Effect of Curing Agent on the Compressive Behavior at Elevated Test Temperature of Carbon Fiber-Reinforced Epoxy Composites
Abstract
1. Introduction
2. Experiments
2.1. Materials
2.2. Productions of CFRP Laminates
2.3. Adhesive Development and Sample Preparation
2.4. Thermal Analysis
2.5. Dynamic Mechanical Analysis
2.6. Compressive Testing of Epoxy Resins
2.7. Celanese Compressive Testing of Carbon Fiber-Reinforced Composites
3. Results and Discussions
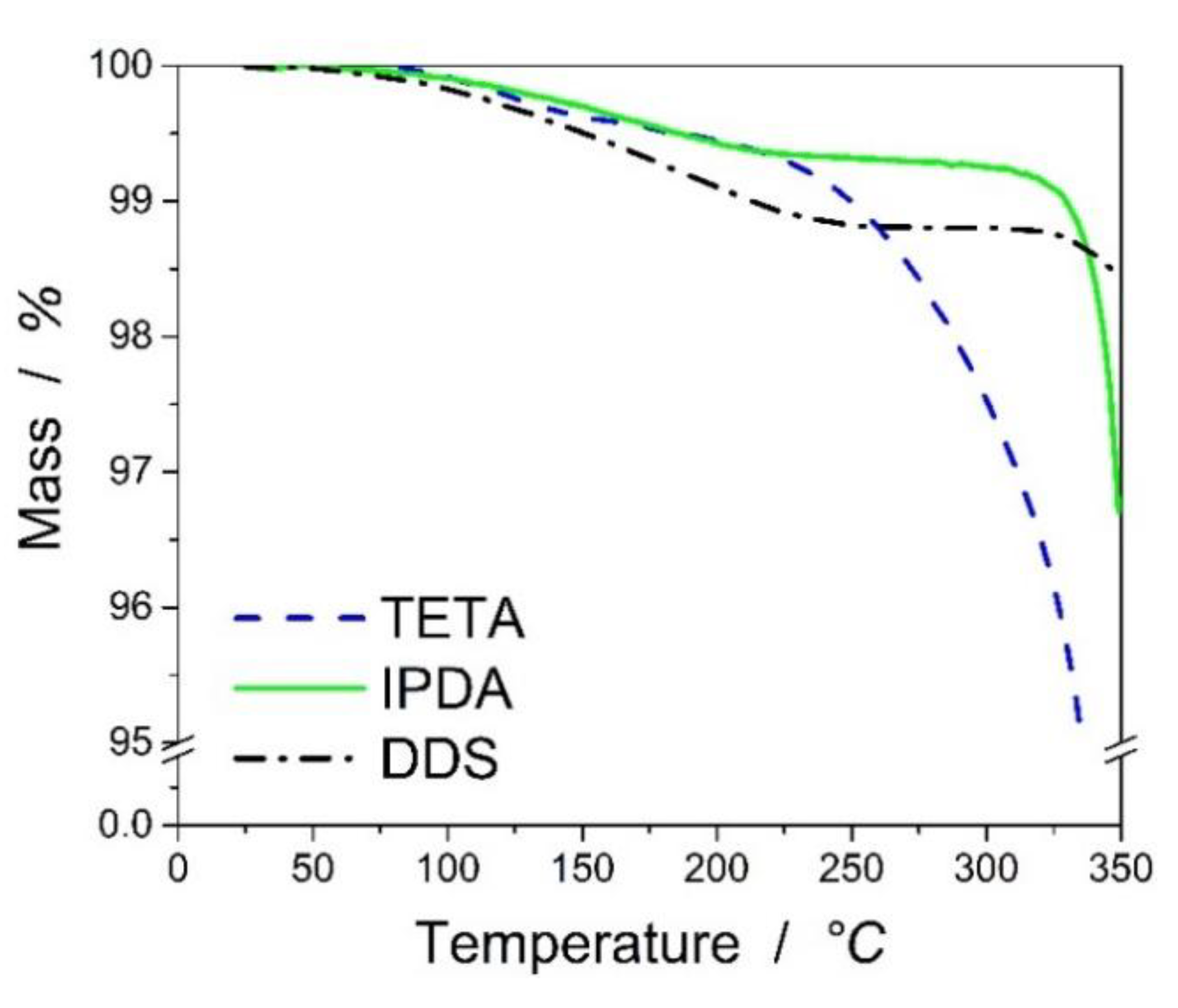
3.1. Thermal Analysis of the Neat Epoxy Systems
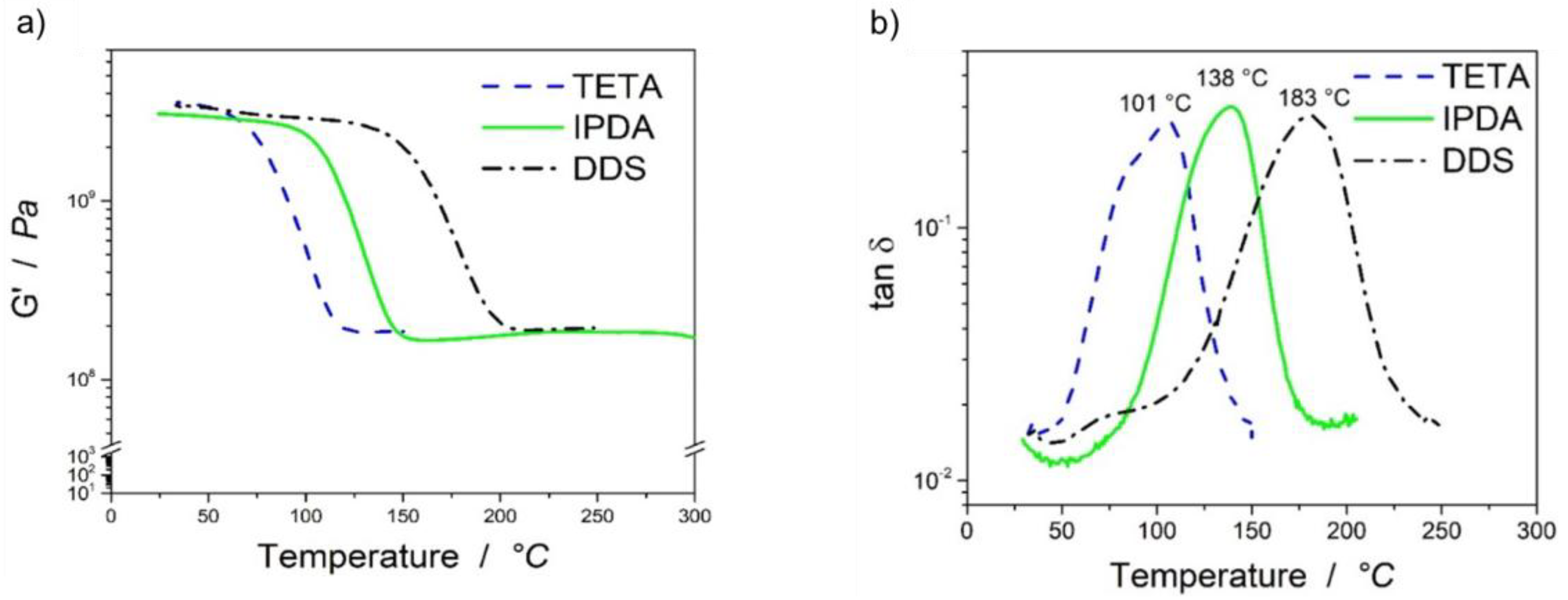
3.2. Determination of Network Density
3.3. Compressive Test of the Neat Resin Systems
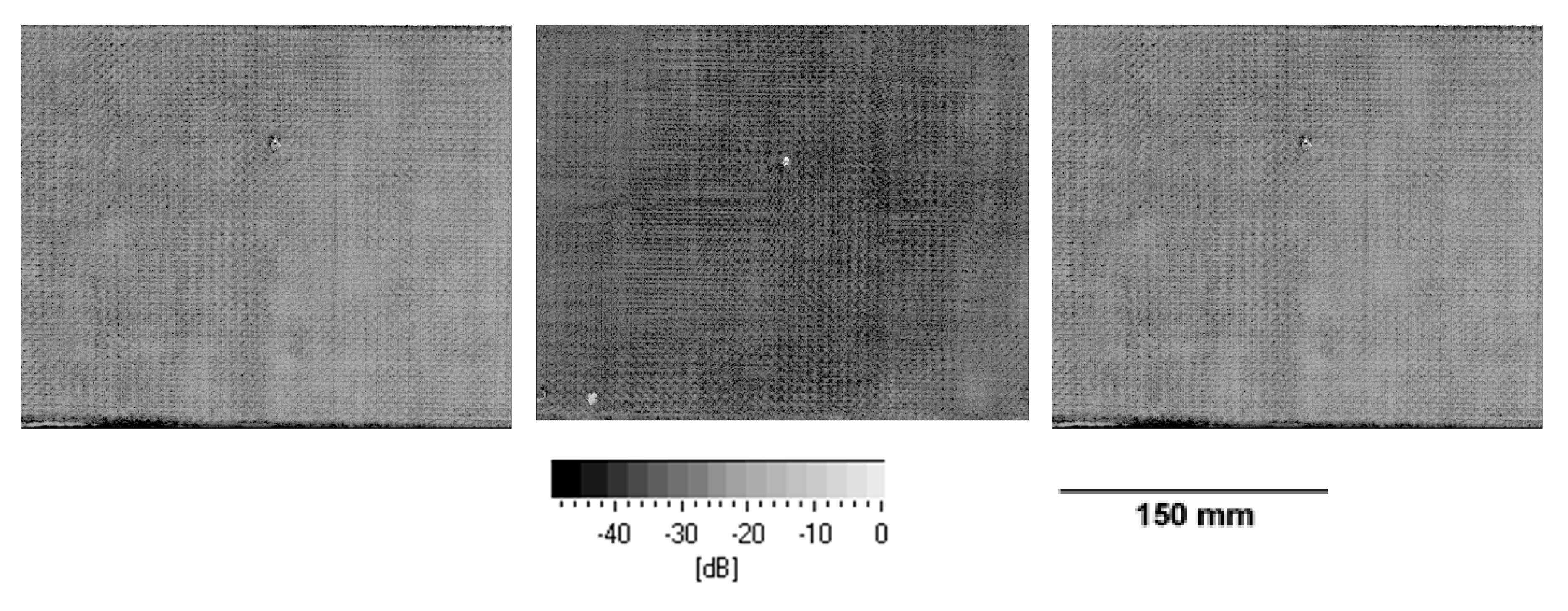
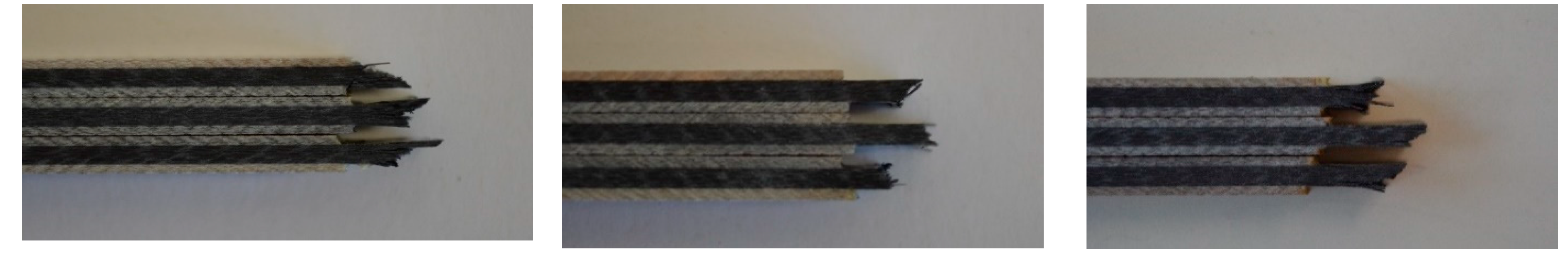
3.4. Ultrasound Testing and Optical Microscopy Measurements of the Laminates
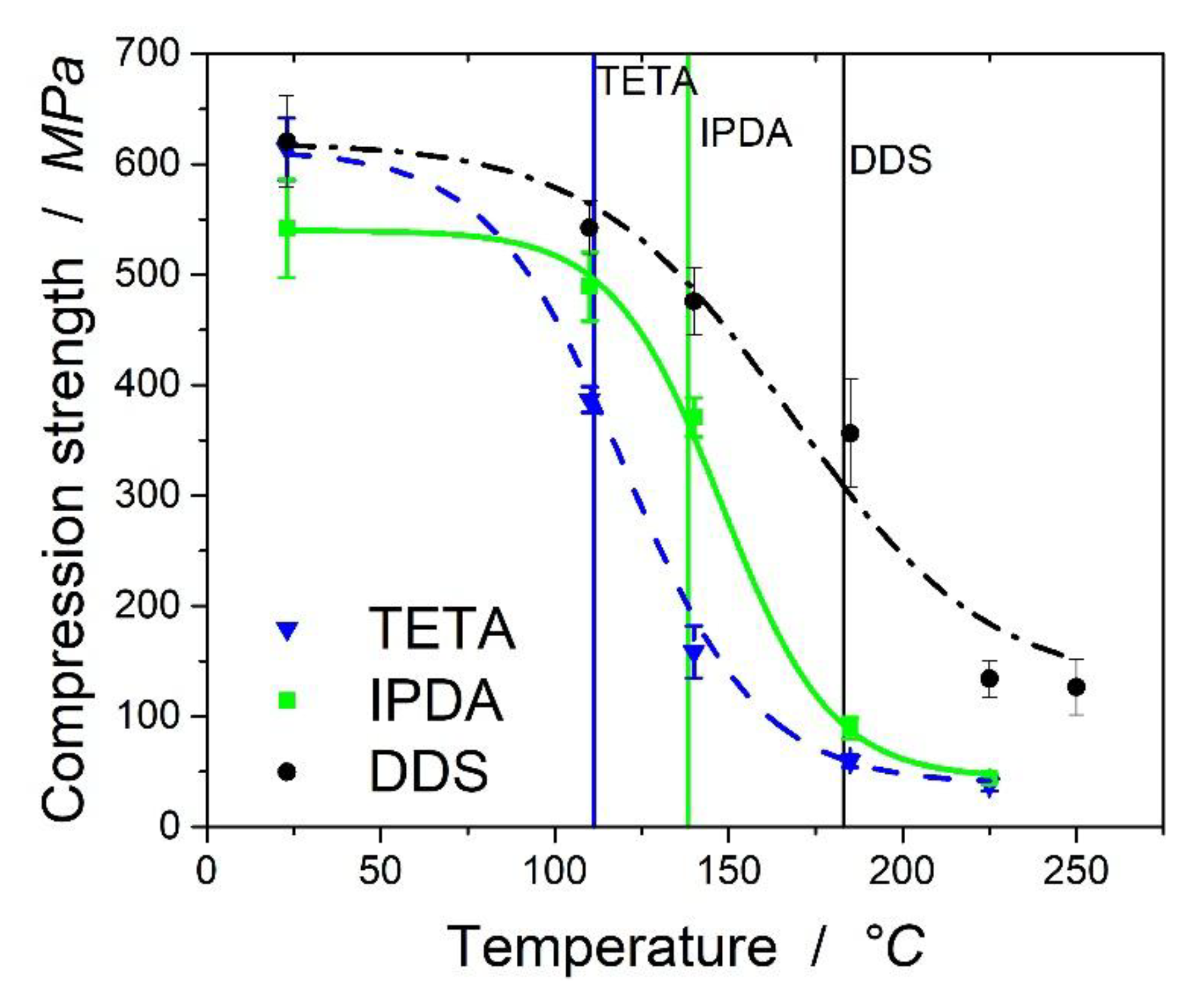
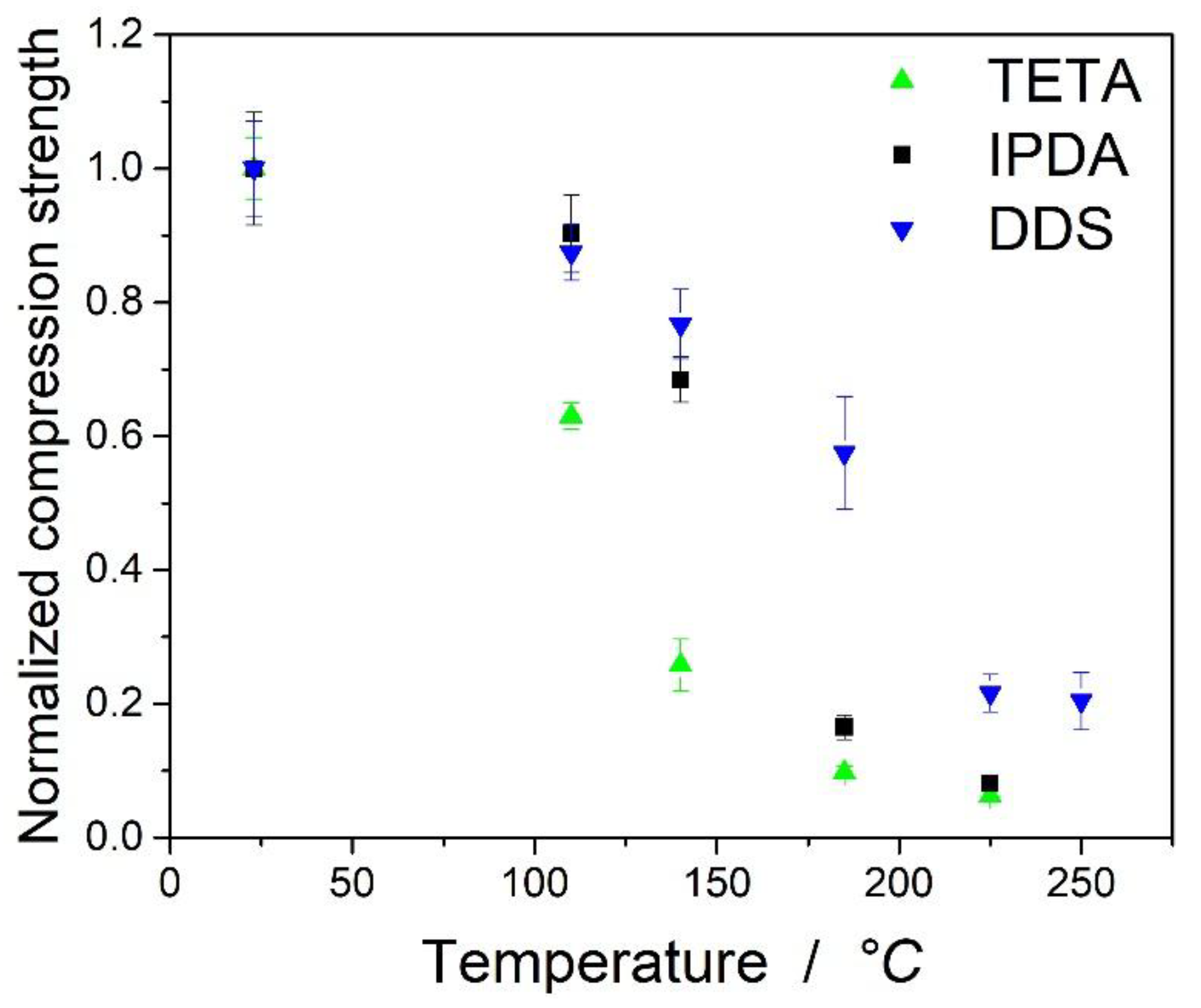
3.5. Celanese Compressive Test of the Fiber-Reinforced Composites at High Temperatures
4. Conclusions
- The differences in network densities calculated from theoretical considerations by Murayama do not seem to be as significant as those calculated from DMTA. This can be attributed to the neglect of the chemical structure and topology in the model by Murayama.
- The compressive strength of the laminates at room temperature is dependent on the modulus of the resin. A higher compressive modulus resulted in higher compressive strength in the Celanese compressive test. This could be explained by the fact that the laminate samples underwent a rather low deformation.
- A high correlation could be found between the thermo-mechanical properties measured in torsion by the DMTA and the scope of the compressive strength in the Celanese compressive tests. The compressive behavior of the composites could be fitted well with the equations suggested by Feih (R2 > 97%).
Author Contributions
Funding
Conflicts of Interest
References
- Pan, C.; Zhang, J.; Shan, Y. Modeling and Analysis of Helicopter Thermal and Infrared Radiation. Chin. J. Aeronaut. 2011, 24, 558–567. [Google Scholar] [CrossRef]
- Weidmann, E.P.; Wiedemann, J.; Binner, T.; Reister, H. Underhood Temperature Analysis in Case of Natural Convection. SAE Tech. Pap. 2015, 1, 2045. [Google Scholar] [CrossRef]
- Gibson, A.G.; Wright, P.N.H.; Wu, Y.-S.; Mouritz, A.P.; Mathys, Z.; Gardiner, C.P. Modelling residual mechanical properties of polymer composites after fire. Plast. Rubber Compos. 2013, 32, 81–90. [Google Scholar] [CrossRef]
- Lisle, T.; Bouvet, C.; Hongkarnjanakul, N.; Pastor, M.-L.; Rivallant, S.; Marguerès, P. Measure of fracture toughness of compressive fiber failure in composite structures using infrared thermography. Compos. Sci. Technol. 2015, 112, 22–33. [Google Scholar] [CrossRef]
- Kandare, E.; Kandola, B.K.; Myler, P.; Edwards, G. Thermo-mechanical Responses of Fiber-reinforced Epoxy Composites Exposed to High Temperature Environments. Part I: Experimental Data Acquisition. J. Compos. Mater. 2010, 44, 3093–3114. [Google Scholar] [CrossRef]
- Kandare, E.; Kandola, B.K.; McCarthy, E.D.; Myler, P.; Edwards, G.; Jifeng, Y.; Wang, Y.C. Fiber-reinforced epoxy composites exposed to high temperature environments. Part II: modeling mechanical property degradation. J. Compos. Mater. 2010, 45, 1511–1521. [Google Scholar] [CrossRef]
- Pinho, S.T.; Gutkin, R.; Pimenta, S.; de Carvalho, N.V.; Robinson, P. On longitudinal compressive failure of carbon-fibre-reinforced polymer: from unidirectional to woven, and from virgin to recycled. Philos. Trans. R. Soc. A 2012, 307, 1871–1895. [Google Scholar] [CrossRef] [PubMed]
- García, F.G.; Soares, B.G.; Pita, V.J.R.R.; Sánchez, R.; Rieumont, J. Mechanical properties of epoxy networks based on DGEBA and aliphatic amines. J. Appl. Sci. 2007, 106, 2047–2055. [Google Scholar] [CrossRef]
- Murayama, T.; Bell, J.P. Relation between the network structure and dynamic mechanical properties of a typical amine-cured epoxy polymer. J. Polym. Sci. Part A-2 Polym. Phys. 1970, 8, 437–445. [Google Scholar] [CrossRef]
- Wu, R. Simulation Methods for Complicated Polymer Networks. MOJ Sci. 2017, 1, 1–3. [Google Scholar] [CrossRef][Green Version]
- Koleske, J.V. Paint and Coating Testing Manual: Fourteenth Edition of the Gardner-Sward Handbook, 14th ed.; ASTM: Philadelphia, PA, USA, 1995. [Google Scholar]
- Okada, T.; Nishijima, S.; Honda, Y.; Kobayashi, Y. Physical properties of epoxy and free volume evaluated by positron annihilation spectroscopy. Le J. de Colloq. 1993, 3, 291–294. [Google Scholar] [CrossRef]
- Dlubek, G.; Hassan, E.M.; Krause-Rehberg, R.; Pionteck, J. Free volume of an epoxy resin and its relation to structural relaxation: Evidence from positron lifetime and pressure-volume-temperature experiments. Phys. E 2006, 73, 031803. [Google Scholar] [CrossRef] [PubMed]
- Babayevsky, P.G.; Gillham, J.K. Epoxy thermosetting systems: Dynamic mechanical analysis of the reactions of aromatic diamines with the diglycidyl ether of bisphenol A. J. Appl. Sci. 1973, 17, 2067–2088. [Google Scholar] [CrossRef]
- Morrison, R.T.; Boyd, R.N. Organic Chemistry, 4th ed.; Allyn and Bacon: Boston, MA, USA, 1983. [Google Scholar]
- Baranek, A.D.; Kendrick, L.L.; Narayanan, J.; Tyson, G.E.; Wand, S.; Patton, D.L. Flexible aliphatic-bridged bisphenol-based polybenzoxazines. Polym. Chem. 2012, 3, 2892. [Google Scholar] [CrossRef]
- Nakka, J.S.; Jansen, K.M.B.; Ernst, L.J. Effect of chain flexibility in the network structure on the viscoelasticity of epoxy thermosets. J. Polym. Res. 2011, 18, 1879–1888. [Google Scholar] [CrossRef]
- Safranski, D.L.; Gall, K. Effect of chemical structure and crosslinking density on the thermo-mechanical properties and toughness of (meth)acrylate shape memory polymer networks. Polymer 2008, 49, 4446–4455. [Google Scholar] [CrossRef]
- Gottro, J. Thermoset Characterization Part 17: Applications of Dynamic Mechanical Analysis (DMA), Part 2. Polymer Innovation Blog. [November 07, 2017]. Available online: https://polymerinnovationblog.com/thermoset-characterization-part-17-applications-dynamic-mechanical-analysis-dma-part-2 (accessed on 1 May 2019).
- Jelf, P.; Fleck, N. Compression Failure Mechanisms in Unidirectional Composites. J. Compos. Mater. 1992, 26, 2706–2726. [Google Scholar] [CrossRef]
- Feih, S.; Mathys, Z.; Gibson, A.; Mouritz, A.; Gibson, A. Modelling the tension and compression strengths of polymer laminates in fire. Compos. Sci. Technol. 2007, 67, 551–564. [Google Scholar] [CrossRef]


| Hardener Name | Chemical Structure | Molecular Weight (g mol−1) | AHEW g/eq |
|---|---|---|---|
| Triethylentetramine (TETA) | 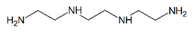 | 146.24 | 37.75 |
| Isophoronediamine (IPDA) |  | 170.30 | 42.58 |
| 4,4‘-Diaminodiphenylsulfon (DDS) | 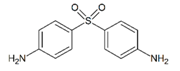 | 248.31 | 62.08 |
| Hardener | Step 1 | Step 2 | Step 3 |
|---|---|---|---|
| TETA | 10 min, 80 °C | 10 min, 100 °C | 50 min, 120 °C |
| IPDA | 60 min, 80 °C | 30 min, 120 °C | 30 min, 160 °C |
| DDS | 60 min, 140 °C | 120 min, 180 °C | 60 min, 200 °C |
| g/cm3 | |||
|---|---|---|---|
| TETA | 142 | 1.19 | 20.41 |
| IPDA | 138 | 1.14 | 20.02 |
| DDS | 134 | 1.23 | 22.33 |
MPa | °C | mol/g | GII KPa | Δ (GI, GII) MPa | |
|---|---|---|---|---|---|
| TETA | 5.756 | 141 | 559 | 3.393 | 5.75 |
| IPDA | 12.076 | 178 | 1069 | 675.5 | 11.4 |
| DDS | 14.169 | 223 | 1152 | 229.9 | 13.4 |
| Hardener Type | Compressive Strength MPa | Compressive Modulus GPa |
|---|---|---|
| TETA | 148 ± 8 | 2.85 ± 0.28 |
| IPDA | 204 ± 17 | 2.74 ± 0.25 |
| DDS | 297 ± 20 | 2.79 ± 0.30 |
| R2 | |||||
|---|---|---|---|---|---|
| MPa | MPa | °C | |||
| TETA | 613.6 | 38.6 | 120 | 0.025 | 0.997 |
| IPDA | 541.2 | 43.4 | 148 | 0.031 | 0.998 |
| DDS | 620.0 | 126.1 | 168 | 0.017 | 0.969 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bard, S.; Demleitner, M.; Weber, R.; Zeiler, R.; Altstädt, V. Effect of Curing Agent on the Compressive Behavior at Elevated Test Temperature of Carbon Fiber-Reinforced Epoxy Composites. Polymers 2019, 11, 943. https://doi.org/10.3390/polym11060943
Bard S, Demleitner M, Weber R, Zeiler R, Altstädt V. Effect of Curing Agent on the Compressive Behavior at Elevated Test Temperature of Carbon Fiber-Reinforced Epoxy Composites. Polymers. 2019; 11(6):943. https://doi.org/10.3390/polym11060943
Chicago/Turabian StyleBard, Simon, Martin Demleitner, Regino Weber, Rico Zeiler, and Volker Altstädt. 2019. "Effect of Curing Agent on the Compressive Behavior at Elevated Test Temperature of Carbon Fiber-Reinforced Epoxy Composites" Polymers 11, no. 6: 943. https://doi.org/10.3390/polym11060943
APA StyleBard, S., Demleitner, M., Weber, R., Zeiler, R., & Altstädt, V. (2019). Effect of Curing Agent on the Compressive Behavior at Elevated Test Temperature of Carbon Fiber-Reinforced Epoxy Composites. Polymers, 11(6), 943. https://doi.org/10.3390/polym11060943