Elasto-Dynamics of Quasicrystals
Abstract
:1. Introduction
2. Elasto-Dynamics of Quasicrystals Followed Bak’s Argument
2.1. Example 1: Basic Equations for Elasto-Dynamics of Anti-Plane Elasticity of Some Quasicrystals
2.2. Example 2: Elasto-Dynamics of Hexagonal Quasicrystals
2.3. Example 3: Elasto-Dynamics of Plane Elasticity of Dodecagonal Quasicrystals
2.4. Example 4: The Approximate Form of Elasto-Dynamics of Two-Dimensional Elasticity (or Simplified Three-Dimensional Elasticity) of Icosahedral Quasicrystals
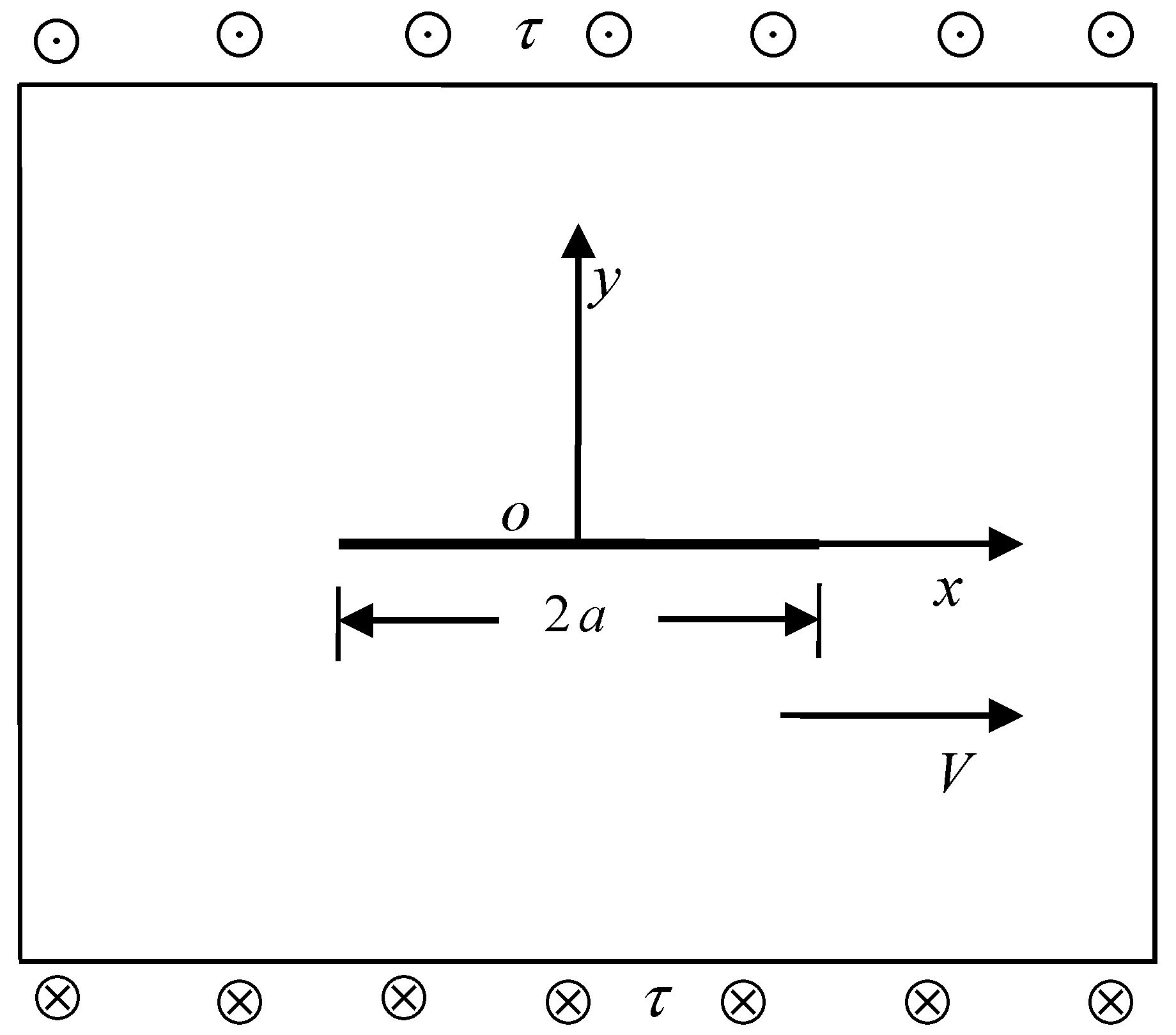
2.5. A Moving Screw Dislocation in Anti-Plane Elasticity of Some Quasicrystals
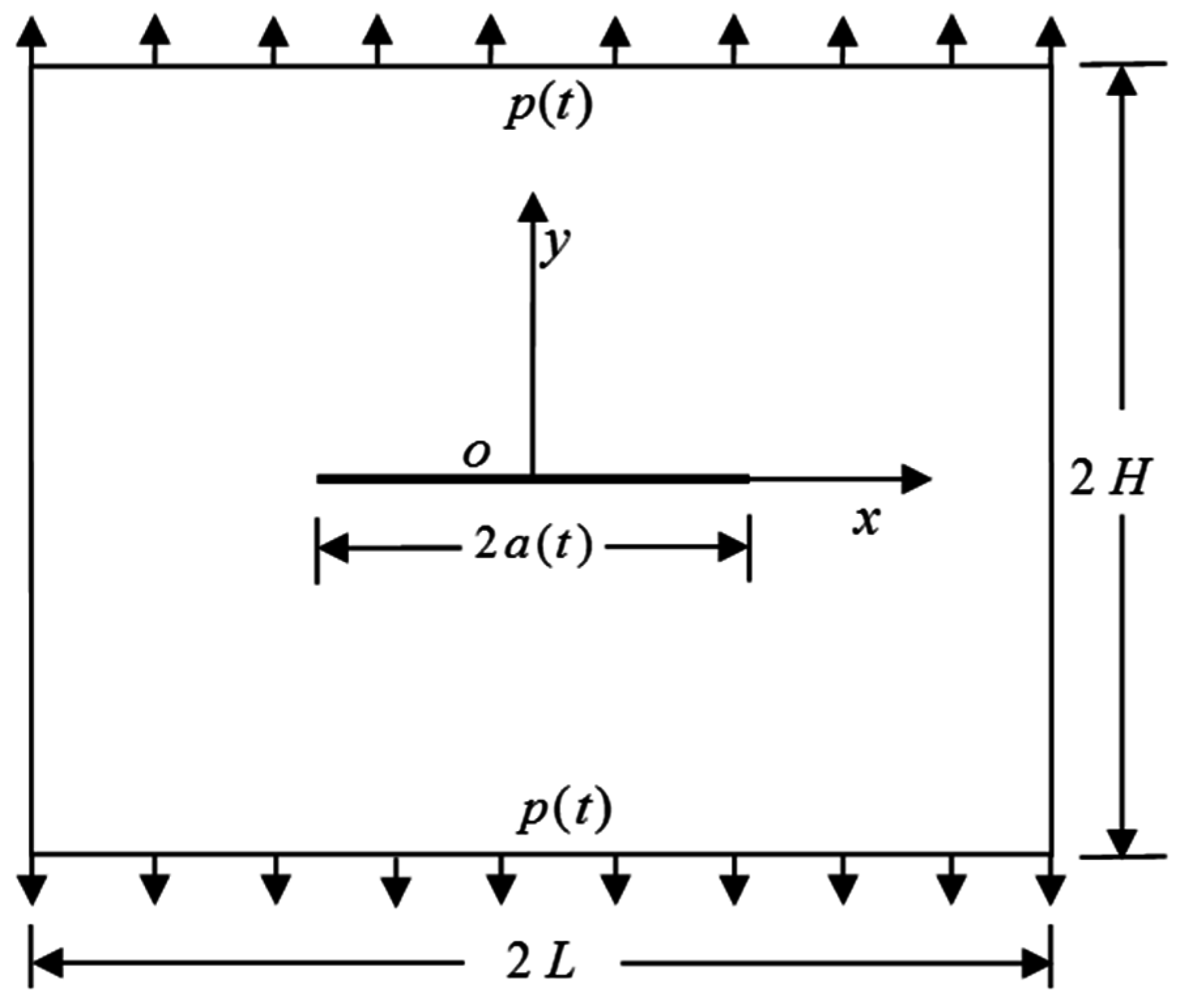
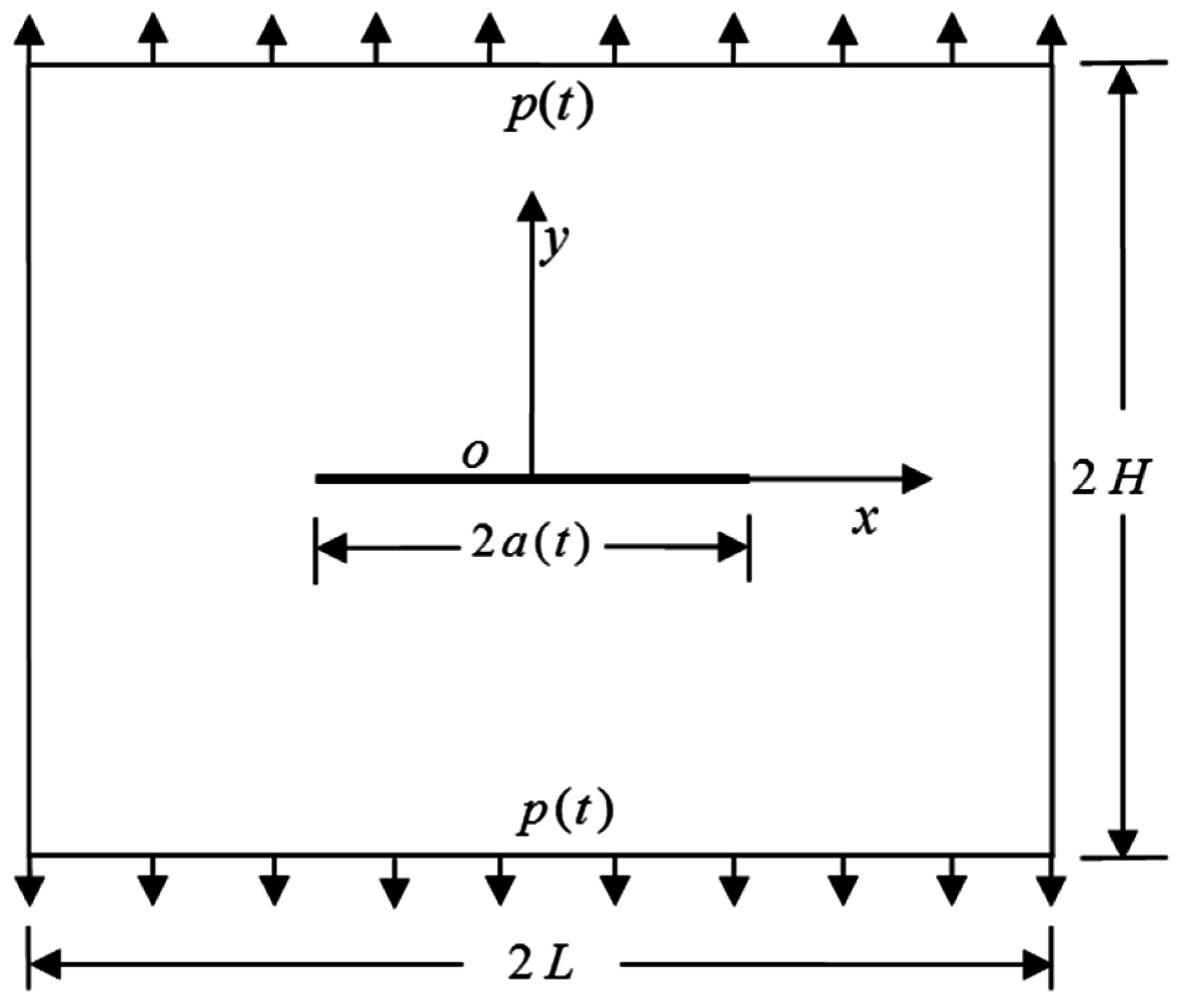
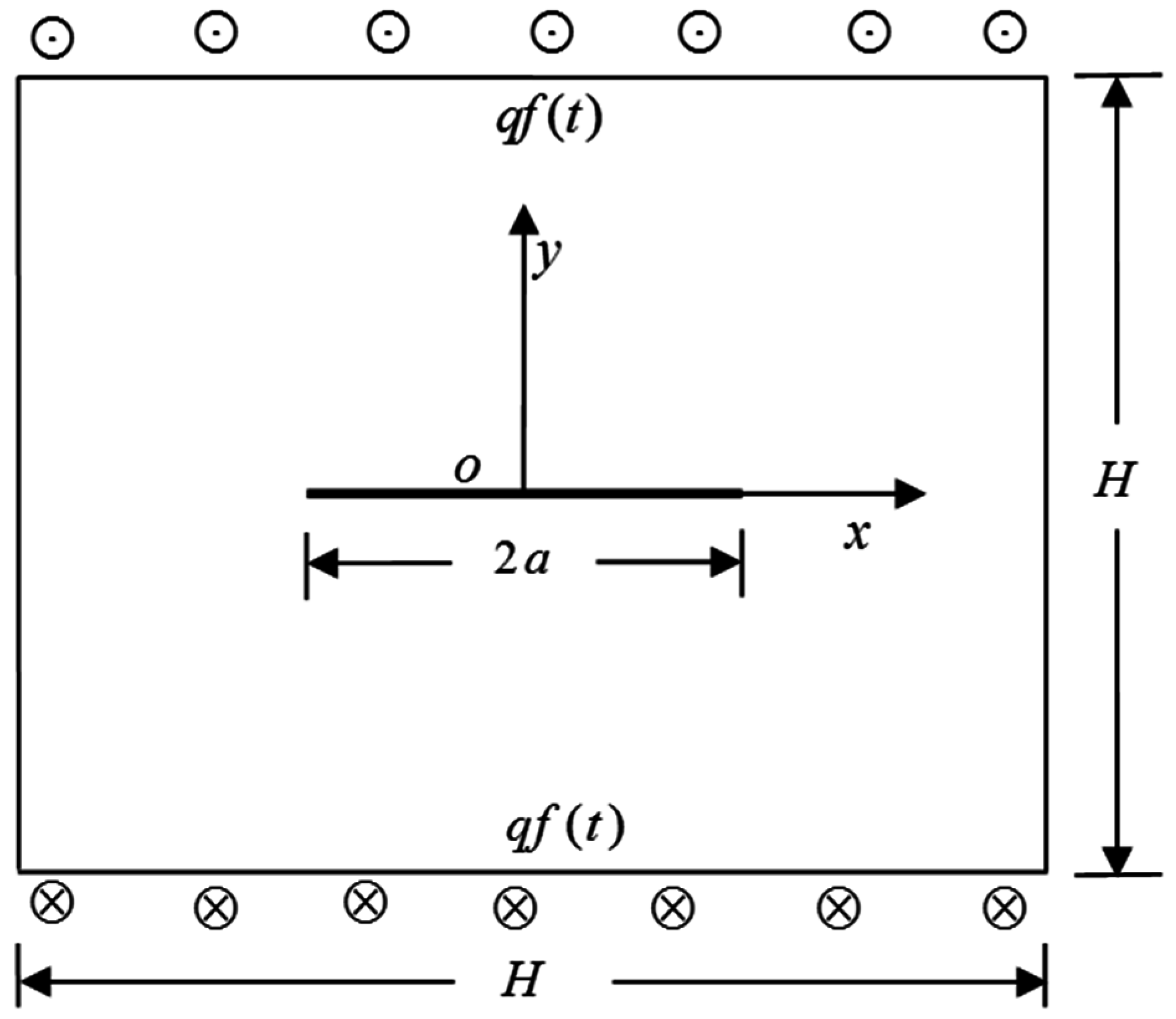
2.6. A Mode III Moving Griffith Crack in Anti-Plane Elasticity
2.7. The Moving Dugdale Model for Plane Elasticity of Dodecagonal Quasicrystals
3. Elasto-/Hydro-Dynamics of Quasicrystals Based on the Argument of Lubensky et al.
3.1. Application 1 of Elasto-/Hydro-Dynamics-Approximate Solution for a Moving Screw Dislocation in Anti-Plane Elasticity
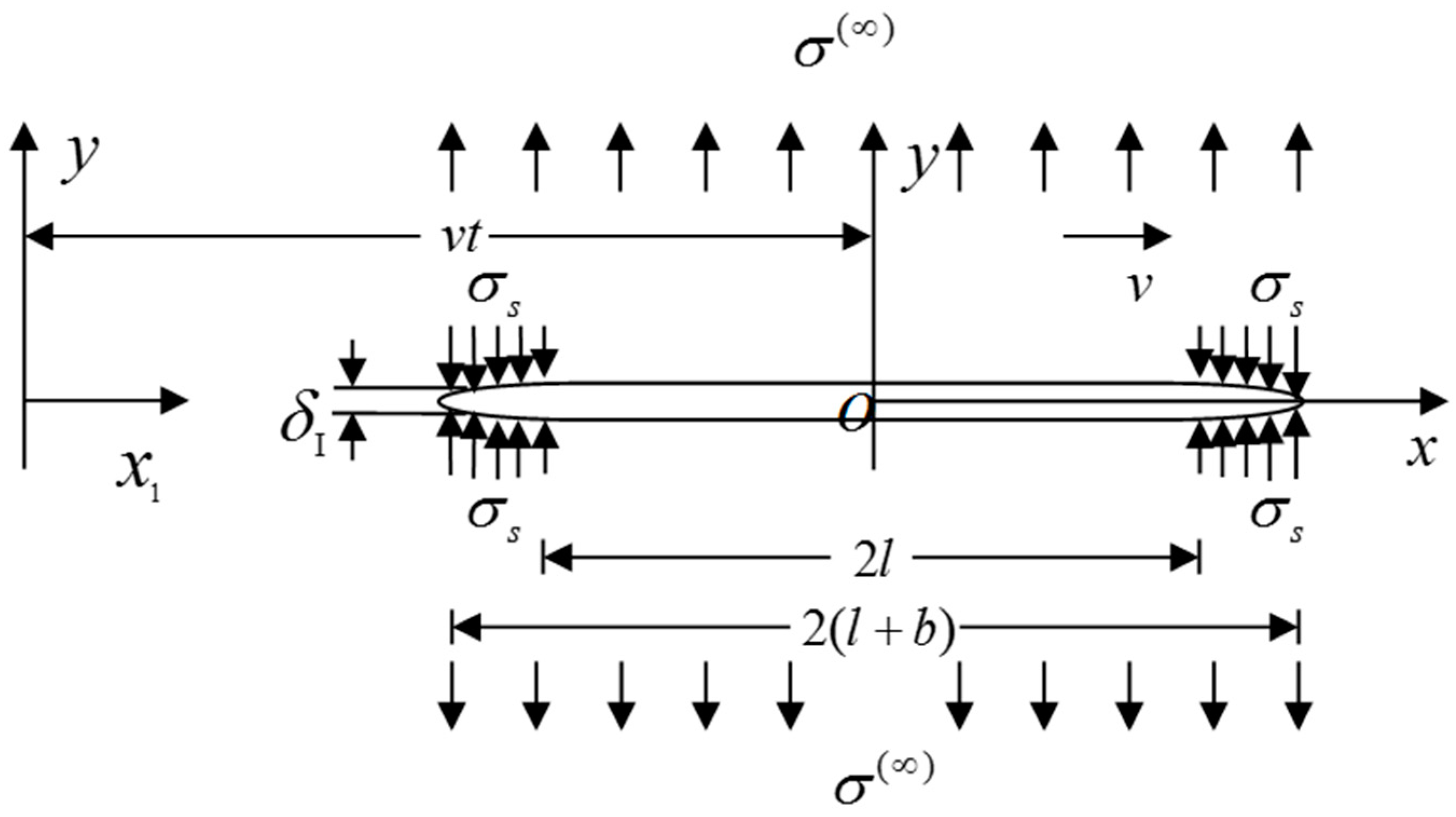
3.2. Application 2 of Elasto-/Hydro-Dynamics—Dynamic Propagating Crack and Solutions of Two-Dimensional Decagonal Quasicrystals
3.3. Application 3 of Elasto-/Hydro-Dynamics-Dynamic Crack Propagation of Icosahedral Quasicrystals
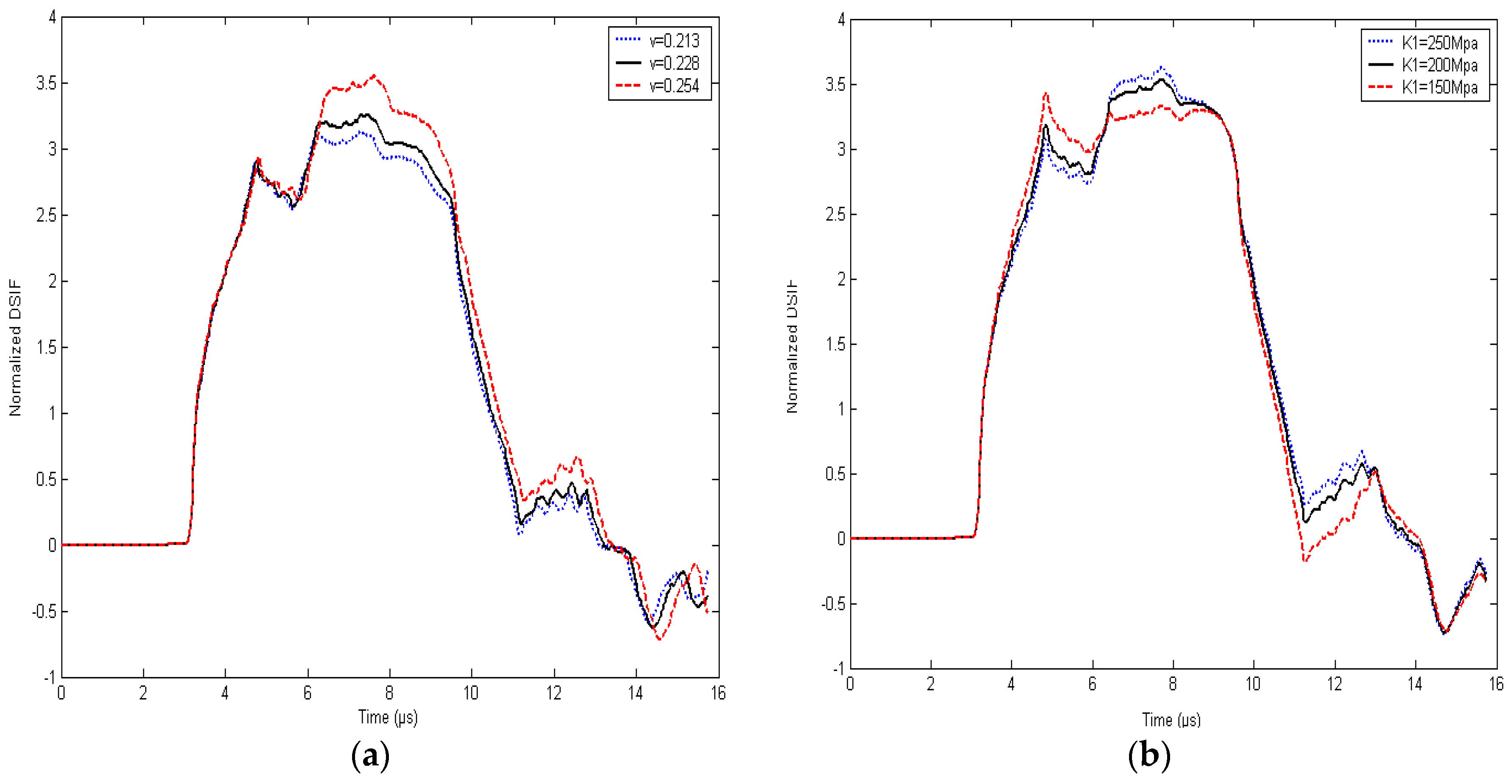
3.3.1. A Cracked Specimen of Icosahedral Quasicrystals of Plane Problem Based on FDM
3.3.2. A Cracked Specimen of Anti-Plane Problem in Icosahedral QCs Based on FDM
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Shechtman, D.; Blech, I.; Gratias, D.; Cahn, J.W. Metallic phase with long-range orientational order and no translational symmetry. Phys. Rev. Lett. 1984, 53, 1951–1953. [Google Scholar] [CrossRef]
- Ohashi, W.; Spaepen, F. Stable Ga-Mg-Zn quasi-periodic crystals with pentagonal dodecahedral solidification morphology. Nature 1987, 330, 555–556. [Google Scholar] [CrossRef]
- Wang, N.; Chen, H.; Kuo, K.H. Two-dimensional quasicrystal with eightfold rotational symmetry. Phys. Rev. Lett. 1987, 59, 1010–1013. [Google Scholar] [CrossRef] [PubMed]
- Janot, C. The structure of quasicrystals. J. Non-Cryst. Solids 1993, 156–158, 852–864. [Google Scholar] [CrossRef]
- Ishimasa, T.; Nissen, H.U. New ordered state between crystalline and am or phous in Ni-Cr particles. Phys. Rev. Lett. 1985, 55, 511–513. [Google Scholar] [CrossRef] [PubMed]
- Feng, Y.C.; Lu, G. Experimental evidence for and a projection model of a cubic quasicrystal. J. Phys. Condens. Matter 1990, 2, 9749–9755. [Google Scholar] [CrossRef]
- Chen, H.; Li, X.Z.; Zhang, Z.; Kuo, K.H. One-dimensional quasicrystals with twolvefold rotational symmetry. Phys. Rev. Lett. 1998, 60, 1645–1648. [Google Scholar] [CrossRef] [PubMed]
- Bohsung, J.; Trebin, H.R. Disclinations in quasicrystals. Phys. Rev. Lett. 1987, 58, 1204–1207. [Google Scholar] [CrossRef] [PubMed]
- Ebert, P.H.; Feuerbacher, M.; Tamura, N. Evidence for a cluster-based on structure of Al-Pd-Mn single quasicrystals. Phys. Rev. Lett. 1996, 77, 3827–3830. [Google Scholar] [CrossRef] [PubMed]
- Li, C.L.; Liu, Y.Y. Low-temperature lattice excitation of icosahedral Al-Mn-Pd quasicrystals. Phys. Rev. B 2001, 63, 064203. [Google Scholar] [CrossRef]
- Rochal, S.B.; Lorman, V.L. Anisotropy of acoustic-phonon properties of an icosahedral quasicrystal at high temperature due to phonon-phason coupling. Phys. Rev. B 2000, 62, 849–874. [Google Scholar] [CrossRef]
- Bak, P. Phenomenological theory of icosahedral in commensurate (quasiperiodic) order in Mn-Al alloys. Phys. Rev. Lett. 1985, 54, 1517–1519. [Google Scholar] [CrossRef] [PubMed]
- Bak, P. Symmetry, stability and elastic properties of icosahedral in commensurate crystals. Phys. Rev. B 1985, 32, 5764–5772. [Google Scholar] [CrossRef]
- Socolar, J.E.S.; Lubensky, T.C.; Steinhardt, P.J. Phonons, phasons and dislocations in quasicrystals. Phys. Rev. B 1986, 34, 3345–3360. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Statistical Physics; Pergamon Press: Elmsford, NY, USA, 1958. [Google Scholar]
- Edagawa, K. Phonon-phason coupling in decagonal quasicrystals. Philos. Mag. 2007, 87, 2789–2798. [Google Scholar] [CrossRef]
- Cheminkov, M.A.; Ott, H.R.; Bianchi, A.; Migliori, A.; Darling, T.W. Elastic moduli of a single quasicrystal of decagonal Al-Ni-Co: Evidence for transverse elastic isotropy. Phys. Rev. Lett. 1998, 80, 321–324. [Google Scholar]
- Tanaka, K.; Mitarai, Y.; Koiwa, M. Elastic constants of Al-based icosahedral quasicrystals. Philos. Mag. A 1996, 73, 1715–1723. [Google Scholar] [CrossRef]
- Ding, D.H.; Yang, W.G.; Hu, C.Z. Generalized elasticity theory of quasicrystals. Phys. Rev. B 1993, 48, 7003–7010. [Google Scholar] [CrossRef]
- Hu, C.Z.; Wang, R.H.; Ding, D.H. Symmetry groups, physical property tensors, elasticity and dislocations in quasicrystals. Rep. Prog. Phys. 2000, 63, 1–39. [Google Scholar] [CrossRef]
- Jeong, H.C.; Steinhardt, P.J. Finite-temperature elasticity phase transition in decagonal quasicrystals. Phys. Rev. B 1993, 48, 9394–9403. [Google Scholar] [CrossRef]
- Fan, T.Y.; Mai, Y.W. Elasticity theory, fracture mechanics and some relevant thermal properties of quasicrystalline materials. Appl. Mech. Rev. 2004, 57, 325–344. [Google Scholar] [CrossRef]
- Fan, T.Y. Mathematical Theory of Elasticity of Quasicrystals and Its Applications; Springer: Heideberg, Germany, 2010. [Google Scholar]
- Levine, D.; Lubensky, T.C.; Ostlund, S. Elasticity and dislocations in pentagonal and icosahedral quasicrystals. Phys. Rev. Lett. 1985, 54, 1520–1523. [Google Scholar] [CrossRef] [PubMed]
- Li, X.F.; Duan, X.Y.; Fan, T.Y. Elastic field for a straight dislocation in a decagonal quasicrystal. J. Phys. Condens. Matter 1999, 11, 703–711. [Google Scholar] [CrossRef]
- Li, X.F.; Fan, T.Y.; Sun, Y.F. A decagonal quasicrystal with a Griffith crack. Philos. Mag. A 1999, 79, 1943–1952. [Google Scholar]
- Chen, W.Q.; Ma, Y.L.; Ding, H.J. On three-dimensional elastic problems of one dimensional hexagonal quasicrystal bodies. Mech. Res. Commun. 2004, 31, 633–641. [Google Scholar] [CrossRef]
- Liu, G.T.; Fan, T.Y.; Guo, R.P. Governing equations and general solutions of plane elasticity of one-dimensional quasicrystals. Int. J. Solids Struct. 2004, 41, 3949–3959. [Google Scholar] [CrossRef]
- Li, L.H.; Fan, T.Y. Complex function method for solving notch problem of point 10 two-dimensional quasicrystal based on the stress potential function. J. Phys. Condens. Matter 2006, 18, 10631–10641. [Google Scholar] [CrossRef]
- Wang, X.; Pan, E. Analytical solutions for some defect problems in 1D hexagonal and 2D octagonal quasicrystals. Pramana—J. Phys. 2008, 70, 911–933. [Google Scholar] [CrossRef]
- Gao, Y.; Xu, S.P.; Zhao, B.S. Boundary conditions for plate bending in one dimensional hexagonal quasicrystals. J. Elast. 2007, 86, 221–233. [Google Scholar] [CrossRef]
- Gao, Y.; Ricoeur, A. The refined theory of one-dimensional quasi-crystals inthick plate structures. J. Appl. Mech. 2011, 78, 031021. [Google Scholar] [CrossRef]
- Coddens, G. On the problem of the relation between phason elasticity and phason dynamics in quasicrystals. Eur. Phys. J. B 2004, 54, 37–65. [Google Scholar] [CrossRef]
- Wang, J.B.; Mancini, L.; Wang, R.H.; Gastaldi, J. Phonon- and phason-type spherical inclusions in icosahedral quasicrystals. J. Phys. Condens. Matter 2003, 15, L363–L370. [Google Scholar] [CrossRef]
- Guo, J.H.; Zhang, Z.Y.; Xing, Y.M. Antiplane analysis for an elliptical inclusion in 1D hexagonal piezoelectric quasicrystal composites. Philos. Mag. 2016, 96, 349–369. [Google Scholar] [CrossRef]
- Radi, E.; Mariano, P.M. Stationary straight cracks in quasicrystals. Int. J. Fract. 2010, 166, 102–120. [Google Scholar] [CrossRef]
- Radi, E.; Mariano, P.M. Steady-state propagation of dislocations in quasi-crystals. Proc. R. Soc. A Math. Phys. 2011, 467, 3490–3508. [Google Scholar] [CrossRef]
- Mariano, P.M.; Planas, J. Phason self-actions in quasicrystals. Physica D 2013, 249, 24946–24957. [Google Scholar] [CrossRef]
- Lazar, M.; Agiasofitou, E. Fundamentals in generalized elasticity and dislocation theory of quasicrystals: Green tensor, dislocation key-formulas and dislocation loops. Philos. Mag. 2014, 94, 4080–4101. [Google Scholar] [CrossRef]
- Li, X.Y. Fundamental solutions of penny-shaped and half infinite plane cracks embedded in an infinite space of one dimensional hexagonal quasi-crystal under thermal loading. Proc. R. Soc. A Math. Phys. 2013, 469, 20130023. [Google Scholar] [CrossRef]
- Li, X.Y. Three-dimensional thermo-elastic general solutions of one-dimensional hexagonal quasi-crystal and fundamental solutions. Phys. Lett. A 2012, 376, 2004–2009. [Google Scholar] [CrossRef]
- Li, X.Y. Elastic field in an infinite medium of one-dimensional hexagonal quasicrystalwith a planar crack. Int. J. Solids Struct. 2014, 51, 1442–1455. [Google Scholar] [CrossRef]
- Sladek, J.; Sladek, V.; Pan, E. Bending analyses of 1D orthorhombic quasicrystal plates. Int. J. Solids Struct. 2013, 50, 3975–3983. [Google Scholar] [CrossRef]
- Li, W.; Chai, Y.Z. Anti-plane problem analysis for icosahedral quasicrystals under shear loadings. Chin. Phys. B 2014, 23, 116201. [Google Scholar] [CrossRef]
- Wollgarten, M.; Beyss, M.; Urban, K.; Liebertz, H.; Koster, U. Direct evidence for plastic deformation of quasicrystals by means of a dislocationmechanism. Phys. Rev. Lett. 1993, 71, 549–552. [Google Scholar] [CrossRef] [PubMed]
- Feuerbacher, M.; Bartsch, M.; Grushko, B.; Messerschmidt, U.; Urban, K. Plastic deformation of decagonal Al-Ni-Co quasicrystals. Philos. Mag. Lett. 1997, 76, 369–376. [Google Scholar] [CrossRef]
- Messerschmidt, U.; Bartsch, M.; Feuerbacher, M.; Geyer, B.; Urban, K. Friction mechanism of dislocation motion in icosahedralAl-Pd-Mn quasicrystals. Philos. Mag. A 1999, 79, 2123–2135. [Google Scholar] [CrossRef]
- Schall, P.; Feuerbacher, M.; Bartsch, M.; Messerschmidt, U.; Urban, K. Dislocation density evolution upon plastic deformation of Al-Pd-Mn single quasicrystals. Philos. Mag. Lett. 1999, 79, 785–796. [Google Scholar] [CrossRef]
- Geyer, B.; Bartsch, M.; Feuerbacher, M.; Urban, K.; Messerschmidt, U. Plastic deformation of icosahedral Al-Pd-Mn single quasicrystals I. Experimental results. Philos. Mag. A 2000, 80, 1151–1163. [Google Scholar] [CrossRef]
- Rosenfeld, R.; Feuerbacher, M. Study of plastically deformed icosahedral Al-Pd-Mn single quasicrystals by transmission electron microscopy. Philos. Mag. Lett. 1995, 72, 375–384. [Google Scholar] [CrossRef]
- Caillard, D.; Vanderschaeve, G.; Bresson, L.; Gratias, D. Transmission electron microscopy study of dislocations and extended defects in as-grown icosahedral Al-Pd-Mn single grains. Philos. Mag. A 2000, 80, 237–253. [Google Scholar] [CrossRef]
- Lubensky, T.C.; Ramaswamy, S.; Toner, J. Hydrodynamics of icosahedral quasicrystals. Phys. Rev. B 1985, 32, 7444–7452. [Google Scholar] [CrossRef]
- Francoual, S.; Livet, F.; de Boissieu, M.; Yakhou, F.; Bley, F.; Letoublon, A.; Caudron, R.; Gastaldi, J. Dynamics of Phason fluctuation in i-Al-Pd-Mn quasicrystals. Phys. Rev. Lett. 2003, 91, 225501. [Google Scholar] [CrossRef] [PubMed]
- Rochal, S.B.; Lorman, V.L. Minimal model of the phonon-phason dynamics on icosahedral quasicrystals and its application for the problem of internal friction in the i-Al-Pd-Mn alloys. Phys. Rev. B 2002, 66, 144204. [Google Scholar] [CrossRef]
- Mikulla, R.; Stadler, J.; Krul, F.; Trebin, H.R.; Gumbsch, P. Crack propagation in quasicrystals. Phys. Rev. Lett. 1998, 81, 3163–3166. [Google Scholar] [CrossRef]
- Takeuchi, S.; Iwanaga, H.; Shibuya, T. Hardness of quasicrystals. Jpn. J. Appl. Phys. 1991, 30, 561–562. [Google Scholar] [CrossRef]
- Rösch, F.; Rudhart, C.; Roth, J.; Trebin, H.R.; Gumbsch, P. Dynamic fracture of icosahedral model quasicrystals: A molecular dynamics study. Phys. Rev. B 2005, 72, 014128. [Google Scholar] [CrossRef]
- Shmuely, M.; Alterman, Z.S. Crack propagation analysis by finite differences. J. Appl. Mech. 1973, 40, 902–908. [Google Scholar] [CrossRef]
- Murti, V.; Vlliappan, S. The use of quarter point element in dynamic crack analysis. Eng. Fract. Mech. 1982, 23, 585–614. [Google Scholar] [CrossRef]
- Fan, T.Y.; Li, X.F.; Sun, Y.F. A moving screw dislocation in one-dimensional hexagonal quasicrystal. Acta Phys. Sin. 1999, 8, 288–295. [Google Scholar]
- Fan, T.Y.; Wang, X.F.; Li, W.; Zhu, A.Y. Elasto-hydrodynamics of quasicrystals. Philos. Mag. 2009, 89, 501–512. [Google Scholar] [CrossRef]
- Zhu, A.Y.; Fan, T.Y. Dynamic crack propagation in a decagonal Al-Ni-Co quasicrystal. J. Phys. Condens. Matter 2008, 20, 295217. [Google Scholar] [CrossRef]
- Wang, X.F.; Fan, T.Y.; Zhu, A.Y. Dynamic behaviour of the icosahedral Al-Pd-Mn quasicrystal with a Griffith crack. Chin. Phys. B 2009, 18, 709–714. [Google Scholar]
- Yoffe, E.H. Moving Griffith crack. Philos. Mag. 1951, 43, 739–750. [Google Scholar] [CrossRef]
- Tupholme, G.E. An anti-plane shear crack moving in one-dimensional hexagonal quasicrystals. Int. J. Solids Struct. 2015, 71, 255–261. [Google Scholar] [CrossRef]
- Li, X.F. A general solution of elasto-hydrodynamics of two dimensionalquasicrystals. Philos. Mag. Lett. 2011, 91, 313–320. [Google Scholar] [CrossRef]
- Agiasofitou, E.; Lazar, M. The elastodynamic model of wave-telegraph type for quasicrystals. Int. J. Solids Struct. 2014, 51, 923–929. [Google Scholar] [CrossRef]
- Agiasofitou, E.; Lazar, M. On the equations of motion of dislocation in quasicrystals. Mech. Res. Commun. 2014, 57, 27–33. [Google Scholar] [CrossRef]
- Amazit, Y.; de Boissieu, M.; Zarembowitch, A. Evidences for elastic isotropy and ultrasonic-attenuation anisotropy in Al-Mn-Pd quasi-crystals. Europhys. Lett. 1992, 20, 703–706. [Google Scholar] [CrossRef]
- Takeuchi, S.; Edagawa, K. Chapter 8 of elasticity and plastic properties of quasicrystals. In Handbook of Metal Physics; Elsevier: Amsterdam, The Netherlands, 2007; pp. 267–311. [Google Scholar]
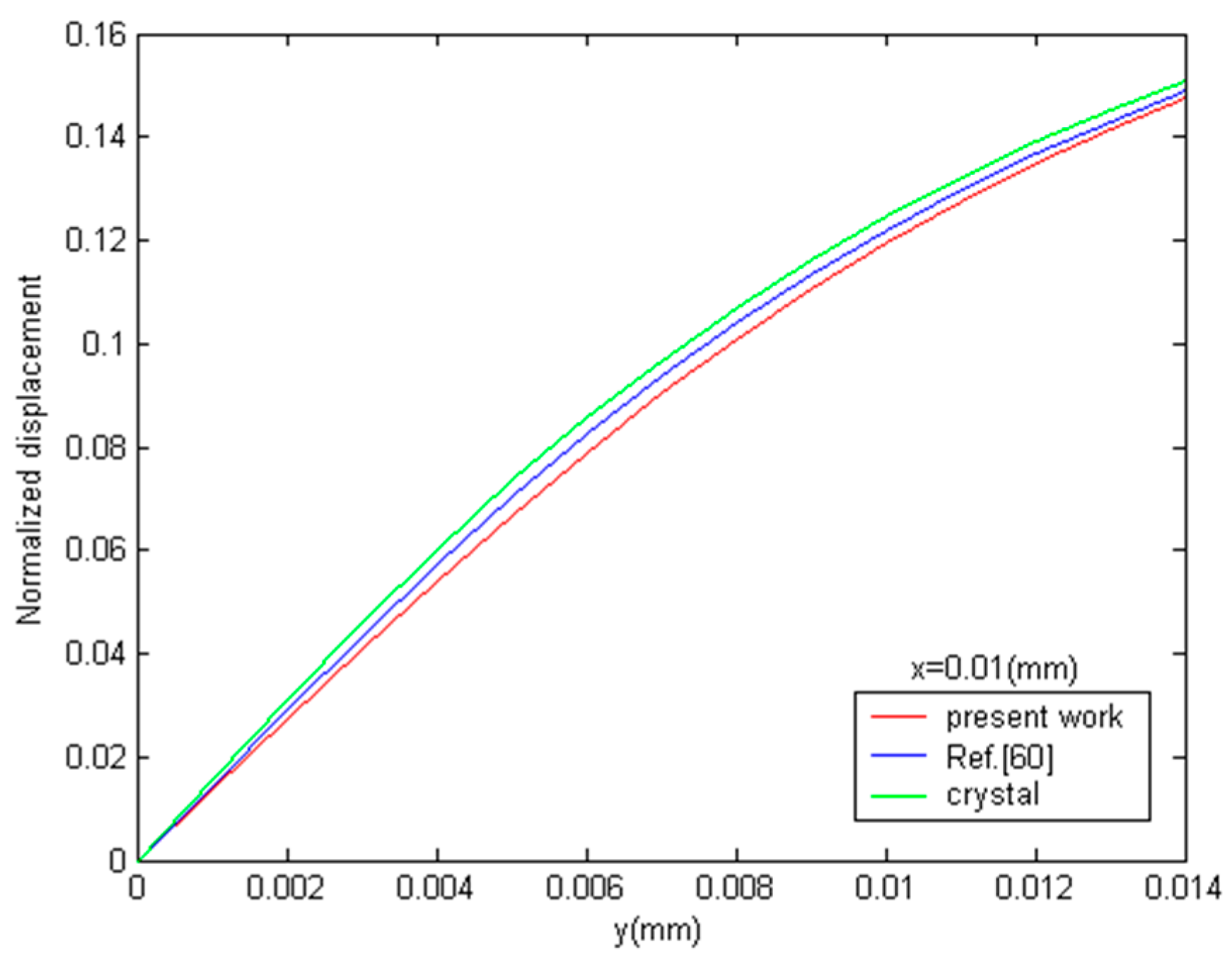

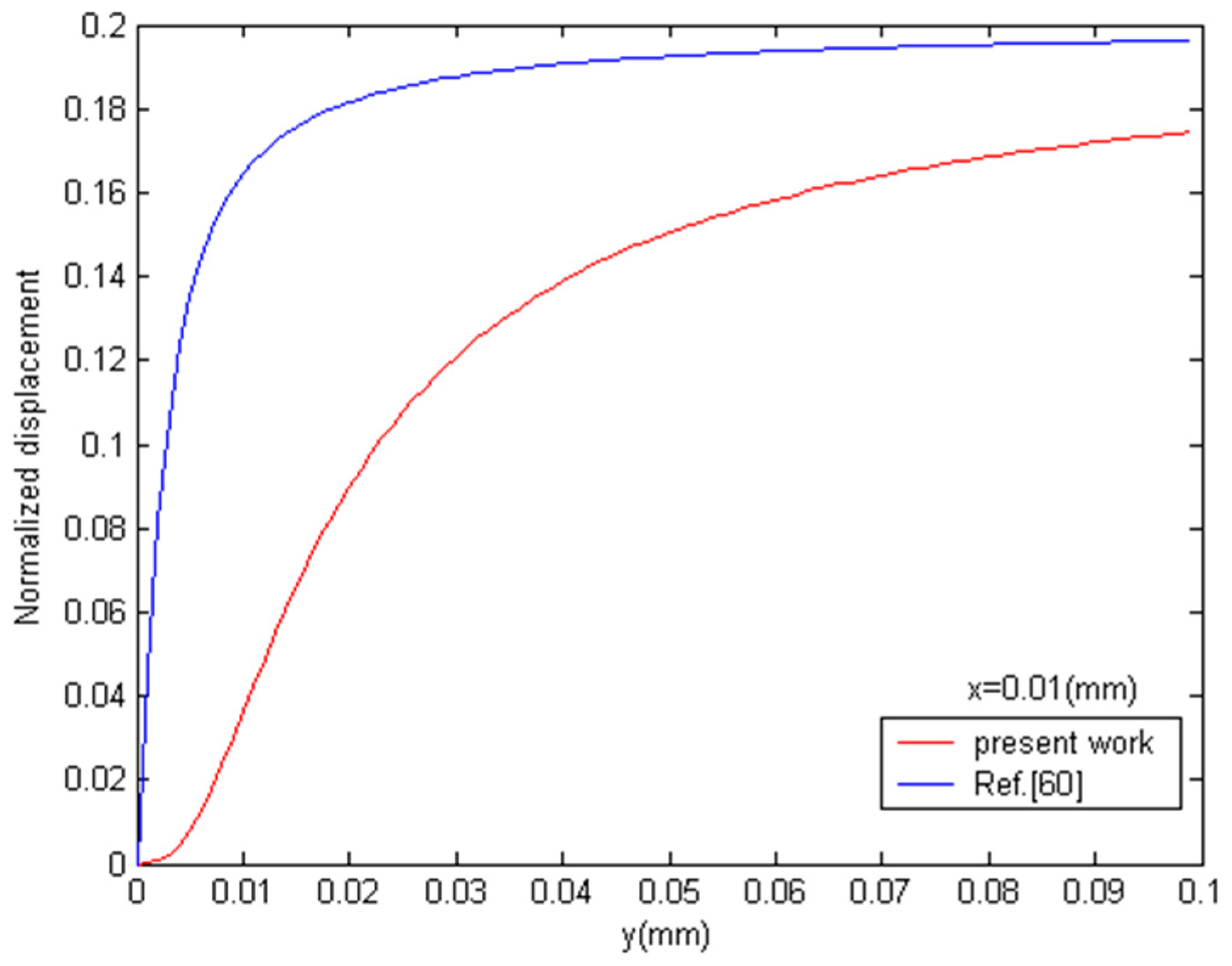
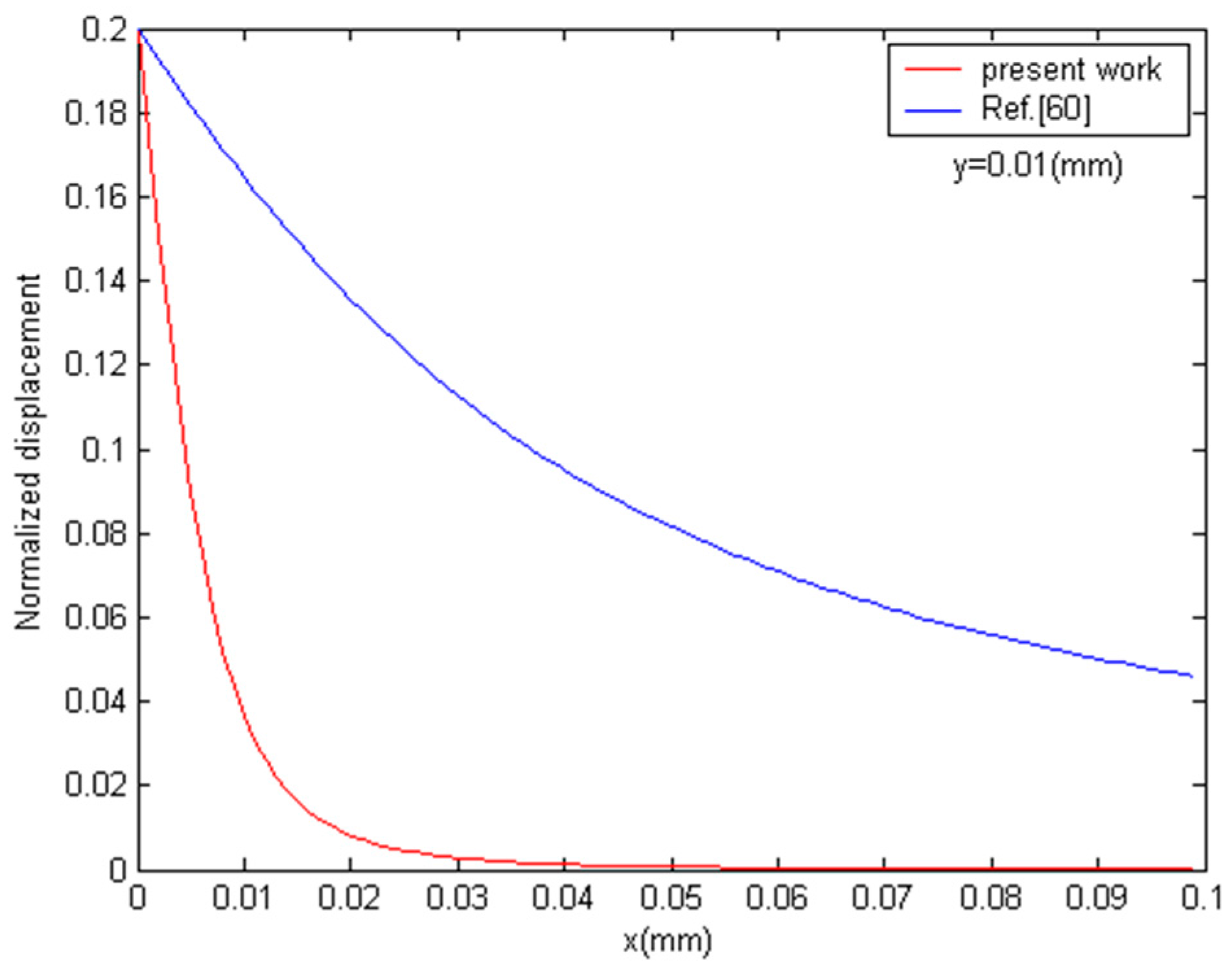

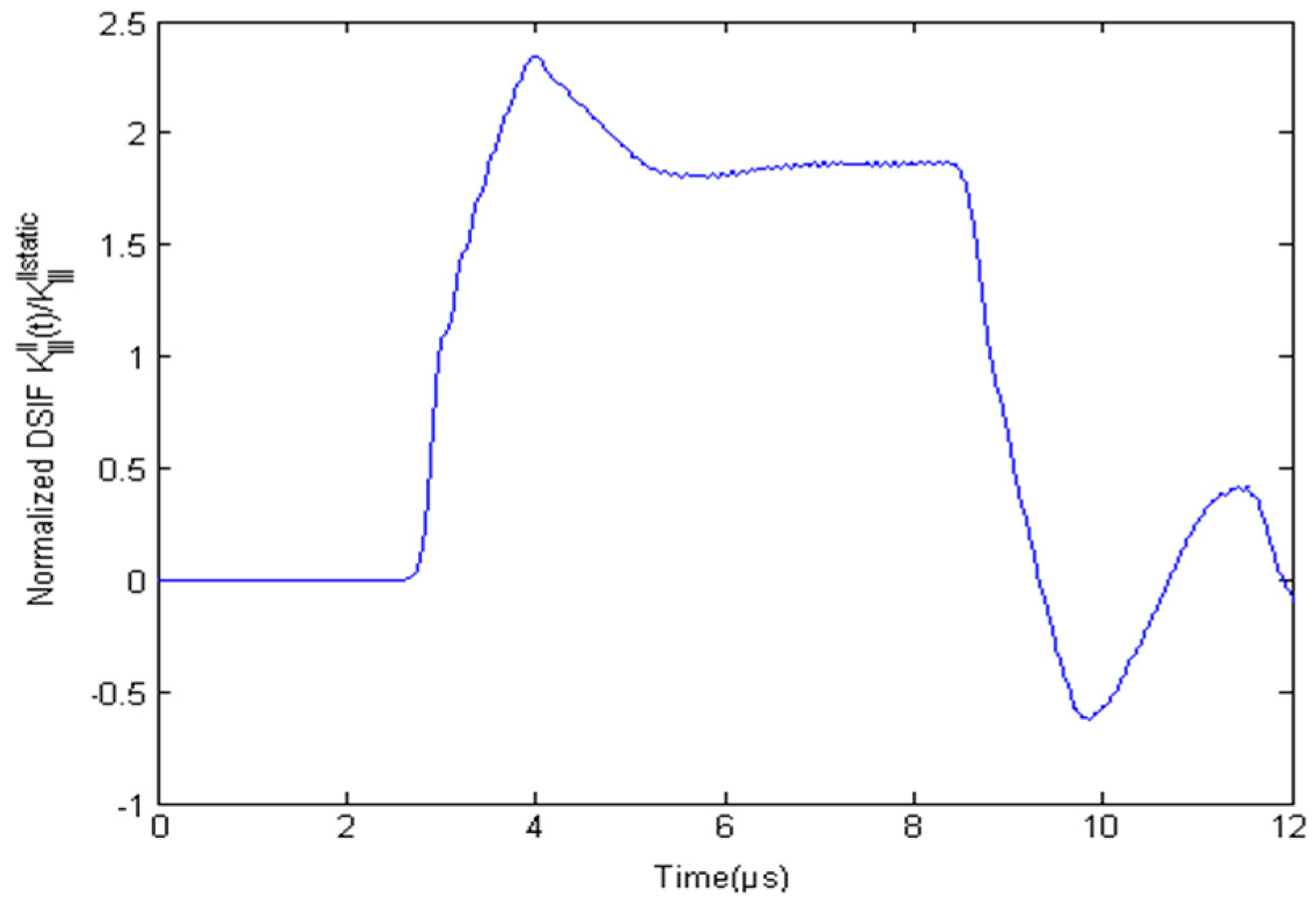
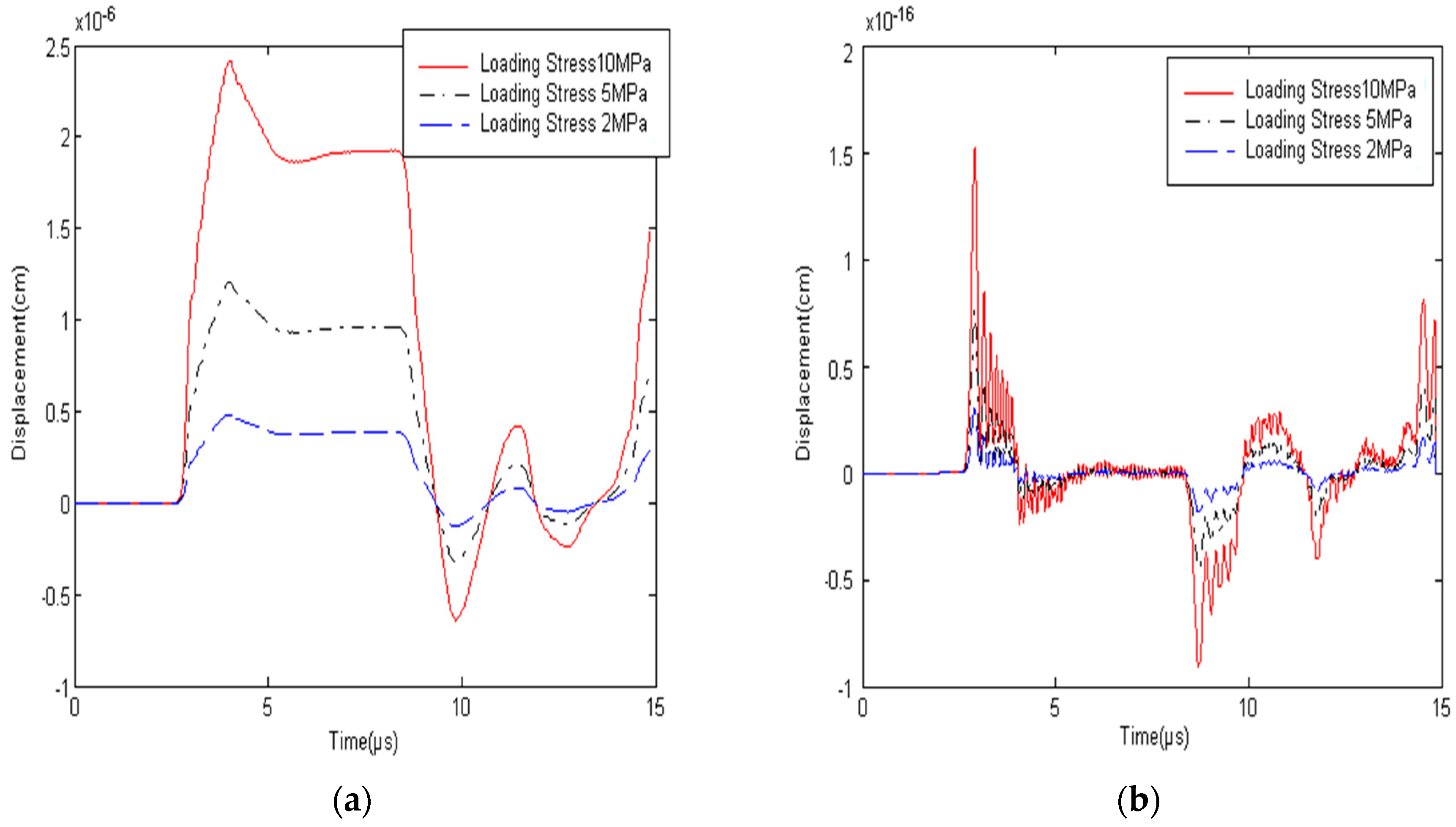
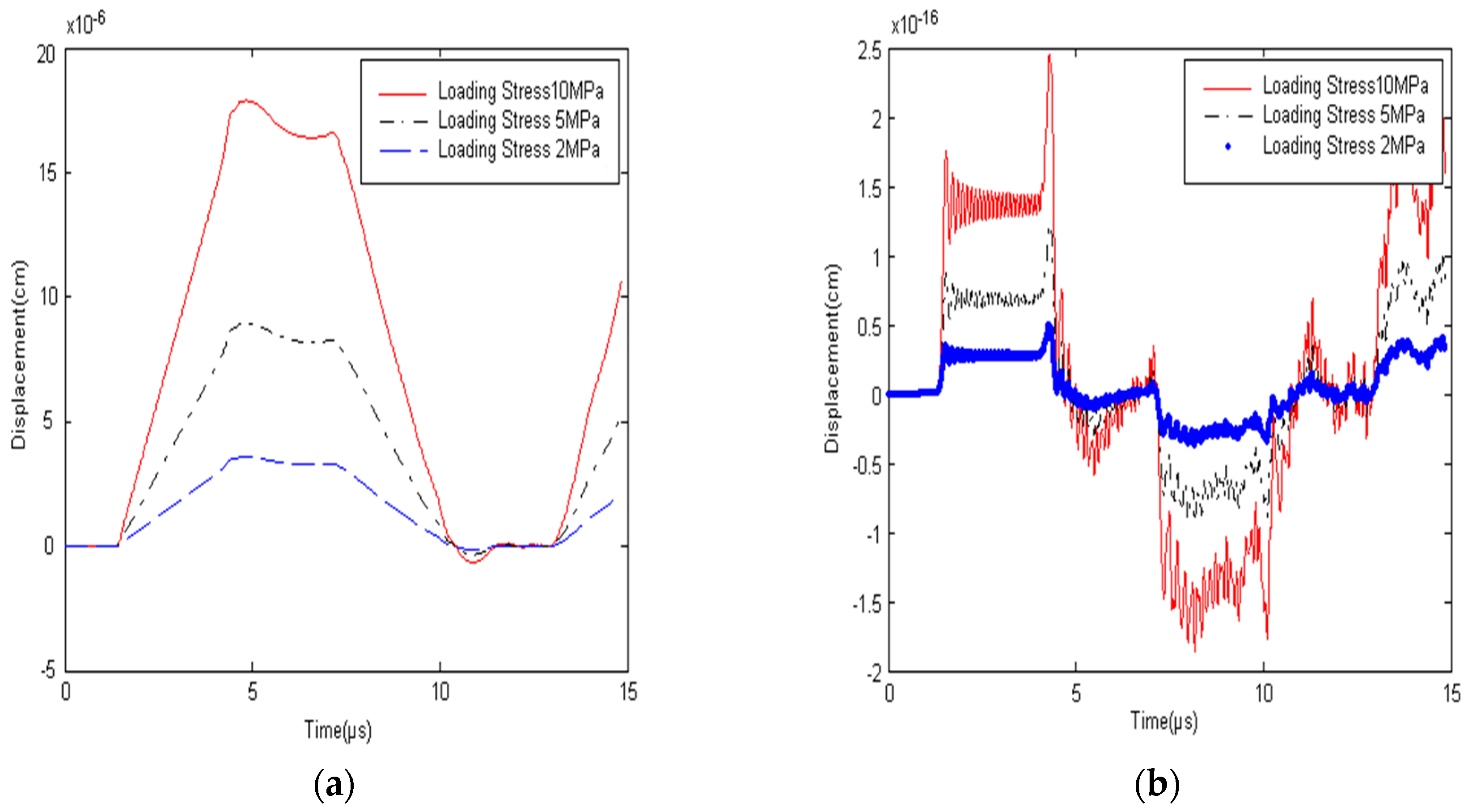
 ) and crack propagation speed (
) and crack propagation speed (  ) for (a); and the stress and displacement near the crack tip for (b).
) for (a); and the stress and displacement near the crack tip for (b).
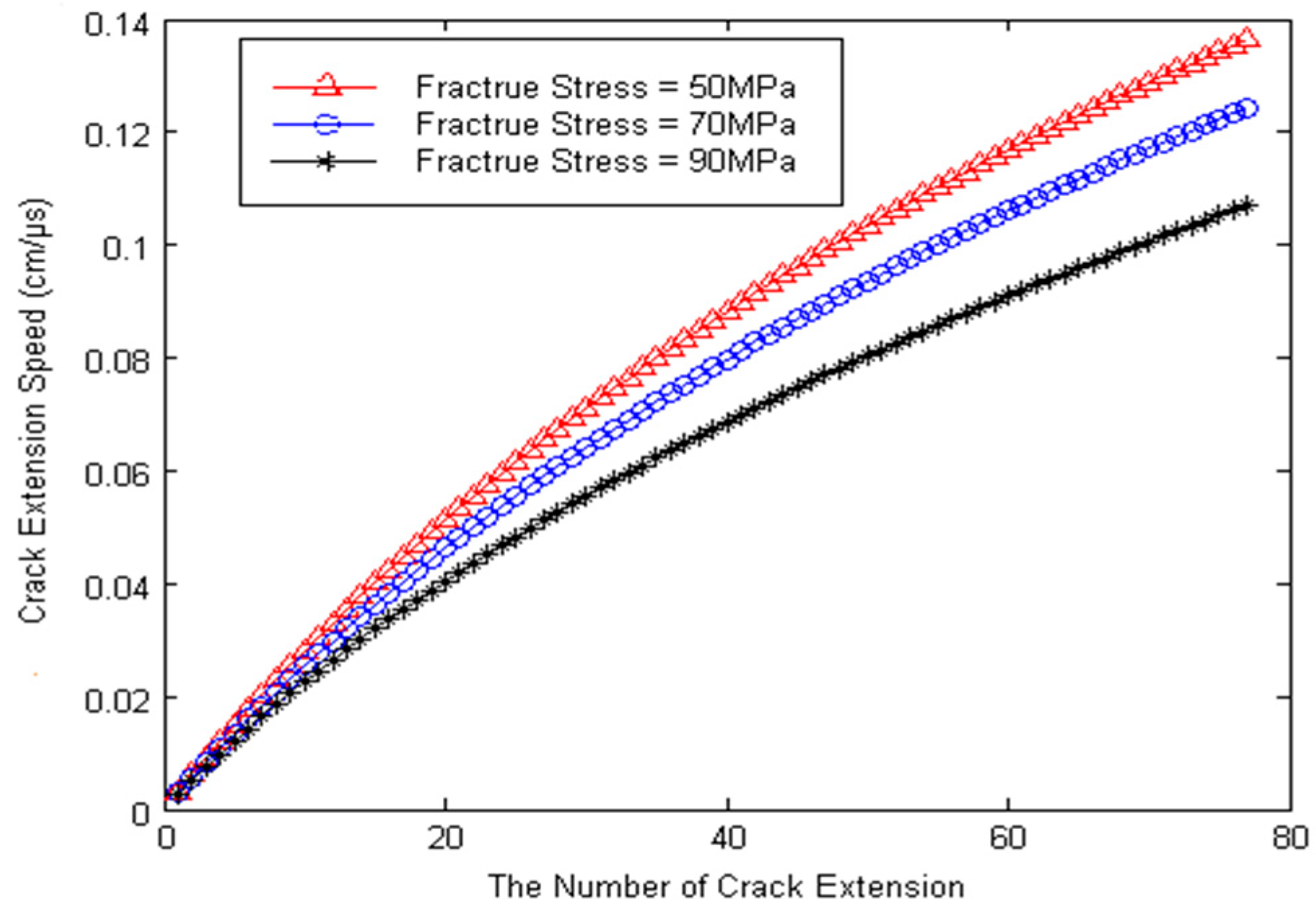
 ) and crack propagation speed (
) and crack propagation speed (  ) for (a); and the stress and displacement near the crack tip for (b).
) for (a); and the stress and displacement near the crack tip for (b).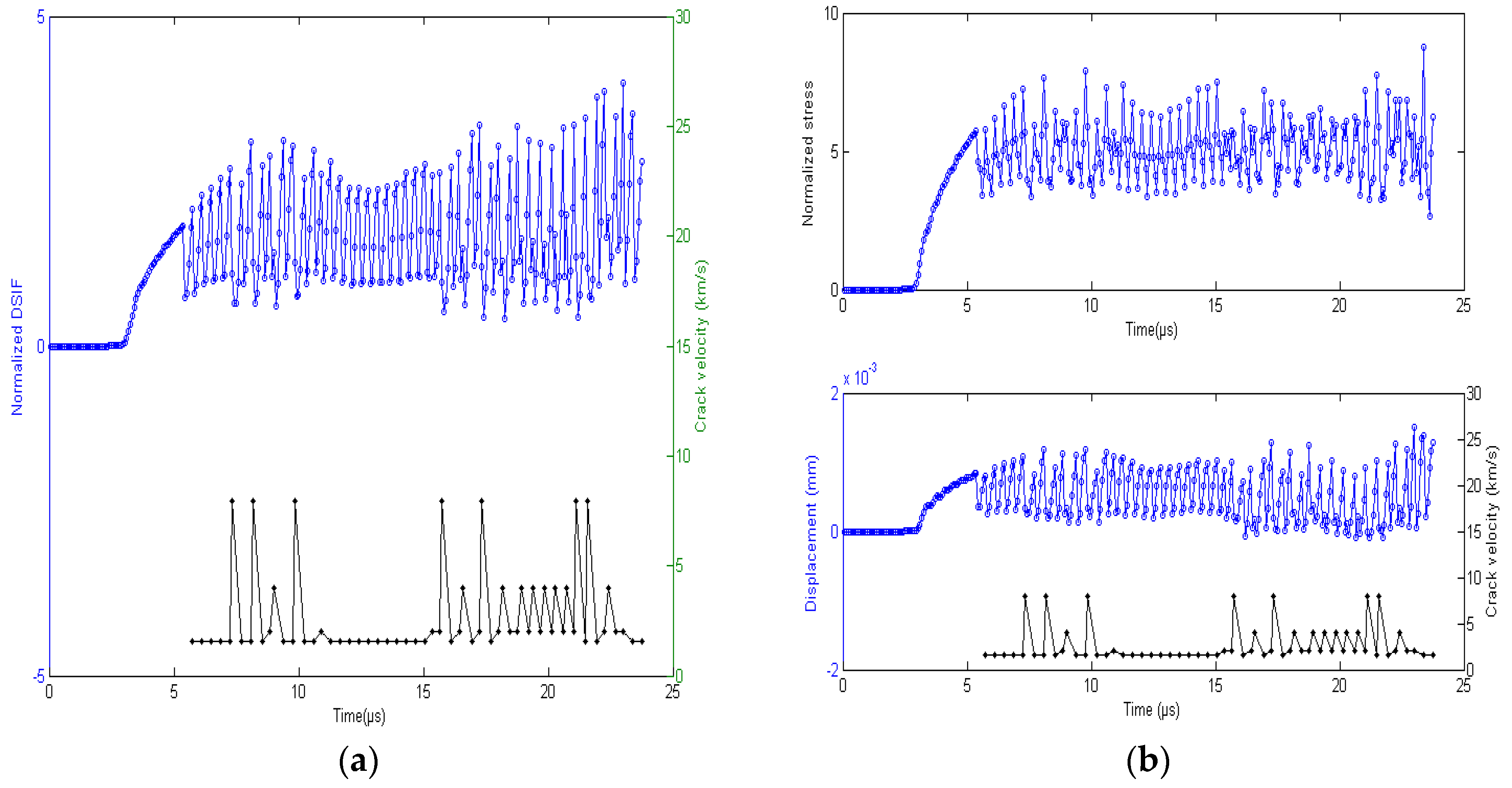
 ) and crack propagation speed (
) and crack propagation speed (  ) for (a); and the stress and displacement near the crack tip for (b).
) for (a); and the stress and displacement near the crack tip for (b).
 ) and crack propagation speed (
) and crack propagation speed (  ) for (a); and the stress and displacement near the crack tip for (b).
) for (a); and the stress and displacement near the crack tip for (b).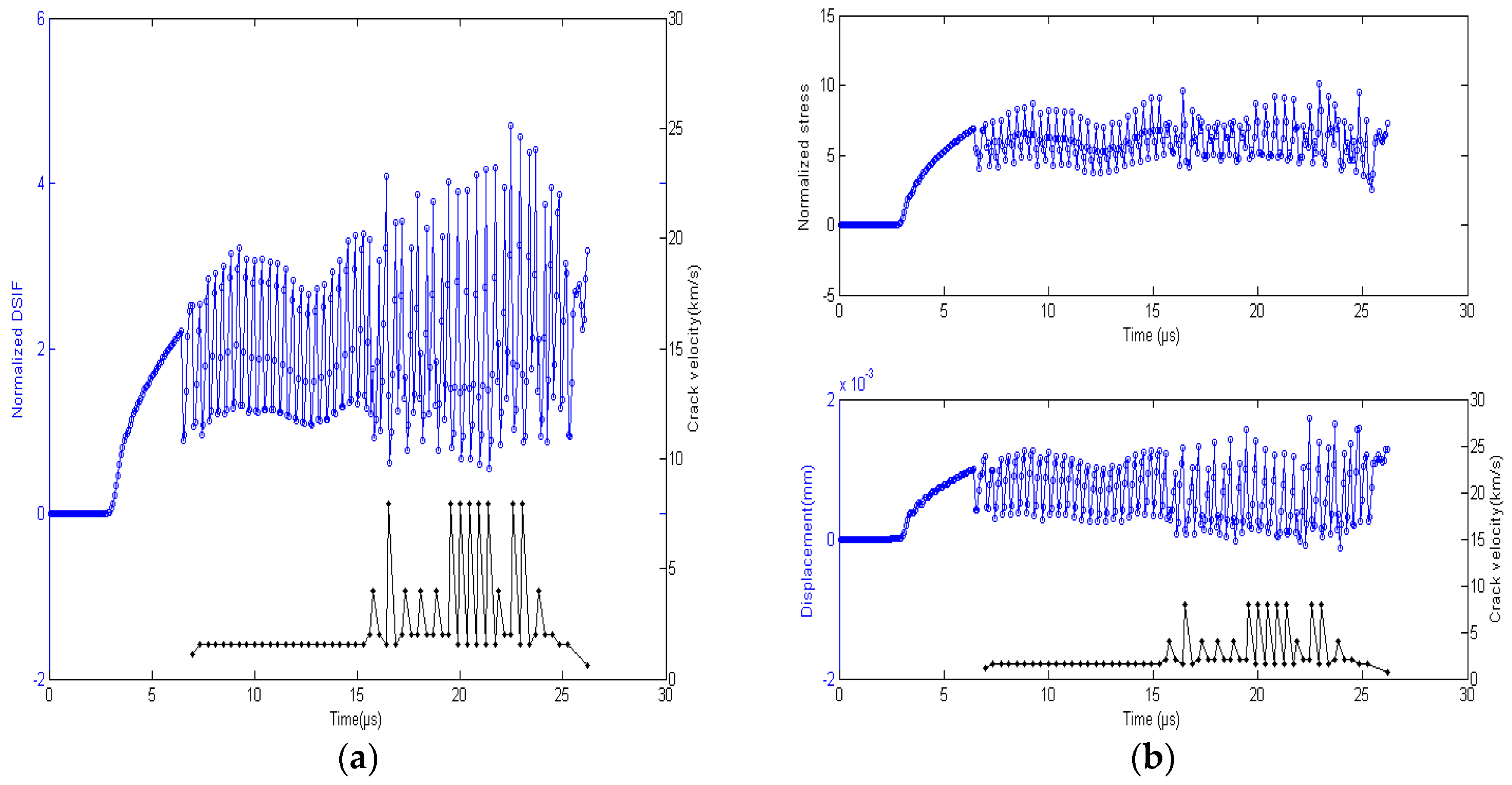
 ) and crack propagation speed (
) and crack propagation speed (  ) for (a); and the stress and displacement near the crack tip for (b).
) for (a); and the stress and displacement near the crack tip for (b).
 ) and crack propagation speed (
) and crack propagation speed (  ) for (a); and the stress and displacement near the crack tip for (b).
) for (a); and the stress and displacement near the crack tip for (b).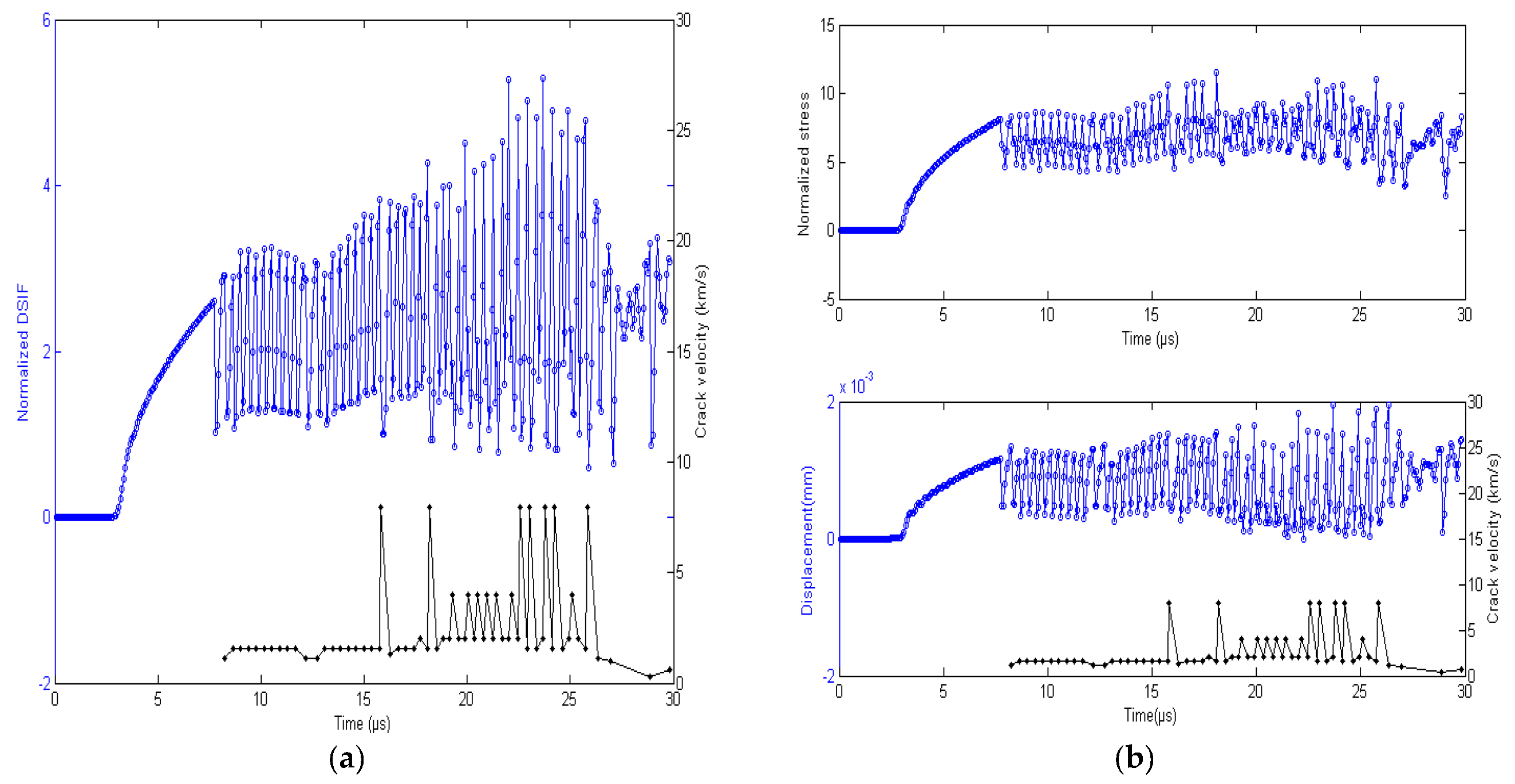


© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, W.; Fan, T. Elasto-Dynamics of Quasicrystals. Crystals 2016, 6, 152. https://doi.org/10.3390/cryst6110152
Li W, Fan T. Elasto-Dynamics of Quasicrystals. Crystals. 2016; 6(11):152. https://doi.org/10.3390/cryst6110152
Chicago/Turabian StyleLi, Wu, and Tianyou Fan. 2016. "Elasto-Dynamics of Quasicrystals" Crystals 6, no. 11: 152. https://doi.org/10.3390/cryst6110152
APA StyleLi, W., & Fan, T. (2016). Elasto-Dynamics of Quasicrystals. Crystals, 6(11), 152. https://doi.org/10.3390/cryst6110152





