Tailoring the Functional Properties of Ferroelectric Perovskite Thin Films: Mechanisms of Dielectric and Photoelectrochemical Enhancement
Abstract
1. Introduction
2. Ab Initio Studies
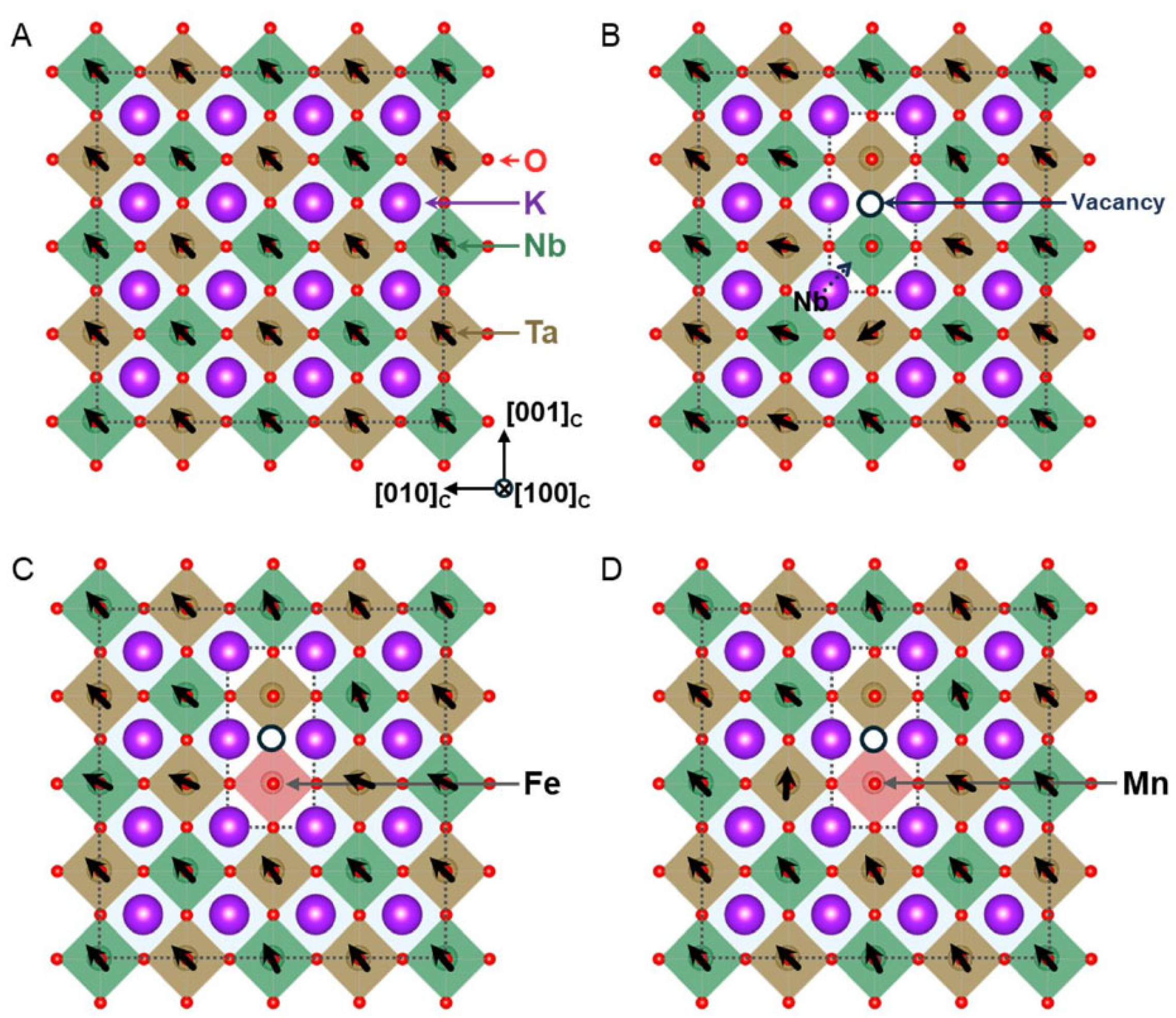
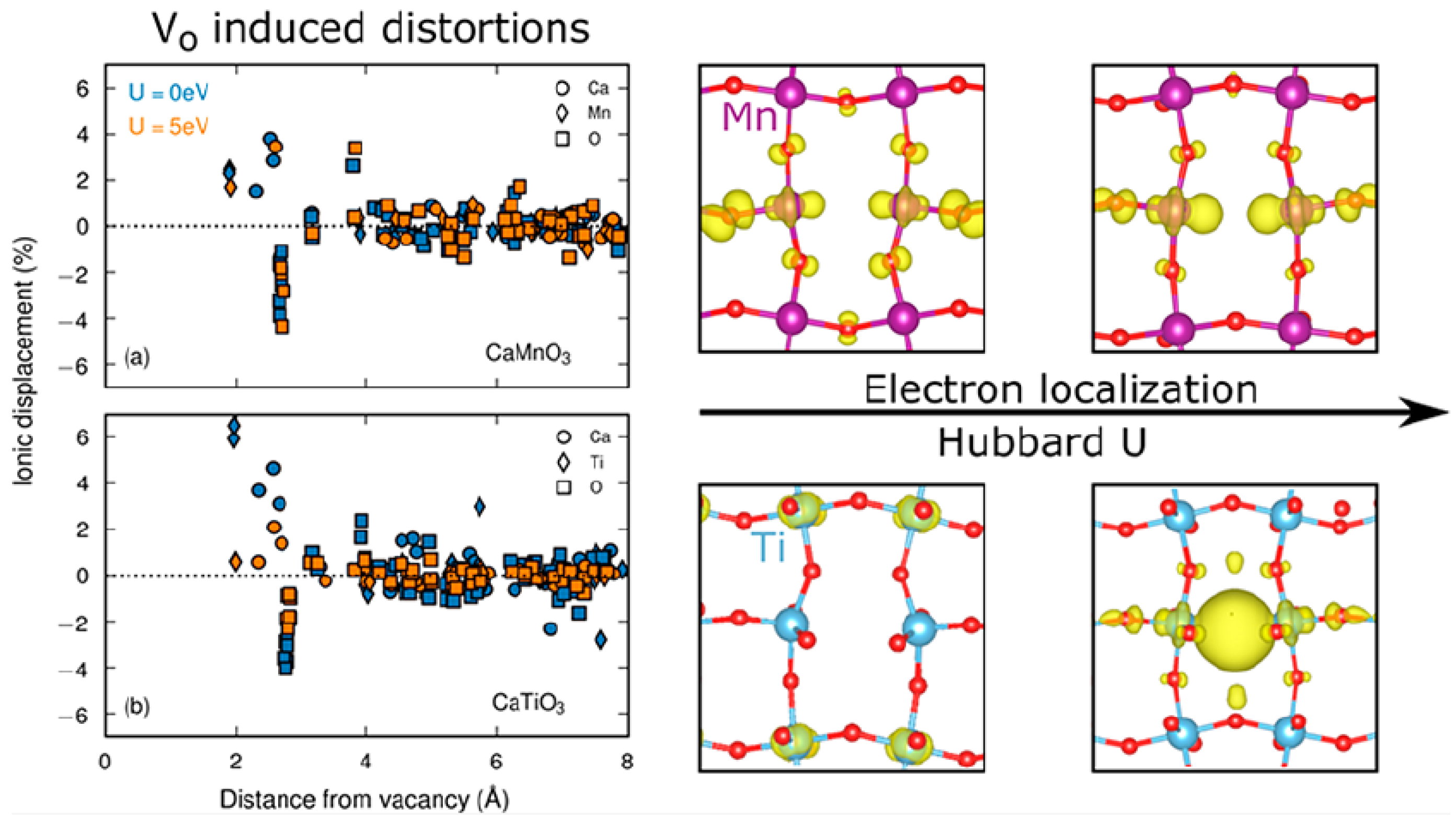
2.1. Doping-Induced Enhancement of Functional Properties
2.2. Strain-Induced Enhancement of Dielectric, Ferroelectric and Photoelectrochemical Properties
2.3. Freestanding Structures and 2D Materials
3. Phase-Field Simulations
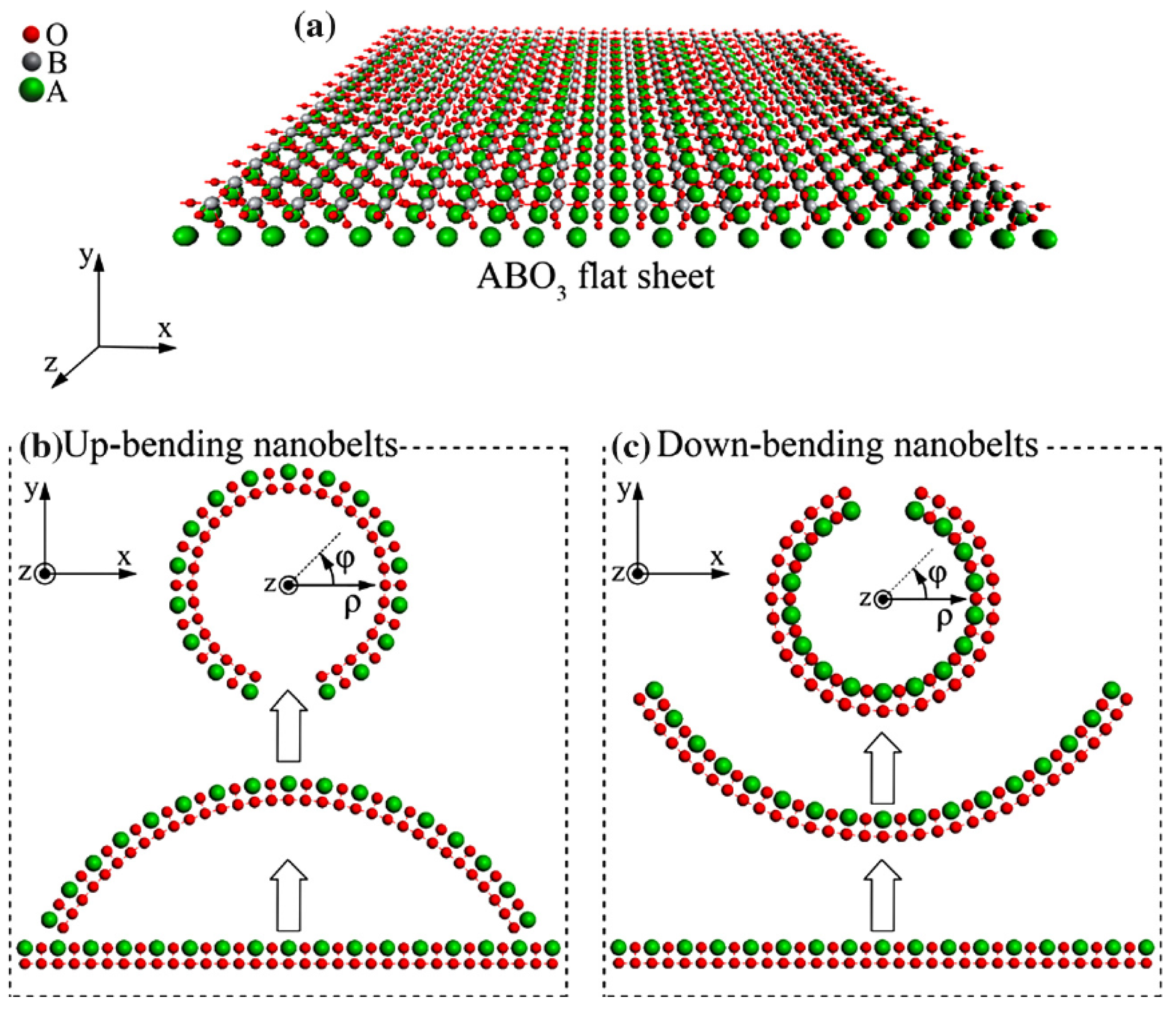
3.1. Freestanding Ferroelectric Membranes
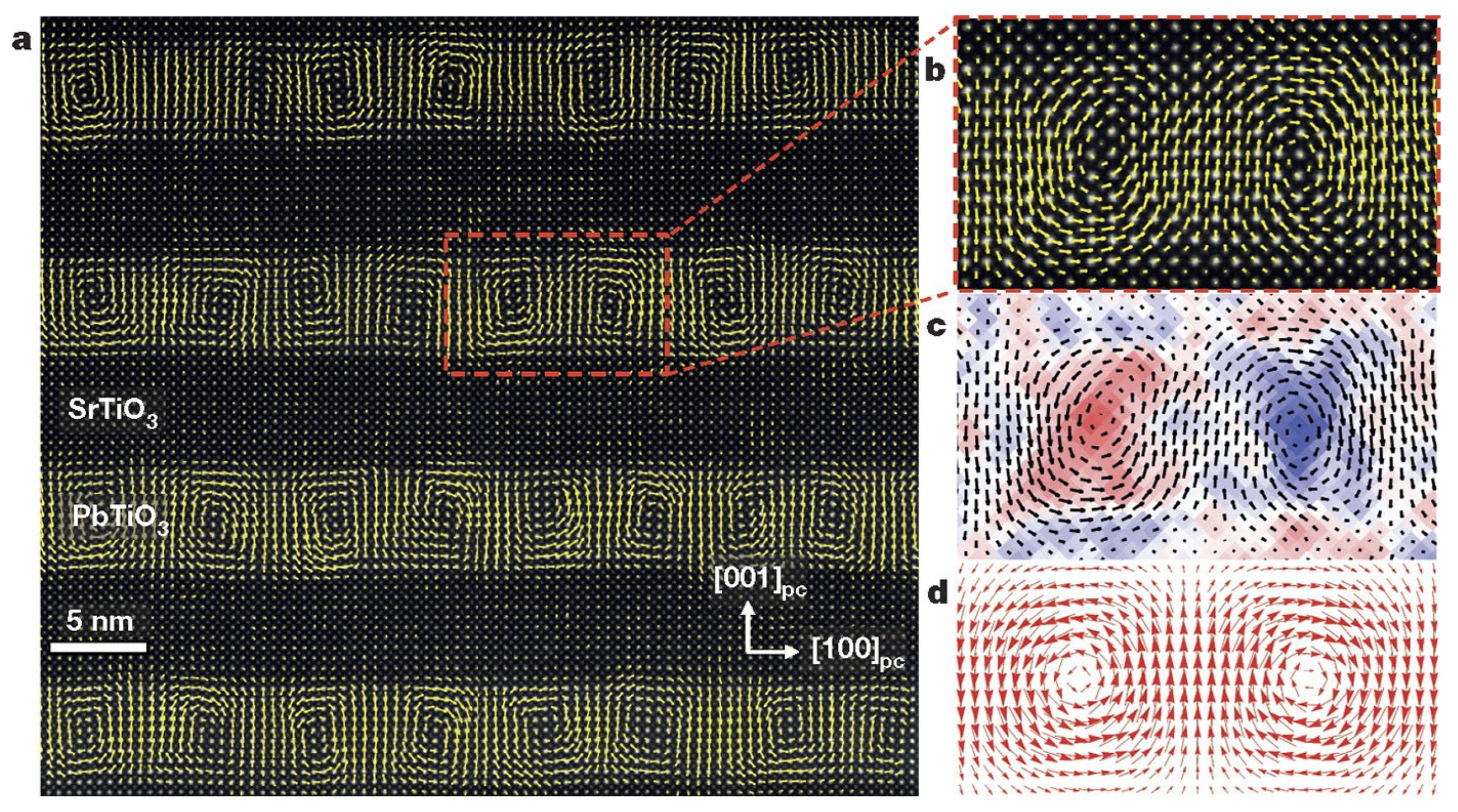
3.2. Topological Structures
3.3. Chemical, Defect and Epitaxial Strain Engineering for Enchanced Functional Properties

4. Experimental Considerations on the Theoretical Approaches
- -
- Strain control beyond the substrate’s influence. The role played by freestanding thin films or heterostructures, as well as interface layers, in overcoming this problem will be very important, as the range of applications for nanodevices is increasing. Different other forms of induced strain have the potential to be used, such as thermal strain.
- -
- Improvements in the control, stability and regulation of polarization in ferroelectric perovskite thin films. Of particular interest for PEC applications, this task is still challenging, due to the fact that any external applied electric field can have detrimental effects on the chemical properties of the sample in in situ PEC systems. Further studies are needed to clarify the real effects of ferroelectric polarization on the whole range of photoelectrochemical processes.
- -
- New materials or heterostructure architectures that can increase PEC performances through stronger polarization or the harvesting of a wider range of external stimuli. Here, freestanding thin films, stand-alone or associated with 2D materials, could have an important role.
- -
- Improvements of the deposition/processing techniques in terms of control of the stoichiometric growth/chemical doping or induced nanostructured features both on the thin film’s surface and in bulk.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sharma, P.; Zhang, Q.; Sando, D.; Lei, C.H.; Liu, Y.; Li, J.; Nagarajan, V.; Seidel, J. Nonvolatile Ferroelectric Domain Wall Memory. Sci. Adv. 2017, 3, e1700512. [Google Scholar] [CrossRef] [PubMed]
- Catalan, G.; Seidel, J.; Ramesh, R.; Scott, J.F. Domain Wall Nanoelectronics. Rev. Mod. Phys. 2012, 84, 119–156. [Google Scholar] [CrossRef]
- Scarisoreanu, N.D.; Craciun, F.; Birjega, R.; Ion, V.; Teodorescu, V.S.; Ghica, C.; Negrea, R.; Dinescu, M. Joining Chemical Pressure and Epitaxial Strain to Yield Y-Doped BiFeO3 Thin Films with High Dielectric Response. Sci. Rep. 2016, 6, 25535. [Google Scholar] [CrossRef]
- Scarisoreanu, N.D.; Craciun, F.; Moldovan, A.; Ion, V.; Birjega, R.; Ghica, C.; Negrea, R.F.; Dinescu, M. High Permittivity (1 − x)Ba(Zr0.2 Ti0.8)O3−x (Ba0.7 Ca0.3)TiO3 (x = 0.45) Epitaxial Thin Films with Nanoscale Phase Fluctuations. ACS Appl. Mater. Interfaces 2015, 7, 23984–23992. [Google Scholar] [CrossRef]
- Han, X.; Ji, Y.; Yang, Y. Ferroelectric Photovoltaic Materials and Devices. Adv. Funct. Mater. 2022, 32, 2109625. [Google Scholar] [CrossRef]
- Zhang, K.; Li, H.; Mu, H.; Li, Y.; Wang, P.; Wang, Y.; Chen, T.; Yuan, J.; Chen, W.; Yu, W.; et al. Spatially Resolved Light-Induced Ferroelectric Polarization in A-In2Se3/Te Heterojunctions. Adv. Mater. 2024, 36, 2405233. [Google Scholar] [CrossRef]
- Yang, L.; Xiong, Y.; Guo, W.; Zhou, M.; Song, K.; Xiao, P.; Cao, G. Manipulation of Charge Transport in Ferroelectric-Semiconductor Hybrid for Photoelectrochemical Applications. Nano Energy 2018, 44, 63–72. [Google Scholar] [CrossRef]
- Andrei, F.; Ion, V.; Birjega, R.; Ghitiu, I.; Zamfir, M.; Moldovan, A.; Teodorescu, V.S.; Dinescu, M.; Marcu, I.-C.; Scarisoreanu, N.D. Strain Engineering of Epitaxial Perovskite-Type LaFeO3/BiFeO3 Heterostructures for Photoelectrochemical Water Splitting. Surf. Interfaces 2025, 62, 106234. [Google Scholar] [CrossRef]
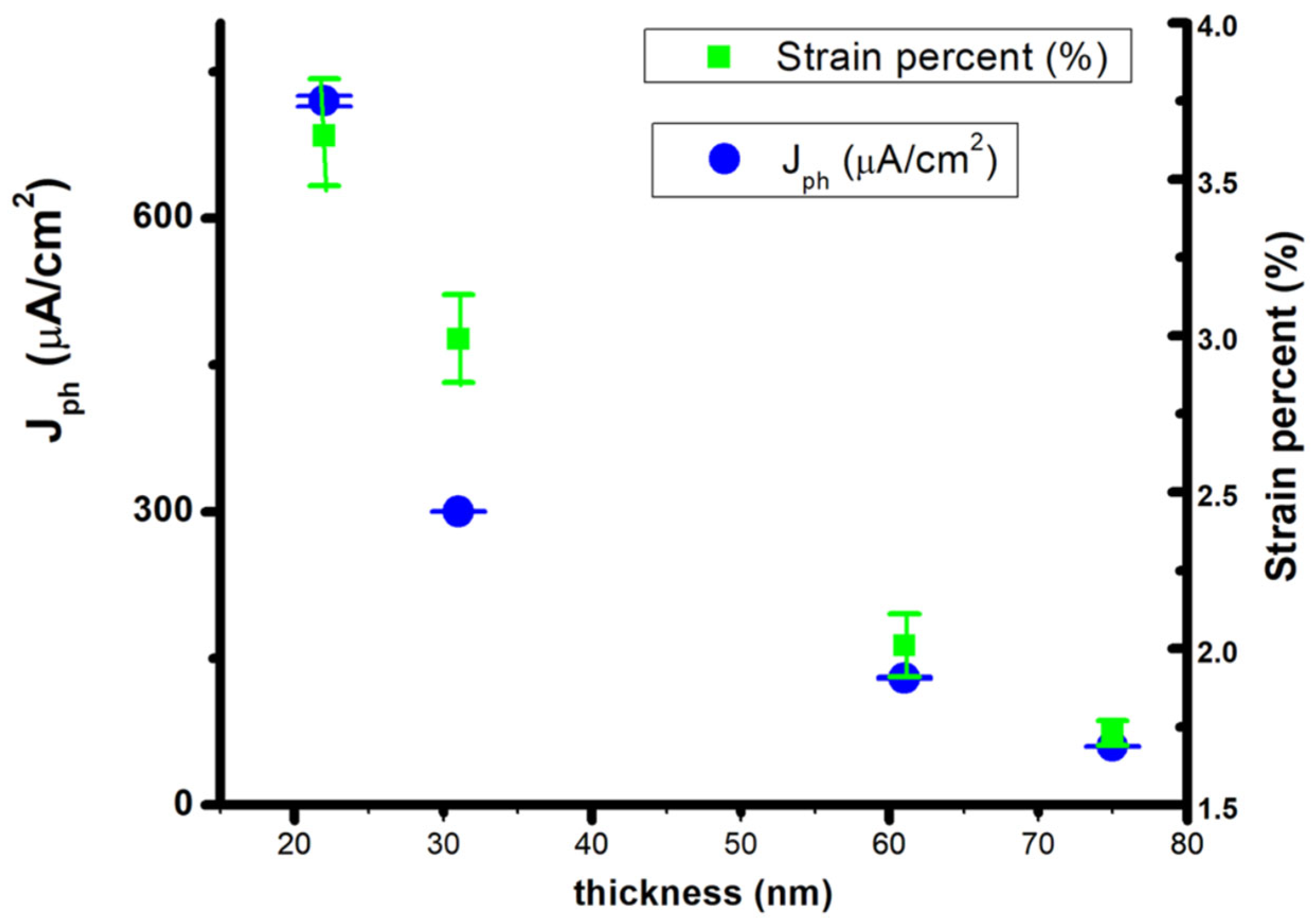
- Andrei, F.; Ion, V.; Bîrjega, R.; Dinescu, M.; Enea, N.; Pantelica, D.; Mihai, M.D.; Maraloiu, V.-A.; Teodorescu, V.S.; Marcu, I.-C.; et al. Thickness-Dependent Photoelectrochemical Water Splitting Properties of Self-Assembled Nanostructured LaFeO3 Perovskite Thin Films. Nanomaterials 2021, 11, 1371. [Google Scholar] [CrossRef]
- Ion, V.; Teodorescu, V.; Birjega, R.; Dinescu, M.; Mitterbauer, C.; Alexandrou, I.; Ghitiu, I.; Craciun, F.; Scarisoreanu, N.D. Lead-Free Perovskite Thin Films with Tailored Pockels-Kerr Effects for Photonics. ACS Appl. Mater. Interfaces 2023, 15, 38039–38048. [Google Scholar] [CrossRef]
- Tao, Q.; Xu, P.; Li, M.; Lu, W. Machine Learning for Perovskite Materials Design and Discovery. NPJ Comput. Mater. 2021, 7, 23. [Google Scholar] [CrossRef]
- Frey, R.; Grosso, B.F.; Fandré, P.; Mächler, B.; Spaldin, N.A.; Mansouri Tehrani, A. Accelerated Search for New Ferroelectric Materials. Phys. Rev. Res. 2023, 5, 023122. [Google Scholar] [CrossRef]
- Sun, Y.; Wang, X.; Hou, C.; Ni, J. Interpretable Machine Learning to Discover Perovskites with High Spontaneous Polarization. J. Phys. Chem. C 2023, 127, 23897–23905. [Google Scholar] [CrossRef]
- Nagai, R.; Akashi, R.; Sugino, O. Completing Density Functional Theory by Machine Learning Hidden Messages from Molecules. NPJ Comput. Mater. 2020, 6, 43. [Google Scholar] [CrossRef]
- del Rio, B.G.; Phan, B.; Ramprasad, R. A Deep Learning Framework to Emulate Density Functional Theory. NPJ Comput. Mater. 2023, 9, 158. [Google Scholar] [CrossRef]
- Alhada-Lahbabi, K.; Deleruyelle, D.; Gautier, B. Machine Learning Surrogate Model for Acceleration of Ferroelectric Phase-Field Modeling. ACS Appl. Electron. Mater. 2023, 5, 3894–3907. [Google Scholar] [CrossRef]
- Mai, H.; Le, T.C.; Chen, D.; Winkler, D.A.; Caruso, R.A. Machine Learning for Electrocatalyst and Photocatalyst Design and Discovery. Chem. Rev. 2022, 122, 13478–13515. [Google Scholar] [CrossRef]
- Haydous, F.; Scarisoreanu, N.D.; Birjega, R.; Ion, V.; Lippert, T.; Dumitrescu, N.; Moldovan, A.; Andrei, A.; Teodorescu, V.S.; Ghica, C.; et al. Rolling Dopant and Strain in Y-Doped BiFeO3 Epitaxial Thin Films for Photoelectrochemical Water Splitting. Sci. Rep. 2018, 8, 15826. [Google Scholar] [CrossRef]
- Seidel, J.; Fu, D.; Yang, S.-Y.; Alarcón-Lladó, E.; Wu, J.; Ramesh, R.; Ager, J.W. Efficient Photovoltaic Current Generation at Ferroelectric Domain Walls. Phys. Rev. Lett. 2011, 107, 126805. [Google Scholar] [CrossRef]
- Huang, H. Ferroelectric Photovoltaics. Nat. Photonics 2010, 4, 134–135. [Google Scholar] [CrossRef]
- Catalan, G.; Scott, J.F. Physics and Applications of Bismuth Ferrite. Adv. Mater. 2009, 21, 2463–2485. [Google Scholar] [CrossRef]
- Tijare, S.N.; Joshi, M.V.; Padole, P.S.; Mangrulkar, P.A.; Rayalu, S.S.; Labhsetwar, N.K. Photocatalytic Hydrogen Generation through Water Splitting on Nano-Crystalline LaFeO3 Perovskite. Int. J. Hydrogen Energy 2012, 37, 10451–10456. [Google Scholar] [CrossRef]
- Liu, Z.K.; Li, X.; Zhang, Q.M. Maximizing the Number of Coexisting Phases near Invariant Critical Points for Giant Electrocaloric and Electromechanical Responses in Ferroelectrics. Appl. Phys. Lett. 2012, 101, 082904. [Google Scholar] [CrossRef]
- Bard, A.J. Photoelectrochemistry. Science (1979) 1980, 207, 139–144. [Google Scholar] [CrossRef]
- Kim, T.W.; Choi, K.-S. Nanoporous BiVO4 Photoanodes with Dual-Layer Oxygen Evolution Catalysts for Solar Water Splitting. Science (1979) 2014, 343, 990–994. [Google Scholar] [CrossRef]
- Wang, J.; Neaton, J.B.; Zheng, H.; Nagarajan, V.; Ogale, S.B.; Liu, B.; Viehland, D.; Vaithyanathan, V.; Schlom, D.G.; Waghmare, U.V.; et al. Epitaxial BiFeO3 Multiferroic Thin Film Heterostructures. Science (1979) 2003, 299, 1719–1722. [Google Scholar] [CrossRef]
- Tang, P.; Chen, H.; Cao, F.; Pan, G. Magnetically Recoverable and Visible-Light-Driven Nanocrystalline YFeO3 Photocatalysts. Catal. Sci. Technol. 2011, 1, 1145. [Google Scholar] [CrossRef]
- Ramachandran, B.; Ramachandra Rao, M.S. Chemical Pressure Effect on Optical Properties in Multiferroic Bulk BiFeO3. J. Appl. Phys. 2012, 112, 073516. [Google Scholar] [CrossRef]
- Fischer, P.; Polomska, M.; Sosnowska, I.; Szymanski, M. Temperature Dependence of the Crystal and Magnetic Structures of BiFeO3. J. Phys. C Solid. State Phys. 1980, 13, 1931–1940. [Google Scholar] [CrossRef]
- Kamba, S.; Nuzhnyy, D.; Savinov, M.; Šebek, J.; Petzelt, J.; Prokleška, J.; Haumont, R.; Kreisel, J. Infrared and Terahertz Studies of Polar Phonons and Magnetodielectric Effect in Multiferroic BiFeO3 Ceramics. Phys. Rev. B 2007, 75, 024403. [Google Scholar] [CrossRef]
- Lacerda, L.H.d.S.; Ribeiro, R.A.P.; de Lázaro, S.R. DFT Approaches for Smart Materials with Ferroelectric Properties. In Functional Properties of Advanced Engineering Materials and Biomolecules; Springer: Cham, Switzerland, 2021; pp. 317–334. [Google Scholar]
- Tan, P.; Huang, X.; Wang, Y.; Xing, B.; Zhang, J.; Hu, C.; Meng, X.; Xu, X.; Li, D.; Wang, X.; et al. Deciphering the Atomistic Mechanism Underlying Highly Tunable Piezoelectric Properties in Perovskite Ferroelectrics via Transition Metal Doping. Nat. Commun. 2024, 15, 10619. [Google Scholar] [CrossRef] [PubMed]
- Giannozzi, P.; Baroni, S.; Bonini, N.; Calandra, M.; Car, R.; Cavazzoni, C.; Ceresoli, D.; Chiarotti, G.L.; Cococcioni, M.; Dabo, I.; et al. QUANTUM ESPRESSO: A Modular and Open-Source Software Project for Quantum Simulations of Materials. J. Phys. Condens. Matter 2009, 21, 395502. [Google Scholar] [CrossRef] [PubMed]
- Wang, T.; Gong, F.; Ma, X.; Pan, S.; Wei, X.-K.; Kuo, C.; Yoshida, S.; Ku, Y.-C.; Wang, S.; Yang, Z.; et al. Large Enhancement of Ferroelectric Properties of Perovskite Oxides via Nitrogen Incorporation. Sci. Adv. 2025, 11, eads8830. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Hafner, J. Ab Initio Molecular Dynamics for Liquid Metals. Phys. Rev. B 1993, 47, 558–561. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Hafner, J. Ab Initio Molecular-Dynamics Simulation of the Liquid-Metal–Amorphous-Semiconductor Transition in Germanium. Phys. Rev. B 1994, 49, 14251–14269. [Google Scholar] [CrossRef]
- Clark, S.J.; Segall, M.D.; Pickard, C.J.; Hasnip, P.J.; Probert, M.I.J.; Refson, K.; Payne, M.C. First Principles Methods Using CASTEP. Z. Kristallogr Cryst. Mater. 2005, 220, 567–570. [Google Scholar] [CrossRef]
- Eno, E.A.; Etiese, D.; Pathmanathan, K.; Agwamba, E.C.; Chukwu, U.G.; Magu, T.O.; Ikeuba, A.I.; Adeyinka, A.S.; Louis, H. Ab-Initio Study of Structural, Electronic, Phonon, X-Ray Spectroscopy, and the Optoelectronic Properties of D-Block Metals (Cr, Mn, Co, and Ni) Substitution of Barium Oxide Based-Perovskites. Chem. Phys. Impact 2023, 7, 100321. [Google Scholar] [CrossRef]
- Islam, M.Z.; Hasan, M.; Rahman, M.F.; Rhaman, M.M. Enhanced Optoelectronic Properties of Ca, Mg, Cr-Doped Half Metallic BaNpO3. SSRN Electron. J. 2023. [Google Scholar] [CrossRef]
- Kacimi-Naciri, H.; Rguiti, M.; Mabrouk, A.; Courtois, C.; Ben Achour, M.A.; Lorgouilloux, Y.; Amrousse, R.; Faska, N.; Bachar, A. DFT-Based and Experimental Study on Sr-Doped BaTiO3: Impacts on Piezoelectric and Ferroelectric Performance. Ceram. Int. 2025, in press. [Google Scholar] [CrossRef]
- Kang, K.T.; Seo, H.I.; Kwon, O.; Lee, K.; Bae, J.-S.; Chu, M.-W.; Chae, S.C.; Kim, Y.; Choi, W.S. Ferroelectricity in SrTiO3 Epitaxial Thin Films via Sr-Vacancy-Induced Tetragonality. Appl. Surf. Sci. 2020, 499, 143930. [Google Scholar] [CrossRef]
- Ou-khouya, A.; Ait Brahim, I.; Ez-Zahraouy, H.; Houba, A.; Mes-Adi, H.; Tahiri, M. First-Principles Calculations to Investigate Impact of Doping by Chalcogen Elements on the Electronic, Structural, and Optical Properties of SrTiO3 Compounds. Chem. Phys. 2024, 581, 112253. [Google Scholar] [CrossRef]
- Blaha, P.; Schwarz, K.; Tran, F.; Laskowski, R.; Madsen, G.K.H.; Marks, L.D. WIEN2k: An APW+lo Program for Calculating the Properties of Solids. J. Chem. Phys. 2020, 152, 074101. [Google Scholar] [CrossRef] [PubMed]
- Martínez-Aguilar, E.; Hmŏk, H.; Siqueiros, J.M. Effect of La Doping on the Ferroelectric and Optical Properties of BiFeO3: A Theoretical-Experimental Study. Mater. Res. Express 2019, 6, 085098. [Google Scholar] [CrossRef]
- Peng, Y.-T.; Chiou, S.-H.; Hsiao, C.-H.; Ouyang, C.; Tu, C.-S. Remarkably Enhanced Photovoltaic Effects and First-Principles Calculations in Neodymium Doped BiFeO3. Sci. Rep. 2017, 7, 45164. [Google Scholar] [CrossRef]
- Wang, Y.; Zheng, L.; Liu, P.; Chen, F.; Chen, R. Tunable Photocatalytic and Magnetic Properties in Y and Transition Metals (TM = Mn, Co, Ni, Cu, Zn) Co-Doped BiFeO3: DFT + U Study*. Ferroelectrics 2023, 615, 212–222. [Google Scholar] [CrossRef]
- Ghosh, A.; Trujillo, D.P.; Choi, H.; Nakhmanson, S.M.; Alpay, S.P.; Zhu, J.-X. Electronic and Magnetic Properties of Lanthanum and Strontium Doped Bismuth Ferrite: A First-Principles Study. Sci. Rep. 2019, 9, 194. [Google Scholar] [CrossRef] [PubMed]
- Tan, Q.; Wang, Q.; Liu, Y. Magnetic Properties and Spontaneous Polarization of La-, Mn- and N-Doped Tetragonal BiFeO3: A First-Principles Study. Materials 2018, 11, 985. [Google Scholar] [CrossRef]
- Yang, R.; Lin, S.; Fang, X.; Gao, X.; Zeng, M.; Liu, J. First-Principles Study on the Magnetic Properties in Mg Doped BiFeO3 with and without Oxygen Vacancies. J. Appl. Phys. 2013, 114, 233912. [Google Scholar] [CrossRef]
- Ahmed, T.; Chen, A.; Yarotski, D.A.; Trugman, S.A.; Jia, Q.; Zhu, J.-X. Magnetic, Electronic, and Optical Properties of Double Perovskite Bi2FeMnO6. APL Mater. 2017, 5, 035601. [Google Scholar] [CrossRef]
- Ghosh, A.; Ahmed, T.; Yarotski, D.A.; Nakhmanson, S.M.; Zhu, J.-X. Oxygen Vacancy Effects on Double Perovskite Bi2FeMnO6: A First-Principles Study. EPL (Europhys. Lett.) 2016, 116, 57002. [Google Scholar] [CrossRef]
- Machado, P.; Scigaj, M.; Gazquez, J.; Rueda, E.; Sánchez-Díaz, A.; Fina, I.; Gibert-Roca, M.; Puig, T.; Obradors, X.; Campoy-Quiles, M.; et al. Band Gap Tuning of Solution-Processed Ferroelectric Perovskite BiFe1– xCoxO3 Thin Films. Chem. Mater. 2019, 31, 947–954. [Google Scholar] [CrossRef] [PubMed]
- Wang, Q.J.; Tan, Q.H.; Liu, Y.K. Enhanced Ferromagnetism in N-Doped BiFeO3: A First-Principles Prediction. J. Alloys Compd. 2015, 644, 30–39. [Google Scholar] [CrossRef]
- Gebhardt, J.; Rappe, A.M. Accuracy and Transferability of Ab. Initio Electronic Band Structure Calculations for Doped BiFeO3. J. Phys. Conf. Ser. 2017, 921, 012009. [Google Scholar] [CrossRef]
- Rodrigues Pela, R.; Vona, C.; Lubeck, S.; Alex, B.; Gonzalez Oliva, I.; Draxl, C. Critical Assessment of G0W0 Calculations for 2D Materials: The Example of Monolayer MoS2. NPJ Comput. Mater. 2024, 10, 77. [Google Scholar] [CrossRef]
- Umadevi, D.; Watson, G.W. Quasiparticle GW Calculations on Lead-Free Hybrid Germanium Iodide Perovskite CH3NH3GeI3 for Photovoltaic Applications. ACS Omega 2019, 4, 5661–5669. [Google Scholar] [CrossRef]
- Kim, S.-J.; Lebègue, S.; Ringe, S.; Kim, H. GW Quasiparticle Energies and Bandgaps of Two-Dimensional Materials Immersed in Water. J. Phys. Chem. Lett. 2022, 13, 7574–7582. [Google Scholar] [CrossRef]
- Biswas, T.; Jain, M. Quasiparticle Band Structure and Optical Properties of Hexagonal-YMnO3. J. Appl. Phys. 2016, 120, 155102. [Google Scholar] [CrossRef]
- Herring, C.J.; Montemore, M.M. Recent Advances in Real-Time Time-Dependent Density Functional Theory Simulations of Plasmonic Nanostructures and Plasmonic Photocatalysis. ACS Nanosci. Au 2023, 3, 269–279. [Google Scholar] [CrossRef]
- Lin, C.; Rohilla, J.; Kuo, H.; Chen, C.; Mark Chang, T.; Sone, M.; Ingole, P.P.; Lo, Y.; Hsu, Y. Density-Functional Theory Studies on Photocatalysis and Photoelectrocatalysis: Challenges and Opportunities. Solar RRL 2024, 8, 2300948. [Google Scholar] [CrossRef]
- Chen, W.; Wang, T.; Yu, C.-C.; Jing, Y.; Li, X.; Xiong, W. Small Polaron-Induced Ultrafast Ferroelectric Restoration in BiFeO3. Phys. Rev. X 2025, 15, 021046. [Google Scholar] [CrossRef]
- Hwang, J.; Feng, Z.; Charles, N.; Wang, X.R.; Lee, D.; Stoerzinger, K.A.; Muy, S.; Rao, R.R.; Lee, D.; Jacobs, R.; et al. Tuning Perovskite Oxides by Strain: Electronic Structure, Properties, and Functions in (Electro)Catalysis and Ferroelectricity. Mater. Today 2019, 31, 100–118. [Google Scholar] [CrossRef]
- Freeland, J.W.; Liu, J.; Kareev, M.; Gray, B.; Kim, J.W.; Ryan, P.; Pentcheva, R.; Chakhalian, J. Orbital Control in Strained Ultra-Thin LaNiO3 /LaAlO3 Superlattices. EPL (Europhys. Lett.) 2011, 96, 57004. [Google Scholar] [CrossRef]
- Akhade, S.A.; Kitchin, J.R. Effects of Strain, d-Band Filling, and Oxidation State on the Surface Electronic Structure and Reactivity of 3 d Perovskite Surfaces. J. Chem. Phys. 2012, 137, 084703. [Google Scholar] [CrossRef]
- Wong, F.J.; Baek, S.-H.; Chopdekar, R.V.; Mehta, V.V.; Jang, H.-W.; Eom, C.-B.; Suzuki, Y. Metallicity in LaTiO3 Thin Films Induced by Lattice Deformation. Phys. Rev. B 2010, 81, 161101. [Google Scholar] [CrossRef]
- Zhang, J.X.; He, Q.; Trassin, M.; Luo, W.; Yi, D.; Rossell, M.D.; Yu, P.; You, L.; Wang, C.H.; Kuo, C.Y.; et al. Microscopic Origin of the Giant Ferroelectric Polarization in Tetragonal-like BiFeO3. Phys. Rev. Lett. 2011, 107, 147602. [Google Scholar] [CrossRef]
- Dong, H.; Chen, C.; Wang, S.; Duan, W.; Li, J. Elastic Properties of Tetragonal BiFeO3 from First-Principles Calculations. Appl. Phys. Lett. 2013, 102, 182905. [Google Scholar] [CrossRef]
- Wei, L.; Ge, D.; Guan, L.; Guo, J.; Liu, B. Bandgap Tailoring of Tetragonal BiFeO3 with Different Magnetic Orders Calculated by First–Principles Calculations. Mater. Res. Express 2019, 6, 045907. [Google Scholar] [CrossRef]
- Fan, Z.; Wang, J.; Sullivan, M.B.; Huan, A.; Singh, D.J.; Ong, K.P. Structural Instability of Epitaxial (001) BiFeO3 Thin Films under Tensile Strain. Sci. Rep. 2014, 4, 4631. [Google Scholar] [CrossRef]
- Xia, L.; Tybell, T.; Selbach, S.M. Bi Vacancy Formation in BiFeO3 Epitaxial Thin Films under Compressive (001)-Strain from First Principles. J. Mater. Chem. C Mater. 2019, 7, 4870–4878. [Google Scholar] [CrossRef]
- Xia, L.; Tybell, T.; Selbach, S.M. First Principles Study of Bismuth Vacancy Formation in (111)-Strained BiFeO3. Materials 2024, 17, 5397. [Google Scholar] [CrossRef]
- Dupé, B.; Prosandeev, S.; Geneste, G.; Dkhil, B.; Bellaiche, L. BiFeO3 Films under Tensile Epitaxial Strain from First Principles. Phys. Rev. Lett. 2011, 106, 237601. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Dansou, C.; Paillard, C.; He, J.; Xiang, H.; Bellaiche, L. Large Photostriction near the Phase Boundary in BiFeO3 under Varying Epitaxial Strain. Phys. Rev. B 2024, 109, 184111. [Google Scholar] [CrossRef]
- Dixit, H.; Beekman, C.; Schlepütz, C.M.; Siemons, W.; Yang, Y.; Senabulya, N.; Clarke, R.; Chi, M.; Christen, H.M.; Cooper, V.R. Understanding Strain-Induced Phase Transformations in BiFeO3 Thin Films. Adv. Sci. 2015, 2, 1500041. [Google Scholar] [CrossRef]
- Ricinschi, D.; Okuyama, M. Ab Initio Study of the Giant Spontaneous Polarization of BiFeO3: Carrier Doping Effects. Ferroelectrics 2007, 355, 101–107. [Google Scholar] [CrossRef]
- Ricinschi, D.; Yun, K.-Y.; Okuyama, M. A Mechanism for the 150 ΜC Cm−2 Polarization of BiFeO3 Films Based on First-Principles Calculations and New Structural Data. J. Phys. Condens. Matter 2006, 18, L97–L105. [Google Scholar] [CrossRef] [PubMed]
- Ritz, E.T.; Benedek, N.A. Strain Game Revisited for Complex Oxide Thin Films: Substrate-Film Thermal Expansion Mismatch in PbTiO3. Phys. Rev. Mater. 2020, 4, 084410. [Google Scholar] [CrossRef]
- Jiang, Z.; Zhang, Z.; Paillard, C.; Xiang, H.; Bellaiche, L. Strain-Induced Bent Domains in Ferroelectric Nitrides. Phys. Rev. B 2024, 110, 054101. [Google Scholar] [CrossRef]
- Tu, J.; Fang, Y.-W.; Lu, Y.; Li, H.; Xi, G.; Ding, J.; Liu, X.; Liu, X.; Yang, Q.; Tian, J.; et al. Strain-Controlled Oxygen Vacancy for Robust Ferroelectric BiSmFe2O6−δ Double-Perovskite Epitaxial Thin Films. Appl. Phys. Rev. 2024, 11, 011413. [Google Scholar] [CrossRef]
- Watanabe, Y. DFT + U Accurate for Strain Effect and Overall Properties of Perovskite Oxide Ferroelectrics and Polaron. J. Appl. Phys. 2024, 135, 224103. [Google Scholar] [CrossRef]
- Waqdim, A.; Agouri, M.; Ouali, M.; Abbassi, A.; Taj, S.; Manaut, B.; El Idrissi, M. Ab Initio Insight into the Physical Properties of New Ferroelectric Perovskite Oxide Materials XGeO3 (X = Sr, Ca). Rev. Mex. De Física 2024, 70, 051603-1. [Google Scholar] [CrossRef]
- Marthinsen, A.; Grande, T.; Selbach, S.M. Microscopic Link between Electron Localization and Chemical Expansion in AMnO3 and ATiO3 Perovskites (A = Ca, Sr, Ba). J. Phys. Chem. C 2020, 124, 12922–12932. [Google Scholar] [CrossRef]
- Ricca, C.; Aschauer, U. Interplay between Polarization, Strain, and Defect Pairs in Fe-Doped SrMnO3−δ. Phys. Rev. Res. 2021, 3, 033237. [Google Scholar] [CrossRef]
- Brehm, J.A.; Neumayer, S.M.; Tao, L.; O’Hara, A.; Chyasnavichus, M.; Susner, M.A.; McGuire, M.A.; Kalinin, S.V.; Jesse, S.; Ganesh, P.; et al. Tunable Quadruple-Well Ferroelectric van Der Waals Crystals. Nat. Mater. 2020, 19, 43–48. [Google Scholar] [CrossRef]
- Ding, W.; Zhu, J.; Wang, Z.; Gao, Y.; Xiao, D.; Gu, Y.; Zhang, Z.; Zhu, W. Prediction of Intrinsic Two-Dimensional Ferroelectrics in In2Se3 and Other III2-VI3 van Der Waals Materials. Nat. Commun. 2017, 8, 14956. [Google Scholar] [CrossRef] [PubMed]
- Xue, F.; Hu, W.; Lee, K.; Lu, L.; Zhang, J.; Tang, H.; Han, A.; Hsu, W.; Tu, S.; Chang, W.; et al. Room-Temperature Ferroelectricity in Hexagonally Layered A-In2 Se3 Nanoflakes down to the Monolayer Limit. Adv. Funct. Mater. 2018, 28, 1803738. [Google Scholar] [CrossRef]
- Higashitarumizu, N.; Kawamoto, H.; Lee, C.-J.; Lin, B.-H.; Chu, F.-H.; Yonemori, I.; Nishimura, T.; Wakabayashi, K.; Chang, W.-H.; Nagashio, K. Purely In-Plane Ferroelectricity in Monolayer SnS at Room Temperature. Nat. Commun. 2020, 11, 2428. [Google Scholar] [CrossRef]
- Orlova, N.N.; Timonina, A.V.; Kolesnikov, N.N.; Deviatov, E.V. Switching Ferroelectricity in SnSe across Phase Transition. EPL (Europhys. Lett.) 2021, 135, 37002. [Google Scholar] [CrossRef]
- Chang, K.; Liu, J.; Lin, H.; Wang, N.; Zhao, K.; Zhang, A.; Jin, F.; Zhong, Y.; Hu, X.; Duan, W.; et al. Discovery of Robust In-Plane Ferroelectricity in Atomic-Thick SnTe. Science (1979) 2016, 353, 274–278. [Google Scholar] [CrossRef]
- Yuan, S.; Luo, X.; Chan, H.L.; Xiao, C.; Dai, Y.; Xie, M.; Hao, J. Room-Temperature Ferroelectricity in MoTe2 down to the Atomic Monolayer Limit. Nat. Commun. 2019, 10, 1775. [Google Scholar] [CrossRef]
- Song, Q.; Occhialini, C.A.; Ergeçen, E.; Ilyas, B.; Amoroso, D.; Barone, P.; Kapeghian, J.; Watanabe, K.; Taniguchi, T.; Botana, A.S.; et al. Evidence for a Single-Layer van Der Waals Multiferroic. Nature 2022, 602, 601–605. [Google Scholar] [CrossRef]
- Jariwala, D.; Marks, T.J.; Hersam, M.C. Mixed-Dimensional van Der Waals Heterostructures. Nat. Mater. 2017, 16, 170–181. [Google Scholar] [CrossRef] [PubMed]
- Kruse, M.; Petralanda, U.; Gjerding, M.N.; Jacobsen, K.W.; Thygesen, K.S.; Olsen, T. Two-Dimensional Ferroelectrics from High Throughput Computational Screening. NPJ Comput. Mater. 2023, 9, 45. [Google Scholar] [CrossRef]
- Li, H.F.; Zhang, G.H.; Zheng, Y.; Wang, B.; Chen, W.J. Ab Initio Study on Mechanical-Bending-Induced Ferroelectric Phase Transition in Ultrathin Perovskite Nanobelts. Acta Mater. 2014, 76, 472–481. [Google Scholar] [CrossRef]
- Sánchez-Santolino, G.; Rouco, V.; Puebla, S.; Aramberri, H.; Zamora, V.; Cabero, M.; Cuellar, F.A.; Munuera, C.; Mompean, F.; Garcia-Hernandez, M.; et al. A 2D Ferroelectric Vortex Pattern in Twisted BaTiO3 Freestanding Layers. Nature 2024, 626, 529–534. [Google Scholar] [CrossRef]
- Dong, G.; Li, S.; Yao, M.; Zhou, Z.; Zhang, Y.-Q.; Han, X.; Luo, Z.; Yao, J.; Peng, B.; Hu, Z.; et al. Super-Elastic Ferroelectric Single-Crystal Membrane with Continuous Electric Dipole Rotation. Science (1979) 2019, 366, 475–479. [Google Scholar] [CrossRef]
- Huang, J.-K.; Wan, Y.; Shi, J.; Zhang, J.; Wang, Z.; Wang, W.; Yang, N.; Liu, Y.; Lin, C.-H.; Guan, X.; et al. High-κ Perovskite Membranes as Insulators for Two-Dimensional Transistors. Nature 2022, 605, 262–267. [Google Scholar] [CrossRef] [PubMed]
- Yang, A.J.; Han, K.; Huang, K.; Ye, C.; Wen, W.; Zhu, R.; Zhu, R.; Xu, J.; Yu, T.; Gao, P.; et al. Van Der Waals Integration of High-κ Perovskite Oxides and Two-Dimensional Semiconductors. Nat. Electron. 2022, 5, 233–240. [Google Scholar] [CrossRef]
- Puebla, S.; Pucher, T.; Rouco, V.; Sanchez-Santolino, G.; Xie, Y.; Zamora, V.; Cuellar, F.A.; Mompean, F.J.; Leon, C.; Island, J.O.; et al. Combining Freestanding Ferroelectric Perovskite Oxides with Two-Dimensional Semiconductors for High Performance Transistors. Nano Lett. 2022, 22, 7457–7466. [Google Scholar] [CrossRef]
- Deeksha; Kour, P.; Ahmed, I.; Sunny; Sharma, S.K.; Yadav, K.; Mishra, Y.K. Transition Metal-based Perovskite Oxides: Emerging Electrocatalysts for Oxygen Evolution Reaction. ChemCatChem 2023, 15, e202300040. [Google Scholar] [CrossRef]
- Collins, J.B.; Levine, H. Diffuse Interface Model of Diffusion-Limited Crystal Growth. Phys. Rev. B 1985, 31, 6119–6122. [Google Scholar] [CrossRef]
- Chen, L.-Q.; Yang, W. Computer Simulation of the Domain Dynamics of a Quenched System with a Large Number of Nonconserved Order Parameters: The Grain-Growth Kinetics. Phys. Rev. B 1994, 50, 15752–15756. [Google Scholar] [CrossRef] [PubMed]
- Aranson, I.S.; Kalatsky, V.A.; Vinokur, V.M. Continuum Field Description of Crack Propagation. Phys. Rev. Lett. 2000, 85, 118–121. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.L.; Hu, S.Y.; Liu, Z.K.; Chen, L.Q. Phase-Field Model of Domain Structures in Ferroelectric Thin Films. Appl. Phys. Lett. 2001, 78, 3878–3880. [Google Scholar] [CrossRef]
- Peng, B.; Peng, R.-C.; Zhang, Y.-Q.; Dong, G.; Zhou, Z.; Zhou, Y.; Li, T.; Liu, Z.; Luo, Z.; Wang, S.; et al. Phase Transition Enhanced Superior Elasticity in Freestanding Single-Crystalline Multiferroic BiFeO3 Membranes. Sci. Adv. 2020, 6, eaba5847. [Google Scholar] [CrossRef]
- Han, L.; Fang, Y.; Zhao, Y.; Zang, Y.; Gu, Z.; Nie, Y.; Pan, X. Giant Uniaxial Strain Ferroelectric Domain Tuning in Freestanding PbTiO3 Films. Adv. Mater. Interfaces 2020, 7, 1901604. [Google Scholar] [CrossRef]
- Xu, R.; Huang, J.; Barnard, E.S.; Hong, S.S.; Singh, P.; Wong, E.K.; Jansen, T.; Harbola, V.; Xiao, J.; Wang, B.Y.; et al. Strain-Induced Room-Temperature Ferroelectricity in SrTiO3 Membranes. Nat. Commun. 2020, 11, 3141. [Google Scholar] [CrossRef]
- Dong, G.; Hu, Y.; Guo, C.; Wu, H.; Liu, H.; Peng, R.; Xian, D.; Mao, Q.; Dong, Y.; Zhao, Y.; et al. Self-Assembled Epitaxial Ferroelectric Oxide Nanospring with Super-Scalability. Adv. Mater. 2022, 34, 2108419. [Google Scholar] [CrossRef]
- Kang, K.T.; Corey, Z.J.; Hwang, J.; Sharma, Y.; Paudel, B.; Roy, P.; Collins, L.; Wang, X.; Lee, J.W.; Oh, Y.S.; et al. Heterogeneous Integration of Freestanding Bilayer Oxide Membrane for Multiferroicity. Adv. Sci. 2023, 10, 2207481. [Google Scholar] [CrossRef]
- Dong, G.; Li, S.; Li, T.; Wu, H.; Nan, T.; Wang, X.; Liu, H.; Cheng, Y.; Zhou, Y.; Qu, W.; et al. Periodic Wrinkle-Patterned Single-Crystalline Ferroelectric Oxide Membranes with Enhanced Piezoelectricity. Adv. Mater. 2020, 32, 2004477. [Google Scholar] [CrossRef]
- Chen, T.; Wang, B.; Zhu, Y.; Zhuang, S.; Chen, L.-Q.; Hu, J.-M. Analytical Model and Dynamical Phase-Field Simulation of Terahertz Transmission across Ferroelectrics. Phys. Rev. B 2024, 109, 094305. [Google Scholar] [CrossRef]
- Guo, C.; Yang, H.; Dong, S.; Tang, S.; Wang, J.; Wang, X.; Huang, H. Advancing Energy-Storage Performance in Freestanding Ferroelectric Thin Films: Insights from Phase-Field Simulations. Adv. Electron. Mater. 2024, 10, 2400001. [Google Scholar] [CrossRef]
- Nelson, C.T.; Winchester, B.; Zhang, Y.; Kim, S.-J.; Melville, A.; Adamo, C.; Folkman, C.M.; Baek, S.-H.; Eom, C.-B.; Schlom, D.G.; et al. Spontaneous Vortex Nanodomain Arrays at Ferroelectric Heterointerfaces. Nano Lett. 2011, 11, 828–834. [Google Scholar] [CrossRef] [PubMed]
- Jia, C.-L.; Urban, K.W.; Alexe, M.; Hesse, D.; Vrejoiu, I. Direct Observation of Continuous Electric Dipole Rotation in Flux-Closure Domains in Ferroelectric Pb(Zr, Ti)O3. Science (1979) 2011, 331, 1420–1423. [Google Scholar] [CrossRef]
- Tang, Y.L.; Zhu, Y.L.; Ma, X.L.; Borisevich, A.Y.; Morozovska, A.N.; Eliseev, E.A.; Wang, W.Y.; Wang, Y.J.; Xu, Y.B.; Zhang, Z.D.; et al. Observation of a periodic array of flux-closure quadrants in strained ferroelectric PbTiO3 films. Science 2015, 348, 547–551. [Google Scholar] [CrossRef]
- Yadav, A.K.; Nelson, C.T.; Hsu, S.L.; Hong, Z.; Clarkson, J.D.; Schlepütz, C.M.; Damodaran, A.R.; Shafer, P.; Arenholz, E.; Dedon, L.R.; et al. Observation of Polar Vortices in Oxide Superlattices. Nature 2016, 530, 198–201. [Google Scholar] [CrossRef]
- Das, S.; Tang, Y.L.; Hong, Z.; Gonçalves, M.A.P.; McCarter, M.R.; Klewe, C.; Nguyen, K.X.; Gómez-Ortiz, F.; Shafer, P.; Arenholz, E.; et al. Observation of Room-Temperature Polar Skyrmions. Nature 2019, 568, 368–372. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; Stoica, V.A.; Paściak, M.; Zhu, Y.; Yuan, Y.; Yang, T.; McCarter, M.R.; Das, S.; Yadav, A.K.; Park, S.; et al. Subterahertz Collective Dynamics of Polar Vortices. Nature 2021, 592, 376–380. [Google Scholar] [CrossRef]
- Wang, Y.J.; Feng, Y.P.; Zhu, Y.L.; Tang, Y.L.; Yang, L.X.; Zou, M.J.; Geng, W.R.; Han, M.J.; Guo, X.W.; Wu, B.; et al. Polar Meron Lattice in Strained Oxide Ferroelectrics. Nat. Mater. 2020, 19, 881–886. [Google Scholar] [CrossRef]
- Rusu, D.; Peters, J.J.P.; Hase, T.P.A.; Gott, J.A.; Nisbet, G.A.A.; Strempfer, J.; Haskel, D.; Seddon, S.D.; Beanland, R.; Sanchez, A.M.; et al. Ferroelectric Incommensurate Spin Crystals. Nature 2022, 602, 240–244. [Google Scholar] [CrossRef]
- Zhao, H.J.; Chen, P.; Prosandeev, S.; Artyukhin, S.; Bellaiche, L. Dzyaloshinskii–Moriya-like Interaction in Ferroelectrics and Antiferroelectrics. Nat. Mater. 2021, 20, 341–345. [Google Scholar] [CrossRef]
- Cao, C.; Lu, Z.; Wang, L.; Li, L.; Deng, D.; Liao, D.; Mo, C. Ce-Doped BiFeO3 as a Photocatalyst to Enhance Photo-Fenton Degradation of Tetracycline. J. Photochem. Photobiol. A Chem. 2024, 446, 115161. [Google Scholar] [CrossRef]
- Zhang, N.; Chen, D.; Niu, F.; Wang, S.; Qin, L.; Huang, Y. Enhanced Visible Light Photocatalytic Activity of Gd-Doped BiFeO3 Nanoparticles and Mechanism Insight. Sci. Rep. 2016, 6, 26467. [Google Scholar] [CrossRef] [PubMed]
- Huang, J.; Kang, Y.; Liu, J.; Yao, T.; Qiu, J.; Du, P.; Huang, B.; Hu, W.; Liang, Y.; Xie, T.; et al. Gradient Tungsten-Doped Bi3TiNbO9 Ferroelectric Photocatalysts with Additional Built-in Electric Field for Efficient Overall Water Splitting. Nat. Commun. 2023, 14, 7948. [Google Scholar] [CrossRef]
- Billah, A.; Anju, A.N.; Hirose, F.; Ahmmad, B. Visible-Light-Driven Oxygen Evolution by a BaTiO3 Based Ferroelectric Photocatalyst via Water Splitting. J. Mater. Chem. A Mater. 2024, 12, 15885–15892. [Google Scholar] [CrossRef]
- Zhang, Z.; Dai, Y.; Li, Z.; Lu, L.; Zhang, X.; Fu, K.; Xu, X.; Tian, W.; Jia, C.; Jiang, Y. Growth Modulation of Super-Tetragonal PbTiO3 Thin Films with Self-Assembled Nanocolumn Structures. Adv. Electron. Mater. 2021, 7, 2100547. [Google Scholar] [CrossRef]
- Enriquez, E.; Li, Q.; Bowlan, P.; Lu, P.; Zhang, B.; Li, L.; Wang, H.; Taylor, A.J.; Yarotski, D.; Prasankumar, R.P.; et al. Induced Ferroelectric Phases in SrTiO3 by a Nanocomposite Approach. Nanoscale 2020, 12, 18193–18199. [Google Scholar] [CrossRef] [PubMed]
- Harrington, S.A.; Zhai, J.; Denev, S.; Gopalan, V.; Wang, H.; Bi, Z.; Redfern, S.A.T.; Baek, S.-H.; Bark, C.W.; Eom, C.-B.; et al. Thick Lead-Free Ferroelectric Films with High Curie Temperatures through Nanocomposite-Induced Strain. Nat. Nanotechnol. 2011, 6, 491–495. [Google Scholar] [CrossRef]
- Li, W.; Zhang, W.; Wang, L.; Gu, J.; Chen, A.; Zhao, R.; Liang, Y.; Guo, H.; Tang, R.; Wang, C.; et al. Vertical Interface Induced Dielectric Relaxation in Nanocomposite (BaTiO3)1−x:(Sm2O3)x Thin Films. Sci. Rep. 2015, 5, 11335. [Google Scholar] [CrossRef]
- Goian, V.; Held, R.; Bousquet, E.; Yuan, Y.; Melville, A.; Zhou, H.; Gopalan, V.; Ghosez, P.; Spaldin, N.A.; Schlom, D.G.; et al. Making EuO Multiferroic by Epitaxial Strain Engineering. Commun. Mater. 2020, 1, 74. [Google Scholar] [CrossRef]
- Song, Y.; Xu, K.; Wang, J.; Huang, H. Thickness-Dependent Dielectric Properties in Doped Relaxor Films by the Phase-Field Model. ACS Appl. Electron. Mater. 2024, 6, 6477–6483. [Google Scholar] [CrossRef]
- Fedeli, P.; Kamlah, M.; Frangi, A. Phase-Field Modeling of Domain Evolution in Ferroelectric Materials in the Presence of Defects. Smart Mater. Struct. 2019, 28, 035021. [Google Scholar] [CrossRef]
- Wang, S.; Yi, M.; Xu, B.-X. A Phase-Field Model of Relaxor Ferroelectrics Based on Random Field Theory. Int. J. Solids Struct. 2016, 83, 142–153. [Google Scholar] [CrossRef]
- Wu, H.H.; Wang, J.; Cao, S.G.; Zhang, T.Y. Effect of Dislocation Walls on the Polarization Switching of a Ferroelectric Single Crystal. Appl. Phys. Lett. 2013, 102, 232904. [Google Scholar] [CrossRef]
- Li, Y.L.; Hu, S.Y.; Choudhury, S.; Baskes, M.I.; Saxena, A.; Lookman, T.; Jia, Q.X.; Schlom, D.G.; Chen, L.Q. Influence of Interfacial Dislocations on Hysteresis Loops of Ferroelectric Films. J. Appl. Phys. 2008, 104, 104110. [Google Scholar] [CrossRef]
- Wu, H.H.; Wang, J.; Cao, S.G.; Chen, L.Q.; Zhang, T.Y. Micro-/Macro-Responses of a Ferroelectric Single Crystal with Domain Pinning and Depinning by Dislocations. J. Appl. Phys. 2013, 114, 164108. [Google Scholar] [CrossRef]
- Wang, J.; Shu, W.; Fang, H.; Kamlah, M. Phase Field Simulations of the Poling Process and Nonlinear Behavior of Ferroelectric Polycrystals with Semiconducting Grain Boundaries. Smart Mater. Struct. 2014, 23, 095016. [Google Scholar] [CrossRef]
- Vidyasagar, A.; Tan, W.L.; Kochmann, D.M. Predicting the Effective Response of Bulk Polycrystalline Ferroelectric Ceramics via Improved Spectral Phase Field Methods. J. Mech. Phys. Solids 2017, 106, 133–151. [Google Scholar] [CrossRef]
- Li, L.; Cheng, X.; Blum, T.; Huyan, H.; Zhang, Y.; Heikes, C.; Yan, X.; Gadre, C.; Aoki, T.; Xu, M.; et al. Observation of Strong Polarization Enhancement in Ferroelectric Tunnel Junctions. Nano Lett. 2019, 19, 6812–6818. [Google Scholar] [CrossRef]
- Li, Y.L.; Hu, S.Y.; Liu, Z.K.; Chen, L.Q. Effect of Substrate Constraint on the Stability and Evolution of Ferroelectric Domain Structures in Thin Films. Acta Mater. 2002, 50, 395–411. [Google Scholar] [CrossRef]
- Li, Y.L.; Chen, L.Q. Temperature-Strain Phase Diagram for BaTiO3 Thin Films. Appl. Phys. Lett. 2006, 88, 072905. [Google Scholar] [CrossRef]
- Li, Y.L.; Cross, L.E.; Chen, L.Q. A Phenomenological Thermodynamic Potential for BaTiO3 Single Crystals. J. Appl. Phys. 2005, 98, 064101. [Google Scholar] [CrossRef]
- Li, Y.L.; Choudhury, S.; Liu, Z.K.; Chen, L.Q. Effect of External Mechanical Constraints on the Phase Diagram of Epitaxial PbZr1−xTixO3 Thin Films—Thermodynamic Calculations and Phase-Field Simulations. Appl. Phys. Lett. 2003, 83, 1608–1610. [Google Scholar] [CrossRef]
- Li, Y.L.; Hu, S.Y.; Chen, L.Q. Ferroelectric Domain Morphologies of (001) PbZr1−xTixO3 Epitaxial Thin Films. J. Appl. Phys. 2005, 97, 034112. [Google Scholar] [CrossRef]
- Karthik, J.; Martin, L.W. Pyroelectric Properties of Polydomain Epitaxial Pb(Zr1−x,Tix)O3 Thin Films. Phys. Rev. B 2011, 84, 024102. [Google Scholar] [CrossRef]
- Zhang, J.X.; Li, Y.L.; Wang, Y.; Liu, Z.K.; Chen, L.Q.; Chu, Y.H.; Zavaliche, F.; Ramesh, R. Effect of Substrate-Induced Strains on the Spontaneous Polarization of Epitaxial BiFeO3 Thin Films. J. Appl. Phys. 2007, 101, 114105. [Google Scholar] [CrossRef]
- Zhang, Y.; Xue, F.; Chen, Z.; Liu, J.-M.; Chen, L.-Q. Presence of a Purely Tetragonal Phase in Ultrathin BiFeO3 Films: Thermodynamics and Phase-Field Simulations. Acta Mater. 2020, 183, 110–117. [Google Scholar] [CrossRef]
- Vasudevarao, A.; Kumar, A.; Tian, L.; Haeni, J.H.; Li, Y.L.; Eklund, C.-J.; Jia, Q.X.; Uecker, R.; Reiche, P.; Rabe, K.M.; et al. Multiferroic Domain Dynamics in Strained Strontium Titanate. Phys. Rev. Lett. 2006, 97, 257602. [Google Scholar] [CrossRef]
- Li, Y.L.; Choudhury, S.; Haeni, J.H.; Biegalski, M.D.; Vasudevarao, A.; Sharan, A.; Ma, H.Z.; Levy, J.; Gopalan, V.; Trolier-McKinstry, S.; et al. Phase Transitions and Domain Structures in Strained Pseudocubic (100) SrTiO3 Thin Films. Phys. Rev. B 2006, 73, 184112. [Google Scholar] [CrossRef]
- Bousquet, E.; Spaldin, N.A.; Ghosez, P. Strain-Induced Ferroelectricity in Simple Rocksalt Binary Oxides. Phys. Rev. Lett. 2010, 104, 037601. [Google Scholar] [CrossRef]
- Kumar, P.; Hoffmann, M.; Nonaka, A.; Salahuddin, S.; Yao, Z. 3D Ferroelectric Phase Field Simulations of Polycrystalline Multi-Phase Hafnia and Zirconia Based Ultra-Thin Films. Adv. Electron. Mater. 2024, 10, 2400085. [Google Scholar] [CrossRef]
- Kim, T.; Kim, G.; Lee, Y.K.; Ko, D.H.; Hwang, J.; Lee, S.; Shin, H.; Jeong, Y.; Jung, S.; Jeon, S. The Opportunity of Negative Capacitance Behavior in Flash Memory for High-Density and Energy-Efficient In-Memory Computing Applications. Adv. Funct. Mater. 2023, 33, 2208525. [Google Scholar] [CrossRef]
- Liu, Z.; Shi, X.; Wang, J.; Huang, H. Electric-Field-Induced Crystallization of Hf0.5Zr0.5O2 Thin Film Based on Phase-Field Modeling. NPJ Quantum Mater. 2024, 9, 44. [Google Scholar] [CrossRef]
- Wang, J.-J.; Su, Y.-J.; Wang, B.; Ouyang, J.; Ren, Y.-H.; Chen, L.-Q. Strain Engineering of Dischargeable Energy Density of Ferroelectric Thin-Film Capacitors. Nano Energy 2020, 72, 104665. [Google Scholar] [CrossRef]
- Hao, L.; Yang, Y.; Huan, Y.; Cheng, H.; Zhao, Y.-Y.; Wang, Y.; Yan, J.; Ren, W.; Ouyang, J. Achieving a High Dielectric Tunability in Strain-Engineered Tetragonal K0.5Na0.5NbO3 Films. NPJ Comput. Mater. 2021, 7, 62. [Google Scholar] [CrossRef]
- Chu, P.; Chen, D.P.; Wang, Y.L.; Xie, Y.L.; Yan, Z.B.; Wan, J.G.; Liu, J.-M.; Li, J.Y. Kinetics of 90° Domain Wall Motions and High Frequency Mesoscopic Dielectric Response in Strained Ferroelectrics: A Phase-Field Simulation. Sci. Rep. 2014, 4, 5007. [Google Scholar] [CrossRef]
- Sheng, G.; Zhang, J.X.; Li, Y.L.; Choudhury, S.; Jia, Q.X.; Liu, Z.K.; Chen, L.Q. Domain Stability of PbTiO3 Thin Films under Anisotropic Misfit Strains: Phase-Field Simulations. J. Appl. Phys. 2008, 104, 054105. [Google Scholar] [CrossRef]
- Sheng, G.; Zhang, J.X.; Li, Y.L.; Choudhury, S.; Jia, Q.X.; Liu, Z.K.; Chen, L.Q. Misfit Strain–Misfit Strain Diagram of Epitaxial BaTiO3 Thin Films: Thermodynamic Calculations and Phase-Field Simulations. Appl. Phys. Lett. 2008, 93, 232904. [Google Scholar] [CrossRef]
- Winchester, B.; Wu, P.; Chen, L.Q. Phase-Field Simulation of Domain Structures in Epitaxial BiFeO3 Films on Vicinal Substrates. Appl. Phys. Lett. 2011, 99, 052903. [Google Scholar] [CrossRef]
- Lu, D.; Hikita, Y.; Baek, D.J.; Merz, T.A.; Sato, H.; Kim, B.; Yajima, T.; Bell, C.; Vailionis, A.; Kourkoutis, L.F.; et al. Strain Tuning in Complex Oxide Epitaxial Films Using an Ultrathin Strontium Aluminate Buffer Layer. Phys. Status Solidi (RRL)–Rapid Res. Lett. 2018, 12, 1700339. [Google Scholar] [CrossRef]
- Deng, X.; Chen, C.; Chen, D.; Cai, X.; Yin, X.; Xu, C.; Sun, F.; Li, C.; Li, Y.; Xu, H.; et al. Strain Engineering of Epitaxial Oxide Heterostructures beyond Substrate Limitations. Matter 2021, 4, 1323–1334. [Google Scholar] [CrossRef]
- People, R.; Bean, J.C. Calculation of Critical Layer Thickness versus Lattice Mismatch for GexSi1−x/Si Strained-Layer Heterostructures. Appl. Phys. Lett. 1985, 47, 322–324. [Google Scholar] [CrossRef]
- Wang, J.-J.; Wang, Y.; Ihlefeld, J.F.; Hopkins, P.E.; Chen, L.-Q. Tunable Thermal Conductivity via Domain Structure Engineering in Ferroelectric Thin Films: A Phase-Field Simulation. Acta Mater. 2016, 111, 220–231. [Google Scholar] [CrossRef]
- Damodaran, A.R.; Agar, J.C.; Pandya, S.; Chen, Z.; Dedon, L.; Xu, R.; Apgar, B.; Saremi, S.; Martin, L.W. New Modalities of Strain-Control of Ferroelectric Thin Films. J. Phys. Condens. Matter 2016, 28, 263001. [Google Scholar] [CrossRef]
- Lu, H.; Tan, Y.; Richarz, L.; He, J.; Wang, B.; Meier, D.; Chen, L.; Gruverman, A. Electromechanics of Domain Walls in Uniaxial Ferroelectrics. Adv. Funct. Mater. 2023, 33, 2213684. [Google Scholar] [CrossRef]
- Agar, J.C.; Damodaran, A.R.; Okatan, M.B.; Kacher, J.; Gammer, C.; Vasudevan, R.K.; Pandya, S.; Dedon, L.R.; Mangalam, R.V.K.; Velarde, G.A.; et al. Highly Mobile Ferroelastic Domain Walls in Compositionally Graded Ferroelectric Thin Films. Nat. Mater. 2016, 15, 549–556. [Google Scholar] [CrossRef] [PubMed]
- Shi, Q.; Parsonnet, E.; Cheng, X.; Fedorova, N.; Peng, R.-C.; Fernandez, A.; Qualls, A.; Huang, X.; Chang, X.; Zhang, H.; et al. The Role of Lattice Dynamics in Ferroelectric Switching. Nat. Commun. 2022, 13, 1110. [Google Scholar] [CrossRef]
- Gurung, A.; Ishtiyaq, M.F.; Alpay, S.P.; Mangeri, J.; Nakhmanson, S. Extrinsic Dielectric Response Due to Domain Wall Motion in Ferroelectric BaTiO3. Comput. Mater. Today 2025, 5, 100016. [Google Scholar] [CrossRef]
- Zhang, M.; Chen, Z.; Yue, Y.; Chen, T.; Yan, Z.; Jiang, Q.; Yang, B.; Eriksson, M.; Tang, J.; Zhang, D.; et al. Terahertz Reading of Ferroelectric Domain Wall Dielectric Switching. ACS Appl. Mater. Interfaces 2021, 13, 12622–12628. [Google Scholar] [CrossRef]
- Sluka, T.; Tagantsev, A.K.; Damjanovic, D.; Gureev, M.; Setter, N. Enhanced Electromechanical Response of Ferroelectrics Due to Charged Domain Walls. Nat. Commun. 2012, 3, 748. [Google Scholar] [CrossRef]
- Zhou, L.; Puntigam, L.; Lunkenheimer, P.; Bourret, E.; Yan, Z.; Kézsmárki, I.; Meier, D.; Krohns, S.; Schultheiß, J.; Evans, D.M. Post-Synthesis Tuning of Dielectric Constant via Ferroelectric Domain Wall Engineering. Matter 2024, 7, 2996–3006. [Google Scholar] [CrossRef]
- Hadjimichael, M.; Li, Y.; Yedra, L.; Dkhil, B.; Zubko, P. Domain Structure and Dielectric Properties of Metal-Ferroelectric Superlattices with Asymmetric Interfaces. Phys. Rev. Mater. 2020, 4, 094415. [Google Scholar] [CrossRef]
- Ion, V.; Craciun, F.; Scarisoreanu, N.D.; Moldovan, A.; Andrei, A.; Birjega, R.; Ghica, C.; Di Pietrantonio, F.; Cannata, D.; Benetti, M.; et al. Impact of Thickness Variation on Structural, Dielectric and Piezoelectric Properties of (Ba, Ca)(Ti, Zr)O3 Epitaxial Thin Films. Sci. Rep. 2018, 8, 2056. [Google Scholar] [CrossRef] [PubMed]
- Guennou, M.; Bouvier, P.; Chen, G.S.; Dkhil, B.; Haumont, R.; Garbarino, G.; Kreisel, J. Multiple High-Pressure Phase Transitions in BiFeO3. Phys. Rev. B 2011, 84, 174107. [Google Scholar] [CrossRef]
- Yang, Y.; Infante, I.C.; Dkhil, B.; Bellaiche, L. Strain Effects on Multiferroic BiFeO3 Films. Comptes Rendus Phys. 2015, 16, 193–203. [Google Scholar] [CrossRef]
- Saito, K.; Ulyanenkov, A.; Grossmann, V.; Ress, H.; Bruegemann, L.; Ohta, H.; Kurosawa, T.; Ueki, S.; Funakubo, H. Structural Characterization of BiFeO3 Thin Films by Reciprocal Space Mapping. Jpn. J. Appl. Phys. 2006, 45, 7311. [Google Scholar] [CrossRef]
- Yao, J.; Yang, Y.; Ge, W.; Li, J.; Viehland, D. Domain Evolution in PbMg1/3Nb2/3O3-60at%PbTiO3 with Temperature and Electric Field. J. Am. Ceram. Soc. 2011, 94, 2479–2482. [Google Scholar] [CrossRef]
- Luo, J.; Maggard, P.A. Hydrothermal Synthesis and Photocatalytic Activities of SrTiO3-Coated Fe2O3 and BiFeO3. Adv. Mater. 2006, 18, 514–517. [Google Scholar] [CrossRef]
- Ederer, C.; Spaldin, N.A. Influence of Strain and Oxygen Vacancies on the Magnetoelectric Properties of Multiferroic Bismuth Ferrite. Phys. Rev. B 2005, 71, 224103. [Google Scholar] [CrossRef]
- Song, J.; Kim, T.L.; Lee, J.; Cho, S.Y.; Cha, J.; Jeong, S.Y.; An, H.; Kim, W.S.; Jung, Y.-S.; Park, J.; et al. Domain-Engineered BiFeO3 Thin-Film Photoanodes for Highly Enhanced Ferroelectric Solar Water Splitting. Nano Res. 2018, 11, 642–655. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghitiu, I.-M.; Nemnes, G.A.; Scarisoreanu, N.D. Tailoring the Functional Properties of Ferroelectric Perovskite Thin Films: Mechanisms of Dielectric and Photoelectrochemical Enhancement. Crystals 2025, 15, 496. https://doi.org/10.3390/cryst15060496
Ghitiu I-M, Nemnes GA, Scarisoreanu ND. Tailoring the Functional Properties of Ferroelectric Perovskite Thin Films: Mechanisms of Dielectric and Photoelectrochemical Enhancement. Crystals. 2025; 15(6):496. https://doi.org/10.3390/cryst15060496
Chicago/Turabian StyleGhitiu, Ioan-Mihail, George Alexandru Nemnes, and Nicu Doinel Scarisoreanu. 2025. "Tailoring the Functional Properties of Ferroelectric Perovskite Thin Films: Mechanisms of Dielectric and Photoelectrochemical Enhancement" Crystals 15, no. 6: 496. https://doi.org/10.3390/cryst15060496
APA StyleGhitiu, I.-M., Nemnes, G. A., & Scarisoreanu, N. D. (2025). Tailoring the Functional Properties of Ferroelectric Perovskite Thin Films: Mechanisms of Dielectric and Photoelectrochemical Enhancement. Crystals, 15(6), 496. https://doi.org/10.3390/cryst15060496






