Temperature-Dependent Spectroscopic Ellipsometry and Modeling of the Optical Properties of Vanadium Dioxide Thin Films
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Surface Morphology
3.2. Temperature-Dependent Spectroscopic Ellipsometry
3.3. Optical Properties of Vanadium Dioxide
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| PCM | Phase change material |
| MIT | Metal–insulator transition |
| PCT | Phase change temperature |
| SEM | Scanning electron microscopy |
| AFM | Atomic force microscopy |
| FWHM | Full width of half maxima |
| RMSE | Root mean square error |
References
- Morin, F.J. Oxides Which Show a Metal-to-Insulator Transition at the Neel Temperature. Phys. Rev. Lett. 1959, 3, 34–36. [Google Scholar] [CrossRef]
- Gong, Z.; Yang, F.; Wang, L.; Chen, R.; Wu, J.; Grigoropoulos, C.P.; Yao, J. Phase change materials in photonic devices. J. Appl. Phys. 2021, 129, 030902. [Google Scholar] [CrossRef]
- Ke, Y.; Wang, S.; Liu, G.; Li, M.; White, T.J.; Long, Y. Vanadium Dioxide: The Multistimuli Responsive Material and Its Applications. Small 2018, 14, 1802025. [Google Scholar] [CrossRef]
- Liu, K.; Lee, S.; Yang, S.; Delaire, O.; Wu, J. Recent progresses on physics and applications of vanadium dioxide. Mater. Today 2018, 21, 875–896. [Google Scholar] [CrossRef]
- Bhupathi, S.; Wang, S.; Ke, Y.; Long, Y. Recent progress in vanadium dioxide: The multi-stimuli responsive material and its applications. Mater. Sci. Eng. R Rep. 2023, 155, 100747. [Google Scholar] [CrossRef]
- Weber, C.; O’Regan, D.D.; Hine, N.D.M.; Payne, M.C.; Kotliar, G.; Littlewood, P.B. Vanadium Dioxide: A Peierls-Mott Insulator Stable against Disorder. Phys. Rev. Lett. 2012, 108, 256402. [Google Scholar] [CrossRef] [PubMed]
- Barker, A.S.; Verleur, H.W.; Guggenheim, H.J. Infrared Optical Properties of Vanadium Dioxide Above and Below the Transition Temperature. Phys. Rev. Lett. 1966, 17, 1286–1289. [Google Scholar] [CrossRef]
- Whittaker, L.; Patridge, C.J.; Banerjee, S. Microscopic and Nanoscale Perspective of the Metal−Insulator Phase Transitions of VO2: Some New Twists to an Old Tale. J. Phys. Chem. Lett. 2011, 2, 745–758. [Google Scholar] [CrossRef]
- Kats, M.A.; Blanchard, R.; Zhang, S.; Genevet, P.; Ko, C.; Ramanathan, S.; Capasso, F. Vanadium Dioxide as a Natural Disordered Metamaterial: Perfect Thermal Emission and Large Broadband Negative Differential Thermal Emittance. Phys. Rev. X 2013, 3, 041004. [Google Scholar] [CrossRef]
- Kana Kana, J.B.; Vignaud, G.; Gibaud, A.; Maaza, M. Thermally driven sign switch of static dielectric constant of VO2 thin film. Opt. Mater. 2016, 54, 165–169. [Google Scholar] [CrossRef]
- Shao, Z.; Cao, X.; Luo, H.; Jin, P. Recent progress in the phase-transition mechanism and modulation of vanadium dioxide materials. NPG Asia Mater. 2018, 10, 581–605. [Google Scholar] [CrossRef]
- Wang, P.-F.; Hu, Q.; Zheng, T.; Liu, Y.; Xu, X.; Sun, J.-L. Optically Monitored Electric-Field-Induced Phase Transition in Vanadium Dioxide Crystal Film. Crystals 2020, 10, 764. [Google Scholar] [CrossRef]
- Polak, P.; Jamroz, J.; Pietrzak, T.K. Observation of Metal–Insulator Transition (MIT) in Vanadium Oxides V2O3 and VO2 in XRD, DSC and DC Experiments. Crystals 2023, 13, 1299. [Google Scholar] [CrossRef]
- Haddad, E.; Kruzelecky, R.V.; Murzionak, P.; Jamroz, W.; Tagziria, K.; Chaker, M.; Ledrogoff, B. Review of the VO2 smart material applications with emphasis on its use for spacecraft thermal control. Front. Mater. 2022, 9, 1013848. [Google Scholar] [CrossRef]
- Jiang, W.; Zheng, T.; Wu, B.; Jiao, H.; Wang, X.; Chen, Y.; Zhang, X.; Peng, M.; Wang, H.; Lin, T.; et al. A versatile photodetector assisted by photovoltaic and bolometric effects. Light Sci. Appl. 2020, 9, 160. [Google Scholar] [CrossRef]
- Zhou, Y.; Chen, X.; Ko, C.; Yang, Z.; Mouli, C.; Ramanathan, S. Voltage-Triggered Ultrafast Phase Transition in Vanadium Dioxide Switches. IEEE Electron Device Lett. 2013, 34, 220–222. [Google Scholar] [CrossRef]
- Wang, H.; Yi, X.; Li, Y. Fabrication of VO2 films with low transition temperature for optical switching applications. Opt. Commun. 2005, 256, 305–309. [Google Scholar] [CrossRef]
- Lee, M.-J.; Park, Y.; Suh, D.-S.; Lee, E.-H.; Seo, S.; Kim, D.-C.; Jung, R.; Kang, B.-S.; Ahn, S.-E.; Lee, C.B.; et al. Two Series Oxide Resistors Applicable to High Speed and High Density Nonvolatile Memory. Adv. Mater. 2007, 19, 3919–3923. [Google Scholar] [CrossRef]
- Pellegrino, L.; Manca, N.; Kanki, T.; Tanaka, H.; Biasotti, M.; Bellingeri, E.; Siri, A.S.; Marré, D. Multistate Memory Devices Based on Free-standing VO2/TiO2 Microstructures Driven by Joule Self-Heating. Adv. Mater. 2012, 24, 2929–2934. [Google Scholar] [CrossRef]
- Shukla, N.; Thathachary, A.V.; Agrawal, A.; Paik, H.; Aziz, A.; Schlom, D.G.; Gupta, S.K.; Engel-Herbert, R.; Datta, S. A steep-slope transistor based on abrupt electronic phase transition. Nat. Commun. 2015, 6, 7812. [Google Scholar] [CrossRef]
- Yi, W.; Tsang, K.K.; Lam, S.K.; Bai, X.; Crowell, J.A.; Flores, E.A. Biological plausibility and stochasticity in scalable VO2 active memristor neurons. Nat. Commun. 2018, 9, 4661. [Google Scholar] [CrossRef]
- Andrews, J.L.; Santos, D.A.; Meyyappan, M.; Williams, R.S.; Banerjee, S. Building Brain-Inspired Logic Circuits from Dynamically Switchable Transition-Metal Oxides. Trends Chem. 2019, 1, 711–726. [Google Scholar] [CrossRef]
- Sangwan, V.K.; Hersam, M.C. Neuromorphic nanoelectronic materials. Nat. Nanotechnol. 2020, 15, 517–528. [Google Scholar] [CrossRef]
- Kumar, S.; Wang, X.; Strachan, J.P.; Yang, Y.; Lu, W.D. Dynamical memristors for higher-complexity neuromorphic computing. Nat. Rev. Mater. 2022, 7, 575–591. [Google Scholar] [CrossRef]
- Cui, Y.; Ke, Y.; Liu, C.; Chen, Z.; Wang, N.; Zhang, L.; Zhou, Y.; Wang, S.; Gao, Y.; Long, Y. Thermochromic VO2 for Energy-Efficient Smart Windows. Joule 2018, 2, 1707–1746. [Google Scholar] [CrossRef]
- Xu, F.; Cao, X.; Luo, H.; Jin, P. Recent advances in VO2-based thermochromic composites for smart windows. J. Mater. Chem. C 2018, 6, 1903–1919. [Google Scholar] [CrossRef]
- Kamalisarvestani, M.; Saidur, R.; Mekhilef, S.; Javadi, F.S. Performance, materials and coating technologies of thermochromic thin films on smart windows. Renew. Sustain. Energy Rev. 2013, 26, 353–364. [Google Scholar] [CrossRef]
- An, Y.; Fu, Y.; Dai, J.-G.; Yin, X.; Lei, D. Switchable radiative cooling technologies for smart thermal management. Cell Rep. Phys. Sci. 2022, 3, 101098. [Google Scholar] [CrossRef]
- Hilfiker, J.; Tompkins, H. Spectroscopic Ellipsometry: Practical Application to Thin Film Characterization; Momentum Press: New York, NY, USA, 2016. [Google Scholar]
- Orji, N.G.; Badaroglu, M.; Barnes, B.M.; Beitia, C.; Bunday, B.D.; Celano, U.; Kline, R.J.; Neisser, M.; Obeng, Y.; Vladar, A.E. Metrology for the next generation of semiconductor devices. Nat. Electron. 2018, 1, 532–547. [Google Scholar] [CrossRef]
- Chen, X.; Liu, S. Optical Scatterometry for Nanostructure Metrology. In Metrology; Gao, W., Ed.; Springer: Singapore, 2019; pp. 1–37. [Google Scholar]
- Swann, J.T.; De Smet, D.J. Ellipsometric investigation of vanadium dioxide films. J. Appl. Phys. 1985, 58, 1335–1338. [Google Scholar] [CrossRef]
- Aspnes, D.E.; Kinsbron, E.; Bacon, D.D. Optical properties of Au: Sample effects. Phys. Rev. B 1980, 21, 3290–3299. [Google Scholar] [CrossRef]
- Wan, C.; Zhang, Z.; Woolf, D.; Hessel, C.M.; Rensberg, J.; Hensley, J.M.; Xiao, Y.; Shahsafi, A.; Salman, J.; Richter, S.; et al. On the Optical Properties of Thin-Film Vanadium Dioxide from the Visible to the Far Infrared. Ann. Der Phys. 2019, 531, 1900188. [Google Scholar] [CrossRef]
- Huang, Y.; Zhang, D.; Liu, Y.; Jin, J.; Yang, Y.; Chen, T.; Guan, H.; Fan, P.; Lv, W. Phase transition analysis of thermochromic VO2 thin films by temperature-dependent Raman scattering and ellipsometry. Appl. Surf. Sci. 2018, 456, 545–551. [Google Scholar] [CrossRef]
- Houska, J.; Kolenaty, D.; Rezek, J.; Vlcek, J. Characterization of thermochromic VO2 (prepared at 250 °C) in a wide temperature range by spectroscopic ellipsometry. Appl. Surf. Sci. 2017, 421, 529–534. [Google Scholar] [CrossRef]
- Kakiuchida, H.; Jin, P.; Nakao, S.; Tazawa, M. Optical Properties of Vanadium Dioxide Film during Semiconductive–Metallic Phase Transition. Jpn. J. Appl. Phys. 2007, 46, L113. [Google Scholar] [CrossRef]
- Petit, C.; Frigerio, J. Optical properties of VO2 thin films in their dielectric and metallic states. In Proceedings of the Optical Systems Design and Production, Berlin, Germany, 26–29 May 1999; Volume 3738. [Google Scholar]
- Floro, J.A.; Hearne, S.J.; Hunter, J.A.; Kotula, P.; Chason, E.; Seel, S.C.; Thompson, C.V. The dynamic competition between stress generation and relaxation mechanisms during coalescence of Volmer–Weber thin films. J. Appl. Phys. 2001, 89, 4886–4897. [Google Scholar] [CrossRef]
- Holzwarth, U.; Gibson, N. The Scherrer equation versus the ‘Debye-Scherrer equation’. Nat. Nanotechnol. 2011, 6, 534. [Google Scholar] [CrossRef]
- Schilbe, P. Raman scattering in VO2. Phys. B Condens. Matter 2002, 316–317, 600–602. [Google Scholar] [CrossRef]
- Huang, H.-T.; Kong, W.; Terry, F.L., Jr. Normal-incidence spectroscopic ellipsometry for critical dimension monitoring. Appl. Phys. Lett. 2001, 78, 3983–3985. [Google Scholar] [CrossRef]
- Vedam, K. Spectroscopic ellipsometry: A historical overview. Thin Solid Films 1998, 313–314, 1–9. [Google Scholar] [CrossRef]
- Nag, J.; Haglund, R.F. Synthesis of vanadium dioxide thin films and nanoparticles. J. Phys. Condens. Matter 2008, 20, 264016. [Google Scholar] [CrossRef]
- Valdes, L.B. Resistivity Measurements on Germanium for Transistors. Proc. IRE 1954, 42, 420–427. [Google Scholar] [CrossRef]
- Chelly, A.; Glass, S.; Belhassen, J.; Karsenty, A. Broad review of four-point probe correction factors: Enhanced analytical model using advanced numerical and experimental cross-examination. Results Phys. 2023, 48, 106445. [Google Scholar] [CrossRef]
- Tauc, J.; Grigorovici, R.; Vancu, A. Optical Properties and Electronic Structure of Amorphous Germanium. Phys. Status Solidi (B) 1966, 15, 627–637. [Google Scholar] [CrossRef]
- Likhachev, D.V.; Malkova, N.; Poslavsky, L. Modified Tauc–Lorentz dispersion model leading to a more accurate representation of absorption features below the bandgap. Thin Solid Films 2015, 589, 844–851. [Google Scholar] [CrossRef]
- Fujiwara, H. Appendix 2: Definitions of Optical Constants. In Spectroscopic Ellipsometry; John Wiley & Sons: Hoboken, NJ, USA, 2007; pp. 347–348. [Google Scholar]
- Collins, R.W.; Ferlauto, A.S. 2—Optical Physics of Materials. In Handbook of Ellipsometry; Tompkins, H.G., Irene, E.A., Eds.; William Andrew Publishing: Norwich, NY, USA, 2005; pp. 93–235. [Google Scholar]
- Abdelkadir, A.A.; Victor, J.-L.; Vignaud, G.; Marcel, C.; Sahal, M.; Maaza, M.; Chaker, M.; Gibaud, A. Analysis of the temperature dependent optical properties of V1−xWxO2 thin films. Thin Solid Films 2023, 772, 139805. [Google Scholar] [CrossRef]
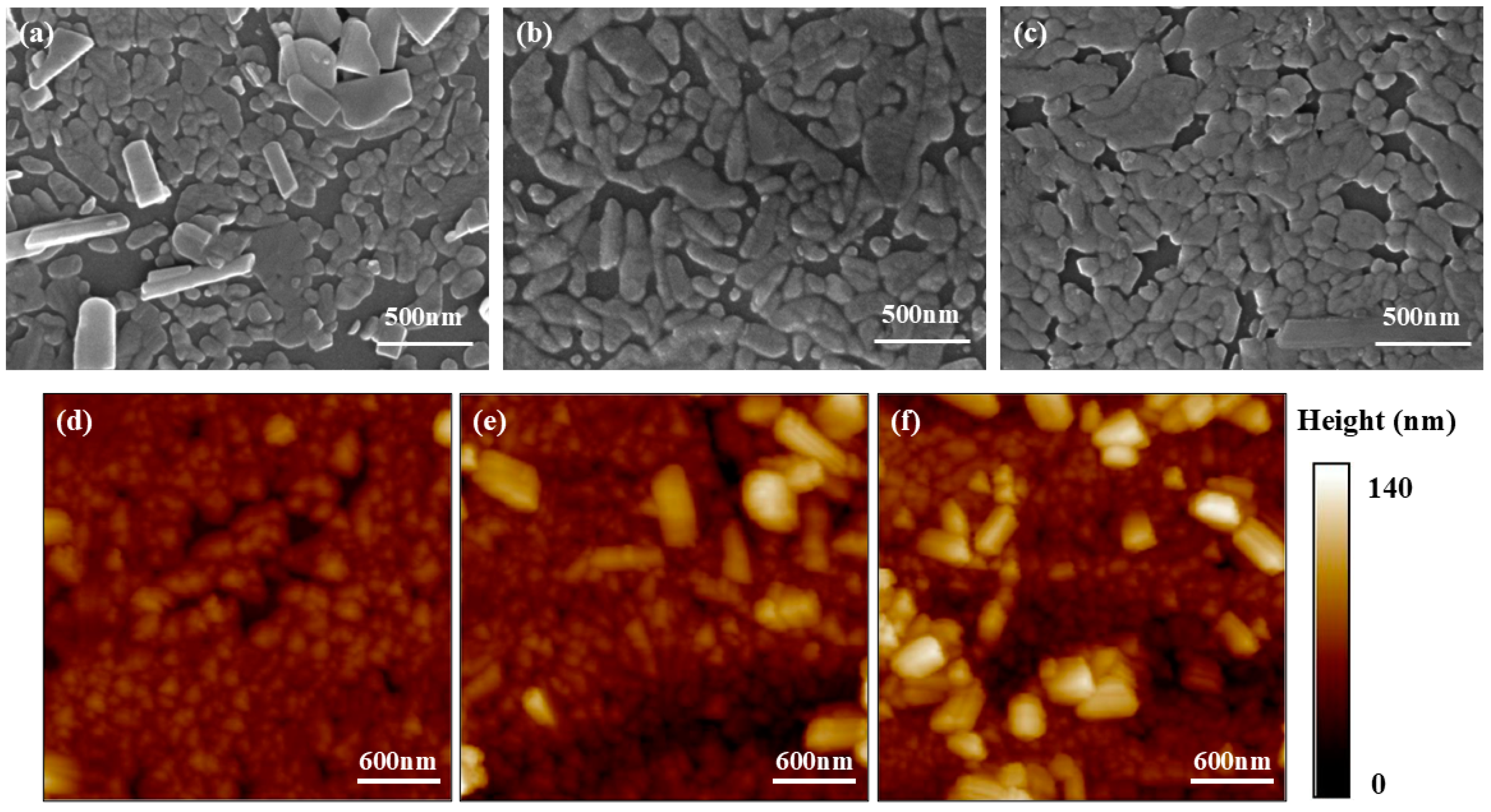
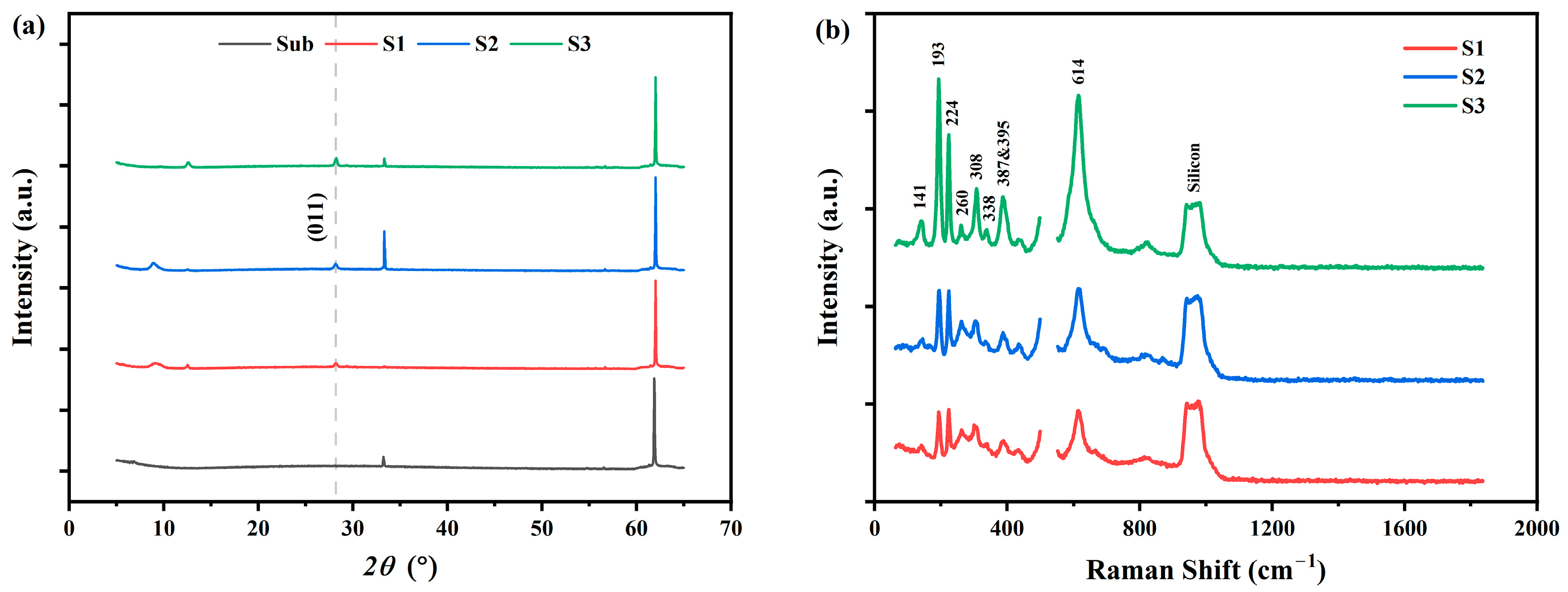
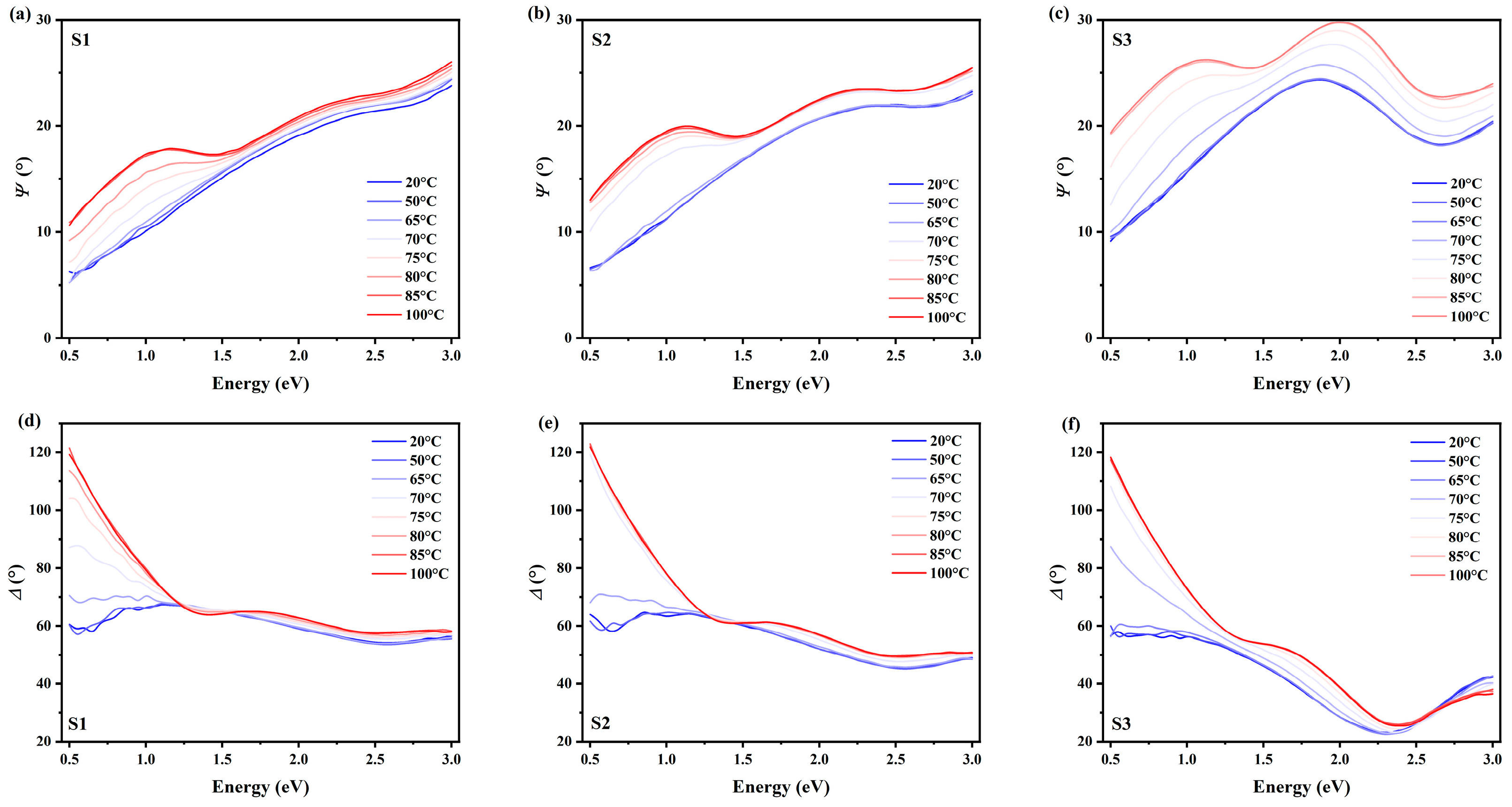





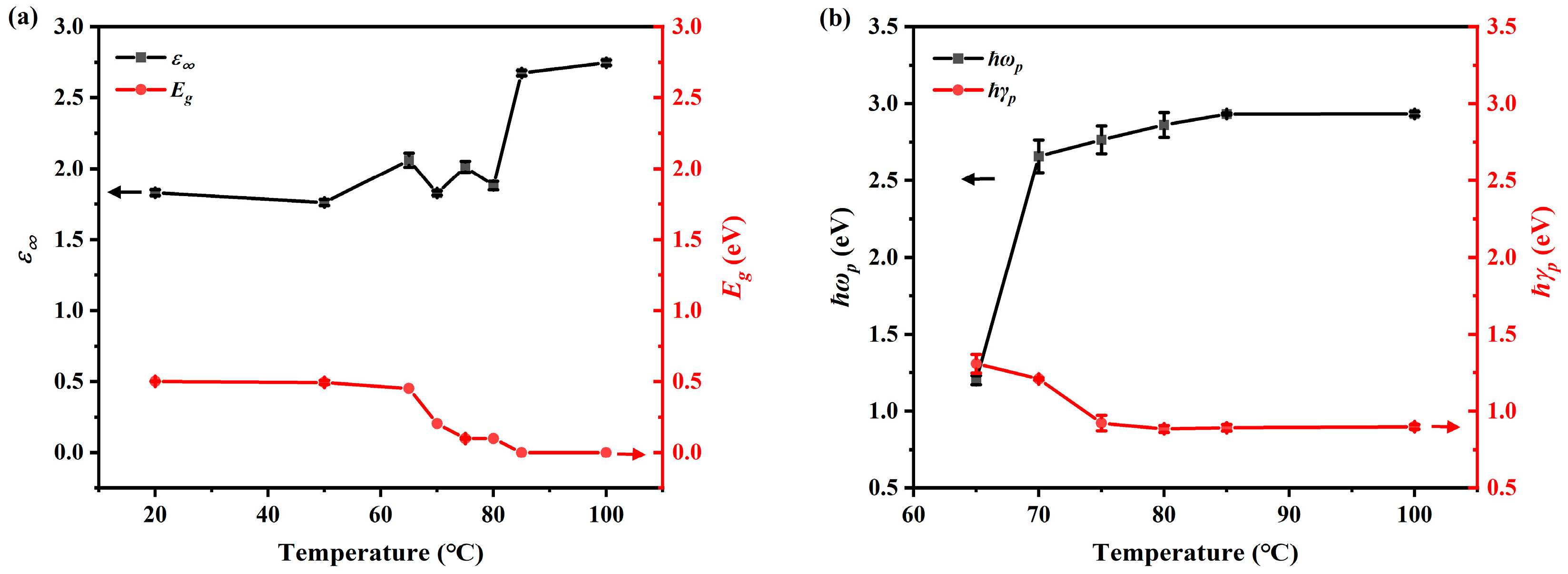
| Sample | (nm) | (h, k, l) | FWHM (°) | D (nm) |
|---|---|---|---|---|
| S1 | 6.19 | (0, 1, 1) | 0.38 | 21 |
| S2 | 12.0 | (0, 1, 1) | 0.36 | 22 |
| S3 | 15.3 | (0, 1, 1) | 0.29 | 27 |
| T (°C) | 20 | 50 | 65 | 70 | 75 | 80 | 85 | 100 |
|---|---|---|---|---|---|---|---|---|
| 1.83 | 1.76 | 2.06 | 1.83 | 2.01 | 1.88 | 2.67 | 2.75 | |
| /eV | / | / | 1.20 | 2.66 | 2.76 | 2.86 | 2.93 | 2.93 |
| /eV | / | / | 1.31 | 1.21 | 0.92 | 0.88 | 0.89 | 0.90 |
| /eV | 0.50 | 0.49 | 0.45 | 0.20 | 0.10 | 0.10 | 0 | 0 |
| RMSE | 0.667 | 0.669 | 0.719 | 1.072 | 1.243 | 1.105 | 1.059 | 1.056 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, X.; Cai, Q.; Qi, J.; Liu, B.; Zheng, Y.; Zhang, R.; Li, J.; Wang, S.; Chen, L.; Lee, Y. Temperature-Dependent Spectroscopic Ellipsometry and Modeling of the Optical Properties of Vanadium Dioxide Thin Films. Crystals 2025, 15, 325. https://doi.org/10.3390/cryst15040325
Sun X, Cai Q, Qi J, Liu B, Zheng Y, Zhang R, Li J, Wang S, Chen L, Lee Y. Temperature-Dependent Spectroscopic Ellipsometry and Modeling of the Optical Properties of Vanadium Dioxide Thin Films. Crystals. 2025; 15(4):325. https://doi.org/10.3390/cryst15040325
Chicago/Turabian StyleSun, Xiaojie, Qingyuan Cai, Jiao Qi, Baojian Liu, Yuxiang Zheng, Rongjun Zhang, Jing Li, Songyou Wang, Liangyao Chen, and Youngpak Lee. 2025. "Temperature-Dependent Spectroscopic Ellipsometry and Modeling of the Optical Properties of Vanadium Dioxide Thin Films" Crystals 15, no. 4: 325. https://doi.org/10.3390/cryst15040325
APA StyleSun, X., Cai, Q., Qi, J., Liu, B., Zheng, Y., Zhang, R., Li, J., Wang, S., Chen, L., & Lee, Y. (2025). Temperature-Dependent Spectroscopic Ellipsometry and Modeling of the Optical Properties of Vanadium Dioxide Thin Films. Crystals, 15(4), 325. https://doi.org/10.3390/cryst15040325









