First-Principles and PSO-Driven Exploration of Ca-Pt Intermetallics: Stable Phases and Pressure-Driven Transitions
Abstract
1. Introduction
2. Calculation Methods
3. Results and Discussion
3.1. Convex Hull
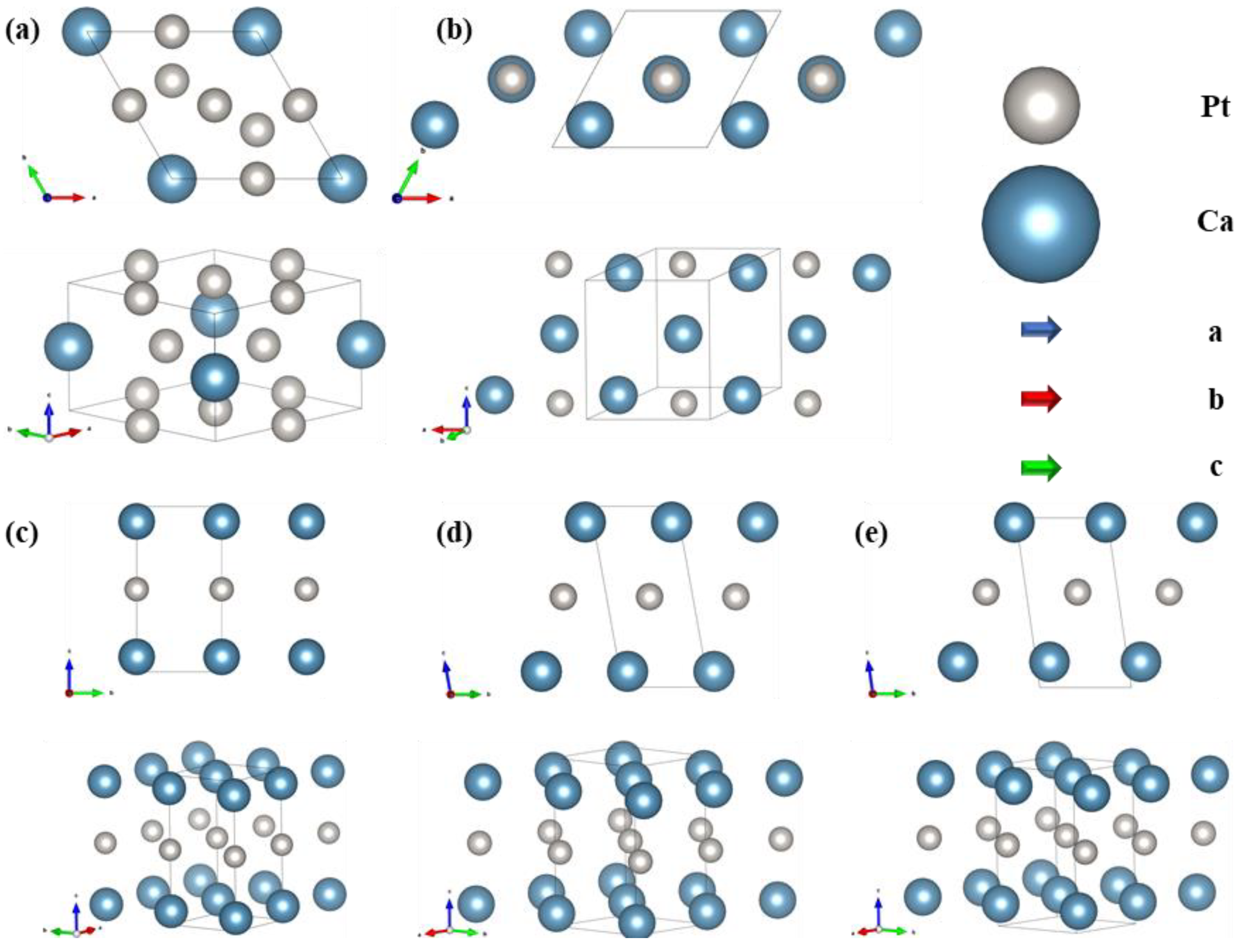
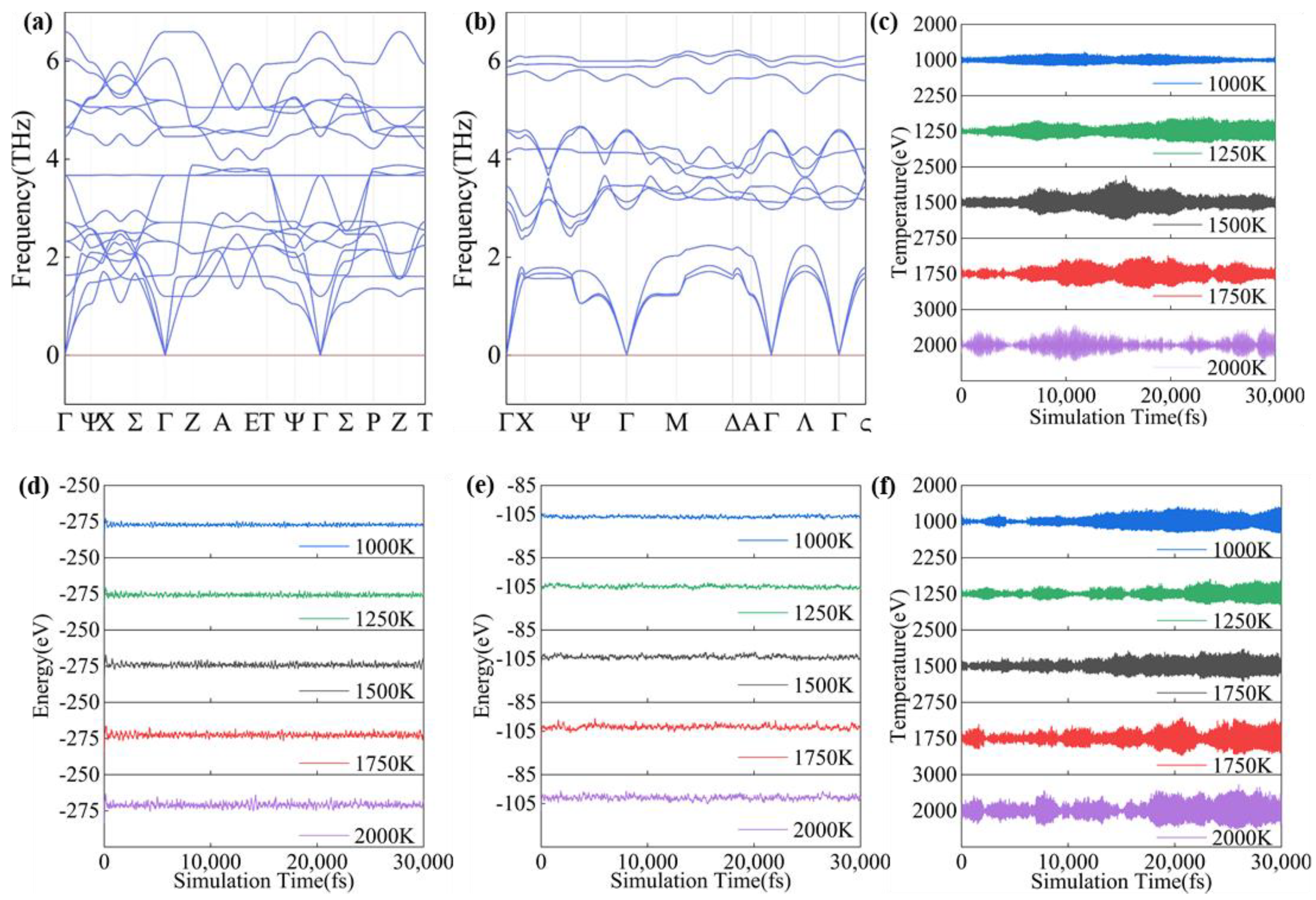
3.2. Structural Characteristics and Stability
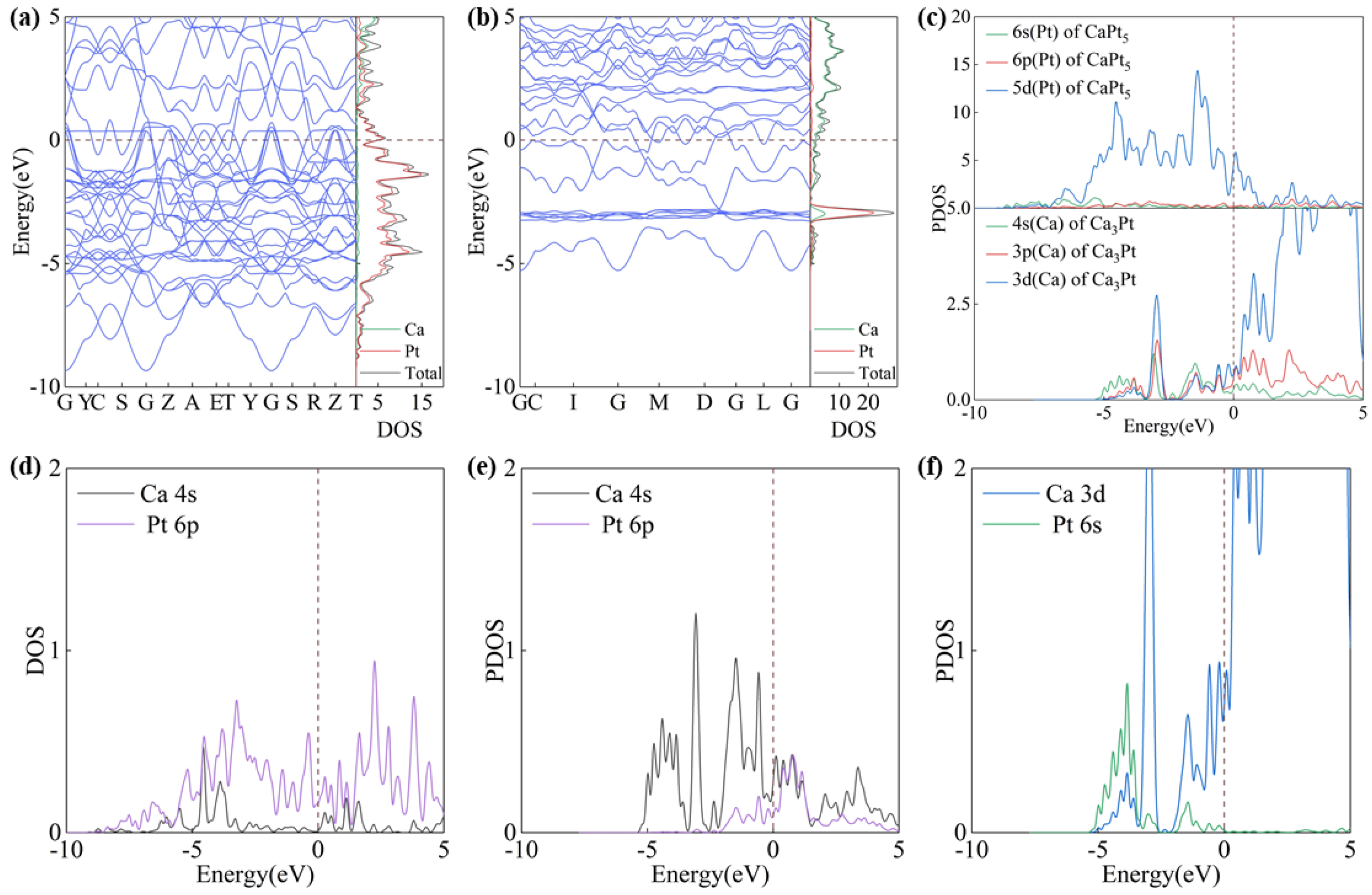
3.3. Electronic Properties
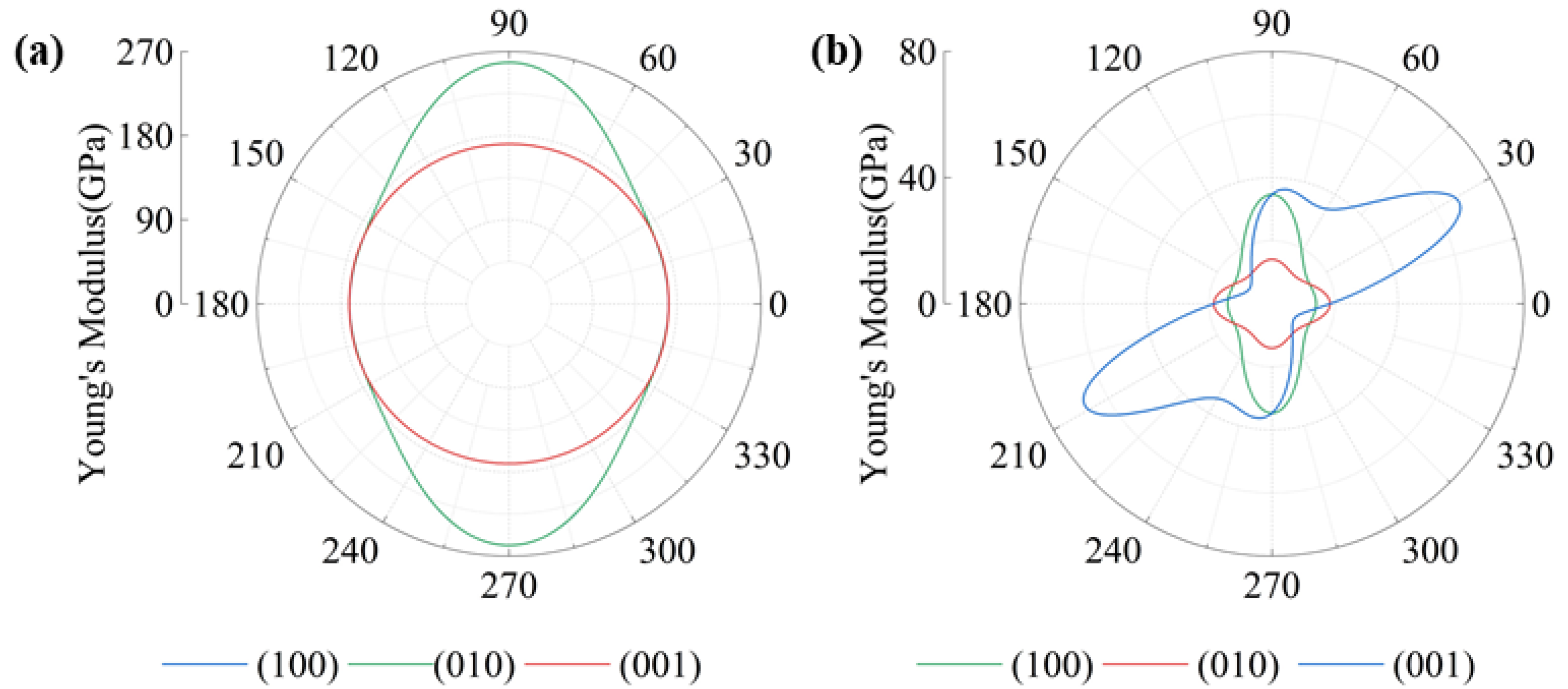
3.4. Mechanical Properties
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yaghi, O.M.; O’Keeffe, M.; Kanatzidis, M. Design of solids from molecular building blocks: Golden opportunities for solid state chemistry. J. Solid State Chem. 2000, 152, 1–2. [Google Scholar] [CrossRef]
- Cava, R.J.; DiSalvo, F.J.; Brus, L.E.; Dunbar, K.R.; Gorman, C.B.; Haile, S.M.; Interrante, L.V.; Musfeldt, J.L.; Navrotsky, A.; Nuzzo, R.G.; et al. Future directions in solid state chemistry: Report of the NSF-sponsored workshop. Prog. Solid State Chem. 2002, 30, 1–101. [Google Scholar] [CrossRef]
- Chamorro, J.R.; McQueen, T.M. Progress toward solid state synthesis by design. Acc. Chem. Res. 2018, 51, 2918–2925. [Google Scholar] [CrossRef]
- Orio, M.; Pantazis, D.A.; Neese, F. Density functional theory. Photosynth. Res. 2009, 102, 443–453. [Google Scholar] [CrossRef]
- Bagayoko, D. Understanding density functional theory (DFT) and completing it in practice. AIP Adv. 2014, 4, 127104. [Google Scholar] [CrossRef]
- Obot, I.B.; Macdonald, D.D.; Gasem, Z.M. Density functional theory (DFT) as a powerful tool for designing new organic corrosion inhibitors. Part 1: An overview. Corros. Sci. 2015, 99, 1–30. [Google Scholar] [CrossRef]
- Kohn, W.; Becke, A.D.; Parr, R.G. Density functional theory of electronic structure. J. Phys. Chem. 1996, 100, 12974–12980. [Google Scholar] [CrossRef]
- Hafner, J. Ab-initio simulations of materials using VASP: Density-functional theory and beyond. J. Comput. Chem. 2008, 29, 2044–2078. [Google Scholar] [CrossRef]
- Clark, S.J.; Segall, M.D.; Pickard, C.J.; Hasnip, P.J.; Probert, M.I.J.; Refson, K.; Payne, M.C. First principles methods using CASTEP. Z. Für Krist. Cryst. Mater. 2005, 220, 567–570. [Google Scholar] [CrossRef]
- Gonze, X.; Amadon, B.; Anglade, P.-M.; Beuken, J.-M.; Bottin, F.; Boulanger, P.; Bruneval, F.; Caliste, D.; Caracas, R.; Côté, M. ABINIT: First-principles approach to material and nanosystem properties. Comput. Phys. Commun. 2009, 180, 2582–2615. [Google Scholar] [CrossRef]
- Crocombette, J.P. Theoretical study of point defects in crystalline zircon. Phys. Chem. Miner. 1999, 27, 138–143. [Google Scholar] [CrossRef]
- Soler, J.M.; Artacho, E.; Gale, J.D.; García, A.; Junquera, J.; Ordejón, P.; Sánchez-Portal, D. The SIESTA method for ab initio order-N materials simulation. J. Phys. Condens. Matter 2002, 14, 2745. [Google Scholar] [CrossRef]
- Zhang, H.; Xie, C.; Chen, L.; Duan, J.; Li, F.; Liu, W. Different reaction mechanisms of SO4•− and •OH with organic compound interpreted at molecular orbital level in Co (II)/peroxymonosulfate catalytic activation system. Water Res. 2023, 229, 119392. [Google Scholar] [CrossRef]
- Giannozzi, P.; Baroni, S.; Bonini, N.; Calandra, M.; Car, R.; Cavazzoni, C.; Ceresoli, D.; Chiarotti, G.L.; Cococcioni, M.; Dabo, I.; et al. QUANTUM ESPRESSO: A modular and open-source software project for quantum simulations of materials. J. Phys. Condens. Matter 2009, 21, 395502. [Google Scholar] [CrossRef] [PubMed]
- Blaha, P.; Schwarz, K.; Tran, F.; Laskowski, R.; Madsen, G.K.H.; Marks, L.D. WIEN2k: An APW+ lo program for calculating the properties of solids. J. Chem. Phys. 2020, 152, 074101. [Google Scholar] [CrossRef]
- Wang, Y.; Lv, J.; Zhu, L.; Ma, Y. CALYPSO: A method for crystal structure prediction. Comput. Phys. Commun. 2012, 183, 2063–2070. [Google Scholar] [CrossRef]
- Glass, C.W.; Oganov, A.R.; Hansen, N. USPEX—Evolutionary crystal structure prediction. Comput. Phys. Commun. 2006, 175, 713–720. [Google Scholar] [CrossRef]
- Eickerling, G.; Reiher, M. The shell structure of atoms. J. Chem. Theory Comput. 2008, 4, 286–296. [Google Scholar] [CrossRef]
- Schwerdtfeger, P.; Smits, O.R.; Pyykkö, P. The periodic table and the physics that drives it. Nat. Rev. Chem. 2020, 4, 359–380. [Google Scholar] [CrossRef]
- Miao, M.; Sun, Y.; Zurek, E.; Lin, H. Chemistry under high pressure. Nat. Rev. Chem. 2020, 4, 508–527. [Google Scholar] [CrossRef]
- Schweizer, A.E.; Kerr, G.T. Thermal decomposition of hexachloroplatinic acid. Inorg. Chem. 1978, 17, 2326–2327. [Google Scholar] [CrossRef]
- Arnold, W.; Eric, S.P.; John, A.R.; Emory, E.T. Handbook of Organic Compounds. Nature 1956, 177, 639–640. [Google Scholar]
- Miedema, A.R.; De Boer, F.R.; De Chatel, P.F. Empirical description of the role of electronegativity in alloy formation. J. Phys. F Met. Phys. 1973, 3, 1558. [Google Scholar] [CrossRef]
- Corbett, J.D. Polyatomic Zintl anions of the post-transition elements. Chem. Rev. 1985, 85, 383–397. [Google Scholar] [CrossRef]
- Pyykko, P.; Desclaux, J.P. Relativity and the periodic system of elements. Acc. Chem. Res. 1979, 12, 276–281. [Google Scholar] [CrossRef]
- Desclaux, J.P. Relativistic Dirac-Fock expectation values for atoms with Z = 1 to Z = 120. At. Data Nucl. Data Tables 1973, 12, 311–406. [Google Scholar] [CrossRef]
- Andersen, T.; Haugen, H.K.; Hotop, H. Binding energies in atomic negative ions: III. J. Phys. Chem. Ref. Data 1999, 28, 1511–1533. [Google Scholar] [CrossRef]
- Arrieta, R.; Brgoch, J. Forming Platinide Phases under Pressure in the Cs–Pt System. J. Phys. Chem. C 2022, 126, 2062–2069. [Google Scholar] [CrossRef]
- Smetana, V.; Mudring, A.V. Cesium platinide hydride 4Cs2Pt CsH: An intermetallic double salt featuring metal anions. Angew. Chem. Int. Ed. 2016, 55, 14838–14841. [Google Scholar] [CrossRef]
- Karpov, A.; Nuss, J.; Wedig, U.; Jansen, M. Cs2Pt: A Platinide (-ii) Exhibiting Complete Charge Separation. Angew. Chem. Int. Ed. 2003, 42, 4818–4821. [Google Scholar] [CrossRef]
- Mao, J.; Wang, Y.; Li, K.; Chen, Y.-C. Pressure-Enriched Chemistry of Pt: Prediction and Synthesis of Dense Sodium Platinides. J. Phys. Chem. C 2021, 125, 11791–11798. [Google Scholar] [CrossRef]
- Bronger, W.; Nacken, B.; Ploog, K. Zur synthese und struktur von Li2Pt und LiPt. J. Less Common Met. 1975, 43, 143–146. [Google Scholar] [CrossRef]
- Nash, C.P.; Boyden, F.M.; Whittig, L.D. Intermetallic compounds of alkali metals with platinum. A novel preparation of a colloidal platinum hydrogenation catalyst. J. Am. Chem. Soc. 1960, 82, 6203–6204. [Google Scholar] [CrossRef]
- McMillan, P.F. High pressure synthesis of solids. Curr. Opin. Solid State Mater. Sci. 1999, 4, 171–178. [Google Scholar] [CrossRef]
- Badding, J.V. High-pressure synthesis, characterization, and tuning of solid state materials. Annu. Rev. Mater. Sci. 1998, 28, 631–658. [Google Scholar] [CrossRef]
- Prewitt, C.T.; Downs, R.T. High-pressure crystal chemistry. Rev. Mineral. 1998, 37, 284–318. [Google Scholar]
- Li, Y.H. Crystal structure, electronic structure, the density of states, optical properties, and superconducting transition temperature of ZrBeSi crystal under pressure. Phys. Status Solidi 2023, 260, 2300196. [Google Scholar] [CrossRef]
- Zhang, M.; Chen, L.; Feng, L.; Tuo, H.-H.; Zhang, Y.; Wei, Q.; Li, P.-F. Pressure-induced phase transition and electronic structure evolution in layered semimetal HfTe2. Chin. Phys. B 2023, 32, 086101. [Google Scholar] [CrossRef]
- Miao, M.; Brgoch, J.; Krishnapriyan, A.; Goldman, A.; Kurzman, J.A.; Seshadri, R. On the stereochemical inertness of the auride lone pair: Ab initio studies of AAu (A = K, Rb, Cs). Inorg. Chem. 2013, 52, 8183–8189. [Google Scholar] [CrossRef]
- Brgoch, J.; Hermus, M. Pressure-Stabilized Ir3−in a Superconducting Potassium Iridide. J. Phys. Chem. C 2016, 120, 20033–20039. [Google Scholar] [CrossRef]
- Zhong, X.; Li, X.; Yang, L.; Wang, D.; Qu, X.; Liu, H. Predicted Stable Structures of the Li–Ag System at High Pressures. J. Phys. Chem. Lett. 2021, 12, 1671–1675. [Google Scholar] [CrossRef]
- Yang, G.; Wang, Y.; Peng, F.; Bergara, A.; Ma, Y. Gold as a 6p-element in dense lithium aurides. J. Am. Chem. Soc. 2016, 138, 4046–4052. [Google Scholar] [CrossRef]
- Lin, J.; Zhang, S.; Guan, W.; Yang, G.; Ma, Y. Gold with +4 and +6 oxidation states in AuF4 and AuF6. J. Am. Chem. Soc. 2018, 140, 9545–9550. [Google Scholar] [CrossRef]
- Lee, K.K.M.; Jeanloz, R. High-pressure alloying of potassium and iron: Radioactivity in the Earth’s core? Geophys. Res. Lett. 2003, 30, 2212. [Google Scholar] [CrossRef]
- Zhou, Y.; Wang, H.; Zhu, C.; Liu, H.; Tse, J.S.; Ma, Y. Prediction of host–guest Na–Fe intermetallics at high pressures. Inorg. Chem. 2016, 55, 7026–7032. [Google Scholar] [CrossRef]
- Zhang, X.; Yao, Y.; Ding, S.; Bergara, A.; Li, F.; Liu, Y.; Zhou, X.-F.; Yang, G. Superconductivity in Li8Au electride. Phys. Rev. B 2023, 107, L100501. [Google Scholar] [CrossRef]
- Wang, Q.; Zhang, S.; Li, H.; Wang, H.; Liu, G.; Ma, J.; Xu, H.; Liu, H.; Ma, Y. Coexistence of superconductivity and electride states in Ca2H with an antifluorite-type motif under compression. J. Mater. Chem. A 2023, 11, 21345–21353. [Google Scholar] [CrossRef]
- Liu, Y.; Cui, T.; Li, D. Emerging d−d orbital coupling between non-d-block main-group elements Mg and I at high pressure. IScience 2023, 26, 106113. [Google Scholar] [CrossRef]
- Zhao, L.; Zhang, X.; Wan, B.; Zhang, Z.; Shen, W.; Zhang, Y.; Fang, C.; Chen, L.; Wang, Q.; He, J.; et al. A pressure-induced high-pressure metallic GeTe phase. J. Chem. Phys. 2023, 158, 134711. [Google Scholar] [CrossRef]
- Zhang, A.; Cui, Y.; Yue, J.; Li, J.; Pan, Y.; Chen, X.; Liu, Y.; Cui, T. Pressure-induced enhancement of thermoelectric performance of CoP3 by the structural phase transition. Acta Mater. 2023, 248, 118773. [Google Scholar] [CrossRef]
- He, X.-L.; Zhang, P.; Ma, Y.; Li, H.; Zhong, X.; Wang, Y.; Liu, H.; Ma, Y. Potential high-temperature superconductivity in the substitutional alloy of (Y, Sr) H11 under high pressure. Phys. Rev. B 2023, 107, 134509. [Google Scholar] [CrossRef]
- Rahman, S.; Wang, L.; Saqib, H.; Errandonea, D.; Yang, L.; Zhao, Y.; Zhuang, Y.; Gao, G.; Wang, L.; Tian, Y. Metallization and superconductivity with Tc > 12 K in transition metal dichalcogenide HfS2 under pressure. Mater. Today Phys. 2023, 34, 101091. [Google Scholar] [CrossRef]
- Wei, X.; Hao, X.; Bergara, A.; Zurek, E.; Liang, X.; Wang, L.; Song, X.; Li, P.; Wang, L.; Gao, G.; et al. Designing ternary superconducting hydrides with A15-type structure at moderate pressures. Mater. Today Phys. 2023, 34, 101086. [Google Scholar] [CrossRef]
- Pei, C.; Zhang, J.; Wang, Q.; Zhao, Y.; Gao, L.; Gong, C.; Tian, S.; Luo, R.; Li, M.; Yang, W.; et al. Pressure-induced superconductivity at 32 K in MoB2. Natl. Sci. Rev. 2023, 10, nwad034. [Google Scholar] [CrossRef]
- Dai, G.; Jia, Y.; Gao, B.; Peng, Y.; Zhao, J.; Ma, Y.; Chen, C.; Zhu, J.; Li, Q.; Yu, R. Pressure-induced superconductivity in the nonsymmorphic topological insulator KHgAs. NPG Asia Mater. 2023, 15, 52. [Google Scholar] [CrossRef]
- Shan, P.F.; Han, X.; Li, X.; Liu, Z.Y.; Yang, P.T.; Wang, B.S.; Wang, J.F.; Liu, H.Y.; Shi, Y.G.; Sun, J.P.; et al. Pressure-induced metallic state in a van der Waals cluster Mott insulator Nb3Cl8. Mater. Today Phys. 2023, 38, 101267. [Google Scholar] [CrossRef]
- Yan, D.; Kong, L.; Xu, B.; Yang, B. One−Step Synthesis Strategy for a Platinum−Based Alloy Catalyst Designed via Crystal−Structure Prediction. Molecules 2024, 29, 5634. [Google Scholar] [CrossRef]
- Nelson, R.; Ertural, C.; Geogre, J.; Deringer, V.L.; Hautier, G. LOBSTER: Local orbital projections, atomic charges, and chemical-bonding analysis from projector-augmented-wave-based density-functional theory. J. Comput. Chem. 2020, 41, 1931–1940. [Google Scholar] [CrossRef]
- Henkelman, G.; Andri, A.; Hannes, J. A fast and robust algorithm for Bader decomposition of charge density. Comput. Mater. Sci. 2006, 36, 354–360. [Google Scholar] [CrossRef]
- Wang, V.; Xu, N.; Liu, J.-C.; Tang, G.; Geng, W.-T. VASPKIT: A user-friendly interface facilitating high-throughput computing and analysis using VASP code. Comput. Phys. Commun. 2021, 267, 108033. [Google Scholar] [CrossRef]
- Wood, E.A.; Compton, V.B. Laves-phase compounds of alkaline earths and noble metals. Acta Crystallogr. 1958, 11, 429–433. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Yan, D. First-Principles and PSO-Driven Exploration of Ca-Pt Intermetallics: Stable Phases and Pressure-Driven Transitions. Crystals 2025, 15, 263. https://doi.org/10.3390/cryst15030263
Wang Y, Yan D. First-Principles and PSO-Driven Exploration of Ca-Pt Intermetallics: Stable Phases and Pressure-Driven Transitions. Crystals. 2025; 15(3):263. https://doi.org/10.3390/cryst15030263
Chicago/Turabian StyleWang, Yifei, and Dengjie Yan. 2025. "First-Principles and PSO-Driven Exploration of Ca-Pt Intermetallics: Stable Phases and Pressure-Driven Transitions" Crystals 15, no. 3: 263. https://doi.org/10.3390/cryst15030263
APA StyleWang, Y., & Yan, D. (2025). First-Principles and PSO-Driven Exploration of Ca-Pt Intermetallics: Stable Phases and Pressure-Driven Transitions. Crystals, 15(3), 263. https://doi.org/10.3390/cryst15030263



