Photoinduced Interactions in Thin Films of Azo Dyes and Planar-Aligned Nematic Liquid Crystal
Abstract
1. Introduction
2. Interaction Potential
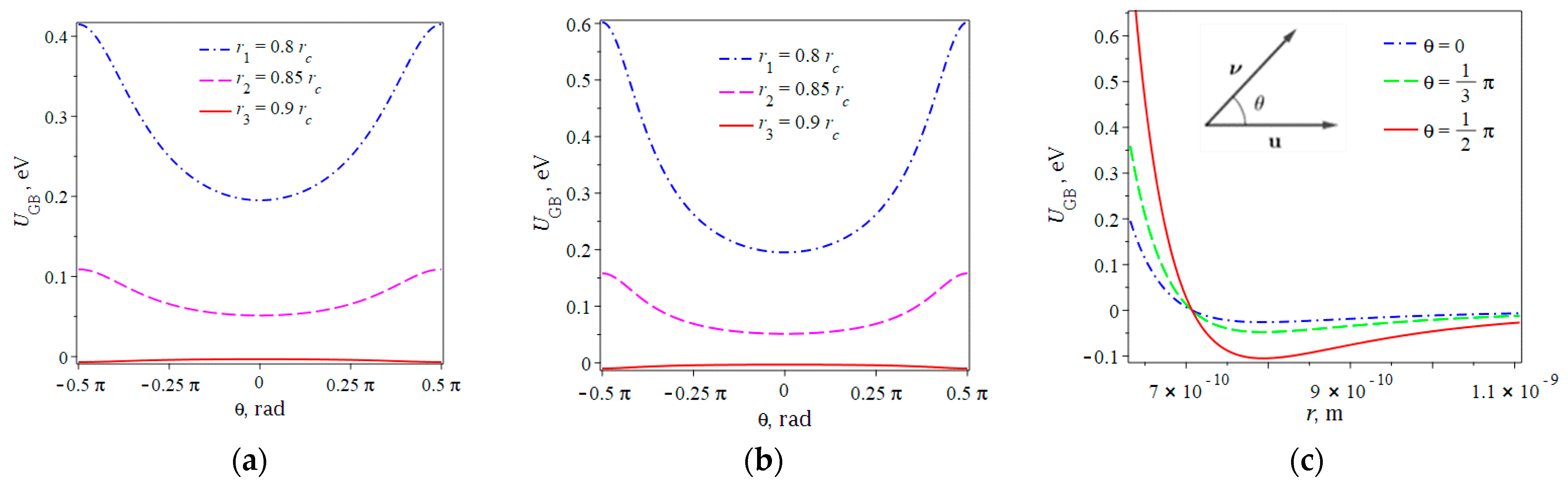
2.1. Dispersion Interactions
2.2. Steric Interactions
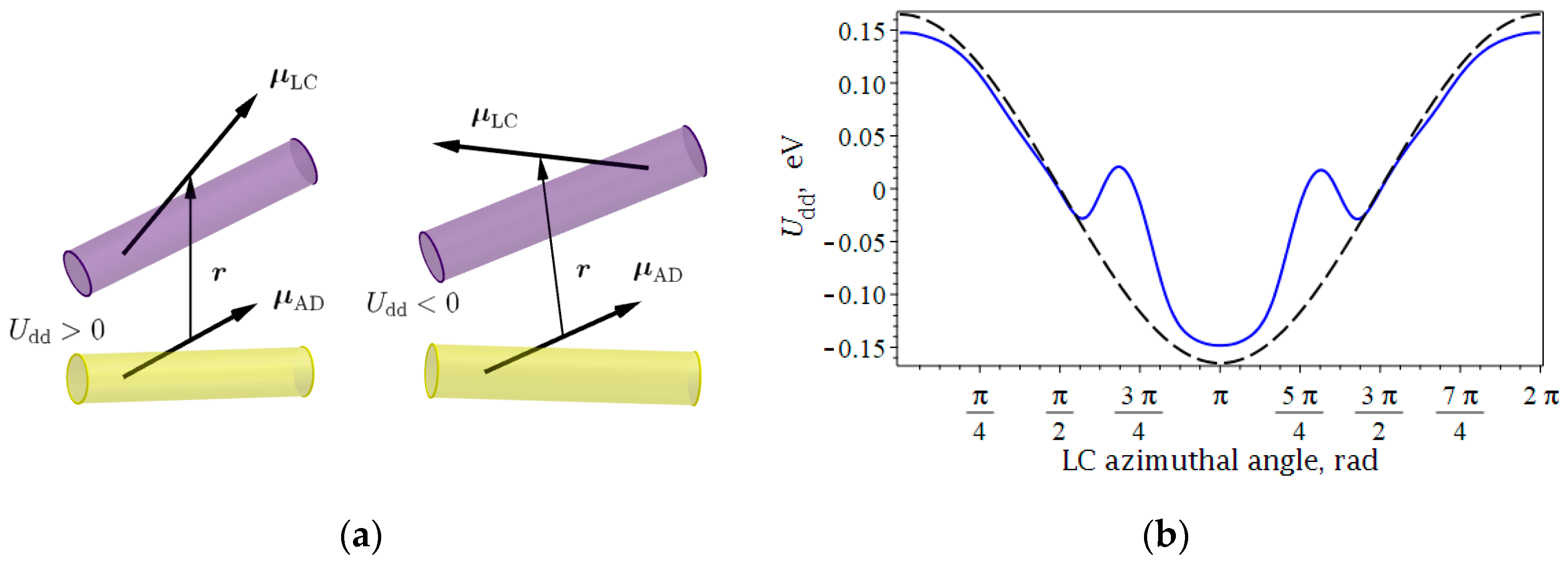
2.3. Photoinduced Potential
3. Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ichimura, K.; Suzuki, Y.; Seki, T.; Hosoki, A.; Aoki, K. Reversible change in alignment mode of nematic liquid crystals regulated photochemically by command surfaces modified with an azobenzene monolayer. Langmuir 1988, 4, 1214–1216. [Google Scholar] [CrossRef]
- Ichimura, K. Photoalignment of Liquid-Crystal Systems. Chem. Rev. 2000, 100, 1847–1874. [Google Scholar] [CrossRef] [PubMed]
- Chigrinov, V.; Pikin, S.; Verevochnikov, A.; Kozenkov, V.; Khazimullin, M.; Ho, J.; Huang, D.D.; Kwok, H.-S. Diffusion model of photoaligning in azo-dye layers. Phys. Rev. E 2004, 69, 061713. [Google Scholar] [CrossRef] [PubMed]
- Nasrollahi, A.; Rella, A.K.; Kumar, V.; Kang, S.-W. Stepwise Progression of Dye-Induced In Situ Photoalignment and Subsequent Stabilization for Noncontact Alignment of Liquid Crystals. ACS Appl. Mater. Interfaces 2024, 16, 24052–24062. [Google Scholar] [CrossRef]
- Quiroga, J.A.; Canga, I.; Alonso, J.; Crespo, D. Reversible Photoalignment of Liquid Crystals: A Path toward the Creation of Rewritable Lenses. Sci. Rep. 2020, 10, 1–9. [Google Scholar] [CrossRef]
- Pan, J.-T.; Zhu, B.-H.; Ma, L.-L.; Chen, W.; Zhang, G.-Y.; Tang, J.; Liu, Y.; Wei, Y.; Zhang, C.; Zhu, Z.-H.; et al. Nonlinear geometric phase coded ferroelectric nematic fluids for nonlinear soft-matter photonics. Nat. Commun. 2024, 15, 8732. [Google Scholar] [CrossRef]
- Folwill, Y.; Zeitouny, Z.; Lall, J.; Zappe, H. A practical guide to versatile photoalignment of azobenzenes. Liq. Cryst. 2021, 48, 862–872. [Google Scholar] [CrossRef]
- Li, C.-Y.; Liu, S.-J.; Wu, H.-J.; Jiang, J.-Q.; Zhao, B.; Rosales-Guzmán, C.; Zhu, Z.-H.; Chen, P.; Lu, Y.-Q. Modal interface for structured light via liquid-crystal planar optics. Phys. Rev. Appl. 2024, 21, 034021. [Google Scholar] [CrossRef]
- Nys, I.; Ropač, P.; Berteloot, B.; Ravnik, M.; Neyts, K. Highly dispersive liquid crystal diffraction gratings with continuously varying periodicity. J. Mol. Liq. 2023, 383, 122062. [Google Scholar] [CrossRef]
- Ferrarini, A.; Moro, G.; Nordio, P.; Luckhurst, G. A shape model for molecular ordering in nematics. Mol. Phys. 1992, 77, 1–15. [Google Scholar] [CrossRef]
- Dadivanyan, A.K.; Pashinina, Y.M.; Chausov, D.N.; Belyaev, V.V.; Solomatin, A.S. Mesogen Molecules Orientation on Crystal Surfaces. Mol. Cryst. Liq. Cryst. 2011, 545, 159–1383. [Google Scholar] [CrossRef]
- Tavarone, R.; Charbonneau, P.; Stark, H. Kinetic Monte Carlo simulations for birefringence relaxation of photo-switchable molecules on a surface. J. Chem. Phys. 2016, 144, 104703. [Google Scholar] [CrossRef] [PubMed]
- Geng, Y.; Yao, L. Effect of azimuthal anchoring energy on rewriting speed of optical rewritable e-paper. Liq. Cryst. 2021, 48, 915–921. [Google Scholar] [CrossRef]
- de Souza, R.F.; Zannoni, C. Rotational diffusion of shape-switching particles dissolved in biaxial liquid crystals. Liq. Cryst. 2023, 51, 919–935. [Google Scholar] [CrossRef]
- Jung, K.H.; Hyun, S.-Y.; Song, D.-M.; Shin, D.-M. The characteristics of polyimide photoalignment layer with chalcone derivatives produced by linear polarized UV light. Opt. Mater. 2003, 21, 663–666. [Google Scholar] [CrossRef]
- Song, D.-M.; Jung, K.-H.; Moon, J.-H.; Shin, D.-M. Photochemistry of chalcone and the application of chalcone-derivatives in photo-alignment layer of liquid crystal display. Opt. Mater. 2003, 21, 667–671. [Google Scholar] [CrossRef]
- Dogra, A.R.; Sharma, V.; Malik, P.; Kumar, P. In-situ homeotropic alignment of dye doped liquid crystal molecules on multilayered self assembled nanoparticles in confined cells for next generation display devices. Opt. Mater. 2024, 154, 115731. [Google Scholar] [CrossRef]
- Ho, J.Y.L.; Chigrinov, V.G.; Kwok, H.S. Variable liquid crystal pretilt angles generated by photoalignment of a mixed polyimide alignment layer. Appl. Phys. Lett. 2007, 90, 2748345. [Google Scholar] [CrossRef]
- Almasri, R.M.; Lim, S.S.; Lovell, N.H.; Ladouceur, F.; Al Abed, A. Alignment assessment of anisotropic liquid crystals through an automated image processing algorithm. J. Mol. Liq. 2024, 408, 125243. [Google Scholar] [CrossRef]
- Ma, H.; Wereley, S.T.; Linnes, J.C.; Kinzer-Ursem, T.L. Computational fluid dynamics method for determining the rotational diffusion coefficient of cells. Phys. Fluids 2024, 36, 0193862. [Google Scholar] [CrossRef]
- Berteloot, B.; Nys, I.; Liu, S.; Neyts, K. Two-Dimensional Liquid-Crystal Photoalignment by Multiple Illumination Steps. ACS Appl. Opt. Mater. 2023, 2, 1295–1302. [Google Scholar] [CrossRef]
- Blinov, L.M. Structure and Properties of Liquid Crystals; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2010; Volume 123. [Google Scholar]
- Guo, Q.; Srivastava, A.; Chigrinov, V.; Kwok, H. Polymer and azo-dye composite: A photo-alignment layer for liquid crystals. Liq. Cryst. 2014, 41, 1465–1472. [Google Scholar] [CrossRef]
- Kiselev, A.D.; Chigrinov, V.G.; Kwok, H.-S. Kinetics of photoinduced ordering in azo-dye films: Two-state and diffusion models. Phys. Rev. E 2009, 80, 011706. [Google Scholar] [CrossRef] [PubMed]
- Gay, J.G.; Berne, B.J. Modification of the overlap potential to mimic a linear site–site potential. J. Chem. Phys. 1981, 74, 3316–3319. [Google Scholar] [CrossRef]
- Chigrinov, V.G.; Kozenkov, V.M.; Kwok, H.-S. Photoalignment of Liquid Crystalline Materials: Physics and Applications; John Wiley & Sons: Hoboken, NJ, USA, 2008; Volume 17. [Google Scholar]
- Ishihara, S.; Mizusaki, M. Alignment control technology of liquid crystal molecules. J. Soc. Inf. Disp. 2020, 28, 44–74. [Google Scholar] [CrossRef]
- Osipov, M. Dipole-dipole interactions and the origin of ferroelectric ordering in polar nematics. Liq. Cryst. 2024, 1–7. [Google Scholar] [CrossRef]
- Dunmur, D.; Palffy-Muhoray, P. A mean field theory of dipole-dipole correlation in nematic liquid crystals. Mol. Phys. 1992, 76, 1015–1023. [Google Scholar] [CrossRef]
- Thoms, E.; Yu, L.; Richert, R. From very low to high fields: The dielectric behavior of the liquid crystal 5CB. J. Mol. Liq. 2022, 368, 120664. [Google Scholar] [CrossRef]
- Agwamba, E.C.; Udoikono, A.D.; Louis, H.; Udoh, E.U.; Benjamin, I.; Igbalagh, A.T.; Edet, H.O.; Ejiofor, E.U.; Ushaka, U.B. Synthesis, characterization, DFT studies, and molecular modeling of azo dye derivatives as potential candidate for trypanosomiasis treatment. Chem. Phys. Impact 2022, 4, 100076. [Google Scholar] [CrossRef]
- Muravsky, A.; Murauski, A.; Kukhta, I.N. Photoinduced hole dipoles’ mechanism of liquid crystal photoalignment. Appl. Opt. 2020, 59, 5102–5107. [Google Scholar] [CrossRef]
- Groh, B.; Dietrich, S. Long-ranged orientational order in dipolar fluids. Phys. Rev. Lett. 1994, 72, 2422–2425. [Google Scholar] [CrossRef] [PubMed]
- Wells, B.A.; Chaffee, A.L. Ewald summation for molecular simulations. J. Chem. Theory Comput. 2015, 11, 3684–3695. [Google Scholar] [CrossRef] [PubMed]
- Onsager, L. The effects of shape on the interaction of colloidal particles. Ann. N. Y. Acad. Sci. 1949, 51, 627–659. [Google Scholar] [CrossRef]
- Zannoni, C. Liquid Crystals and Their Computer Simulations; Cambridge University Press: Cambridge, UK, 2022. [Google Scholar]
- O’Neill, M.; Kelly, S.M. Photoinduced surface alignment for liquid crystal displays. J. Phys. D Appl. Phys. 2000, 33, R67–R84. [Google Scholar] [CrossRef]
- Martínez, L.; Andrade, R.; Birgin, E.G.; Martínez, J.M. Packmol: A package for building initial configurations for molecular dynamics simulations. J. Comput. Chem. 2009, 30, 2157–2164. [Google Scholar] [CrossRef]
- Boychuk, A.; Shibaev, V.; Cigl, M.; Pomeisl, K.; Hamplová, V.; Pociecha, D.; Bubnov, A.; Bobrovsky, A. Photo-orientation Processes in Liquid Crystalline Polymethacrylates with Side Azobenzene Groups Having Lateral Methyl Substituents. Macromolecules 2021, 54, 10499–10509. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kudreyko, A.; Chigrinov, V.; Perestoronina, A. Photoinduced Interactions in Thin Films of Azo Dyes and Planar-Aligned Nematic Liquid Crystal. Crystals 2025, 15, 22. https://doi.org/10.3390/cryst15010022
Kudreyko A, Chigrinov V, Perestoronina A. Photoinduced Interactions in Thin Films of Azo Dyes and Planar-Aligned Nematic Liquid Crystal. Crystals. 2025; 15(1):22. https://doi.org/10.3390/cryst15010022
Chicago/Turabian StyleKudreyko, Aleksey, Vladimir Chigrinov, and Arina Perestoronina. 2025. "Photoinduced Interactions in Thin Films of Azo Dyes and Planar-Aligned Nematic Liquid Crystal" Crystals 15, no. 1: 22. https://doi.org/10.3390/cryst15010022
APA StyleKudreyko, A., Chigrinov, V., & Perestoronina, A. (2025). Photoinduced Interactions in Thin Films of Azo Dyes and Planar-Aligned Nematic Liquid Crystal. Crystals, 15(1), 22. https://doi.org/10.3390/cryst15010022








