From the Fibonacci Icosagrid to E8 (Part I): The Fibonacci Icosagrid, an H3 Quasicrystal
Abstract
1. Introduction
- It is ordered but not periodic.
- It has long-range quasiperiodic translational order and long-range orientational order. In other words, for any finite patch within the quasicrystal, you can find an infinite number of identical patches at other locations with translational and rotational transformations.
- It has finite types of prototiles/unit cells.
- It has a discrete diffraction pattern.
2. Fibonacci Mutigrid—A New Kind of Quasicrystal
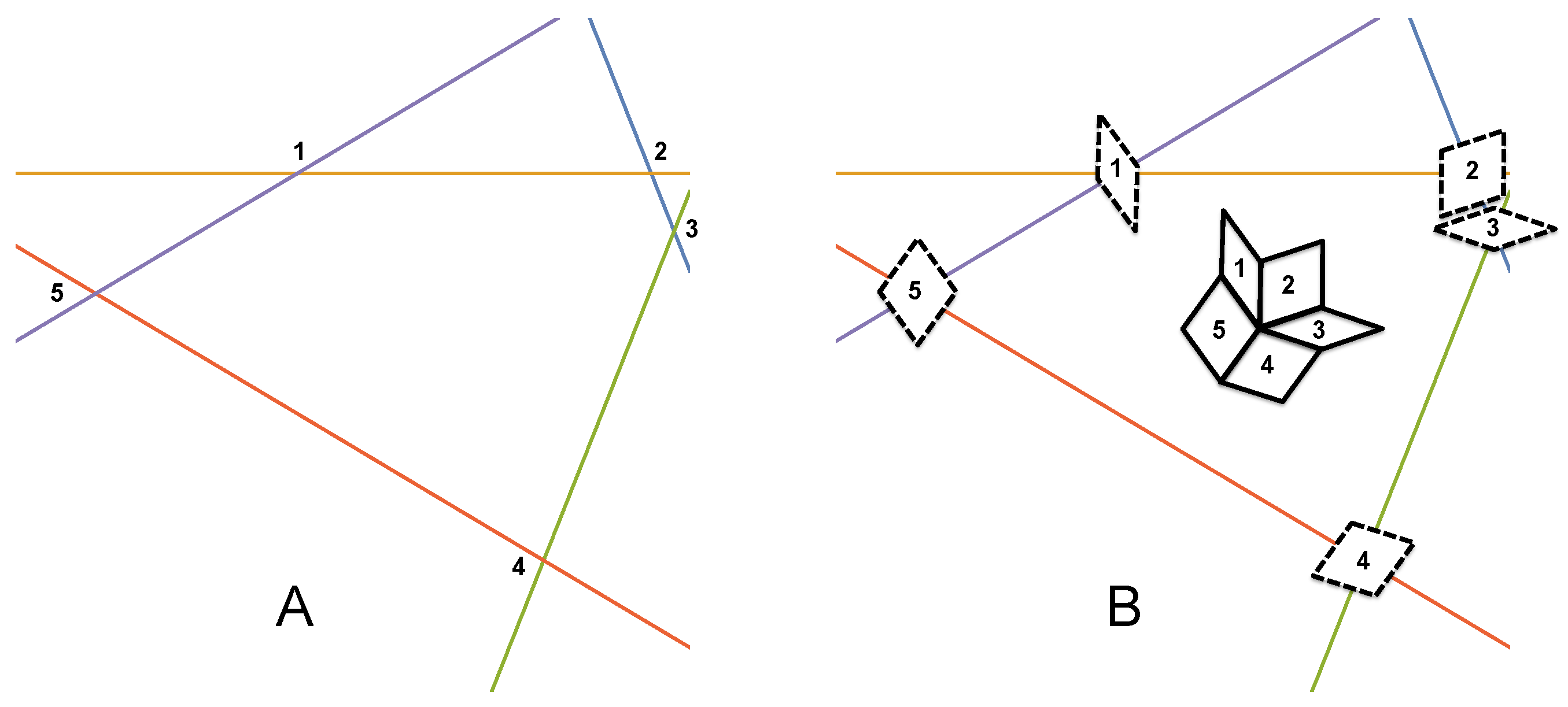
2.1. Review of the Dual-Grid Method
- The last step is to place the rhombi edge to edge while maintaining their original topological connectedness. For example, as shown in Figure 2B, although the cells are translated to be placed edge to edge, cell 1 is always connected to cell 2 through the vertical edge, cell 2 is connected to cell 3 through the other non-vertical edge, and so on.
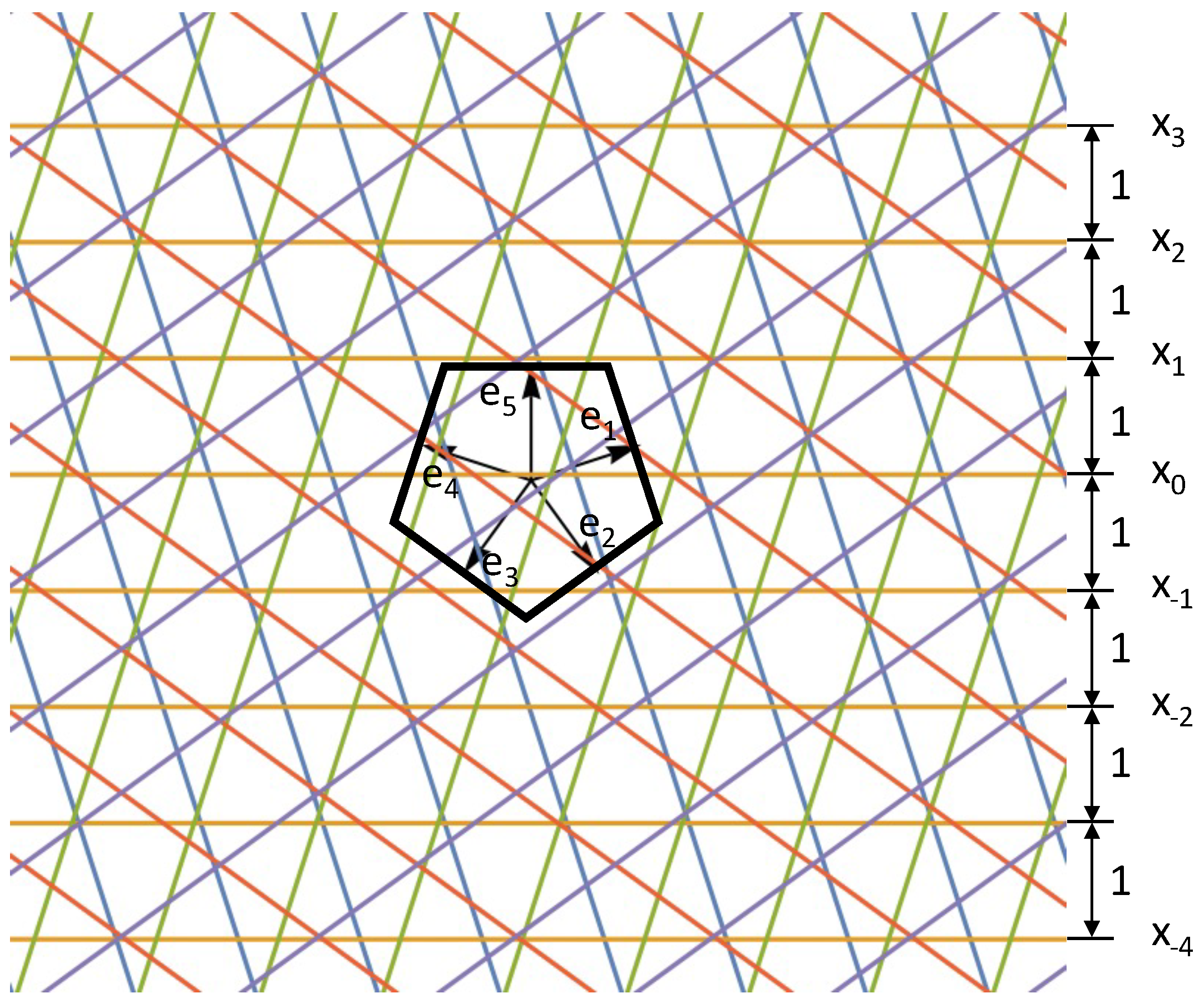
2.2. Fibonacci Multigrid Method
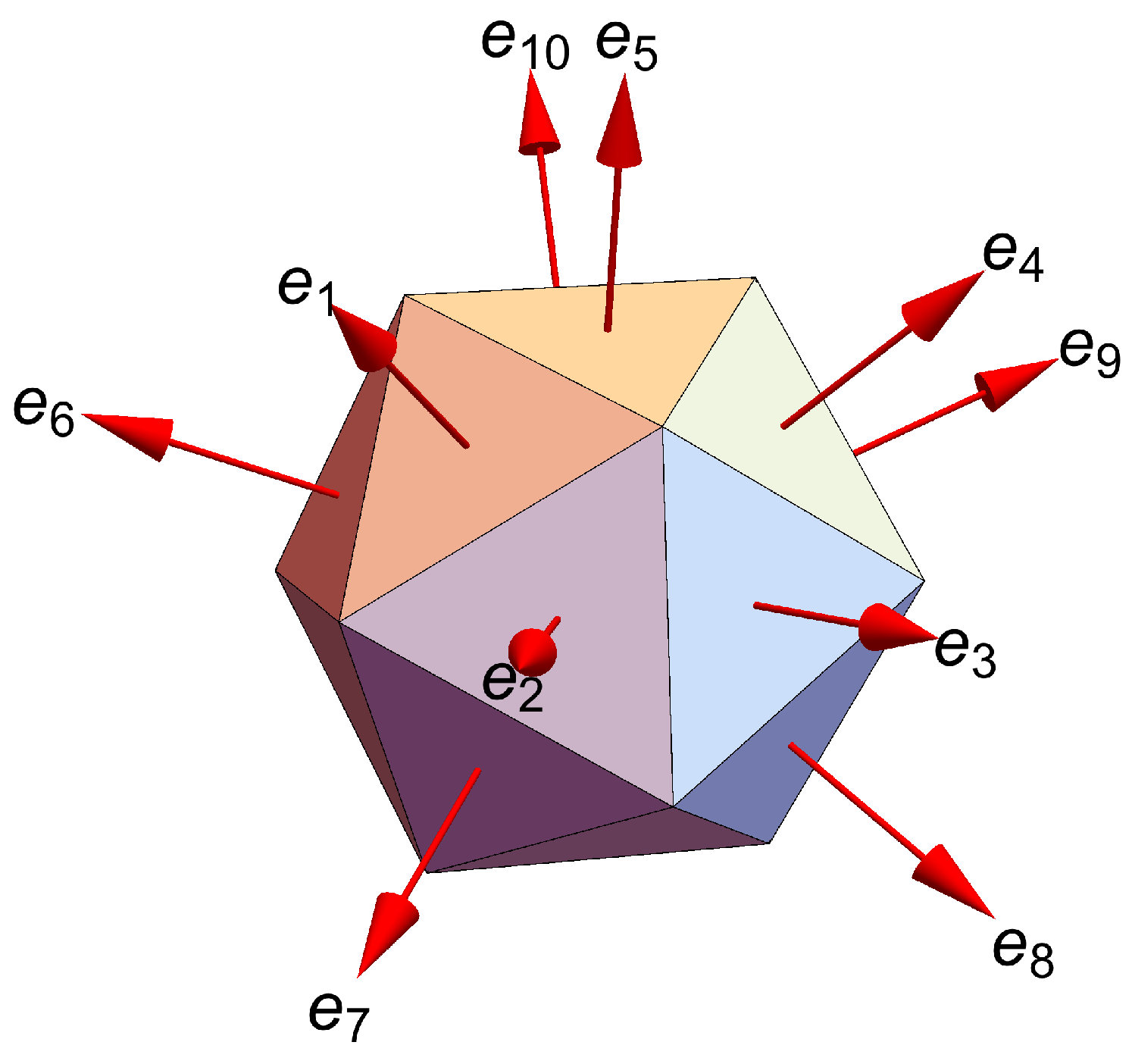
- Consider a d-dimensional polytope centered around the origin, such as a convex regular polytope. Identify the normal vectors for each facet (-dimensional face or hyperface).
- Extend a hyperplane from each facet to infinity. The normal vectors of each facet are given by a normal vector .
- Along each normal vector, place an infinite number of parallel hyperplanes that are aperiodically spaced by the Fibonacci chain. Choose the Fibonacci chain that contains a 180-degree rotational symmetry at the origin. The first letter in the Fibonacci chain is an edge extending from the origin to the facet.
- Find the quasicrystal by identifying all interaction vertices between the Fibonacci-spaced hyperplanes.
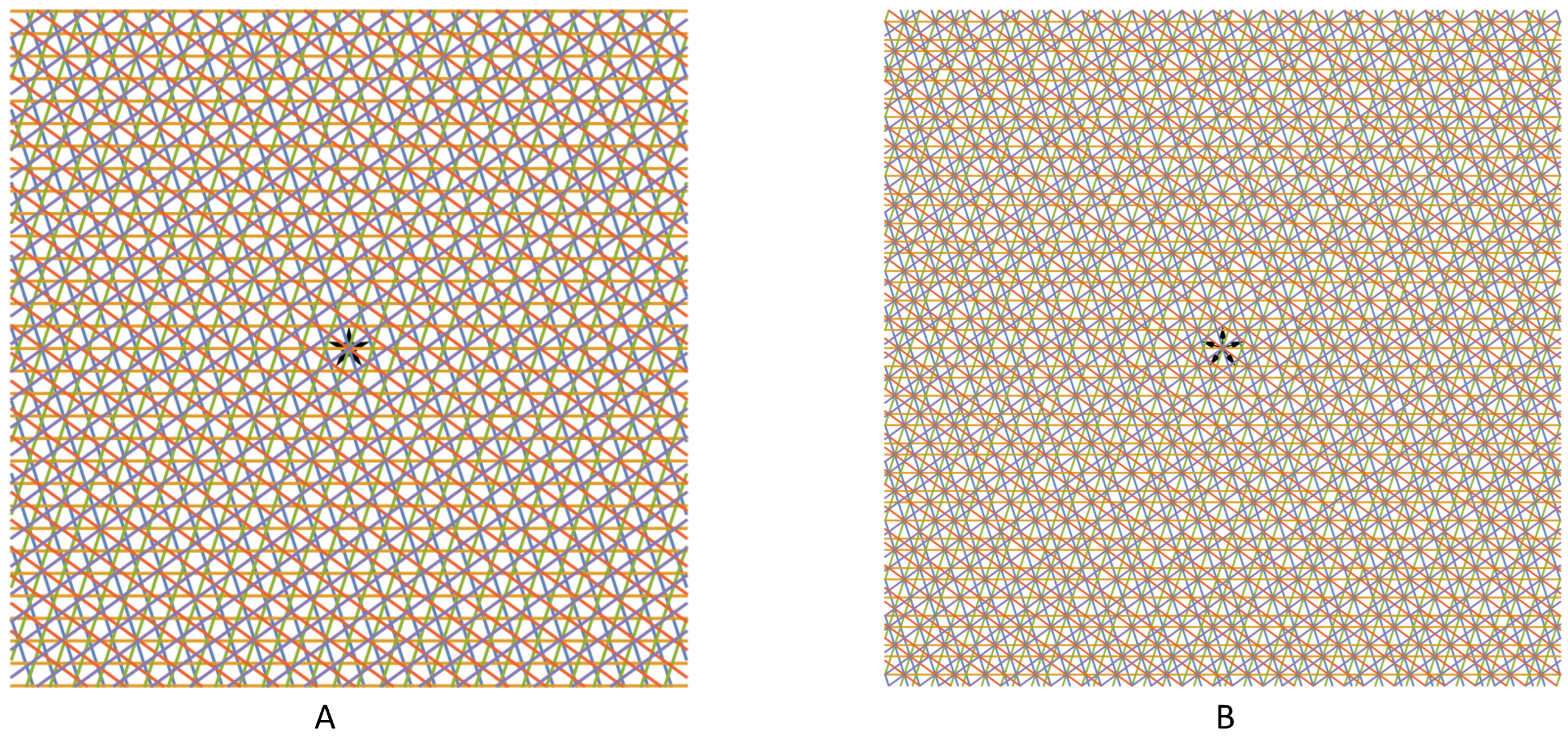
3. Fibonacci Icosagrid and Fibonacci Tetragrid
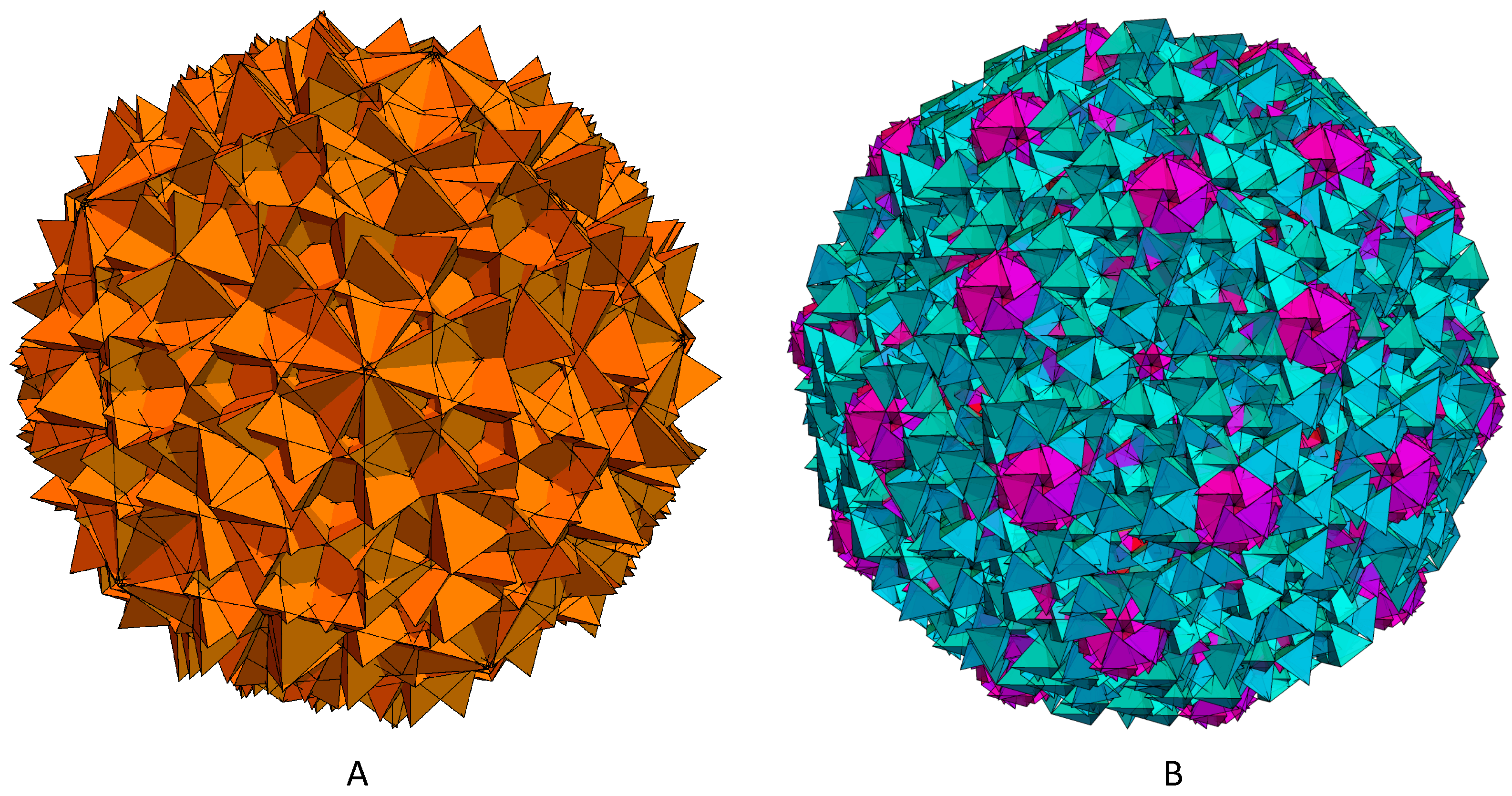
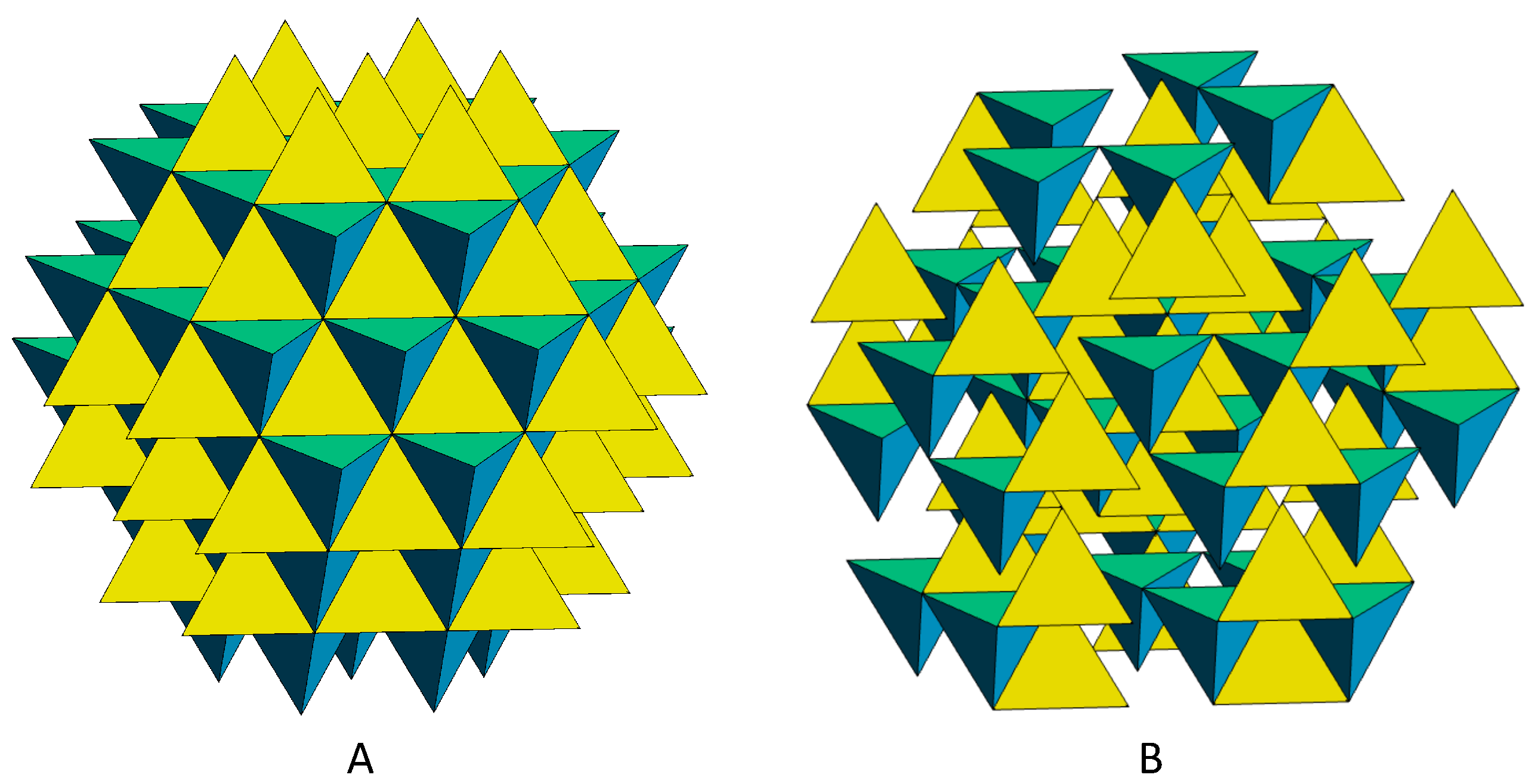
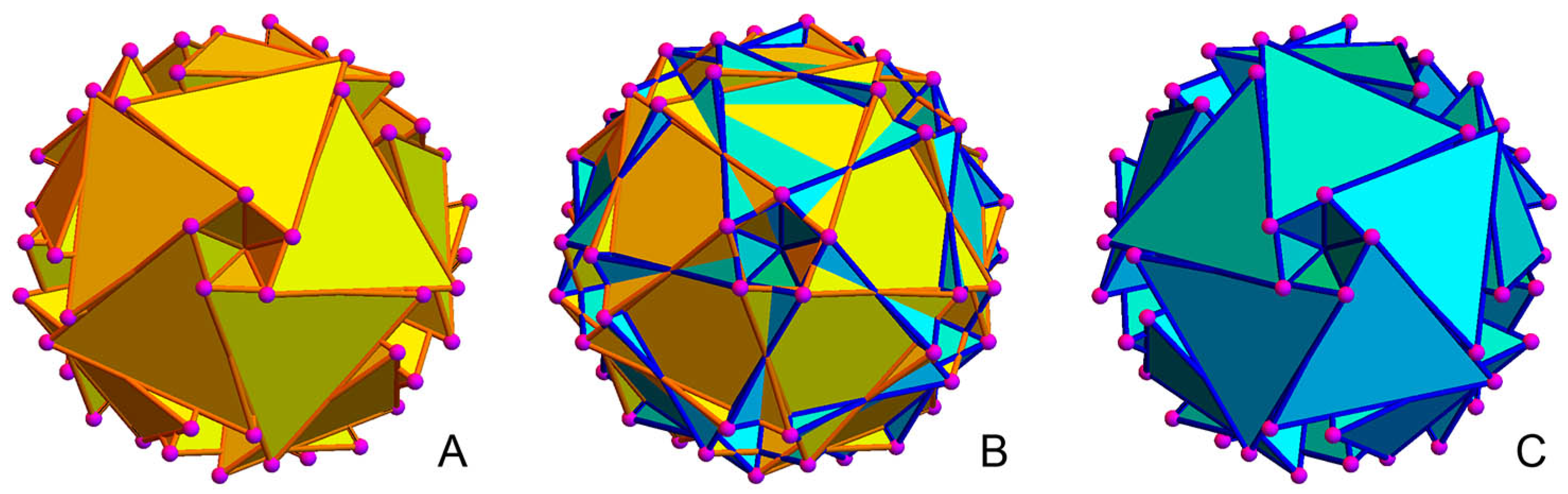
3.1. Icosagrid and Its 1/5th, Tetragrid
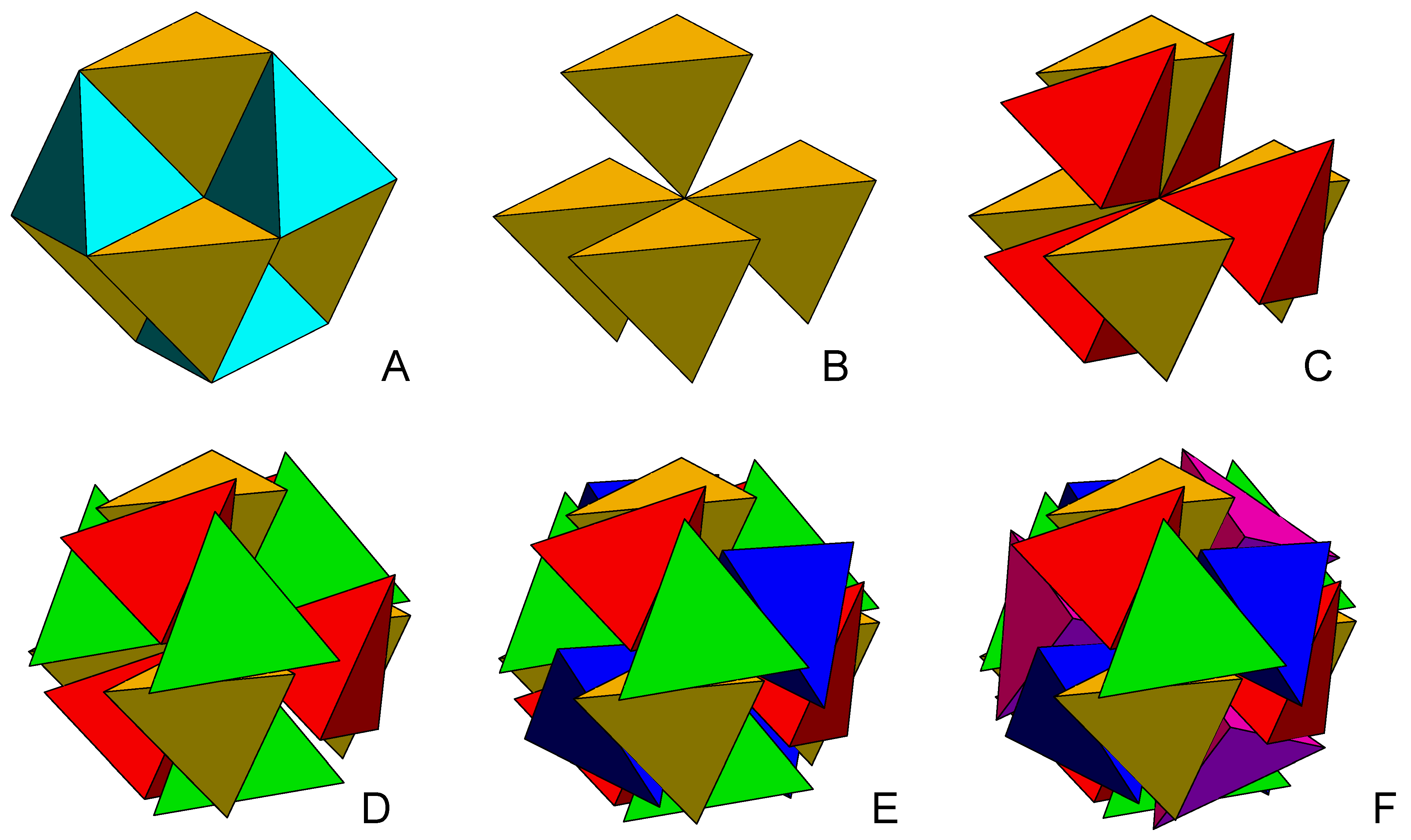
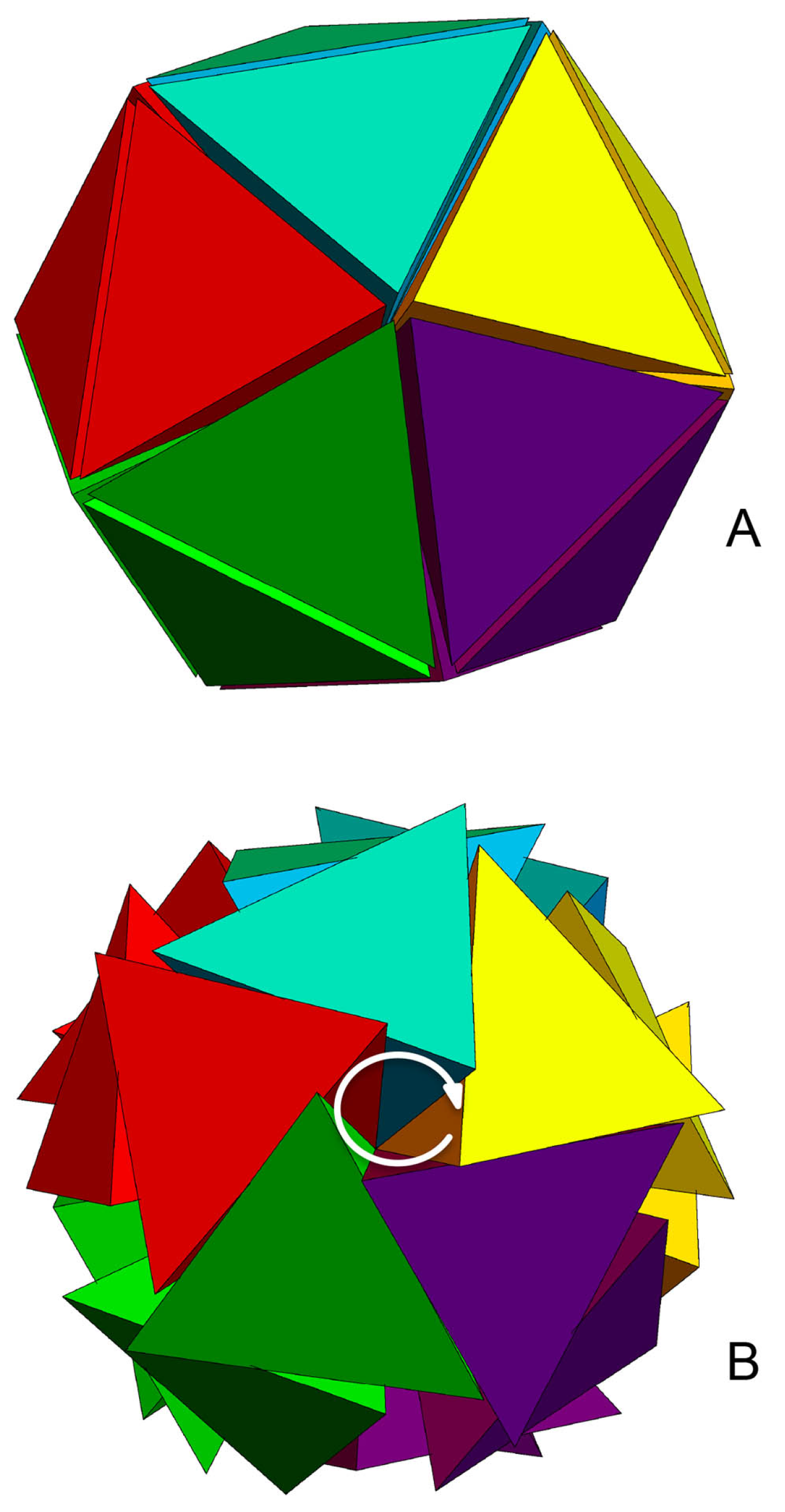
- Our procedure starts at the origin of the tetragrid, where we identify eight tetrahedral cells converging at this point. These are divided into two sets based on orientation: four cells in one orientation and the remaining four in the dual orientation, as illustrated by the yellow tetrahedra in Figure 9A.
- We select the four tetrahedral cells of identical orientation, as shown in Figure 9B, and replicate them to create four copies.
- Two of these copies are then positioned together, ensuring they share their central point. Three of their tetrahedral faces are aligned to be parallel and in contact. This arrangement incorporates a relative rotation angle of —referred to as the golden rotation, as demonstrated in Figure 9C between the gold and red tetrahedron-4-clusters (see [30]).
- This process is repeated three additional times to integrate the remaining three copies—colored green, blue, and pink—into the existing gold and red structure, as depicted in Figure 9C–E. The culmination of this procedure results in a composite structure of five tetrahedron-4-clusters, forming a twisted 20-tetrahedron cluster, or the 20-group (20G), as shown in Figure 9F.
- Subsequently, each tetrahedron-4-cluster is expanded into its full tetragrid form, with only the tetrahedra of the same orientation being considered active. This configuration yields a structure analogous to a chiral icosagrid, depicted in Figure 7A. Conversely, activating the tetrahedral cells of the alternate orientation results in an icosagrid of opposite chirality.
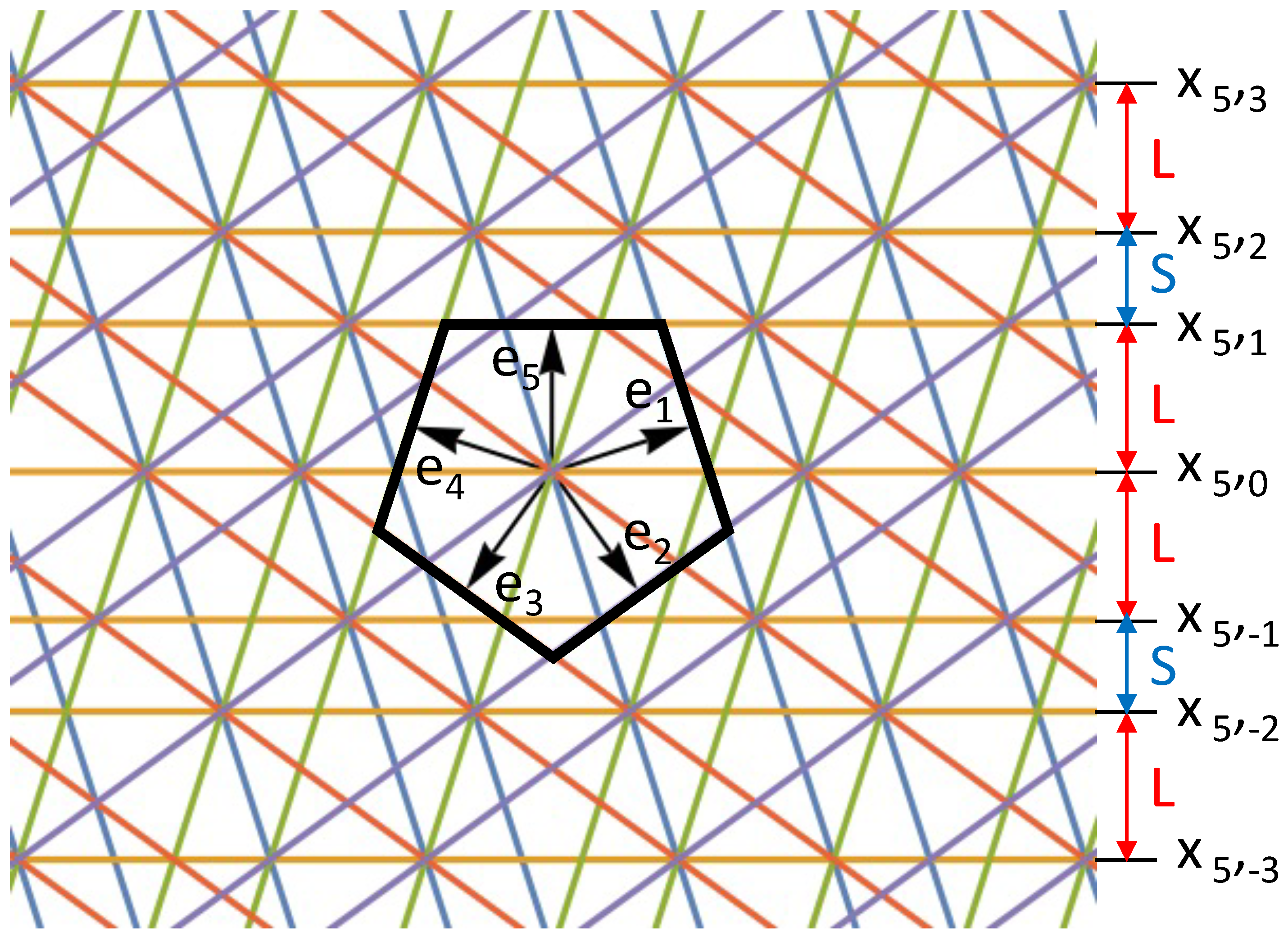
3.2. Fibonacci Icosagrid (FIG) and Its 1/5th, Fibonacci Tetragrid (FTG)
3.3. The Possible Mapping of the FIG to the Lattice and the Golden Twisting
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| FPG | Fibonacci pentagrid quasicrystal |
| FIG | Fibonacci icosagrid quasicrystal |
| FTG | Fibonacci tetragrid quasicrystal |
| ESQC | Elser–Sloane quasicrystal |
| C5C | compound of five cuboctahedra |
| 20G | 20-group of tetrahedra, chirally twisted, with one shared vertex |
| 4G | 4-group of tetrahedrally arranged regular tetrahedra, with one shared vertex |
References
- Shechtman, D.; Blech, I.; Gratias, D.; Cahn, J.W. Metallic phase with long-range orientational order and no translational symmetry. Phys. Rev. Lett. 1984, 53, 1951. [Google Scholar] [CrossRef]
- Steinhardt, P.J. The Second Kind of Impossible; Harvard Science Book Talk; Simon & Schuster: New York, NY, USA, 2019. [Google Scholar]
- Baake, M.; Grimm, U. Aperiodic Order; Cambrage University Press: Cambridge, UK, 2013. [Google Scholar]
- Steinhardt, P.J.; Östlund, S. Physics of Quasicrystals; World Scientific: Singapore; Teaneck, NJ, USA, 1987. [Google Scholar]
- Senechal, M.L. Quasicrystals and Geometry; Cambrige University Press: Cambridge, UK, 1995. [Google Scholar]
- Rivier, N. A botanical quasicrystal. J. Phys. Colloq. 1986, 47, C3-299–C3-309. [Google Scholar] [CrossRef]
- Miekisz, J.; Radin, C. The unstable chemical structure of quasicrystalline alloys. Phys. Lett. A 1986, 119, 133–134. [Google Scholar] [CrossRef]
- Levine, D.; Steinhardt, P.J. Quasicrystals. I. Definition and structure. Phys. Rev. B 1986, 34, 596. [Google Scholar] [CrossRef] [PubMed]
- Henley, C.L. Cell geometry for cluster-based quasicrystal models. Phys. Rev. B 1991, 43, 993. [Google Scholar] [CrossRef] [PubMed]
- Moody, R. (Ed.) The Mathematics of Long-Range Aperiodic Order; Springer: Netherlands, The Netherlands, 1997; Volume 489. [Google Scholar]
- Patera, J. (Ed.) Quasicrystals and Discrete Geometry; American Mathematical Soc.: Toronto, ON, Canada, 1998; Volume 10. [Google Scholar]
- Connes, A. Non-Commutative Geometry; Springer: Berlin/Heidelberg, Germany, 1988. [Google Scholar]
- Socolar, J.E.; Lubensky, T.; Steinhardt, P.J. Phonons, phasons, and dislocations in quasicrystals. Phys. Rev. B 1986, 34, 3345. [Google Scholar] [CrossRef]
- Poon, S. Electronic properties of quasicrystals an experimental review. Adv. Phys. 1992, 41, 303–363. [Google Scholar] [CrossRef]
- Bogomolov, V.N.; Gaponenko, S.V.; Germanenko, I.N.; Kapitonov, A.M.; Petrov, E.P.; Gaponenko, N.V.; Prokofiev, A.V.; Ponyavina, A.N.; Silvanovich, N.I.; Samoilovich, S.M. Photonic band gap phenomenon and optical properties of artificial opals. Phys. Rev. E 1997, 55, 7619. [Google Scholar] [CrossRef]
- Bindi, L.; Kolb, W.; Eby, G.N.; Asimow, P.D.; Wallace, T.C.; Steinhardt, P.J. Accidental synthesis of a previously unknown quasicrystal in the first atomic bomb test. Proc. Natl. Acad. Sci. USA 2021, 118, e2101350118. [Google Scholar] [CrossRef]
- Bindi, L.; Dmitrienko, V.E.; Steinhardt, P.J. Are quasicrystals really so rare in the Universe? Am. Mineral. 2020, 105, 1121–1125. [Google Scholar] [CrossRef]
- Fisher, I.; Islam, Z.; Panchula, A.; Cheon, K.; Kramer, M.; Canfield, P.; Goldman, A. Growth of large-grain R-Mg-Zn quasicrystals from the ternary melt (R= Y, Er, Ho, Dy and Tb). Philos. Mag. B 1998, 77, 1601–1615. [Google Scholar] [CrossRef]
- Nagao, K.; Inuzuka, T.; Nishimoto, K.; Edagawa, K. Experimental Observation of Quasicrystal Growth. Phys. Rev. Lett. 2015, 115, 075501. [Google Scholar] [CrossRef]
- Han, I.; Wang, K.L.; Cadotte, A.T.; Xi, Z.; Parsamehr, H.; Xiao, X.; Glotzer, S.C.; Shahani, A.J. Formation of a single quasicrystal upon collision of multiple grains. Nat. Commun. 2021, 12, 5790. [Google Scholar] [CrossRef]
- Lifshitz, R. The definition of quasicrystals. arXiv 2000, arXiv:cond-mat/0008152. [Google Scholar]
- Else, D.V.; Huang, S.J.; Prem, A.; Gromov, A. Quantum many-body topology of quasicrystals. arXiv 2021, arXiv:2103.13393. [Google Scholar] [CrossRef]
- Baggioli, M. Homogeneous Holographic Viscoelastic Models & Quasicrystals. Phys. Rev. Res. 2020, 2, 022022. [Google Scholar] [CrossRef]
- Baggioli, M.; Landry, M. Effective Field Theory for Quasicrystals and Phasons Dynamics. arXiv 2020, arXiv:2008.05339. [Google Scholar] [CrossRef]
- Amaral, M.; Fang, F.; Hammock, D.; Irwin, K. Geometric State Sum Models from Quasicrystals. Phys. Sci. 2021, 1, 155–168. [Google Scholar] [CrossRef]
- Amaral, M.; Chester, D.; Fang, F.; Irwin, K. Exploiting Anyonic Behavior of Quasicrystals for Topological Quantum Computing. Symmetry 2022, 14, 1780. [Google Scholar] [CrossRef]
- Socolar, J.E.; Steinhardt, P.J. Quasicrystals. II. Unit-cell configurations. Phys. Rev. B 1986, 34, 617. [Google Scholar] [CrossRef]
- Bruijn, N.D. Algebraic theory of Penrose’s non-periodic tilings of the plane. Indag. Math. Proc. 1981, 84, 39–66. [Google Scholar] [CrossRef]
- Elser, V.; Sloane, N.J.A. A highly symmetric four-dimensional quasicrystal. J. Phys. A 1987, 20, 6161–6168. [Google Scholar] [CrossRef]
- Fang, F.; Irwin, K.; Kovacs, J.; Sadler, G. Cabinet of Curiosities: The Interesting Geometry of the Angleβ = arccos ((3ϕ − 1)/4). Fractal Fract. 2019, 3, 48. [Google Scholar] [CrossRef]
- Niu, K.; Fang, M.; Ren, X.; Huang, Z.; Ren, H.; Wu, X.; Sha, W.E.I. Linear and nonlinear spin-orbital coupling in golden-angle spiral quasicrystals. Opt. Express 2020, 28, 334–344. [Google Scholar] [CrossRef]
- Fang, F.; Clawson, R.; Irwin, K. Closing Gaps in Geometrically Frustrated Symmetric Clusters: Local Equivalence between Discrete Curvature and Twist Transformations. Mathematics 2018, 6, 89. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fang, F.; Irwin, K. From the Fibonacci Icosagrid to E8 (Part I): The Fibonacci Icosagrid, an H3 Quasicrystal. Crystals 2024, 14, 152. https://doi.org/10.3390/cryst14020152
Fang F, Irwin K. From the Fibonacci Icosagrid to E8 (Part I): The Fibonacci Icosagrid, an H3 Quasicrystal. Crystals. 2024; 14(2):152. https://doi.org/10.3390/cryst14020152
Chicago/Turabian StyleFang, Fang, and Klee Irwin. 2024. "From the Fibonacci Icosagrid to E8 (Part I): The Fibonacci Icosagrid, an H3 Quasicrystal" Crystals 14, no. 2: 152. https://doi.org/10.3390/cryst14020152
APA StyleFang, F., & Irwin, K. (2024). From the Fibonacci Icosagrid to E8 (Part I): The Fibonacci Icosagrid, an H3 Quasicrystal. Crystals, 14(2), 152. https://doi.org/10.3390/cryst14020152





