Phononic Band Structure by Calculating Effective Parameters of One-Dimensional Metamaterials
Abstract
:1. Introduction
2. Homogenization Theoretical Formalism
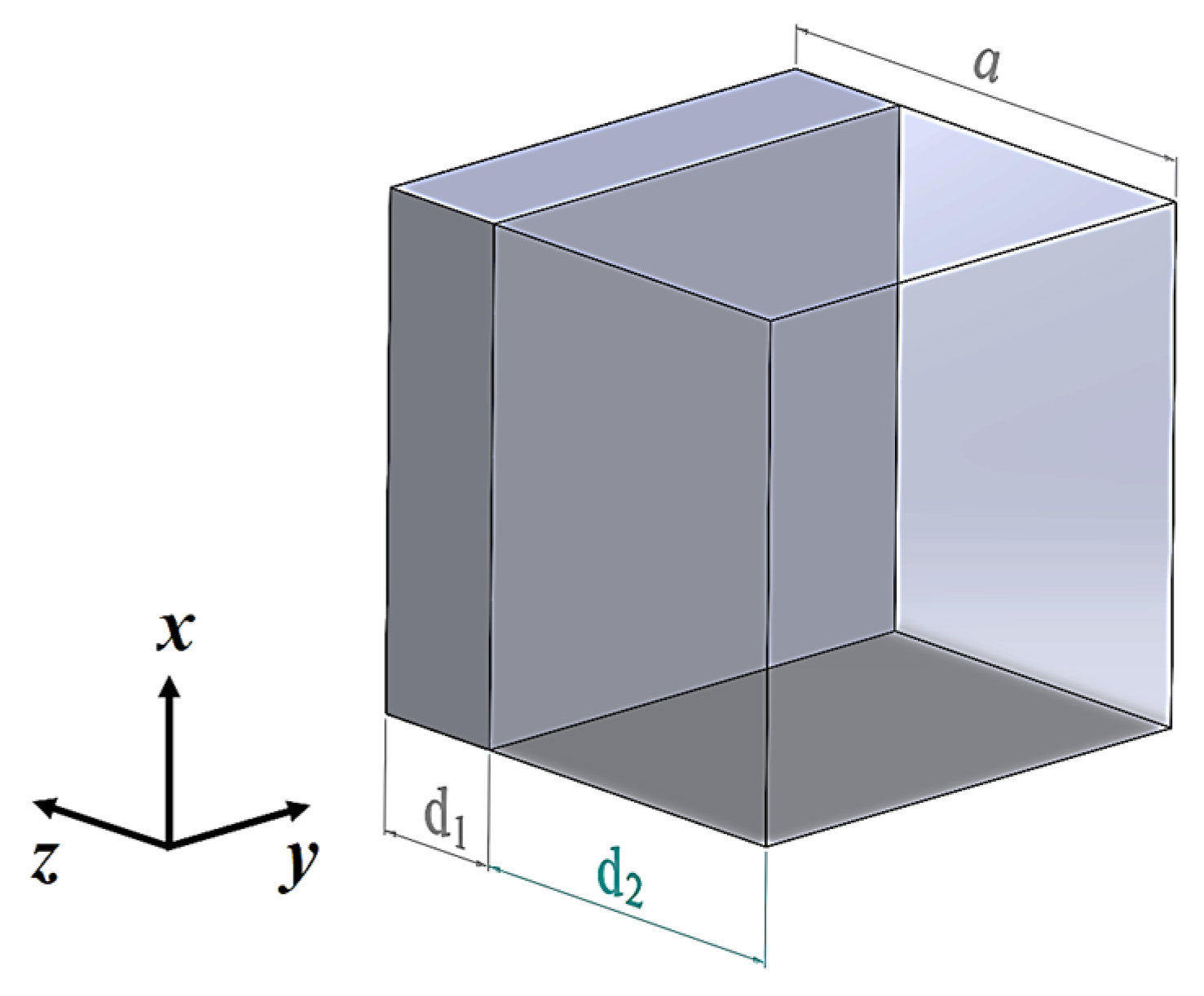
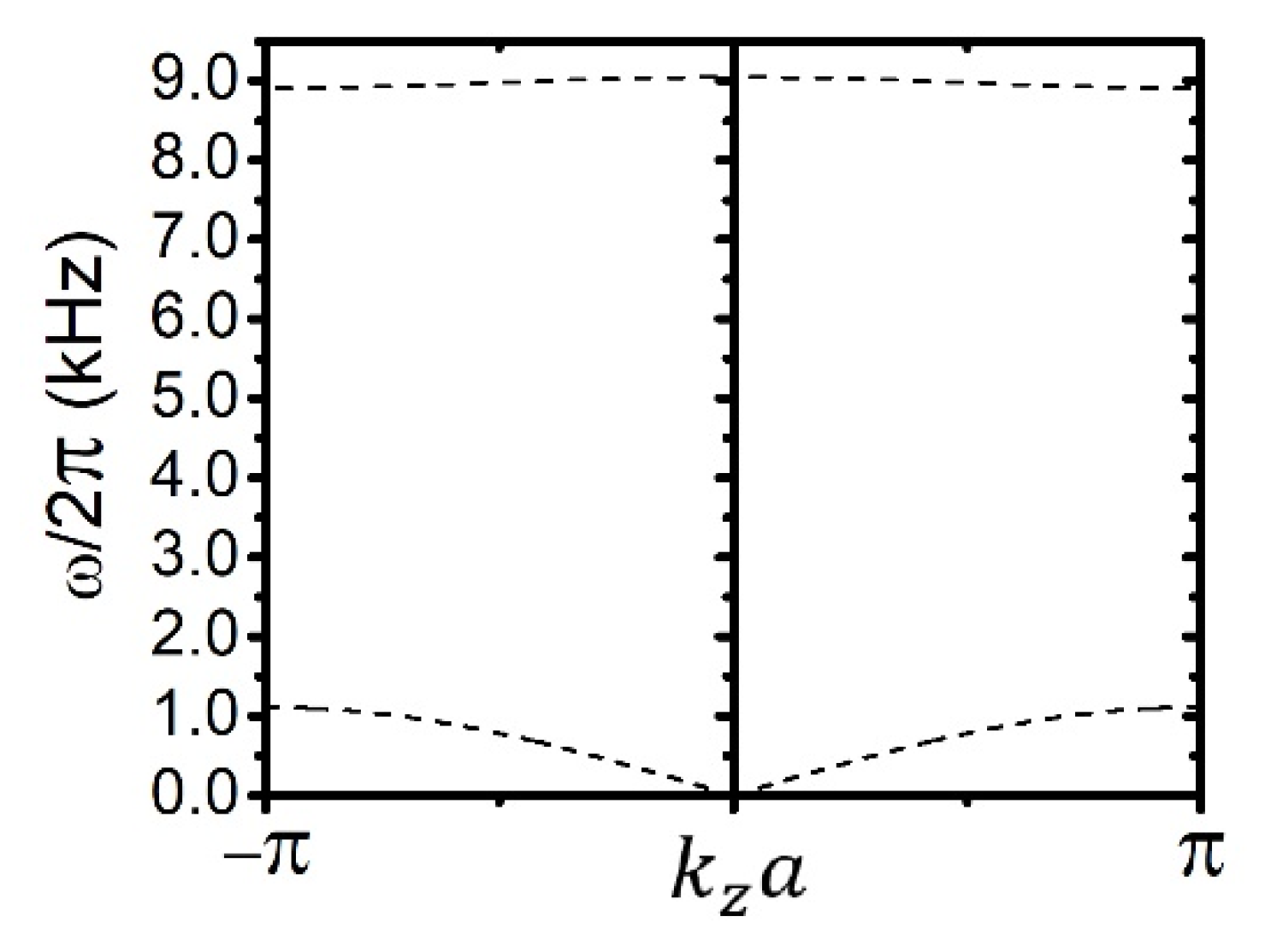
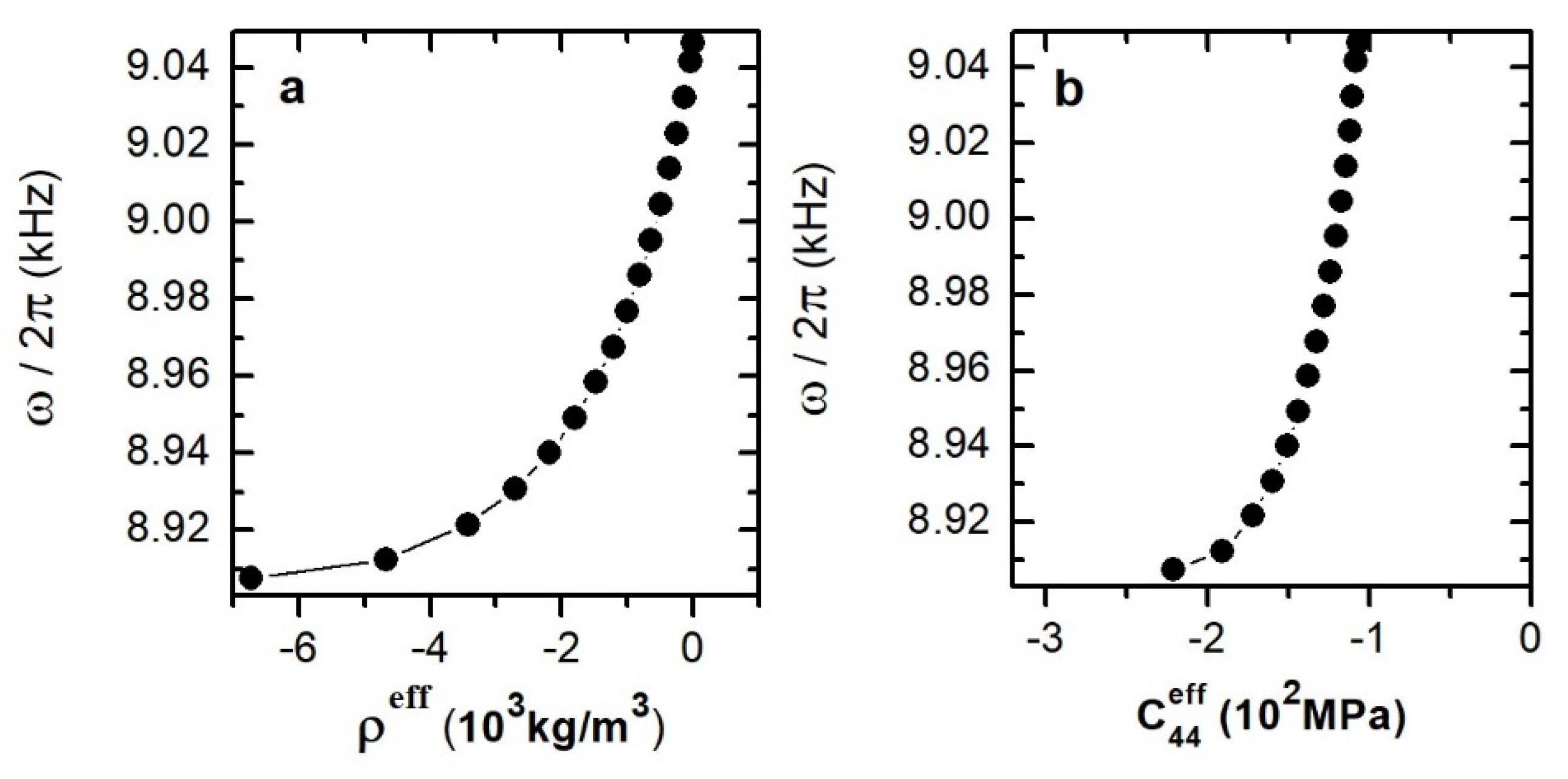
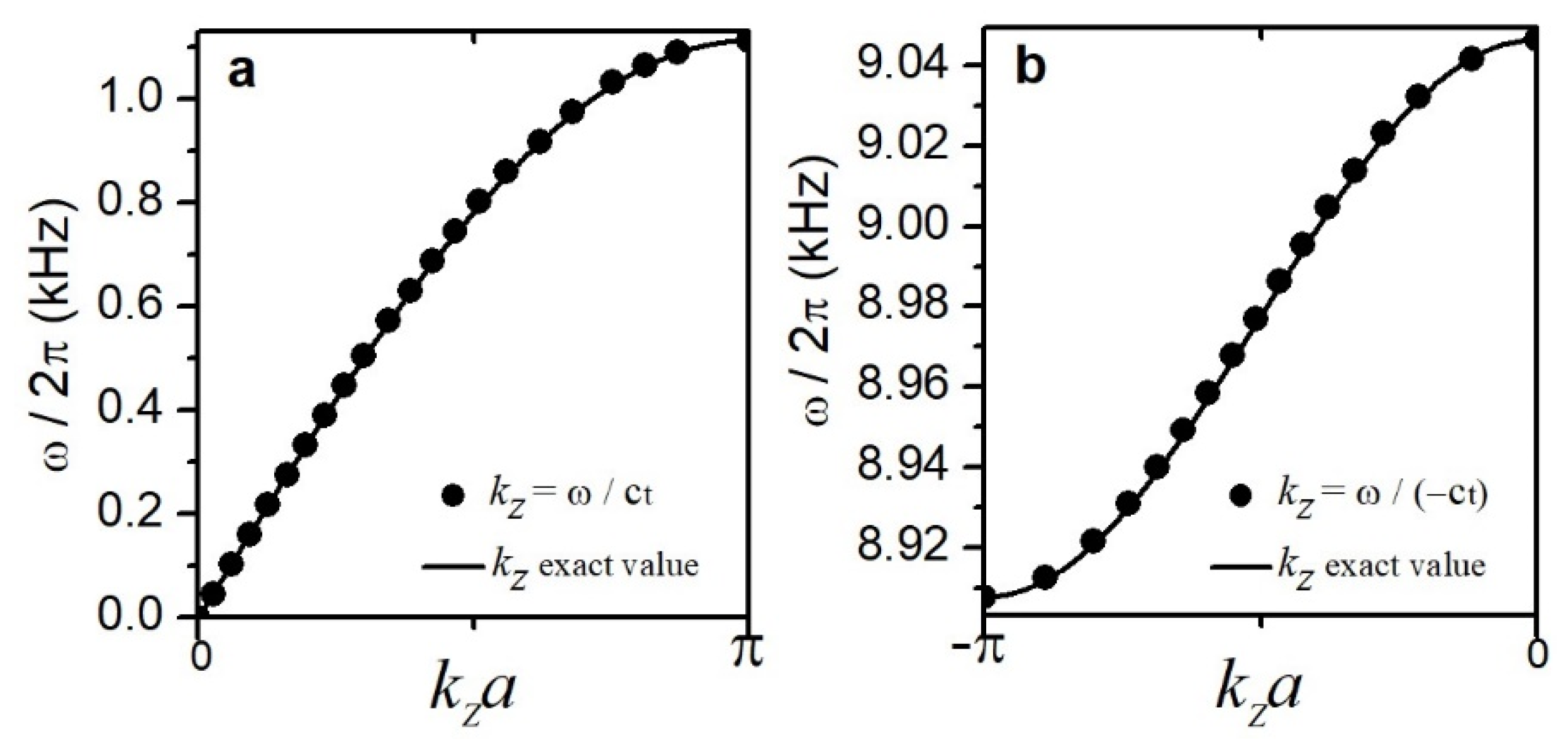
3. Numerical Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ni, Q.; Cheng, J. Anisotropy of effective velocity for elastic wave propagation in two-dimensional phononic crystals at low frequencies. Phys. Rev. B 2005, 72, 014305. [Google Scholar] [CrossRef]
- Khelif, A.; Adibi, A. Phononic Crystals: Fundamentals and Applications, 1st ed.; Springer: New York, NY, USA, 2016; pp. 1–22. [Google Scholar]
- Gumen, L.N.; Arriaga, J.; Krokhin, A.A. Metafluid with anisotropic dynamic mass. Low Temp. Phys. 2011, 37, 975–978. [Google Scholar] [CrossRef] [Green Version]
- Kushwaha, M.S.; Halevi, P.; Dobrzynski, L.; Djafari-Rouhani, B. Acoustic band structure of periodic elastic composites. Phys. Rev. Lett. 1993, 71, 2022–2025. [Google Scholar] [CrossRef] [PubMed]
- Sigalas, M.; Economou, E. Elastic and acoustic wave band structure. J. Sound Vib. 1992, 158, 377–382. [Google Scholar] [CrossRef]
- Li, Y.F.; Huang, X.; Meng, F.; Zhou, S. Evolutionary topological design for phononic band gap crystals. Struct. Multidisc. Optim. 2016, 54, 595–617. [Google Scholar] [CrossRef]
- Li, Y.F.; Meng, F.; Li, S.; Jia, B.; Zhou, S.; Huang, X. Designing broad phononic band gaps for in-plane modes. Phys. Lett. A 2018, 382, 679–684. [Google Scholar] [CrossRef]
- Aravantinos-Zafiris, N.; Lucklum, F.; Sigalas, M.M. Complete phononic band gaps in the 3D Yablonovite structure with spheres. Ultrasonics 2021, 110, 106265. [Google Scholar] [CrossRef]
- Liu, J.; Guo, H.; Wang, T. A Review of Acoustic Metamaterials and Phononic Crystals. Crystals 2020, 10, 305. [Google Scholar] [CrossRef] [Green Version]
- Holliman, J.E., Jr.; Schaef, H.T.; McGrail, B.P.; Miller, Q.R. Review of foundational concepts and emerging directions in metamaterial research: Design, phenomena, and applications. Mater. Adv. 2022, 3, 8390–8406. [Google Scholar] [CrossRef]
- Ren, X.; Das, R.; Tran, P.; Ngo, T.D.; Xie, Y.M. Auxetic metamaterials and structures: A review. Smart Mater. Struct. 2018, 27, 023001. [Google Scholar] [CrossRef]
- Han, D.; Ren, X.; Zhang, Y.; Zhang, X.Y.; Zhang, X.G.; Luo, C.; Xie, Y.M. Lightweight auxetic metamaterials: Design and characteristic study. Compos. Struct. 2022, 293, 115706. [Google Scholar] [CrossRef]
- Lakes, R.S. Foam structures with a negative Poisson’s ratio. Science 1987, 235, 1038–1040. [Google Scholar] [CrossRef] [PubMed]
- Rothenburg, L.; Berlin, A.I.; Bathurst, R.J. Microstructure of isotropic materials with negative Poisson’s ratio. Nature 1991, 354, 470–472. [Google Scholar] [CrossRef]
- Grima, J.N.; Evans, K.E. Auxetic behavior from rotating squares. J. Mater. Sci. Lett. 2000, 19, 1563–1565. [Google Scholar] [CrossRef]
- Brillouin, L. Wave Propagation in Periodic Structures: Electric Filters and Crystal Lattices, 1st ed.; McGraw-Hill Book Company Inc.: New York, NY, USA, 1953. [Google Scholar]
- Li, N.; Ren, J.; Wang, L.; Zhang, G.; Hänggi, P.; Li, B. Colloquium: Phononics: Manipulating heat flow with electronic analogs and beyond. Rev. Modern Phys. 2012, 84, 1045–1066. [Google Scholar] [CrossRef] [Green Version]
- Chen, A.-L.; Li, Z.-Y.; Ma, T.-X.; Li, X.-S.; Wang, Y.-S. Heat reduction by thermal wave crystals. Int. J. Heat Mass Transf. 2018, 121, 215–222. [Google Scholar] [CrossRef] [Green Version]
- Yeung, W.K.; Lam, T.T. A numerical scheme for non-Fourier heat conduction Part I: One-dimensional problem formulation and applications. Numer. Heat Transf. Part B Fund. 1998, 33, 215–233. [Google Scholar] [CrossRef]
- Lam, T.T.; Yeung, W.K. A numerical scheme for non-Fourier heat conduction, Part II: Two-dimensional problem formulation and verification. Numer. Heat Transf. Part B Fund. 2002, 41, 543–564. [Google Scholar] [CrossRef]
- Zhang, M.K.; Cao, B.Y.; Guo, Y.C. Numerical studies on dispersion of thermal waves. Int. J. Heat Mass Transf. 2013, 67, 1072–1082. [Google Scholar] [CrossRef]
- Chen, T.; Weng, C.-N.; Chen, J.-S. Cloak for curvilinearly anisotropic media in conduction. Appl. Phys. Lett. 2008, 93, 114103. [Google Scholar] [CrossRef]
- Fan, C.Z.; Gao, Y.; Huang, J.P. Shaped graded materials with an apparent negative thermal conductivity. Appl. Phys. Lett. 2008, 92, 251907. [Google Scholar] [CrossRef]
- Kuon, I.; Ohtaka, K. Photonic Crystals: Physics, Fabrication and Applications, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Yablonovitch, E. Inhibited spontaneous emission in solid-state physics and electronics. Phys. Rev. Lett. 1987, 58, 2059–2062. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- John, S. Strong localization of photons in certain disordered dielectric superlattices. Phys. Rev. Lett. 1987, 58, 2486–2489. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kuramochi, E. Manipulating and trapping light with photonic crystals from fundamental studies to practical applications. J. Mater. Chem. C 2016, 4, 11032–11049. [Google Scholar] [CrossRef]
- Milton, G.W.; Briane, M.; Willis, J.R. On cloaking for elasticity and physical equations with a transformation invariant form. New J. Phys. 2006, 8, 248. [Google Scholar] [CrossRef]
- Farhat, M.; Guenneau, S.; Enoch, S. Broadband cloaking of bending waves via homogenization of multiply perforated radially symmetric and isotropic thin elastic plates. Phys. Rev. B Condens. Matter Mater. Phys. 2012, 85, 020301. [Google Scholar] [CrossRef]
- Restrepo-Florez, J.M.; Maldovan, M. Mass Separation by Metamaterials. Sci. Rep. 2016, 6, 21971. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Restrepo-Florez, J.M.; Maldovan, M. Rational design of mass diffusion metamaterial concentrators based on coordinate transformation. J. Appl. Phys. 2016, 120, 084902. [Google Scholar] [CrossRef]
- Jo, S.-H.; Youn, B.D. A Phononic Crystal with Differently Configured Double Defects for Broadband Elastic Wave Energy Localization and Harvesting. Crystals 2021, 11, 643. [Google Scholar] [CrossRef]
- Chen, Y.; Li, G.; Sun, R.; Chen, G. Wave Dispersion in One-Dimensional Nonlinear Local Resonance Phononic Crystals with Perturbation Method. Crystals 2021, 11, 774. [Google Scholar] [CrossRef]
- Chen, L.; Liu, Y.; Kong, H. Acoustic Tunneling Study for Hexachiral Phononic Crystals Based on Dirac-Cone Dispersion Properties. Crystals 2021, 11, 1577. [Google Scholar] [CrossRef]
- Sigalas, M.; Economou, E. Band structure of elastic waves in two dimensional systems. Solid State Commun. 1993, 86, 141–143. [Google Scholar] [CrossRef]
- Hussein, M.I.; Leamy, M.J.; Ruzzene, M. Dynamics of phononic materials and structures: Historical origins, recent progress, and future outlook. Appl. Mech. Rev. 2014, 66, 38. [Google Scholar] [CrossRef]
- Laude, V. Phononic Crystals: Artificial Crystals for Sonic, Acoustic, and Elastic Waves; Walter de Gruyter GmbH & Co KG Llc.: Berlin/Heidelberg, Germany, 2015; Volume 26. [Google Scholar]
- Veselago, V.G. The electrodynamics of substances with simultaneously negative values of ε and μ. Sov. Phys. Usp. 1968, 10, 509. [Google Scholar] [CrossRef]
- Li, J.; Chan, C.T. Double-negative acoustic metamaterial. Phys. Rev. B 2004, 70, 055602(R). [Google Scholar] [CrossRef] [Green Version]
- Dong, H.-W.; Zhao, S.-D.; Wang, Y.-S.; Zhang, C. Topology optimization of anisotropic broadband double-negative elastic metamaterials. J. Mech. Phys. Solids 2017, 105, 54–80. [Google Scholar] [CrossRef] [Green Version]
- Wu, Y.; Lai, Y.; Zhang, Z.-Q. Effective medium theory for elastic metamaterials in two dimensions. Phys. Rev. B 2007, 76, 205313. [Google Scholar] [CrossRef] [Green Version]
- Zhou, X.; Hu, G. Analytic model of elastic metamaterials with local resonances. Phys. Rev. B 2009, 79, 195109. [Google Scholar] [CrossRef] [Green Version]
- Wu, Y.; Lai, Y.; Zhang, Z.-Q. Elastic Metamaterials with Simultaneously Negative Effective Shear Modulus and Mass Density. Phys. Rev. Lett. 2011, 107, 105506. [Google Scholar] [CrossRef] [Green Version]
- Lai, Y.; Wu, Y.; Sheng, P.; Zhang, Z.-Q. Hybrid elastic solids. Nat. Mater 2011, 10, 620–624. [Google Scholar] [CrossRef]
- Flores Méndez, J.; Pinón Reyes, A.C.; Heredia Jiménez, A.H.; Ambrosio Lázaro, R.C.; Morales-Sánchez, A.; Moreno Moreno, M.; Luna-López, J.A.; Severiano Carrillo, F.; Meraz Melo, M.A. Discretization Approach for the Homogenization of Three-Dimensional Solid-Solid Phononic Crystals in the Quasi-Static Limit: Density and Elastic Moduli. Appl. Sci. 2022, 12, 2987. [Google Scholar] [CrossRef]
- Zhang, S.; Xia, C.G.; Fang, N. Broadband Acoustic Cloak for Ultrasound Waves. Phys. Rev. Lett. 2011, 106, 024301. [Google Scholar] [CrossRef] [PubMed]
- Popa, B.I.; Zigoneanu, L.; Cummer, S.A. Experimental Acoustic Ground Cloak in Air. Phys. Rev. Lett. 2011, 106, 253901. [Google Scholar] [CrossRef] [PubMed]
- Zigoneanu, L.; Popa, B.I.; Cummer, S.A. Three-dimensional broadband omnidirectional acoustic ground cloak. Nat. Mater. 2014, 13, 352–355. [Google Scholar] [CrossRef] [Green Version]
- Bi, Y.; Jia, H.; Sun, Z.; Yang, Y.; Zhao, H.; Yang, J. Experimental demonstration of three-dimensional broadband underwater acoustic carpet cloak. Appl. Phys. Lett. 2018, 112, 223502. [Google Scholar] [CrossRef] [Green Version]
- Esfahlani, H.; Karkar, S.; Lissek, H.; Mosig, J.R. Acoustic carpet cloak based on an ultrathin metasurface. Phys. Rev. B 2016, 94, 014302. [Google Scholar] [CrossRef] [Green Version]
- Park, J.J.; Park, C.M.; Lee, K.J.B.; Lee, S.H. Acoustic superlens using membrane-based metamaterials. Appl. Phys. Lett. 2015, 106, 051901. [Google Scholar] [CrossRef]
- Yang, X.; Yin, J.; Yu, G.; Peng, L.; Wang, N. Acoustic superlens using Helmholtz-resonator-based metamaterials. Appl. Phys. Lett. 2015, 107, 193505. [Google Scholar] [CrossRef]
- Kaina, N.; Lemoult, F.; Fink, M.; Lerosey, G. Negative refractive index and acoustic superlens from multiple scattering in single negative metamaterials. Nature 2015, 525, 77–81. [Google Scholar] [CrossRef]
- Park, C.M.; Lee, S.H. Zero-reflection acoustic metamaterial with a negative refractive index. Sci. Rep. 2019, 9, 3372. [Google Scholar] [CrossRef] [Green Version]
- Ding, Y.; Liu, Z.; Qiu, C.; Shi, J. Metamaterial with Simultaneously Negative Bulk Modulus and Mass Density. Phys. Rev. Lett. 2007, 99, 093904. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Zhang, X.; Mao, Y.; Zhu, Y.Y.; Yang, Z.; Chan, C.T.; Sheng, P. Locally Resonant Sonic Materials. Science 2000, 289, 1734. [Google Scholar] [CrossRef] [PubMed]
- Kittel, C. Introduction to Solid State Physics, 8th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2005. [Google Scholar]
- Ciesielski, A. An Introduction to Rubber Technology, 1st ed.; RAPRA Technology: Shrewsbury, UK, 1999. [Google Scholar]
- Deymier, P.A. Acoustic Metamaterials and Phononic Crystals, 1st ed.; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Kushwaha, M.S. Classical band structure of periodic elastic composites. Int. J. Mod. Phys. B 1996, 10, 977–1094. [Google Scholar] [CrossRef]
- Rytov, S.M. Acoustic properties of a thinly laminated medium. Sov. Phys. Acoust. 1956, 2, 68. [Google Scholar]
- Lee, S.H.; Park, C.M.; Seo, Y.M.; Wang, Z.G.; Kim, C.K. Composite acoustic medium with simultaneously negative density and modulus. Phys. Rev. Lett. 2010, 104, 054301. [Google Scholar] [CrossRef]
- Liu, X.N.; Hu, G.K.; Huang, G.L.; Sun, C.T. An elastic metamaterial with simultaneously negative mass density and bulk modulus. Appl. Phys. Lett. 2011, 98, 251907. [Google Scholar] [CrossRef] [Green Version]
- Liang, Z.; Feng, T.; Lok, S.; Liu, F.; Ng, K. Space-coiling metamaterials with double negativity and conical dispersion. Sci. Rep. 2013, 3, 1614. [Google Scholar] [CrossRef] [Green Version]
- Xie, Y.; Popa, B.I.; Zigoneanu, L.; Cummer, S.A. Measurement of a broadband negative index with space-coiling acoustic metamaterials. Phys. Rev. Lett. 2013, 110, 175501. [Google Scholar] [CrossRef] [Green Version]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Flores Méndez, J.; Heredia Jiménez, A.H.; Minquiz, G.M.; Morales-Sánchez, A.; Moreno, M.; Luna López, J.A.; Severiano, F.; Piñón Reyes, A.C. Phononic Band Structure by Calculating Effective Parameters of One-Dimensional Metamaterials. Crystals 2023, 13, 931. https://doi.org/10.3390/cryst13060931
Flores Méndez J, Heredia Jiménez AH, Minquiz GM, Morales-Sánchez A, Moreno M, Luna López JA, Severiano F, Piñón Reyes AC. Phononic Band Structure by Calculating Effective Parameters of One-Dimensional Metamaterials. Crystals. 2023; 13(6):931. https://doi.org/10.3390/cryst13060931
Chicago/Turabian StyleFlores Méndez, Javier, Aurelio H. Heredia Jiménez, Gustavo M. Minquiz, A. Morales-Sánchez, Mario Moreno, José Alberto Luna López, Francisco Severiano, and A. C. Piñón Reyes. 2023. "Phononic Band Structure by Calculating Effective Parameters of One-Dimensional Metamaterials" Crystals 13, no. 6: 931. https://doi.org/10.3390/cryst13060931
APA StyleFlores Méndez, J., Heredia Jiménez, A. H., Minquiz, G. M., Morales-Sánchez, A., Moreno, M., Luna López, J. A., Severiano, F., & Piñón Reyes, A. C. (2023). Phononic Band Structure by Calculating Effective Parameters of One-Dimensional Metamaterials. Crystals, 13(6), 931. https://doi.org/10.3390/cryst13060931







