Modelling the Structure and Optical Properties of Reduced Graphene Oxide Produced by Laser Ablation: Insights from XPS and Time-Dependent DFT
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Geim, A.K. Graphene: Status and prospects. Science 2009, 324, 1530–1534. [Google Scholar] [CrossRef]
- Bonaccorso, F.; Sun, Z.; Hasan, T.; Ferrari, A.C. Graphene photonics and optoelectronics. Nat. Photon. 2010, 4, 611–622. [Google Scholar] [CrossRef]
- Mueller, T.; Xia, F.; Avouris, P. Graphene photodetectors for high-speed optical communications. Nat. Photon. 2010, 4, 297–301. [Google Scholar] [CrossRef]
- Mahakul, P.C.; Subramaniam, B.V.R.S.; Mahanandia, P. Mesoscopic investigation of the effect of MWCNT/rGO network on the performance of P3HT:PC60BM solar cells. Mater. Chem. Phys. 2019, 226, 113–117. [Google Scholar] [CrossRef]
- Liu, R.; Qiu, R.; Zou, T.; Liu, C.; Chen, J.; Dai, Q.; Zhang, S.; Zhou, H. SnO2-rGO nanocomposite as an efficient electron transport layer for stable perovskite solar cells on AZO substrate. Nanotechnology 2018, 30, 075202. [Google Scholar] [CrossRef]
- Jiang., X.; Wang, Z.; Han, W.; Liu, Q.; Lu, S.; Wen, Y.; Hou, J.; Huang, F.; Peng, S.; He, D.; et al. High performance silicon–organic hybrid solar cells via improving conductivity of PEDOT:PSS with reduced graphene oxide. Appl. Surf. Sci. 2017, 407, 398–404. [Google Scholar] [CrossRef]
- Gopi, C.V.V.M.; Singh, S.; Reddy, A.E.; Kim, H.J. CNT@rGO@MoCuSe composite as an efficient counter electrode for quantum dot-sensitized solar cells. ACS Appl. Mater. Interfaces 2018, 10, 10036–10042. [Google Scholar] [CrossRef]
- Liu, J.; Xu, M.; Wang, B.; Zhou, Z.; Wang, L. Fluorescence sensor for detecting protamines based on competitive interactions of polyacrylic acid modified with sodium 4-amino-1-naphthalenesulfonate with protamines and aminated graphene oxide. RSC Adv. 2017, 7, 1432–1438. [Google Scholar] [CrossRef]
- Li, Z.; Gadipelli, S.; Yang, Y.; Guo, Z. Design of 3D Graphene-Oxide Spheres and Their Derived Hierarchical Porous Structures for High Performance Supercapacitors. Small 2017, 13, 1702474. [Google Scholar] [CrossRef]
- Dideikin, A.T.; Vul’, A.Y. Graphene oxide and derivatives: The place in graphene family. Front. Phys. 2019, 6, 149. [Google Scholar] [CrossRef]
- Tarcan, R.; Todor-Boer, O.; Petrovai, I.; Leordean, C.; Astilean, S.; Botiz, I. Reduced graphene oxide today. J. Mater. Chem. C 2020, 8, 1198–1224. [Google Scholar] [CrossRef]
- Bacon, M.; Bradley, S.J.; Nann, T. Graphene quantum dots. Part. Part. Syst. Charact. 2014, 31, 415–428. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Fal′ko, V.I.; Colombo, L.; Gellert, P.R.; Schwab, M.G.; Kim, K. A roadmap for graphene. Nature 2012, 490, 192–200. [Google Scholar] [CrossRef]
- Kakavelakis, G.; Maksudov, T.; Konios, D.; Paradisanos, I.; Kioseoglou, G.; Stratakis, E.; Kymakis, E. Efficient and highly air stable planar inverted perovskite solar cells with reduced graphene oxide doped PCBM electron transporting layer. Adv. Energy Mater. 2017, 7, 1602120. [Google Scholar] [CrossRef]
- Naderi, H.R.; Sobhani-Nasab, A.; Rahimi-Nasrabadi, M.; Ganjali, M.R. Decoration of nitrogen-doped reduced graphene oxide with cobalt tungstate nanoparticles for use in high-performance supercapacitors. Appl. Surf. Sci. 2017, 423, 1025–1034. [Google Scholar] [CrossRef]
- Yamaguchi, H.; Eda, G.; Mattevi, C.; Kim, H.K.; Chhowalla, M. Highly uniform 300 mm wafer-Scale deposition of single and multilayered chemically derived graphene thin films. ACS Nano 2010, 4, 524–528. [Google Scholar] [CrossRef] [PubMed]
- He, L.; Tjong, S.C. Silver-decorated reduced graphene oxides as novel building blocks for transparent conductive films. RSC Adv. 2017, 7, 2058–2065. [Google Scholar] [CrossRef]
- Wang, X.; Tian, H.; Mohammad, M.A.; Li, C.; Wu, C.; Yang, Y.; Ren, T.L. A spectrally tunable all-graphene-based flexible field-effect light-emitting device. Nat. Commun. 2015, 6, 7767. [Google Scholar] [CrossRef]
- Yu, X.X.; Yin, H.; Li, H.-X.; Zhao, H.; Li, C.; Zhu, M.-Q. A novel high-performance self-powered UV-vis-NIR photodetector based on a CdS nanorod array/reduced graphene oxide film heterojunction and its piezo-phototronic regulation. J. Mater. Chem. C 2018, 6, 630–636. [Google Scholar] [CrossRef]
- Xu, Y.; Zhao, L.; Bai, H.; Hong, W.; Li, C.; Shi, G. Chemically converted graphene induced molecular flattening of 5,10,15,20-Tetrakis(1-methyl-4-pyridinio)porphyrin and its application for optical detection of cadmium(II) ions. J. Am. Chem. Soc. 2009, 131, 13490–13497. [Google Scholar] [CrossRef]
- Siklitskaya, A.; Gacka, E.; Larowska, D.; Mazurkiewicz-Pawlicka, M.; Malolepszy, A.; Stobiński, L.; Marciniak, B.; Lewandowska-Andrałojć, A.; Kubas, A. Lerf–Klinowski-type models of graphene oxide and reduced graphene oxide are robust in analyzing non-covalent functionalization with porphyrins. Sci. Rep. 2021, 11, 7977. [Google Scholar] [CrossRef] [PubMed]
- Kymakis, E.; Petridis, C.; Anthopoulos, T.D.; Stratakis., E. Laser-assisted reduction of graphene oxide for flexible, large-area optoelectronics. IEEE J. Sel. Top. Quant. Electr. 2014, 20, 106–115. [Google Scholar] [CrossRef]
- Bhattacharjya, D.; Kim, C.-H.; Kim, J.-H.; You, I.-K.; In, J.B.; Lee, S.-M. Fast and controllable reduction of graphene oxide by low-cost CO2 laser for supercapacitor application. Appl. Surf. Sci. 2018, 462, 353–361. [Google Scholar] [CrossRef]
- Evlashin, S.; Dyakonov, P.; Khmelnitsky, R.; Dagesyan, S.; Klokov, A.; Sharkov, A.; Timashev, P.; Minaeva, S.; Maslakov, K.; Svyakhovskiy, S.; et al. Controllable Laser Reduction of Graphene Oxide Films for Photoelectronic Applications. ACS Appl. Mater. Interfaces 2016, 8, 28880–28887. [Google Scholar] [CrossRef] [PubMed]
- Rodriguez, R.D.; Khalelov, A.; Postnikov, P.S.; Lipovka, A.; Dorozhko, E.; Amin, I.; Murastov, G.V.; Chen, J.-J.; Sheng, W.; Trusova, M.E.; et al. Beyond graphene oxide: Laser engineering functionalized graphene for flexible electronics. Mater. Horiz. 2020, 7, 1030–1041. [Google Scholar] [CrossRef]
- Zhao, Y.; Han, Q.; Cheng, Z.; Jiang, L.; Qu, L. Integrated graphene systems by laser irradiation for advanced devices. Nano Today 2017, 12, 14–30. [Google Scholar] [CrossRef]
- Donate-Buendia, C.; Torres-Mendieta, R.; Pyatenko, A.; Falomir, E.; Fernández-Alonso, M.; Mínguez-Vega, G. Fabrication by laser irradiation in a continuous flow jet of carbon quantum dots for fluorescence imaging. ACS Omega 2018, 3, 2735–2742. [Google Scholar] [CrossRef]
- Kang, S.; Ryu, J.H.; Lee, B.; Jung, K.H.; Shim, K.B.; Han, H.; Kim., K.M. Laser wavelength modulated pulsed laser ablation for selective and efficient production of graphene quantum dots. RSC Adv. 2019, 9, 13658–13663. [Google Scholar] [CrossRef]
- Ershov, I.V.; Lavrentyev, A.A.; Prutsakova, N.V.; Holodova, O.M.; Mardasova, I.V.; Zhdanova, T.P.; Kozakov, A.T. Characterization of graphenic carbon produced by pulsed laser ablation of sacrificial carbon tapes. Appl. Sci. 2021, 11, 11972. [Google Scholar] [CrossRef]
- Boukhvalov, D.W.; Katsnelson, M.I. Modeling of Graphite Oxide. J. Am. Chem. Soc. 2008, 130, 10697–10701. [Google Scholar] [CrossRef]
- Lahaye, R.J.W.E.; Jeong, H.K.; Park, C.Y.; Lee, Y.H. Density functional theory study of graphite oxide for different oxidation levels. Phys. Rev. B 2009, 79, 125435. [Google Scholar] [CrossRef]
- Lundie, M.; Šljivančanin, Z.; Tomić., S. Electronic and optical properties of reduced graphene oxide. J. Mater. Chem. C 2015, 3, 7632–7641. [Google Scholar] [CrossRef]
- Hernández Rosas, J.J.; Ramírez Gutiérrez, R.E.; Escobedo-Morales, A.; Chigo Anota, E. First principles calculations of the electronic and chemical properties of graphene, graphane, and graphene oxide. J. Mol. Model. 2011, 17, 1133–1139. [Google Scholar] [CrossRef] [PubMed]
- Gacka, E.; Burdzinski, G.; Marciniak, B.; Kubas, A. Interaction of light with a non-covalent zinc porphyrin–graphene oxide nanohybrid. Phys. Chem. Chem. Phys. 2020, 22, 13456–13466. [Google Scholar] [CrossRef] [PubMed]
- Gacka, E.; Wojcik, A.; Mazurkiewicz-Pawlicka, M.; Malolepszy, A.; Stobiński, L.; Kubas, A.; Hug, G.L.; Marciniak, B.; Lewandowska-Andralojc. A. Noncovalent Porphyrin–Graphene Oxide Nanohybrids: The pH-Dependent Behavior. J. Phys. Chem. C 2019, 123, 3368–3380. [Google Scholar] [CrossRef]
- Szabo, T.; Berkesi, O.; Forgo, P.; Josepovits, K.; Sanakis, Y.; Petridis, D.; Dekany, I. Evolution of Surface Functional Groups in a Series of Progressively Oxidized Graphite Oxides. Chem. Mater. 2006, 18, 2740–2749. [Google Scholar] [CrossRef]
- Gao, W.; Alemany, L.B.; Ci, L.; Ajayan, P.M. New insights into the structure and reduction of graphite oxide. Nature Chem. 2009, 1, 403–408. [Google Scholar] [CrossRef]
- Dreyer, D.R.; Todd, A.D.; Bielawski., C.W. Harnessing the chemistry of graphene oxide. Chem. Soc. Rev. 2014, 43, 5288–5301. [Google Scholar] [CrossRef]
- Lerf, A.; He, H.; Forster, M.; Klinowski, J. Structure of graphite oxide revisited. J. Phys. Chem. B 1998, 102, 4477–4482. [Google Scholar] [CrossRef]
- Diez, N.; Sliwak, A.; Gryglewicz, S.; Grzyb, B.; Gryglewicz, G. Enhanced reduction of graphene oxide by high-pressure hydrothermal treatment. RSC Adv. 2015, 5, 81831. [Google Scholar] [CrossRef]
- Zhang, D.; Gokce, B.; Barcikowski, S. Laser Synthesis and Processing of Colloids: Fundamentals and Applications. Chem. Rev. 2017, 117, 3990–4103. [Google Scholar] [CrossRef] [PubMed]
- Giannozzi, P.; Andreussi, O.; Brumme, T.; Bunau, O.; Nardelli, M.B.; Calandra, M.; Car, R.; Cavazzoni, C.; Ceresoli, D.; Cococcioni, M.; et al. Advanced capabilities for materials modelling with Quantum ESPRESSO. J. Phys. Condens. Matter. 2017, 29, 465901. [Google Scholar] [CrossRef] [PubMed]
- Ge, X.; Binnie, S.J.; Rocca, D.; Gebauer, R.; Baroni, S. turboTDDFT 2.0—Hybrid functionals and new algorithms within time-dependent density-functional perturbation theory. Comp. Phys. Comm. 2014, 185, 2080–2089. [Google Scholar] [CrossRef]
- Gruning, M.; Marini, A.; Gonze, X. Implementation and testing of Lanczos-based algorithms for Random-Phase Approximation eigenproblems. Comput. Mater. Sci. 2011, 50, 2148–2156. [Google Scholar] [CrossRef]
- Clar, E. Polycyclic Hydrocarbons; Academic Press and Springer-Verlag: London, UK, 1964; Volume 1–2. [Google Scholar]
- Rieger, R.; Mullen, K. Forever young: Polycyclic aromatic hydrocarbons as model cases for structural and optical studies. J. Phys. Org. Chem. 2010, 23, 315–325. [Google Scholar] [CrossRef]
- Fetzer, J.C. Large (C >= 24) Polycyclic Aromatic Hydrocarbons; John Wiley & Sons: New York, NY, USA, 2000. [Google Scholar]
- Malcioglu, O.B.; Gebauer, R.; Rocca, D.; Baroni, S. turboTDDFT—A code for the simulation of molecular spectra using the Liouville–Lanczos approach to time-dependent density-functional perturbation theory. Comp. Phys. Commun. 2011, 182, 1744–1754. [Google Scholar] [CrossRef]

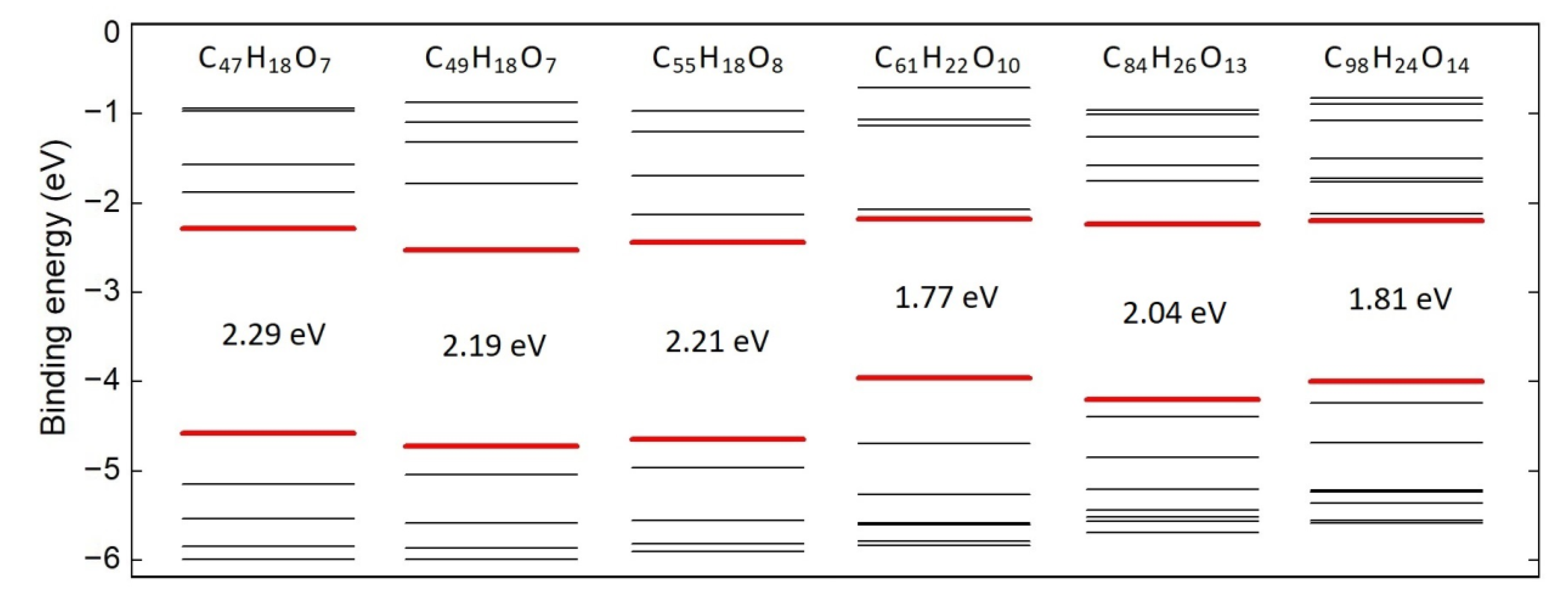
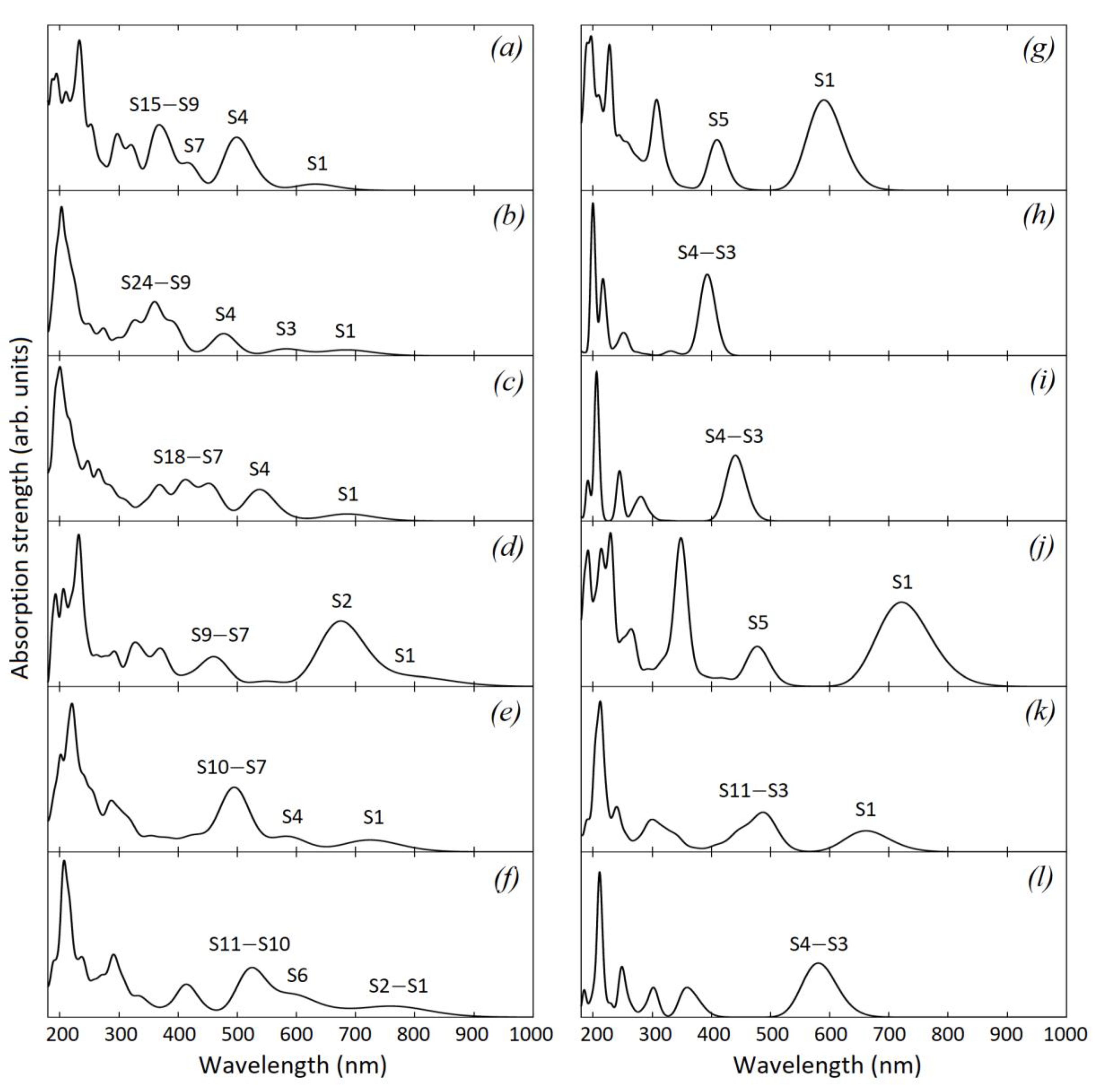
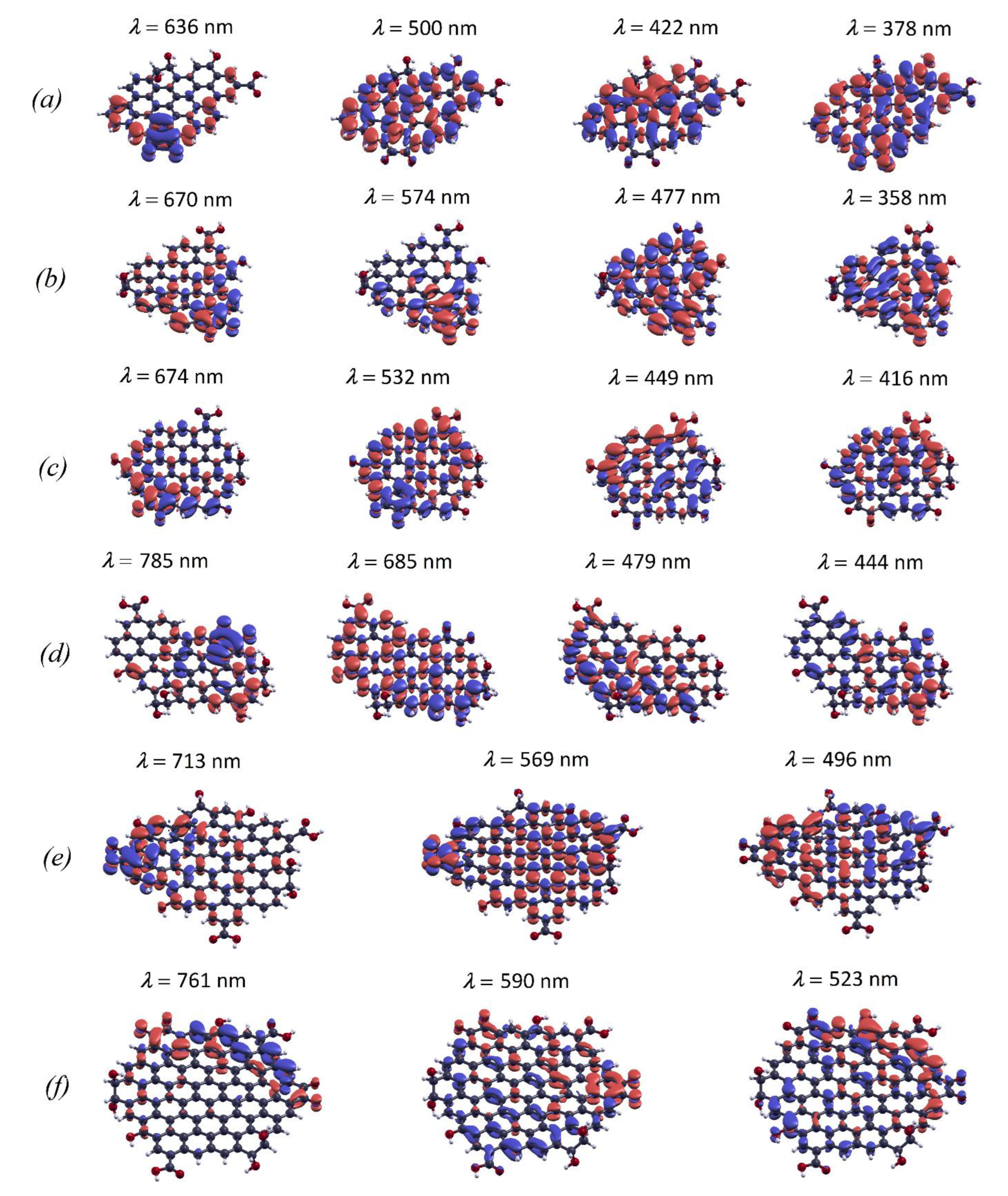
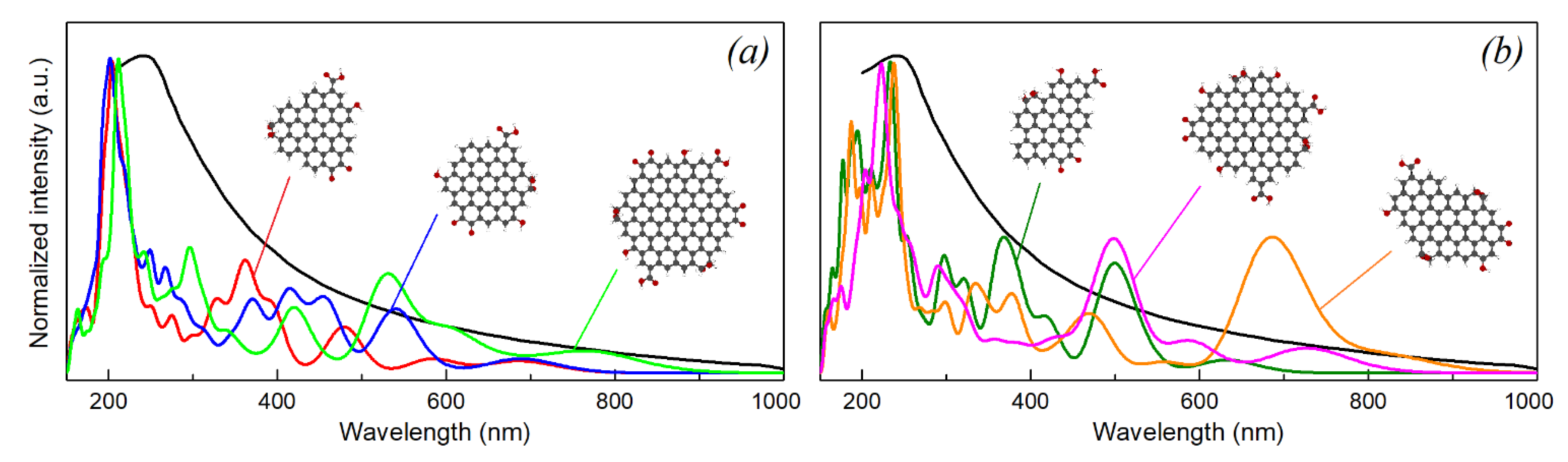
| Compound | S → S Transition | E (eV) | λ (nm) | Σχii (e2a2/eV) | Transition Components |
|---|---|---|---|---|---|
| C47H18O7 | S0 → S1 | 1.95 | 636 | 0.08 | H → L (99%) |
| S0 → S4 | 2.48 | 500 | 0.43 | H → L + 1 (58%) H → L + 2 (18%) | |
| S0 → S7 | 2.94 | 422 | 0.20 | H-1 → L + 1 (53%) H → L + 2 (25%) | |
| S0 → S13 | 3.39 | 366 | 0.29 | H-5 → L (75%) | |
| C49H18O7 | S0 → S1 | 1.85 | 670 | 0.18 | H → L (95%) |
| S0 → S3 | 2.16 | 574 | 0.17 | H-1 → L (92%) | |
| S0 → S4 | 2.60 | 477 | 0.30 | H → L + 1 (72%) | |
| S0 → S9 | 3.20 | 387 | 0.40 | H → L + 2 (40%) H-1 → L + 1 (21%) | |
| S0 → S15 | 3.46 | 358 | 0.17 | H-1 → L + 2 (34%) H-2 → L + 1 (17%) | |
| C55H18O8 | S0 → S1 | 1.84 | 674 | 0.12 | H → L (92%) |
| S0 → S4 | 2.33 | 532 | 0.52 | H → L + 1 (44%) H-1 → L (40%) | |
| S0 → S7 | 2.76 | 449 | 0.51 | H → L + 2 (55%) H-1 → L + 1 (21%) | |
| S0 → S9 | 2.98 | 416 | 0.29 | H-1 → L + 2 (45%) H → L + 3 (40%) | |
| S0 → S11 | 3.03 | 409 | 0.22 | H → L + 3 (46%) H-1 → L + 2 (29%) | |
| C61H22O10 | S0 → S1 | 1.58 | 785 | 0.18 | H → L (85%) |
| S0 → S2 | 1.81 | 685 | 1.43 | H → L + 1 (84%) | |
| S0 → S7 | 2.59 | 479 | 0.17 | H → L + 2 (51%) H-1 → L + 1 (21%) | |
| S0 → S9 | 2.79 | 444 | 0.24 | H-2 → L (78%) | |
| C84H26O13 | S0 → S1 | 1.74 | 713 | 0.41 | H → L (93%) |
| S0 → S4 | 2.18 | 569 | 0.28 | H → L + 1 (66%) H-1 → L + 2 (10%) | |
| S0 → S7 | 2.50 | 496 | 1.30 | H-1 → L + 1 (41%) H → L + 2 (29%) | |
| S0 → S10 | 2.63 | 471 | 0.80 | H-1 → L + 2 (33%) H → L + 3 (26%) H-3 → L (26%) | |
| C98H24O14 | S0 → S1 + S2 | 1.61–1.63 | 761–770 | 0.43 | H → L (61%) H → L + 1 (27%) |
| S0 → S6 | 2.10 | 590 | 0.72 | H → L + 2 (48%) H → L + 3 (18%) | |
| S0 → S11 | 2.37 | 523 | 1.09 | H-1 → L + 3 (48%) H-2 → L + 1 (13%) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ershov, I.V.; Lavrentyev, A.A.; Bazhin, I.V.; Holodova, O.M.; Prutsakova, N.V.; Zhdanova, T.P.; Romanov, D.L. Modelling the Structure and Optical Properties of Reduced Graphene Oxide Produced by Laser Ablation: Insights from XPS and Time-Dependent DFT. Crystals 2023, 13, 600. https://doi.org/10.3390/cryst13040600
Ershov IV, Lavrentyev AA, Bazhin IV, Holodova OM, Prutsakova NV, Zhdanova TP, Romanov DL. Modelling the Structure and Optical Properties of Reduced Graphene Oxide Produced by Laser Ablation: Insights from XPS and Time-Dependent DFT. Crystals. 2023; 13(4):600. https://doi.org/10.3390/cryst13040600
Chicago/Turabian StyleErshov, Igor V., Anatoly A. Lavrentyev, Igor V. Bazhin, Olga M. Holodova, Natalia V. Prutsakova, Tatiana P. Zhdanova, and Dmitry L. Romanov. 2023. "Modelling the Structure and Optical Properties of Reduced Graphene Oxide Produced by Laser Ablation: Insights from XPS and Time-Dependent DFT" Crystals 13, no. 4: 600. https://doi.org/10.3390/cryst13040600
APA StyleErshov, I. V., Lavrentyev, A. A., Bazhin, I. V., Holodova, O. M., Prutsakova, N. V., Zhdanova, T. P., & Romanov, D. L. (2023). Modelling the Structure and Optical Properties of Reduced Graphene Oxide Produced by Laser Ablation: Insights from XPS and Time-Dependent DFT. Crystals, 13(4), 600. https://doi.org/10.3390/cryst13040600









