Study on Oscillatory Mechanism of Solutocapillary Convection and Influence of Aspect Ratio on Flow Characteristics during Crystal Growth
Abstract
1. Introduction
2. Models and Methods
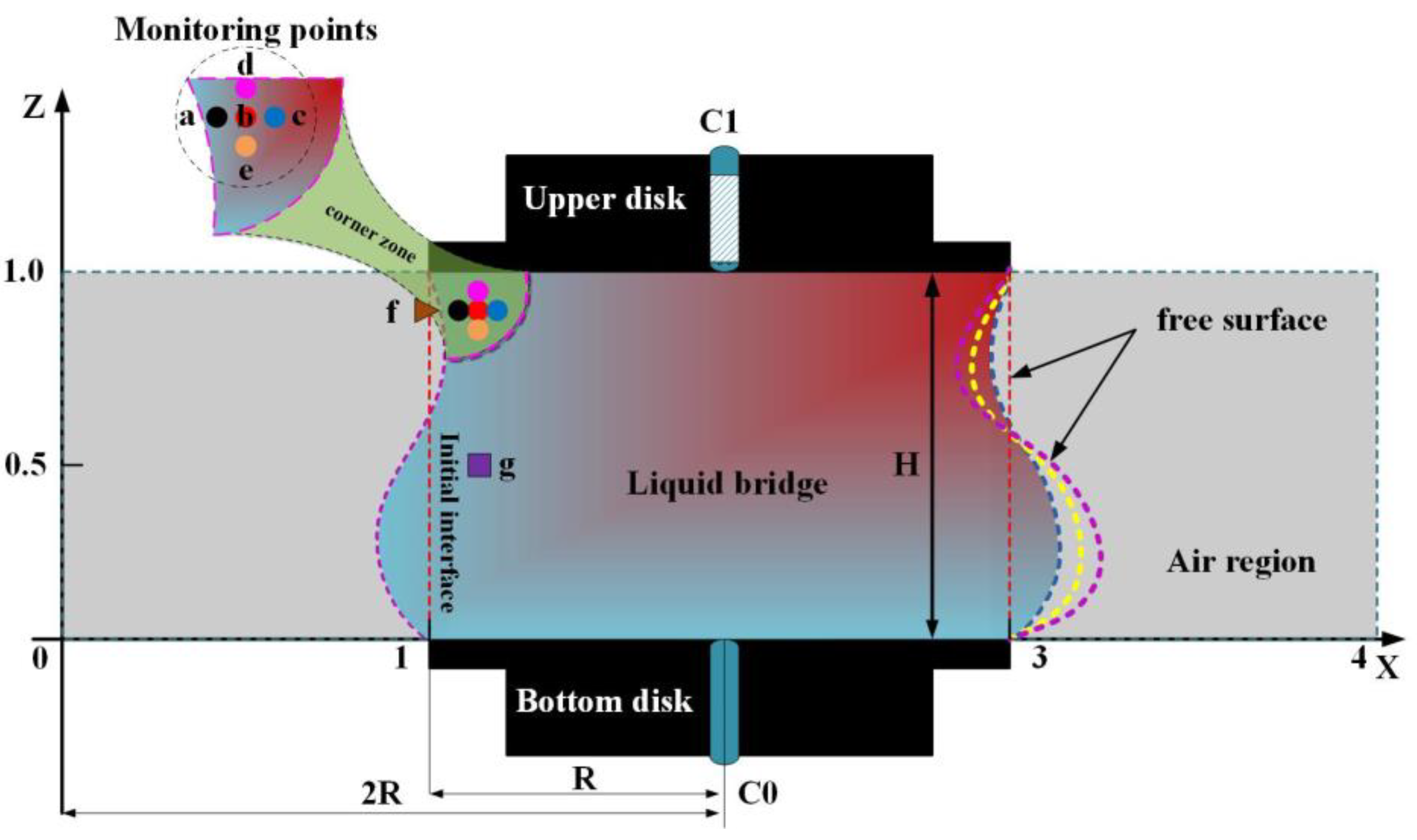
2.1. Geometric Model
2.2. Governing Equations and Boundary Conditions
2.3. Level Set Method
3. Results and discussions
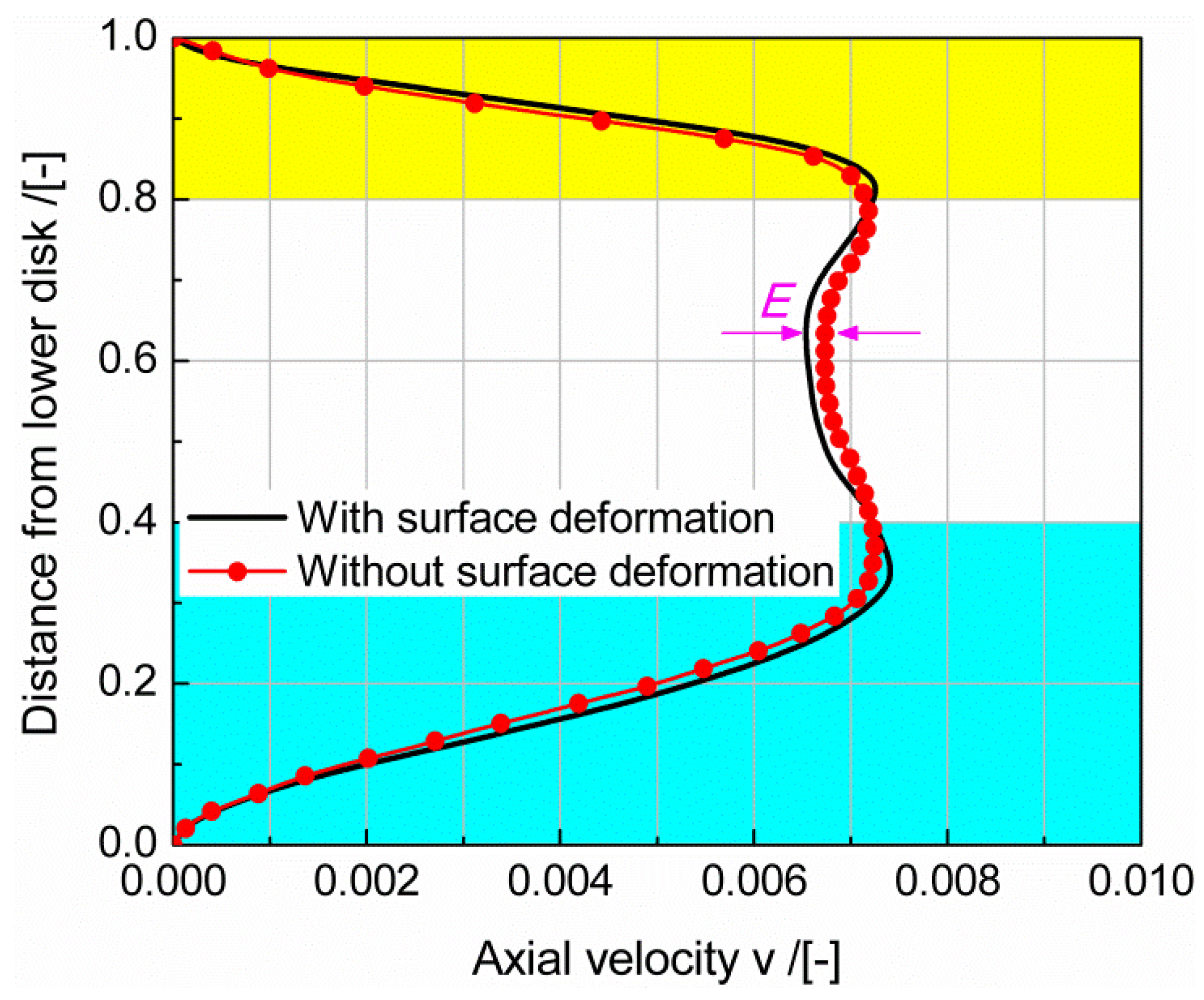
3.1. Code Validation
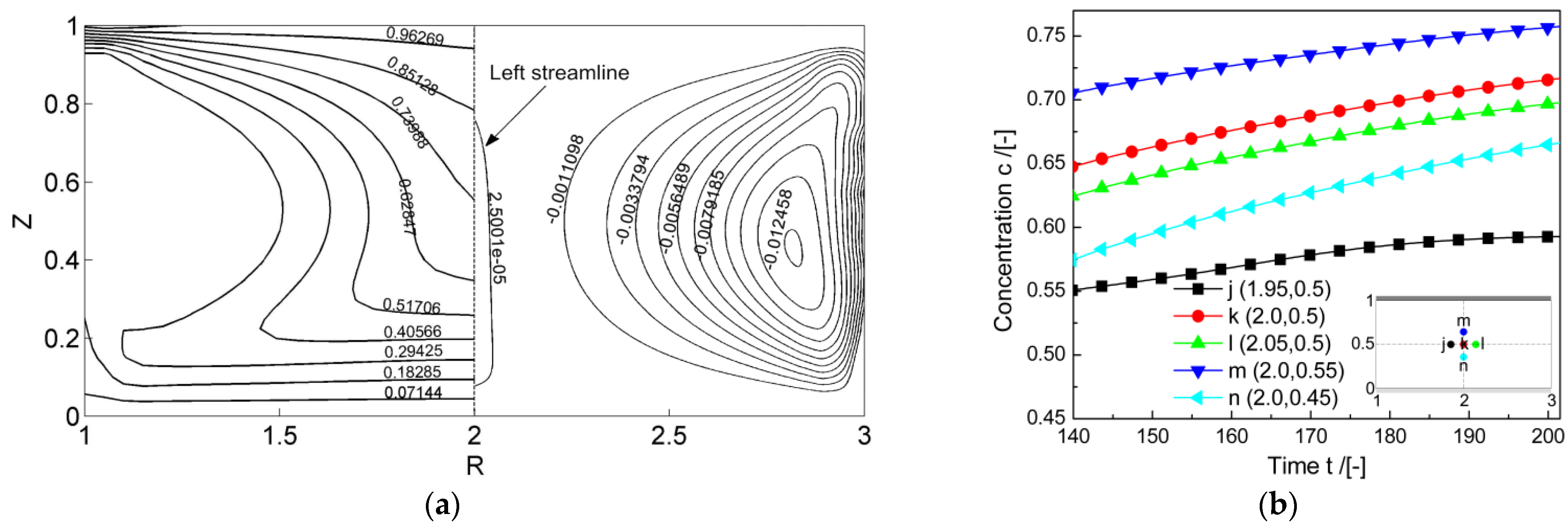
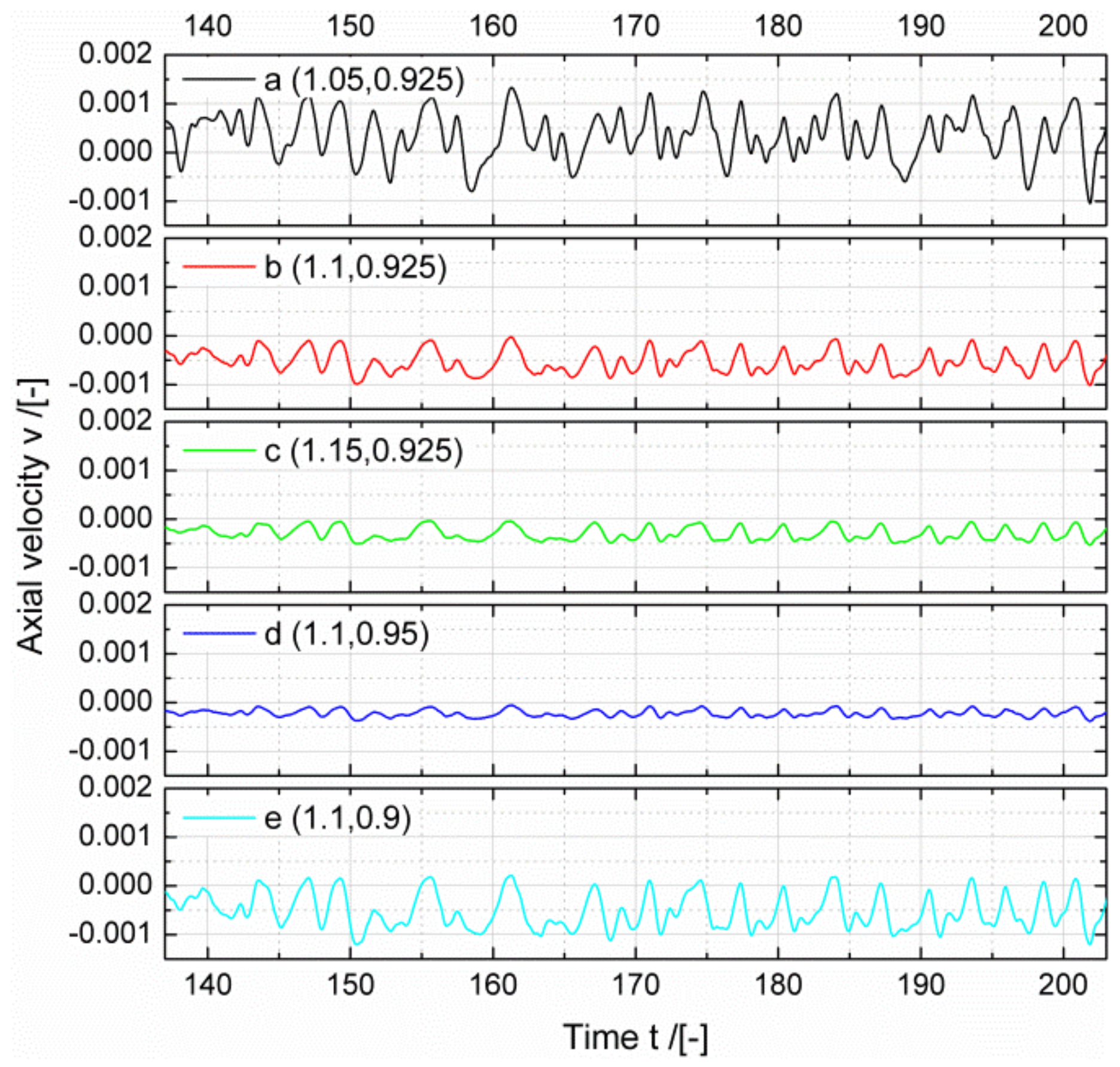
3.2. Oscillatory Mechanism of Solutocapillary Convection in Liquid Bridge
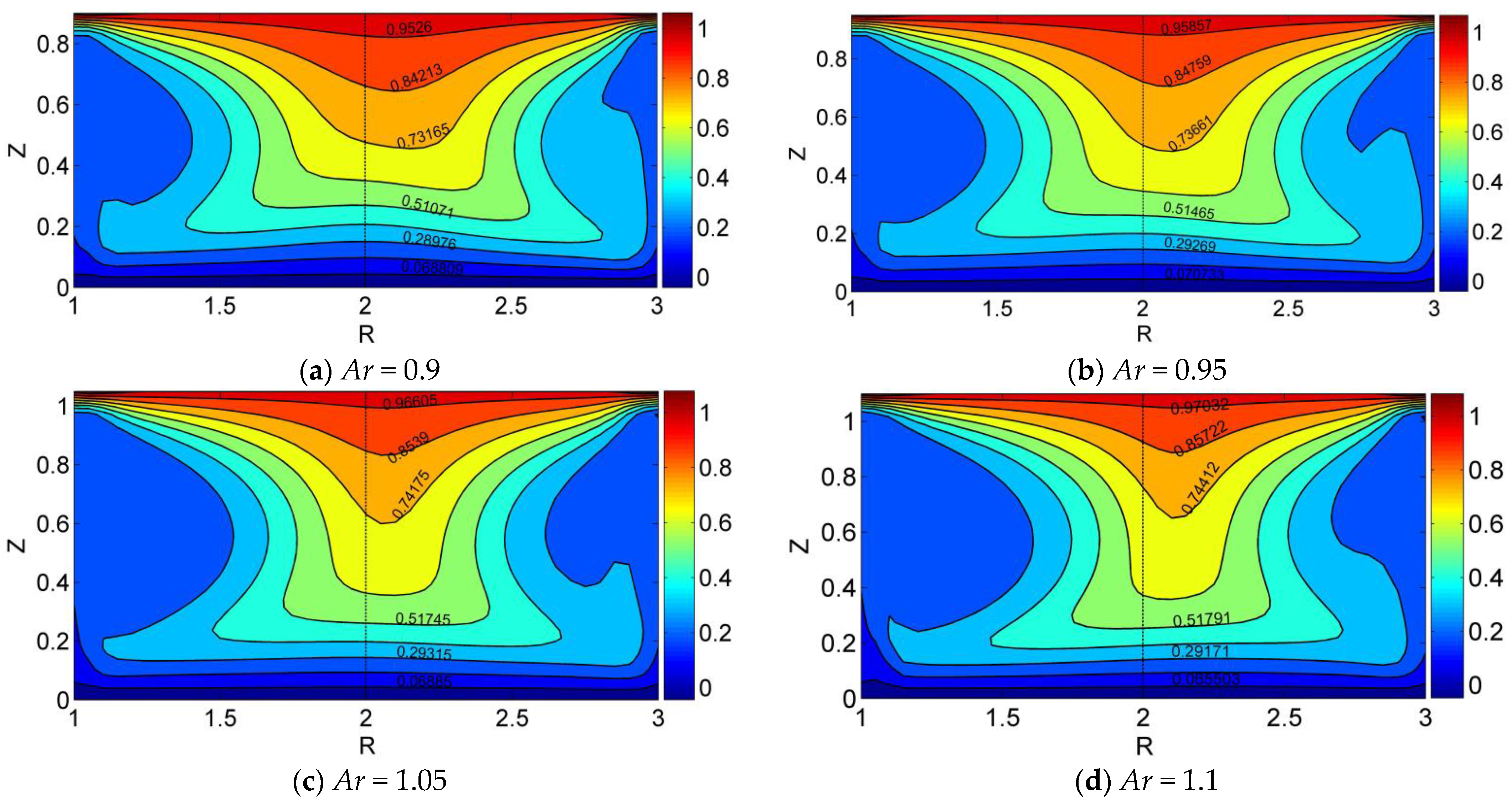
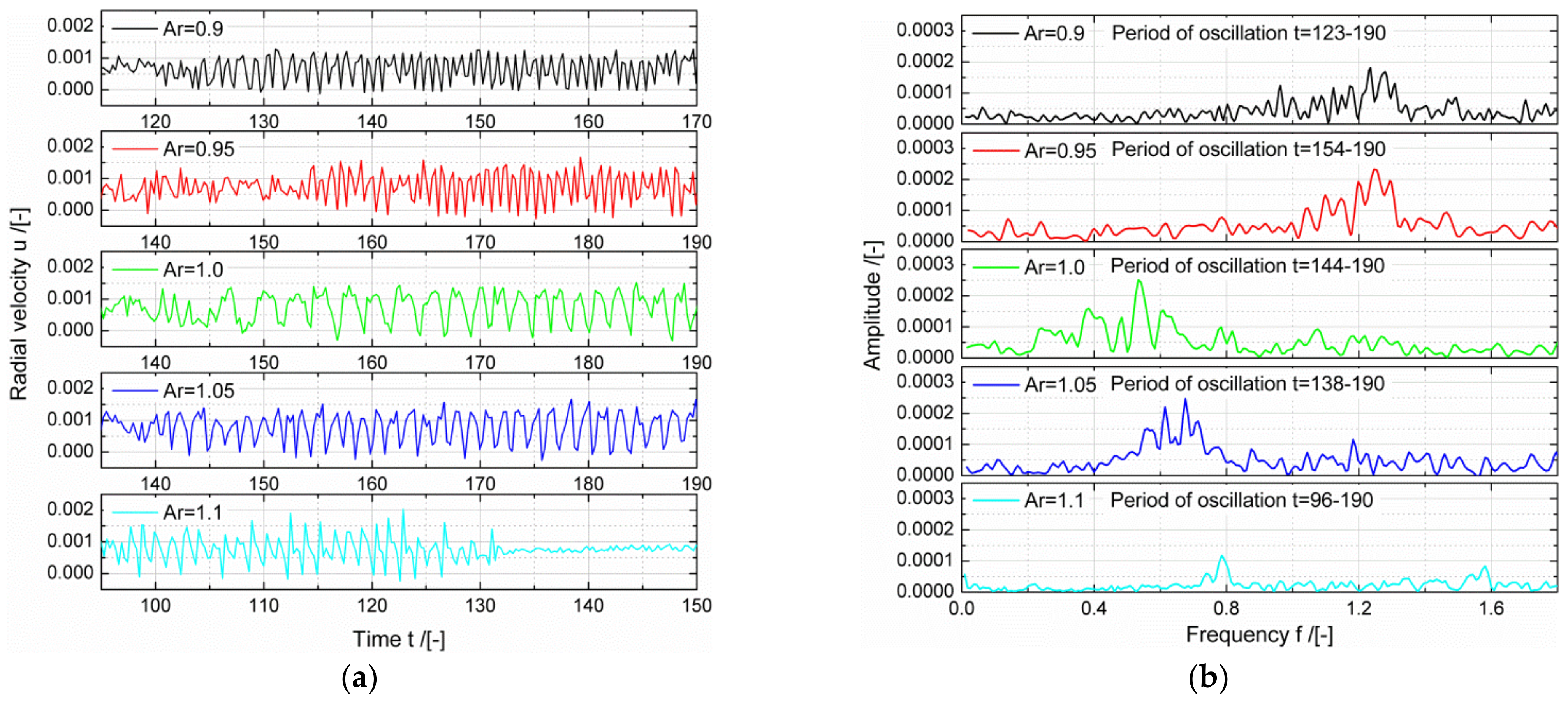
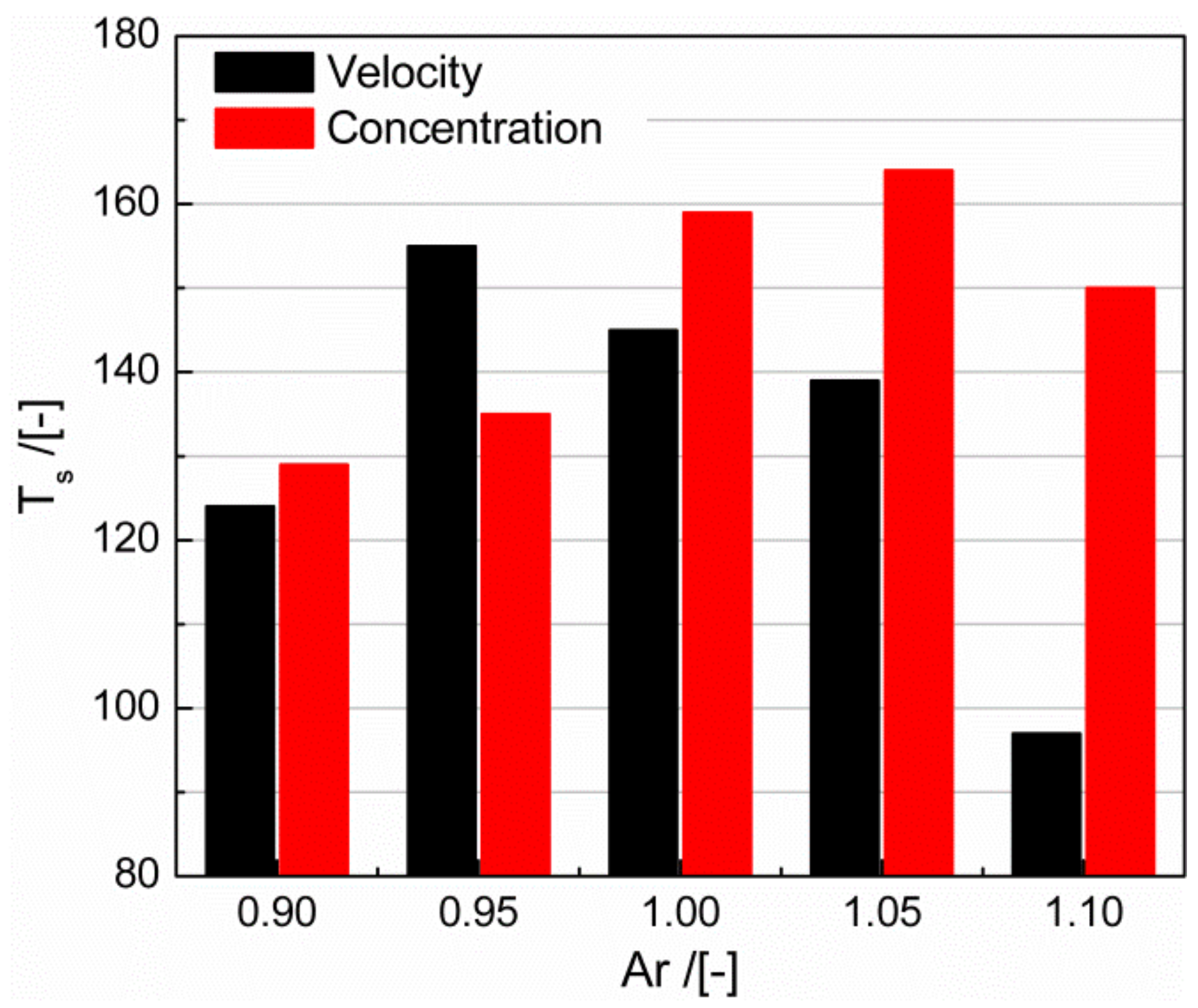
3.3. Influence of Aspect Ratio on Flow Characteristics of Oscillatory Solutocapillary Convection in Liquid Bridge
4. Conclusions
- The complex coupling effect of the oscillation among the concentration, velocity and surface leads to the oscillating solutecapillary convection starting from the high concentration corner area. The oscillation at the middle height is later than that in the corner area, and the oscillation of the concentration and velocity is stronger because it is closer to the surface. The oscillation intensity of the concentration in the corner area is relatively larger than that in the middle height. The oscillation of the radial velocity is stronger than that of the axial velocity and has obvious regularity, while the oscillation of the axial velocity at the middle height is significantly stronger than that of the radial velocity and has obvious regularity. The oscillation on the free surface belongs to high-frequency oscillation, and the oscillation of the transverse displacement of the free surface in the corner region is stronger than that of the free surface at the intermediate height.
- Within a certain height range, the smaller the height of the liquid bridge (corresponding to 0.9 ≤ Ar ≤ 1.0), the more stable the solutocapillary convection. The larger the height (corresponding to 1.0 ≤ Ar ≤ 1.05), the greater the amplitudes of the oscillations for the concentration, velocity, and surface. At a constant concentration difference, there is no obvious positive correlation between the onset time of the velocity and concentration oscillation and different aspect ratios. There is a relationship of mutual promotion and offset between the coupling oscillations of the concentration, velocity and surface transverse displacement.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | Amplitude |
| Ar | aspect ratio, H/R |
| C′ | dimensionless concentration, (C1-C)/(C1-C0) |
| ∆C | concentration difference, C1-C0 |
| CaC | concentration capillary, σC ∆C/σ0 |
| D | concentration diffusion coefficient |
| D′ | viscous stress tensor |
| f | frequency |
| H | liquid bridge height |
| L | characteristic length |
| n | unit normal vector of gas-liquid interface |
| p | dimensionless pressure, p/(ρlU2) |
| R | liquid bridge radius |
| Re | Reynolds number, ρlUL/μl |
| Sc | Schmidt number |
| T | period |
| t | time |
| U | characteristic velocity |
| u | fluid velocity |
| V | dimensionless velocity |
| We | Weber number, ρlU2L/σ |
| u,v | velocity in the X and Z directions |
| X,Z | radial and axial coordinates |
| - | dimensionless |
| Greek symbols | |
| μ | dynamic viscosity |
| ρ | density |
| κ | curvature of gas-liquid interface |
| δ(d) | dirac trigonometric function |
| σ0 | initial surface tension |
| σC | concentration coefficient of surface tension |
| φ | level set function |
| Subscripts | |
| 0 | lower disk |
| 1 | upper disk |
| g | gas |
| l | liquid |
References
- Laknath, R.; Mendis, A.; Sekimoto, A.; Okano, Y.; Minakuchi, H.; Dost, S. A numerical study on the exact onset of flow instabilities in thermo-solutal Marangoni convection driven by opposing forces in a half-zone liquid bridge under zero gravity. J. Chem. Eng. JPN. 2021, 54, 424–430. [Google Scholar] [CrossRef]
- Kawaji, M.; Liang, R.Q.; Nasr-Esfahany, M.; Simic-Stefani, S.; Yodab, S. The effect of small vibrations on Marangoni convection and the free surface of a liquid bridge. Acta Astronaut. 2006, 58, 622–632. [Google Scholar] [CrossRef]
- Wang, Y.; Zeng, Z.; Liu, H.; Zhang, L.Q.; Yin, L.M.; Xiao, Y.; Liu, Y. Flow instabilities in thermocapillary liquid bridges between two coaxial disks with different radii. Int. J. Heat Mass Tran. 2022, 183, 122182. [Google Scholar] [CrossRef]
- Liang, R.Q.; Zhou, J.L.; Yang, S.; Zhang, Y.Y.; Shi, J.H.; Xiao, S. Experimental study on transition characteristics of theremo-solutocapillary convection under buoyancy. Exp. Therm. Fluid Sci. 2019, 106, 38–47. [Google Scholar] [CrossRef]
- Bratukhin, Y.K.; Kostarev, K.G.; Viviani, A.; Zuev, A.L. Experimental study of Marangoni bubble migration in normal gravity. Exp. Fluids 2005, 38, 594–605. [Google Scholar] [CrossRef]
- Campbell, T.A.; Schweizer, M.; Dold, P.; Cröll, A.; Benz, K.W. Float zone growth and characterization of Ge1−xSix (x ≤ 10 at %) single crystals. J. Cryst. Growth 2001, 226, 231–239. [Google Scholar] [CrossRef]
- Benz, K.W.; Dold, P. Crystal growth under microgravity: Present results and future prospects towards the International Space Station. J. Cryst. Growth 2002, 237, 1638–1645. [Google Scholar] [CrossRef]
- Witkowski, L.M.; Walker, J.S. Solutocapillary instabilities in liquid bridges. Phys. Fluids 2002, 14, 2647–2656. [Google Scholar] [CrossRef]
- Walker, J.S.; Dold, P.; Cröll, A.; Volz, M.P.; Szofran, F.R. Solutocapillary convection in the float-zone process with a strong magnetic field. Int J Heat Mass Tran. 2002, 45, 4695–4702. [Google Scholar] [CrossRef]
- Lin, K.; Dold, P.; Benz, K.W. Numerical study of influences of buoyancy and solutal Marangoni convection on flow structures in a germanium-silicon floating zone. Cryst. Res. Technol. 2005, 40, 550–556. [Google Scholar] [CrossRef]
- Lyubimova, T.P.; Scuridyn, R.V. Numerical modelling of three-dimensional thermo- and solutocapillary-induced flows in a floating zone during crystal growth. Eur. Phys. J.-Spec. Top. 2011, 192, 41–46. [Google Scholar] [CrossRef]
- Minakuchi, H.; Takagi, Y.; Okano, Y.; Gima, S. The relative contributions of thermo-solutal Marangoni convections on flow patterns in a liquid bridge. J. Cryst. Growth 2014, 385, 61–65. [Google Scholar] [CrossRef]
- Zhou, X.M.; Huai, X.L. Free surface deformation of thermo-solutocapillary convection in axisymmetric liquid bridge. Microgravity Sci. Technol. 2015, 27, 39–47. [Google Scholar] [CrossRef]
- Viviani, A.; Denisova, M.; Kostarev, K.; Zuev, A. The threshold origin of solutocapillary Marangoni convection on a bounded free surface. Acta Astronaut. 2014, 102, 200–206. [Google Scholar] [CrossRef]
- Mizev, A.; Birikh, R. Interaction between buoyant and solutocapillary convections induced by a surface-active source placed under the free surface. Eur. Phys. J-Spec. Top. 2011, 192, 145–153. [Google Scholar] [CrossRef]
- Yang, S.; Qin, D.C.; Zhang, Y.P.; Xu, L.; Fu, Y.D.; Cui, J.; Pan, H. Experimental study on the influence of gravitational tilt angle on the spatio-temporal evolution of solutocapillary convection. Symmetry 2022, 12, 2485. [Google Scholar] [CrossRef]
- Lappa, M.; Savino, R.; Monti, R. Three-dimensional numerical simulation of Marangoni instabilities in liquid bridges: Influence of geometrical aspect ratio. Int. J. Numer. Meth Fluids 2001, 36, 53–90. [Google Scholar] [CrossRef]
- Tang, Z.M.; Hu, W.R. Influence of aspect ratio on the onset of thermocapillary oscillatory convection in a floating half zone of large Prandtl number fluid. Chin. Phys. Lett. 2003, 20, 526–528. [Google Scholar]
- Yan, A.A.; Li, K.; Cao, Z.H.; Hu, W.R. Aspect-ratio dependent oscillatory thermocapillary convection in the floating half zone. Sci. China-Phys. Mech. 2011, 54, 338–341. [Google Scholar] [CrossRef]
- Fan, J.G.; Liang, R.Q. Thermal-solutal capillary convection in binary mixture liquid bridge with various aspect ratios under microgravity. J. Cryst. Growth 2022, 586, 126630. [Google Scholar] [CrossRef]
- Zhang, Y.; Huang, H.L.; Zhang, X.D.; Zou, Y.; Tang, S.J. The effect of aspect ratio and axial magnetic field on thermocapillary convection in liquid bridges with a deformable free-surface. Eng. Appl. Comp. Fluid. 2015, 10, 16–28. [Google Scholar] [CrossRef]
- Yano, T.; Nishino, K. Effect of liquid bridge shape on the oscillatory thermal Marangoni convection. Eur. Phys. J-Spec. Top. 2015, 224, 289–298. [Google Scholar] [CrossRef]
- Mendis, R.L.A.; Sekimoto, A.; Okano, Y.; Minakuchi, H.; Dost, S. The relative Contribution of solutal Marangoni convection to thermal Marangoni flow instabilities in a liquid bridge of smaller aspect ratios under zero gravity. Crystals 2021, 11, 116. [Google Scholar] [CrossRef]
- Kan, K.; Yang, Z.X.; Lyu, P.; Zheng, Y.; Shen, L. Numerical study of turbulent flow past a rotating axial-flow pump based on a level-set immersed boundary method. Renew. Energ. 2020, 168, 960–971. [Google Scholar] [CrossRef]
- Kan, K.; Chen, H.X.; Zheng, Y.; Zhou, D.Q.; Binama, M.; Dai, J. Transient characteristics during power-off process in a shaft extension tubular pump by using a suitable numerical model. Renew. Energ. 2020, 164, 109–121. [Google Scholar] [CrossRef]
- Osher, S.; Sethian, J.A. Fronts propagating with curvature-dependent speed: Algorithms based on Hamilton-Jacobi formulations. J. Comput. Phys. 1988, 79, 12–49. [Google Scholar] [CrossRef]
- Sussman, M.; Smereka, P.; Osher, S. A level set approach for computing solutions to incompressible two-phase flow. J. Comput. Phys. 1994, 114, 146–159. [Google Scholar] [CrossRef]
- Chang, Y.C.; Hou, T.Y.; Merriman, B.; Osher, S. A level set formulation of Eulerian interface capturing methods for incompressible fluid flows. J. Comput. Phys. 1996, 124, 449–464. [Google Scholar] [CrossRef]
- Chen, J.C.; Zhang, L.; Li, Y.R.; Yu, J.J. Three-dimensional numerical simulation of pure solutocapillary flow in a shallow annular pool for mixture fluid with high Schmidt number. Microgravity Sci. Technol. 2016, 28, 49–57. [Google Scholar] [CrossRef]
- Semenov, S.N.; Schimpf, M.E. Molecular thermodiffusion (thermophoresis) in liquid mixtures. Phys. Rev. E 2005, 72, 041202. [Google Scholar] [CrossRef]







| Toluene/n-Hexane Mixture | ||
|---|---|---|
| Density, ρ (25 °C) | [kg/m3] | 699 |
| Dynamic viscosity, μ (25 °C) | [Pa·s] | 3.37 × 10−4 |
| Kinematic viscosity, ν (25 °C) | [m2/s] | 4.82 × 10−7 |
| Surface tension, σ (25 °C) | [N/m] | 2.1 × 10−2 |
| Concentration coefficient of surface tension, σC | [N/m] | −8.62 × 10−3 |
| Schmidt number, Sc | [-] | 142.8 |
| Grids | Axial Velocity | Radial Velocity | Concentration |
|---|---|---|---|
| 41 × 21 | 0.002132 | 0.0001817 | 0.2337 |
| 81 × 41 | 0.002178 | 0.0001907 | 0.2376 |
| 101 × 51 | 0.002168 | 0.0001955 | 0.2365 |
| 121 × 61 | 0.002189 | 0.0001872 | 0.2384 |
| Monitoring Points at the Upper Corner | Monitoring Point on Free Surface of the Upper Corner | Monitoring Point at the Intermediate Height |
|---|---|---|
| a (1.05, 0.925) d (1.1, 0.95) | f (1.0, 0.925) | g (1.1, 0.5) |
| b (1.1, 0.925) e (1.1, 0.9) | ||
| c (1.15, 0.925) |
| Ar | 0.9 | 0.95 | 1.0 | 1.05 | 1.1 |
|---|---|---|---|---|---|
| Monitoring point h at upper corner | (1.15, 0.855) | (1.15, 0.9025) | (1.15, 0.95) | (1.15, 0.9975) | (1.15, 1.045) |
| Monitoring point i at intermediate height | (1.15, 0.45) | (1.15, 0.475) | (1.15, 0.5) | (1.15, 0.525) | (1.15, 0.55) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, S.; Liang, R.; Yang, S. Study on Oscillatory Mechanism of Solutocapillary Convection and Influence of Aspect Ratio on Flow Characteristics during Crystal Growth. Crystals 2023, 13, 298. https://doi.org/10.3390/cryst13020298
Zhang S, Liang R, Yang S. Study on Oscillatory Mechanism of Solutocapillary Convection and Influence of Aspect Ratio on Flow Characteristics during Crystal Growth. Crystals. 2023; 13(2):298. https://doi.org/10.3390/cryst13020298
Chicago/Turabian StyleZhang, Shuo, Ruquan Liang, and Shuo Yang. 2023. "Study on Oscillatory Mechanism of Solutocapillary Convection and Influence of Aspect Ratio on Flow Characteristics during Crystal Growth" Crystals 13, no. 2: 298. https://doi.org/10.3390/cryst13020298
APA StyleZhang, S., Liang, R., & Yang, S. (2023). Study on Oscillatory Mechanism of Solutocapillary Convection and Influence of Aspect Ratio on Flow Characteristics during Crystal Growth. Crystals, 13(2), 298. https://doi.org/10.3390/cryst13020298












