The Effects of Chlorine Doping on the Mechanical Properties of Bi2O2Se
Abstract
1. Introduction
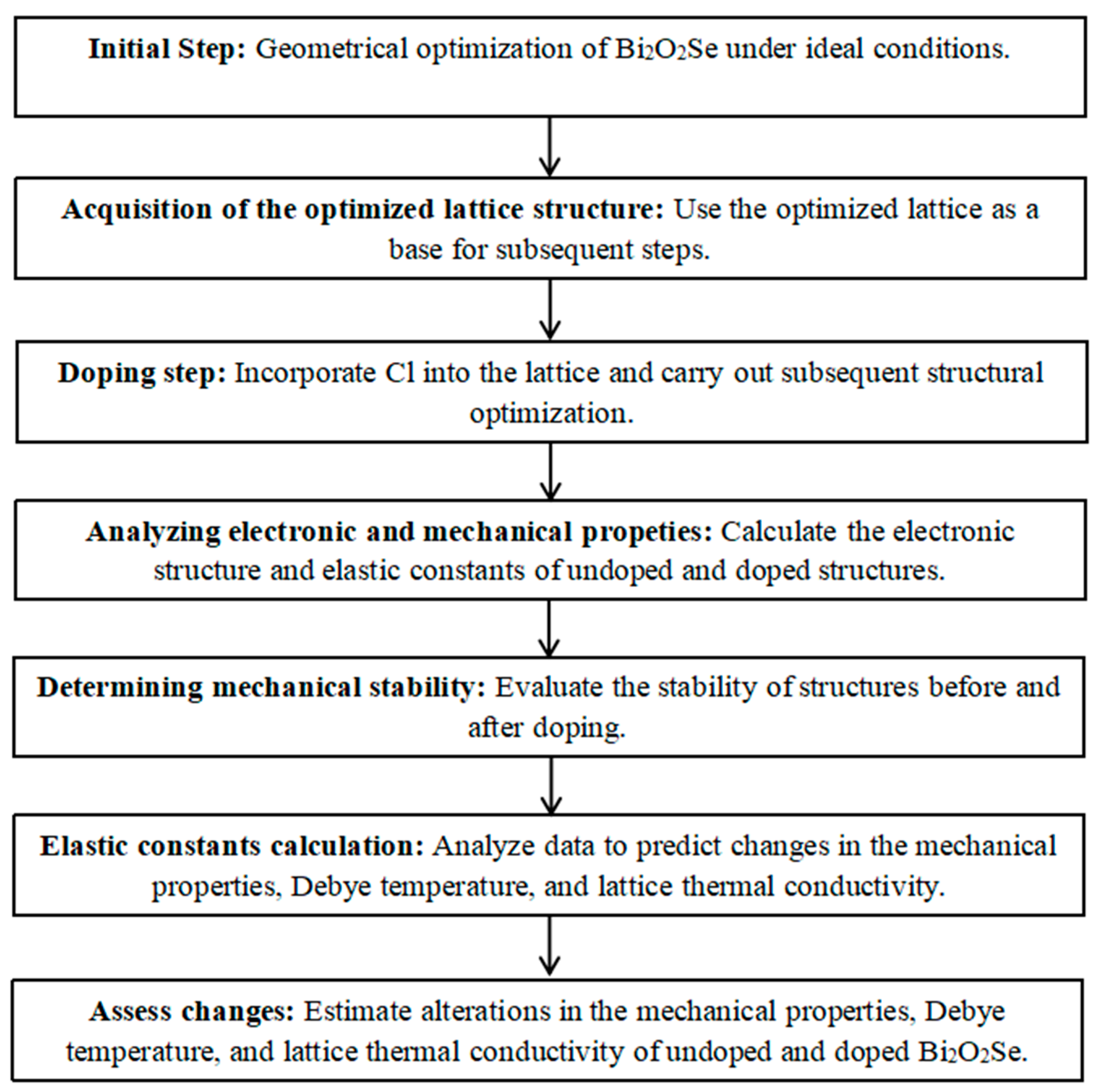
2. Computational Details
3. Results and Discussions
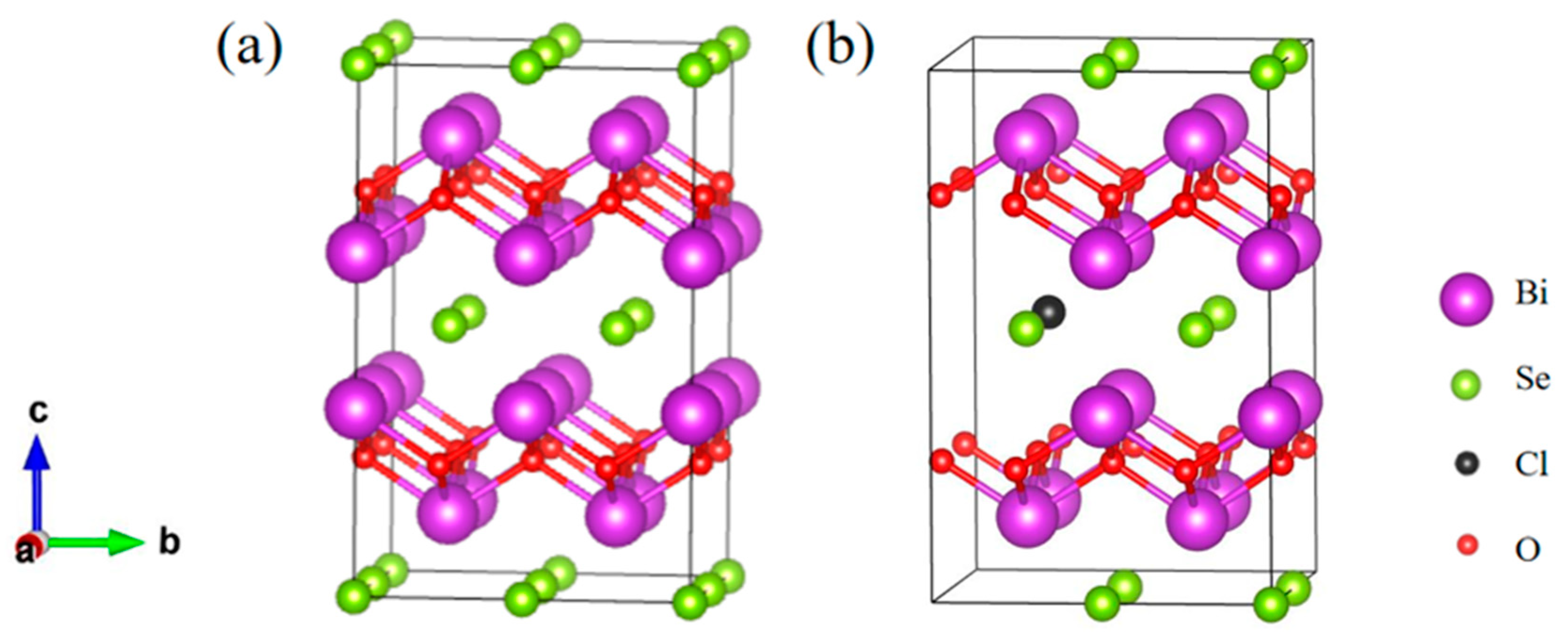
3.1. Structural Properties of Bi2O2Se and Bi2O2Se0.875Cl0.125
3.2. The Influence of Cl Doping on the Electronic Structure of Bi2O2Se
3.3. Mechanical Properties of Bi2O2Se and Bi2O2Se0.875Cl0.125
3.3.1. Elastic Constants of Bi2O2Se and Bi2O2Se0.875Cl0.125
3.3.2. Elastic Moduli of Bi2O2Se and Bi2O2Se0.875Cl0.125
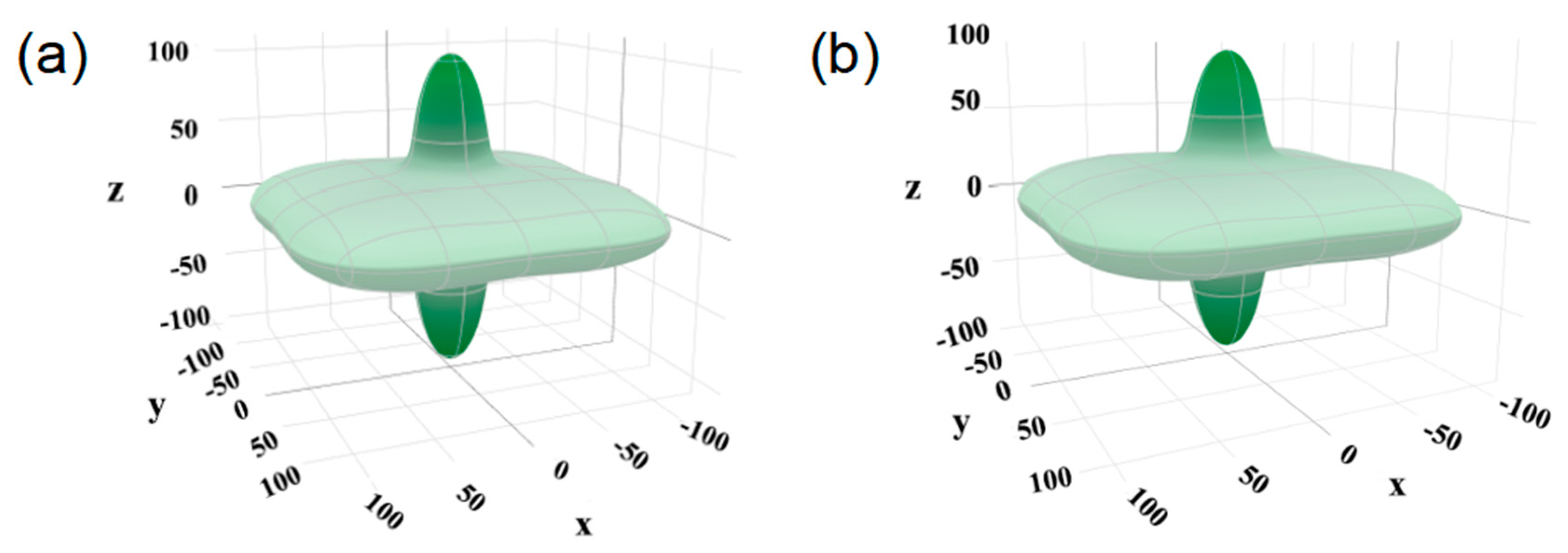
3.3.3. Ductility and Elastic Anisotropy of Bi2O2Se and Bi2O2Se0.875Cl0.125
3.3.4. Debye Temperature of Bi2O2Se and Bi2O2Se0.875Cl0.125
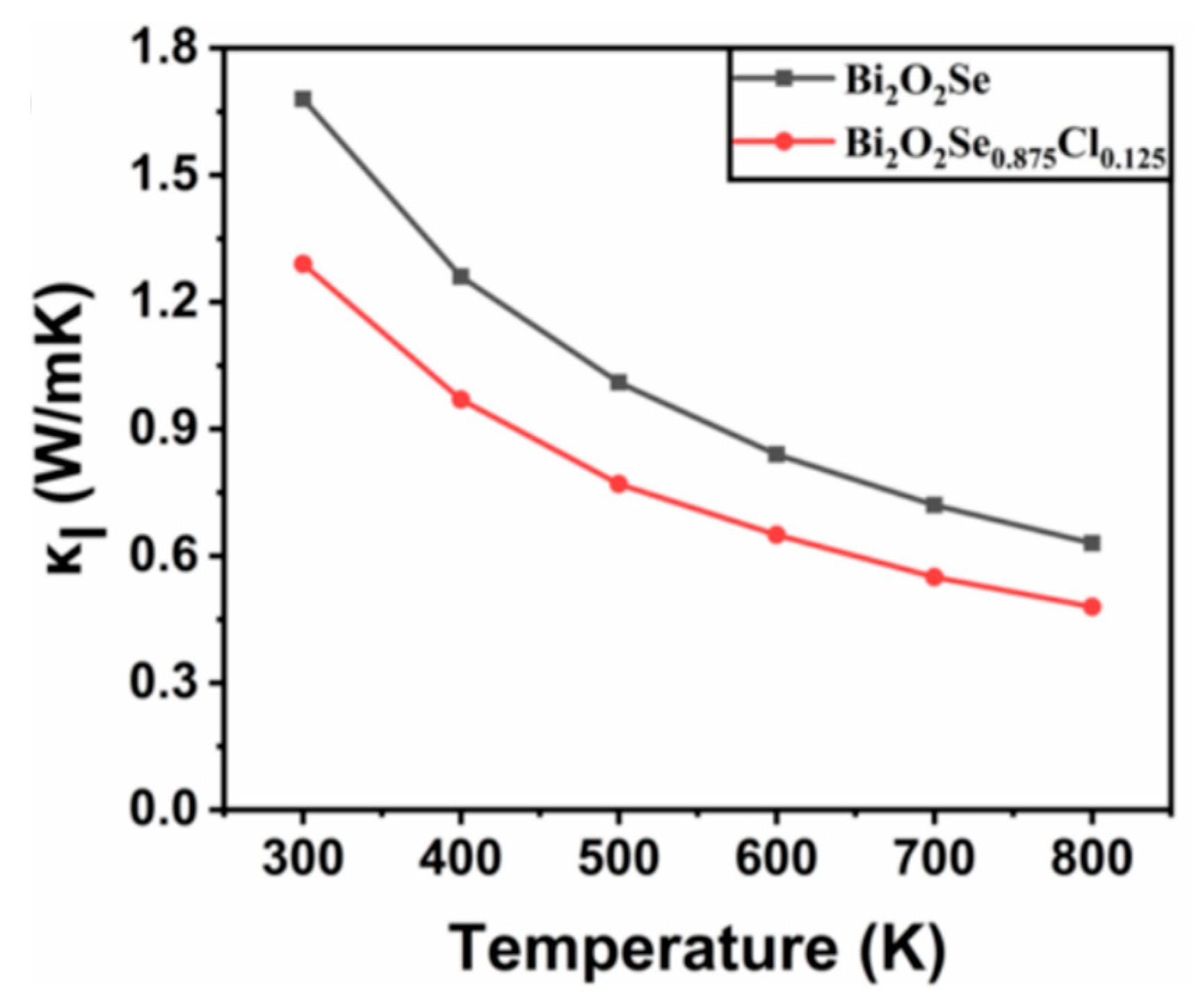
3.3.5. Lattice Thermal Conductivity of Bi2O2Se and Bi2O2Se0.875Cl0.125
4. Conclusions
- The Cl doping results in an increase of 0.74% in the lattice constant a0 (3.946 Å) of Bi2O2Se, while c0 (12.287 Å) is decreased by 0.57% and the volume is expanded by 0.91%. At the same time, the calculated <Bi-O> bond lengths (2.339 Å) is increased by 0.09%, and the <Bi-Se> bond length (3.336 Å) is increased by 0.76%.
- The Cl doping has significant influences on the electronic structure of Bi2O2Se. Different from the semiconductor character of the pristine Bi2O2Se, the Bi2O2Se0.875Cl0.125 exhibits metallic properties, since a certain number of electrons are distributed at the Fermi level.
- As compared with the elastic constants of Bi2O2Se, the calculated elastic constants C11 = 143.17 GPa, C12 = 63.39 GPa, C33 = 106.87 GPa, C44 = 11.96 GPa and C66 = 53.74 GPa for Bi2O2Se0.875Cl0.125 are reduced by 10.18%, 13.81%, 11.88%, 10.81%, and 6.88%, respectively, while the calculated value of C13 (46.38 GPa) is increased by 4.84%. These results suggest that Cl doping has remarkable effects on the mechanical properties of Bi2O2Se. Both the doped and undoped Bi2O2Se satisfy the criteria of mechanical stability and exhibit elastic anisotropy.
- The Cl doping leads to a reduction of 7.40%, 11.46%, and 11.05% in the bulk modulus, shear modulus, and Young’s modulus of Bi2O2Se, respectively, suggesting that Cl doping leads to a more plastic deformation of Bi2O2Se, which could help to prevent cracking under extreme temperature gradients.
- The calculated B/G value of Bi2O2Se0.875Cl0.125 is 2.94, which is 4.63% higher than that of pure Bi2O2Se, indicating that Cl doping enhances the ductility of Bi2O2Se.
- The calculated Debye temperature θ of Bi2O2Se0.875Cl0.125 is 212.5 K, which is 11.6 K lower than that of pure Bi2O2Se (224.1 K). This lower Debye temperature helps to improve the toughness and processability of Bi2O2Se.
- The lattice thermal conductivity of both Bi2O2Se and Bi2O2Se0.875Cl0.125 decreases with increasing temperature. Cl doping reduces the lattice thermal conductivity of Bi2O2Se considerably. For example, the lattice thermal conductivity of Bi2O2Se0.875Cl0.125 at 300 K is 1.29 W/mK, which is 23.21% lower than that of pure Bi2O2Se (1.68 W/mK). The lower lattice thermal conductivity is beneficial in inhibiting heat transfer and minimizing thermal energy loss.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, N.; Li, M.; Xiao, H.; Gao, Z.; Liu, Z.; Zu, X.; Li, S.; Qiao, L. Band degeneracy enhanced thermoelectric performance in layered oxyselenides by first-principles calculations. npj Computat. Mater. 2021, 7, 18. [Google Scholar] [CrossRef]
- Wang, N.; Li, M.; Xiao, H.; Zu, X.; Qiao, L. Layered LaCuOSe: A Promising Anisotropic Thermoelectric Material. Phys. Rev. Appl. 2020, 13, 024038. [Google Scholar] [CrossRef]
- Liu, W.; Jie, Q.; Kim, H.S.; Ren, Z. Current progress and future challenges in thermoelectric power generation: From materials to devices. Acta Mater. 2015, 87, 357–376. [Google Scholar] [CrossRef]
- Yang, J.; Stabler, F.R. Automotive Applications of Thermoelectric Materials. J. Electron. Mater. 2009, 38, 1245–1251. [Google Scholar] [CrossRef]
- Wang, N.; Shen, C.; Sun, Z.; Xiao, H.; Zhang, H.; Yin, Z.; Qiao, L. High-Temperature Thermoelectric Monolayer Bi2TeSe2 with High Power Factor and Ultralow Thermal Conductivity. ACS Appl. Energy Mater. 2022, 5, 2564–2572. [Google Scholar] [CrossRef]
- Ren, D.S.; Feng, X.N.; Liu, L.S.; Hsu, H.J.; Lu, L.G.; Wang, L.; He, X.M.; Ouyang, M.G. Investigating the relationship between internal short circuit and thermal runaway of lithium-ion batteries under thermal abuse condition. Energy Storage Mater. 2021, 34, 563–573. [Google Scholar] [CrossRef]
- Hong, J.C.; Wang, Z.P.; Yao, Y.T. Fault prognosis of battery system based on accurate voltage abnormity prognosis using long short-term memory neural networks. Appl. Energy 2019, 251, 14. [Google Scholar] [CrossRef]
- Liu, J.; Tian, L.; Mou, Y.; Jia, W.; Zhang, L.; Liu, R. Electronic and mechanical property of high electron mobility semiconductor Bi2O2Se. J. Alloys Compd. 2018, 764, 674–678. [Google Scholar] [CrossRef]
- Pang, Z.; Li, T. Mechanics and strain engineering of bulk and monolayer Bi2O2Se. J. Mech. Phys. Solids 2021, 157, 104626. [Google Scholar] [CrossRef]
- Wang, Y.X.; Yan, Z.X.; Liu, W.; Zhou, G.L.; Gu, J.B. Ab initio study of the mechanical properties and thermal conductivity of Bi2O2X (X = Se, Te) under pressure. Solid State Sci. 2020, 106, 106299. [Google Scholar] [CrossRef]
- Zhang, Z.; Chen, J.; Zhang, W.; Yu, Z.; Yu, C.; Lu, H. Systematically investigate mechanical and electrical properties of Bi2O2Se by Te atom substitution and compare it with homologue Bi2O2Te from first-principles calculations. Mater. Today Commun. 2020, 24, 101182. [Google Scholar] [CrossRef]
- Piriz, S.; Fernandez-Werner, L.; Pardo, H.; Jasen, P.; Faccio, R.; Mombru, A.W. Mechanical properties and electronic structure of edge-doped graphene nanoribbons with F, O, and Cl atoms. Phys. Chem. Chem. Phys. 2017, 19, 21474–21480. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Gu, Y.; Wang, S.; Li, Q.; Li, M.; Zhang, Z. Enhanced dielectric and mechanical properties in chlorine-doped continuous CNT sheet reinforced sandwich polyvinylidene fluoride film. Carbon 2016, 107, 405–414. [Google Scholar] [CrossRef]
- Park, H.; Woo, D.; Lee, J.M.; Park, S.J.; Lee, S.; Kim, H.J.; Yoon, E.; Lee, G.D. First principles investigation on energetics, structure, and mechanical properties of amorphous carbon films doped with B, N, and Cl. Sci. Rep. 2019, 9, 18961. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758. [Google Scholar] [CrossRef]
- Qiao, L.; Zhang, S.; Xiao, H.Y.; Singh, D.J.; Zhang, K.H.L.; Liu, Z.J.; Zu, X.T.; Li, S. Orbital controlled band gap engineering of tetragonal BiFeO3 for optoelectronic applications. J. Mater. Chem. C 2018, 6, 1239–1247. [Google Scholar] [CrossRef]
- Heyd, J.; Scuseria, G.E.; Ernzerhof, M. Hybrid functionals based on a screened Coulomb potential. J. Chem. Phys. 2003, 118, 8207–8215. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- Yao, H.; Ouyang, L.; Ching, W.-Y. Ab Initio Calculation of Elastic Constants of Ceramic Crystals. J. Am. Ceram. Soc. 2007, 90, 3194–3204. [Google Scholar] [CrossRef]
- Wu, J.; Tan, C.; Tan, Z.; Liu, Y.; Yin, J.; Dang, W.; Wang, M.; Peng, H. Controlled Synthesis of High-Mobility Atomically Thin Bismuth Oxyselenide Crystals. Nano Lett. 2017, 17, 3021–3026. [Google Scholar] [CrossRef]
- Chen, Q.; Zhang, P.; Qin, M.; Lou, Z.; Gong, L.; Xu, J.; Kong, J.; Yan, H.; Gao, F. Effect of La3+, Ag+ and Bi3+ doping on thermoelectric properties of SrTiO3: First-principles investigation. Ceram. Int. 2022, 48, 13803–13816. [Google Scholar] [CrossRef]
- Boiler, I. Die Kristallstruktur von Bi2O2Se. Monatsh. Chem. 1973, 104, 916–919. [Google Scholar] [CrossRef]
- Wu, J.; Yuan, H.; Meng, M.; Chen, C.; Sun, Y.; Chen, Z.; Dang, W.; Tan, C.; Liu, Y.; Yin, J.; et al. High electron mobility and quantum oscillations in non-encapsulated ultrathin semiconducting Bi2O2Se. Nat. Commun. 2017, 12, 530–534. [Google Scholar] [CrossRef] [PubMed]
- Tan, X.; Lan, J.-L.; Ren, G.; Liu, Y.; Lin, Y.-H.; Nan, C.-W. Enhanced thermoelectric performance of n-type Bi2O2Se by Cl-doping at Se site. J. Am. Ceram. Soc. 2017, 100, 1494–1501. [Google Scholar] [CrossRef]
- Hu, K.; Han, J.; Xu, B.; Lin, Y.H. Thermoelectric power factor of doped Bi2O2Se: A computational study. Phys. Chem. Chem. Phys. 2020, 22, 27096–27104. [Google Scholar] [CrossRef] [PubMed]
- Li, J.Q.; Cheng, C.; Duan, M.Y. The electronic and optical properties of multi-layer Bi2O2X (X = S, Se, Te) by first-principles calculations. Appl. Surf. Sci. 2023, 618, 156541. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, B.; Yu, G.; Zhang, J.; Ma, S.; Yuan, S.; Sun, T.; Wang, Y. Electronic structure and thermoelectric properties of Bi2O2Se with GGA and TB-mBJ potentials. Jpn. J. Appl. Phys. 2019, 58, 015501. [Google Scholar] [CrossRef]
- Yeh, J.W.; Chen, S.K.; Lin, S.J.; Gan, J.Y.; Chin, T.S.; Shun, T.T.; Tsau, C.H.; Chang, S.Y. Nanostructured high-entropy alloys with multiple principal elements: Novel alloy design concepts and outcomes. Adv. Eng. Mater. 2004, 6, 299–303. [Google Scholar] [CrossRef]
- Li, M.; Wang, N.; Jiang, M.; Xiao, H.; Zhang, H.; Liu, Z.; Zu, X.; Qiao, L. Improved thermoelectric performance of bilayer Bi2O2Se by the band convergence approach. J. Mater. Chem. C 2019, 7, 11029–11039. [Google Scholar] [CrossRef]
- Zhu, X.L.; Liu, P.F.; Xie, G.; Wang, B.T. First-principles study of thermal transport properties in the two- and three-dimensional forms of Bi2O2Se. Phys. Chem. Chem. Phys. 2019, 21, 10931–10938. [Google Scholar] [CrossRef] [PubMed]
- Wang, F.; Di Valentin, C.; Pacchioni, G. Electronic and Structural Properties of WO3: A Systematic Hybrid DFT Study. J. Phys. Chem. C 2011, 115, 8345–8353. [Google Scholar] [CrossRef]
- Garza, A.J.; Scuseria, G.E. Predicting Band Gaps with Hybrid Density Functionals. J. Phys. Chem. Lett. 2016, 7, 4165–4170. [Google Scholar] [CrossRef] [PubMed]
- He, J.; Franchini, C. Assessing the performance of self-consistent hybrid functional for band gap calculation in oxide semiconductors. J. Phys. Condens. Matter 2017, 29, 454004. [Google Scholar] [CrossRef] [PubMed]
- Li, M.; Hu, J.; Gong, H.; Ren, Q.; Liao, Y.; Xiao, H.; Qiu, Q.; Feng, S.; Zu, X. First-principles study of point defects in U3Si2: Effects on the mechanical and electronic properties. Phys. Chem. Chem. Phys. 2022, 24, 4287–4297. [Google Scholar] [CrossRef]
- Koc, H.; Ozisik, H.; Deligoz, E.; Mamedov, A.M.; Ozbay, E. Mechanical, electronic, and optical properties of Bi(2)S(3) and Bi(2)Se(3) compounds: First principle investigations. J. Mol. Model. 2014, 20, 2180. [Google Scholar] [CrossRef] [PubMed]
- Chung, D.H.; Buessem, W.R. The Voigt-Reuss-Hill (VRH) Approximation and the Elastic Moduli of Polycrystalline ZnO, TiO2 (Rutile), and α-Al2O3. J. Appl. Phys. 1968, 39, 2777–2782. [Google Scholar] [CrossRef]
- Voigt, W. Lehrbuch der Kristallphysik: Teubner-Leipzig; Macmillan: New York, NY, USA, 1928; p. 739. [Google Scholar]
- Reuss, A. Calculation of the flow limits of mixed crystals on the basis of the plasticity of monocrystals. Z. Angew. Math. Mech. 1929, 9, 49. [Google Scholar] [CrossRef]
- Sagar, R.U.R.; Khan, U.; Galluzzi, M.; Aslam, S.; Nairan, A.; Anwar, T.; Ahmad, W.; Zhang, M.; Liang, T. Transfer-Free Growth of Bi2O2Se on Silicon Dioxide via Chemical Vapor Deposition. ACS Appl. Electron. Mater. 2020, 2, 2123–2131. [Google Scholar] [CrossRef]
- Mahan, G.D. Figure of merit for thermoelectrics. J. Appl. Phys. 1989, 65, 1578–1583. [Google Scholar] [CrossRef]
- Majumder, R.; Hossain, M.M. First-principles study of structural, electronic, elastic, thermodynamic and optical properties of topological superconductor LuPtBi. Comput. Condens. Matter 2019, 21, e00402. [Google Scholar] [CrossRef]
- Nye, J.F.; Lindsay, R.B. Physical Properties of Crystals: Their Representation by Tensors and Matrices. Phys. Today 1957, 10, 26. [Google Scholar] [CrossRef]
- Shi, X.; Chen, H.; Hao, F.; Liu, R.; Wang, T.; Qiu, P.; Burkhardt, U.; Grin, Y.; Chen, L. Room-temperature ductile inorganic semiconductor. Nat. Mater. 2018, 17, 421–426. [Google Scholar] [CrossRef] [PubMed]
- Pugh, S.F. XCII. Relations between the elastic moduli and the plastic properties of polycrystalline pure metals. Lond. Edinb. Dublin Philos. Mag. J. Sci. 2009, 45, 823–843. [Google Scholar] [CrossRef]
- Li, M.; Wang, N.; Zhang, S.; Hu, J.; Xiao, H.; Gong, H.; Liu, Z.; Qiao, L.; Zu, X. A review of the properties, synthesis and applications of lanthanum copper oxychalcogenides. J. Phys. D Appl. Phys. 2022, 55, 273002. [Google Scholar] [CrossRef]
- MacIsaac, D.; Kanner, G.; Anderson, G. Basic physics of the incandescent lamp (lightbulb). PhTea 1999, 37, 520–525. [Google Scholar] [CrossRef][Green Version]
- Tvergaard, V.H.; Hutchinsonm, J.W. Microcracking in Ceramics Induced by Thermal Expansion or Elastic Anisotropy. J. Am. Ceram. Soc. 1988, 71, 157–166. [Google Scholar] [CrossRef]
- Ravindran, P.; Fast, L.; Korzhavyi, P.A.; Johansson, B.; Wills, J.; Eriksson, O. Density functional theory for calculation of elastic properties of orthorhombic crystals: Application to TiSi2. J. Appl. Phys. 1998, 84, 4891–4904. [Google Scholar] [CrossRef]
- Gaillac, R.; Pullumbi, P.; Coudert, F.X. ELATE: An open-source online application for analysis and visualization of elastic tensors. J. Phys. Condens. Matter 2016, 28, 275201. [Google Scholar] [CrossRef] [PubMed]
- Debye, P. Zur theorie der spezifischen wärmen. Ann. Phys. 1912, 344, 789–839. [Google Scholar]
- Feng, J.; Xiao, B.; Wan, C.L.; Qu, Z.X.; Huang, Z.C.; Chen, J.C.; Zhou, R.; Pan, W. Electronic structure, mechanical properties and thermal conductivity of Ln2Zr2O7 (Ln = La, Pr, Nd, Sm, Eu and Gd) pyrochlore. Acta Mater. 2014, 72, 1742–1760. [Google Scholar] [CrossRef]
- Anderson, O.L. A simplified method for calculating the debye temperature from elastic constants. J. Phys. Chem. Solids 1963, 24, 909–917. [Google Scholar] [CrossRef]
- Schreiber, E.; Anderson, O.L.; Soga, N.; Bell, J.F. Elastic constants and their measurement. J. Appl. Mech. 1975, 42, 747–748. [Google Scholar] [CrossRef]
- Tian, J.; Zhao, Y.; Hou, H.; Wang, B. The Effect of Alloying Elements on the Structural Stability, Mechanical Properties, and Debye Temperature of Al(3)Li: A First-Principles Study. Materials 2018, 11, 1471. [Google Scholar] [CrossRef] [PubMed]
- Morelli, D.T.; Slack, G.A. High Lattice Thermal Conductivity of Solids; Springer: New York, NY, USA, 2006; pp. 37–68. [Google Scholar]
- Li, Y.; Wang, J.; Wang, J. Approaching extremely low thermal conductivity by crystal structure engineering in Mg2Al4Si5O18. J. Mater. Res. 2015, 30, 3729–3739. [Google Scholar] [CrossRef]

| a0 | c0 | Volume | <Bi-O> | <Bi-Se> | ||
|---|---|---|---|---|---|---|
| Bi2O2Se | Our cal. | 3.917 | 12.357 | 189.592 | 2.337 | 3.311 |
| Exp. [23] | 3.88 | 12.16 | 183.06 | --- | --- | |
| Other cal. [22] | 3.90 | 12.39 | 188.45 | --- | --- | |
| Bi2O2Se0.875Cl0.125 | Our cal. | 3.946 | 12.287 | 191.320 | 2.339 | 3.336 |
| Compounds | C11 | C12 | C13 | C33 | C44 | C66 | |
|---|---|---|---|---|---|---|---|
| Bi2O2Se | Our Cal. | 159.39 | 73.55 | 44.24 | 121.28 | 13.41 | 57.71 |
| Other Cal. [8] | 155.48 | 71.56 | 43.47 | 119.01 | 11.23 | 56.41 | |
| Bi2O2Se0.875Cl0.125 | Our Cal. | 143.17 | 63.39 | 46.38 | 106.87 | 11.96 | 53.74 |
| Compounds | B | G | E | |
|---|---|---|---|---|
| Bi2O2Se | Our Cal. | 83.21 | 29.59 | 79.36 |
| Other Cal. [8] | 81.42 | 27.38 | 73.56 | |
| Other Cal. [10] | --- | --- | --- | |
| Exp. [40] | --- | --- | 66 | |
| Exp. [41] | 71.5 | --- | --- | |
| Bi2O2Se0.875Cl0.125 | Our Cal. | 77.05 | 26.20 | 70.59 |
| Compounds | |||||
|---|---|---|---|---|---|
| Bi2O2Se | Our Cal. | 3638 | 1787 | 2007 | 224.1 |
| Other Cal. [8] | 3584 | 1727 | 1939 | -- | |
| Other Cal. [10] | 1440 | 2630 | 1600 | 181.0 | |
| Bi2O2Se0.875Cl0.125 | Our Cal. | 3513 | 1699 | 1909 | 212.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, B.; Qi, H.; Li, M.; Zu, X.; Qiao, L.; Xiao, H. The Effects of Chlorine Doping on the Mechanical Properties of Bi2O2Se. Crystals 2023, 13, 1492. https://doi.org/10.3390/cryst13101492
Li B, Qi H, Li M, Zu X, Qiao L, Xiao H. The Effects of Chlorine Doping on the Mechanical Properties of Bi2O2Se. Crystals. 2023; 13(10):1492. https://doi.org/10.3390/cryst13101492
Chicago/Turabian StyleLi, Buda, Hangbo Qi, Menglu Li, Xiaotao Zu, Liang Qiao, and Haiyan Xiao. 2023. "The Effects of Chlorine Doping on the Mechanical Properties of Bi2O2Se" Crystals 13, no. 10: 1492. https://doi.org/10.3390/cryst13101492
APA StyleLi, B., Qi, H., Li, M., Zu, X., Qiao, L., & Xiao, H. (2023). The Effects of Chlorine Doping on the Mechanical Properties of Bi2O2Se. Crystals, 13(10), 1492. https://doi.org/10.3390/cryst13101492









