Mechanical Stabilities and Properties of Graphene-like 2D III-Nitrides: A Review
Abstract
1. Introduction
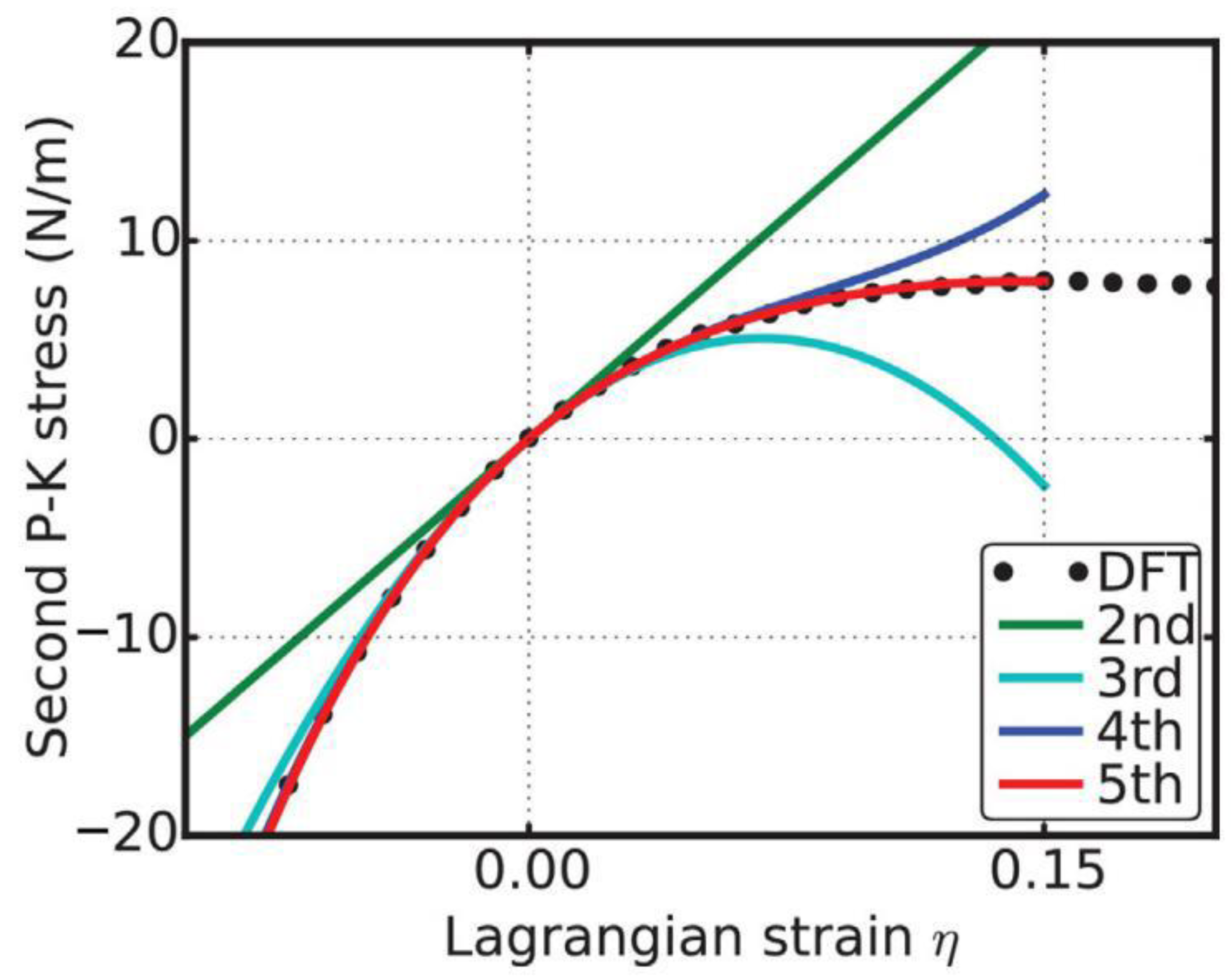
2. Five-Order Non-Linear Elasticity (FONE) Method
3. Density Functional Theory Calculations
4. Results and Analysis
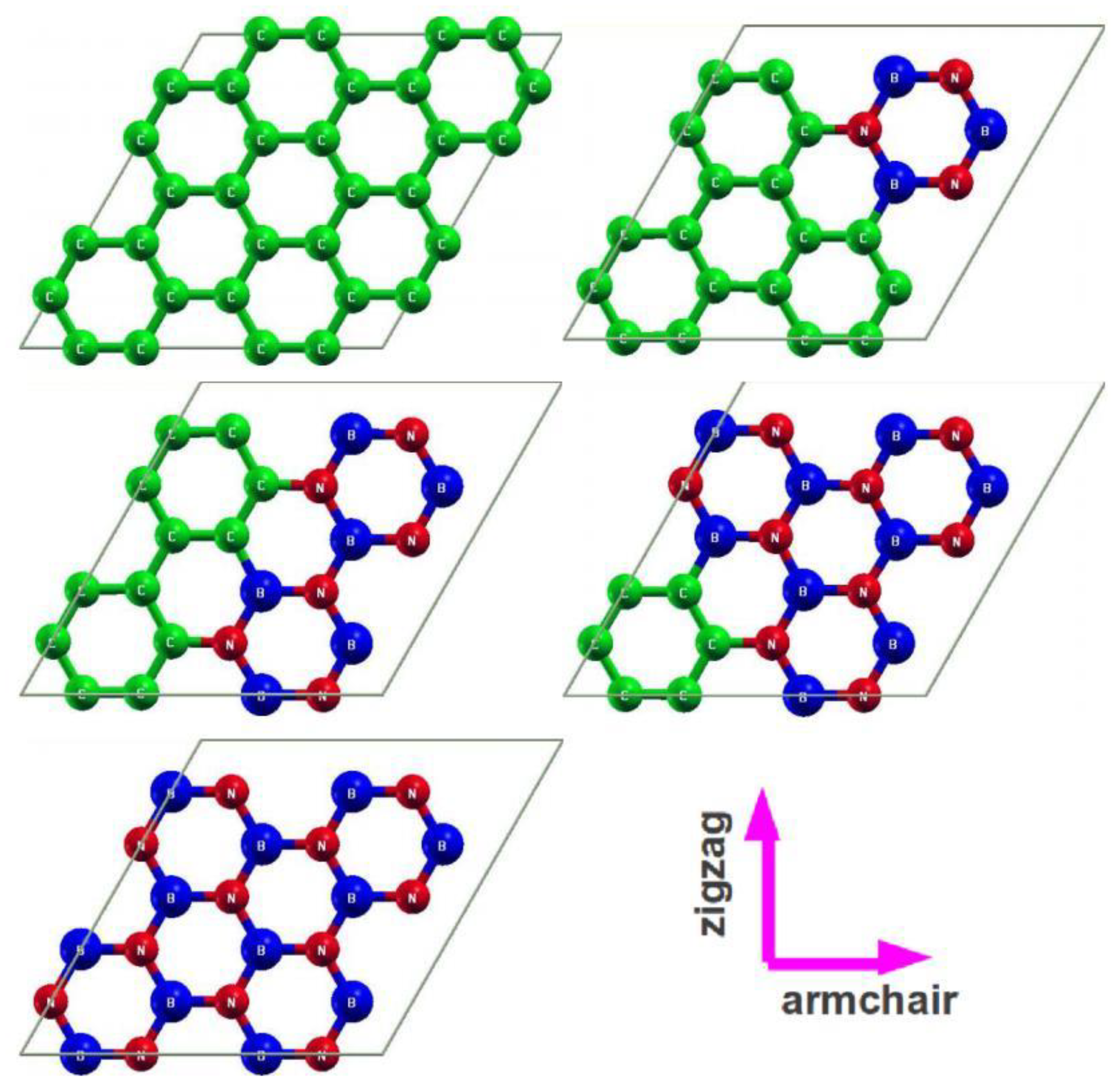
4.1. g-BNC
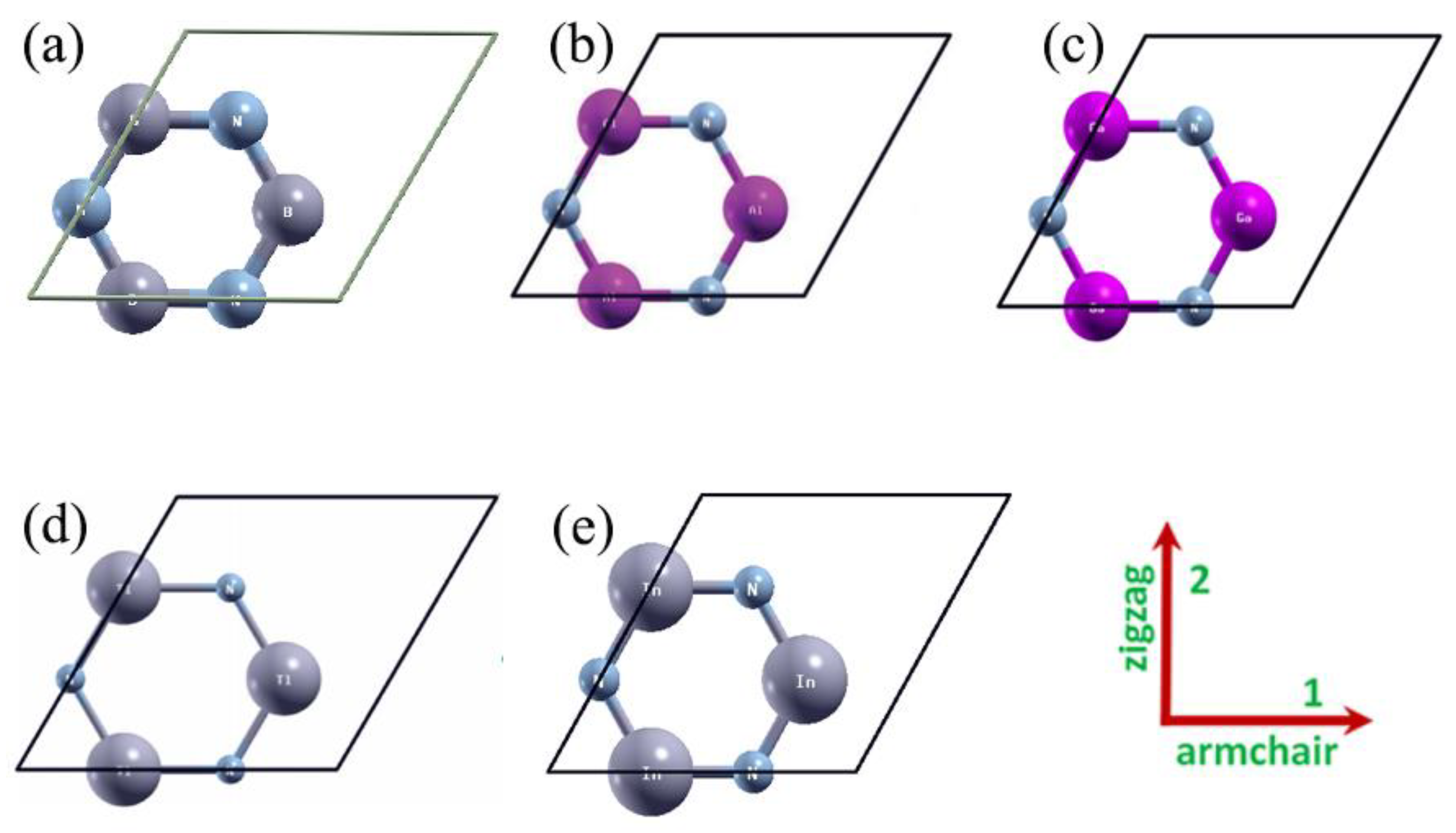
4.1.1. Geometry
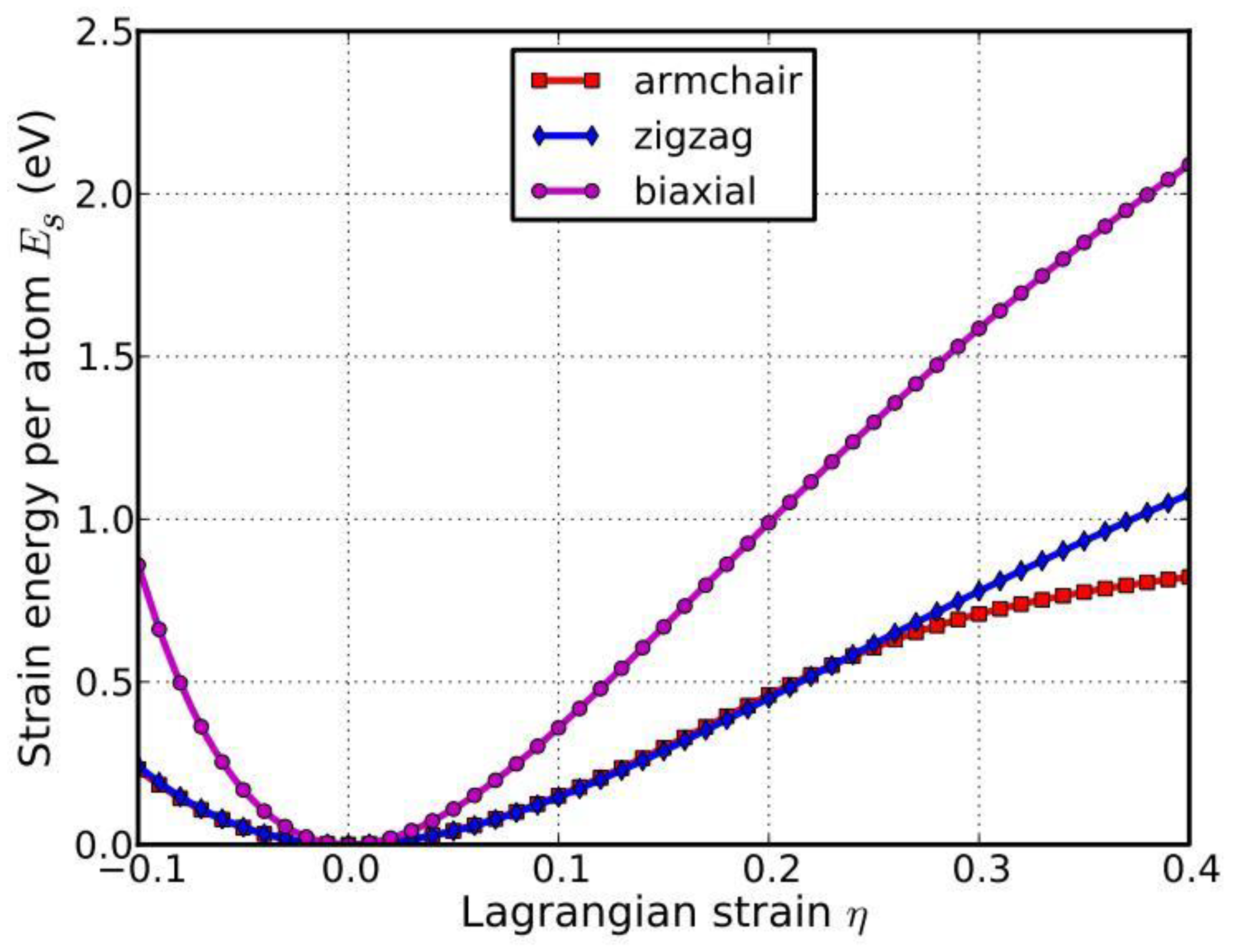
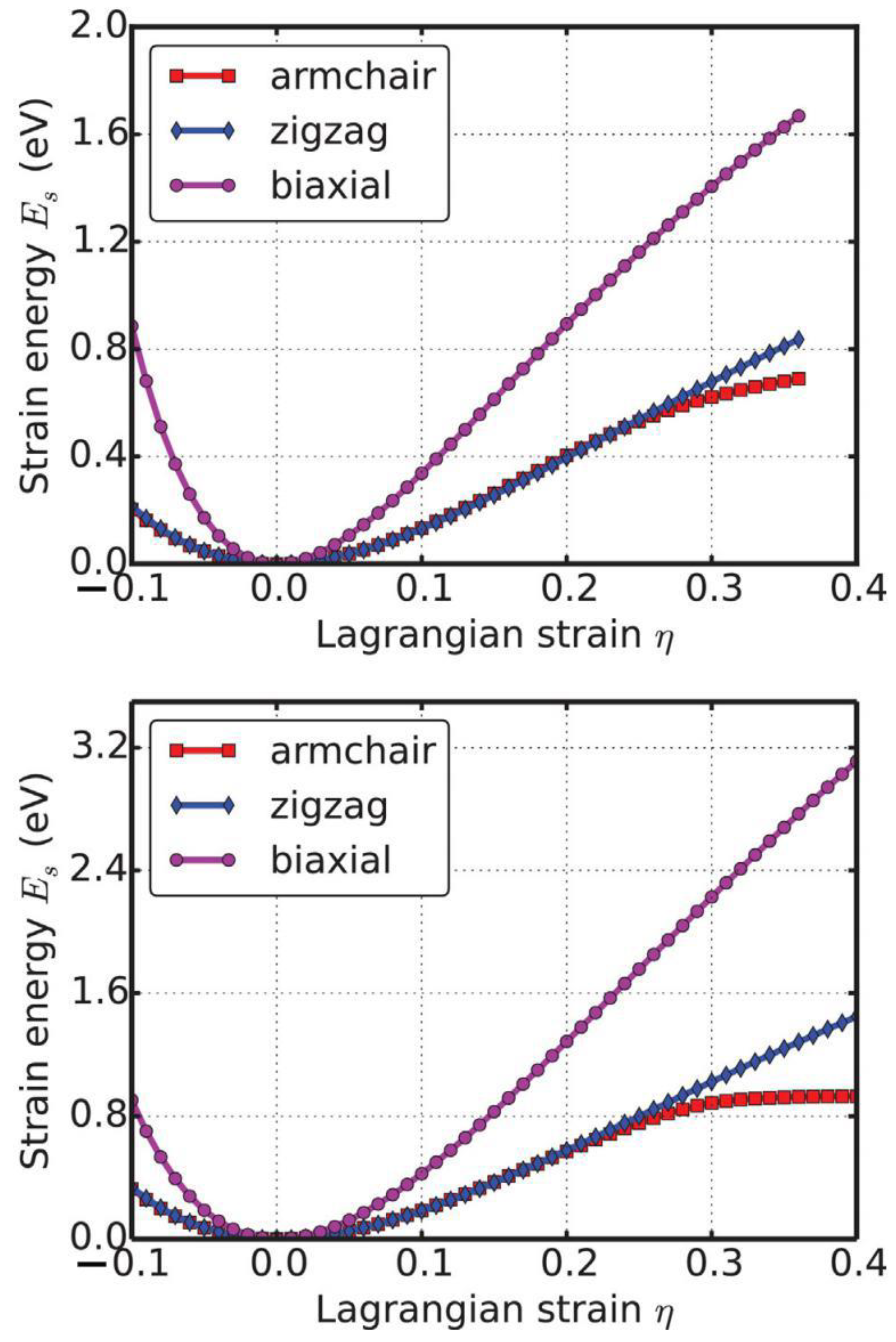
4.1.2. Strain Energy
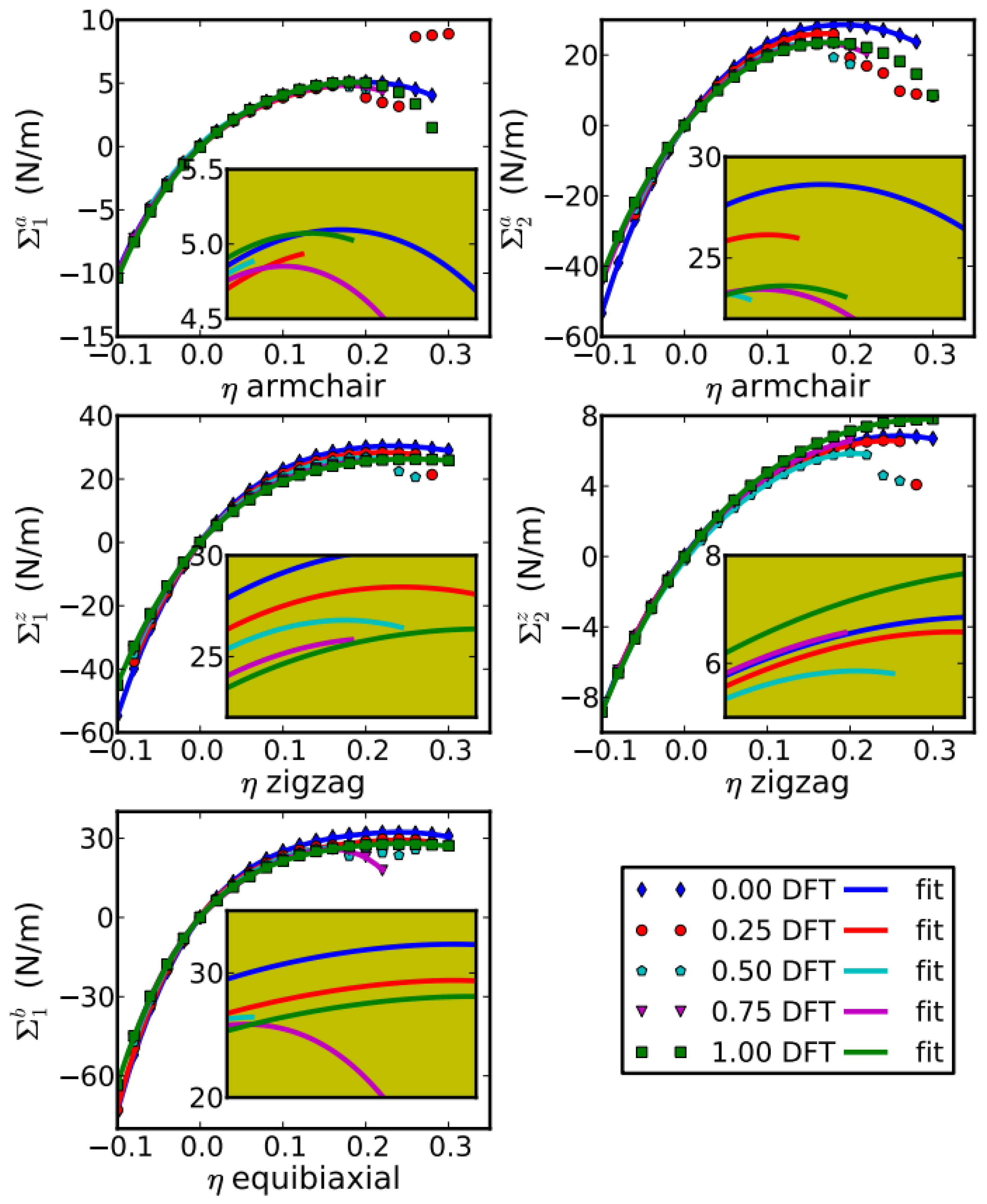
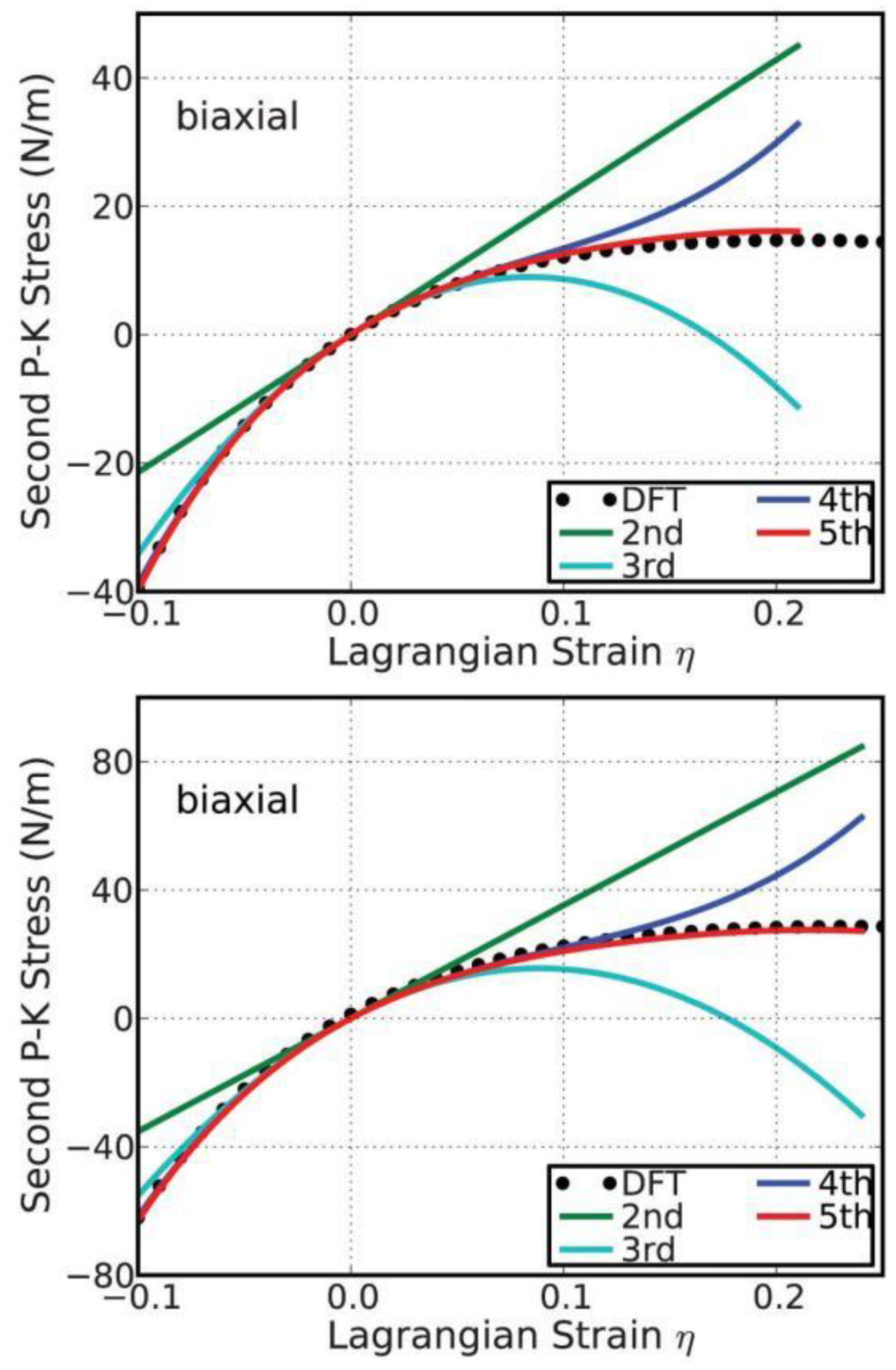
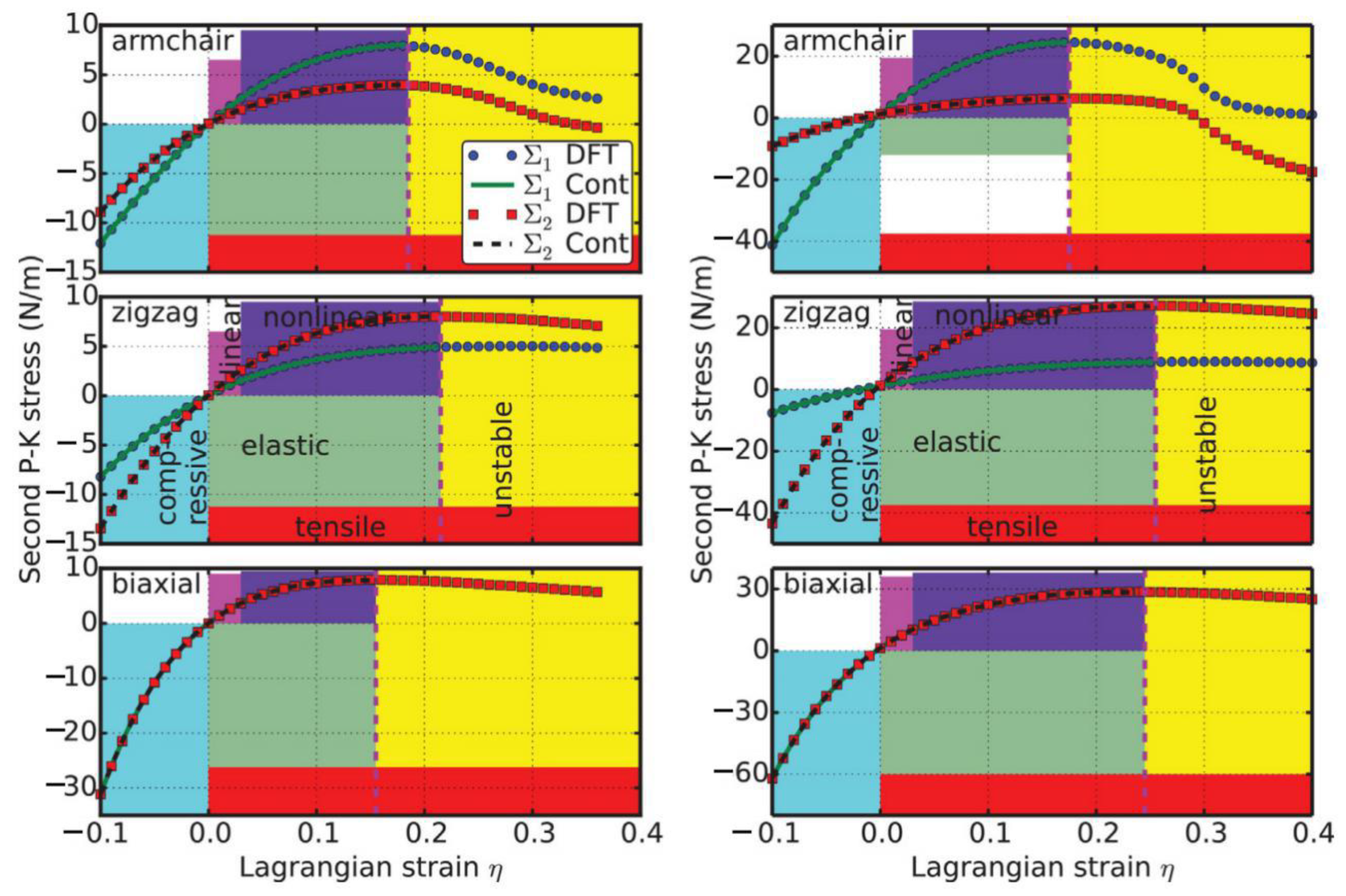
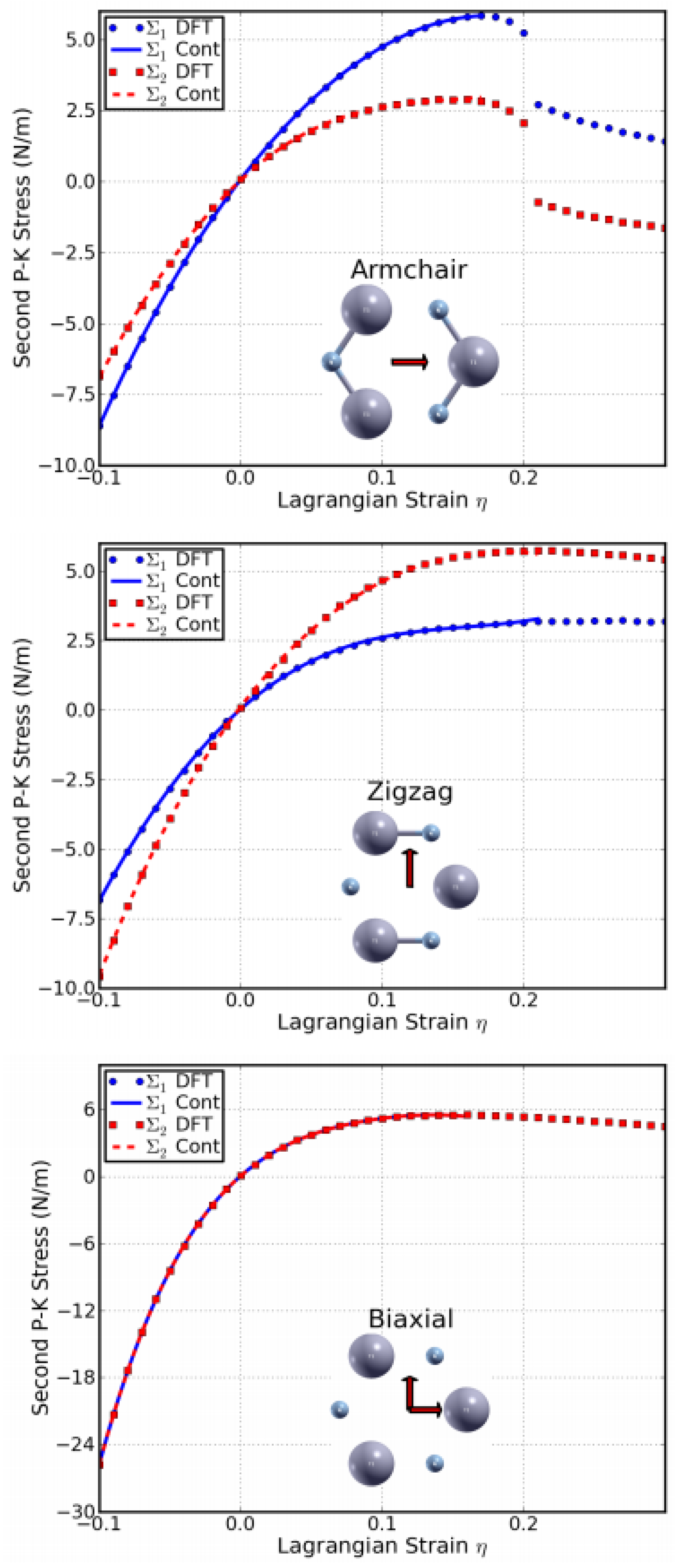
4.1.3. Stress–Strain Response
4.1.4. Elastic Constants
4.2. g-AlN
4.2.1. Atomic Structure
4.2.2. Strain Energy
4.2.3. Stress–Strain Curves
4.2.4. Elastic Constants
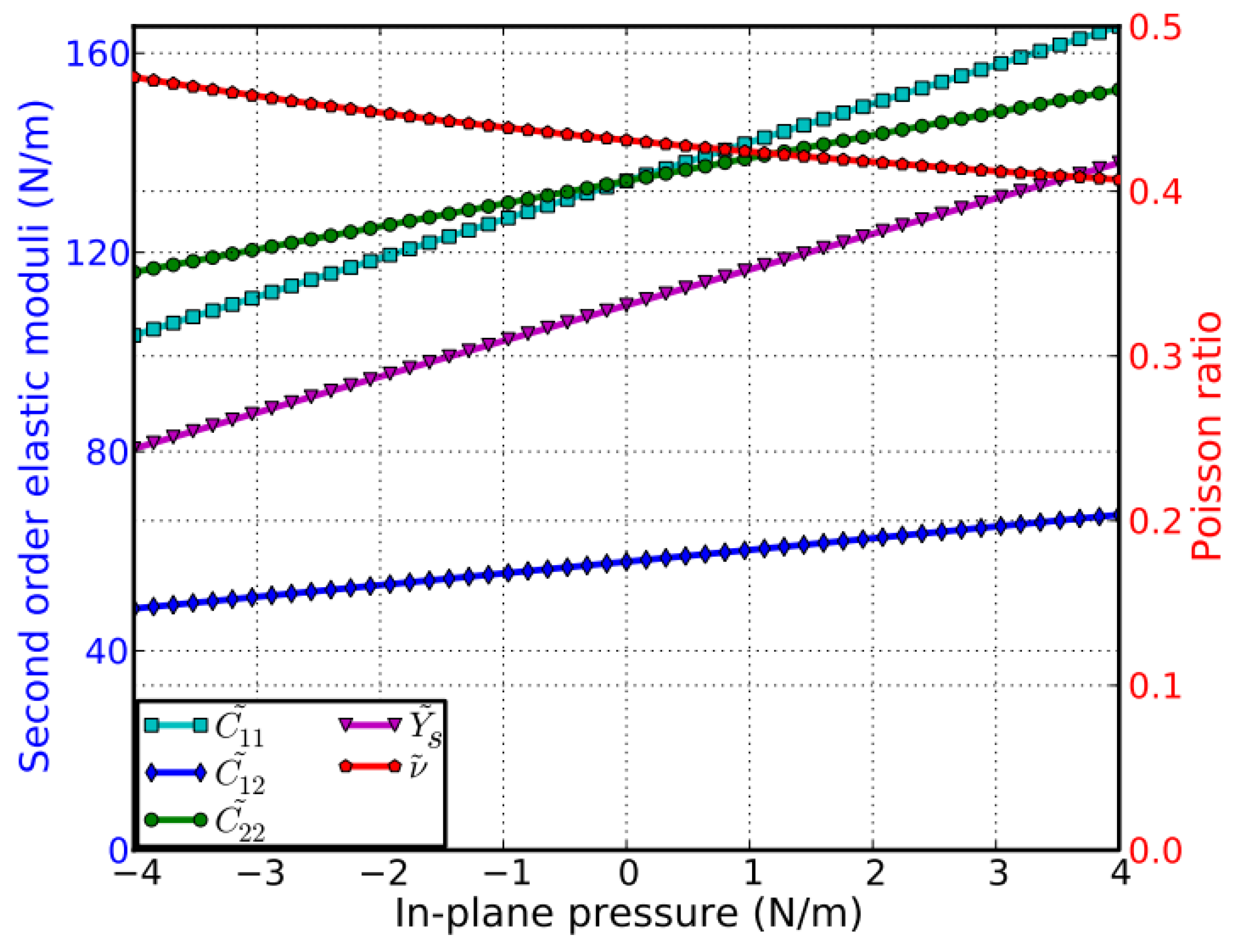
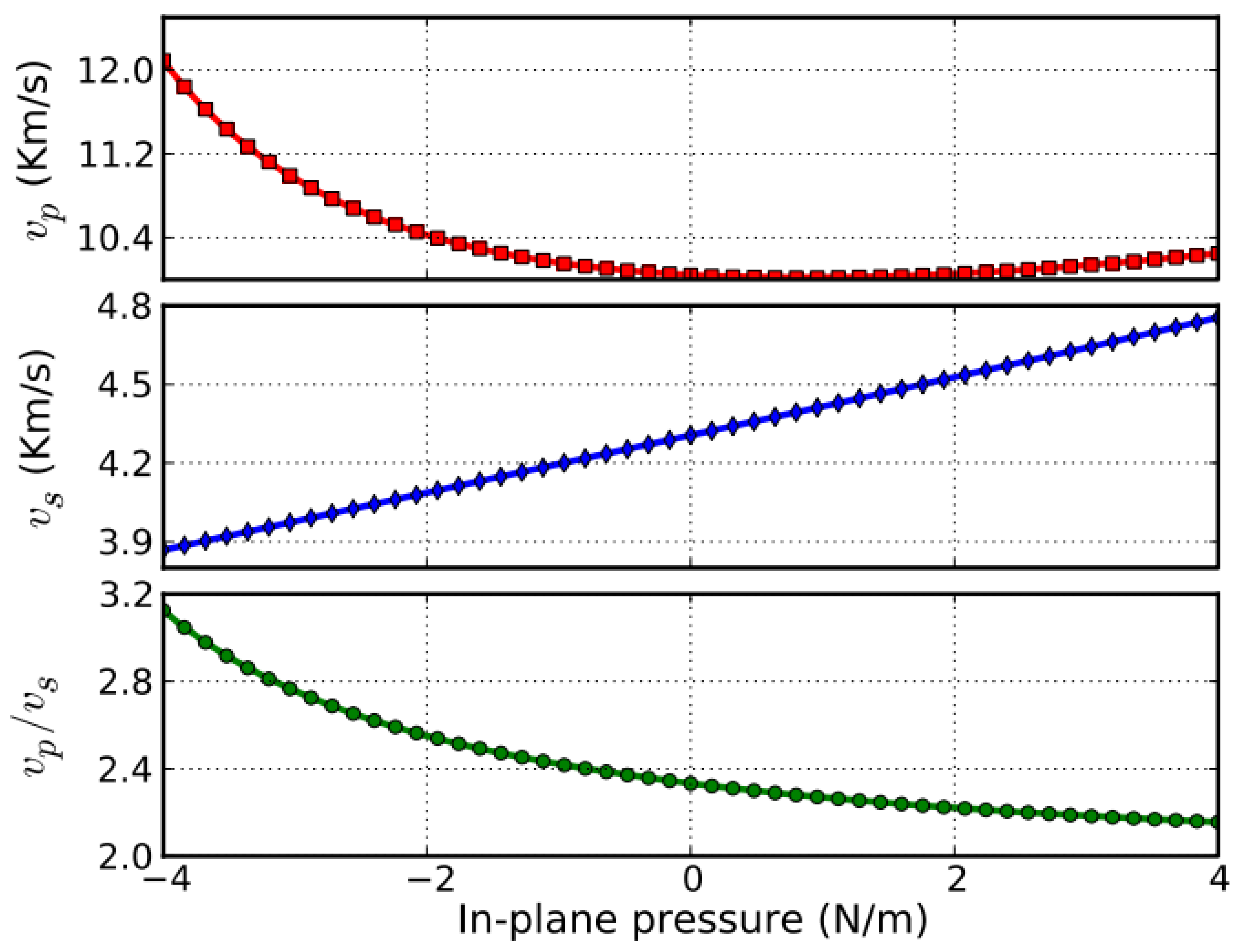
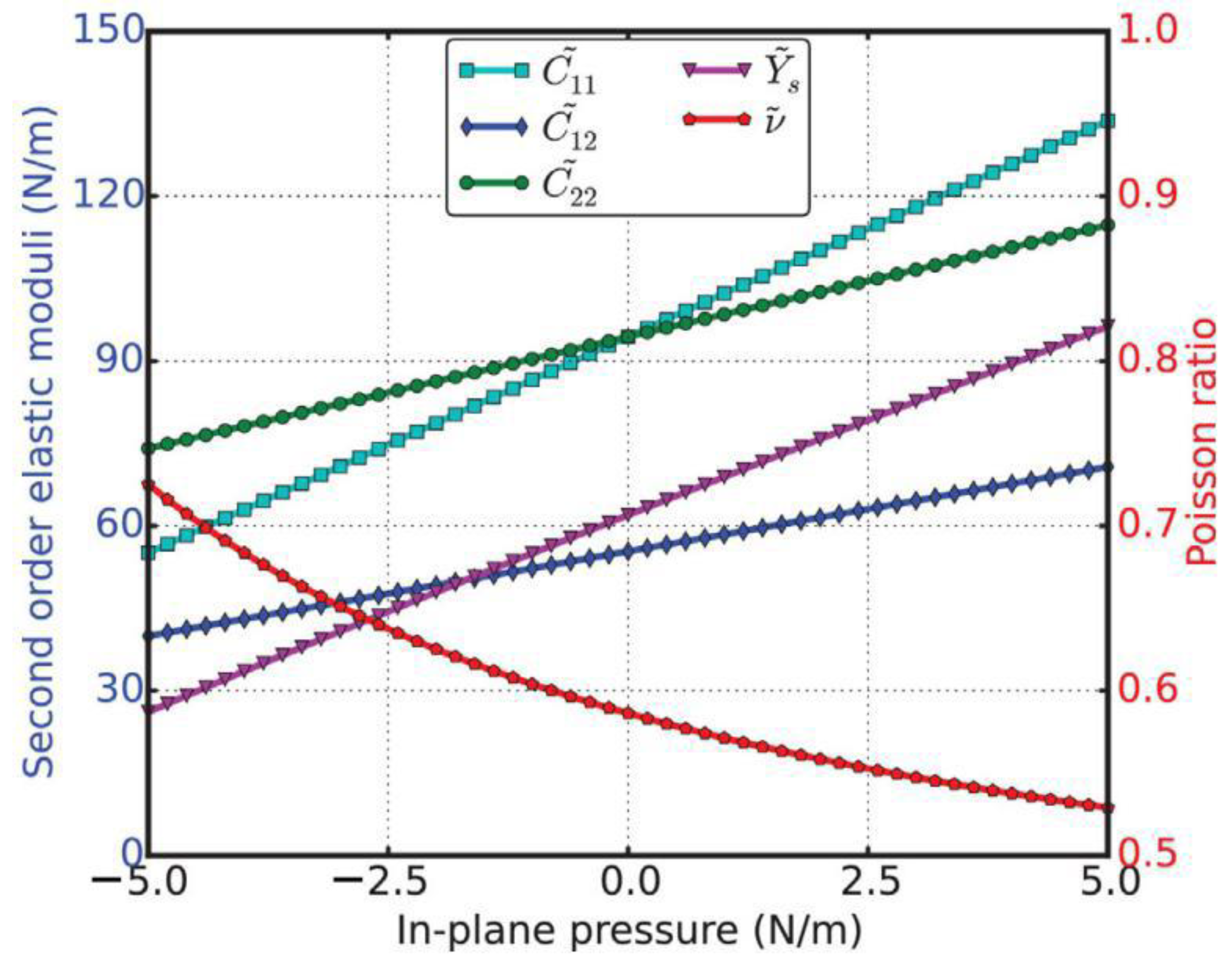
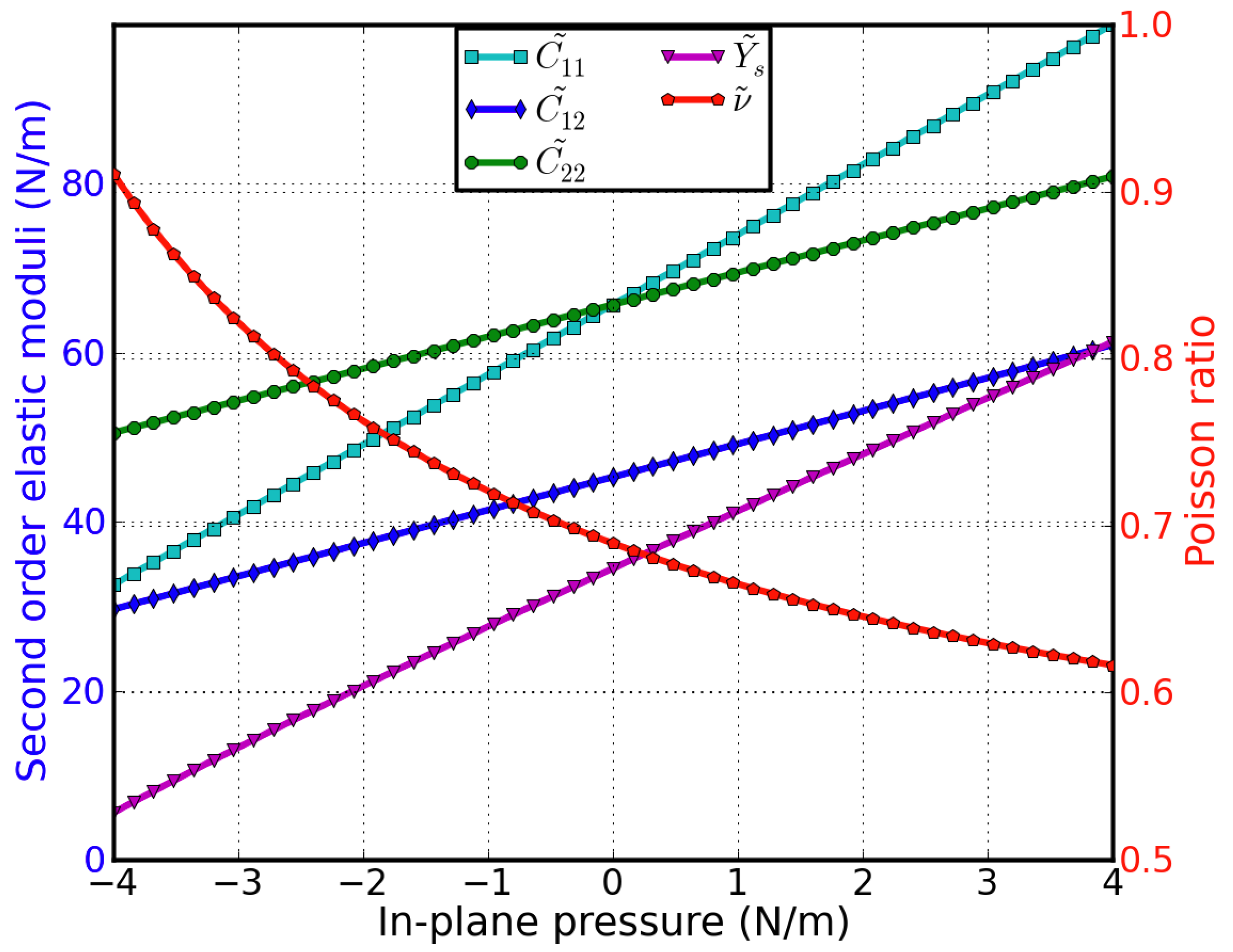
4.2.5. Pressure Effect on the Elastic Moduli
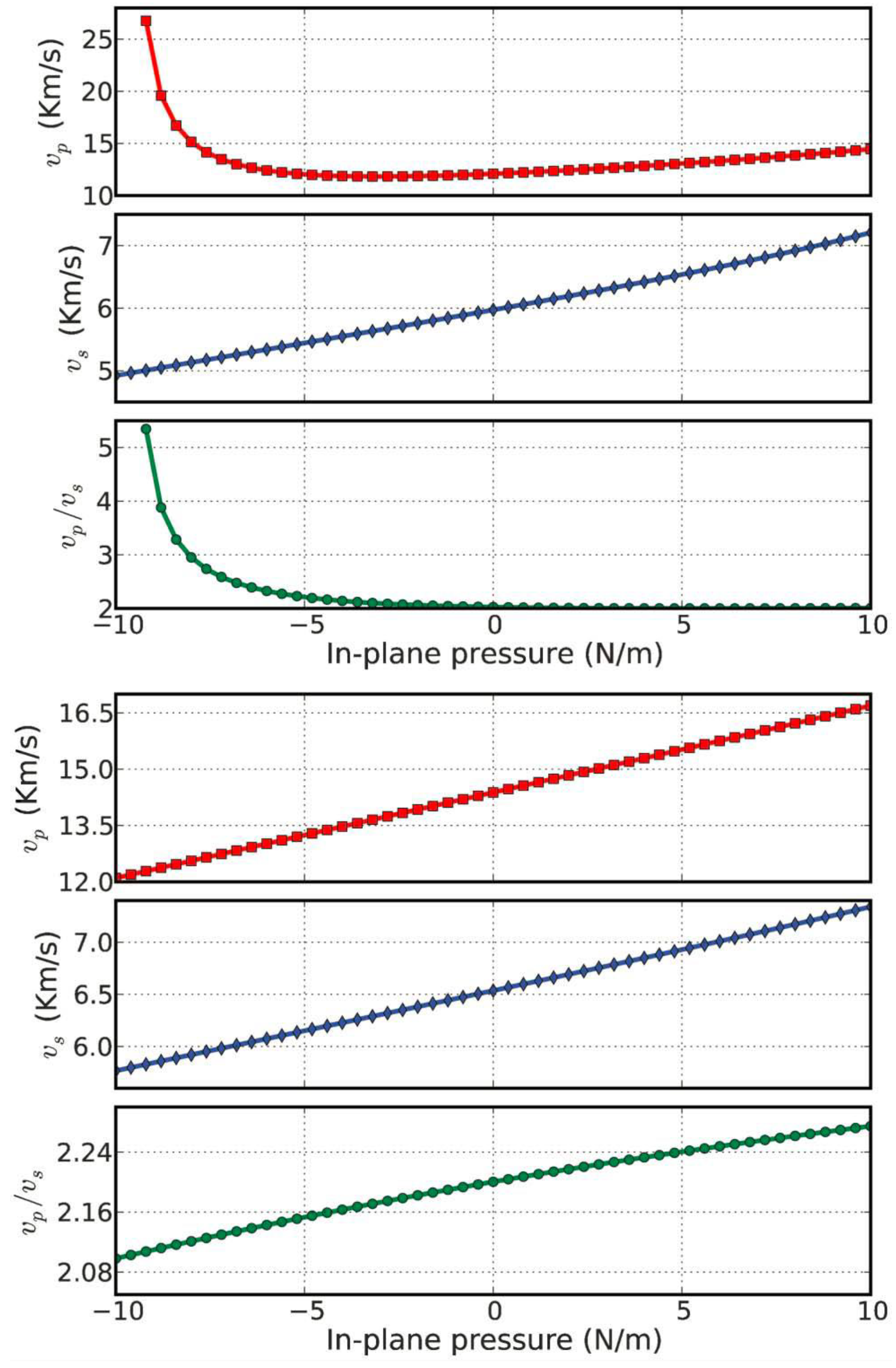
4.2.6. Pressure Effect on the Velocities of Sound
4.3. g-GaN
4.3.1. Atomic Structure
4.3.2. Strain Energy
4.3.3. Stress–Strain Curves
4.3.4. Elastic Constants
4.3.5. Effect of Pressure on the Elastic Moduli
4.3.6. Promising Applications
4.4. g-InN
4.4.1. Atomic Structure
4.4.2. Strain Energy
4.4.3. Stress–Strain Curves
4.4.4. Elastic Constants
4.4.5. Pressure Effect on the Elastic Moduli
4.4.6. Mechanical Instabilities
4.5. g-TlN
4.5.1. Atomic Structure
4.5.2. Strain Energy
4.5.3. Stress–Strain Curves
4.5.4. Elastic Constants
4.5.5. Pressure Effect on the Elastic Moduli
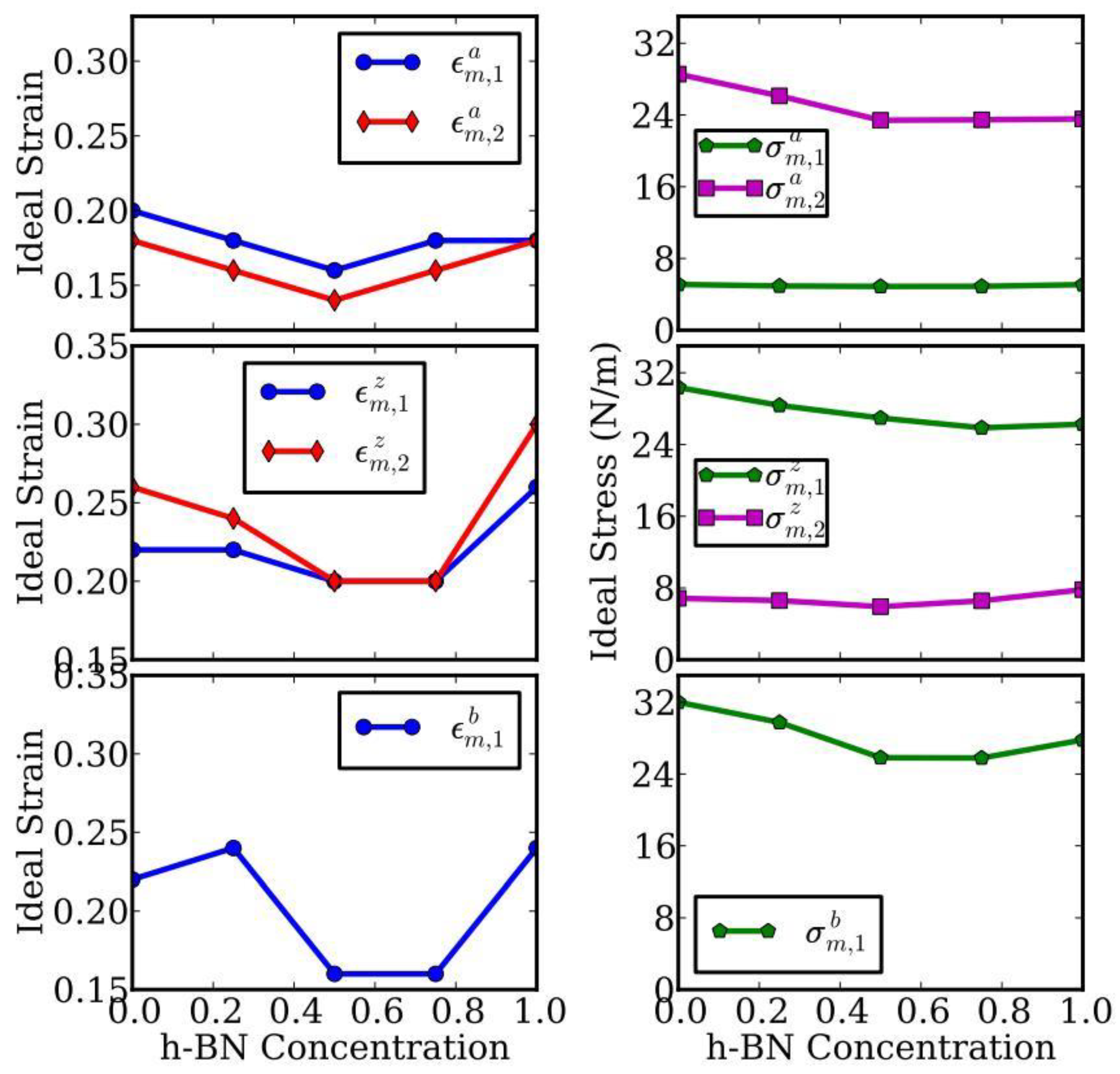
5. Discussion
6. Conclusions and Outlooks
- (I)
- With the knowledge of the ideal strength, one can predict and design the reinforcement efficiency when these 2D materials are used as one component of a composite.
- (II)
- With the knowledge of the ultimate tensile strain and stress, the upper limit of the mechanical loading is set for the practical applications of these materials and designs of nitrides.
- (III)
- With the knowledge of the elastic properties, the efficiency of the strain engineering could be predicted.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jiang, L.; Walczyk, D.; Mooney, L.; Putney, S. Manufacturing of mycelium-based biocomposites. In Proceedings of the International SAMPE Technical Conference, Long Beach, CA, USA, 6–9 May 2013; pp. 1944–1955. [Google Scholar]
- Jiang, L. A New Process for Manufacturing Biocomposite Laminate and Sandwich Parts using Mycelium as a Binder. In Proceedings of the American Society for Composites 29th Technical Conference, 16th US-Japan Conference on Composite Materials & ASTM-D30 Meeting, La Jolla, CA, USA, 8–10 September 2014; pp. 1–16. [Google Scholar]
- Jiang, L.; Walczyk, D.; McIntyre, G. Vaccum Infusion of Mycelium-bound Biocomposite Preforms with Natural Resins. In Proceedings of the Composites and Advanced Materials Expo Conference, Orlando, FL, USA, 13–16 October 2014; pp. 2797–2809. [Google Scholar]
- Béché, A.; Bougerol, C.; Cooper, D.; Daudin, B.; Rouvière, J.L. Measuring two dimensional strain state of AlN quantum dots in GaN nanowires by nanobeam electron diffraction. J. Phys. Conf. Ser. 2011, 326, 012047. [Google Scholar] [CrossRef]
- Park, H.J.; Tay, R.Y.; Wang, X.; Zhao, W.; Kim, J.H.; Ruoff, R.S.; Feng Ding, F.; Teo, E.H.T.; Zonghoon Lee, Z. Double-Spiral Hexagonal Boron Nitride and Shear Strained Coalescence Boundary. Nano Lett. 2019, 19, 4229–4236. [Google Scholar] [CrossRef] [PubMed]
- Song, L.M.; Shujuan Zhang, S.J.; Sun, S.H.; Wei, J.F.; Liu, E.M. Performance and mechanism on hydrogen evolution of two-dimensional boron nitride under mechanical vibration. Fuel 2023, 331, 125765. [Google Scholar] [CrossRef]
- Li, J.; Du, Y.H.; Mu, J.J.; Tian, Y.Y.; Yin, H.; Lv, Y.W.; Gao, L.L.; Zhang, M. Structure and property study by first-principles calculations: Twodimensional semi-hydrogenated-semi-oxidized bilayer BN (111)-oriented nanosheets. Diam. Rela. Mater. 2020, 102, 107666. [Google Scholar] [CrossRef]
- Tuoc, V.N.; Lien, L.T.H.; Huan, T.D.; Nguyen Ngoc Trung, N.N. Structural, Electronic and Mechanical Properties of Few-Layer GaN Nanosheet: A First-Principle Study. Mater. Trans. 2020, 61, 1438–1444. [Google Scholar] [CrossRef]
- Jafari, H.; Ravan, B.A.; Faghihnasiri, M. Mechanical and electronic properties of single-layer TiN and AlN under strain. Solid State Commun. 2018, 282, 21–27. [Google Scholar] [CrossRef]
- Kourra, M.H.; Sadki, K.; Drissi, L.B.; Bousmina, M. Mechanical response, thermal conductivity and phononic properties of group III-V 2D hexagonal compounds. Mater. Chem. Phys. 2021, 267, 124685. [Google Scholar] [CrossRef]
- Le, M.Q. Fracture and strength of single-atom-thick hexagonal materials. Comput. Mater. Sci. 2022, 201, 110854. [Google Scholar] [CrossRef]
- Lee, C.; Wei, X.; Kysar, J.W.; Hone, J. Measurement of the Elastic Properties and Intrinsic Strength of Monolayer Graphene. Science 2008, 321, 385–388. [Google Scholar] [CrossRef]
- Liu, F.; Ming, P.; Li, J. Ab initio calculation of ideal strength and phonon instability of graphene under tension. Phys. Rev. B 2007, 76, 064120. [Google Scholar] [CrossRef]
- Xiao, T.; Xu, X.; Liao, K. Formation mechanism of wide stacking faults in nanocrystalline Al. J. Appl. Phys. 2004, 95, 8145. [Google Scholar] [CrossRef]
- Khare, R.; Mielke, S.L.; Paci, J.T.; Zhang, S.; Ballarini, R.; Schatz, G.C.; Belytschko, T. Coupled quantum mechanical/molecular mechanical modeling of the fracture of defective carbon nanotubes and graphene sheets. Phys. Rev. B 2007, 75, 075412. [Google Scholar] [CrossRef]
- Lu, Q.; Huang, R. Nonlinear mechanics of single-atomic-layer graphene sheets. Int. J. Appl. Mech. 2009, 1, 443. [Google Scholar] [CrossRef]
- Cadelano, E.; Palla, P.L.; Giordano, S.; Colombo, L. Nonlinear Elasticity of Monolayer Graphene. Phys. Rev. Lett. 2009, 102, 235502. [Google Scholar] [CrossRef]
- Wei, X.; Fragneaud, B.; Marianetti, C.A.; Kysar, J.W. Nonlinear elastic behavior of graphene: Ab initio calculations to continuum description. Phys. Rev. B 2009, 80, 205407. [Google Scholar] [CrossRef]
- Hiki, Y. Higher Order Elastic Constants of Solids. Annu. Rev. Mater. Sci. 1981, 11, 51–73. [Google Scholar] [CrossRef]
- Ravindran, P.; Vidya, R.; Vajeeston, P.; Kjekshus, A.; Fjellvåg, H. Ground- and excited-state properties of inorganic solids from full-potential density-functional calculations. J. Solid State Chem. 2003, 176, 338–374. [Google Scholar] [CrossRef]
- Crisfield, M.A. Non-Linear Finite Element Analysis of Solids and Structures, 2nd ed.; John Wiley & Sons, Ltd.: Chichester, UK, 1991. [Google Scholar]
- Brugger, K. Thermodynamic Definition of Higher Order Elastic Coefficients. Phys. Rev. 1964, 133, A1611–A1612. [Google Scholar] [CrossRef]
- Nye, J.F. Physical Properties of Crystals. Math. Gaz. 1958, 43, 329–330. [Google Scholar]
- Zhou, J.; Huang, R. Internal lattice relaxation of single-layer graphene under in-plane deformation. J. Mech. Phys. Solids. 2008, 56, 1609–1623. [Google Scholar] [CrossRef]
- Wang, R.; Wang, S.; Wu, X.; Liang, X. First-principles calculations on third-order elastic constants and internal relaxation for monolayer graphene. Phys. Rev. B Condens. Matter 2010, 405, 3501–3506. [Google Scholar] [CrossRef]
- Marianetti, C.A.; Yevick, H.G. Failure Mechanisms of Graphene under Tension. Phys. Rev. Lett. 2010, 105, 245502. [Google Scholar] [CrossRef] [PubMed]
- Peng, Q.; De, S. Tunable band gaps of mono-layer hexagonal BNC heterostructures. Phys. E. 2012, 44, 1662–1666. [Google Scholar] [CrossRef]
- Peng, Q.; Zamiri, A.R.; Ji, W.; De, S. Elastic properties of hybrid graphene/boron nitride monolayer. Acta Mechanica. 2012, 223, 2591–2596. [Google Scholar] [CrossRef]
- Kresse, G.; Hafner, J. Ab initio molecular dynamics for liquid metals. Phys. Rev. B 1993, 47, 558–561. [Google Scholar] [CrossRef]
- Kresse, G.; Hafner, J. Ab initio molecular-dynamics simulation of the liquid-metalamorphous- semiconductor transition in germanium. Phys. Rev. B 1994, 49, 14251–14269. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comput. Mater. Sci. 1996, 6, 15–50. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B Condens. Matter. 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Inhomogeneous electron gas. Phys. Rev. 1964, 136, B864–B871. [Google Scholar] [CrossRef]
- Kohn, W.; Sham, L.J. Self-consistent equations including exchange and correlation effects. Phys. Rev. 1965, 140, A1133–A1138. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953–17979. [Google Scholar] [CrossRef] [PubMed]
- Jones, R.O.; Gunnarsson, O. The density functional formalism, its applications and prospects. Rev. Mod. Phys. 1989, 61, 689–746. [Google Scholar] [CrossRef]
- Peng, Q.; Ji, W.; De, S. Mechanical properties of the hexagonal boron nitride monolayer: Ab initio study. Comput. Mater. Sci. 2012, 56, 11–17. [Google Scholar] [CrossRef]
- Peng, Q.; Ji, W.; De, S. Mechanical properties of graphyne monolayers: A first-principles study. Phys. Chem. Chem. Phys. 2012, 14, 13385–13391. [Google Scholar] [CrossRef]
- Peng, Q.; Liang, C.; Ji, W.; De, S. A First Principles Investigation of the Mechanical Properties of g-TlN. Model. Numer. Simul. Mater. Sci. 2012, 2, 76–84. [Google Scholar] [CrossRef][Green Version]
- Peng, Q.; Liang, C.; Ji, W.; De, S. A first principles investigation of the mechanical properties of g-ZnO: The graphene-like hexagonal zinc oxide monolayer. Comput. Mater. Sci. 2013, 68, 320–324. [Google Scholar] [CrossRef]
- Ci, L.; Song, L.; Jin, C.; Jariwala, D.; Wu, D.; Li, Y.; Srivastava, A.; Wang, Z.F.; Storr, K.; Balicas, L.; et al. Atomic layers of hybridized boron nitride and graphene domains. Nat. Mater. 2010, 9, 430–435. [Google Scholar] [CrossRef]
- Peng, Q.; Liang, C.; Ji, W.; De, S. A theoretical analysis of the effect of the hydrogenation of graphene to graphane on its mechanical properties. Phys. Chem. Chem. Phys. 2013, 15, 2003–2011. [Google Scholar] [CrossRef]
- Peng, Q.; Liang, C.; Ji, W.; De, S. Mechanical properties of g-GaN: A first principles study. Appl. Phys. A-Mater. 2013, 113, 483–490. [Google Scholar] [CrossRef]
- Peng, Q.; Liang, C.; Ji, W.; De, S. A first-principles study of the mechanical properties of g-GeC. Mech. Mater. 2013, 64, 135–141. [Google Scholar] [CrossRef]
- Peng, Q.; Chen, X.J.; Ji, W.; De, S. Chemically tuning mechanics of graphene by BN. Adv. Eng. Mater. 2013, 15, 718–727. [Google Scholar] [CrossRef]
- Peng, Q.; Crean, J.; Dearden, A.K.; Huang, C.; Wen, X.; Bordas, S.P.A.; De, S. Defect engineering of 2D monatomic-layer materials. Mod. Phys. Lett. B 2013, 27, 1330017. [Google Scholar] [CrossRef]
- Peng, Q.; De, S. Elastic limit of silicane. Nanoscale 2014, 6, 12071–12079. [Google Scholar] [CrossRef] [PubMed]
- Peng, Q.; Ji, W.; De, S. First-principles study of the effects of mechanical strains on the radiation hardness of hexagonal boron nitride monolayers. Nanoscale 2013, 5, 695–703. [Google Scholar] [CrossRef]
- Peng, Q.; De, S. Mechanical properties and instabilities of ordered graphene oxide C6O monolayers. RSC Adv. 2013, 3, 24337–24344. [Google Scholar] [CrossRef]
- Peng, Q.; Han, L.; Lian, J.; Wen, X.; Liu, S.; Chen, Z.; Koratkar, N.; De, S. Mechanical degradation of graphene by epoxidation: Insights from first-principles calculations. Phys. Chem. Chem. Phys. 2015, 17, 19484–19490. [Google Scholar] [CrossRef]
- Peng, Q.; Han, L.; Wen, X.; Liu, S.; Chen, Z.; Lian, J.; De, S. Mechanical properties and stabilities of α-boron monolayers. Phys. Chem. Chem. Phys. 2015, 17, 2160–2168. [Google Scholar] [CrossRef]
- Zhang, Z.; Xie, Y.; Peng, Q.; Chen, Y. Geometry, stability and thermal transport of hydrogenated graphene nanoquilts. Solid State Commun. 2015, 213–214, 31–36. [Google Scholar] [CrossRef][Green Version]
- Peng, Q.; Han, L.; Wen, X.D.; Liu, S.; Chen, Z.F.; Lian, J.; De, S. Mechanical properties and stabilities of g-ZnS monolayers. RSC Adv. 2015, 5, 11240–11247. [Google Scholar] [CrossRef]
- Peng, Q.; Chen, X.J.; Liu, S.; De, S. Mechanical stabilities and properties of graphene-like aluminum nitride predicted from first-principles calculations. RSC Adv. 2013, 3, 7083–7092. [Google Scholar] [CrossRef]
- Peng, Q.; Wen, X.D.; De, S. Mechanical stabilities of silicene. RSC ADV 2013, 3, 13772–13781. [Google Scholar] [CrossRef]
- Peng, Q.; Dearden, A.K.; Lian, J.; Han, L.; Liu, S.; Wen, X.D.; De, S. New materials graphyne, graphdiyne, graphone, and graphane: Review of properties, synthesis, and application in nanotechnology. Nanotechnol. Sci. Appl. 2014, 7, 1–29. [Google Scholar] [CrossRef] [PubMed]
- Peng, Q.; De, S. Outstanding mechanical properties of monolayer MoS2 and its application in elastic energy storage. Phys. Chem. Chem. Phys. 2013, 15, 19427–19437. [Google Scholar] [CrossRef] [PubMed]
- Peng, Q.; Dearden, A.K.; Chen, X.J.; Huang, C.; Wen, X.; De, S. Peculiar pressure effect on Poisson ratio of graphone as a strain damper. Nanoscale 2015, 7, 9975–9979. [Google Scholar] [CrossRef]
- Peng, Q.; Wang, G.; Liu, G.R.; De, S. Structures, mechanical properties, equations of state, and electronic properties of β-HMX under hydrostatic pressures: A DFT-D2 study. Phys. Chem. Chem. Phys. 2014, 16, 19972–19983. [Google Scholar] [CrossRef]
- Zhang, Z.; Xie, Y.; Peng, Q.; Chen, Y. Thermal transport in MoS2/Graphene hybrid nanosheets. Nanotechnology 2015, 26, 375402. [Google Scholar] [CrossRef]
- Peng, Q.; Chen, Z.; De, S. A Density Functional Theory Study of the Mechanical Properties of Graphane with van der Waals Corrections. Mech. Adv. Mater. Struct. 2015, 22, 717–721. [Google Scholar] [CrossRef]
- Peng, Q.; Xin Sun, X.; Wang, H.; Yunbo Yang, Y.B.; Wen, X.D.; Huang, C.; Liu, S.; De, S. Theoretical prediction of a graphene-like structure of indium nitride: A promising excellent material for optoelectronics. Appl. Mater. Today 2017, 7, 169–178. [Google Scholar] [CrossRef]
- Liu, L.; Feng, Y.P.; Shen, Z.X. Structural and electronic properties of h-BN. Phys. Rev. B 2003, 68, 104102. [Google Scholar] [CrossRef]
- Baskin, Y.; Meyer, L. Lattice Constants of Graphite at Low Temperatures. Phys. Rev. 1955, 100, 544. [Google Scholar] [CrossRef]
- Peng, Q.; Wang, G.; Liu, G.R.; De, S. Van der Waals Density Functional Theory vdW-DFq for Semihard Materials. Crystals 2019, 9, 243. [Google Scholar] [CrossRef]
- Topsakal, M.; Cahangirov, S.; Ciraci, S. The response of mechanical and electronic properties of graphane to the elastic strain. Appl. Phys. Lett. 2010, 96, 091912. [Google Scholar] [CrossRef]
- Kudin, K.N.; Scuseria, G.E.; Yakobson, B.I. C2F, BN, and C nanoshell elasticity from ab initio computations. Phys. Rev. B 2001, 64, 235406. [Google Scholar] [CrossRef]
- Hernández, E.; Goze, C.; Bernier, P.; Rubio, A. Elastic Properties of C and BxCyNz Composite Nanotubes. Phys. Rev. Lett. 1998, 80, 4502–4505. [Google Scholar] [CrossRef]
- Greaves, G.N.; Greer, A.L.; Lakes, R.S.; Rouxel, T. Author Correction: Poisson’s ratio and modern materials. Nat. Mater. 2019, 18, 406. [Google Scholar] [CrossRef]
- Davydov, S.Y. Third-order elastic moduli of single-layer graphene. Phys. Solid State 2011, 53, 665–668. [Google Scholar] [CrossRef]
- Topsakal, M.; Aktürk, E.; Ciraci, S. First-principles study of two- and one-dimensional honeycomb structures of boron nitride. Phys. Rev. B 2009, 79, 115442. [Google Scholar] [CrossRef]
- Şahin, H.; Cahangirov, S.; Topsakal, M.; Bekaroglu, E.; Akturk, E.; Senger, R.T.; Ciraci, S. Monolayer honeycomb structures of group-IV elements and III-V binary compounds: First-principles calculations. Phys. Rev. B 2009, 80, 155453. [Google Scholar] [CrossRef]
- de Almeida, E.F.; de Brito Mota, F.; de Castilho, C.M.C.; Kakanakova-Georgieva, A.; Gueorguiev, G.K. Defects in hexagonal-AlN sheets by first-principles calculations. Eur. Phys. J. B 2012, 85, 48. [Google Scholar] [CrossRef]
- Wang, Y.; Shi, S. Structural and electronic properties of monolayer hydrogenated honeycomb III–V sheets from first-principles. Solid State Commun. 2010, 150, 1473–1478. [Google Scholar] [CrossRef]
- Zheng, F.-L.; Zhang, J.-M.; Zhang, Y.; Ji, V. First-principles study of the perfect and vacancy defect AlN nanoribbon. Phys. Rev. B Condens. Matter. 2010, 405, 3775–3781. [Google Scholar] [CrossRef]
- Hou, S.M.; Zhang, J.X.; Shen, Z.Y.; Zhao, X.Y.; Xue, Z.Q. First-principles calculations on the open end of single-walled AlN nanotubes. Phys. E. 2005, 27, 45–50. [Google Scholar] [CrossRef]
- Cerda, E.; Mahadevan, L. Geometry and Physics of Wrinkling. Phys. Rev. Lett. 2003, 90, 074302. [Google Scholar] [CrossRef] [PubMed]
- Bao, W.; Miao, F.; Chen, Z.; Zhang, H.; Jang, W.; Dames, C.; Lau, C.N. Controlled ripple texturing of suspended graphene and ultrathin graphite membranes. Nat. Nanotechnol. 2009, 4, 562–566. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, F. Maximum asymmetry in strain induced mechanical instability of graphene: Compression versus tension. Appl. Phys. Lett. 2011, 99, 241908. [Google Scholar] [CrossRef]
- Tsoukleri, G.; Parthenios, J.; Papagelis, K.; Jalil, R.; Ferrari, A.C.; Geim, A.K.; Novoselov, K.S.; Galiotis, C. Subjecting a Graphene Monolayer to Tension and Compression. Small 2009, 5, 2397–2402. [Google Scholar] [CrossRef]
- Yoon, D.; Son, Y.-W.; Cheong, H. Negative Thermal Expansion Coefficient of Graphene Measured by Raman Spectroscopy. Nano Lett. 2011, 11, 3227–3231. [Google Scholar] [CrossRef]
- Pan, W.; Xiao, J.; Zhu, J.; Yu, C.; Zhang, G.; Ni, Z.; Watanabe, K.; Taniguchi, T.; Shi, Y.; Wang, X. Biaxial Compressive Strain Engineering in Graphene/Boron Nitride Heterostructures. Sci. Rep. 2012, 2, 893. [Google Scholar] [CrossRef]
- Quhe, R.; Zheng, J.; Luo, G.; Liu, Q.; Qin, R.; Zhou, J.; Yu, D.; Nagase, S.; Mei, W.-N.; Gao, Z.; et al. Tunable and sizable band gap of single-layer graphene sandwiched between hexagonal boron nitride. NPG Asia Mater. 2012, 4, e6. [Google Scholar] [CrossRef]
- Everest, F.A. ; The Master Handbook of Acoustics, 4th ed.; McGraw-Hill Companies: New York, NY, USA, 2001; pp. 41–79. [Google Scholar]
- Kipshidze, D.G.; Schenk, H.P.; Fissel, A.; Kaiser, U.; Schulze, J.; Richter, W.; Weihnacht, M.; Kunze, R.; Kräusslich, J. Molecular-beam epitaxy of a strongly lattice-mismatched heterosystem AlN/Si(111) for application in SAW devices. Semiconductors 1999, 33, 1241–1246. [Google Scholar] [CrossRef]
- Benes, E.; Groschl, M.; Seifert, F.; Pohl, A. Comparison between BAW and SAW sensor principles. IEEE Trans. Sonics Ultrason. 1998, 45, 1314–1330. [Google Scholar] [CrossRef] [PubMed]
- Weigel, R.; Morgan, D.P.; Owens, J.M.; Ballato, A.; Lakin, K.M.; Hashimoto, K.; Ruppel, C.C.W. Microwave acoustic materials, devices, and applications. IEEE Trans. Microwave Theory Technol. 2002, 50, 738–749. [Google Scholar] [CrossRef]
- Zhuang, H.L.; Singh, A.K.; Hennig, R.G. Computational discovery of single-layer III–V materials. Phys. Rev. B 2013, 87, 165415. [Google Scholar] [CrossRef]
- Ueno, M.; Yoshida, M.; Onodera, A.; Shimomura, O.; Takemura, K. Stability of the wurtzite-type structure under high pressure: GaN and InN. Phys. Rev. B 1994, 49, 14–21. [Google Scholar] [CrossRef]
- Brugger, K. Determination of 3rd-order elastic coefficients in crystals. J. Appl. Phys. 1965, 36, 768. [Google Scholar] [CrossRef]
- Singh, A.K.; Zhuang, H.L.; Hennig, R.G. Ab initio synthesis of single-layer III–V materials. Phys. Rev. B 2014, 89, 245431. [Google Scholar] [CrossRef]
- Li, T. Ideal strength and phonon instability in single-layer MoS2. Phys. Rev. B 2012, 85, 235407. [Google Scholar] [CrossRef]
- Staszczak, G.; Gorczyca, I.; Suski, T.; Wang, X.Q.; Christensen, N.E.; Svane, A.; Dimakis, E.; Moustakas, T.D. Photoluminescence and pressure effects in short period InN/nGaN superlattices. J. Appl. Phys. 2013, 113, 123101. [Google Scholar] [CrossRef]
- Lepkowski, S.P.; Majewski, J.A.; Jurczak, G. Nonlinear elasticity in III-N compounds: Ab initio calculations. Phys. Rev. B 2005, 72, 245201. [Google Scholar] [CrossRef]
- Yan, Z. Thermal Properties of Graphene and Applications for Thermal Management of High-Power Density Electronics. Ph.D. Thesis, University of California, Riverside, CA, USA, 2013. [Google Scholar]
- Pollock, D.T.; Yang, Z.; Wen, J.T. Dryout avoidance control for multi-evaporator vapor compression cycle cooling. Appl. Energy 2015, 160, 266–285. [Google Scholar] [CrossRef]
- Yang, Z.; Pollock, D.T.; Wen, J.T. Gain-scheduling control of vapor compression cycle for transient heat-flux removal. Control. Eng. Pract. 2015, 39, 67–89. [Google Scholar] [CrossRef]
- Yang, Z.; Pollock, D.T.; Wen, J.T. Multivariable Control of Vapor Compression Cycle With Transient Heat Flux. In Proceedings of the ASME 2013 International Mechanical Engineering Congress and Exposition Conference, San Diego, CA, USA, 15–21 November 2013. IMECE2013-63610. [Google Scholar]
- Pollock, D.T.; Yang, Z.; Wen, J.T.; Peles, Y.; Jensen, M.K. Model-based control of vapor compression cycles for transient heat-flux removal. Int. J. Heat Mass Transfer. 2014, 77, 662–683. [Google Scholar] [CrossRef]
- Pollock, D.T.; Yang, Z.; Wen, J.T. Dryout Avoidance Control for Multi-Evaporator Vapor Compression Cycles With Transient Heat Flux. In Proceedings of the ASME 2014 International Mechanical Engineering Congress and Exposition Conference, Montreal, QC, Canada, 14–20 November 2014. IMECE2014-39398. [Google Scholar]
- Pollock, D.T.; Yang, Z.; Wen, J.T.; Peles, Y.; Jensen, M.K. Modeling and control of single and multiple evaporator vapor compression cycles for electronics cooling. In Proceedings of the American Control Conference, Washington, DC, USA, 17–19 June 2013; p. 6580071. [Google Scholar]
- Zheng, F.F.; Xiao, X.; Xie, J.; Zhou, L.J.; Li, Y.Y.; Dong, H.L. Structures, properties and applications of two-dimensional metal nitrides: From nitride MXene to other metal nitrides. 2D Mater. 2022, 9, 022001. [Google Scholar] [CrossRef]

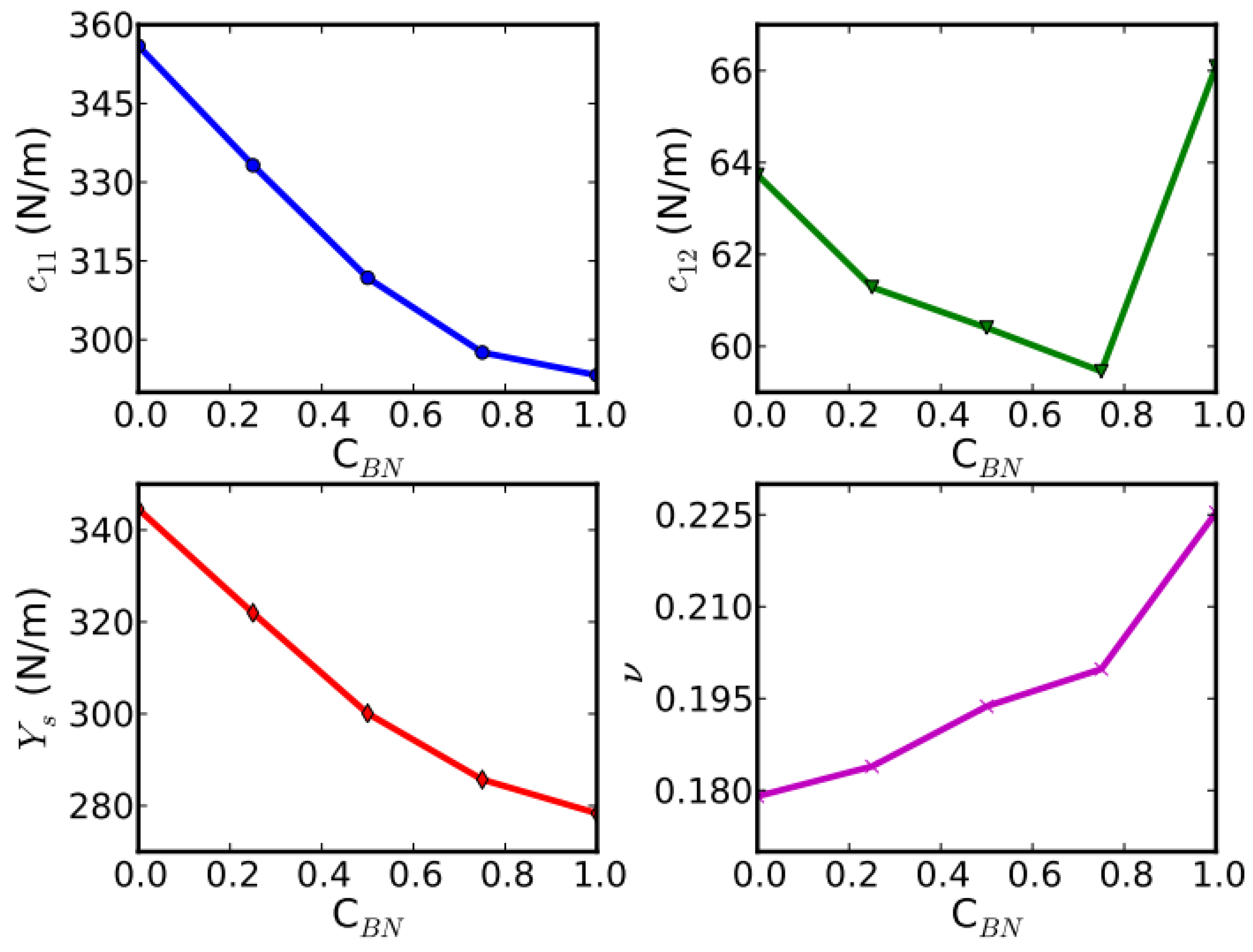
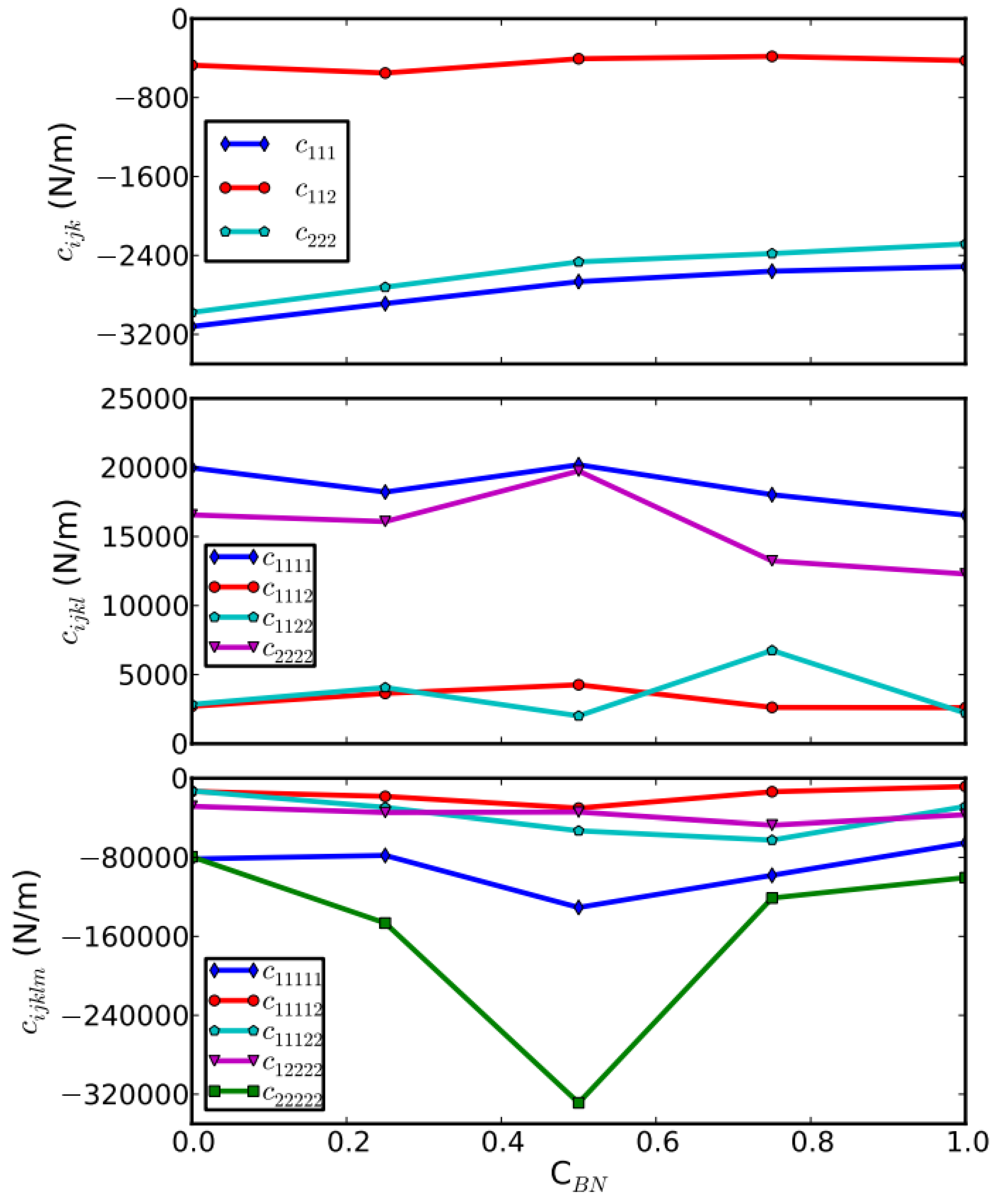

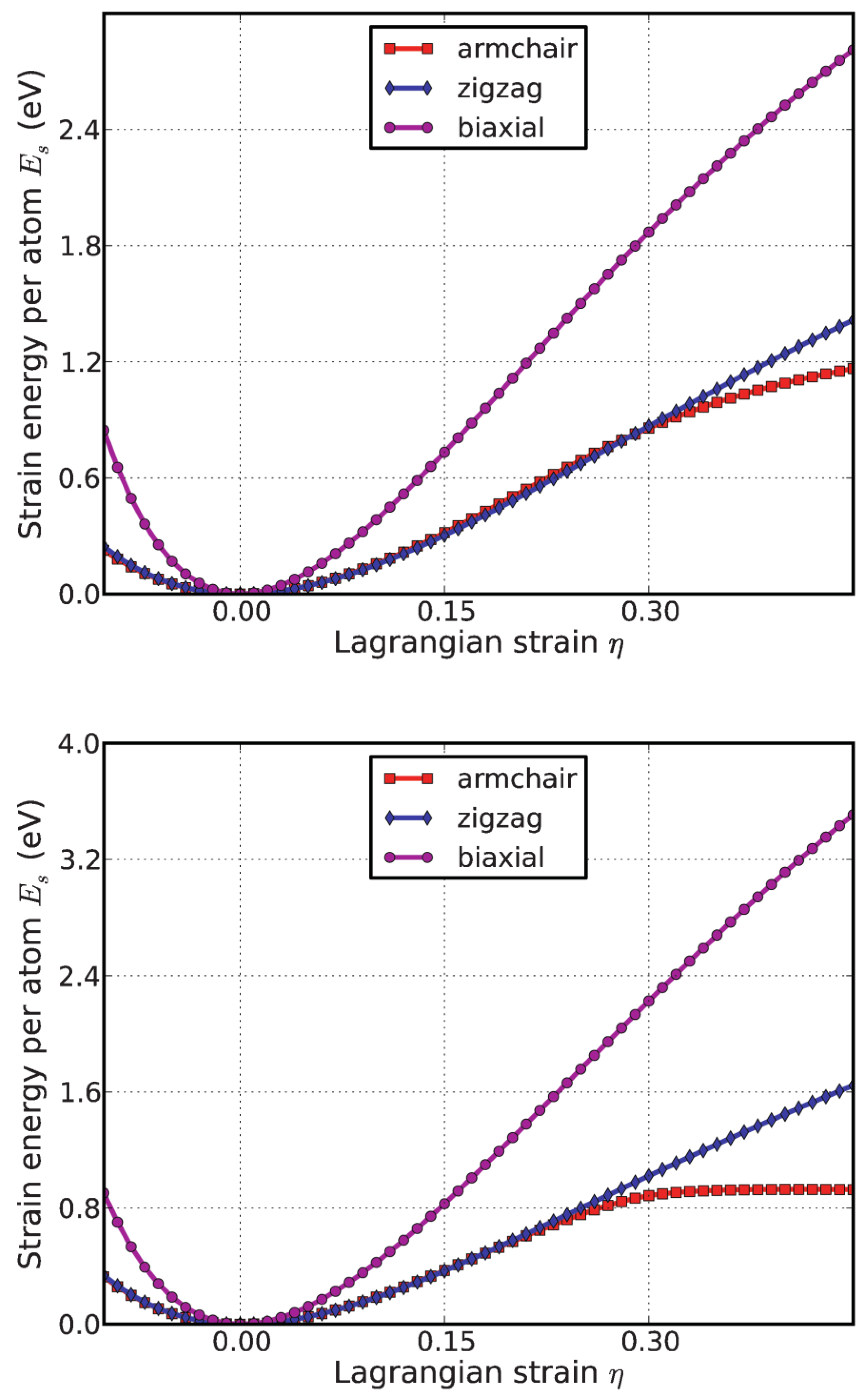
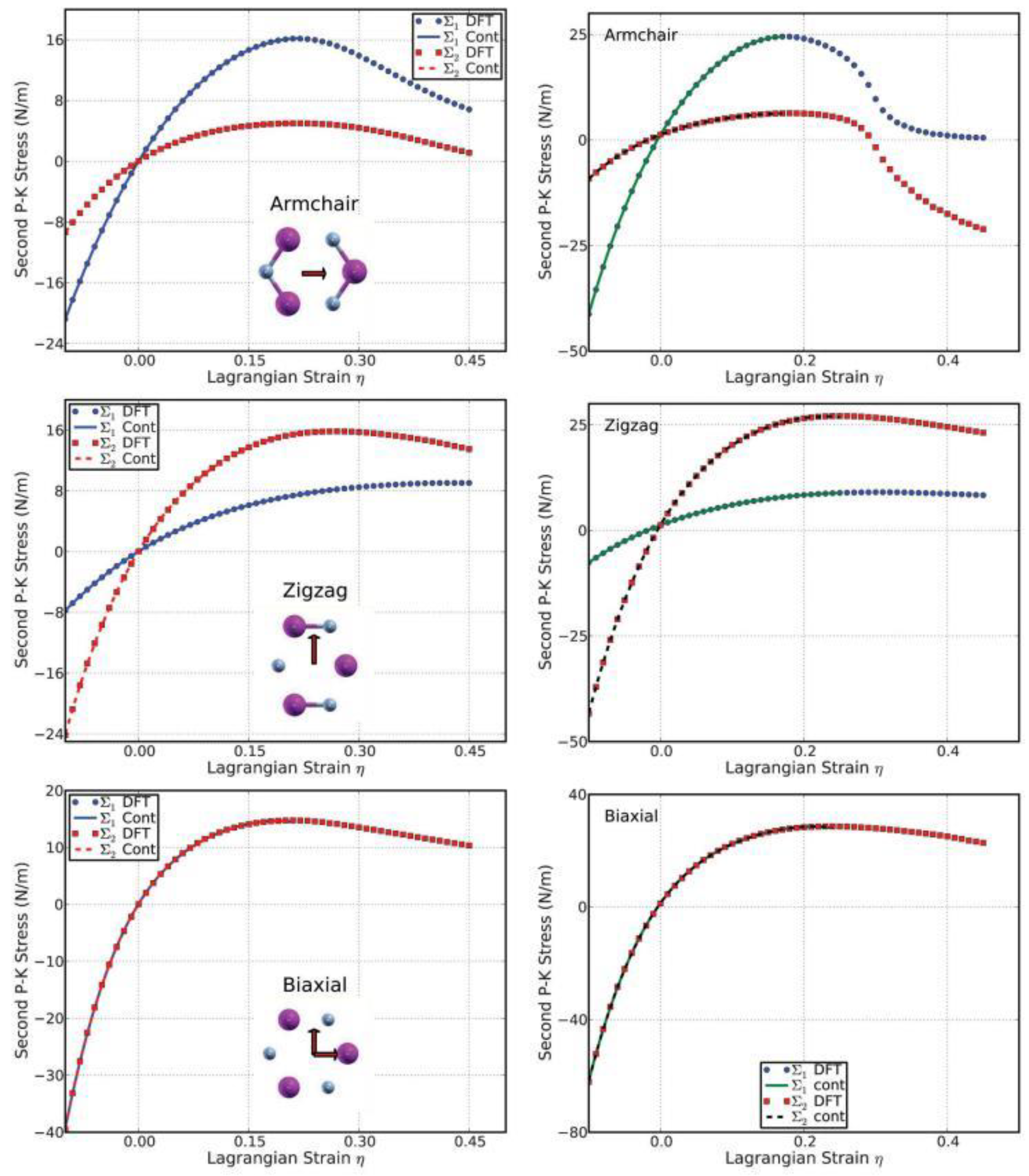



| 0.00 | 0.25 | 0.50 | 0.75 | 1.00 | Graphene a | |
|---|---|---|---|---|---|---|
| a | 2.468 | 2.484 | 2.496 | 2.506 | 2.512 | 2.446 |
| Ys | 344.6 | 321.9 | 300.0 | 285.7 | 278.3 | 348 |
| V | 0.1790 | 0.1839 | 0.1937 | 0.1998 | 0.2254 | 0.169 |
| Qi | 356.0 | 333.2 | 311.8 | 297.6 | 293.2 | 358.1 |
| C12 | 63.7 | 61.3 | 60.4 | 59.4 | 66.1 | 60.4 |
| Cm | −3121 | −2888 | −2666 | −2560 | −2514 | −2817 |
| C112 | −472 | −551 | −407 | −383 | −425 | −337 |
| C222 | −2978 | −2722 | −2466 | −2381 | −2284 | −2693 |
| Gm | 19,980 | 18,220 | 20,195 | 18,030 | 16,547 | 13,416 |
| C1112 | 2706 | 3643 | 4267 | 2628 | 2609 | 759 |
| C1122 | 2843 | 4065 | 2012 | 6755 | 2215 | 2582.8 |
| C2222 | 16,568 | 16,085 | 19,746 | 13,234 | 12,288 | 10,358.9 |
| Q11111 | −81,498 | −77,944 | −130,712 | −98,102 | −65,265 | −31,383.8 |
| Q11112 | −13,378 | −18,430 | −30,317 | −13,725 | −8454 | −88.4 |
| G11122 | −12,852 | −29,572 | −52,986 | −62,434 | −28,556 | −12,960.5 |
| G12222 | −28,504 | −34,777 | −34,287 | −47,317 | −36,955 | −13,046.6 |
| C22222 | −79,311 | −146,507 | −328,759 | −121,135 | −100,469 | −33,446.7 |
| g-BN | g-AlN | g-GaN | g-InN | g-TIN | |
|---|---|---|---|---|---|
| Σma | 23.6 | 16.2 | 11.9 | 8.0 | 5.8 |
| ηma | 0.18 | 0.22 | 0.18 | 0.18 | 0.17 |
| Σmz | 26.3 | 15.9 | 12.1 | 8.0 | 5.7 |
| ηmz | 0.26 | 0.27 | 0.22 | 0.21 | 0.21 |
| Σmb | 27.8 | 14.8 | 11.7 | 8.0 | 5.5 |
| ηmb | 0.24 | 0.21 | 0.16 | 0.15 | 0.16 |
| g-BN | g-AlN | g-GaN | g-InN | g-TlN | |
|---|---|---|---|---|---|
| A | 2.512 | 3.127 | 3.207 | 3.585 | 3.73 |
| Ys | 278.3 | 135.7 | 109.4 | 62.0 | 34.5 |
| Ν | 0.225 | 0.366 | 0.431 | 0.586 | 0.689 |
| C11 | 293.2 | 156.7 | 134.4 | 94.4 | 65.7 |
| C12 | 66.1 | 57.4 | 57.9 | 55.4 | 45.3 |
| C111 | −2513.6 | −1265.7 | −1038.6 | −716.7 | −485.2 |
| C112 | −425 | −328.3 | −452.2 | −461.0 | −434 |
| C222 | −2284.2 | −968.9 | −880.6 | −609.1 | −420.6 |
| C1111 | 16,547 | 8753 | 5478 | 2978 | 1977 |
| C1112 | 2609 | 699 | 2710 | 3307 | 623 |
| C1122 | 2215 | 4604 | 4964 | 2514 | 7317 |
| C2222 | 12,288 | 2447 | 2165 | −1745 | 1362 |
| C11111 | −65,265 | −46,941 | −22,786 | 3382 | −13,969 |
| C11112 | −8454 | −2801 | 12,073 | −591 | 16,509 |
| C11122 | −28,556 | −13,643 | −29,688 | −17,843 | −23,086 |
| C12222 | −36,955 | −18,237 | 26,909 | −9615 | 32,898 |
| C22222 | −100,469 | −3855 | −9539 | 40,801 | −11,812 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ye, C.; Peng, Q. Mechanical Stabilities and Properties of Graphene-like 2D III-Nitrides: A Review. Crystals 2023, 13, 12. https://doi.org/10.3390/cryst13010012
Ye C, Peng Q. Mechanical Stabilities and Properties of Graphene-like 2D III-Nitrides: A Review. Crystals. 2023; 13(1):12. https://doi.org/10.3390/cryst13010012
Chicago/Turabian StyleYe, Chao, and Qing Peng. 2023. "Mechanical Stabilities and Properties of Graphene-like 2D III-Nitrides: A Review" Crystals 13, no. 1: 12. https://doi.org/10.3390/cryst13010012
APA StyleYe, C., & Peng, Q. (2023). Mechanical Stabilities and Properties of Graphene-like 2D III-Nitrides: A Review. Crystals, 13(1), 12. https://doi.org/10.3390/cryst13010012







