High Temperature Superconducting Flux Pumps for Contactless Energization
Abstract
1. Introduction
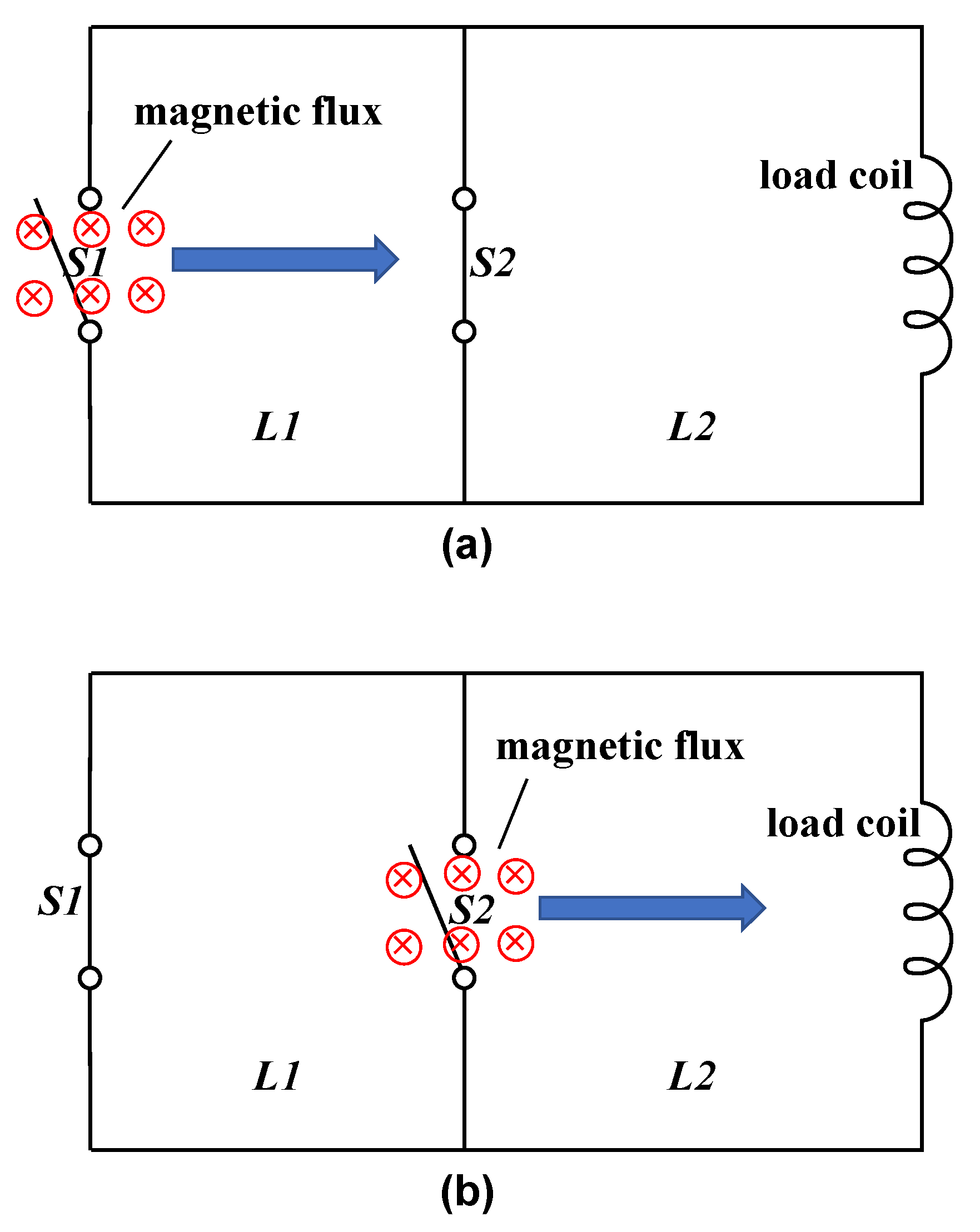
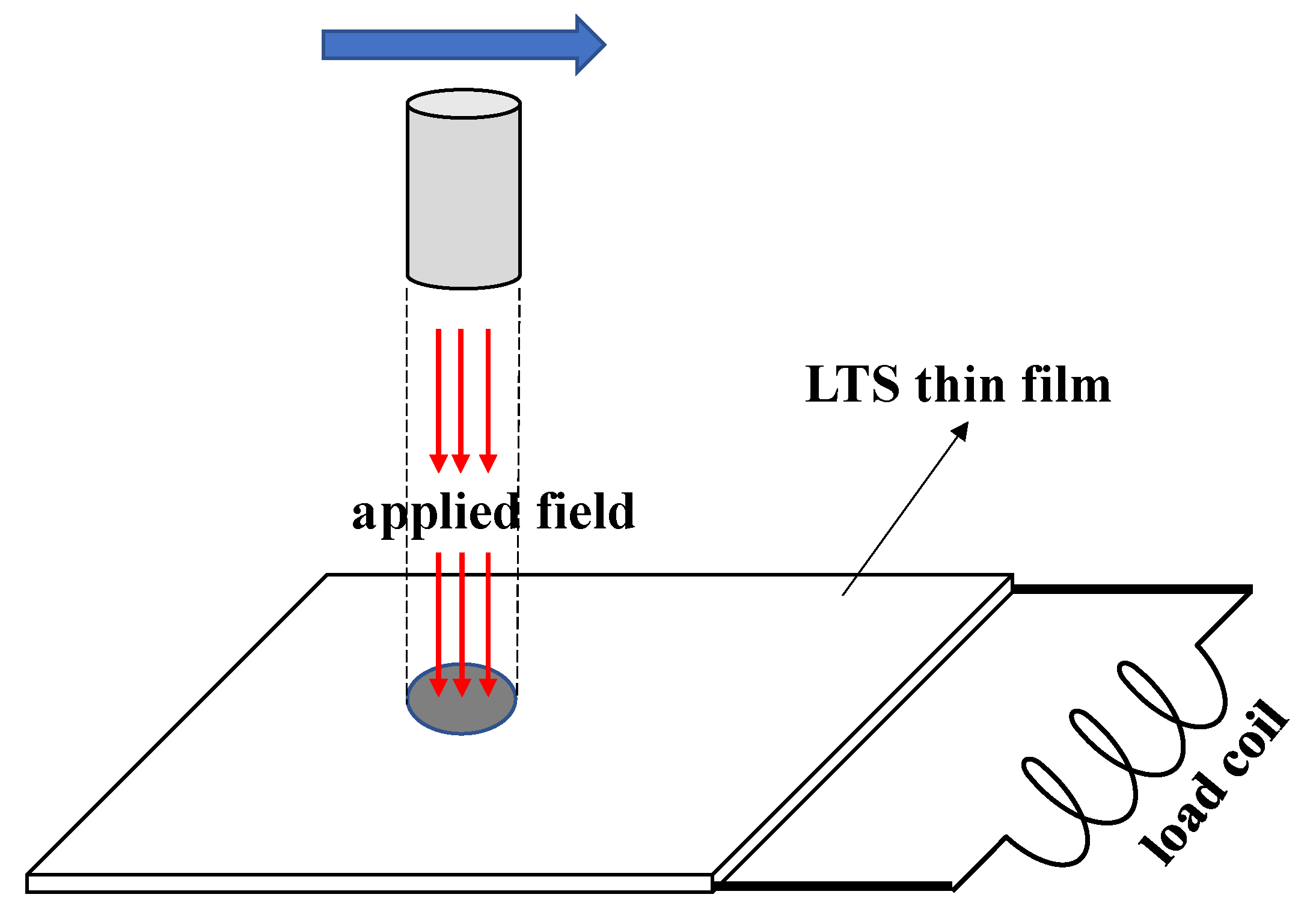
2. Low Temperature Superconducting Flux Pump
3. Travelling Wave HTS Flux Pumps
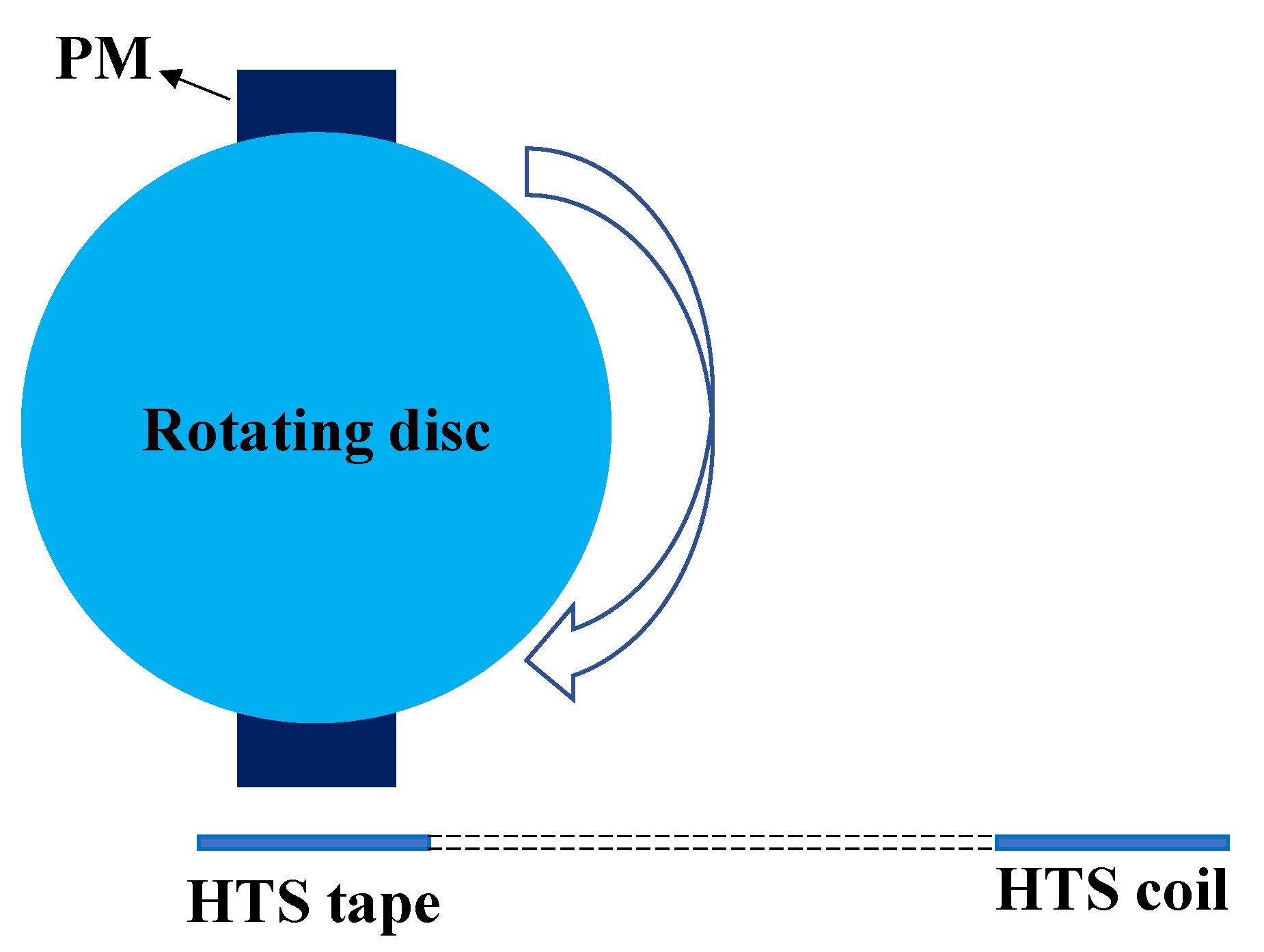
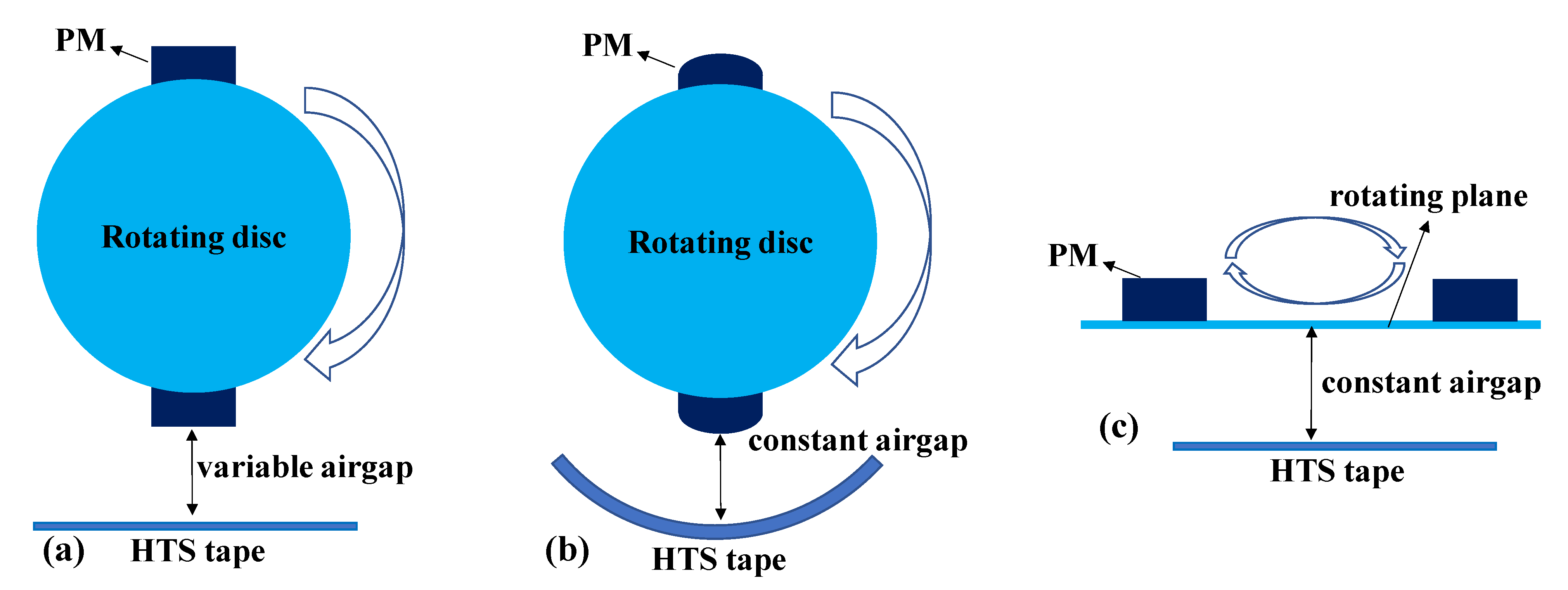
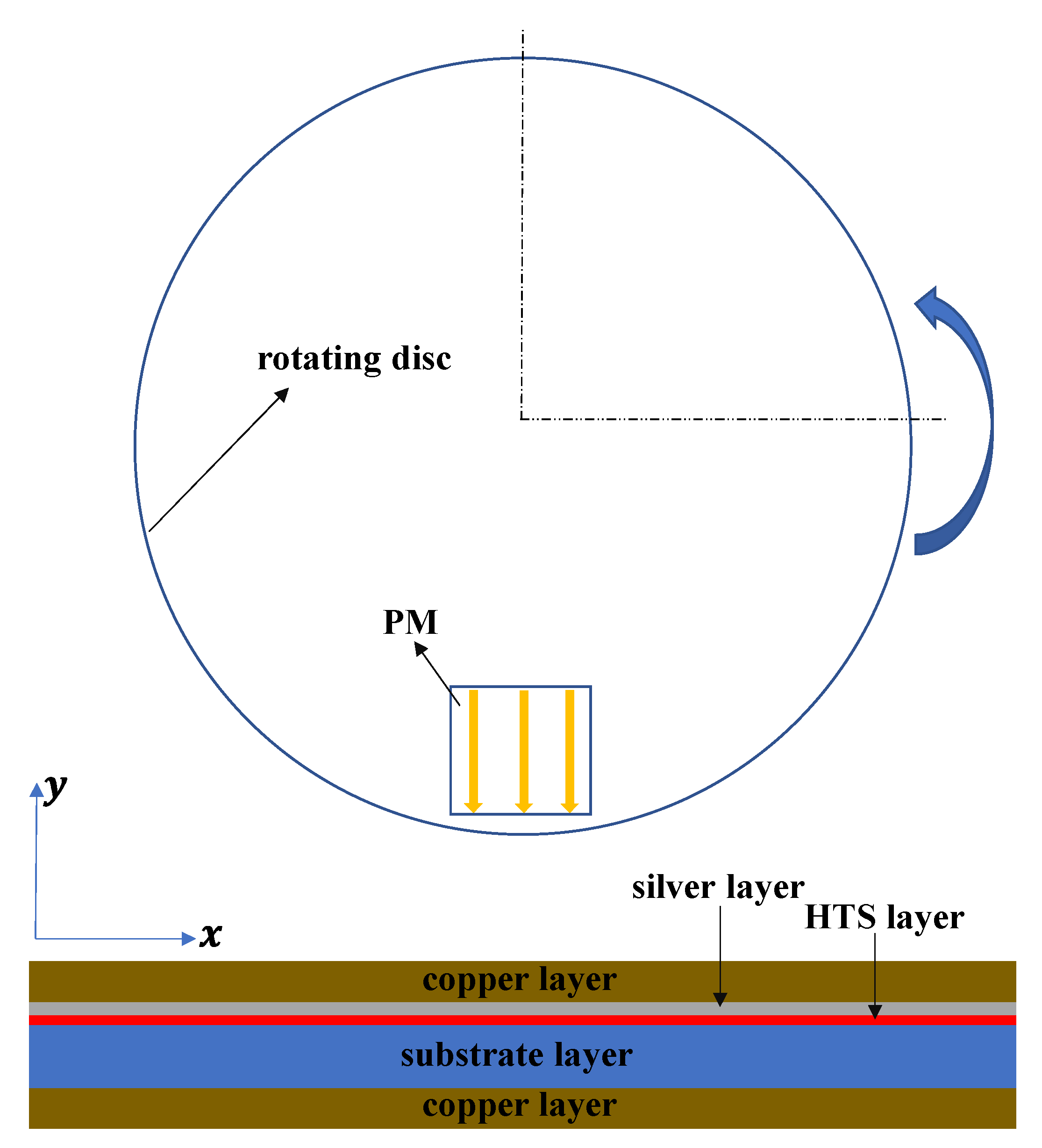
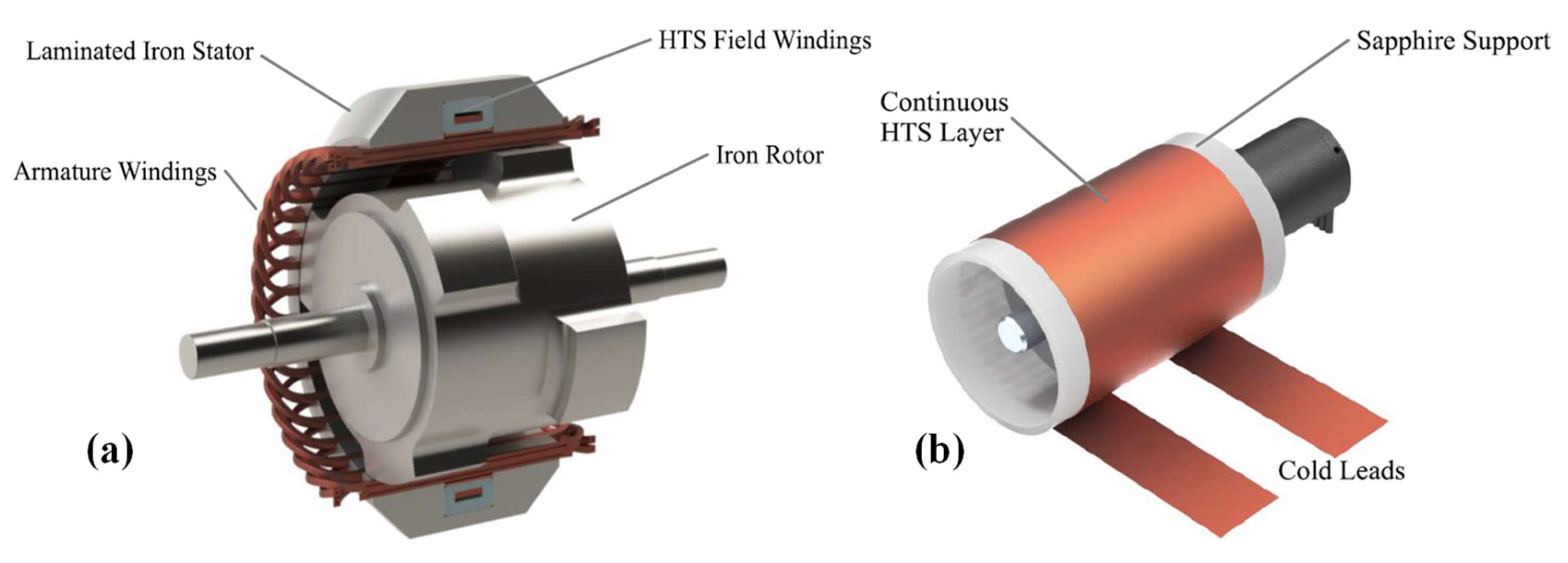
3.1. Rotary HTS Flux Pumps
3.2. Linear HTS Flux Pumps
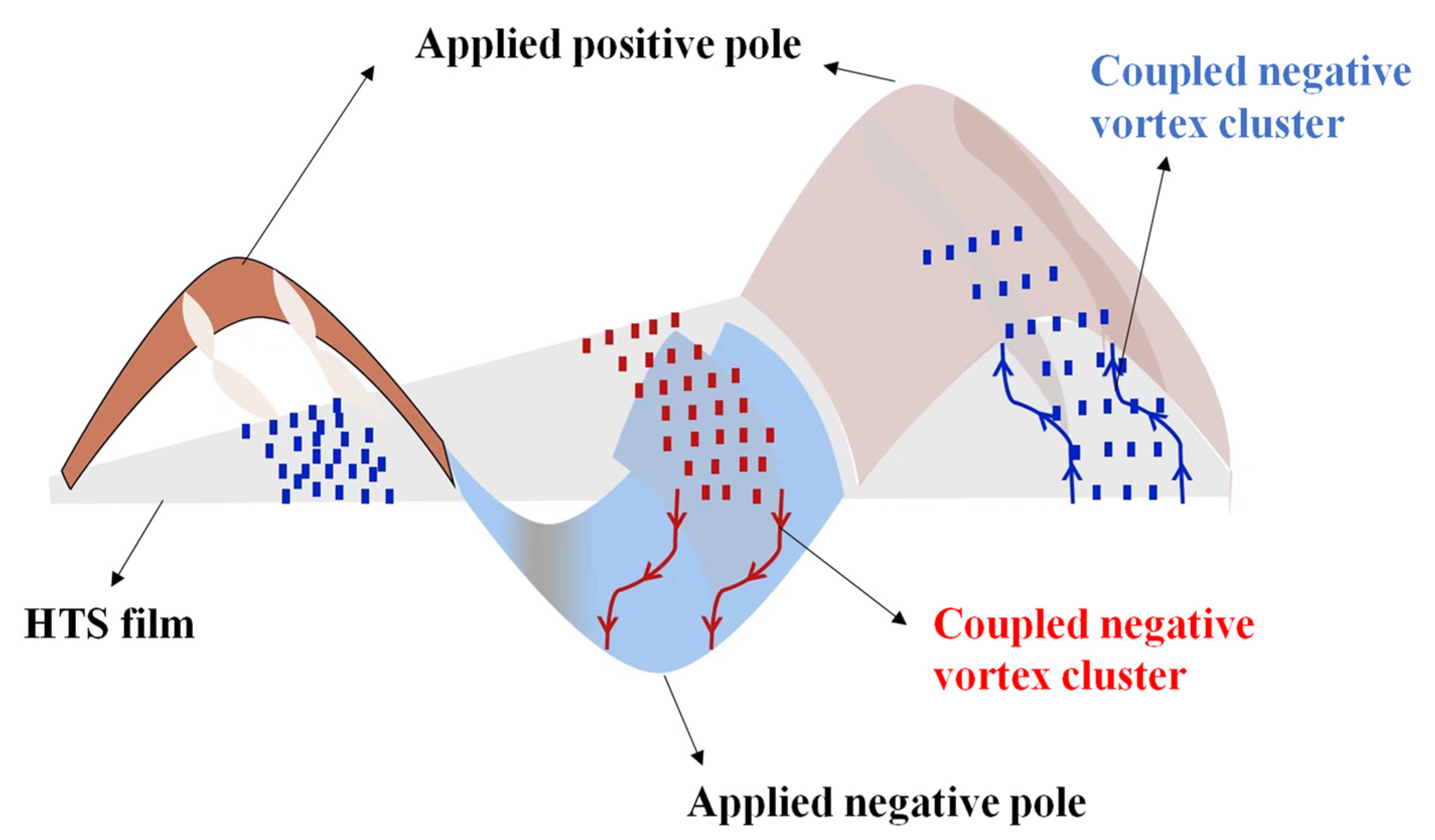
3.3. Underlying Physics
3.4. Modeling Techniques
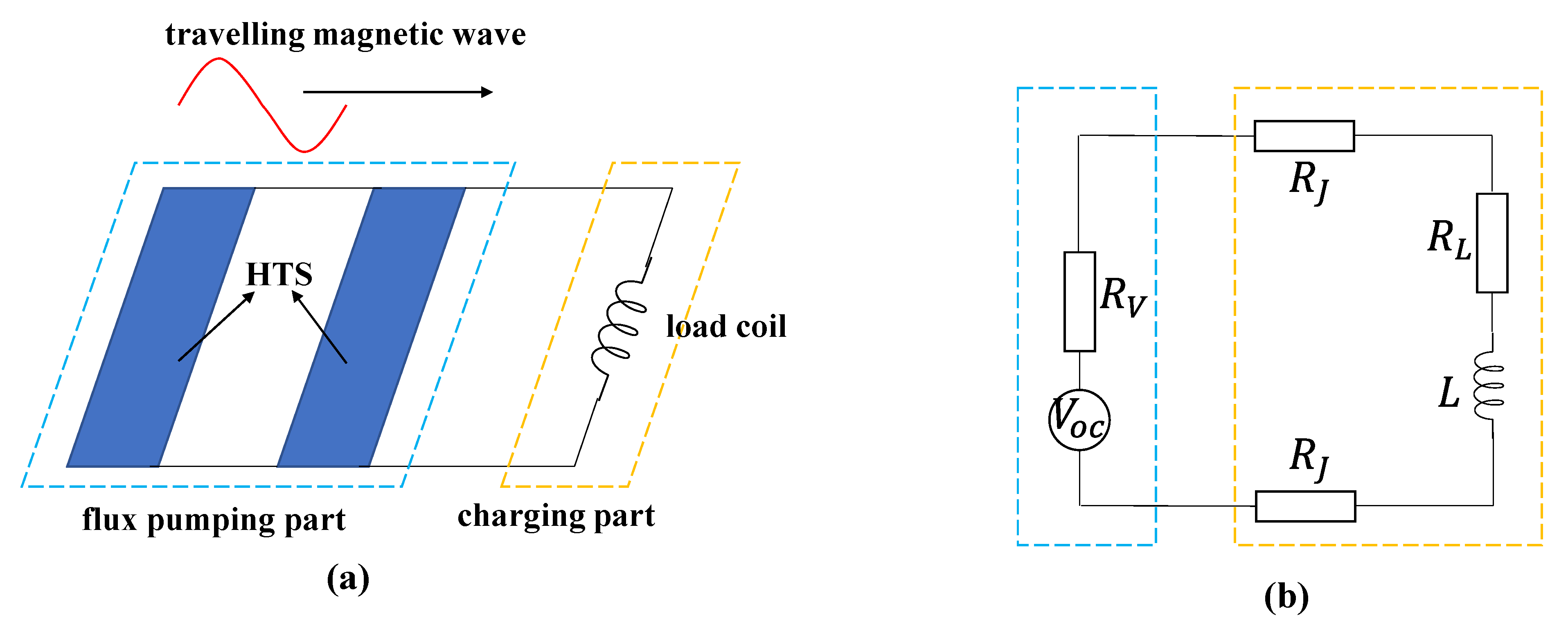
4. Transformer-Rectifier HTS Flux Pumps
4.1. AC Field Switched Transformer-Rectifier HTS Flux Pumps
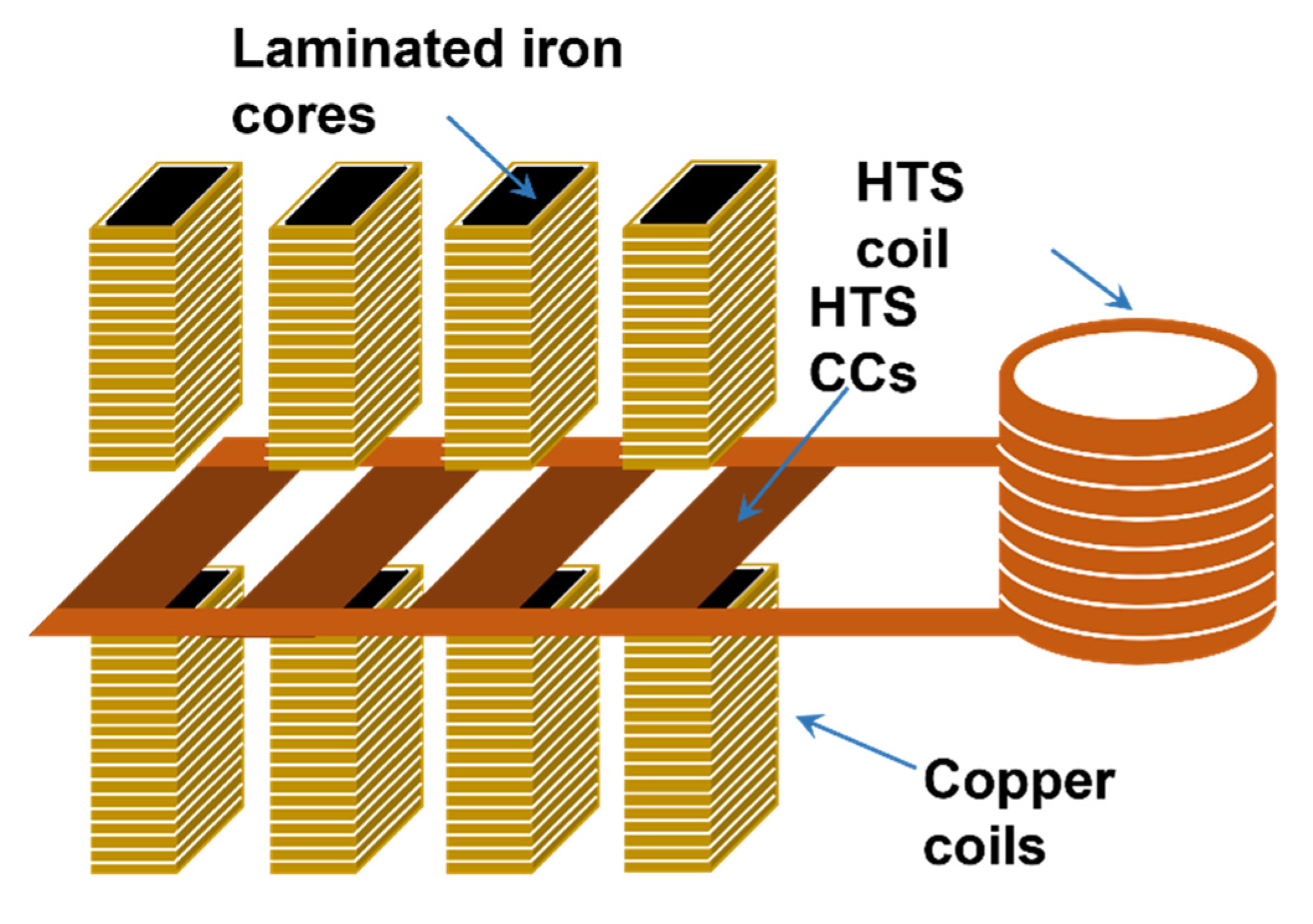
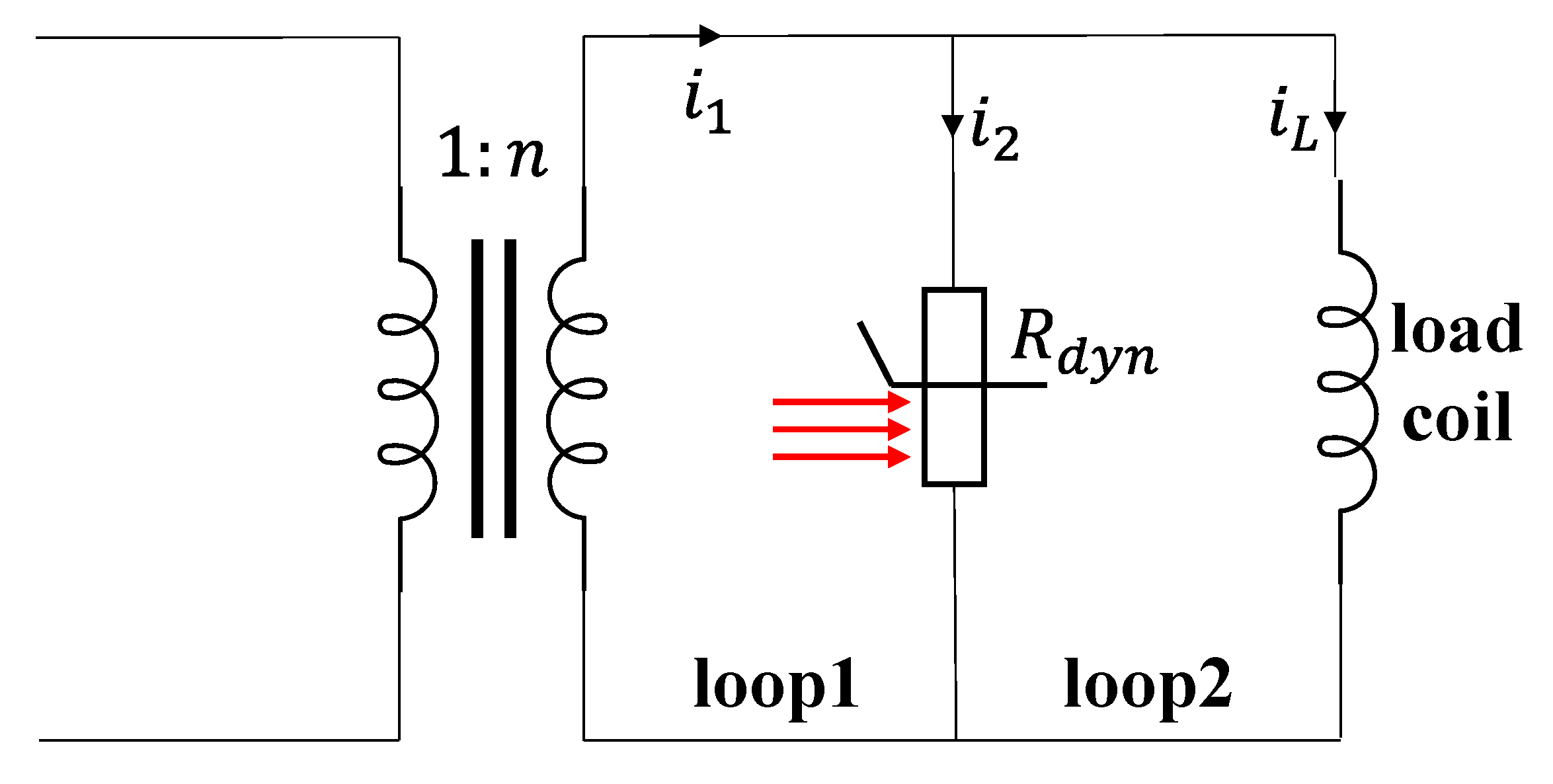
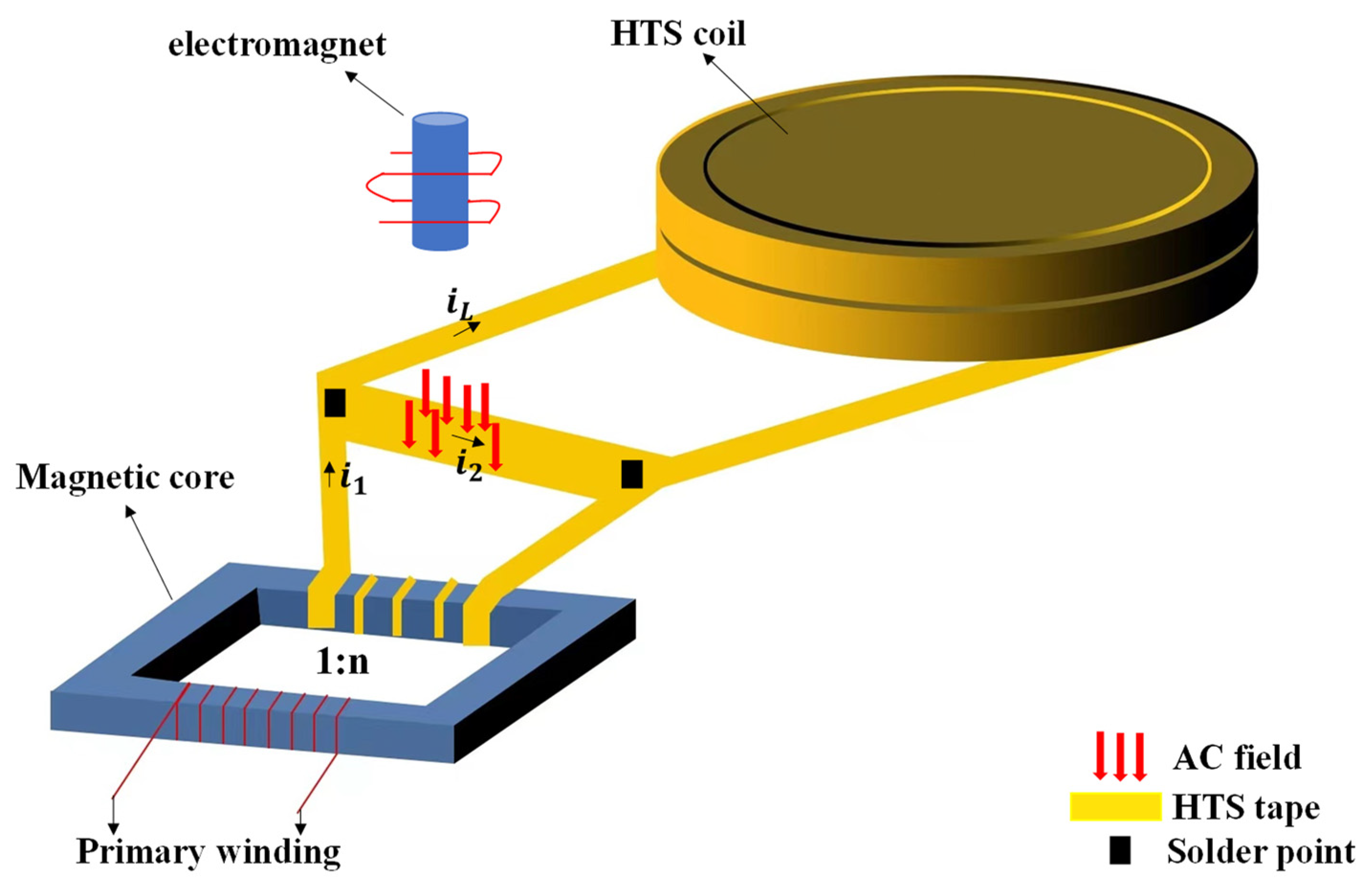
4.1.1. Topology
4.1.2. Mechanism
4.2. Self-Regulating Transformer-Rectifier HTS Flux Pumps
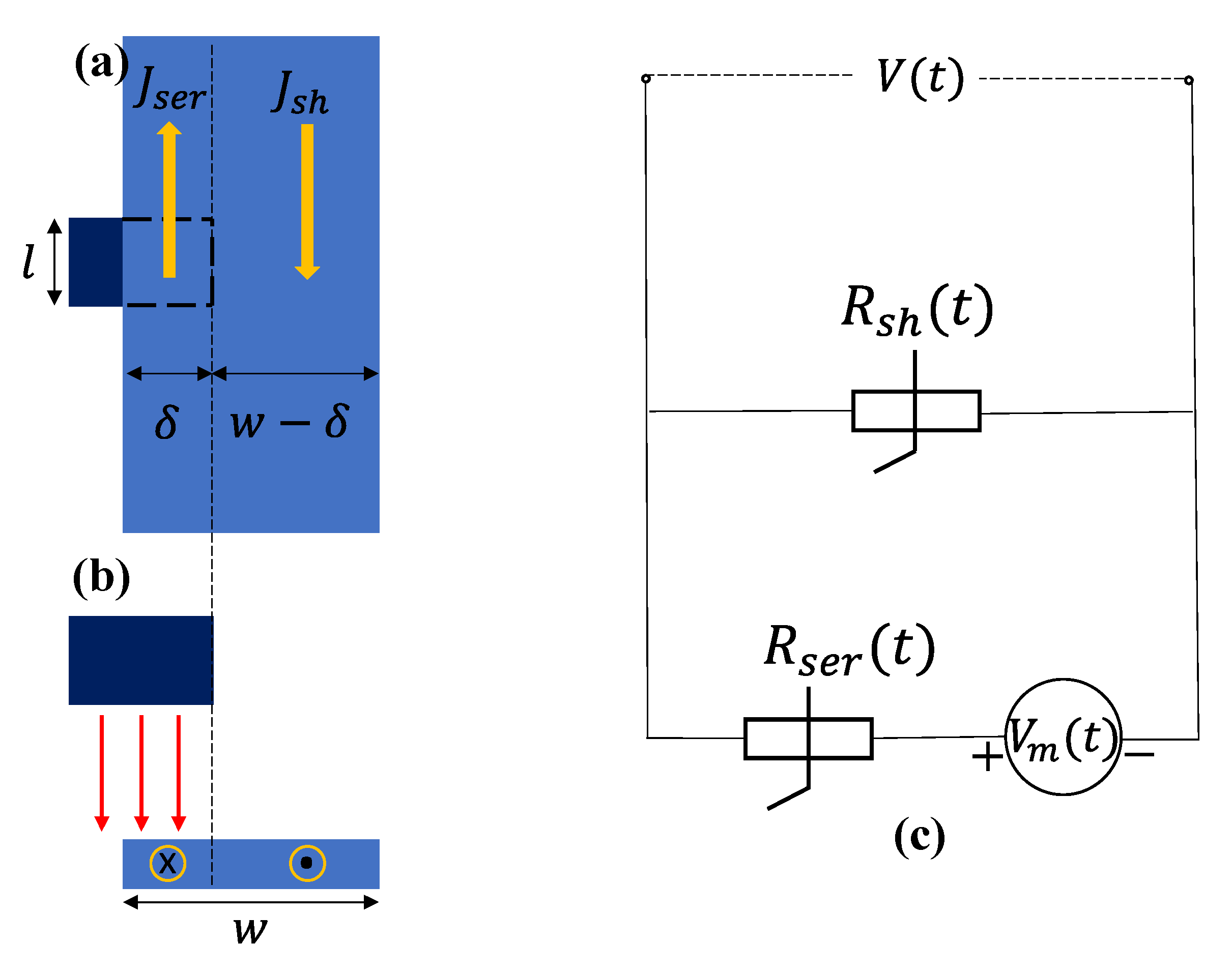
4.2.1. Topology
4.2.2. Mechanism
4.3. Modeling Techniques
5. Other HTS Flux Pumps
6. Discussion
7. Applications
8. Conclusions
- Most work on HTS flux pumps presented in the current literature focuses on operational research and theoretical analysis, namely, how to increase the pumped current and figure out the underlying mechanism. Limited attention has been put on the losses associated with HTS flux pumps [154], which directly determines the efficiency of the flux pump system. The scenario, where conducting superconductors are exposed to external AC fields, appears in almost all HTS flux pumps, which means certain AC losses must exist [155,156,157]. Especially for the AC field switched transfer-rectifier HTS flux pump, in which their working principles are essentially based on AC losses. Moreover, in order to scale up the pumped current, one will need to solder multiple HTS loads together with multiple HTS bridges (switches). As a result, it is inevitable that extra heat losses due to non-zero joint resistances will be produced [113]. A recent work [158] has described the energy balance for HTS dynamos, in which it was demonstrated that due to the interactions of induced currents with rotating PMs, a significant part of the mechanical power supplied to the rotor is converted into Joule dissipation with HTSCs. If these losses are not well modulated, the HTS components may be placed at the risk of quenching, which will result in failure of the whole system.
- The most obvious criterion to evaluate the performance of an HTS flux pump is the output voltage (in open circuit condition) or pumping current (in closed loop condition). Ideally, we want the output to be as high as possible. However, it should be highlighted that the peak of the output is not the only concern. In many cases, the ripple plays a critical role. For instance, in a synchronous machine, if the excitation current in field winding is not maintained constant, the electromagnetic force generated by the external rotating field changes accordingly, which will cause output fluctuation or even fatal damage to the machine. Due to external vibrations, such as noise from the input system, ripples can be expected in any type of HTS flux pump. In particular, the self-regulating transfer-rectifier flux pump is operated in the flux flow regime (sharp E-J relation), where a small turbulence in the current can result in very large ripples. Hence, it is necessary to integrate a reliable control system to HTS flux pumps to stabilize the output. The authors of [159,160] proposed a proportion–integral–differential (PID) loop for a rotary HTS flux pump and [161] demonstrated a feedback circuit for a transformer-rectifier flux pump, both showing great effectiveness for smoothing the output ripple. A sensitive control system is desired for more sophisticated applications.
- One of the key obstacles that hinders the advancement of the HTS flux pump is the operating cost. The HTS components, which are not cheap themselves, plus the expensive compulsory cryogenic system make the construction and operation of such devices costly. Alternatively, accurate simulation can be utilized to investigate HTS flux pumps without undertaking real experiments, saving substantial costs. The modeling of HTS flux pumps has mainly relied on FEM models, which are extremely helpful for acquiring details about the electromagnetic behavior involved in HTS flux pumps. However, FEM models are often time consuming, because they need to mathematically simulate the whole process to replicate the real case. Moreover, FEM models require users to have abundant knowledge about the problem they are modeling. These features are absolutely not preferred from the perspective of industrial design and optimization, where the interests will be capturing certain specifications that can evaluate the device performance rather than delving into the underlying physics. Recent work in [69] proposed an innovative statistical model based on machine learning techniques, for capturing the output characteristics of a rotary HTS flux pump. In that model, the output voltage can be immediately predicted according to several design parameters with very high accuracy. Such machine learning enabled models can be further developed, which are expected to become powerful design tools for HTS flux pumps and other superconducting applications.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Weijers, H.; Trociewitz, U.; Markiewicz, W.; Jiang, J.; Myers, D.; Hellstrom, E.; Xu, A.; Jaroszynski, J.; Noyes, P.; Viouchkov, Y. High field magnets with HTS conductors. IEEE Trans. Appl. Supercond. 2010, 20, 576–582. [Google Scholar] [CrossRef]
- Hahn, S.; Park, D.K.; Voccio, J.; Bascunan, J.; Iwasa, Y. No-Insulation (NI) HTS Inserts for > 1 GHz LTS/HTS NMR Magnets. IEEE Trans. Appl. Supercond. 2011, 22, 4302405. [Google Scholar] [CrossRef] [PubMed]
- Abrahamsen, A.B.; Mijatovic, N.; Seiler, E.; Zirngibl, T.; Træholt, C.; Nørgård, P.B.; Pedersen, N.F.; Andersen, N.H.; Østergaard, J. Superconducting wind turbine generators. Supercond. Sci. Technol. 2010, 23, 034019. [Google Scholar] [CrossRef]
- Sivasubramaniam, K.; Zhang, T.; Lokhandwalla, M.; Laskaris, E.; Bray, J.; Gerstler, B.; Shah, M.; Alexander, J. Development of a high speed HTS generator for airborne applications. IEEE Trans. Appl. Supercond. 2009, 19, 1656–1661. [Google Scholar] [CrossRef]
- Miki, M.; Tokura, S.; Hayakawa, H.; Inami, H.; Kitano, M.; Matsuzaki, H.; Kimura, Y.; Ohtani, I.; Morita, E.; Ogata, H. Development of a synchronous motor with Gd–Ba–Cu–O bulk superconductors as pole-field magnets for propulsion system. Supercond. Sci. Technol. 2006, 19, S494. [Google Scholar] [CrossRef]
- Umemoto, K.; Aizawa, K.; Yokoyama, M.; Yoshikawa, K.; Kimura, Y.; Izumi, M.; Ohashi, K.; Numano, M.; Okumura, K.; Yamaguchi, M. Development of 1 MW-class HTS motor for podded ship propulsion system. J. Phys. Conf. Ser. 2010, 234, 032060. [Google Scholar] [CrossRef]
- Nick, W.; Frank, M.; Klaus, G.; Frauenhofer, J.; Neumuller, H.-W. Operational experience with the world’s first 3600 rpm 4 MVA generator at Siemens. IEEE Trans. Appl. Supercond. 2007, 17, 2030–2033. [Google Scholar] [CrossRef]
- Sung, H.-J.; Kim, G.-H.; Kim, K.; Jung, S.-J.; Park, M.; Yu, I.-K.; Kim, Y.-G.; Lee, H.; Kim, A.-R. Practical design of a 10 MW superconducting wind power generator considering weight issue. IEEE Trans. Appl. Supercond. 2013, 23, 5201805. [Google Scholar] [CrossRef]
- Karmaker, H.; Ho, M.; Chen, E.; Kulkarni, D. Direct drive HTS wind generator design for commercial applications. In Proceedings of the 2014 International Conference on Electrical Machines (ICEM), Berlin, Germany, 2–5 September 2014; pp. 491–495. [Google Scholar]
- Sung, H.-J.; Kim, G.-H.; Kim, K.; Park, M.; Yu, I.-K.; Kim, J.-Y. Design and comparative analysis of 10 MW class superconducting wind power generators according to different types of superconducting wires. Phys. C Supercond. 2013, 494, 255–261. [Google Scholar] [CrossRef]
- Filipenko, M.; Kühn, L.; Gleixner, T.; Thummet, M.; Lessmann, M.; Möller, D.; Böhm, M.; Schröter, A.; Häse, K.; Grundmann, J. Concept design of a high power superconducting generator for future hybrid-electric aircraft. Supercond. Sci. Technol. 2020, 33, 054002. [Google Scholar] [CrossRef]
- Boll, M.; Corduan, M.; Biser, S.; Filipenko, M.; Pham, Q.H.; Schlachter, S.; Rostek, P.; Noe, M. A holistic system approach for short range passenger aircraft with cryogenic propulsion system. Supercond. Sci. Technol. 2020, 33, 044014. [Google Scholar] [CrossRef]
- Lvovsky, Y.; Stautner, E.W.; Zhang, T. Novel technologies and configurations of superconducting magnets for MRI. Supercond. Sci. Technol. 2013, 26, 093001. [Google Scholar] [CrossRef]
- Corato, V.; Bagni, T.; Biancolini, M.; Bonifetto, R.; Bruzzone, P.; Bykovsky, N.; Ciazynski, D.; Coleman, M.; Della Corte, A.; Dembkowska, A. Progress in the design of the superconducting magnets for the EU DEMO. Fusion Eng. Des. 2018, 136, 1597–1604. [Google Scholar] [CrossRef]
- Zhang, M.; Yuan, W.; Hilton, D.K.; Canassy, M.D.; Trociewitz, U.P. Study of second-generation high-temperature superconducting magnets: The self-field screening effect. Supercond. Sci. Technol. 2014, 27, 095010. [Google Scholar] [CrossRef]
- Khodzhibagiyan, H.; Agapov, N.; Akishin, P.; Blinov, N.; Borisov, V.; Bychkov, A.; Galimov, A.; Donyagin, A.; Karpinskiy, V.; Korolev, V. Superconducting magnets for the NICA accelerator collider complex. IEEE Trans. Appl. Supercond. 2013, 24, 4001304. [Google Scholar] [CrossRef]
- Rossi, L. Superconducting magnets for the LHC main lattice. IEEE Trans. Appl. Supercond. 2004, 14, 153–158. [Google Scholar] [CrossRef]
- Yokoyama, K.; Oka, T.; Okada, H.; Fujine, Y.; Chiba, A.; Noto, K. Solid-liquid magnetic separation using bulk superconducting magnets. IEEE Trans. Appl. Supercond. 2003, 13, 1592–1595. [Google Scholar] [CrossRef]
- Tomita, M.; Murakami, M. High-temperature superconductor bulk magnets that can trap magnetic fields of over 17 tesla at 29 K. Nature 2003, 421, 517–520. [Google Scholar] [CrossRef]
- Durrell, J.H.; Dennis, A.R.; Jaroszynski, J.; Ainslie, M.D.; Palmer, K.G.; Shi, Y.; Campbell, A.M.; Hull, J.; Strasik, M.; Hellstrom, E. A trapped field of 17.6 T in melt-processed, bulk Gd-Ba-Cu-O reinforced with shrink-fit steel. Supercond. Sci. Technol. 2014, 27, 082001. [Google Scholar] [CrossRef]
- Patel, A.; Baskys, A.; Mitchell-Williams, T.; McCaul, A.; Coniglio, W.; Hänisch, J.; Lao, M.; Glowacki, B.A. A trapped field of 17.7 T in a stack of high temperature superconducting tape. Supercond. Sci. Technol. 2018, 31, 09LT01. [Google Scholar] [CrossRef]
- Toth, J.; Bole, S. Design, construction, and first testing of a 41.5 T all-resistive magnet at the NHMFL in Tallahassee. IEEE Trans. Appl. Supercond. 2017, 28, 4300104. [Google Scholar] [CrossRef]
- Iijima, Y.; Kaneko, N.; Hanyu, S.; Sutoh, Y.; Kakimoto, K.; Ajimura, S.; Saitoh, T. Development of IBAD/PLD process for long length Y-123 conductors in Fujikura. Phys. C Supercond. Appl. 2006, 445, 509–514. [Google Scholar] [CrossRef]
- Freyhardt, H.C. YBaCuO and REBaCuO HTS for applications. Int. J. Appl. Ceram. Technol. 2007, 4, 203–216. [Google Scholar] [CrossRef]
- Shiohara, Y.; Yoshizumi, M.; Izumi, T.; Yamada, Y. Present status and future prospect of coated conductor development and its application in Japan. Supercond. Sci. Technol. 2008, 21, 034002. [Google Scholar] [CrossRef]
- Sugano, M.; Osamura, K.; Prusseit, W.; Semerad, R.; Kuroda, T.; Itoh, K.; Kiyoshi, T. Reversible strain dependence of critical current in 100 A class coated conductors. IEEE Trans. Appl. Supercond. 2005, 15, 3581–3584. [Google Scholar] [CrossRef]
- Lee, J.-H.; Lee, H.; Lee, J.-W.; Choi, S.-M.; Yoo, S.-I.; Moon, S.-H. RCE-DR, a novel process for coated conductor fabrication with high performance. Supercond. Sci. Technol. 2014, 27, 044018. [Google Scholar] [CrossRef]
- Ballarino, A. Current leads, links and buses. arXiv 2015, arXiv:1501.07166. [Google Scholar]
- Wegener, L. Status of Wendelstein 7-X construction. Fusion Eng. Des. 2009, 84, 106–112. [Google Scholar] [CrossRef]
- Ballarino, A. Current leads for the LHC magnet system. IEEE Trans. Appl. Supercond. 2002, 12, 1275–1280. [Google Scholar] [CrossRef]
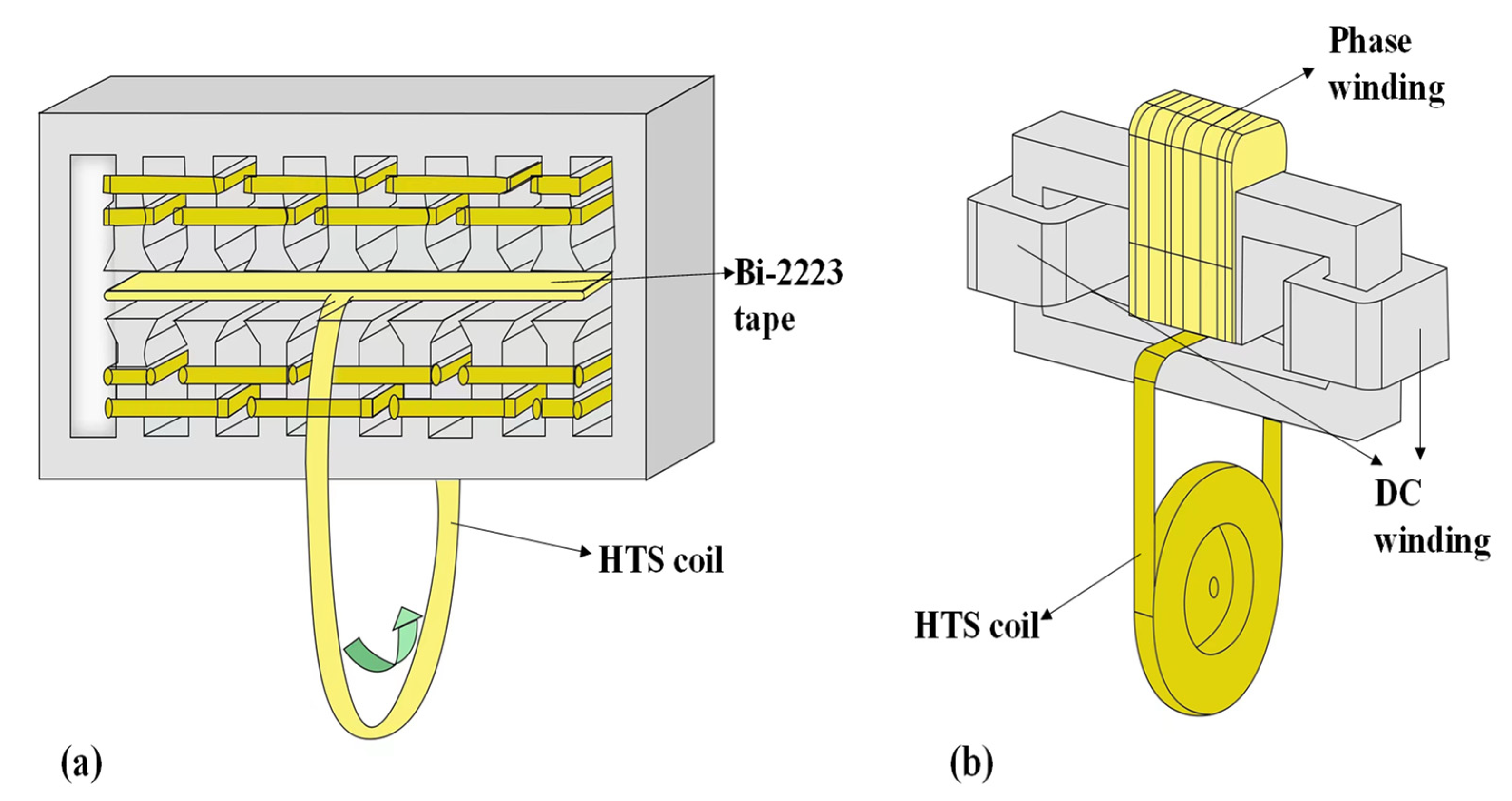
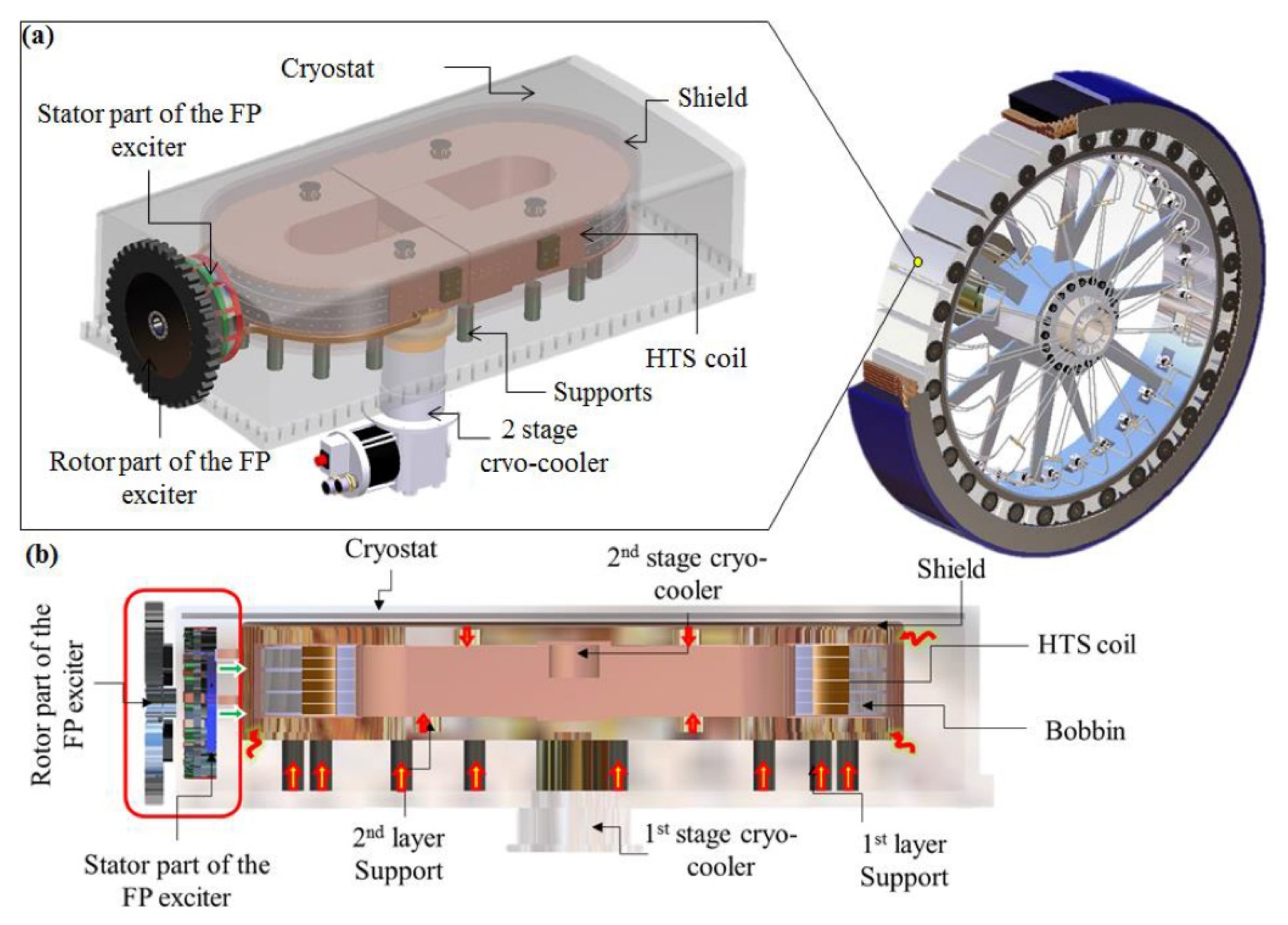
- Bumby, C.W.; Badcock, R.A.; Sung, H.-J.; Kim, K.-M.; Jiang, Z.; Pantoja, A.E.; Bernardo, P.; Park, M.; Buckley, R.G. Development of a brushless HTS exciter for a 10 kW HTS synchronous generator. Supercond. Sci. Technol. 2016, 29, 024008. [Google Scholar] [CrossRef]
- Sung, H.; Badcock, R.; Jiang, Z.; Choi, J.; Park, M.; Yu, I. Design and heat load analysis of a 12 MW HTS wind power generator module employing a brushless HTS exciter. IEEE Trans. Appl. Supercond. 2016, 26, 5205404. [Google Scholar] [CrossRef]
- Jeon, H.; Lee, J.; Han, S.; Kim, J.H.; Hyeon, C.J.; Kim, H.M.; Park, D.; Do Chung, Y.; Ko, T.K.; Yoon, Y.S. Methods for increasing the saturation current and charging speed of a rotary HTS flux-pump to charge the field coil of a synchronous motor. IEEE Trans. Appl. Supercond. 2018, 28, 5202605. [Google Scholar] [CrossRef]
- Kalsi, S.S. Applications of High Temperature Superconductors to Electric Power Equipment; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Feigel’man, M.; Geshkenbein, V.; Vinokur, V. Flux creep and current relaxation in high-T c superconductors. Phys. Rev. B 1991, 43, 6263. [Google Scholar] [CrossRef] [PubMed]
- Hildebrandt, A.F.; Wahlquist, H.; Elleman, D.D. Configurations of superconducting shells required for near critical uniform magnetic fields. J. Appl. Phys. 1962, 33, 1798–1800. [Google Scholar] [CrossRef]
- Hildebrandt, A.F.; Elleman, D.D.; Whitmore, F.C.; Simpkins, R. Some Experimental Consequences of Flux Conservation within Multiply-Connected Superconductors. J. Appl. Phys. 1962, 33, 2375–2377. [Google Scholar] [CrossRef]
- Rose-Innes, A. A superconducting magnetic flux compressor. Cryogenics 1973, 13, 103–105. [Google Scholar] [CrossRef]
- Van Beelen, H.; AJPT Arnold, M.; Sypkens, H.; De Bruyn Ouboter, R.; Beenakker, J.; Taconis, K. A 25 000 gauss, 175 amperes Nb-25% Zr wire magnet fed by a flux pump. Phys. Lett. 1963, 7. [Google Scholar] [CrossRef]
- Van Suchtelen, J.; Volger, J.; Van Houwelingen, D. The principle and performance of a superconducting dynamo. Cryogenics 1965, 5, 256–266. [Google Scholar] [CrossRef]
- Van Beelen, H.; Houckgeest, J.V.B.; Ouboter, R.D.B.; Taconis, K. Remarks on a moving-flux experiment in superconducting sheets. Physica 1967, 36, 107–117. [Google Scholar] [CrossRef]
- Atherton, D. High-Efficiency Superconducting Homopolar dc Generators. J. Appl. Phys. 1968, 39, 2639. [Google Scholar] [CrossRef]
- Van Beelen, H.; De Langen, M.; Kerkdijk, C. Comments on the efficiency of flux pumping into superconducting circuits. Physica 1969, 42, 265–276. [Google Scholar] [CrossRef]
- Purcell, J.; Payne, E. Superconducting rectifiers. In Advances in Cryogenic Engineering; Springer: Berlin/Heidelberg, Germany, 1961; pp. 149–153. [Google Scholar]
- Hempstead, C.; Kim, Y.; Strnad, A. Inductive behavior of superconducting magnets. J. Appl. Phys. 1963, 34, 3226–3236. [Google Scholar] [CrossRef]
- Buchhold, T. Superconductive power supply and its application for electrical flux pumping. Cryogenics 1964, 4, 212–217. [Google Scholar] [CrossRef]
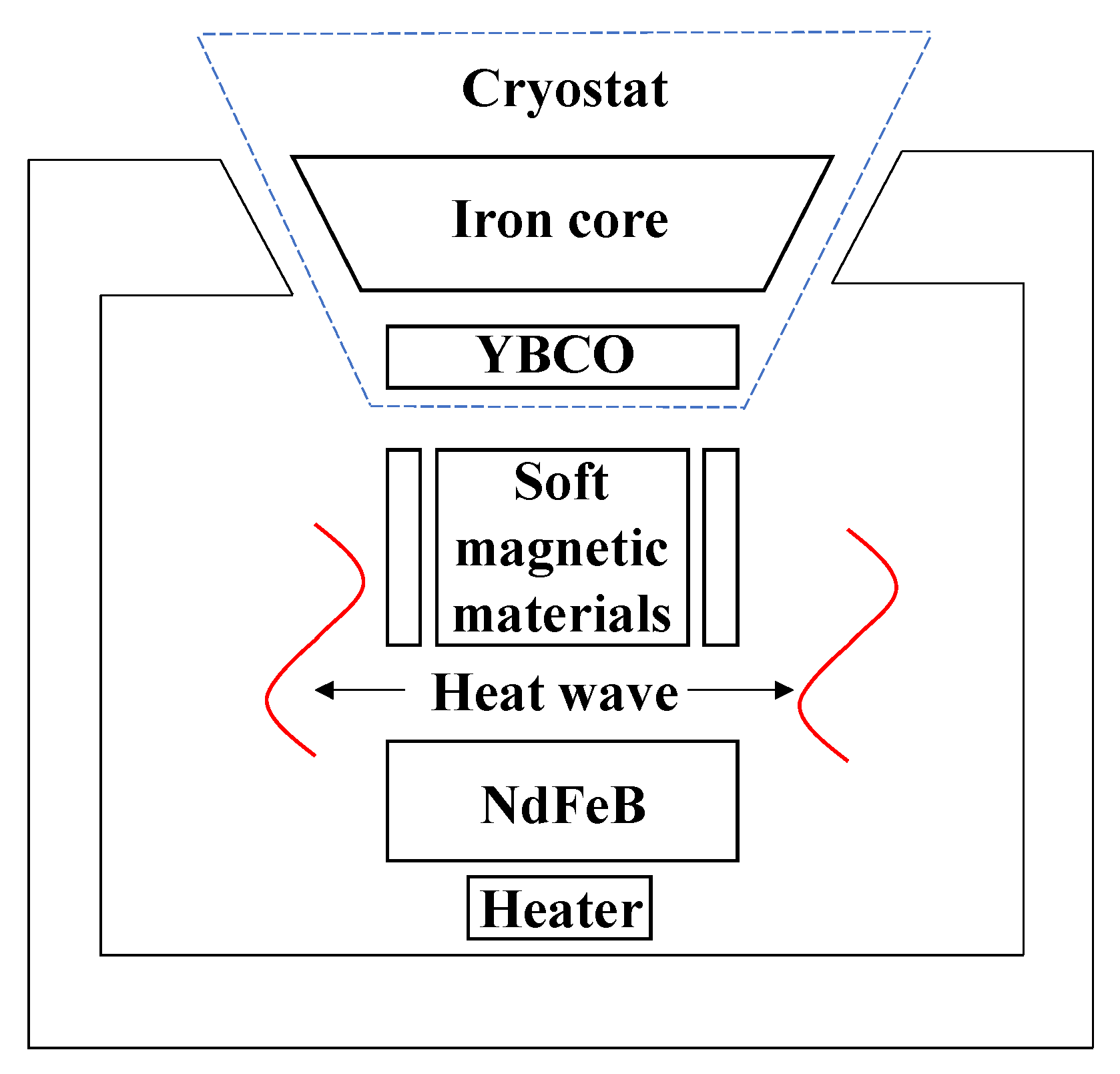
- Coombs, T.; Hong, Z.; Zhu, X. A thermally actuated superconducting flux pump. Phys. C Supercond. 2008, 468, 153–159. [Google Scholar] [CrossRef]
- Coombs, T.; Hong, Z.; Yan, Y.; Rawlings, C. The next generation of superconducting permanent magnets: The flux pumping method. IEEE Trans. Appl. Supercond. 2009, 19, 2169–2173. [Google Scholar] [CrossRef]
- Yan, Y.; Li, Q.; Coombs, T. Thermally actuated magnetization flux pump in single-grain YBCO bulk. Supercond. Sci. Technol. 2009, 22, 105011. [Google Scholar] [CrossRef]
- Coombs, T.A.; Geng, J.; Fu, L.; Matsuda, K. An overview of flux pumps for HTS coils. IEEE Trans. Appl. Supercond. 2016, 27, 4600806. [Google Scholar] [CrossRef]
- Coombs, T. Superconducting flux pumps. J. Appl. Phys. 2019, 125, 230902. [Google Scholar] [CrossRef]
- Zhai, Y.; Tan, Z.; Liu, X.; Shen, B.; Coombs, T.A.; Wang, F. Research Progress of Contactless Magnetization Technology: HTS Flux Pumps. IEEE Trans. Appl. Supercond. 2020, 30, 4602905. [Google Scholar] [CrossRef]
- Van de Klundert, L.; ten Kate, H.H. Fully superconducting rectifiers and fluxpumps Part 1: Realized methods for pumping flux. Cryogenics 1981, 21, 195–206. [Google Scholar] [CrossRef]
- van de Klundert, L.; ten Kate, H. On fully superconducting rectifiers and fluxpumps. A review. Part 2: Commutation modes, characteristics and switches. Cryogenics 1981, 21, 267–277. [Google Scholar] [CrossRef][Green Version]
- Mulder, G.; Ten Kate, H.; Krooshoop, H.; van de Klundert, L. Development of a thermally switched superconducting rectifier for 100 kA. IEEE Trans. Magn. 1991, 27, 2333–2336. [Google Scholar] [CrossRef]
- Oomen, M.P.; Leghissa, M.; Ries, G.; Proelss, N.; Neumueller, H.-W.; Steinmeyer, F.; Vester, M.; Davies, F. HTS flux pump for cryogen-free HTS magnets. IEEE Trans. Appl. Supercond. 2005, 15, 1465–1468. [Google Scholar] [CrossRef]
- Hoffmann, C.; Pooke, D.; Caplin, A.D. Flux pump for HTS magnets. IEEE Trans. Appl. Supercond. 2010, 21, 1628–1631. [Google Scholar] [CrossRef]
- Hoffmann, C.; Walsh, R.; Karrer-Mueller, E.; Pooke, D. Design parameters for an HTS flux pump. Phys. Procedia 2012, 36, 1324–1329. [Google Scholar] [CrossRef]
- Bumby, C.W.; Pantoja, A.E.; Sung, H.-J.; Jiang, Z.; Kulkarni, R.; Badcock, R.A. Through-wall excitation of a magnet coil by an external-rotor HTS flux pump. IEEE Trans. Appl. Supercond. 2016, 26, 0500505. [Google Scholar] [CrossRef]
- Storey, J.G.; Pantoja, A.E.; Jiang, Z.; Hamilton, K.; Badcock, R.A.; Bumby, C.W. Impact of annular yoke geometry on performance of a dynamo-type HTS flux pump. IEEE Trans. Appl. Supercond. 2018, 28, 5203906. [Google Scholar] [CrossRef]
- Pantoja, A.E.; Storey, J.G.; Badcock, R.A.; Jiang, Z.; Phang, S.; Bumby, C.W. Output during continuous frequency ramping of a dynamo-type HTS flux pump. IEEE Trans. Appl. Supercond. 2018, 28, 5202205. [Google Scholar] [CrossRef]
- Storey, J.G.; Pantoja, A.E.; Jiang, Z.; Badcock, R.A.; Bumby, C.W. Optimizing rotor speed and geometry for an externally mounted HTS dynamo. IEEE Trans. Appl. Supercond. 2019, 29, 5202705. [Google Scholar] [CrossRef]
- Jiang, Z.; Bumby, C.W.; Badcock, R.A.; Sung, H.-J.; Long, N.J.; Amemiya, N. Impact of flux gap upon dynamic resistance of a rotating HTS flux pump. Supercond. Sci. Technol. 2015, 28, 115008. [Google Scholar] [CrossRef]
- Bumby, C.W.; Phang, S.; Pantoja, A.E.; Jiang, Z.; Storey, J.G.; Sung, H.-J.; Park, M.; Badcock, R.A. Frequency dependent behavior of a dynamo-type HTS flux pump. IEEE Trans. Appl. Supercond. 2016, 27, 5200705. [Google Scholar] [CrossRef]
- Pantoja, A.E.; Jiang, Z.; Badcock, R.A.; Bumby, C.W. Impact of stator wire width on output of a dynamo-type HTS flux pump. IEEE Trans. Appl. Supercond. 2016, 26, 4805208. [Google Scholar] [CrossRef]
- Badcock, R.A.; Phang, S.; Pantoja, A.E.; Jiang, Z.; Storey, J.G.; Sung, H.-J.; Park, M.; Bumby, C.W. Impact of magnet geometry on output of a dynamo-type HTS flux pump. IEEE Trans. Appl. Supercond. 2016, 27, 5200905. [Google Scholar] [CrossRef]
- Zhang, H.; Yao, M.; Kails, K.; Machura, P.; Mueller, M.; Jiang, Z.; Xin, Y.; Li, Q. Modelling of electromagnetic loss in HTS coated conductors over a wide frequency band. Supercond. Sci. Technol. 2020, 33, 025004. [Google Scholar] [CrossRef]
- Zhang, H.; Chen, H.; Jiang, Z.; Yang, T.; Xin, Y.; Mueller, M.; Li, Q. A full-range formulation for dynamic loss of high-temperature superconductor coated conductors. Supercond. Sci. Technol. 2020, 33, 05LT01. [Google Scholar] [CrossRef]
- Wen, Z.; Zhang, H.; Mueller, M. Sensitivity analysis and machine learning modelling for the output characteristics of rotary HTS flux pumps. Supercond. Sci. Technol. 2021, 34, 125019. [Google Scholar] [CrossRef]
- Mataira, R.; Ainslie, M.D.; Badcock, R.; Bumby, C.W. Modeling of Stator Versus Magnet Width Effects in High-$ T_c $ Superconducting Dynamos. IEEE Trans. Appl. Supercond. 2020, 30, 5204406. [Google Scholar] [CrossRef]
- Fu, L.; Matsuda, K.; Baghdadi, M.; Coombs, T. Linear flux pump device applied to high temperature superconducting (HTS) magnets. IEEE Trans. Appl. Supercond. 2015, 25, 4603804. [Google Scholar] [CrossRef]
- Fu, L.; Matsuda, K.; Lecrevisse, T.; Iwasa, Y.; Coombs, T. A flux pumping method applied to the magnetization of YBCO superconducting coils: Frequency, amplitude and waveform characteristics. Supercond. Sci. Technol. 2016, 29, 04LT01. [Google Scholar] [CrossRef]
- Fu, L.; Matsuda, K.; Coombs, T. Linear flux pump device applied to HTS magnets: Further characteristics on wave profile, number of poles, and control of saturated current. IEEE Trans. Appl. Supercond. 2016, 26, 0500304. [Google Scholar] [CrossRef]
- Fu, L.; Matsuda, K.; Shen, B.; Coombs, T. Charging an HTS Coil: Flux Pump With an HTS Square Bridge. IEEE Trans. Appl. Supercond. 2021, 31, 3800405. [Google Scholar] [CrossRef]
- Coombs, T.; Fagnard, J.-F.; Matsuda, K. Magnetization of 2-G coils and artificial bulks. IEEE Trans. Appl. Supercond. 2014, 24, 8201005. [Google Scholar] [CrossRef]
- Geng, J.; Matsuda, K.; Fu, L.; Fagnard, J.-F.; Zhang, H.; Zhang, X.; Shen, B.; Dong, Q.; Baghdadi, M.; Coombs, T. Origin of dc voltage in type II superconducting flux pumps: Field, field rate of change, and current density dependence of resistivity. J. Phys. D Appl. Phys. 2016, 49, 11LT01. [Google Scholar] [CrossRef]
- Bumby, C.; Jiang, Z.; Storey, J.; Pantoja, A.; Badcock, R. Anomalous open-circuit voltage from a high-T c superconducting dynamo. Appl. Phys. Lett. 2016, 108, 122601. [Google Scholar] [CrossRef]
- Mataira, R.; Ainslie, M.; Badcock, R.; Bumby, C. Origin of the DC output voltage from a high-Tc superconducting dynamo. Appl. Phys. Lett. 2019, 114, 162601. [Google Scholar] [CrossRef]
- Mataira, R.; Ainslie, M.; Pantoja, A.; Badcock, R.; Bumby, C. Mechanism of the high-T c superconducting dynamo: Models and experiment. Phys. Rev. Appl. 2020, 14, 024012. [Google Scholar] [CrossRef]
- Wang, W.; Coombs, T. Macroscopic magnetic coupling effect: The physical origination of a high-temperature superconducting flux pump. Phys. Rev. Appl. 2018, 9, 044022. [Google Scholar] [CrossRef]
- Wei, J.; Wang, W.; Yang, Z.; Ye, H.; Zhang, Y.; Li, H.; Xu, H.; Shen, B.; Zhai, Y.; Zhou, Q. Build Charging Database of Linear-Motor Type Flux Pump and Analyze the Influence of DC-Bias Field Using Fixed Step Size Search Algorithm. IEEE Trans. Appl. Supercond. 2021, 31, 0500305. [Google Scholar] [CrossRef]
- Kajikawa, K.; Hayashi, T.; Yoshida, R.; Iwakuma, M.; Funaki, K. Numerical evaluation of AC losses in HTS wires with 2D FEM formulated by self magnetic field. IEEE Trans. Appl. Supercond. 2003, 13, 3630–3633. [Google Scholar] [CrossRef]
- Hong, Z.; Campbell, A.; Coombs, T. Numerical solution of critical state in superconductivity by finite element software. Supercond. Sci. Technol. 2006, 19, 1246. [Google Scholar] [CrossRef]
- Brambilla, R.; Grilli, F.; Martini, L. Development of an edge-element model for AC loss computation of high-temperature superconductors. Supercond. Sci. Technol. 2006, 20, 16. [Google Scholar] [CrossRef]
- Ainslie, M.D.; Yuan, W.; Hong, Z.; Pei, R.; Flack, T.J.; Coombs, T.A. Modeling and electrical measurement of transport AC loss in HTS-based superconducting coils for electric machines. IEEE Trans. Appl. Supercond. 2010, 21, 3265–3268. [Google Scholar] [CrossRef]
- Ainslie, M.D.; Flack, T.J.; Campbell, A.M. Calculating transport AC losses in stacks of high temperature superconductor coated conductors with magnetic substrates using FEM. Phys. C Supercond. 2012, 472, 50–56. [Google Scholar] [CrossRef]
- Brambilla, R.; Grilli, F.; Martini, L.; Bocchi, M.; Angeli, G. A finite-element method framework for modeling rotating machines with superconducting windings. IEEE Trans. Appl. Supercond. 2018, 28, 5207511. [Google Scholar] [CrossRef]
- Santos, B.M.O.; Dias, F.J.M.; Sass, F.; Sotelo, G.G.; Polasek, A.; de Andrade, R. Simulation of superconducting machine with stacks of coated conductors using hybrid ah formulation. IEEE Trans. Appl. Supercond. 2020, 30, 5207309. [Google Scholar] [CrossRef]
- Liang, F.; Venuturumilli, S.; Zhang, H.; Zhang, M.; Kvitkovic, J.; Pamidi, S.; Wang, Y.; Yuan, W. A finite element model for simulating second generation high temperature superconducting coils/stacks with large number of turns. J. Appl. Phys. 2017, 122, 043903. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, M.; Yuan, W. An efficient 3D finite element method model based on the T–A formulation for superconducting coated conductors. Supercond. Sci. Technol. 2016, 30, 024005. [Google Scholar] [CrossRef]
- Berrospe-Juarez, E.; Zermeño, V.M.; Trillaud, F.; Grilli, F. Real-time simulation of large-scale HTS systems: Multi-scale and homogeneous models using the T–A formulation. Supercond. Sci. Technol. 2019, 32, 065003. [Google Scholar] [CrossRef]
- Grilli, F.; Pardo, E.; Morandi, A.; Gömöry, F.; Solovyov, M.; Zermeño, V.M.; Brambilla, R.; Benkel, T.; Riva, N. Electromagnetic modeling of superconductors with commercial software: Possibilities with two vector potential-based formulations. IEEE Trans. Appl. Supercond. 2020, 31, 5900109. [Google Scholar] [CrossRef]
- Benkel, T.; Lao, M.; Liu, Y.; Pardo, E.; Wolfstädter, S.; Reis, T.; Grilli, F. T–A-formulation to model electrical machines with HTS coated conductor coils. IEEE Trans. Appl. Supercond. 2020, 30, 5205807. [Google Scholar] [CrossRef]
- Quéval, L.; Liu, K.; Yang, W.; Zermeño, V.M.; Ma, G. Superconducting magnetic bearings simulation using an H-formulation finite element model. Supercond. Sci. Technol. 2018, 31, 084001. [Google Scholar] [CrossRef]
- Ainslie, M.D.; Quéval, L.; Mataira, R.C.; Bumby, C.W. Modelling the frequency dependence of the open-circuit voltage of a high-T c superconducting dynamo. IEEE Trans. Appl. Supercond. 2021, 31, 4900407. [Google Scholar] [CrossRef]
- Pardo, E.; Šouc, J.; Frolek, L. Electromagnetic modelling of superconductors with a smooth current–voltage relation: Variational principle and coils from a few turns to large magnets. Supercond. Sci. Technol. 2015, 28, 044003. [Google Scholar] [CrossRef]
- Pardo, E.; Kapolka, M. 3D computation of non-linear eddy currents: Variational method and superconducting cubic bulk. J. Comput. Phys. 2017, 344, 339–363. [Google Scholar] [CrossRef]
- Brambilla, R.; Grilli, F.; Martini, L.; Sirois, F. Integral equations for the current density in thin conductors and their solution by the finite-element method. Supercond. Sci. Technol. 2008, 21, 105008. [Google Scholar] [CrossRef]
- Grilli, F.; Brambilla, R.; Martini, L.F.; Sirois, F.; Nguyen, D.N.; Ashworth, S.P. Current density distribution in multiple YBCO coated conductors by coupled integral equations. IEEE Trans. Appl. Supercond. 2009, 19, 2859–2862. [Google Scholar] [CrossRef]
- Brambilla, R.; Grilli, F.; Nguyen, D.N.; Martini, L.; Sirois, F. AC losses in thin superconductors: The integral equation method applied to stacks and windings. Supercond. Sci. Technol. 2009, 22, 075018. [Google Scholar] [CrossRef]
- Morandi, A.; Fabbri, M.; Ribani, P.L.; Dennis, A.; Durrell, J.; Shi, Y.; Cardwell, D. The measurement and modeling of the levitation force between single-grain YBCO bulk superconductors and permanent magnets. IEEE Trans. Appl. Supercond. 2018, 28, 3601310. [Google Scholar] [CrossRef]
- Fabbri, M.; Forzan, M.; Lupi, S.; Morandi, A.; Ribani, P.L. Experimental and numerical analysis of DC induction heating of aluminum billets. IEEE Trans. Magn. 2009, 45, 192–200. [Google Scholar] [CrossRef]
- Perini, E.; Giunchi, G.; Geri, M.; Morandi, A. Experimental and Numerical Investigation of the Levitation Force Between Bulk Permanent Magnet and MgB2 Disk. IEEE Trans. Appl. Supercond. 2009, 19, 2124–2128. [Google Scholar] [CrossRef]
- Prigozhin, L.; Sokolovsky, V. Fast solution of the superconducting dynamo benchmark problem. Supercond. Sci. Technol. 2021, 34, 065006. [Google Scholar] [CrossRef]
- Prigozhin, L.; Sokolovsky, V. Two-dimensional model of a high-T c superconducting dynamo. IEEE Trans. Appl. Supercond. 2021, 31, 5201107. [Google Scholar] [CrossRef]
- Sokolovsky, V.; Prigozhin, L.; Kozyrev, A.B. Chebyshev spectral method for superconductivity problems. Supercond. Sci. Technol. 2020, 33, 085008. [Google Scholar] [CrossRef]
- Ainslie, M.; Grilli, F.; Quéval, L.; Pardo, E.; Perez-Mendez, F.; Mataira, R.; Morandi, A.; Ghabeli, A.; Bumby, C.; Brambilla, R. A new benchmark problem for electromagnetic modelling of superconductors: The high-T c superconducting dynamo. Supercond. Sci. Technol. 2020, 33, 105009. [Google Scholar] [CrossRef]
- Zhang, H.; Machura, P.; Kails, K.; Chen, H.; Mueller, M. Dynamic loss and magnetization loss of HTS coated conductors, stacks, and coils for high-speed synchronous machines. Supercond. Sci. Technol. 2020, 33, 084008. [Google Scholar] [CrossRef]
- Ghabeli, A.; Pardo, E.; Kapolka, M. 3D modeling of a superconducting dynamo-type flux pump. Sci. Rep. 2021, 11, 10296. [Google Scholar] [CrossRef]
- Ghabeli, A.; Ainslie, M.; Pardo, E.; Quéval, L.; Mataira, R. Modeling the charging process of a coil by an HTS dynamo-type flux pump. Supercond. Sci. Technol. 2021, 34, 084002. [Google Scholar] [CrossRef]
- Geng, J.; Matsuda, K.; Fu, L.; Shen, B.; Zhang, X.; Coombs, T. Operational research on a high-Tc rectifier-type superconducting flux pump. Supercond. Sci. Technol. 2016, 29, 035015. [Google Scholar] [CrossRef]
- Geng, J.; Coombs, T. Mechanism of a high-Tc superconducting flux pump: Using alternating magnetic field to trigger flux flow. Appl. Phys. Lett. 2015, 107, 142601. [Google Scholar] [CrossRef]
- Geng, J.; Painter, T.; Long, P.; Gawith, J.; Yang, J.; Ma, J.; Dong, Q.; Shen, B.; Li, C.; Coombs, T. A kilo-ampere level HTS flux pump. Supercond. Sci. Technol. 2019, 32, 074004. [Google Scholar] [CrossRef]
- Zhang, H.; Geng, J.; Coombs, T. Magnetizing high-Tc superconducting coated conductor stacks using a transformer–rectifier flux pumping method. Supercond. Sci. Technol. 2018, 31, 105007. [Google Scholar] [CrossRef]
- Zhou, P.; Ma, G.; Deng, Y.; Nie, X.; Zhai, Y.; Liu, K.; Zhang, H.; Li, Y. A contactless self-regulating HTS flux pump. IEEE Trans. Appl. Supercond. 2020, 30, 3603006. [Google Scholar] [CrossRef]
- Geng, J.; Bumby, C.W.; Badcock, R.A. Maximising the current output from a self-switching kA-class rectifier flux pump. Supercond. Sci. Technol. 2020, 33, 045005. [Google Scholar] [CrossRef]
- Zhai, Y.; Zhou, P.; Li, J.; Sun, C.; Li, Y.; Xiao, L.; Deng, Y.; Ma, G. Performance Investigation of Contactless Self-Regulating HTS Flux Pump. IEEE Trans. Appl. Supercond. 2021, 31, 4603105. [Google Scholar] [CrossRef]
- Li, C.; Geng, J.; Shen, B.; Ma, J.; Gawith, J.; Coombs, T.A. Investigation on the transformer-rectifier flux pump for high field magnets. IEEE Trans. Appl. Supercond. 2019, 29, 4301105. [Google Scholar] [CrossRef]
- Jiang, Z.; Hamilton, K.; Amemiya, N.; Badcock, R.; Bumby, C. Dynamic resistance of a high-Tc superconducting flux pump. Appl. Phys. Lett. 2014, 105, 112601. [Google Scholar] [CrossRef]
- Zhang, H.; Hao, C.; Xin, Y.; Mueller, M. Demarcation currents and corner field for dynamic resistance of HTS-coated conductors. IEEE Trans. Appl. Supercond. 2020, 30, 6601305. [Google Scholar] [CrossRef]
- Oomen, M.; Rieger, J.; Leghissa, M.; ten Haken, B.; ten Kate, H.H. Dynamic resistance in a slab-like superconductor with Jc (B) dependence. Supercond. Sci. Technol. 1999, 12, 382. [Google Scholar] [CrossRef]
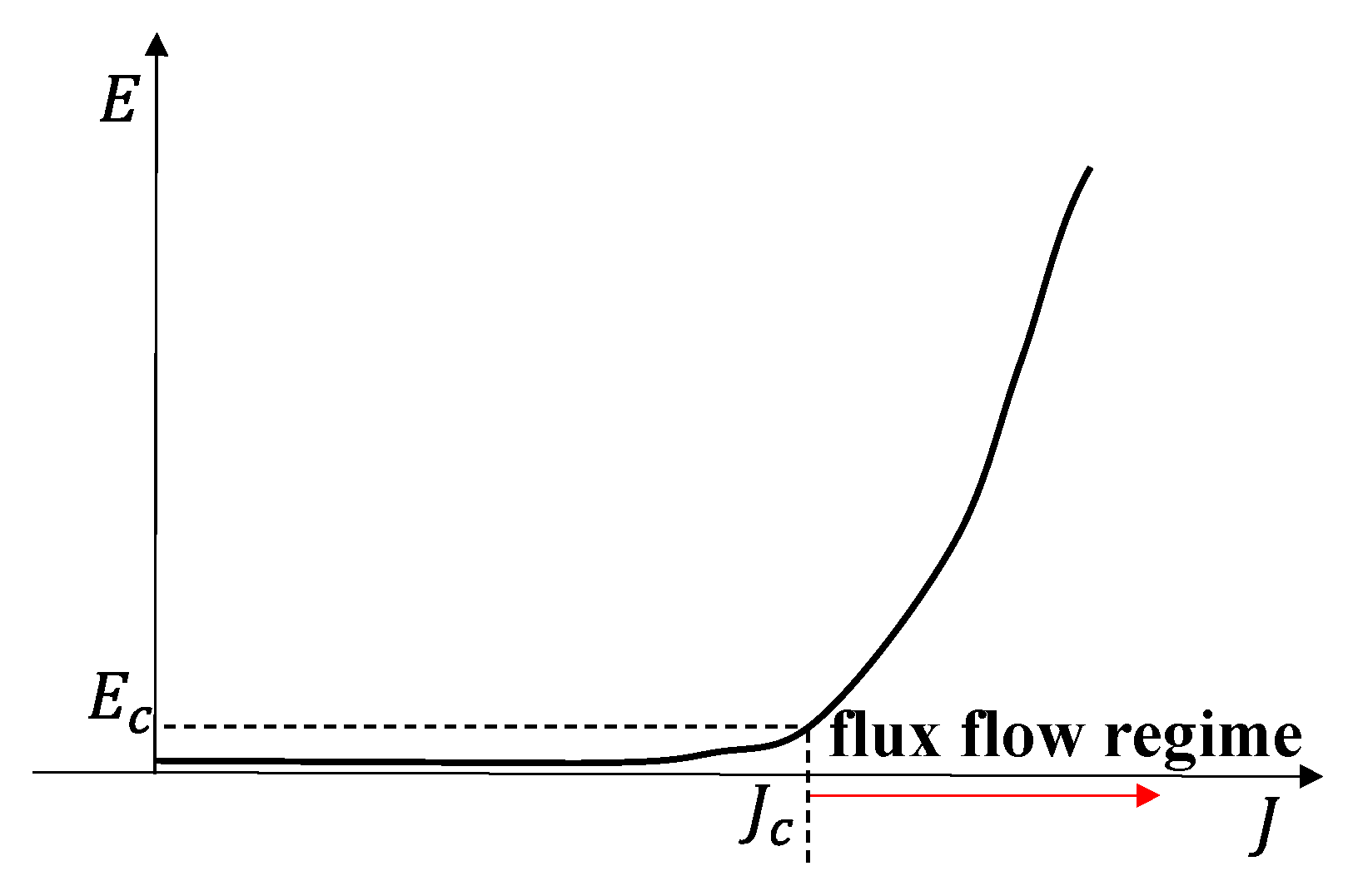
- Geng, J.; Coombs, T. An HTS flux pump operated by directly driving a superconductor into flux flow region in the E–J curve. Supercond. Sci. Technol. 2016, 29, 095004. [Google Scholar] [CrossRef]
- Geng, J.; Coombs, T.A. Modeling methodology for a HTS flux pump using a 2D H-formulation. Supercond. Sci. Technol. 2018, 31, 125015. [Google Scholar] [CrossRef]
- Li, C.; Yang, J.; Shen, B.; Ma, J.; Gawith, J.; Öztürk, Y.; Geng, J.; Coombs, T.A. A HTS flux pump simulation methodology based on the electrical circuit. IEEE Trans. Appl. Supercond. 2020, 30, 4702905. [Google Scholar] [CrossRef]
- Bai, Z.; Ding, S.; Li, C.; Li, C.; Yan, G. A newly developed pulse-type microampere magnetic flux pump. IEEE Trans. Appl. Supercond. 2010, 20, 1667–1670. [Google Scholar]
- Bai, Z.; Yan, G.; Wu, C.; Ding, S.; Chen, C. A novel high temperature superconducting magnetic flux pump for MRI magnets. Cryogenics 2010, 50, 688–692. [Google Scholar] [CrossRef]
- Bai, Z.; Chen, C.; Wu, Y.; Zhen, Z. Effect of various pulse wave forms for pulse-type magnetic flux pump. Cryogenics 2011, 51, 530–533. [Google Scholar] [CrossRef]
- Bai, Z.; Ma, C.; Chen, C.; Pang, Y. Study on the excitation of a Bi-2223 small superconducting coil by a pulse-type magnetic flux pump. IEEE Trans. Appl. Supercond. 2017, 27, 4801705. [Google Scholar] [CrossRef]
- Bai, Z.; Cui, X.; Ma, C. Characteristics of Pumping Current in a YBCO Coil by a Pulse-Type Magnetic Flux Pump. IEEE Trans. Appl. Supercond. 2021, 31, 4602806. [Google Scholar] [CrossRef]
- Wang, W.; Lei, Y.; Huang, S.; Wang, P.; Huang, Z.; Zhou, Q. Charging 2G HTS double pancake coils with a wireless superconducting DC p ower supply for persistent current operation. IEEE Trans. Appl. Supercond. 2018, 28, 0600804. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, W.; Ye, H.; Wang, X.; Gao, Y.; Zhou, Q.; Liu, X.; Lei, Y. Compact linear-motor type flux pumps with different wavelengths for high-temperature superconducting magnets. IEEE Trans. Appl. Supercond. 2020, 30, 5000305. [Google Scholar] [CrossRef]
- Ye, H.; Wang, W.; Zhang, Y.; Wang, X.; Gao, Y.; Zhou, Q.; Liu, X.; Lei, Y. Measuring the output voltage of a linear-motor type flux pump with an insulated HTS coil. IEEE Trans. Appl. Supercond. 2020, 30, 3800105. [Google Scholar] [CrossRef]
- Ye, H.; Wang, W.; Zhang, Y.; Li, H.; Wei, J.; Xu, H.; Zhou, Q.; Liu, X.; Lei, Y. A Linear-Motor Type HTS Flux Pump With Pumped Current Exceeding 640 A. IEEE Trans. Appl. Supercond. 2021, 31, 3800705. [Google Scholar] [CrossRef]
- Zhang, H.; Yang, T.; Li, W.; Xin, Y.; Li, C.; Iacchetti, M.F.; Smith, A.C.; Mueller, M. Origin of the anomalous electromechanical interaction between a moving magnetic dipole and a closed superconducting loop. Supercond. Sci. Technol. 2022, 35, 045009. [Google Scholar] [CrossRef]
- Gawith, J.; Geng, J.; Li, C.; Shen, B.; Zhang, X.; Ma, J.; Coombs, T. A half-bridge HTS transformer–rectifier flux pump with two AC field-controlled switches. Supercond. Sci. Technol. 2018, 31, 085002. [Google Scholar] [CrossRef]
- Leuw, B.; Geng, J.; Rice, J.H.; Moseley, D.A.; Badcock, R.A. A half-wave superconducting transformer-rectifier flux pump using J c (B) switches. Supercond. Sci. Technol. 2022, 35, 035009. [Google Scholar] [CrossRef]
- Sung, H.-J.; Go, B.-S.; Jiang, Z.; Park, M.; Yu, I.-K. Heat loss analysis-based design of a 12 MW wind power generator module having an HTS flux pump exciter. Phys. C Supercond. Appl. 2016, 530, 133–137. [Google Scholar] [CrossRef]
- Sung, H.-J.; Go, B.-S.; Park, H.; Badcock, R.A.; Park, M.; Yu, I.-K. Design, fabrication, and analysis of HTS coils for a 10-kW wind power generator employing a brushless exciter. IEEE Trans. Appl. Supercond. 2017, 27, 5202305. [Google Scholar] [CrossRef]
- Tuvdensuren, O.; Sung, H.; Go, B.; Le, T.; Park, M.; Yu, I. Structural design and heat load analysis of a flux pump-based HTS module coil for a large-scale wind power generator. J. Phys. Conf. Ser. 2018, 1054, 012084. [Google Scholar] [CrossRef]
- Kalsi, S.; Badcock, R.; Hamilton, K.; Storey, J. Homopolar superconducting AC machines, with HTS dynamo driven field coils, for aerospace applications. IOP Conf. Ser. Mater. Sci. Eng. 2020, 756, 012028. [Google Scholar] [CrossRef]
- Kim, J.H.; Quach, H.L.; Boo, C.-J.; Yoon, Y.S.; Jeon, H.; Han, S.; Ko, T.K.; Kim, H.-W.; Jo, Y.-S.; Park, H.J. Fabrication and charging test of HTS field windings using HTS contactless rotary excitation device. IEEE Trans. Appl. Supercond. 2019, 29, 5203207. [Google Scholar] [CrossRef]
- Kim, J.H.; Hyeon, C.J.; Quach, H.L.; Chae, S.H.; Chae, Y.S.; Moon, J.H.; Boo, C.-J.; Yoon, Y.S.; Lee, J.; Jeon, H. Design, analysis, and fabrication of salient field-pole for a 1-kW-class HTS rotating machine. Cryogenics 2019, 97, 126–132. [Google Scholar] [CrossRef]
- Kim, J.H.; Chae, Y.S.; Quach, H.L.; Yoon, Y.S.; Jeon, H.; Han, S.; Ko, T.K.; Lee, J.; Kim, H.-W.; Jo, Y.-S. Fabrication and performance testing of a 1-kW-class high-temperature superconducting generator with a high-temperature superconducting contactless field exciter. Supercond. Sci. Technol. 2020, 33, 095003. [Google Scholar] [CrossRef]
- Parkinson, B.J.; Slade, R.; Mallett, M.J.; Chamritski, V. Development of a cryogen free 1.5 T YBCO HTS magnet for MRI. IEEE Trans. Appl. Supercond. 2012, 23, 4400405. [Google Scholar] [CrossRef]
- Terao, Y.; Ozaki, O.; Ichihara, C.; Kawashima, S.; Hase, T.; Kitaguchi, H.; Kobayashi, S.-i.; Sato, K.-i.; Nakajima, I.; Oonishi, N. Newly designed 3 T MRI magnet wound with Bi-2223 tape conductors. IEEE Trans. Appl. Supercond. 2013, 23, 4400904. [Google Scholar] [CrossRef]
- Parkinson, B.; Bouloukakis, K.; Slade, R. A compact 3 T all HTS cryogen-free MRI system. Supercond. Sci. Technol. 2017, 30, 125009. [Google Scholar] [CrossRef]
- Yokoyama, S.; Miura, H.; Matsuda, T.; Inoue, T.; Morita, Y.; Eguchi, R.; Otake, S.; Tanabe, H.; Sato, S.; Kiss, T. Design and cooling properties of high stable field REBCO superconducting magnet for MRI. IEEE Trans. Appl. Supercond. 2020, 30, 4400904. [Google Scholar] [CrossRef]
- Wang, X.; Wang, W.; Lei, Y.; Gao, Y.; Huang, S.; Liu, X.; Zhou, Q. Electromagnetic design of 1.5 T no-insulation REBCO coil system charged by multiflux pumps for dedicated MRI. IEEE Trans. Appl. Supercond. 2019, 29, 4601805. [Google Scholar] [CrossRef]
- Öztürk, Y.; Shen, B.; Williams, R.; Gawith, J.; Yang, J.; Ma, J.; Carpenter, A.; Coombs, T. Current status in building a compact and mobile HTS MRI instrument. IEEE Trans. Appl. Supercond. 2021, 31, 4400405. [Google Scholar] [CrossRef]
- Baig, T.; Yao, Z.; Doll, D.; Tomsic, M.; Martens, M. Conduction cooled magnet design for 1.5 T, 3.0 T and 7.0 T MRI systems. Supercond. Sci. Technol. 2014, 27, 125012. [Google Scholar] [CrossRef]
- Cosmus, T.C.; Parizh, M. Advances in whole-body MRI magnets. IEEE Trans. Appl. Supercond. 2010, 21, 2104–2109. [Google Scholar] [CrossRef]
- Hahn, S.; Kim, K.; Kim, K.; Hu, X.; Painter, T.; Dixon, I.; Kim, S.; Bhattarai, K.R.; Noguchi, S.; Jaroszynski, J. 45.5-tesla direct-current magnetic field generated with a high-temperature superconducting magnet. Nature 2019, 570, 496–499. [Google Scholar] [CrossRef]
- Miller, J.R. The NHMFL 45-T hybrid magnet system: Past, present, and future. IEEE Trans. Appl. Supercond. 2003, 13, 1385–1390. [Google Scholar] [CrossRef]
- Hamilton, K.; Mataira, R.; Geng, J.; Bumby, C.; Carnegie, D.; Badcock, R. Practical estimation of HTS dynamo losses. IEEE Trans. Appl. Supercond. 2020, 30, 4703105. [Google Scholar] [CrossRef]
- Zhang, H.; Yao, M.; Jiang, Z.; Xin, Y.; Li, Q. Dependence of dynamic loss on critical current and n-value of HTS coated conductors. IEEE Trans. Appl. Supercond. 2019, 29, 8201907. [Google Scholar] [CrossRef]
- Zhang, H.; Wen, Z.; Grilli, F.; Gyftakis, K.; Mueller, M. Alternating current loss of superconductors applied to superconducting electrical machines. Energies 2021, 14, 2234. [Google Scholar] [CrossRef]
- Grilli, F.; Pardo, E.; Stenvall, A.; Nguyen, D.N.; Yuan, W.; Gömöry, F. Computation of losses in HTS under the action of varying magnetic fields and currents. IEEE Trans. Appl. Supercond. 2013, 24, 78–110. [Google Scholar] [CrossRef]
- Morandi, A.; Russo, G.; Fabbri, M.; Soldati, L. Energy balance, efficiency and operational limits of the dynamo type flux pump. Supercond. Sci. Technol. 2022, 35, 065011. [Google Scholar] [CrossRef]
- Walsh, R.M.; Slade, R.; Pooke, D.; Hoffmann, C. Characterization of current stability in an HTS NMR system energized by an HTS flux pump. IEEE Trans. Appl. Supercond. 2013, 24, 4600805. [Google Scholar] [CrossRef]
- Jeon, H.; Lee, J.; Han, S.; Kim, J.H.; Hyeon, C.J.; Kim, H.M.; Kang, H.; Ko, T.K.; Yoon, Y.S. PID control of an electromagnet-based rotary HTS flux pump for maintaining constant field in HTS synchronous motors. IEEE Trans. Appl. Supercond. 2018, 28, 5207605. [Google Scholar] [CrossRef]
- Geng, J.; Wang, B.; Baghdadi, M.; Li, J.; Shen, B.; Zhang, H.; Li, C.; Zhang, X.; Coombs, T.A. Feedback control of a rectifier type HTS flux pump: Stabilizing load current with minimized losses. IEEE Trans. Appl. Supercond. 2017, 27, 0500104. [Google Scholar] [CrossRef]
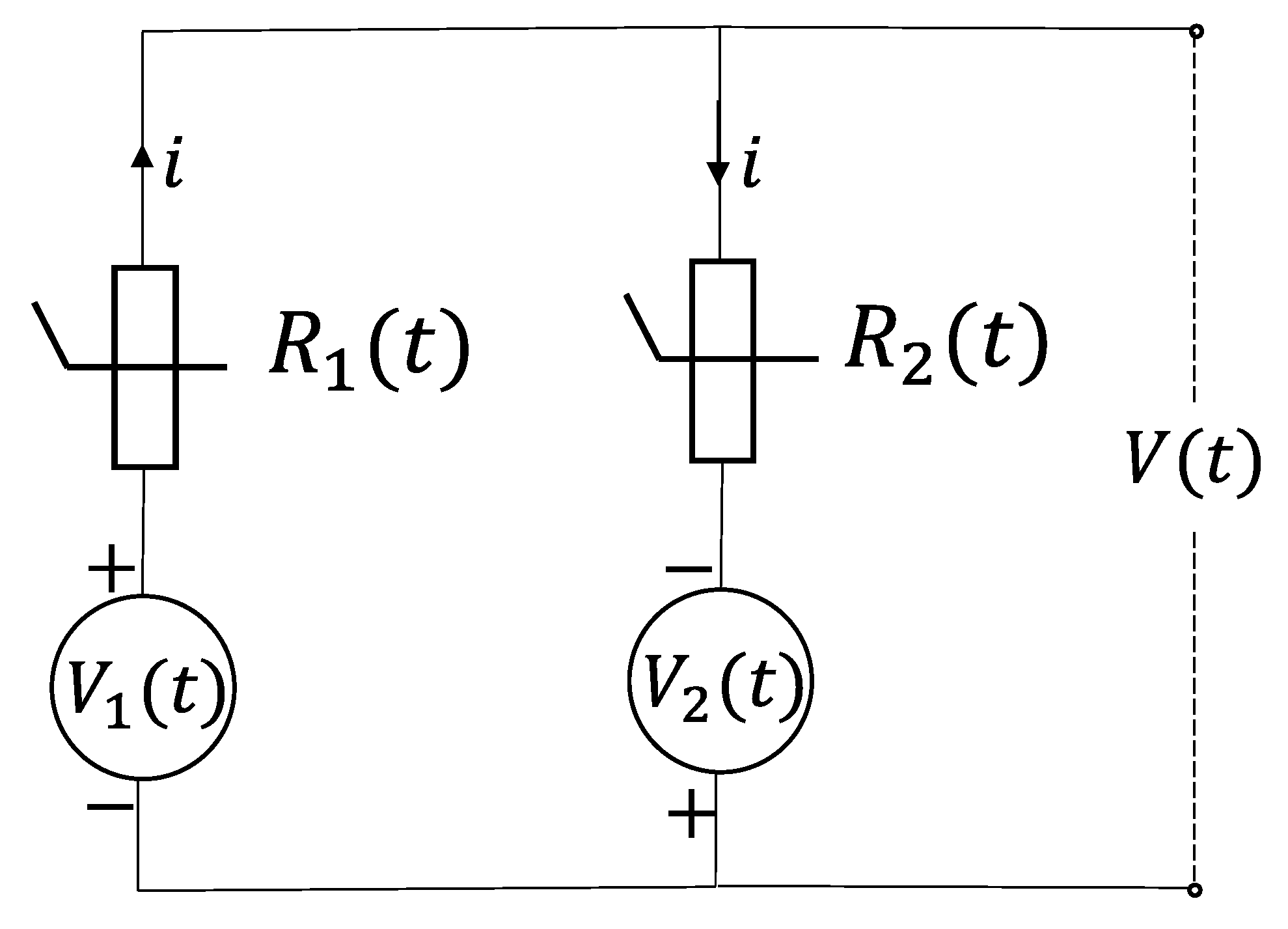

| Type | Pros | Cons | Notes |
|---|---|---|---|
| Rotary HTS flux pump | Simplest structure, easiest operation, least material consumption. | Mechanical drive is required to create relative motion between static and moving parts. | Losses are presented and difficult to mitigate due to the single loop topology. |
| Linear HTS flux pump | Concise configuration. The external field can be tuned easily. | Magnetic framework is required to divert flux path. | |
| AC field switched transformer-rectifier | The flux pumping process consists of several independent steps, thus highly flexible. | The system is sophisticated and imposes many operational considerations. | Losses are presented but can be modulated since the transport current and charging current are separated. |
| Self-regulating transformer-rectifier | It can operate at completely current-driven mode without any external field involved. | Potentially unstable due to the sharp E-J relation. | |
| Power electronics switched | Power electronics devices such as MOSFETs, IGBTs are cheap and widely available. | Commonly in power electronics devices, the on-state resistance is inevitable. | The operating frequency and output ripple are highly associated with electronics device standards. |
| Strength | 1.5 T | 3.0 T | 7.0 T |
|---|---|---|---|
| Operating temperature (K) | 4.2 | 4.2 | 4.2 |
| Amount of LHe (L) | 1700 | <3000 | 4000 |
| Length including 10 cm for cryogenics (m) | 1.25–1.70 | 1.60–1.80 | 3.0 |
| Stored energy (MJ) | 2–4 | 10–15 | 50–90 |
| Peak magnetic field * (T) | <9 | <9 | <9 |
| Coil operating current density * (A/mm2) | <250 | <250 | <250 |
| Ampere-length (kA-km) | 15–25 | 35–60 | 120–180 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wen, Z.; Zhang, H.; Mueller, M. High Temperature Superconducting Flux Pumps for Contactless Energization. Crystals 2022, 12, 766. https://doi.org/10.3390/cryst12060766
Wen Z, Zhang H, Mueller M. High Temperature Superconducting Flux Pumps for Contactless Energization. Crystals. 2022; 12(6):766. https://doi.org/10.3390/cryst12060766
Chicago/Turabian StyleWen, Zezhao, Hongye Zhang, and Markus Mueller. 2022. "High Temperature Superconducting Flux Pumps for Contactless Energization" Crystals 12, no. 6: 766. https://doi.org/10.3390/cryst12060766
APA StyleWen, Z., Zhang, H., & Mueller, M. (2022). High Temperature Superconducting Flux Pumps for Contactless Energization. Crystals, 12(6), 766. https://doi.org/10.3390/cryst12060766







