Analysis of Electromagnetic Effects on Vibration of Functionally Graded GPLs Reinforced Piezoelectromagnetic Plates on an Elastic Substrate
Abstract
:1. Introduction
2. Plate Configuration
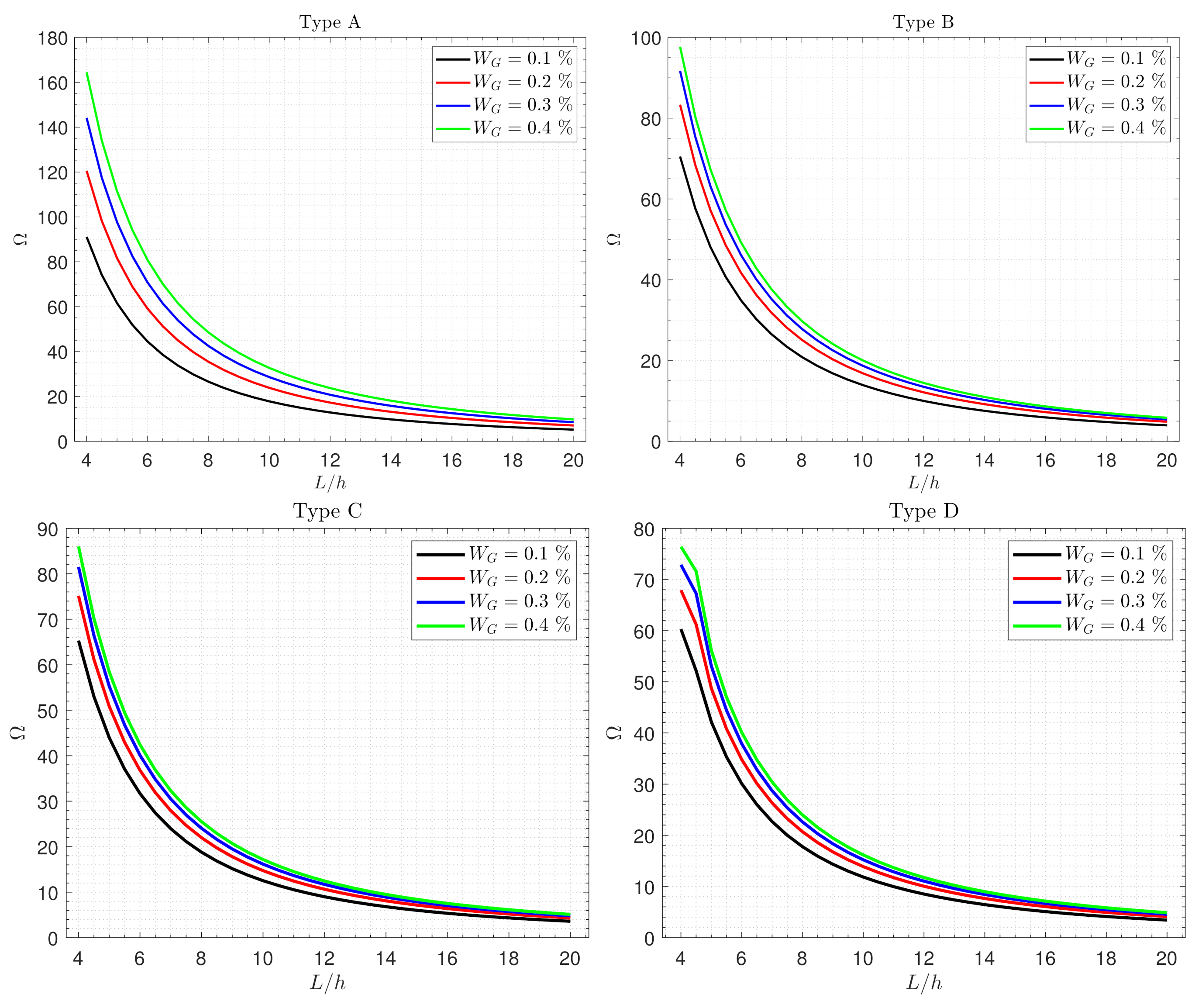
2.1. Type A
2.2. Type B
2.3. Type C
2.4. Type D
3. Constitutive Equations
4. Governing Equations
5. Solution Procedure
6. Results and Discussion
6.1. Verification
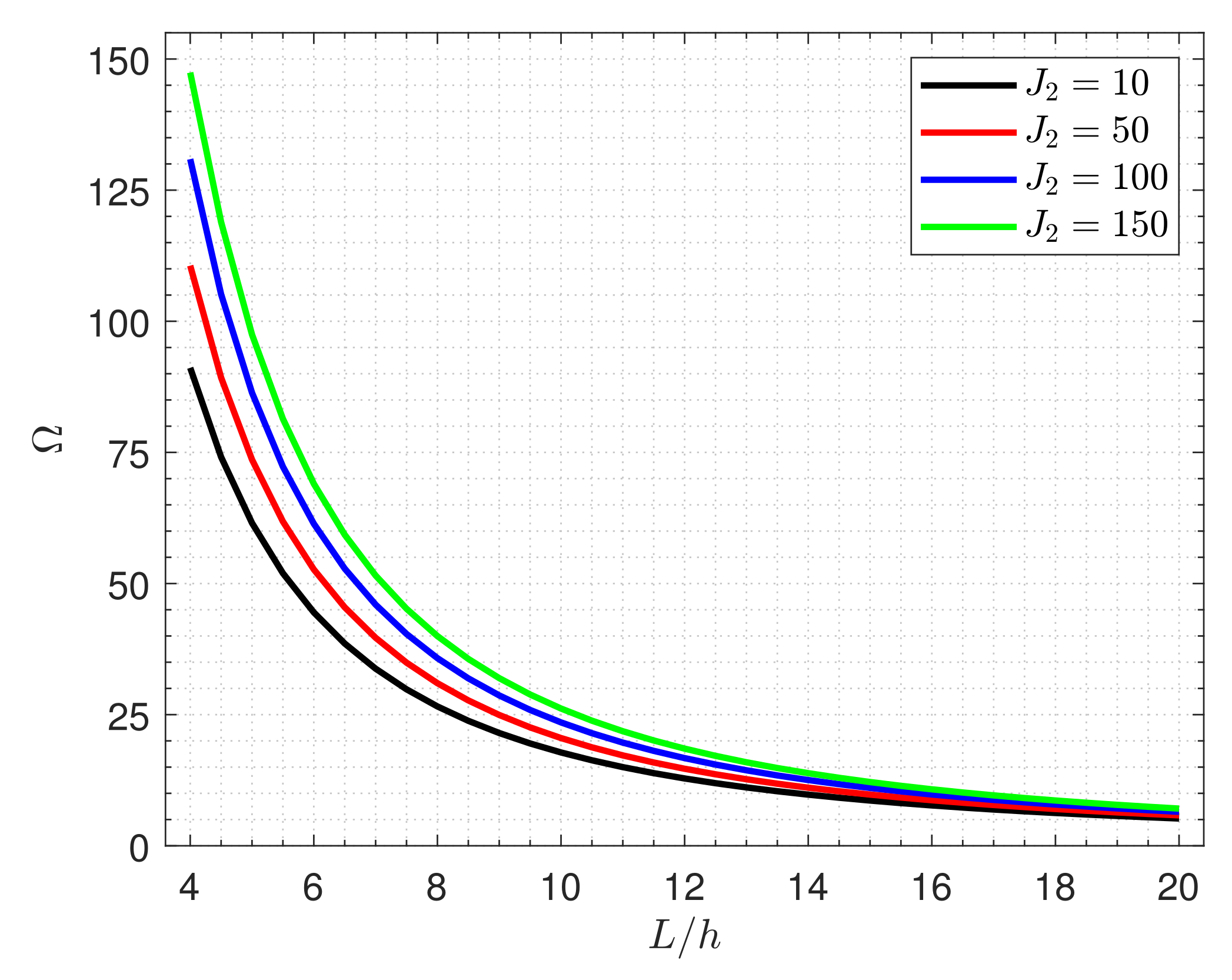
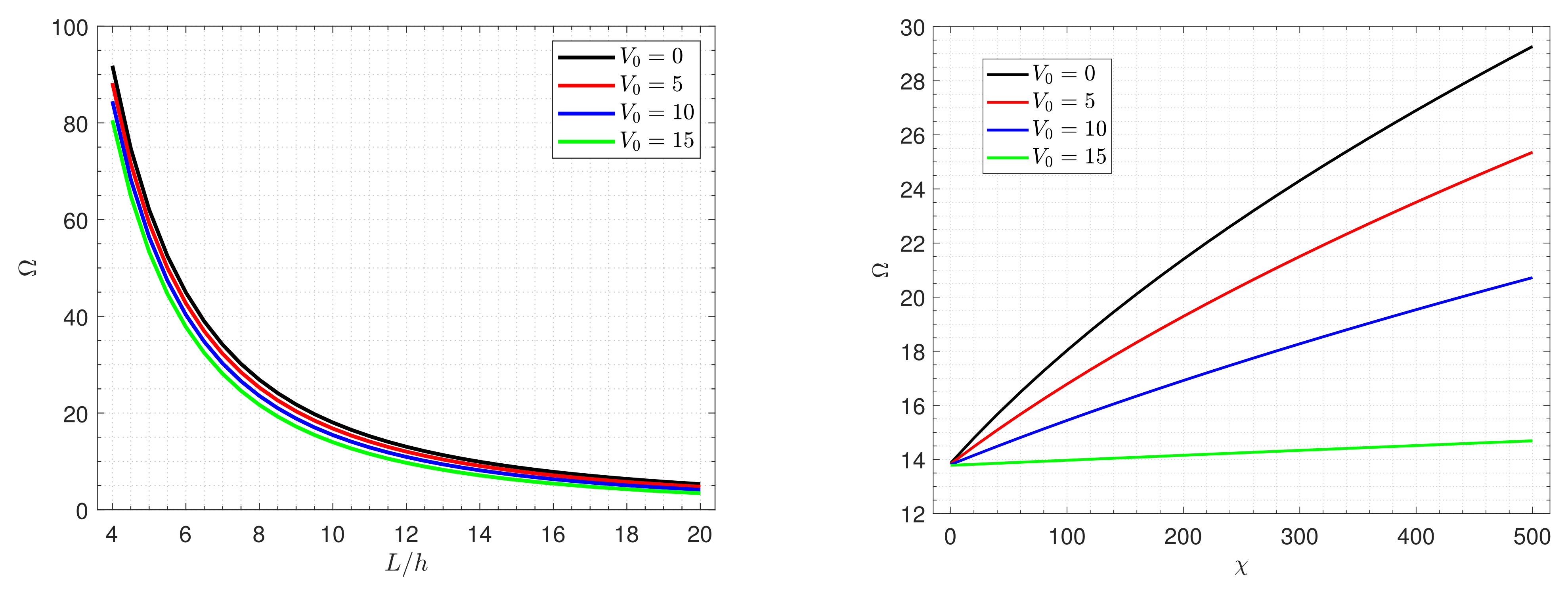
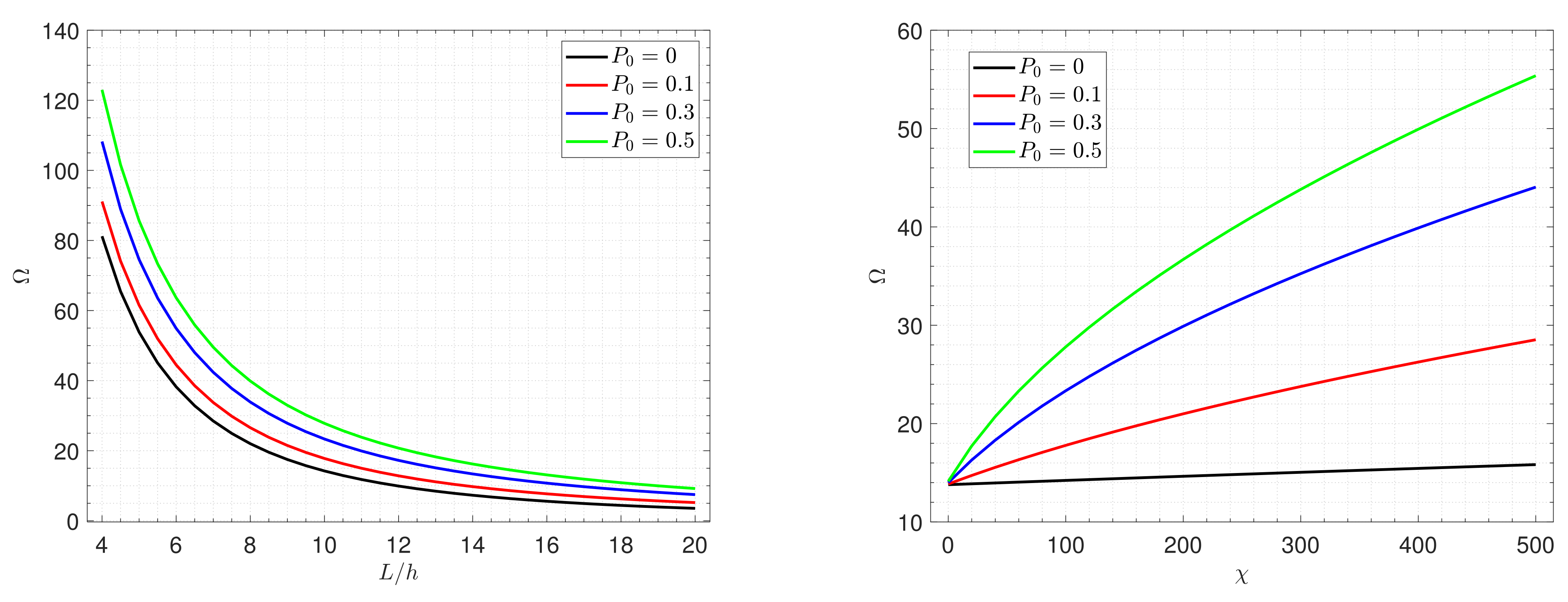
6.2. Parametric Results
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sun, J.; Xu, X.; Lim, C.W.; Zhou, Z.; Xiao, S. Accurate thermo-electro-mechanical buckling of shear deformable piezoelectric fiber-reinforced composite cylindrical shells. Compos. Struct. 2016, 141, 221–231. [Google Scholar] [CrossRef]
- Sobhy, M. Piezoelectric bending of gpl-reinforced annular and circular sandwich nanoplates with fg porous core integrated with sensor and actuator using dqm. Arch. Civ. Mech. Eng. 2021, 21, 1–18. [Google Scholar] [CrossRef]
- Phung-Van, P.; Tran, L.V.; Ferreira, A.J.M.; Nguyen-Xuan, H.; Abdel-Wahab, M.J.N.D. Nonlinear transient isogeometric analysis of smart piezoelectric functionally graded material plates based on generalized shear deformation theory under thermo-electro-mechanical loads. Nonlinear Dyn. 2017, 87, 879–894. [Google Scholar] [CrossRef]
- Phung-Van, P.; Nguyen, L.B.; Tran, L.V.; Dinh, T.D.; Thai, C.H.; Bordas, S.P.A.; Abdel-Wahab, M.; Nguyen-Xuan, H. An efficient computational approach for control of nonlinear transient responses of smart piezoelectric composite plates. Int. J. Non-Linear Mech. 2015, 76, 190–202. [Google Scholar] [CrossRef] [Green Version]
- Phung-Van, P.; De Lorenzis, L.; Thai, C.H.; Abdel-Wahab, M.; Nguyen-Xuan, H. Analysis of laminated composite plates integrated with piezoelectric sensors and actuators using higher-order shear deformation theory and isogeometric finite elements. Comput. Mater. Sci. 2015, 96, 495–505. [Google Scholar] [CrossRef]
- Sahu, M.; Hajra, S.; Lee, K.; Deepti, P.L.; Mistewicz, K.; Kim, H.J. Piezoelectric nanogenerator based on lead-free flexible PVDF-barium titanate composite films for driving low power electronics. Crystals 2021, 11, 85. [Google Scholar] [CrossRef]
- Yan, X.; Zheng, M.; Zhu, M.; Hou, Y. Soft and hard piezoelectric ceramics for vibration energy harvesting. Crystals 2020, 10, 907. [Google Scholar] [CrossRef]
- Hu, K.-Q.; Li, G.-Q. Electro-magneto-elastic analysis of a piezoelectromagnetic strip with a finite crack under longitudinal shear. Mech. Mater. 2005, 37, 925–934. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, Q.; Yang, X.; Ma, J. Size-dependent vibration of laminated composite nanoplate with piezo-magnetic face sheets. Eng. Comput. 2021, 1–17. [Google Scholar] [CrossRef]
- Bin, W.; Jiangong, Y.; Cunfu, H. Wave propagation in non-homogeneous magneto-electro-elastic plates. J. Sound Vib. 2008, 317, 250–264. [Google Scholar] [CrossRef]
- Khorasani, M.; Soleimani-Javid, Z.; Arshid, E.; Amir, S.; Civalek, O. Vibration analysis of graphene nanoplatelets’ reinforced composite plates integrated by piezo-electromagnetic patches on the piezo-electromagnetic media. Waves Random Complex Media 2021, 1–31. [Google Scholar] [CrossRef]
- Naskar, S.; Shingare, K.B.; Mondal, S.; Mukhopadhyay, T. Flexoelectricity and surface effects on coupled electromechanical responses of graphene reinforced functionally graded nanocomposites: A unified size-dependent semi-analytical framework. Mech. Syst. Signal Process. 2022, 169, 108757. [Google Scholar] [CrossRef]
- Samadi, A.; Hosseini, S.M.; Mohseni, M. Investigation of the electromagnetic microwaves absorption and piezoelectric properties of electrospun Fe3O4-GO/PVDF hybrid nanocomposites. Org. Electron. 2018, 59, 149–155. [Google Scholar] [CrossRef]
- Ke, L.-L.; Wang, Y.-S.; Yang, J.; Kitipornchai, S. Free vibration of size-dependent magneto-electro-elastic nanoplates based on the nonlocal theory. Acta Mech. Sin. 2014, 30, 516–525. [Google Scholar] [CrossRef]
- Ke, L.-L.; Wang, Y.-S. Free vibration of size-dependent magneto-electro-elastic nanobeams based on the nonlocal theory. Phys. E Low-Dimens. Syst. Nanostruct. 2014, 63, 52–61. [Google Scholar] [CrossRef]
- Li, Y.S.; Ma, P.; Wang, W. Bending, buckling, and free vibration of magnetoelectroelastic nanobeam based on nonlocal theory. J. Intell. Mater. Syst. Struct. 2016, 27, 1139–1149. [Google Scholar] [CrossRef]
- Pan, E.; Han, F. Exact solution for functionally graded and layered magneto-electro-elastic plates. Int. J. Eng. Sci. 2005, 43, 321–339. [Google Scholar] [CrossRef]
- Farajpour, A.; Yazdi, M.R.H.; Rastgoo, A.; Loghmani, M.; Mohammadi, M. Nonlocal nonlinear plate model for large amplitude vibration of magneto-electro-elastic nanoplates. Compos. Struct. 2016, 140, 323–336. [Google Scholar] [CrossRef]
- Farajpour, A.; Rastgoo, A.; Farajpour, M.R. Nonlinear buckling analysis of magneto-electro-elastic cnt-mt hybrid nanoshells based on the nonlocal continuum mechanics. Compos. Struct. 2017, 180, 179–191. [Google Scholar] [CrossRef]
- Jamalpoor, A.; Ahmadi-Savadkoohi, A.; Hosseini, M.; Hosseini-Hashemi, S. Free vibration and biaxial buckling analysis of double magneto-electro-elastic nanoplate-systems coupled by a visco-pasternak medium via nonlocal elasticity theory. Eur. J. Mech. A/Solids 2017, 63, 84–98. [Google Scholar] [CrossRef]
- Mehditabar, A.; Rahimi, G.H.; Sadrabadi, S.A. Three-dimensional magneto-thermo-elastic analysis of functionally graded cylindrical shell. Appl. Math. Mech. 2017, 38, 479–494. [Google Scholar] [CrossRef]
- Zenkour, A.M.; Aljadani, M.H. Buckling analysis of actuated functionally graded piezoelectric plates via a quasi-3d refined theory. Mech. Mater. 2020, 151, 103632. [Google Scholar] [CrossRef]
- Meskini, M.; Ghasemi, A.R. Electro-magnetic potential effects on free vibration of rotating circular cylindrical shells of functionally graded materials with laminated composite core and piezo electro-magnetic two face sheets. J. Sandw. Struct. Mater. 2021, 23, 2772–2797. [Google Scholar] [CrossRef]
- Abazid, M.A.; Sobhy, M. Thermo-electro-mechanical bending of FG piezoelectric microplates on Pasternak foundation based on a four-variable plate model and the modified couple stress theory. Microsyst. Technol. 2018, 24, 1227–1245. [Google Scholar] [CrossRef]
- Monaco, G.T.; Fantuzzi, N.; Fabbrocino, F.; Luciano, R. Critical temperatures for vibrations and buckling of magneto-electro-elastic nonlocal strain gradient plates. Nanomaterials 2021, 11, 87. [Google Scholar] [CrossRef]
- Monaco, G.T.; Fantuzzi, N.; Fabbrocino, F.; Luciano, R. Trigonometric solution for the bending analysis of magneto-electro-elastic strain gradient nonlocal nanoplates in hygro-thermal environment. Mathematics 2021, 9, 567. [Google Scholar] [CrossRef]
- Chen, J.; Guo, J.; Pan, E. Wave propagation in magneto-electro-elastic multilayered plates with nonlocal effect. J. Sound Vib. 2017, 400, 550–563. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Dabbagh, A. Wave dispersion characteristics of rotating heterogeneous magneto-electro-elastic nanobeams based on nonlocal strain gradient elasticity theory. J. Electromagn. Waves Appl. 2018, 32, 138–169. [Google Scholar] [CrossRef]
- Abazid, M.A. The nonlocal strain gradient theory for hygrothermo-electromagnetic effects on buckling, vibration and wave propagation in piezoelectromagnetic nanoplates. Int. J. Appl. Mech. 2019, 11, 1950067. [Google Scholar] [CrossRef]
- Arefi, M.; Zenkour, A.M. Wave propagation analysis of a functionally graded magneto-electro-elastic nanobeam rest on visco-pasternak foundation. Mech. Res. Commun. 2017, 79, 51–62. [Google Scholar] [CrossRef]
- Sobhy, M. Analytical buckling temperature prediction of fg piezoelectric sandwich plates with lightweight core. Mater. Res. Express 2021, 8, 095704. [Google Scholar] [CrossRef]
- Sobhy, M. Stability analysis of smart FG sandwich plates with auxetic core. Int. J. Appl. Mech. 2021, 13, 2150093. [Google Scholar] [CrossRef]
- Potts, J.R.; Dreyer, D.R.; Bielawski, C.W.; Ruoff, R.S. Graphene-based polymer nanocomposites. Polymer 2011, 52, 5–25. [Google Scholar] [CrossRef] [Green Version]
- Papageorgiou, D.G.; Kinloch, I.A.; Young, R.J. Mechanical properties of graphene and graphene-based nanocomposites. Prog. Mater. Sci. 2017, 90, 75–127. [Google Scholar] [CrossRef]
- Yang, J.; Zhang, Y.; Li, Y.; Wang, Z.; Wang, W.; An, Q.; Tong, W. Piezoelectric nanogenerators based on graphene oxide/pvdf electrospun nanofiber with enhanced performances by in-situ reduction. Mater. Today Commun. 2021, 26, 101629. [Google Scholar] [CrossRef]
- Forsat, M.; Musharavati, F.; Eltai, E.; Zain, A.M.; Mobayen, S.; Mohamed, A.M. Vibration characteristics of microplates with GNPs-reinforced epoxy core bonded to piezoelectric-reinforced CNTs patches. Adv. Nano Res. 2021, 11, 115–140. [Google Scholar]
- Thai, C.H.; Phung-Van, P. A meshfree approach using naturally stabilized nodal integration for multilayer FG GPLRC complicated plate structures. Eng. Anal. Bound. Elem. 2020, 117, 346–358. [Google Scholar] [CrossRef]
- Thai, C.H.; Ferreira, A.J.M.; Tran, T.D.; Phung-Van, P. Free vibration, buckling and bending analyses of multilayer functionally graded graphene nanoplatelets reinforced composite plates using the NURBS formulation. Compos. Struct. 2019, 220, 749–759. [Google Scholar] [CrossRef]
- Phung-Van, P.; Lieu, Q.X.; Ferreira, A.J.M.; Thai, C.H. A refined nonlocal isogeometric model for multilayer functionally graded graphene platelet-reinforced composite nanoplates. Thin-Walled Struct. 2021, 164, 107862. [Google Scholar] [CrossRef]
- Mao, J.J.; Lu, H.M.; Zhang, W.; Lai, S.K. Vibrations of graphene nanoplatelet reinforced functionally gradient piezoelectric composite microplate based on nonlocal theory. Compos. Struct. 2020, 236, 111813. [Google Scholar] [CrossRef]
- Sobhy, M. Magneto-electro-thermal bending of fg-graphene reinforced polymer doubly-curved shallow shells with piezoelectromagnetic faces. Compos. Struct. 2018, 203, 844–860. [Google Scholar] [CrossRef]
- Mao, J.-J.; Zhang, W. Linear and nonlinear free and forced vibrations of graphene reinforced piezoelectric composite plate under external voltage excitation. Compos. Struct. 2018, 203, 551–565. [Google Scholar] [CrossRef]
- Mao, J.-J.; Zhang, W. Buckling and post-buckling analyses of functionally graded graphene reinforced piezoelectric plate subjected to electric potential and axial forces. Compos. Struct. 2019, 216, 392–405. [Google Scholar] [CrossRef]
- Sobhy, M.; Abazid, M.A.; Mukahal, F.H.H.A. Electro-thermal buckling of fg graphene platelets-strengthened piezoelectric beams under humid conditions. Adv. Mech. Eng. 2022. [Google Scholar] [CrossRef]
- Abolhasani, M.M.; Shirvanimoghaddam, K.; Naebe, M. Pvdf/graphene composite nanofibers with enhanced piezoelectric performance for development of robust nanogenerators. Compos. Sci. Technol. 2017, 138, 49–56. [Google Scholar] [CrossRef] [Green Version]
- Xu, K.; Wang, K.; Zhao, W.; Bao, W.; Liu, E.; Ren, Y.; Wang, M.; Fu, Y.; Zeng, J.; Li, Z.; et al. The positive piezoconductive effect in graphene. Nat. Commun. 2015, 6, 1–6. [Google Scholar] [CrossRef] [Green Version]
- Al Mukahal, F.H.H.; Sobhy, M. Wave propagation and free vibration of FG graphene platelets sandwich curved beam with auxetic core resting on viscoelastic foundation via DQM. Arch. Civ. Mech. Eng. 2022, 22, 1–21. [Google Scholar] [CrossRef]
- Allam, M.N.M.; Radwan, A.F.; Sobhy, M. Hygrothermal deformation of spinning FG graphene sandwich cylindrical shells having an auxetic core. Eng. Struct. 2022, 251, 113433. [Google Scholar] [CrossRef]
- Sobhy, M.; Alakel Abazid, M. Mechanical and thermal buckling of FG-GPLs sandwich plates with negative Poisson’s ratio honeycomb core on an elastic substrate. Eur. Phys. J. Plus 2022, 137, 1–21. [Google Scholar] [CrossRef]
- Shimpi, R.P. Refined plate theory and its variants. AIAA J. 2002, 40, 137–146. [Google Scholar] [CrossRef]
- Zenkour, A.M.; Sobhy, M. Axial magnetic field effect on wave propagation in bi-layer fg graphene platelet-reinforced nanobeams. Eng. Comput. 2021, 37, 1–17. [Google Scholar] [CrossRef]
- Reddy, J.N. A simple higher-order theory for laminated composite plates. J. Appl. Mech. Dec. 1984, 51, 745–752. [Google Scholar] [CrossRef]
- Touratier, M. An efficient standard plate theory. Int. J. Eng. Sci. 1991, 29, 901–916. [Google Scholar] [CrossRef]
- Karama, M.; Afaq, K.S.; Mistou, S. Mechanical behaviour of laminated composite beam by the new multi-layered laminated composite structures model with transverse shear stress continuity. Int. J. Solids Struct. 2003, 40, 1525–1546. [Google Scholar] [CrossRef]
- Zhang, S.; Xia, R.; Lebrun, L.; Anderson, D.; Shrout, T.R. Piezoelectric materials for high power, high temperature applications. Mater. Lett. 2005, 59, 3471–3475. [Google Scholar] [CrossRef]
- Thai, H.-T.; Choi, D.-H. A refined plate theory for functionally graded plates resting on elastic foundation. Compos. Sci. Technol. 2011, 71, 1850–1858. [Google Scholar] [CrossRef]
- Hasani Baferani, A.; Saidi, A.R.; Ehteshami, H. Accurate solution for free vibration analysis of functionally graded thick rectangular plates resting on elastic foundation. Compos. Struct. 2011, 93, 1842–1853. [Google Scholar] [CrossRef]




| Boundary Conditions | The Functions | ||||
|---|---|---|---|---|---|
| S | S | S | S | ||
| C | S | S | S | ||
| C | C | S | S | ||
| C | C | C | S | ||
| C | C | C | C | ||
| n | Present | Published | ||||
|---|---|---|---|---|---|---|
| TDPT | SDPT | RDPT | Ref. [56] | Ref. [57] | ||
| 0 | 0.20 | 0.41543 | 0.41550 | 0.41547 | 0.4154 | 0.4154 |
| 0.15 | 0.24543 | 0.24545 | 0.24544 | 0.2454 | 0.2454 | |
| 0.10 | 0.11346 | 0.11346 | 0.11346 | 0.1135 | 0.1134 | |
| 0.05 | 0.02910 | 0.02910 | 0.02910 | 0.0291 | 0.0291 | |
| 0.5 | 0.20 | 0.35530 | 0.35535 | 0.35533 | 0.3553 | 0.3606 |
| 0.15 | 0.20913 | 0.20915 | 0.20914 | 0.2091 | 0.2121 | |
| 0.10 | 0.09636 | 0.09637 | 0.09637 | 0.0964 | 0.0975 | |
| 0.05 | 0.02466 | 0.02466 | 0.02466 | 0.0247 | 0.0249 | |
| 1 | 0.20 | 0.32066 | 0.32070 | 0.32069 | 0.3207 | 0.3299 |
| 0.15 | 0.18862 | 0.18864 | 0.18863 | 0.1886 | 0.1939 | |
| 0.10 | 0.08687 | 0.08687 | 0.08687 | 0.0869 | 0.0891 | |
| 0.05 | 0.02223 | 0.02223 | 0.02223 | 0.0222 | 0.0227 | |
| 2 | 0.20 | 0.28935 | 0.28934 | 0.28934 | 0.2894 | 0.3016 |
| 0.15 | 0.17067 | 0.17066 | 0.17066 | 0.1707 | 0.1778 | |
| 0.10 | 0.07879 | 0.07879 | 0.07879 | 0.0788 | 0.0819 | |
| 0.05 | 0.02020 | 0.02019 | 0.02019 | 0.0202 | 0.0209 | |
| 5 | 0.20 | 0.26676 | 0.26657 | 0.26662 | 0.2668 | 0.2765 |
| 0.15 | 0.15894 | 0.15887 | 0.15888 | 0.1589 | 0.1648 | |
| 0.10 | 0.07405 | 0.07403 | 0.07403 | 0.0740 | 0.0767 | |
| 0.05 | 0.01910 | 0.01910 | 0.01910 | 0.0191 | 0.0197 | |
| n | Present | Published | ||||
|---|---|---|---|---|---|---|
| TDPT | SDPT | RDPT | Ref. [56] | Ref. [57] | ||
| 0 | 0.20 | 0.42728 | 0.42735 | 0.42733 | 0.4273 | 0.4273 |
| 0.15 | 0.25188 | 0.25190 | 0.25189 | 0.2519 | 0.2519 | |
| 0.10 | 0.11625 | 0.11626 | 0.11626 | 0.1163 | 0.1162 | |
| 0.05 | 0.02979 | 0.02979 | 0.02979 | 0.0298 | 0.0298 | |
| 0.5 | 0.20 | 0.37048 | 0.37053 | 0.37051 | 0.3705 | 0.3758 |
| 0.15 | 0.21744 | 0.21745 | 0.21745 | 0.2174 | 0.2204 | |
| 0.10 | 0.09998 | 0.09999 | 0.09999 | 0.1000 | 0.1012 | |
| 0.05 | 0.02556 | 0.02556 | 0.02556 | 0.0256 | 0.0258 | |
| 1 | 0.20 | 0.33825 | 0.33829 | 0.33828 | 0.3382 | 0.3476 |
| 0.15 | 0.19827 | 0.19829 | 0.19828 | 0.1983 | 0.2036 | |
| 0.10 | 0.09108 | 0.09109 | 0.09109 | 0.0911 | 0.0933 | |
| 0.05 | 0.02327 | 0.02327 | 0.02327 | 0.0233 | 0.0238 | |
| 2 | 0.20 | 0.30977 | 0.30976 | 0.30976 | 0.3098 | 0.3219 |
| 0.15 | 0.18186 | 0.18185 | 0.18185 | 0.1819 | 0.1889 | |
| 0.10 | 0.08367 | 0.08367 | 0.08367 | 0.0837 | 0.0867 | |
| 0.05 | 0.02140 | 0.02140 | 0.02140 | 0.0214 | 0.0221 | |
| 5 | 0.20 | 0.29019 | 0.29002 | 0.29006 | 0.2902 | 0.2999 |
| 0.15 | 0.17164 | 0.17157 | 0.17159 | 0.1716 | 0.1775 | |
| 0.10 | 0.07954 | 0.07952 | 0.07952 | 0.0795 | 0.0821 | |
| 0.05 | 0.02045 | 0.02045 | 0.02045 | 0.0205 | 0.0210 | |
| n | Present | Published | ||||
|---|---|---|---|---|---|---|
| TDPT | SDPT | RDPT | Ref. [56] | Ref. [57] | ||
| 0 | 0.20 | 0.61618 | 0.61623 | 0.61621 | 0.6162 | 0.6162 |
| 0.15 | 0.35604 | 0.35606 | 0.35605 | 0.3560 | 0.3560 | |
| 0.10 | 0.16188 | 0.16188 | 0.16188 | 0.1619 | 0.1619 | |
| 0.05 | 0.04110 | 0.04110 | 0.04110 | 0.0411 | 0.0411 | |
| 0.5 | 0.20 | 0.59543 | 0.59545 | 0.59544 | 0.5954 | 0.6026 |
| 0.15 | 0.34236 | 0.34237 | 0.34237 | 0.3424 | 0.3460 | |
| 0.10 | 0.15500 | 0.15500 | 0.15500 | 0.1550 | 0.1563 | |
| 0.05 | 0.03924 | 0.03924 | 0.03924 | 0.0392 | 0.0395 | |
| 1 | 0.20 | 0.58553 | 0.58555 | 0.58554 | 0.5855 | 0.5978 |
| 0.15 | 0.33613 | 0.33614 | 0.33613 | 0.3361 | 0.3422 | |
| 0.10 | 0.15197 | 0.15197 | 0.15197 | 0.1520 | 0.1542 | |
| 0.05 | 0.03844 | 0.03844 | 0.03844 | 0.0384 | 0.0388 | |
| 2 | 0.20 | 0.58023 | 0.58023 | 0.58023 | 0.5802 | 0.5970 |
| 0.15 | 0.33298 | 0.33298 | 0.33297 | 0.3330 | 0.3412 | |
| 0.10 | 0.15051 | 0.15051 | 0.15051 | 0.1505 | 0.1535 | |
| 0.05 | 0.03807 | 0.03807 | 0.03807 | 0.0381 | 0.0386 | |
| 5 | 0.20 | 0.58349 | 0.58344 | 0.58345 | 0.5835 | 0.5993 |
| 0.15 | 0.33495 | 0.33492 | 0.33493 | 0.3350 | 0.3427 | |
| 0.10 | 0.15153 | 0.15152 | 0.15152 | 0.1515 | 0.1543 | |
| 0.05 | 0.03836 | 0.03836 | 0.03836 | 0.0384 | 0.0388 | |
| Present | Published | ||
|---|---|---|---|
| Ref. [29] | Ref. [14] | ||
| 1, 1 | 0.3830 | 0.3829 | 0.3698 |
| 1, 2 | 0.9330 | 0.9329 | 0.9247 |
| 2, 2 | 1.4571 | 1.4568 | 1.4800 |
| Type | SSSS | CSSS | CCSS | CCCS | CCCC | |
|---|---|---|---|---|---|---|
| Type A | 10 | 15.60604 | 20.17570 | 20.69866 | 24.26245 | 24.59618 |
| 15 | 7.69551 | 9.79339 | 9.97522 | 11.66440 | 11.79149 | |
| 20 | 4.70668 | 5.90897 | 5.97521 | 6.96373 | 7.01009 | |
| 25 | 3.23406 | 4.01599 | 4.03570 | 4.68846 | 4.70050 | |
| 30 | 2.38941 | 2.94103 | 2.93993 | 3.40587 | 3.40216 | |
| Type B | 10 | 13.91824 | 17.85522 | 17.87654 | 21.13514 | 20.96808 |
| 15 | 6.64805 | 8.53231 | 8.55383 | 10.15398 | 10.10229 | |
| 20 | 3.94721 | 5.03870 | 5.04273 | 5.98381 | 5.95221 | |
| 25 | 2.64537 | 3.35515 | 3.34959 | 3.96789 | 3.94248 | |
| 30 | 1.91426 | 2.41261 | 2.40245 | 2.83992 | 2.81771 | |
| Type C | 10 | 11.02065 | 14.12403 | 14.54444 | 16.95287 | 17.24037 |
| 15 | 5.36317 | 6.79055 | 6.94550 | 8.08732 | 8.20204 | |
| 20 | 3.24892 | 4.06724 | 4.13150 | 4.79916 | 4.84784 | |
| 25 | 2.21630 | 2.74827 | 2.77484 | 3.21523 | 3.23516 | |
| 30 | 1.62823 | 2.00317 | 2.01206 | 2.32603 | 2.33213 | |
| Type D | 10 | 10.54877 | 13.31021 | 13.62532 | 15.82989 | 16.05832 |
| 15 | 5.16099 | 6.42669 | 6.52518 | 7.56204 | 7.63701 | |
| 20 | 3.14148 | 3.86961 | 3.90012 | 4.50712 | 4.53008 | |
| 25 | 2.15131 | 2.62703 | 2.63159 | 3.03336 | 3.03577 | |
| 30 | 1.58541 | 1.92249 | 1.91608 | 2.20366 | 2.19722 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sobhy, M.; Al Mukahal, F.H.H. Analysis of Electromagnetic Effects on Vibration of Functionally Graded GPLs Reinforced Piezoelectromagnetic Plates on an Elastic Substrate. Crystals 2022, 12, 487. https://doi.org/10.3390/cryst12040487
Sobhy M, Al Mukahal FHH. Analysis of Electromagnetic Effects on Vibration of Functionally Graded GPLs Reinforced Piezoelectromagnetic Plates on an Elastic Substrate. Crystals. 2022; 12(4):487. https://doi.org/10.3390/cryst12040487
Chicago/Turabian StyleSobhy, Mohammed, and F. H. H. Al Mukahal. 2022. "Analysis of Electromagnetic Effects on Vibration of Functionally Graded GPLs Reinforced Piezoelectromagnetic Plates on an Elastic Substrate" Crystals 12, no. 4: 487. https://doi.org/10.3390/cryst12040487
APA StyleSobhy, M., & Al Mukahal, F. H. H. (2022). Analysis of Electromagnetic Effects on Vibration of Functionally Graded GPLs Reinforced Piezoelectromagnetic Plates on an Elastic Substrate. Crystals, 12(4), 487. https://doi.org/10.3390/cryst12040487






