Application of the Alexander–Haasen Model for Thermally Stimulated Dislocation Generation in FZ Silicon Crystals
Abstract
:1. Introduction
2. Description of Experiment
3. Numerical Model
3.1. Heat Transfer
3.1.1. Temperature Field
3.1.2. Heat Induction
3.1.3. Equation Coupling
3.2. Dislocation Density Dynamics
3.2.1. Constitutive Equations
3.2.2. Plastic Stress
3.2.3. Equation Coupling
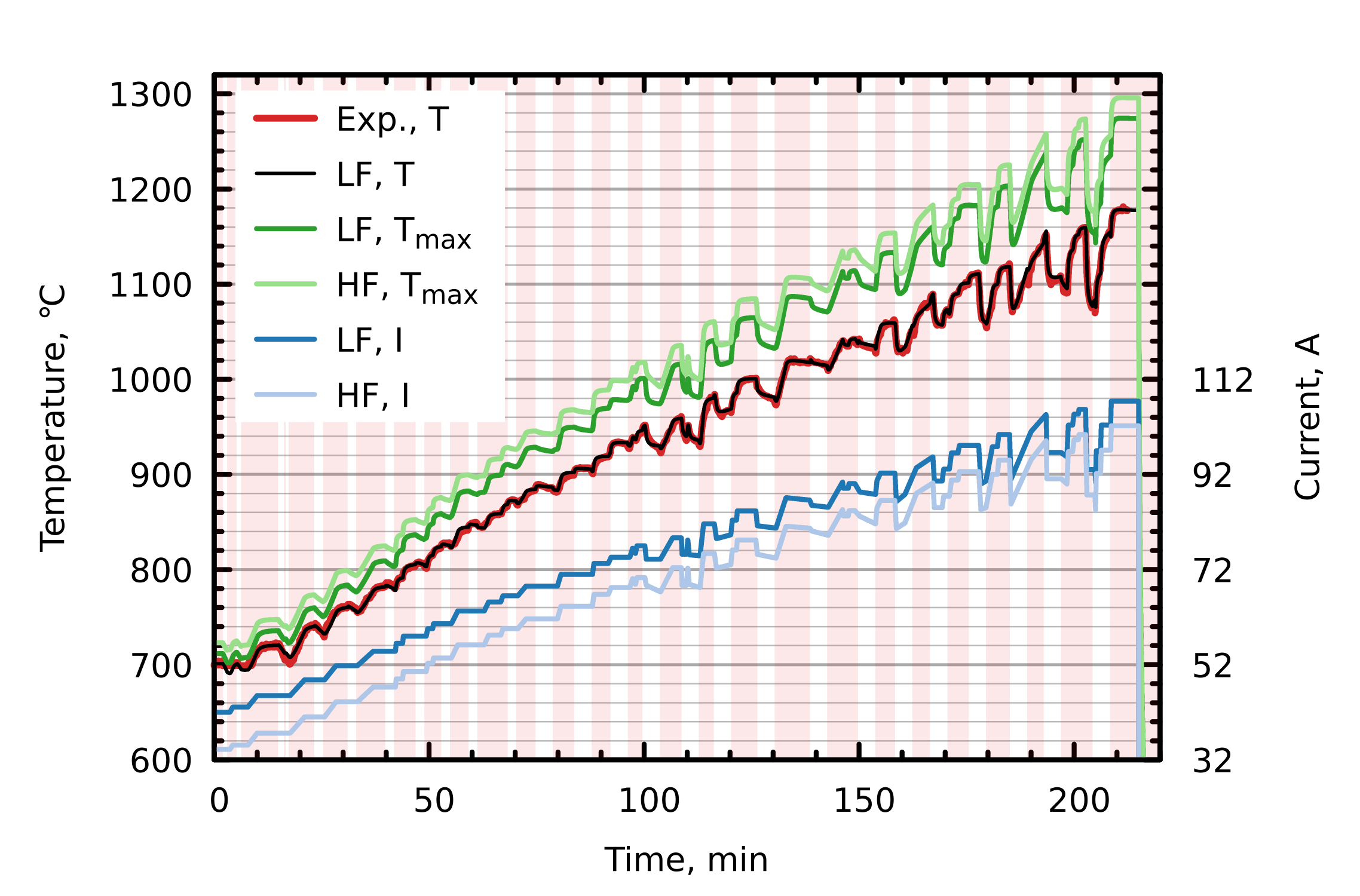
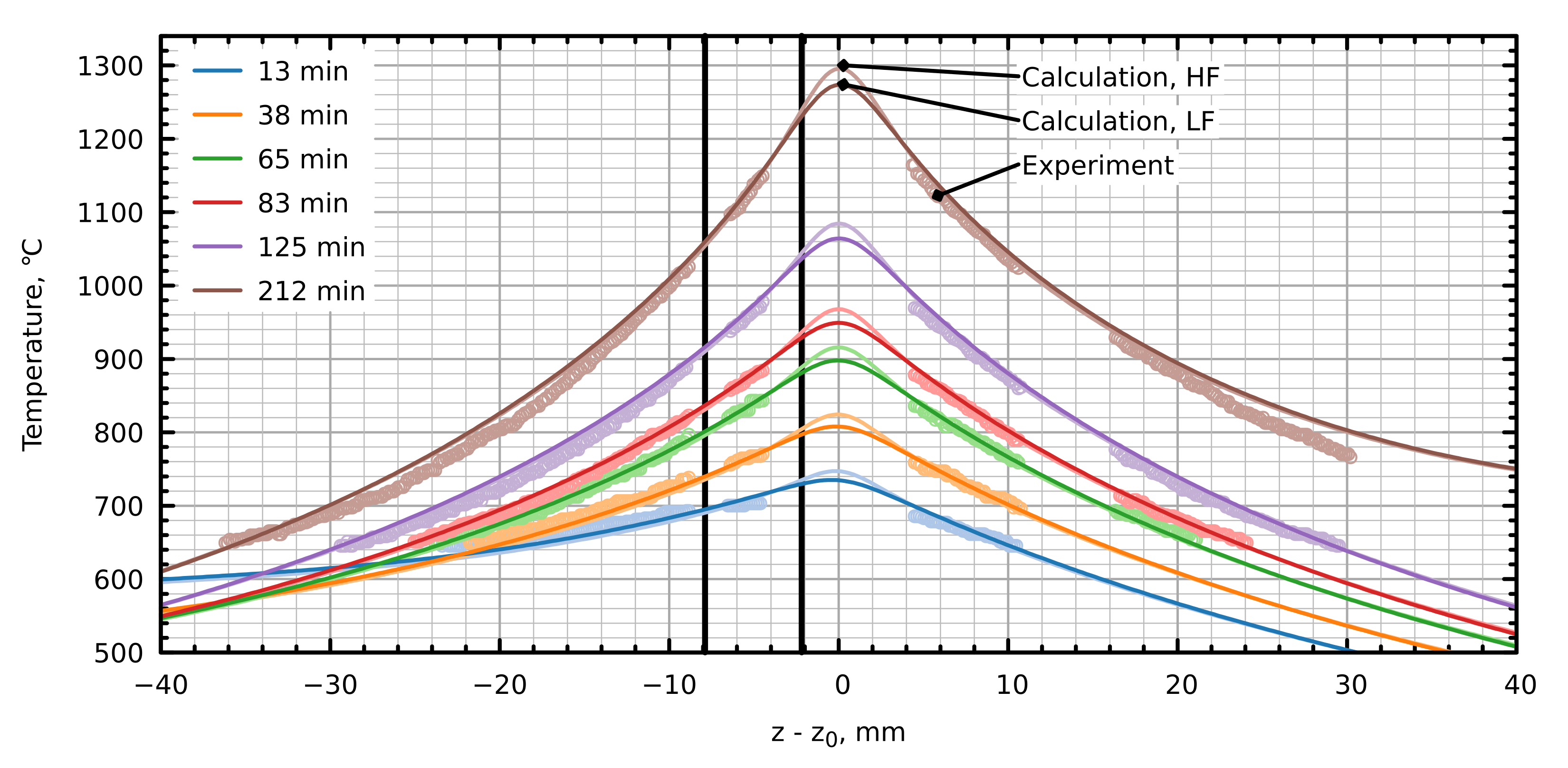
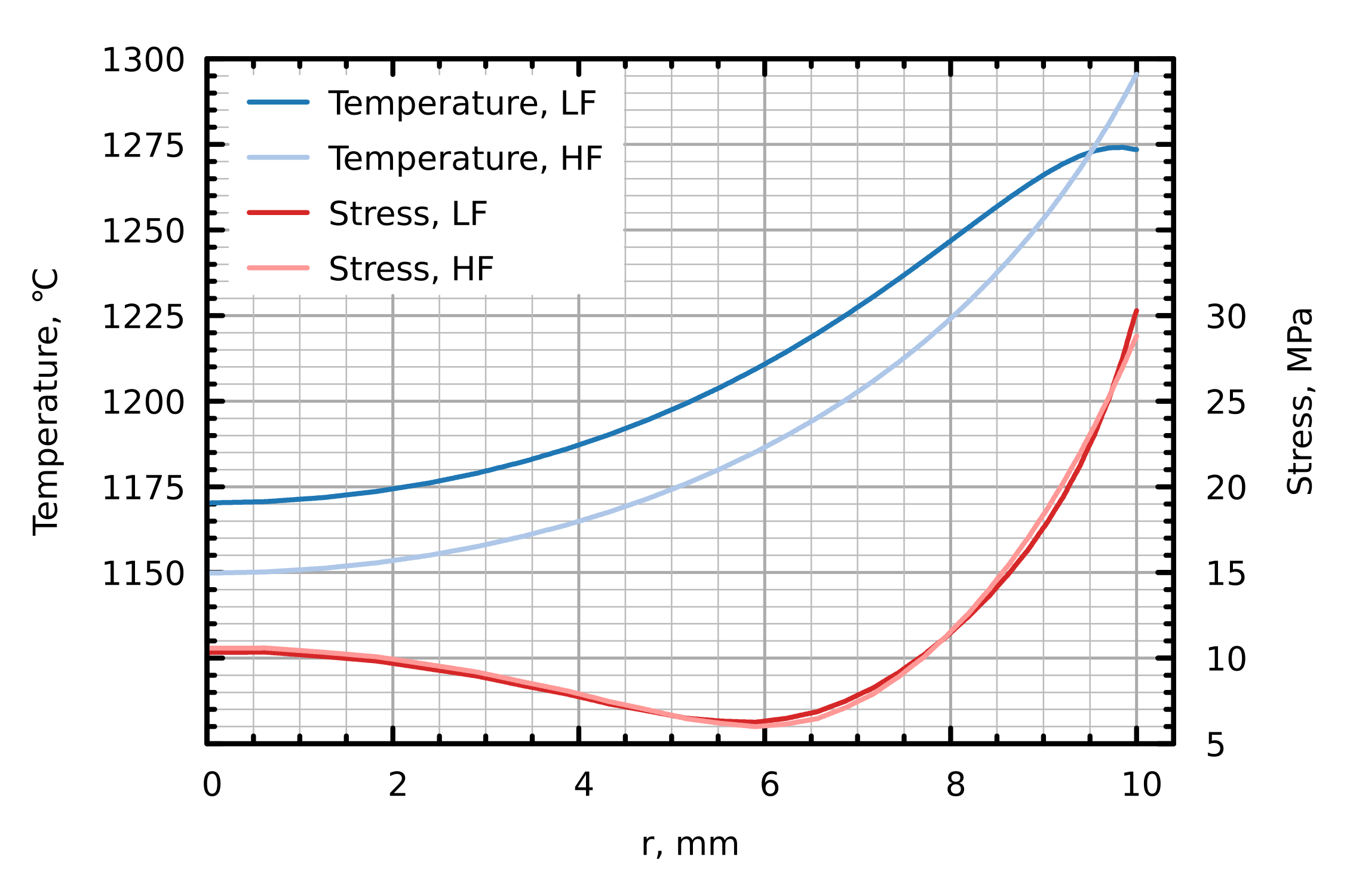
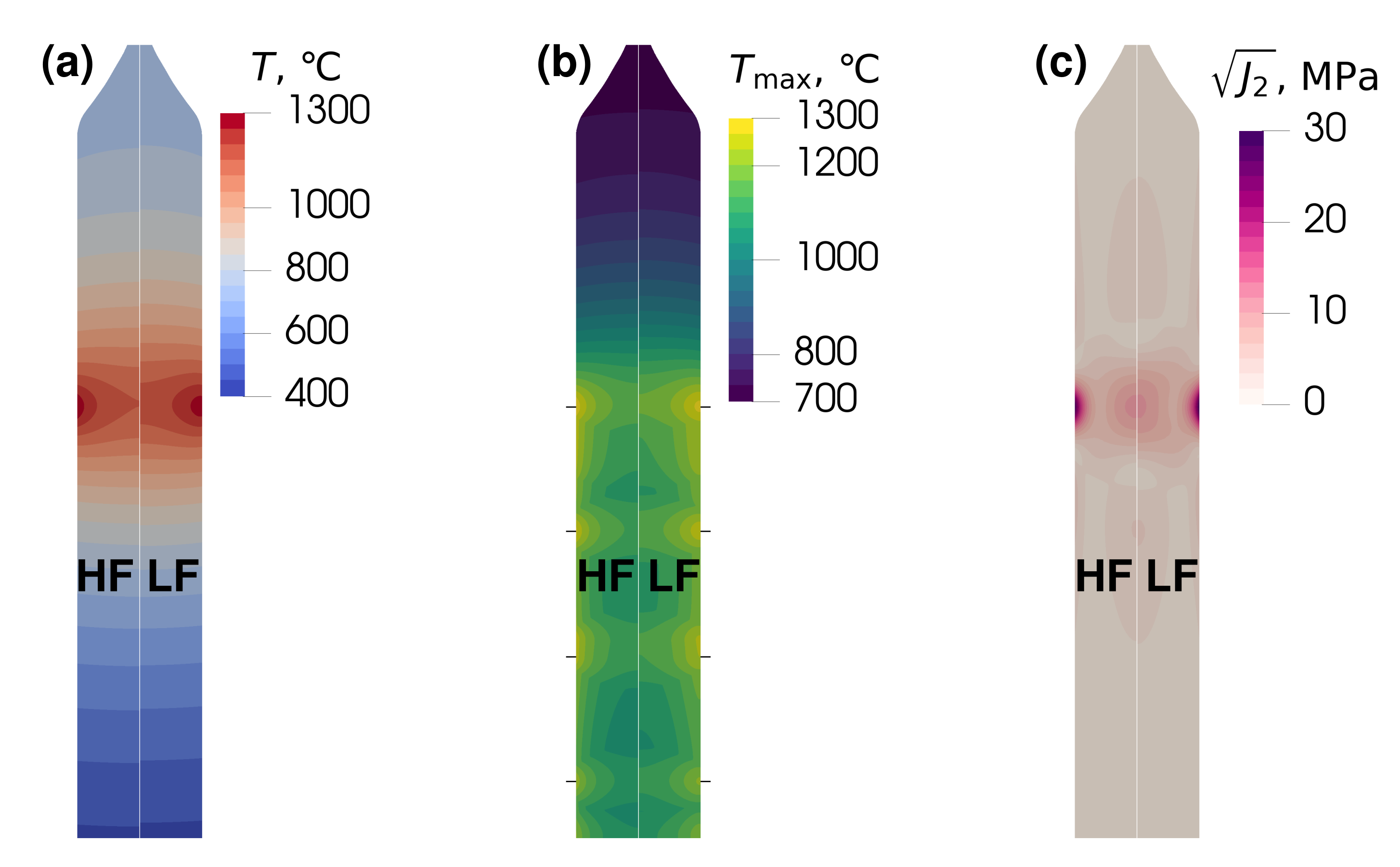
4. Results and Discussion
4.1. Heat Transfer and Elastic Stress
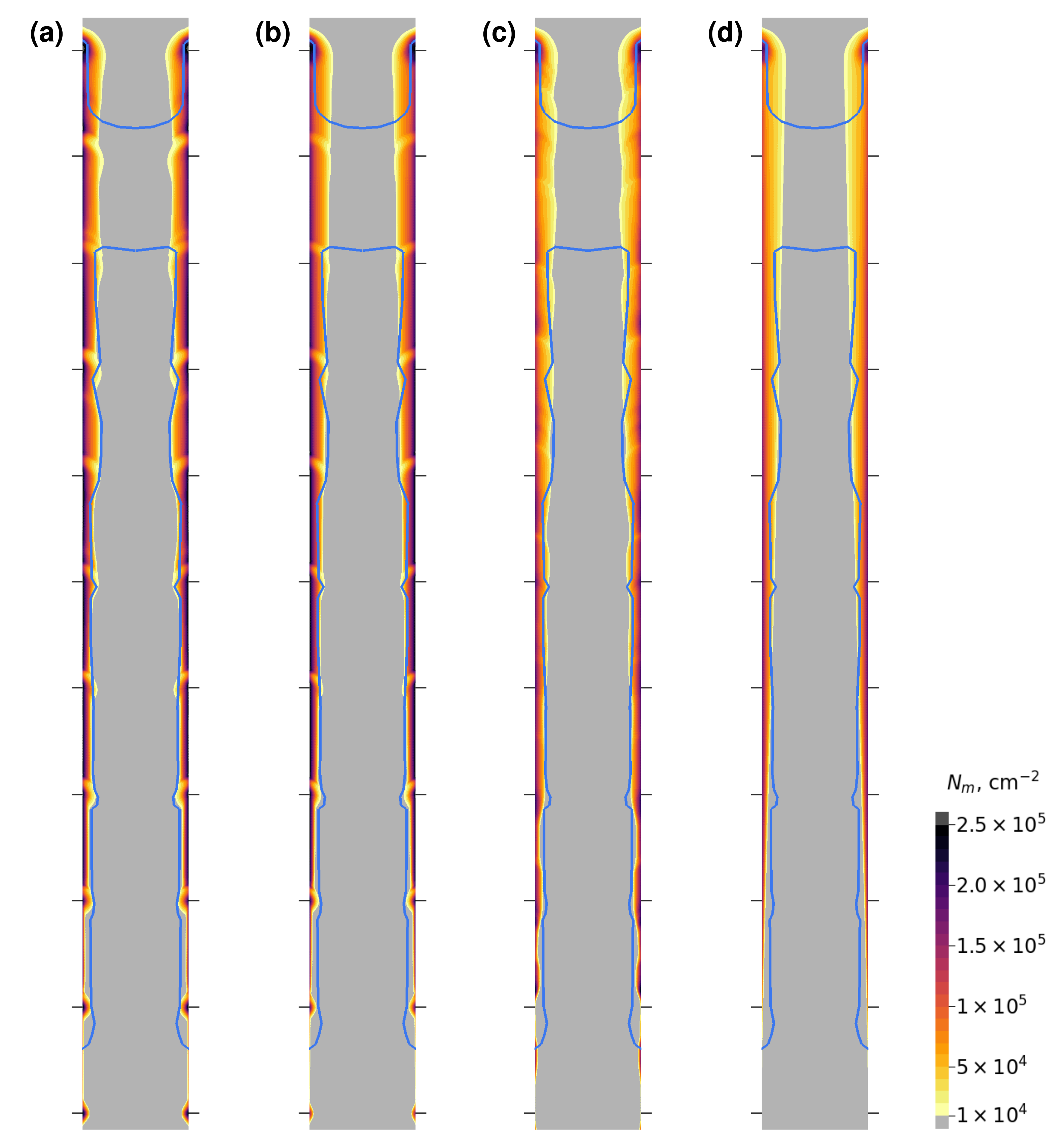
4.2. Dislocation Density Dynamics
5. Summary and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AH | Alexander–Haasen |
| CRSS | Critical resolved shear stress |
| CZ | Czochralski |
| EM | Electromagnetic |
| FZ | Floating-zone |
| HF | High-frequency |
| LF | Low-frequency |
Appendix A. Derivation of 1D Temperature Distribution for HF and LF Heat Induction Models
References
- Dash, W.C. Growth of silicon crystals free from dislocations. J. Appl. Phys. 1959, 30, 459–474. [Google Scholar] [CrossRef]
- Rost, H.J.; Menzel, R.; Siche, D.; Juda, U.; Kayser, S.; Kießling, F.M.; Sylla, L.; Richter, T. Defect formation in Si-crystals grown on large diameter bulk seeds by a modified FZ-method. J. Cryst. Growth 2018, 500, 5–10. [Google Scholar] [CrossRef]
- Menzel, R.; Rost, H.J.; Kießling, F.M.; Sylla, L. Float-zone growth of silicon crystals using large-area seeding. J. Cryst. Growth 2019, 515, 32–36. [Google Scholar] [CrossRef]
- Stoddard, N.; Russell, J.; Hixson, E.C.; She, H.; Krause, A.; Wolny, F.; Bertoni, M.; Naerland, T.U.; Sylla, L.; von Ammon, W. NeoGrowth silicon: A new high purity, low-oxygen crystal growth technique for photovoltaic substrates. Prog. Photovoltaics Res. Appl. 2018, 26, 324–331. [Google Scholar] [CrossRef]
- Jordan, A.S.; Caruso, R.; VonNeida, A.R.; Nielsen, J.W. A comparative study of thermal stress induced dislocation generation in pulled GaAs, InP, and Si crystals. J. Appl. Phys. 1981, 52, 3331–3336. [Google Scholar] [CrossRef]
- Miyazaki, N.; Uchida, H.; Munakata, T.; Fujioka, K.; Sugino, Y. Thermal stress analysis of silicon bulk single crystal during Czochralski growth. J. Cryst. Growth 1992, 125, 102–111. [Google Scholar] [CrossRef]
- Muižnieks, A.; Raming, G.; Mühlbauer, A.; Virbulis, J.; Hanna, B.; Ammon, W. Stress-induced dislocation generation in large FZ- and CZ-silicon single crystals—Numerical model and qualitative considerations. J. Cryst. Growth 2001, 230, 305–313. [Google Scholar] [CrossRef]
- Alexander, H.; Haasen, P. Dislocations and plastic flow in the diamond structure. In Solid State Physics; Seitz, F., Turnbull, D., Ehrenreich, H., Eds.; Elsevier: Amsterdam, The Netherlands, 1969; Volume 22, pp. 27–158. [Google Scholar] [CrossRef]
- Dillon, O.W.; Tsai, C.T.; de Angelis, R.J. Dislocation dynamics during the growth of silicon ribbon. J. Appl. Phys. 1986, 60, 1784–1792. [Google Scholar] [CrossRef]
- Völkl, J.; Müller, G. A new model for the calculation of dislocation formation in semiconductor melt growth by taking into account the dynamics of plastic deformation. J. Cryst. Growth 1989, 97, 136–145. [Google Scholar] [CrossRef]
- Tsai, C.T.; Dillon, O.W.; de Angelis, R.J. The constitutive equation for silicon and its use in crystal growth modeling. J. Eng. Mater. Technol. 1990, 112, 183–187. [Google Scholar] [CrossRef]
- Miyazaki, N.; Okuyama, S. Development of finite element computer program for dislocation density analysis of bulk semiconductor single crystals during Czochralski growth. J. Cryst. Growth 1998, 183, 81–88. [Google Scholar] [CrossRef]
- Dadzis, K.; Behnken, H.; Bähr, T.; Oriwol, D.; Sylla, L.; Richter, T. Numerical simulation of stresses and dislocations in quasi-mono silicon. J. Cryst. Growth 2016, 450, 14–21. [Google Scholar] [CrossRef]
- Gallien, B.; Albaric, M.; Duffar, T.; Kakimoto, K.; M’Hamdi, M. Study on the usage of a commercial software (Comsol-Multiphysics®) for dislocation multiplication model. J. Cryst. Growth 2017, 457, 60–64. [Google Scholar] [CrossRef]
- Yonenaga, I.; Sumino, K. Dislocation dynamics in the plastic deformation of silicon crystals I. Experiments. Phys. Status Solidi A 1978, 50, 685–693. [Google Scholar] [CrossRef]
- Suezawa, M.; Sumino, K.; Yonenaga, I. Dislocation dynamics in the plastic deformation of silicon crystals. II. Theoretical analysis of experimental results. Phys. Status Solidi A 1979, 51, 217–226. [Google Scholar] [CrossRef]
- Gao, B.; Jiptner, K.; Nakano, S.; Harada, H.; Miyamura, Y.; Sekiguchi, T.; Kakimoto, K. Applicability of the three-dimensional Alexander-Haasen model for the analysis of dislocation distributions in single-crystal silicon. J. Cryst. Growth 2015, 411, 49–55. [Google Scholar] [CrossRef]
- Rost, H.J.; Buchovska, I.; Dadzis, K.; Juda, U.; Renner, M.; Menzel, R. Thermally stimulated dislocation generation in silicon crystals grown by the Float-Zone method. J. Cryst. Growth 2020, 552, 125842. [Google Scholar] [CrossRef]
- Arndt, D.; Bangerth, W.; Davydov, D.; Heister, T.; Heltai, L.; Kronbichler, M.; Maier, M.; Pelteret, J.P.; Turcksin, B.; Wells, D. The deal.II library, version 8.5. J. Numer. Math. 2017, 25, 137–145. [Google Scholar] [CrossRef]
- MACPLAS: MAcroscopic Crystal PLAsticity Simulator. Available online: https://github.com/aSabanskis/MACPLAS (accessed on 16 November 2021).
- Ratnieks, G. Modelling of the Floating Zone Growth of Silicon Single Crystals with Diameter up to 8 Inch. Ph.D. Thesis, University of Latvia, Riga, Latvia, 2007. [Google Scholar]
- Muhlbauer, A.; Muiznieks, A.; Leßmann, H.J. The calculation of 3D high-frequency electromagnetic fields during induction heating using the BEM. IEEE Trans. Magn. 1993, 29, 1566–1569. [Google Scholar] [CrossRef]
- Fulkerson, W.; Moore, J.P.; Williams, R.K.; Graves, R.S.; McElroy, D.L. Thermal conductivity, electrical resistivity, and Seebeck coefficient of silicon from 100 to 1300°K. Phys. Rev. 1968, 167, 765–782. [Google Scholar] [CrossRef]
- Sabanskis, A.; Plāte, M.; Sattler, A.; Miller, A.; Virbulis, J. Evaluation of the performance of published point defect parameter sets in cone and body phase of a 300 mm Czochralski silicon crystal. Crystals 2021, 11, 460. [Google Scholar] [CrossRef]
- Sabanskis, A.; Virbulis, J. Simulation of the influence of gas flow on melt convection and phase boundaries in FZ silicon single crystal growth. J. Cryst. Growth 2015, 417, 51–57. [Google Scholar] [CrossRef]
- Geuzaine, C.; Remacle, J.F. Gmsh: A 3-D finite element mesh generator with built-in pre- and post-processing facilities. Int. J. Numer. Methods Eng. 2009, 79, 1309–1331. [Google Scholar] [CrossRef]
- Okada, Y.; Tokumaru, Y. Precise determination of lattice parameter and thermal expansion coefficient of silicon between 300 and 1500 K. J. Appl. Phys. 1984, 56, 314–320. [Google Scholar] [CrossRef]


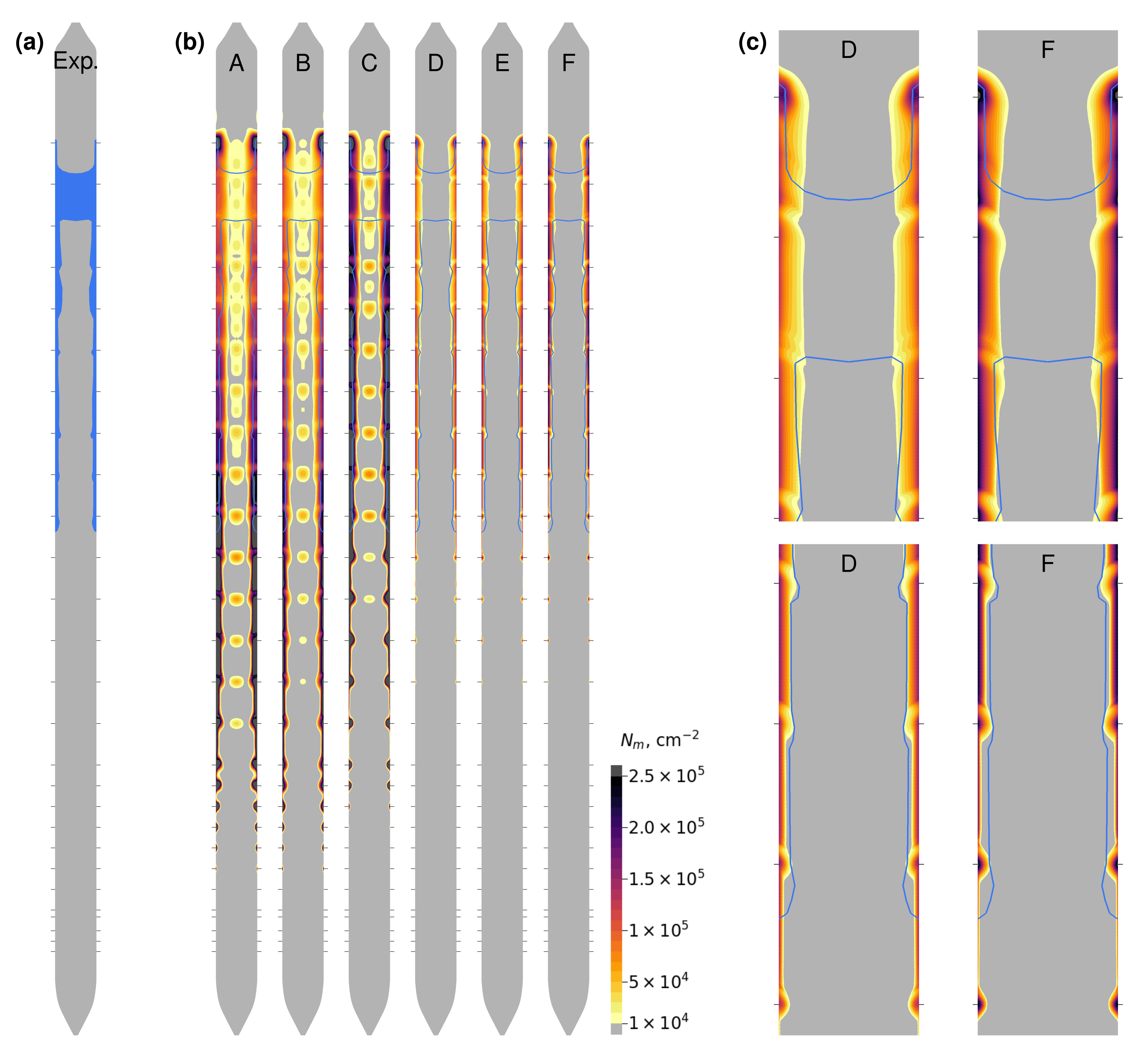
| Case | Heat Induction Mode | Dislocation Parameters |
|---|---|---|
| A | LF | Basic parameter set |
| B | HF | Basic parameter set |
| C | LF | |
| D | LF | |
| E | LF | , |
| F | LF | , |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sabanskis, A.; Dadzis, K.; Menzel, R.; Virbulis, J. Application of the Alexander–Haasen Model for Thermally Stimulated Dislocation Generation in FZ Silicon Crystals. Crystals 2022, 12, 174. https://doi.org/10.3390/cryst12020174
Sabanskis A, Dadzis K, Menzel R, Virbulis J. Application of the Alexander–Haasen Model for Thermally Stimulated Dislocation Generation in FZ Silicon Crystals. Crystals. 2022; 12(2):174. https://doi.org/10.3390/cryst12020174
Chicago/Turabian StyleSabanskis, Andrejs, Kaspars Dadzis, Robert Menzel, and Jānis Virbulis. 2022. "Application of the Alexander–Haasen Model for Thermally Stimulated Dislocation Generation in FZ Silicon Crystals" Crystals 12, no. 2: 174. https://doi.org/10.3390/cryst12020174
APA StyleSabanskis, A., Dadzis, K., Menzel, R., & Virbulis, J. (2022). Application of the Alexander–Haasen Model for Thermally Stimulated Dislocation Generation in FZ Silicon Crystals. Crystals, 12(2), 174. https://doi.org/10.3390/cryst12020174








