Kretschmann-Based Optical Sensor via Thermally Tunable Refractive Index
Abstract
1. Introduction
2. Theoretical Consideration
2.1. Optical Dispersion Relations
2.2. Chemical Materials
2.2.1. Material and Methods
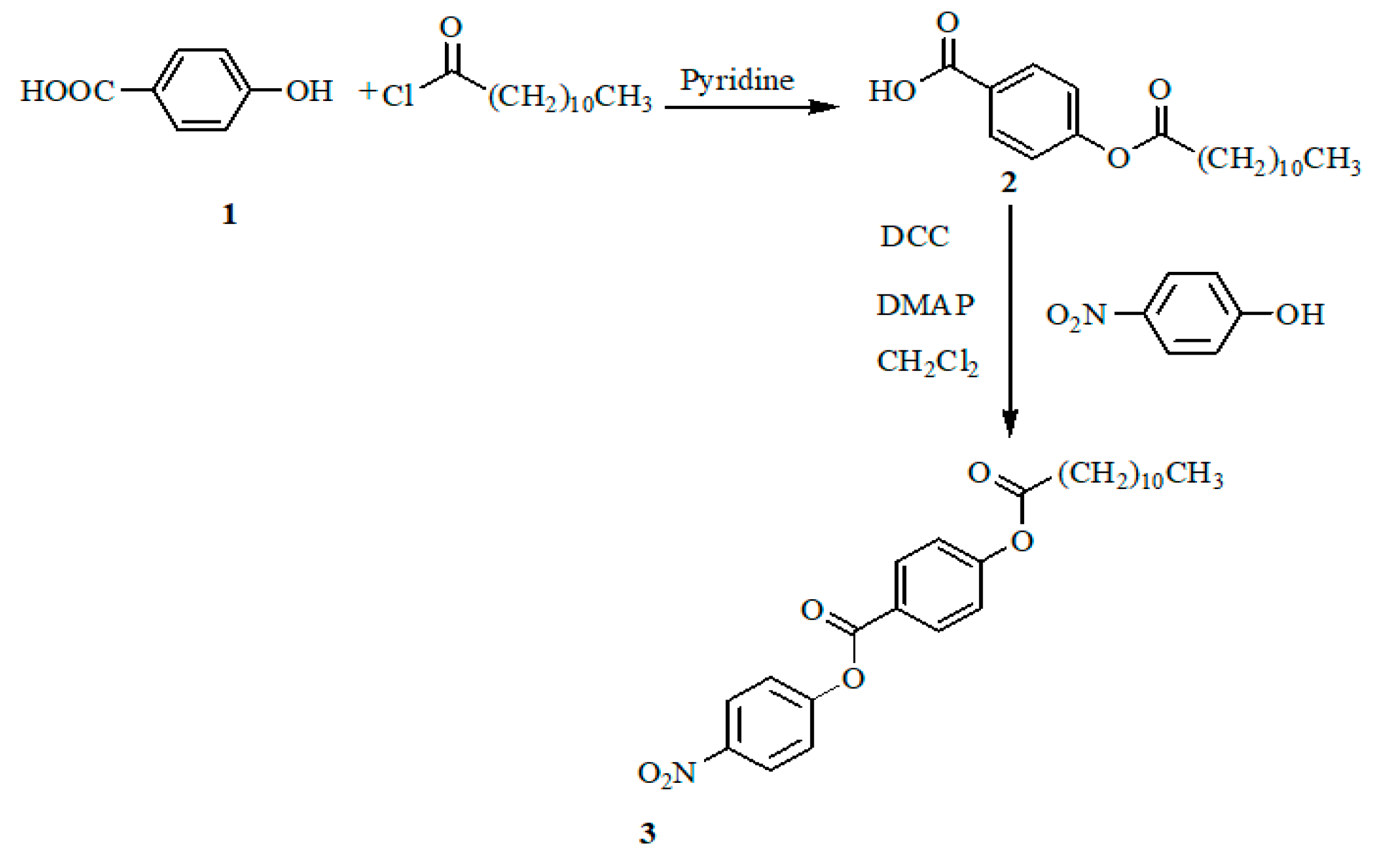
2.2.2. Procedure
a-4-(Dodecanoyloxy) Benzoic Acid (2)
b-4-Nitrophenyl-4-(dodecanoyloxy) Benzoate (3)
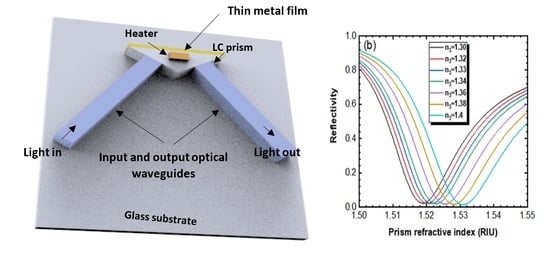
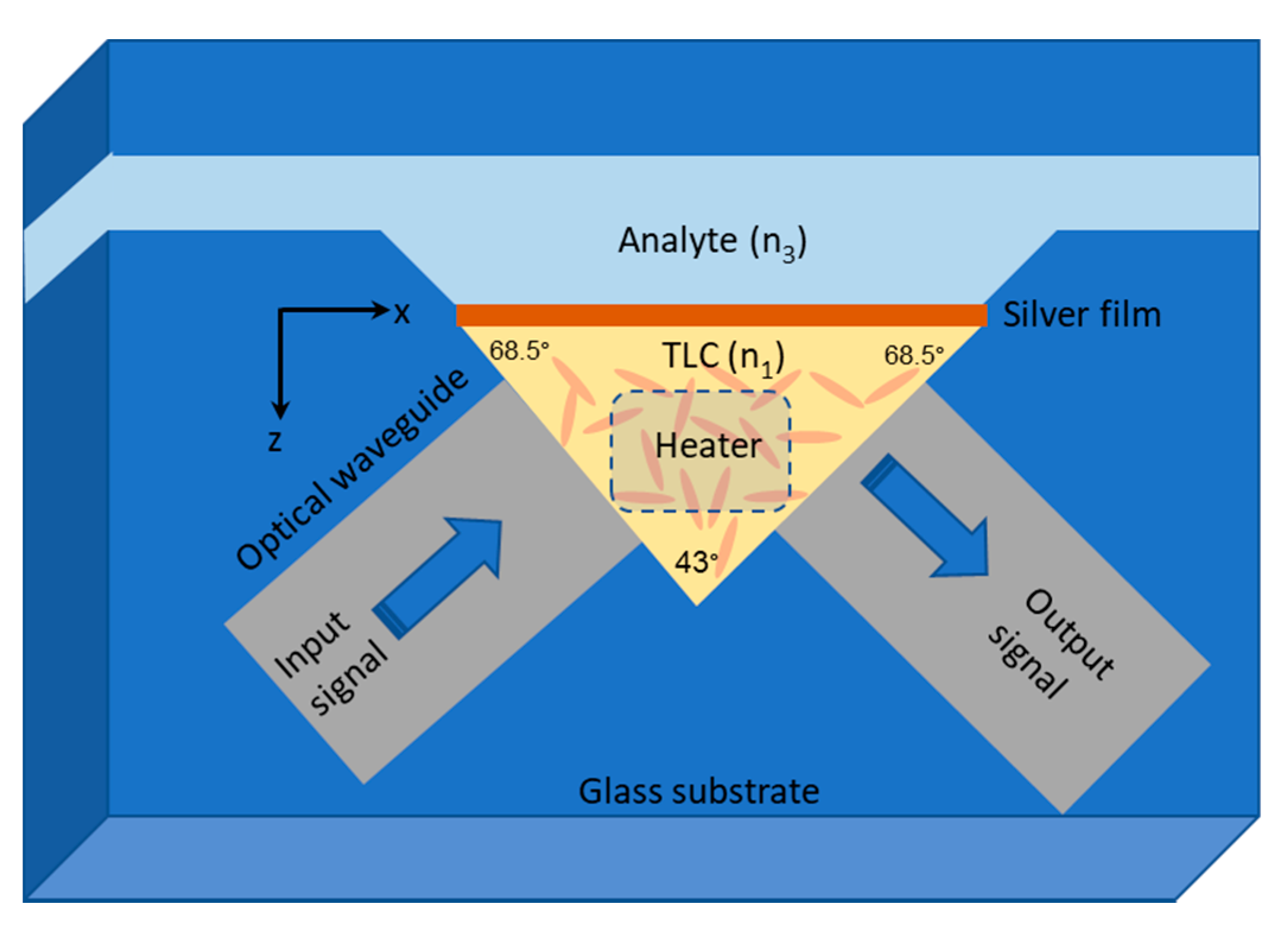
2.3. Optical Measurements
2.4. Mesophase Temperature Detection
3. Results
3.1. Chemical Preparation
3.2. Mesophase Temperature Detection
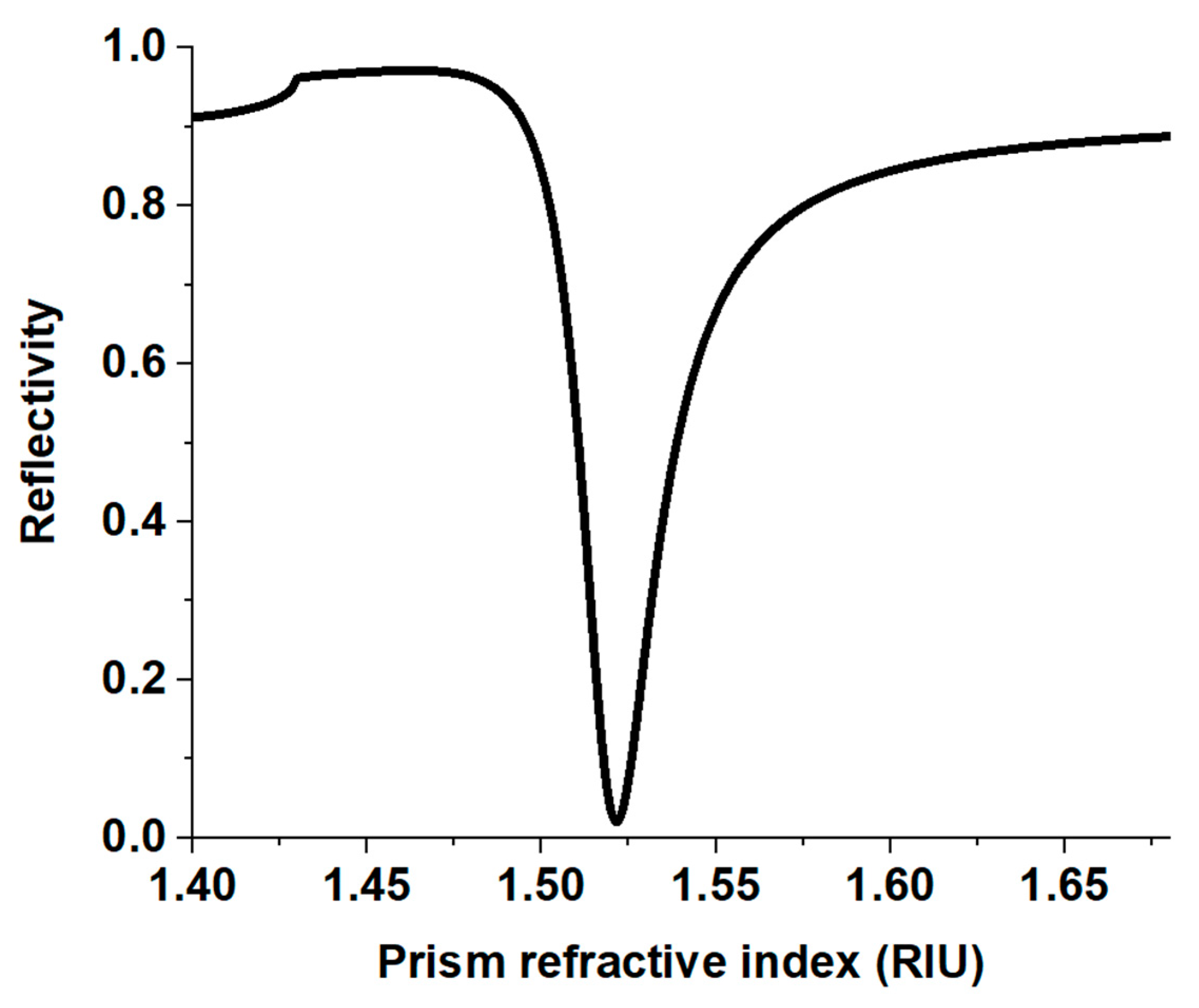
3.3. Optical Parameters
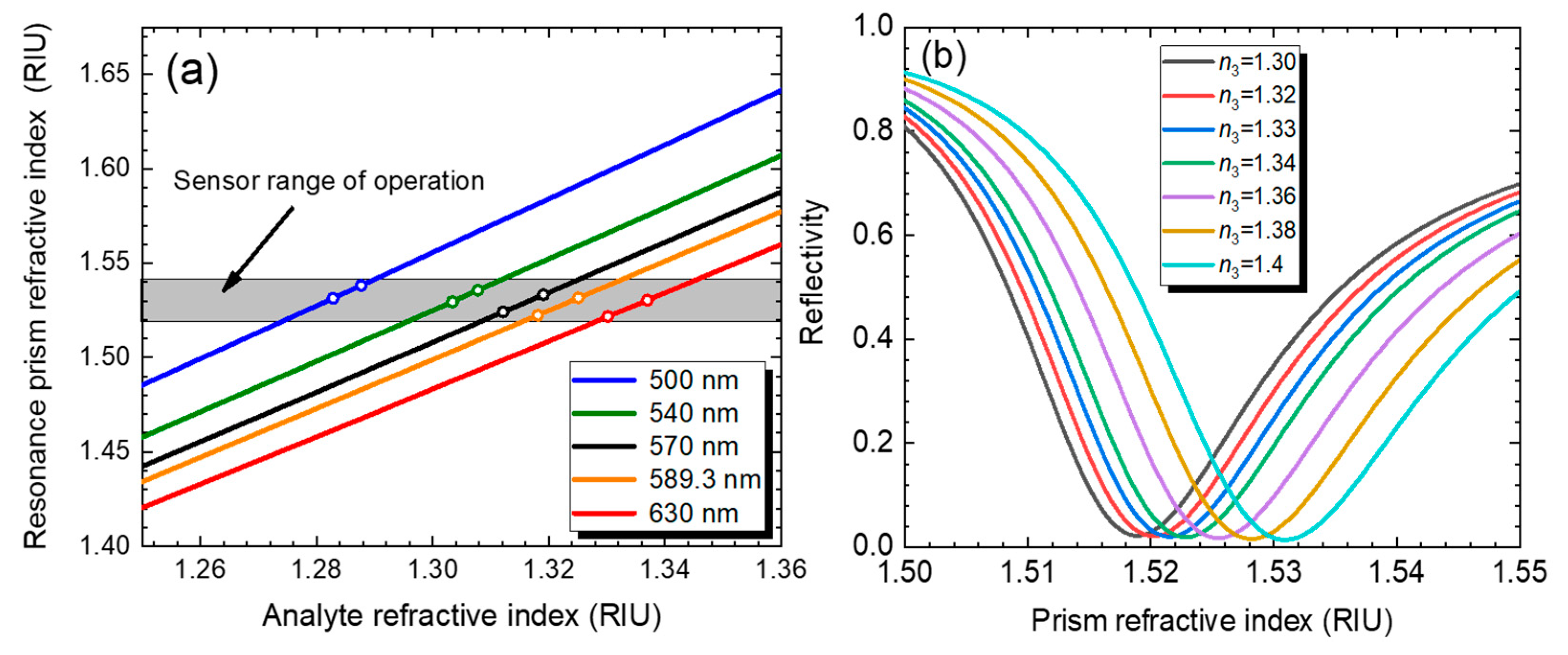
3.4. Refractive Index Sensing Application
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Bao, J.; Zong, X.; Li, L.; Yu, K.; Liu, Y. Liquid crystal filled dual-channel self-calibration optical-fiber surface plasmon resonance thermometer. Appl. Opt. 2020, 59, 720–725. [Google Scholar] [CrossRef]
- Tabassum, R.; Kant, R. Recent trends in surface plasmon resonance based fiber–optic gas sensors utilizing metal oxides and carbon nanomaterials as functional entities. Sens. Actuators B Chem. 2020, 310, 127813. [Google Scholar] [CrossRef]
- Tao, F.; Zhang, H.-F.; Yang, X.-H.; Cao, D. Surface plasmon polaritons of the metamaterial four-layered structures. JOSA B 2009, 26, 50–59. [Google Scholar] [CrossRef]
- Liu, Z.; Feng, W.; Huang, Z.; Guo, L.J. Polarization-controlled efficient and unidirectional surface plasmon polariton excitation enabled by metagratings in a generalized Kretschmann configuration. Opt. Express 2021, 29, 3659–3668. [Google Scholar] [CrossRef] [PubMed]
- Joseph, S.; Sarkar, S.; Joseph, J. Grating-Coupled Surface Plasmon-Polariton Sensing at a Flat Metal--Analyte Interface in a Hybrid-Configuration. ACS Appl. Mater. Interfaces 2020, 12, 46519–46529. [Google Scholar] [CrossRef]
- Kretschmann, E.; Raether, H.; Institut für Angewandte Physik der Universität Hamburg. Radiative decay of non-radiative surface plasmons excited by light. Z. Naturforsch. A 1968, 23, 2135–2136. [Google Scholar] [CrossRef]
- Genchev, Z.D.; Nedelchev, N.M.; Mateev, E.; Stoyanov, H.Y. Analytical approach to the prism coupling problem in the Kretschmann configuration. Plasmonics 2008, 3, 21–26. [Google Scholar] [CrossRef]
- Gupta, B.D.; Srivastava, S.K.; Verma, R. Fiber Optic Sensors Based on Plasmonics; World Scientific: Singapore, 2015. [Google Scholar]
- Gwon, H.R.; Lee, S.H. Spectral and angular responses of surface plasmon resonance based on the Kretschmann prism configuration. Mater. Trans. 2010, 51, 1150–1155. [Google Scholar] [CrossRef]
- Pawlik, G.; Walasik, W.; Jarema, M.; Mitus, A.C.; Khoo, I.C. Temperature dependence of refractive index gradient in nanosphere dispersed liquid crystal (NDLC) metamaterial at infrared frequencies: Monte Carlo study. Opt. Mater. 2012, 34, 1656–1659. [Google Scholar] [CrossRef]
- Hu, D.J.J.; Shum, P.; Lu, C.; Sun, X.; Ren, G.; Yu, X.; Wang, G. Design and analysis of thermally tunable liquid crystal filled hybrid photonic crystal fiber coupler. Opt. Commun. 2009, 282, 2343–2347. [Google Scholar] [CrossRef]
- Yakuphanoglu, F.; Durmuş, M.; Okutan, M.; Köysal, O.; Ahsen, V. The refractive index dispersion and the optical constants of liquid crystal metal-free and nickel(II) phthalocyanines. Phys. B Condens. Matter 2006, 373, 262–266. [Google Scholar] [CrossRef]
- Okutan, M.; San, S.E.; Köysal, O.; Yakuphanoglu, F. Investigation of refractive index dispersion and electrical properties in carbon nano-balls’ doped nematic liquid crystals. Phys. B Condens. Matter 2005, 362, 180–186. [Google Scholar] [CrossRef]
- Guo, Y.; Li, J.; Li, S.; Liu, Y.; Meng, X.; Bi, W.; Lu, H.; Cheng, T.; Hao, R. Amphibious sensor of temperature and refractive index based on D-shaped photonic crystal fibre filled with liquid crystal. Liq. Cryst. 2020, 47, 882–894. [Google Scholar] [CrossRef]
- Kieser, B.; Pauluth, D.; Gauglitz, G. Nematic liquid crystals as sensitive layers for surface plasmon resonance sensors. Anal. Chim. Acta 2001, 434, 231–237. [Google Scholar] [CrossRef]
- Liu, C.; Wang, F.; Lv, J.; Sun, T.; Liu, Q.; Fu, C.; Mu, H.; Chu, P.K. A highly temperature-sensitive photonic crystal fiber based on surface plasmon resonance. Opt. Commun. 2016, 359, 378–382. [Google Scholar] [CrossRef]
- Watad, I.; Abdulhalim, I. Phase-shifted polarimetric surface plasmon resonance sensor using a liquid crystal retarder and a diverging beam. Opt. Lett. 2019, 44, 1607–1610. [Google Scholar] [CrossRef] [PubMed]
- Lu, M.; Zhang, X.; Liang, Y.; Li, L.; Masson, J.-F.; Peng, W. Liquid crystal filled surface plasmon resonance thermometer. Opt. Express 2016, 24, 10904. [Google Scholar] [CrossRef] [PubMed]
- Lee, S.; Song, H.; Ahn, H.; Kim, S.; Choi, J.; Kim, K. Fiber-Optic Localized Surface Plasmon Resonance Sensors Based on Nanomaterials. Sensors 2021, 21, 819. [Google Scholar] [CrossRef]
- Algorri, J.F.; García-Cámara, B.; García-García, A.; Urruchi, V.; Sánchez-Pena, J.M. Fiber optic temperature sensor based on amplitude modulation of metallic and semiconductor nanoparticles in a liquid crystal mixture. J. Light. Technol. 2015, 33, 2451–2455. [Google Scholar] [CrossRef]
- Algorri, J.F.; Garcia-Camara, B.; Urruchi, V.; Sánchez-Pena, J.M. High-sensitivity Fabry-Pérot temperature sensor based on liquid crystal doped with nanoparticles. IEEE Photonics Technol. Lett. 2014, 27, 292–295. [Google Scholar] [CrossRef]
- Smith, D.Y.; Inokuti, M.; Karstens, W. A generalized cauchy dispersion formula and the refractivity of elemental semiconductors. J. Phys. Condens. Matter 2001, 13, 3883–3893. [Google Scholar] [CrossRef]
- Ghosh, G. Dispersion-equation coefficients for the refractive index and birefringence of calcite and quartz crystals. Opt. Commun. 1999, 163, 95–102. [Google Scholar] [CrossRef]
- Wondraczek, L.; Gross, G.-P.; Heide, G.; Kloess, G.; Frischat, G.H. Abbe numbers and refractive indices of tektites and volcanic glasses. J. Non. Cryst. Solids 2003, 323, 127–130. [Google Scholar] [CrossRef]
- Yokokura, H.; Era, S.; Abe, H.; Nakata, T.; Kitamura, T.; Mukoh, A. Liquid-crystal compound, liquid crystal composition and liquid crystal display device. EU-EP0149754A2, 31 July 1985. [Google Scholar]
- Li, J.; Gauza, S.; Wu, S.T. Temperature effect on liquid crystal refractive indices. J. Appl. Phys. 2004, 96, 19–24. [Google Scholar] [CrossRef]
- Li, J.; Gauzia, S.; Wu, S.-T. High temperature-gradient refractive index liquid crystals. Opt. Express 2004, 12, 2002. [Google Scholar] [CrossRef]
- Loiko, P.; Ghanbari, S.; Matrosov, V.; Yumashev, K.; Major, A. Dispersion and anisotropy of thermo-optical properties of Alexandrite laser crystal. Opt. Mater. Express 2018, 8, 3000. [Google Scholar] [CrossRef]
- Gryga, M.; Vala, D.; Kolejak, P.; Gembalova, L.; Ciprian, D.; Hlubina, P. One-dimensional photonic crystal for Bloch surface waves and radiation modes-based sensing. Opt. Mater. Express 2019, 9, 4009. [Google Scholar] [CrossRef]
- Kurihara, K.; Suzuki, K. Theoretical understanding of an absorption-based surface plasmon resonance sensor based on Kretchmann’s theory. Anal. Chem. 2002, 74, 696–701. [Google Scholar] [CrossRef] [PubMed]
- Rakić, A.D.; Djurišić, A.B.; Elazar, J.M.; Majewski, M.L. Optical properties of metallic films for vertical-cavity optoelectronic devices. Appl. Opt. 1998, 37, 5271–5283. [Google Scholar] [CrossRef] [PubMed]
- Liu, C.; Zhang, X.; Gao, Y.; Wei, Y.; Wu, P.; Su, Y.; Wu, P. Fiber SPR refractive index sensor with the variable core refractive index. Appl. Opt. 2020, 59, 1323–1328. [Google Scholar] [CrossRef]
- Cetin, A.E.; Mertiri, A.; Huang, M.; Erramilli, S.; Altug, H. Thermal tuning of surface plasmon polaritons using liquid crystals. Adv. Opt. Mater. 2013, 1, 915–920. [Google Scholar] [CrossRef]
- Du, Y.; Shyu, L.; Hu, W. The Effect of Combining Stationary Wavelet Transform and Independent Component Analysis in the Multi-channel SEMGs Hand Motion Identification System. J. Med Biol. Eng. 2006, 26, 9–14. [Google Scholar]
- Hsu, H.F.; Lin, Y.T.; Huang, Y.T.; Lu, M.F.; Chen, C.H. In Situ Regeneration of Si-based ARROW-B Surface Plasmon Resonance Biosensors. J. Med Biol. Eng. 2015, 35, 305–314. [Google Scholar] [CrossRef][Green Version]
- Zida, S.I.; Yang, C.C.; Khung, Y.L.; Lin, Y.D. Fabrication and Characterization of an Aptamer-Based N-type Silicon Nanowire FET Biosensor for VEGF Detection. J. Med Biol. Eng. 2020, 40, 601–609. [Google Scholar] [CrossRef]
- Gomaa, L.; Shaaban, A.; Hameed, M.F.; Obayya, S.S. Competitiveness of the BPM in studying the optical beams at critical incidence on dielectric interfaces. Opt. Quantum Electron. 2017, 49, 51. [Google Scholar] [CrossRef]
- Shaaban, A.; Du, Y.-C.; Gomaa, L. Effects of smoothing functions on the transformation of TM to TE propagation problems in the framework of FFT-BPM: A comparative study. Opt. Commun. 2021, 478, 126374. [Google Scholar] [CrossRef]
- Shaaban, A.; Hameed, M.F.; Gomaa, L.R.; Obayya, S.S. Accurate calculation of Goos-Hänchen shift at critical angle for complex laser beam profiles using beam propagation method. Optik 2018, 157, 1106–1114. [Google Scholar] [CrossRef]
- Shaaban, A.; Sayed, M.; Hameed, M.F.O.; Saleh, H.I.; Gomaa, L.; Du, Y.-C.; Obayya, S. Fast parallel beam propagation method based on multi-core and many-core architectures. Optik 2019, 180, 484–491. [Google Scholar] [CrossRef]
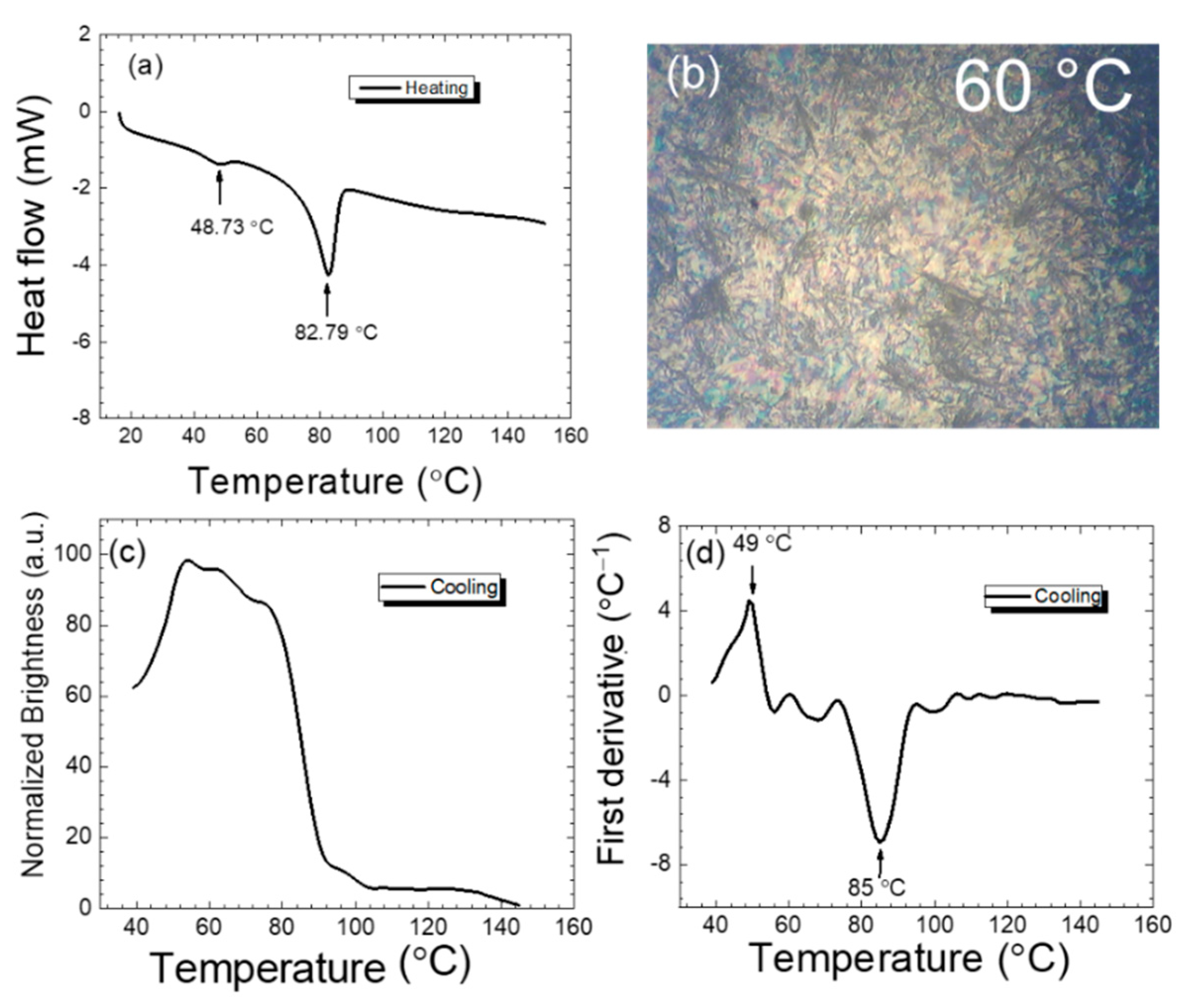

| Phase Transition | Heating | Cooling |
|---|---|---|
| Crystalline to liquid crystal phase transition temperature (°C) | 48.73 | 49 |
| Liquid crystal to isotropic phase transition temperature (oC) | 82.79 | 85 |
| λ (nm) | n0 | s × 10−4 (K−1) |
|---|---|---|
| 500 | 1.59927 | 1.90232 |
| 540 | 1.59089 | 1.71868 |
| 570 | 1.61333 | 2.49419 |
| 589.3 | 1.61153 | 2.49419 |
| 630 | 1.60766 | 2.4039 |
| T (oC) | A | B × 10−3 (μm2) | C × 10−4 (μm4) | VD |
|---|---|---|---|---|
| 57.48 | 1.51394 | 4.84 | 2.42616 | 47.321 |
| 60 | 1.51267 | 5.16 | 2.15102 | 46.438 |
| 62.3 | 1.51402 | 3.86 | 4.33966 | 45.991 |
| 65 | 1.51264 | 4.11 | 4.3272 | 44.386 |
| 67.5 | 1.51207 | 4.02 | 4.62173 | 43.603 |
| 70 | 1.51012 | 4.74 | 3.77997 | 42.857 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shaban, H.; Shaaban, A.; Salem, M.S.; Gomaa, L.R.; Medhat, M.; Du, Y.-C. Kretschmann-Based Optical Sensor via Thermally Tunable Refractive Index. Crystals 2021, 11, 616. https://doi.org/10.3390/cryst11060616
Shaban H, Shaaban A, Salem MS, Gomaa LR, Medhat M, Du Y-C. Kretschmann-Based Optical Sensor via Thermally Tunable Refractive Index. Crystals. 2021; 11(6):616. https://doi.org/10.3390/cryst11060616
Chicago/Turabian StyleShaban, Hassanein, Adel Shaaban, Marwa S. Salem, Lotfy Rabeh Gomaa, Mohamed Medhat, and Yi-Chun Du. 2021. "Kretschmann-Based Optical Sensor via Thermally Tunable Refractive Index" Crystals 11, no. 6: 616. https://doi.org/10.3390/cryst11060616
APA StyleShaban, H., Shaaban, A., Salem, M. S., Gomaa, L. R., Medhat, M., & Du, Y.-C. (2021). Kretschmann-Based Optical Sensor via Thermally Tunable Refractive Index. Crystals, 11(6), 616. https://doi.org/10.3390/cryst11060616