Hot Electron Plasmon-Resonant Grating Structures for Enhanced Photochemistry: A Theoretical Study
Abstract
1. Introduction
2. Methods
3. Theory
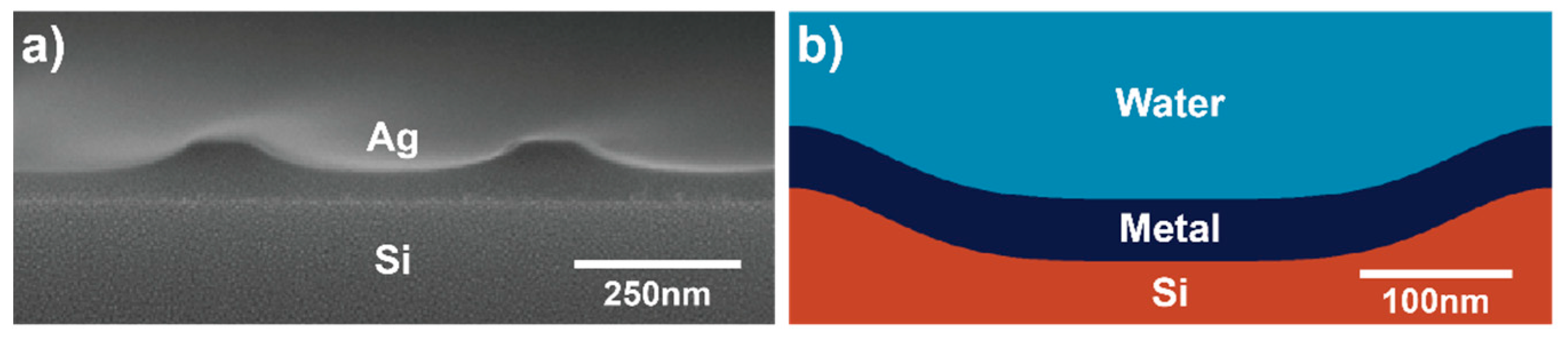
4. Corrugated Grating Structure
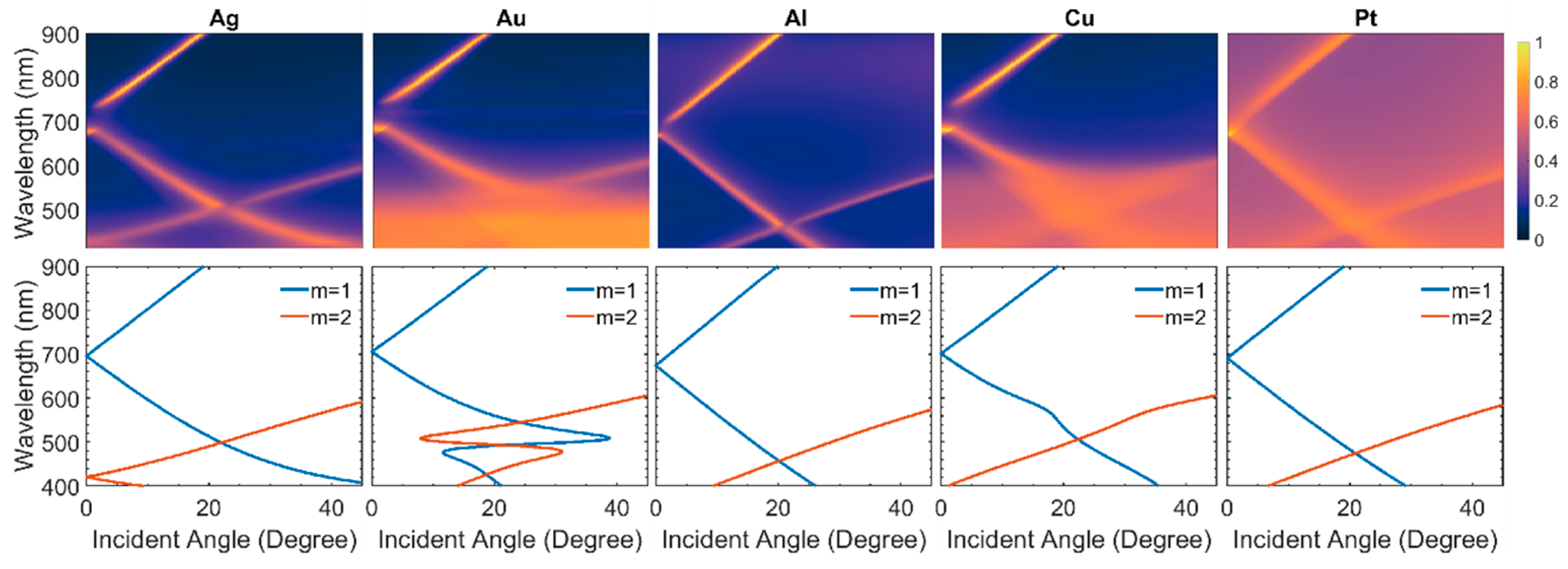
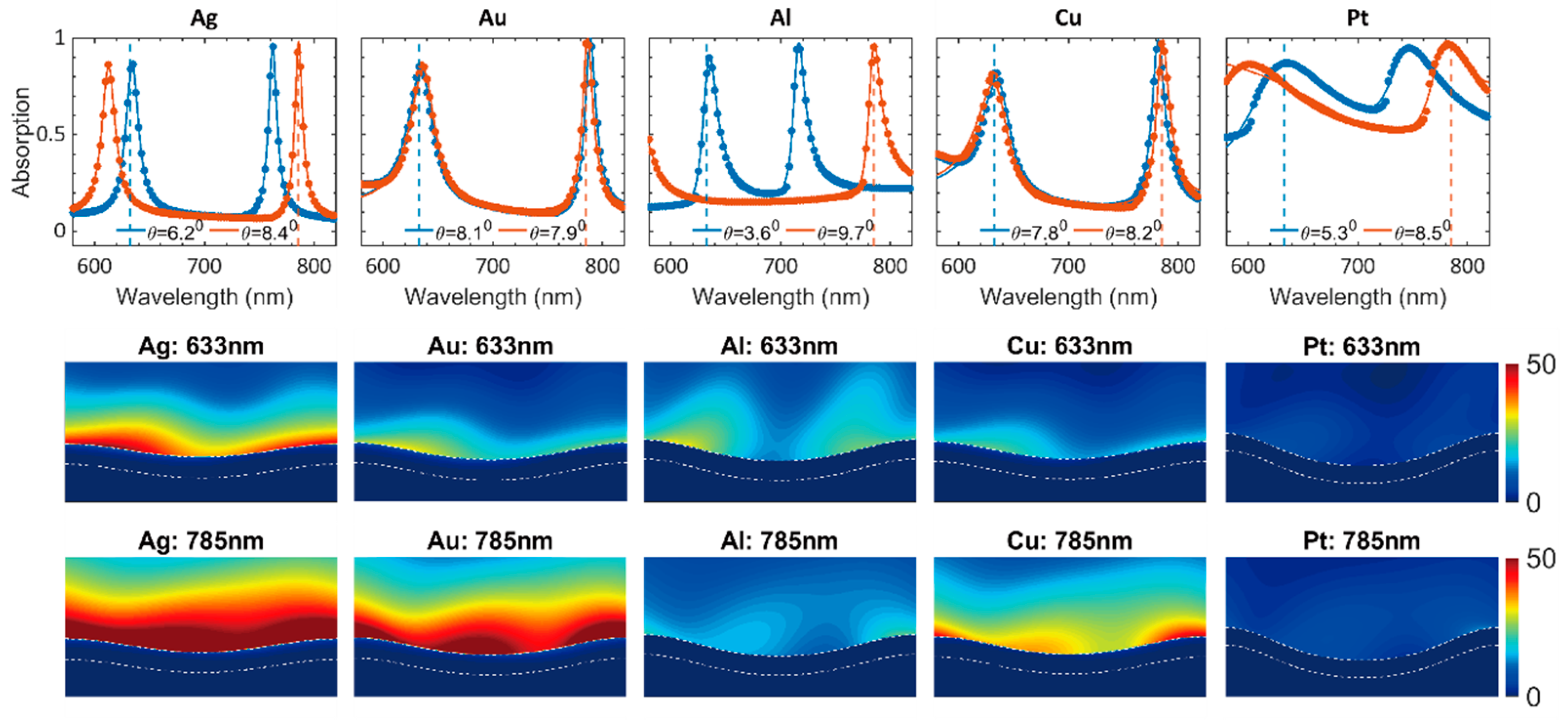
5. Tuning the Corrugation for Each Material
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Brongersma, M.L.; Halas, N.J.; Nordlander, P. Plasmon-induced hot carrier science and technology. Nat. Nanotechnol. 2015, 10, 25–34. [Google Scholar] [CrossRef] [PubMed]
- Narang, P.; Sundararaman, R.; Atwater, H.A. Plasmonic hot carrier dynamics in solid-state and chemical systems for energy conversion. Nanophotonics 2016, 5, 96–111. [Google Scholar] [CrossRef]
- Chalabi, H.; Schoen, D.; Brongersma, M.L. Hot-Electron Photodetection with a Plasmonic Nanostripe Antenna. Nano Lett. 2014, 14, 1374–1380. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Valentine, J. Harvesting the loss: Surface plasmon-based hot electron photodetection. Nanophotonics 2016, 6, 177–191. [Google Scholar] [CrossRef]
- Zhang, Y.; He, S.; Guo, W.; Hu, Y.; Huang, J.; Mulcahy, J.R.; Wei, W.D. Surface-Plasmon-Driven Hot Electron Pho-tochemistry. Chem. Rev. 2018, 118, 2927–2954. [Google Scholar] [CrossRef]
- Liu, Z.; Hou, W.; Pavaskar, P.; Aykol, M.; Cronin, S.B. Plasmon Resonant Enhancement of Photocatalytic Water Splitting Under Visible Illumination. Nano Lett. 2011, 11, 1111–1116. [Google Scholar] [CrossRef]
- Hou, B.; Shen, L.; Shi, H.; Kapadia, R.; Wang, B. Hot electron-driven photocatalytic water splitting. Phys. Chem. Chem. Phys. 2017, 19, 2877–2881. [Google Scholar] [CrossRef]
- Mukherjee, S.; Libisch, F.; Large, N.; Neumann, O.; Brown, L.V.; Cheng, J.; Lassiter, J.B.; Carter, E.A.; Nordlander, P.; Halas, N.J. Hot Electrons Do the Impossible: Plasmon-Induced Dissociation of H2on Au. Nano Lett. 2013, 13, 240–247. [Google Scholar] [CrossRef] [PubMed]
- Mukherjee, S.; Zhou, L.; Goodman, A.M.; Large, N.; Ayala-Orozco, C.; Zhang, Y.; Nordlander, P.; Halas, N.J. Hot-Electron-Induced Dissociation of H2on Gold Nanoparticles Supported on SiO2. J. Am. Chem. Soc. 2014, 136, 64–67. [Google Scholar] [CrossRef] [PubMed]
- Brown, A.M.; Sundararaman, R.; Narang, P.; Goddard, I.W.A.; Atwater, H.A. Nonradiative Plasmon Decay and Hot Carrier Dynamics: Effects of Phonons, Surfaces, and Geometry. ACS Nano 2016, 10, 957–966. [Google Scholar] [CrossRef] [PubMed]
- Sundararaman, R.; Narang, P.; Jermyn, A.S.; Iii, W.A.G.; Atwater, H.A. Theoretical predictions for hot-carrier generation from surface plasmon decay. Nat. Commun. 2014, 5, 5788. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Shi, H.; Shen, L.; Wang, Y.; Cronin, S.B.; Dawlaty, J.M. Ultrafast Dynamics of Hot Electrons in Nanostructures: Distinguishing the Influence on Interband and Plasmon Resonances. ACS Photonics 2019, 6, 2295–2302. [Google Scholar] [CrossRef]
- Wang, Y.; Aravind, I.; Cai, Z.; Shen, L.; Gibson, G.N.; Chen, J.; Wang, B.; Shi, H.; Song, B.; Guignon, E.F.; et al. Hot Electron Driven Photocatalysis on Plasmon-Resonant Grating Nanostructures. ACS Appl. Mater. Interfaces 2020, 12, 17459–17465. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Shen, L.; Wang, Y.; Hou, B.; Gibson, G.N.; Poudel, N.; Chen, J.; Shi, H.; Guignon, E.; Cady, N.C.; et al. Hot electron-driven photocatalysis and transient absorption spectroscopy in plasmon resonant grating structures. Faraday Discuss. 2019, 214, 325–339. [Google Scholar] [CrossRef] [PubMed]
- Shen, L.; Poudel, N.; Gibson, G.N.; Hou, B.; Chen, J.; Shi, H.; Guignon, E.; Page, W.D.; Pilar, A.; Cronin, S.B. Plas-mon resonant amplification of a hot electron-driven photodiode. Nano Res. 2018, 11, 2310–2314. [Google Scholar] [CrossRef]
- Shen, L.; Gibson, G.N.; Poudel, N.; Hou, B.; Chen, J.; Shi, H.; Guignon, E.; Cady, N.C.; Page, W.D.; Pilar, A.; et al. Plasmon resonant amplification of hot electron-driven photocatalysis. Appl. Phys. Lett. 2018, 113, 113104. [Google Scholar] [CrossRef]
- Lo, H.Y.; Chan, C.Y.; Ong, H.C. Direct measurement of radiative scattering of surface plasmon polariton reso-nance from metallic arrays by polarization-resolved reflectivity spectroscopy. Appl. Phys. Lett. 2012, 101, 223108. [Google Scholar] [CrossRef]
- Wonjoo, S.; Zheng, W.; Shanhui, F. Temporal coupled-mode theory and the presence of non-orthogonal modes in lossless multimode cavities. IEEE J. Quantum Electron. 2004, 40, 1511–1518. [Google Scholar] [CrossRef]
- Seok, T.J.; Jamshidi, A.; Kim, M.; Dhuey, S.; Lakhani, A.; Choo, H.; Schuck, P.J.; Cabrini, S.; Schwartzberg, A.M.; Bokor, J.; et al. Radiation Engineering of Optical Antennas for Maximum Field Enhancement. Nano Lett. 2011, 11, 2606–2610. [Google Scholar] [CrossRef]
- Liu, X.; Gao, J.; Wang, Y.; Wang, X.; Yang, H.; Hu, H.; Gao, J.; Bourouina, T.; Cui, T. Simultaneous field enhancement and loss inhibition based on surface plasmon polariton mode hybridization. Nanophotonics 2020, 9, 2809–2816. [Google Scholar] [CrossRef]
- Maier, S.A. Plasmonic field enhancement and SERS in the effective mode volume picture. Opt. Express 2006, 14, 1957–1964. [Google Scholar] [CrossRef]
- Cao, Z.; Zhang, L.; Chan, C.Y.; Ong, H.C. Interplay between absorption and radiative decay rates of surface plasmon polaritons for field enhancement in periodic arrays. Opt. Lett. 2014, 39, 501–504. [Google Scholar] [CrossRef]
- Krishnan, A.; O’Gorman, A.B.; Povinelli, M.L. Design of switchable, narrowband thermal absorption peaks in met-al-vanadium-dioxide gratings. J. Opt. 2020, 22, 094002. [Google Scholar] [CrossRef]
- Cao, Z.; Lo, H.-Y.; Ong, H.-C. Determination of absorption and radiative decay rates of surface plasmon polaritons from nanohole array. Opt. Lett. 2012, 37, 5166–5168. [Google Scholar] [CrossRef]
- Johnson, S.G. The NLopt Nonlinear-Optimization Package. Available online: http://github.com/stevengj/nlopt (accessed on 26 January 2021).
- Cao, Z.L.; Ong, H.C. Determination of the absorption and radiative decay rates of dark and bright plasmonic modes. Opt. Express 2014, 22, 16112–16129. [Google Scholar] [CrossRef]




| Original Gratings | Optimized Geometry | |||||||
|---|---|---|---|---|---|---|---|---|
| A (nm) | σ (nm) | A (nm) | σ (nm) | |||||
| Ag | 58.9 | 125 | 7.05 | 6.04 | 41.4 | 235 | 13.00 | 23.00 |
| Au | 58.9 | 125 | 4.37 | 4.85 | 46.2 | 198 | 5.90 | 17.60 |
| Al | 58.9 | 125 | 5.14 | 5.82 | 59.5 | 198 | 7.06 | 7.44 |
| Cu | 58.9 | 125 | 3.65 | 4.77 | 50.5 | 215 | 4.47 | 12.20 |
| Pt | 58.9 | 125 | 1.50 | 1.97 | 95.7 | 178 | 1.73 | 2.48 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aravind, I.; Wang, Y.; Cai, Z.; Shen, L.; Zhao, B.; Yang, S.; Wang, Y.; Dawlaty, J.M.; Gibson, G.N.; Guignon, E.; et al. Hot Electron Plasmon-Resonant Grating Structures for Enhanced Photochemistry: A Theoretical Study. Crystals 2021, 11, 118. https://doi.org/10.3390/cryst11020118
Aravind I, Wang Y, Cai Z, Shen L, Zhao B, Yang S, Wang Y, Dawlaty JM, Gibson GN, Guignon E, et al. Hot Electron Plasmon-Resonant Grating Structures for Enhanced Photochemistry: A Theoretical Study. Crystals. 2021; 11(2):118. https://doi.org/10.3390/cryst11020118
Chicago/Turabian StyleAravind, Indu, Yu Wang, Zhi Cai, Lang Shen, Bofan Zhao, Sisi Yang, Yi Wang, Jahan M. Dawlaty, George N. Gibson, Ernest Guignon, and et al. 2021. "Hot Electron Plasmon-Resonant Grating Structures for Enhanced Photochemistry: A Theoretical Study" Crystals 11, no. 2: 118. https://doi.org/10.3390/cryst11020118
APA StyleAravind, I., Wang, Y., Cai, Z., Shen, L., Zhao, B., Yang, S., Wang, Y., Dawlaty, J. M., Gibson, G. N., Guignon, E., Cady, N. C., Page, W. D., Pilar, A., & Cronin, S. B. (2021). Hot Electron Plasmon-Resonant Grating Structures for Enhanced Photochemistry: A Theoretical Study. Crystals, 11(2), 118. https://doi.org/10.3390/cryst11020118