Electronic, Optical, and Thermoelectric Properties of Bulk and Monolayer Germanium Tellurides
Abstract
:1. Introduction
2. Computational Methods
2.1. DFT Parameters
2.2. Optical Coefficients
2.3. Thermoelectric Transport Coefficients
3. Results and Discussion
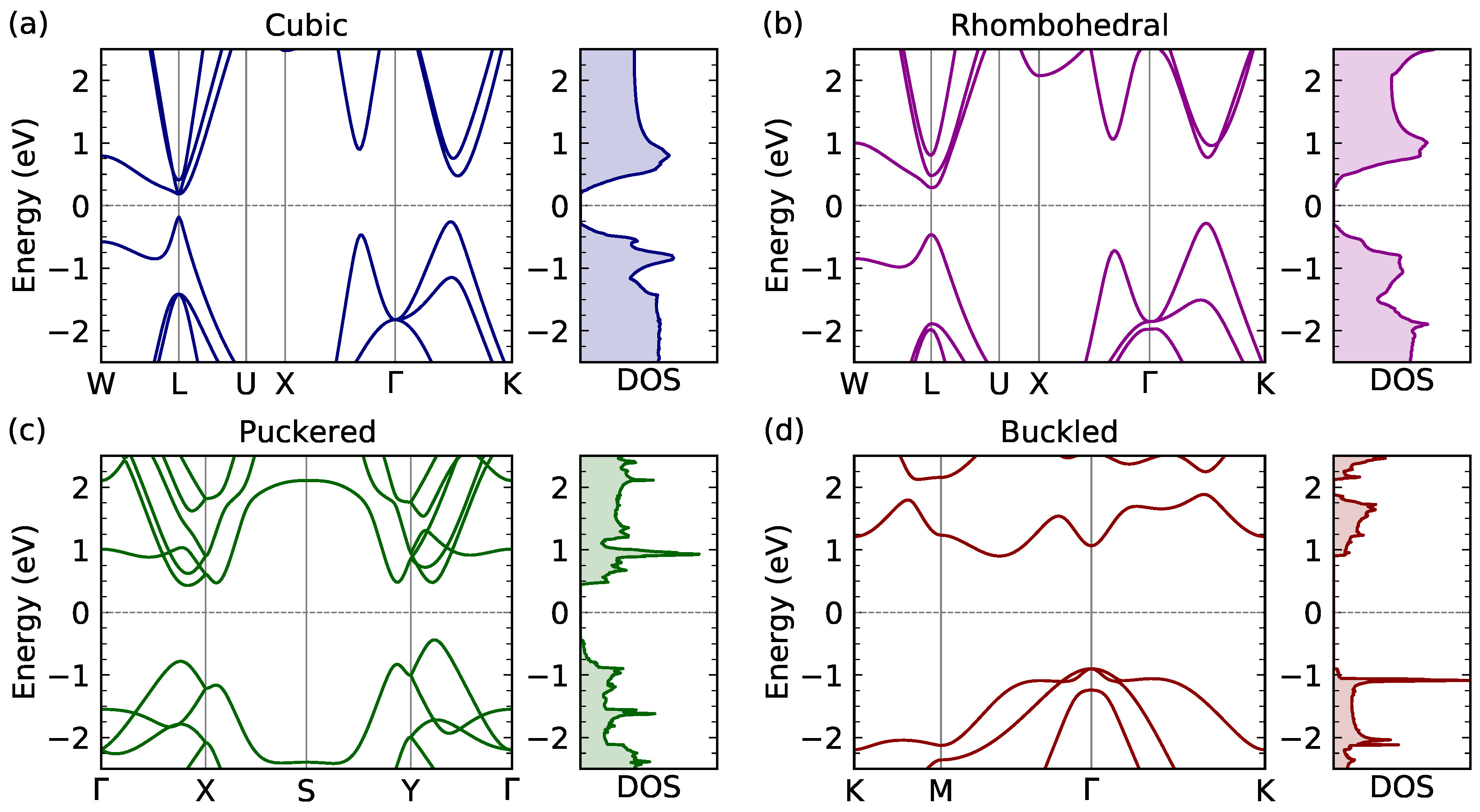
3.1. Electronic Band Structures
3.2. Absorption Spectra
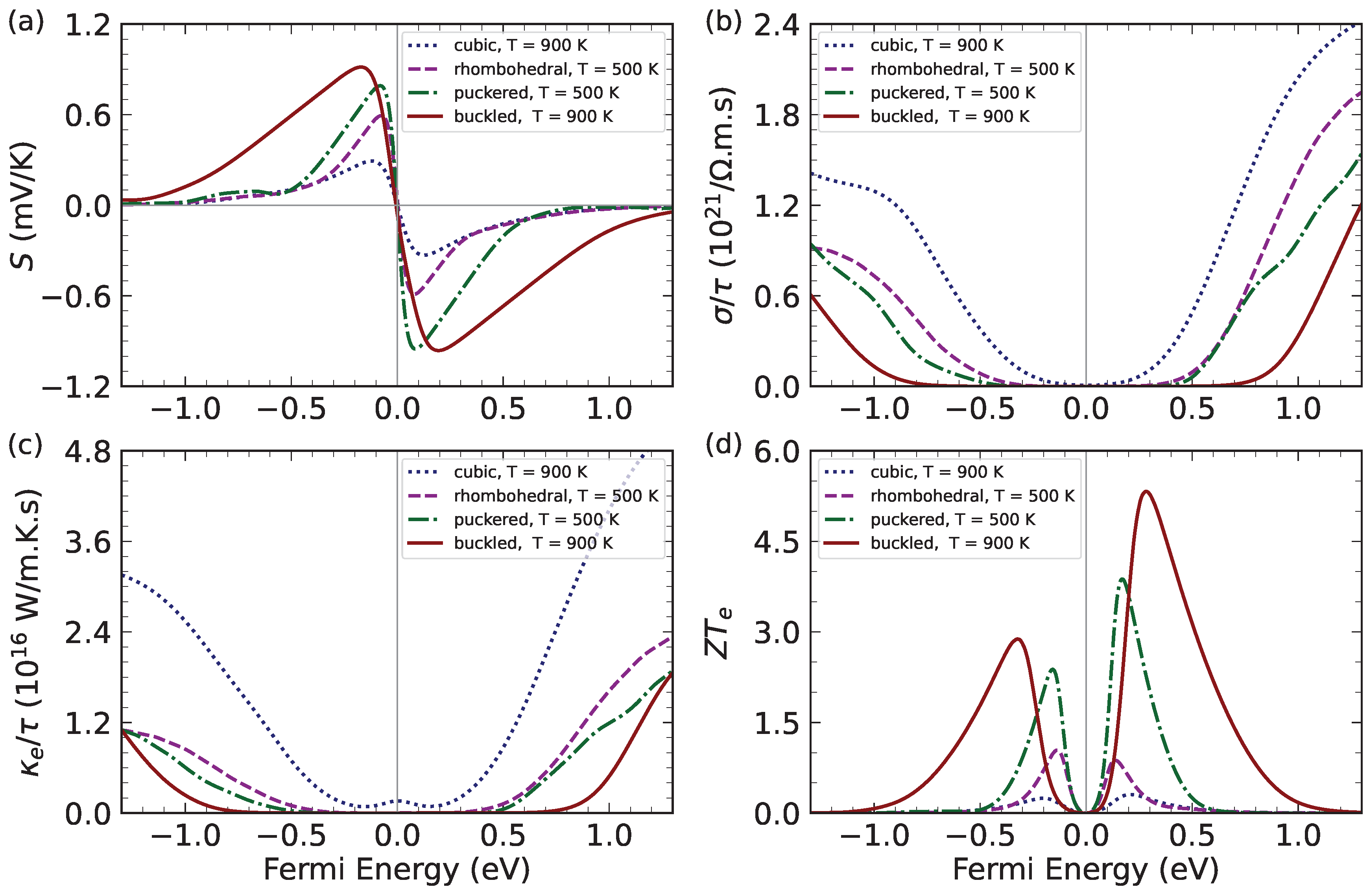
3.3. Thermoelectric Properties
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hamakawa, Y. Solar PV energy conversion and the 21st century’s civilization. Sol. Energy Mater. Sol. Cells 2002, 74, 13–23. [Google Scholar] [CrossRef]
- Becquerel, E. Memoire sur les effects electriques produits sous I’influence des rayons solaires. Comptes Rendus 1839, 9, 561–567. [Google Scholar]
- Seebeck, T.J. Magnetische Polarisation der Metalle und Erze Durch Temperatur-Differenz; Abhandlungen der Königlichen Akademie der Wissenschaften: Berlin, Germany, 1825; pp. 265–373. [Google Scholar]
- Lewis, J.E. Optical properties and energy gap of GeTe from reflectance studies. Phys. Status Solidi B 1973, 59, 367–377. [Google Scholar] [CrossRef]
- Chattopadhyay, T.; Boucherle, J.X.; vonSchnering, H.G. Neutron diffraction study on the structural phase transition in GeTe. J. Phys. C 1987, 20, 1431. [Google Scholar] [CrossRef]
- Singh, D.J. Optical properties of cubic and rhombohedral GeTe. J. Appl. Phys. 2013, 113, 203101. [Google Scholar] [CrossRef] [Green Version]
- Wuttig, M.; Yamada, N. Phase-change materials for rewriteable data storage. Nat. Mater. 2007, 6, 824–832. [Google Scholar] [CrossRef]
- Raoux, S. Phase Change Materials. Annu. Rev. Mater. Sci. 2009, 39, 25–48. [Google Scholar] [CrossRef]
- Li, J.; Chen, Z.; Zhang, X.; Sun, Y.; Yang, J.; Pei, Y. Electronic origin of the high thermoelectric performance of GeTe among the p-type group IV monotellurides. NPG Asia Mater. 2017, 9, e353. [Google Scholar] [CrossRef] [Green Version]
- Li, J.; Zhang, X.; Chen, Z.; Lin, S.; Li, W.; Shen, J.; Witting, I.T.; Faghaninia, A.; Chen, Y.; Jain, A.; et al. Low-Symmetry Rhombohedral GeTe Thermoelectrics. Joule 2018, 2, 976–987. [Google Scholar] [CrossRef]
- Feynman, R.P. There’s plenty of room at the bottom. Eng. Sci. Caltech Mag. 1960, 25, 22–36. [Google Scholar]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric field effect in atomically thin carbon films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Katsnelson, M.I.; Grigorieva, I.V.; Dubonos, S.V.; Firsov, A.A. Two-dimensional gas of massless Dirac fermions in graphene. Nature 2005, 438, 197–200. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Jiang, Z.; Zhang, Y.; Morozov, S.V.; Stormer, H.L.; Zeitler, U.; Maan, J.C.; Boebinger, G.S.; Kim, P.; Geim, A.K. Room-temperature quantum Hall effect in graphene. Science 2007, 315, 1379. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, H.; Neal, A.T.; Zhu, Z.; Luo, Z.; Xu, X.; Tománek, D.; Ye, P.D. Phosphorene: An unexplored 2D semiconductor with a high hole mobility. ACS Nano 2014, 8, 4033–4041. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mak, K.F.; Lee, C.; Hone, J.; Shan, J.; Heinz, T.F. Atomically thin MoS2: A new direct-gap semiconductor. Phys. Rev. Lett. 2010, 105, 136805. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bandurin, D.A.; Tyurnina, A.V.; Yu, G.L.; Mishchenko, A.; Zólyomi, V.; Morozov, S.V.; Kumar, R.K.; Gorbachev, R.V.; Kudrynskyi, Z.R.; Pezzini, S.; et al. High electron mobility, quantum Hall effect and anomalous optical response in atomically thin InSe. Nat. Nanotechnol. 2017, 12, 223–227. [Google Scholar] [CrossRef]
- Wdowik, U.D.; Parlinski, K.; Rols, S.; Chatterji, T. Soft-phonon mediated structural phase transition in GeTe. Phys. Rev. B 2014, 89, 224306. [Google Scholar] [CrossRef]
- Wan, W.; Liu, C.; Xiao, W.; Yao, Y. Promising ferroelectricity in 2D group IV tellurides: A first-principles study. Appl. Phys. Lett. 2017, 111, 132904. [Google Scholar] [CrossRef] [Green Version]
- Zhang, P.; Zhao, F.; Long, P.; Wang, Y.; Yue, Y.; Liu, X.; Feng, Y.; Li, R.; Hu, W.; Li, Y.; et al. Sonication-assisted liquid-phase exfoliated α-GeTe: A two-dimensional material with high Fe3+ sensitivity. Nanoscale 2018, 10, 15989–15997. [Google Scholar] [CrossRef]
- Zhang, D.; Hu, S.; Sun, Y.; Liu, X.; Wang, H.; Wang, H.; Chen, Y.; Ni, Y. XTe (X = Ge, Sn, Pb) monolayers: Promising thermoelectric materials with ultralow lattice thermal conductivity and high-power factor. ES Energy Environ. 2020, 10, 59–65. [Google Scholar]
- Wang, Q.; Wu, L.; Urban, A.; Cao, H.; Lu, P. Anisotropic to Isotropic Transition in Monolayer Group-IV Tellurides. Materials 2021, 14, 4495. [Google Scholar] [CrossRef]
- Ge, G.; Zhang, Y.; Yan, H.; Yang, J.; Zhou, L.; Sui, X. Complexly electronic structure induced largely tunable anisotropic mobility for monolayer GeTe by uniaxial strain. Appl. Surf. Sci. 2021, 538, 148009. [Google Scholar] [CrossRef]
- Qiao, M.; Chen, Y.; Wang, Y.; Li, Y. The germanium telluride monolayer: A two dimensional semiconductor with high carrier mobility for photocatalytic water splitting. J. Mater. Chem. A 2018, 6, 4119–4125. [Google Scholar] [CrossRef]
- Zhang, X.; Zhao, F.; Wang, Y.; Liang, X.; Zhang, Z.; Feng, Y.; Li, Y.; Tang, L.; Feng, W. Two-Dimensional GeTe: Air Stability and Photocatalytic Performance for Hydrogen Evolution. ACS Appl. Mater. Interfaces 2020, 12, 37108–37115. [Google Scholar] [CrossRef] [PubMed]
- Giannozzi, P.; Andreussi, O.; Brumme, T.; Bunau, O.; Buongiorno Nardelli, M.; Calandra, M.; Car, R.; Cavazzoni, C.; Ceresoli, D.; Cococcioni, M.; et al. Advanced capabilities for materials modelling with Quantum ESPRESSO. J. Phys. Condens. Matter 2017, 29, 465901. [Google Scholar] [CrossRef] [Green Version]
- Goldsmid, H.J. Introduction to Thermoelectricity; Springer: Berlin, Germay, 2010. [Google Scholar]
- Madsen, G.K.H.; Singh, D.J. BoltzTraP. A code for calculating band-structure dependent quantities. Comput. Phys. Commun. 2006, 175, 67–71. [Google Scholar] [CrossRef] [Green Version]
- Madsen, G.K.H.; Carrete, J.; Verstraete, M.J. BoltzTraP2, a program for interpolating band structures and calculating semi-classical transport coefficients. Comput. Phys. Commun. 2018, 231, 140–145. [Google Scholar] [CrossRef] [Green Version]
- Momma, K.; Izumi, F. VESTA: A three-dimensional visualization system for electronic and structural analysis. J. Appl. Crystallogr. 2008, 41, 653–658. [Google Scholar] [CrossRef]
- Jeong, K.; Park, S.; Park, D.; Ahn, M.; Han, J.; Yang, W.; Jeong, H.S.; Cho, M.H. Evolution of crystal structures in GeTe during phase transition. Sci. Rep. 2017, 7, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Nonaka, T.; Ohbayashi, G.; Toriumi, Y.; Mori, Y.; Hashimoto, H. Crystal structure of GeTe and Ge2Sb2Te5 meta-stable phase. Thin Solid Film. 2000, 370, 258–261. [Google Scholar] [CrossRef]
- Zhang, D.; Zhou, Z.; Wang, H.; Yang, Z.; Liu, C. Tunable Electric Properties of Bilayer α-GeTe with Different Interlayer Distances and External Electric Fields. Nanoscale Res. Lett. 2018, 13, 400. [Google Scholar] [CrossRef]
- Zhang, X.; Jin, K.H.; Mao, J.; Zhao, M.; Liu, Z.; Liu, F. Prediction of intrinsic topological superconductivity in Mn-doped GeTe monolayer from first-principles. Npj Comput. Mater. 2021, 7, 44. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- Hamann, D.R. Optimized norm-conserving Vanderbilt pseudopotentials. Phys. Rev. B 2013, 88, 085117. [Google Scholar] [CrossRef] [Green Version]
- Schlipf, M.; Gygi, F. Optimization algorithm for the generation of ONCV pseudopotentials. Comput. Phys. Commun. 2015, 196, 36–44. [Google Scholar] [CrossRef] [Green Version]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar] [CrossRef] [Green Version]
- Heyd, J.; Scuseria, G.E.; Ernzerhof, M. Hybrid functionals based on a screened Coulomb potential. J. Chem. Phys. 2003, 118, 8207–8215. [Google Scholar] [CrossRef] [Green Version]
- Giustino, F. Materials Modelling Using Density Functional Theory: Properties and Predictions; Oxford University Press: Oxford, UK, 2014. [Google Scholar]
- Dresselhaus, M.; Dresselhaus, G.; Cronin, S.B.; Souza Filho, A.G. Solid State Properties; Springer: Berlin, Germany, 2018. [Google Scholar]
- Aryasetiawan, F.; Gunnarsson, O. The GW method. Rep. Prog. Phys. 1998, 61, 237. [Google Scholar] [CrossRef] [Green Version]
- Tung, Y.W.; Cohen, M.L. Relativistic band structure and electronic properties of SnTe, GeTe, and PbTe. Phys. Rev. 1969, 180, 823. [Google Scholar] [CrossRef]
- Korzhuev, M.A. On the fundamental energy gap in GeTe. Phys. Status Solidi B 1982, 112, K39–K41. [Google Scholar] [CrossRef]
- Okoye, C.M.I. Electronic and optical properties of SnTe and GeTe. J. Phys. Condens. Matter 2002, 14, 8625. [Google Scholar] [CrossRef]
- Kim, M.R.; Ma, D. Quantum-dot-based solar cells: Recent advances, strategies, and challenges. J. Phys. Chem. Lett. 2015, 6, 85–99. [Google Scholar] [CrossRef] [PubMed]
- Bernardi, M.; Palummo, M.; Grossman, J.C. Extraordinary sunlight absorption and one nanometer thick photovoltaics using two-dimensional monolayer materials. Nano Lett. 2013, 13, 3664–3670. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Tan, X.; Yin, K.; Liu, H.; Tang, X.; Shi, J.; Zhang, Q.; Uher, C. Convergence of Conduction Bands as a Means of Enhancing Thermoelectric Performance of n-Type Mg2Si1−xSnx Solid Solutions. Phys. Rev. Lett. 2012, 108, 166601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tang, Y.; Gibbs, Z.M.; Agapito, L.A.; Li, G.; Kim, H.S.; Nardelli, M.B.; Curtarolo, S.; Snyder, G.J. Convergence of multi-valley bands as the electronic origin of high thermoelectric performance in CoSb3 skutterudites. Nat. Mater. 2015, 14, 1223–1228. [Google Scholar] [CrossRef] [PubMed]
- Hicks, L.D.; Dresselhaus, M.S. Effect of quantum-well structures on the thermoelectric figure of merit. Phys. Rev. B 1993, 47, 12727. [Google Scholar] [CrossRef]
- Hung, N.T.; Hasdeo, E.H.; Nugraha, A.R.T.; Dresselhaus, M.S.; Saito, R. Quantum Effects in the Thermoelectric Power Factor of Low-Dimensional Semiconductors. Phys. Rev. Lett. 2016, 117, 036602. [Google Scholar] [CrossRef] [PubMed]
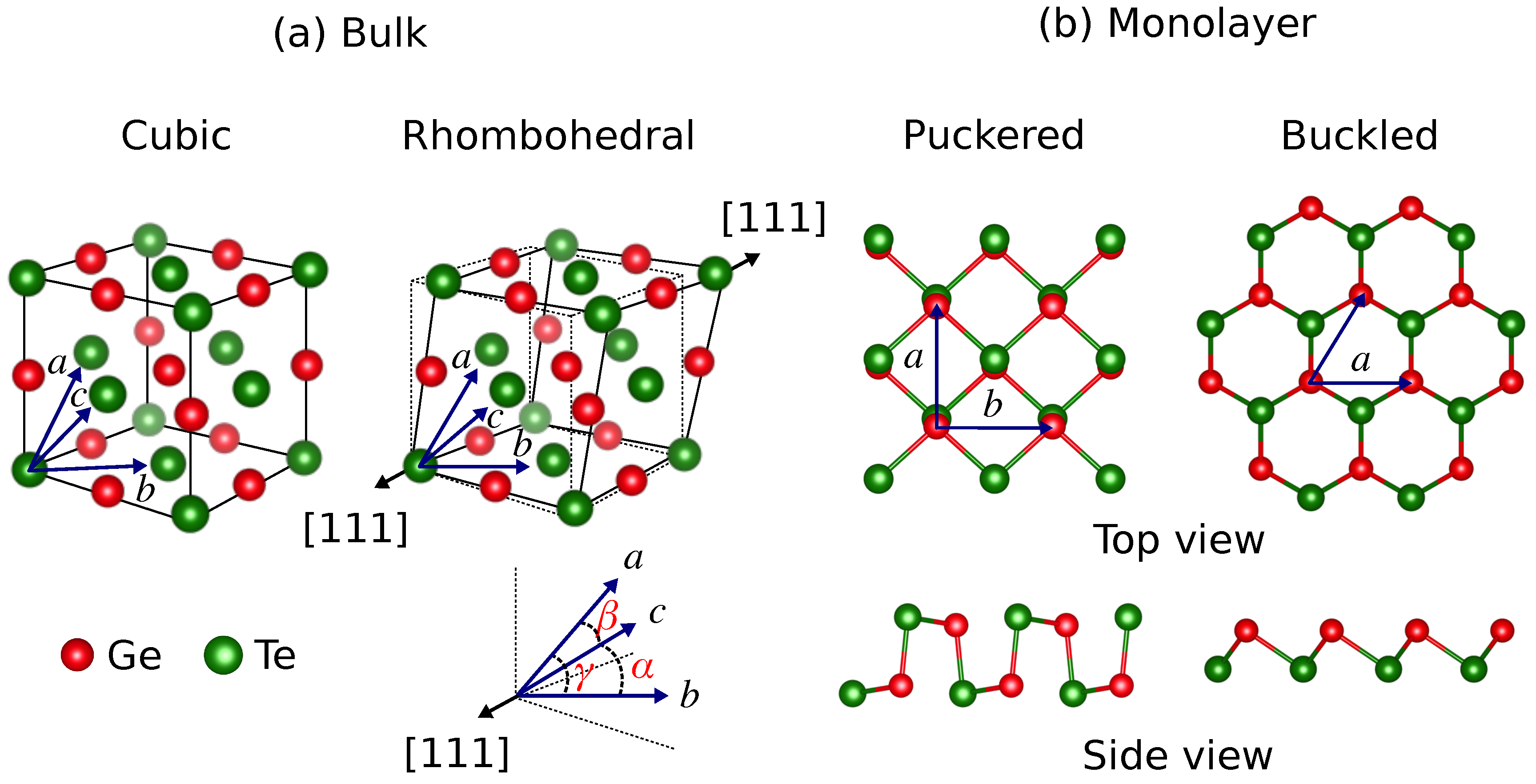

| GeTe Structure | This Work | Reference Data |
|---|---|---|
| cubic (bulk) | , | [31], [5], [32] |
| rhombohedral (bulk) | , | [20], [31], [5] |
| puckered (monolayer) | ) | (, ) [19] |
| buckled (monolayer) | [33], [34], [20,24] |
| Method | Cubic | Rhombohedral | Puckered | Buckled |
|---|---|---|---|---|
| ONCV-GGA | ||||
| HSE |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sinambela, W.V.; Wella, S.A.; Arsyad, F.S.; Hung, N.T.; Nugraha, A.R.T. Electronic, Optical, and Thermoelectric Properties of Bulk and Monolayer Germanium Tellurides. Crystals 2021, 11, 1290. https://doi.org/10.3390/cryst11111290
Sinambela WV, Wella SA, Arsyad FS, Hung NT, Nugraha ART. Electronic, Optical, and Thermoelectric Properties of Bulk and Monolayer Germanium Tellurides. Crystals. 2021; 11(11):1290. https://doi.org/10.3390/cryst11111290
Chicago/Turabian StyleSinambela, Wenny V., Sasfan A. Wella, Fitri S. Arsyad, Nguyen Tuan Hung, and Ahmad R. T. Nugraha. 2021. "Electronic, Optical, and Thermoelectric Properties of Bulk and Monolayer Germanium Tellurides" Crystals 11, no. 11: 1290. https://doi.org/10.3390/cryst11111290
APA StyleSinambela, W. V., Wella, S. A., Arsyad, F. S., Hung, N. T., & Nugraha, A. R. T. (2021). Electronic, Optical, and Thermoelectric Properties of Bulk and Monolayer Germanium Tellurides. Crystals, 11(11), 1290. https://doi.org/10.3390/cryst11111290








