Chalcogen-Nitrogen Bond: Insights into a Key Chemical Motif
Abstract
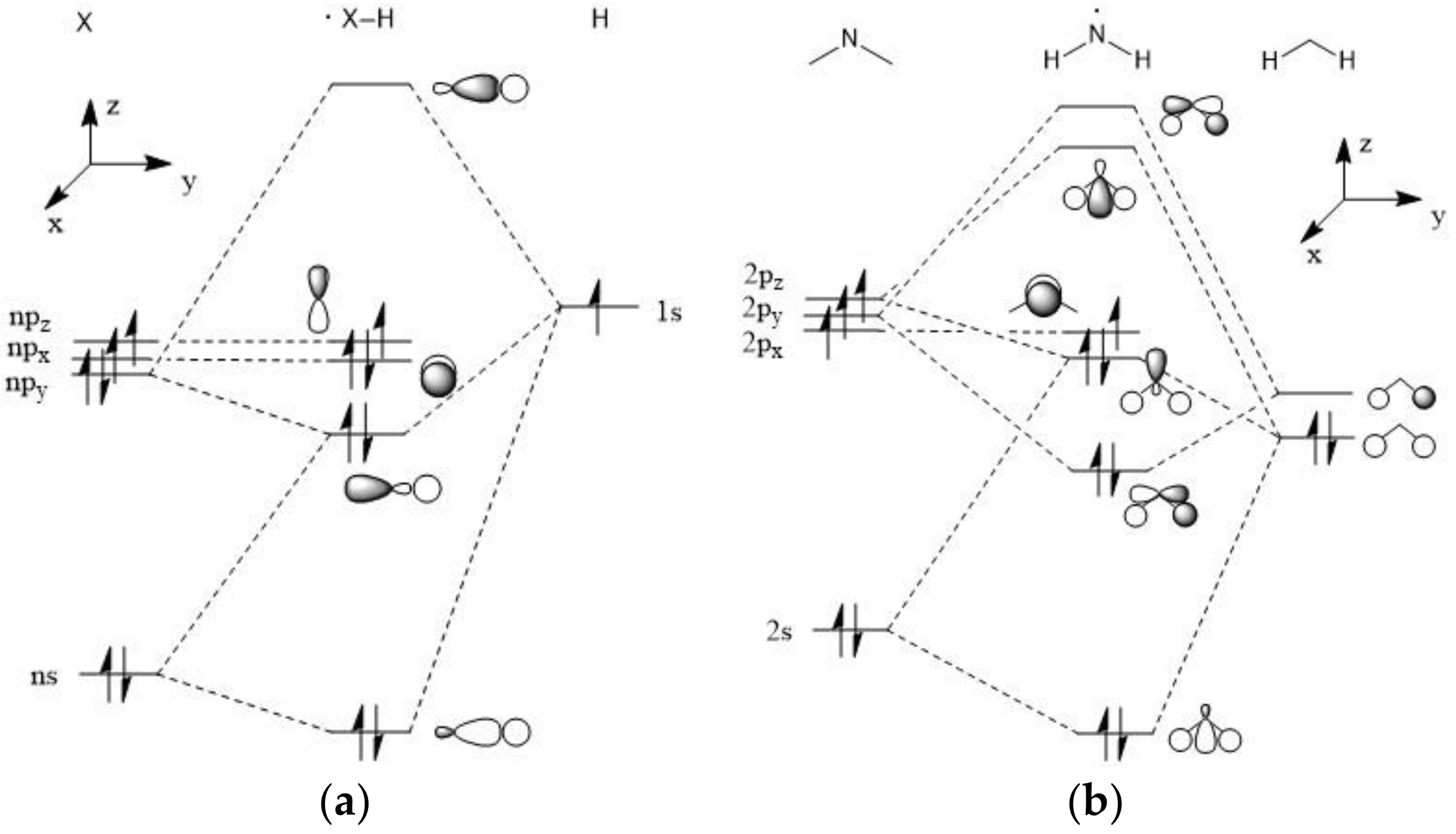
1. Introduction
2. Results
3. Discussion
4. Methods
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Joshi, P.; More, M.; Jadhav, A.; Khanna, P. Materials and biological applications of 1,2,3-selenadiazoles: A review. Mater. Today Chem. 2020, 16, 100255. [Google Scholar] [CrossRef]
- Alberto, E.E.; Nascimento, V.D.; Braga, A.L. Catalytic application of selenium and tellurium compounds as glutathione peroxidase enzyme mimetics. J. Braz. Chem. Soc. 2010, 21, 2032–2041. [Google Scholar] [CrossRef]
- Barbosa, N.V.; Nogueira, C.W.; Nogara, P.A.; De Bem, A.F.; Aschner, M.; Barbosa, N.B.D.V. Organoselenium compounds as mimics of selenoproteins and thiol modifier agents. Metallomics 2017, 9, 1703–1734. [Google Scholar] [CrossRef]
- Chivers, T.; Laitinen, R.S. Neutral binary chalcogen–nitrogen and ternary S,N,P molecules: New structures, bonding insights and potential applications. Dalton Trans. 2020, 49, 6532–6547. [Google Scholar] [CrossRef]
- Engman, L.; Hallberg, A. Expedient synthesis of ebselen and related compounds. J. Org. Chem. 1989, 54, 2964–2966. [Google Scholar] [CrossRef]
- Sarma, B.K.; Manna, D.; Minoura, M.; Mugesh, G. Synthesis, Structure, Spirocyclization Mechanism, and Glutathione Peroxidase-like Antioxidant Activity of Stable Spirodiazaselenurane and Spirodiazatellurane. J. Am. Chem. Soc. 2010, 132, 5364–5374. [Google Scholar] [CrossRef]
- Zade, S.S.; Panda, S.; Tripathi, S.K.; Singh, H.B.; Wolmershäuser, G. Convenient Synthesis, Characterization and GPx-Like Catalytic Activity of Novel Ebselen Derivatives. Eur. J. Org. Chem. 2004, 2004, 3857–3864. [Google Scholar] [CrossRef]
- Kersting, B.; DeLion, M. Synthesis of Benzisochalcogenol and -azole Derivatives via ortho Metalation of Isophthalamides. Zeitschrift für Naturforschung B 1999, 54, 1042–1047. [Google Scholar] [CrossRef]
- Bhowmick, D.; Mugesh, G. Introduction of a catalytic triad increases the glutathione peroxidase-like activity of diaryl diselenides. Org. Biomol. Chem. 2015, 13, 9072–9082. [Google Scholar] [CrossRef]
- Sarma, B.K.; Mugesh, G. Antioxidant Activity of the Anti-Inflammatory Compound Ebselen: A Reversible Cyclization Pathway via Selenenic and Seleninic Acid Intermediates. Chem. A Eur. J. 2008, 14, 10603–10614. [Google Scholar] [CrossRef]
- Singh, V.P.; Singh, H.B.; Butcher, R.J. Synthesis and Glutathione Peroxidase-Like Activities of Isoselenazolines. Eur. J. Org. Chem. 2011, 2011, 5485–5497. [Google Scholar] [CrossRef]
- Orian, L.; Toppo, S. Organochalcogen peroxidase mimetics as potential drugs: A long story of a promise still unfulfilled. Free. Radic. Biol. Med. 2014, 66, 65–74. [Google Scholar] [CrossRef]
- Wolters, L.P.; Orian, L. Peroxidase Activity of Organic Selenides: Mechanistic Insights from Quantum Chemistry. Curr. Org. Chem. 2015, 20, 189–197. [Google Scholar] [CrossRef]
- Azad, G.K.; Tomar, R.S. Ebselen, a promising antioxidant drug: Mechanisms of action and targets of biological pathways. Mol. Biol. Rep. 2014, 41, 4865–4879. [Google Scholar] [CrossRef]
- Müller, A.; Cadenas, E.; Graf, P.; Sies, H. A novel biologically active seleno-organic compound-1. Glutathione peroxidase-like activity in vitro and antioxidant capacity of PZ 51 (Ebselen). Biochem. Pharm. 1984, 33, 3235–3239. [Google Scholar] [CrossRef]
- Sies, H. Ebselen, a selenoorganic compound as glutathione peroxidase mimic. Free. Radic. Biol. Med. 1993, 14, 313–323. [Google Scholar] [CrossRef]
- Nogueira, C.W.; Rocha, J.B. Toxicology and pharmacology of selenium: Emphasis on synthetic organoselenium compounds. Arch. Toxicol. 2011, 85, 1313–1359. [Google Scholar] [CrossRef]
- Zhao, R.; Holmgren, A. A Novel Antioxidant Mechanism of Ebselen Involving Ebselen Diselenide, a Substrate of Mammalian Thioredoxin and Thioredoxin Reductase. J. Biol. Chem. 2002, 277, 39456–39462. [Google Scholar] [CrossRef]
- Antony, S.; Bayse, C.A. Modeling the Mechanism of the Glutathione Peroxidase Mimic Ebselen. Inorg. Chem. 2011, 50, 12075–12084. [Google Scholar] [CrossRef]
- Ribaudo, G.; Orian, L. Organodiselenides: Organic Catalysis and Drug Design Learning from Glutathione Peroxidase. Curr. Org. Chem. 2019, 23, 1381–1402. [Google Scholar] [CrossRef]
- Wendel, A.; Fausel, M.; Safayhi, H.; Tiegs, G.; Otter, R. A novel biologically active seleno-organic compound—II. Biochem. Pharmacol. 1984, 33, 3241–3245. [Google Scholar] [CrossRef]
- Kil, J.; Lobarinas, E.; Spankovich, C.; Griffiths, S.K.; Antonelli, P.J.; Lynch, E.D.; Le Prell, C.G. Safety and efficacy of ebselen for the prevention of noise-induced hearing loss: A randomised, double-blind, placebo-controlled, phase 2 trial. Lancet 2017, 390, 969–979. [Google Scholar] [CrossRef]
- Singh, N.; Halliday, A.C.; Thomas, J.M.; Kuznetsova, O.V.; Baldwin, R.; Woon, E.C.Y.; Aley, P.K.; Antoniadou, I.; Sharp, T.; Vasudevan, S.R.; et al. A safe lithium mimetic for bipolar disorder. Nat. Commun. 2013, 4, 1–7. [Google Scholar] [CrossRef]
- Singh, N.; Sharpley, A.L.; Emir, U.E.; Masaki, C.; Herzallah, M.M.; Gluck, M.A.; Sharp, T.; Harmer, C.J.; Vasudevan, S.R.; Cowen, P.J.; et al. Effect of the Putative Lithium Mimetic Ebselen on Brain Myo-Inositol, Sleep, and Emotional Processing in Humans. Neuropsychopharmacol 2015, 41, 1768–1778. [Google Scholar] [CrossRef]
- Jin, Z.; Du, X.; Xu, Y.; Deng, Y.; Liu, M.; Zhao, Y.; Zhang, B.; Li, X.; Zhang, L.; Peng, C.; et al. Structure of Mpro from SARS-CoV-2 and discovery of its inhibitors. Nature 2020, 582, 289–293. [Google Scholar] [CrossRef]
- Sies, H.; Parnham, M.J. Potential therapeutic use of ebselen for COVID-19 and other respiratory viral infections. Free. Radic. Biol. Med. 2020, 156, 107–112. [Google Scholar] [CrossRef]
- Ribaudo, G.; Ongaro, A.; Oselladore, E.; Zagotto, G.; Memo, M.; Gianoncelli, A. A computational approach to drug repurposing against SARS-CoV-2 RNA dependent RNA polymerase (RdRp). J. Biomol. Struct. Dyn. 2020, 1–8. [Google Scholar] [CrossRef]
- Menéndez, C.A.; Byléhn, F.; Perez-Lemus, G.R.; Alvarado, W.; De Pablo, J.J. Molecular characterization of ebselen binding activity to SARS-CoV-2 main protease. Sci. Adv. 2020, 6, eabd0345. [Google Scholar] [CrossRef]
- Sargsyan, K.; Lin, C.-C.; Chen, T.; Grauffel, C.; Chen, Y.-P.; Yang, W.-Z.; Yuan, H.S.; Lim, C. Multi-targeting of functional cysteines in multiple conserved SARS-CoV-2 domains by clinically safe Zn-ejectors. Chem. Sci. 2020, 11, 9904–9909. [Google Scholar] [CrossRef]
- Ma, C.; Hu, Y.; Townsend, J.A.; Lagarias, P.I.; Marty, M.T.; Kolocouris, A.; Wang, J. Ebselen, Disulfiram, Carmofur, PX-12, Tideglusib, and Shikonin Are Nonspecific Promiscuous SARS-CoV-2 Main Protease Inhibitors. Acs Pharm. Transl. Sci. 2020, 3, 1265–1277. [Google Scholar] [CrossRef]
- Thenin-Houssier, S.; De Vera, I.M.S.; Pedro-Rosa, L.; Brady, A.; Richard, A.; Konnick, B.; Opp, S.; Buffone, C.; Fuhrmann, J.; Kota, S.; et al. Ebselen, a Small-Molecule Capsid Inhibitor of HIV-1 Replication. Antimicrob. Agents Chemother. 2016, 60, 2195–2208. [Google Scholar] [CrossRef]
- Mukherjee, S.; Weiner, W.S.; Schroeder, C.E.; Simpson, D.S.; Hanson, A.M.; Sweeney, N.L.; Marvin, R.K.; Ndjomou, J.; Kolli, R.; Isailovic, D.; et al. Ebselen Inhibits Hepatitis C Virus NS3 Helicase Binding to Nucleic Acid and Prevents Viral Replication. ACS Chem. Biol. 2014, 9, 2393–2403. [Google Scholar] [CrossRef]
- Björgvinsson, M.; Roesky, H.W. The structures of compounds containing selenium-nitrogen and tellurium-nitrogen bonds. Polyhedron 1991, 10, 2353–2370. [Google Scholar] [CrossRef]
- Mahmudov, K.T.; Kopylovich, M.N.; Da Silva, M.F.C.G.; Pombeiro, A.J.L. Chalcogen bonding in synthesis, catalysis and design of materials. Dalton Trans. 2017, 46, 10121–10138. [Google Scholar] [CrossRef]
- Rendeková, J.; Vlasáková, D.; Arsenyan, P.; Vasiljeva, J.; Nasim, M.J.; Witek, K.; Domínguez-Álvarez, E.; Żesławska, E.; Mániková, D.; Tejchman, W.; et al. The Selenium-Nitrogen Bond as Basis for Reactive Selenium Species with Pronounced Antimicrobial Activity. Curr. Org. Synth. 2018, 14, 1082–1090. [Google Scholar] [CrossRef]
- Orian, L.; Mauri, G.; Roveri, A.; Toppo, S.; Benazzi, L.; Bosello-Travain, V.; De Palma, A.; Maiorino, M.; Miotto, G.; Zaccarin, M.; et al. Selenocysteine oxidation in glutathione peroxidase catalysis: An MS-supported quantum mechanics study. Free. Radic. Biol. Med. 2015, 87, 1–14. [Google Scholar] [CrossRef]
- Salmeen, A.; Andersen, J.N.; Myers, M.P.; Meng, T.-C.; Hinks, J.A.; Tonks, N.K.; Barford, D. Redox regulation of protein tyrosine phosphatase 1B involves a sulphenyl-amide intermediate. Nature 2003, 423, 769–773. [Google Scholar] [CrossRef]
- Montfort, R.L.M.; Van Congreve, M.; Tisi, D.; Carr, R.; Jhoti, H. Reduction of the sulphenyl-amide bond. Nature 2003, 423, 773–777. [Google Scholar] [CrossRef]
- Poole, L.B.; Nelson, K.J. Discovering mechanisms of signaling-mediated cysteine oxidation. Curr. Opin. Chem. Biol. 2008, 12, 18–24. [Google Scholar] [CrossRef]
- Lee, J.-W.; Soonsanga, S.; Helmann, J.D. A complex thiolate switch regulates the Bacillus subtilis organic peroxide sensor OhrR. Proc. Natl. Acad. Sci. USA 2007, 104, 8743–8748. [Google Scholar] [CrossRef]
- Yang, J.; Groen, A.; Lemeer, S.; Jans, A.; Slijper, M.; Roe, S.M.; Hertog, J.D.; Barford, D. Reversible Oxidation of the Membrane Distal Domain of Receptor PTPα Is Mediated by a Cyclic Sulfenamide†. Biochemistry 2007, 46, 709–719. [Google Scholar] [CrossRef]
- Nogara, P.A.; Orian, L.; Rocha, J.B. The Se S/N interactions as a possible mechanism of δ-aminolevulinic acid dehydratase enzyme inhibition by organoselenium compounds: A computational study. Comput. Toxicol. 2020, 15, 100127. [Google Scholar] [CrossRef]
- Cozzolino, A.F.; Vargas-Baca, I. The supramolecular chemistry of 1,2,5-chalcogenadiazoles. J. Organomet. Chem. 2007, 692, 2654–2657. [Google Scholar] [CrossRef]
- Cozzolino, A.F.; Yang, Q.; Vargas-Baca, I. Engineering Second-Order Nonlinear Optical Activity by Means of a Noncentrosymmetric Distortion of the [Te−N]2 Supramolecular Synthon. Cryst. Growth Des. 2010, 10, 4959–4964. [Google Scholar] [CrossRef]
- Suturina, E.A.; Semenov, N.A.; Lonchakov, A.V.; Bagryanskaya, I.Y.; Gatilov, Y.V.; Irtegova, I.G.; Vasilieva, N.V.; Lork, E.; Mews, R.; Gritsan, N.P.; et al. Interaction of 1,2,5-Chalcogenadiazole Derivatives with Thiophenolate: Hypercoordination with Formation of Interchalcogen Bond versus Reduction to Radical Anion. J. Phys. Chem. A 2011, 115, 4851–4860. [Google Scholar] [CrossRef]
- Tsuzuki, S.; Sato, N. Origin of Attraction in Chalgogen–Nitrogen Interaction of 1,2,5-Chalcogenadiazole Dimers. J. Phys. Chem. B 2013, 117, 6849–6855. [Google Scholar] [CrossRef]
- Bortoli, M.; Ahmad, S.M.; Hamlin, T.A.; Bickelhaupt, F.M.; Orian, L. Nature and strength of chalcogen–π bonds. Phys. Chem. Chem. Phys. 2018, 20, 27592–27599. [Google Scholar] [CrossRef]
- Bickelhaupt, F.M.; Baerends, E.J. Kohn-Sham Density Functional Theory: Predicting and Understanding Chemistry. In Reviews in Computational Chemistry; Lipkovitz, K.B., Boyd, D.B., Eds.; Wiley-VCH: New York, NY, USA, 2000; Volume 15, pp. 1–86. ISBN 9780470125922. [Google Scholar]
- Bickelhaupt, F.M.; Houk, K.N. Analyzing Reaction Rates with the Distortion/Interaction-Activation Strain Model. Angew. Chem. Int. Ed. 2017, 56, 10070–10086. [Google Scholar] [CrossRef]
- Te Velde, G.; Bickelhaupt, F.M.; Baerends, E.J.; Fonseca Guerra, C.; Van Gisbergen, S.J.A.; Snijders, J.G.; Ziegler, T. Chemistry with ADF. J. Comput. Chem. 2001, 22, 931–967. [Google Scholar] [CrossRef]
- ADF, version 103; SCM, Theoretical Chemistry; Vrije Universiteit: Amsterdam, The Netherlands, 2019.
- Becke, A.D. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A 1988, 38, 3098–3100. [Google Scholar] [CrossRef]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colic-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef]
- Johnson, B.G.; Gill, P.M.W.; Pople, J.A. The performance of a family of density functional methods. J. Chem. Phys. 1993, 98, 5612–5626. [Google Scholar] [CrossRef]
- Russo, T.V.; Martin, R.L.; Hay, P.J. Density functional calculations on first-row transition metals. J. Chem. Phys. 1994, 101, 7729–7737. [Google Scholar] [CrossRef]
- Van Lenthe, E.; Baerends, E.J.; Snijders, J.G. Relativistic regular two-component Hamiltonians. J. Chem. Phys. 1993, 99, 4597–4610. [Google Scholar] [CrossRef]
- Van Lenthe, E.; Baerends, E.J.; Snijders, J.G. Relativistic total energy using regular approximations. J. Chem. Phys. 1994, 101, 9783–9792. [Google Scholar] [CrossRef]
- Van Lenthe, E.; Snijders, J.G.; Baerends, E.J. The zero-order regular approximation for relativistic effects: The effect of spin–orbit coupling in closed shell molecules. J. Chem. Phys. 1996, 105, 6505–6516. [Google Scholar] [CrossRef]
- Van Lenthe, E.; Ehlers, A.; Baerends, E.-J. Geometry optimizations in the zero order regular approximation for relativistic effects. J. Chem. Phys. 1999, 110, 8943–8953. [Google Scholar] [CrossRef]
- Grimme, S.; Ehrlich, S.; Goerigk, L. Effect of the damping function in dispersion corrected density functional theory. J. Comput. Chem. 2011, 32, 1456–1465. [Google Scholar] [CrossRef]
- Zaccaria, F.; Wolters, L.P.; Guerra, C.F.; Orian, L. Insights on selenium and tellurium diaryldichalcogenides: A benchmark DFT study. J. Comput. Chem. 2016, 37, 1672–1680. [Google Scholar] [CrossRef]
- Bortoli, M.; Bruschi, M.; Swart, M.; Orian, L. Sequential oxidations of phenylchalcogenides by H2O2: Insights into the redox behavior of selenium via DFT analysis. New J. Chem. 2020, 44, 6724–6731. [Google Scholar] [CrossRef]
- Bortoli, M.; Zaccaria, F.; Tiezza, M.D.; Bruschi, M.; Guerra, C.F.; Bickelhaupt, F.M.; Orian, L. Oxidation of organic diselenides and ditellurides by H2O2 for bioinspired catalyst design. Phys. Chem. Chem. Phys. 2018, 20, 20874–20885. [Google Scholar] [CrossRef]
- Zhao, Y.; Truhlar, D.G. A new local density functional for main-group thermochemistry, transition metal bonding, thermochemical kinetics, and noncovalent interactions. J. Chem. Phys. 2006, 125, 194101. [Google Scholar] [CrossRef]
- Zhao, Y.; Truhlar, D.G. The M06 suite of density functionals for main group thermochemistry, thermochemical kinetics, noncovalent interactions, excited states, and transition elements: Two new functionals and systematic testing of four M06-class functionals and 12 other functionals. Theor. Chem. Accounts 2008, 120, 215–241. [Google Scholar] [CrossRef]
- Zhao, Y.; Truhlar, D.G. How Well Can New-Generation Density Functionals Describe the Energetics of Bond-Dissociation Reactions Producing Radicals? J. Phys. Chem. A 2008, 112, 1095–1099. [Google Scholar] [CrossRef]
- Liakos, D.G.; Guo, Y.; Neese, F. Comprehensive Benchmark Results for the Domain Based Local Pair Natural Orbital Coupled Cluster Method (DLPNO-CCSD(T)) for Closed- and Open-Shell Systems. J. Phys. Chem. A 2019, 124, 90–100. [Google Scholar] [CrossRef]
- Neese, F. The ORCA Program System: Software Update—Version 4.0. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2018, 8, e1327. [Google Scholar] [CrossRef]
- Neese, F. The ORCA program system. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2012, 2, 73–78. [Google Scholar] [CrossRef]
- Neese, F.; Wolf, A.; Fleig, T.; Reiher, M.; Hess, B.A. Calculation of electric-field gradients based on higher-order generalized Douglas-Kroll transformations. J. Chem. Phys. 2005, 122, 204107. [Google Scholar] [CrossRef]
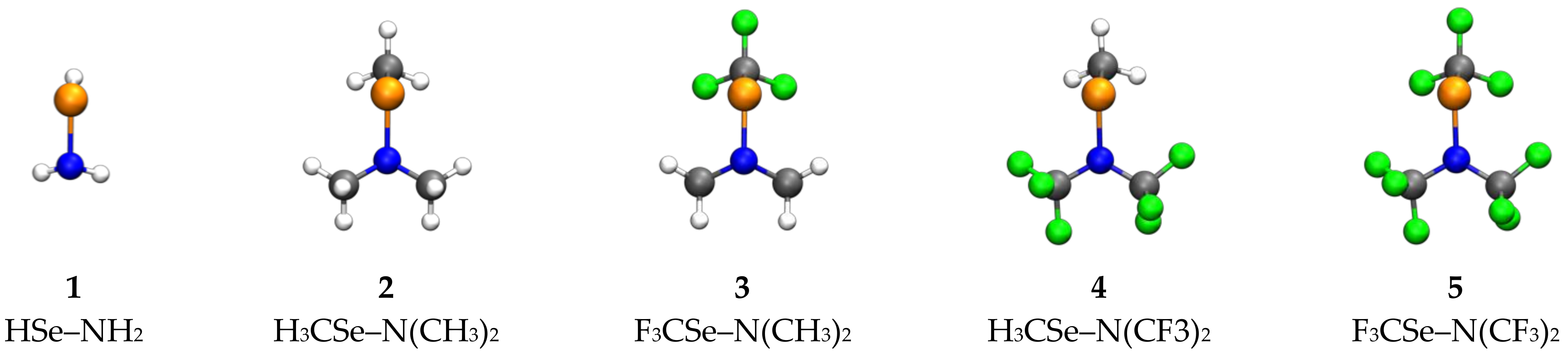


| X–N Length (Å) | |||
|---|---|---|---|
| R, R’ | S | Se | Te |
| 1 | 1.73 | 1.90 | 2.08 |
| 2 | 1.72 | 1.92 | 2.10 |
| 3 | 1.68 | 1.86 | 2.06 |
| 4 | 1.74 | 1.92 | 2.11 |
| 5 | 1.71 | 1.89 | 2.09 |
| Mulliken Atomic Charges | |||||||
|---|---|---|---|---|---|---|---|
| BLYP-QZ | M06-2X-QZ | ||||||
| R, R’ | S | Se | Te | S | Se | Te | |
| 1 | X | 0.5455 | 0.4846 | 0.7099 | 0.1871 | 0.1981 | 0.5581 |
| N | −0.7209 | −0.7470 | −0.8062 | −0.4879 | −0.4552 | −0.5809 | |
| 2 | X | 0.2647 | 0.3141 | 0.3630 | 0.2858 | 0.4658 | 0.5621 |
| N | −0.2197 | −0.2369 | −0.3436 | −0.3817 | −0.4306 | −0.5780 | |
| 3 | X | 0.4265 | 0.4623 | 0.4964 | 0.3158 | 0.3522 | 0.6443 |
| N | −0.1447 | −0.1860 | −0.2778 | −0.3935 | −0.4181 | −0.6699 | |
| 4 | X | 0.3173 | 0.4696 | 0.5575 | 0.3068 | 0.5820 | 0.7278 |
| N | 0.2421 | 0.1109 | −0.0094 | −0.1698 | −0.3122 | −0.4935 | |
| 5 | X | 0.4773 | 0.5797 | 0.6748 | 0.3078 | 0.4650 | 0.7760 |
| N | 0.2285 | 0.1411 | −0.0116 | −0.1947 | −0.2513 | −0.4801 | |
| RX–NR’2 | ∆Eint (kcal mol−1) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| S | Se | Te | |||||||||
| Struct | R | R’ | BLYP | M06 | CC | BLYP | M06 | CC | BLYP | M06 | CC |
| 1 | H | H | −71.0 | −73.0 | −67.6 | −62.3 | −59.8 | −62.5 | −59.7 | −53.7 | −61.4 |
| 2 | CH3 | CH3 | −59.0 | −65.5 | −62.1 | −50.9 | −50.2 | −56.5 | −47.5 | −42.5 | −54.0 |
| 3 | CF3 | CH3 | −64.9 | −71.9 | −68.2 | −56.3 | −56.2 | −62.7 | −49.8 | −45.1 | −56.6 |
| 4 | CH3 | CF3 | −68.8 | −77.6 | −74.3 | −64.0 | −66.9 | −72.2 | −63.8 | −63.9 | −73.6 |
| 5 | CF3 | CF3 | −67.2 | −75.4 | −72.5 | −61.7 | −63.5 | −69.8 | −60.8 | −59.5 | −70.0 |
| S | Se | Te | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| ∆Velstat | ∆EPauli | ∆EOI | ∆Edisp | ∆Velstat | ∆EPauli | ∆EOI | ∆Edisp | ∆Velstat | ∆EPauli | ∆EOI | ∆Edisp | |
| 1 | −166.5 | 359.2 | −261.6 | −2.1 | −132.9 | 265.5 | −192.6 | −2.3 | −108.5 | 193.8 | −142.4 | −2.7 |
| 2 | −176.2 | 355.7 | −232.4 | −6.2 | −130.8 | 235.9 | −150.1 | −5.9 | −124.9 | 213.1 | −129.2 | −6.5 |
| 3 | −197.2 | 397.0 | −258.5 | −6.3 | −155.8 | 285.8 | −179.6 | −6.6 | −140.1 | 238.4 | −141.5 | −6.7 |
| 4 | −164.5 | 357.8 | −256.1 | −6.1 | −122.1 | 245.5 | −181.0 | −6.4 | −113.1 | 220.3 | −164.0 | −7.0 |
| 5 | −166.1 | 368.0 | −262.7 | −6.4 | −129.5 | 266.8 | −192.3 | −6.7 | −115.9 | 229.6 | −167.4 | −7.2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bortoli, M.; Madabeni, A.; Nogara, P.A.; Omage, F.B.; Ribaudo, G.; Zeppilli, D.; Rocha, J.B.T.; Orian, L. Chalcogen-Nitrogen Bond: Insights into a Key Chemical Motif. Catalysts 2021, 11, 114. https://doi.org/10.3390/catal11010114
Bortoli M, Madabeni A, Nogara PA, Omage FB, Ribaudo G, Zeppilli D, Rocha JBT, Orian L. Chalcogen-Nitrogen Bond: Insights into a Key Chemical Motif. Catalysts. 2021; 11(1):114. https://doi.org/10.3390/catal11010114
Chicago/Turabian StyleBortoli, Marco, Andrea Madabeni, Pablo Andrei Nogara, Folorunsho B. Omage, Giovanni Ribaudo, Davide Zeppilli, Joao B. T. Rocha, and Laura Orian. 2021. "Chalcogen-Nitrogen Bond: Insights into a Key Chemical Motif" Catalysts 11, no. 1: 114. https://doi.org/10.3390/catal11010114
APA StyleBortoli, M., Madabeni, A., Nogara, P. A., Omage, F. B., Ribaudo, G., Zeppilli, D., Rocha, J. B. T., & Orian, L. (2021). Chalcogen-Nitrogen Bond: Insights into a Key Chemical Motif. Catalysts, 11(1), 114. https://doi.org/10.3390/catal11010114