Choices in the 11–20 Game: The Role of Risk Aversion
Abstract
1. Introduction
2. Related Literature
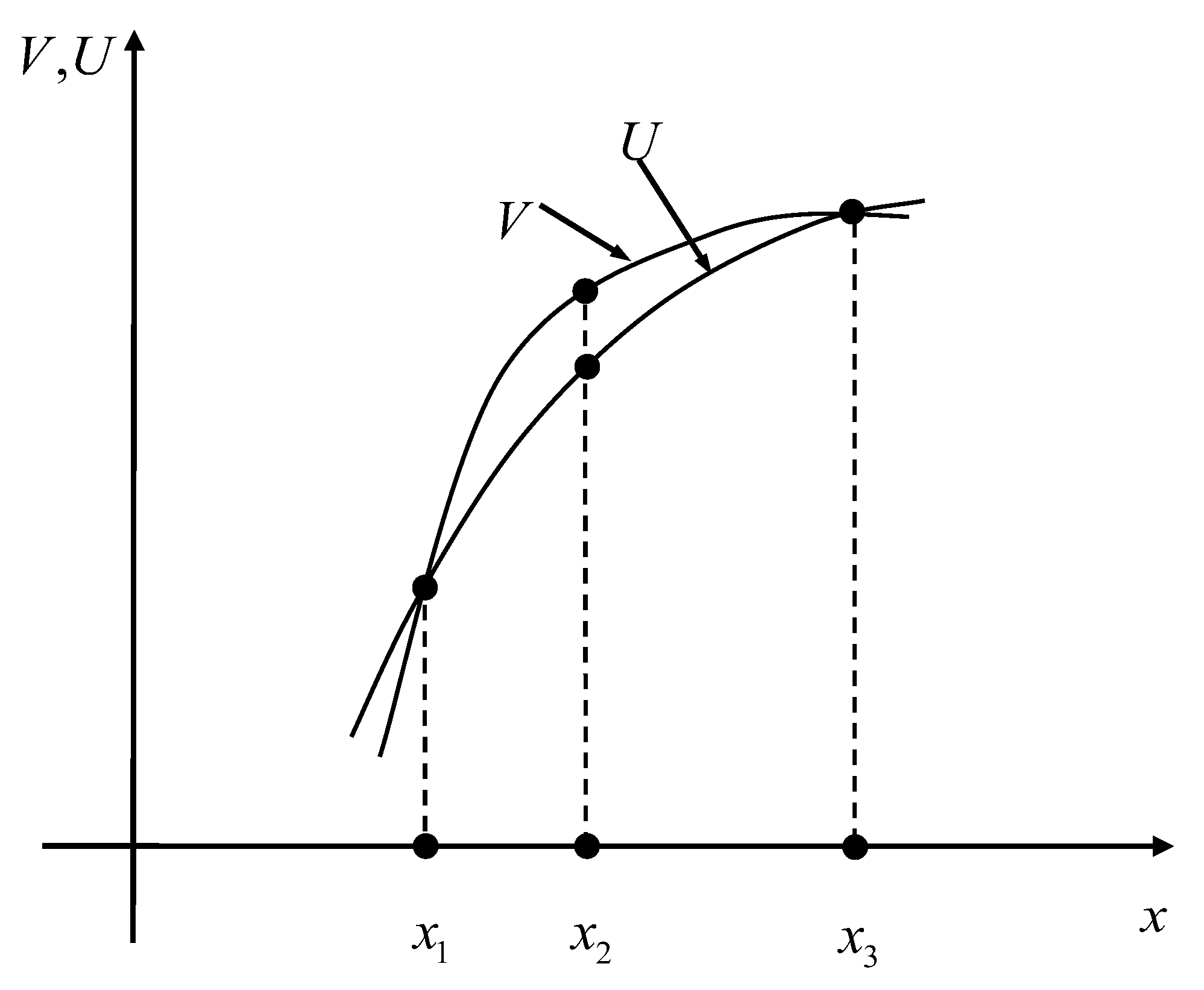
3. Theoretical Analysis
4. Experimental Design
5. Experimental Results
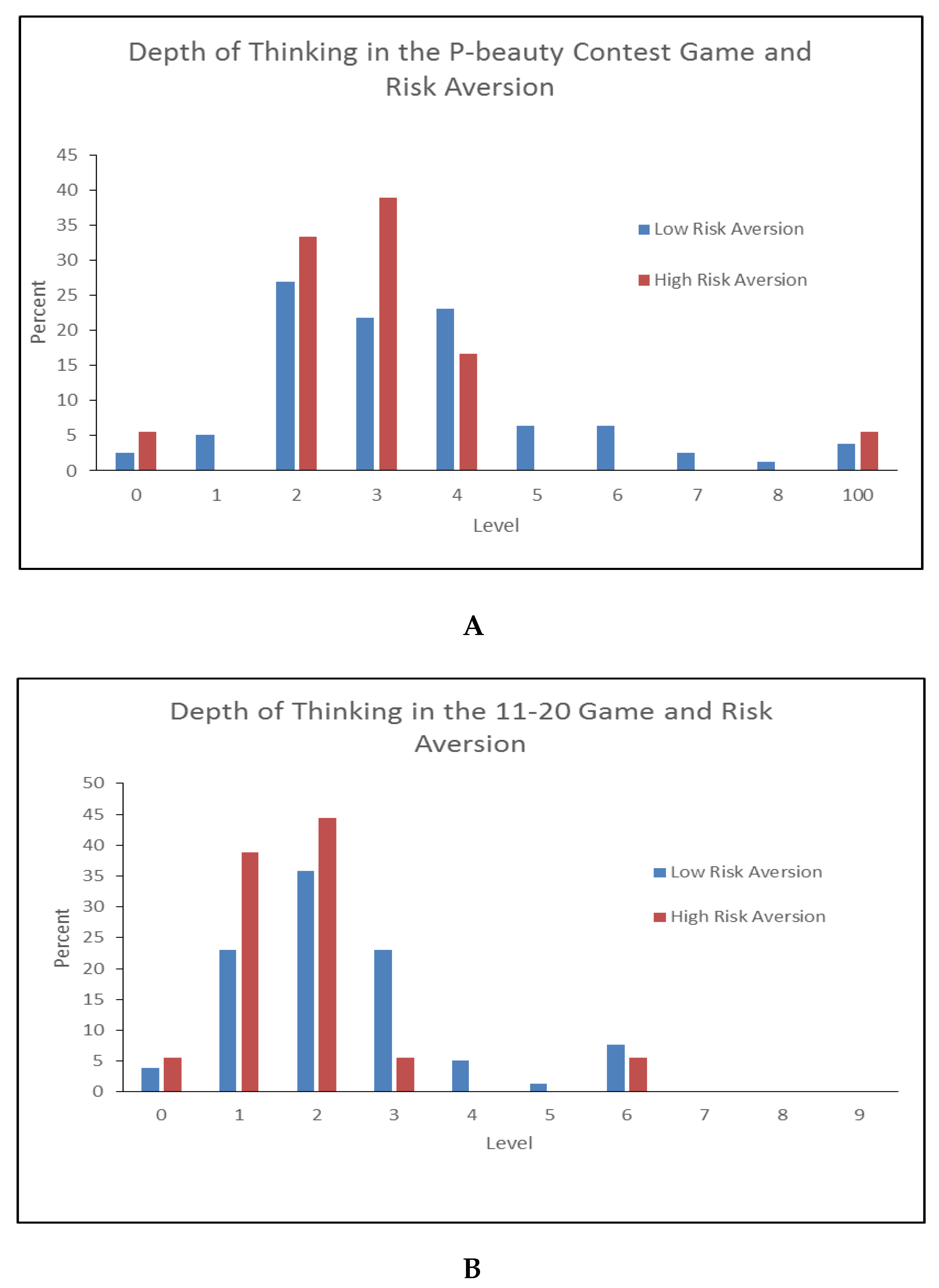
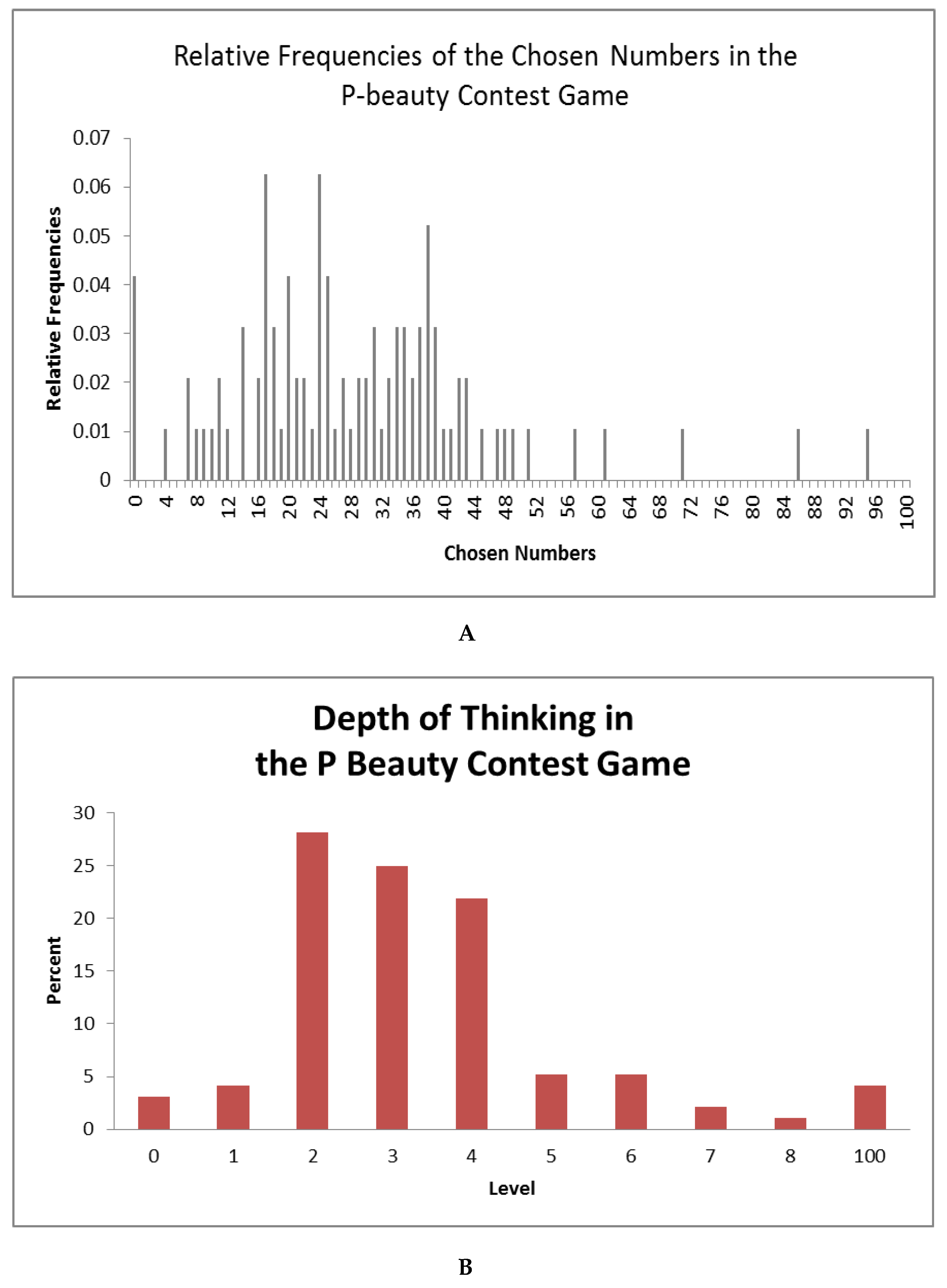
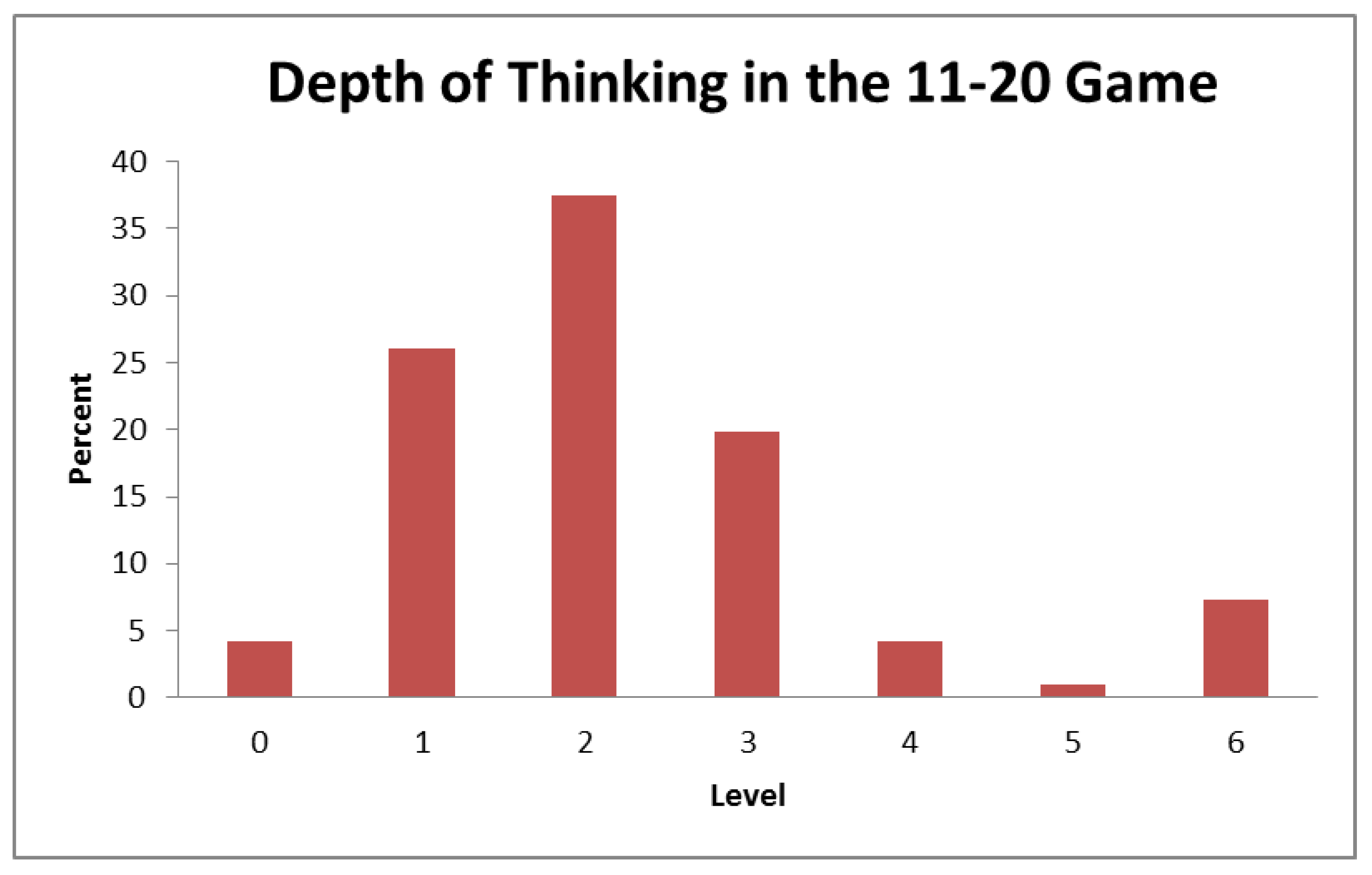
5.1. Measurement of the Depth of Thinking
5.2. Risk aversion and choice in the 11–20 Game
5.3. The relationship between choices in the P beauty contest game and the 11–20 game
5.4. Comparison of results in P Beauty to Nagel (1995)
5.5. Comparison of results in 11–20 to Arad and Rubinstein (2012)
6. Discussion
Appendix A
| Choice | Gamble A | Gamble B | Expected Value of Gamble A | Expected Value of Gamble B |
|---|---|---|---|---|
| 1 | 1/10 , RMB 20 ; 9/10, RMB 16 | 1/10 , RMB 38.5 ; 9/10, RMB 1 | 16.4 | 4.75 |
| 2 | 2/10 , RMB20 ; 8/10, RMB16 | 2/10 , RMB 38.5 ; 8/10, RMB 1 | 16.8 | 8.5 |
| 3 | 3/10 , RMB20 ; 7/10, RMB16 | 3/10 , RMB 38.5 ; 7/10, RMB 1 | 17.2 | 12.25 |
| 4 | 4/10 , RMB20 ; 6/10, RMB16 | 4/10 , RMB 38.5 ; 6/10, RMB 1 | 17.6 | 16 |
| 5 | 5/10 , RMB20 ; 5/10, RMB16 | 5/10 , RMB 38.5 ; 5/10, RMB 1 | 18 | 19.75 |
| 6 | 6/10 , RMB20 ; 4/10, RMB16 | 6/10 , RMB 38.5 ; 4/10, RMB 1 | 18.4 | 23.5 |
| 7 | 7/10 , RMB20 ; 3/10, RMB16 | 7/10 , RMB 38.5 ; 3/10, RMB 1 | 18.8 | 27.25 |
| 8 | 8/10 , RMB20 ; 2/10, RMB16 | 8/10 , RMB 38.5 ; 2/10, RMB 1 | 19.2 | 31 |
| 9 | 9/10 , RMB20 ; 1/10, RMB16 | 9/10 , RMB 38.5 ; 1/10, RMB 1 | 19.6 | 34.75 |
| 10 | 10/10 , RMB20 ; 0/10, RMB16 | 10/10 , RMB 38.5 ; 0/10, RMB 1 | 20 | 38.5 |
| Choice in which Subject Switched to Gamble B | Proportion of Choices |
|---|---|
| 1 | 0 |
| 2 | 0 |
| 3 | 0.07 |
| 4 | 0.15 |
| 5 | 0.25 |
| 6 | 0.16 |
| 7 | 0.26 |
| 8 | 0.08 |
| 9 | 0.04 |
| 10 | 0 |
| Dependent Variable: Depth of Thinking in P Beauty Contest game | |
|---|---|
| Depth of Thinking in the 11–20 Game | −0.02 |
| (0.11) | |
| Constant | 3.31 *** |
| (0.32) | |
| R-squared | 0.001 |
| Number of Observations | 92 |
Appendix B
Appendix C
References
- Keynes, J.M. The General Theory of Interest, Employment and Money; Palgrave Macmillan: London, UK, 1936. [Google Scholar]
- Nagel, R. Unraveling in guessing games: An experimental study. Am. Econ. Rev. 1995, 1313–1326. [Google Scholar]
- Arad, A.; Rubinstein, A. The 11–20 money request game: A level-k reasoning study. Am. Econ. Rev. 2012, 102, 3561–3573. [Google Scholar] [CrossRef]
- Holt, C.A.; Laury, S.K. Risk aversion and incentive effects. Am. Econ. Rev. 2002, 92, 1644–1655. [Google Scholar] [CrossRef]
- Goeree, J.K.; Louis, P.; Zhang, J. Noisy introspection in the “11–20” game. Econ. J. 2018, 128, 1509–1530. [Google Scholar] [CrossRef]
- Goeree, J.K.; Holt, C.A. A model of noisy introspection. Games Econ. Behav. 2004, 46, 365–382. [Google Scholar] [CrossRef]
- Alaoui, L.; Penta, A. Endogenous depth of reasoning. Rev. Econ. Stud. 2015, 83, 1297–1333. [Google Scholar] [CrossRef]
- Georganas, S.; Healy, P.J.; Weber, R.A. On the persistence of strategic sophistication. J. Econ. Theory 2015, 159, 369–400. [Google Scholar] [CrossRef]
- Costa-Gomes, M.A.; Crawford, V.P. Cognition and behavior in two-person guessing games: An experimental study. Am. Econ. Rev. 2006, 96, 1737–1768. [Google Scholar] [CrossRef]
| 1 | Alaoui and Penta (2015) [7] present a model in which the player’s depth of thinking is endogenously determined. In their approach, individuals act as if they follow a cost-benefit analysis. Our approach is related to their approach in the sense that players face a trade-off over whether to forego a higher fixed payoff (cost) for the possibility of obtaining the reward (benefit). |
| 2 | Unfortunately, this result cannot be generalized to the general case that allows arbitrary utility functions or allows arbitrary distribution of the risk aversion level. For example, for the CARA utility, it can be verified that the equilibrium thresholds may not be monotonic. |
| 3 | In the literature, an alternative method for classifying the depth of thinking is to use 50 as a reference point for level 0. We do not use 50 as the reference point because doing so would require dropping data points above 50. Nevertheless, our result remains qualitatively the same, and significant, if we use 50 as the reference point. |
| 4 | Highly risk-averse subjects are defined as those who switch from gamble A to gamble B in choice 8 or later (i.e., the subject has chosen 7 safe choices (gamble A)). Our design very closely follows that of Holt and Laury (2002) [4]. This group of subjects is also described as very risk averse by Holt and Laury (2002) [4]. Table A1 (online appendix) reports the expected value of the gambles, assuming that the subjects take the objective probability as given. We can observe that, if a subject is risk neutral, then he should switch from gamble A to gamble B starting with choice 5. Thus, an individual who switched to gamble B at choice 8 or later must be highly risk averse. Holt and Laury (2002) [3] estimate the coefficient of relative risk aversion of their subjects using the utility function u(x) = x1 − r/(1−r) for x > 0. It is found that the coefficient of relative risk aversion increases with the number of safe choices. For example, when the subject switched at choice 8, the implied range of relative risk aversion is 0.68 < r < 0.97; thus, they classify the subject as “very risk averse”. |
| 5 | The risk premium of subjects who switched to gamble B in choice n is equal to [(the expected value of gamble A in choice n—the expected value of gamble B in choice n) + (the expected value of gamble A in choice (n−1)—the expected value of gamble B in choice (n−1))]/2. |
| Number | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 |
|---|---|---|---|---|---|---|---|---|---|---|
| Equilibrium when players are risk neutral (%) | 0 | 0 | 0 | 0 | 25 | 25 | 20 | 15 | 10 | 5 |
| Equilibrium when players have utility u(x) = x1−r/(1 − r) with r = 1.37 (%) | 0 | 0 | 0 | 0 | 0 | 22.2 | 30.5 | 23.3 | 16 | 8 |
| Equilibrium when players have utility u(x)=1−eαx with α = 0.15 (%) | 0 | 0 | 0 | 0 | 0 | 0 | 20 | 38.1 | 27.2 | 14.7 |
| Experimental Results by Arad and Rubinstein (2012) (%) | 4 | 0 | 3 | 6 | 1 | 6 | 32 | 30 | 12 | 6 |
| Our Experimental Results (%) | 0 | 0 | 0 | 7.3 | 1 | 4.2 | 19.8 | 37.5 | 26 | 4.2 |
| Dependent Variable: Low Depth of Thinking | Dependent Variable: Depth of Thinking | |||
|---|---|---|---|---|
| (1) P Beauty Contest Game | (2)11–20 Game | (3)P Beauty Contest Game | (4)11–20 Game | |
| Risk Premium5 | 0.00004 | −1.99e−06 *** | ||
| (0.00005) | (7.27e−07) | |||
| High Risk Aversion | 0.04 | 0.27 *** | ||
| (0.13) | (0.09) | |||
| Constant | 6.34 *** | 2.34 *** | ||
| (2.10) | (0.16) | |||
| R-squared | 0.04 | 0.02 | ||
| Pseudo R-squared | 0.001 | 0.03 | ||
| Number of Observations | 96 | 96 | 89 | 89 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, K.K.; Rong, K. Choices in the 11–20 Game: The Role of Risk Aversion. Games 2018, 9, 53. https://doi.org/10.3390/g9030053
Li KK, Rong K. Choices in the 11–20 Game: The Role of Risk Aversion. Games. 2018; 9(3):53. https://doi.org/10.3390/g9030053
Chicago/Turabian StyleLi, King King, and Kang Rong. 2018. "Choices in the 11–20 Game: The Role of Risk Aversion" Games 9, no. 3: 53. https://doi.org/10.3390/g9030053
APA StyleLi, K. K., & Rong, K. (2018). Choices in the 11–20 Game: The Role of Risk Aversion. Games, 9(3), 53. https://doi.org/10.3390/g9030053




