Dynamic Contributions to a Public Project: The Impact of Rising Marginal Benefit and Completion Benefits
Abstract
1. Introduction
2. Experiment
2.1. Theoretical Analysis
2.2. Parameterization of Experiments
2.3. Experimental Design
3. Results
3.1. Group Level Results
3.2. Individual Level Results
3.3. Evidence of Confusion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
Experiment Instructions: RMB CB Treatment




References
- Croson, R.T.A. Theories of Commitment, Altruism and Reciprocity: Evidence from Linear Public Goods Games. Econ. Inq. 2007, 45, 199–216. [Google Scholar] [CrossRef]
- Schelling, T.C. The Strategy of Conflict; Harvard University Press: Cambridge, MA, USA, 1960. [Google Scholar]
- Ledyard, J.O. Public Goods: A Survey of Experimental Research. In Handbook of Experimental Economics; Kagel, J., Roth, A., Eds.; Princeton University Press: Princeton, NJ, USA, 1995. [Google Scholar]
- Marx, L.M.; Matthews, S.A. Dynamic Voluntary Contribution to a Public Project. Rev. Econ. Stud. 2000, 67, 327–358. [Google Scholar]
- Compte, O.; Jehiel, P. Gradualism in Bargaining and Contribution Games. Rev. Econ. Stud. 2004, 71, 975–1000. [Google Scholar] [CrossRef]
- Duffy, J.; Ochs, J.; Vesterlund, L. Giving Little by Little: Dynamic Voluntary Contribution Games. J. Public Econ. 2007, 91, 1708–1730. [Google Scholar] [CrossRef]
- Choi, S.; Gale, D.; Kariv, S. Sequential Equilibrium in Monotone Games: Theory-Based Analysis of Experimental Data. J. Econ. Theory 2008, 143, 302–330. [Google Scholar] [CrossRef]
- Battaglini, M.; Nunnari, S.; Palfrey, T.R. The Dynamic Free Rider Problem: An Experimental Study. Am. Econ. J. Microecon. 2016, 8, 268–308. [Google Scholar] [CrossRef]
- Gachter, S.; Mengel, F.; Tsakas, E.; Vostroknutov, A. Growth and inequality in public good provision. J. Public Econ. 2017, 150, 1–13. [Google Scholar] [CrossRef]
- Andreoni, J. Giving with Impure Altruism: Applications to Charity and Ricardian Equivalence. J. Polit. Econ. 1989, 97, 1447–1458. [Google Scholar] [CrossRef]
- Vesterlund, L. The Informational Value of Sequential Fundraising. J. Public Econ. 2003, 87, 627–657. [Google Scholar] [CrossRef]
- Palfrey, T.R.; Rosenthal, H. Participation and the provision of discrete public goods: A strategic analysis. J. Public Econ. 1984, 24, 171–193. [Google Scholar] [CrossRef]
- Marks, M.B.; Croson, R. Alternative rebate rules in the provision of a threshold public good: An experimental investigation. J. Public Econ. 1998, 67, 195–220. [Google Scholar] [CrossRef]
- Croson, R.T.A.; Marks, M.B. Step returns in threshold public goods: A meta- and experimental analysis. Exp. Econ. 2000, 2, 239–259. [Google Scholar] [CrossRef]
- Fischbacher, U. z-Tree: Zurich Toolbox for Ready-Made Economic Experiments. Exp. Econ. 2007, 10, 171–178. [Google Scholar] [CrossRef]
- Andreoni, J. Leadership Giving in Charitable Fundraising. J. Public Econ. Theory 2006, 8, 1–22. [Google Scholar] [CrossRef]
- Bracha, A.; Menietti, M.; Vesterlund, L. Seeds to Succeed? Sequential giving to public projects. J. Public Econ. 2011, 95, 416–427. [Google Scholar] [CrossRef]
- Rondeau, D.; List, J.A. Matching and Challenge Gifts to Charity: Evidence from Laboratory and Natural Field Experiments. Exp. Econ. 2008, 11, 253–267. [Google Scholar] [CrossRef]
- Tavoni, A.; Dannenberg, A.; Kallis, G.; Löschel, A. Inequality, communication, and the avoidance of disastrous climate change in a public goods game. Proc. Natl. Acad. Sci. USA 2011, 108, 11825–11829. [Google Scholar] [CrossRef] [PubMed]
- Isaac, R.M.; Walker, J.M. Group Size Effects in Public Goods Provision: The Voluntary Contribution Mechanism. Q. J. Econ. 1988, 53, 179–200. [Google Scholar] [CrossRef]
| 1 | Strong preferences for reciprocity have been shown to drive behavior in VCM experiments, see Croson (2007) [1]. |
| 2 | Shelling (1960) [2] originated this theoretical idea. |
| 3 | See Ledyard (1995) [3] for a review of static public goods experiments. |
| 4 | The model of Compte and Jehiel (2004) [5] provides similar conclusions about how contributions can be enhanced in dynamic funding environments. In the place of a completion benefit, Compte and Jehiel (2004) rely upon the existence of outside options and the ability of subjects to terminate the project to facilitate cooperative equilibria. |
| 5 | |
| 6 | A rising marginal benefit of contribution as a project nears completion would also be consistent with the warm giving hypothesis of Andreoni (1989) [10] because later contributions could be viewed by contributors as more likely to influence whether others will be able to enjoy a completed project. |
| 7 | Fundraisers often provide explicit completion benefits to contributors in the form of plaques or bricks placed in completed building projects that display the names of contributors. |
| 8 | If , , and , the CMB CB treatment becomes a traditional provision point VCM with no rebate. See for example, Marks and Croson (1998) [13]. Provision point VCMs can have funding equilibria in one-round games. |
| 9 | Zero-provision of the group project would also be a possible equilibrium of the multi-round game. |
| 10 | For this to be true, it would be sufficient that . |
| 11 | Note that λ2 is parametrized to be the mean of λ1, λ2, and λ3. This allows the CMB and RMB treatments to be comparable, as the return to a completed project is identical between these treatments. |
| 12 | Duffy et al. (2007) [6] contains treatments analogous to our CMB and CMB CB treatments. Using the symbols of our model, their experiments are parameterized as follows: n = 3, T = 4, Zi = 6, Pi = $0.10, , λ2 = $0.05, and B = $0.10. |
| 13 | Our design is structured such that the th token does not pay the individual marginal benefit, in the CMB CB treatment or λ3 in the RMB CB treatment, and only pays the completion benefit. Alternatively, as is done in Duffy et al. (2007) [6], the payoff function could have been structured such that the th token did pay the individual marginal benefit with B = $0.30 − λ2 in the CMB CB treatment, and B = $0.30 − λ3 in the RMB CB treatment. The designs are mathematically identical, and the predictions of our model would not change. |
| 14 | Subjects include: economics, business, history, geography, and earth sciences. |
| 15 | Computer screenshots and software are available upon request from the authors. |
| 16 | Instructions for the RMB CB treatment are included in the appendix, other instructions are similar. Copies of the instructions for all treatments are available upon request from the authors. |
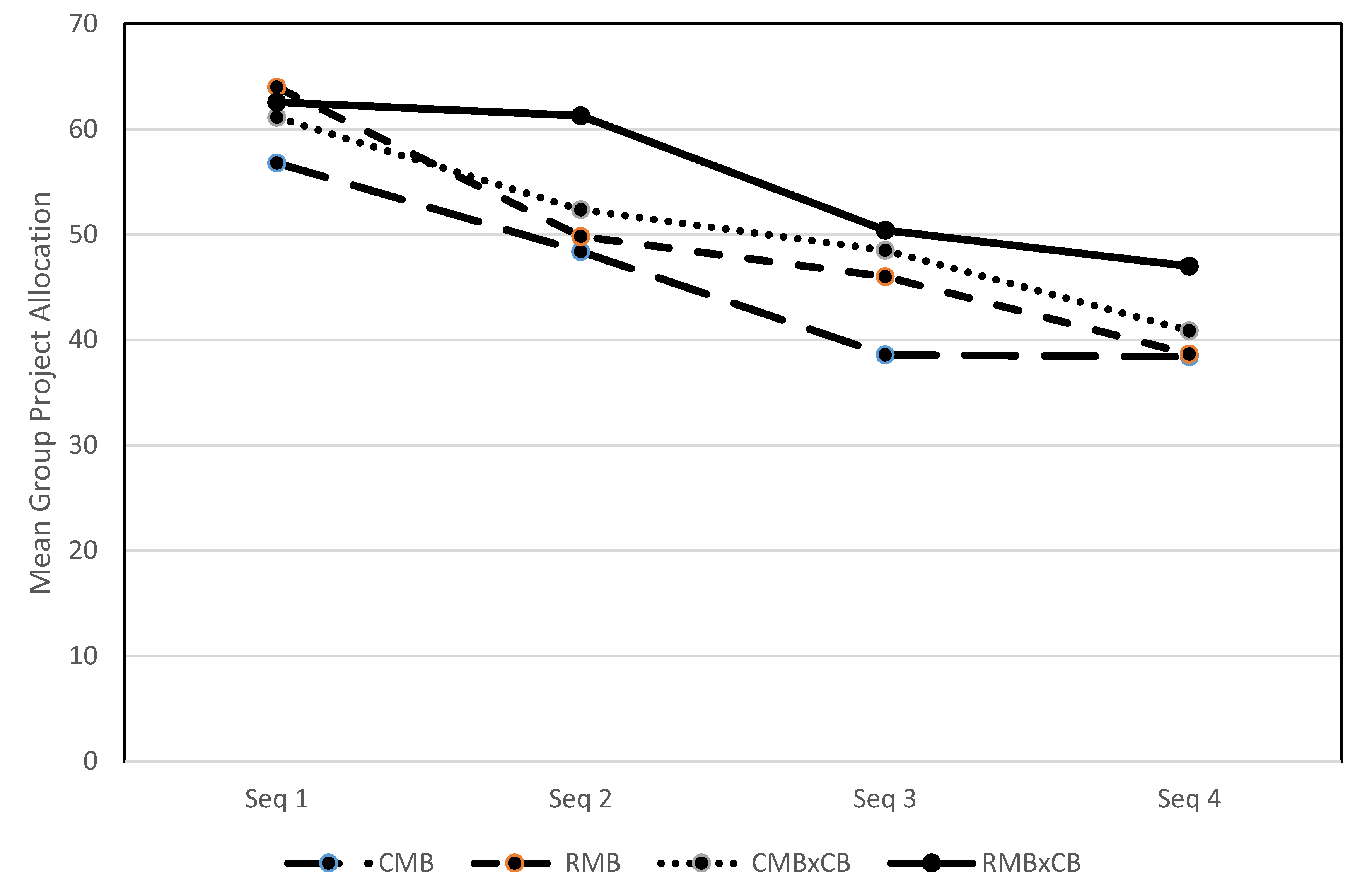
| 17 | When the data analysis presented in this section is repeated using only data from the first sequence, there is no statistical difference in contributions or completion rates between treatments. Subjects may be forming beliefs about how others will behave in the game based on their previous interactions. Examining Figure 1, it appears the existence of the completion benefit prevents contributions from decaying as much as the CMB and RMB treatments as the sequences progress. |
| 18 | There is one outlying group with a total group contribution of 91 tokens in the RMB treatment. Members of this group made mistaken choices as 30 additional tokens were contributed to the group project in rounds following the round in which the project was completed. All results are qualitatively similar if this group is removed from the data. |
| 19 | Recall that new groups were formed for each sequence within an experimental session. |
| 20 | Sequence 1: Round 3 (p = 0.003); Sequence 2: Round 4 (p = 0.08), Round 6 (p = 0.08), Sequence 3: Round 3 (p = 0.08), Round 7 (p = 0.09), Round 10 (p = 0.02); Sequence 4: Round 6 (p = 0.04). |

| Treatment Condition | Number of Experimental Sessions | Number of Subjects | Total Number of 10-Round Sequences Completed by 5-Subject Groups |
|---|---|---|---|
| CMB | 2 | 25 | 20 |
| RMB | 2 | 30 | 24 |
| CMB CB | 3 | 40 | 32 |
| RMB CB | 3 | 35 | 28 |
| Treatment | Number of Observations | Mean | Standard Deviation | Minimum Observation | Maximum Observation |
|---|---|---|---|---|---|
| CMB | 20 | 45.55 | 13.20 | 20 | 70 |
| RMB | 24 | 49.63 | 16.45 | 12 | 91 |
| CMB CB | 32 | 50.72 | 12.77 | 25 | 70 |
| RMB CB | 28 | 55.32 | 11.19 | 26 | 73 |
| (a) Coefficients | ||||
|---|---|---|---|---|
| Independent Variable | Coefficient Estimate | Standard Error | Ho: Coefficient = 0 | |
| t | p-Value | |||
| Constant | 56.185 | 1.411 | 39.83 | 0.000 |
| RMB | 4.075 | 4.399 | 0.93 | 0.378 |
| CMB CB | 5.169 | 1.064 | 4.86 | 0.001 |
| RMB CB | 9.771 | 1.177 | 8.30 | 0.000 |
| 2nd Sequence | −7.923 | 2.019 | −3.92 | 0.003 |
| 3rd Sequence | −14.808 | 2.011 | −7.36 | 0.000 |
| 4th Sequence | −19.808 | 1.854 | −10.68 | 0.000 |
| (b) Hypothesis tests across treatments and sequences | ||||
| Compared Treatments | Ho | p-Value | ||
| CMB vs. RMB | RMB = 0 | 0.378 | ||
| CMB vs. CMB CB | CMB CB = 0 | 0.001 | ||
| CMB vs. RMB CB | RMB CB = 0 | 0.000 | ||
| RMB vs. CMB CB | RMB − CMB × CB = 0 | 0.813 | ||
| RMB vs. RMB CB | RMB − RMB × CB = 0 | 0.240 | ||
| CMB CB vs. RMB CB | CMB × CB − RMB × CB = 0 | 0.014 | ||
| 2nd Seq vs. 3rd Seq | 2nd Seq − 3rd Seq = 0 | 0.011 | ||
| 2nd Seq vs. 4th Seq | 2nd Seq − 4th Seq = 0 | 0.000 | ||
| 3rd Seq vs. 4th Seq | 3rd Seq − 4th Seq = 0 | 0.008 | ||
| (a) Coefficients | |||||
| Independent Variable | Coefficient Estimate | Standard Error | Ho: Coefficient = 0 | ||
| Z | p-Value | ||||
| Constant | −0.329 | 0.571 | −0.58 | 0.565 | |
| RMB | 0.839 | 0.742 | 1.13 | 0.258 | |
| CMB x CB | 1.482 | 0.669 | 2.21 | 0.027 | |
| RMB x CB | 2.213 | 0.753 | 2.94 | 0.003 | |
| 2nd Sequence | −1.649 | 0.745 | −2.21 | 0.027 | |
| 3rd Sequence | −2.817 | 0.470 | −5.99 | 0.000 | |
| 4th Sequence | −3.635 | 0.657 | −5.53 | 0.000 | |
| (b) Marginal Effects | |||||
| Independent Variable | Marginal Effect | Standard Error | Ho: coefficient = 0 | ||
| Z | p-Value | ||||
| RMB | 0.172 | 0.163 | 1.06 | 0.290 | |
| CMB x CB | 0.306 | 0.136 | 2.25 | 0.024 | |
| RMB x CB | 0.471 | 0.155 | 3.03 | 0.002 | |
| 2nd Sequence | −0.245 | 0.078 | −3.13 | 0.002 | |
| 3rd Sequence | −0.362 | 0.043 | −8.36 | 0.000 | |
| 4th Sequence | −0.429 | 0.051 | −8.48 | 0.000 | |
| (c) Hypothesis tests across treatments and sequences | |||||
| Conditional Means Compared | Ho | p-Value | |||
| CMB vs. RMB | RMB = 0 | 0.258 | |||
| CMB vs. CMB CB | CMB CB = 0 | 0.027 | |||
| CMB vs. RMB CB | RMB CB = 0 | 0.003 | |||
| RMB vs. CMB CB | RMB − CMB × CB = 0 | 0.238 | |||
| RMB vs. RMB CB | RMB − RMB × CB = 0 | 0.041 | |||
| CMB CB vs. RMB CB | CMB × CB − RMB × CB = 0 | 0.157 | |||
| 2nd Seq vs. 3rd Seq | 2nd Seq − 3rd Seq = 0 | 0.126 | |||
| 2nd Seq vs. 4th Seq | 2nd Seq − 4th Seq = 0 | 0.001 | |||
| 3rd Seq vs. 4th Seq | 3rd Seq − 4th Seq = 0 | 0.336 | |||
| (a) Groups that Reach 20 Tokens Before Round Four | ||||
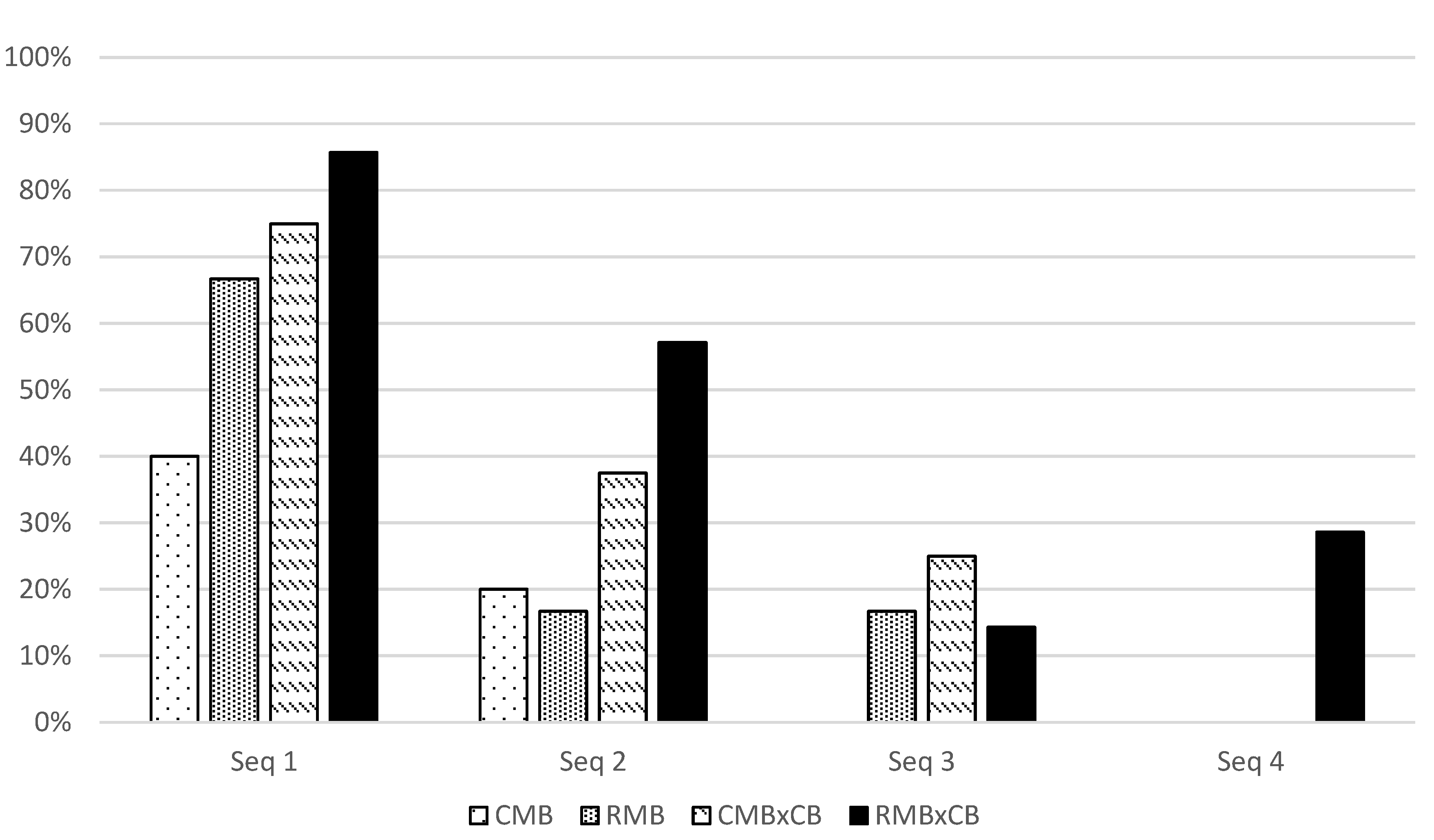
| Treatment | Total Number of Groups | Number of Groups that Reach Minimum of 20 Tokens before Round 4 (Percentage of Total Groups) | Number of Groups that Complete Project Conditional on Reaching Minimum of 20 Tokens before Round 4 (Percentage of Such Groups) | Average Percentage of Remaining Project Completed Conditional on Reaching 20 Tokens before Round 4 |
| CMB | 20 | 8 (40.00%) | 3 (37.50%) | 88.09% |
| RMB | 24 | 13 (54.17%) | 6 (46.15%) | 125.34% * |
| CMB CB | 32 | 23 (71.88%) | 11 (47.83%) | 81.82% |
| RMB CB | 28 | 17 (60.71%) | 11 (64.71%) | 107.63% |
| (b) Groups that do not Reach 20 Tokens Before Round Four | ||||
| Treatment | Total Number of Groups | Number of Groups that Complete Project Conditional on not Reaching 20 Tokens before Round 4 (Percentage of Such Groups) | Average Percentage of Remaining Project Completed Conditional on Not Reaching 20 Tokens before Round 4 | |
| CMB | 20 | 0 (0.00%) | 57.40% | |
| RMB | 24 | 0 (0.00%) | 58.09% | |
| CMB CB | 32 | 0 (0.00%) | 55.36% | |
| RMB CB | 28 | 2 (18.00%) | 74.22% | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baker, R.; Halloran, M. Dynamic Contributions to a Public Project: The Impact of Rising Marginal Benefit and Completion Benefits. Games 2018, 9, 43. https://doi.org/10.3390/g9030043
Baker R, Halloran M. Dynamic Contributions to a Public Project: The Impact of Rising Marginal Benefit and Completion Benefits. Games. 2018; 9(3):43. https://doi.org/10.3390/g9030043
Chicago/Turabian StyleBaker, Ronald, and Matthew Halloran. 2018. "Dynamic Contributions to a Public Project: The Impact of Rising Marginal Benefit and Completion Benefits" Games 9, no. 3: 43. https://doi.org/10.3390/g9030043
APA StyleBaker, R., & Halloran, M. (2018). Dynamic Contributions to a Public Project: The Impact of Rising Marginal Benefit and Completion Benefits. Games, 9(3), 43. https://doi.org/10.3390/g9030043




