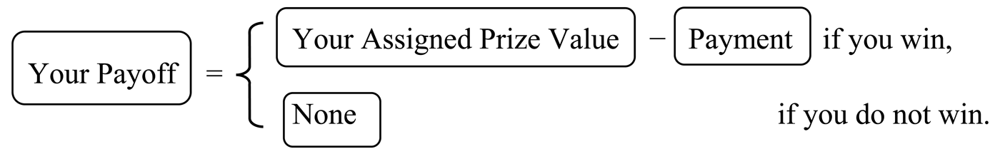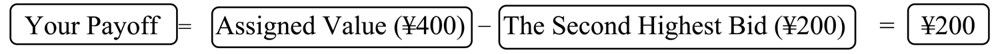Spite and Reciprocity in Auctions

Abstract

The paper presents a complete information model of bidding in second price sealed-bid and ascending-bid (English) auctions, in which potential buyers know the unit valuation of other bidders and may spitefully prefer that their rivals earn a lower surplus. Bidders with spiteful preferences should overbid in equilibrium when they know their rival has a higher value than their own, and bidders with a higher value underbid to reciprocate the spiteful overbidding of the lower value bidders. The model also predicts different bidding behavior in second price as compared to ascending-bid auctions. The paper also presents experimental evidence broadly consistent with the model. In the complete information environment, lower value bidders overbid more than higher value bidders, and they overbid more frequently in the second price auction than in the ascending price auction. Overall, the lower value bidder submits bids that exceed value about half the time. These patterns are not found in the incomplete information environment, consistent with the model.

1. Introduction

One of the most basic and apparently innocuous assumptions about behavior in games is that players will adopt dominant strategies. One reason why players may avoid dominant strategies, as expressed in monetary payoffs, is because they have social preferences such as spite or conditional Results and Discussion cooperation. Recent laboratory research in public good mechanism design, for example, has documented extensive failure by subjects to follow dominant strategies even in fairly simple environments, perhaps due to a desire to cooperate with others who are also cooperative (Attiyeh et al. [1]; Kawagoe and Mori [2]; Cason et al. [3]). Mixed results also exist in experiments assessing the incentive-compatibility of second price (Vickrey) auctions. For example, Kagel and Levin [4] find that 58 to 67 percent of bids exceed value, and Harstad [5] reports that severe overbidding does not decline over time, while others such as Coppinger et al. [6], Cox et al. [7], Kagel et al. [8], and Chew and Nishimura [9] report consistency with value-revealing bidding.

Overbidding is much less pronounced in the English, ascending-bid auction. Especially in the “Japanese” version of ascending-bid auction [10,11], which is isomorphic to the second price auction, the equilibrium bidding strategy is more transparent, which has led some researchers to conclude that the subtlety of the dominant strategy in the sealed bid second-price auction is a primary reason some bidders fail to follow it. Learning is also difficult in the second price auction because the use of a weakly dominated strategy may often not cause any loss in actual payoff (Kagel and Levin [4]). Moreover, even with standard (own-payoff maximizing) preferences, many Nash equilibria exist in these auction formats other than the dominant strategy equilibrium.

This paper explores the importance of alternative, spiteful preferences as an explanation for overbidding in second price and ascending-bid auctions. A spiteful agent has utility that increases when the earnings of her rivals decrease, and so she may be willing to sacrifice some monetary payoff in order to reduce the other agent's monetary payoff (Saijo and Nakamura [12]). The following section contains our formal definition, which features a reciprocal motive; i.e., subjects feel more spiteful towards others who treat them spitefully. The key design feature of second price and ascending-bid auctions that make them incentive-compatible under standard preferences makes them particularly prone to manipulation by bidders who have spiteful preferences. Because an individual's monetary payoff conditional on winning the auction is independent of her bid, if she cares only about her monetary payoff has no incentive to change her bid to lower her price. But if she fails to win her bid may determine the payoff of the winner. Therefore, if she is spiteful she can increase her bid to increase her (spiteful) utility. Agents who have spiteful preferences would not consider a bid equal to value to be a dominant strategy.

We construct a two-bidder, intention-based reciprocal decision model which belongs to the class of reciprocity models including Rabin [13] and Segal and Sobel [14], and extend it to the sequential decision making of the ascending-bid auction in the spirit of Dufwenberg and Kirchsteiger [15] and Falk and Fischbacher [16]. Intention is measured by the distance between a buyer's bid choice and her standard value-revealing bid which we consider as the intention neutral reference. A bidder with a low value for the object may behave spitefully when her opponent expects to win a positive surplus. This can be interpreted as part of the disutility of losing the auction, since she is in a disadvantageous position. This may prompt her to place a spiteful bid higher than her value, hoping to reduce her opponent's winning surplus. For example, suppose it is common knowledge that bidder 1 values the object at 800 yen, while bidder 2 values the object at 700 yen. A spiteful bidder 2 could bid, say, 750 rather than her value 700 in a second-price auction to reduce bidder 1's winning surplus from 800 – 700 = 100 to 800 – 750 = 50. A novelty of our analysis is in incorporating retaliation by the bidder who has a higher value. The higher value bidder may place a deliberately low bid in order to penalize the spiteful conduct by the lower value bidder, even though such a retaliatory bid reduces his chance of winning. Continuing the example, bidder 1 could bid less than his value of 800, such as 760, in order to limit the spiteful actions of bidder 2. Bidder 1 could even penalize a spiteful bidder 2 by bidding lower than 750.

Another novel feature of our analysis is that we consider a complete information environment, which strengthens the impact of social preferences such as spite and reciprocity. This is intended to approximate conditions in which bidders have some information about rivals' values or costs, such as in local government procurement settings with repeated competition between the same set of bidders. In the incomplete information environment typically employed in the auction literature, adding spiteful and reciprocal preferences as we have modeled them still results in bids equal to value in the unique (but not dominant strategy) symmetric equilibrium. By contrast, bidders with spiteful and reciprocal preferences should overbid in equilibrium when they have complete information about their rival's value and they know their rival has a higher value than their own.

Spiteful and reciprocal preferences also make the second price and the ascending-bid auction forms non-isomorphic. In an ascending-bid auction, an auctioneer or clock raises a calling price until there remains only one active bidder. A climbing calling price gradually reduces the winner's payoff. Taking this effect into account, in our sequential decision model the bidders are more aware of the extent of the other's spitefulness when they reach each new, higher calling price, because they can infer that their rival did not drop out. This makes the bidder with the higher value willing to retaliate at an earlier stage. Consequently, for the same level of spiteful preferences, in response the lower value bidders should overbid less in the ascending-bid auction than in the second price auction. Thus, the upper bound of the set of equilibria in ascending-bid auctions is likely to be lower.

The second part of the paper presents experimental evidence that provides some qualified support for the predictions of this model. In the complete information environment, lower value bidders overbid more than higher value bidders, and they overbid more frequently in the second price auction than in the ascending price auction. Overall, the lower value bidder submits bids that exceed value about one-half the time. These patterns are not found in the data we collected for the incomplete information environment, consistent with the model. Similar to most of the literature on incomplete information second price and ascending bid auctions, bids are near values for both low-and high-value bidders.

Researchers have recently measured and explored the impact of social preferences that include reciprocity and spite in a variety of environments, but often in non-competitive contexts such as public good provision, two-agent bargaining and simple games. A small amount of research has studied the impact of spite in auctions, starting with Morgan et al.'s [17] theoretical analysis. Their model, which we discuss below in more detail, features non-reciprocal spite and does not predict differences between the second price and ascending price auctions for the two-bidder setting we employ. Cooper and Fang's [18] experimental study also considers (like us) a two-bidder environment for simplicity, but only second price auctions. They provide bidders with noisy information about their rival's value, with varying degrees of accuracy, and find that overbidding is consistent both with spite and “joy-of-winning” motivations. Andreoni et al. [19] also report a laboratory experiment in which bidders may have information about rivals' value draws. They consider first and second price auctions, all with four competing bidders, and test predictions regarding equilibrium strategies in three different information structures. Their results provide strong support for theory, but they also observe overbidding by lower value bidders in their second price auctions that is consistent with a spite motive. Herrmann and Orzen [20] identify spiteful behavior in two-bidder contests that share some strategic similarities to auctions.

Our results are also consistent with spiteful bidder preferences, and we observe overbidding and underbidding in a pattern consistent with our model of reciprocal spite. Lower value bidders overbid relative to their values, but in response the higher value bidders underbid to punish this overbidding (or at least make overbidding risky). In equilibrium these spiteful social preferences substantially reduce the size of the set of Nash equilibria. Moreover, this combination of spite and reciprocity is the reason that isomorphism fails for the second price and ascending price auction, and the particular pattern of larger and more frequent overbids in the second price auction predicted by the model is also observed in the experimental data.

2. The Model: Spite Bidding with and without Reciprocity

2.1. Known Values (Complete Information)

Consider, for simplicity, the case of two buyers with unit demand of values {v₁,v₂}, with v₁ > v₂. In this subsection, we assume that both buyers know each other's values. Although this assumption of complete information about a rival bidder's values is unusual, we consider it for two reasons. First, complete information is a reasonable approximation of auction markets that take place repeatedly between the same set of bidders, such as procurement auctions where bid histories provide information about values (Arora et al. [21]; Cason et al. [22]), and other settings with repeated bidding such as for internet search auctions (Edleman et al. [23]). Second, spiteful motivations are more likely to be triggered in the complete information environment, since bidders know their relative value position. This allows the model to highlight the effect of spiteful reciprocity while eliminating the complexity arising from value uncertainty. This view is also consistent in spirit with the increased information about rivals studied in Andreoni et al. [19] and Cooper and Fang [18] that also consider implications of spite in auctions. Thus, our complete information model provides a useful benchmark for the case of well-informed bidders, which presents the starkest contrast to the more standard incomplete information context, considered below in Section 2.2. As will be seen, an analysis of this complete information context reveals new implications for bidder behavior in auctions.

We consider all values and bids in terms of minimum transaction unit ε > 0, corresponding for example to a minimum currency unit. Let v_i ∈ V, i ∈ {1,2}, where V = {0, ε,2ε, ⋯, v̅−ε, v̅},v̅ = u̅ε, and u̅ is a finite positive integer greater than 1. Each buyer chooses a bid b_i ∈ B_i = B, i ∈ {1,2}, where B_i = B = {0, ε, 2ε, ⋯, b̅ − ε, b̅}, i ∈ {1,2}, a set of bids commonly available to the two buyers, where b̅ = c̅ε > v̅ and c̅ is a finite positive integer greater than 1. In what follows, we consider only pure bid strategies.

A. Second Price Auction

In the second price auction with two buyers, the winner's payment is equal to the loser's bid. Thus, buyer i's monetary payoff is given by π_i : B_i × B_j → ℜ, i, j ∈ {1,2}, i ≠ j, such that

$${\pi }_i(b_i,b_j)=(v_i-b_j)I_{b_i>b_j}+0\cdot I_{b_i<b_j}+(1/2)(v_i-b_j)I_{b_i=b_j}$$

(1)

where I_A is an index function which assumes value 1 when the statement A holds, and zero otherwise. The first term in the RHS of (1) is her winning payoff, the second term is her losing payoff (zero), and the third term is her expected payoff from a tie.

Case 1: The Conventional Model

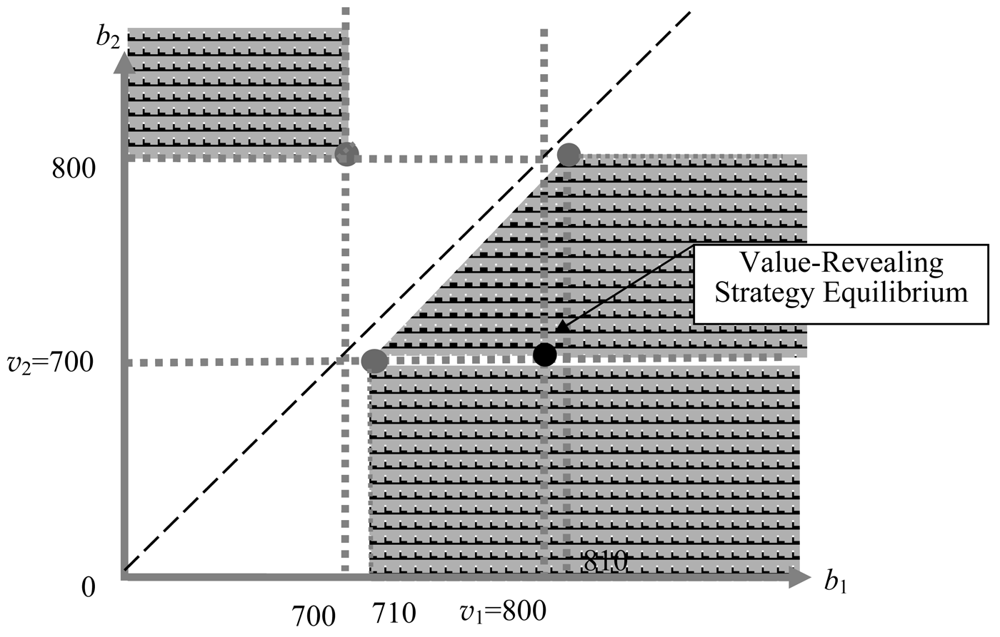
It is well known that the second price auction has multiple Nash equilibria. Figure 1 shows the set of Nash equilibria with two buyers who receive payoff (1) when (v₁, v₂) = (800, 700). The upper left shaded area is the set of inefficient equilibria where buyer 2 with the lower value wins. The efficient lower right area includes a value-revealing dominant strategy Nash equilibrium [24,25].

In the following, we show that introducing spiteful motivations narrows the set of Nash equilibria and that the equilibrium set also differs between when bidders are spiteful with and without reciprocity.

Case 2: Spite-without-Reciprocity

Morgan et al. [17] present a model of two spiteful buyers who obtain a utility loss when they lose that depends on their rival's amount of monetary winning payoff [26]. There is no element of reciprocity in their model, since buyers become unconditionally spiteful whenever they lose. So we call this case the spite-without-reciprocity model. In their model, buyer i's utility can be represented by

$$u_i(b_i,b_j)=(v_i-b_j)I_{b_i>b_j}-{\eta }_i(v_j-b_i)\cdot I_{b_i<b_j}+(1/2)[(v_i-b_j)-{\eta }_i(v_j-b_i)]I_{b_i=b_j}$$

(2)

for i, j ∈ {1, 2}, i ≠ j, where the coefficient η_i is a positive real number.

The black dotted line in Figure 2 shows, the set of Nash equilibria for the case with (v₁, v₂) = (800, 700), ε = 10, η₁ = 0.3, and η₂ = 0.8. The equilibrium set is much smaller compared to Figure 1, and all of its elements are efficient. When spiteful preferences are stronger, however, as shown by the orange dotted line with η₁ = 1 and η₂ = 4, all elements of the equilibrium set are inefficient. The condition that determines whether the equilibrium set is efficient is η₁η₂ < 1. Two points should be noted; one is that the lower bound of the equilibrium set is strictly above (b₁, b₂) = (v₂ + ε, v₂) or (b₁, b₂) = (v₂, v₂ + ε). The other is that the value-revealing strategy (b₁, b₂) = (v₁, v₂) is no longer an equilibrium and there is no equilibrium bid strategy that generates the equivalent monetary outcome of the value-revealing strategy.

Case 3: Spite-with-Reciprocity

The main innovation of our model is to incorporate the possibility of retaliation against a bidder's spiteful behavior. We label this spite-with-reciprocity model. We construct buyers' utility in the spirit of the reciprocity model proposed by Segal and Sobel [14] with some modifications on the specific functional forms [27–31]:

$$U_i(b_i,b_j)=(v_i-b_j)I_{b_i>b_j}+{\gamma }_i\cdot {\alpha }_i^j(b_j)[(v_j-b_i)-R_j]\cdot I_{b_i<b_j}+(1/2)\left[(v_i-b_j)+{\gamma }_i\cdot {\alpha }_i^j(b_j)[(v_j-b_i)-R_j]\right]I_{b_i=b_j}$$

(3)

for i, j ∈ {1,2}, i ≠ j. The first term in the RHS of (3) is buyer i 's monetary payoff when she wins. The second term is the payoff in the event of loss that consists of monetary payoff zero (which is omitted) and the term which is typically labeled as psychological payoff in reciprocity models. This term is a product of the difference between her rival's monetary payoff v_j − b_i and rival's reference winning payoff (hereafter reference payoff) R_j, and a weight ${\alpha }_i^j(b_j)$ with coefficient γ_i which is a non-negative real number [32]. Note that when γ_i= 0 or ${\alpha }_i^j(b_j)=0$, the utility (3) becomes conventional utility (1). When ${\alpha }_i^j(b_j)$ is a negative constant and the reference payoff R_j = 0 For i, j ∈ {1, 2} and i ≠ j, the utility (3) becomes the utility with spite-without-reciprocity of (2). Thus, as usual in reciprocity models, the reference payoff R_j and the weight ${\alpha }_i^j(b_j)$ play the key role in characterizing reciprocity. In this paper, we regard R₁ = v₁ − v₂ and R₂ = 0 as the reference because these payoffs are realized under the value-revealing bid strategy b_k = v_k, k = i, j, which is neutral of any spiteful intention since it is the dominant strategy in the conventional model. Also, we assume the weight ${\alpha }_i^j(b_j)$ is non-positive to represent the bidder's spite intention [33]. Reciprocity models commonly interpret the weight ${\alpha }_i^j(b_j)$ to reflect the buyer i's view of buyer j's intention toward buyer i, and represents buyer j's intention by a deviation of buyer j's action from the spite neutral reference strategy b_j = v_j.

Specifically, consider the case of buyer 1. If b₂ ∈ {v₂, ⋯, v₁ − ε}, buyer 1 should speculate what makes buyer 2 place a bid b₂ > v₂ since such bids increase the likelihood of a negative monetary payoff if buyer 2's purpose is to win. It is reasonable for buyer 1 to perceive buyer 2's bid deviation from v₂ as spiteful, because buyer 2 reduces buyer 1's winning surplus to v₁ − b₂ from v₁ − v₂. Hence, the size of bid deviation v₂ − b₂ corresponds to buyer 1's lost surplus. How much damage the lost surplus means to buyer 1 must be measured relative to the range of buyer 1's potential winning payoff possible with buyer 2's spite bid, which is v₁ − v₂. Thus, we define buyer 1's weight such that ${\alpha }_1^2(b_2)=min\{v_2-b_2,0\}/(v_1-v_2+\epsilon )$ [34]. Recognizing buyer 2's spiteful intention, buyer 1 may reciprocate by placing a deliberately low bid in order to let buyer 2 win with negative winning surplus of v₂ − b₁.

Consider next the case of buyer 2. The size of bid deviation v₁ − b₁ reflects the minimum winning surplus that buyer 1 claims. The larger payoff buyer 1 claims, the more spiteful buyer 2 becomes. The size of bid deviation v₁ − b₁ also corresponds to buyer 1's maximum potential winning surplus that she is willing to forego to retaliate against buyer 2. The impact of bid deviation v₁ − b₁ should be measured relative to the payoff range v₁ − v₂. Thus, we define buyer 2's weight by ${\alpha }_2^1(b_1)=-max\{v_1-b_1,0\}/(v_1-v_2+\epsilon )$. With these specifications for weights, it is easy to check that the losing payoff of each buyer i, i ∈ {1,2} is increasing in her own bid b_i.

Segal and Sobel [14] showed that the Nash equilibrium concept is directly applicable to their general reciprocity model (see [27]). Accordingly, we define the equilibrium of the second price auction as follows.

Definition 1 (Equilibrium in the second price auction)

A strategy profile $(b_i^{\ast },b_j^{\ast })\in B_i\times B_j$ generates an equilibrium in the second price sealed-bid auction, if for each i, j ∈ {1,2}, i ≠ j, $b_i^{\ast }\in B{R}_i(b_j^{\ast })$, where BR_i : B_j → B_i is buyer i's best response correspondence defined by

$$B{R}_i(b_j)=\left\{b_i\in B_i\mid U_i(b_i,b_j)\ge U_i(b_i^{\prime },b_j),\forall b_i^{\prime }\in B_i\right\},\text{for}\text{a}\text{given}{b}_j\in B_j.$$

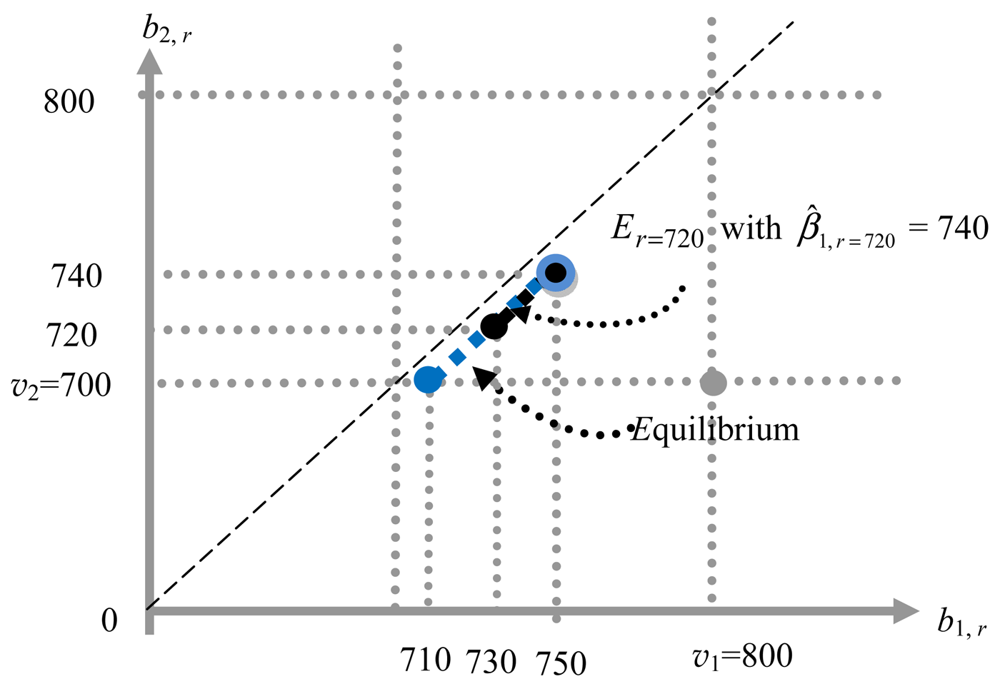
Thus, we can identify the equilibrium set for any (v₁, v₂) ∈ V × V, v₁ > v₂, and γ₁ ∈ ℜ₊, which is formally stated in Proposition 1 below. (Proofs of all propositions and lemmas are collected in Appendices A and B.) Figure 3 shows an example when (v₁, v₂) = (800, 700) and γ₁ = 2. The equilibrium set is represented by a dotted line segment whose lower bound is (b₁, b₂) = (v₂ + ε, v₂) = (710, 700) and upper bound is (b₁, b₂) = (β̂₁ + ε, β̂₁)= (760, 750), where β̂₁ ∈ B₁ is the threshold bid for buyer 1 in the following sense [35]; when her rival bids above β̂₁, buyer 1 strictly prefers to lose, and weakly prefers to win otherwise. Lemma 1 below summarizes the property of β̂_i, i ∈ {1,2} (For details about β̂_i and the proof of Lemma 1, see Appendix A-1.) [36].

Lemma 1

(i) There exists a unique threshold bid β̂_i ∈ B, for each i ∈ {1,2}. (ii) β̂₁ ∈ {v₂, ⋯, v₁ − ε} and β̂₂ = v₂.

Proposition 1

The equilibrium set with buyers of the spite-with-reciprocity type is given by

$$E=\left\{(b+\epsilon ,b)\in B_1\times B_2\mid (v_2={\widehat{\beta }}_2)\le b\le {\widehat{\beta }}_1\right\}.$$

The boundaries of equilibrium set are defined by the two buyers' threshold bids, and the equilibrium set in Figure 3 is much smaller than the one in Figure 1 [37]. Unlike the conventional and the spite-without-reciprocity models, the equilibrium set is always efficient. We can conclude that introducing buyers of spite-with-reciprocity type does not impede efficiency, and it even potentially improves the performance of second price auction, since it eliminates inefficient outcomes from the equilibrium set [38]. Although the value-revealing bid strategy is no longer an equilibrium, the equilibrium bid strategy (b₁, b₂) = (v₂ + ε, v₂) = (β̂₂ + ε, β̂₂), the lower bound of E, generates the equivalent outcome.

B. Ascending-Bid Auction

In an ascending-bid auction the calling price rises by unit ε, and this increase occurs in each unit of time in the clock version. We assume that the initial price is low enough so that both buyers are active at the start. The auction terminates when either buyer withdraws from bidding. If both buyers withdraw simultaneously, the winner is chosen randomly with equal probabilities, and the winner has to pay her own withdrawal bid.

Let r ∈ B denote the calling price, with r = 0 corresponding to the initial stage before the auction starts. At each r, each buyer chooses a bid at which she plans to withdraw. Such a planned withdrawal bid of buyer i at decision point r is denoted by b_i,r ∈ B_r, where B_i,r = B_r = {r, r + ε, −, b̅}, i ∈ {1,2} for all r ∈ B. Note that the decision problem at r = 0 is equivalent to that in the second price auction. Each buyer makes sequential decision at each decision point r as the calling price rises, until she arrives at the actual withdrawal point where b_i,r = r. Those sequential decisions of planned withdrawal bids (hereafter bids) {b_i,r} are behavioral strategies. Since the ascending-bid auction does not allow buyers to reenter after they withdraw, our analysis focuses on the buyers' behavioral strategies. The auction terminates at the decision point where min{b_1,r, b_2,r} = r.

We are particularly interested in buyers' behavior when r climbs past v₂. When this occurs there is no doubt that buyer 2's bid exceeds v₂, which means to buyer 1 that buyer 2 is spiteful. By letting r go beyond v₂, buyer 2 eliminates the upper part of possible payoff range of buyer 1 located above v₁ − r. The extent of spitefulness toward buyer 1 of the same size of bid deviation v₂ − b₂ must be different for different range of buyer 1's possible winning payoffs, and so must be the effect of the bid deviation v₁ − b₁ on buyer 2. We extend our model (3) to the extensive form in the spirit of Dufwenberg and Kirchsteiger [15] based on the behavioral strategies, through modifying the weight ${\alpha }_i^j(b_j)$, i, j ∈ {1,2}, i ≠ j, of (3) in the following way. The new weight ${\alpha }_i^j(b_{j,r},r)$ depends on a behavioral strategy b_j,r at each decision point r, and especially when r ∈ {v₂ + ε, ⋯, v₁ − ε}, the weight ${\alpha }_i^j(b_{j,r},r)$ is updated with the new, smaller denominator v₁ − max{v₂, r} + ε. Buyer i's utility at r is therefore given by

$$U_i(b_{i,r},b_{j,r},r)=(v_i-b_{j,r})I_{b_{i,r}>b_{j,r}}+{\gamma }_i\cdot {\alpha }_i^j(b_{j,r},r)(v_j-b_{i,r}-R_j)\cdot I_{b_{i,r}<b_{j,r}}+(1/2)[(v_i-b_{j,r})+{\gamma }_i\cdot {\alpha }_i^j(b_{j,r},r)(v_j-b_{i,r}-R_j)]I_{b_{i,r}=b_{j,r}}$$

(4)

for i, j ∈ {1,2}, i ≠ j, where

$${\alpha }_i^j(b_{j,r},r)=\{\begin{array}{ll}\hfill min\{v_j-b_{j,r},0\}/(v_1-max\{v_2,r\}+\epsilon ),\hfill & \text{if}{v}_i\ge v_j\hfill \\ \hfill -max\{v_j-b_{j,r},0\}/(v_1-max\{v_2,r\}+\epsilon ),\hfill & \text{otherwise},\hfill \end{array}\begin{array}{c}\text{for}r\in \{0,\cdots ,v_1-\epsilon \},\text{and}\end{array}$$

${\alpha }_i^j(\cdot ,r)=0$ for r ∈ {v₁, ⋯,b̅}. Note that the absolute value of ${\alpha }_i^j(b_{j,r},r)$ becomes larger as r rises when r ∈ {v₂ + ε, ⋯, v₁ − ε}. This means that each buyer becomes more sensitive to the other buyer's spite intention [39,40].

For each r, we consider the equilibrium set analogous of E in Proposition 1, which we call interim equilibrium set, denoted by E_r.

Definition 2 (Interim equilibrium)

At each r ∈ B, let BR_i,r(b_j,r) denote buyer i's best response correspondence against a given b_j,r ∈ B_r such that, $B{R}_{i,r}(b_{j,r})=\left\{b_{i,r}\in B_r\mid U_{i,r}(b_{i,r},b_{j,r},r)\ge U_{i,r}(b_{i,r}^{\prime },b_{j,r},r),\forall b_{i,r}^{\prime }\in B_r\right\}$ for i, j ∈ {1,2}, i ≠ j. A bid strategy profile $(b_{1,r}^{\ast },b_{2,r}^{\ast })\in B_r\times B_r$ at given r ∈ B is an interim equilibrium, if $b_{i,r}^{\ast }\in B{R}_{i,r}(b_{j,r}^{\ast })$, i, j ∈ {1,2}, i ≠ j.

For a given r, an interim equilibrium set can be stated as E_r = {(b_1,r, b_2,r) ∈ B_r × B_r ∣ b_i,r ∈ BR_i,r(b_j,r), i ={1,2}, i ≠ j}. The set E₀ at r = 0 is the same as E in the second price auction. Let us call E_rnull, if its only element is (b_1,r, b_2,r) = (r, r). Proposition 2 below describes the properties of non-null interim equilibrium sets. Each non-null E_r has its lower bound (b_1,r, b_2,r) = (max{r, v₂} + ε, max{r, v₂}) and its upper bound (b_1,r, b_2,r)=(β̂_1,r + ε, β̂_1,r), where β̂_1,r ∈ B_r is the threshold bid for buyer 1 at decision point r, which is the counterpart of β̂₁ in the second price auction [41]. Lemma 2 summarizes the properties of β̂_i,r, i ∈ {1,2}. By the rule of the ascending-bid auction that prohibits reentry, if E_r is null for some, r = r′, then E_r is null for all r ∈ {r′ + ε, ⋯, b̅}. Lemma 3 shows that the calling price r̂ identified in Lemma 2 (i) is the lowest r beyond which E_r becomes null. Consequently, the auction will never continue beyond r̂.

Lemma 2

• There exists r̂ ∈ {v₂, ⋯, v₁ − ε} such that r̂ = min{r ∈ B∣r = β̂_1,r}.

• β̂_2,r = v₂ when r ∈ {0, ⋯, v₂}, otherwise no buyer 2's threshold bid exists. There exists unique β̂_1,r ∈ {max{v₂, r}, ⋯, v₁ − ε} for each r ∈ {0, ⋯, r̂} with β̂_1,0 = β̂₁. No buyer 1's threshold bid exists for r ∈ {r̂ + ε, ⋯, b} except r = v₁ where β̂_1,r = v₁.

• β̂_1,r = β̂₁ for all r ∈ {0, ε, ⋯,v₂} and β̂_1,r is non-increasing in r, for all r ∈ {v₂ + ε, ⋯, r̂}.

Lemma 3

E_r is not null for all r ∈ {0, ⋯, r̂} and E_r is null otherwise.

Buyer i does not prefer to win against any of her rival's possible bids b_j,r ∈ B_r, when r falls in the range where no β̂_i,r exists (see Appendix A-4). Based on Lemma 2 and 3, we can derive buyers' best response correspondences. (The list of the best response correspondences is available in Appendix (A-5.). By Lemma 3, we can restrict our equilibrium analyses to the case r ∈ {0, ⋯, r̂}.

Proposition 2

• The interim equilibrium set E_r for all r ∈ {0, ε, ⋯, r̂ − ε} is given by E_r = {(b + ε, b) ∈ B_r × B_r∣max{v₂, r} ≤ b ≤ β̂_1,r}.

• For r = r̂ = β̂_1,r, E_r̂ = {(r̂ + ε, r̂), (r̂, r̂)} if β̂_{1, r̂} = β̂_1,r̂, and E_r̂ = {(r̂ + ε, r̂)} otherwise.

Let B^r denote the set of bid profiles whose lower bid is greater than r, defined by B^r = {(b₁, b₂)∣min{b₁, b₂} ∈ {r + ε, r + 2ε, ⋯, b̅}}. Then, for a given r ∈ {0, ε, ⋯, r̂}, the set E_r\(E_r ∩ B^r) consists of interim equilibrium bid profiles that terminate the auction at r. Thus, these bid strategy profiles constitute equilibrium that ultimately determine the price in the ascending-bid auction. Hence, we define the equilibrium as follows.

Definition 3 (Equilibrium in the ascending-bid auction)

A bid strategy profile $(b_1^{\ast },b_2^{\ast })\in B\times B$ is an equilibrium in the ascending-bid auction, if $(b_1^{\ast },b_2^{\ast })\in {\cup }_{r=0}^{\widehat{r}}\left[E_r\backslash \left(E_r\cap {\mathit{\text{B}}}^r\right)\right]$ [42].

Proposition 2 together with Lemma 2 implies that we have an inclusion relation among all non-null interim equilibrium sets of the following sort: E_r̂ ⊂ E_r̂−ε ⊂⋯⊂E_v2 =⋯=E₀ = E [43]. The next proposition identifies the equilibrium set in the ascending-bid auction.

Proposition 3

The bid strategy profile $(b_1^{\ast },b_2^{\ast })\in B\times B$ is equilibrium in the ascending-bid auction if and only if $(b_1^{\ast },b_2^{\ast })\in E_0\backslash \left(E_0\cap {\mathit{\text{B}}}^{\widehat{r}}\right)=E\backslash \left(E\cap {\mathit{\text{B}}}^{\widehat{r}}\right)$.

It is immediate that the equilibrium set E \ (E ∩ B^r̂) ⊆ E. The upper bound of the equilibrium set of the ascending-bid auction coincides with the upper bound of the set E_r̂ which is (b_1,r, b_2,r) = (r̂ + ε, r̂). It is bounded by the upper bound of the equilibrium set E in the second price auction (b₁, b₂) = (β̂₁ + ε, β̂₁), since β̂_1,r ≤ β̂₁. Furthermore, (β̂₁ + ε, β̂₁) is bounded by the upper bound of the equilibrium set in the conventional model (v₁, v₁ − ε).

Figure 4 depicts an interim equilibrium set E_r when v₁ = 800, v₂ = 700, γ₁ = 2, ε = 10, r = 720, and β̂_1,r=720 = 740 as the dark dotted line between (b₁, b₂) = (r + ε, r) = (730, 720) and (b_1,r, b_2,r) = (β̂_1,r + ε, β̂_1,r) = (750, 740). The set E₇₂₀ in Figure 4 is the proper subset of the equilibrium set E in the second price auction of Figure 3. In this example, since the threshold bid β̂_1,r ∈ B_r remains the same at 740 for r = 730 and 740 (due to the discreteness of B_r [44]), r̂ = 740. Consequently, the equilibrium set of the ascending-bid auction comes down to the blue dotted line connecting between (710, 700) and (750, 740).

The analysis up to this point boils down to the following testable hypothesis: if the bidders are all self-regarding money-maximizing preference types, it is known that the prices observed in the ascending-bid auction should coincide in distribution with those in the second price auction. This also is the case when bidders are the type of spite-without-reciprocity, because the upper bound of corresponding interim equilibrium sets remains the same, which is easy to check. But if some bidders are the type of spite-with-reciprocity, it immediately follows from Proposition 3 that higher prices should be less frequent in the ascending-bid auction than in the second price auction.

2.2. Unknown Values (Incomplete Information)

In the incomplete information case, there are two main differences compared to the complete information case. First, the two players are now perceived as symmetric buyers (ex ante), so that we omit the subscript when there is no risk of confusion. Second, we no longer have a reason to restrict buyers' value sets and bid sets to be discrete with the minimum bid unit ε to ensure the existence of equilibrium.

Let V̅ = [0, v̅] be a closed interval from which each buyer's value is drawn independently. Let G:V̅ → [0, 1] be the cumulative probability distribution of each buyer's value with density function g:V̅ → [0,1], which is common knowledge. In what follows, we consider a buyer whose private value is v ∈V̅, and she perceives her opponent's value as a random variable z ∈ V̅ that follows the cumulative probability distribution G with density function g.

We consider the second price auction as the special case of the ascending-bid auction where the calling price is zero. Thus, our analysis focuses on the ascending-bid auction. Let B̅_r = [r, b̅] ⊆ B̅, with b̅ > v̅, and r ∈ B̅₀ = B̅ denote buyer's bid set at decision point when the calling price is r. Consider a continuous and continuously differentiable function b_r : V̅ → B̅_r, with b_r(0) = 0 to represent buyer's withdrawal bid strategy, (hereafter bid strategy for short) at a given decision point r. We focus on a symmetric equilibrium where both buyers employ the same bid strategy b_r(·), for all r ∈ B̅. Since such a symmetric equilibrium bid function must be strictly increasing in its argument [45,46], each buyer can construct the probability distribution of her opponent's bid from G via the inverse bid strategy function $b_r^{-1}:{\overline{B}}_r\to \overline{V}$. As the calling price r increases, the possibility of the opponent's bid being less than r is eliminated, so that each buyer updates G conditional on $z\in [b_r^{-1}(r),\overline{b}]$.

Suppose that at a given r, a buyer with value v makes a bid b_r (x) as if her value is x ∈ V̅. She expects that her opponent with value z will make a withdrawal bid b_r(z). For a given z, we can construct buyer i's deterministic utility U(b_r(x), b_r(z), r) based on the utility (4), where b_i,r, b_j,r, v_i, and v_j correspond to b_r (x), b_r (z), v, and z, respectively. Then the buyer's expected utility is obtained by taking expectation of U(b_r (x), b_r (z), r) with respect to random variable z, denoted by EU_z(x, r) ≡ E_z[U(b_r(x), b_r(z), r)]. The exact form of buyer's expected utility EU_z(x, r) is provided by (B2) in Appendix B-1. Buyer's decision problem at each r is to choose a bid strategy function b_r(x) that maximizes EU_z(x, r) when x = v, for every v ∈ V̅.

Following the same steps in the preceding subsection 2.1B, let us define symmetric interim equilibrium as follows.

Definition 4

Let a function $b_r^{\ast }:\overline{V}\to {\overline{B}}_r$ be continuous, continuously differentiabe, and strictly increasing bid strategy for a given r ∈ B̅. Then, the function $b_r^{\ast }(\cdot )$ generates a symmetric interim equilibrium at r if it maximizes EU_z ( x, r) ∣_x=v for all v ∈ V̅.

For each buyer, her ultimate withdrawal decision point is given by $r=b_r^{\ast }(v)$, where her optimal bid coincides with the current calling price.

Definition 5

Let a function b* : V̅ → B̅ be continuous, continuously differentiable, and strictly increasing bid strategy. Then, the function b*(·) generates a symmetric equilibrium in the ascending-bid auction if it maximizes ${E{U}_z(x,r)\mid }_{x=v=b{\ast }^{-1}(r)}$ for all v ∈ V̅.

Then the symmetric equilibrium bid function is sequentially rational if it also generates symmetric interim equilibrium at each r ∈ [0, b*⁻¹(v)] ⊂ B̅for all v ∈ V̅.

Proposition 4

There exists a unique symmetric interim equilibrium strategy b*(·) such that b*(v) = v, for all relevant r ∈ [0, b*⁻¹(v)] ⊂ B̅ and for all v ∈ V̅.

Proposition 4 asserts that the value-revealing bid strategy is a unique symmetric equilibrium bid strategy (but not a dominant strategy) in both the second price and ascending-bid auctions. The intuition behind Proposition 4 is simple. In the incomplete information environment, buyers are no longer aware of their relative value position, which is the driving force for their spiteful bids in the complete information case. This result contrasts with Morgan et al. [17] who predict overbidding by all buyers with the spite-without-reciprocity type. Moreover, in contrast to the complete information case considered in the previous section, the incomplete information case with buyers of the spite-with-reciprocity type does not generate any differences in bidding or winning prices between the second price and ascending-bid auctions.

3. Experimental Design

The theoretical model in the previous section generates a range of empirical implications that we evaluated in a controlled laboratory experiment. The experiment consisted of seven sessions of 12 subjects each (84 total subjects), all conducted with undergraduate econ major students at Shinshu University. Subjects bid in a series of two-bidder auctions with one item for sale. Motivated by the differing testable implications derived above, the principal treatment variables were the auction format (ascending-bid versus second price sealed-bid) and information conditions (complete versus incomplete). Both of these treatment variables were varied within sessions, and in four sessions all subjects bid in both formats and both information conditions. In the remaining three sessions subjects only bid in complete information, sealed-bid auctions. Subjects submitted bids for 6 to 10 consecutive periods within each treatment configuration.

A secondary treatment variable was the matching rule. This was also varied within sessions, so sometimes subjects bid against the same opponent for 6 to 10 periods, and at other times subjects bid against randomly-changing opponents every period. We included fixed pairings in some sessions because the multiple equilibria (cf Figures 1–4) may require some coordination. Fixed pairings make this coordination more plausible. The matching rule was common knowledge. The presentation order of both the principal and secondary treatment variables was varied across sessions to control for order effects.

In the complete information treatment, the two possible resale values for the two bidders were 700 and 800 yen. These two values were randomly assigned each period, and this was common knowledge. Therefore, after a bidder learned that her resale value was 800 yen, for example, she knew with certainty that the other bidder's resale value was 700 yen. In the incomplete information treatment, resale values were drawn independently for each bidder each period from the discrete uniform distribution between 500 and 800 yen. The uniform distribution is the most commonly-used distribution in the extensive literature on independent private value auctions (Kagel, [47]). This probability distribution was common knowledge, but individuals only learned their own value draw. Bids were constrained to 10-yen increments, but value draws could be any whole yen amount in the feasible range. In all ascending-bid auction treatments the clock price increased in 10-yen increments.

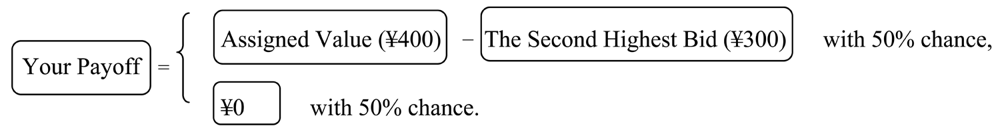
Subjects received the difference between their resale value and their price paid when they won the auction. The price was determined by the lowest bid or the first drop-out price, depending on the auction format, with the highest or the remaining bidder winning the auction. (Consistent with the theoretical model, ties were resolved randomly.) Subjects received written instructions to describe the auction rules and procedures, which they first read in silence before the experimenter read them aloud. The instructions included both equation and payoff table explanations describing the relationship between bidder actions, allocations, and payoffs. A translation of the instructions is shown in Appendix C. At the conclusion of the session subjects received their cumulative auction earnings in cash, along with a 1,000 yen show-up payment. Payments (including this show-up payment) averaged about 4,500 yen, and ranged between 1,590 and 10,788 yen. Sessions typically lasted about 150 min.

4. Experimental Results

4.1. Overview

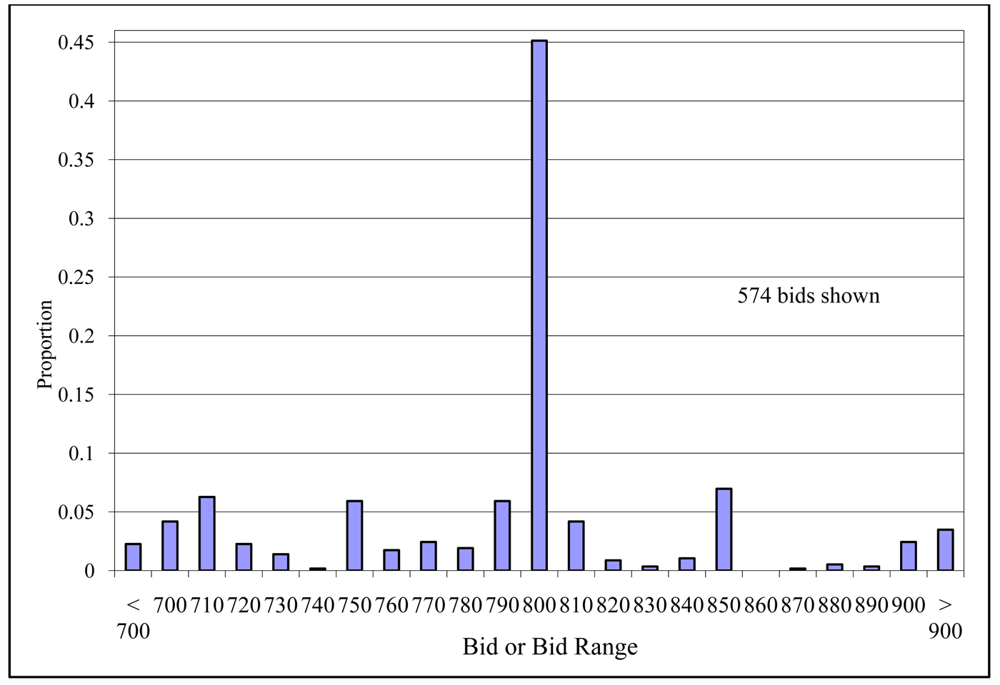
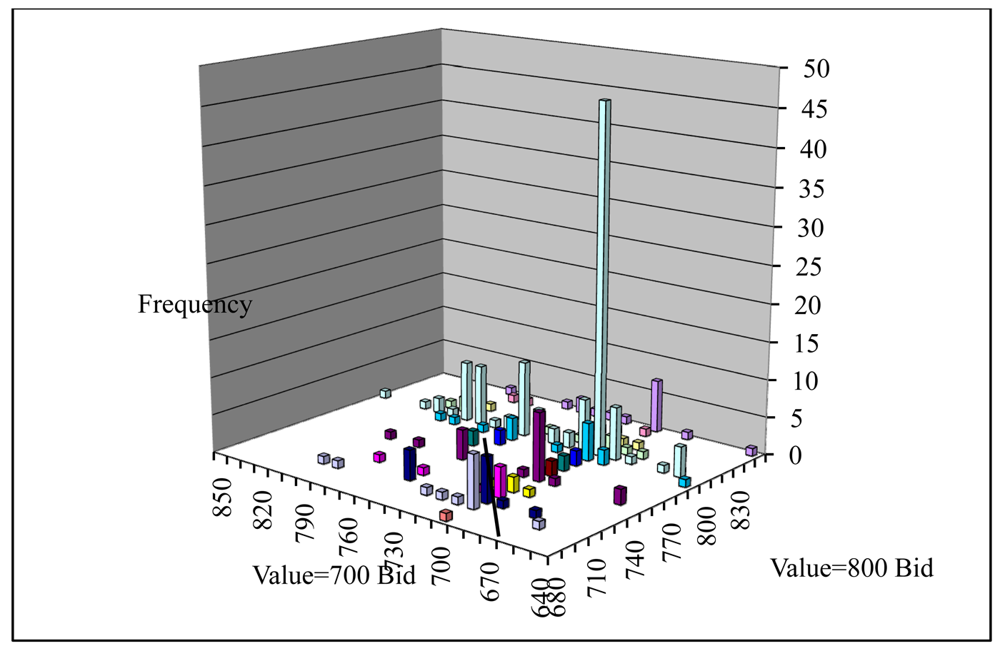
In order to orient the reader, we first summarize the data using a series of figures before turning to formal hypothesis testing. Recall that in the complete information environment, the valuations are either 700 or 800 yen. Figures 5 and 6 display the frequency distribution of bids for the low-value (700) and high-value (800) bidder, respectively [48]. In the ascending-bid auction, 30 of the 291 bids for the low value bidder are not observed directly, since the low-value bidder won the auction when the high-value bidder dropped out. These censored bids are at least as high as this drop-out price, so the minimum bid consistent with these prices (displayed on Figure 5) presents only the lower bound of the intended bid by this low-value bidder [49]. The statistical tests below account for this censoring.

In all panels of these figures, the modal bid equals the bidder's value. Overbidding by the low-value bidder, however, is pronounced in Figure 5. About one-half of all low-value bids exceed 700 (51 percent in the ascending-bid auction and 47 percent in the second-price sealed-bid auction). Conditional on overbidding, the figure suggests that more aggressive bids such as 750 and 790 are more common in the sealed-bid auction. Figure 6 indicates that underbidding is more common than overbidding for the high-value bidder in the sealed-bid auction.

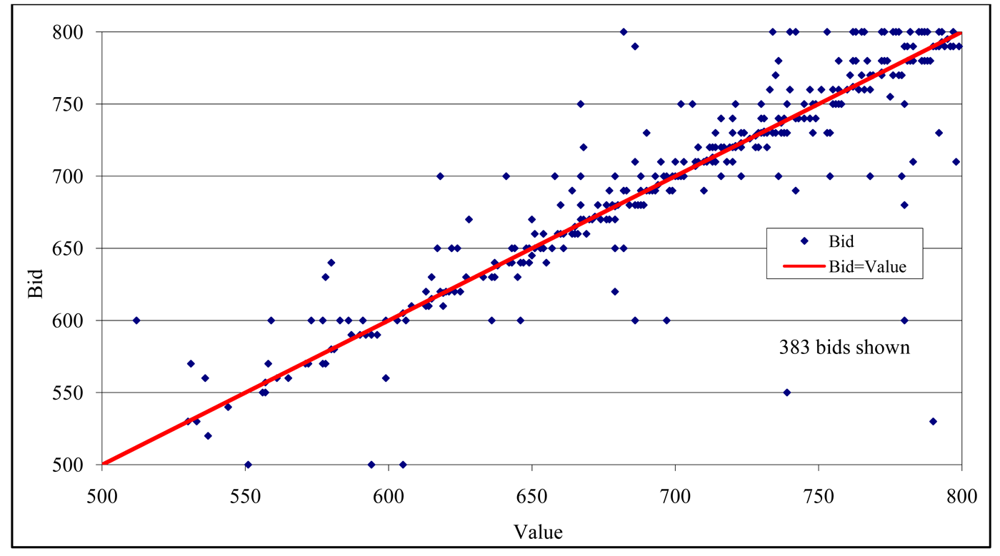
Figure 7 summarizes the bid combinations for the complete information sealed bid auctions in the treatment in which pairs of bidders are randomly re-assigned each period. The modal bid pair is on the value-revealing strategy equilibrium (700, 800), but other pairs are common. Most of the pairs lie to the right of the line drawn on the surface of this diagram. This line indicates where the low-value bid equals the high-value bid. Therefore, the high-value bidder nearly always wins the auction even though many bids deviate from the standard preference, dominant strategy equilibrium.

Figure 8 presents the time series path of bids for three example fixed pairs of bidders in this complete information sealed bid environment. Because of these fixed pairings, subjects could react directly to each other's bids in the previous periods. Some pairs (not shown on this figure) often bid repeatedly equal to their value, but many other pairs frequently changed their bids across rounds as illustrated by the three pairs in Figure 8. Typically, bid pairs remain below the Bid1 = Bid2 line that distinguishes the efficient and inefficient allocations. Pairs were also quite heterogeneous. For example, Pair 2 exhibited substantial underbidding by the high-value bidder (even leading to two cases where the low-value bidder won), whereas Pair 3 did not exhibit any such underbidding.

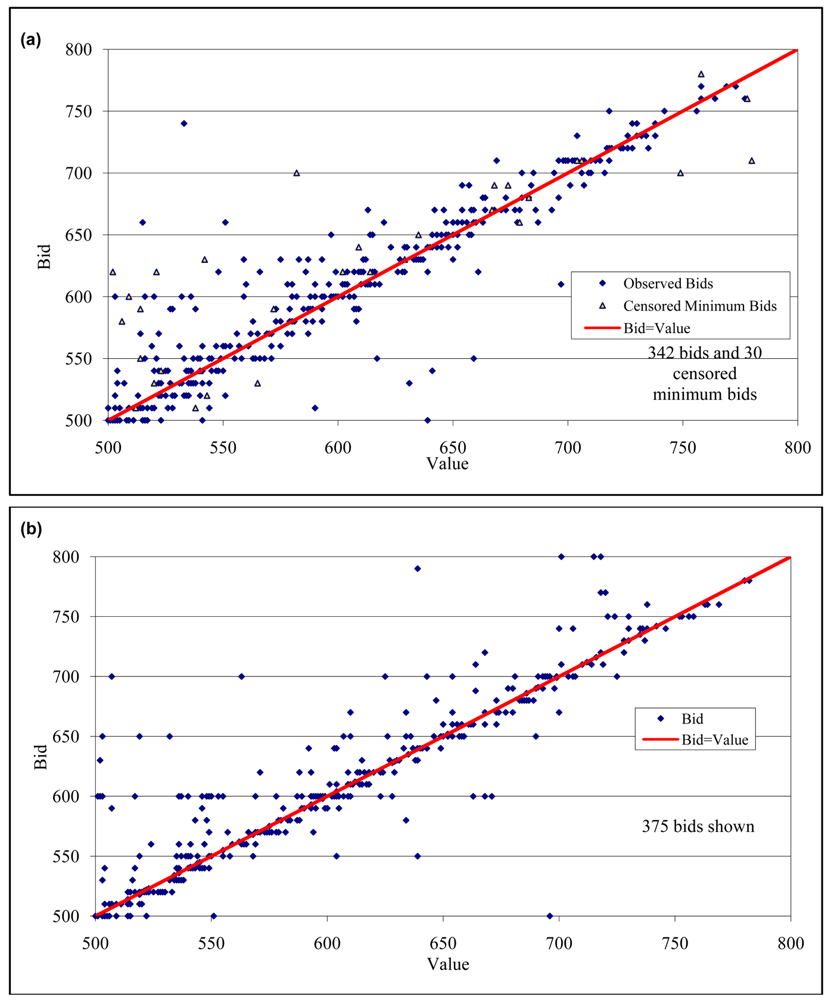
Figures 9 and 10 summarize the bids for the incomplete information environment. Recall that values are drawn from U[500,800]. The figures display bids separately for the buyer with the highest and the lowest value draws, although subjects only observed their own value draw and therefore did not know their ranking. For reference the figures indicate a solid line where bid = value. Again, we do not include the ascending-bid auction bids for the highest value bidder, since this bidder nearly always won the auction and so his bid is typically not observed.

Careful inspection of the figures should remind the reader that bids were constrained to 10-yen intervals, while value draws could correspond to any integer yen amount. Therefore, by design the bidders will typically not be able to bid exactly equal to their drawn value. Overbidding and underbidding appear about equally common on the figures, and on average bids are within one percent of value.

4.2. Hypothesis Testing: Complete Information Environment

This section reports tests of the hypotheses generated by the complete information reciprocity model presented in Section 2.1.

Hypothesis H1

In the complete information environment, (a) low-value bidders overbid relative to their values, and (b) overbidding is more common for low-value bidders than for high-value bidders.

Figure 5 above illustrates widespread overbidding by the low-value bidders. This indicates support for H1. To document the extent of this overbidding, we determined how frequently individuals bid above their value when they had the low value draw in the complete information environment, across both auction institutions. Thirty-nine of the 84 subjects (46 percent) submitted bids greater than their values in at least one-half of these cases. In other words, nearly half of the subjects submitted bids that exceeded their value at least half of the time when they knew that they had the lower value draw. By contrast, only eight out of 84 subjects (10 percent) submitted bids that were less than their values in at least half of these opportunities.

Table 1. Regression models of bid deviations from value and overbidding: Complete information environment, sealed bid auction.

Model	All Bidders		Frequent Over-Bidders
	1 (Random Effects GLS)	2 (Random Effects Probit)	3 (Random Effects GLS)	4 (Random Effects Probit)
Dependent Variable	Bid − Value	= 1 if Bid > Value	Bid − Value	= 1 if Bid > Value
Dummy Variable = 1 if	−35.03	1.30**	−6.12	1.54**
Lower Value	(47.56)	(0.12)	(38.10)	(0.15)
Dummy Variable = 1 for	5.40	0.22*	20.72	0.08
Fixed Pairings	(5.95)	(0.11)	(26.49)	(0.13)
1/period	94.99	−0.11	155.03	−0.26
	(85.23)	(0.19)	(170.91)	(0.23)
Intercept	−21.17	−1.61**	−28.47	−0.45*
	(27.18)	(0.22)	(46.29)	(0.20)
Observations	1150	1150	542	542
Number of Bidders	84	84	39	39
R2 or Log-likelihood	0.01	−470.0	0.01	−273.5

Notes: Standard errors (in parentheses) are based on a subjects random effects model and for the GLS regressions in columns 1 and 3 are calculated to be robust to unmodeled correlation of choices within clusters defined by sessions.^*denotes significantly different from zero at the five-percent level, and^**denotes significantly different from zero at the one-percent level.

reports results from several random-effects regression models to formally test part (b) of Hypothesis H1. These models include a dummy variable to indicate when the bid is submitted by the lower value bidder, and they also control for a time trend (using a standard form 1/period) and for the fixed versus random matching of bidding pairs. The estimates only use the sealed bid auction data, since as already noted the ascending price bids for the high-value bidder are heavily censored because this bidder typically wins.

The regression shown in column 1 determines whether bids relative to values are different between the low-value and the high-value bidders. The difference (Bid – Value) is actually lower for the low-value bidder, but this is mainly because of a small number of “throw-away” and overtly collusive bids, which were more common in the periods with fixed pairs of bidders. Although such bids were relatively rare, they add substantial variance and are a major reason that the regression coefficient estimate does not approach statistical significance. By contrast, the random effect probit model in column 2 is more robust to such outliers, and it indicates that the likelihood of overbidding is much higher for low-value bidders. Low-value bidders overbid 47 percent of the time, whereas high-value bidders overbid only 20 percent of the time. This difference is highly significant and is consistent with Hypothesis H1.

The theoretical model's predictions are based on agents who have spiteful preferences, which suggests that empirical results might be sharper when the analysis is focused more narrowly on those types of subjects. Therefore, columns 3 and 4 present estimates for the subset of subjects who bid above their value at least half the time when they had the low value draw. These 39 subjects represent roughly half the sample and their bids most clearly reveal spiteful preferences. Conclusions drawn for this spiteful subset of bidders are similar to those drawn for the entire sample.

Hypothesis H2

In the complete information environment, (a) low-value bidders bid higher in the second-price sealed-bid auction than in the ascending-bid auction, and (b) overbids (especially large overbids) are more common in the second-price sealed-bid auction than in the ascending-bid auction.

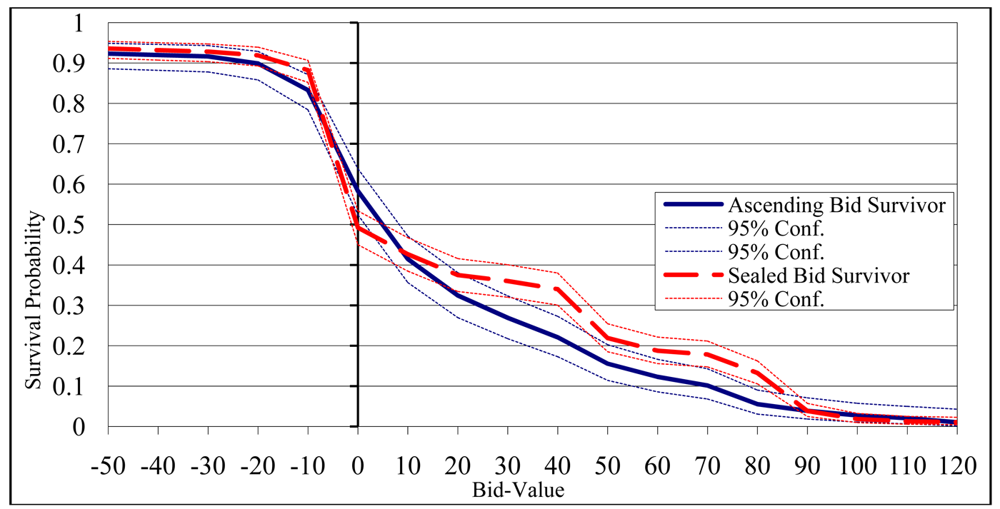
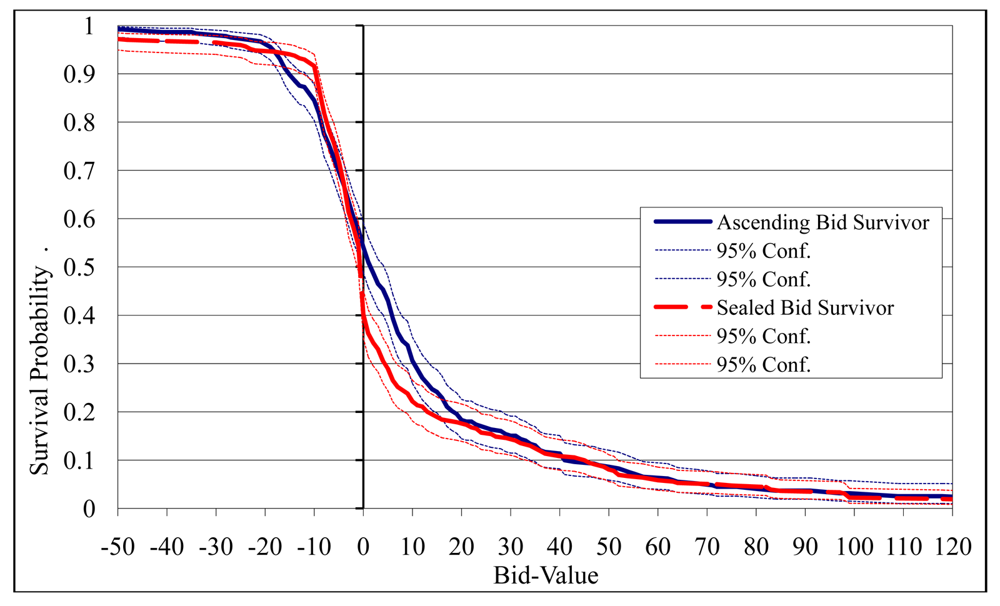
The figures and the summary statistics presented above provide some suggestive evidence in support of H2. For a formal statistical test, however, we must account for the censoring of the bids in the ascending-bid auction. Recall that for this institution we do not observe the bid of the winning bidder—only the price at which the other bidder drops out. This censoring occurs for 30 of the 305 (10 percent) of the low-value bidders' bids. We employ survival analysis to account for this censoring, where “failure” occurs when the rival bidder drops out. The approach we use accounts for differing censoring points since the rival bidder drops out at different prices in different periods.

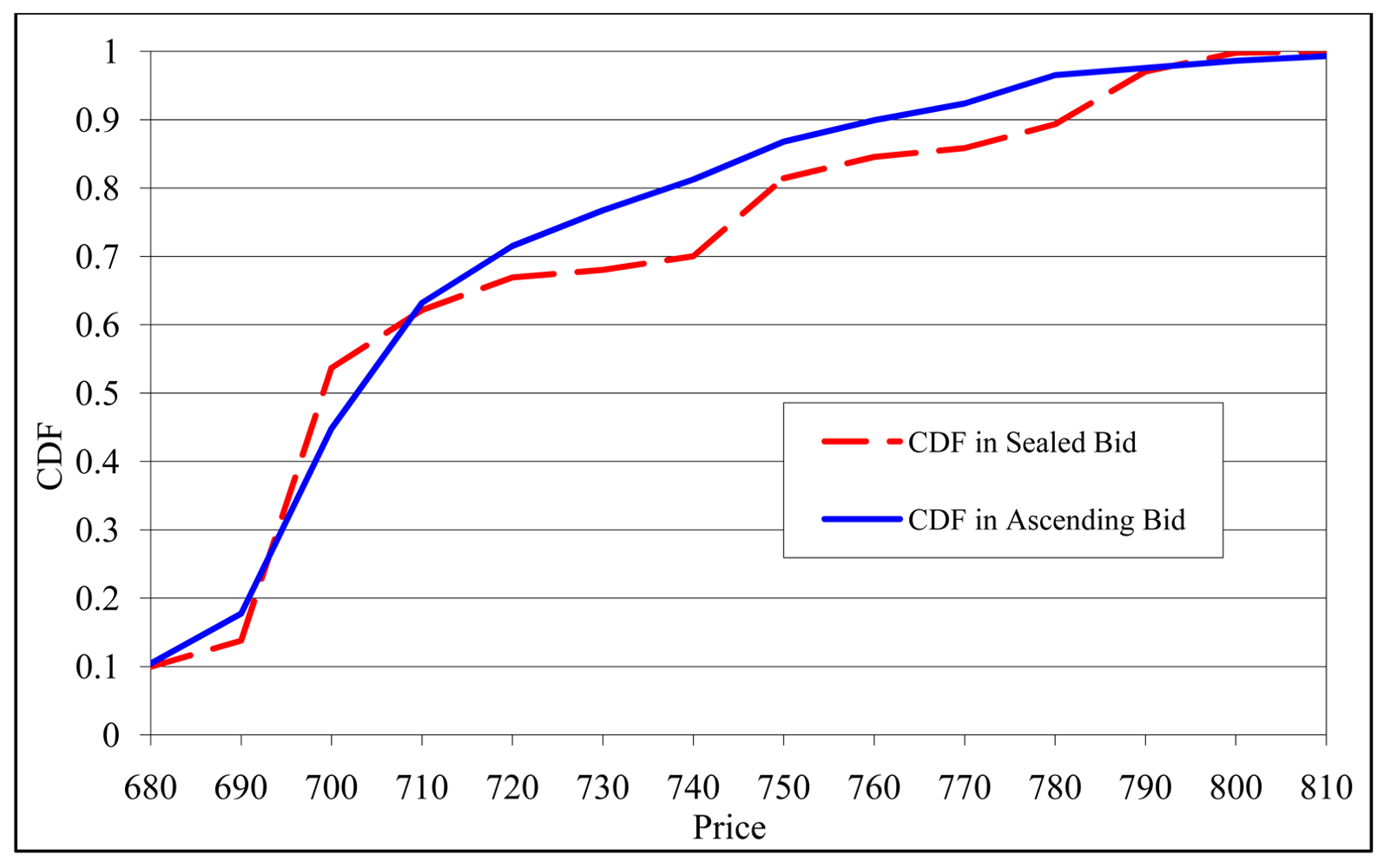
Figure 11 presents a comparison of the Kaplan-Meier nonparametric estimate of the survival function S(x) = Prob (bid > x) for the two auction forms for the low-value bidders (e.g., see Cameron and Trivedi [50], Chapter 17). The median bid for the ascending-bid auction estimated using this method is 710, compared to 700 for the sealed bid auction. Overbidding (defined as any bid > 700) occurs with probability 0.58 in the ascending-bid auction, and with probability 0.49 in the sealed bid auction. The bid of 700, however, is the only place where the survivor function is higher for the ascending-bid auction. This is due to the higher mode of 700 in the sealed bid auction (cf Figure 5).

For all other bids < 800, the survivor function estimates imply that the sealed bid auction has a higher probability of observing bids exceeding all particular bid prices that are higher than 700. For example, if we define large overbid as a bid greater than or equal to 750, large overbidding occurs with probability 0.22 in the ascending-bid auction, and with probability 0.34 in the sealed bid auction. A log-rank test rejects the null hypothesis that these survivor functions are equal ( ${\chi }_{1\text{d}.\text{f}}^2=7.69$ ; one-tailed p-value < 0.01). We therefore conclude that the data provide modest support Hypothesis H2, but only for the case of large overbids and not small overbids.

Since large overbids by low-value bidders are more common in the sealed-bid auction, a natural auxiliary hypothesis is that transaction prices are also higher in the sealed-bid auction:

Hypothesis H3

In the complete information environment, (a) transaction prices are higher in the second-price sealed-bid auction than in the ascending-bid auction, and (b) prices above 700 (especially greatly above 700) are more common in the second-price sealed-bid auction than in the ascending-bid auction.

Figure 12 indicates that the cumulative distributions of transaction prices for the two auction institutions are ordered consistent with Hypothesis H3, since the sealed-bid CDF is lower than the ascending-bid CDF for the critical range of prices between 710 and 790.

Table 2. Regression models of transaction prices and likelihood of high prices: Complete information environment.

Model	All Prices		Excluding Prices < 711
	1 (Random Effects GLS)	2 (Random Effects Probit)	3 (Random Effects GLS)	4 (Random Effects Probit)
Dependent Variable	Price	= 1 if Price > 740	Price	= 1 if Price > 740
Dummy Variable = 1 if	−16.73	0.04	11.36**	0.77**
Sealed-Bid Auction	(12.51)	(0.12)	(3.07)	(0.16)
Dummy Variable = 1	-28.73**	−0.05	−4.73	−0.17
for Fixed Pairings	(10.87)	(0.10)	(2.91)	(0.15)
1/period	27.14	−0.02	−3.98	−0.20
	(19.21)	(0.17)	(4.92)	(0.25)
Intercept	697.19**	−0.66**	754.09**	0.22
	(18.18)	(0.21)	(3.59)	(0.18)
Observations	887	887	312	312
Number of Sessions	7	7	7	7
R2 or Log-likelihood	0.01	−465.6	0.05	−178.7

Notes: Standard errors (in parentheses) are based on session random effects models.^*denotes significantly different from zero at the five-percent level, and^**denotes significantly different from zero at the one-percent level.

indicates, however, that when considering all prices the data fail to reject the hypothesis that prices are equal across institutions (model 1), or that high prices are equally likely in either auction institution (model 2). Many of the prices are in the range of 690 to 710, which occur when the low-value bidder adopts a value-revealing strategy. Therefore, in order to focus on periods in which the low-value bidder exhibits some spiteful behavior, columns 3 and 4 report these same models after excluding the prices that are less than 711. Within this subset of data, which represents 35 percent of the price observations in columns 1 and 2, column 3 shows that transaction prices are significantly higher (by 11 yen) in the sealed-bid auction compared to the ascending-bid auction. Column 4 shows that the estimated likelihood that prices within this subsample exceed 740 increases from 25 percent in the ascending-bid auction to 54 percent in the sealed-bid auction. We therefore conclude that the data support the price differences indicated by Hypothesis H3 only when excluding lower prices that arise from value-revealing bid strategies.

4.3. Hypothesis Testing: Incomplete Information Environment

Section 2.2 established that even with spiteful preferences, in the incomplete information (unknown values) environment a unique symmetric equilibrium strategy exists where each bidder bids at her own value. Figures 9 and 10 indicate that significant dispersion of bids occurs both above and below value in the incomplete information data, partly due to the restriction that bids must be in 10-yen increments. Nevertheless, the data do not reject the null hypothesis that a linear bid function fit on the incomplete information sealed bid data has an intercept of 0 and a slope of 1 ( ${\chi }_{2\text{d}.\text{f}}^2=1.02$ ; p-value = 0.60), consistent with the equilibrium model and much of the experimental literature on second price and ascending bid auctions with incomplete information. To test the model, however, we need to determine whether bids shift between the complete and incomplete information environments as predicted by spiteful preferences.

Hypothesis H4

(a) Low-value bidders bid higher and (b) high-value bidders bid lower in the complete information environment compared to the incomplete information environment.

Support for this hypothesis may be difficult to obtain since in the incomplete information environment subjects do not know when they have the low or high value draw. They may have reasonably confident beliefs when they have very low value draws near 500 or very high value draws near 800, but not when they have intermediate values in the range between 600 and 700.

Because bids are typically not observed for the higher value bidder in the ascending-bid auction, to test this hypothesis we consider only the sealed bid auction where all bids are observed. In order to make the two environments comparable, we normalize all bids by subtracting the associated value draw. We then regress this difference on a dummy variable for the complete information environment, using the same control variables as in the regressions reported above. To be consistent with Hypothesis H4, the dummy variable for the complete information environment should be positive for low-value bidders (H4a) and negative for high-value bidders (H4b). The results, shown in

Table 3. Regression models of bid deviations from value to compare the complete and incomplete information environments.

Model	All Bidders		Frequent Over-Bidders
	1 (Lower Value Bidders)	2 (Higher Value Bidders)	3 (Lower Value Bidders)	4 (Higher Value Bidders)
Dependent Variable	Bid − Value	Bid − Value	Bid − Value	Bid − Value
Dummy Variable = 1 if	−43.03	6.34	24.86*	27.86
Complete Info.	(40.96)	(14.48)	(12.07)	(29.76)
Environment
Dummy Variable = 1 for	−20.28	21.53	−27.16	51.78
Fixed Pairings	(17.24)	(12.32)	(18.27)	(42.97)
1/period	20.57	88.07	20.61	203.74
	(18.21)	(82.36)	(23.37)	(218.76)
Intercept	27.66	−34.26	15.41**	−90.53
	(20.02)	(36.82)	(4.40)	(101.01)
Observations	957	956	424	391
Number of Bidders	84	84	39	39
R2	0.00	0.01	0. 02	0.02

Notes: Standard errors (in parentheses) are based on a subjects random effects model and are calculated to be robust to unmodeled correlation of choices within clusters defined by sessions.^*denotes significantly different from zero at the five-percent level,^**denotes significantly different from zero at the one-percent level.

, indicate support for Hypothesis H4a but not for H4b, and the support is not strong because it only holds when restricting the analysis to the subsample of frequently overbidding subjects. Estimates are rather imprecise, in part because of a small number of low “throw-away” or collusive bids in the complete information environment noted earlier.

Another implication of the equilibrium result that bids should equal values in the incomplete information environment is that there should not be significant differences between bidding behavior for low and high value bidders.

Hypothesis H5

In the incomplete information environment, overbidding is not more common for low-value bidders than for high-value bidders.

This hypothesis is the counterpart of Hypothesis H1 (b), where for the complete information environment the hypothesis was that overbidding is more common for low-value bidders than for high-value bidders. Recall that Table 1 presented models of bid deviations and overbidding that partially supported Hypothesis H1 (b). The likelihood of overbidding is much higher for low-value bidders in the complete information environment, but the deviation between bid and value was not significantly different between low- and high-value bidders.

Table 4. Regression models of bid deviations from value and overbidding: Incomplete information environment, sealed bid auction.

Model	All Bidders		Frequent Over-Bidders
	1 (Random Effects GLS)	2 (Random Effects Probit)	3 (Random Effects GLS)	4 (Random Effects Probit)
Dependent Variable	Bid − Value	= 1 if Bid > Value	Bid − Value	= 1 if Bid > Value
Dummy Variable = 1 if	−4.30	−0.05	−13.69	−0.10
Lower Value	(6.70)	(0.13)	(7.25)	(0.20)
Dummy Variable = 1 for	−3.58	0.86**	−15.11	1.11**
Fixed Pairings	(5.40)	(0.13)	(13.10)	(0.20)
1/period	−2.30	−0.76**	11.07	−0.43
	(5.22)	(0.23)	(10.44)	(0.35)
Intercept	3.16	−0.85**	17.28*	0.05
	(3.36)	(0.30)	(6.99)	(0.35)
Observations	763	763	273	273
Number of Bidders	48	48	17	17
R2 or Log-likelihood	0.00	−325.8	0.02	−136.2

Notes: Standard errors (in parentheses) are based on a subjects random effects model and for the GLS regressions in columns 1 and 3 are calculated to be robust to unmodeled correlation of choices within clusters defined by sessions.^*denotes significantly different from zero at the five-percent level, and^**denotes significantly different from zero at the one-percent level.

reports the identical models for the incomplete information environment, but this time the research hypothesis (H5) corresponds to the statistical null hypothesis that the dummy variable for the lower value is not significantly different from zero [51]. Consistent with Hypothesis H5, we find no evidence that bidding behavior is different for the low- and high-value bidders.

The final hypothesis is the incomplete information counterpart to Hypothesis H2. Recall that with complete information, overbids by the low-value bidder are predicted to be larger in the second-price compared to ascending-bid auction. By contrast, with incomplete information there should be no systematic difference between the bids across auction institutions.

Hypothesis H6

In the incomplete information environment, (a) lower value bidders do not bid higher in the second-price sealed-bid auction than in the ascending-bid auction, and (b) overbids (especially large overbids) are not more common in the second-price sealed-bid auction than in the ascending-bid auction.

We test Hypothesis H6 in exactly the same way that we tested Hypothesis H2. To account for the bid censoring in the ascending-bid auction, we again employ survival analysis. In the incomplete information environment, this censoring occurs for 30 of the 372 (8 percent) of the low-value bidders' bids. Figure 13 presents a comparison of the Kaplan-Meier nonparametric estimate of the survival function for the two auction forms for the low-value bidders. The median bid for the ascending-bid auction estimated using this method is one yen above value, compared to one yen below value for the sealed bid auction. Overbidding (defined as any bid > value) occurs with probability 0.54 in the ascending-bid auction, and with probability 0.40 in the sealed bid auction. That is, similar to results in the experimental literature with incomplete information about rival values, bids are above and below value with approximately equal frequency. (Recall that bids exactly on values are not common in our environment due to the restriction that bids had to be in 10-yen increments.) There is virtually no evidence that the frequency of large overbids is different for the two auction institutions, and the survivor functions are essentially identical for all bids that are 20 or more yen greater than value. Moreover, a log-rank test fails to reject the null hypothesis that these survivor functions are equal ( ${\chi }_{1\text{d}.\text{f}}^2=2.03$ ; p-value = 0.15). We therefore conclude that the data support Hypothesis H6: Overbidding by low value bidders in the incomplete information environment is not different in the sealed-bid and ascending-bid auctions.

5. Conclusions

We have investigated bidding behavior in both complete and incomplete information environments for two-person second price sealed-bid auctions and ascending-bid auctions for a single indivisible object with independent private values. Our intention-based bidding model features individuals who are reciprocally spiteful. A lower value bidder may be spiteful in the sense that he receives a positive psychological payoff when he loses if he reduces the winners' payoff. A high-value bidder who faces a spiteful bidder's over-bidding would reciprocate by underbidding to increase the likelihood that the spiteful bidder wins and incurs a negative monetary payoff. The possibility of this underbidding causes such spiteful bidders to refrain from bold overbidding. Our theoretical analysis in the complete information environment indicates that the equilibrium bidding strategy differs from the Nash equilibrium strategy set generated without spite and reciprocity, in the following three respects. First, the equilibrium strategy set is much smaller and does not contain any inefficient outcomes. Second, although a strategy of “bidding at one's value” is no longer part of an equilibrium strategy profile, the equivalent outcome in which the lower value bidder bids at her own value and loses is one of the equilibrium outcomes. Third, the threat of reciprocal underbidding is more important in ascending-bid auctions than second price sealed-bid auctions, since a rising calling price directly reveals the spiteful intention of a low-value bidder. This leads to a lower equilibrium spiteful over-bidding in ascending-bid auctions, which implies an even smaller equilibrium set with lower price upper bound.

As summarized in

Table 5. Summary of theoretical predictions and hypotheses support.

	Complete Information				Incomplete Information
	Theoretical Prediction	Consistent with Hypothesis:			Theoretical Prediction	Consistent with Hypothesis:
		H1	H2	H3		H4	H5	H6
Conventional Model	S = A, b(v) = v	No	No	No	S = A, b(v) = v	No	Yes	Yes
Spite without Reciprocity	S = A, b(v) ≠ v	Yes	No	No	S = A, b(v) > v	No	Yes	Yes
Spite with Reciprocity	S = A, b(v) = v	Yes	Yes (for large overbids)	Yes (for prices > 710	S = A, b(v) = v	Yes (for spiteful low value bidders)	Yes	Yes

S: Bidding in a second price auctionA: Bidding in an ascending-bid auctionb(·): bid strategy

, our experimental results provide qualified support for the model's theoretical predictions. In the complete information setting, nearly half of the bids are consistent with spiteful overbidding. Bidders with lower private values are more prone to overbid in both auction formats, and large overbids and high prices are more common in the second price auctions than in the ascending-bid auctions. However, such systematic overbidding disappears when bidders' private values are unknown in the incomplete information setting. This is also consistent with the model, which predicts value-revealing bidding behavior as the unique symmetric Nash equilibrium, although this is not a dominant strategy equilibrium. Overall, results tend to be more consistent with the spite model that features reciprocity than the conventional or the spite-without-reciprocity models.

Subjects' decision-making seems to be different when they do or do not know each other's values. When they have complete information about all bidders' values, this allows them to evaluate their relative payoffs. A low-value bidder in our environment who bids 750, for example, knows that this bid will likely reduce the winning bidder's payoff by half relative to the payoff if all bids equal values. A bidder with the higher value can also perceive the spiteful intentions of her opponent's bid in the complete information environment. It is this spiteful intention that induces underbidding by the higher value bidder. This is the driving force behind our theoretical result that bidders make more timid overbids in the ascending-bid auction, because the rising calling price directly reveals the lower value bidder's spiteful intention. This can be interpreted as an additional evidence of negative reciprocity at work, but here in the context of an auction, consistent with negative reciprocity observed in the context of ultimatum and related games (e.g., see Charness and Rabin [28]).