Optimal Contest Design When Policing Damaging Behavior
Abstract
1. Introduction
2. Literature Review
3. A Contest with Limited Aggressiveness
3.1. Environment
3.2. Contestants’ Behavior in Equilibrium
- (a)
- , contestants exert more effort when the organizer sets a higher prize spread or tolerates more aggressiveness, and . However, a higher inspection rate reduces effort, .
- (b)
- , all contest instruments motivate contestants’ effort, , , and .
- (c)
- , a higher inspection rate or limit on aggressiveness increases contestants’ effort, and . However, a higher prize spread reduces effort,
4. Optimal Contest Design
4.1. Organizer’s Problem
- (a)
- ,
- (b)
- , and
- (c)
- .
4.2. Simulation
5. Valueless Aggressiveness
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Proofs
Appendix B. Comparative Statics When δ Follows a Uniform Dist
Appendix C. The N-Player Game
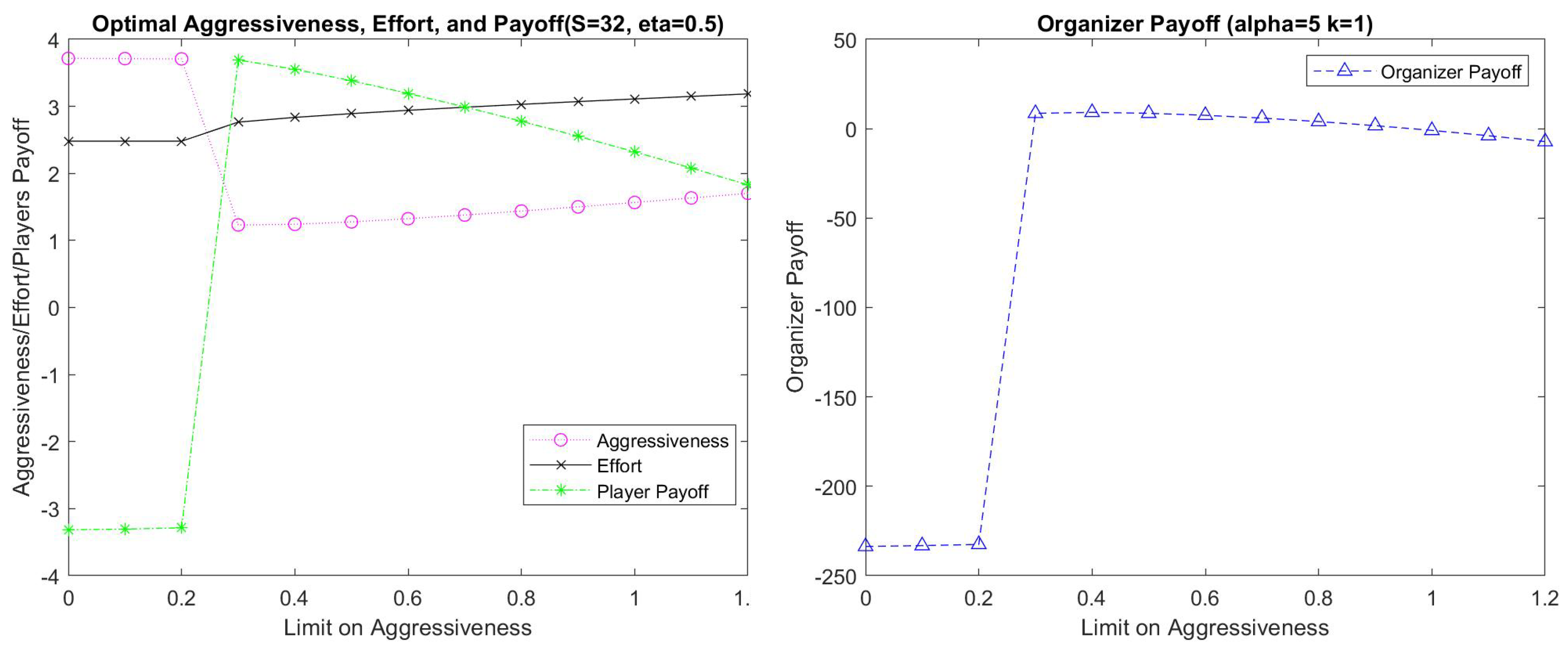
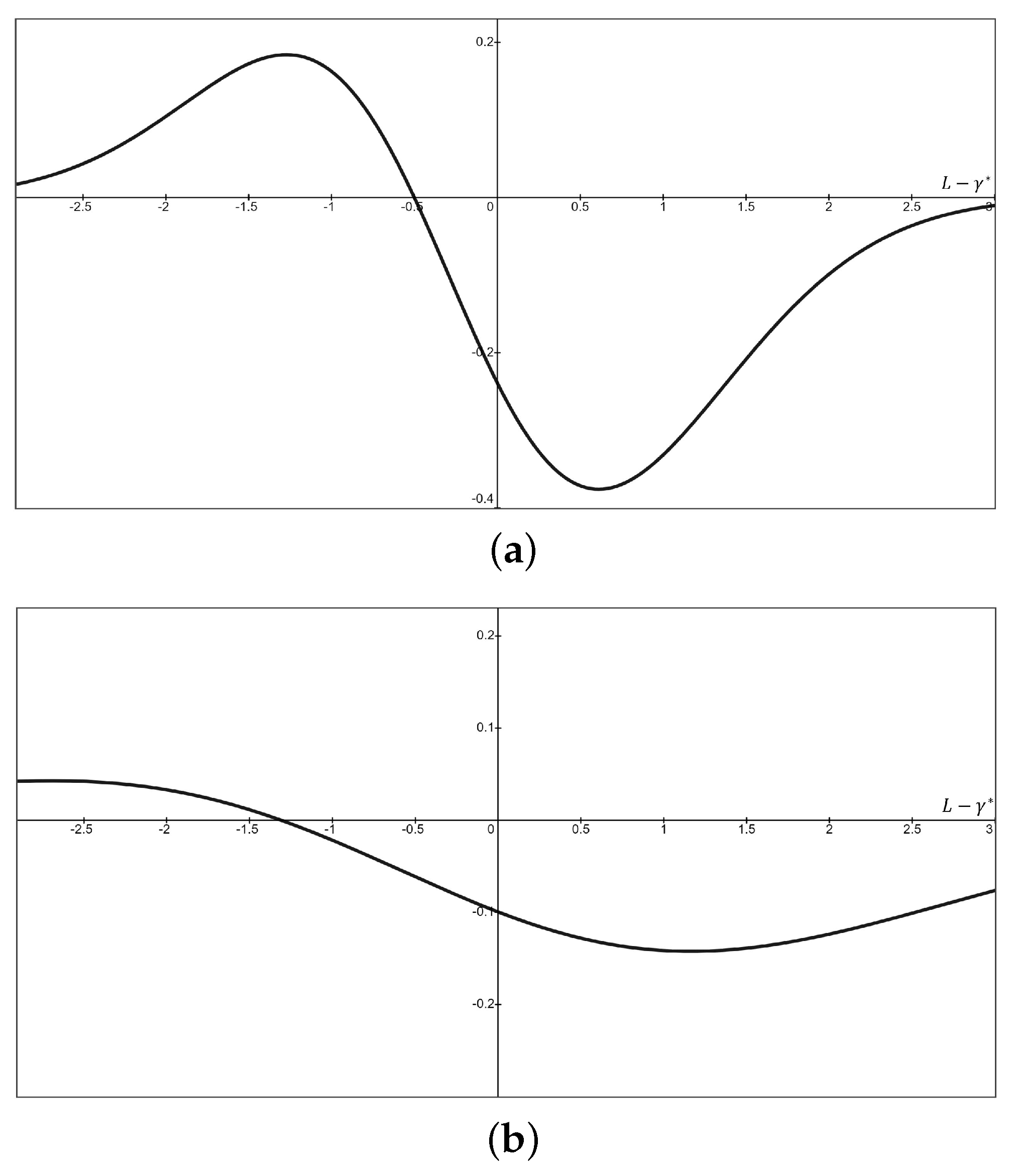
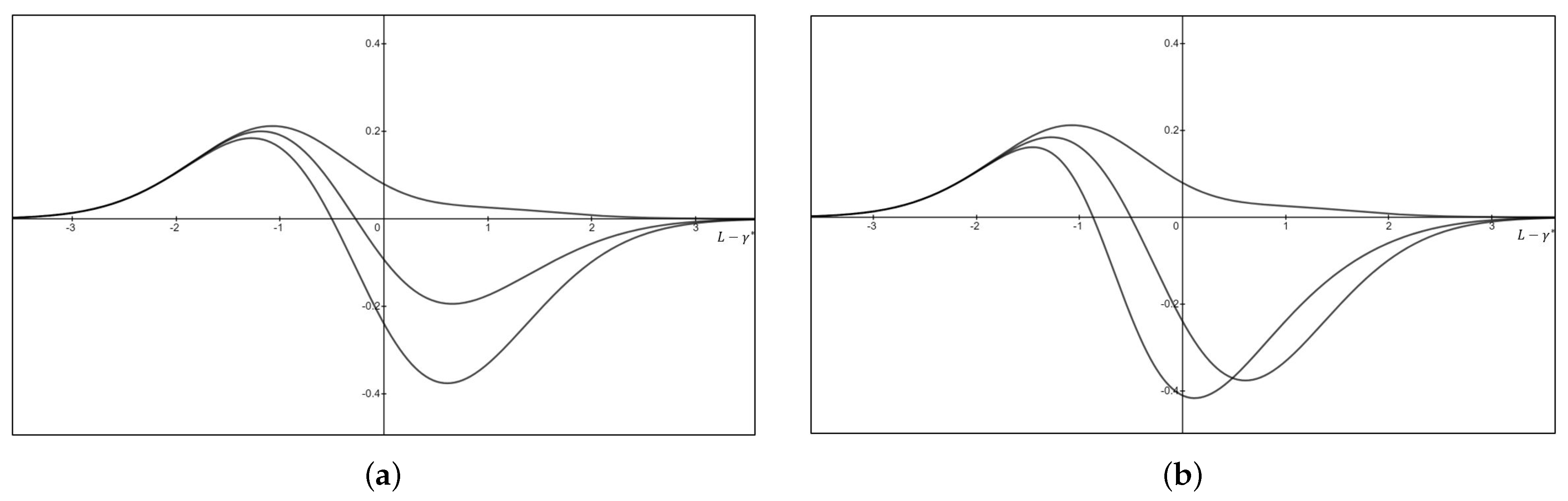
| 1 | In Proposition 2, the comparisons between how fast the marginal cost of aggressive actions changes with such actions, , and how quickly the marginal benefit changes with it, , reflect the incentive for aggressive actions. |
| 2 | This is contrary to the notion that one should utilize a tool less often when it is less precise. |
| 3 | is sufficiently large so that contestants always participate in the contest. However, the organizer will decide whether to hold the contest. |
| 4 | A common assumption in the tournament. |
| 5 | guarantees the local maximum because the second order condition for is less than 0 and the Hessian determinant for is greater than 0 (See the proof for Proposition 2 in Appendix: proofs). This condition indicates that the marginal cost of aggressiveness increases faster than the marginal benefit of such actions to contestants. We later denote as A. |
| 6 | Gilpatric and Reiser [2] find that if the enforcement prohibiting misconduct cannot effectively deter such conduct, that enforcement is not optimal. Their finding is analogous to findings about “marginal deterrence” in the enforcement literature. The notion has been articulated by Marchese di Beccaria [14], Shackleton [15], and Bentham [16] as “to induce a man to choose always the least mischievous of two offenses; therefore, where two offenses come in competition, the punishment for the greater offense must be sufficient to induce a man to prefer the less”. Stigler [17] originated the term marginal deterrence, which was later developed by Wilde [18], Mookherjee and Png [19], Shavell [20], and Friedman and Sjostrom [21]. They studied conditions under which marginal deterrence requires gradual penalties. Mookherjee and Png [22] extend the model to a general setting in which the activity level is a continuous variable, and individuals derive heterogeneous benefits. These researchers show that it is optimal to establish marginal expected penalties everywhere less than the marginal harm. |
| 7 | The corner solutions such as and non-interior solutions such as S tends to infinity do not contribute much to our contest design, thus, we focus on the conditions that can produce interior solutions. |
| 8 | If we add more digits to , , or , can be improved and falls under . For example, when , , and , and . The same issue occurs when . |
References
- Lazear, E.P. Pay equality and industrial politics. J. Political Econ. 1989, 97, 561–580. [Google Scholar] [CrossRef]
- Gilpatric, S.M.; Reiser, C.M. Why Zero Tolerance of Misconduct Is Undesirable in Contests. Econ. Inq. 2017, 55, 1145–1160. [Google Scholar] [CrossRef]
- Berentsen, A. The economics of doping. Eur. J. Political Econ. 2002, 18, 109–127. [Google Scholar] [CrossRef]
- Haugen, K.K. The performance-enhancing drug game. J. Sports Econ. 2004, 5, 67–86. [Google Scholar] [CrossRef]
- Konrad, K.A. Tournaments and Multiple Productive Inputs: The Case of Performance Enhancing Drugs; Technical report; IZA Discussion Papers: Bonn, Germany, 2005. [Google Scholar]
- Curry, P.A.; Mongrain, S. Deterrence in rank-order tournaments. Rev. Law Econ. 2009, 5, 723–740. [Google Scholar] [CrossRef]
- Kräkel, M. Doping and cheating in contest-like situations. Eur. J. Political Econ. 2007, 23, 988–1006. [Google Scholar] [CrossRef]
- Gilpatric, S.M. Cheating in contests. Econ. Inq. 2011, 49, 1042–1053. [Google Scholar] [CrossRef]
- Mohan, V.; Hazari, B. Cheating in contests: Anti-doping regulatory problems in sport. J. Sports Econ. 2016, 17, 736–747. [Google Scholar] [CrossRef]
- Music, K. The undesirable consequences of doping regulations: Why stricter efforts might strengthen doping incentives. J. Sports Econ. 2020, 21, 281–303. [Google Scholar] [CrossRef]
- Becker, G.S. Crime and punishment: An economic approach. J. Political Econ. 1968, 76, 169–217. [Google Scholar] [CrossRef]
- Ehrlich, I. The deterrent effect of criminal law enforcement. J. Leg. Stud. 1972, 1, 259–276. [Google Scholar] [CrossRef]
- Polinsky, A.M.; Shavell, S. The economic theory of public enforcement of law. J. Econ. Lit. 2000, 38, 45–76. [Google Scholar] [CrossRef]
- Marchese di Beccaria, C. An Essay on Crimes and Punishments; Newbery, E., Ed.; Available online: https://archive.org/details/essayoncrimespun00becc/page/86/mode/2up (accessed on 2 May 2023).
- Shackleton, R. The Spirit of Laws; Dodesley, P., Ed.; Printed for J. Almon, opposite Burlington-House, Piccadilly: London, UK, 1767; Available online: https://catalog.hathitrust.org/Record/008603896 (accessed on 2 May 2023).
- Bentham, J. The Collected Works of Jeremy Bentham: An Introduction to the Principles of Morals and Legislation; Clarendon Press: Oxford, UK, 1996. [Google Scholar]
- Stigler, G.J. The optimum enforcement of laws. J. Political Econ. 1970, 78, 526–536. [Google Scholar] [CrossRef]
- Wilde, L.L. Criminal choice, nonmonetary sanctions, and marginal deterrence: A normative analysis. Int. Rev. Law Econ. 1992, 12, 333–344. [Google Scholar] [CrossRef]
- Mookherjee, D.; Png, I.P. Monitoring vis-a-vis Investigation in Enforcement of Law. Am. Econ. Rev. 1992, 3, 556–565. [Google Scholar]
- Shavell, S. A note on marginal deterrence. Int. Rev. Law Econ. 1992, 12, 345–355. [Google Scholar] [CrossRef]
- Friedman, D.; Sjostrom, W. Hanged for a sheep: The economics of marginal deterrence. J. Leg. Stud. 1993, 22, 345–366. [Google Scholar] [CrossRef]
- Mookherjee, D.; Png, I.P. Marginal deterrence in enforcement of law. J. Political Econ. 1994, 102, 1039–1066. [Google Scholar] [CrossRef]
| Case (a) | |
| (10) | |
| (11) | |
| (12) | |
| Case (b) | |
| (10) | |
| (11) | |
| (12) | |
| Case (c) | |
| (10) | |
| (11) | |
| (12) |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | |
|---|---|---|---|---|---|---|---|---|---|---|
| 0.7 | 1.5 | 2.2 | 18 | 23.9 | 29 | 34 | 38.4 | 42.4 | 48.8 | |
| 0 | 0 | 0 | 0.53 | 0.54 | 0.54 | 0.54 | 0.54 | 0.54 | 0.544 | |
| any | any | any | 0.5 | 0.6 | 0.8 | 1 | 1.2 | 1.3 | 1.44 | |
| 0.4889 | 1.9602 | 4.4116 | 8.4794 | 16.2553 | 25.7818 | 37.0398 | 50.0381 | 64.6665 | 81.1578 | |
| 0.8667 | 3.4667 | 7.8000 | 13.8667 | 21.6667 | 31.2000 | 42.4667 | 55.4667 | 70.2000 | 86.6667 | |
| 0.1862 | 0.3989 | 0.5851 | 2.5428 | 3.2953 | 3.9822 | 4.6538 | 5.2652 | 5.7122 | 6.4603 | |
| 0.6667 | 1.3333 | 2.0000 | 2.6667 | 3.3333 | 4.0000 | 4.6667 | 5.3333 | 6.0000 | 6.6667 | |
| 0.2793 | 0.5984 | 0.8777 | 0.9134 | 1.0429 | 1.2596 | 1.473 | 1.6657 | 1.843 | 2.0317 | |
| 0.2 | 0.4 | 0.6 | 0.8 | 1 | 1.2 | 1.4 | 1.6 | 1.8 | 2 | |
| −0.4134 | −0.4429 | −0.4596 | −0.473 | −0.4657 | −0.5438 | −0.5817 |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | |
|---|---|---|---|---|---|---|---|---|---|---|
| 0.7 | 1.5 | 2.2 | 2.9 | 3.7 | 27.2 | 33.5 | 38.4 | 42.4 | 46.7 | |
| 0 | 0 | 0 | 0 | 0 | 0.53 | 0.54 | 0.54 | 0.54 | 0.54 | |
| any | any | any | any | any | 0.9 | 1 | 1.2 | 1.4 | 1.8 | |
| 0.4889 | 1.9602 | 4.4116 | 7.8410 | 12.2547 | 20.4111 | 31.6450 | 44.6381 | 59.2665 | 75.7454 | |
| 0.8667 | 3.4667 | 7.8000 | 13.8667 | 21.6667 | 31.2000 | 42.4667 | 55.4667 | 70.2000 | 86.6667 | |
| 0.1862 | 0.3989 | 0.5851 | 0.7713 | 0.9841 | 3.7992 | 4.6039 | 5.2652 | 5.7122 | 6.5964 | |
| 0.6667 | 1.3333 | 2.0000 | 2.6667 | 3.3333 | 4.0000 | 4.6667 | 5.3333 | 6.0000 | 6.6667 | |
| 0.2793 | 0.5984 | 0.8777 | 1.1569 | 1.4761 | 1.3603 | 1.4562 | 1.6657 | 1.8430 | 2.1509 | |
| 0.2 | 0.4 | 0.6 | 0.8 | 1 | 1.2 | 1.4 | 1.6 | 1.8 | 2 | |
| −0.4603 | −0.4562 | −0.4657 | −0.443 | −0.3509 |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | |
|---|---|---|---|---|---|---|---|---|---|---|
| 0.7 | 1.5 | 2.2 | 2.9 | 35.7 | 43.7 | 51.1 | 60.3 | 68.29 | 75.92 | |
| 0 | 0 | 0 | 0 | 0.72 | 0.72 | 0.72 | 0.725 | 0.726 | 0.726 | |
| any | any | any | any | 0 | 0.1 | 0.3 | 0.45 | 0.63 | 0.83 | |
| 0.4889 | 1.9602 | 4.4116 | 7.841 | 14.4104 | 23.9275 | 35.1668 | 48.2043 | 62.9355 | 79.4011 | |
| 0.8667 | 3.4667 | 7.8000 | 13.8667 | 21.6667 | 31.2000 | 42.4667 | 55.4667 | 70.2000 | 86.6667 | |
| 0.1862 | 0.3989 | 0.5851 | 0.7713 | 3.2424 | 3.8824 | 4.5252 | 5.2911 | 5.9729 | 6.6364 | |
| 0.6667 | 1.3333 | 2.0000 | 2.6667 | 3.3333 | 4.0000 | 4.6667 | 5.3333 | 6.0000 | 6.6667 | |
| 0.2793 | 0.5984 | 0.8777 | 1.1569 | 1.0936 | 1.3018 | 1.5182 | 1.6441 | 1.8262 | 2.0291 | |
| 0.2 | 0.4 | 0.6 | 0.8 | 1 | 1.2 | 1.4 | 1.6 | 1.8 | 2 | |
| −1.0936 | −1.2018 | −1.2182 | −1.1941 | −1.1962 | −1.1991 |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | |
|---|---|---|---|---|---|---|---|---|---|---|
| 0.7 | 1.5 | 2.2 | 2.9 | 3.7 | 4.4 | 51.1 | 57.7 | 65.1 | 75.3 | |
| 0 | 0 | 0 | 0 | 0 | 0 | 0.72 | 0.72 | 0.72 | 0.725 | |
| any | any | any | any | any | any | 0.3 | 0.6 | 0.8 | 0.86 | |
| 0.4889 | 1.9602 | 4.4116 | 7.841 | 12.2547 | 17.6466 | 27.9668 | 40.9348 | 55.6357 | 72.1473 | |
| 0.8667 | 3.4667 | 7.8000 | 13.8667 | 21.6667 | 31.2000 | 42.4667 | 55.4667 | 70.2000 | 86.6667 | |
| 0.1862 | 0.3989 | 0.5851 | 0.7713 | 0.9841 | 1.1702 | 4.5252 | 5.1884 | 5.8353 | 6.6083 | |
| 0.6667 | 1.3333 | 2.0000 | 2.6667 | 3.3333 | 4.0000 | 4.6667 | 5.3333 | 6.0000 | 6.6667 | |
| 0.2793 | 0.5984 | 0.8777 | 1.1569 | 1.4761 | 1.7553 | 1.5182 | 1.7417 | 1.9572 | 2.0534 | |
| 0.2 | 0.4 | 0.6 | 0.8 | 1 | 1.2 | 1.4 | 1.6 | 1.8 | 2 | |
| −1.2182 | −1.1417 | −1.1572 | −1.1934 |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | |
|---|---|---|---|---|---|---|---|---|---|---|
| 0.3 | 0.6 | 0.9 | 20 | 25.2 | 30.4 | 35.6 | 40.8 | 45.9 | 51.16 | |
| 0 | 0 | 0 | 0.608 | 0.612 | 0.617 | 0.619 | 0.620 | 0.622 | 0.623 | |
| any | any | any | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| 0.0784 | 0.3136 | 0.7057 | 4.3622 | 10.329 | 17.6414 | 26.2936 | 36.2829 | 47.6083 | 60.2687 | |
| 0.6667 | 2.6667 | 6.0000 | 10.6667 | 16.6667 | 24.0000 | 32.6667 | 42.6667 | 54.0000 | 66.6667 | |
| 0.0798 | 0.1596 | 0.2394 | 2.6454 | 3.3174 | 3.9894 | 4.6617 | 5.3328 | 5.9957 | 6.6642 | |
| 0.6667 | 1.3333 | 2.0000 | 2.6667 | 3.3333 | 4.0000 | 4.6667 | 5.3333 | 6.0000 | 6.6667 | |
| 0.1197 | 0.2394 | 0.3590 | 0.2116 | 0.1987 | 0.1941 | 0.1913 | 0.1917 | 0.1853 | 0.1833 | |
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| −0.2116 | −0.1987 | −0.1941 | −0.1913 | −0.1917 | −0.1853 | −0.1833 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gilpatric, S.M.; Hong, Y. Optimal Contest Design When Policing Damaging Behavior. Games 2023, 14, 48. https://doi.org/10.3390/g14030048
Gilpatric SM, Hong Y. Optimal Contest Design When Policing Damaging Behavior. Games. 2023; 14(3):48. https://doi.org/10.3390/g14030048
Chicago/Turabian StyleGilpatric, Scott M., and Ye Hong. 2023. "Optimal Contest Design When Policing Damaging Behavior" Games 14, no. 3: 48. https://doi.org/10.3390/g14030048
APA StyleGilpatric, S. M., & Hong, Y. (2023). Optimal Contest Design When Policing Damaging Behavior. Games, 14(3), 48. https://doi.org/10.3390/g14030048






