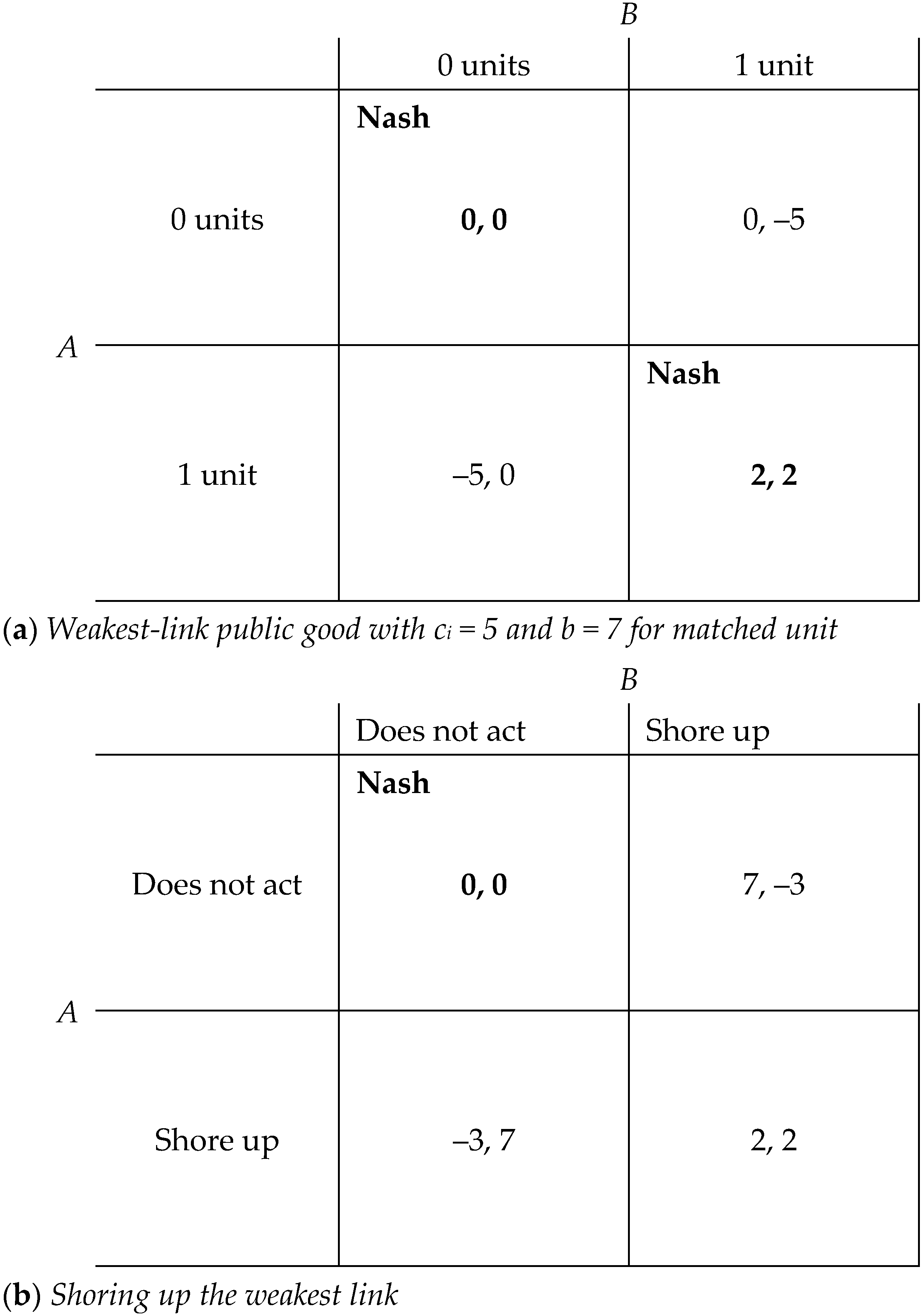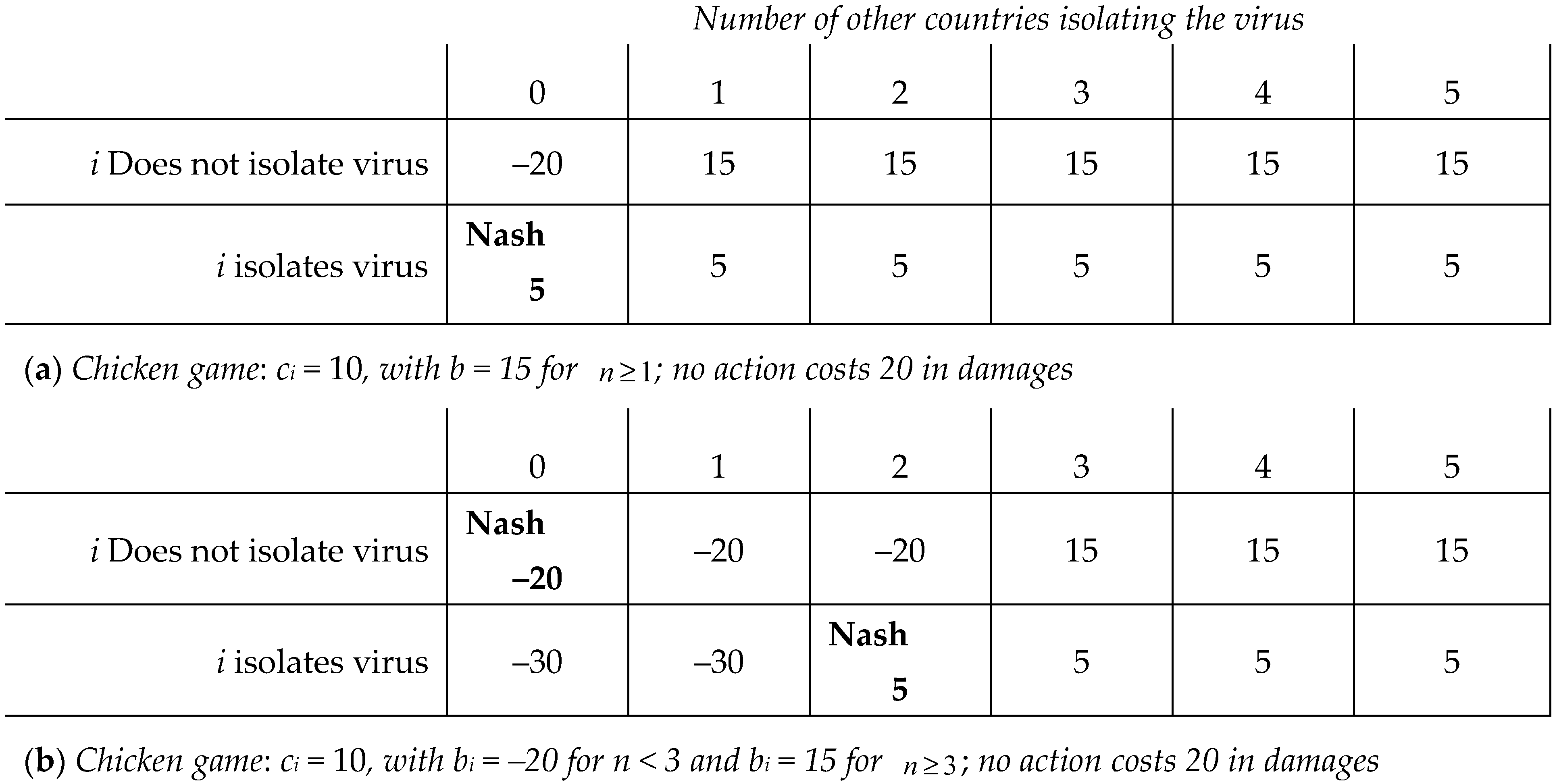COVID-19 Activities: Publicness and Strategic Concerns

Abstract

This paper considers the diverse public good characteristics of COVID-19 activities along with their strategic implications. The underlying aggregator technologies, which relate individual contributions to the amount consumed, affect the prognosis for the supply of COVID-related activities. Weakest-link activities assume a particularly pivotal role in curbing the spread of COVID-19. For instance, the propagation of COVID-19 through new strains is disproportionately influenced by those countries with the smallest vaccination rates or least isolation actions. Diverse income distribution among at-risk countries raises the need to “shore up” weakest-link countries’ provision to lift global supply. Generally, shoring-up actions result in a Prisoner’s Dilemma with unfavorable collective action prospects. As the number of countries requiring shoring up increases, the less favorable is the prospect for addressing provision shortfalls. Also, as the number of capable countries to do the shoring up increases, the prospect for successful action diminishes. The paper also examines the strategic implications of other aggregators—e.g., best shot and better shot—associated with COVID-inhibiting actions. To address best-shot anti-COVID actions, countries must pool or coordinate actions to meet a threshold. A host of institutions—e.g., the World Health Organization or public-private partnerships—can facilitate shoring-up weakest-link activities or coordinating best-shot actions.

1. Introduction

Global pandemics are not new to the world. The Black Death during the mid-1300s decimated Europe, wiping out a third of its population (Zacher [1]). Beginning in spring 1918 toward the end of World War I, the Spanish flu killed over 50 million worldwide and infected one-third of the global population (Centers for Disease Control and Prevention (CDC) [2]).1 The flu’s death toll far eclipsed that of World War I, estimated to have killed 15 to 22 million ([1]; Hobson [3]). The spread of the Black Death was bolstered by Asian-European trade growth stemming from the Silk Road and associated sea routes, while the diffusion of Spanish flu was augmented by trade and immigration growth responding to the end of World War I [1]. Like those two earlier pandemics, COVID-19 transmission was fostered by international transportation and exchange.

COVID-19 infections are believed to have originated in Wuhan, China, in December 2019 at the Huanan Seafood Wholesale Market (Christensen [4]). The rapid dissemination of infections from the Wuhan cluster was propelled by globalization and high-speed commercial air travel. Enhanced urbanization and its high population density further sped the diffusion of the virus (Connolly [5]; Eichenberger et al. [6]; Rasul [7]). The growing interconnectedness among population centers today increased the virus dissemination among neighboring cities and regions. By 8 December 2022, global infection reached 651.7 million cases with 6.65 million deaths (Worldometers [8]).

COVID-protection measures created huge economic consequences in the world’s globally integrated economies ([7]; Caggiano et al. [9]; Susskind and Vines [10]; Walmsley et al. [11]). Prophylactic efforts to stem the spread of COVID-19, such as lockdowns and quarantines, curbed production and commerce, resulting in reduced national income, smaller economic growth, and greater unemployment. In the United States, the unemployment rate rose dramatically to 14.7% in April 2020 with gross domestic product (GDP) falling by almost 32% in the second quarter of 2020 before rebounding during the second half of 2020 (Alpert [12]). The unprecedented second-quarter economic rebound in the United States was fueled by the Federal Reserve’s reductions in interest rates and the US government’s large fiscal stimulus packages. The most noteworthy of which was the $1.9 trillion American Rescue Plan Act (ARPA), signed by President Biden in March 2021 [12]. ARPA followed other expansionary executive orders and legislation during 2020. Similar employment and GDP reductions characterized the G20 economies between February 2020, marking the start of lockdowns, and April 2020, which resulted in a decline in industrial production of around 28% (International Labour Organisation and the Organisation of Economic Co-operation and Development [13]).

The purpose of the current paper is to investigate COVID-19 activities as public goods—e.g., achieving herd immunity, testing population members, tracking COVID-19 infections, imposing quarantines, developing an effective COVID-19 vaccine, isolating new COVID strains, instituting lockdowns, and observing social distancing [14,15,16,17,18]2—in order to identify their prognoses for success. In addition to nonrival and nonexcludable benefits, past studies of health-related activities note differences in their “aggregator technologies”, which indicate how individual contributions determine the overall quantities of the public good activities available for consumption (Buchholz and Sandler [19]; Hirshleifer [20]; Sandler and Arce [21]; Sonntag [22]). For instance, a best-shot activity equates the maximum contribution of the individual suppliers to the available consumption amount for everyone. Aggregator technologies influence how the underlying game differs among contributors, leading to essential implications for provision success or failure. Moreover, the associated game affects public policy recommendations regarding such things as income redistribution policy among contributors, the need for novel participants (e.g., nongovernment organizations (NGOs), multilateral organizations, global health networks, or public-private partnerships), and the required efforts to bolster provision efficiency. Some COVID-related activities may fare better than others owing to their underlying aggregator and implied strategic structure. Weakest-link COVID-related activities, whose overall level equals that of the smallest contribution, do not encourage free riding or relying on the provision of others because to contribute nothing means that the available quantity for consumption is then zero. For those who can afford to contribute and who values the activity, there is no rationale for free riding. If, however, a potential contributor or country is income-challenged, income redistribution to that country may be required to raise its contribution and achieve an acceptable consumption level of the activity for all countries. Alternatively, a better-endowed contributor can transfer the activity in-kind to the income-challenged country to lift the available public good level (Vicary [23]; Vicary and Sandler [24]). Such income or in-kind transfers “shore up” weakest-link contributors and, as shown later, can reintroduce free-riding incentives among rich contributors’ efforts to address provision shortfalls. The shoring up can be assisted by novel organizations or agents at the international level (e.g., the World Health Organization (WHO)) to raise or coordinate provision. Other aggregators confront diverse provision concerns as analyzed below.

Unlike the current study, the earlier literature [14,15,16,17,18,19,20,21,22] did not focus on how the underlying aggregators of COVID-related activities influenced the prospects for successful provision. For instance, the need to shore up weakest-link anti-COVID actions, given the great disparities in countries’ incomes, has not been explored to date in an explicit game formulation. In the current paper, the analysis of shoring up of weakest-link COVID activities highlights the dilemma posed by the many countries needing a boost in providing COVID inoculations or effective quarantines. By relating other COVID activities to aggregator technologies, the current paper not only identifies the underlying games but also the prognosis for success. In so doing, the paper indicates the role of international institutions to augment anti-COVID activities. The previous literature [15,18,19,22] mentioned aggregator technologies but did not spell out their game implications regarding COVID activities per se. For many alternative game formulations, the analysis here indicates the effects of the number of countries on the prognosis for successful collective action.

The paper contains many findings. For instance, a large proportion of COVID activities adhere to weakest-link and best-shot aggregators. The favorable outlook for weakest-link actions may be lost when some countries’ provision is stymied by their small income levels. For global weakest-link public goods tied to COVID, actions to shore up the supply capacity of poor countries are required and pose challenging collective action difficulties given the plethora of weakest-link countries as well, ironically, as the number of capable countries that can do the shoring up. Some best-shot activities—e.g., developing effective vaccines—present a similar collective action concern once the vaccine is discovered. Namely, vaccines must be produced, sold, and then distributed to the population in poor countries, which require help from richer countries if infection rates and new strains are to be controlled. The latter constitutes a dynamic collective action worry. Some best-shot actions necessitate the pooling and coordination of actions among countries worldwide. Moreover, vaccine development may, at times, abide by a better-shot aggregator where smaller than the maximum contribution may still provide, though diminished, benefits. For instance, less effective vaccines with lower immunization rates may possess other attributes (e.g., storage and transport at higher temperatures) favoring some developing countries.

The remainder of the paper contains six sections. Section 2 briefly reviews the infection-propagating model governing the progress of COVID and indicates the condition for stability or convergence of infections. In Section 3, the four public good properties of COVID activities are emphasized, followed in Section 4 with an analysis of weakest-link COVID actions and their strategic implications. The focus on the strategic aspects is tied to shoring up income-poor countries, whose minimal or even zero contributions jeopardizes everyone’s safety. Next, Section 5 considers best-shot and better-shot COVID endeavors and their strategic aspects. In Section 6, cases where no action for an activity spells dire consequences are examined in a chicken-game framework that stresses mixed-strategy equilibrium. Concluding remarks are contained in Section 7.

2. The Infection-Propagating Model as It Pertains to COVID

The propagation and potential convergence of contagious viral diseases are modeled using the susceptible-infected-removed (SIR) representation of community spread (Cooper et al. [25,26]).3 Susceptible (S) individuals denote at-risk population members, whose infected (I) individuals are those who contracted the disease and are contagious. Removed (R_m) individuals are those who leave the infected population through infection-induced immunity or death. For COVID, the total at-risk population over time is not defined nor kept constant because of new strains so that susceptible individuals may not decrease monotonically [25]. During surge periods, susceptible people may increase as recovered patients become reinfected. Allowing for such surges yields an extended SIR or eSIR model in which the susceptible population is a variable that adjusts to new strains and waves of infection.

Ignoring deaths and vaccinations, the classic SIR model is governed by the following three interrelated differential equations [25]:

$$\frac{dS\left(t\right)}{dt}=aS\left(t\right)I\left(t\right)$$

(1)

$$\frac{dI\left(t\right)}{dt}=aS\left(t\right)I\left(t\right)-bI\left(t\right)$$

(2)

$$\frac{d{R}_m\left(t\right)}{dt}=bI\left(t\right)$$

(3)

where t denotes time, a represents the transmission rate, and b is the decay rate for infections. In Equation (1), the change in the susceptible population equals the transmission rate times the product of susceptible and infected individuals, S(t)I(t). The change in infections in Equation (2) over time is the difference between new cases of at-risk people, aS(t)I(t), and the removal of infected individuals, bI(t), characterized by Equation (3).

According to [25], the effective reproductive rate, R_e for $dI\left(t\right)/dt=0$ is $R_e=aS\left(t\right)/b$, which captures the progression of the disease. If $R_e<1$, the infected population converges to zero over time. If, however, $R_e>1$, the infected population increases over time. Thus, R_e represents a dividing or separating threshold between the disease dying out or becoming an epidemic. When recovered patients become susceptible again, S(t) can increase or surge, thereby pushing R_e above one as a new epidemic or wave results, as was true for COVID-19. Moreover, if a new strain becomes more infectious by a larger transmission rate as occurred for Delta and Omicron variants, then R_e is raised in value and a new wave of infections ensues.

The discovery of an effective vaccine can reduce the transmission rate, a, resulting in smaller changes in infections over time by Equation (1), which can bolster the likelihood of $R_e<1$ unless new virulent strains and surges occur. With more contagious strains, the vaccine loses its effectiveness and the transmission rate, a, increases. At first, the discovery of an effective vaccine held out hope that vaccinated and recovered patients’ immunity would eradicate the disease, as was true for smallpox once herd immunity was attained. The ability of new COVID strains to evade vaccines’ antibodies along with the reinfection of cured patients dashed the hopes for COVID herd immunity for the present. Nevertheless, vaccines still serve crucial purposes. High vaccination rates not only reduce serious illness, but also curtail the appearance of new strains and limit surges. However, the eventual eradication of COVID, like flu, is likely not achievable leading to the periodic need for fortified vaccines that target new and old COVID strains.

3. Publicness of COVID Activities

Fighting the COVID-19 virus involves myriad activities (e.g., testing population members, tracking outbreaks, and observing social distancing), whose benefits vary in their publicness aspects. The publicness variations of COVID-fighting actions make for a rich and nuance discussion. In general, public goods possess four dimensions of publicness (Cornes and Sandler [27]; Kaul et al. [28]). First, public good benefits are nonexcludable if once the good or activity is supplied by one country, the associated benefits are automatically available to other countries regardless of payment. Nonexcludable benefits encourage free riding whereby non-providing countries rely on other countries’ provision. Free riders use their own income to purchase excludable private goods. If immunization reduces COVID transmission rates, this reduction is consumed by all susceptible people at home or abroad, regardless of payment, in a globally connected world. Second, a public good’s benefits are nonrival so that consumption by one person or country of the good does not, in the least, diminish the good’s benefits still available to others to consume. One country’s action to limit the spread of COVID reduces the infection risk for all susceptible countries. As countries take advantage of the safer environment, their derived benefits do not limit the safety available to other countries. Additionally, developing an effective COVID treatment regimen not only benefits the inventing country but all other at-risk countries at an undiminished rate. COVID-19-related actions that generate nonexcludable and nonrival benefits for those within the range of benefit spillovers constitute pure public goods (Samuelson [29]), which cannot be parceled out and sold in markets. Benefit nonrivalry means that exclusion, if practiced, is undesirable since consumption implies zero marginal cost of use. Exclusion of a nonrival good denies some potential consumers the good’s benefit when they cannot afford the access price. From a social vantage, marginal gains to potential consumers are then eliminated by exclusion when there are no grounds for doing so given the zero marginal cost of use.

Third, public goods’ benefits possess different ranges of spillovers that affect a single nation, neighboring countries, a regional cluster of countries, or the entire globe (Kaul et al. [28,30]; Sandler [31]; George and Sandler [32]).4 Thus, COVID activities may give rise to national, regional, or global public goods (GPGs). Social-distancing measures and the wearing of face masks primarily offer localized national benefits [16], while quarantine measures at ports of entry provide regional and global benefits. The development of an effective vaccine or treatment regimen produces worldwide benefits associated with GPGs. Fourth, public good activities abide by aggregator technologies indicating how individual provision levels determine the consumption amount of the good ([20]; Harrison and Hirshleifer [33]).

Partially rival benefits from crowding can allow for exclusion and the use of user fees (tolls) to account for marginal crowding cost that an added consumer imposes on other paying consumers. Club goods are partially rival but excludable goods, whose user fees can finance the shared good by capturing consumers’ taste differences through revealed visitation rates [19]. Hospitals are club goods that treat COVID patients, while action to cleanse COVID contamination is also a club good.

3.1. Aggregator Technologies and COVID-19 Actions

Like other health-related public goods, COVID actions display a large number of aggregator technologies, some of which are more promising for collective action than others. Unlike the analysis here, earlier articles on health public goods did not consider how COVID-related activities’ aggregators influenced the underlying game analyses per se [21,22]. Discussion here is limited to six aggregators even though many others apply.

Until Hirshleifer’s [20] insightful breakthrough, the sole public good aggregator considered by Samuelson [29] and subsequent articles was summation for which public good levels equal the sum of individual contributions. With summation, one contributor’s, say a country’s, provision is perfectly substitutable for that of another. Perfect substitutability encourages free riding and the undersupply of the activity. A contributing country equates its marginal provision cost to its marginal provision benefit, thereby ignoring the sum of the marginal gains that other countries receive from the contributing country’s provision. The greater the range of benefit spillovers, the larger is the potential inefficiency from ignoring such spillovers. To correct the resulting inefficiency, countries must account for spillover benefits to other countries when providing summation public goods or else be coordinated by an international organization, such as WHO, public-private partnerships (e.g., GAVI, the Vaccine Alliance), or NGOs. For COVID, summation-abiding activities may involve stockpiling personal protective equipment globally, testing population members, expanding hospital surge capacity, or accumulating knowledge on the disease. In such instances, each contributors’ action adds to the total that could benefit all. For instance, a central storage facility for personal protective equipment can be used to dispense equipment as needed to surge areas, not unlike an insurance pool to cover contingencies. Currently, such actions are done independently by countries.

A highly relevant aggregator for some essential COVID actions—i.e., immunizing to augment population immunity, tracking COVID outbreaks, imposing quarantines, and maintaining port-of entry standards—is weakest link in which the smallest provision amount fixes the available consumption level for everyone. The least effort to quarantine the COVID virus determines the safety of at-risk populations since viruses (in infected people) that elude the quarantine can rapidly disperse far and wide in today’s interconnected world. If herd immunity were possible, then even a sole country with insufficiently immune population jeopardizes all countries. This applies to the failed polio eradication efforts thus far where neither Afghanistan nor Pakistan has yet achieved herd immunity.

Weakest-link public goods possess a better provision prognosis than summation public goods [31,33]. This follows partly from the lack of free-riding incentives associated with weakest linkage because a noncontributor receives no spillover benefits by bringing effective provision down to zero. The hallmark of weakest-link activities is matching behavior (see Section 4) in which countries match the smallest provision level. If all countries possess the same income and tastes, each desires the same quantity, which, when matched, results in an optimum amount. Problems may arise for heterogeneous countries since the poorest country or countries may be unable to match an acceptable minimal contribution, thereby jeopardizing all others by lowering the effective provision level. Such a scenario requires a rich country, collective of rich countries, or multilateral organization to raise these income-challenged countries’ supply effort. Actions to assist poor countries constitute shoring up the weakest link.

At the opposite end of the aggregator spectrum is best-shot public good where the largest effort determines the overall public good level. Provision below that of the best-shot country achieves nothing. For COVID, best-shot activities include identifying the virus, developing an effective vaccine, engineering disease-detection tests, devising anti-COVID drugs, establishing treatment regimens, and identifying new COVID variants. In the strictest best-shot case, a matching supply by another country is redundant (but see the later discussion of better-shot activities). The realization of best-shot public goods may require the pooling of efforts—e.g., the development of mRNA vaccine by Pfizer and BioNTech.5 Countries can redistribute income or resources to the country with the best chances of achieving the best-shot good or breakthrough. Additionally, the needed capacity can also be enhanced by NGOs, multilaterals, and other institutions.

If the cumulative contribution to an activity must surpass a benchmark for benefits to materialize, then the activity adheres to a threshold aggregator. Consider the institution of a lockdown period to keep COVID-19 new infections in check—the so-called flattening of the infection curve to allow hospitals to cope with admissions. Unless the lockdown interval is sufficiently long and inclusive to allow existing and developing cases to exceed the contagious period [26], the lockdown is inadequate to reduce the spread of the virus. Threshold aggregators also apply to social-distancing efforts, installing effective health infrastructure, and imposing tracking and tracing of cases. For instance, unless tracking follows most of the infections and contacts, the virus spread will not be halted sufficiently. To attain and surpass some thresholds, countries may need to coordinate and pool their activities. This pooling is also required by state and local governments within a country. Additionally multi-stakeholder organizations (e.g., public-private partnerships), charitable trusts, multilateral organizations, and NGOs can exert reinforcing influences to attain thresholds.

An additional aggregator is weighted sum for which contributors’ provisions are assigned different weights so that one contributor’s supply is not perfectly substitutable for that of another. These weights may be based on spatial location where, for instance, countries efforts nearer to the epicenter of an infectious outbreak are assigned larger weights. Such weights affect free riding—namely, countries whose supply is assigned lower (higher) weights offer less (more) free-riding opportunities. If benefits are largely localized, the need for transnational policy intervention is curtailed. For sufficiently large weights, transnational intervention must account for the different weights associated with contributors’ provision.

The final highlighted aggregator corresponds to zero weights given to the contributions of others owing to rival and excludable benefits, characteristic of private goods. In such cases, markets can ideally allocate resources to their most valued use; however, an efficient allocation may not result in a “desirable” distribution of the private good. Take the instance of COVID-19 vaccine distribution after its discovery and production. Rich countries can afford the vaccine for their people in contrast to many poor countries. If the latter countries obtain inadequate vaccine supplies, then COVID case there will soar, resulting in new variants and trade disruption. Although vaccine is a private good, its consumption gives rise to a jointly produced benefit in terms of limiting new strains. This jointly produced externality justifies rich countries pooling efforts to supply doses abroad as the Biden administration and some EU countries are doing.

3.2. Other Implications

Less strict forms of best-shot and weakest-link aggregators are also germane in the fight against COVID-19. Better-shot public goods arise when the largest provision has the greatest marginal gain, followed by progressively smaller marginal gains as the provision level of other contributors declines. Anti-COVID-19 mRNA vaccines have the greatest effectiveness, while Johnson & Johnson (J&J) Novavax viral-vector vaccine is not as effective. Nevertheless J&J vaccine possesses some favorable properties—the need for fewer doses and high-temperature storage—that made it still useful. Moreover, the J&J vaccine is tolerated by some people who could not take the mRNA vaccine. Other anti-COVID-19 better-shot, but not best-shot, vaccines include Russian Sputnik V and Chinese Sinopharm vaccines. Sputnik V is a viral-vector vaccine, while Sinopharm is an inactivated virus platform. Vaccines with lower efficacy yield smaller marginal benefits.

A softer variant of weakest link is weaker link in which the smallest provision amount provides the greatest marginal benefit, followed by the next smallest supply, and so on. With weaker-link activities, providers do not necessarily have to match one another’s contributions [21]. The shoring up of laggard contributors’ action can be accomplished to a lesser extent with weaker-link activities since full matching is unnecessary.

The introduction of aggregator technology results in more nuanced policy recommendations for COVID-tied activities. Weakest-link actions means that some countries must bolster those of poorer challenged countries, while best-shot action implies coordination among capable countries to avoid duplication. Each aggregator is associated with different strategic, and hence game, considerations to which I turn next.

4. COVID-19 and Weakest-Link Actions

To keep the analysis simple, I use discrete-form games to illustrate strategic considerations. If continuous choice of activities is permitted, then continuous games can be specified via subscriptions models for the private provision of public goods [27].

In Figure 1a, there are two players—country A and B—each of whom supply 0 or 1 unit of a weakest-link activity (e.g., establishing a quarantine). Country A is the row player, while country B is the column player.6 Country A’s net gain for each of the four strategic combinations appears to the left of the comma in the cell, while country B’s net gain for each strategic combination is to the right of the comma in the cell. For matched contributions,

Country A and B each receives 7 in benefits before unit cost of 5 is deducted, while, for an unmatched unit, there are no benefits to offset unit cost. When neither country contributes to the weakest-link action, each earns nothing in the upper left-hand cell. If country A contributes 1 unit and country B provides nothing, country A nets $-$5 when covering cost while country B gets 0 owing to the absence of benefit spillovers. For the opposite contribution scenario in the upper right-hand cell, the payoffs are reversed. When both countries contribute, each gets 2 as matched benefit of 7 is reduced by 5 in provision cost. The game has no dominant strategy since $0>-5$ but $2>0$ for both countries. In contrast to a Prisoners’ Dilemma, this assurance game does not have free riding as a dominant strategy (Runge [34]). Moreover, the game contains two pure-strategy Nash equilibriums as indicated, corresponding to no contributions or both countries contributing. At these Nash equilibriums, neither country is unilaterally incentivized to alter its strategy as countries compare their payoff from moving from the Nash equilibrium. If one country assumes leadership and contributes, the other country is better off following suit.

Since there is always an odd number of Nash equilibriums to a discrete game (Dixit et al. [35]), there is a mixed-strategy Nash equilibrium for which both countries randomize their action between contributing or not. Let country B choose not contributing $\left(1-q\right)$ percent of the time and choose contributing one unit q percent of the time. Analogously, let country A choose not contributing $\left(1-p\right)$ percent of the time and choose contributing one unit p percent of the time. By applying country B’s randomization, $\left(1-q\right)$ and q, to country A’s payoff for each of its two strategic choices and then equating country A’s expected payoffs from not contributing to its expected payoffs from contributing, one gets the mixed-strategy equilibrium, $q=5/7$. A similar exercise for country B’s expected payoffs based on country A’s randomization yields $p=5/7$.7 At the mixed-strategy Nash equilibrium, neither country wants to alter its randomization as the expected payoff from each strategy is equal. Given the 0 payoff for the absence of a matched unit contributed, $p=q=c/b=5/7,$ which increases with unit cost and decreases with the matched unit’s benefit. As such, reduced unit cost or greater matched benefit lowers the assurance likelihood that a country requires to contribute, which, in turn, fosters collective action. If a coordination mechanism can be devised for the two countries, then nonzero weakest-link contributions become more likely.

As COVID-19 increases in severity along with the degree of contagion, benefit from inoculation increases and required assurance decreases, namely mixed-strategy equilibrium randomization p and q decreases, thus augmenting likely inoculation rates. With the possibility of reinfection growing with new strains, the weakest-link characterization of inoculation becomes more appropriate. As more was learned about vaccines and the absence of side effects, the cost per unit falls, which, in turn, augments vaccinations with the decline in the required assurance likelihood at the mixed-strategy equilibrium. If, however, new variants limit the vaccine’s effectiveness, then vaccination benefit falls, and the necessary assurance probabilities rise for provision. This COVID-related discussion of mixed-strategy equilibriums and their drivers is new to the literature.

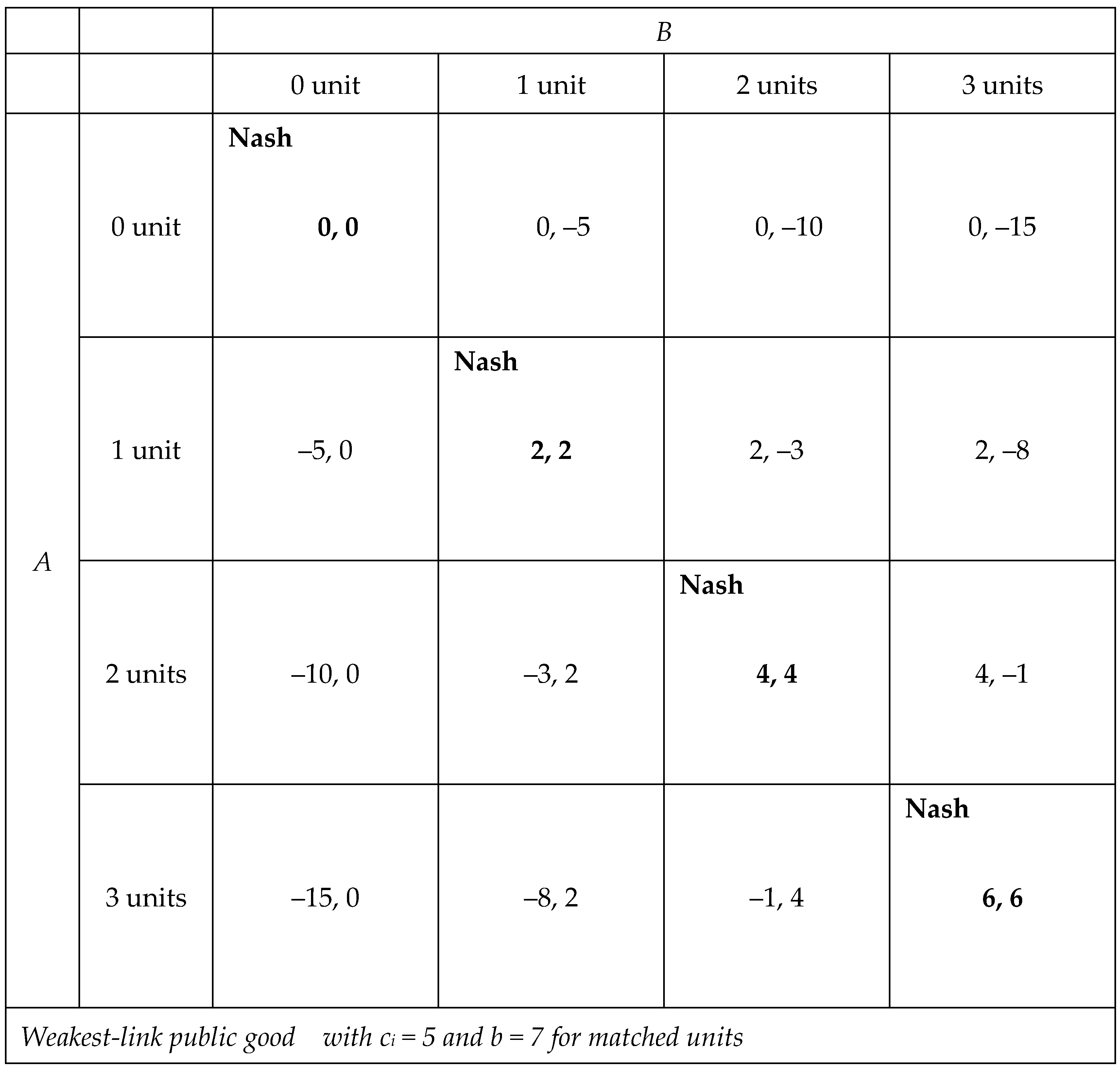
In the Appendix A, Figure A1 extends the weakest-link case to permit country A and B to choose among 0, 1, 2, or 3 units where $c_i=5$ for each contributed unit and $b=7$ for each matched unit. The weakest-link level of the activity corresponds to the smallest amount provided—e.g., the least effective quarantine enforced. Thus, if country A gives 2 units and country B contributes 3 units, then the effective contribution is 2 units with net benefits of 4 as 14 in benefits are reduced by two-unit cost of 10. The boldfaced two-by-two matrix in the upper left-hand corner of the four-by-four matrix in Figure A1 corresponds to Figure 1a. The four pure-strategy Nash equilibriums are along the main diagonal where matching, the hallmark of weakest-link public goods, occurs. The focused equilibrium is the three-unit match, which requires that both countries can afford three units. If one country is income-challenged, then the minimum contribution is that country’s provision unless the other country comes to its assistance through an in-kind or monetary transfer [23,24]. Such shoring-up efforts are discussed next.

To investigate efforts to shore up a noncontributing “weakest-link” country, first consider the two-country case in Figure 1b, where matching efforts result in the same main diagonal payoffs as before. If country A shores up resource-challenged country B, then country B free rides for a benefit of 7, while A nets $-3$ as it must cover the cost of its unit and that of country B, which is 10—see the lower left-hand cell in Figure 1b. The payoffs are reversed when country B shores up country A’s inability to contribute—see the upper right-hand cell in Figure 1b. The game matrix in Figure 1b is a Prisoners’ Dilemma where both countries have a dominant strategy to not shore up, resulting in a deficient outcome with zero payoffs. If, therefore, one country is income-challenged, then the collective action prospect for weakest-link activities, prevalent for COVID-19, loses their relative optimism.

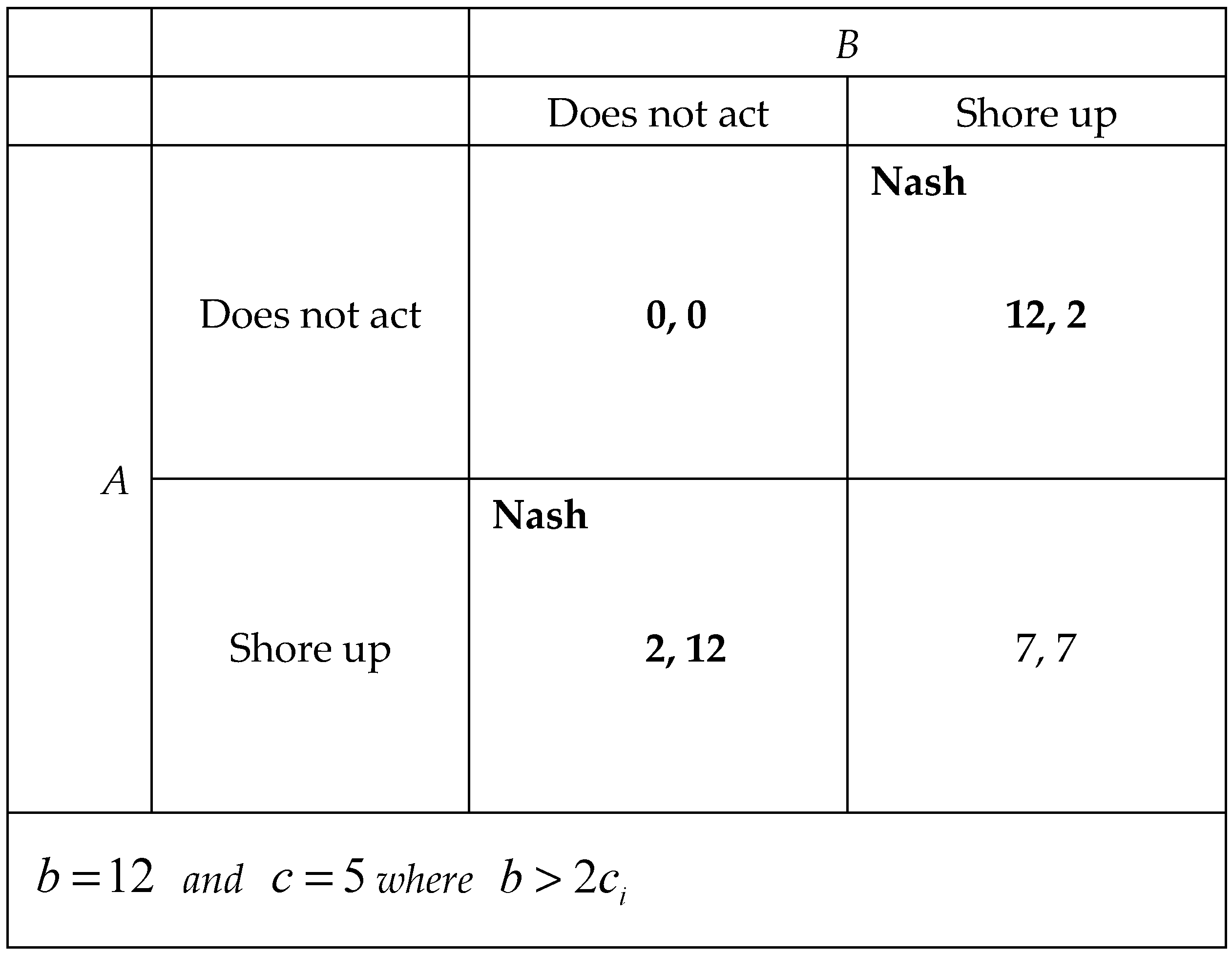
When $b>2c_i$, a chicken game results.8 This is displayed in Figure A2 in the Appendix A, where a matched unit yields $b=12>10=2c_i$ in benefit. As a consequence, neither country has a dominant strategy and the two pure-strategy Nash equilibriums involve a single country doing the shoring up, so that some form of coordination is needed. If one country leads and commits convincingly to do nothing, then the other country is incentivized to shore up the non-acting country.9 Hence, sufficient gains from a matched unit may potentially bring about collective action in a shoring-up game among two countries. The mixed-strategy Nash equilibrium randomization occurs when $p=q=2/7$, which requires very little assurance to shore up, given the relatively high benefit of a matched unit.

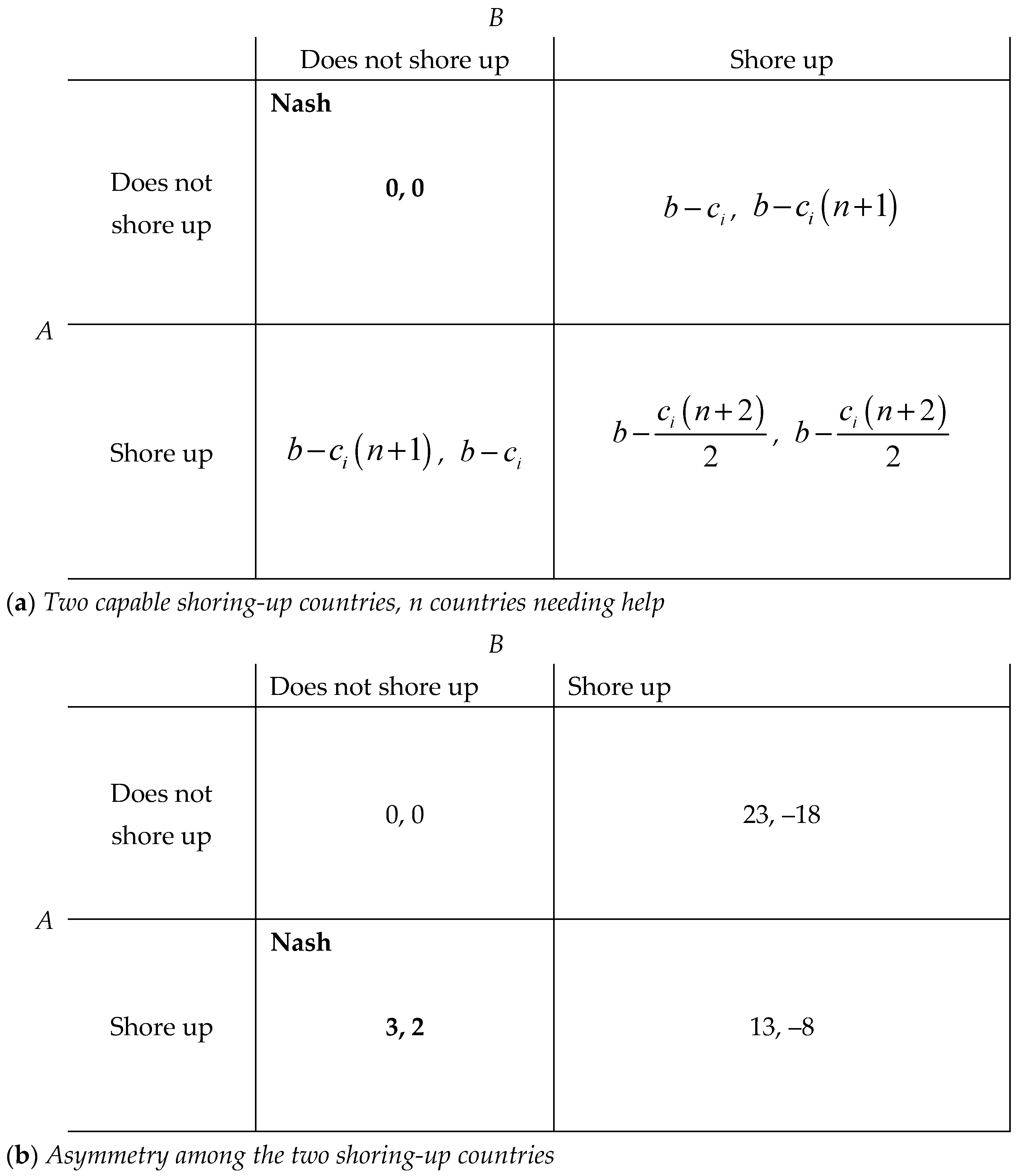
In Figure 2a, the matrix game corresponds to two capable countries—country A and B representing say the United States and China—and $n$ poor countries requiring shoring up. Only the vantage of the two capable countries is germane, resulting in the displayed two-by-two matrix game since the poor countries contribute nothing. The associated game parameters are $c_i$ for unit cost and $b>c_i$ for the benefit associated with a matched unit. In the off-diagonal cells, the shoring-up country nets $b-c_i\left(n+1\right)$ as it must cover its cost and that of the n countries needing provision assistance, while the capable free-riding country clears $b-c_i$ as it covers just its own provision cost. If both capable countries combine forces to shore up the n challenged countries, then the capable countries’ shared cost is $c_i\left(n+2\right)/2$. For $b-c_i\left(n+1\right)<0$, the sole Nash equilibrium for the resulting Prisoners’ Dilemma corresponds to the two capable countries doing nothing for which mutual inaction results in zero gain for both countries. The matched unit must provide a benefit larger than $c_i\left(n+1\right)$ for a chicken game to result, which is less likely as the number of countries requiring assistance increases. In the case of COVID inoculations and the development of more virulent and contagious strains among the unvaccinated, the benefit from a matched unit may be sufficiently high for a capable country to act on its own or to pool its efforts among select capable countries as was true for the eradication of smallpox or the ongoing efforts to eradicate polio [36,37].10 For many COVID-19 weakest-link actions, the number of weakest-link countries is great.

In Figure 2a, the largest sum of payoffs among the countries is associated with unilateral and mutual shoring up, where the two capable countries gain $2b-c_i\left(n+2\right)$ in total and the incapable countries gain $7n$ in aggregate free-rider benefits. Reducing unit cost not only leads to a heightened likelihood of shoring up but also to a greater overall gain for unilateral or multilateral shoring-up action. The same holds for an increase in matched benefits, b.

Next, the analysis is generalized to m capable shoring-up countries and n countries needing their provision to be covered (not displayed in an $m\times m$ matrix game). The $m$ capable countries can choose between shoring up or not. The payoffs for $m$ mutually shoring-up countries are $b-\left[c_i\left(n+m\right)/m\right]=x$, which becomes smaller with larger n and larger with greater m. The latter follows because

$$\partial x/\partial m=\left(c_{i}n\right)/m^2>0$$

(4)

Thus, the presence of more capable shoring-up countries might make for a better collective action prognosis [38,39,40,41]11 if they coordinate efforts at helping less fortunate countries. Unfortunately, this is not the case because a capable country that free rides—i.e., does not shore up—nets $b-c_i$ which exceeds x. A dominant strategy to not shore up remains even when there are more capable countries so that the capable countries faces an m-country Prisoners’ Dilemma.

The final weakest-link scenario to be considered here concerns two capable, but asymmetric, shoring-up countries and four countries needing assistance. In Figure 2b, the matrix game includes capable countries denoted by A and B where $c_i=5$ but a matched unit gives 28 in benefits to country A and just 7 in benefits to country B and to those countries being shored up. When country B shores up alone, it nets $-18\left(=7-25\right)$ as it covers its provision cost and that of the four challenged countries. With country B’s unilateral shoring-up effort, country A nets $23\left(=28-5\right)$ as it just covers its own provision cost. If, however, country A unilaterally shores up the weakest-link countries, then country A clears $3\left(=28-25\right)$, while free-rider country B nets $2\left(=7-5\right)$. In Figure 2b, the sole Nash equilibrium has country A shoring up alone. Thus, sufficient benefit asymmetry can escape the usual Prisoners’ Dilemma associated with shoring up. Analogously, asymmetric cost can also avoid a shoring-up Prisoners’ Dilemma. The reader can easily extend the analysis to more capable and more challenged (incapable) countries.

5. Best-Shot Scenario for COVID Action and Importance of Coordination

Efforts to address the COVID-19 threats are associated with myriad best-shot activities including isolating the virus and its novel strains, and discovering an effective vaccine.

In a classic and strict best-shot scenario, the largest individual contribution provides benefits in contrast to smaller contributions that add nothing [20]. For illustration, consult the matrix game displayed in Figure 3a played between country A and B, each of which provides 0, 1, or 2 units of the best-shot activity.12 Once again, country A is the row player and country B is the column player. Of course, this game can be readily extended to more units of provision. A single unit offers 7 in benefits to each of the countries, while a two-unit block from a single country yields 13 in benefits to each of the countries, allowing for diminishing returns in benefits. The benefits associated with each country simultaneously providing a single unit is just 7 and not 13, because a two-unit block offers more benefits than two single-unit contributions. Again, unit cost equals 5 for the contributing countries.

For the matrix game in Figure 3a, mutual inaction results in zero payoffs all around in the upper left-hand cell. When country A solely supplies one unit, it nets 2 after covering cost, while country B receives the free-rider gain of 7. With roles reversed, payoffs are reversed accordingly for the two countries. If country A contributes 2 units and country B provides no units, then country A nets $3\left(=13-10\right)$ and country B gets a free ride worth 13—see the lower left-hand cell marked with Nash. When country A supplies 2 units and country B supplies a single unit, country A again receives 3 but country B nets $8\left(=13-5\right)$. Other payoff combinations are computed similarly. The two pure-strategy Nash equilibriums are in the two corner off-diagonal cells in matrix 3a where one country provides 2 units and the other free rides. A mixed-strategy equilibrium exists as the countries randomize over providing nothing or two units of the best-shot good. The hallmark of best-shot COVID activities involves a sole country supplying the maximum allowable contribution and the other or others free riding. As such, potential best-shot countries must coordinate so that there is no wasteful duplication of effort. If, for example, both countries contribute 2 units, then each gain just 3 after cost is deducted in Figure 3a. Duplication of effort characterized COVID-19 vaccine development by the United States, China, and Russia with each pursuing their own vaccines, which can be motivated based on selective or private incentives [38]. Only laboratories in the United States and the United Kingdom coordinated their search for an mRNA vaccine. Games such as Figure 3a are termed coordination games and indicates the need for countries to coordinate or pool actions with respect to best-shot COVID activities. The simple discrete-game representation here makes that clear, which has not been pointed out in COVID literature addressing best-shot activities [10,15].

Best-shot activities, unlike some weakest-link ones, are generally suboptimally supplied, because the best-shot supplier does not account for the marginal benefits conferred on other countries from it breakthrough prospect when deciding its pursuit efforts [33]. Best-shot discoveries can be sped up by directing resources and personnel to the country with the best prognosis for success, but since that country is generally rich, such redistributions are not always looked upon favorably.

In the case of COVID vaccines or antiviral treatment discovery, once either best-shot good is discovered, the associated derived products (namely, vaccine or treatment doses) are private goods in terms of excludable and rival benefits [14]. Ironically, the best-shot activity results in a marketable good, whose undersupply to poor countries generates a weakest-link activity—e.g., inoculations—that puts all countries at risk from new variants and surges. As such, vaccines and antiviral distribution imply weakest-link concerns requiring shoring-up actions with their collective action difficulties.

If smaller units of a potential best-shot activity offer marginal gains that are less than those of a larger effort or effectiveness, then the activity is better shot. In this case, the largest provision block does not necessarily make smaller contributions redundant or useless. For COVID vaccines, their efficacy differs among the various platforms—namely, mRNA, viral vector, and inactivated viral vector ([14]; CDC [42]). Benefits from less-effective vaccines may arise from the smaller number of doses, less demanding transport temperatures, or greater patient tolerance, making such vaccines useful in some situations.13

Figure 3b represents a stylized discrete game for a better-shot activity’s provision, where 0, 1, and 2 effectiveness units are possible. The cost per unit remains at 5. Country A’s initial unit provided gives 7 in benefit, while its two units yield 13 in benefit as before. By contrast, country B’s initial unit renders 6 in benefit, while its two-unit block offers 11 in benefit. The number of units represents the effectiveness of the vaccine. Each country uses its own vaccine, if available, even when inferior, where China is an apt example. If the country does not develop a vaccine, then it relies on that of the other country. In the top row of matrix 3b, country A free rides on country B’s vaccine for benefits of 6 and 11, corresponding to 1 and 2 units of B’s vaccine effectiveness, respectively, while country B nets $1\left(=6-5\right)$ and $1\left(=11-10\right)$ corresponding to its two provision levels of one and two units, respectively. The other payoff combinations are found similarly—e.g., when country A and B each provide a vaccine effectiveness of 1, country A clears 2 and country B gets 1 from using its own vaccines. If, however, country A develops a more effective vaccine corresponding to 2 units and country B free rides, than country A nets 3 and country B receives 13.

For this better-shot scenario, there are three pure-strategy equilibriums—the two off-diagonal corner cells and the cell where country A free rides on country B’s single unit effectiveness—each of which are marked Nash. With better-shot activities, more equilibriums, some not requiring coordination, are possible. In fact, some underlying payoffs may allow for even more equilibriums. In the literature [10,15,19,21,22], the strategic implications of better-shot COVID activities have not been indicated.

6. No-Action, Dire-Consequences Games

A best-shot COVID situation, such as isolating the virus, may not only warn countries worldwide of the new threat but also allow them to study the disease, which can result in the development of vaccines, treatment regimes, and antiviral drugs. Until the virus is isolated, the world community is blindsided. To capture the essential strategic aspects of this activity, I put forward alternative chicken games played by n identical countries. The cost for isolating the virus is assumed to be 10 with public benefits of 15 for all countries once the virus is identified. If the virus is not isolated, then a damage of 20 is imposed on all countries as they are helpless to do much to offset the virus.

In Figure 4, the upper matrix game displays the net payoffs to representative country i. For simplicity, only six countries are initially considered, but n can be any number of countries. When country i isolates the virus, it nets $5\left(=15-10\right)$, while avoiding the dire consequence of –20 for no action by any of the countries. The payoff of country i for isolating COVID is 5 in the bottom row no matter how many other countries also isolate the virus. In the top row of matrix 4a, if neither country i nor another country isolates the virus, then country i (and the other countries) suffers 20 in losses. When country i does not act but one or more countries isolate the virus, country i receives 15 as a free rider. The Nash equilibriums involve the representative country isolating the virus alone. Because $5>-20$, country i will not want to alter its strategy in that scenario if given the opportunity. Since country i can be any of the six countries, there are six pure-strategy Nash equilibriums—namely, a single acting country. For n homogeneous countries, the assumed payoffs remain the same in the n cells in the top and bottom rows, so that there are n pure-strategy Nash equilibriums with a single country acting.

As a consequence, the countries confront a coordination problem where precisely a single country must come forward to avoid the losses from inaction. Such, n-player chicken games are not uncommon and are analogous to the “bystander” game where a rape victim cries out for help and n potential saviors hear the cry and each must independently decide whether or not to assist [35], (pp. 454–458). Because there is no need for two players to act, there is the danger that no action ensues as all may wait for someone to assume the burden. As shown next, this failure to act worsens as the number of potential isolating countries, n, increases.

Let P be the probability that a representative country does not act in terms of a best-shot COVID scenario. Country i’s mixed-strategy Nash equilibrium must be consulted to discern how the number of countries influences possible action being taken. For independent probabilities, the likelihood that no other country acts is $P^{n-1}$, where the probability that at least one of the other countries acts is $1-P^{n-1}$. Given the parameters in Figure 4a, country i’s mixed-strategy equilibrium between action and no action equates the expected payoff from no action on country i’s part (involving the expected payoff of no action by others and the expected payoff of action by one other country) to the payoff of country i’s own action: namely,

$$-20P^{n-1}+b\left(1-P^{n-1}\right)=b-c_i$$

(5)

where b = 15 and c_i = 10. I keep b and c_i generic for interpretation purposes.

Solving for P yields:

$$P={\left(\frac{c_i}{b+20}\right)}^{1/\left(n-1\right)}$$

(6)

which is representative country i’s mixed-strategy equilibrium probability of not acting, so that the probability that at least one country acts is

$$1-P^n=1-{\left(\frac{c_i}{b+20}\right)}^{n/\left(n-1\right)}$$

(7)

As n increases from 2 to infinity, the probability of some country acting decreases from $1-{\left(\frac{c_i}{b+20}\right)}^2$ to $1-\left(\frac{c_i}{b+20}\right)$, assuming that $b+20>c_i$. Hence, the larger the group of at-risk countries, the less likely action is to avert disaster. Increase in a representative country’s cost of action reduces the probability of action, while increases in the benefit of action or the loss from inaction raises the probability of action. In the case of vaccine development, the losses from no breakthrough are so large that vaccine discovery was a near certainty even in a world with well over two hundred countries.

Thus far, the case of homogeneous countries has been examined. Consider vaccine development where most countries do not have the capability to find the vaccine. Thus, the required coordination concerns a relatively select number of countries, making some action among capable countries more likely. Asymmetric countries allow some rich countries to face a great benefit, b, and a larger loss from inaction or failure, both of which augments such countries’ likelihood of actions. Sufficient asymmetries can coordinate action.

In Figure 4b, a threshold aggregator version of chicken is displayed for six countries, where a minimum of three countries must work in conjunction to avoid the losses of 20. In the bottom row, country i nets $-30\left(=-20-10\right)$ when less than two other countries join i’s efforts as the loss from failure and the cost of provision impact country i. Once the three-country threshold is attained, country i clears 5 in the remaining cells in the bottom row of matrix 4b. For the top row, country i loses 20 when it and two other countries fail to act. Once the participation threshold is met, country i gets 15 in free-rider benefits. The game has $1+\frac{6!}{3!3!}$ pure-strategy Nash equilibriums, corresponding to no one acting and exactly three of six countries coordinating actions. By increasing the number of equilibriums, the achievement of action becomes more assured. Again, the important parameters for the previous game—e.g., large losses from inaction—bolster the likelihood of action for the required threshold of countries.

7. Concluding Remarks

The paper explores how COVID public good activities abide by alternative aggregator technologies that relate to how countries’ or contributors’ provision influences the amount of the activities available for consumption. For instance, the consumption stemming from weakest-link activities corresponds to the smallest provision level given; in contrast, the consumption arising from best-shot activities corresponds to the largest provision level given. Other aggregators—summation, weaker link, threshold, and better shot—are discussed in relation to COVID activities. Throughout the analysis, the paper employs simple discrete games with illustrative benefit and cost values to identify the provision prospects. When provision prospects are grim, some policies are suggested. The analysis is conceptual and does not hinge on real-world numbers, which are difficult to obtain. Moreover, the study focuses on the strategic implications of COVID activities. As such, the analysis differs from the few articles [10,15,18,19] that mention aggregator technologies with respect to COVID actions but do not exploit games to illustrate provision prospects and pitfalls. Moreover, earlier articles do not pursue mixed-strategy Nash equilibriums, when such equilibriums are relevant. In the current analysis, the number of countries becomes an important parameter in the prognosis for collective action for COVID activities related to weakest-link and best-shot aggregators.

If the providing countries of a weakest-link COVID activity, possess similar tastes and income, then the prognosis for efficient action is promising. When, however, many countries are income-constrained to achieve acceptable provision levels, rich countries must shore up provision shortfalls by numerous poor countries. Often, shoring up results in a Prisoners’ Dilemma game among capable countries that are incentivized to rely on the shoring-up efforts of other capable countries. The shoring-up provision dilemma of weakest-link COVID activities may be exacerbated by greater numbers of capable or needy countries. However, the dilemma may be avoided with an asymmetric capable country that greatly values provision benefits over shoring-up costs. Multilateral organizations, NGOS, charitable foundations, health networks of countries, and public-private partnerships can direct resources to the capable country to meet necessary shoring-up action. When, however, the benefits from shoring up dwarf shoring-up cost, a chicken game results and coordination among potential capable countries becomes more promising.

Best-shot COVID activities necessitate coordination among potential providers to avoid wasteful duplication. Given the huge inequality among countries, many best-shot activities have a good prospect of provision. This is illustrated by the rapid development of effective COVID vaccines; however, duplication occurred as China and Russia engineered less effective non-mRNA vaccines. Once the vaccine was developed by private, but publicly subsidized pharmaceutical firms in the United States and the United Kingdom, the vaccine’s international distribution concern became a weakest-link activity that required shoring-up actions. Thus, one activity following one aggregator technology can morph into another with a different aggregator at further development stages. As such, the direction of income redistribution can change since weakest-link aggregators favor more equal income distribution in contrast to best-shot aggregators wanting less equal income distribution. To address best-shot COVID issues, the WHO can provide coordination among countries. For weakest-link COVID actions, rich countries can combine their efforts with those of NGOs, multilateral organizations, charitable foundations, health-promoting networks, and public-private partnerships to bolster provision.

The infection of recovered and once-immunized patients with new COVID strains means that the eventual achievement of herd immunity is highly unlikely. Thus, COVID vaccines, like flu, must be refreshed, tailored to new strains, and administered annually to offer up-to-date protection. This best-shot updating of vaccines is relatively easy for the pharmaceutical companies that developed the first COVID vaccines. The shoring-up issue concerns within-country vaccine fatigue and the transnational distribution of doses to poor countries.

The use of simple discrete games can be used to investigate the collective action prospect for public good activities involving other diseases, environmental concerns, conflict issues (e.g., peacekeeping), financial stability, and other global and regional public good concerns. An analysis of aggregator technologies as they apply to international public good concerns indicate that policies must be tailored to the strategic implications of such aggregators.