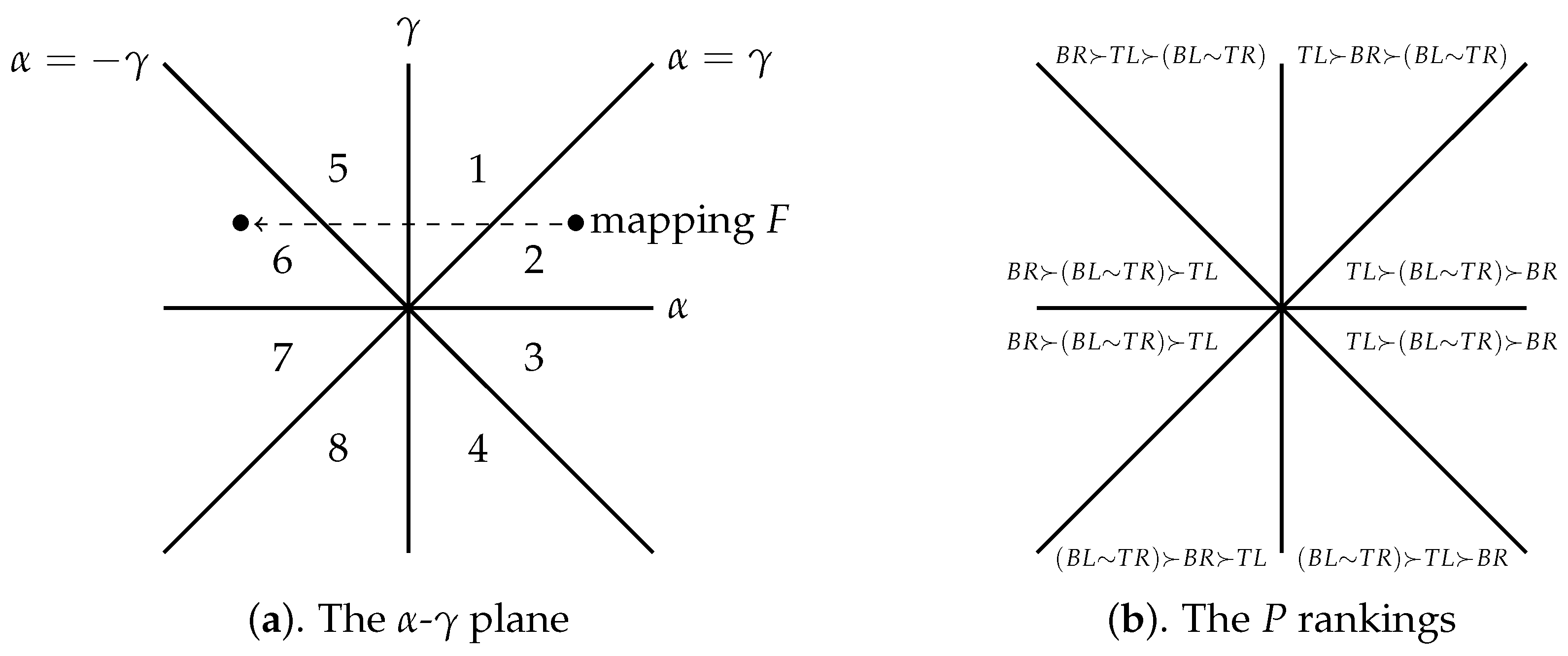With Potential Games, Which Outcome Is Better?

Abstract

Lower one- or two-dimensional coordination, or potential games, are popularly used to model interactive behavior, such as innovation diffusion and cultural evolution. Typically, this involves determining the “better” of competing solutions. However, examples have demonstrated that different measures of a “good” choice can lead to conflicting conclusions; a fact that reflects the history of game theory in equilibrium selection. This behavior is totally explained while extending the analysis to the full seven-dimensional class of potential games, which includes coordination games.

1. Introduction

When Schelling (1960) wrote Strategy of Conflict, it pivoted attention from zero-sum games to the more general behavior allowed by games with mutually beneficial outcomes (which was appropriate during this Cold War period) [1]. Specifically, Schelling made a case for coordination games, which Lewis (1969) used to discuss culture and convention [2]. This behavioral notion of mutually beneficial outcomes was further explored by Rosenthal (1973) with his development of the congestion game [3]. Monderer and Shapley (1996) built on the congestion game with “common interest” games; namely the potential games (which include coordination games) [4]. More recently, Young (2006, 2011) and Newton and Sercombe (2020) took this analysis a step further by modeling, with potential games, how populations on networks evolve from one convention to another [5,6,7]. The natural question in this work is to discover whether the status quo or an innovation will be accepted.

This issue of finding the “better outcome” (e.g., an innovation or the status quo), which is a theme of this paper, is a fundamental and general concern for game theory; answers require selecting a measure of comparison. A natural choice is to prefer those outcomes where the players receive larger payoffs. Rather than payoff dominance, another refinement of Nash equilibria offered by Harsanyi and Selton (1988) is risk dominance [8].1 The choice used by Young (2006, 2011) and later by Newton and Sercombe (2020) is to maximize social welfare. Still, other measures can be constructed [5,6,7].

With the social welfare measure, Young constructed a clever ad-hoc example where, although it is seemingly profitable to adopt the innovation, the innovation is worse than the status quo [5]. Young’s observation underscores the important need to understand when and why a model’s conclusions can change. This includes his concerns of identifying when and why a new cultural convention is “better”. Is there a boundary between the quality of innovations?

Conflicting conclusions must be anticipated because different measures emphasize different traits of games. Thus, answering the "better" question requires determining which aspects of a game a given measure ignores or accentuates. The approach used here to address this concern is new; it appeals to a recent decomposition (or coordinate system) created for the space of games [9,10,11,12] (in what follows, the [9,10,11] papers are designated by [JS1], and the book [12] by [JS2]). There are many ways to decompose games, where the emphases reflect different objectives. An early approach was to express a game in terms of its zero sum and identical play components, which plays a role in the more recent Kalai and Kalai bargaining solution [13]. Others include examining harmonic and potential games [14] and strategic behavior such as in [15,16]. While some overlap must be expected, the material in [JS1] and [JS2] appears to be the first to strictly separate and emphasize Nash and non-Nash structures.

Indeed, in [JS1], [JS2], and this paper, appropriate aspects of a game are used to extract all information, and only this information, needed for the game’s Nash structures; this is the game’s “Nash” component. Other coordinate directions (orthogonal to, and hence independent of the Nash structures) identify those features of a game that require interaction among the players, such as coordination, cooperation, externalities, and so forth. By isolating the attributes that induce behavior among players, these terms define the game’s “Behavioral” component. The final component, the kernel, is akin to adding the same value to each of a player’s payoffs. While this is a valuable variable with transferable assets, or to avoid having games with negative payoffs, it plays no role in the analysis of most settings. In [JS2], the [JS1] decomposition is extended to handle more player and strategies.2

One objective of this current paper is to develop a coordinate system that is more convenient to use with a wide variety of choices that include potential games (a later paper extends this to more players and strategies). An advantage of using these coordinates is that they intuitively organize the strategic and payoff structures of all games. This is achieved by extracting from each payoff entry the behavioral portions that capture ways in which players agree or disagree (e.g., in accepting or rejecting an innovation) and affect payoff values. Of interest is how this structure applies to all $2\times 2$ normal form games. When placing the emphasis on potential games, these coordinates cover their full seven-dimensional space, so they subsume the lower dimensional models in the literature.

By being a change of basis of the original decomposition, this system still highlights the unexpected facts that Nash equilibria and similar solution concepts (e.g., solution notions based on “best response” such as standard Quantal Response Equilibria) ignore nontrivial aspects of a game’s payoff structure; see [11]. In fact, this is the precise feature that answers certain concerns in the innovation diffusion literature. Young’s example [5], for instance, turns out to combine disagreement between two natural measures of "good" outcomes: one measure depends on unilateral deviations; the other aggregates the collective payoff. Newton and Sercombe re-parametrize Young’s model to further explore this disagreement [7]. As we show, Young’s example and the Newton and Sercombe arguments stem from a game’s tension between group cooperative behavior and individualistic forces.

Other contributions of this current paper are to

• describe the payoff structure of these games;
• characterize the full seven-dimensional space of $2\times 2$ potential games;
• analyze the behavioral tension between individual and cooperative forces in potential games;
• explain why different measures can reach different conclusions; and,
• relate the results to risk-dominance when possible.

The paper begins with an overview of the coordinate system for all $2\times 2$ normal form games. After identifying the source of all conflict with symmetric potential games, the full seven-dimensional class is described.

2. Overview of the Coordinate System

As standard with coordinate systems, the one developed for games in [JS1] can be adjusted to meet other needs. The choice given here [JS2] reflects central features of potential games.

Consider an arbitrary $2\times 2$ game $\mathcal{G}$ (

Table 1. Arbitrary Game $\mathcal{G}$ in Normal-Form.

	$+1$		$-1$
$+1$	$a_1$	$b_1$	$a_3$	$b_2$
$-1$	$a_2$	$b_3$	$a_4$	$b_4$

), where each agent’s strategies are labeled $+1$ and $-1$ (cells also will be denoted by TL (top-left), BR (bottom-right), etc.).

A weakness of this representation is captured by the

Table 2. An Example.

	$+1$		$-1$
$+1$	$-3$	7	7	3
$-1$	$-1$	5	$-1$	$-3$

game. Information about which strategy each player prefers, whether they do, or do not want to coordinate with the other player, and where to find group opportunities is packed into the entries. Yet, in general, this structure is not readily available from the Table 2 form.

The coordinate system described here significantly demystifies a game by unpacking its valued behavioral information. This is done by decomposing a game into four orthogonal components, where each captures a specified essential trait: individual preference, individual coordinative pressures, pure externality (or Behavioral), and kernel components (see

Table 3. The Four Components of a $2\times 2$ Game.

	$+1$		$-1$			$+1$		$-1$
$+1$	${\alpha }_1$	${\alpha }_2$	${\alpha }_1$	$-{\alpha }_2$	$+1$	${\gamma }_1$	${\gamma }_2$	$-{\gamma }_1$	$-{\gamma }_2$
$-1$	$-{\alpha }_1$	${\alpha }_2$	$-{\alpha }_1$	$-{\alpha }_2$	$-1$	$-{\gamma }_1$	$-{\gamma }_2$	${\gamma }_1$	${\gamma }_2$
a. Individual Preference Component					b. Coordinative Pressure Component
	$+1$		$-1$			$+1$		$-1$
$+1$	${\beta }_1$	${\beta }_2$	$-{\beta }_1$	${\beta }_2$	$+1$	${\kappa }_1$	${\kappa }_2$	${\kappa }_1$	${\kappa }_2$
$-1$	${\beta }_1$	$-{\beta }_2$	$-{\beta }_1$	$-{\beta }_2$	$-1$	${\kappa }_1$	${\kappa }_2$	${\kappa }_1$	${\kappa }_2$
c. Pure Externality Component					d. Kernel Component

) (the orthogonality comment follows by identifying games with vectors). The original game is the sum of the four parts.

To associate these components with behavioral traits of any $2\times 2$ game, the individual preference component identifies an agent’s inherent preference for strategy $+1$ or $-1$. If ${\alpha }_i>0$, then agent i prefers strategy $+1$ to $-1$independent of what the other agent plays. In turn, ${\alpha }_i<0$ means that agent i’s individual preference is for strategy $-1$. The Table 2 values will turn out to be ${\alpha }_1=2,{\alpha }_2=3$, which indicates that both players prefer $+1$ (or TL).

The coordinative pressure component ${\gamma }_j$ reflects a conforming stress a game imposes on agent j. Independent of the ${\alpha }_i$ sign, a ${\gamma }_j>0$ value rewards agent j a positive payoff by conforming with agent i’s preferred ${\alpha }_i$ choice. Conversely, when ${\gamma }_j<0$, agent j’s payoff is improved by playing a strategy different than what agent i wants. With Table 2, ${\gamma }_1=-3$ while ${\gamma }_2=-1$, so neither player is strategically supportive of personally reinforcing the other agent’s preferred choice.

The pure externality component represents consequences that an agent’s action imposes on the other agent.3 If agent i plays $+1$, for instance, then, independent of what agent j does, agent j receives an extra ${\beta }_j$ payoff! Acting alone, however, agent j is powerless to change this portion of the payoff. To see why this statement is true, should Column select L in Table 3c, then no matter what strategy Row chooses, this extra advantage remains ${\beta }_1$. The sign of ${\beta }_j$ indicates which of agent i’s strategies contributes to, or detracts from, agent j’s payoff. In Table 2, the ${\beta }_1=-3,{\beta }_2=2$ values convert TR into a potential group opportunity.

A subtle but important behavioral distinction is reflected by the $\gamma$ and $\beta$ terms. The ${\gamma }_j$ values capture whether, in seeking a personally preferred (Nash) outcome, an agent should, or should not, coordinate with the other agent’s preferred interests. In contrast, the ${\beta }_j$ values identify externalities and opportunities to encourage both to cooperate. For a supporting story, suppose the strategies are to take highway 1 or $-1$ to drive to a desired location. A ${\gamma }_1<0$ value indicates the first agent’s personal preference to avoid being on the same highway as the second. However, it should it be winter time, then the second agent, who always has a truck with a plow when driving on highway 1, creates a positive externality that can be captured with a $\beta$ value.

The final component is the kernel, which for Table 2 is ${\kappa }_1=1,{\kappa }_2=3$. This can be treated as an inflationary term that adds the same ${\kappa }_i$ value to each of the $ith$ agent’s payoffs. Methods that compare payoffs cancel the kernel value, so, as in this paper, the kernel can be ignored.

It is important to point out that the individual and coordinative pressure components contain all information from a game that is needed to compute the Nash equilibrium and to analyze related strategic solution concepts [JS1]. To appreciate why this is so, recall that the Nash information relies on payoff comparisons with unilateral deviations. But with the pure externality and kernel components, all unilateral payoff differences equal zero, so they contain no Nash information. This also means that “best response” solutions and methods ignore, and are not affected by the wealth of a game’s $\beta$ information (for explicit examples, see [11]).

By involving eight orthogonal directions and independent variables, these components span the eight-dimensional space of all $2\times 2$ games. Consequently, any $2\times 2$ game can be expressed and analyzed in terms of these eight coordinates. The equations converting a game into this form are

$$\begin{array}{ccc}{\alpha }_1=\frac{1}{4}[(a_1+a_3)-(a_2+a_4)],& {\alpha }_2=\frac{1}{4}[(b_1+b_3)-(b_2+b_4)],& {\gamma }_1=\frac{1}{4}[(a_1+a_4)-(a_2+a_3)],\\ {\gamma }_2=\frac{1}{4}[(b_1+b_4)-(b_2+b_3)],& {\kappa }_1=\frac{1}{4}[a_1+a_2+a_3+a_4],& {\kappa }_2=\frac{1}{4}[b_1+b_2+b_3+b_4],\\ {\beta }_1=\frac{1}{2}[a_1+a_2]-{\kappa }_1,& {\beta }_2=\frac{1}{2}[b_1+b_2]-{\kappa }_2.\end{array}$$

(1)

For interpretations, ${\kappa }_j$ is agent j’s average payoff, ${\beta }_j$ is agent j’s average payoff should the other agent play 1 minus the inflationary ${\kappa }_j$ value, ${\alpha }_j$ is half the difference of agent j’s average payoff if the other agent plays 1 and the average if the other agent plays $-1$, and ${\gamma }_j$ is half the difference of the $jth$ agent’s average TL, BR payoff, and average BL and TR payoff.

To illustrate the derivation of Equation (1), the ${\alpha }_1$ value of the

Table 4. Decomposing a game.

	$+1$		$-1$			$+1$		$-1$
$+1$	12	10	2	2	$+1$	1	0	1	0
$-1$	0	4	6	0	$-1$	$-1$	0	$-1$	0
a. $\mathcal{G}:$ A special case					b. ${\mathcal{G}}^{{\alpha }_1}:$ Where ${\alpha }_1=1,{\alpha }_2=0$

a game is computed. All that is needed is a standard vector analysis to find how much of game $\mathcal{G}$ is in the ${\alpha }_1$ coordinate direction, which is denoted by ${\mathcal{G}}^{{\alpha }_1}$, where ${\alpha }_1=1$ and ${\alpha }_2=0$. The sum of the squares of the ${\mathcal{G}}^{{\alpha }_1}$ entries (which in the following notation equals $[{\mathcal{G}}^{{\alpha }_1},{\mathcal{G}}^{{\alpha }_1}]$) is $1^2+0^2+1^2+0^2+{(-1)}^2+0^2+{(-1)}^2+0^2=4,$ so, according to vector analysis, ${\alpha }_1=\frac{1}{4}[\mathcal{G},{\mathcal{G}}^{{\alpha }_1}]$. Here, $[\mathcal{G},{\mathcal{G}}^{{\alpha }_1}]$ is the sum of the products of corresponding entries from each cell. (Identifying a game’s payoffs with components of a vector in ${\mathbb{R}}^8$, $[{\mathcal{G}}_1,{\mathcal{G}}_2]$ is the scalar product of the vectors.) In this example, $[\mathcal{G},{\mathcal{G}}^{{\alpha }_1}]=\left(12\right)\left(1\right)+\left(10\right)\left(0\right)+\left(2\right)\left(1\right)+\left(2\right)\left(0\right)+\left(0\right)(-1)+\left(4\right)\left(0\right)+\left(6\right)(-1)+\left(0\right)\left(0\right)=8,$, so ${\alpha }_1=\frac{1}{4}[\mathcal{G},{\mathcal{G}}^{{\alpha }_1}]=2.$. Similarly, by defining a corresponding ${\mathcal{G}}^{{\alpha }_2},{\mathcal{G}}^{{\gamma }_1},\cdots ,$, the remaining values are ${\alpha }_2=3,{\gamma }_1=4,{\gamma }_2=1,{\beta }_1=1,{\beta }_2=2,{\kappa }_1=5,$ and ${\kappa }_2=4.$ The Equation (1) expressions can be recovered in this manner.

This decomposition simplifies the analysis by extracting the portion from each payoff that contributes to these different attributes of a game. Illustrating with Table 1, rather than handling each entry separately, behavior can be analyzed with the separated impact of the components. For instance, the $a_1$ entry is $a_1={\alpha }_1+{\gamma }_1+{\beta }_1+{\kappa }_1,$ which, for Table 2, is $a_1=-3=2-3-3+1.$

To connect this notation with [JS1], [JS2], a game’s Nash component, denoted by ${\mathcal{G}}^N$, is the sum of the individual preference and coordinative pressure components as given in

Table 5. The ${\mathcal{G}}^N$ Nash component.

	$+1$		$-1$
$+1$	${\eta }_{1,1}={\alpha }_1+{\gamma }_1$	${\eta }_{1,2}={\alpha }_2+{\gamma }_2$	${\eta }_{2,1}={\alpha }_1-{\gamma }_1$	$-{\eta }_{1,2}=-{\alpha }_2-{\gamma }_2$
$-1$	$-{\eta }_{1,1}=-{\alpha }_1-{\gamma }_1$	${\eta }_{2,2}={\alpha }_2-{\gamma }_2$	$-{\eta }_{2,1}=-{\alpha }_1+{\gamma }_1$	$-{\eta }_{2,2}=-{\alpha }_2+{\gamma }_2$

.

Principal facts about $2\times 2$ games follow.4 As a reminder, a game is a potential game if there exists a global payoff function that aggregates the unilateral incentive structure of the game. More precisely, the payoff difference obtained by an agent unilaterally deviating is reflected in the change of the potential function. Potential games are often called “common interest” games. Coordination games have pure strategy Nash equilibria precisely where the agents play the same strategy (i.e., the strategy profiles $(+1,+1)$ and $(-1,-1)$). On the other hand, anti-coordination games have pure strategy Nash equilibria when the agents play different strategies (i.e., the strategy profiles $(+1,-1)$ and $(-1,+1)$).

Theorem 1.

Generically (that is, all ${\mathcal{G}}^N$ and ${\beta }_j$ entries are nonzero), the following hold for $2\times 2$ normal form games $\mathcal{G}$.

 1.

A $\mathcal{G}$ cell is pure Nash if and only if all of the cell’s ${\mathcal{G}}^N$ entries are positive.

 2.

$\mathcal{G}$ is a potential game if and only if ${\gamma }_1={\gamma }_2$.

 3.

$\mathcal{G}$ is a coordination game if and only if $|{\gamma }_1|>|{\alpha }_1|$, $|{\gamma }_2|>|{\alpha }_2|$ and $sgn{\gamma }_1=sgn{\gamma }_2=1$. If $sgn{\gamma }_1=sgn{\gamma }_2=-1$, then $\mathcal{G}$ is an anti-coordination game.

 4.

All of the payoffs in a normal-form game, as in Table 3, can be expressed with the utility functions for agents 1 and 2 given by, respectively,

$${\pi }_1(t_1,t_2)={\alpha }_1{t}_1+{\gamma }_1{t}_1{t}_2+{\beta }_1{t}_2+{\kappa }_{1}and {\pi }_2(t_1,t_2)={\alpha }_2{t}_2+{\gamma }_2{t}_1{t}_2+{\beta }_2{t}_1+{\kappa }_2,$$

where $t_1$ and $t_2$ represent the strategy choice (either $+1$ or $-1$) of agents 1 and 2,

 5.

A potential function for a game with components described in Table 3 can be transformed into

$$P(t_1,t_2)={\alpha }_1{t}_1+{\alpha }_2{t}_2+\gamma t_1{t}_2,where\gamma ={\gamma }_1={\gamma }_2.$$

(2)

 6.

A potential game’s potential function is invariant to the pure externality and kernel components.

To explain certain comments, recall that a potential game has a potential function; if an agent changes a strategy, the change in the agent’s payoff equals the change in the potential function. To illustrate, suppose the first agent changes from strategy $t_1=1$ to $t_1=-1$, while agent 2 remains at $t_2=1.$ According to Table 2, the change in the first agent’s payoff is

$$[-{\alpha }_1-{\gamma }_1+{\beta }_1+{\kappa }_1]-[{\alpha }_1+{\gamma }_1+{\beta }_1+{\kappa }_1]=-2[{\alpha }_1+{\gamma }_1],$$

or $-2[{\alpha }_1+\gamma ]$ for potential games. According to Equation (2), the change in the potential is the same value

$$P(-1,1)-P(1,1)=[-{\alpha }_1+{\alpha }_2+\left(1\right)(-1)\gamma ]-[{\alpha }_1+{\alpha }_2+\left(1\right)\left(1\right)\gamma ]=-2[{\alpha }_1+\gamma ].$$

Statement 1 is proved in [JS1]. To prove the second assertion, in (Chap. 2 of [JS2]) it is shown that game $\mathcal{G}$ is a potential game if and only if it is orthogonal to the $2\times 2$ matching pennies game ${\mathcal{G}}^{pennies}$; 5this orthogonality condition is $[\mathcal{G},{\mathcal{G}}^{pennies}]=0$. A direct computation shows that the individual preference, pure externality, and kernel components always satisfy this condition. The coordinative pressure component satisfies the condition if and only if ${\gamma }_1={\gamma }_2$.

Statement 4 is a direct computation. Statement 5 is a direct computation showing that changes in an agent’s strategy have the same change in the potential function as in the player’s payoff. Statement 6 follows, because $P(t_1,t_2)$ (Equation (2)) does not have $\beta$ or $\kappa$ values. A proof of the remaining statement 3 is in [JS2]. For intuition, the players in a coordination game coordinate their strategies, which is the defining feature of the coordinative pressure component. Thus, for $\mathcal{G}$ to be a coordination game, the $\gamma$ values must dominate the game’s Nash structure.

3. Disagreement in Potential Games

These coordinates lead to explanations why different behavioral measures can differ about which is the "better" outcome for certain games. This discussion is motivated by innovation diffusion, which is typically modeled by using coordination games with two equilibria, so a key step is to identify the preferred equilibria. A common choice is the risk-dominant pure Nash equilibria. In part, this is because these equilibria have been connected to the long-run behavior of dynamics, such as log-linear learning [18]. Because a coordination game is a potential game, the potential function’s global maximum is the risk-dominant equilibrium (Theorem 2).

However, as developed next, there are many games where a maximizing strategy for the potential function differs from the profile that maximizes social welfare. This difference is what allows agents to do “better” by using a profile other than the one leading to the risk-dominant equilibrium. Young [5,6] creates such an example using the utilitarian measure of social welfare, which sums all of the payoffs in a given strategy profile, and Newton and Sercombe [7] discuss similar ideas. A first concern is whether their examples are sufficiently isolated that they can be ignored, or whether they are so prevalent that they must be taken seriously. As we show, the second holds.

An explanation for what causes these conflicting conclusions emerges from the Table 2 decomposition and Theorem 1. A way to illustrate these results is to create any number of new, more complex examples. To do so, start with the fact (Theorem 2.5 in [JS2]) that with $2\times 2$ games and two Nash equilibria, a Nash cell is risk dominant over the other Nash cell if and only if the product of the $\eta$ values (see Table 4) of the first is larger than the product of the $\eta$ values of the second. (To handle some of our needs, this result is refined in Theorem 2.) Of significance is that, although the $\beta$ terms obviously affect payoff values, they play no role in this risk-adverse dominance analysis.

To illustrate, let ${\gamma }_1={\gamma }_2=4$ (Theorems 1-2; to have a potential game) and ${\alpha }_1={\alpha }_2=1$ (Theorem 1-3; to have a coordination game). This defines the

Table 6. Conflicting measures.

	$+1$		$-1$			$+1$		$-1$
$+1$	5	5	$-3$	$-5$	$+1$	2	2	0	$-8$
$-1$	$-5$	$-3$	3	3	$-1$	$-8$	0	6	6
a. First example					b. Conflicting behavior

a game where the TL Nash cell (with $\left({\eta }_{1,1}\right)\left({\eta }_{1,2}\right)=25$) is risk dominant over the BR Nash cell (with $(-{\eta }_{1,1})(-{\eta }_{1,2})=9$). There remain simple ways to modify this game that make the payoffs of any desired cell, say BR, more attractive than the risk dominant TL. All that is needed is to select ${\beta }_j$ values that increase the payoffs for the appropriate Table 3c cell; for the BR choice, this requires choosing negative ${\beta }_1,{\beta }_2$ values (Table 3c). Although these values never affect the risk-dominant analysis, they enhance each player’s BR payoff while reducing their TL payoffs. The ${\beta }_1={\beta }_2=-3$ choices define the Table 6b game where each player receives a significantly larger payoff from BR than from the risk dominant TL! In both games, the Nash and risk dominance structures remain unchanged.

These coordinates make it possible to easily create a two-dimensional family of games with such properties. To do so, add Table 3c to the Table 6a game, and then select appropriate ${\beta }_1,{\beta }_2$ values to emphasize the payoffs of different cells. For instance, using Young’s welfare measure (the sum of a cell’s payoffs), no matter which cell is selected, suitable $\beta$ values exist to make that cell preferred to TL. It follows from the Table 3c structure, for instance, that a way to enhance the TR payoffs is to use ${\beta }_1<0$ and ${\beta }_2>0$ choices. Adding these values to the Table 6a game defines the TR cell values of $-3+|{\beta }_1|$ and $-5+{\beta }_2$ while the TL values are $5-|{\beta }_1|$ and $5+{\beta }_2$. Thus, the sum of TR cell values dominates the sum of TL values if and only if

$$(-3+|{\beta }_1\left|\right)+(-5+{\beta }_2)>(5-|{\beta }_1\left|\right)+(5+{\beta }_2),\mathrm{or}\mathrm{iff}-{\beta }_1=\left|{\beta }_1\right|>9.$$

(3)

$$\begin{array}{|cccc|}\hline 10& 11& 2& 7\\ 8& 4& 8& 6\\ \hline\end{array}=\begin{array}{|cccc|}\hline \hfill 1& \hfill 2& \hfill -3& \hfill -2\\ \hfill -1& \hfill -1& \hfill 3& \hfill 1\\ \hline\end{array}+\begin{array}{|cccc|}\hline \hfill 2& \hfill 2& \hfill -2& \hfill 2\\ \hfill 2& \hfill -2& \hfill -2& \hfill -2\\ \hline\end{array}+\begin{array}{|cccc|}\hline 7& 7& 7& 7\\ 7& 7& 7& 7\\ \hline\end{array}.$$

(4)

$$\mathrm{Conflict} \mathrm{among} 50-50, \mathrm{payoff}, \mathrm{and} \mathrm{risk} \mathrm{dominance}$$

The coordinates also make it possible to compare other measures by mimicking the above approach. Games with payoff dominant strategies that differ from the risk adverse ones, for example, require appropriate $\beta$ values. To explain, if BR is risk dominant, then, as in the Equation (4) game (from Section 2.6.4 in [JS2]), the product of its $\eta$ values from BR in ${\mathcal{G}}^N$ (first bimatrix on the right) is larger than the product of the TL ${\mathcal{G}}^N$ values. This product condition ensures that the only way the payoff and risk dominant cells can differ is by introducing TL $\beta$ components; this is illustrated with ${\beta }_1={\beta }_2=2$ in the second bimatrix in Equation (4). More generally and using just elementary algebra as in Equation (3), the regions (in the space of games) where the two concepts differ now can finally be determined.

For another measure, consider the 50–50 choice. This is where, absent any information about an opponent, it seems reasonable to assume there is a 50–50 chance the opponent will select one Nash cell over the other. This assumption suggests using an expected value analysis to identify which strategy a player should select. To discover what coordinate information this measure uses, if TL and BR are the two Nash cells, then for Row and this 50–50 assumption, the expected return from playing T is $\frac{1}{2}\left[\right|{\eta }_{1,1}|-|{\eta }_{2,1}\left|\right]+\frac{1}{2}[{\beta }_1-{\beta }_1]+\frac{1}{2}[{\kappa }_1+{\kappa }_1]=\frac{1}{2}\left[\right|{\eta }_{1,1}|-|{\eta }_{2,1}\left|\right]+{\kappa }_1.$ Similarly, the expected value of playing B is $\frac{1}{2}[-|{\eta }_{1,1}|+|{\eta }_{2,1}\left|\right]+\frac{1}{2}[{\beta }_1-{\beta }_1]+\frac{1}{2}[{\kappa }_1+{\kappa }_1]=\frac{1}{2}[-|{\eta }_{1,1}|+|{\eta }_{2,1}\left|\right]+{\kappa }_1.$ Consequently, an agent’s larger $\eta$ value completely determines the 50-50 choice. However, if the risk adverse cell of ${\mathcal{G}}^N$ is not also ${\mathcal{G}}^N$ payoff dominant, as true with the first bimatrix on the right in Equation (4), and if both players adopt the 50-50 measure, they will select a non-Nash outcome. Indeed, in Equation (4), BR is risk dominant, TL is payoff dominant, and BL, which is not a Nash cell (and Pareto inferior to both Nash cells), is the 50–50 choice. Again, elementary algebra of the Equation (3) form identifies the large region of games where this behavior can arise. (By using appropriate $\beta$ values, it is easy to create 50–50 outcomes that are disastrous.)

3.1. The Potential and Welfare Functions

Moving beyond examples, these coordinates can fully identify those games for which different measures agree or disagree, which is one of the objectives of this paper. The importance of this analysis is that it underscores our earlier comment that this conflict between the conclusions of measures is a fundamental concern that is suffered by a surprisingly large percentage of all $2\times 2$ games.

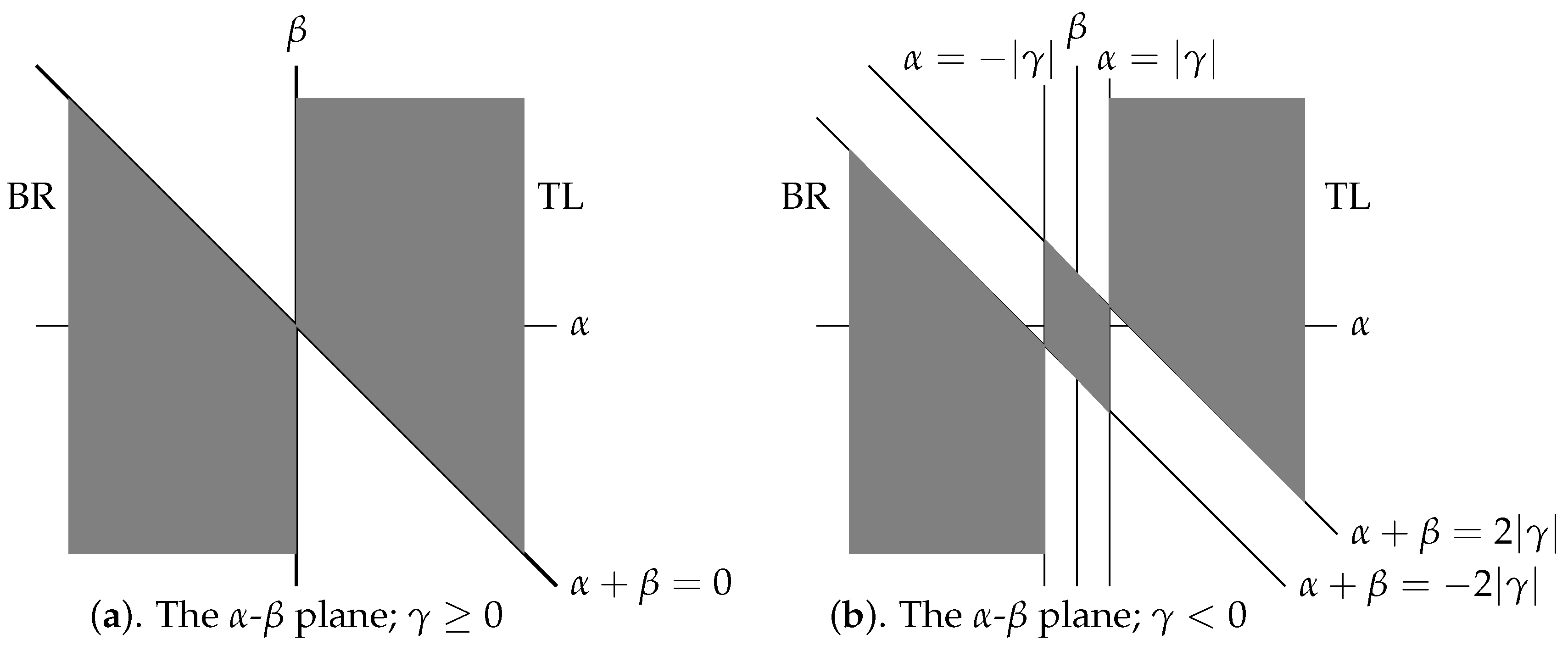
Our outcomes are described using maps of the space of $2\times 2$ games. The maps show where the potential and social welfare functions (e.g., the ones used by Young [5,6] and Newton and Sercombe [7]) agree, or disagree, on which is the "better" choice of two equilibria. Not only do these diagrams demonstrate the preponderance of this conflict, but they identify which behavior a specific game will experience. As an illustration, the dark regions of Figure 1 single out those potential games (so ${\gamma }_1={\gamma }_2$), where ${\alpha }_1={\alpha }_2=\alpha$ and ${\beta }_1={\beta }_2=\beta$, which are without conflict; for games in the unshaded regions, different measures support different outcomes.

Thanks to the coordinate system for games, the game theoretic analysis is surprisingly simple; it merely uses a slightly more abstract version of the Equation (3) analysis. To illustrate with the above 50-50 discussion, if the Nash cells are TL and BR, then ${\eta }_{1,1},-{\eta }_{2,1},{\eta }_{1,2},-{\eta }_{2,2}$ are all positive (if the Nash cells are BL and TR, all of these entries are negative). Consequently, the two surfaces ${\eta }_{1,1}+{\eta }_{2,1}=0$ and ${\eta }_{1,2}+{\eta }_{2,2}=0$ separate which one of an agent’s $\eta$ values is larger. Even though the discussion applies to the four dimensional space of $\eta$ values, one can envision the huge wedges these surfaces define where the $\eta$ values force the 50–50 approach to select a non-Nash outcome.

A similar approach applies to all of the maps derived here. In Figure 1a, the dividing surface separating which Nash cell is selected by potential function outcomes is $\alpha =0$; if $\alpha >0$, the game’s top Nash choice for the potential function is TL; if $\alpha <0$, the top Nash choice is BR. However, the social welfare conclusion is influenced by $\beta$ values, so it will turn out that the separating line between a social welfare function selecting TL or BR is the $\alpha +\beta =0$ line. Above this line, TR is the preferred Nash choice; below it is BR. Given this legend, Figure 1a demonstrates those games for which the different measures agree or disagree about the top choices, and the magnitude of the problem. Stated simply, regions that emphasize behavioral terms place emphasis on payoff and social welfare dominant measures; regions that emphasize Nash strategic terms emphasize risk dominant measures.

Stated differently, difficulties in what follows do not reflect the game theory; the coordinate system handles all of these problems. Instead, all of the complications (there are some) reflect the geometric intricacy of the seven-dimensional space of $2\times 2$ potential games. Consequently, readers that are interested in applying this material to specific games should emphasize the maps and their legends (given in the associated theorems). Readers that are interested in the geometry of the space of potential functions will find the following technical analysis of value. However, first, a fundamental conclusion about potential games is derived.

3.2. A Basic “Risk Dominant” Theorem

The coordinates explicitly display a tension between what individuals can achieve on their own (Nash behavior) and with cooperative forces. With a focus on individualistic forces, the potential function is useful because its local maxima are pure Nash equilibria. Even more, as known, the potential’s global maximum is the risk dominant equilibrium. This fact is re-derived for $2\times 2$ potential games in a manner that now highlights the roles of a potential game’s coordinates.

Theorem 2.

For a $2\times 2$ potential game $\mathcal{G}$, its potential function has a global maximum at the strategy profile $(t^{\prime },t^{″})$ if and only if $(t^{\prime },t^{″})$ is $\mathcal{G}$’s risk-dominant Nash equilibrium. With two Nash equilibria where one is risk dominant, $(t^{\prime },t^{″})$ is the risk dominant strategy if and only if the following inequalities hold

$$\mathbf{a}.({\alpha }_1{t}^{\prime }+{\alpha }_2{t}^{″})>0,\mathbf{b}.\left|\gamma \right|>|{\alpha }_1|,|{\alpha }_2|,\mathbf{c}.t^{\prime }{t}^{″}\gamma >0.$$

(5)

As shown in the proof, inequality c identifies the Nash cells; e.g., if $\gamma <0$, then $t^{\prime }{t}^{″}=-1$, so the Nash cells are BL and TR. With two Nash cells, the inequality a identifies which one is a global maximum of the potential function. Similarly, inequality b requires $\gamma$ to have a sufficiently large value to create two Nash cells. Of importance, Equation (5) does not include $\beta$ values!

To illustrate these inequalities, let ${\alpha }_1=1,{\alpha }_2=-2,$ and $\gamma =3$. By satisfying Equation (5b), there are two Nash cells. According to Equation (5c), $t^{\prime }{t}^{″}=1$, so $t^{\prime }=t^{″}$, which positions the Nash cells at TL and BR. From Equation (5a), $t^{\prime }-2t^{\prime }>0$, or $t^{\prime }<0$, so the risk dominant strategy is the $t^{\prime }=t^{″}=-1$ BR cell. Conversely, to create an example where a desired cell, say TR, is risk dominant, the $t^{\prime }=1,t^{″}=-1$ values require (Equation (5c)) $\gamma <0$ and (Equation (5a)) ${\alpha }_1>{\alpha }_2.$ Finally, select $\gamma <0$ that satisfies Equation (5b); e.g., ${\alpha }_1=1,{\alpha }_2=-1$ and $\gamma =-2$ suffice.

The Equation (5) inequalities lead to the following conclusion.

Corollary 1.

If a $2\times 2$ potential game $\mathcal{G}$ has two pure Nash equilibria where one is risk dominant, then $\mathcal{G}$ is a coordination game. If $\gamma >0$, the Nash cells are at TL and BR, where $\mathcal{G}$ is a coordination game. If $\gamma <0$, the Nash cells are at BL and TR, where $\mathcal{G}$ is an anti-coordination game.6

Proof of Corollary 1.

According to Theorem 2, the Corollary 1 hypothesis ensures that Equation (5) hold. With the b inequality, it follows from Theorem 1-3 that $\mathcal{G}$ is a coordination game. In a $2\times 2$ game, pure Nash cells are diagonally opposite. If $\gamma >0$, it follows from Equation (5), c that the Nash strategies satisfy $t^{\prime }{t}^{″}=1,$ so the Nash cells are at TL (for $t^{\prime }=t^{″}=1$) and BR (for $t^{\prime }=t^{″}=-1$), and that this is a coordination game. Similarly, if $\gamma <0$, then $t^{\prime }{t}^{″}=-1$, so the Nash cells are at BL (for $t^{\prime }=-1,t^{″}=1$) and TR (for $t^{\prime }=1,t^{″}=-1$) to define an anti-coordination game. □

Proof of Theorem 2.

In a non-degenerate case (i.e., $P(t_1,t_2)$ is not a constant function), P has a maximum, so there exists at least one pure Nash cell. If a game has a unique pure strategy Nash equilibrium, then, by default, it is risk-dominant and P’s unique maximum.

Assume there are two Nash cells; properties that the potential, P, must satisfy at a global maximum are derived. Pure Nash equilibria must be diametrically opposite in a normal form representation, so if $\mathcal{G}$ has two pure strategy Nash equilibria where one is $(t^{\prime },t^{″})$, then the other one is at $(-t^{\prime },-t^{″})$. Consequently, if P has a global maximum at $(t^{\prime },t^{″}),$, then P has a local maximum at $(-t^{\prime },-t^{″})$, so $P(t^{\prime },t^{″})>P(-t^{\prime },-t^{″}).$ According to Equation (2), this inequality holds iff $[{\alpha }_1{t}^{\prime }+{\alpha }_2{t}^{″}+\gamma \left(t^{\prime }\right)\left(t^{\prime \prime }\right)]-[{\alpha }_1(-t^{\prime })+{\alpha }_2(-t^{\prime \prime })+\gamma (-t^{\prime })(-t^{\prime \prime })]=2[{\alpha }_1{t}^{\prime }+{\alpha }_2{t}^{″}]>0$, which is inequality Equation (5)a.

The local maximum structure of $P(-t^{\prime },-t^{″})$ requires that $P(-t^{\prime },-t^{″})>P(t^{\prime },-t^{″})$ and $P(-t^{\prime },-t^{″})>P(t^{\prime },-t^{″}).$ Again, according to Equation (2), the first inequality is true if

$${\alpha }_1(-t^{\prime })+{\alpha }_2(-t^{″})+\gamma (-t^{\prime })(-t^{″})>{\alpha }_1{t}^{\prime }+{\alpha }_2(-t^{″})+\gamma \left(t^{\prime }\right)(-t^{″}),$$

or $\gamma t^{\prime }{t}^{\prime }>{\alpha }_1{t}^{\prime }$. Similarly, the second inequality is true iff $\gamma t^{\prime }{t}^{″}>{\alpha }_2{t}^{″}.$ Thus, for a potential game with two Nash cells, P has a global maximum at $(t^{\prime },t^{″})$ iff

$$[{\alpha }_1{t}^{\prime }+{\alpha }_2{t}^{″}]>0,\gamma t^{\prime }{t}^{″}>{\alpha }_1{t}^{\prime },\gamma t^{\prime }{t}^{″}>{\alpha }_2{t}^{″},\gamma t^{\prime }{t}^{″}>0.$$

(6)

The last inequality follows from the first one, which requires at least one of ${\alpha }_1{t}^{\prime },{\alpha }_2{t}^{″}$ to be positive. Thus, the $\gamma t^{\prime }{t}^{″}>0$ inequality follows from either the second or third inequality.

All that is needed to establish the equivalence of the Equation (6) inequalities and those of Equation (5) is that Equation (5b) is equivalent to the two middle inequalities of Equation (6). Equation (5b) implies the two middle inequalities of Equation (6) is immediate. In the opposite direction, the first Equation (6) inequality requires at least one of ${\alpha }_1{t}^{\prime }$ or ${\alpha }_2{t}^{″}$ to be positive. If it is ${\alpha }_{j}t$, then because $\left|\gamma \right|=\gamma t^{\prime }{t}^{″}$, this positive term requires the appropriate middle inequality of Equation (6) to be $\left|\gamma \right|>|{\alpha }_j|$. If it holds for both terms, the proof is completed. If it holds for only one term, say ${\alpha }_1{t}^{\prime }>0$, but ${\alpha }_2{t}^{″}<0$, then the first Equation (6) inequality requires that $|{\alpha }_1|>|{\alpha }_2|$, which completes the proof.

The second step requires showing that $(t^{\prime },t^{″})$ is a risk-dominant Nash equilibrium if Equation (5) holds. According to Harsanyi and Selten (1988), $(t^{\prime },t^{″})$ is a game’s risk-dominant Nash equilibrium if

$$(P(-t^{\prime },t^{″})-P(t^{\prime },t^{″}))(P(t^{\prime },-t^{″})-P(t^{\prime },t^{″}))>(P(t^{\prime },-t^{″})-P(-t^{\prime },-t^{″}))(P(-t^{\prime },t^{″})-P(-t^{\prime },-t^{″})),$$

(7)

which is $(-2{\alpha }_1{t}^{\prime }-2\gamma t^{\prime }{t}^{″})(-2{\alpha }_2{t}^{\prime }-2\gamma t^{\prime }{t}^{″})>(2{\alpha }_1{t}^{\prime }-2\gamma t^{\prime }{t}^{″})(2{\alpha }_2{t}^{\prime }-2\gamma t^{\prime }{t}^{″}).$ This inequality reduces to

$$\gamma t^{\prime }{t}^{″}({\alpha }_1{t}^{\prime }+{\alpha }_2{t}^{″})>0.$$

(8)

If $t^{\prime }{t}^{″}=1$, the Nash cells are at TL and BR, so both entries of these two Table 4 cells must be positive (Theorem 1-1). For the TL cell, this means that $\gamma >-{\alpha }_1,-{\alpha }_2,$, while for the BR cell it requires $\gamma >{\alpha }_1,{\alpha }_2.$ Consequently, $\gamma >|{\alpha }_1|,|{\alpha }_2|$ and $\gamma >0$: inequalities b and c of Equation (5) are satisfied. That inequality a of Equation (5) holds follows from $\gamma t^{\prime }{t}^{″}>0$ and Equation (8).

Similarly, if $t^{\prime }{t}^{″}=-1$, then BL and TR are the Nash cells. Again, each entry of each of these Table 4 cells must be positive: from BL, we have that $-{\alpha }_1,{\alpha }_2>\gamma ,$, while from TR we have that ${\alpha }_1,-{\alpha }_2>\gamma .$ Consequently, $\gamma <0,$$\gamma t^{\prime }{t}^{″}>0$ and $\left|\gamma \right|>|{\alpha }_1|,|{\alpha }_2|;$ these are inequalities b and c of Equation (5). That inequality a holds again follows from Equation (8). □

A consequence of Theorem 2 is that the potential function can serve as a comparison measure of Nash outcomes. Other natural measures reflect the overall well-being of all agents, such as the utilitarian social welfare function that sums each strategy profile’s payoffs. To obtain precise conclusions, our results use this social welfare function. However, as indicated later, everything extends to several other measures.

3.3. Symmetric Games

Without question, it is difficult to understand the structures of a four-dimensional object, leave alone the seven-dimensions of the space of $2\times 2$ potential games. Thus, to underscore the ideas, we start with the simpler (but important) symmetric games (all symmetric games are potential games); doing so reduces the dimension of the space of games from seven to four (with the kernel). This is where ${\alpha }_1={\alpha }_2=\alpha ,{\beta }_1={\beta }_2=\beta ,{\gamma }_1={\gamma }_2=\gamma ,$ and ${\kappa }_1={\kappa }_2=\kappa .$ Ignoring the kernel term, the coordinates are given in

Table 7. Decomposition for a symmetric potential game.

	$+1$		$-1$			$+1$		$-1$
$+1$	$\alpha +\gamma$	$\alpha +\gamma$	$\alpha -\gamma$	$-\alpha -\gamma$	$+1$	$\beta$	$\beta$	$-\beta$	$\beta$
$-1$	$-\alpha -\gamma$	$\alpha -\gamma$	$-\alpha +\gamma$	$-\alpha +\gamma$	$-1$	$\beta$	$-\beta$	$-\beta$	$-\beta$
a. Nash terms					b. Behavioral, externality terms

.

To interpret Table 7, the Nash entries combine terms, where each agent prefers a particular strategy independent of what the other agent does (if $\alpha >0$, each agent prefers to play 1; if $\alpha <0$ each agent prefers $-1$) with terms that impose coordination pressures. That is, if $\gamma >0$, the game’s Nash structure inflicts a pressure on both agents to coordinate; if $\gamma <0$, the game’s joint pressure is for anti-coordination. With symmetric games, the payoffs for both agents agree at TL and at BR, so if one of these cells is social welfare top-ranked (the sum of the entries is larger than the sum of entries of any other cell), the cell also has the stronger property of being payoff dominant.

All $2\times 2$ symmetric games can be expressed with these four $\alpha ,\beta ,\gamma ,\kappa$ variables (Equation (1)). Ignoring $\kappa$, the remaining variables define a three-dimensional system. To tie all of this in with commonly used symmetric games, if BR is the sole Nash point, then the above defines a Prisoner’s Dilemma game if $-\alpha +\gamma >0$ and $\alpha +\gamma +\beta >-\alpha +\gamma -\beta$, the second of which reduces to $\beta +\alpha >0$. Similarly, the Hawk–Dove game with Nash points at BL and TR, and “hawk” strategy as $+1$, has $-\alpha -\gamma >0$ and $\alpha -\gamma >0$ for the Nash points, and $\beta <0$ to enhance the BR payoffs, so $\gamma <0$, $\alpha <-\left|\gamma \right|$, and $\beta <0.$ A coordination game simply has $\gamma >\left|\alpha \right|$ (these inequalities allow the different games to easily be located in Figure 1 and elsewhere). As stated, because ${\gamma }_1={\gamma }_2$, it follows (Theorem 1-2) that all $2\times 2$ symmetric games are potential games with the potential function (Equation (2))

$$P(t_1,t_2)=\alpha (t_1+t_2)+\gamma t_1{t}_2$$

(9)

A computation (Table 7) proves that the social welfare function (the sum of a cell’s entries) is

$$w(t_1,t_2)=(\alpha +\beta )(t_1+t_2)+2\gamma t_1{t}_2.$$

(10)

Our goal is to identify those games for which the potential and social functions agree, or disagree, on the ordering of strategies. Here, the following results are useful.

Theorem 3.

A $2\times 2$ symmetric potential game $\mathcal{G}$ satisfies

$$w(t_1,t_2)=2P(t_1,t_2)+(\beta -\alpha )(t_1+t_2).$$

(11)

If $\alpha =\beta$, the potential function and the welfare function rankings of $\mathcal{G}$’s four cells agree.

Both the potential and welfare functions are indifferent about the ranking of the BL and TR cells, denoted as $BL\sim TR$. If one of these cells is a Nash cell for $\mathcal{G}$, then so is the other one, but neither is risk dominant.

Equation (11) explicitly demonstrates that $\beta$ values—the game’s behavioral or externality values—are solely responsible for all of the differences between how the potential and social welfare functions rank $\mathcal{G}$’s cells.

Proof. 

Adding and subtracting $\alpha (t_1+t_2)$ to Equation (10) leads to Equation (11). Thus, if $\alpha =\beta$, then $\frac{1}{2}w(t_1,t_2)=P(t_1,t_2),$ so both functions have the same ranking of $\mathcal{G}$’s four cells.

The BL and TR cells correspond, respectively, to $(t_1=-1,t_2=1)$ and $(t_1=1,t_2=-1)$, where $t_1+t_2=0.$ Thus, (Equation (11)), the $\frac{1}{2}w$, and P values for each of these cells is $\gamma$. As both measures have the same value for each cell, both have a tie ranking for the two cells denoted by $BL\sim TR$.

The Nash entries of BL and TR are the same but in different order (Table 6a). Thus, if both entries of one of these cells are positive, then so are both entries of the other. This means that both are Nash cells (Theorem 1-1). The risk dominant assertion follows because inequality a of Equation (5) is not satisfied; it equals zero. Equivalently, P does not have a unique global maximum in this case. □

A Map of Games and Symmetries

A portion of a map that describes the structure of all $2\times 2$ games (Chapter 3, [JS2]) is expanded here to concentrate on the symmetric games. While variables $\alpha ,\gamma ,\beta$ require a three-dimensional space, the potential function does not depend on $\beta$, so Figure 2a highlights the $\alpha$-$\gamma$ plane. Treat the positive $\beta$ axis as coming out orthogonal to the page.

Changes in the potential game and P (Equation (9)) depend on $\alpha ,\gamma ,\gamma -\alpha ,$ and $\gamma +\alpha$ values, which suggests dividing the $\alpha$-$\gamma$ plane into sectors where these terms have different signs. That is, divide the plane into eight regions (Figure 2a) with the lines $\alpha =0$, $\gamma =0$, $\alpha =\gamma$, and $-\alpha =\gamma$. The first two lines represent changes in a game’s structure by varying the $\alpha$ and $\gamma$ signs. For instance, reversing the sign of $\alpha$ changes which strategy the agents prefer; swapping the $\gamma$ sign exchanges a game’s coordination, anti-coordination features. The other two lines are where certain payoffs (Table 6a) change sign, which affects the game’s Nash structure. The labelling of the regions follows:

$$\begin{array}{cccccccc}\left(1\right)& \hfill \gamma >\alpha >0& \left(2\right)& \hfill \alpha >\gamma >0& \left(3\right)& \hfill \alpha >-\gamma >0& \left(4\right)& \hfill -\gamma >\alpha >0\\ \left(5\right)& \hfill \gamma >-\alpha >0& \left(6\right)& \hfill -\alpha >\gamma >0& \left(7\right)& \hfill -\alpha >-\gamma >0& \left(8\right)& \hfill -\gamma >-\alpha >0\end{array}$$

A natural symmetry simplifies the analysis. In Table 6, interchanging each matrix’s rows and then columns creates an equivalent game, where the $t^{\prime },t^{″}$ cell in the original becomes the $-t^{\prime },-t^{″}$ cell in the image. This equivalent game is identified in Figure 2a with the mapping

$$F(\alpha ,\gamma ,\beta )=(-\alpha ,\gamma ,-\beta ).$$

(12)

Geometrically, F flips a point on the right (a symmetric potential game) about the $\gamma$ axis to a point on the left (which corresponds to the described changing of rows and columns of the original game); e.g., in Figure 2a, the bullet in region 2 is flipped to the bullet in region 6. Similarly, the original $\beta$ value is flipped to the other side of the $\alpha$-$\gamma$ plane. Consequently, anything stated about a $(t^{\prime },t^{″})$ strategy or cell for a game in region k holds for a $(-t^{\prime },-t^{″})$ strategy or cell of the corresponding game in region ($k+4$). Thanks to this symmetry, by determining the P ranking of region k to the right of the $\gamma$ axis, the P ranking of region $k+4$ is also known.

The following theorem describes each region’s P ranking. The reason the decomposition structure simplifies the analysis is that all of the comments about Nash cells follow directly from Table 6a. If, for instance, $\gamma >\alpha >0$ (region 1), then only cells TL and BR have all positive entries (Table 6a), so they are the only Nash cells. Similarly, if $-\gamma >\alpha >0$ (region 4), only cells BL and TR have all positive entries, so they are the Nash cells (Theorem 1-1). Each cell’s P value is specified in matrix

Table 8. A symmetric potential game’s P and $\frac{w}{2}$ values.

	$+1$	$-1$		$+1$	$-1$
$+1$	$2\alpha +\gamma$	$-\gamma$	$+1$	$\gamma +[\alpha +\beta ]$	$-\gamma$
$-1$	$-\gamma$	$\gamma -2\alpha$	$-1$	$-\gamma$	$\gamma -[\alpha +\beta ]$
a. P values			b. $\frac{w}{2}$ values

a, so the P ranking of the cells follows immediately. Region 2, for instance, has $\alpha >\gamma >0$, so TL is P’s top-ranked cell. Whether BL is P-ranked over BR holds (Table 8a) iff $-\gamma >\gamma -2\alpha$, or $\alpha >\gamma$, which is the case. This leads to P’s ranking of $TL\succ (BL\sim TR)\succ BR$ for all region 2 games (here, $A\succ B$ means A is ranked over B and $A\sim B$ means they are tied). Each cell’s $\frac{1}{2}w$’s value (half the social welfare function), which comes from Equation (11), is given in Table 8b.

Theorem 4.

The following hold for a $2\times 2$ symmetric potential game.

 1.

Region 1 has Nash equilibria at TL and BR, where TL is risk dominant. The P ranking of the cells is $TL\succ BR\succ (BL\sim TR).$ The region 5 P ranking is $BR\succ TL\succ (BL\sim TR)$; BR is risk dominant.

 2.

Regions 2 and 3 have a single Nash cell at TL, where, for each region, the P ranking of the cells is $TL\succ (BL\sim TR)\succ BR.$ The P ranking in regions 6 and 7 is $BR\succ (BL\sim TR)\succ TL.$

 3.

Region 4 has Nash cells at BL and TR, where neither is risk dominant. The P ranking is $(BL\sim TR)\succ TL\succ BR.$ The region 8 P ranking is $(BL\sim TR)\succ BR\succ TL.$

The content of this theorem is displayed in Figure 2b. Notice, with $\alpha >0$, the potential function has BR bottom ranked unless BR is a Nash cell. This makes sense; $\alpha >0$ means (Table 3a) that both agents prefer a “$+1$” strategy, so they prefer T and L. In fact, with $\alpha >0$, the only way TL loses its top-ranked P status is with a sufficiently strong negative $\gamma$ value (region 4 of Figure 2a). This also makes sense; a negative $\gamma$ value (Table 3b) captures the game’s anti-coordination flavor, which, if strong enough, can crown BL and TR as Nash cells.

Similar comments hold for $\alpha <0$; this is because TL and BR reverse roles in the P rankings (properties of F (Equation (12)). Thus, the P ranking of region 1 is $TL\succ BR\succ (BL\sim TR),$ so the P ranking of region 5 is $BR\succ TL\succ (BL\sim TR).$ Accordingly, for $\alpha <0$, P always bottom-ranks TL unless TL is a Nash cell, which reflects that $\alpha <0$ is where the players have a preference for B and R.

The next theorem describes where the potential and social welfare function rankings agree or disagree. As its proof relies on Table 8b values, it is carried out in the same manner as for Theorem 4. Namely, to determine whether TL is ranked above BL or TR, it must be determined (Table 8b) whether $\gamma +(\alpha +\beta )>-\gamma$, or whether $2\gamma >\alpha +\beta >0$. Next, according to (Table 8b), the social welfare function ranks BR above BL (or TR) iff $\gamma -(\alpha +\beta )>-\gamma$, or $2\gamma >\alpha +\beta >0$. Thus, if $2\gamma >\alpha +\beta >0$, the social welfare ranking is $TL\succ BR\succ (BL\sim TR).$

Theorem 5.

For a $2\times 2$ symmetric potential game with a coordinative flavor of $\gamma >0$, the social welfare function (Equation (10)) ranks the cells in the following manner:

 1.

If $(\alpha +\beta )>2\gamma ,$ the ranking is $TL\succ (BL\sim TR)\succ BR$ and TL is payoff dominant,

 2.

if $2\gamma >(\alpha +\beta )>0$, the ranking is $TL\succ BR\succ (BL\sim TR)$ and TL is payoff dominant,

 3.

if $0>(\alpha +\beta )>-2\gamma$, the ranking is $BR\succ TL\succ (BL\sim TR)$ and BR is payoff dominant, and

 4.

if $-2\gamma >(\alpha +\beta )$, the ranking is $BR\succ (BL\sim TR)\succ TL$ where BR is payoff dominant.

For games with an anti-coordinative flavor of $\gamma <0$, the social welfare rankings are

 5.

if $(\alpha +\beta )>-2\gamma$, the ranking is $TL\succ (TR\sim BL)\succ BR$ and TL is payoff dominant;

 6.

if $-2\gamma >(\alpha +\beta )>0$, the ranking is $(BL\sim TR)\succ TL\succ BR$,

 7.

if $0>(\alpha +\beta )>2\gamma$, the ranking is $(BL\sim TR)\succ BR\succ TL,$ and

 8.

if $0>2\gamma >(\alpha +\beta )$, the ranking is $BR\succ (BL\sim TR)\succ TL$ and BR is payoff dominant.

As with Theorem 4, this theorem ignores certain equalities, such as $\alpha +\beta =2\gamma >0$, but the social welfare ranking is the obvious choice. This equality captures the transition between parts 1 and 2, so its associated ranking is $TL\succ BR\sim (BL\sim TR).$

A message of these theorems is to anticipate strong differences between the potential and social welfare rankings. Each game in region 1 of Figure 2a, for instance, has the unique P ranking of $TL\succ BR\succ (BL\sim TR)$, so TL is P’s “best” cell. In contrast, each game in each region has four different social welfare rankings7 where most of them involve ranking conflicts! In region 1 of Figure 2a, for instance, an admissible social welfare ranking (Theorem 5-4) is $BR\succ (BL\sim TR)\succ TL$, where the payoff dominant BR is treated as being significantly better than P’s top choice of TL.

The coordinates explicitly identify why these differences arise: The potential function ignores $\beta$, while the $\beta$ values contribute to the social welfare rankings. By influencing a game’s payoffs and identifying (positive or negative) externalities that players can impose on each other, the $\beta$ values constitute important information about the game. To illustrate, Table 5 has two different symmetric games; they differ only in that the first game has $\beta =0$ with no externalities while the second has $\beta =-3$, which is a sizable externality favoring BR payoffs (Table 6b). Both of the games are in region 1 of Figure 2, so both have the same P ranking of $TL\succ BR\succ (BL\sim TR)$ (Theorem 4-1), where TL is judged the better of the two Nash cells. However, the social welfare ranking for the Table 5b game is $BR\succ TL\succ (BL\sim TR)$, which disagrees with the P ranking by crowning BR as the superior cell. By examining this Table 5b game, which includes externality information, it would seem to be difficult to argue otherwise.

Viewed from this externality perspective, Theorem 5 makes excellent sense. It asserts that, with sufficiently large positive $\beta$ values, the social welfare function favors TL, which must be expected. The decomposition (Table 6b) requires $\beta >0$ to favor TL payoffs. Conversely, $\beta <0$ enhances the BR payoffs.

The potential and social welfare rankings can even reverse each other. According to Theorem 4-2, all games in region 2 of Figure 2a have a single Nash cell with the P ranking of $TL\succ (BR\sim TR)\succ BR.$ This region requires $\alpha >\gamma >0$, so the

Table 9. Conflicting measures.

	$+1$		$-1$			$+1$		$-1$
$+1$	4	4	2	$-4$	$+1$	$-2$	$-2$	8	$-10$
$-1$	$-4$	2	$-2$	$-2$	$-1$	$-10$	8	4	4
a. Example with $\beta =0$					b. Example with $\beta =-6$

a example is constructed with $\alpha =3,\gamma =1$. For this game, where $\beta =0$, the P and social welfare rankings agree. To modify the game to obtain the reversed social welfare ranking of $BR\succ (BL\sim TR)\succ TL$, where the non-Nash cell BR will be the social welfare function’s best choice, Theorem 5 describes precisely what to do; select $\beta$ values that satisfy $(\alpha +\beta )<-2\gamma$. For Table 9, this means that $\beta <-2\left(1\right)-3=-5.$ The $\beta =-6$ choice leads to the Table 9b game, where the social welfare ranking reverses that of the potential function. Again, it is difficult to argue against this game’s social welfare ranking.

4. Conflict and Agreement

These negative conclusions, where potential and social welfare rankings disagree, can be overly refined for many purposes. Similar to an election, the interest may be in the winner rather than who is in second, third, or fourth place. Thus, an effective but cruder measure is to determine where potential and social welfare functions have the same top-ranked cell.

All of the conflict in potential and social welfare rankings are strictly caused by $\beta$ values, which suggests identifying those $\beta$ values that allow the same potential and social welfare preferred cell. It is encouraging how answers follow from $\alpha$ and $\beta$ comparisons.

Corollary 2.

For symmetric $2\times 2$ games, the following hold for $\gamma \ge 0$:

 1.

The potential and social welfare functions have TL as the top-ranked and payoff dominant cell for $\alpha +\beta >0$ and $\alpha >0$ (shaded Figure 1a region on the right).

 2.

If $\alpha +\beta <0$ and $\alpha >0$ (unshaded region region on the right of Figure 1a), then BR is the social welfare top-ranked and payoff dominant cell, but the top-ranked P cell is $TL$.

 3.

If $\alpha +\beta <0$ and $\alpha <0$ (shaded Figure 1a region on the left), both functions have the BR cell top-ranked. BR also is the payoff dominant cell.

 4.

If $\alpha +\beta >0$ and $\alpha <0$ (unshaded region on the left of Figure 1a), TL is the social welfare top-ranked and payoff dominant cell, while the P top-ranked cell is $BR$.

The content of this corollary serves as a legend for the Figure 1a map; the shaded regions are where both measures have the same top-ranked cell. A simple way to interpret this figure is that for all games to the right of the $\beta$ axis ($\alpha >0$), P’s top-ranked cell is TL, while to the left it is BR. In contrast, above the $\alpha +\beta =0$ slanted line, the social welfare’s top-ranked cell is TL, while below it is BR. Thus, in the unshaded regions, one measure has BR top-ranked, while the other has TL.

This corollary and Figure 1a show that if the $\alpha$ value (indicating a preference of the agents for T and L or B and R) is not overly hindered by the externality forces (e.g., if $\alpha >0$ and $\beta >-\alpha$) then the potential and social welfare functions share the same top ranked cell. But should conflict arise between these two fundamental variables, where the $\alpha$ and $\beta$ values favor cells in opposite directions, disagreement arises between the choices of the top ranked P and social welfare cells.

Proof. 

The proof follows directly from Table 8. With $\gamma \ge 0,$ P’s top-ranked cell is TL for $\alpha >0$ (to the right of the Figure 1a $\beta$ axis), and BR for $\alpha <0$ (Table 8a). According to Table 8b, the social welfare’s top-ranked cell is TL iff $\gamma +[\alpha +\beta ]>\gamma -[\alpha +\beta ]$, or iff $\alpha +\beta >0$; this is the region above the Figure 1a slanted line. The same computation shows that the social welfare’s top-ranked cell is BR for the region below the slanted line. This completes the proof. □

Everything becomes slightly more complicated with $\gamma <0$. The reason is that this $\gamma <0$ anti-coordination factor permits BL and TR to become Nash cells. This characteristic is manifested in Figure 1b, where the Figure 1a $\alpha =0$ and $\alpha +\beta =0$ lines are separated into strips.

The content of the next corollary is captured by Figure 1b, where the potential and social welfare’s top-ranked cells agree in the three shaded regions. To interpret Figure 1b, P’s top-ranked cell is BR for all games to the left of the vertical strip ($\alpha <-\left|\gamma \right|$), cells BL and TR (or $BL\sim TR$) in the vertical strip ($-\left|\gamma \right|<\alpha <\left|\gamma \right|$), and cell TL to the right of the vertical strip ($\alpha >\left|\gamma \right|$). Similarly, the social welfare’s top-ranked cell is BR below the slanted strip, $BL\sim TR$ in the slanted strip, and TL above the slanted strip. As $\gamma \to 0$, the width of the strips shrink and Figure 1b merges into Figure 1a.

Corollary 3.

For symmetric $2\times 2$ potential games, the following hold for $\gamma <0$:

 1.

The top-ranked P cell is TL iff $\alpha >\left|\gamma \right|$ (the Figure 1b region to the right of the $\alpha =\left|\gamma \right|$ vertical line). In this region, the social welfare ranking is TL (to agree with P) for $(\alpha +\beta )>2\left|\gamma \right|$ (shaded region on the right of Figure 1b), but conflicts with P’s choice with the social welfare top-ranking of $BL\sim TR$ if $2\left|\gamma \right|>(\alpha +\beta )>-2\left|\gamma \right|$ (the portion of the strip below the shaded region on the right of Figure 1b), and with the top-ranked BR for $-2\left|\gamma \right|>(\alpha +\beta )$ (the unshaded region below the strip on the right of Figure 1b).

 2.

For $-\left|\gamma \right|<\alpha <\left|\gamma \right|$ (the vertical strip of Figure 1b), both BL and TR are P’s top-ranked cells with the ranking $BL\sim TR$. In this strip, the social welfare function has the same $BL\sim TR$ ranking only if $-2\left|\gamma \right|<(\alpha +\beta )<2\left|\gamma \right|$ (the shaded trapezoid). Outside of this region in the strip, the social welfare top-ranked cell differs from P’s $BL\sim TR$ choice by being TL for $(\alpha +\beta )>2\left|\gamma \right|$ (above the trapezoid) and BR for $(\alpha +\beta )<-2\left|\gamma \right|$ (below the trapezoid).

 3.

The top-ranked cell for P is BR for $\alpha <-\left|\gamma \right|$ (to the left of the vertical strip). The social welfare function’s top-ranked cell also is BR for $\alpha +\beta <-2\left|\gamma \right|$ (shaded Figure 1b region on the left). However, in this region, the social welfare function has BL and TR top ranked, or $BL\sim TR$, for $-2\left|\gamma \right|<(\alpha +\beta )<2\left|\gamma \right|$ (the portion of the slanted strip above the shaded region) and TL top ranked for $(\alpha +\beta )>2\left|\gamma \right|$ (above the slanted strip).

Proof. 

The proof follows directly from Table 8. With $\gamma <0$, it follows from Table 8a that P’s top-ranked cell is TL if it is preferred to either BL or TR, which is if $2\alpha +\gamma >-\gamma$ or if $\alpha >\left|\gamma \right|$. This is the Figure 1b region to the right of the $\alpha =\left|\gamma \right|$ vertical line. Similarly, P’s top-ranked cell is BR if $\gamma -2\alpha >-\gamma$, or if $-\alpha >\left|\gamma \right|$; this is the region to the left of the $\alpha =-\left|\gamma \right|$ vertical line. The same computation shows that in the vertical strip $-\left|\gamma \right|<\alpha <\left|\gamma \right|$, P’s top-ranked cells are the two Nash cells BL and TR, where P’s ranking is $BL\sim TR$.

Using the same approach with Table 8b, it follows that the social welfare’s top-ranked cell is TL if it has a higher score than BL or TR, which is if $-\left|\gamma \right|+(\alpha +\beta )>\left|\gamma \right|$, or if $\alpha +\beta >2\left|\gamma \right|.$ This is the region above the $\alpha +\beta =\left|\gamma \right|$ slanted line. Similarly, the social welfare top ranked cells are $BL\sim TR$ for $-2\left|\gamma \right|<(\alpha +\beta )<2\left|\gamma \right|$, which is the slanted strip (which expanded the Figure 1a slanting line), and BR for $(\alpha +\beta )<-2\left|\gamma \right|$, which is the region below the slanting strip. This completes the proof. □

4.1. Changing ${\beta }_1,{\beta }_2$

The cause of conflict between potential and social welfare rankings now is clear; the first ignores $\beta$ values while the second depends upon them. However, a feature of the previous section is that if TL or BR ended up being the social welfare top-ranked cell, it also was the payoff dominant cell. This property is a direct consequence of the symmetric game structure where the behavioral terms (Table 6b) always favored one of these two cells.

To recognize the many other possibilities, change the $\beta$ structure from $\beta ={\beta }_1={\beta }_2$ to $\beta ={\beta }_1=-{\beta }_2$. This affects Table 6b by changing the sign of player 2’s entries, so the game’s externality features now emphasize either BL or TR. The social welfare function becomes

$$w=2\gamma t_1{t}_2+\alpha (t_1+t_2)+\beta (t_2-t_1)$$

(13)

A reason for considering this case is that any $({\beta }_1,{\beta }_2)$ can be uniquely expressed as

$$({\beta }_1,{\beta }_2)=(b_1,b_1)+(b_2,-b_2)\mathrm{where}{b}_1=\frac{{\beta }_1+{\beta }_2}{2},b_2=\frac{{\beta }_1-{\beta }_2}{2}.$$

(14)

Thus, combining Figure 1 with the impact of ($\beta ,-\beta )$ captures the general complexity.

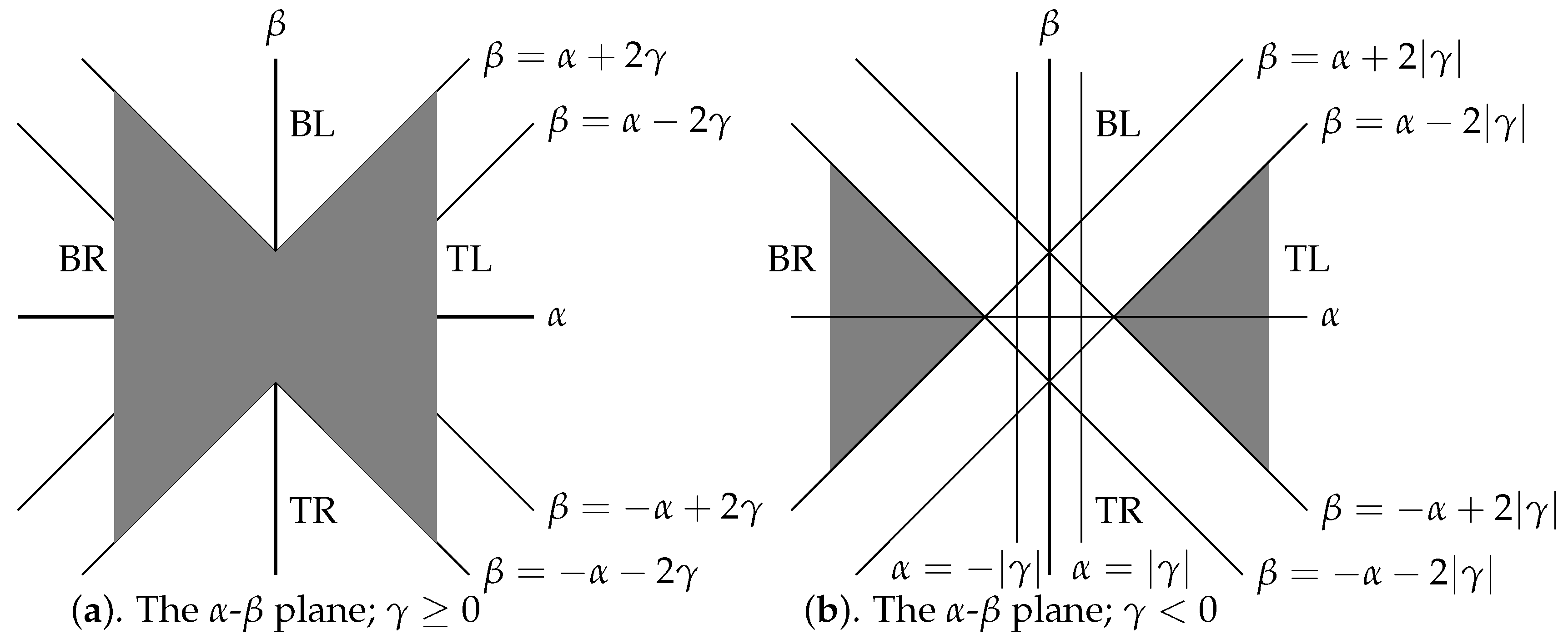
Because the decomposition isolates appropriate variables for each measure, Table 8 is the main tool to derive the Figure 3 results. In this new setting, Table 8 is replaced with

Table 10. A quasi-symmetric potential game’s P and $\frac{w}{2}$ values, with $(\beta ,-\beta )$.

	$+1$	$-1$		$+1$	$-1$
$+1$	$2\alpha +\gamma$	$-\gamma$	$+1$	$\gamma +\alpha$	$-\gamma -\beta$
$-1$	$-\gamma$	$\gamma -2\alpha$	$-1$	$-\gamma +\beta$	$\gamma -\alpha$
a. P values			b. $\frac{w}{2}$ values

, where part a restates the potential function values for each cell and b gives half of the social welfare function’s values.

As with Figure 1a, if $\gamma \ge 0,$ then P’s top-ranked cell is TL for $\alpha >0$ and BR for $\alpha <0.$ The same holds for Figure 3a. According to Table 10b, the social welfare function ranks TL over BL if $\gamma +\alpha >-\gamma +\beta$, or if $\beta <2\gamma +\alpha$. In Fig. Figure 3a, this is the region below the $\beta =\alpha +2\gamma$ slanted line. Similarly, the social welfare function ranks TL over TR if $\alpha +2\gamma >-\beta$, or $\beta >-\alpha -2\gamma$, which is the Figure 3a region above the $\beta =-\alpha -2\gamma$ line. P’s top ranked cell is BR if $\alpha <0$, which is the Figure 3a region to the left of the $\beta$ axis. A similar analysis shows that the social welfare function ranks BR above TR if $\beta >\alpha -2\gamma$, or the region above the Figure 3a $\beta =\alpha -2\gamma$ line. Finally, this function ranks BR above BL if $\beta <-\alpha +2\gamma$, which is the region below the $\beta =-\alpha +2\gamma$ line.

Consequently, agreement between the two measure’s top-ranked cell is in Figure 3a shaded regions, where BR is the common choice to the left of the $\beta$ axis and TL is for the region to the left. Conflict occurs in the unshaded region where BL is the welfare’s top-cell on the top and TR is for the region below. Again, these outcomes capture the $\beta$ structure where, now, positive $\beta$ values emphasize the BL cell and negative values enhance the TR entries. Contrary to Figure 1a, the upper unshaded region now is where BL, rather than TL, is the welfare function’s top-ranked cell.

Consistent with Figure 1b, the situation becomes more complicated with the anti-coordination $\gamma <0.$ Again, P’s top ranked cell is BR to the left of the vertical strip, $BL\sim TR$ in the strip, and TR to the right of the strip. Similar algebraic comparisons show that the social welfare’s top-ranked cell is BL in the upper unshaded region of Figure 3b, including the portion of the $\beta$ axis. Similarly, TR is the welfare function’s top-ranked cell in the lower unshaded region. Consequently, the two large shaded regions are where agreement occurs (going from left to right, BR, TL).

With the Table 6a Nash structure, outcomes for all possible $({\beta }_1,{\beta }_2)$ values can be computed from Figure 1 and Figure 3. To illustrate with $\gamma =-0.5,\alpha =-2,{\beta }_1=11,{\beta }_2=1$, it follows from $\left|\gamma \right|<-\alpha$ that BR is P’s top-ranked cell. The information for the welfare function comes from Equation (14) where $b_1=6,b_2=5.$ To find half of the welfare functions value, substitute $\alpha =2\gamma =-0.5,\beta =6$ in Table 8b, substitute $\alpha =2\gamma =-0.5,\beta =6$ in Equation (13b), and add the values. It already follows from plotting these values in Figure 1b and Figure 3b that the outcome is either TL or BL.

4.2. Changing ${\alpha }_1,{\alpha }_2$

The general setting for a potential game involves variables ${\alpha }_1,{\alpha }_2,{\beta }_1,{\beta }_2,\gamma$. This suggests mimicking what was done with $\beta$ by carrying out an analysis using $\alpha ={\alpha }_1=-{\alpha }_2$; this is simple, but not necessary. The reason is that most needed information about P’s top-ranked cell comes from Equation (5). As this expression shows, with appropriate choices of ${\alpha }_1$, ${\alpha }_2$, and $\gamma$, any cell can be selected to be P’s risk-dominant, top-ranked choice, any admissible pair of cells can be Nash cells, where a designated one is risk dominant, and any cell can be selected to be the sole Nash cell. Finding how the behavioral terms (the ${\beta }_1,{\beta }_2$ values) can change which cell is the welfare function’s top-ranked cell has been reduced to elementary algebra.

All that is needed to obtain answers is to have a generalized form of Table 8 and Table 10, which is given in

Table 11. A potential game’s P and w values.

	$+1$	$-1$
$+1$	${\alpha }_1+{\alpha }_2+\gamma$	${\alpha }_1-{\alpha }_2-\gamma$
$-1$	$-{\alpha }_1+{\alpha }_2-\gamma$	$-{\alpha }_1-{\alpha }_2+\gamma$
a. P values
	$+1$	$-1$
$+1$	${\alpha }_1+{\alpha }_2+{\beta }_1+{\beta }_2+2\gamma$	${\alpha }_1-{\alpha }_2-{\beta }_1+{\beta }_2-2\gamma$
$-1$	$-{\alpha }_1+{\alpha }_2+{\beta }_1-{\beta }_2-2\gamma$	$-{\alpha }_1-{\alpha }_2-{\beta }_1-{\beta }_2+2\gamma$
b. w values

. The Table 11a values come from the general form of the potential function in Equation (2). The Table 11b values for the welfare function come from a direct computation of its equation

$$w(t_1,t_2)=({\alpha }_1+{\beta }_2)t_1+({\alpha }_2+{\beta }_1)t_2+2\gamma t_1{t}_2.$$

(15)

In the manner employed above, Theorem 6 is a sample of results. Here, use Equation (5) to determine the potential function structure, and Theorem 6 to compare social welfare (and $\beta$) values.

Theorem 6.

The social welfare function (Equation (15)) is maximized at TL if and only if ${\alpha }_1+{\beta }_2+{\alpha }_2+{\beta }_1>0$ and ${\beta }_i+{\alpha }_{\neg i}>-2\gamma$, where $i=1,2$ and $\neg i$ denotes the agent who is not i. The welfare function is maximized at TR if and only if ${\alpha }_2+{\beta }_1<-2\gamma ,{\alpha }_1+{\beta }_2>2\gamma$, and ${\alpha }_1+{\beta }_2>{\alpha }_2+{\beta }_1$. The welfare function is maximized at BL if and only if ${\alpha }_1+{\beta }_2<-2\gamma ,{\alpha }_2+{\beta }_1>2\gamma$, and ${\alpha }_2+{\beta }_1>{\alpha }_1+{\beta }_2$. Finally, the welfare function is maximized at BR if and only if ${\alpha }_1+{\beta }_2+{\alpha }_2+{\beta }_1<0$ and ${\beta }_i+{\alpha }_{\neg i}<2\gamma$, for $i=1,2$.

5. Discussion

Questions regarding the various measures for game theory have proved to be difficult to analyze. There is an excellent reason for this complexity; answers must depend upon the particular payoffs of a game, but it was not clear what portions of each payoff contribute to which aspects of a game. As such, a surprising and welcomed property of the coordinate system is how it identifies how all of a game’s entries interact; the coordinates precisely dissect and extract from each payoff entry its contribution to the different attributes of a game.

Support for these comments come from equations such as Equation (9) for the potential function and Equation (10) for the welfare function. The different signs of $t_1{t}_2$ and $t_i$ coefficients, for instance, nicely capture the complexity of a standard approach; it indicates there exists a twisting of certain portions of the payoff entries that are needed to carry out an analysis. The decomposition’s separation of which parts of a payoff entry affect Nash structures and which affect payoff and externality factors explain why different measures of a game can have different conclusions. What illustrates the power of doing so is how the discovery and proofs of many subtle results now reduce to elementary algebraic computations.

Our analysis described how and why differences can arise among potential function, payoff dominance, and social welfare conclusions about games. Everything extends more generally. As the decomposition demonstrates, expect methods, learning approaches, and measures that emphasize “best response”, comparisons of individual payoff differences, and obtaining Nash equilibria to ignore behavioral terms. Should the objective be to identify properties of Nash structures, doing so simplifies the analysis by eliminating the redundant (for a Nash analysis) ${\beta }_j$ variables. However, by not including $\beta$ terms, it must be expected that answers from these approaches about games will differ from those measures that capture the value of payoffs, such as the social welfare function and payoff dominance. They must; the two different classes of measures depend upon different information about the games.