Example of a Finite Game with No Berge Equilibria at All
Abstract
:1. Introduction
2. Basic Notions and Definitions
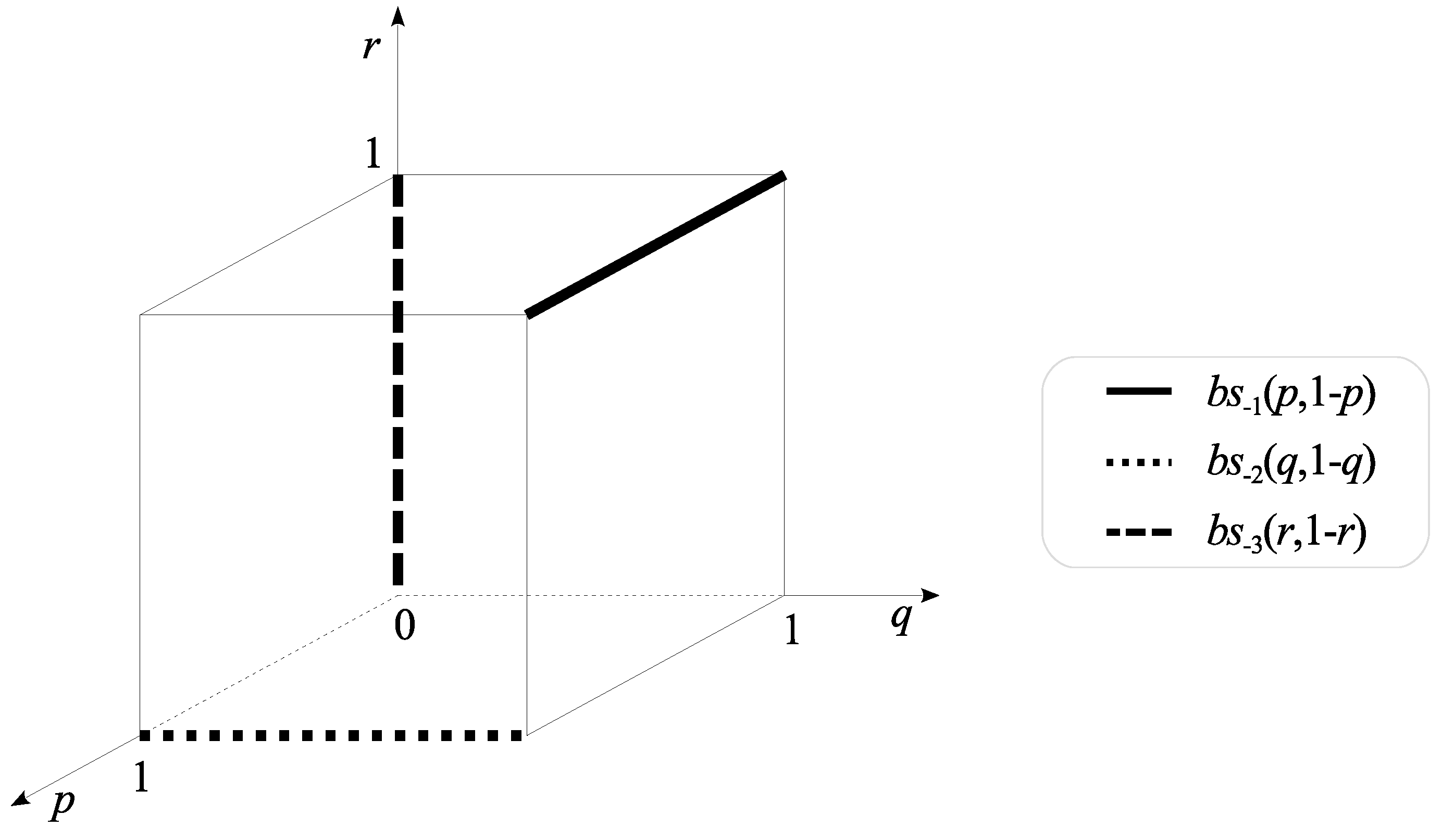
3. Example of a Three-Player Game with No Berge Equilibria at All
4. Summary
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Berge, C. Théorie Générale des Jeux à n Personnes; Gauthier-Villars: Paris, France, 1957. [Google Scholar]
- Colman, A.M. Mutual support in games: Some properties of Berge equilibria. J. Math. Psychol. 2011, 55, 166. [Google Scholar] [CrossRef]
- Larbani, M.; Zhukovskii, V.I. Berge equilibrium in normal form static games: A literature review. Izv. Inst. Mat. Inform. Udmurt. Gos. Univ. 2017, 49, 80. [Google Scholar] [CrossRef]
- Nash, J. Equilibrium points in n-person games. Proc. Natl. Acad. Sci. USA 1950, 36, 48. [Google Scholar] [CrossRef]
- Peters, H. Game Theory. A Multi-Leveled Approach; Springer: Berlin, Germany, 2008. [Google Scholar]
- Musy, O.; Pottier, A.; Tazdaït, T. A new theorem to find Berge equilibria. Int. Game Theory Rev. 2012, 14, 1250005. [Google Scholar] [CrossRef]
- Bytner, P. Berge Equilibria in Pure and Mixed Strategies. Master’s Thesis, University of Gdańsk, Gdańsk, Poland, 2016. (In Polish). [Google Scholar]
| 1 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pykacz, J.; Bytner, P.; Frąckiewicz, P. Example of a Finite Game with No Berge Equilibria at All. Games 2019, 10, 7. https://doi.org/10.3390/g10010007
Pykacz J, Bytner P, Frąckiewicz P. Example of a Finite Game with No Berge Equilibria at All. Games. 2019; 10(1):7. https://doi.org/10.3390/g10010007
Chicago/Turabian StylePykacz, Jarosław, Paweł Bytner, and Piotr Frąckiewicz. 2019. "Example of a Finite Game with No Berge Equilibria at All" Games 10, no. 1: 7. https://doi.org/10.3390/g10010007
APA StylePykacz, J., Bytner, P., & Frąckiewicz, P. (2019). Example of a Finite Game with No Berge Equilibria at All. Games, 10(1), 7. https://doi.org/10.3390/g10010007




