Design and Algorithm Verification of a Gyroscope-Based Inertial Navigation System for Small-Diameter Spaces in Multilateral Horizontal Drilling Applications
Abstract
:1. Introduction

- The attitude error of the device should be less than 0.1° and the position error should be less than 1 m for 1000 m-deep wells.
- The device installs in a steel tube of 24-mm maximal diameter and the size of the three-dimensional device should be less than 150 mm × 150 mm × 10 mm (length, width and height).
- The temperature range of the device should be between 40 and 100 °C.
- The device should be able to perform well in a shock and vibration environment ranging up to 15 g RMS (5–500 Hz).
2. Fundamental Theory of Miniature Gyroscope-Based While Drilling (MGWD) System
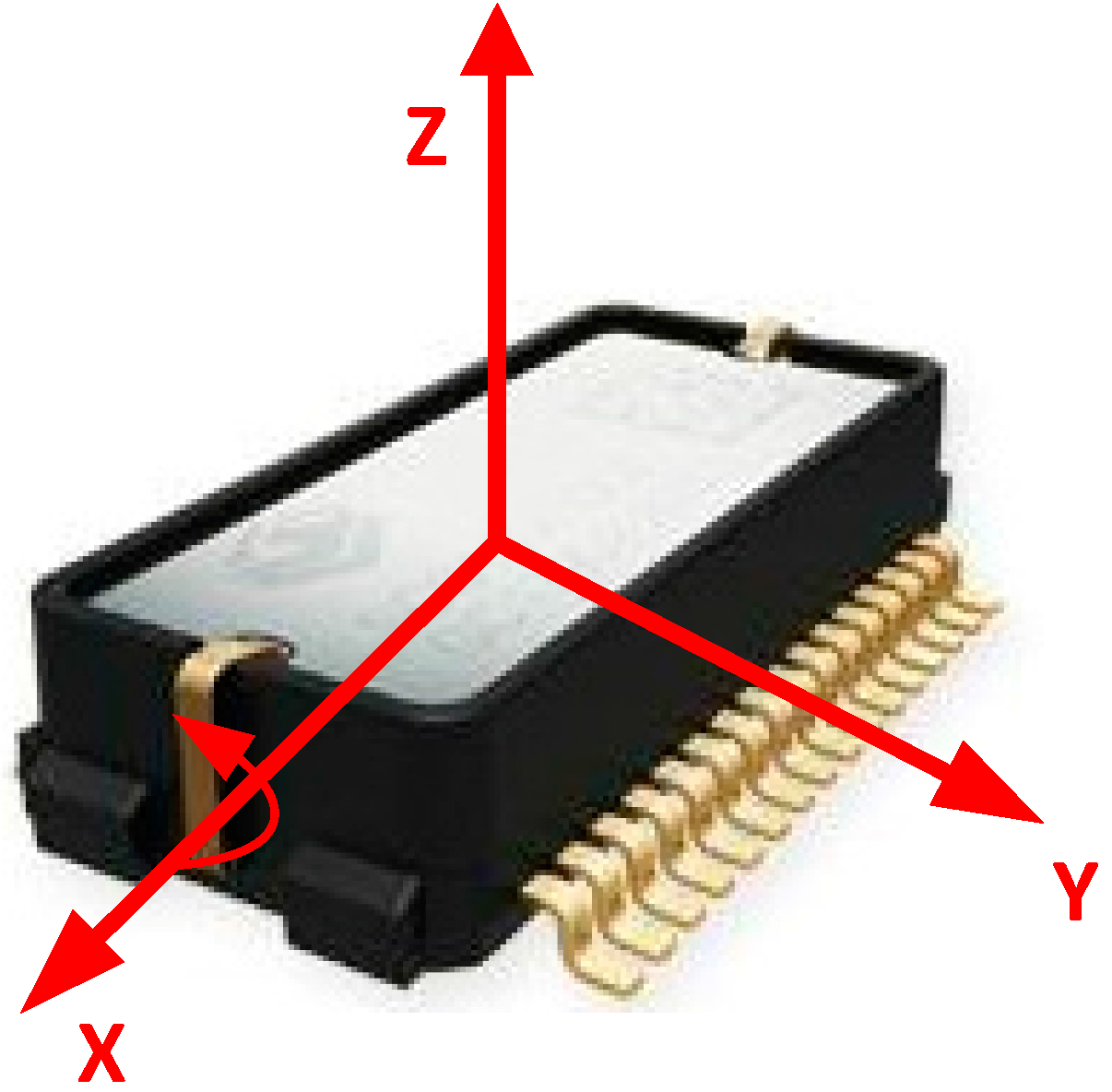
2.1. Definition of Coordinate Frames
| Parameters | Gyroscope | Parameters | Accelerometer |
|---|---|---|---|
| Offset Short Term Instability | <2.1°/h | Offset Error | ±70 mg |
| Angular Random Walk | 0.86°/ | Linearity Error | ±40 mg |
| Noise Density | 0.02(°/s/ | Noise | 5–7 mg |
| Temperature | −40–+125 °C | Temperature | −40–+125 °C |
2.2. MGWD System Navigation Solution
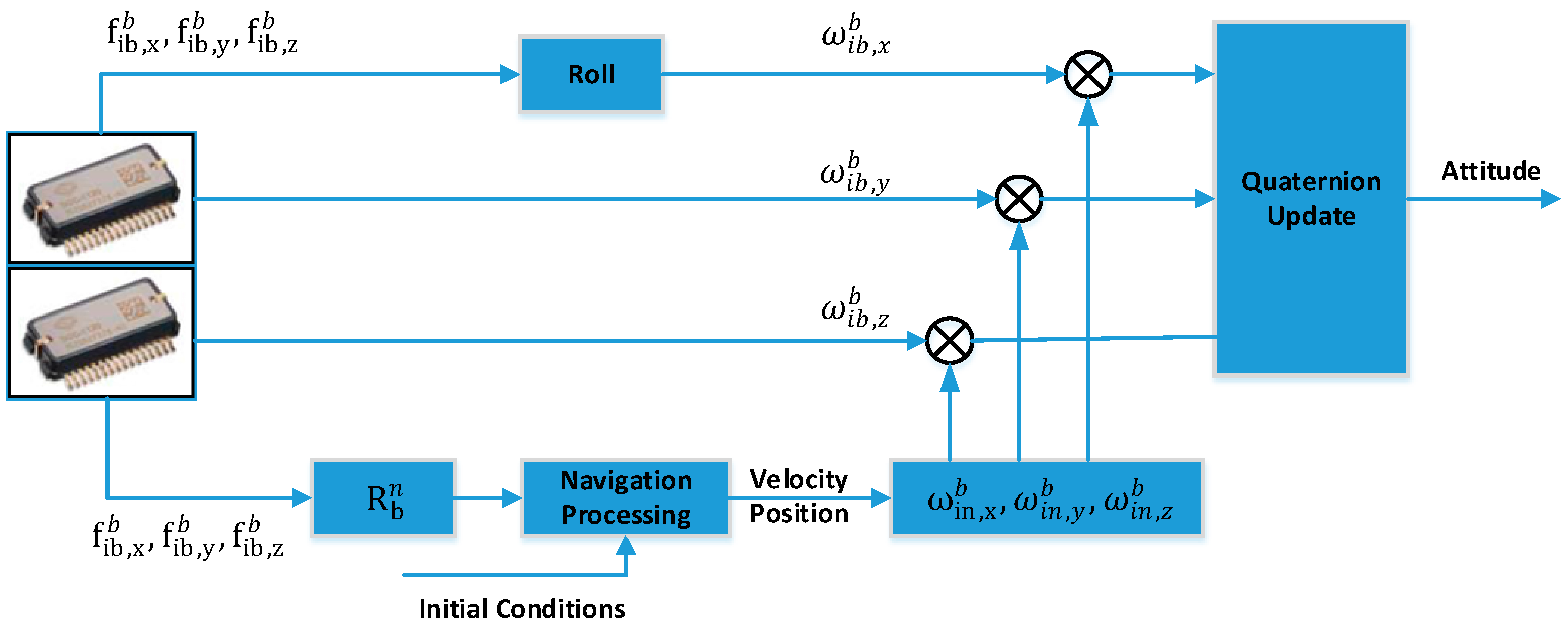
2.3. MGWD System Error Model
2.4. Kalman Filter Theory
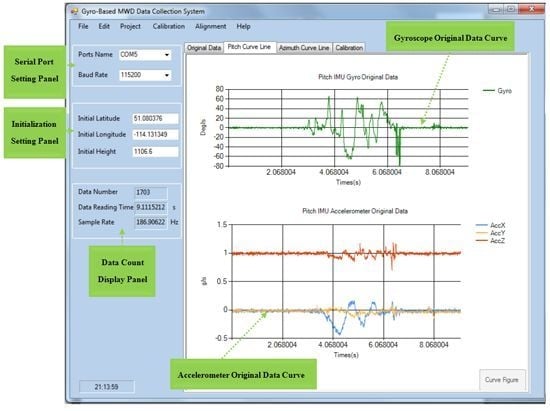
3. MGWD System Design and Testing
3.1. MGWD System Design

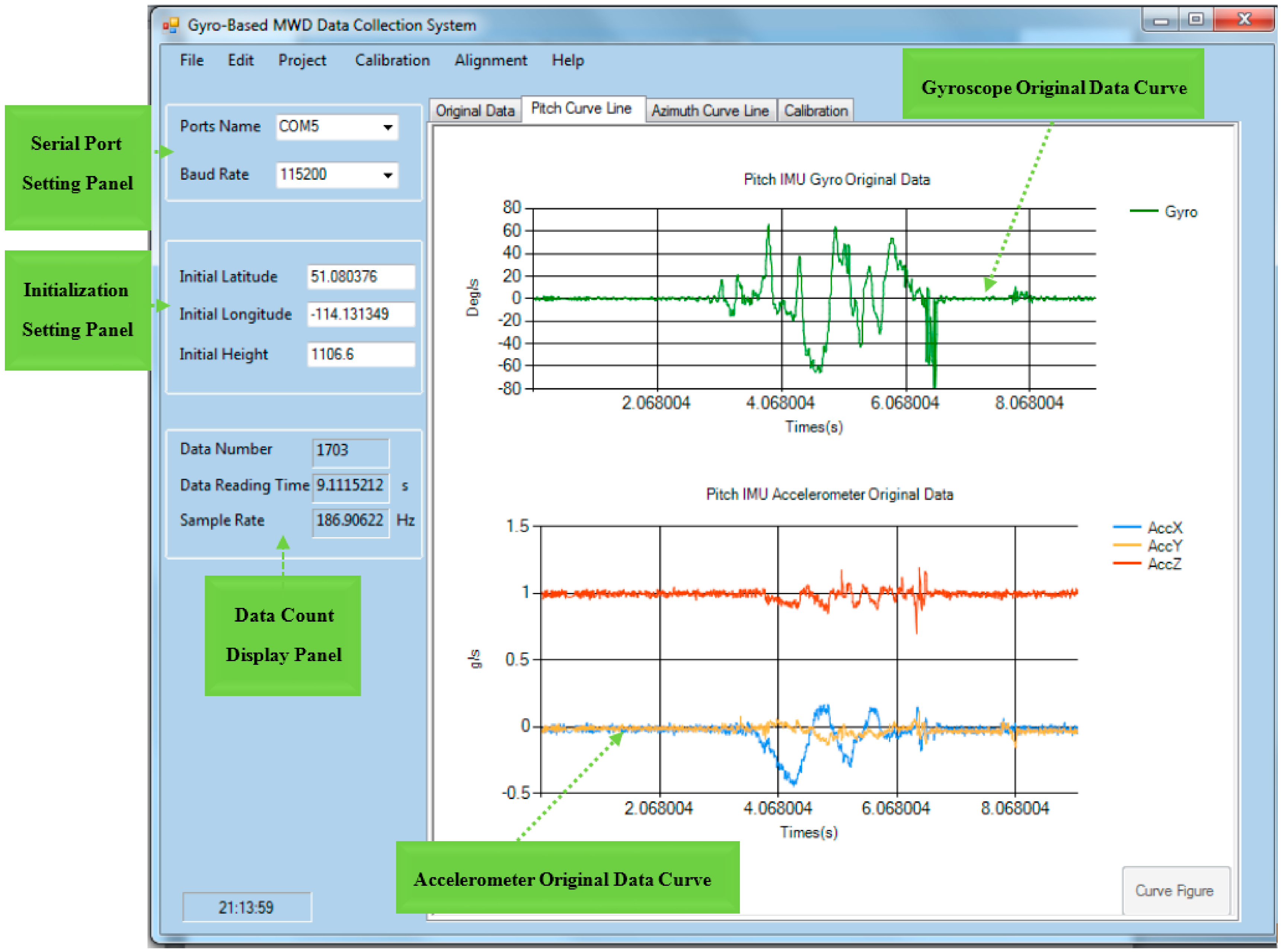
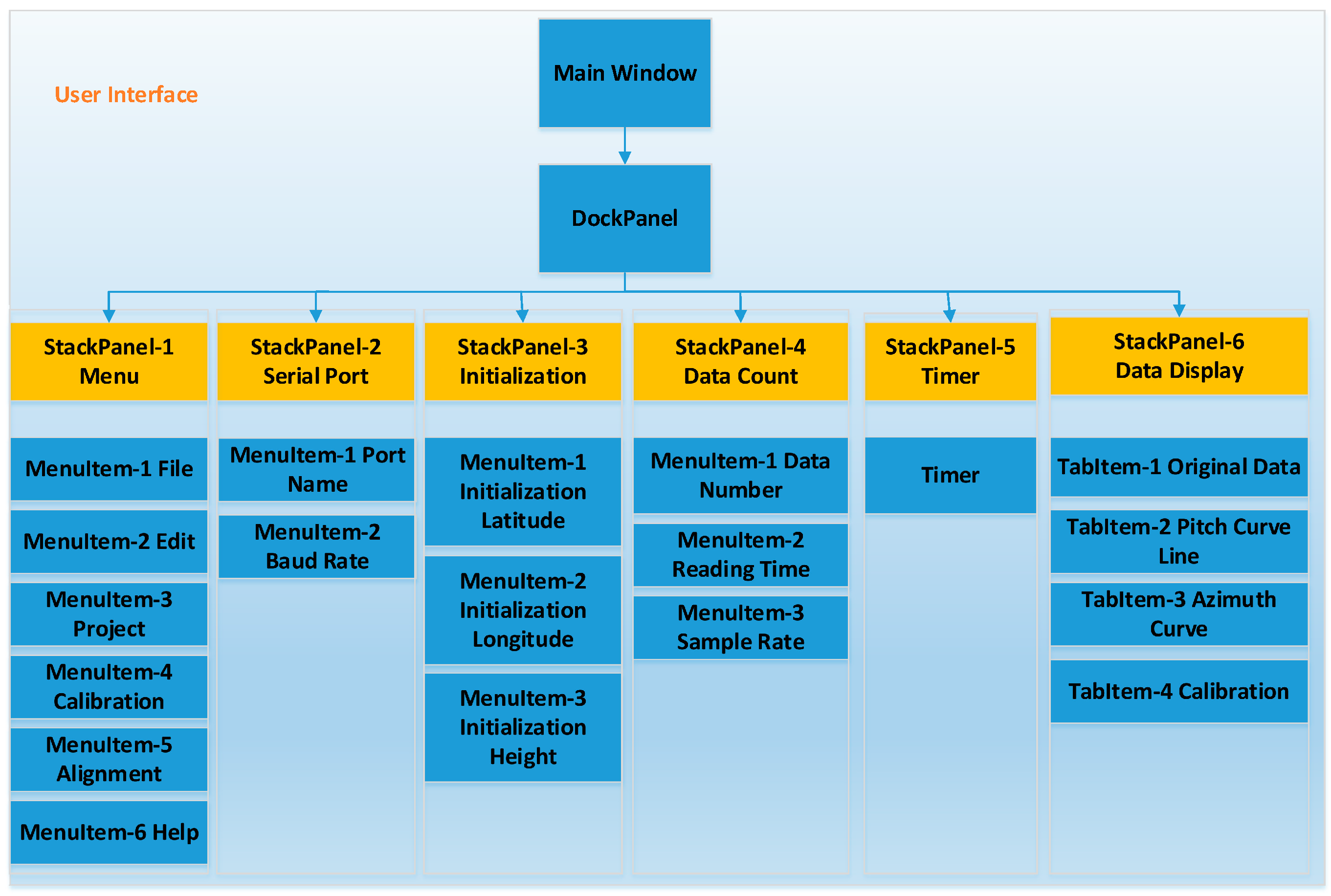
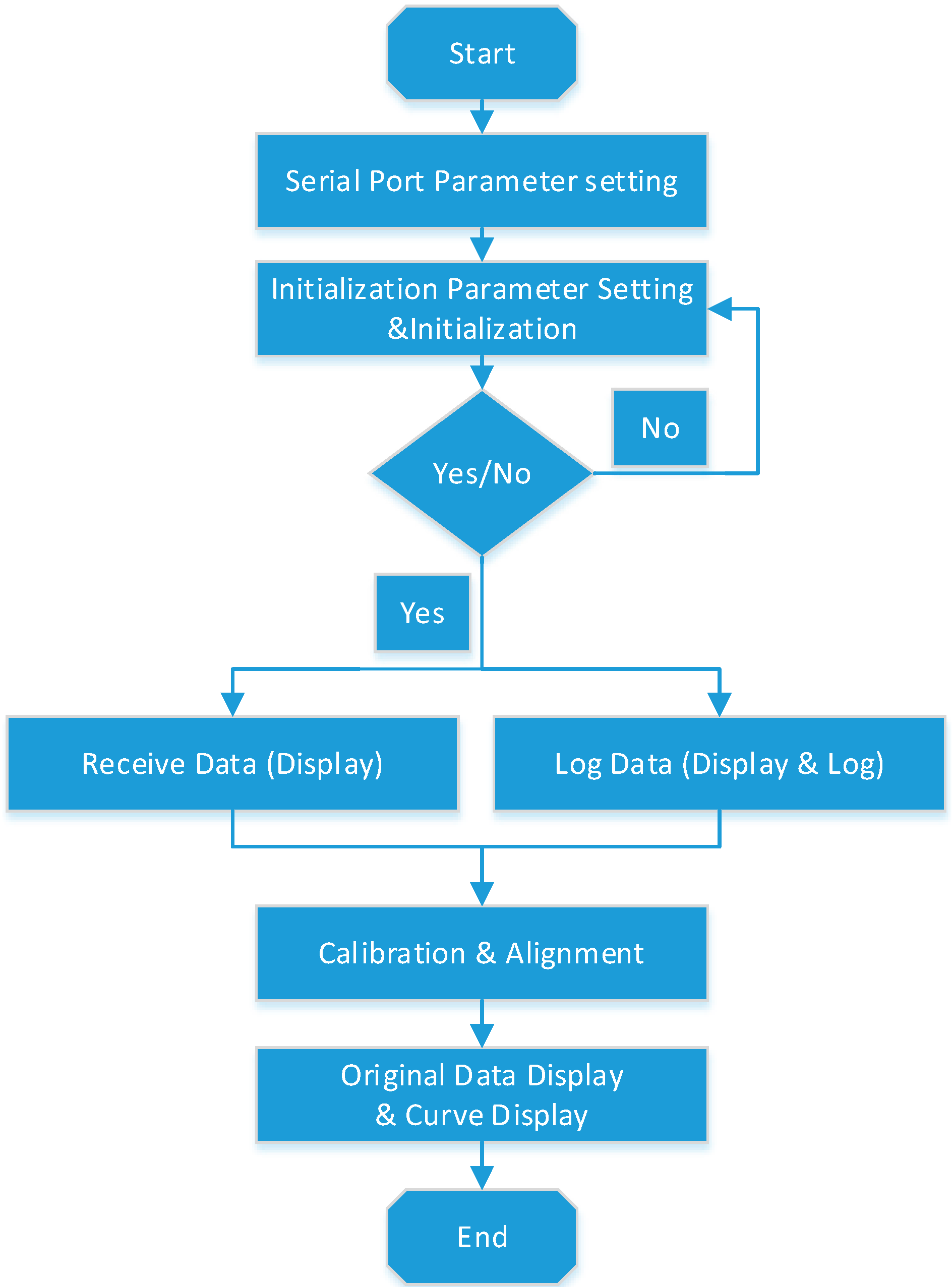
3.2 MGWD System Test
3.2.1. Allan Variance Analysis
| Sensor | Angular Random Walk | Bias Stability |
|---|---|---|
| Y-Axis Gyroscope | 0.004466835 (°/h/) | 0.00120876 (°/s) |
| Sensor | Velocity Random Walk | Bias Stability |
| Z-Axis Accelerometer | 0.052257815 (m/s/) | 0.000554625 m/s2 |

3.2.2. Stochastic Error Model
| Axis | Gyroscope Calibration Error (°/s) | Accelerometer Calibration Error (m/s2) |
|---|---|---|
| X-Axis | −0.021121495327103 | −0.007083371298406 |
| Y-Axis | −0.002242990654206 | −0.020187699316629 |
| Z-Axis | −0.016448598130841 | 0.002405466970394 |
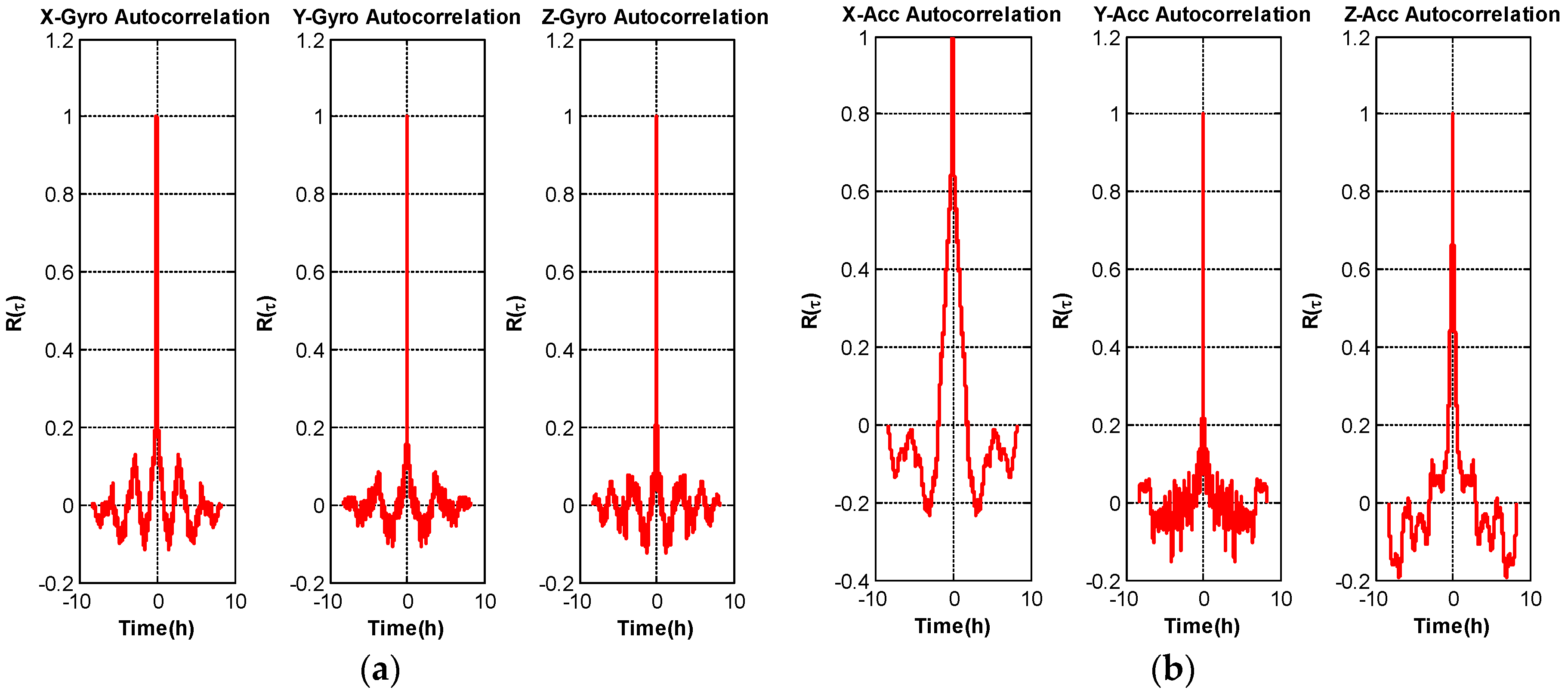
| Axis | a1 | a2 | a3 | a4 | βf,0 |
|---|---|---|---|---|---|
| X-Axis Accelerometer | 0.3308 | −0.0995 | 0.2036 | 0.3347 | 0.0034 |
| Y-Axis Accelerometer | 0.3307 | −0.1118 | 0.1956 | 0.3350 | 2.0947 × 10−4 |
| Z-Axis Accelerometer | 0.1249 | −0.0801 | 0.0382 | 0.0724 | 3.3090 × 10−6 |
| Axis | b1 | b2 | b3 | b4 | βw,0 |
|---|---|---|---|---|---|
| X-Axis Gyroscope | −0.0866 | −0.5159 | −0.0947 | −0.1270 | 2.2871 × 10−7 |
| Y-Axis Gyroscope | −0.8466 | −0.1105 | 0.2948 | −0.1642 | 7.1662 × 10−7 |
| Z-Axis Gyroscope | −0.2683 | −0.0026 | 0.0192 | −0.3496 | 6.9822 × 10−7 |
3.2.3. MGWD System Alignment

4. Results and Discussion
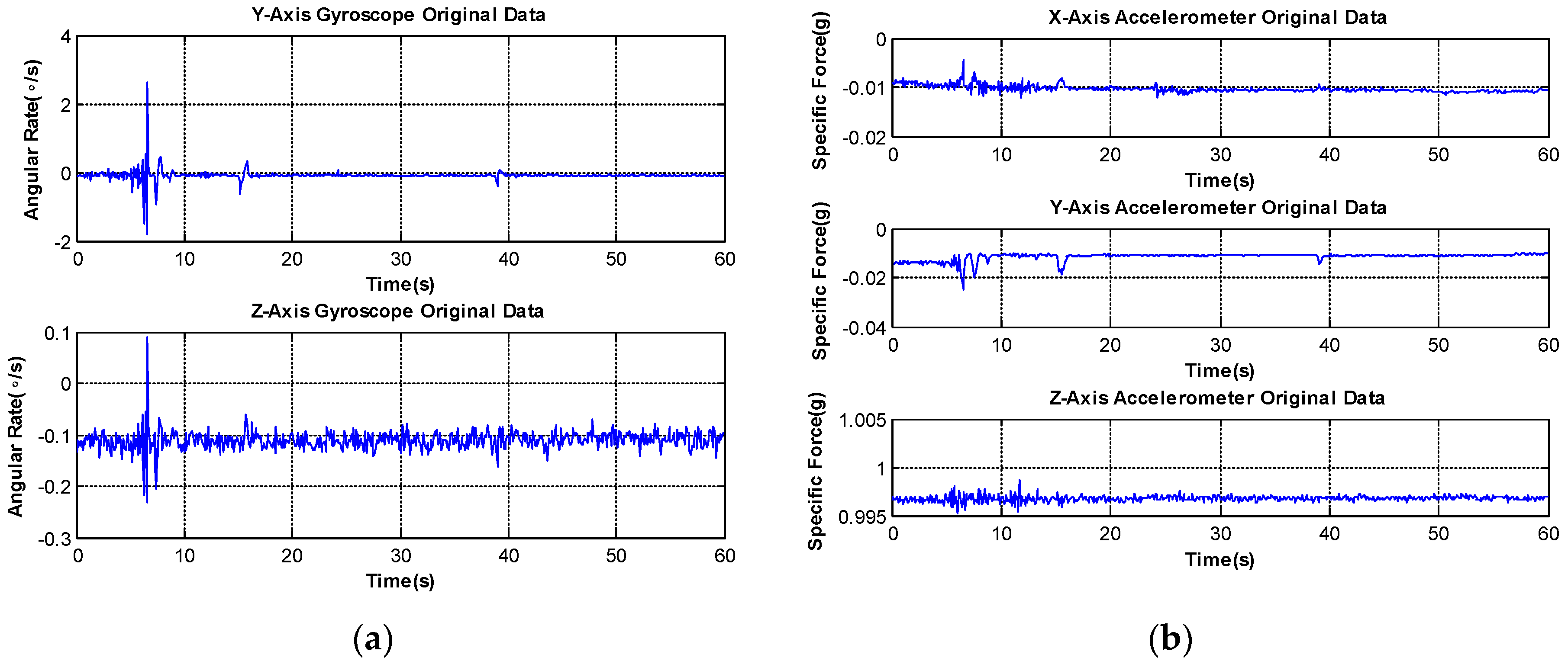
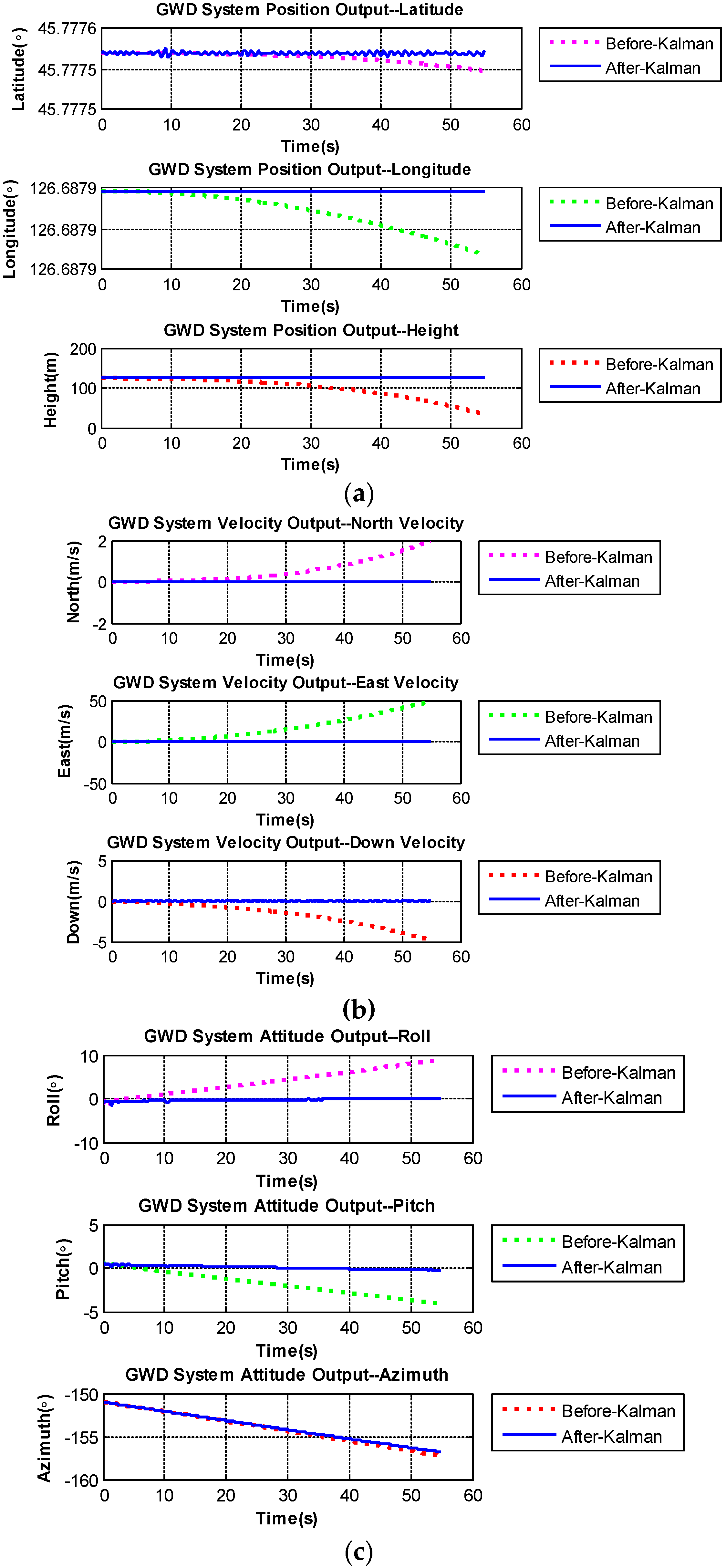
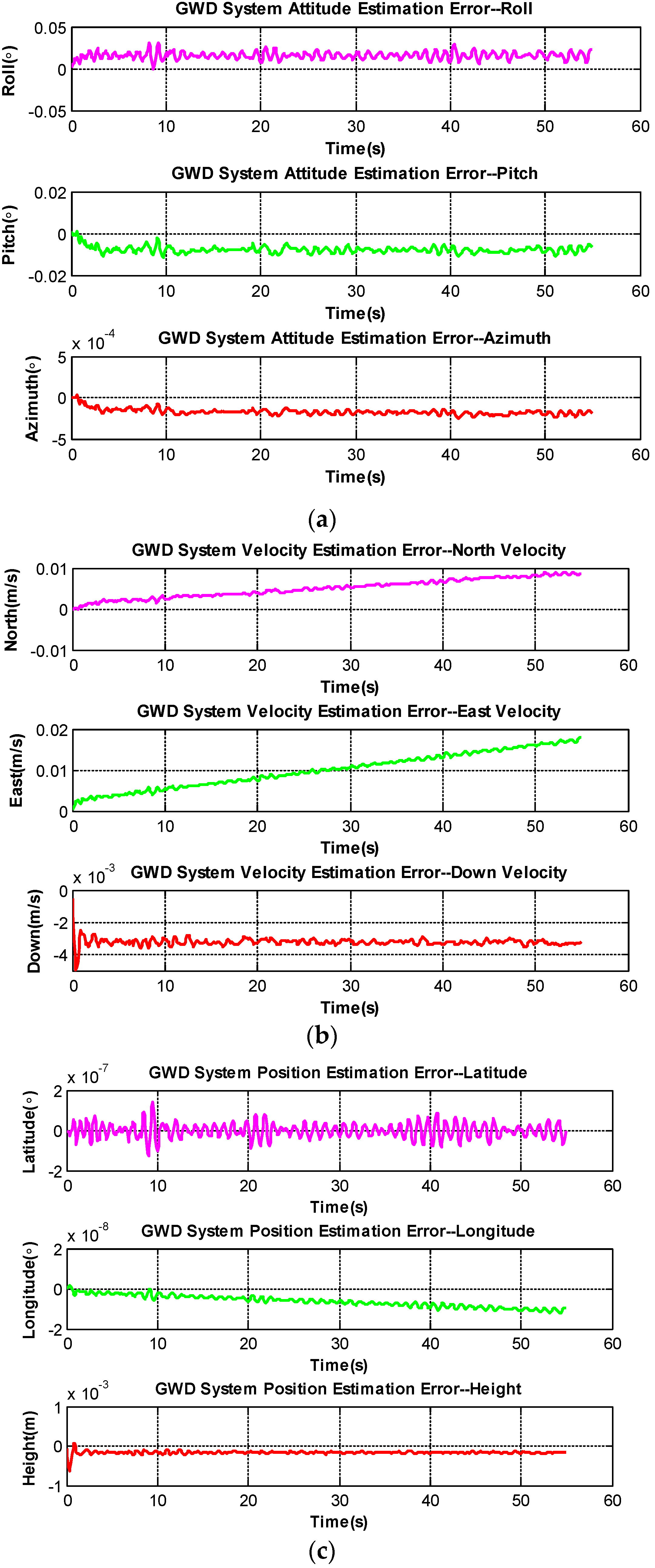

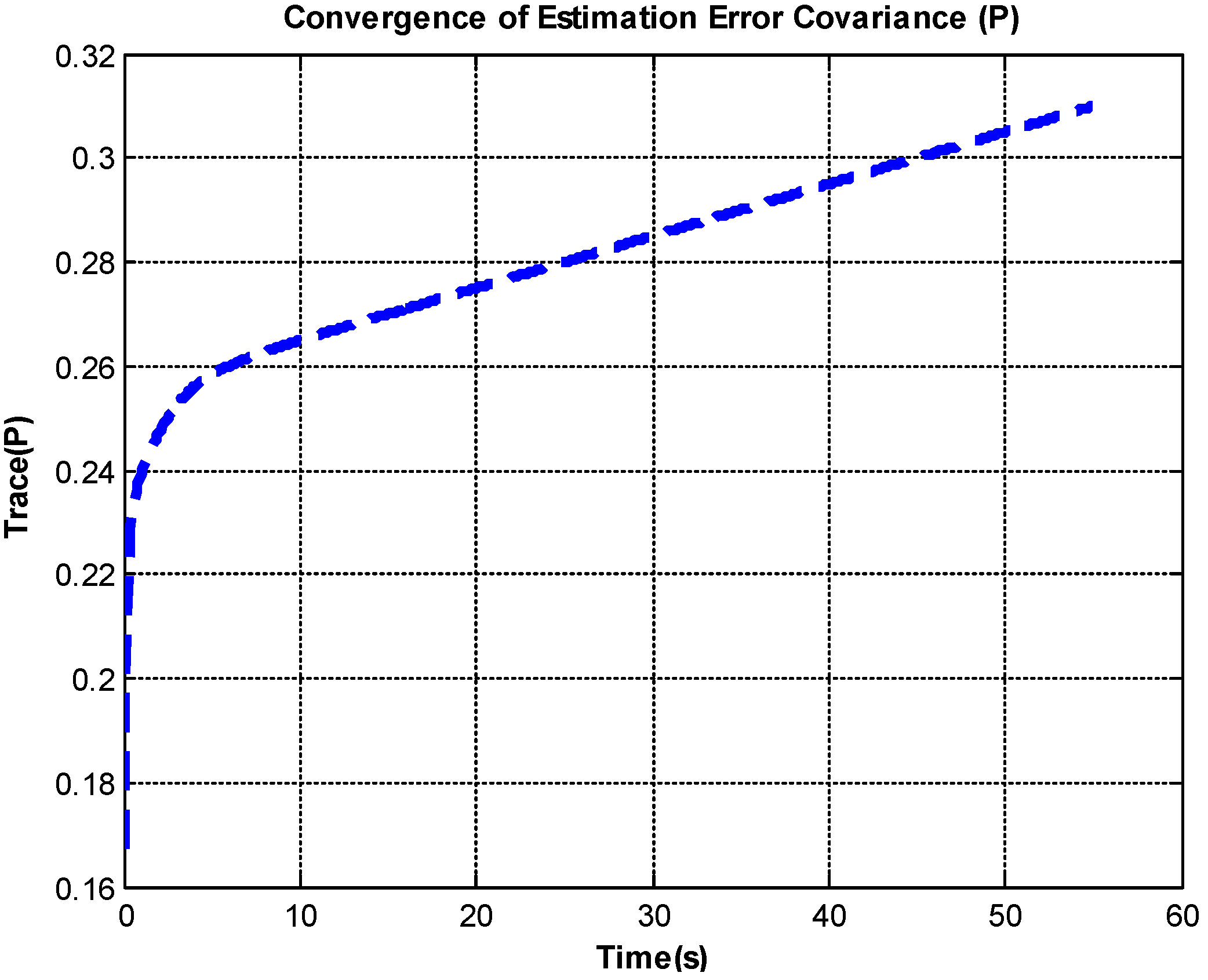
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
References
- Ledroz, A.G.; Pecht, E.; Cramer, D.; Mintchev, M.P. Fog-based navigation in downhole environment during horizontal drilling utilizing a complete inertial measurement unit: Directional measurement-while-drilling surveying. IEEE Trans. Instrum. Meas. 2005, 54, 1997–2006. [Google Scholar] [CrossRef]
- Hill, D.; Neme, E.; Ehlig-Economides, C.; Mollinedo, M. Reentry drilling gives new life to aging fields. Oilfield Rev. 1996, 8, 4–17. [Google Scholar]
- Pendleton, L.E. Horizontal drilling review. In Archie Conference on Reservoir Definition and Description; Society of Petroleum Engineers: Houston, TX, USA, 1991; p. 6. [Google Scholar]
- Longbottom, J.R.; Jano, J.C.; Cox, D.C.; Welch, W.R.; White, P.M.; Nivens, H.W.; Jacquier, R.C.; Holbrook, P.D.; Freeman, T.A.; Mills, D.H. Multilateral Well Drilling And Completion Method and Apparatus. EN Patent number EP0701045 A2, 15 October 1996. [Google Scholar]
- Von Flatern, R. Operators are Ready for More Sophisticated Multilateral Well Technology. Available online: http://www.researchgate.net/publication/255035866_Operators_are_ready_for_more_sophisticated_multilateral_well_technology (accessed on 2 December 2015).
- Wang, Z. Mems-Based Downhole Inertial Navigation Systems for Directional Drilling Applications. Master’s Thesis, University of Calgary, Calgary, AB, Canada, 2015. [Google Scholar]
- Ishak, I.B.; Macaulay, R.C.; Stephenson, P.M.; Al Mantheri, S.M. Review of horizontal drilling. In Middle East Oil Show; Society of Petroleum Engineers: Manama, Bahrain, 1995. [Google Scholar]
- Matheson, E.; Lee, R.G.M. The first use of gravity MWD in offshore drilling delivers reliable azimuth measurements in close proximity to sources of magnetic interference. In Proceedings of the IADC/SPE Drilling Conference, Dallas, TX, USA, 2–4 March 2004.
- Zijsling, D.H.; Wilson, R.A. Improved Magnetic Surveying Techniquesfield Experience. In Offshore Europe; Society of Petroleum Engineers: Aberdeen, UK, 1989. [Google Scholar]
- Noureldin, A.; Irvine-Halliday, D.; Mintchev, M.P. Accuracy limitations of fog-based continuous measurement-while-drilling surveying instruments for horizontal wells. IEEE Trans. Instrum. Meas. 2002, 51, 1177–1191. [Google Scholar] [CrossRef]
- Torgeir, T.I.E.; Fjogstad, A.; Saasen, A.; Amundsen, P.A.; Omland, T.H. Drilling fluid affects MWD magnetic azimuth and wellbore position. In Proceedings of the IADC/SPE Drilling Conference, Dallas, TX, USA, 2–4 March 2004.
- Cheatham, C.A.; Shih, S.; Churchwell, D.L.; Woody, J.M.; Rodney, P.F. Effects of magnetic interference on directional surveys in horizontal wells. In Proceeding of SPE/IADC Drilling Conference, New Orleans, LA, USA, 18–21 February 1992.
- Savage, P.G. Strapdown Analytics; Strapdown Associates, Inc.: Maple Plain, MN, USA, 2000; Volume 1. [Google Scholar]
- Britting, K.R. Inertial Navigation Systems; Wiley-Interscience: New York, NY, USA, 1971; p. 249. [Google Scholar]
- Edvardsen, I.; Nyrnes, E.; Johnsen, M.G.; Hansen, T.L.; Løvhaug, U.P.; Matzka, J. Improving the accuracy and reliability of MWD magnetic wellbore directional surveying in the Barents Sea. In Proceedings of the SPE Annual Technical Conference and Exhibition, Oreleans, LA, USA, 30 September–2 Ocotber 2013.
- Van Nispen, J.; Howe, N.A. Directional surveying using inertial techniques—Field experience in the northern north sea. In Proceedings of the SPE Offshore Europe, Aberdeen, UK, 15–18 September 1981.
- Noureldin, A.; Tabler, H.; Irvine-Halliday, D.; Mintchev, M. Testing the applicability of fiber optic gyroscopes for azimuth monitoring for measurement-while-drilling processes in the oil industry. In Proceedings of the Position Location and Navigation Symposium, Atlanta, GA, USA, 22–26 April 2000; pp. 291–298.
- Uttecht, G.W.; de Wardt, J.P. Application of small-diameter inertial grade gyroscopes significantly reduces Borehole position uncertainty. In Proceedings of the IADC/SPE Drilling Conference, New Orleans, LA, USA, 20–23 February 1983.
- Elgizawy, M.; Noureldin, A.M.; El-Sheimy, N. MEMS gyroscope-while-drilling environment qualification testing. In Proceedings of the IADC/SPE Drilling Conference and Exhibition, New Orleans, LA, USA, 2–4 February 2010.
- Richardson, D.A. A gyroscope guidance sensor for ultra-deepwater applications. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 2–5 May 2014.
- Noureldin, A. New Measurement-While-Drilling Surveying Technique Utilizing Sets of Fiber Optic Rotation Sensors; University of Calgary: Calgary, AB, Canada, 2002. [Google Scholar]
- Pecht, E.; Mintchev, M.P. Observability analysis for ins alignment in horizontal drilling. IEEE Trans. Instrum. Meas. 2007, 56, 1935–1945. [Google Scholar] [CrossRef]
- Shin, E.-H. Accuracy Improvement of Low Cost INS/GPS for Land Applications; University of Calgary: Calgary, AB, Canada, 2001. [Google Scholar]
- Mintchev, M.P.; Pecht, E.; Cloutier, J.; Dzhurkov, A. In Drilling Alignment. U.S. Patent 7823661 B2, 2 November 2010. [Google Scholar]
- Jurkov, A.S.; Cloutier, J.; Pecht, E.; Mintchev, M.P. Experimental feasibility of the in-drilling alignment method for inertial navigation in measurement-while-drilling. IEEE Trans. Instrum. Meas. 2011, 1088–1089. [Google Scholar] [CrossRef]
- Wang, Z.; Poscente, M.; Filip, D.; Dimanchev, M.; Mintchev, M. Rotary in-drilling alignment using an autonomous mems-based inertial measurement unit for measurement- while-drilling processes. IEEE Trans. Instrum. Mag. 2014, 16, 26–34. [Google Scholar] [CrossRef]
- Titterton, D.H.; Weston, J.L. Strapdown Inertial Navigation Technology, 2nd ed.; American Institute of Aeronautics and Astronautics: New York, NY, USA, 2004. [Google Scholar]
- Oy, M.E. Scc1300-d04 Combined Gyroscope and 3-Axis Accelerometer with Digital Spi Interfaces. Available online: http://www.murata.com/~/media/webrenewal/products/sensor/gyro/scr1100/scr1100-d04%20datasheet%20v2%201.ashx?la=en-us (accessed on 25 November 2015).
- Noureldin, A.; Tabler, H.; Smith, W.; Irvine-Halliday, D.; Mintchev, M.P. Continous Measurement-While-Drilling Surveying. U.S. Patent No. 6823602 B2, 5 September 2003. [Google Scholar]
- Groves, P.D. Principles of Gnss, Inertial, and Multisensor Integrated Navigation System; Artech House: Boston, MA, USA; London, UK, 2008; p. 505. [Google Scholar]
- El-Sheimy, N. Dynamic Error State Model. In Inertial Techniques and INS/DGPS Integration: Derivation of the INS; ENGO 623-Course Note; University of Calgary: Calgary, AB, Canada, 2006; pp. 3–5. [Google Scholar]
- Brian D.O. Anderson, J.B.M. Optimal Filter; Prentice Hall Inc.: EngleWood Cliffs, NJ, USA, 1979. [Google Scholar]
- Simon, D. Optimal State Estimation: Kalman, H Infinity, and Nonlinear Approaches; John Wiley and Sons, Inc: Hoboken, NJ, USA, 2006. [Google Scholar]
- Bar-Shalom, Y.; Rong, X.L.; Kirubarajan, T. Estimation with Applications to Tracking and Navigation; John Wiley and Sons, Inc.: Toroto, ON, Canada, 2001; p. 188. [Google Scholar]
- Wu, Y.; Zhang, H.; Wu, M.; Hu, X.; Hu, D. Observability of strapdown ins alignment: A global perspective. IEEE Trans. Aerosp. Electron. Syst. 2012, 48, 78–102. [Google Scholar]
- Moore, B. Principal component analysis in linear systems: Controllability, observability, and model reduction. IEEE Trans. Autom. Control 1981, 26, 17–32. [Google Scholar] [CrossRef]
- IEEE. IEEE Standard Specification Format Guide and Test Procedure for Single-Axis Interferometric Fiber Optic Gyros; Standard 952-1997; IEEE: New York, NY, USA, 1998. [Google Scholar]
- Nassar, S. Improving the Inertial Navigation System (INS) Error Model for Ins and Insdgps Applications; University of Calgary: Calgary, AB, Canada, 2003. [Google Scholar]
- Quinchia, A.G.; Falco, G.; Falletti, E.; Dovis, F.; Ferrer, C. A comparison between different error modeling of mems applied to GPS/INS integrated systems. Sensors 2013, 13, 9549–9588. [Google Scholar] [CrossRef] [PubMed]
- Gelb, A. Applied Optimal Estimation; The MIT Press: Cambridge, MA, USA, 1974; p. 382. [Google Scholar]
- Von Storch, H.; Zwiers, F.W. Statistical Analysis in Climate Research; Cambridge University Press: Cambridge, UK, 2002; p. 496. [Google Scholar]
- El-Sheimy, N. Inertial Techniques and INS/DGPS Integration: Initial Alignment of INS; ENGO 623-Course Note; University of Calgary: Calgary, AB, Canada, 2006; p. 43. [Google Scholar]
- Jiang, Y.F. Error analysis of analytic coarse alignment methods. IEEE Trans. Aerosp. Electron. Syst. 1998, 34, 334–337. [Google Scholar] [CrossRef]
- Noureldin, A.; Karamat, T.B.; Eberts, M.D.; El-Shafie, A. Performance enhancement of mems-based INS/GPS integration for low-cost navigation applications. IEEE Trans. Veh. Technol. 2009, 58, 1077–1096. [Google Scholar] [CrossRef]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, T.; Yuan, G.; Lan, H.; Mintchev, M. Design and Algorithm Verification of a Gyroscope-Based Inertial Navigation System for Small-Diameter Spaces in Multilateral Horizontal Drilling Applications. Micromachines 2015, 6, 1946-1970. https://doi.org/10.3390/mi6121467
Li T, Yuan G, Lan H, Mintchev M. Design and Algorithm Verification of a Gyroscope-Based Inertial Navigation System for Small-Diameter Spaces in Multilateral Horizontal Drilling Applications. Micromachines. 2015; 6(12):1946-1970. https://doi.org/10.3390/mi6121467
Chicago/Turabian StyleLi, Tao, Gannan Yuan, Haiyu Lan, and Martin Mintchev. 2015. "Design and Algorithm Verification of a Gyroscope-Based Inertial Navigation System for Small-Diameter Spaces in Multilateral Horizontal Drilling Applications" Micromachines 6, no. 12: 1946-1970. https://doi.org/10.3390/mi6121467
APA StyleLi, T., Yuan, G., Lan, H., & Mintchev, M. (2015). Design and Algorithm Verification of a Gyroscope-Based Inertial Navigation System for Small-Diameter Spaces in Multilateral Horizontal Drilling Applications. Micromachines, 6(12), 1946-1970. https://doi.org/10.3390/mi6121467