Design and Fabrication of Ultrathin Metallic Phase Shifters for Visible and Near-Infrared Wavelengths
Abstract
1. Introduction
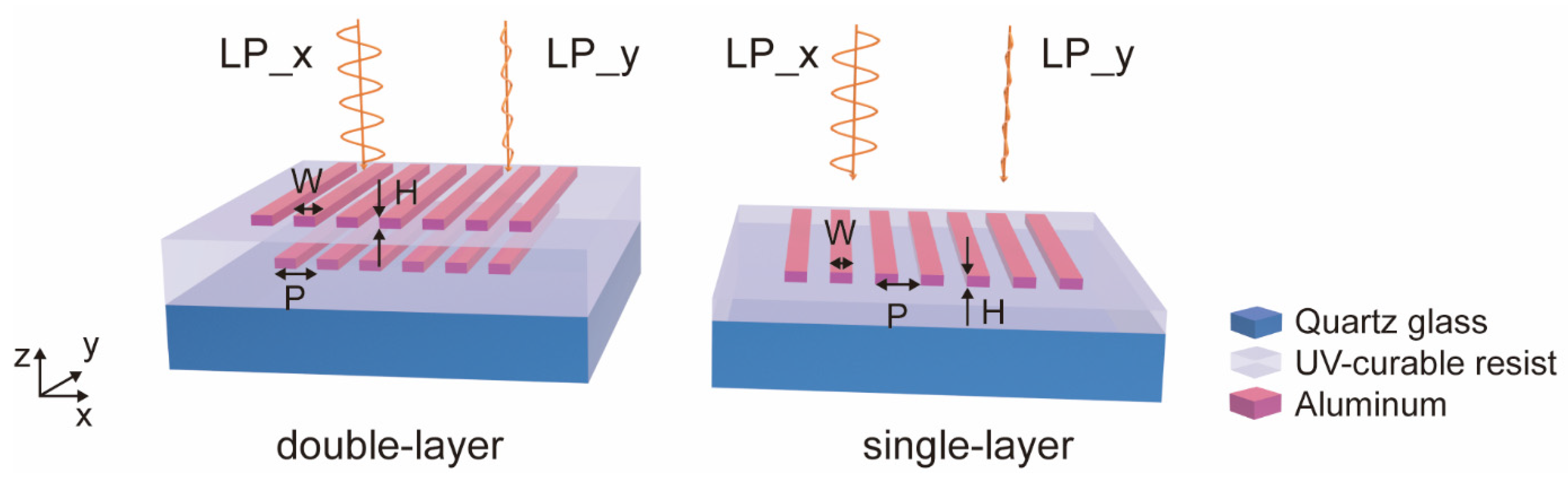
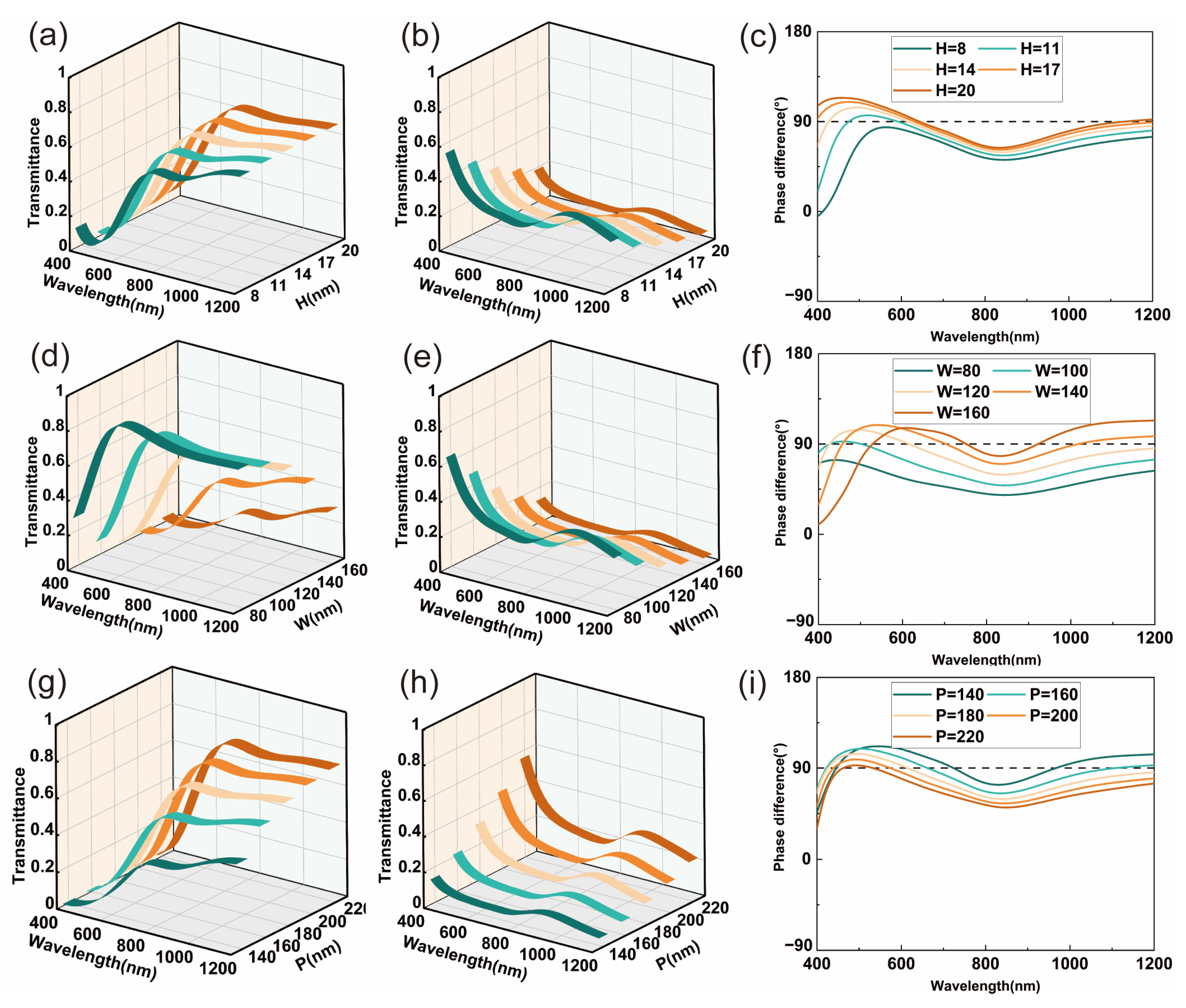
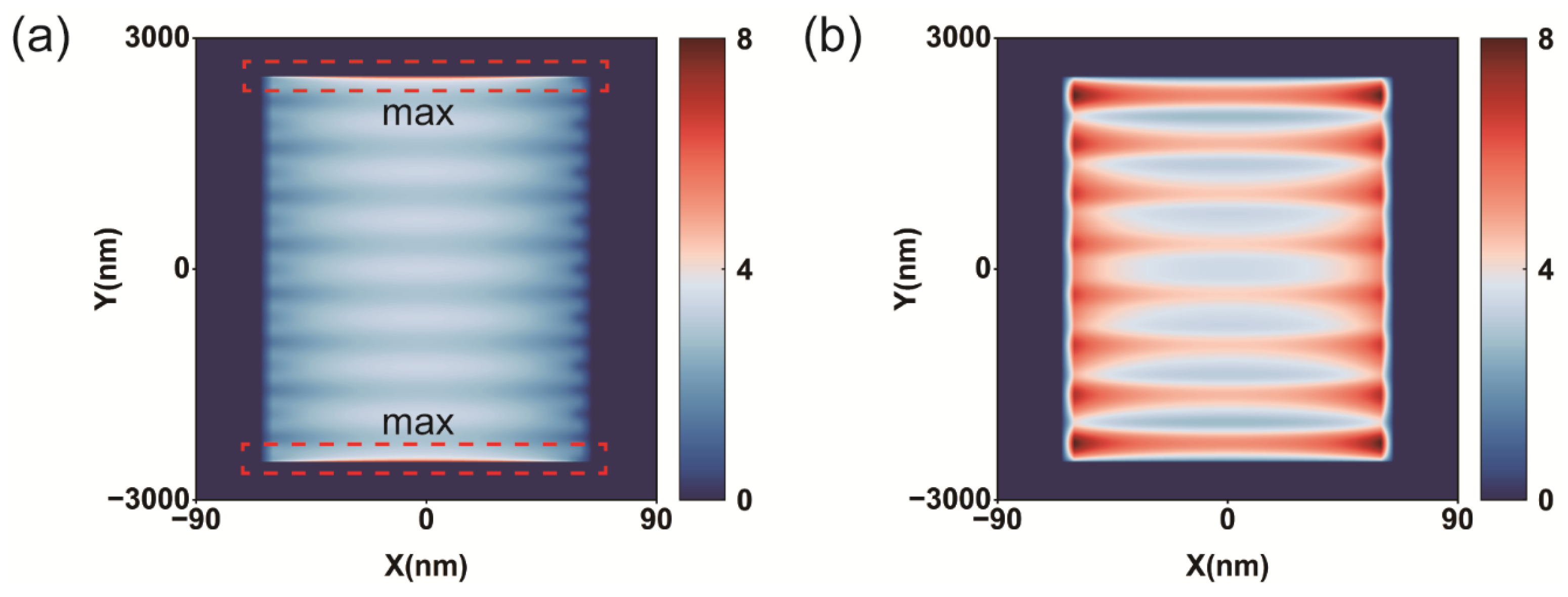
2. Design and Simulation
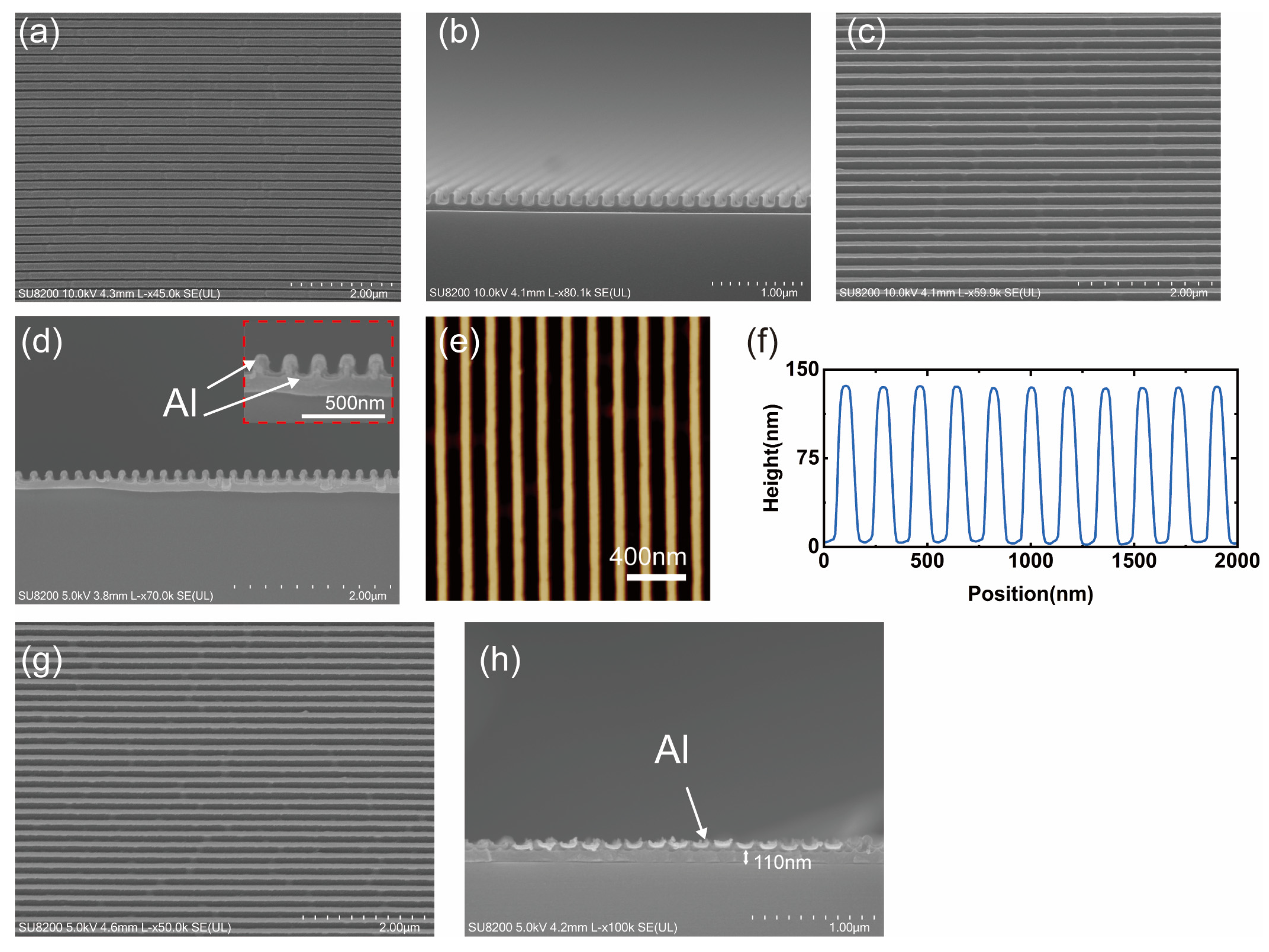
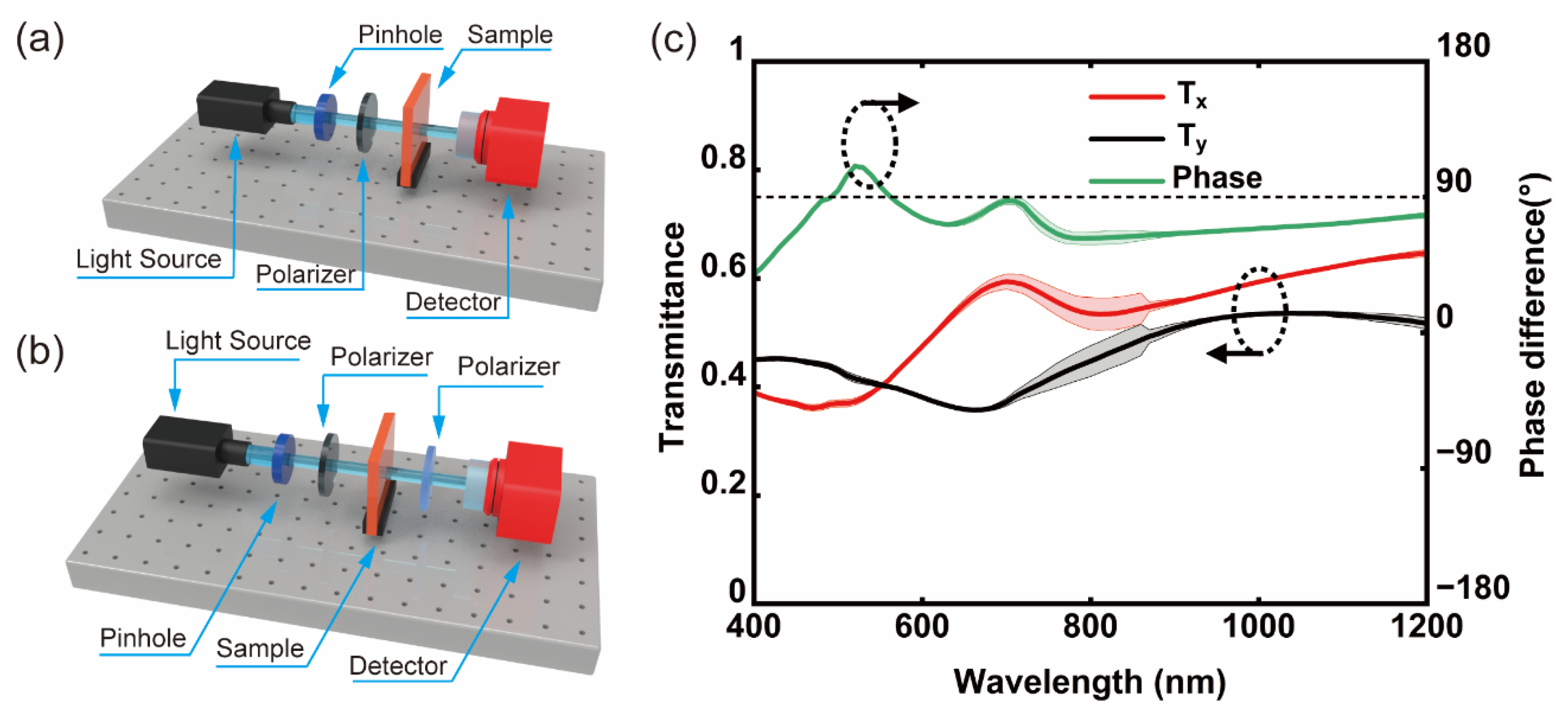
3. Device Fabrication and Characterization
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kwiatkowski, G.; Jähnig, F.; Steinhauser, J.; Wespi, P.; Ernst, M.; Kozerke, S. Direct Hyperpolarization of Micro- and Nanodiamonds for Bioimaging Applications—Considerations on Particle Size, Functionalization and Polarization Loss. J. Magn. Reson. 2018, 286, 42–51. [Google Scholar] [CrossRef] [PubMed]
- Ding, C.; Li, C.; Deng, F. Axially-Offset Differential Interference Contrast Microscopy via Polarization Wavefront Shaping. Opt. Express 2019, 27, 3837–3850. [Google Scholar] [CrossRef] [PubMed]
- Ishikawa, K.; Yatabe, K.; Ikeda, Y.; Oikawa, Y.; Onuma, T.; Niwa, H.; Yoshii, M. Optical Sensing of Sound FIelds: Non-Contact, Quantitative, and Single-Shot Imaging of Sound Using High-Speed Polarization Camera. Proc. Mtgs. Acoust. 2024, 29, 030005. [Google Scholar]
- Qin, X.; Huang, W.; Xu, M.; Jia, S. Error Analysis and Calibration Based on Division-of-Aperture Bionic Polarization Navigation Systems. Opt. Commun. 2024, 569, 130844. [Google Scholar] [CrossRef]
- Backer, A.S.; Biebricher, A.S.; King, G.A.; Wuite, G.J.L.; Heller, I.; Peterman, E.J.G. Single-Molecule Polarization Microscopy of DNA Intercalators Sheds Light on the Structure of S-DNA. Sci. Adv. 2019, 5, eaav1083. [Google Scholar] [CrossRef] [PubMed]
- Morizet, J.; Ducourthial, G.; Supatto, W.; Boutillon, A.; Legouis, R.; Schanne-Klein, M.-C.; Stringari, C.; Beaurepaire, E. High-Speed Polarization-Resolved Third-Harmonic Microscopy. Optica 2019, 6, 385–388. [Google Scholar] [CrossRef]
- Wang, C.; Chen, M.; Liu, H.; Teng, C.; Deng, H.; Yuan, L. Wideband Circular Polarization Converter Based on Graphene Metasurface at Terahertz Frequencies. Opt. Eng. 2019, 58, 1. [Google Scholar] [CrossRef]
- Cao, L.; Dou, J.; Zhao, J.; Li, B.; Hu, Y. Generation of Mode-Switchable Nanosecond Pulsed Cylindrical Vector Beams and Vortex Beams from a Q-Switched Solid-State Laser. Opt. Commun. 2023, 545, 129715. [Google Scholar] [CrossRef]
- Yi, D.-E.; Yan, Y.-B.; Liu, H.-T.; Si-Lu; Jin, G.-F. Broadband Achromatic Phase Retarder by Subwavelength Grating. Opt. Commun. 2003, 227, 49–55. [Google Scholar] [CrossRef]
- Zhu, R.; Zhou, J.; Li, B.; Huang, Y. Measurement Error Caused by Quarter-Wave Plate in the Dynamic Interferometer: Theory and Correction Method. Opt. Commun. 2021, 502, 127406. [Google Scholar] [CrossRef]
- Ma, X. Stokes Parameters Measurement System Designed by Symmetric Division of Amplitude with Double Quarter-Wave Plate. Opt. Commun. 2023, 549, 129888. [Google Scholar] [CrossRef]
- Zhang, B.; Gong, Y. Achromatic Terahertz Quarter Waveplate Based on Silicon Grating. Opt. Express 2015, 23, 14897–14902. [Google Scholar] [CrossRef]
- Mu, T.; Zhang, C.; Li, Q.; Liang, R. Achromatization of Waveplate for Broadband Polarimetric System. Opt. Lett. 2015, 40, 2485–2488. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Zhang, D.; Wen, Q.; Zhong, Z.; Wen, T. Tunable Linear-to-Circular Terahertz Polarization Convertor Enabled by a Plasmonic Nanocomposite Metasurface. Opt. Express 2023, 31, 39557. [Google Scholar] [CrossRef]
- Qu, X.; Ding, J.; Yuan, Y.; Guo, C. A Novel Broadband Polarisation Conversion Reflective Metasurface. IET Microw. Antennas Prop. 2023, 17, 93–103. [Google Scholar] [CrossRef]
- Khan, M.I.; Khalid, Z.; Tahir, F.A. Linear and Circular-Polarization Conversion in X-Band Using Anisotropic Metasurface. Sci. Rep. 2019, 9, 4552. [Google Scholar] [CrossRef] [PubMed]
- Zhao, X.-B.; Xu, L.; Yang, Y.; Yang, X.; Wu, N. 3-D Printed Conformal Dielectric Linear-to-Circular Polarization Converters for Cylindrical and Spherical Surfaces. IEEE Antennas Wirel. Propag. Lett. 2021, 20, 2539–2543. [Google Scholar] [CrossRef]
- Pan, W.; Ren, X.; Chen, Q. A Terahertz quarter wave plate based on staggered split ring resonators. Prog. Electromagn. Res. Lett. 2019, 85, 117–123. [Google Scholar] [CrossRef]
- Chang, C.-C.; Zhao, Z.; Li, D.; Taylor, A.J.; Fan, S.; Chen, H.-T. Broadband Linear-to-Circular Polarization Conversion Enabled by Birefringent Off-Resonance Reflective Metasurfaces. Phys. Rev. Lett. 2019, 123, 237401. [Google Scholar] [CrossRef] [PubMed]
- Shi, Z.; Khorasaninejad, M.; Huang, Y.-W.; Roques-Carmes, C.; Zhu, A.Y.; Chen, W.T.; Sanjeev, V.; Ding, Z.-W.; Tamagnone, M.; Chaudhary, K.; et al. Single-Layer Metasurface with Controllable Multiwavelength Functions. Nano Lett. 2018, 18, 2420–2427. [Google Scholar] [CrossRef]
- Asadulina, S.; Bogdanov, A.; Yermakov, O. All-Dielectric Meta-Waveguides for Flexible Polarization Control of Guided Light. Laser Photonics Rev. 2024, 18, 2300544. [Google Scholar] [CrossRef]
- Wang, S.; Wen, S.; Deng, Z.-L.; Li, X.; Yang, Y. Metasurface-Based Solid Poincaré Sphere Polarizer. Phys. Rev. Lett. 2023, 130, 123801. [Google Scholar] [CrossRef] [PubMed]
- Guo, J.; Wang, T.; Quan, B.; Zhao, H.; Gu, C.; Li, J.; Wang, X.; Situ, G.; Zhang, Y. Polarization Multiplexing for Double Images Display. Opto-Electron. Adv. 2019, 2, 6. [Google Scholar] [CrossRef]
- Xie, X.; Li, X.; Pu, M.; Ma, X.; Liu, K.; Guo, Y.; Luo, X. Plasmonic Metasurfaces for Simultaneous Thermal Infrared Invisibility and Holographic Illusion. Adv. Funct. Mater. 2018, 28, 1706673. [Google Scholar] [CrossRef]
- Bai, N.; Zhong, F.; Shen, J.; Fan, H.; Sun, X. A Thermal-Insensitive Ultra-Broadband Metamaterial Absorber. J. Phys. D Appl. Phys. 2021, 54, 095101. [Google Scholar] [CrossRef]
- Zhou, J.; Liu, X.; Zhang, H.; Liu, M.; Yi, Q.; Liu, Z.; Wang, J. Cross-Shaped Titanium Resonators Based Metasurface for Ultra-Broadband Solar Absorption. IEEE Photonics J. 2021, 13, 4800108. [Google Scholar] [CrossRef]
- Thiel, M.; Fischer, J.; Von Freymann, G.; Wegener, M. Direct Laser Writing of Three-Dimensional Submicron Structures Using a Continuous-Wave Laser at 532 Nm. Appl. Phys. Lett. 2010, 97, 221102. [Google Scholar] [CrossRef]
- Malinauskas, M.; Farsari, M.; Piskarskas, A.; Juodkazis, S. Ultrafast Laser Nanostructuring of Photopolymers: A Decade of Advances. Phys. Rep. 2013, 533, 1–31. [Google Scholar] [CrossRef]
- Jonušauskas, L.; Gailevičius, D.; Mikoliūnaitė, L.; Sakalauskas, D.; Šakirzanovas, S.; Juodkazis, S.; Malinauskas, M. Optically Clear and Resilient Free-Form µ-Optics 3D-Printed via Ultrafast Laser Lithography. Materials 2017, 10, 12. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Zhang, R.; Fan, Y.; Cheng, H.; Guan, C.; Chu, J. A Novel Method for the Design of a Full Stokes Polarimeter Based on Dielectric Metasurfaces. Optik 2022, 261, 169198. [Google Scholar] [CrossRef]
- Liu, J.; Chu, J.; Zhang, R.; Liu, R.; Fu, J. Wide Field of View and Full Stokes Polarization Imaging Using Metasurfaces Inspired by the Stomatopod Eye. Nanophotonics 2023, 12, 1137–1146. [Google Scholar] [CrossRef]
- Sun, T.; Hu, J.; Zhu, X.; Xu, F.; Wang, C. Broadband Single-Chip Full Stokes Polarization-Spectral Imaging Based on All-Dielectric Spatial Multiplexing Metalens. Laser Photonics Rev. 2022, 16, 2100650. [Google Scholar] [CrossRef]
- Yue, Z.; Liu, J.; Li, J.; Li, J.; Zheng, C.; Wang, G.; Chen, M.; Xu, H.; Wang, Q.; Xing, X.; et al. Multifunctional Terahertz Metasurfaces for Polarization Transformation and Wavefront Manipulation. Nanoscale 2021, 13, 14490–14496. [Google Scholar] [CrossRef] [PubMed]
- Zuo, J.; Bai, J.; Choi, S.; Basiri, A.; Chen, X.; Wang, C.; Yao, Y. Chip-Integrated Metasurface Full-Stokes Polarimetric Imaging Sensor. Light Sci. Appl. 2023, 12, 218. [Google Scholar] [CrossRef]
- Chou, S.Y.; Krauss, P.R.; Renstrom, P.J. Imprint of Sub-25 Nm Vias and Trenches in Polymers. Appl. Phys. Lett. 1995, 67, 3114–3116. [Google Scholar] [CrossRef]
- Yun, J.-G.; Kim, S.-J.; Yun, H.; Lee, K.; Sung, J.; Kim, J.; Lee, Y.; Lee, B. Broadband Ultrathin Circular Polarizer at Visible and Near-Infrared Wavelengths Using a Non-Resonant Characteristic in Helically Stacked Nano-Gratings. Opt. Express 2017, 25, 14260. [Google Scholar] [CrossRef] [PubMed]
- Baena, J.D.; Del Risco, J.P.; Slobozhanyuk, A.P.; Glybovski, S.B.; Belov, P.A. Self-Complementary Metasurfaces for Linear-to-Circular Polarization Conversion. Phys. Rev. B 2015, 92, 245413. [Google Scholar] [CrossRef]
- Päivänranta, B.; Passilly, N.; Pietarinen, J.; Laakkonen, P.; Kuittinen, M.; Tervo, J. Low-Cost Fabrication of Form-Birefringent Quarter-Wave Plates. Opt. Express 2008, 16, 16334. [Google Scholar] [CrossRef]
- Zhang, P.; Tan, Y.; Liu, W.; Chen, W. Methods for Optical Phase Retardation Measurement: A Review. Sci. China Technol. Sci. 2013, 56, 1155–1164. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, Q.; Chu, J.; Guan, C.; Zhang, C.; Zhang, R. Design and Fabrication of Ultrathin Metallic Phase Shifters for Visible and Near-Infrared Wavelengths. Micromachines 2025, 16, 74. https://doi.org/10.3390/mi16010074
Guo Q, Chu J, Guan C, Zhang C, Zhang R. Design and Fabrication of Ultrathin Metallic Phase Shifters for Visible and Near-Infrared Wavelengths. Micromachines. 2025; 16(1):74. https://doi.org/10.3390/mi16010074
Chicago/Turabian StyleGuo, Qing, Jinkui Chu, Chuanlong Guan, Chuxiao Zhang, and Ran Zhang. 2025. "Design and Fabrication of Ultrathin Metallic Phase Shifters for Visible and Near-Infrared Wavelengths" Micromachines 16, no. 1: 74. https://doi.org/10.3390/mi16010074
APA StyleGuo, Q., Chu, J., Guan, C., Zhang, C., & Zhang, R. (2025). Design and Fabrication of Ultrathin Metallic Phase Shifters for Visible and Near-Infrared Wavelengths. Micromachines, 16(1), 74. https://doi.org/10.3390/mi16010074






