Variable Range Hopping Model Based on Gaussian Disordered Organic Semiconductor for Seebeck Effect in Thermoelectric Device
Abstract
:1. Introduction
2. Model Methods
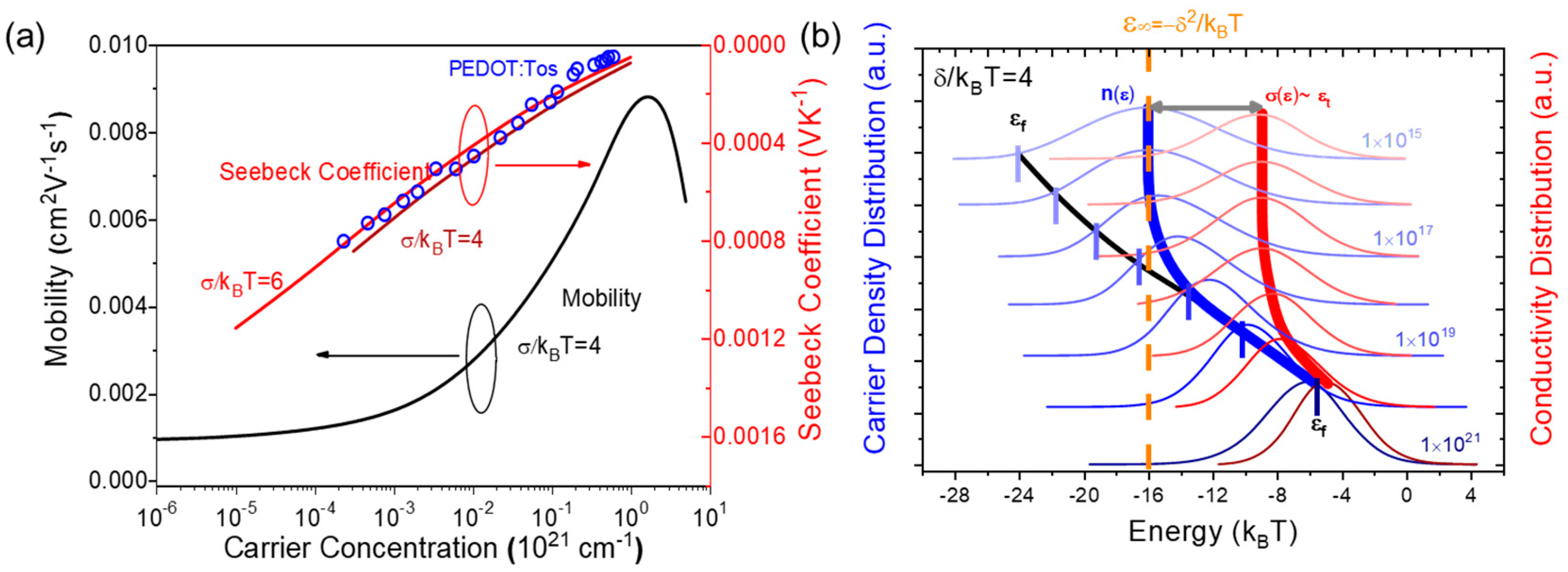
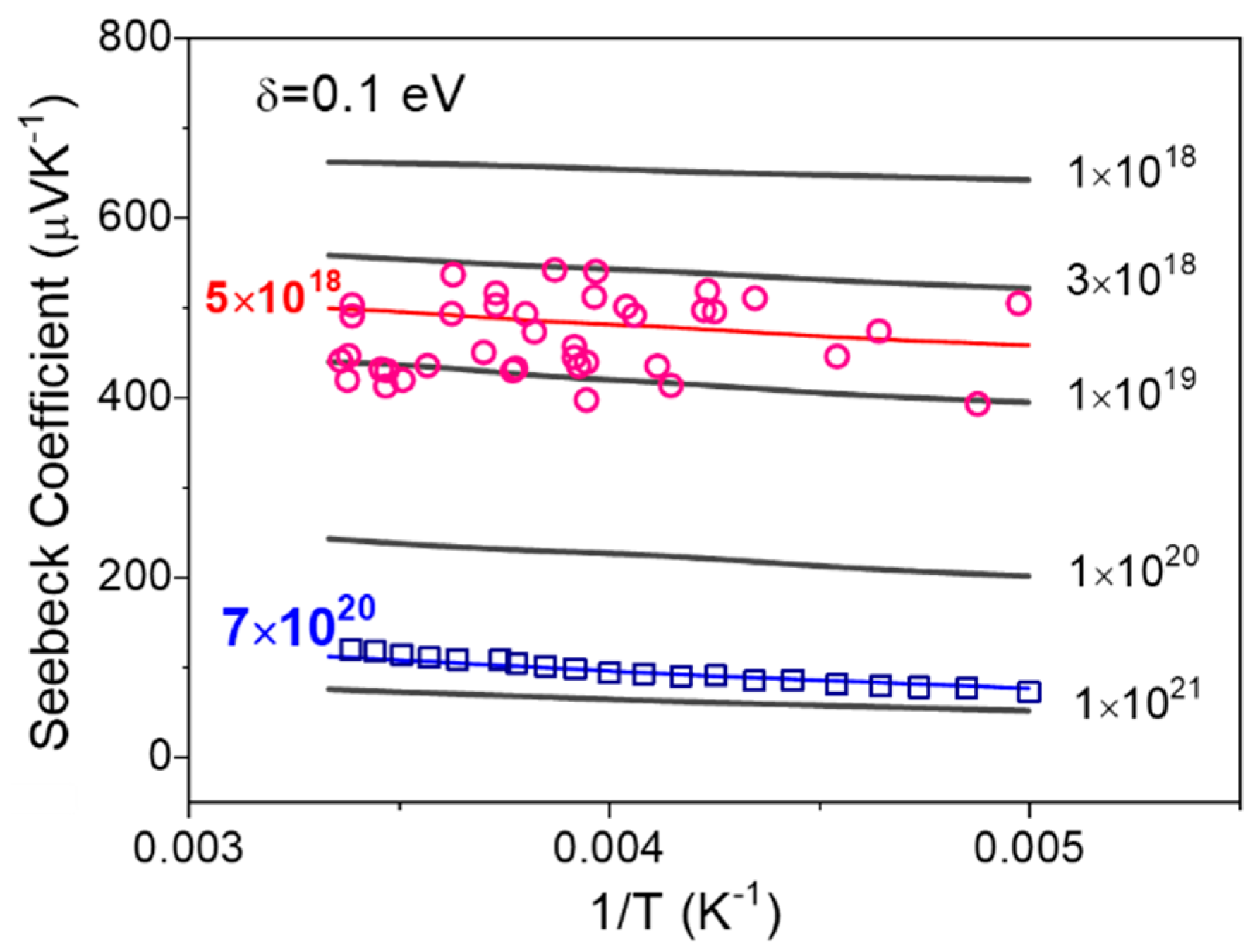
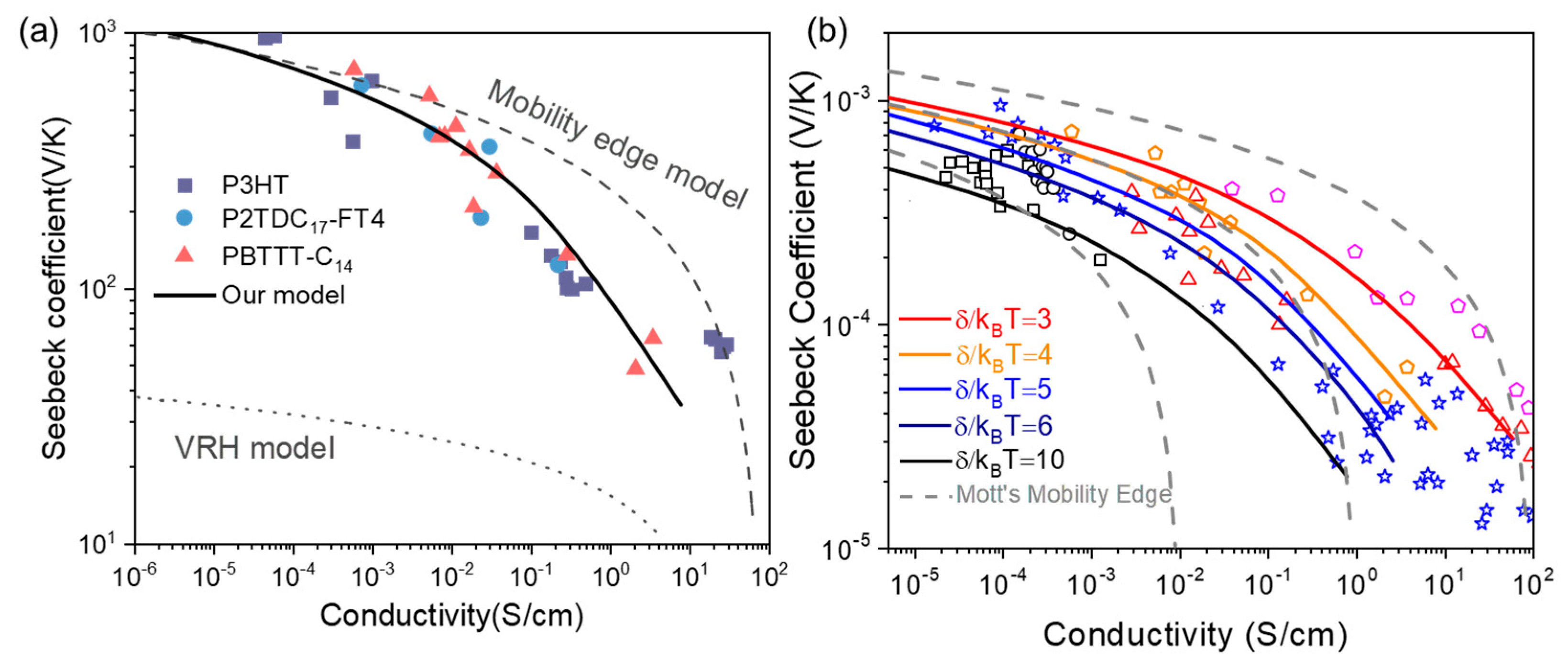
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Shi, X.-L.; Zou, J.; Chen, Z.-G. Advanced Thermoelectric Design: From Materials and Structures to Devices. Chem. Rev. 2020, 120, 7399–7515. [Google Scholar] [CrossRef] [PubMed]
- Snyder, G.J.; Ursell, T.S. Thermoelectric Efficiency and Compatibility. Phys. Rev. Lett. 2003, 91, 148301. [Google Scholar] [CrossRef] [Green Version]
- Zhang, F.; Di, C.-A. Exploring Thermoelectric Materials from High Mobility Organic Semiconductors. Chem. Mater. 2020, 32, 2688–2702. [Google Scholar] [CrossRef]
- Zhang, F.; Zang, Y.; Huang, D.; Di, C.-A.; Gao, X.; Sirringhaus, H.; Zhu, D. Modulated Thermoelectric Properties of Organic Semiconductors Using Field-Effect Transistors. Adv. Funct. Mater. 2015, 25, 3004–3012. [Google Scholar] [CrossRef]
- Hannewald, K.; Stojanovic, V.M.; Schellekens, J.M.T.; Bobbert, P.A.; Kresse, G.; Hafner, J. Theory of polaron band-width narrowing in organic molecular crystals. Phys. Rev. B 2004, 69, 075211. [Google Scholar] [CrossRef] [Green Version]
- Troisi, A.; Orlandi, G. Charge-Transport Regime of Crystalline Organic Semiconductors: Diffusion Limited by Thermal Off-Diagonal Electronic Disorder. Phys. Rev. Lett. 2006, 96, 086601. [Google Scholar] [CrossRef] [PubMed]
- Mott, N.F. Conduction in Non-Crystalline Systems: I. Localized Electronic States in Disordered Systems. Philos. Mag. 1968, 17, 1259–1268. [Google Scholar] [CrossRef]
- Baranovski, S. Charge Transport in Disordered Solids with Applications in Electronics; John Wiley & Sons: Chichester, UK, 2006. [Google Scholar]
- Kang, S.; Snyder, G. Charge-transport model for conducting polymers. Nat. Mater. 2017, 16, 252–257. [Google Scholar] [CrossRef]
- Bässler, H. Charge Transport in Disordered Organic Photoconductors a Monte Carlo Simulation Study. Phys. Stat. Sol. B 1993, 175, 15. [Google Scholar] [CrossRef]
- Lu, N.; Li, L.; Liu, M. Universal carrier thermoelectric-transport model based on percolation theory in organic semi-conductors. Phys. Rev. B 2015, 91, 195205. [Google Scholar] [CrossRef]
- Li, L.; Lu, N.; Liu, M.; Bässler, H. General Einstein relation model in disordered organic semiconductors under quasiequilibrium. Phys. Rev. B 2014, 90, 214107. [Google Scholar] [CrossRef]
- Mehraeen, S.; Coropceanu, V.; Brédas, J.-L. Role of band states and trap states in the electrical properties of organic semiconductors: Hopping versus mobility edge model. Phys. Rev. B 2013, 87, 195209. [Google Scholar] [CrossRef] [Green Version]
- Vissenberg, M.C.J.M.; Matters, M. Theory of the field-effect mobility in amorphous organic transistors. Phys. Rev. B 1998, 57, 12964–12967. [Google Scholar] [CrossRef] [Green Version]
- Mott, N.F.; Davis, E.A. Electronic Processes in Non-Crystalline Materials; Clarendon Press: Oxford, UK, 1971. [Google Scholar]
- Fritzsche, H. A general expression for the thermoelectric power. Solid State Commun. 1971, 9, 1813–1815. [Google Scholar] [CrossRef]
- Arkhipov, V.I.; Heremans, P.; Emelianova, E.V.; Bässler, H. Effect of doping on the density-of-states distribution and carrier hopping in disordered organic semiconductors. Phys. Rev. B 2005, 71, 045214. [Google Scholar] [CrossRef]
- Tanase, C.; Meijer, E.J.; Blom, P.W.M.; De Leeuw, D.M. Unification of the Hole Transport in Polymeric Field-Effect Transistors and Light-Emitting Diodes. Phys. Rev. Lett. 2003, 91, 216601. [Google Scholar] [CrossRef] [Green Version]
- Oelerich, J.O.; Huemmer, D.; Baranovskii, D. How to find out the density of states in disordered organic semiconductors. Phys. Rev. Lett. 2012, 108, 226403. [Google Scholar] [CrossRef]
- Li, L.; Meller, G.; Kosina, H. Einstein relation in hopping transport of organic semiconductors. Appl. Phys. Lett. 2008, 92, 013307. [Google Scholar] [CrossRef]
- Bubnova, O.; Khan, Z.U.; Malti, A.; Braun, S.; Fahlman, M.; Berggren, M.; Crispin, X. Optimization of the thermoelectric figure of merit in the conducting polymer poly (3,4-ethylenedioxythiophene). Nat. Mater. 2011, 10, 429. [Google Scholar] [CrossRef]
- Gao, X.; Uehara, K.; Klug, D.D.; Patchkovskii, S.; Tse, J.S.; Tritt, T.M. Theoretical studies on the thermopower of semiconductors and low-band-gap crystalline polymers. Phys. Rev. B 2005, 72, 125202. [Google Scholar] [CrossRef]
- Kaiser, A.B. Thermoelectric power and conductivity of heterogeneous conducting polymers. Phys. Rev. B 1989, 40, 2806. [Google Scholar] [CrossRef] [PubMed]
- Park, Y. Structure and morphology: Relation to thermopower properties of conductive polymers. Synth. Met. 1991, 45, 173. [Google Scholar] [CrossRef]
- Glaudell, A.M.; Cochran, J.E.; Patel SNChabinyc, M.L. Impact of the doping method on conductivity and thermopower in semiconducting polythiophenes. Adv. Energy Mater. 2015, 5, 1401072. [Google Scholar] [CrossRef]
- Kaiser, A.B. Electronic transport properties of conducting polymers and carbon nanotubes. Rep. Prog. Phys. 2001, 64, 1–49. [Google Scholar] [CrossRef]
- Sun, J.; Yeh, M.L.; Jung, B.J.; Zhang, B.; Feser, J.; Majumdar, A.; Katz, H.E. Simultaneous increase in seebeck coefficient and conductivity in a doped poly (alkylthiophene) blend with defined density of states. Macromolecules 2010, 43, 2897. [Google Scholar] [CrossRef]
- Zhang, Q.; Sun, Y.M.; Xu, W.; Zhu, D.B. What to expect from conducting polymers on the playground of thermoelectricity: Lessons learned from four high-mobility polymeric semiconductors. Macromolecules 2014, 47, 609. [Google Scholar] [CrossRef]
- Fan, Z.; Ouyang, J. Thermoelectric Properties of PEDOT:PSS. Adv. Energy Mater. 2019, 5, 1800769. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Y.; Wang, J. Variable Range Hopping Model Based on Gaussian Disordered Organic Semiconductor for Seebeck Effect in Thermoelectric Device. Micromachines 2022, 13, 707. https://doi.org/10.3390/mi13050707
Zhao Y, Wang J. Variable Range Hopping Model Based on Gaussian Disordered Organic Semiconductor for Seebeck Effect in Thermoelectric Device. Micromachines. 2022; 13(5):707. https://doi.org/10.3390/mi13050707
Chicago/Turabian StyleZhao, Ying, and Jiawei Wang. 2022. "Variable Range Hopping Model Based on Gaussian Disordered Organic Semiconductor for Seebeck Effect in Thermoelectric Device" Micromachines 13, no. 5: 707. https://doi.org/10.3390/mi13050707
APA StyleZhao, Y., & Wang, J. (2022). Variable Range Hopping Model Based on Gaussian Disordered Organic Semiconductor for Seebeck Effect in Thermoelectric Device. Micromachines, 13(5), 707. https://doi.org/10.3390/mi13050707




