Towards Transient Electronics through Heat Triggered Shattering of Off-the-Shelf Electronic Chips
Abstract
:1. Introduction
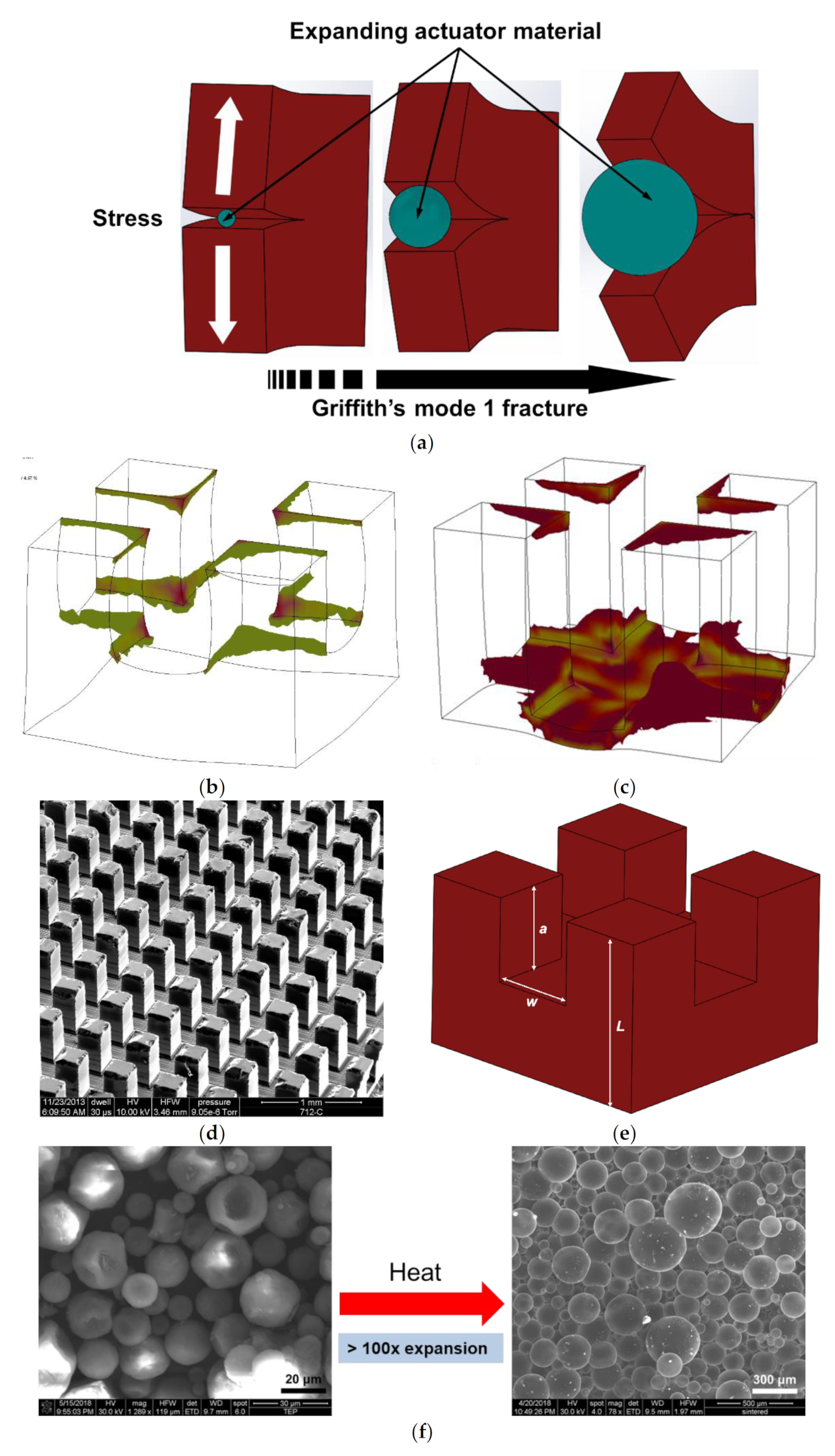
2. Transience Mechanism
3. Materials and Methods
3.1. Expansion Mechanism
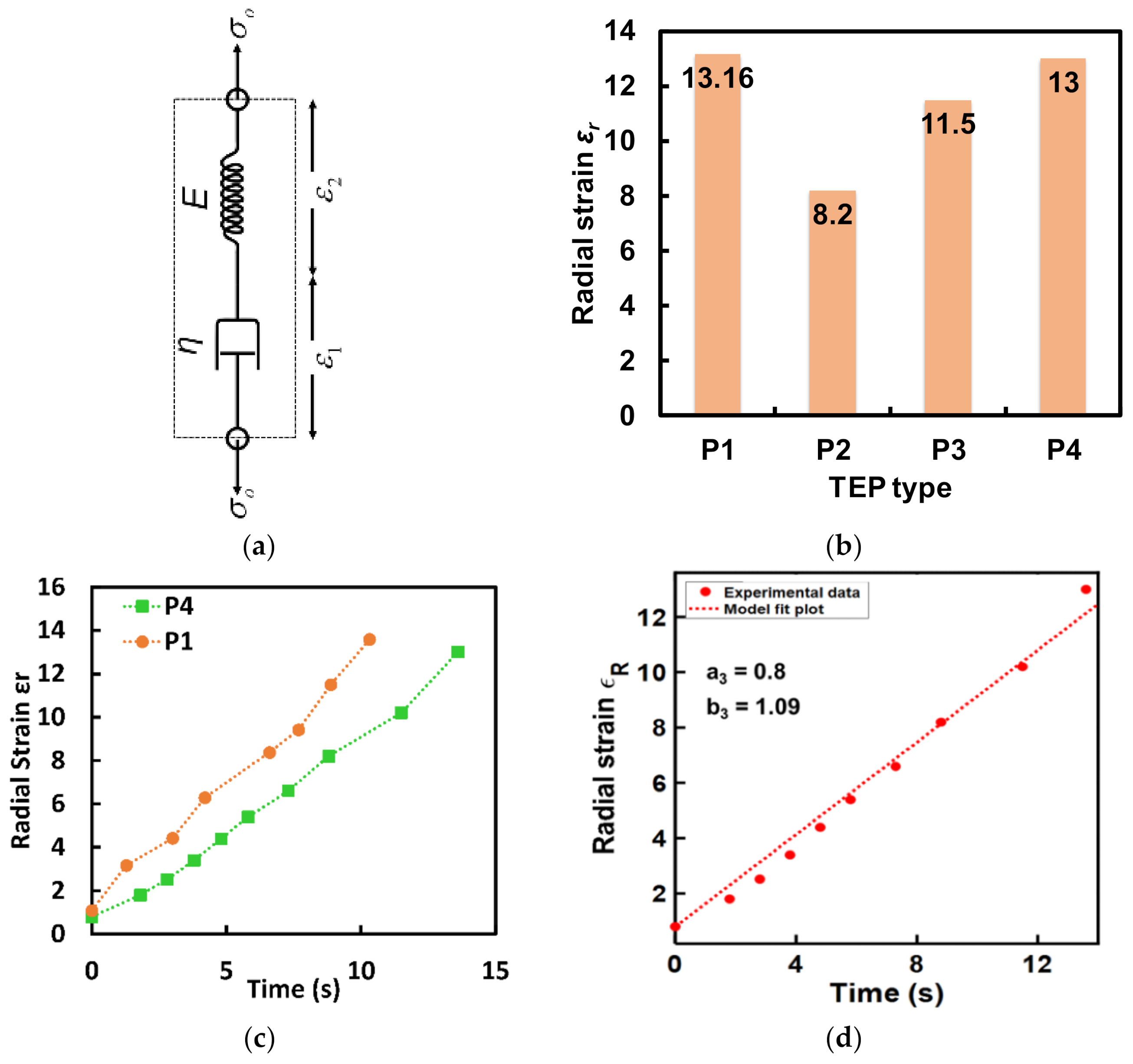
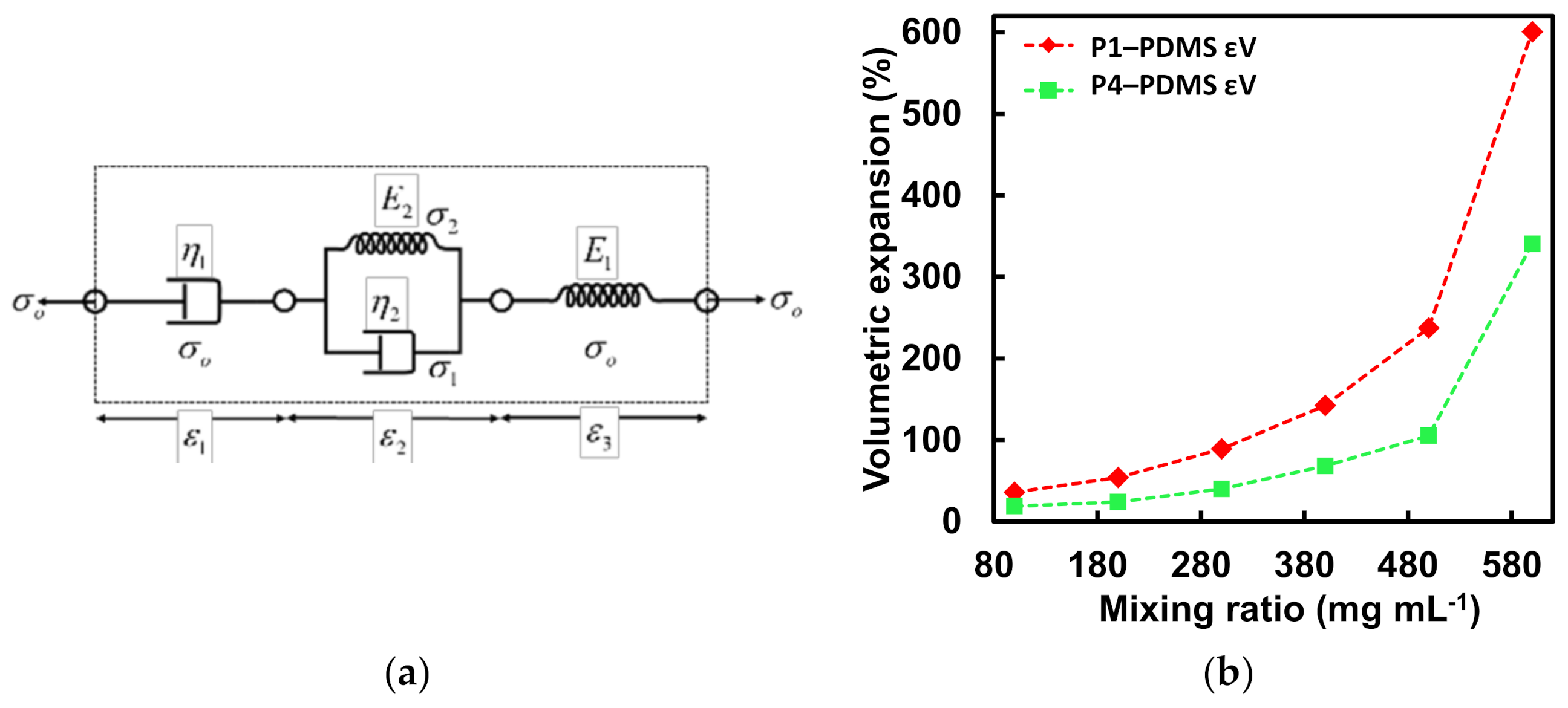
3.2. Expansion Kinetics
4. Device Fabrication
4.1. Backside Grooves
4.2. TE Transience Test Chip
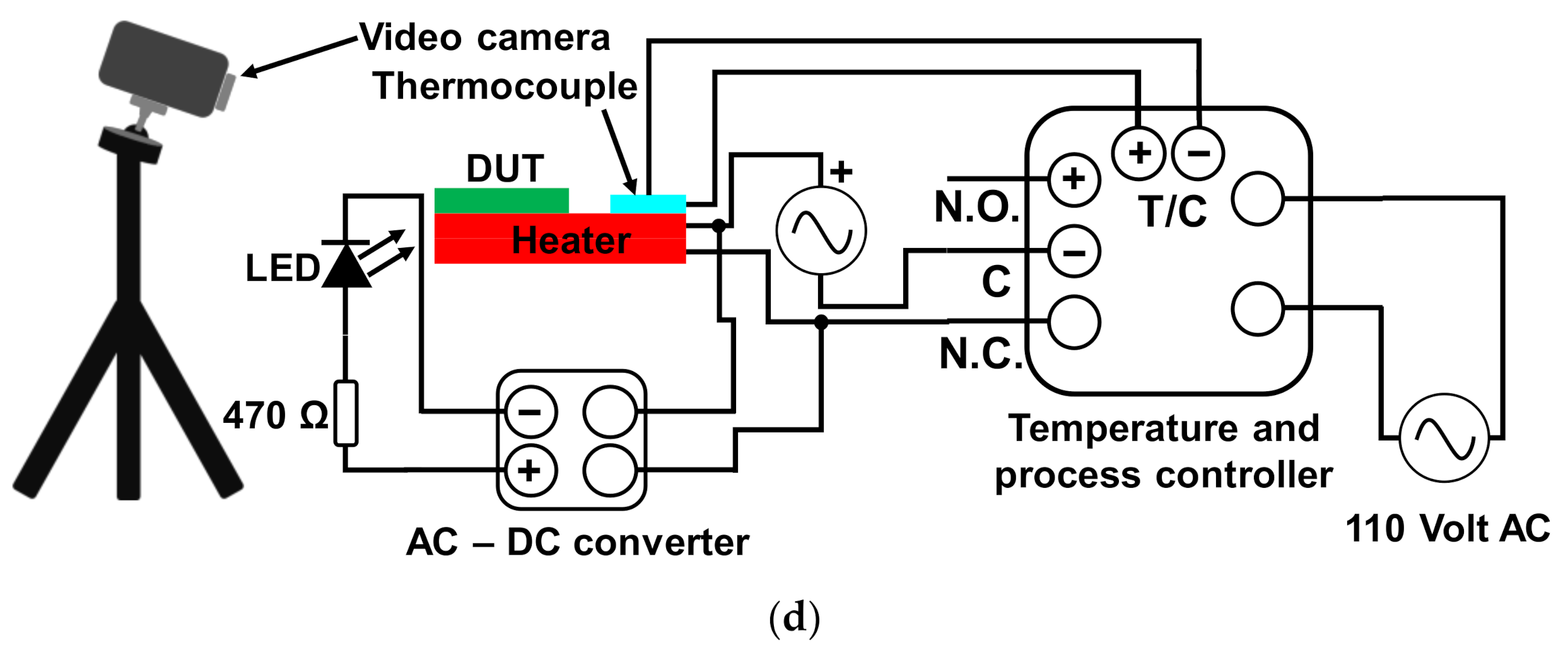
4.3. Thermal Transience Test Set-Up
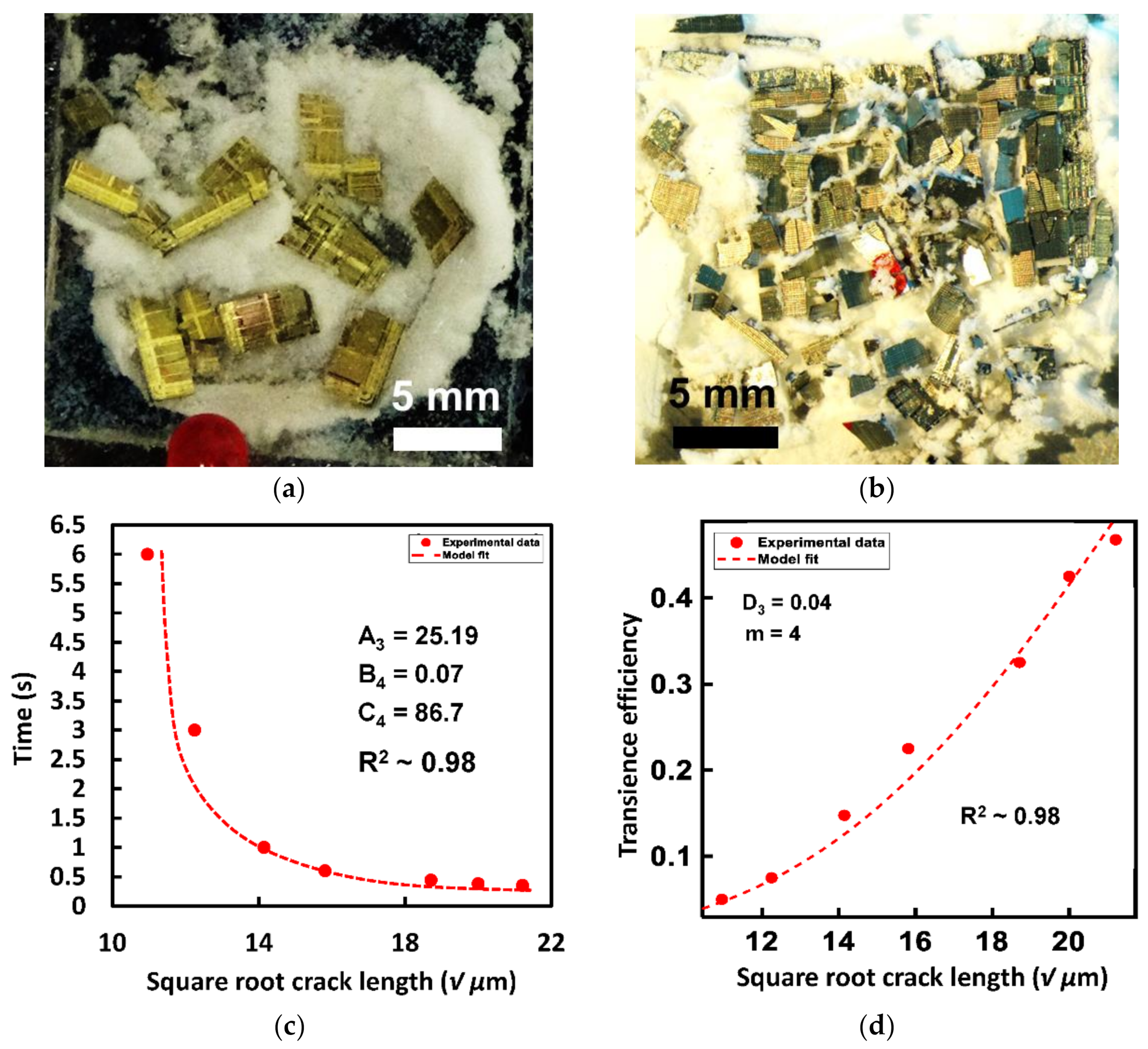
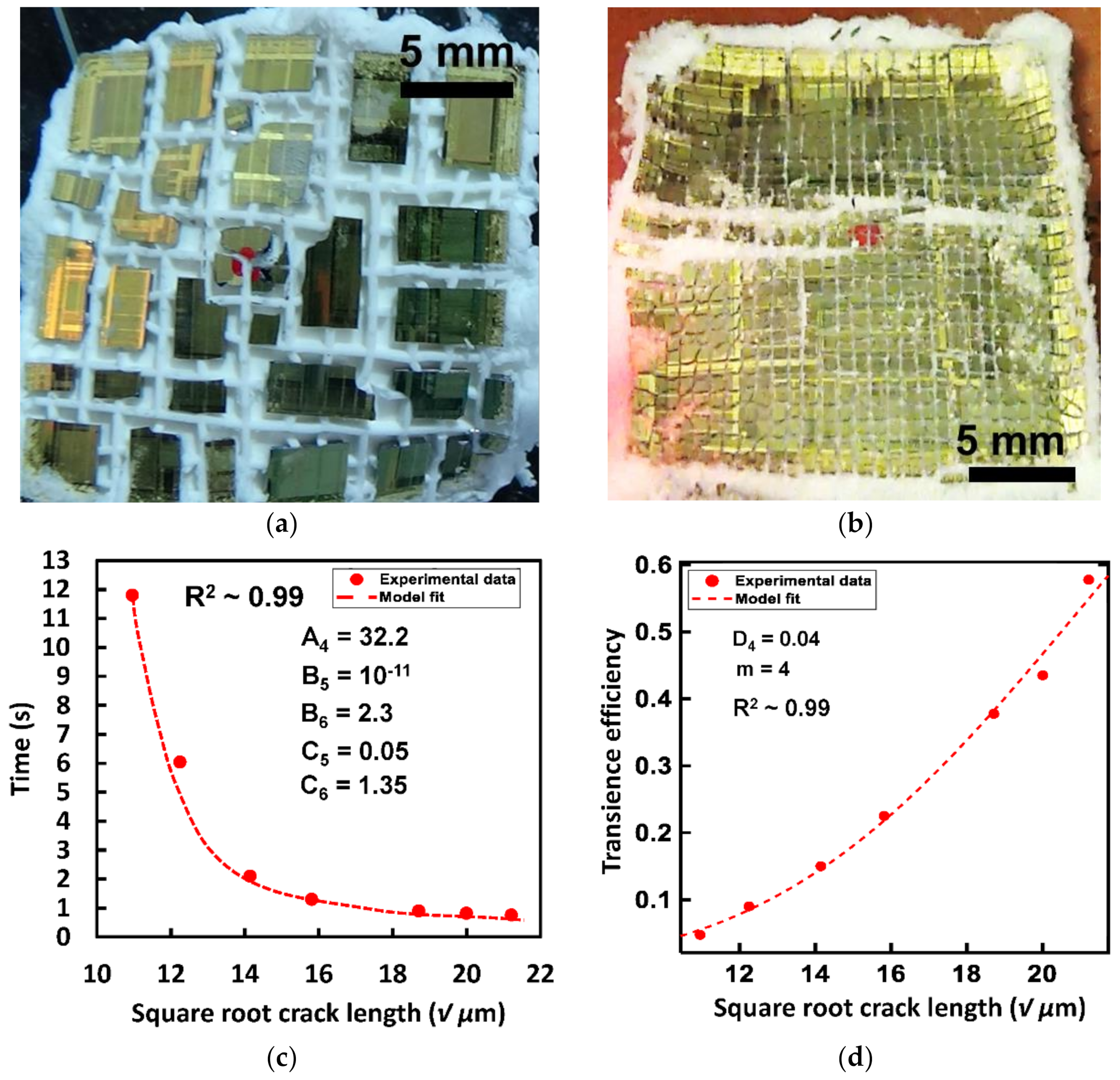
5. Results and Discussions
5.1. Heat Triggered Transience via TEP Microspheres
5.2. Heat Triggered Transience via TE Elastomer
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Quadir, S.E.; Chen, J.; Forte, D.; Asadizanjani, N.; Shahbazmohamadi, S.; Wang, L.; Chandy, J.; Tehranipoor, M. A Survey on Chip to System Reverse Engineering. Acm. J. Emerg. Tech. Com. 2017, 13, 1–34. [Google Scholar] [CrossRef]
- Privacy Right Clearinghouse, Data Breaches by Breach Type. Available online: https://privacyrights.org/data-breaches (accessed on 29 December 2021).
- Ponemon Institute, 2020 Cost of Data Breach Study: Global Overview. Available online: https://www.ibm.com/security/data-breach (accessed on 29 December 2021).
- Lord, N. What is Data Encryption. Available online: https://digitalguardian.com/blog/what-data-encryption (accessed on 29 December 2021).
- Brecht, D. Tales from the Crypt: Hardware vs. Software. Available online: https://www.infosecurity-magazine.com/magazine-features/tales-crypt-hardware-software/ (accessed on 29 December 2021).
- Müller, T.; Latzo, T.; Freiling, F.C. Secure deletion for flash-based self-encrypting drives. In Proceedings of the 29th Chaos Communication Congress, Hamburg, Germany, 27–30 December 2012. [Google Scholar]
- Boteanu, D. Bypassing Self-Encrypting Drives in Enterprise Environments. Available online: https://docplayer.net/9511959-Bypassing-self-encrypting-drives-sed-in-enterprise-environments-daniel-boteanu-kevvie-fowler-november-12-th-2015.html (accessed on 29 December 2021).
- Gu, X.; Lou, W.; Song, R.; Zhao, Y.; Zhang, L. Simulation research on a novel micro-fluidic self-destruct device for microchips. In Proceedings of the IEEE 5th International Conference on Nano/Micro Engineered and Molecular Systems, Xiamen, China, 20–23 January 2010. [Google Scholar]
- Smolker, G. Method and Apparatus for Protecting Sensitive Information Contained in Thin-Film Microelectonic Circuitry. U.S. Patent 3,882,323, 6 May 1975. [Google Scholar]
- Shield, D.J.; Davis, D.L. Method and Apparatus for Fast Self Detruction of a CMOS Integrated Circuit. U.S. Patent 5,736,7776, 7 April 1998. [Google Scholar]
- Keister, F.Z.; Smolker, G.S. Single Layer Self-Destruct Circuit Produced by Co-deposition of Tungsten Oxide and Aluminium. U.S. Patent 3,742,120, 26 June 1973. [Google Scholar]
- Hwang, S.W.; Kang, S.K.; Huang, X.; Brenckle, M.A.; Omenetto, F.G.; Rogers, J.A. Materials for Programmed, Functional Transformation in Transient Electronic Systems. Adv. Mater. 2014, 27, 47–52. [Google Scholar] [CrossRef] [PubMed]
- Keim, S.; Brunner, J.G.; Fabry, B.; Virtanen, S. Control of magnesium corrosion and biocompatibility with biomimetic coatingsr. J. Biomed. Mater. Res B 2011, 96B, 84–90. [Google Scholar] [CrossRef] [PubMed]
- Kirkland, N.T.; Birbilis, N.; Staiger, M.P. Assessing the corrosion of biodegradable magnesium implants: A critical review of current methodologies and their limitations. Acta Biomater. 2012, 8, 925–936. [Google Scholar] [CrossRef]
- Kang, S.K.; Hwang, S.W.; Yu, S.; Seo, J.H.; Corbin, E.A.; Shin, J.; Wie, D.S.; Bashir, R.; Ma, Z.; Rogers, J.A. Transient Eletronics: Biodegradable Thin Metal Foils and Spin-On Glass Materials for Transient Electronics. Adv. Funct. Mater. 2015, 25, 1789. [Google Scholar] [CrossRef]
- Yin, L.; Bozler, C.; Harburg, D.V.; Omenetto, F.; Rogers, J.A. Materials and fabrication sequences for water soluble silicon integrated circuits at the 90 nm node. Appl. Phys. Lett. 2015, 106, 0141051. [Google Scholar] [CrossRef]
- Hwang, S.W.; Kim, D.H.; Tao, H.; Kim, T.I.; Kim, S.; Yu, K.J.; Panilaitis, B.; Jeong, J.W.; Song, J.K.; Omenetto, F.G.; et al. Materials and fabrication processes for transient and bioresorbable high-performance electronics. Adv. Funct. Mater. 2013, 23, 4087–4093. [Google Scholar] [CrossRef]
- Witte, F. The history of biodegradable magnesium implants: A review. Acta. Biomater. 2010, 6, 1680–1692. [Google Scholar] [CrossRef]
- Hermawan, H.; Purnama, A.; Dube, D.; Couet, J.; Mantovani, D. Fe-Mn Alloys for Metallic Biodegradable Stents: Degradation and Cell Viability Studies. Acta. Biomater. 2010, 6, 1852–1860. [Google Scholar] [CrossRef]
- Dagdeviren, C.; Hwang, S.W.; Su, Y.; Kim, S.; Cheng, H.; Gur, O.; Haney, R.; Omenetto, F.G.; Huang, Y.; Rogers, J.A. Transient, biocompatible electronics and energy harvesters based on ZnO. Small 2013, 9, 3398–3404. [Google Scholar] [CrossRef]
- Vladu, M.I. “Green” electronics: Biodegradable and biocompatible materials and devices for sustainable future. Chem. Soc. Rev. 2014, 43, 588–610. [Google Scholar] [CrossRef] [Green Version]
- Zelikin, A.N.; Lynn, D.M.; Farhadi, J.; Martin, I.; Shastri, V.; Langer, R. Erodible conducting polymers for potential biomedical applications. Angew. Chem. Int. Ed. Engl. 2002, 41, 141–144. [Google Scholar] [CrossRef]
- Xia, Y.; Sun, K.; Ouyang, J. Solution-processed metallic conducting polymer films as transparent electrode of optoelectronic devices. J. Adv. Mater. 2012, 24, 2436–2440. [Google Scholar] [CrossRef] [PubMed]
- Wu, H.; Hu, L.; Rowell, M.W.; Kong, D.; Cha, J.J.; McDonough, J.R.; Zhu, J.; Yang, Y.; McGehee, M.D.; Cui, Y. Electrospun Metal Nanofiber Webs as High-Performance Transparent Electrode. Nano Lett. 2010, 10, 4242–4248. [Google Scholar] [CrossRef] [PubMed]
- Jiang, H.; Moon, K.; Li, Y.; Wong, C.P. Surface Functionalized Silver Nanoparticles for Ultrahigh Conductive Polymer Composites. Chem. Mater 2006, 18, 2969–2973. [Google Scholar] [CrossRef]
- Merilampi, S.; Laine-Ma, T.; Ruuskanen, P. The characterization of electrically conductive silver ink patterns on flexible substrates. Microelectron Reliab. 2009, 49, 782–790. [Google Scholar] [CrossRef]
- Hwang, S.W.; Song, J.K.; Huang, X.; Cheng, H.; Kang, S.K.; Kim, B.H.; Kim, J.H.; Yu, S.; Huang, Y.; Rogers, J.A. High-performance biodegradable/transient electronics on biodegradable polymers. Adv. Mater. 2014, 26, 3905–3911. [Google Scholar] [CrossRef]
- Bettinger, C.J.; Bao, Z. Organic thin-film transistors fabricated on resorbable biomaterial substrates. Adv. Mater. 2010, 22, 651. [Google Scholar] [CrossRef] [Green Version]
- Hernandez, H.L.; Kang, S.K.; Lee, O.P.; Hwang, S.W.; Kaitz, J.A.; Inci, B.; Park, C.W.; Chung, S.; Sottos, N.R.; Moore, J.S.; et al. Triggered transience of metastable poly(phthalaldehyde) for transient electronics. Adv. Mater. 2014, 26, 7637–7642. [Google Scholar] [CrossRef]
- Jung, Y.H.; Chang, T.H.; Zhang, H.; Yao, C.; Zheng, Q.; Yang, V.W.; Mi, H.; Kim, M.; Cho, S.J.; Park, D.W.; et al. High-performance green flexible electronics based on biodegradable cellulose nanofibril paper. Nat. Commun. 2015, 6, 7170–7171. [Google Scholar] [CrossRef]
- Hwang, S.W.; Tao, H.; Kim, D.H.; Cheng, H.; Song, J.K.; Rill, E.; Brenckle, M.A.; Panilaitis, B.; Won, S.M.; Kim, Y.S.; et al. A physically transient form of silicon electronics. Science 2012, 337, 1640–1644. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kang, S.K.; Park, G.; Kim, K.; Hwang, S.W.; Cheng, H.; Shin, J.; Chung, S.; Kim, M.; Yin, L.; Lee, J.C.; et al. Dissolution chemistry and biocompatibility of silicon- and germanium-based semiconductors for transient electronics. ACS Appl. Mater. Inter. 2015, 7, 9297–9305. [Google Scholar] [CrossRef] [PubMed]
- Hwang, S.W.; Park, G.; Edwards, C.; Corbin, E.A.; Kang, S.K.; Cheng, H.; Song, J.K.; Kim, J.H.; Yu, S.; Ng, J.; et al. Dissolution chemistry and biocompatibility of single-crystalline silicon nanomembranes and associated materials for transient electronics. ACS Nano 2014, 8, 5843–5851. [Google Scholar] [CrossRef] [PubMed]
- Fu, K.K.; Wang, Z.; Dai, J.; Carter, M.; Hu, L. Transient Electronics: Materials and Devices. Chem. Mater. 2016, 28, 3527–3539. [Google Scholar] [CrossRef]
- Dubrova, E. Hardware Security. Available online: https://www.kth.se/social/files/59102ef5f276540f03507109/hardware_security__2017_05_08.pdf (accessed on 29 December 2021).
- Pandey, S.S.; Banerjee, N.; Xie, Y.; Mastrangelo, C.H. Self-Destructing Secured Microchips by On-Chip Triggered Energetic and Corrosive Attacks for Transient Electronics. Adv. Mater. Technol. 2018, 3, 1800044. [Google Scholar] [CrossRef]
- Banerjee, N.; Xie, Y.; Rahman, M.M.; Kim, H.; Mastrangelo, C.H. From chips to dust: The mems shatter secure chip. In Proceedings of the 2014 IEEE 27th International Conference on Micro Electro Mechanical Systems (MEMS), San Francisco, CA, USA, 26–30 January 2014. [Google Scholar]
- Gardfors, T. Synthesis and Evaluation of Expancel Microspheres. Available online: https://www.diva-portal.org/smash/get/diva2:662295/FULLTEXT01.pdf (accessed on 29 December 2021).
- Jonsson, M.; Nordin, O.; Kron, A.L.; Malmström, E. Thermally expandable microspheres with excellent expansion characteristics at high temperature. J. Appl. Polym. Sci. 2010, 117, 384–392. [Google Scholar] [CrossRef]
- Huang, Y. Development of High-Speed Optical Coherence Tomography for Time-Lapse Nondestructive Characterization of Samples. Ph.D. Thesis, Lehigh University, Bethlehem, PA, USA, November 2004. [Google Scholar]
- Roylance, D. Introduction to Fracture Mechanics. Available online: https://web.mit.edu/course/3/3.11/www/modules/frac.pdf (accessed on 29 December 2021).
- Patricio, M.; Mattheji, R.M.M. Crack Propagation Analysis. Available online: https://www.win.tue.nl/analysis/reports/rana07-23.pdf (accessed on 29 December 2021).
- Kuttulamadom, M.A.; Hamzehlouia, S.; Mears, M.L. Effect of Machining Feed on Surface Roughness in Cutting 6061 Aluminum. Available online: https://pdfs.semanticscholar.org/b737/c62c26de8d9ad394d944bbe0595611fe058d.pdf (accessed on 29 December 2021).
- Vagues, M. Analysing Backside Chipping Issues of the Die at Wafer Saw. Available online: https://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.566.9979&rep=reP1&type=pdf (accessed on 29 December 2021).
- Schijve, J. Stress concentration at notches. In Fatigue of Structure and Materials; Schijve, J., Ed.; Springer: Berlin/Heidelberg, Germany, 2009; pp. 59–88. [Google Scholar]
- Basu, B.; Tiwari, D.; Kundu, D.; Prasad, R. Is Weibull Distribution the Most Appropriate Statistical Strength Distribution for Brittle Materials? Ceram. Int. 2009, 35, 237–246. [Google Scholar] [CrossRef] [Green Version]
- Weibull, W. A Statistical Theory of the Strength of Materials; Generalstabens Litografiska Anstalts Förlag: Stockholm, Sweden, 1939. [Google Scholar]
- Bertalan, Z.; Shekhawat, A.; Sethna, J.P.; Zapperi, S. Fracture Strength: Stress Concentration, Extreme Value Statistics, and the Fate of the Weibull Distribution. Phys. Rev. Appl. 2014, 2, 034008. [Google Scholar] [CrossRef] [Green Version]
- Lopez, O.B.; Vodenitcharova, T.; Hoffman, M.; Leo, A.J. Surface fracture of polycrystalline silicon wafers: Experimental study. J. Am. Ceram. Soc. 2009, 92, 2713. [Google Scholar] [CrossRef]
- Deluca, M.; Bermejo, R.; Pletz, M.; Supancic, P. Strength and fracture analysis of silicon-based components for embedding. J. Eur. Ceram. Soc. 2010, 31, 549–558. [Google Scholar] [CrossRef]
- Gazzani, S.E.; Nassiet, V.; Habas, J.P.; Freydier, C.; Hilleshein, A. High Temperature Epoxy Foam: Optimization of Process Parameters. Polymers 2016, 8, 215. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kuo, A.C.M. Poly(dimethylsiloxane). In Polymer Data Handbook; Mark, J.E., Ed.; Oxford University Press: New York, NY, USA, 1999. [Google Scholar]
- Fincan, M. Assessing Viscoelastic Properties of Polydimethylsiloxane (PDMS) Using Loading and Unloading of the Macroscopic Compression Test. Ph.D. Thesis, University of South Florida, Tampa, FL, USA, April 2015. [Google Scholar]
- Duffy, S.F. Linear Viscoelasticity. Available online: https://academic.csuohio.edu/duffy_s/Linear_Visco.pdf (accessed on 29 December 2020).


| Commercial Name | Size (μm) | Tstart (°C) | Tend (°C) | Grade |
|---|---|---|---|---|
| 461-DU-20 | 6–9 | 100–106 | 143–151 | P1 |
| 461-DU-40 | 9–15 | 98–104 | 144–152 | P2 |
| 920-DU-80 | 18–24 | 123–133 | 180–195 | P3 |
| 920-DU-120 | 28–38 | 133–143 | 192–207 | P4 |
| f(t) | L{f(t)} |
| α | α/s |
| H(t) | 1/s |
| δ(t − τ) | e−τs |
| dδ(t)/dt | s |
| e−αt | 1/(α+s) |
| (1 − e−αt)/α | 1/s(α+s) |
| t/α − (1 − e−αt)/α2 | 1/s2(α + s) |
| tn | n!/s1+n, n ≥ 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pandey, S.; Mastrangelo, C. Towards Transient Electronics through Heat Triggered Shattering of Off-the-Shelf Electronic Chips. Micromachines 2022, 13, 242. https://doi.org/10.3390/mi13020242
Pandey S, Mastrangelo C. Towards Transient Electronics through Heat Triggered Shattering of Off-the-Shelf Electronic Chips. Micromachines. 2022; 13(2):242. https://doi.org/10.3390/mi13020242
Chicago/Turabian StylePandey, Shashank, and Carlos Mastrangelo. 2022. "Towards Transient Electronics through Heat Triggered Shattering of Off-the-Shelf Electronic Chips" Micromachines 13, no. 2: 242. https://doi.org/10.3390/mi13020242
APA StylePandey, S., & Mastrangelo, C. (2022). Towards Transient Electronics through Heat Triggered Shattering of Off-the-Shelf Electronic Chips. Micromachines, 13(2), 242. https://doi.org/10.3390/mi13020242





