Thermal Poling of Optical Fibers: A Numerical History
Abstract
1. Introduction
2. Early Evidence of Second-Order Non-Linearities in Silica Fibers and Thermal Poling
2.1. First Theoretical Explanation of Thermal Poling: Single-Carrier Model
2.2. Multiple-Carrier Model for Space-Charge Region Formation
3. From Poling of Bulk Glasses to Silica Optical Fibers
3.1. From Conventional Poling to Cathode-Less Poling
3.2. Induction Poling
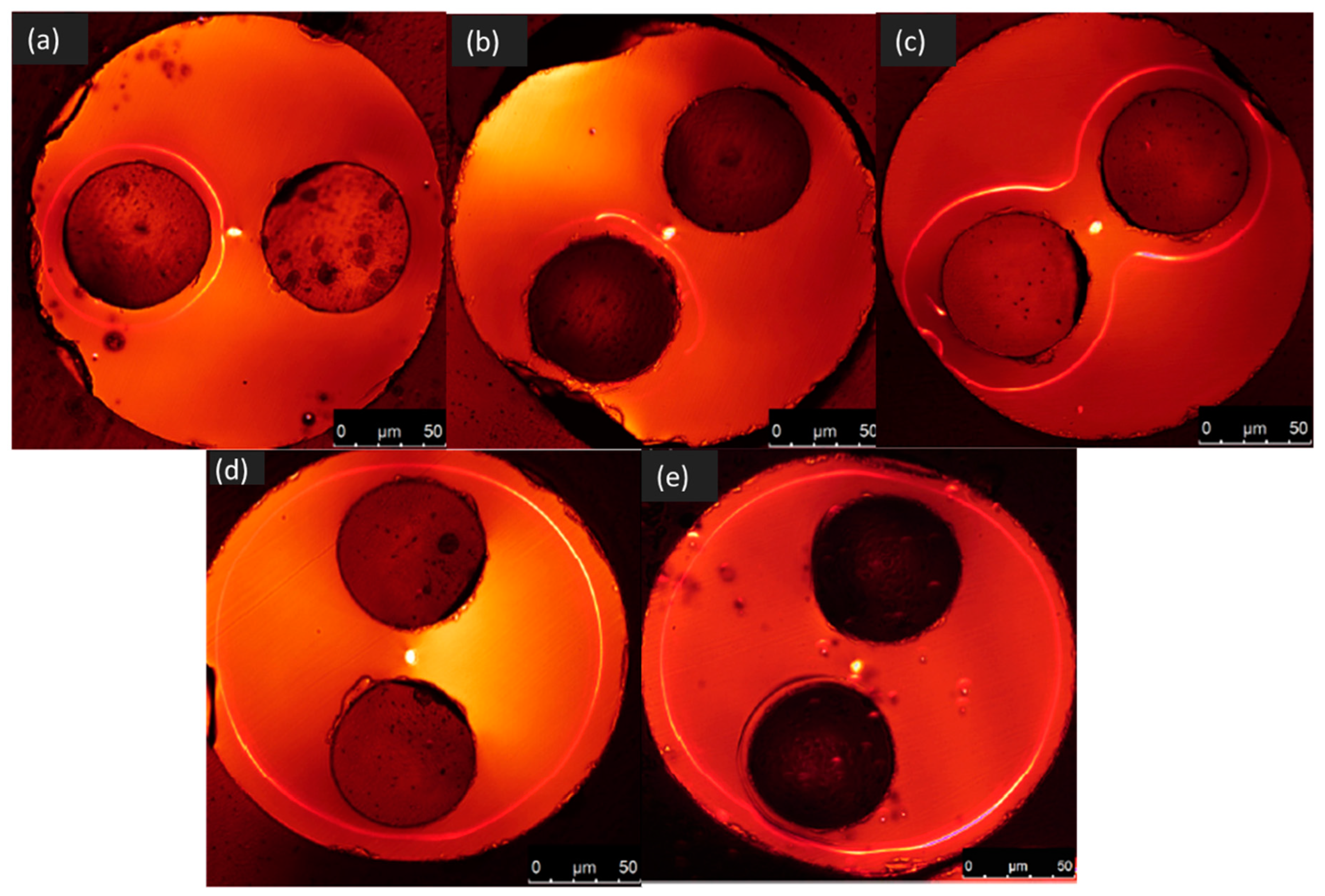
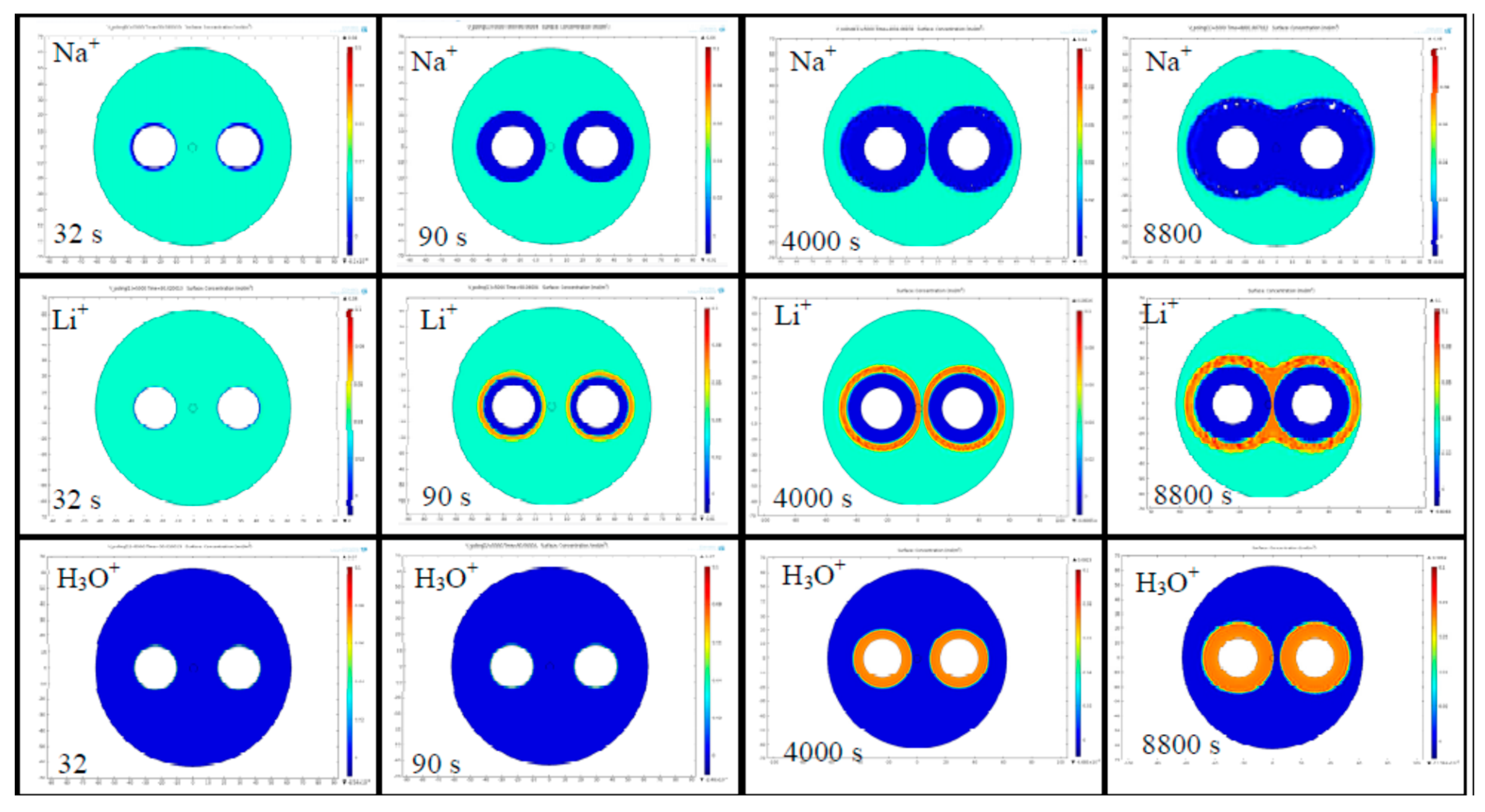
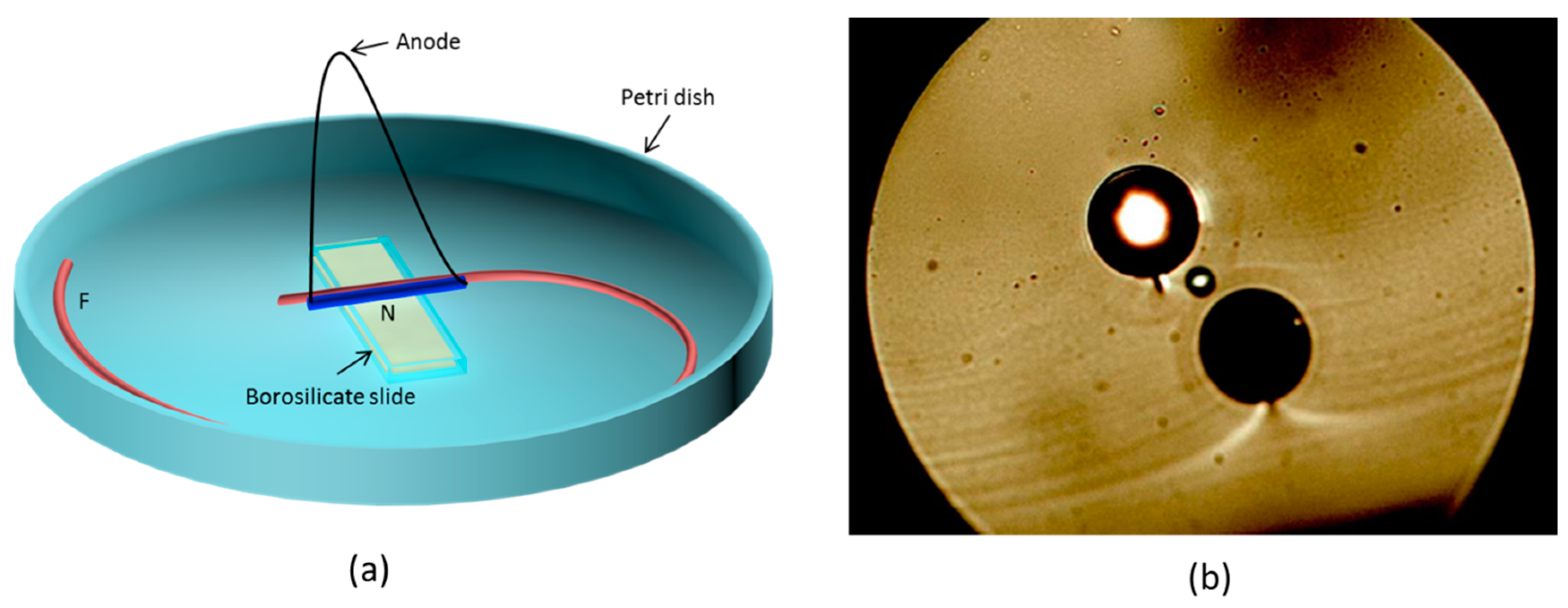
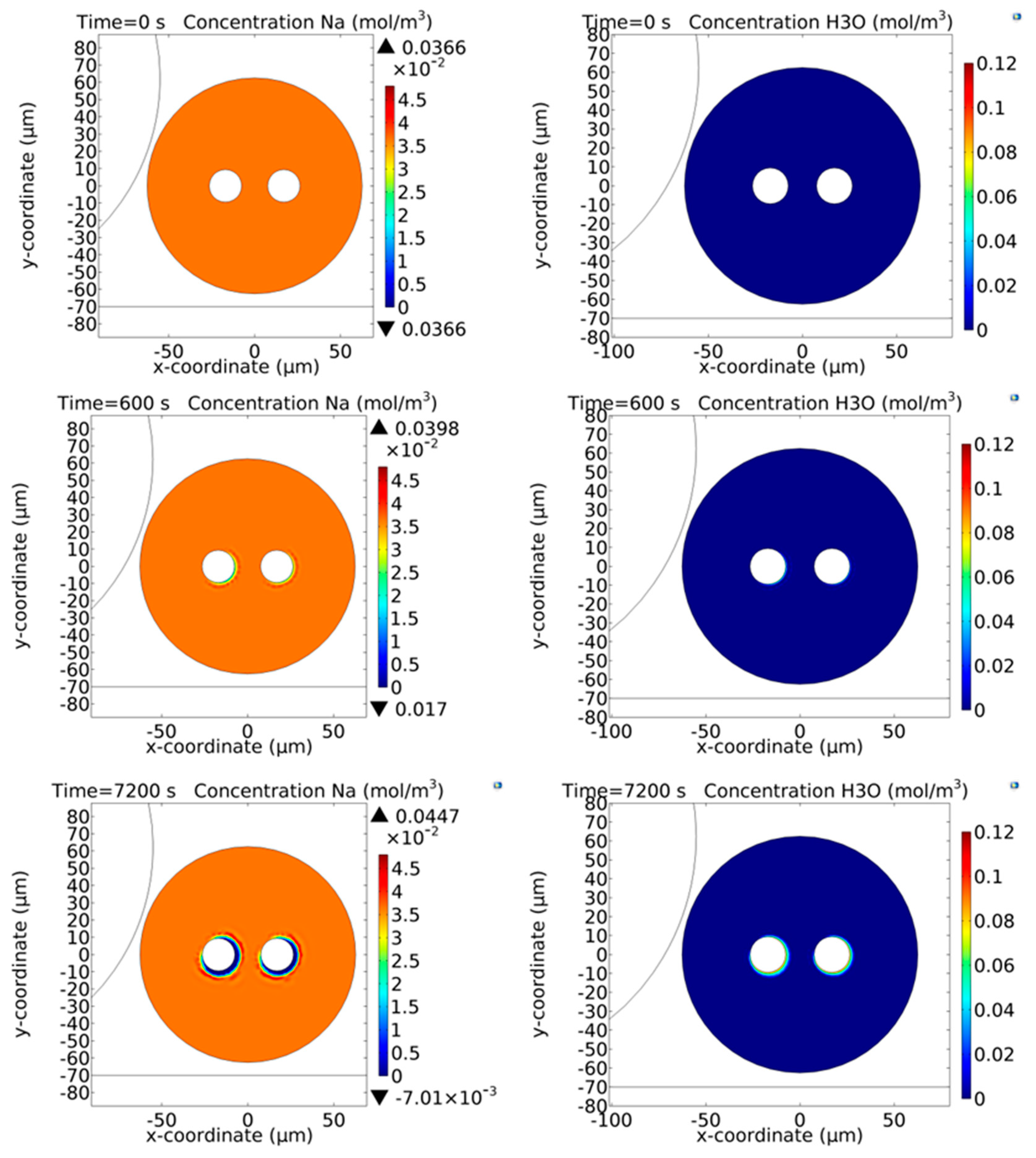
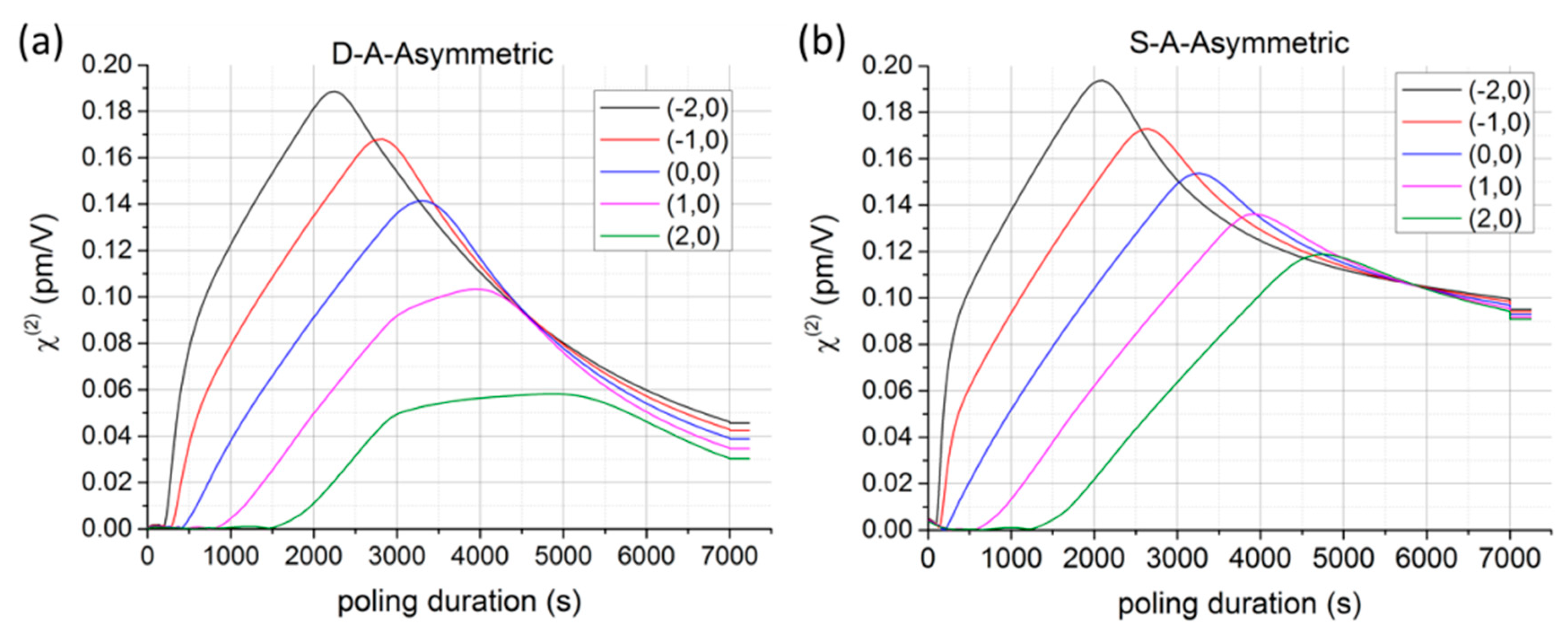
3.3. Single Anode Poling
4. Conclusions
Funding
Conflicts of Interest
References
- Baird, J.L. An Improved Method of and Means for Producing Optical Images. British Patent No. 285 738, 1928. [Google Scholar]
- Hansell, C.W. Picture Transmission, 1751584. 1930. Available online: https://patentimages.storage.googleapis.com/07/66/87/78111772a09892/US1751584.pdf (accessed on 20 November 2018).
- Kapron, F.P.; Keck, D.B.; Maurer, R.D. Radiation Losses in Glass Optical Waveguides. Appl. Phys. Lett. 1970, 423–425. [Google Scholar] [CrossRef]
- Agrawal, G.P. Fiber-Optic Communication Systems; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Ainslie, B.J. A Review of the Fabrication and Properties of Erbium-Doped Fibers for Optical Amplifiers. J. Lightwave Technol. 1991, 9, 220–227. [Google Scholar] [CrossRef]
- Chen, J.; Liu, B.; Zhang, H. Review of Fiber Bragg Grating Sensor Technology. Front. Optoelectron. China 2011, 4, 204–212. [Google Scholar] [CrossRef]
- Dudley, J.M.; Taylor, J.R. Supercontinuum Generation in Optical Fibers; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Butcher, P.N.; Cotter, D. The Elements of Nonlinear Optics, volume 9; Cambridge Studies in Modern Optics: Cambridge, UK, 1990. [Google Scholar]
- Myers, R.A.; Mukherjee, N.; Brueck, S.R. Large Second-Order Nonlinearity in Poled Fused Silica. Opt. Lett. 1991, 16, 1732–1734. [Google Scholar] [CrossRef]
- Kazansky, P.G.; Dong, L.; Russell, P.S. High Second-Order Nonlinearities in Poled Silicate Fibers. Opt. Lett. 1994, 19, 701–703. [Google Scholar] [CrossRef]
- Batchko, R.G.; Miller, G.D.; Alexandrovski, A.; Fejer, M.M.; Byer, R.L. Limitations of High-Power Visible Wavelength Periodically Poled Lithium Niobite Devices Due to Green-Induced Infrared Absorption and Thermal Lensing. In Proceedings of the Lasers and Electro-Optics, CTuD6G, San Francisco, CA, USA, 3–8 May 1998. [Google Scholar]
- Furukawa, Y.; Kitamura, K.; Alexandrovski, A.; Route, R.K.; Fejer, M.M.; Foulon, G. Green-Induced Infrared Absorption in MgO Doped LiNbO3. Appl. Phys. Lett. 2001, 78, 1970–1972. [Google Scholar] [CrossRef]
- Yamane, M.; Asahara, Y. Glasses for Photonics; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Fujii, Y.; Kawasaki, B.S.; Hill, K.O.; Johnson, D.C. Sum-Frequency Light Generation in Optical Fibers. Opt. Lett. 1980, 5, 48–50. [Google Scholar] [CrossRef]
- Sasaki, Y.; Ohmori, Y. Phasematched Sumfrequency Light Generation in Optical Fibers. Appl. Phys. Lett. 1981, 39, 466–468. [Google Scholar] [CrossRef]
- Ohmori, Y.; Sasaki, Y. Two-Wave Sum-Frequency Light Generation in Optical Fibers. IEEE Trans. Microw. Theory Tech. MTT 1982, 30, 604–608. [Google Scholar] [CrossRef]
- Gabriagues, J.M.; Fersing, L. Second and Third Harmonic Generation in Optical Fibers. In Conference on Lasers and Electro-Optics; Optical Society of America: Washington, DC, USA, 1984. [Google Scholar]
- Osterberg, U.; Margulis, W. Dye Laser Pumped by Nd:YAG Laser Pulses Frequency Doubled in a Glass Optical Fibers. Opt. Lett. 1986, 11, 516–518. [Google Scholar] [CrossRef]
- Osterberg, U.; Margulis, W. Experimental Studies on Efficient Frequency Doubling in Glass Optical Fibers. Opt. Lett. 1987, 12, 57–59. [Google Scholar] [CrossRef] [PubMed]
- Stolen, R.H.; Tom, H.W.K. Self-Organized Phase-Matched Harmonic Generation in Optical Fibers. Opt. Lett. 1987, 12, 585–587. [Google Scholar] [CrossRef] [PubMed]
- Bethune, D.S. Quadrupole Second-Harmonic Generation for a Focused Beam of Arbitrary Transverse Structure and Polarization. Opt. Lett. 1981, 6, 287–289. [Google Scholar] [CrossRef] [PubMed]
- Farries, M.C.; Russell, P.St.J.; Fermann, M.E.; Payne, D.N. Second-Harmonic Generation in an Optical Fiber by Self-Written χ(2) Grating. Electron. Lett. 1987, 23, 322–324. [Google Scholar] [CrossRef]
- Kashyap, R. Phase-Matched Periodic Electric-Field-Induced Second-Harmonic Generation in Optical Fibers. J. Opt. Soc. Am. B 1989, 6, 313–328. [Google Scholar] [CrossRef]
- Mukherjee, N.; Myers, R.A.; Brueck, S.R.J. Dynamics of Second-Harmonic Generation in Fused Silica. J. Opt. Soc. Am. B 1994, 11, 665–669. [Google Scholar] [CrossRef]
- Alley, T.G.; Brueck, S.R.J.; Myers, R.A. Space Charge Dynamics in Thermally Poled Fused Silica. J. Non-Cryst. Solids 1998, 242, 165–176. [Google Scholar] [CrossRef]
- Krieger, U.K.; Lanford, W.A. Field Assisted Transport of Na+ ions, Ca2+ ions and Electrons in Commercial Soda-Lime Glass I: Experimental. J. Non-Cryst. Solids 1988, 102, 50–61. [Google Scholar] [CrossRef]
- Kazansky, P.G.; Smith, A.R.; Russell, P.St.J.; Yang, G.M.; Sessler, G.M. Thermally Poled Silica Glass: Laser Induced Pressure Pulse Probe of Charge Distribution. Appl. Phys. Lett. 1996, 68, 269–271. [Google Scholar] [CrossRef]
- Pureur, D.; Liu, A.C.; Digonnet, M.J.; Kino, G.S. Absolute Measurement of the Second-Order Nonlinearity Profile in Poled Silica. Opt. Lett. 1998, 23, 588–590. [Google Scholar] [CrossRef]
- Kudlinski, A.; Quiquempois, Y.; Martinelli, G. Modeling of the chi(2) Susceptibility Time-Evolution in Thermally Poled Fused Silica. Opt. Express. 2005, 13, 8015–8024. [Google Scholar] [CrossRef] [PubMed]
- Kudlinski, A.; Martinelli, G.; Quiquempois, Y. Time Evolution of Second-Order Nonlinear Profiles Induced within Thermally Poled Silica Samples. Opt. Lett. 2005, 30, 1039–1041. [Google Scholar] [CrossRef] [PubMed]
- Faccio, D.; Pruneri, V.; Kazansky, P.G. Dynamics of the Second-Order Nonlinearity in Thermally Poled Silica Glass. Appl. Phys. Lett. 2001, 79, 2687–2689. [Google Scholar] [CrossRef][Green Version]
- Fujiwara, T.; Wong, D.; Fleming, S. Large Electrooptic Modulation in a Thermally-Poled Germanosilicate Fiber. IEEE Photonics Technol. Lett. 1995, 7, 1177–1179. [Google Scholar] [CrossRef]
- Myrén, N.; Olsson, H.; Norin, L.; Sjödin, N.; Helander, P.; Svennebrink, J.; Margulis, W. Wide Wedge-Shaped Depletion Region in Thermally Poled Fiber with Alloy Electrodes. Opt. Express. 2004, 12, 6093–6099. [Google Scholar] [CrossRef] [PubMed]
- Kazansky, P.G.; Pruneri, V. Electric-Field Poling of Quasi-Phase-Matched Optical Fibers. J. Opt. Soc. Am. B 1997, 14, 3170–3179. [Google Scholar] [CrossRef]
- Wong, D.; Xu, W.; Fleming, S.; Janos, M.; Lo, K.-M. Frozen-in Electrical Field in Thermally Poled Fibers. Opt. Fiber Technol. 1999, 5, 235–241. [Google Scholar] [CrossRef]
- Blazkiewicz, P.; Xu, W.; Wong, D.; Fleming, S.; Ryan, T. Modification of Thermal Poling Evolution Using Novel Twin-Hole Fibers. J. Lightwave Technol. 2001, 19, 1149–1154. [Google Scholar] [CrossRef]
- Margulis, W.; Tarasenko, O.; Myrén, N. Who Needs a Cathode? Creating a Second-Order Nonlinearity by Charging Glass Fiber with Two Anodes. Opt. Express 2009, 17, 15534–15540. [Google Scholar] [CrossRef]
- An, H.; Fleming, S. Investigating the Effectiveness of Thermally Poling Optical Fibers with Various Internal Electrode Configurations. Opt. Express 2012, 20, 7436–7444. [Google Scholar] [CrossRef]
- Camara, A.; Tarasenko, O.; Margulis, W. Study of Thermally Poled Fibers with a Two-Dimensional Model. Opt. Express 2014, 22, 17700–17715. [Google Scholar] [CrossRef] [PubMed]
- Alley, T.G.; Brueck, S.R.J. Visualization of the Nonlinear Optical Space-Charge Region of Bulk Thermally Poled Fused-Silica Glass. Opt. Lett. 1998, 23, 1170–1172. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Qian, L. Real-time χ(2) Evolution in Twin-Hole Fiber During Thermal Poling and Repoling. J. Opt. Soc. Am. B 2009, 26, 1412–1416. [Google Scholar] [CrossRef]
- De Lucia, F.; Huang, D.; Corbari, C.; Healy, N.; Sazio, P.J.A. Optical Fiber Poling by Induction. Opt. Lett. 2014, 39, 6513–6516. [Google Scholar] [CrossRef] [PubMed]
- De Lucia, F.; Sazio, P.J.A. Optimized Optical Fiber Poling Configurations. Opt. Mater. X 2019, 1, 100016. [Google Scholar] [CrossRef]
- De Lucia, F.; Huang, D.; Corbari, C.; Healy, N.; Sazio, P.J.A. Optical Fiber Poling by Induction: Analysis by 2D Numerical Modeling. Opt. Lett. 2016, 41, 1700–1703. [Google Scholar] [CrossRef]
- De Lucia, F.; Bannerman, R.; Englebert, N.; Nunez Velazquez, M.M.A.; Leo, F.; Gates, J.; Gorza, S.-P.; Sahu, J.; Sazio, P.J.A. Single is Better than Double: Theoretical and Experimental Comparison between Two Thermal Poling Configurations of Optical Fibers. Opt. Express 2019, 27, 27761–27776. [Google Scholar] [CrossRef]
- De Lucia, F.; Keefer, D.W.; Corbari, C.; Sazio, P.J.A. Thermal Poling of Silica Optical Fibers Using Liquid Electrodes. Opt. Lett. 2017, 42, 69–72. [Google Scholar] [CrossRef]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
De Lucia, F.; Sazio, P.J.A. Thermal Poling of Optical Fibers: A Numerical History. Micromachines 2020, 11, 139. https://doi.org/10.3390/mi11020139
De Lucia F, Sazio PJA. Thermal Poling of Optical Fibers: A Numerical History. Micromachines. 2020; 11(2):139. https://doi.org/10.3390/mi11020139
Chicago/Turabian StyleDe Lucia, Francesco, and Pier J. A. Sazio. 2020. "Thermal Poling of Optical Fibers: A Numerical History" Micromachines 11, no. 2: 139. https://doi.org/10.3390/mi11020139
APA StyleDe Lucia, F., & Sazio, P. J. A. (2020). Thermal Poling of Optical Fibers: A Numerical History. Micromachines, 11(2), 139. https://doi.org/10.3390/mi11020139




