Validation of Atmospheric Wind Fields from MIGHTI/ICON: A Comprehensively Comparative Analysis with Meteor Radars, FPI and TIMED/TIDI
Abstract
1. Introduction
2. Materials and Methods
2.1. MIGHTI
2.2. Meteor Radar
2.3. FPI
2.4. TIDI
2.5. Methodologies
3. Comparison Results
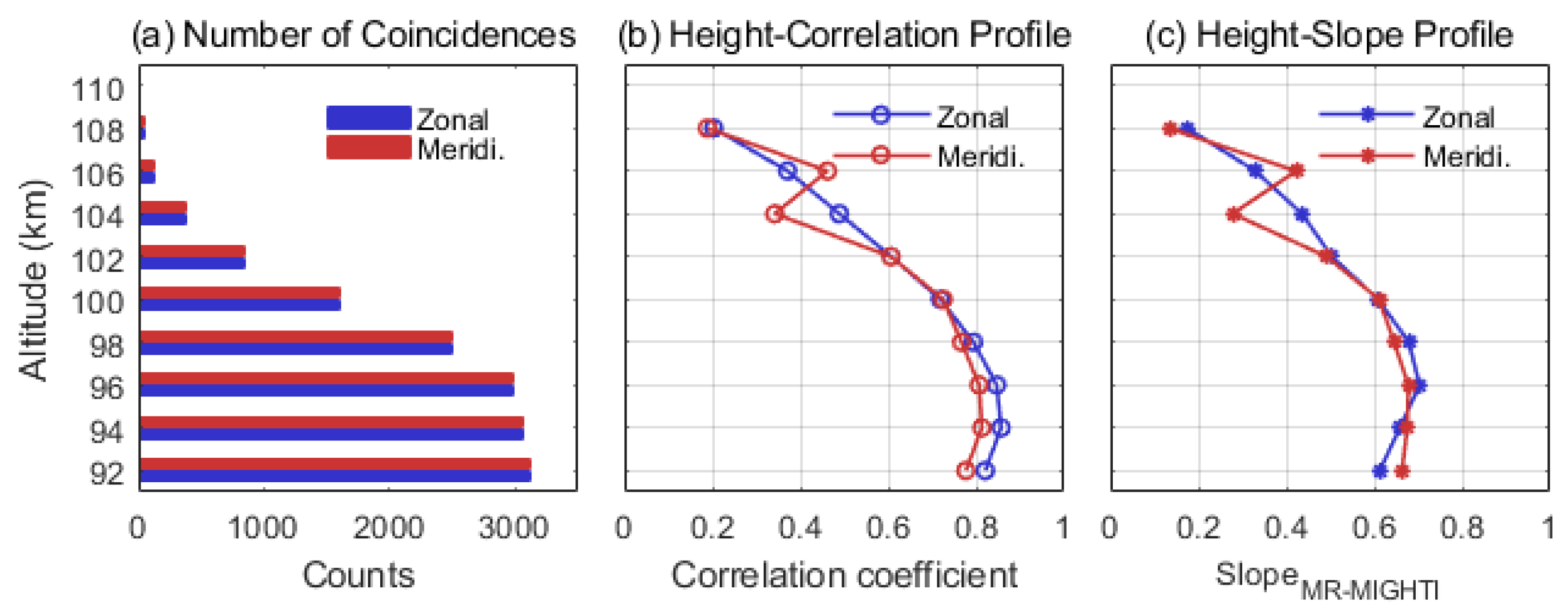
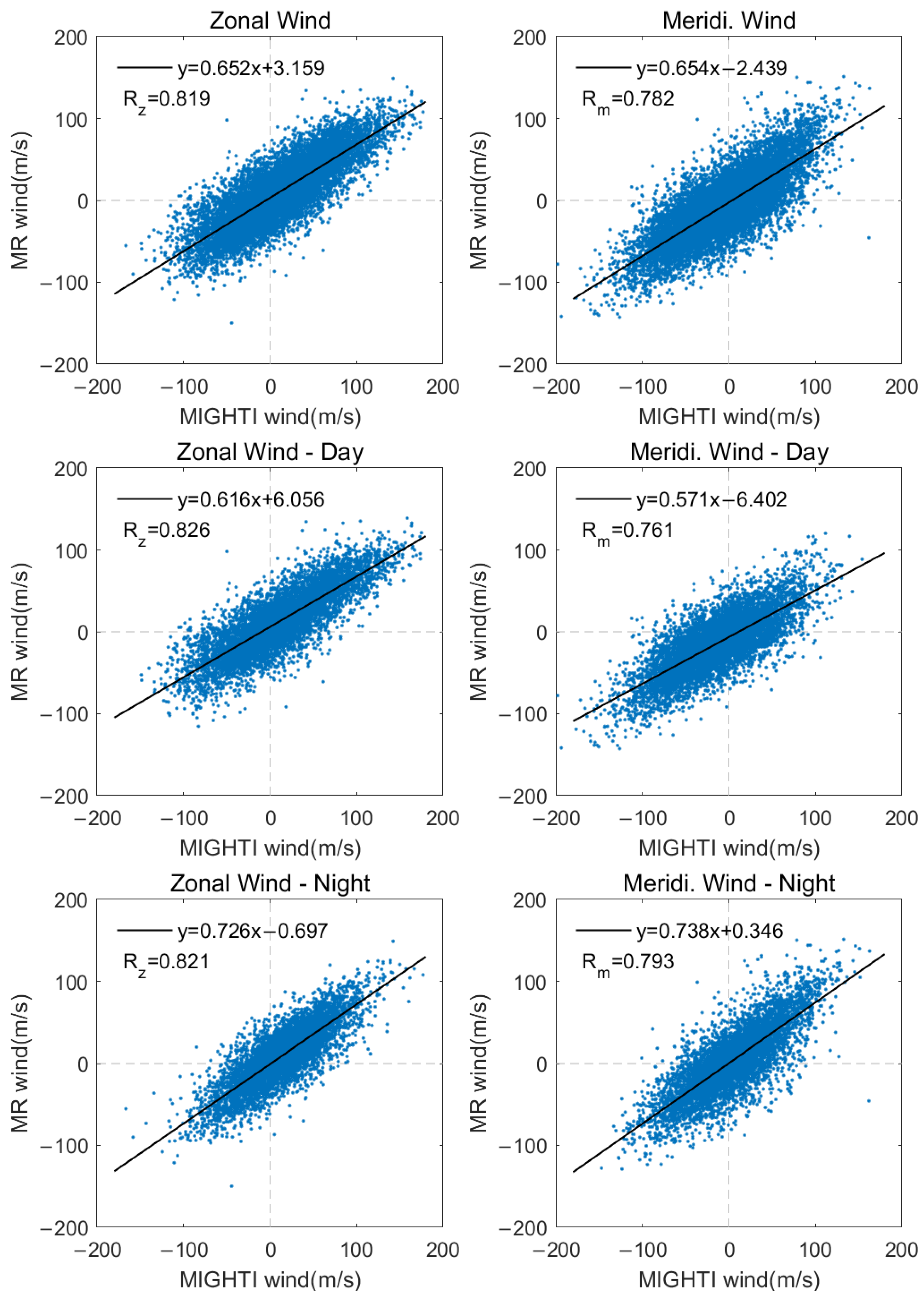
3.1. MIGHTI vs. MR
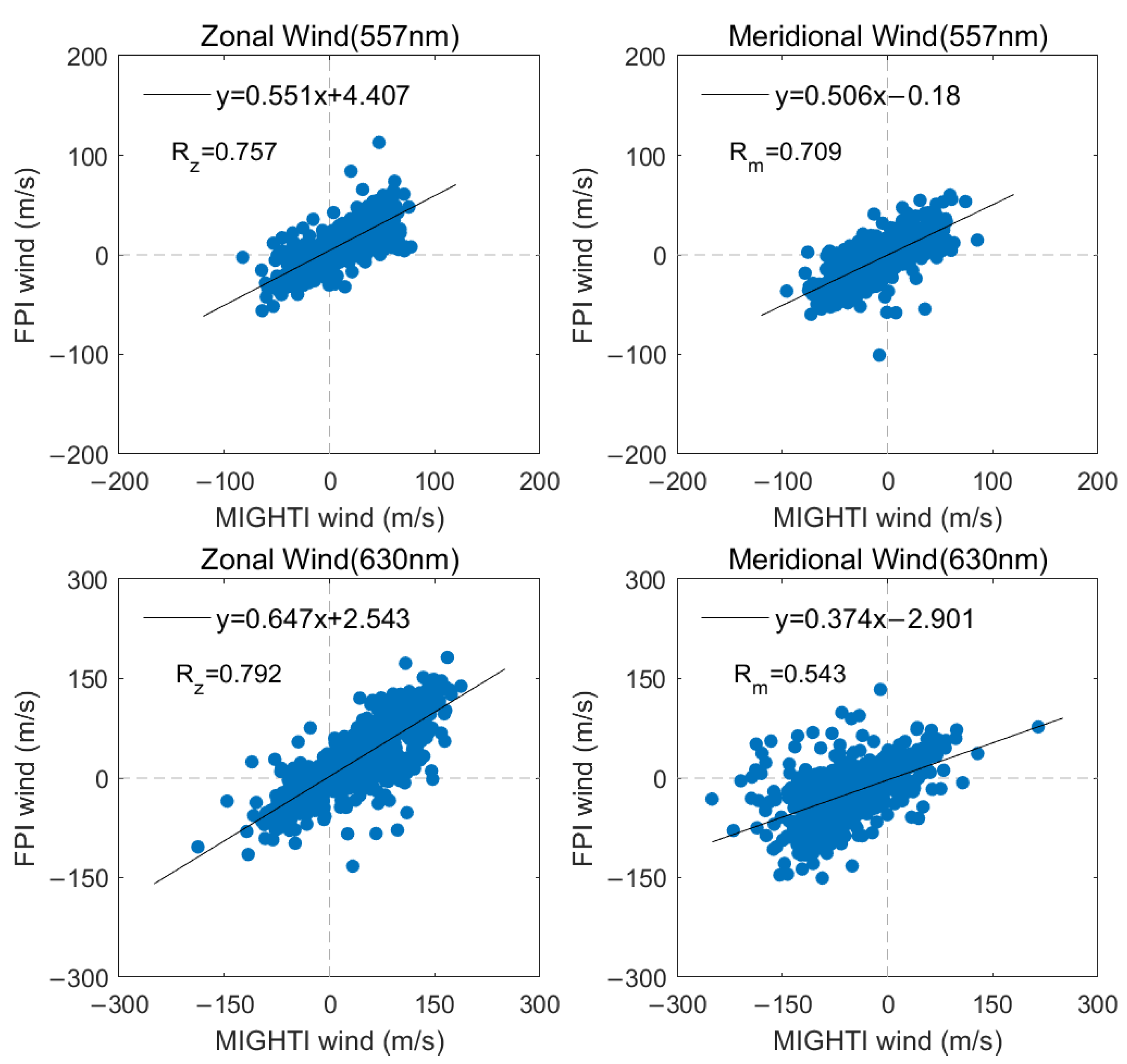
3.2. MIGHTI vs. FPI
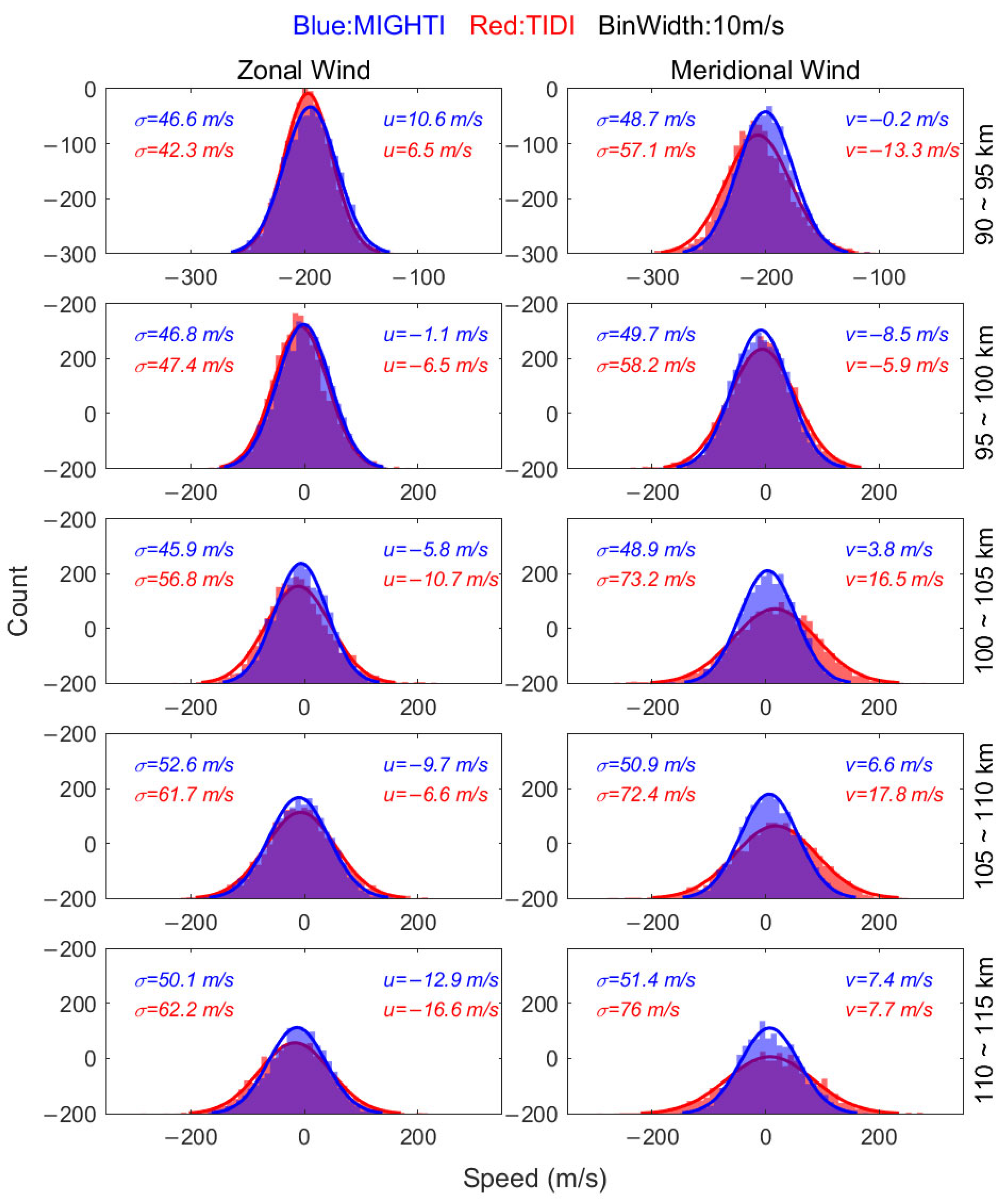
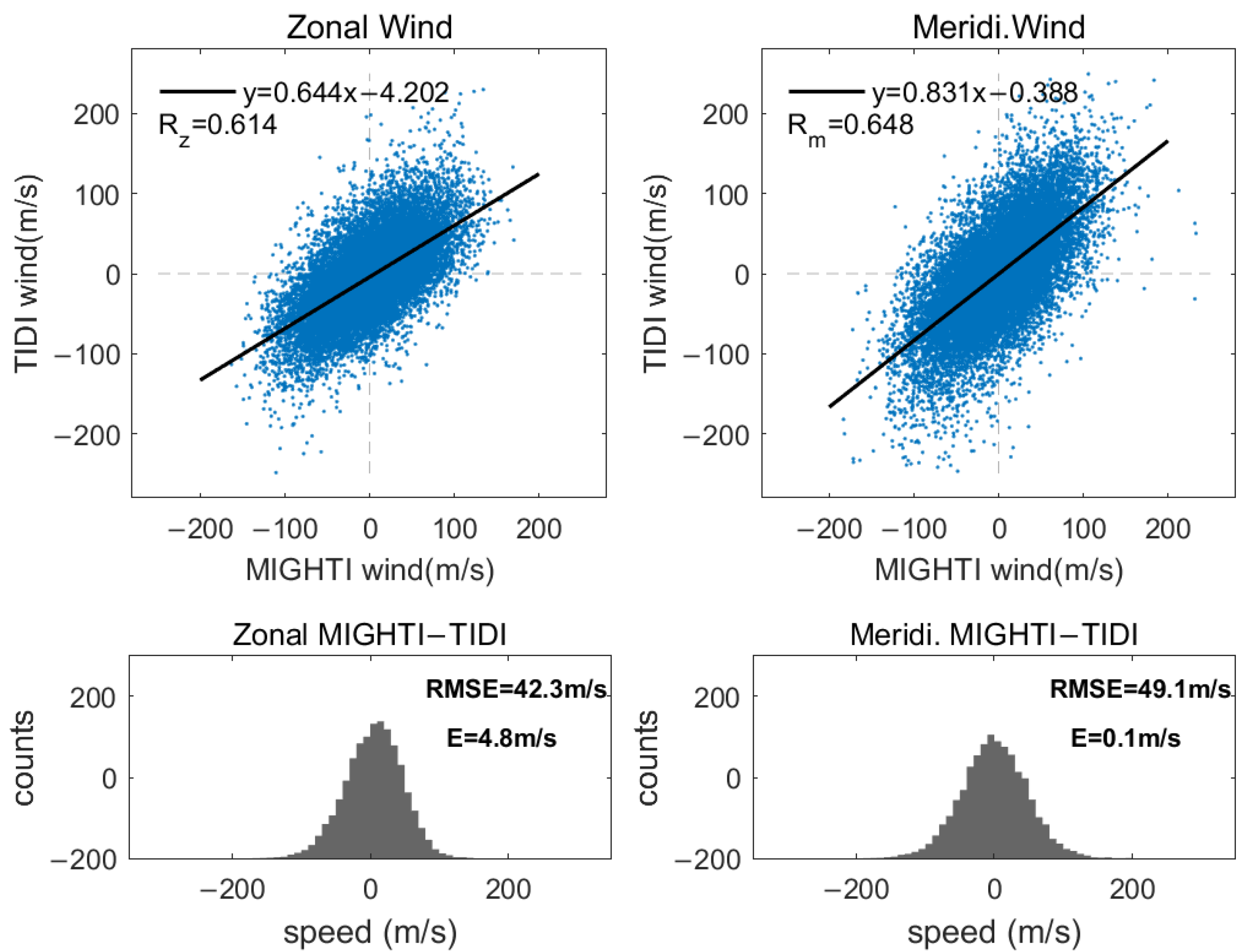
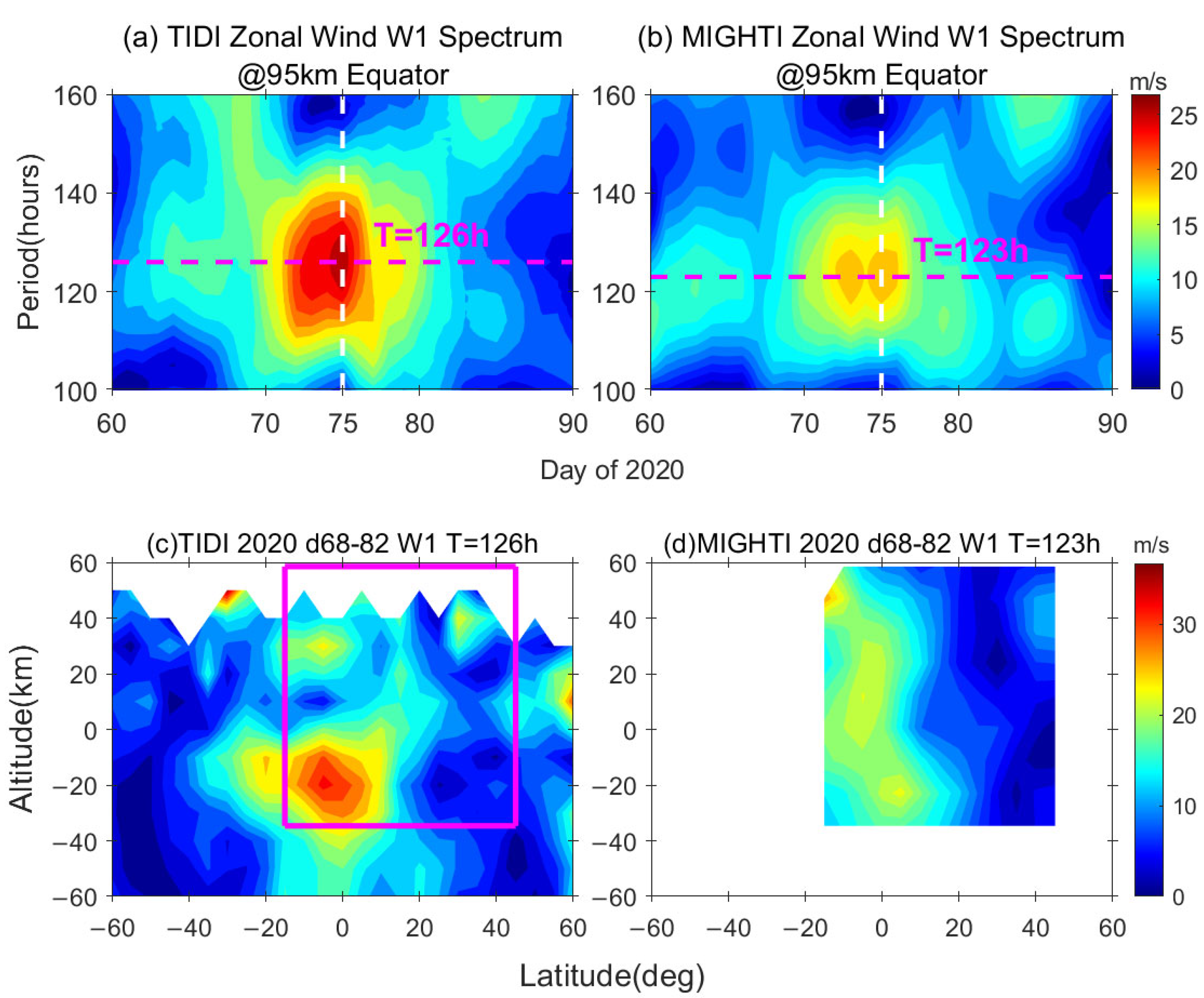
3.3. MIGHTI vs. TIDI
3.3.1. Neutral Wind
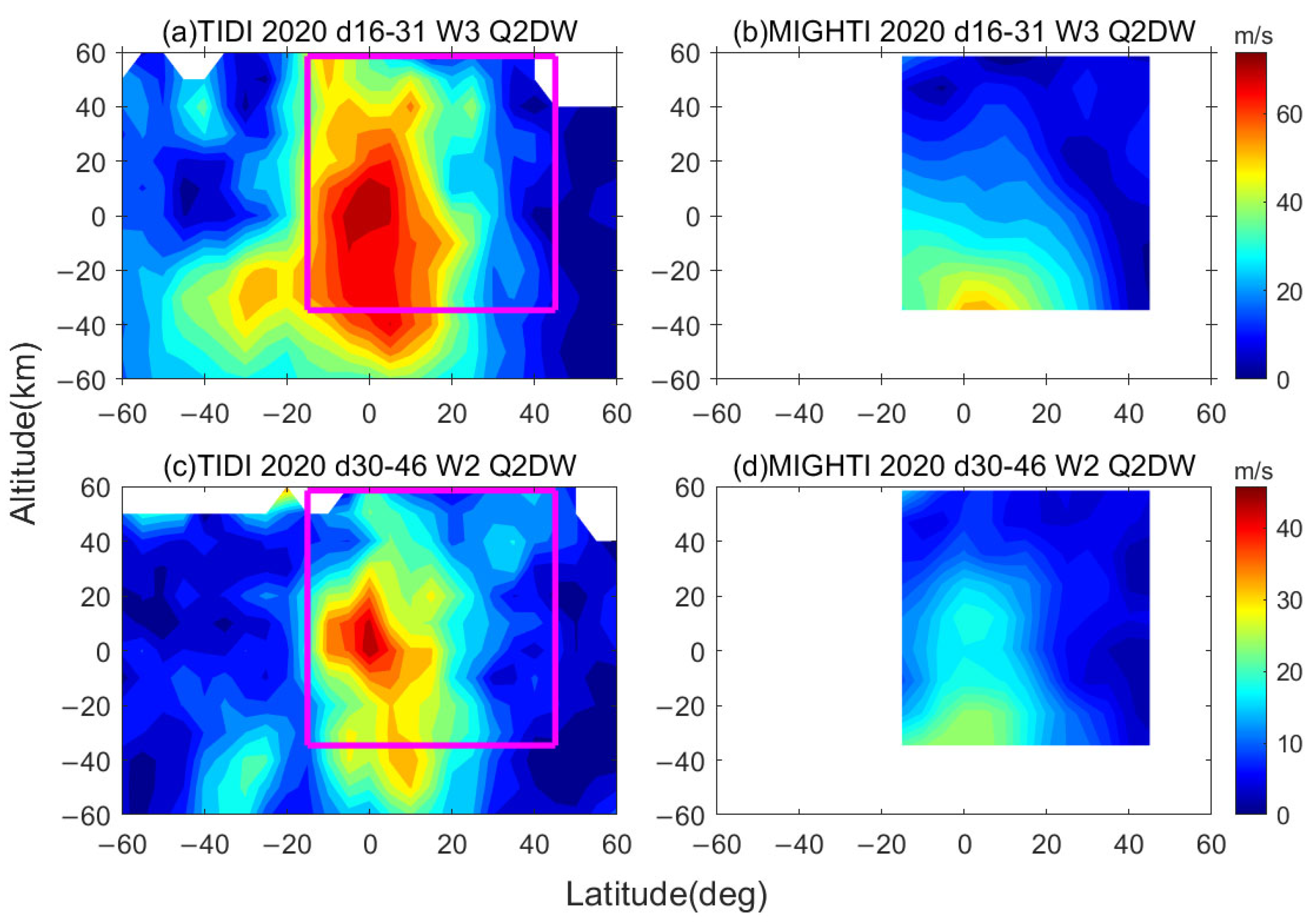
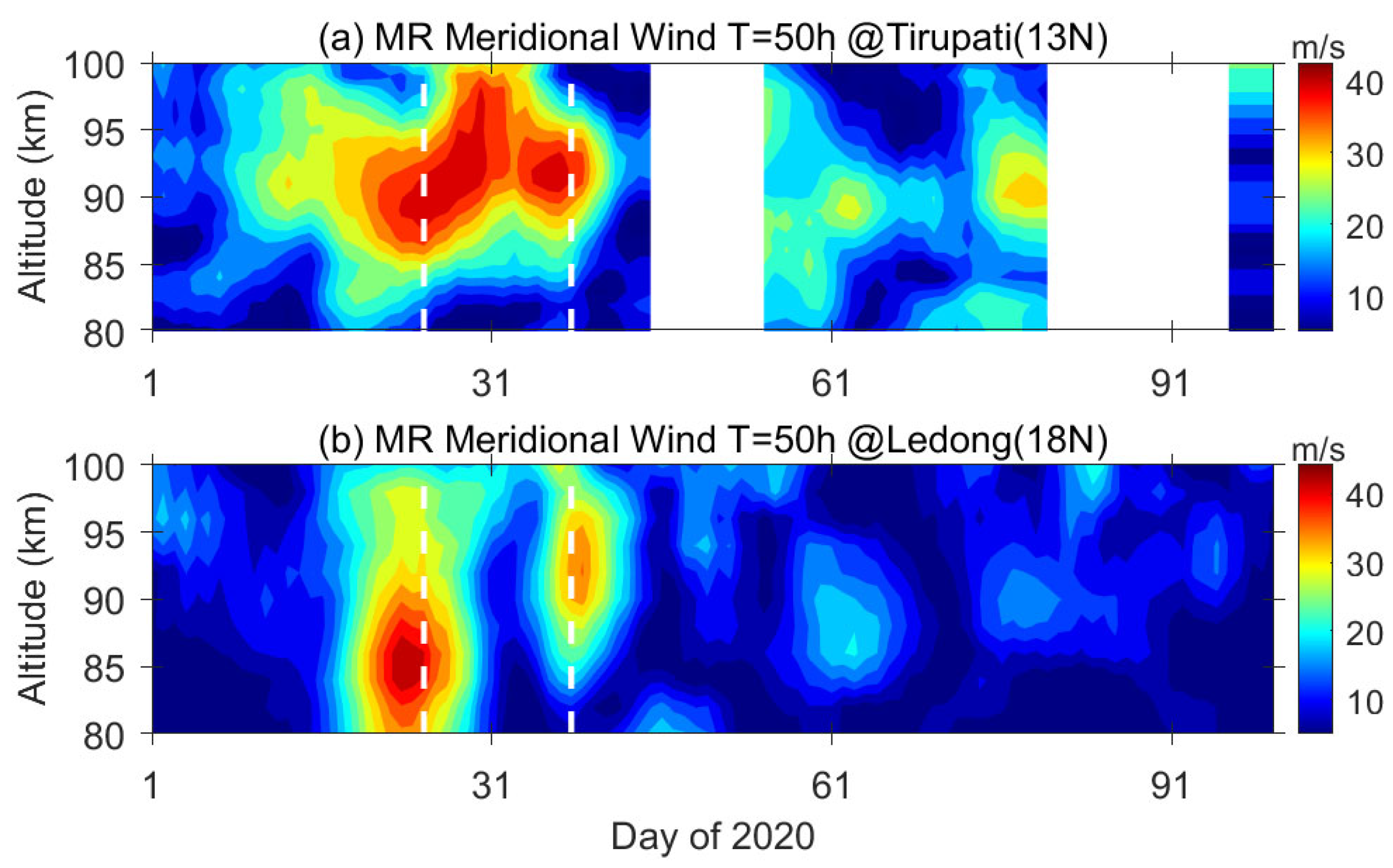
3.3.2. Planetary Wave
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- He, Y.; Zhu, X.; Sheng, Z.; He, M. Resonant Waves Play an Important Role in the Increasing Heat Waves in Northern Hemisphere Mid-Latitudes Under Global Warming. Geophys. Res. Lett. 2023, 50, e2023GL104839. [Google Scholar] [CrossRef]
- He, Y.; Zhu, X.; Sheng, Z.; He, M. Identification of Stratospheric Disturbance Information in China Based on the Round-Trip Intelligent Sounding System. Atmos. Chem. Phys. 2024, 24, 3839–3856. [Google Scholar] [CrossRef]
- Andrews, D.G.; Holton, J.R.; Leovy, C.B. Middle Atmosphere Dynamics; Academic Press: Cambridge, MA, USA, 1987. [Google Scholar]
- Gu, S. Planetary Waves and Their Roles in Atmospheric Coupling. Ph.D. Thesis, University of Science and Technology of China, Hefei, China, 2015. [Google Scholar]
- Salby, M.L. Rossby Normal Modes in Nonuniform Background Configurations. Part II. Equinox and Solstice Conditions. J. Atmos. Sci. 1981, 38, 1827–1840. [Google Scholar] [CrossRef]
- Tang, Q.; Zhou, Y.; Du, Z.; Zhou, C.; Qiao, J.; Liu, Y.; Chen, G. A Comparison of Meteor Radar Observation over China Region with Horizontal Wind Model (HWM14). Atmosphere 2021, 12, 98. [Google Scholar] [CrossRef]
- Wang, Y.; Li, G.; Ning, B.; Yang, S.; Sun, W.; Yu, Y. All-Sky Interferometric Meteor Radar Observations of Zonal Structure and Drifts of Low-Latitude Ionospheric E Region Irregularities. Earth Space Sci. 2019, 6, 2653–2662. [Google Scholar] [CrossRef]
- Zhou, X.; Yue, X.; Yu, Y.; Hu, L. Day-To-Day Variability of the MLT DE3 Using Joint Analysis on Observations From TIDI-TIMED and a Meteor Radar Meridian Chain. J. Geophys. Res. Atmos. 2022, 127, e2021JD035794. [Google Scholar] [CrossRef]
- Wu, Q.; Gablehouse, R.D.; Solomon, S.C.; Killeen, T.L.; She, C.-Y. A New Fabry-Perot Interferometer for Upper Atmosphere Research. In Proceedings of the Instruments, Science, and Methods for Geospace and Planetary Remote Sensing, Honolulu, HI, USA, 30 December 2004; Volume 5660, pp. 218–227. [Google Scholar]
- Wei, Y.; Gu, S.-Y.; Yang, Z.; Huang, C.; Li, N.; Hu, G.; Dou, X. Inversion of Wind and Temperature from Low SNR FPI Interferograms. Remote Sens. 2023, 15, 1934. [Google Scholar] [CrossRef]
- Gu, S.; Hou, X.; Li, N.; Yi, W.; Ding, Z.; Chen, J.; Hu, G.; Dou, X. First Comparative Analysis of the Simultaneous Horizontal Wind Observations by Collocated Meteor Radar and FPI at Low Latitude through 892.0-Nm Airglow Emission. Remote Sens. 2021, 13, 4337. [Google Scholar] [CrossRef]
- Sun, R.; Gu, S.-Y.; Dou, X.; Wei, Y.; Qin, Y.; Yang, Z. Decadal Quasi-2-Day Wave Observations in the Equatorial Mesopause Region by a Meteor Radar over Kototabang (0.2°S, 100.3°E) and TIMED/TIDI and Comparison with Quasi-2-Day Wave Observations at Mid-Latitudes. Remote Sens. 2023, 15, 1122. [Google Scholar] [CrossRef]
- Niciejewski, R.; Wu, Q.; Skinner, W.; Gell, D.; Cooper, M.; Marshall, A.; Killeen, T.; Solomon, S.; Ortland, D. TIMED Doppler Interferometer on the Thermosphere Ionosphere Mesosphere Energetics and Dynamics Satellite: Data Product Overview. J. Geophys. Res. 2006, 111, 2005JA011513. [Google Scholar] [CrossRef]
- Englert, C.R.; Harlander, J.M.; Brown, C.M.; Marr, K.D.; Miller, I.J.; Stump, J.E.; Hancock, J.; Peterson, J.Q.; Kumler, J.; Morrow, W.H.; et al. Michelson Interferometer for Global High-Resolution Thermospheric Imaging (MIGHTI): Instrument Design and Calibration. Space Sci. Rev. 2017, 212, 553–584. [Google Scholar] [CrossRef]
- Immel, T.J.; England, S.L.; Mende, S.B.; Heelis, R.A.; Englert, C.R.; Edelstein, J.; Frey, H.U.; Korpela, E.J.; Taylor, E.R.; Craig, W.W.; et al. The Ionospheric Connection Explorer Mission: Mission Goals and Design. Space Sci. Rev. 2017, 214, 13. [Google Scholar] [CrossRef] [PubMed]
- Englert, C.R.; Harlander, J.M.; Marr, K.D.; Harding, B.J.; Makela, J.J.; Fae, T.; Brown, C.M.; Ratnam, M.V.; Rao, S.V.B.; Immel, T.J. Michelson Interferometer for Global High-Resolution Thermospheric Imaging (MIGHTI) On-Orbit Wind Observations: Data Analysis and Instrument Performance. Space Sci. Rev. 2023, 219, 27. [Google Scholar] [CrossRef] [PubMed]
- Makela, J.J.; Baughman, M.; Navarro, L.A.; Harding, B.J.; Englert, C.R.; Harlander, J.M.; Marr, K.D.; Benkhaldoun, Z.; Kaab, M.; Immel, T.J. Validation of ICON-MIGHTI Thermospheric Wind Observations: 1. Nighttime Red-Line Ground-Based Fabry-Perot Interferometers. JGR Space Phys. 2021, 126, e2020JA028726. [Google Scholar] [CrossRef] [PubMed]
- Harding, B.J.; Chau, J.L.; He, M.; Englert, C.R.; Harlander, J.M.; Marr, K.D.; Makela, J.J.; Clahsen, M.; Li, G.; Ratnam, M.V.; et al. Validation of ICON-MIGHTI Thermospheric Wind Observations: 2. Green-Line Comparisons to Specular Meteor Radars. JGR Space Phys. 2021, 126, e2020JA028947. [Google Scholar] [CrossRef] [PubMed]
- Dhadly, M.S.; Englert, C.R.; Drob, D.P.; Emmert, J.T.; Niciejewski, R.; Zawdie, K.A. Comparison of ICON/MIGHTI and TIMED/TIDI Neutral Wind Measurements in the Lower Thermosphere. JGR Space Phys. 2021, 126, e2021JA029904. [Google Scholar] [CrossRef] [PubMed]
- Zhou, C.; Liu, Y.; Du, Z.; Fan, Z.; Sun, H.; Zhou, C. Validation of MIGHTI/ICON Atmospheric Wind Observations over China Region Based on Meteor Radar and Horizontal Wind Model (HWM14). Atmosphere 2022, 13, 1078. [Google Scholar] [CrossRef]
- He, M.; Chau, J.L.; Forbes, J.M.; Zhang, X.; Englert, C.R.; Harding, B.J.; Immel, T.J.; Lima, L.M.; Bhaskar Rao, S.V.; Ratnam, M.V.; et al. Quasi-2-Day Wave in Low-Latitude Atmospheric Winds as Viewed from the Ground and Space During January–March, 2020. Geophys. Res. Lett. 2021, 48, e2021GL093466. [Google Scholar] [CrossRef]
- Yamazaki, Y.; Harding, B.J.; Qiu, L.; Stolle, C.; Siddiqui, T.A.; Miyoshi, Y.; Englert, C.R.; England, S.L. Monthly Climatologies of Zonal-Mean and Tidal Winds in the Thermosphere as Observed by ICON/MIGHTI During April 2020–March 2022. Earth Space Sci. 2023, 10, e2023EA002962. [Google Scholar] [CrossRef]
- Holdsworth, D.A.; Reid, I.M.; Cervera, M.A. Buckland Park All-Sky Interferometric Meteor Radar. Radio Sci. 2004, 39, RS5009. [Google Scholar] [CrossRef]
- Rao, S.V.B.; Eswaraiah, S.; Venkat Ratnam, M.; Kosalendra, E.; Kishore Kumar, K.; Sathish Kumar, S.; Patil, P.T.; Gurubaran, S. Advanced Meteor Radar Installed at Tirupati: System Details and Comparison with Different Radars. J. Geophys. Res. Atmos. 2014, 119, 11-893–11-904. [Google Scholar] [CrossRef]
- Yu, Y.; Wan, W.; Ning, B.; Liu, L.; Wang, Z.; Hu, L.; Ren, Z. Tidal Wind Mapping from Observations of a Meteor Radar Chain in December 2011. J. Geophys. Res. Space Phys. 2013, 118, 2321–2332. [Google Scholar] [CrossRef]
- Kristoffersen, S.K.; Ward, W.E.; Meek, C.E. Wind Comparisons between Meteor Radar and Doppler Shifts in Airglow Emissions Using Field-Widened Michelson Interferometers. Atmos. Meas. Tech. 2024, 17, 3995–4014. [Google Scholar] [CrossRef]
- Hasebe, F.; Tsuda, T.; Nakamura, T.; Burrage, M.D. Validation of HRDI MLT Winds with Meteor Radars. Ann. Geophys. 1997, 15, 1142–1157. [Google Scholar] [CrossRef][Green Version]
- Yuan, W.; Xu, J.; Ma, R.; Wu, Q.; Jiang, G.; Gao, H.; Liu, X.; Chen, S. First Observation of Mesospheric and Thermospheric Winds by a Fabry-Perot Interferometer in China. Chin. Sci. Bull. 2010, 55, 4046–4051. [Google Scholar] [CrossRef]
- Wei, Y.; Gu, S.-Y.; Li, N.; Qin, Y.; Sun, R.; Wang, D.; Hu, G.; Le, H.; Yuan, W. Passive Optical Observation of Mesosphere and Thermosphere Wind Over Three Stations in China. J. Geophys. Res. Space Phys. 2024, 129, e2023JA032214. [Google Scholar] [CrossRef]
- Killeen, T.L.; Wu, Q.; Solomon, S.C.; Ortland, D.A.; Skinner, W.R.; Niciejewski, R.J.; Gell, D.A. TIMED Doppler Interferometer: Overview and Recent Results. J. Geophys. Res. Space Phys. 2006, 111, A10S01. [Google Scholar] [CrossRef]
- Wu, Q.; Ortland, D.A.; Killeen, T.L.; Roble, R.G.; Hagan, M.E.; Liu, H.-L.; Solomon, S.C.; Xu, J.; Skinner, W.R.; Niciejewski, R.J. Global Distribution and Interannual Variations of Mesospheric and Lower Thermospheric Neutral Wind Diurnal Tide: 1. Migrating Tide. J. Geophys. Res. 2008, 113, 2007JA012542. [Google Scholar] [CrossRef]
- Wu, D.L.; Hays, P.B.; Skinner, W.R. A Least Squares Method for Spectral Analysis of Space-Time Series. J. Atmos. Sci. 1995, 52, 3501–3511. [Google Scholar] [CrossRef]
- Chen, Z.; Liu, Z.; Feng, J.; Hu, L.; Yan, C.; Yuan, W.; Wu, X.; Zhen, B.; Zhou, X. Meteor Radar Prototype Testing and Data Quality Comparison Analysis for Phase II of Chinese Meridian Project. Rev. Geophys. Planet. Phys. 2024, 55, 120–130. [Google Scholar] [CrossRef]
- Yu, T.; Xia, C.; Zuo, X.; Huang, C.; Mao, T.; Liu, L.; Liu, Z. A Comparison of Mesospheric and Low-Thermospheric Winds Measured by Fabry-Perot Interferometer and Meteor Radar over Central China. J. Geophys. Res. Space Phys. 2016, 121, 10-037–10-051. [Google Scholar] [CrossRef]
- Gault, W.A.; Thuillier, G.; Shepherd, G.G.; Zhang, S.P.; Wiens, R.H.; Ward, W.E.; Tai, C.; Solheim, B.H.; Rochon, Y.J.; McLandress, C.; et al. Validation of O(1S) Wind Measurements by WINDII: The WIND Imaging Interferometer on UARS. J. Geophys. Res. Atmos. 1996, 101, 10405–10430. [Google Scholar] [CrossRef]
- Burrage, M.D.; Skinner, W.R.; Gell, D.A.; Hays, P.B.; Marshall, A.R.; Ortland, D.A.; Manson, A.H.; Franke, S.J.; Fritts, D.C.; Hoffman, P.; et al. Validation of Mesosphere and Lower Thermosphere Winds from the High Resolution Doppler Imager on UARS. J. Geophys. Res. 1996, 101, 10365–10392. [Google Scholar] [CrossRef]
- Forbes, J.M.; Portnyagin, Y.I.; Skinner, W.; Vincent, R.A.; Solovjova, T.; Merzlyakov, E.; Nakamura, T.; Palo, S. Climatological Lower Thermosphere Winds as Seen by Ground-Based and Space-Based Instruments. Ann. Geophys. 2004, 22, 1931–1945. [Google Scholar] [CrossRef]
- Wu, Q.; Killeen, T.L.; Ortland, D.A.; Solomon, S.C.; Gablehouse, R.D.; Johnson, R.M.; Skinner, W.R.; Niciejewski, R.J.; Franke, S.J. TIMED Doppler Interferometer (TIDI) Observations of Migrating Diurnal and Semidiurnal Tides. J. Atmos. Sol.-Terr. Phys. 2006, 68, 408–417. [Google Scholar] [CrossRef]
- Xiao, Y.; Feng, Y.; Wen, Z.; Fu, D. Doppler Asymmetric Spatial Heterodyne Interferometry for Wind Measurement in Middle and Upper Atmosphere (Invited). Acta Photonica Sin. 2022, 51, 0851516. [Google Scholar] [CrossRef]
- Tang, L.; Gu, S.-Y.; Sun, R.; Dou, X. Multi-Year Behavioral Observations of Quasi-2-Day Wave Activity in High-Latitude Mohe (52.5°N, 122.3°E) and Middle-Latitude Wuhan (30.5°N, 114.6°E) Using Meteor Radars. Remote Sens. 2024, 16, 311. [Google Scholar] [CrossRef]
- Gu, S.; Li, T.; Dou, X.; Wu, Q.; Mlynczak, M.G.; Russell, J.M. Observations of Quasi-Two-Day Wave by TIMED/SABER and TIMED/TIDI. JGR Atmos. 2013, 118, 1624–1639. [Google Scholar] [CrossRef]
- Gu, S.-Y.; Wei, Y.; Sha, X.; Tang, L.; Li, N. A Case Study of the Wavenumber Transition between Westward Quasi-2 Day Wave s = 3 and s = 4 Modes in the Mesosphere. Atmosphere 2023, 14, 442. [Google Scholar] [CrossRef]
- Harris, T.J.; Vincent, R.A. The Quasi-two-day Wave Observed in the Equatorial Middle Atmosphere. J. Geophys. Res. 1993, 98, 10481–10490. [Google Scholar] [CrossRef]
- Plumb, R.A. Baroclinic Instability of the Summer Mesosphere: A Mechanism for the Quasi-Two-Day Wave? J. Atmos. Sci. 1983, 40, 262–270. [Google Scholar] [CrossRef]
- Randel, W.J. Observations of the 2-Day Wave in NMC Stratospheric Analyses. J. Atmos. Sci. 1994, 51, 306–313. [Google Scholar] [CrossRef]
- Forbes, J.M. Tidal and Planetary Waves. In Geophysical Monograph Series; Johnson, R.M., Killeen, T.L., Eds.; American Geophysical Union: Washington, DC, USA, 2013; pp. 67–87. ISBN 978-1-118-66424-7. [Google Scholar]
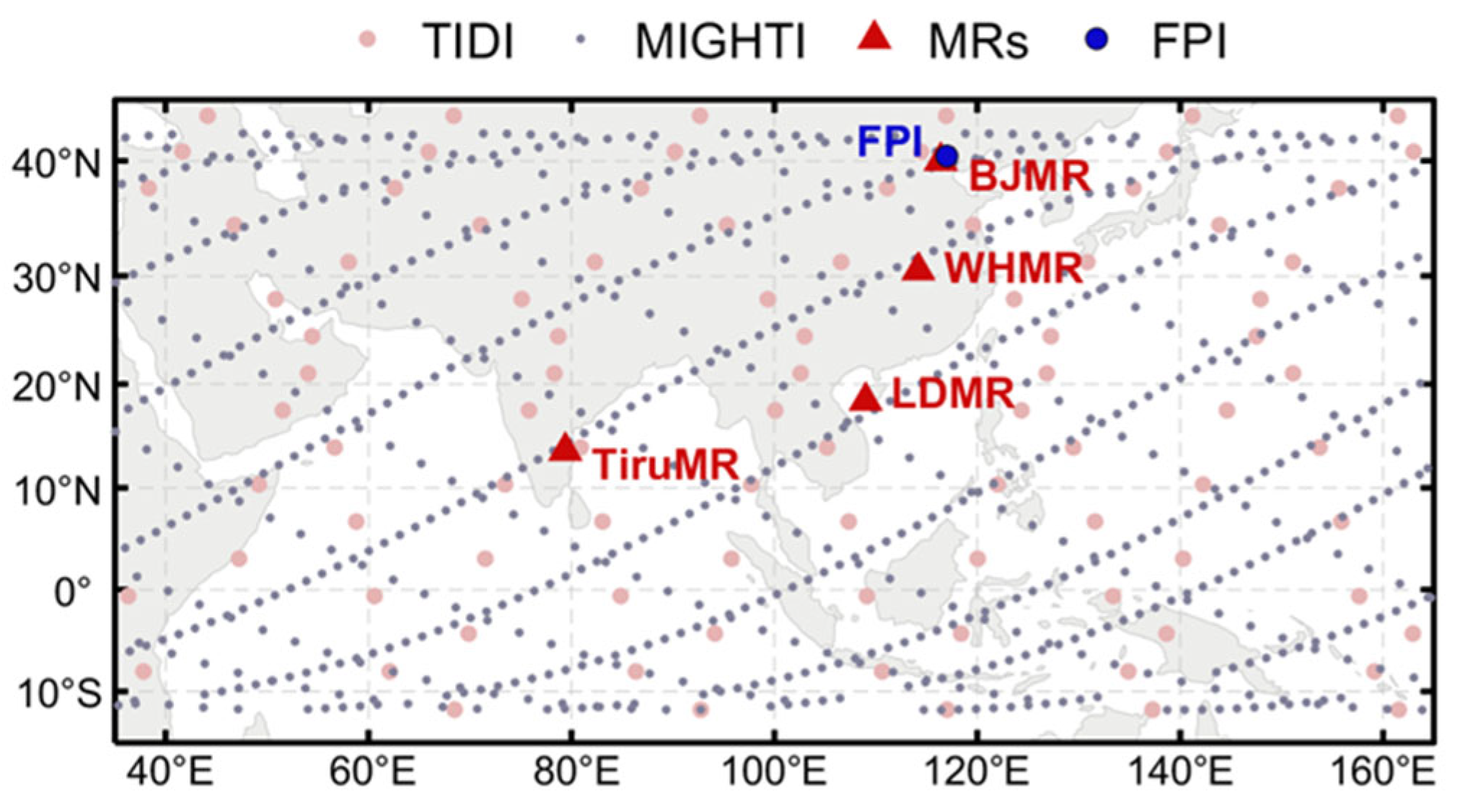



| Station | Latitude | Longitude | Frequency | Peak Power | Availability |
|---|---|---|---|---|---|
| Tirupati | 13.6°N | 79.4°E | 35.25 MHz | 40 kW | 2019.12–2020.4 |
| Ledong | 18.4°N | 109.0°E | 38.9 MHz | 20 kW | 2019.12–2022.10 |
| Wuhan | 30.5°N | 114.6°E | 38.9 MHz | 20 kW | 2019.12–2022.10 |
| Beijing | 40.3°N | 116.2°E | 38.9 MHz | 10 kW | 2019.12–2021.12 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gong, D.; Gu, S.; Qin, Y.; Li, N.; Chen, Y.; Yuan, W.; Wei, Y. Validation of Atmospheric Wind Fields from MIGHTI/ICON: A Comprehensively Comparative Analysis with Meteor Radars, FPI and TIMED/TIDI. Remote Sens. 2025, 17, 794. https://doi.org/10.3390/rs17050794
Gong D, Gu S, Qin Y, Li N, Chen Y, Yuan W, Wei Y. Validation of Atmospheric Wind Fields from MIGHTI/ICON: A Comprehensively Comparative Analysis with Meteor Radars, FPI and TIMED/TIDI. Remote Sensing. 2025; 17(5):794. https://doi.org/10.3390/rs17050794
Chicago/Turabian StyleGong, Dini, Shengyang Gu, Yusong Qin, Na Li, Yiding Chen, Wei Yuan, and Yafei Wei. 2025. "Validation of Atmospheric Wind Fields from MIGHTI/ICON: A Comprehensively Comparative Analysis with Meteor Radars, FPI and TIMED/TIDI" Remote Sensing 17, no. 5: 794. https://doi.org/10.3390/rs17050794
APA StyleGong, D., Gu, S., Qin, Y., Li, N., Chen, Y., Yuan, W., & Wei, Y. (2025). Validation of Atmospheric Wind Fields from MIGHTI/ICON: A Comprehensively Comparative Analysis with Meteor Radars, FPI and TIMED/TIDI. Remote Sensing, 17(5), 794. https://doi.org/10.3390/rs17050794








