Comprehensive Discussion on Remote Sensing Modeling and Dynamic Electromagnetic Scattering for Aircraft with Speed Brake Deflection
Abstract
1. Introduction
2. Method Description
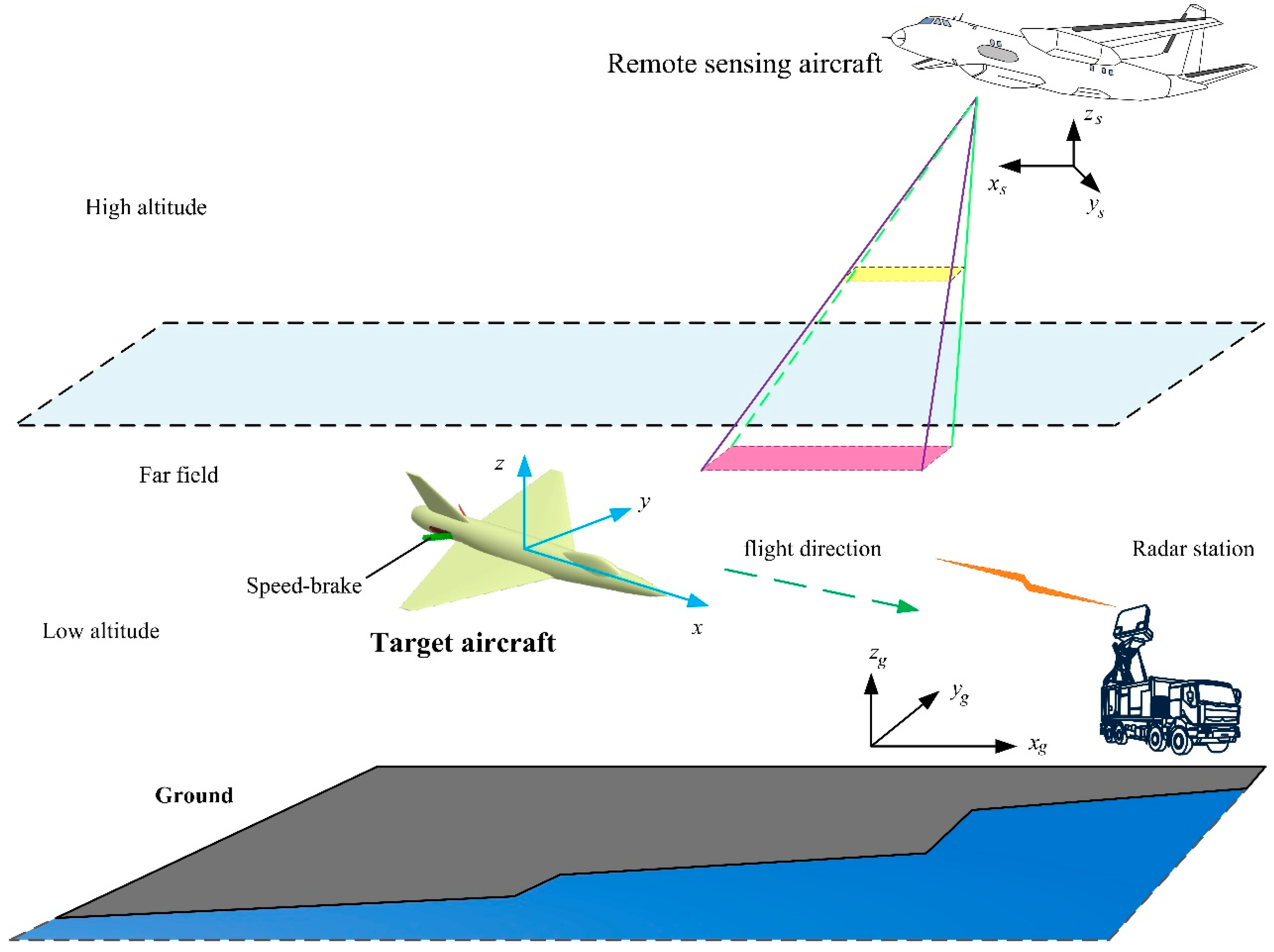
2.1. Remote Sensing Modeling
2.2. Dynamic Electromagnetic Scattering
3. Model Establishment
4. Results and Discussion
4.1. Analysis of Remote Sensing
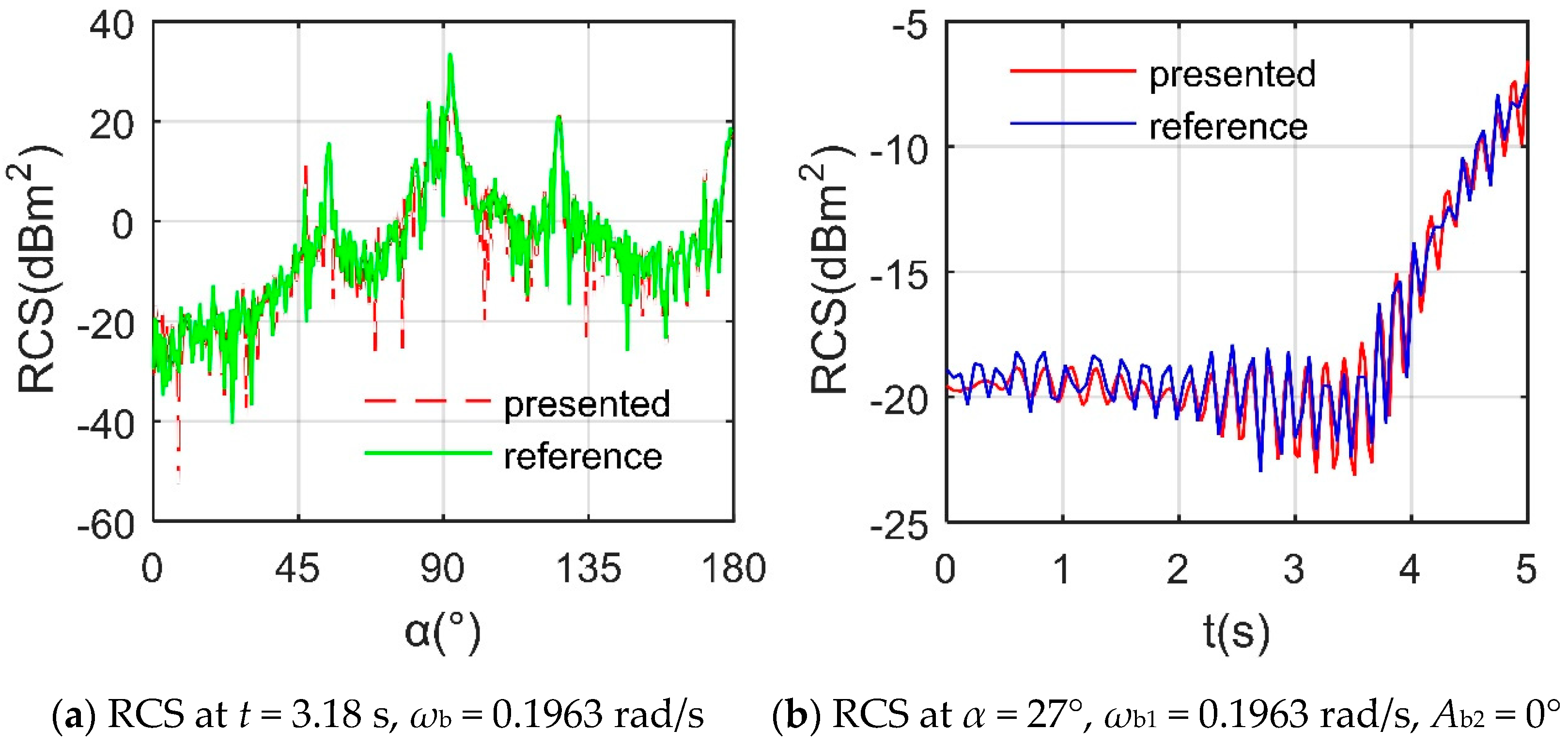
4.2. Dynamic Scattering Analysis
4.3. Comprehensive Discussion
5. Conclusions
- (1)
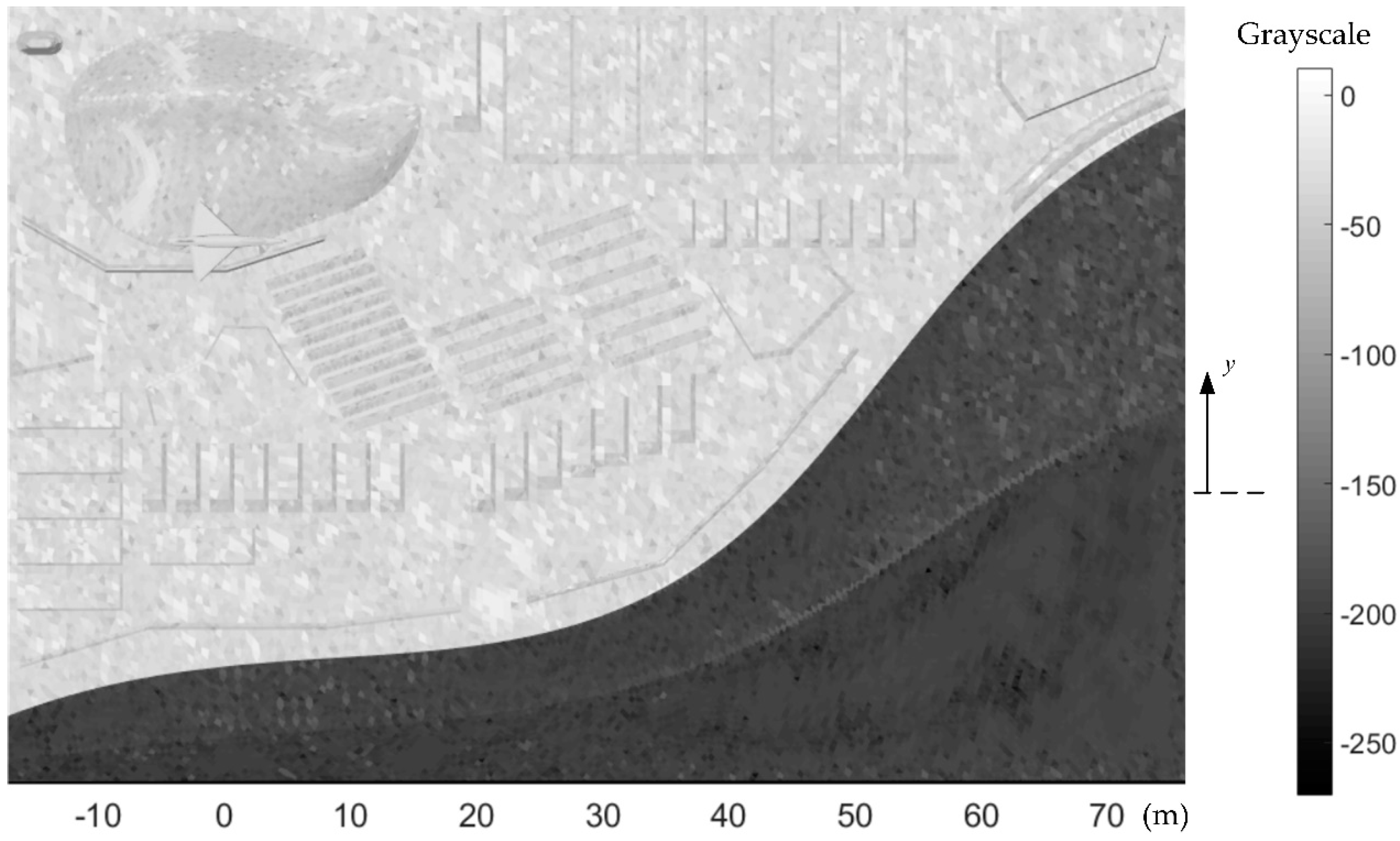
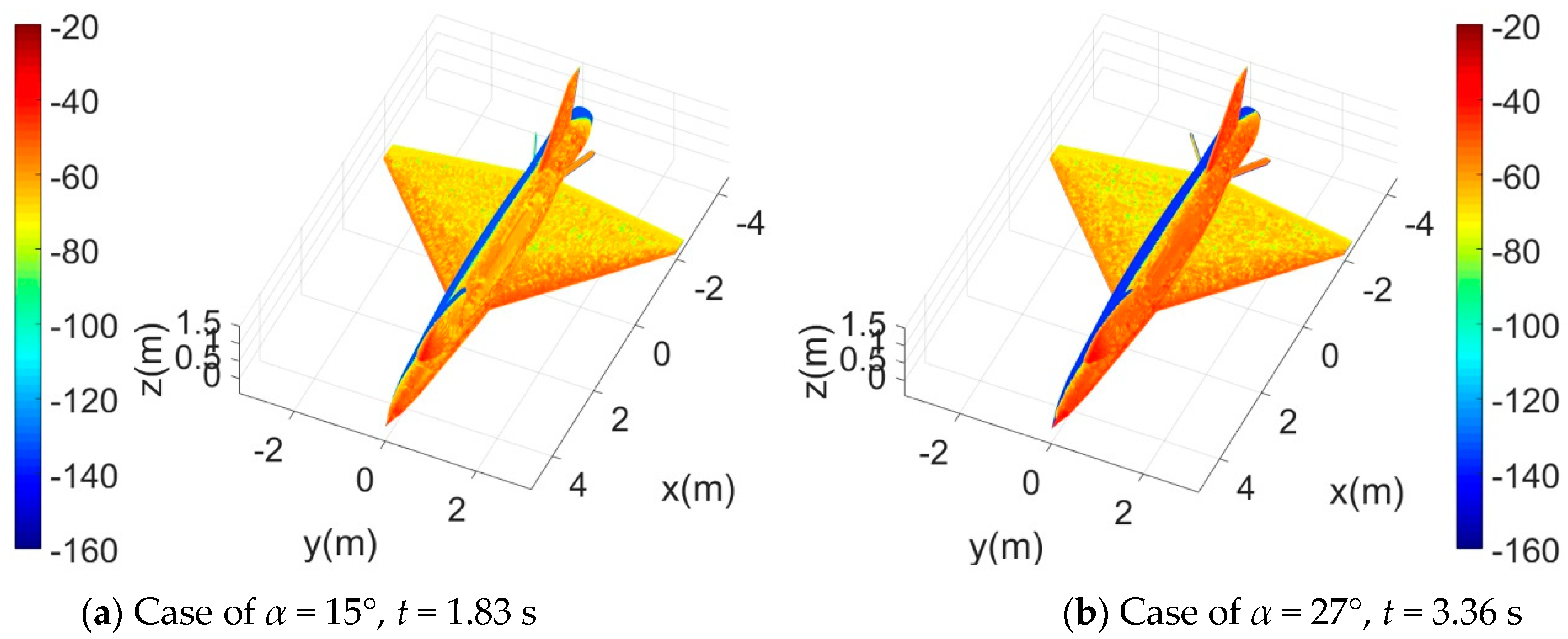
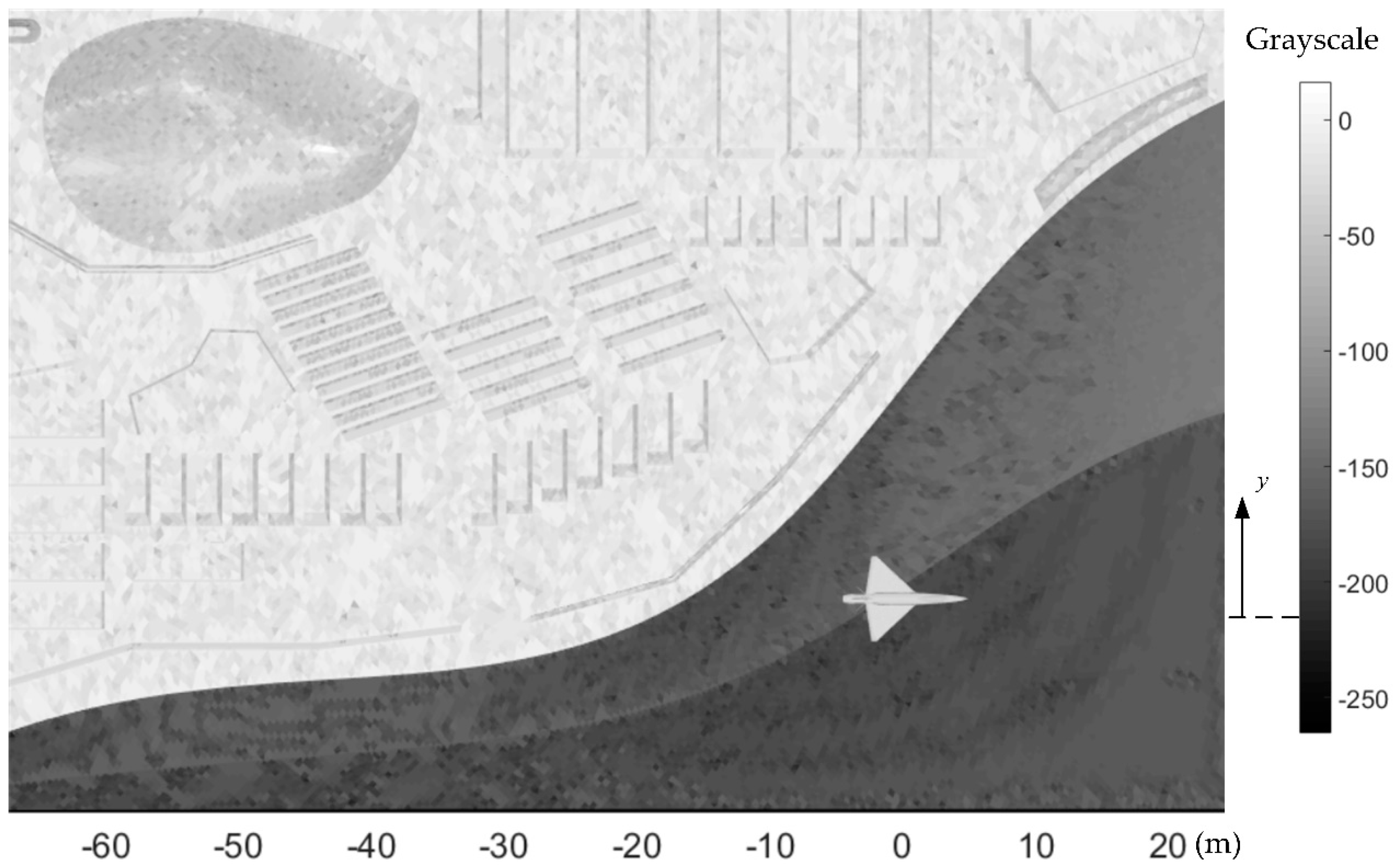
- This remote sensing imaging method can capture the grayscale characteristics of many reference objects on the ground. When the target aircraft enters the observation area, some complex ground reference objects can easily confuse the grayscale projection of the thin speed brake with the ground without taking local magnification measures.
- (2)
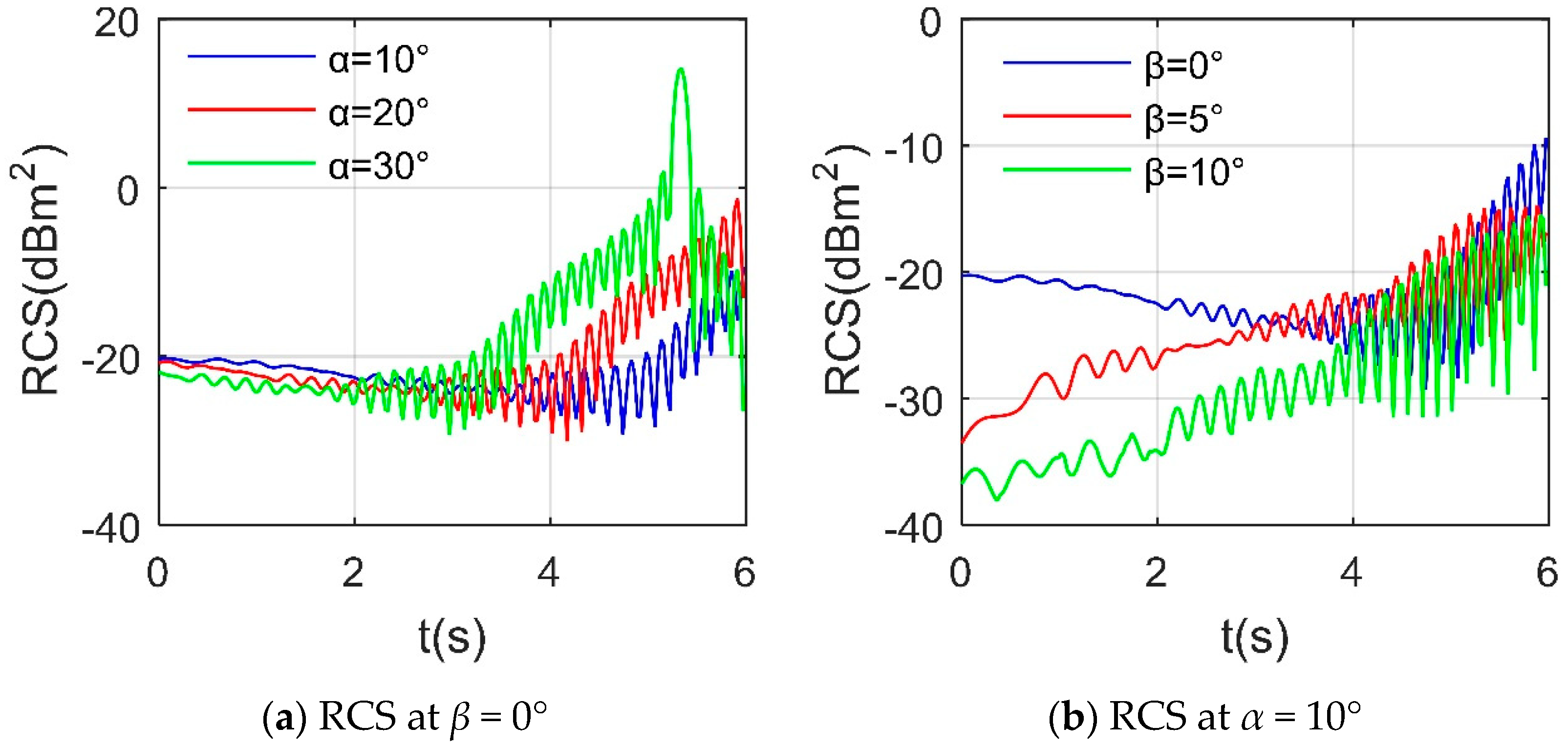
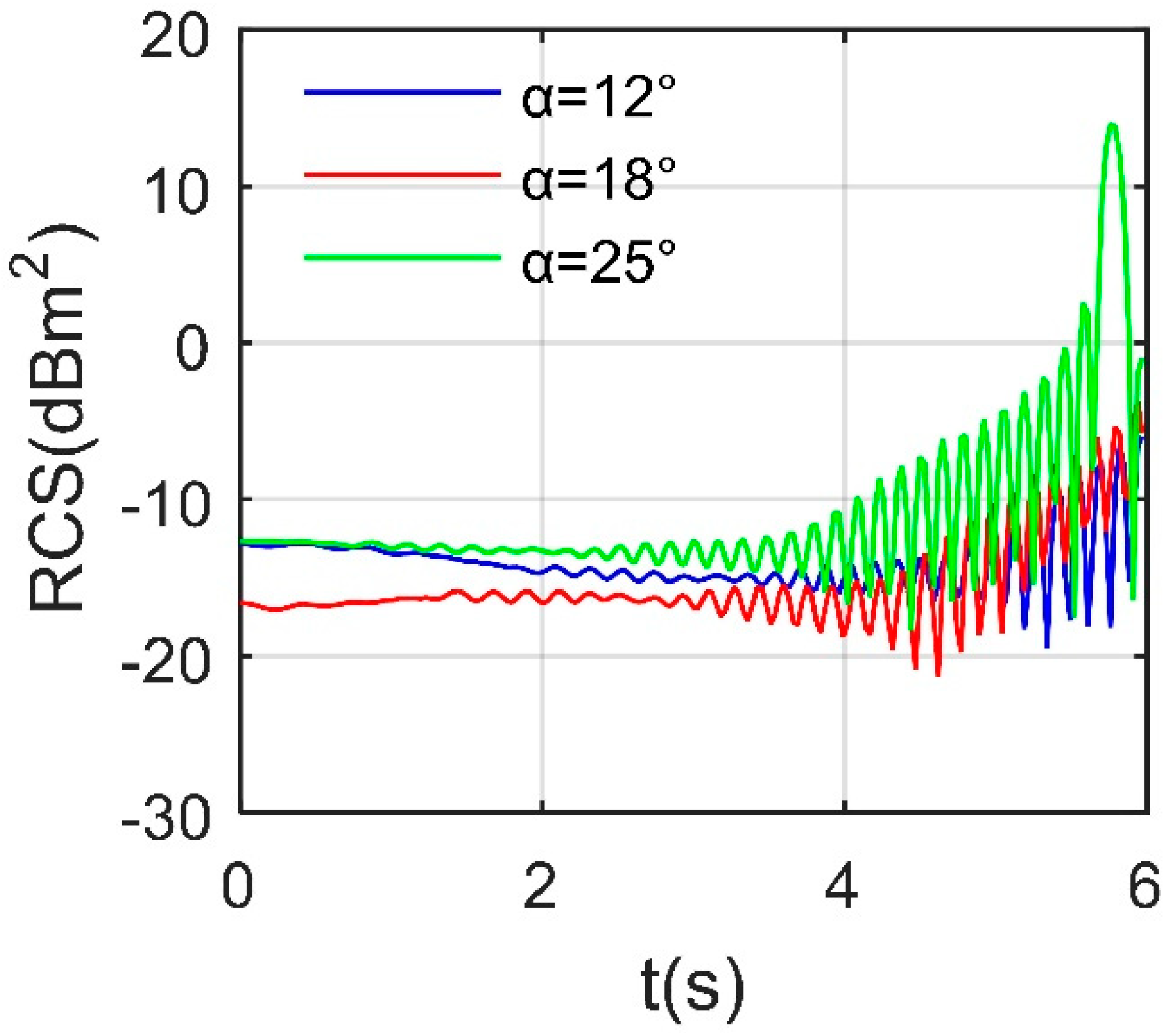
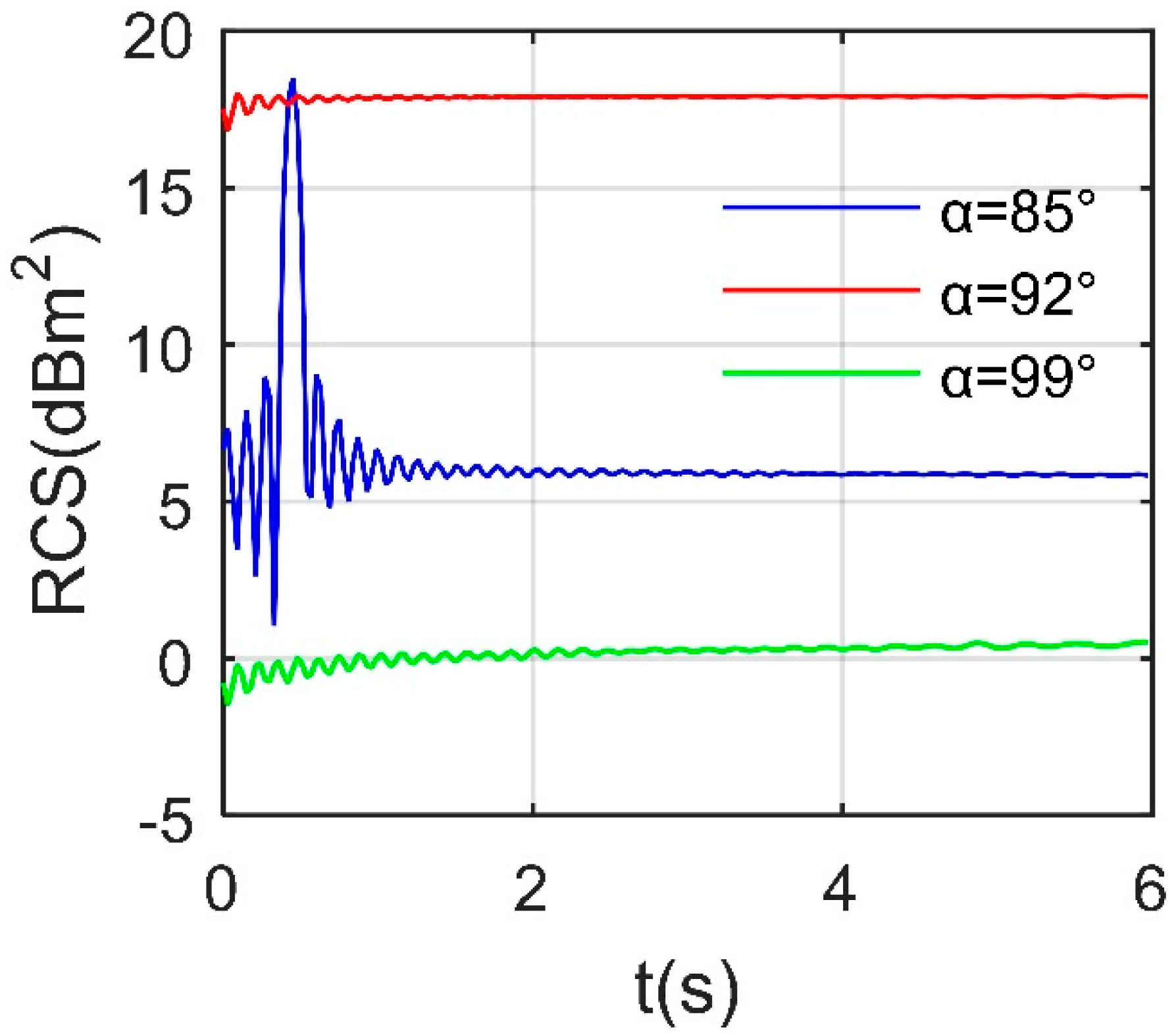
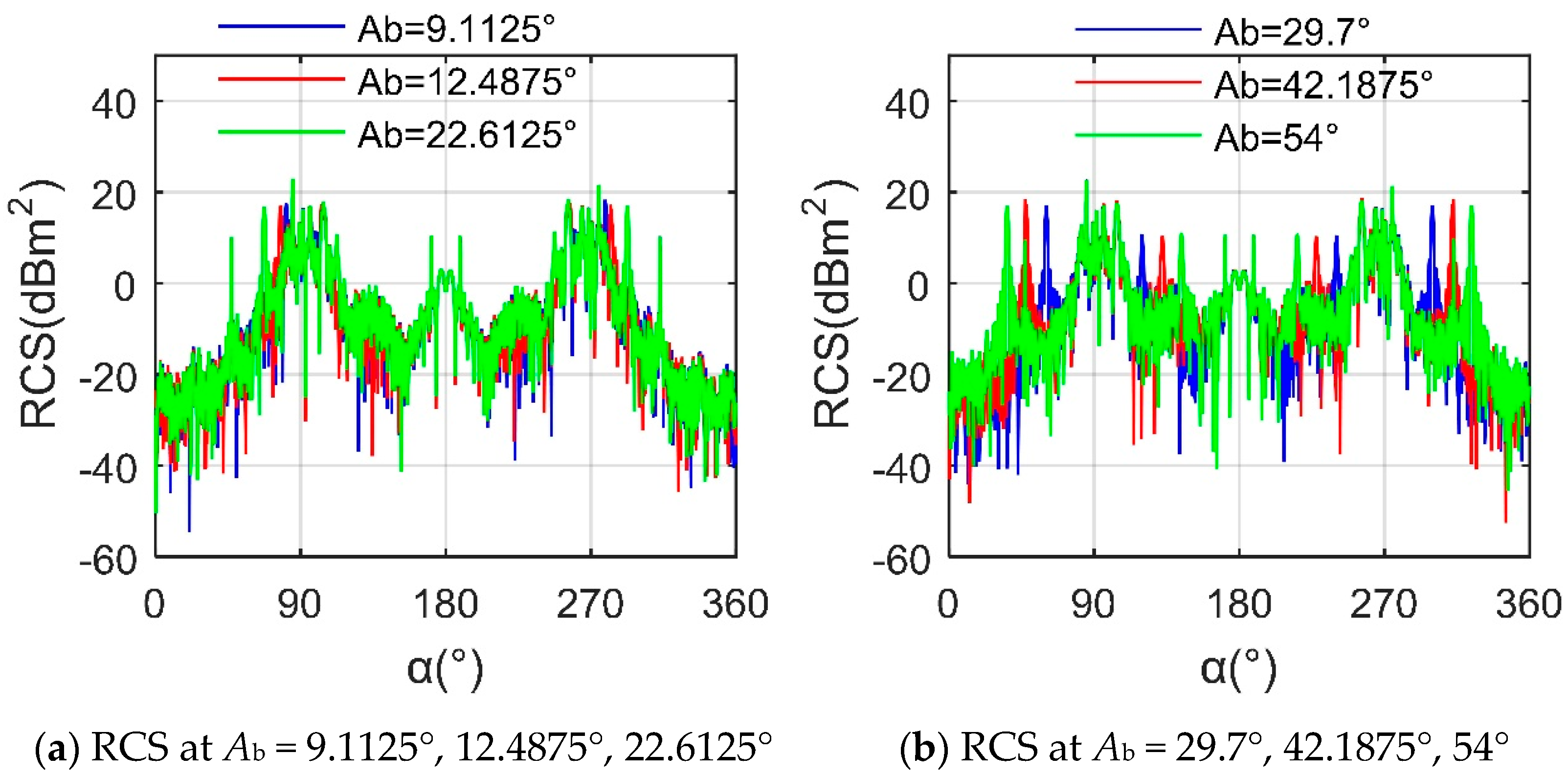
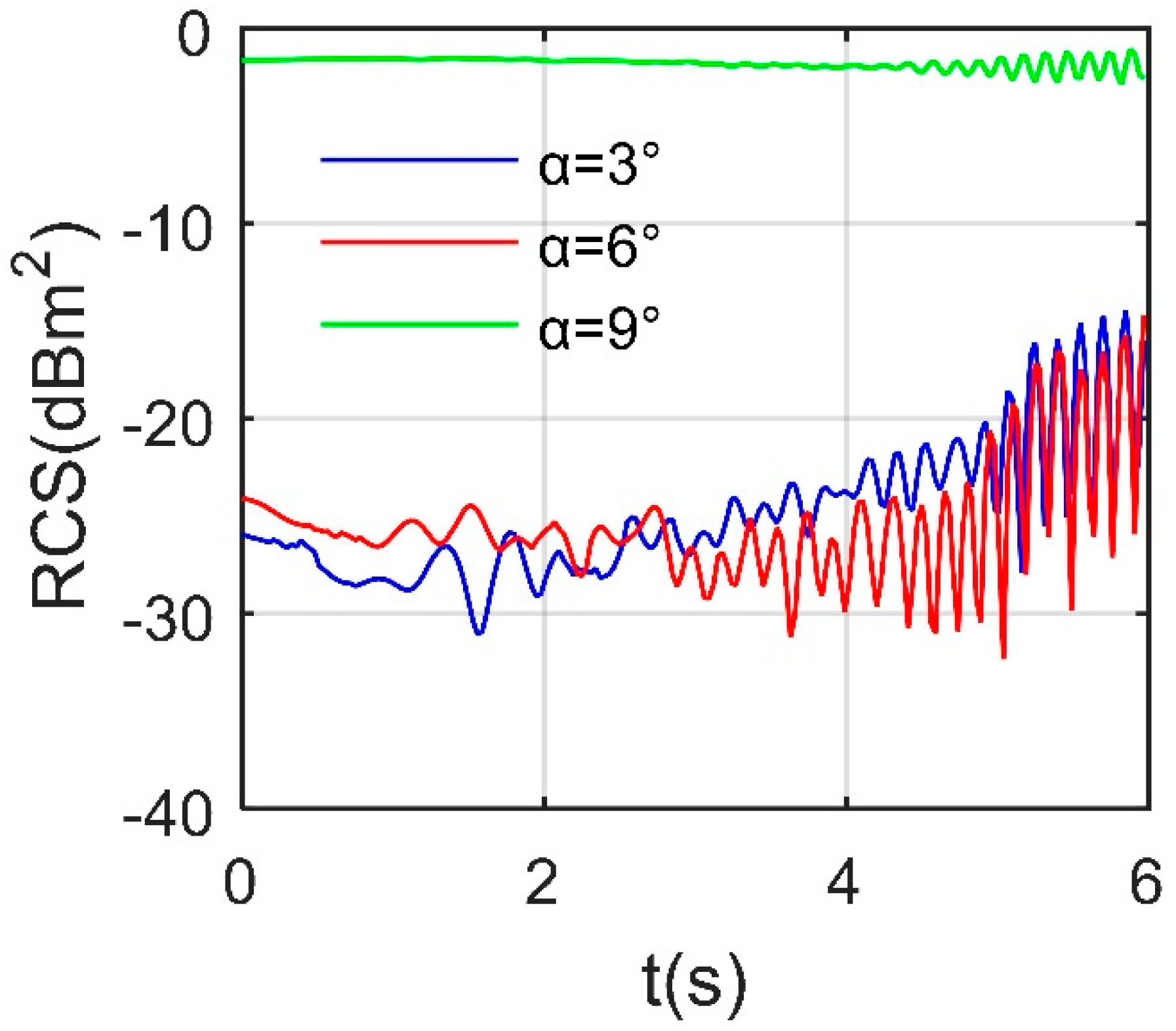
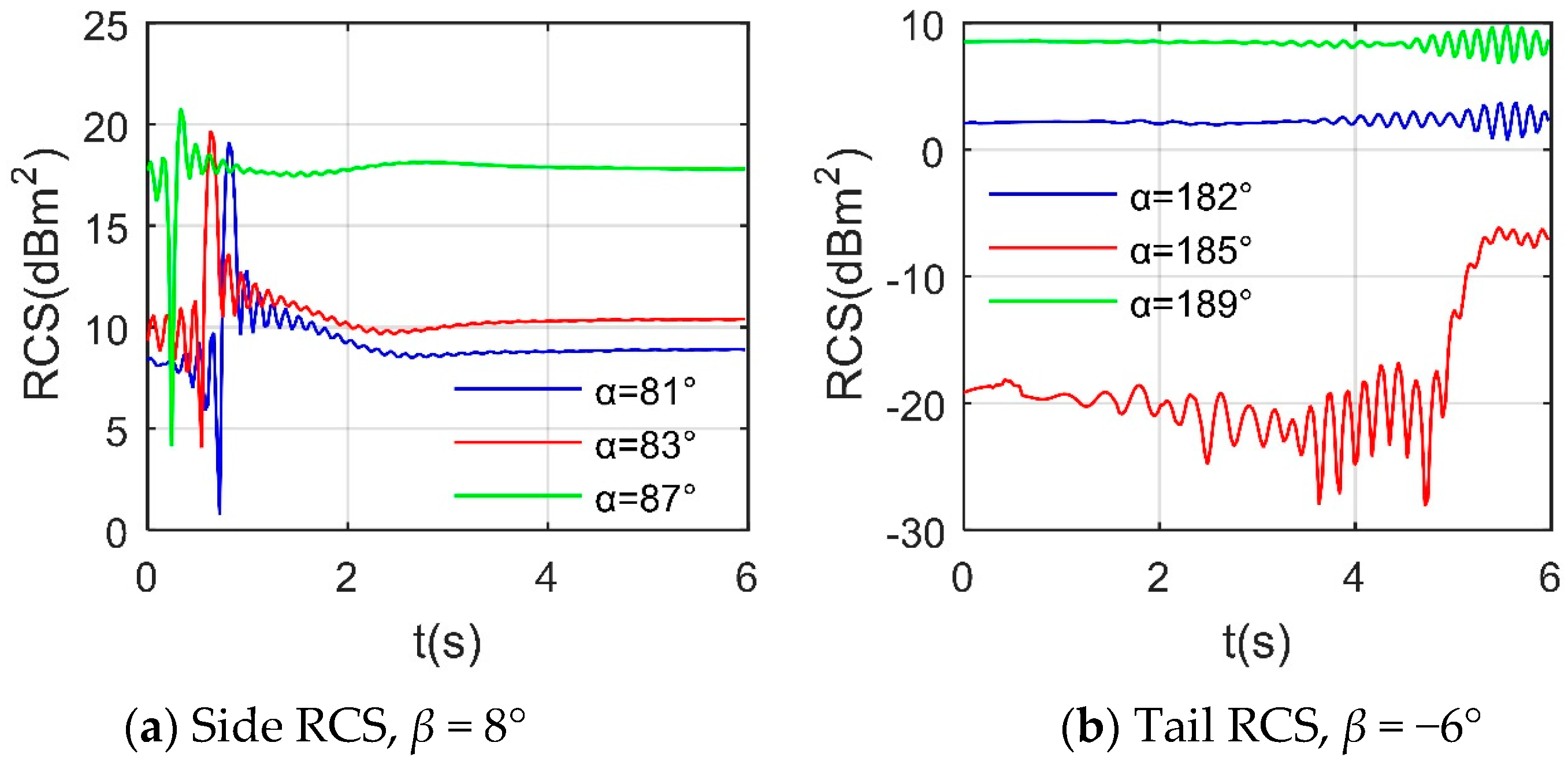
- The dynamic RCS of the speed brake at the forward azimuth shows a large amplitude in the second half of the deflection process, where the increase in elevation angle can reduce the mean and initial value of this dynamic RCS. When the speed brake is deflected on one side, the amplitude of the change in RCS at an example forward azimuth of the aircraft may exceed 33.36 dBm2, while the dynamic characteristics and mean value of the aircraft’s RCS vary greatly under different lateral azimuths.
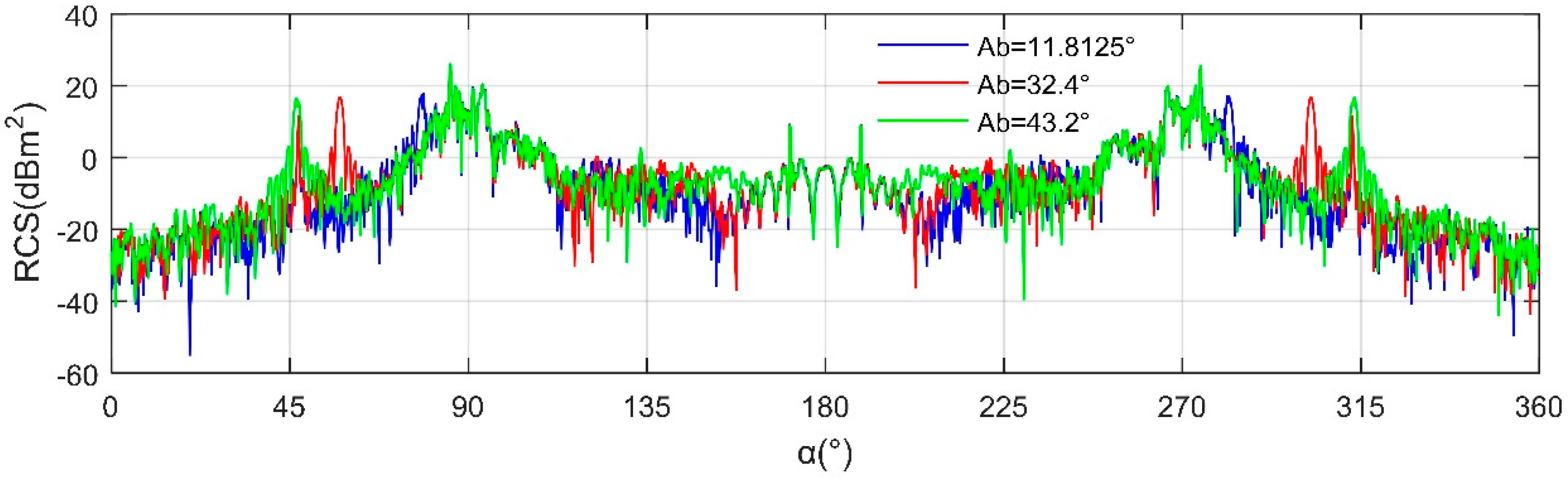
- (3)
- When two speed brakes are opened at a fixed angle, this will introduce a local peak value to the RCS–azimuth curve of the aircraft. The first half of the dynamic deflection of the two speed brakes will introduce large fluctuations to the RCS of the aircraft in the lateral azimuth. When the projection of the target aircraft crosses the water boundary or enters the water area, the fuselage, wings, and vertical tail are easily recognizable, and the opening state and deflection angle of the speed brake can be determined.
Funding
Data Availability Statement
Conflicts of Interest
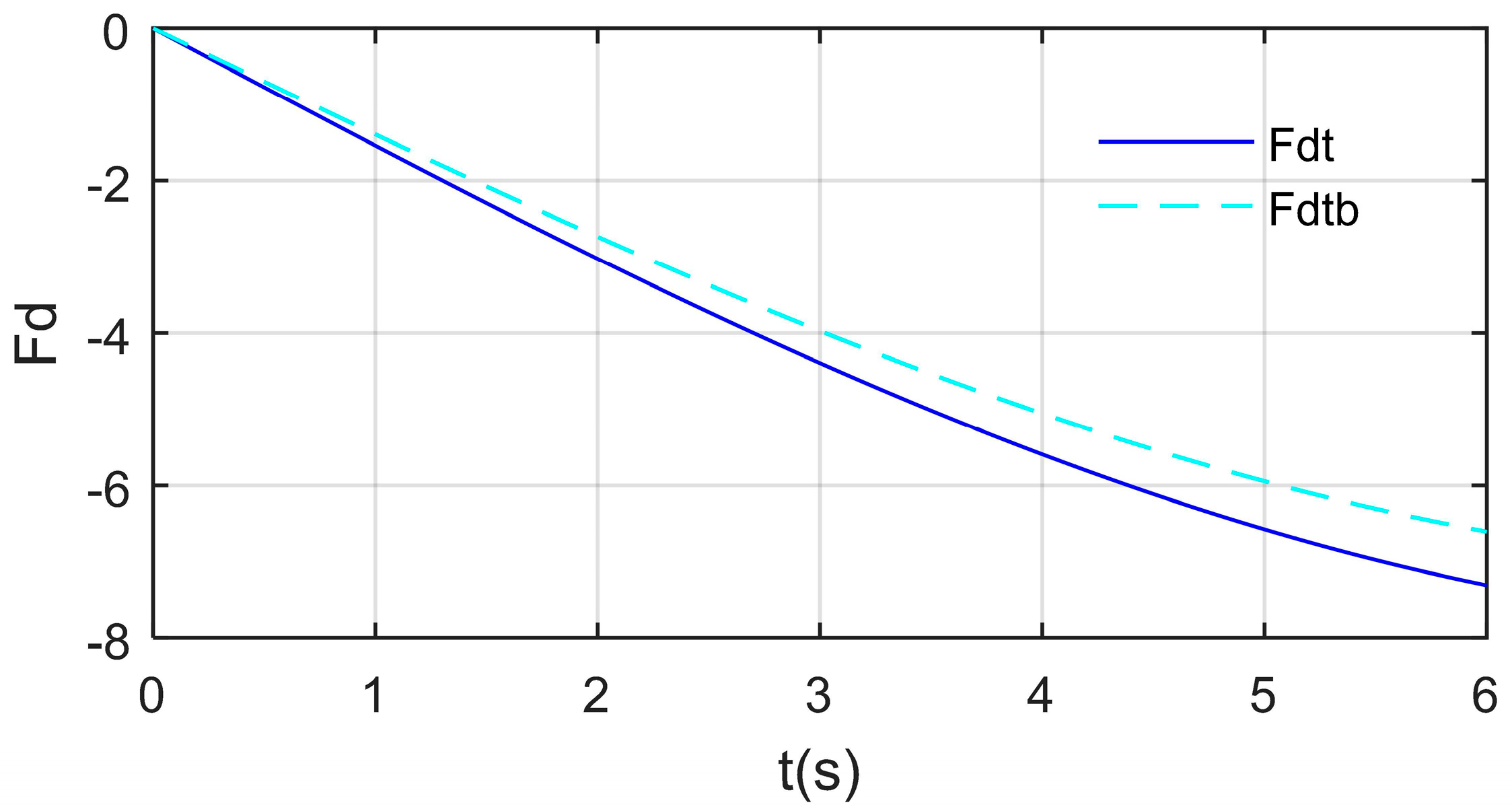
Appendix A

References
- Lockhart, K.; Sandino, J.; Amarasingam, N.; Hann, R.; Bollard, B.; Gonzalez, F. Unmanned Aerial Vehicles for Real-Time Vegetation Monitoring in Antarctica: A Review. Remote Sens. 2025, 17, 304. [Google Scholar] [CrossRef]
- Liang, H.S. Stealth technology for radar onboard next generation fighter. Mod. Radar. 2018, 40, 11–14. [Google Scholar]
- Cui, W.; Liu, J.; Sun, Y.; Li, Q.; Xiao, Z. Airbrake controls of pitching moment and pressure fluctuation for an oblique tail fighter model. Aerosp. Sci. Technol. 2018, 81, 294–305. [Google Scholar] [CrossRef]
- Wang, G.; Zhang, M.H.; Mao, J.; Sang, W.M.; Chen, Z.L.; Wang, L. and Zhang, B. High-lift technology for spoiler on blended-wing-body civil aircraft. Acta Aeronaut. Et Astronaut. Sinica 2019, 40, 623045. [Google Scholar]
- Teillet, P.M.; Fedosejevs, G.; Gauthier, R.P. Operational radiometric calibration of broadscale satellite sensors using hyperspectral airborne remote sensing of prairie rangeland: First trials. Metrologia 1998, 35, 639. [Google Scholar] [CrossRef]
- Cheng, X.Q.; Wong, C.W.; Hussain, F. Flat plate drag reduction using plasma-generated streamwise vortices. J. Fluid Mech. 2021, 918, A24. [Google Scholar] [CrossRef]
- Wang, W.; Liu, P. Numerical Study of Spoiler Aided by Flap on Roll Control and Airbrake Civil. Aircr. Des. Res. 2017, 1, 38–44. [Google Scholar]
- Yan, X.; Chen, R. Augmented flight dynamics model for pilot workload evaluation in tilt-rotor aircraft optimal landing procedure after one engine failure. Chin. J. Aeronaut. 2019, 32, 92–103. [Google Scholar] [CrossRef]
- Zuo, L.X.; Wang, J.J. Experimental study of the effect of AMT on aerodynamic performance of tailless flying wing aircraft. Acta Aerodyn. Sin. 2010, 28, 132–137. [Google Scholar]
- Mao, L.; Wang, X.; Chi, Y.; Pang, S.; Wang, X.; Huang, Q. A Three-Dimensional FDTD (2,4) Subgridding Algorithm for the Airborne Ground-Penetrating Radar Detection of Landslide Models. Remote Sens. 2025, 17, 1107. [Google Scholar] [CrossRef]
- Li, Z.J.; Ma, D.L. Control characteristics analysis of drag yawing control devices of flying wing configuration. J. Beijing Univ. Aeronaut. Astronaut. 2014, 40, 695–700. [Google Scholar]
- Zhou, Z.; Huang, J. Study of the Radar Cross-Section of Turbofan Engine with Biaxial Multirotor Based on Dynamic Scattering Method. Energies 2020, 13, 5802. [Google Scholar] [CrossRef]
- Qu, X.B.; Zhang, W.G.; Shi, J.P. Control allocation method for split-drag-rudders with asymmetrical deflection. J. Syst. Simul. 2016, 28, 627–639. [Google Scholar]
- Dash, S.M.; Triantafyllou, M.S.; Alvarado, P.V.Y. A numerical study on the enhanced drag reduction and wake regime control of a square cylinder using dual splitter plates. Comput. Fluids 2020, 199, 104421. [Google Scholar] [CrossRef]
- Liu, J.; Zheng, L.Q. Study on Flow Mechanism and Aerodynamic Characteristic of High-Lift Devices with Drooped Spoiler. J. Northwestern Polytech. Univ. 2017, 35, 813–820. [Google Scholar]
- Li, S.; Simonian, A.; Chin, B.A. Sensors for agriculture and the food industry. Electrochem. Soc. Interface 2010, 19, 41–46. [Google Scholar] [CrossRef]
- Liu, C.; Liu, P. Research on Aerodynamic Characteristics of AHDF Civil Aviation Aircraft. Aeronaut. Comput. Tech. 2021, 51, 84–87. [Google Scholar]
- Zhou, Z.; Huang, J. An optimization model of parameter matching for aircraft catapult launch. Chin. J. Aeronaut. 2020, 33, 191–204. [Google Scholar] [CrossRef]
- Li, T.S. Analysis and Treatment on Left Front Deceleration Beam Crack for a Certain Type of Aircraft. Aviat. Maint. Eng. 2019, 3, 97–99. [Google Scholar]
- Saleem, M.; Saifullah, Y. Coding Artificial Magnetic Conductor Ground and Their Application to High-Gain, Wideband Radar Cross-Section Reduction of a 2×2 Antenna Array. Phys. Status Solidi 2021, 218, 2100088. [Google Scholar] [CrossRef]
- Chen, L.; Duan, P.F.; Yuan, C. Research on development status and key technology of stealth air-to-air missiles. Aero Weapon. 2022, 29, 14–21. [Google Scholar]
- Ezuma, M.; Anjinappa, C.K.; Funderburk, M. Radar cross section based statistical recognition of UAVs at microwave frequencies. IEEE Trans. Aerosp. Electron. Syst. 2021, 58, 27–46. [Google Scholar] [CrossRef]
- Bu, L.; Fu, T.; Chen, D.; Cao, H.; Zhang, S.; Han, J. Doppler-Spread Space Target Detection Based on Overlapping Group Shrinkage and Order Statistics. Remote Sens. 2024, 16, 3413. [Google Scholar] [CrossRef]
- Yin, P.; Jia, G.W.; Yang, X.X. Research on the development of foreign military uav stealth design. Aerodyn. Missile J. 2021, 12, 69–74. [Google Scholar]
- Zhou, Z.; Huang, J. Numerical investigations on radar cross-section of helicopter rotor with varying blade pitch. Aerosp. Sci. Technol. 2022, 123, 107452. [Google Scholar] [CrossRef]
- Ye, S.B.; Xiong, J.J. Dynamic RCS Behavior of Helicopter Rotating Blades. Acta Aeronaut. Et Astronaut. Sinica 2006, 27, 816–822. [Google Scholar]
- Zhou, Z.; Huang, J. Mixed design of radar/infrared stealth for advanced fighter intake and exhaust system. Aerosp. Sci. Technol. 2021, 110, 106490. [Google Scholar] [CrossRef]
- Xu, X.; Gong, S.X. The forward-backward IPO algorithm for calculating open-ended cavities. Space Electron. Technol. 2008, 1, 72–76. [Google Scholar]
- Song, S.; Cho, C.; Oh, T. Effect of driving frequency on reduction of radar cross section due to dielectric-barrier-discharge plasma in Ku-band. IEEE Trans. Plasma Sci. 2021, 49, 1548–1556. [Google Scholar] [CrossRef]
- Rodríguez-López, L.; Bravo Alvarez, L.; Duran-Llacer, I.; Ruíz-Guirola, D.E.; Montejo-Sánchez, S.; Martínez-Retureta, R.; López-Morales, E.; Bourrel, L.; Frappart, F.; Urrutia, R. Leveraging Machine Learning and Remote Sensing for Water Quality Analysis in Lake Ranco, Southern Chile. Remote Sens. 2024, 16, 3401. [Google Scholar] [CrossRef]
- Sui, M.; Xu, X.J. Electromagnetic scattering calculation of complex structure using iterative physical optics based on curved surface patches. Chin. J. Radio Sci. 2012, 27, 892–896. [Google Scholar]
- Zhou, Z.; Huang, J. Study of RCS characteristics of tilt-rotor aircraft based on dynamic calculation approach. Chin. J. Aeronaut. 2022, 35, 426–437. [Google Scholar] [CrossRef]
- Zhou, Z.; Huang, J. Y-type quadrotor radar cross-section analysis. Aircr. Eng. Aerosp. Technol. 2023, 95, 535–545. [Google Scholar] [CrossRef]
- Zhang, T. Iterative Physical Optics and Its Acceleration Methods in Electromagnetic Scattering from Target and Rough Sea Surface; Xidian University: Xi’an, China, 2015. [Google Scholar]
- Xu, X.; Feng, C.; Han, L. Classification of Radar Targets with Micro-Motion Based on RCS Sequences Encoding and Convolutional Neural Network. Remote Sens. 2022, 14, 5863. [Google Scholar] [CrossRef]
- Zhou, Z.; Huang, J. X-Band Radar Cross-Section of Tandem Helicopter Based on Dynamic Analysis Approach. Sensors 2021, 21, 271. [Google Scholar] [CrossRef]
- Zhou, Z.; Huang, J. Z-folding aircraft electromagnetic scattering analysis based on hybrid grid matrix transformation. Sci. Rep. 2022, 12, 4452. [Google Scholar] [CrossRef]




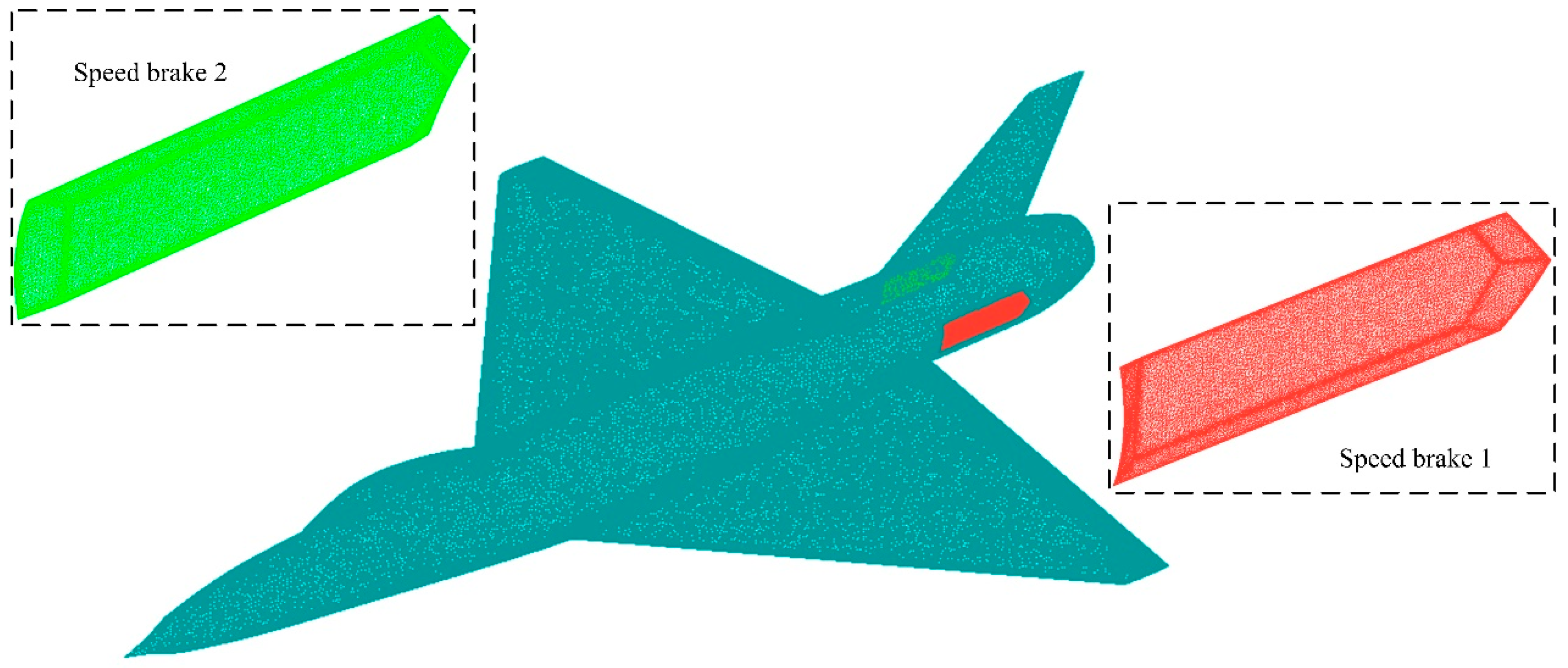





| Parameter | Awl (°) | Awt (°) | Lrf (m) | Lf (m) |
| Value | 47.121 | 8.727 | 0.78 | 9.5 |
| Parameter | Wf (m) | Xb2 (m) | Atl (°) | Hv (m) |
| Value | 6.4 | 2.855 | 38.437 | 1.52 |
| Parameter | Lbl (mm) | Hbe (mm) | Lbe (mm) |
| Value | 862.81 | 22.78 | 60.24 |
| Parameter | Wb1 (mm) | Hb1 (mm) | Ltv (mm) |
| Value | 220 | 40 | 82.71 |
| Region | Max (mm) | Region | Max (mm) |
|---|---|---|---|
| Global minimum size | 1 | Outside edge of speed brake | 1 |
| Front edge of speed brake | 2 | Inside edge of speed brake | 2 |
| Speed brake surface | 8 | Wing trailing edge | 5 |
| Leading edge of wing | 8 | Vertical tail edge | 15 |
| Nozzle edge | 15 | Fuselage surface | 50 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Z. Comprehensive Discussion on Remote Sensing Modeling and Dynamic Electromagnetic Scattering for Aircraft with Speed Brake Deflection. Remote Sens. 2025, 17, 1706. https://doi.org/10.3390/rs17101706
Zhou Z. Comprehensive Discussion on Remote Sensing Modeling and Dynamic Electromagnetic Scattering for Aircraft with Speed Brake Deflection. Remote Sensing. 2025; 17(10):1706. https://doi.org/10.3390/rs17101706
Chicago/Turabian StyleZhou, Zeyang. 2025. "Comprehensive Discussion on Remote Sensing Modeling and Dynamic Electromagnetic Scattering for Aircraft with Speed Brake Deflection" Remote Sensing 17, no. 10: 1706. https://doi.org/10.3390/rs17101706
APA StyleZhou, Z. (2025). Comprehensive Discussion on Remote Sensing Modeling and Dynamic Electromagnetic Scattering for Aircraft with Speed Brake Deflection. Remote Sensing, 17(10), 1706. https://doi.org/10.3390/rs17101706







