Research on the Detection Method of Martian Atmospheric Temperature and Pressure Profile Based on Laser Occultation Technology
Abstract
1. Introduction
2. Laser Occultation Principle
3. Simulation of Mars Laser Occultation Model
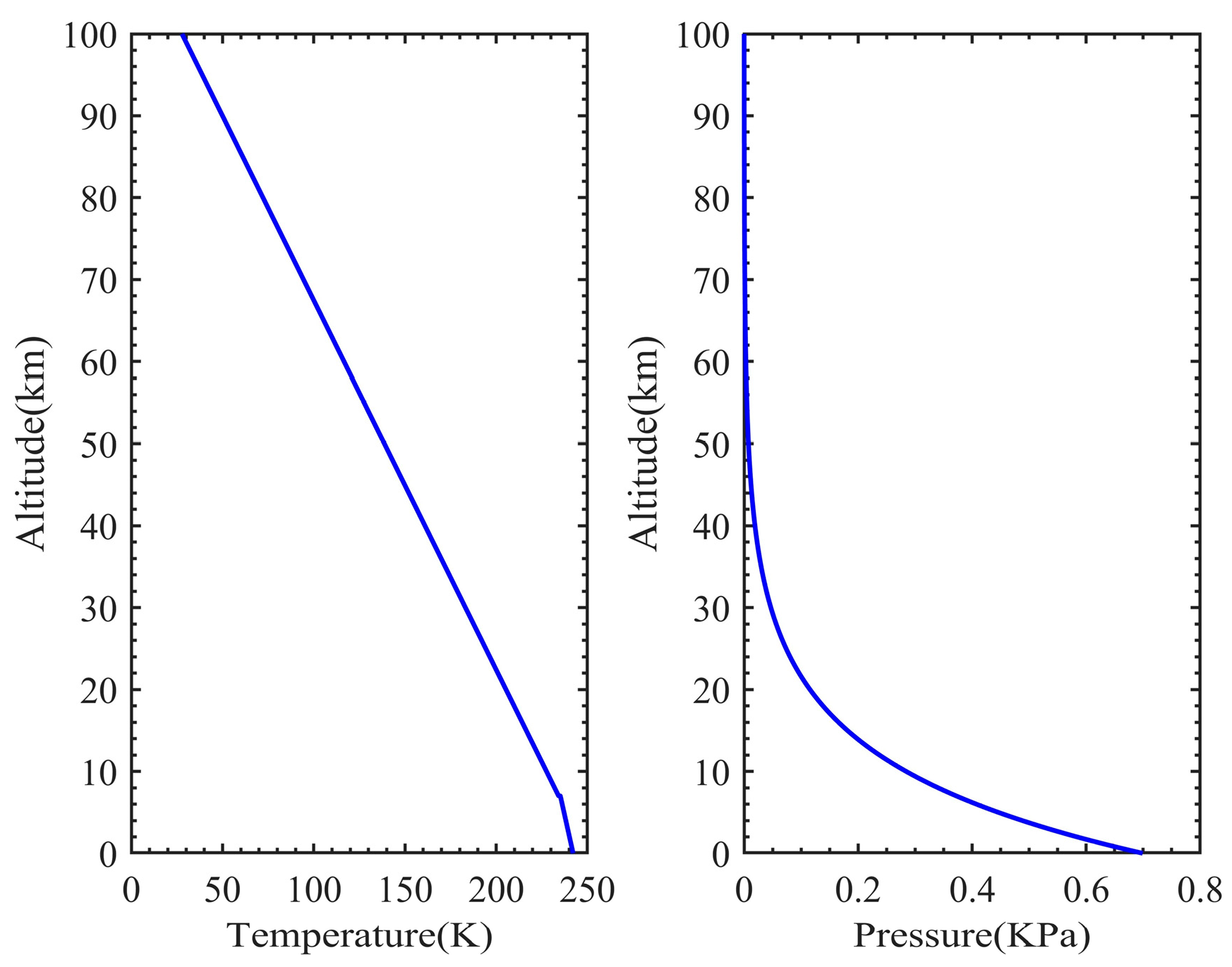
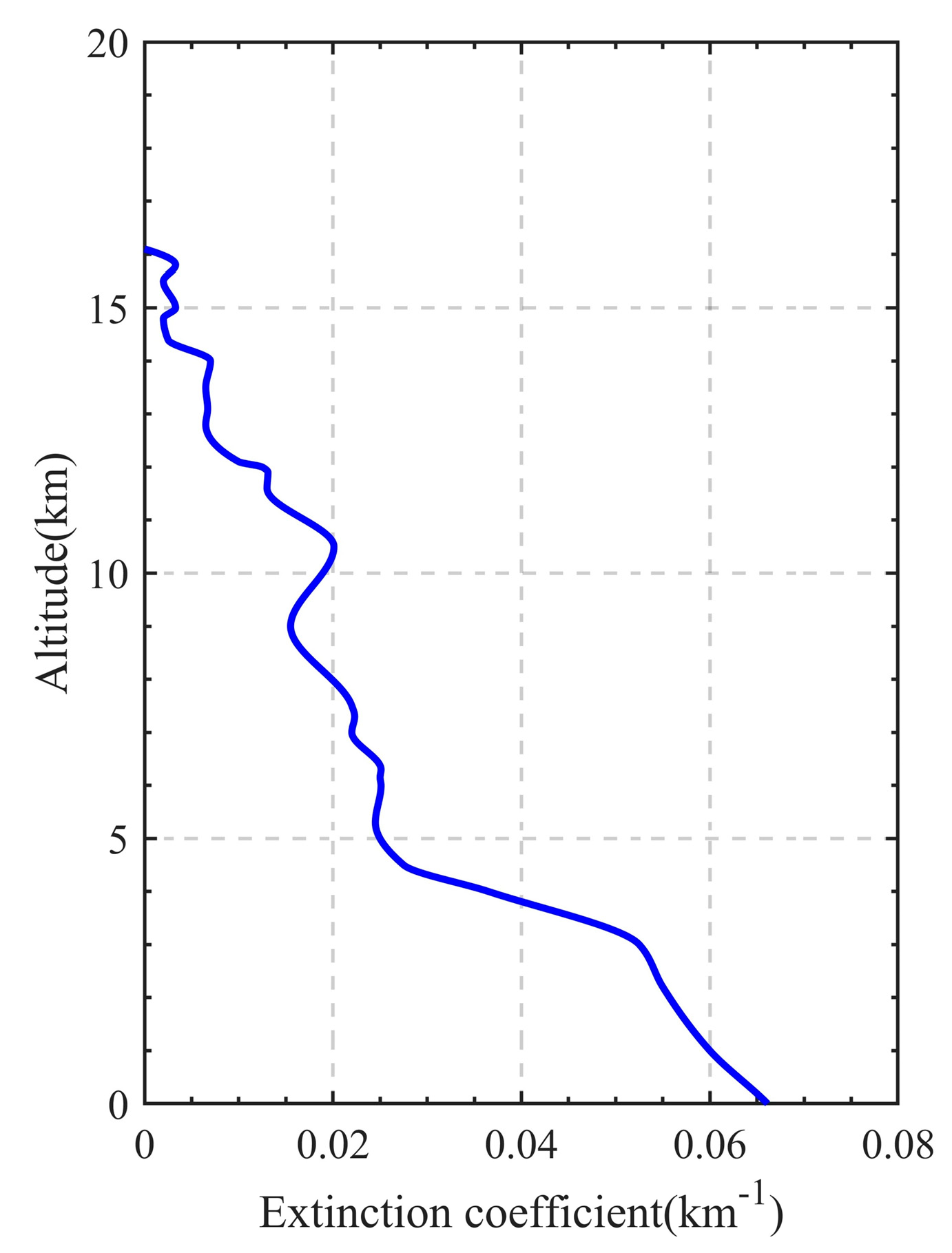
3.1. Mars Atmosphere Model
3.2. Occultation Beam Trajectory Model
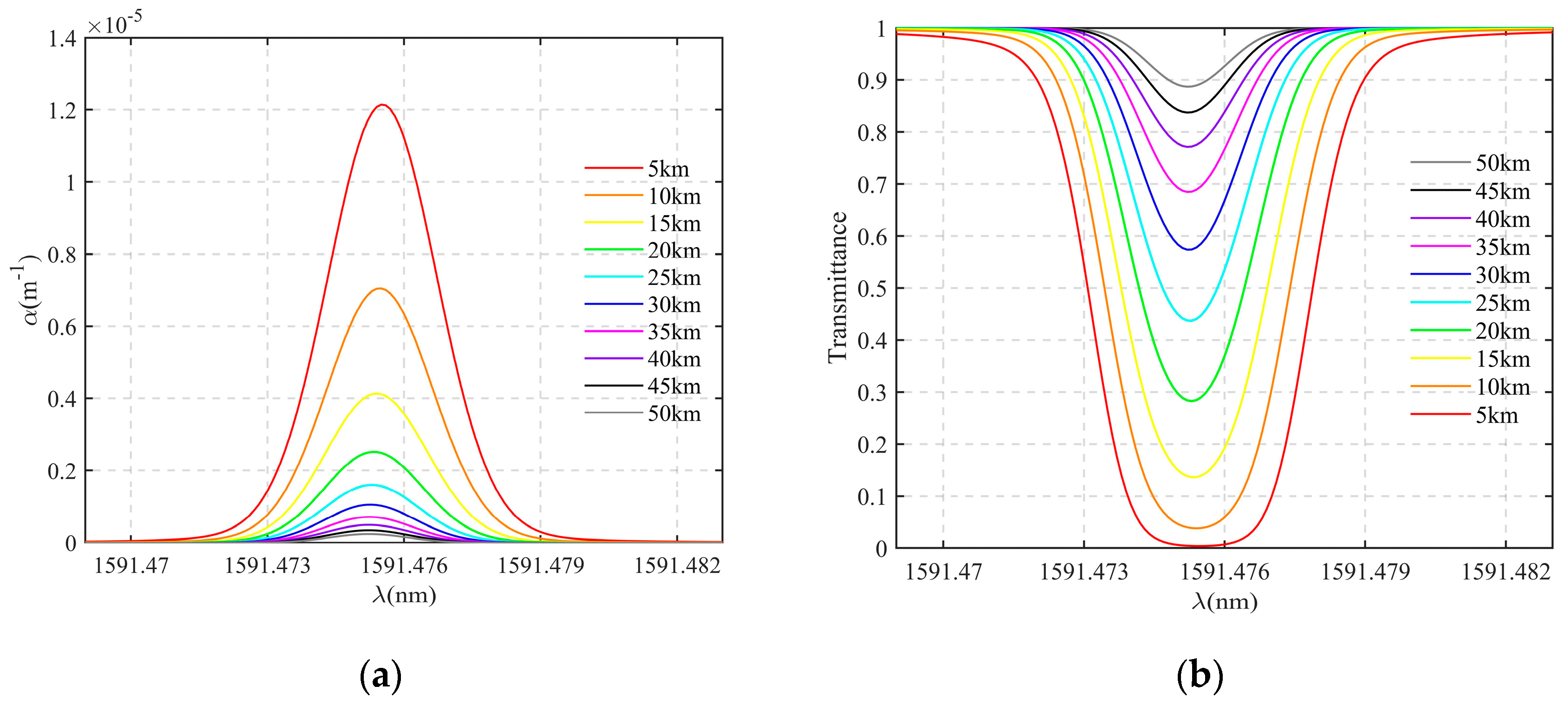
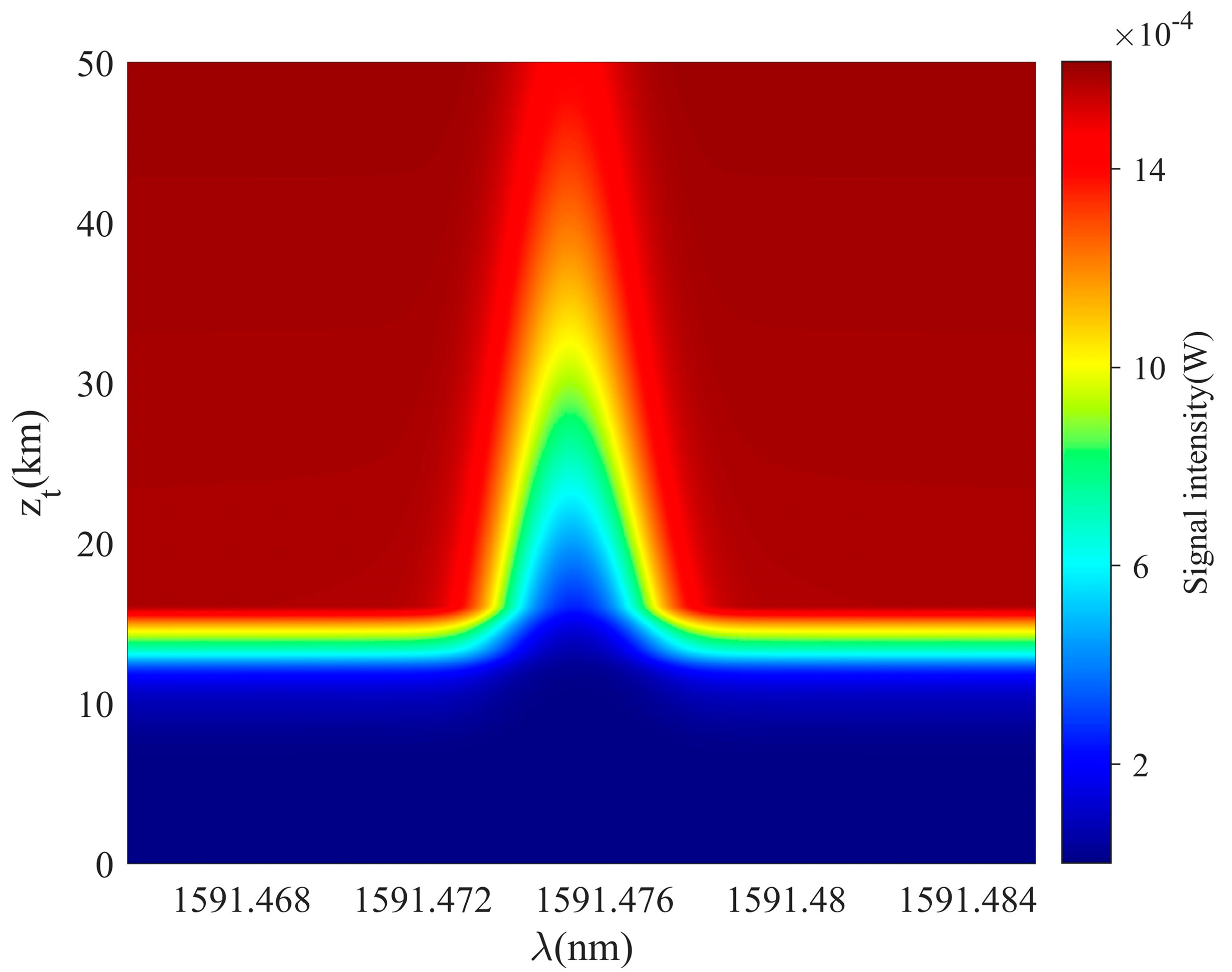
3.3. The Signal Radiation Intensity Calculation Model
4. Analysis of the Inversion Method and Simulation Results
4.1. The Inversion Method of Martian Atmospheric Temperature and Pressure Profiles Based on Carbon Dioxide Absorption Spectrum
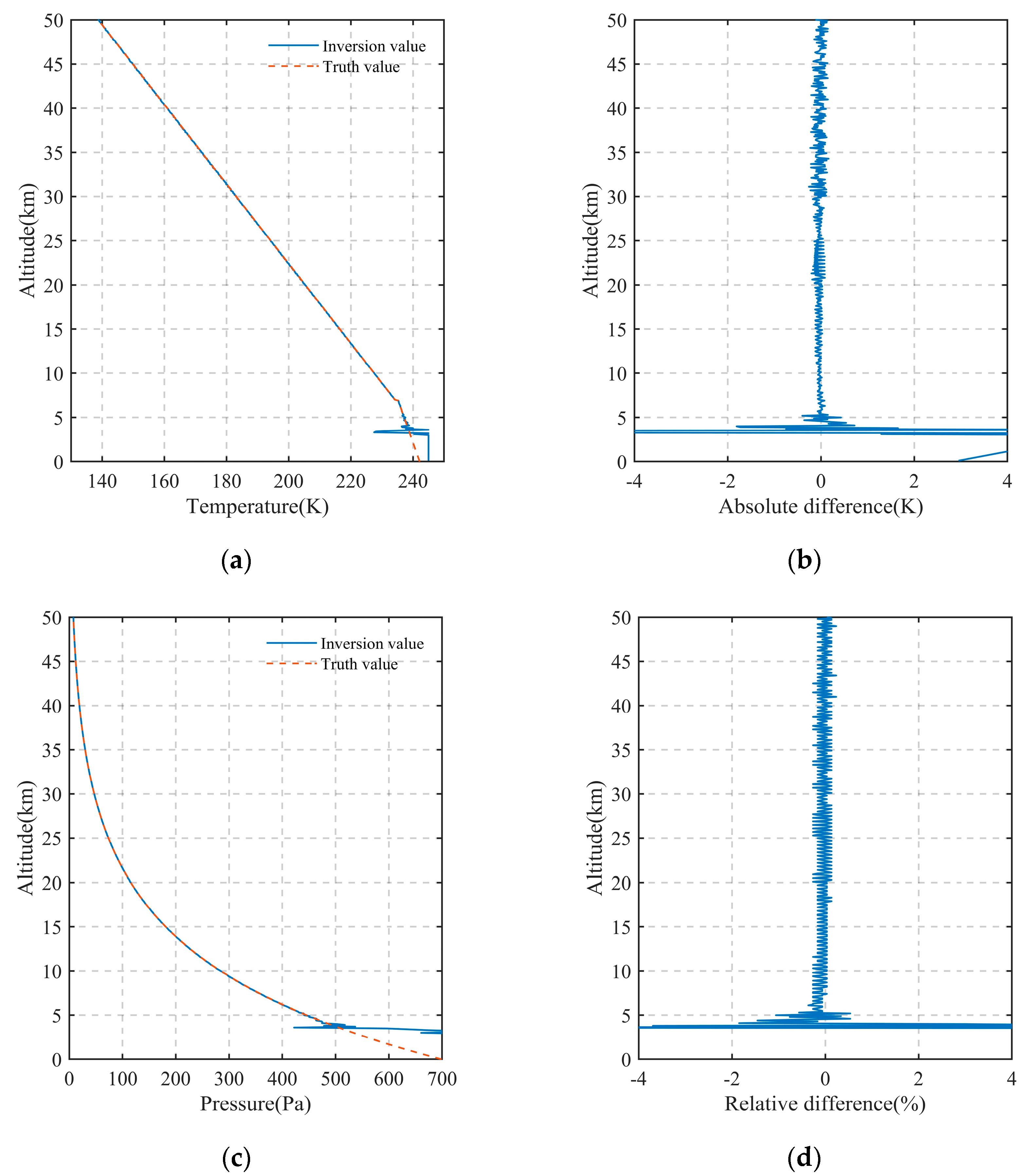
4.2. Inversion Results and Error Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Fonseca, R.M.; Zorzano, M.P.; Martín-Torres, J. MARSWRF Prediction of Entry Descent Landing Profiles: Applications to Mars Exploration. Earth Space Sci. 2019, 6, 1440–1459. [Google Scholar] [CrossRef]
- Broadfoot, A.L.; Sandel, B.R.; Shemansky, D.E.; Atreya, S.K.; Donahue, T.M.; Moos, H.W.; Bertaux, J.L.; Blamont, J.E.; Ajello, J.M.; Strobel, D.F.; et al. Ultraviolet spectrometer experiment for the Voyager mission. Space Sci. Rev. 1977, 21, 183–205. [Google Scholar] [CrossRef]
- Wickert, J.; Reigber, C.; Beyerle, G.; König, R.; Marquardt, C.; Schmidt, T.; Grunwaldt, L.; Galas, R.; Meehan, T.K.; Melbourne, W.G.; et al. Atmosphere sounding by GPS radio occultation: First results from CHAMP. Geophys. Res. Lett. 2001, 28, 3263–3266. [Google Scholar] [CrossRef]
- Bertaux, J.L.; Vandaele, A.C.; Korablev, O.; Villard, E.; Fedorova, A.; Fussen, D.; Quemerais, E.; Belyaev, D.; Mahieux, A.; Montmessin, F.; et al. A warm layer in Venus’ cryosphere and high-altitude measurements of HF, HCl, H2O, and HDO. Nature 2007, 450, 646–649. [Google Scholar] [CrossRef]
- Liao, M.; Zhang, P.; Yang, G.-L.; Bi, Y.-M.; Liu, Y.; Bai, W.-H.; Meng, X.-G.; Du, Q.-F.; Sun, Y.-Q. Preliminary validation of the refractivity from the new radio occultation sounder GNOS/FY-3C. Atmos. Meas. Technol. 2016, 9, 781–792. [Google Scholar] [CrossRef]
- Fjeldbo, G.; Eshleman, V.R.; Garriott, O.K.; Smith, F.L. The two-frequency bistatic radar-occultation method for the study of planetary ionospheres. J. Geophys. Res. 1965, 70, 3701–3710. [Google Scholar] [CrossRef]
- Kliore, A.; Cain, D.L.; Levy, G.S.; Eshleman, V.R.; Fjeldbo, G.; Drake, F.D. Occultation Experiment: Results of the First Direct Measurement of Mars’s Atmosphere and Ionosphere. Science 1965, 149, 1243–1248. [Google Scholar] [CrossRef]
- Fjeldbo, G.; Fjeldbo, W.C.; Eshleman, V.R. Models for the Atmosphere of Mars based on the Mariner 4 Occultation Experiment. J. Geophys. Res. 1966, 71, 2307–2316. [Google Scholar] [CrossRef]
- Kliore, A.J. Radio Occultation Exploration of Mars. Symp. Int. Astron. Union 2015, 65, 295–316. [Google Scholar] [CrossRef]
- Hinson, D.P.; Simpson, R.A.; Twicken, J.D.; Tyler, G.L.; Flasar, F.M. Initial results from radio occultation measurements with Mars Global Surveyor. J. Geophys. Res. Planets 1999, 104, 26997–27012. [Google Scholar] [CrossRef]
- Tyler, G.L.; Balmino, G.; Hinson, D.P.; Sjogren, W.L.; Smith, D.E.; Simpson, R.A.; Asmar, S.W.; Priest, P.; Twicken, J.D. Radio science observations with Mars Global Surveyor: Orbit insertion through one Mars year in mapping orbit. J. Geophys. Res. Planets 2001, 106, 23327–23348. [Google Scholar] [CrossRef]
- Hinson, D.P.; Smith, M.D.; Conrath, B.J. Comparison of atmospheric temperatures obtained through infrared sounding and radio occultation by Mars Global Surveyor. J. Geophys. Res. Planets 2004, 109, E12002. [Google Scholar] [CrossRef]
- McCleese, D.J.; Haskins, R.D.; Schofield, J.T.; Zurek, R.W.; Leovy, C.B.; Paige, D.A.; Taylor, F.W. Atmosphere and climate studies of Mars using the Mars Observer pressure modulator infrared radiometer. J. Geophys. Res. Planets 1992, 97, 7735–7757. [Google Scholar] [CrossRef]
- Zurek, R.W.; Smrekar, S.E. An overview of the Mars Reconnaissance Orbiter (MRO) science mission. J. Geophys. Res. Planets 2007, 112, E05S01. [Google Scholar] [CrossRef]
- Ao, C.O.; Edwards, C.D.; Kahan, D.S.; Pi, X.; Asmar, S.W.; Mannucci, A.J. A first demonstration of Mars crosslink occultation measurements. Radio Sci. 2015, 50, 997–1007. [Google Scholar] [CrossRef]
- Hu, X.; Wu, X.C.; Song, S.L.; Ma, M.L.; Zhou, W.L.; Xu, Q.C.; Li, L.; Xiao, C.Y.; Li, X.; Wang, C.; et al. First Observations of Mars Atmosphere and Ionosphere with Tianwen-1 Radio-Occultation Technique on 5 August 2021. Remote Sens. 2022, 14, 2718. [Google Scholar] [CrossRef]
- Gröller, H.; Montmessin, F.; Yelle, R.V.; Lefèvre, F.; Forget, F.; Schneider, N.M.; Koskinen, T.T.; Deighan, J.; Jain, S.K. MAVEN/IUVS Stellar Occultation Measurements of Mars Atmospheric Structure and Composition. J. Geophys. Res. Planets 2018, 123, 1449–1483. [Google Scholar] [CrossRef]
- Kirchengast, G.; Bernath, P.; Buehler, S. ACCURATE–climate benchmark profiling of greenhouse gases and thermodynamic variables and wind from space (ESA Earth Explorer opportunity mission EE-8 proposal). In Proceedings of the ESA Earth Explorer Opportunity Mission EE8 Proposal, Scientific Report 36–2010, Graz, Austria, 6–11 September 2010; Available online: https://wegccon.uni-graz.at/opac2010/pdf_presentation/opac_2010_kirchengast_gottfried_presentation57.pdf (accessed on 29 October 2024).
- Trainer, M.G.; Wong, M.H.; McConnochie, T.H.; Franz, H.B.; Atreya, S.K.; Conrad, P.G.; Lefèvre, F.; Mahaffy, P.R.; Malespin, C.A.; Manning, H.L.K.; et al. Seasonal Variations in Atmospheric Composition as Measured in Gale Crater, Mars. J. Geophys. Res. Planets 2019, 124, 3000–3024. [Google Scholar] [CrossRef]
- Mars Atmosphere Model. Available online: https://www.grc.nasa.gov/WWW/K-12/airplane/atmosmrm.html (accessed on 29 October 2024).
- Rothman, L.S.; Jacquemart, D.; Barbe, A.; Chris Benner, D.; Birk, M.; Brown, L.R.; Carleer, M.R.; Chackerian, C.; Chance, K.; Coudert, L.H.; et al. The HITRAN 2004 molecular spectroscopic database. J. Quant. Spectrosc. Radiat. Transf. 2005, 96, 139–204. [Google Scholar] [CrossRef]
- Langford, J.I. A rapid method for analysing the breadths of diffraction and spectral lines using the Voigt function. J. Appl. Crystallogr. 1978, 11, 10–14. [Google Scholar] [CrossRef]
- Dickinson, C.; Whiteway, J.A.; Komguem, L.; Moores, J.E.; Lemmon, M.T. Lidar measurements of clouds in the planetary boundary layer on Mars. Geophys. Res. Lett. 2010, 37, L18203. [Google Scholar] [CrossRef]
- Komguem, L.; Whiteway, J.A.; Dickinson, C.; Daly, M.; Lemmon, M.T. Phoenix LIDAR measurements of Mars atmospheric dust. Icarus 2013, 223, 649–653. [Google Scholar] [CrossRef]
- Jiang, P.; Yuan, J.; Wu, K.; Wang, L.; Xia, H. Turbulence Detection in the Atmospheric Boundary Layer Using Coherent Doppler Wind Lidar and Microwave Radiometer. Remote Sens. 2022, 14, 2951. [Google Scholar] [CrossRef]
- Shen, X.; Kong, W.; Chen, T.; Liu, Y.; Huang, G.; Shu, R. An inter-satellite laser occultation method profiling atmospheric temperature and pressure from troposphere to lower mesosphere. J. Quant. Spectrosc. Radiat. Transf. 2024, 328, 109174. [Google Scholar] [CrossRef]
- Bertaux, J.-L.; Fonteyn, D.; Korablev, O.; Chassefière, E.; Dimarellis, E.; Dubois, J.P.; Hauchecorne, A.; Cabane, M.; Rannou, P.; Levasseur-Regourd, A.C.; et al. The study of the Martian atmosphere from top to bottom with SPICAM light on Mars Express. Planet. Space Sci. 2000, 48, 1303–1320. [Google Scholar] [CrossRef]
- López-Valverde, M.A.; Funke, B.; Brines, A.; Stolzenbach, A.; Modak, A.; Hill, B.; González-Galindo, F.; Thomas, I.; Trompet, L.; Aoki, S.; et al. Martian Atmospheric Temperature and Density Profiles During the First Year of NOMAD/TGO Solar Occultation Measurements. J. Geophys. Res. Planets 2023, 128, e2022JE007278. [Google Scholar] [CrossRef]






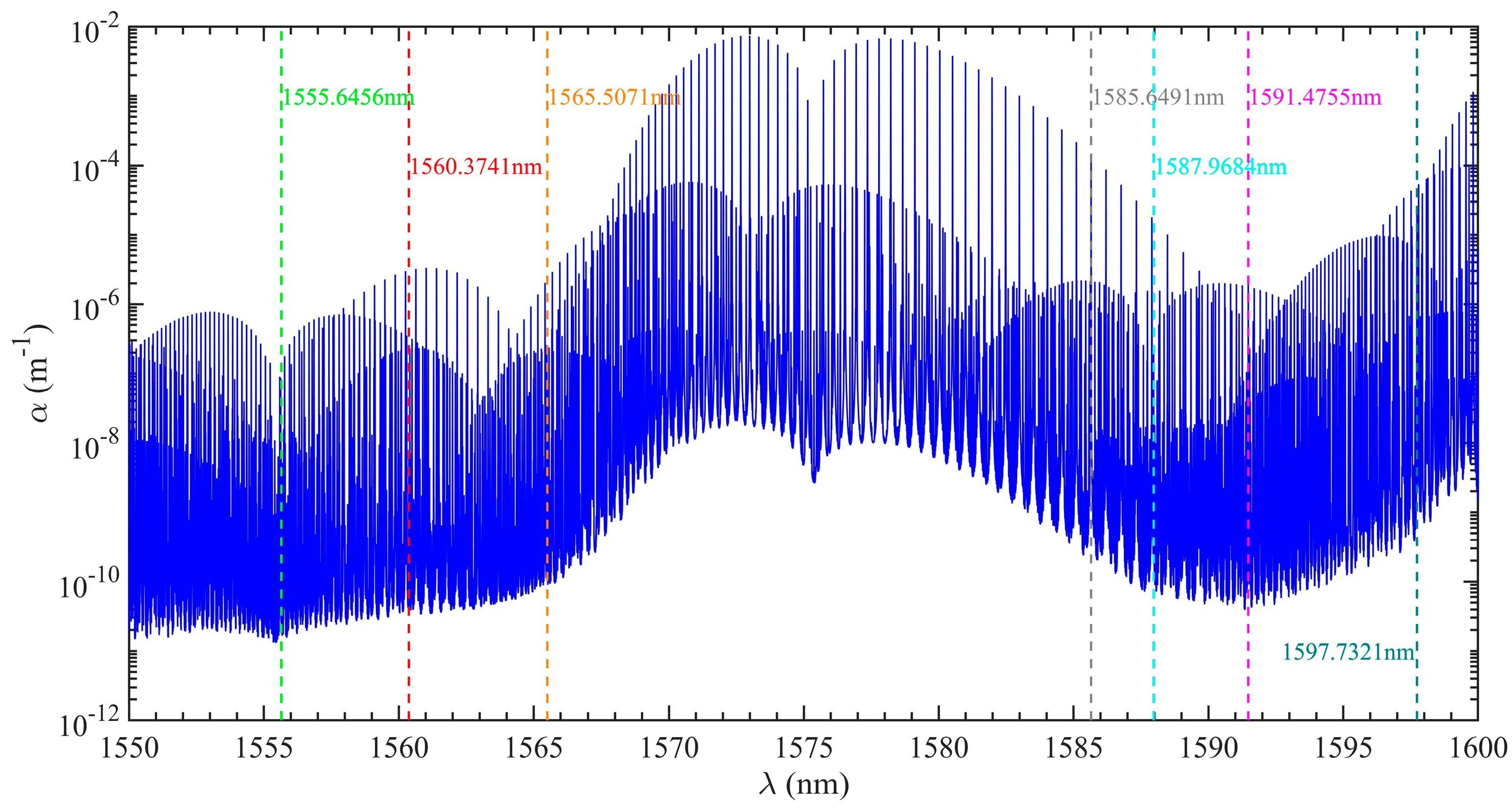
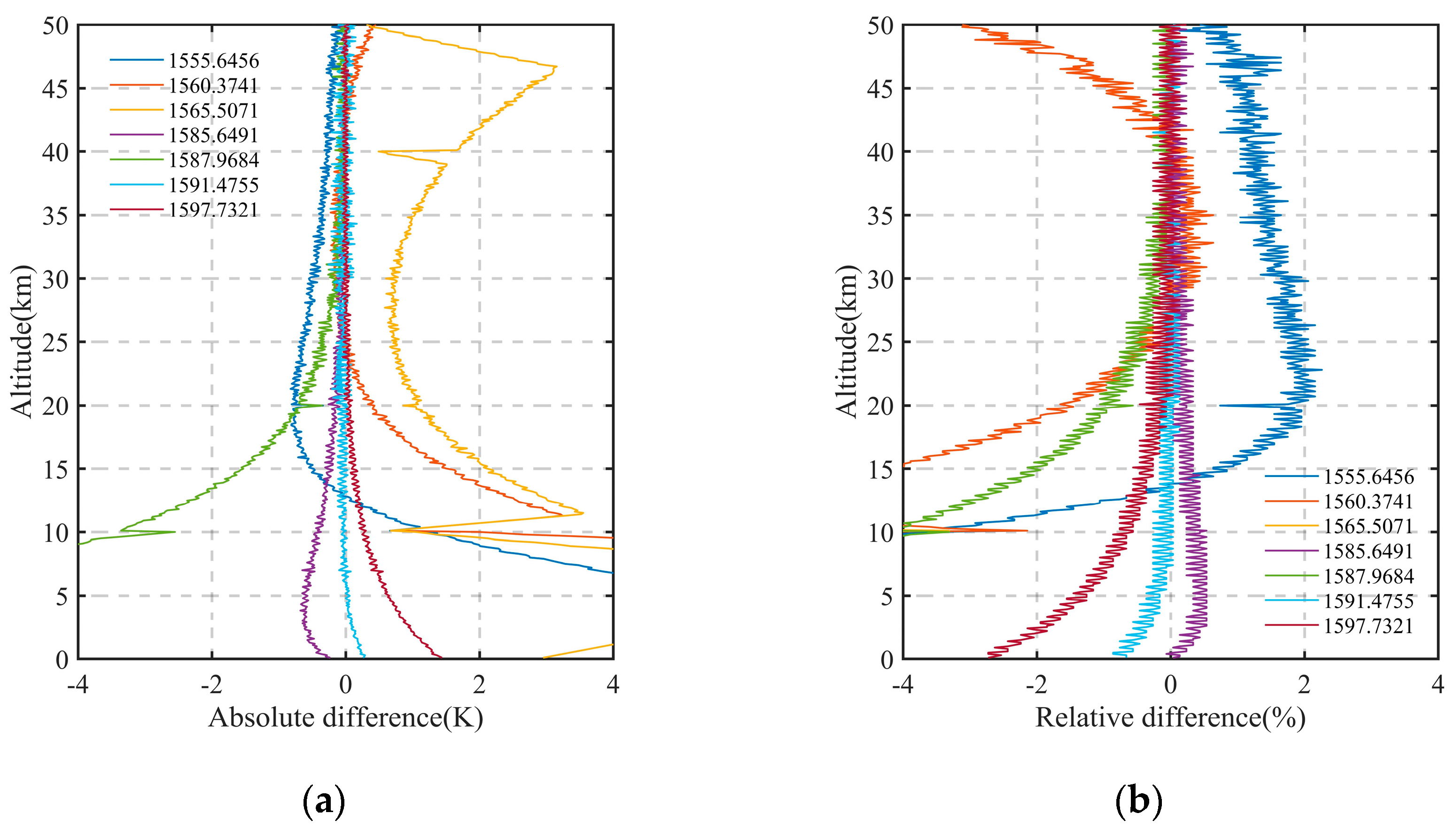
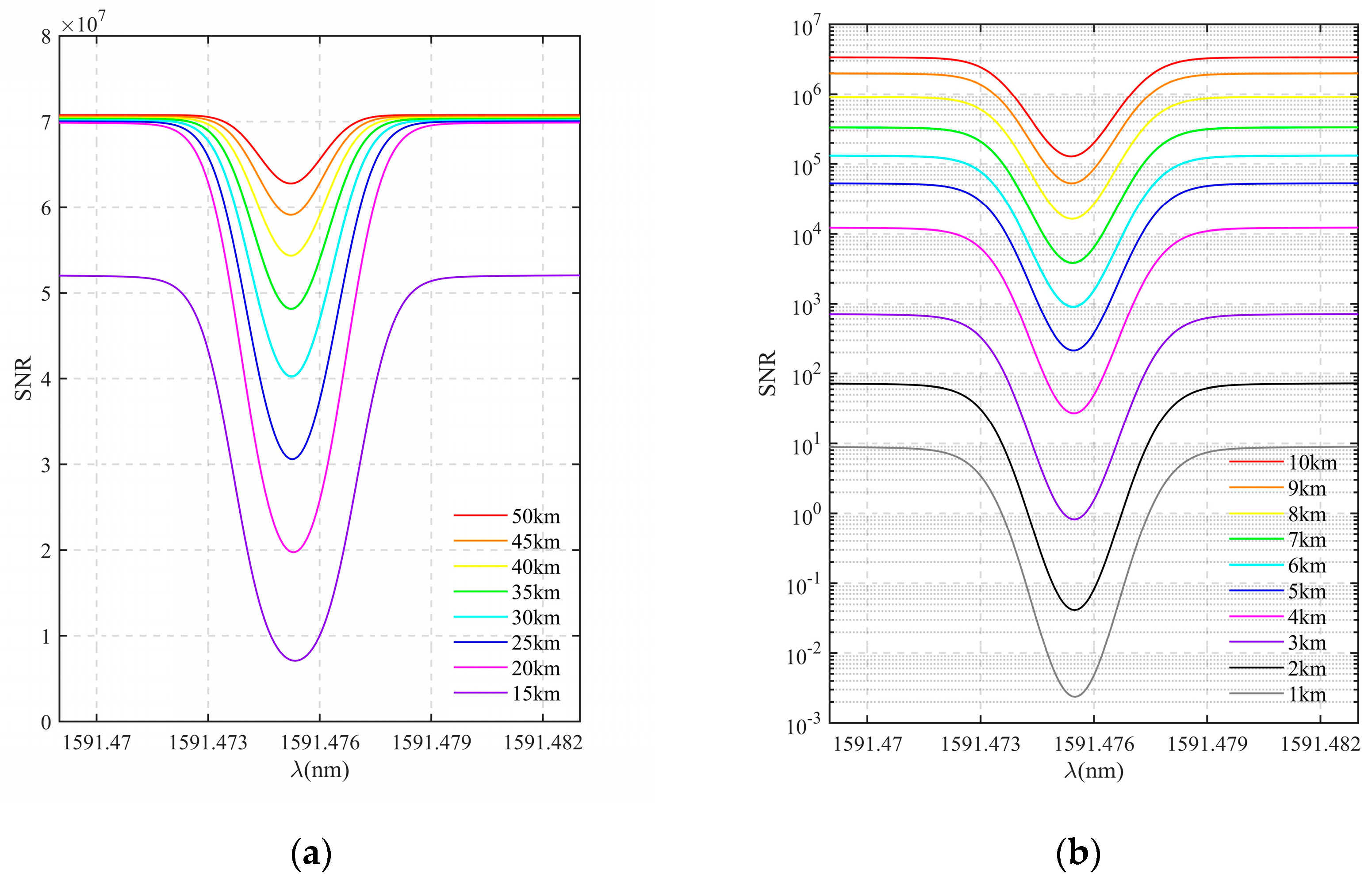
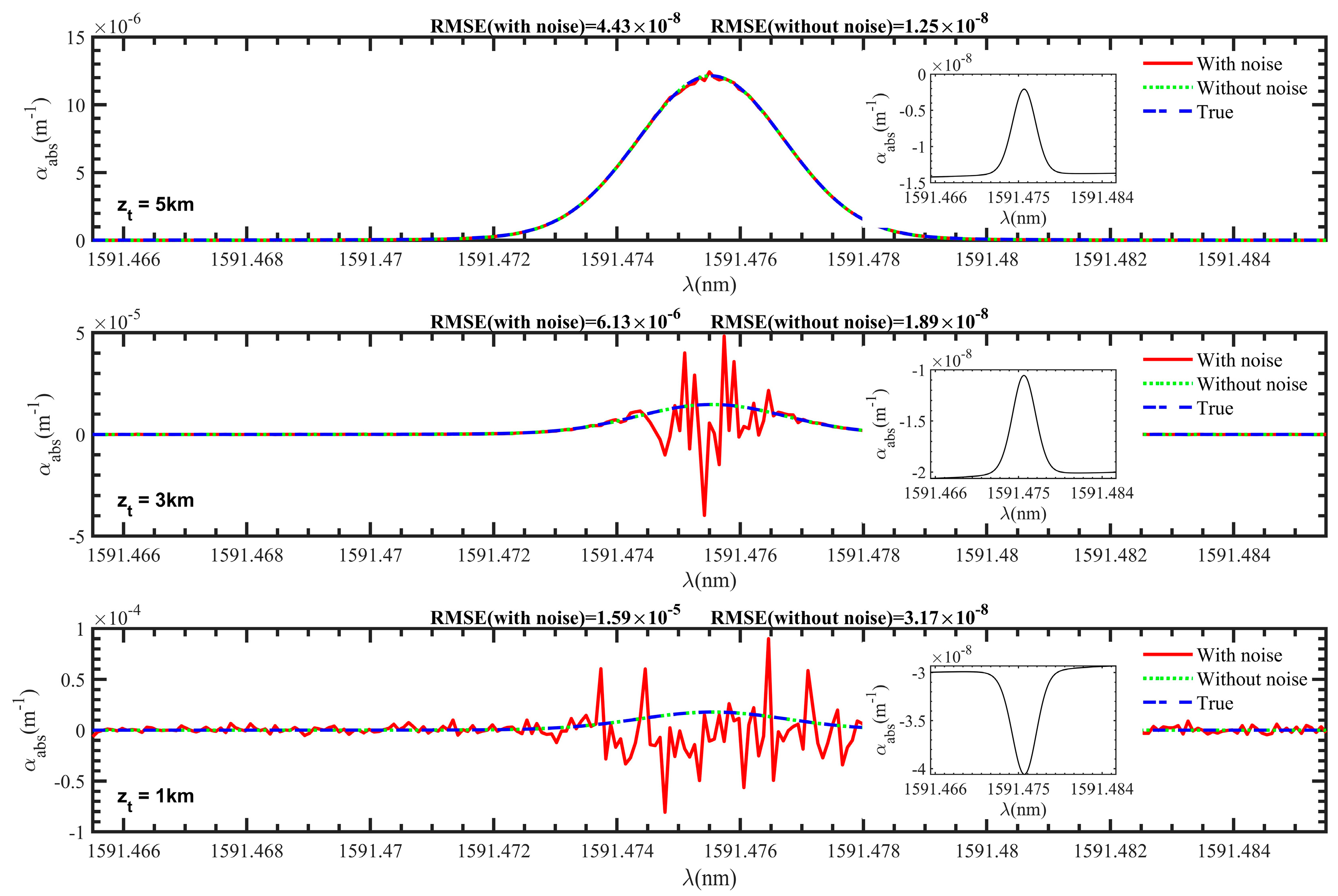
| System Parameters | Value (Unit) |
|---|---|
| Laser power | 1 kW |
| Laser divergence angle | 10 μrad |
| Laser pulse time width | 1 μs |
| Laser repetition rate | 200 kHz |
| Laser wavelength | 1.55–1.60 μm |
| Transmitting system optical diameter | 0.3 m |
| Transmitting system efficiency | 90% |
| Receiving telescope diameter | 0.3 m |
| Receiving system efficiency | 40% |
| Field of view | 1 mrad |
| Detector bandwidth | 500 kHz |
| Detector noise equivalent density | 32 fW/ |
| Detector noise equivalent power | 0.02 nW |
| Technology | Reference Model | Detection Altitude | Vertical Resolution | Detection Accuracy | References |
|---|---|---|---|---|---|
| Radio occultation | Viking data | 1 km~50 km | 500 m | Temperature: 0.4 K~10 K Pressure: 0.4%~6% | [10,11] |
| TES data | 0 km~100 km | 10 km | Temperature: <2 K | [12] | |
| RS data | 10 km~100 km | 500 m | Pressure: 0.3%~5% | ||
| MARS GCM | 0 km~80 km | 5 km | Temperature: <2 K Pressure: 1%~2% | [13] | |
| Stellar occultation | SPICAM Light | 20 km~160 km | 1 km | Temperature: <5 K | [27] |
| 80 km~120 km | 2 km | Temperature: <5 K | |||
| LMD-MGCM | 20 km~90 km | 3 km | Temperature: 2 K~5 K | [28] | |
| Laser occultation | NASA model | 5 km~50 km | 100 m | Temperature: <0.43 K Pressure: <1.06% | This paper |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Kong, W.; Shen, X.; Chen, T.; Huang, G. Research on the Detection Method of Martian Atmospheric Temperature and Pressure Profile Based on Laser Occultation Technology. Remote Sens. 2025, 17, 87. https://doi.org/10.3390/rs17010087
Liu Y, Kong W, Shen X, Chen T, Huang G. Research on the Detection Method of Martian Atmospheric Temperature and Pressure Profile Based on Laser Occultation Technology. Remote Sensing. 2025; 17(1):87. https://doi.org/10.3390/rs17010087
Chicago/Turabian StyleLiu, Ye, Wei Kong, Xue Shen, Tao Chen, and Genghua Huang. 2025. "Research on the Detection Method of Martian Atmospheric Temperature and Pressure Profile Based on Laser Occultation Technology" Remote Sensing 17, no. 1: 87. https://doi.org/10.3390/rs17010087
APA StyleLiu, Y., Kong, W., Shen, X., Chen, T., & Huang, G. (2025). Research on the Detection Method of Martian Atmospheric Temperature and Pressure Profile Based on Laser Occultation Technology. Remote Sensing, 17(1), 87. https://doi.org/10.3390/rs17010087






