Suitability Index for the Placement of Solar Plants Based on Inequality Measurements and on Satellite Images
Abstract
1. Introduction
2. Material and Methods
2.1. Data
2.2. Methods Applied
2.2.1. Exceedance Probabilities and Interquartile Range
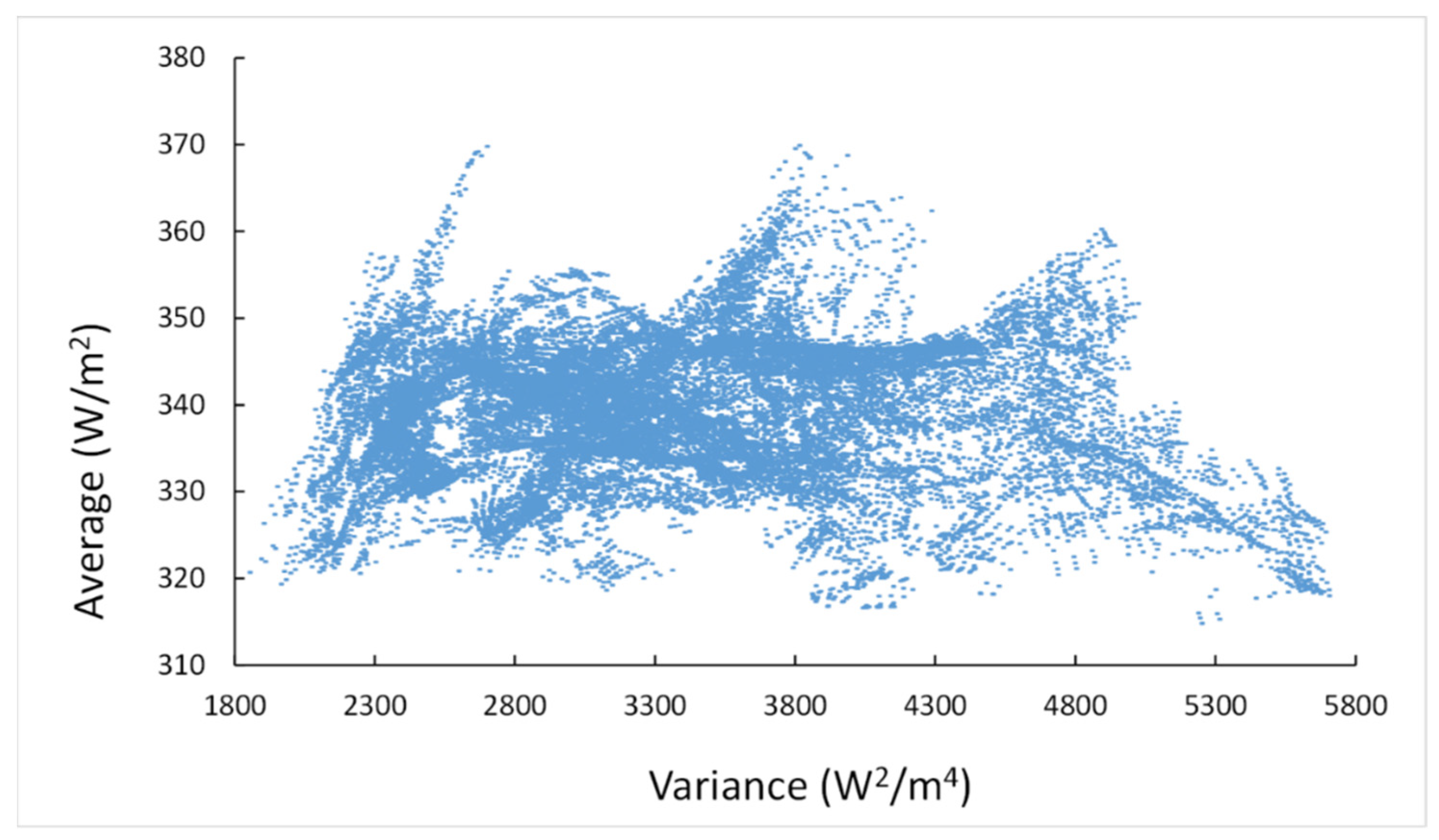
2.2.2. The Average and the Variance
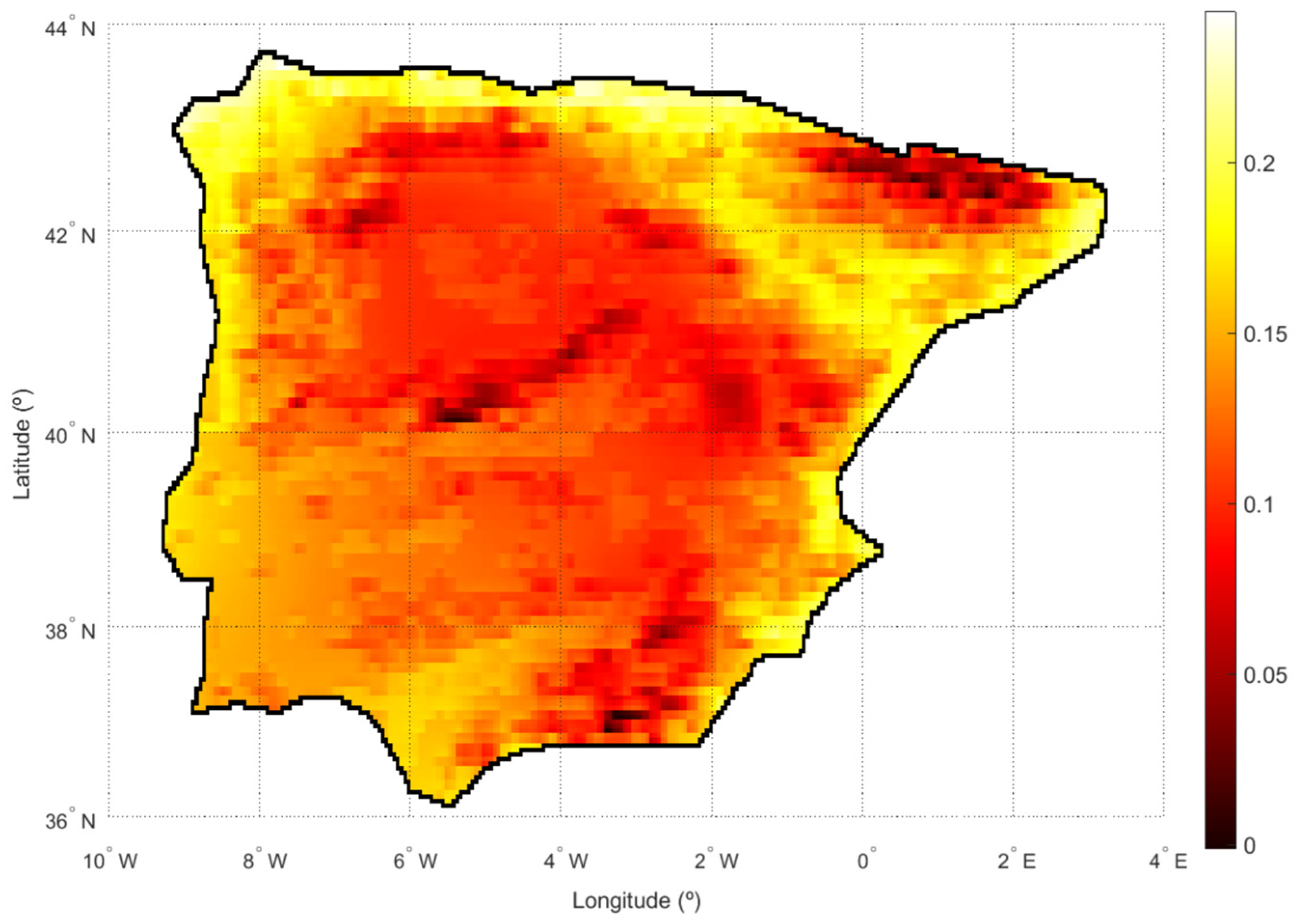
2.2.3. Suitability Index Based on Theil
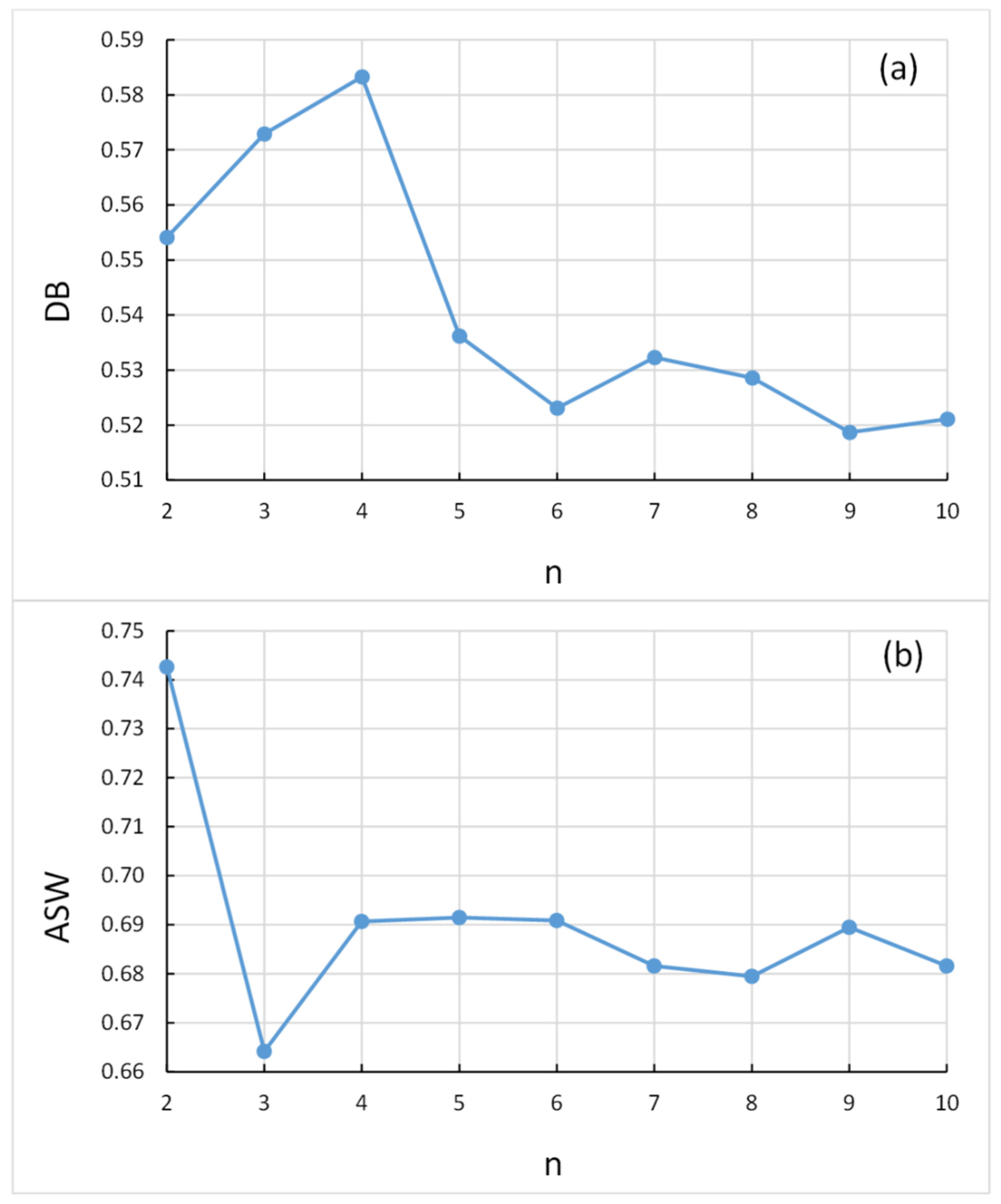
2.2.4. Clustering Analysis
- The Davies method
- The Silhouette method
2.2.5. Storage
3. Results
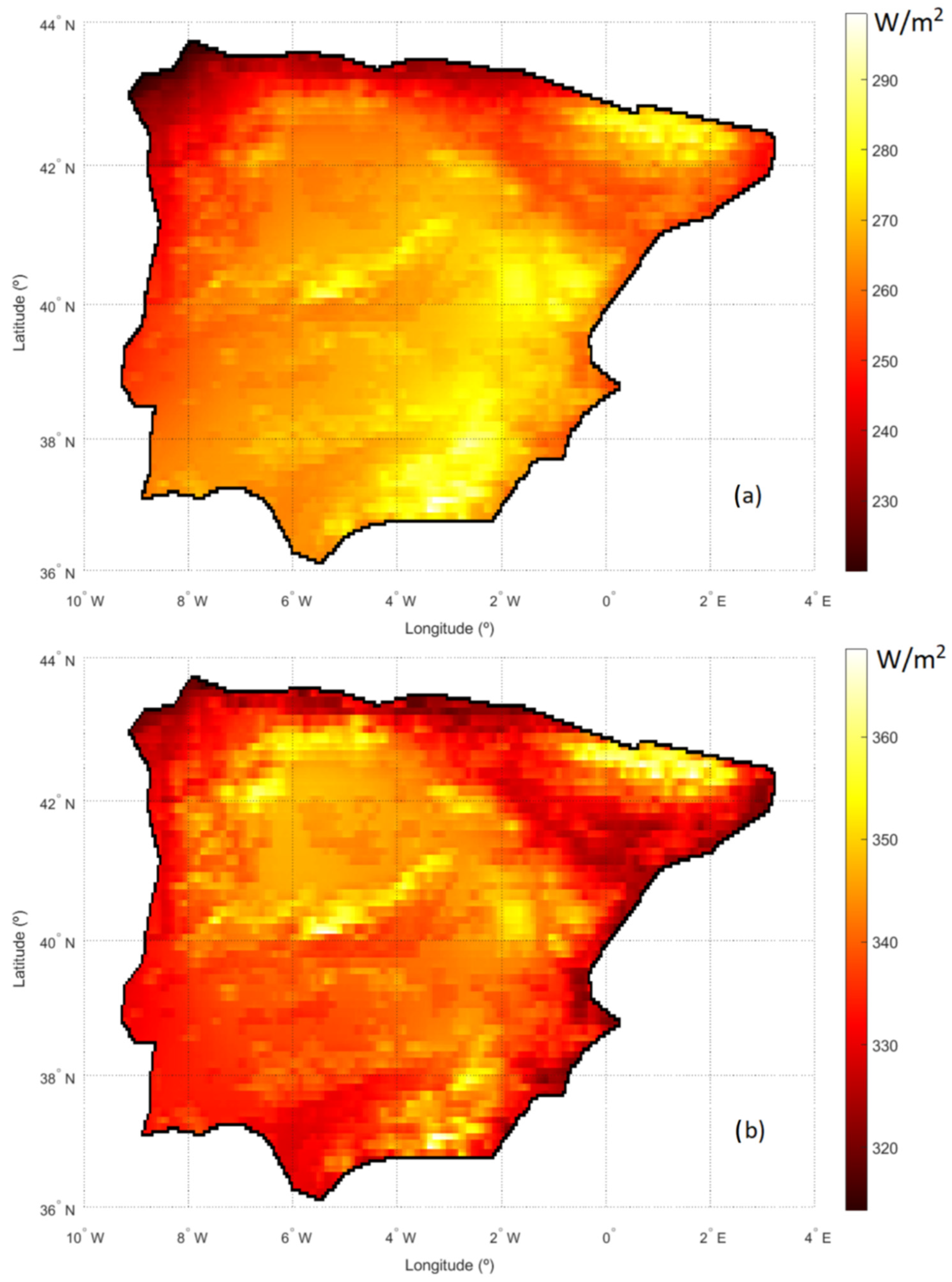
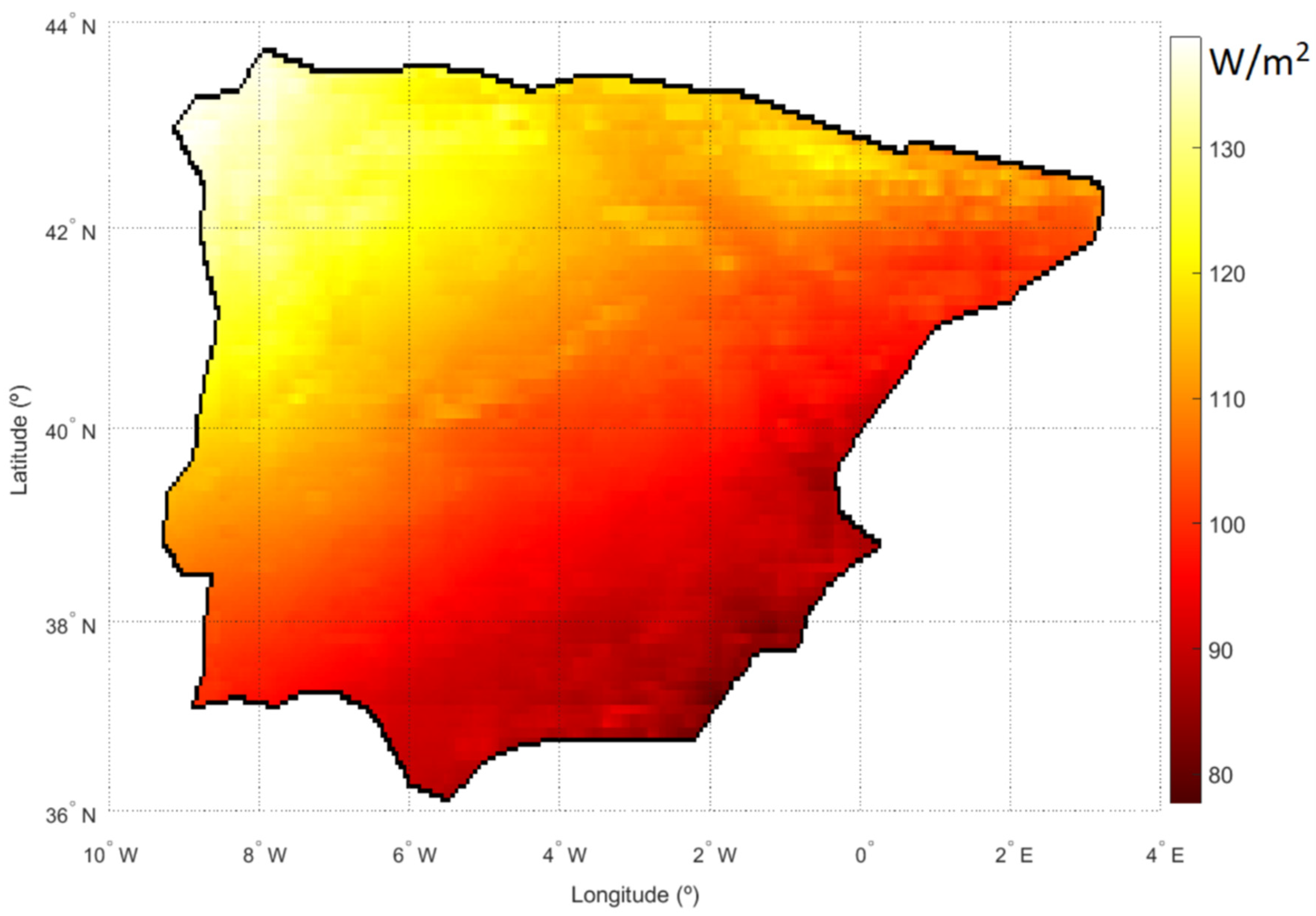
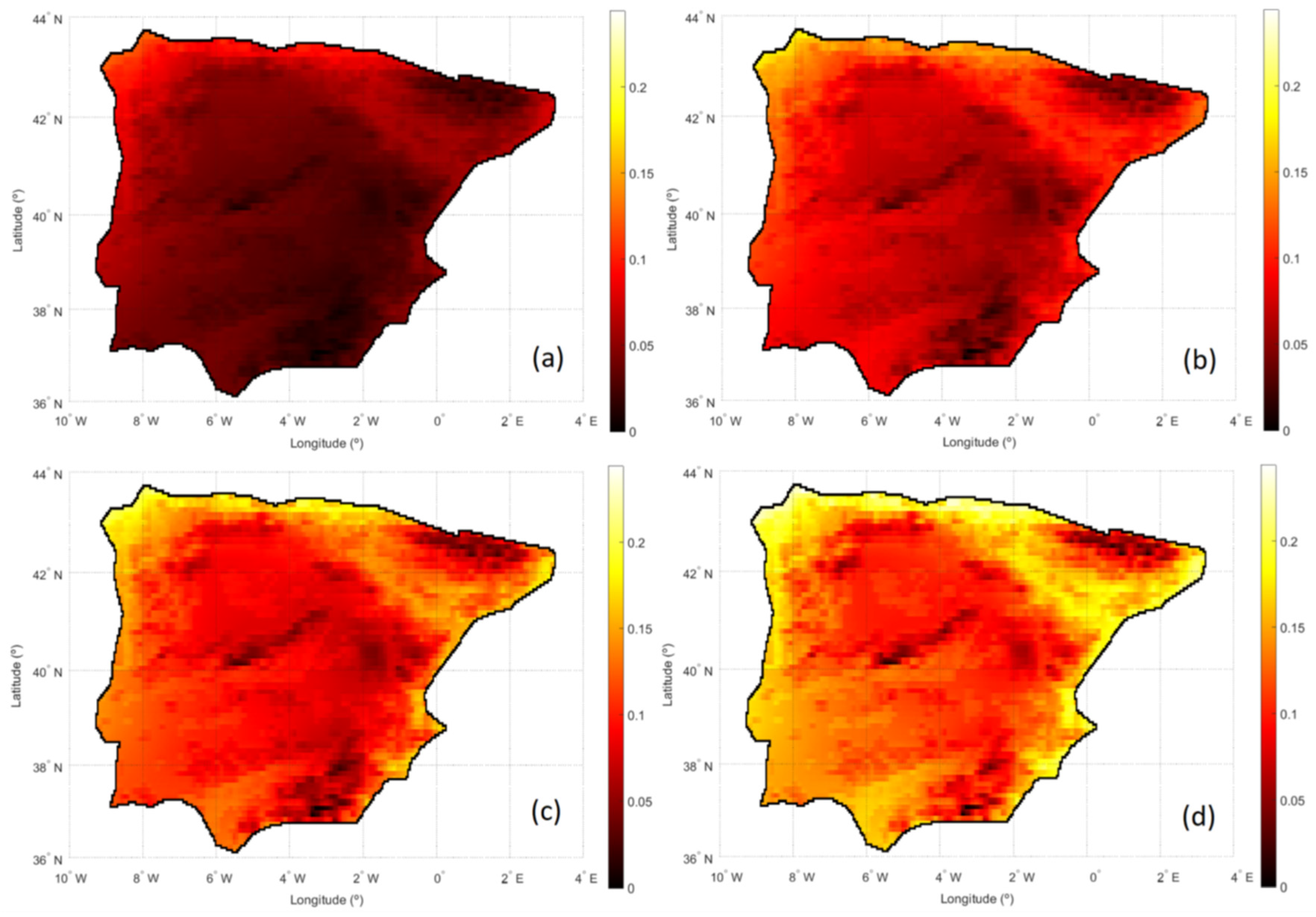
3.1. Exceedance Probabilities and Interquartile Range
3.2. Average and Variance
3.3. Suitability Index Based on Theil (SIT)
4. Discussion
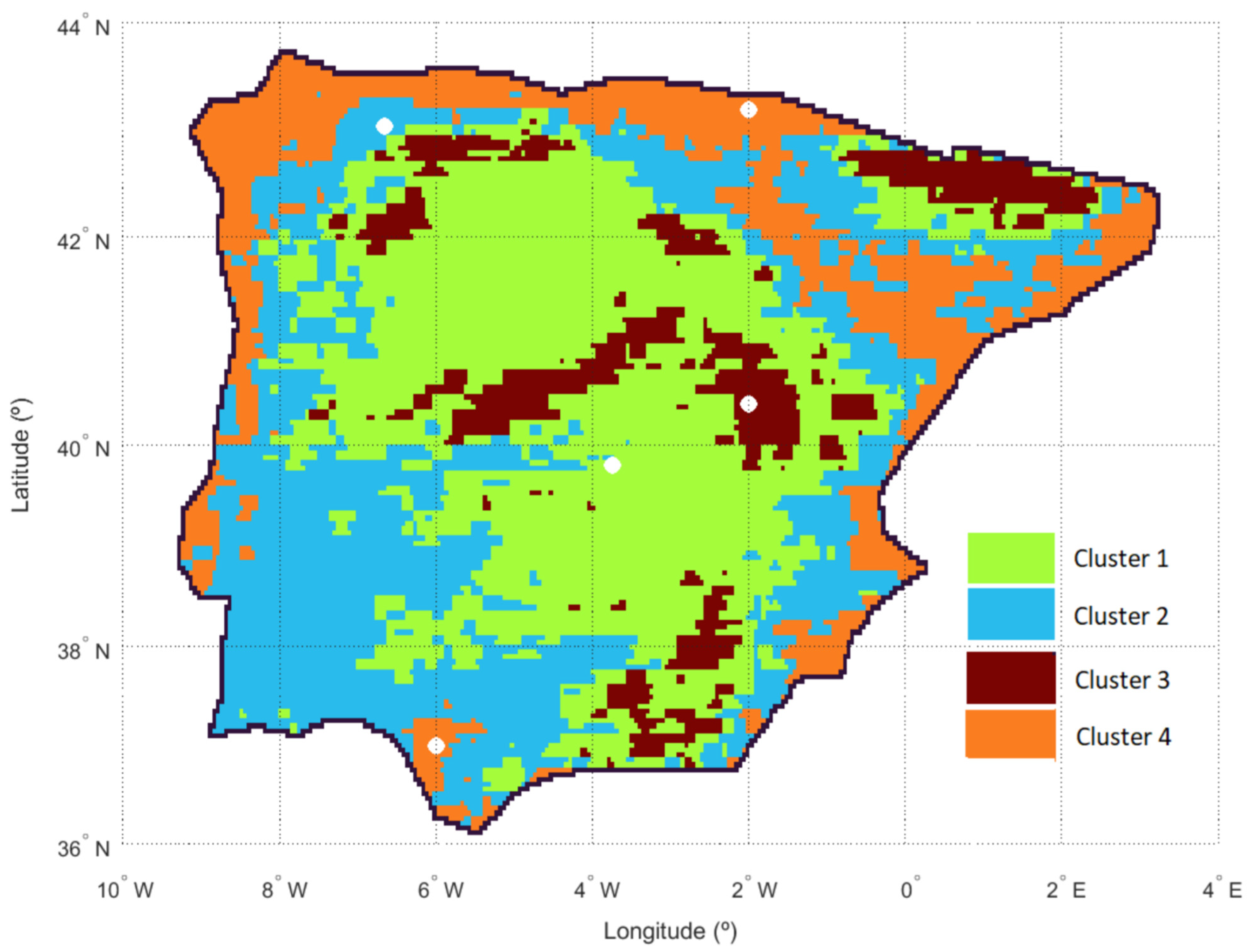
4.1. Clusters from the SIT
4.2. Analysis of the Selected Points
4.3. Assessment with Storage
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- World Bank Group. Solar Resource and Photovoltaic Potential of Indonesia; Energy Sector Management Assistance Program; World Bank Group: Washington, DC, USA, 2017; Available online: http://documents.worldbank.org/curated/en/729411496240730378/Solar-resource-and-photovoltaic-potential-of-Indonesia (accessed on 2 February 2024).
- World Bank Group. Solar Resource and Photovoltaic Potential of Myanmar; Energy Sector Management Assistance Program; World Bank Group: Washington, DC, USA, 2017; Available online: http://documents.worldbank.org/curated/en/509371496240201777/Solar-resource-and-photovoltaic-power-potential-of-Myanmar (accessed on 2 February 2024).
- Suehrcke, H.; McCormick, P. The frequency distribution of instantaneous insolation values. Sol. Energy 1988, 40, 413–422. [Google Scholar] [CrossRef]
- Olseth, J.A.; Skartveit, A. A probability density function for daily insolation within the temperate storm belts. Sol. Energy 1984, 33, 533–542. [Google Scholar] [CrossRef]
- Skartveit, A.; Olseth, J. The probability density and autocorrelation of short-term global and beam irradiance. Sol. Energy 1992, 49, 477–487. [Google Scholar] [CrossRef]
- Stein, J.S.; Hansen, C.W.; Reno, M.J. The Variability Index: A New and Novel Metric for Quantifying Irradiance and PV Output Variability. In Proceedings of the World Renewable Energy Forum, Denver, CO, USA, 13–17 May 2012. [Google Scholar]
- Peerlings, E. Cloud Gazing and Catching the Sun’s Rays: Quantifying Cloud Caused Variability, In Solar Irradiance. Master’s Thesis, Wageningen University, Wageningen, The Netherlands, 9 March 2019. [Google Scholar]
- Dobos, A.; Gilman, P.; Kasberg, M. P50/P90 analysis for solar energy systems using the system advisor model. In Proceedings of the World Renewable Energy Forum, Denver, CO, USA, 13–17 May 2012. [Google Scholar]
- Vindel, J.M.; Trincado, E. Discontinuity in the Production Rate Due to the Solar Resource Intermittency. J. Clean. Prod. 2021, 321, 128976. [Google Scholar] [CrossRef]
- Ratings, F. Rating Criteria for Solar Power Projects, Utility-Scale Photovoltaic and Concentrating Solar Power. Fitch Ratings. 2011. Available online: www.fitchratings.com (accessed on 1 November 2023).
- Vignola, F.; McMahan, A.; Grover, C. Chapter 5. Bankable solar-radiation datasets. In Solar Energy Forecasting and Resource Assessment; Elsevier: Amsterdam, The Netherlands, 2013; Volume 131, p. 97. [Google Scholar] [CrossRef]
- Gueymard, C.; Wilcox, S.M. Spatial and temporal variability in the solar resource: Assessing the value of short-term measurements at potential solar power plant sites. In Proceedings of the Solar Conference 2009, Buffalo, NY, USA, 11–16 May 2009. [Google Scholar]
- Fernández-Peruchena, C.M.; Gastón, M. A simple and efficient procedure for increasing the temporal resolution of global horizontal solar irradiance series. Renew. Energy 2016, 86, 375–383. [Google Scholar] [CrossRef]
- Polo, J.; Fernández-Peruchena, C.; Salamalikis, V.; Mazorra-Aguiar, L.; Turpin, M.; Martín-Pomares, L.; Kazantzidis, A.; Blanc, P.; Remund, J. Benchmarking on improvement and site-adaptation techniques for modelled solar radiation datasets. Sol. Energy 2020, 201, 469–479. [Google Scholar] [CrossRef]
- Fernández-Peruchena, C.M.; Polo, J.; Martín, L.; Mazorra, L. Site-Adaptation of modelled solar radiation data: The site adapt procedure. Remote Sens. 2020, 12, 2127. [Google Scholar] [CrossRef]
- Hoyer-Klick, C.; Beyer, H.G.; Dumortier, D.; Schroedter Homscheidt, M.; Wald, L.; Martinoli, M.; Schillings, C.; Gschwind, B.; Menard, L.; Gaboardi, E.; et al. MESoR e management and exploitation of solar resource knowledge. In Proceedings of the solarPACES, Berlin, Germany, 15–18 September 2009. [Google Scholar]
- Vignola, F.; Harlan, P.; Perez, R.; Kmiecik, M. Analysis of satellite derived beam and global solar radiation data. Sol. Energy 2007, 81, 768–772. [Google Scholar] [CrossRef]
- Zelenka, A.; Perez, R.; Seals, R.; Renne, D. Effective accuracy of satellite-derived hourly irradiances. Theor. Appl. Climatol. 1999, 62, 199–207. [Google Scholar] [CrossRef]
- Cano, D.; Monget, J.M.; Aubuisson, M.; Guillard, H.; Regas, N.; Wald, L. A method for the determination of the global solar radiation from meteorological satellite data. Sol. Energy 1986, 37, 631–639. [Google Scholar] [CrossRef]
- Moser, W.; Raschke, E. Mapping of global radiation and of cloudiness from METEOSAT image data. Theory and ground truth comparisons. Meteorol. Rundsch. 1983, 36, 33–41. [Google Scholar]
- Gautier, C.; Diak, G.; Masse, S. A simple physical model to estimate incident solar radiation at the surface from GOES satellite data. J. Appl. Meteorol. 1980, 19, 1005–1012. [Google Scholar] [CrossRef]
- Yuzer, E.O.; Bozkurt, A. Deep learning model for regional solar radiation estimation using satellite images. Ain Shams Eng. J. 2003, 14, 10205. [Google Scholar] [CrossRef]
- López, M.; Aler, R.; Galván, I.M.; Rodríguez, F.J.; Pozo, A.D. Improving Solar Radiation Nowcasts by Blending Data-Driven, Satellite-Images-Based and All-Sky-Imagers-Based Models Using Machine Learning Techniques. Remote Sens. 2003, 15, 2328. [Google Scholar] [CrossRef]
- Verbois, H.; Saint-Drenan, Y.-M.; Libois, Q.; Michel, Y.; Cassas, M.; Dubus, L.; Blanc, P. Improvement of satellite-derived surface solar irradiance estimations using spatio-temporal extrapolation with statistical learning. Sol. Energy 2023, 258, 175–193. [Google Scholar] [CrossRef]
- Oliveti, G.; Arcuri, N.; Ruffolo, S. Effect of climatic variability on the performance of solar plants with interseasonal storage. Renew. Energy 2000, 19, 235–241. [Google Scholar] [CrossRef]
- Dohse, K.; Jürgens, U.; Nialsch, T. From “fordism” to “toyotism”? The social organization of the labor process in the Japanese automobile Industry. J. Politics Soc. 1985, 14, 115–146. [Google Scholar] [CrossRef]
- Bischoff, M.; Jahn, J. Economic objectives, uncertainties and decision making in the energy sector. J. Bus. Econ. 2016, 86, 85–102. [Google Scholar] [CrossRef]
- Harrouni, S.; Guessoum, A. Using fractal dimension to quantify long-range persistence in global solar radiation. Chaos Solit. Fractals 2009, 41, 1520–1530. [Google Scholar] [CrossRef]
- Bertok, B.; Bartos, A. Renewable energy storage and distribution scheduling for microgrids by exploiting recent developments in process network synthesis. J. Clean. Prod. 2020, 244, 118520. [Google Scholar] [CrossRef]
- Strongin, S.; Eugeni, F. The Cost of Uncertainty. Chic. Fed. Lett. 1991, 43, 1. Available online: https://login.bucm.idm.oclc.org/login?url=https://www-proquest-com.bucm.idm.oclc.org/docview/214569638?accountid=14514 (accessed on 2 February 2024).
- Libra, M.; Mrázek, D.; Tyukhov, I.; Severová, L.; Poulek, V.; Mach, J.; Šubrt, T.; Beránek, V.; Svoboda, R.; Sedláček, J. Reduced real lifetime of PV panels—Economic consequences. Sol. Energy 2023, 259, 229–234. [Google Scholar] [CrossRef]
- Dong, S.; Kremers, E.; Brucoli, M.; Rothman, R.; Brow, S. Improving the feasibility of household and community energy storage: A techno-enviro-economic study for the UK. Renew. Sustain. Energy Rev. 2020, 131, 110009. [Google Scholar] [CrossRef]
- Olczak, P.; Żelazna, A.; Stecuła, K.; Matuszewska, D.; Lelek, Ł. Environmental and economic analyses of different size photovoltaic installation in Poland. Energy Sustain. Dev. 2022, 70, 160–169. [Google Scholar] [CrossRef]
- Markowitz, H. Portfolio Selection: Efficient Diversification of Investments; Blackwell: Malden, MA, USA, 1991. [Google Scholar]
- Pfeifroth, U.; Kothe, S.; Müller, R.; Trentmann, J.; Hollmann, R.; Fuchs, P.; Werscheck, M. Surface Radiation Data Set—Heliosat (SARAH)—Edition 2, Satellite Application Facility on Climate Monitoring. 2017. Available online: https://wui.cmsaf.eu/safira/action/viewDoiDetails?acronym=SARAH_V002 (accessed on 1 March 2023).
- CM-SAF. Validation Report Meteosat Solar Surface Radiation and Effective Cloud Albedo Climate Data Record SARAH-2. 2016. Available online: https://www.cmsaf.eu/SharedDocs/Literatur/document/2016/saf_cm_dwd_val_meteosat_hel_2_1_pdf.html (accessed on 1 March 2023).
- CM-SAF. Algorithm Theoretical Baseline Document Meteosat Solar Surface Radiation and Effective Cloud Albedo Climate Data Records—Heliosat SARAH-2. 2017. Available online: https://wui.cmsaf.eu/safira/action/viewProduktSearch (accessed on 1 March 2023).
- Levy, J. Radical Uncertainty. Crit. Q. 2020, 62, 15–28. [Google Scholar] [CrossRef]
- Robicheck, A.A.N.; Myers, S.C. Conceptual problems in the use of risk-adjusted discount rates. J. Financ. 1996, 21, 727–730. [Google Scholar] [CrossRef]
- Copeland, D.E.; Weston, J.F. Financial Theory and Corporate Policy; Addison Wesley: Boston, MA, USA, 1983. [Google Scholar]
- Altimir, O.; Crivelli, A.; Piñera, S. Análisis de Descomposición. Una Generalización del Método de Theil, Development Research Center del Banco Mundial and Comisión Económica para América Latina. 1977. Available online: https://hdl.handle.net/11362/32365 (accessed on 2 February 2024).
- Gini, C. On the Measure of Concentration with Special Reference to Income and Statistics; General Series; Colorado College Publication: Denver, CO, USA, 1936; Volume 208, pp. 73–79. [Google Scholar]
- Theil, H. Statistical Decomposition Analysis: With Applications in the Social and Administrative Sciences, Studies in Mathematical and Managerial Economics; North-Holland Publishing: Amsterdam, The Netherlands, 1972; Volume 14. [Google Scholar]
- Theil, H. More on Log-Change Index Numbers. Rev. Econ. Stat. 1974, 56, 552–554. [Google Scholar] [CrossRef]
- Rohde, N. Lorenz Curves and Generalised Entropy Inequality Measures. In Modeling Income Distributions and Lorenz Curves, Economic Studies in Equality, Social Exclusion and Well-Being; Chotikapanich, D., Ed.; Springer: New York, NY, USA, 2008; Volume 5, pp. 271–283. [Google Scholar]
- Shorrocks, A.F. The Class of Additively Decomposable Inequality Measures. Econometrica 1980, 48, 613–625. [Google Scholar] [CrossRef]
- Theil, H. Economics and Information Theory; North-Holland Pub. Co: Amsterdam, The Netherlands, 1967. [Google Scholar]
- Jin, X.; Han, J. K-Means Clustering. In Encyclopedia of Machine Learning; Sammut, C., Webb, G.I., Eds.; Springer: Boston, MA, USA, 2011. [Google Scholar] [CrossRef]
- Davies, D.L.; Bouldin, D.W. A cluster separation measure. IEEE Trans. Pattern Anal. Mach. Intell. 1979, PAMI-1, 224–227. [Google Scholar] [CrossRef]
- Rousseeuw, P.J. Silhouettes: A graphical aid to the interpretation and validation of cluster analysis. J. Comput. Appl. Math 1987, 20, 53–65. [Google Scholar] [CrossRef]
- Walsh, M.; de la Torre, D.; Shapouri, H.; Slinksky, S. Bioenergy crop production in the United States. Environ. Resour. Econ. 2003, 24, 313–333. [Google Scholar] [CrossRef]
- Lankoski, J.; Ollikainen, M. Bioenergy Crop Production and Climate Policies: A von Thunen Model and the Case of Reed Canary Grass in Finland. Eur. Rev. Agric. Econ. 2008, 35, 519–546. [Google Scholar] [CrossRef]
- Von Thünen, J. The Isolated State in Relation to Agriculture and Political Economy; Palgrave Macmillan: London, UK, 2009. [Google Scholar]


| Latitude | Longitude | P10 | P50 | IQR | SIT |
|---|---|---|---|---|---|
| 43.05°N | 6.65°W | 251.2 | 341.0 | 127.0 | 0.133 |
| 39.8°N | 3.75°W | 266.0 | 338.2 | 99.9 | 0.123 |
| 40.4°N | 2°W | 278.2 | 350.8 | 101.8 | 0.071 |
| 43.2°N | 2°W | 240.1 | 326.2 | 116.2 | 0.199 |
| 37.0°N | 6°W | 262.3 | 329.3 | 91.2 | 0.166 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Trincado, E.; Vindel, J.M. Suitability Index for the Placement of Solar Plants Based on Inequality Measurements and on Satellite Images. Remote Sens. 2024, 16, 1039. https://doi.org/10.3390/rs16061039
Trincado E, Vindel JM. Suitability Index for the Placement of Solar Plants Based on Inequality Measurements and on Satellite Images. Remote Sensing. 2024; 16(6):1039. https://doi.org/10.3390/rs16061039
Chicago/Turabian StyleTrincado, Estrella, and Jose María Vindel. 2024. "Suitability Index for the Placement of Solar Plants Based on Inequality Measurements and on Satellite Images" Remote Sensing 16, no. 6: 1039. https://doi.org/10.3390/rs16061039
APA StyleTrincado, E., & Vindel, J. M. (2024). Suitability Index for the Placement of Solar Plants Based on Inequality Measurements and on Satellite Images. Remote Sensing, 16(6), 1039. https://doi.org/10.3390/rs16061039








