Unusual Sunrise and Sunset Terminator Variations in the Behavior of Sub-Ionospheric VLF Phase and Amplitude Signals Prior to the Mw7.8 Turkey Syria Earthquake of 6 February 2023
Abstract
1. Introduction
2. TBB VLF Amplitude and Phase Investigations
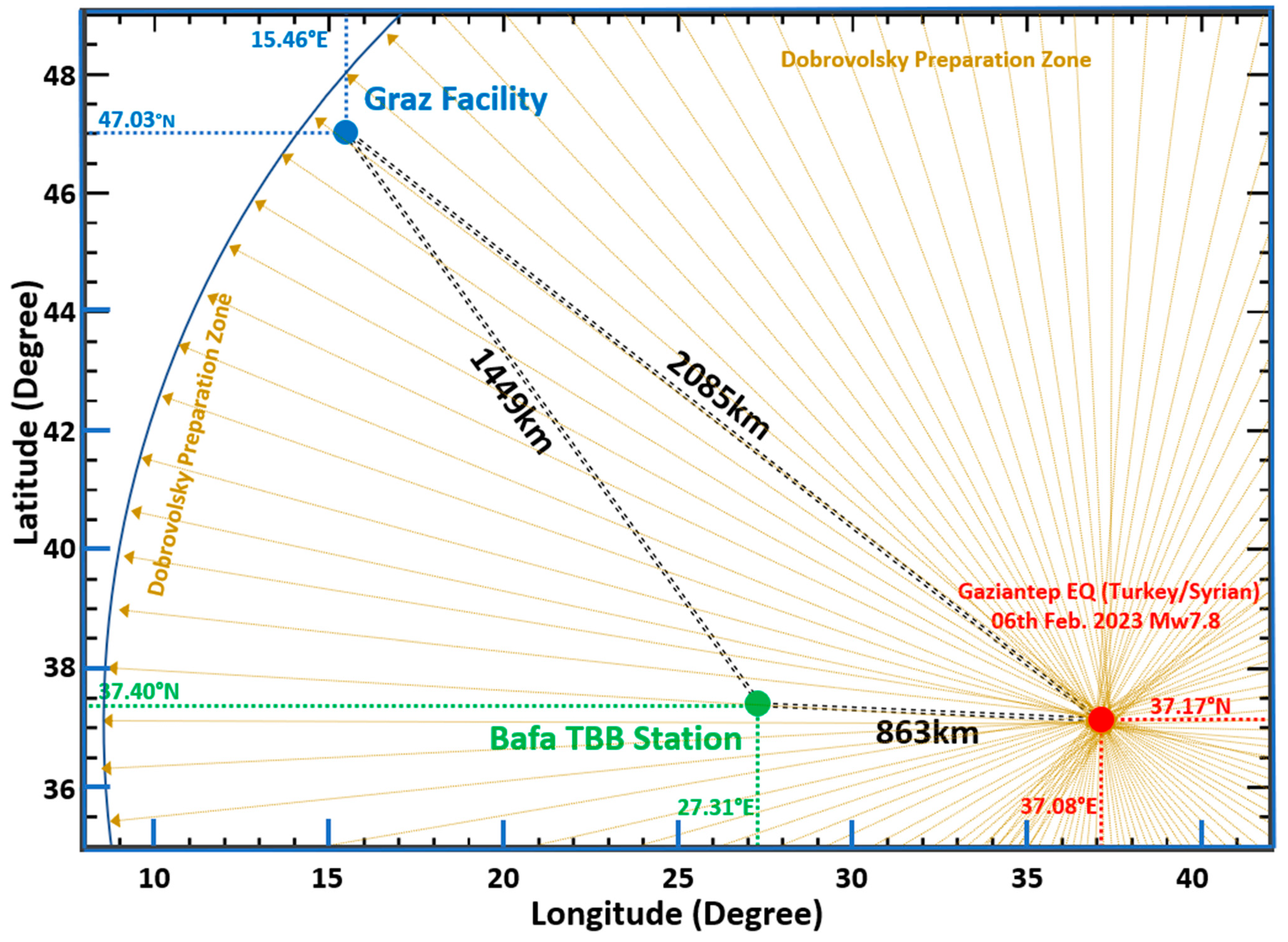
2.1. Features of Turkey–Syrian Earthquakes on 6 February 2023 and Dobrovolsky Preseismic Area
2.2. Overview Variations in the Amplitude and the Phase of TBB Transmitter Signal
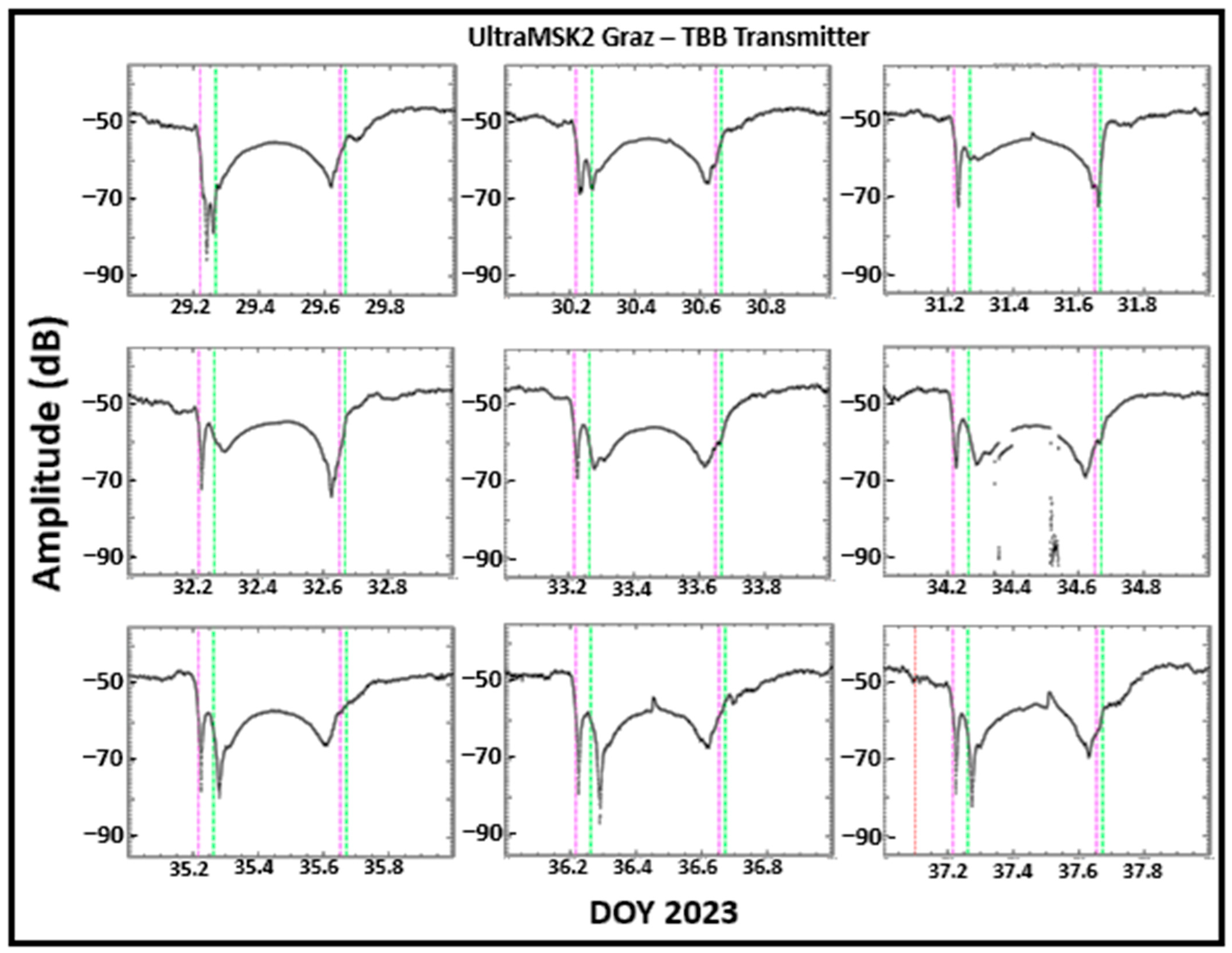
2.2.1. TBB Amplitude Variations
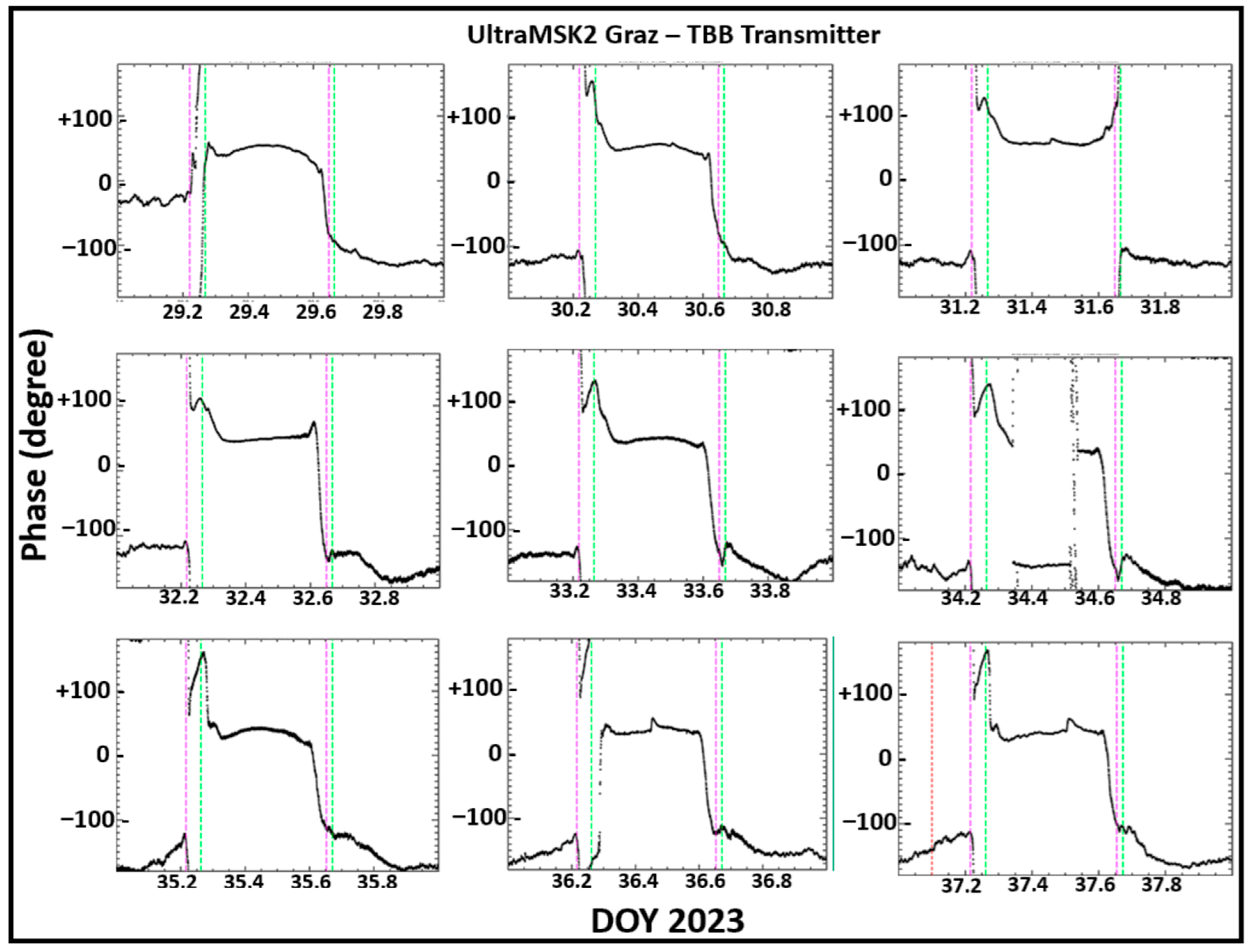
2.2.2. TBB Phase Variations
3. Time Shifts of Sunrise and Sunset Using Multi-Terminators Method (MTM)
3.1. Case Study of TBB Amplitude and Phase Variations as Recorded on 2 February 2023
3.1.1. Spectral Features Recorded on 2 February 2023
3.1.2. Terminator Method Versus Multi-Terminator Method
3.1.3. Reduction in Selected Minima and Maxima Anomalies for the Event of 2 February 2023
- one minimum close to the TBB transmitter sunrise terminator for the amplitude,
- one maximum close to the Graz station sunrise terminator for the amplitude,
- one minimum close to the EQ epicenter sunset terminator for the amplitude,
- one maximum close to the TBB transmitter sunset terminator for the amplitude,
- one minimum close to the TBB transmitter sunrise terminator for the phase,
- one maximum close to the Graz station sunrise terminator for the phase,
- one minimum close to the TBB transmitter sunset terminator for the phase,
- one maximum close to the Graz station sunset terminator for the phase.
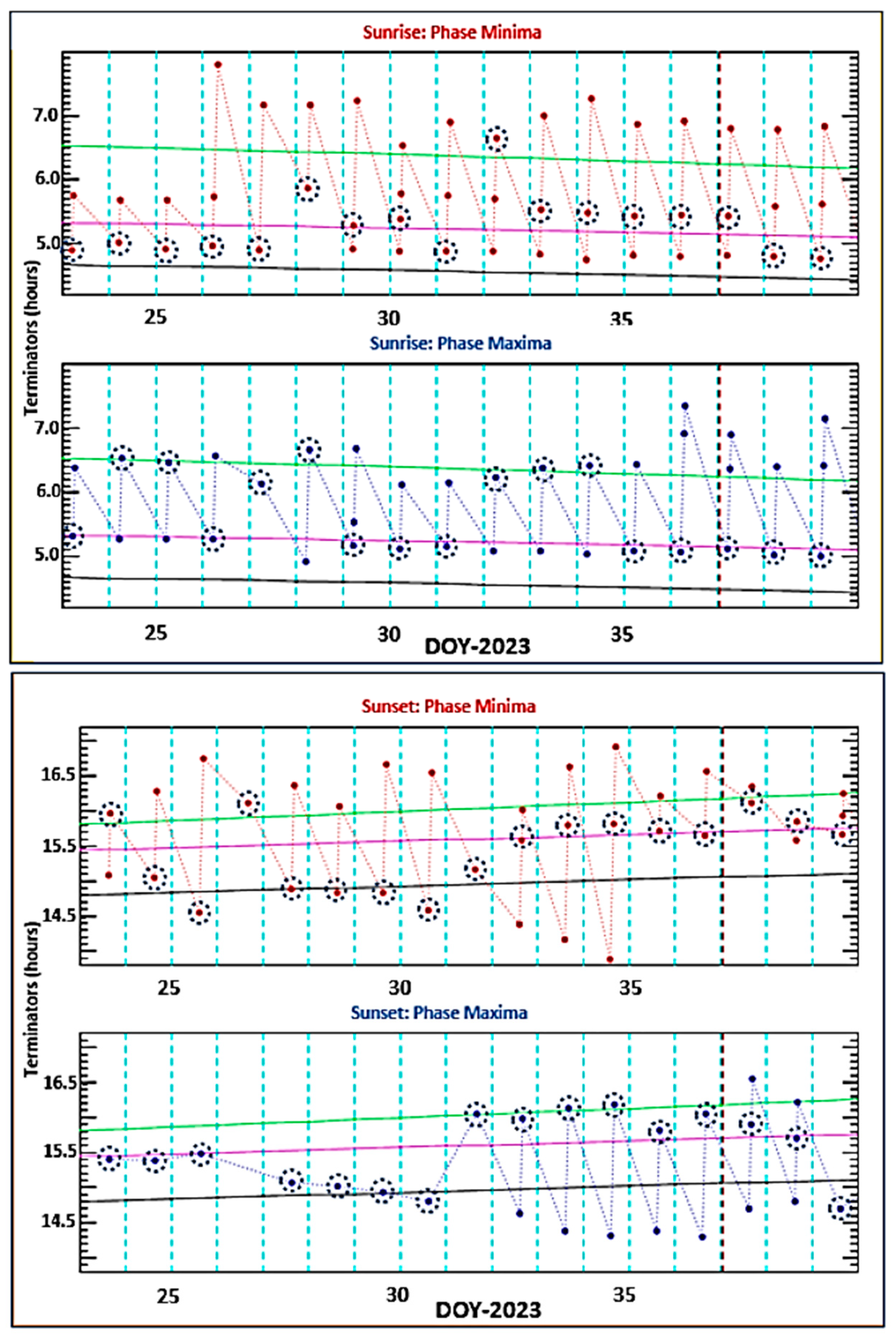
3.2. Anomalies in TBB Amplitude Signal at Sunrise and Sunset Terminators
3.3. Anomalies in TBB Phase Signal at Sunrise and Sunset Terminators
3.4. Summary of the Main Anomalies in Amplitude and Phase of TBB Signal
- Amplitude
- ➢
- Sunrise—Minima: EQ (41%), TB (47%) and GR (12%)
- ➢
- Sunrise—Maxima: EQ (07%), TB (41%) and GR (52%)
- ➢
- Sunset—Minima: EQ (70%), TB (07%) and GR (23%)
- ➢
- Sunset—Maxima: EQ (44%), TB (37%) and GR (29%)
- Phase
- ➢
- Sunrise—Minima: EQ (35%), TB (59%) and GR (06%)
- ➢
- Sunrise—Maxima: EQ (00%), TB (59%) and GR (41%)
- ➢
- Sunset—Minima: EQ (41%), TB (41%) and GR (18%)
- ➢
- Sunset—Maxima: EQ (31%), TB (38%) and GR (31%)
4. Discussion
4.1. Significant Terminator Anomalies Recorded by Graz Facility Are Those Linked to EQ Epicenter Regions
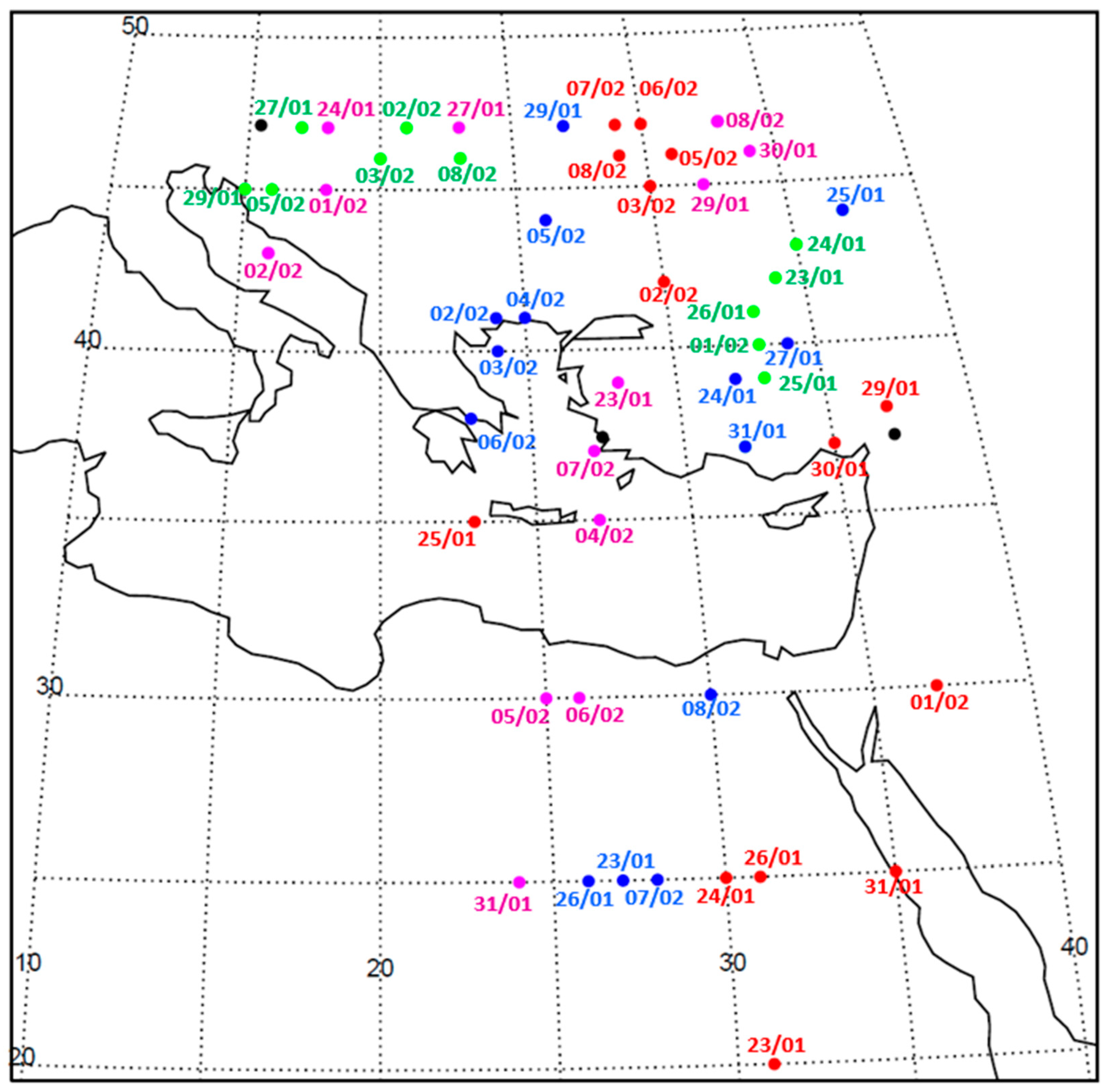
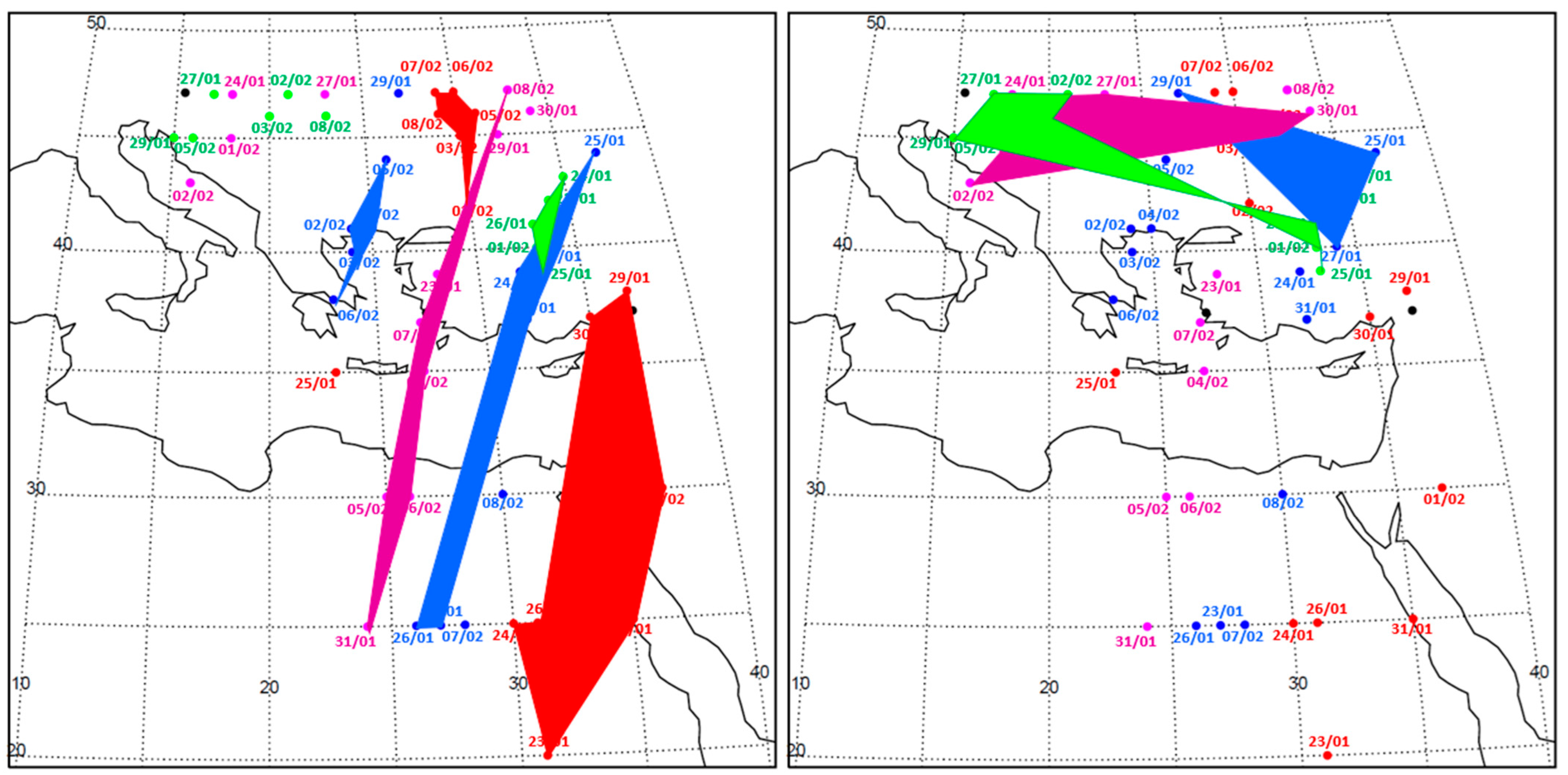
4.2. Geographical Longitude and Latitude Locations of Terminator Anomalies
- the amplitude for a given DOY (first column), the sunrise (second column), the sunset (third column), longitude (fourth column), and latitude (fifth column). Those points are shown in the upper-left panel of Figure 7.
- the phase for a given DOY (first column), the sunrise (sixth column), the sunset (seventh column), the longitude (eighth column), and latitude (ninth column). Those points are shown in the lower-left panel of Figure 7.
- the amplitude for a given DOY (first column), the sunrise (second column), the sunset (third column), longitude (fourth column), and latitude (fifth column). Those points are shown in the upper-right panel of Figure 7.
- the phase for a given DOY (first column), the sunrise (sixth column), the sunset (seventh column), the longitude (eighth column), and latitude (ninth column). Those points are shown in the lower-right panel of Figure 7.
4.3. Scenario of the Dynamics of the Radio Preparation Seismic Zone
4.4. Physical Mechanisms at the Origin of EQ Terminator Time Shift Anomalies
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Parrot, M.; Achache, J.; Berthelier, J.J.; Blanc, E.; Deschamps, A.; Lefeuvre, F.; Menvielle, M.; Plantet, J.L.; Tarits, P.; Villain, J.P. High-frequency seismo-electromagnetic effects. Phys. Earth Planet. Inter. 1993, 77, 65–83. [Google Scholar] [CrossRef]
- Hayakawa, M. Earthquake Prediction with Radio Techniques; John Wiley and Sons: Singapore, 2015; 296p. [Google Scholar]
- Cicerone, R.D.; Ebel, J.E.; Britton, J. A systematic compilation of earthquake precursors. Tectonophysics 2009, 476, 371–396. [Google Scholar] [CrossRef]
- Hayakawa, M. On the fluctuation spectra of seismo-electromagnetic phenomena. Nat. Hazards Earth Syst. Sci. 2011, 11, 301–308. [Google Scholar] [CrossRef]
- Yazdania, M.; Loosemoreb, M.; Mojtahedic, M.; Sandersonc, D.; Haghania, M. Progress and landscape of disaster science: In- sights from computational analyses. Int. J. Disaster Risk Reduct. 2024, 108, 104536. [Google Scholar] [CrossRef]
- Pulinets, S.A.; Boyarchuk, K. Ionospheric Precursors of Earthquakes; Springer: Berlin/Heidelberg, Germany, 2004; 315p. [Google Scholar]
- Pulinets, S.; Ouzounov, D. Lithosphere–atmosphere–ionosphere coupling (LAIC) model—A unified concept for earthquake precursors validation. J. Asian Earth Sci. 2011, 41, 371–382. [Google Scholar] [CrossRef]
- Pulinets, S.; Ouzounov, D.; Karelin, A.; Davidenko, D. Lithosphere–Atmosphere–Ionosphere–Magnetosphere coupling—A concept for pre-earthquake signals generation. In Pre-Earthquake Processes: A Multidisciplinary Approach to Earthquake Prediction Studies (Geophysical Monograph 234); Ouzounov, D., Pulinets, S., Hattori, K., Taylor, P., Eds.; American Geophysical Union: Washington, DC, USA; John Wiley and Sons: Hoboken, NJ, USA, 2018; pp. 79–98. [Google Scholar]
- Kuo, C.L.; Huba, J.D.; Joyce, G.; Lee, L.C. Ionosphere plasma bubbles and density variations induced by pre-earthquake rock currents and associated surface charges. J. Geophys. Res. 2011, 116, A10317. [Google Scholar] [CrossRef]
- Liu, J.Y.; Chuo, Y.J.; Shan, S.J.; Tsai, Y.B.; Chen, Y.I.; Pulinets, S.A.; Yu, S.B. Pre-earthquake ionospheric anomalies registered by continuous GPS TEC measurements. Ann. Geophys. 2004, 22, 1585–1593. [Google Scholar] [CrossRef]
- Freund, F. Earthquake forewarning—A multidisciplinary challenge from the ground up to space. Acta Geophys. 2013, 61, 775–807. [Google Scholar] [CrossRef]
- Kuo, C.L.; Ho, Y.Y.; Lee, L.C. Electrical Coupling between the ionosphere and surface charges in the earthquake fault zone. In Pre-Earthquake Processes: A Multidisciplinary Approach to Earthquake Prediction Studies (Geophysical Monograph 234); Ouzounov, D., Pulinets, S., Hattori, K., Taylor, P., Eds.; American Geophysical Union: Washington, DC, USA; John Wiley and Sons: Hoboken, NJ, USA, 2018; pp. 99–124. [Google Scholar]
- Denisenko, V.V.; Boudjada, M.Y.; Lammer, H. Propagation of seismogenic electric currents through the Earth’s atmosphere. J. Geophys. Res. 2018, 123, 4290–4297. [Google Scholar] [CrossRef]
- Liu, J.Y.; Chen, Y.I.; Chuo, Y.J.; Chen, C.S. A statistical investigation of pre-earthquake ionospheric anomaly. J. Geophys. Res. 2006, 111, A05304. [Google Scholar]
- Zakharenkova, I.E.; Shagimuratov, I.I.; Tepenitzina, N.Y.; Krankowski, A. Anomalous modification of the ionospheric total electron content prior to the 26 September 2005 Peru earthquake. J. Atmos. Sol.–Terr. Phys. 2008, 70, 1919–1928. [Google Scholar] [CrossRef]
- Namgaladze, A.A.; Zolotov, O.V.; Karpov, M.I.; Romanovskaya, Y.V. Manifestations of the earthquake preparations in the ionosphere total electron content variations. Nat. Sci. 2012, 11, 848–855. [Google Scholar] [CrossRef][Green Version]
- Hayakawa, M.; Molchanov, O.A.; Ondoh, T.; Kawai, E. Precursory signature of the Kobe earthquake on VLF subionospheric signal. J. Atmos. Electr. 1996, 16, 247–257. [Google Scholar]
- Molchanov, O.A.; Hayakawa, M. Subionospheric VLF signal perturbations possibly related to earthquakes. J. Geophys. Res. Space Phys. 1998, 103, 17489–17504. [Google Scholar] [CrossRef]
- Ghimire, B.D.; Chaudhary, H.N.D.; Schnoor, P.W.; Bhatta, K.; Chhettri, M.P.; Khadka, B. A Study on the Terminator Times for the Signal of 52.10 kHz Transmitted from Crimrod, UK Received at Kiel Lonwave Monitor, Germany. Int. J. Recent Res. Rev. 2016, 9, 21–26. [Google Scholar]
- Chakrabarti, S.K.; Saha, M.; Khan, R.; Mandal, S.; Acharyya, K.; Saha, R. Possible Detection of Ionospheric Disturbances during Sumatra-Andaman Islands. Indian J. Radio Space Phys. 2005, 34, 314–317. [Google Scholar]
- Yoshida, M.; Yamauchi, T.; Horie, T.; Hayakawa, M. On the generation mechanism of terminator times in subionospheric VLF/LF propagation and its possible application to seismogenic effects. Nat. Hazards Earth Syst. Sci. 2008, 8, 129–134. [Google Scholar] [CrossRef]
- Ray, S.; Chakrabarti, S.K. A study of the behavior of the terminator time shifts using multiple VLF propagation paths during the Pakistan earthquake (M = 7.2) of 18 January 2011. Nat. Hazards Earth Syst. Sci. 2013, 13, 1501–1506. [Google Scholar] [CrossRef]
- Sasmal, S.; Chakrabarti, S.K.; Ray, S. Unusual behavior of VLF signals observed from Sitapur during the Earthquake at Honshu Japan on 11 March, 2011. Indian J. Phys. 2014, 88, 103–119. [Google Scholar] [CrossRef]
- Ghosh, S.; Chakraborty, S.; Sasmal, S.; Basak, T.; Chakrabarti, S.K.; Samanta, A. Comparative study of the possible lower ionospheric anomalies in very low frequency (VLF) signal during Honshu, 2011 and Nepal, 2015 earthquakes. Geomat. Nat. Hazards Risk 2019, 10, 1596–1612. [Google Scholar] [CrossRef]
- Rapoport, Y.; Grimalsky, V.; Krankowski, A.; Pulinets, S.; Fedorenko, A.; Petrishchevskii, S. Algorithm for modeling electromagnetic channel of seismo-ionospheric coupling (SIC) and the variations in the electron concentration. Acta Geophys. 2019, 68, 253–278. [Google Scholar] [CrossRef]
- Rapoport, Y.; Grimalsky, V.; Fedun, V.; Agapitov, O.; Bonnell, J.; Grytsai1, A.; Milinevsky, G.; Liashchuk, A.; Rozhnoi, A.; Solovieva, M.; et al. Model of the propagation of very low-frequency beams in the Earth–ionosphere waveguide: Principles of the tensor impedance method in multi-layered gyrotropic waveguides. Ann. Geophys. 2020, 38, 207–230. [Google Scholar] [CrossRef]
- Rapoport, Y.; Reshetnyk, V.; Grytsai, A.; Grimalsky, V.; Liashchuk, O.; Fedorenko, A.; Hayakawa, M.; Krankowski, A.; Błaszkiewicz, L.; Flisek, P. Spectral Analysis and Information Entropy Approaches to Data of VLF Disturbances in the Waveguide Earth-Ionosphere. Sensors 2022, 22, 8191. [Google Scholar] [CrossRef] [PubMed]
- Boudjada, M.Y.; Biagi, P.F.; Eichelberger, H.U.; Nico, G.; Galopeau, P.H.M.; Ermini, A.; Solovieva, M.; Hayakawa, M.; Lammer, H.; Voller, W.; et al. Analysis of Pre-Seismic Ionospheric Disturbances Prior to 2020 Croatian Earthquakes. Remote Sens. 2024, 16, 529. [Google Scholar] [CrossRef]
- Dobrovolsky, I.R.; Zubkov, S.I.; Myachkin, V.I. Estimation of the size of earthquake preparation zones. Pageoph 1979, 117, 1025–1044. [Google Scholar] [CrossRef]
- Hayakawa, M. Probing the lower ionospheric perturbations associated with earthquakes by means of subionospheric VLF/LF propagation. Earthq. Sci. 2011, 24, 609–637. [Google Scholar] [CrossRef]
- Beer, T. Atmospheric Waves; Adam Hilger: London, UK, 1974. [Google Scholar]
- Hines, C.O. Tidal oscillations, shorter period gravity waves and shear waves. Up. Atmos. Motion 1974, 18, 96–109. [Google Scholar]
- Molchanov, O.A.; Hayakawa, M.; Miyaki, K. VLF/LF sounding of the lower ionosphere to study the role of atmospheric oscillations in the lithosphere-ionosphere coupling. Adv. Polar Up. Atmos. Res. 2001, 15, 146–158. [Google Scholar]
- Miyaki, K.; Hayakawa, M.; Molchanov, O.A. The role of gravity waves in the lithosphere–ionosphere coupling, as revealed from the subionospheric LF propagation data. In Seismo-Electromagnetics: Lithosphere–Atmosphere–Ionosphere Coupling; TERRAPUB: Tokyo, Japan, 2002; pp. 229–232. [Google Scholar]
- Rozhnoi, A.; Solovieva, M.; Molchanov, O.; Biagi, P.F.; Hayakawa, M. Observation evidences of atmospheric Gravity Waves induced by seismic activity from analysis of subionospheric LF signal spectra. Nat. Hazards Earth Syst. Sci. 2007, 7, 625–628. [Google Scholar] [CrossRef]
- Muto, F.; Kasahara, Y.; Hobara, Y.; Hayakawa, M.; Rozhnoi, A.; Solovieva, M.; Molchanov, O.A. Further study on the role of atmospheric gravity waves on the seismo-ionospheric perturbations as detected by subionospheric VLF/LF propagation. Nat. Hazards Earth Syst. Sci. 2009, 9, 1111–1118. [Google Scholar] [CrossRef][Green Version]
- Korepanov, V.; Hayakawa, M.; Yampolski, Y.; Lizunov, G. AGW as a seismo-ionospheric coupling responsible agent. Phys. Chem. Earth Parts A/B/C 2009, 34, 485–495. [Google Scholar] [CrossRef]
- Hayakawa, M.; Kasahara, Y.; Nakamura, T.; Hobara, Y.; Rozhnoi, A.; Solovieva, M.; Molchanov, O.; Korepanov, V. Atmospheric gravity waves as a possible candidate for seismo-ionospheric perturbation. J. Atmos. Electr. 2011, 31, 129–140. [Google Scholar] [CrossRef]
- Nakamura, T.; Korepanov, V.; Kasahara, Y.; Hobara, Y.; Hayakawa, M. An evidence on the lithosphere-ionosphere coupling in terms of atmospheric gravity waves on the basis of a combined analysis of surface pressure, ionospheric perturbations and ground-based ULF variations. J. Atmos. Electr. 2013, 33, 53–68. [Google Scholar] [CrossRef][Green Version]
- Chakraborty, S.; Sasmal, S.; Chakrabarti, S.K.; Bhattacharya, A. Observational signatures of unusual outgoing longwave radiation (OLR) and atmospheric gravity waves (AGW) as precursory effects of May 2015 Nepal earthquakes. J. Geodyn. 2018, 113, 43–51. [Google Scholar] [CrossRef]
- Yang, S.-S.; Asano, T.; Hayakawa, M. Abnormal gravity wave activity in the stratosphere prior to the 2016 Kumamoto earthquakes. J. Geophys. Res. 2019, 124, 1410–1425. [Google Scholar] [CrossRef]
- Nina, A.; Pulinets, S.; Biagi, P.F.; Nico, G.; Mitrovic, S.; Radovanovic, M.; Popovic, L.C. Variation in natural short-period ionospheric noise, and acoustic and gravity waves revealed by the amplitude analysis of a VLF radio signal on the occasion of the Kraljevo earthquake (Mw=5.4). Sci. Total Environ. 2020, 710, 136406. [Google Scholar] [CrossRef]
- Yang, S.-S.; Hayakawa, M. Gravity Wave Activity in the Stratosphere before the 2011 Tohoku Earthquake as the Mechanism of Lithosphere-atmosphere-ionosphere Coupling. Entropy 2020, 22, 110. [Google Scholar] [CrossRef]
- Akhoondzadeh, M.; Marchetti, D. Study of the Preparation Phase of Turkey’s Powerful Earthquake (6 February 2023) by a Geophysical Multi-Parametric Fuzzy Inference System. Remote Sens. 2023, 15, 2224. [Google Scholar] [CrossRef]
- Contadakis, M.E.; Pikridas, C.; Bitharis, S.; Scordilis, E. TEC variation over Europe during the intense tectonic activity in the area of Turkey on February of 2023. In Proceedings of the European Geosciences Union General Assembly 2024, Vienna, Austria, 14–19 April 2024. EGU24-6275. [Google Scholar]
- Maletckii, B.; Astafyeva, E.; Sanchez, S.A.; Kherani, E.A.; de Paula, E.R. The 6 February 2023 Türkiye earthquake sequence as detected in the ionosphere. J. Geophys. Res. 2023, 128, e2023JA031663. [Google Scholar] [CrossRef]
- Vesnin, A.; Yasyukevich, Y.; Perevalova, N.; ¸Sentürk, E. Ionospheric Response to the 6 February 2023 Turkey–Syria Earthquake. Remote Sens. 2023, 15, 2336. [Google Scholar] [CrossRef]
- Schwingenschuh, K.; Prattes, G.; Besser, B.P.; Mocnik, K.; Stachel, M.; Aydogar, Ö.; Jernej, I.; Boudjada, M.Y.; Stangl, G.; Rozhnoi, A.; et al. The Graz seismo-electromagnetic VLF facility. Nat. Hazards Earth Syst. Sci. 2011, 11, 1121–1127. [Google Scholar] [CrossRef]
- Biagi, P.F.; Colella, R.; Schiavulli, L.; Ermini, A.; Boudjada, M.; Eichelberger, H.; Schwingenschuh, K.; Katzis, K.; Contadakis, M.E.; Skeberis, C.; et al. The INFREP network: Present situation and recent results. Open J. Earthq. Res. 2019, 8, 154–196. [Google Scholar] [CrossRef]
- Biagi, P.F.; Maggipinto, T.; Righetti, F.; Loiacono, D.; Schiavulli, L.; Ligonzo, T.; Ermini, A.; Moldovan, I.A.; Moldovan, A.S.; Buyuksarac, A.; et al. The European VLF/LF Radio Network to Search for Earthquake Precursors: Setting Up and Natural/Man-Made Disturbances. Nat. Hazards Earth Syst. Sci. 2011, 11, 333–341. [Google Scholar] [CrossRef]
- Galopeau, P.H.M.; Maxworth, A.; Boudjada, M.Y.; Eichelberger, H.U.; Meftah, M.; Biagi, P.F.; Schwingenschuh, K. A VLF/LF facility network for preseismic electromagnetic investigations. Geosci. Instrum. Method. Data. 2023, 12, 231–237. [Google Scholar] [CrossRef]
- Rozhnoi, A.; Solovieva, M.; Fedun, V.; Gallagher, P.; McCauley, J.; Boudjada, M.Y.; Shelyag, S.; Eichelberger, H.U. Strong influence of solar X-ray flares on low-frequency electromagnetic signals in middle latitudes. Ann. Geophys. 2019, 37, 843–850. [Google Scholar] [CrossRef]
- Davidson, M. Elements of Mathematical Astronomy; Hutchinson & Co., Ltd.: London, UK, 1962; 142p. [Google Scholar]
- Pulinets, S.; Krankowski, A.; Hernandez-Pajares, M.; Marra, S.; Cherniak, I.; Zakharenkova, I.; Rothkaehl, H.; Kotulak, K.; Davidenko, D.; Blaszkiewicz, L.; et al. Ionosphere Sounding for Pre-seismic Anomalies Identification (INSPIRE): Results of the Project and Perspectives for the Short-Term Earthquake Forecast. Front. Earth Sci. 2021, 9, 610193. [Google Scholar] [CrossRef]
- Schirninger, C.; Eichelberger, H.U.; Magnes, W.; Boudjada, M.Y.; Schwingenschuh, K.; Pollinger, A.; Besser, B.P.; Biagi, P.F.; Solovieva, M.; Wang, J.; et al. Satellite measured ionospheric magnetic field variations over natural hazards sites. Remote Sens. 2021, 13, 2360. [Google Scholar] [CrossRef]
- Yang, B.-Y.; Li, Z.; Huang, J.-P.; Yang, X.-M.; Yin, H.-C.; Li, Z.-Y.; Lu, H.-X.; Li, W.-J.; Shen, X.-H.; Zeren, Z.; et al. EMD based statistical analysis of nighttime pre-earthquake ULF electric field disturbances observed by CSES. Front. Astron. Space Sci. 2023, 9, 1077592. [Google Scholar] [CrossRef]



| Figure | Type | DOY | Time (UT) | Amplitude (dB) | Phase (Degree) | |
|---|---|---|---|---|---|---|
| Figure 4—right panel | Sunrise | Minimum | 033.227 | 05:27 | −68.99 | +110.77° |
| 2 February 2023 | Sunrise | Maximum | 033.245 | 05:54 | −54.94 | +105.89° |
| Amplitude | Sunrise | Minimum | 033.280 | 06:43 | −66.48 | +097.56° |
| Sunrise | Maximum | 033.293 | 07:03 | −63.81 | +076.30° | |
| Sunrise | Minimum | 033.310 | 07:27 | −64.42 | +057.49° | |
| Sunset | Minimum | 033.615 | 14:46 | −65.96 | −004.88° | |
| Sunset | Maximum | 033.645 | 15:30 | −61.04 | −131.33° | |
| Sunset | Maximum | 033.657 | 15:46 | −59.52 | −151.95° | |
| Sunset | Minimum | 033.661 | 15:53 | −60.15 | −146.46° | |
| Figure 4—left panel | Sunrise | Minimum | 033.201 | 04:50 | −48.11 | −143.32° |
| 2 February 2023 | Sunrise | Maximum | 033.212 | 05:05 | −51.84 | −126.03° |
| Phase | Sunrise | Jump | 033.224 | 05:22 | −63.00 | −179.98° |
| Sunrise | Jump | 033.224 | 05:22 | −63.32 | +177.15° | |
| Sunrise | Minimum | 033.231 | 05:32 | −63.04 | +082.86° | |
| Sunrise | Maximum | 033.266 | 06:23 | −61.19 | +130.76° | |
| Sunrise | Minimum | 033.291 | 07:00 | −64.26 | +075.69° | |
| Sunset | Minimum | 033.590 | 14:10 | −63.12 | +029.26° | |
| Sunset | Maximum | 033.600 | 14:23 | −63.80 | +035.48° | |
| Sunset | Minimum | 033.658 | 15:48 | −59.80 | −154.75° | |
| Sunset | Maximum | 033.672 | 16:08 | −57.06 | −122.19° | |
| Sunset | Minimum | 033.693 | 16:38 | −52.09 | −136.03° |
| Figure | Type | DOY | Time (UT) | Amplitude (dB) | Phase (Degree) | |
|---|---|---|---|---|---|---|
| Figure 4—right panel | Sunrise | Minimum | 033.280 | 06:43 | −66.48 | +097.56° |
| 2 February 2023 | Sunset | Minimum | 033.661 | 15:53 | −60.15 | −146.46° |
| Amplitude | ||||||
| Figure 4—left panel | Sunrise | Minimum | 033.291 | 07:00 | −64.26 | +075.69° |
| 2 February 2023 | Sunset | Minimum | 033.658 | 15:48 | −59.80 | −154.75° |
| Phase |
| Figure | Type | DOY | Time (UT) | Closest Terminator | |
|---|---|---|---|---|---|
| Figure 4—right panel | Sunrise | Minimum | 033.227 | 05:27 | TBB transmitter |
| 2 February 2023 | Sunrise | Maximum | 033.245 | 05:54 | Graz reception |
| Amplitude | Sunset | Minimum | 033.615 | 14:46 | EQ epicenter |
| Sunset | Maximum | 033.645 | 15:30 | TBB transmitter | |
| Figure 4—left panel | Sunrise | Minimum | 033.231 | 05:32 | TBB transmitter |
| 2 February 2023 | Sunrise | Maximum | 033.266 | 06:23 | Graz reception |
| Phase | Sunset | Minimum | 033.658 | 15:48 | TBB transmitter |
| Sunset | Maximum | 033.672 | 16:08 | Graz reception |
| DOY | 023 | 024 | 025 | 026 | 027 | 028 | 029 | 030 | 031 | 032 | 033 | 034 | 035 | 036 | 037 | 038 | 039 | ||
| Sunrise | |||||||||||||||||||
| Amplitude | Figure 5 | EQ | EQ | TB | EQ | GR | GR | EQ | EQ | EQ | EQ | TB | TB | TB | TB | TB | TB | TB | |
| Minima | 1st Panel | Time | 04:32 | 04:44 | 05:30 | 04:38 | 06:22 | 06:43 | 04:39 | 04:42 | 04:24 | 04:23 | 05:27 | 05:26 | 05:23 | 05:23 | 05:24 | 05:22 | 05:23 |
| Amplitude | Figure 5 | TB | TB | TB | TB | GR | GR | GR | TB | TB | TB | GR | GR | GR | GR | EQ | GR | GR | |
| Maxima | 2nd Panel | Time | 05:02 | 05:01 | 04:59 | 05:03 | 06:37 | 06:32 | 06:34 | 04:56 | 04:52 | 04:54 | 05:54 | 05:55 | 05:55 | 06:03 | 04:41 | 05:48 | 05:51 |
| DOY | 023 | 024 | 025 | 026 | 027 | 028 | 029 | 030 | 031 | 032 | 033 | 034 | 035 | 036 | 037 | 038 | 039 | ||
| Sunset | |||||||||||||||||||
| Amplitude | Figure 5 | GR | GR | GR | GR | EQ | EQ | EQ | EQ | TB | EQ | EQ | EQ | EQ | EQ | EQ | EQ | EQ | |
| Minima | 3rd Panel | Time | 15:42 | 15:47 | 15:50 | 15:45 | 14:52 | 14:59 | 14:53 | 15:00 | 15:26 | 15:01 | 14:46 | 14:55 | 14:39 | 14:55 | 15:07 | 15:14 | 15:11 |
| Amplitude | Figure 5 | EQ | EQ | EQ | EQ | TB | GR | TB | GR | EQ | TB | TB | TB | GR | EQ | EQ | TB | ||
| Maxima | 4th Panel | Time | 14:48 | 14:40 | 15:05 | 14:56 | 15:24 | 16:10 | 15:16 | 16:33 | 15:09 | 15:30 | 15:48 | 15:22 | 16:27 | 14:54 | 13:55 | 15:34 | |
| DOY | 023 | 024 | 025 | 026 | 027 | 028 | 029 | 030 | 031 | 032 | 033 | 034 | 035 | 036 | 037 | 038 | 039 | ||
| Sunrise | |||||||||||||||||||
| Phase | Figure 6 | EQ | TB | EQ | EQ | EQ | TB | TB | TB | EQ | GR | TB | TB | TB | TB | TB | TB | EQ | |
| Minima | 1st Panel | Time | 04:54 | 05:01 | 04:55 | 04:58 | 04:54 | 05:52 | 05:47 | 05:23 | 04:53 | 06:39 | 05:32 | 05:29 | 05:26 | 05:27 | 05:24 | 04:48 | 04:46 |
| Phase | Figure 6 | TB | GR | GR | TB | GR | GR | TB | TB | TB | GR | GR | GR | TB | TB | TB | TB | TB | |
| Maxima | 2nd Panel | Time | 05:19 | 06:32 | 06:28 | 05:19 | 06:08 | 06:40 | 05:10 | 05:07 | 05:09 | 06:14 | 06:23 | 06:25 | 05:05 | 05:04 | 05:07 | 05:01 | 05:00 |
| DOY | 023 | 024 | 025 | 026 | 027 | 028 | 029 | 030 | 031 | 032 | 033 | 034 | 035 | 036 | 037 | 038 | 039 | ||
| Sunset | |||||||||||||||||||
| Phase | Figure 6 | GR | EQ | EQ | GR | EQ | EQ | EQ | EQ | EQ | TB | TB | TB | TB | TB | GR | TB | TB | |
| Minima | 3rd Panel | Time | 15:58 | 15:03 | 14:33 | 16:07 | 14:53 | 14:50 | 14:50 | 14:35 | 15:10 | 15:35 | 15:48 | 15:49 | 15:43 | 15:39 | 16:07 | 15:51 | 15:40 |
| Phase | Figure 6 | TB | TB | TB | EQ | EQ | EQ | EQ | GR | GR | GR | GR | TB | GR | TB | TB | EQ | ||
| Maxima | 4th Panel | Time | 15:24 | 15:23 | 15:29 | 15:04 | 15:01 | 14:56 | 14:48 | 16:03 | 15:59 | 16:08 | 16:11 | 15:49 | 16:03 | 15:54 | 15:42 | 14:42 | |
| Amplitude Minima | Amplitude Minima | |||||||
|---|---|---|---|---|---|---|---|---|
| Figure 7: Upper-Left Panel | Figure 7: Lower-Left Panel | |||||||
| DOY | Sunrise UT | Sunset UT | Longitude Degree | Latitude Degree | Sunrise UT | Sunrise UT | Longitude Degree | Latitude Degree |
| 023 | 04:32 | 15:42 | 31°E | 20°N | 04:54 | 15:58 | 27°E | 25°N |
| 024 | 04:44 | 15:47 | 30°E | 25°N | 05:01 | 15:03 | 32°E | 39°N |
| 025 | 05:30 | 15:30 | 23°E | 35°N | 04:55 | 13:33 | 37°E | 44°N |
| 026 | 04:38 | 15:45 | 31°E | 25°N | 04:58 | 16:07 | 26°E | 25°N |
| 027 | 06:22 | 14:52 | --- | --- | 04:54 | 14:53 | 34°E | 40°N |
| 028 | 06:43 | 14:59 | --- | --- | 05:52 | 14:50 | --- | --- |
| 029 | 04:39 | 14:53 | 37°E | 38°N | 05:47 | 14:50 | 27°E | 47°N |
| 030 | 04:42 | 15:00 | 35°E | 37°N | 05:23 | 14:35 | 33°E | 47°N |
| 031 | 04:24 | 15:26 | 35°E | 25°N | 04:53 | 15:10 | 32°E | 37°N |
| 032 | 04:23 | 15:01 | 37°E | 30°N | 06:36 | 15:35 | --- | --- |
| 033 | 05:27 | 14:46 | 30°E | 42°N | 05:32 | 15:48 | 24°E | 41°N |
| 034 | 05:26 | 14:55 | 30°E | 45°N | 05:29 | 15:49 | 24°E | 40°N |
| 035 | 05:23 | 14:39 | 33°E | 47°N | 05:26 | 15:43 | 25°E | 41°N |
| 036 | 05:23 | 14:55 | 31°E | 46°N | 05:27 | 15:39 | 26°E | 44°N |
| 037 | 05:24 | 15:00 | 30°E | 47°N | 05:24 | 16:07 | 23°E | 38°N |
| 038 | 05:22 | 15:14 | 29°E | 47°N | 04:48 | 15:51 | 28°E | 25°N |
| 039 | 05:23 | 15:11 | 29°E | 46°N | 04:46 | 15:40 | 30°E | 30°N |
| Amplitude Maxima | Amplitude Maxima | |||||||
|---|---|---|---|---|---|---|---|---|
| Figure 7: Upper-Right Panel | Figure 7: Lower-Right Panel | |||||||
| DOY | Sunrise UT | Sunset UT | Longitude Degree | Latitude Degree | Sunrise UT | Sunrise UT | Longitude Degree | Latitude Degree |
| 023 | 05:02 | 14:48 | 34°E | 42°N | 05:19 | 15:24 | 28°E | 39°N |
| 024 | 05:01 | 14:40 | 35°E | 43°N | 06:32 | 15:23 | 18°E | 47°N |
| 025 | 04:59 | 15:05 | 33°E | 39°N | 06:28 | 15:29 | 18°E | 47°N |
| 026 | 05:03 | 14:56 | 33°E | 41°N | 05:19 | --- | --- | --- |
| 027 | 06:37 | 15:24 | 17°E | 47°N | 06:08 | 15:04 | 23°E | 47°N |
| 028 | 06:32 | --- | --- | --- | 06:40 | 15:01 | --- | --- |
| 029 | 06:34 | 16:10 | 15°E | 45°N | 05:10 | 14:56 | 32°E | 45°N |
| 030 | 04:56 | 15:16 | 32°E | 37°N | 05:07 | 14:48 | 34°E | 46°N |
| 031 | 04:52 | 16:33 | 22°E | 10°N | 05:09 | 16:03 | 24°E | 25°N |
| 032 | 04:54 | 15:09 | 33°E | 40°N | 06:14 | 15:59 | 18°E | 45°N |
| 033 | 05:54 | 15:30 | 21°E | 47°N | 06:23 | 16:08 | 16°E | 43°N |
| 034 | 05:55 | 15:48 | 20°E | 46°N | 06:25 | 16:11 | 16°E | 43°N |
| 035 | 05:55 | 15:22 | 23°E | 47°N | 05:05 | 15:49 | 27°E | 35°N |
| 036 | 06:03 | 16:27 | 16°E | 45°N | 05:04 | 16:03 | 25°E | 30°N |
| 037 | 04:41 | 14:54 | 37°E | 44°N | 05:07 | 15:54 | 26°E | 30°N |
| 038 | 05:48 | 13:55 | --- | --- | 05:01 | 15:42 | 27°E | 37°N |
| 039 | 05:51 | 15:34 | 23°E | 46°N | 05:00 | 14:42 | 33°E | 47°N |
| Figure 7 | Name | Band1 Begin | End | Name | Band2 Begin | End |
|---|---|---|---|---|---|---|
| Upper-left panel | Band_Am_Min1 | 31°E-20°N | 37°E-38°N | Band_Am_Min2 | 23°E-35°N | 33°E-47°N |
| Upper-right panel | Band_Am_Max1 | 32°E-37°N | 37°E-44°N | Band_Am_Max2 | 15°E-45°N | 23°E-47°N |
| Lower-left panel | Band_Ph_Min1 | 26°E-25°N | 37°E-44°N | Band_Ph_Min2 | 15°E-45°N | 23°E-47°N |
| Lower-left panel | Band_Ph_Max1 | 24°E-25°N | 33°E-47°N | Band_Ph_Min2 | 16°E-43°N | 18°E-47°N |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boudjada, M.Y.; Biagi, P.F.; Eichelberger, H.U.; Nico, G.; Schwingenschuh, K.; Galopeau, P.H.M.; Solovieva, M.; Contadakis, M.; Denisenko, V.; Lammer, H.; et al. Unusual Sunrise and Sunset Terminator Variations in the Behavior of Sub-Ionospheric VLF Phase and Amplitude Signals Prior to the Mw7.8 Turkey Syria Earthquake of 6 February 2023. Remote Sens. 2024, 16, 4448. https://doi.org/10.3390/rs16234448
Boudjada MY, Biagi PF, Eichelberger HU, Nico G, Schwingenschuh K, Galopeau PHM, Solovieva M, Contadakis M, Denisenko V, Lammer H, et al. Unusual Sunrise and Sunset Terminator Variations in the Behavior of Sub-Ionospheric VLF Phase and Amplitude Signals Prior to the Mw7.8 Turkey Syria Earthquake of 6 February 2023. Remote Sensing. 2024; 16(23):4448. https://doi.org/10.3390/rs16234448
Chicago/Turabian StyleBoudjada, Mohammed Y., Pier F. Biagi, Hans U. Eichelberger, Giovanni Nico, Konrad Schwingenschuh, Patrick H. M. Galopeau, Maria Solovieva, Michael Contadakis, Valery Denisenko, Helmut Lammer, and et al. 2024. "Unusual Sunrise and Sunset Terminator Variations in the Behavior of Sub-Ionospheric VLF Phase and Amplitude Signals Prior to the Mw7.8 Turkey Syria Earthquake of 6 February 2023" Remote Sensing 16, no. 23: 4448. https://doi.org/10.3390/rs16234448
APA StyleBoudjada, M. Y., Biagi, P. F., Eichelberger, H. U., Nico, G., Schwingenschuh, K., Galopeau, P. H. M., Solovieva, M., Contadakis, M., Denisenko, V., Lammer, H., Voller, W., & Giner, F. (2024). Unusual Sunrise and Sunset Terminator Variations in the Behavior of Sub-Ionospheric VLF Phase and Amplitude Signals Prior to the Mw7.8 Turkey Syria Earthquake of 6 February 2023. Remote Sensing, 16(23), 4448. https://doi.org/10.3390/rs16234448










