The Spacecraft Parabolic Antenna Payload Orientation Estimation Method Based on the Step Effect of Measured Radar Cross Section Sequences
Abstract
1. Introduction
2. Methods
2.1. Analysis of the Electromagnetic Scattering Properties of Spacecraft Carrying a Parabolic Antenna Payload
2.1.1. Simulation Calculation of Electromagnetic Scattering Characteristics
2.1.2. Analysis of Electromagnetic Scattering Characteristics
2.2. Mathematical Model of Parabolic Antenna Pointing Estimation
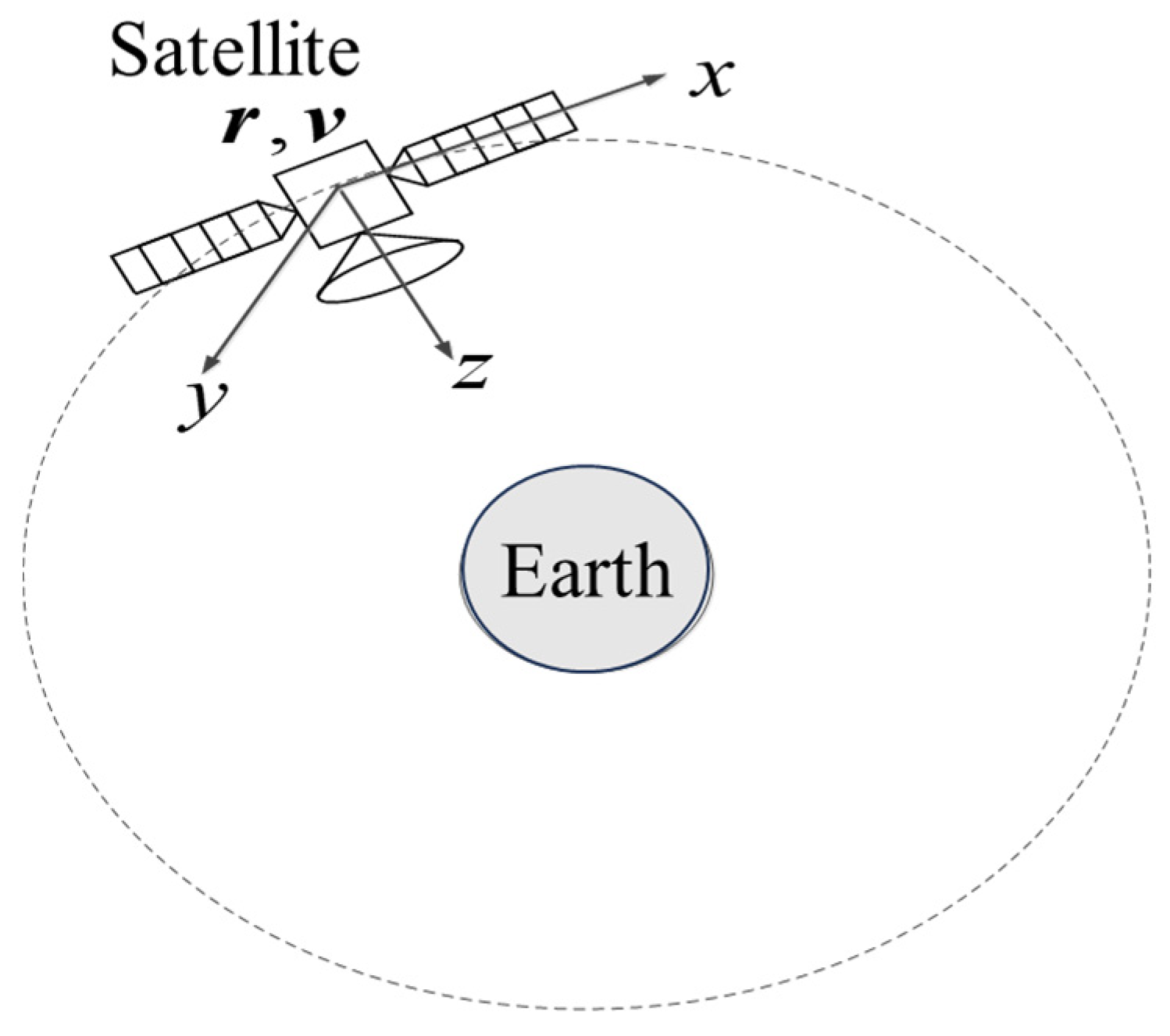
2.2.1. Satellite Orbital Coordinate System
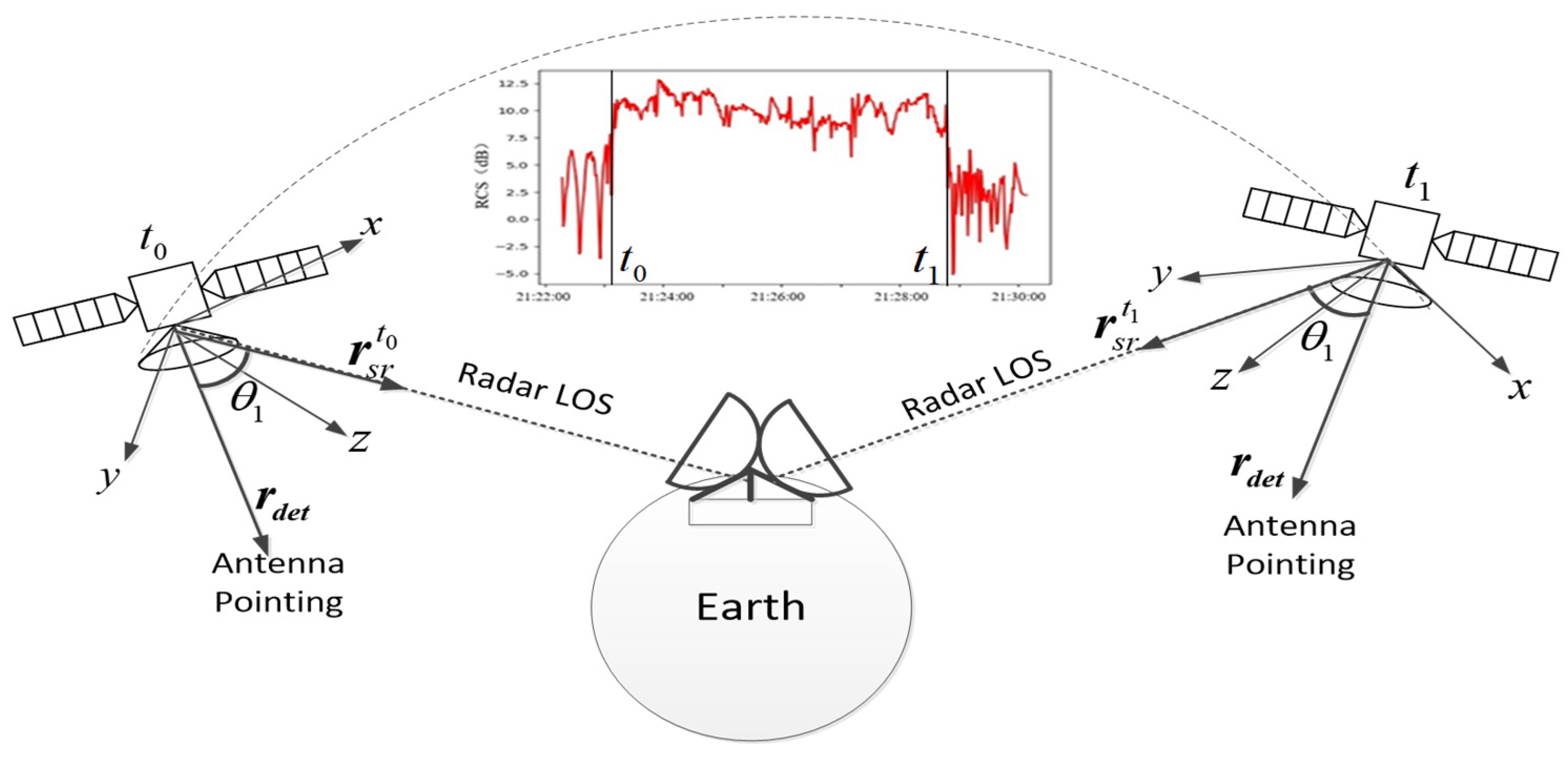
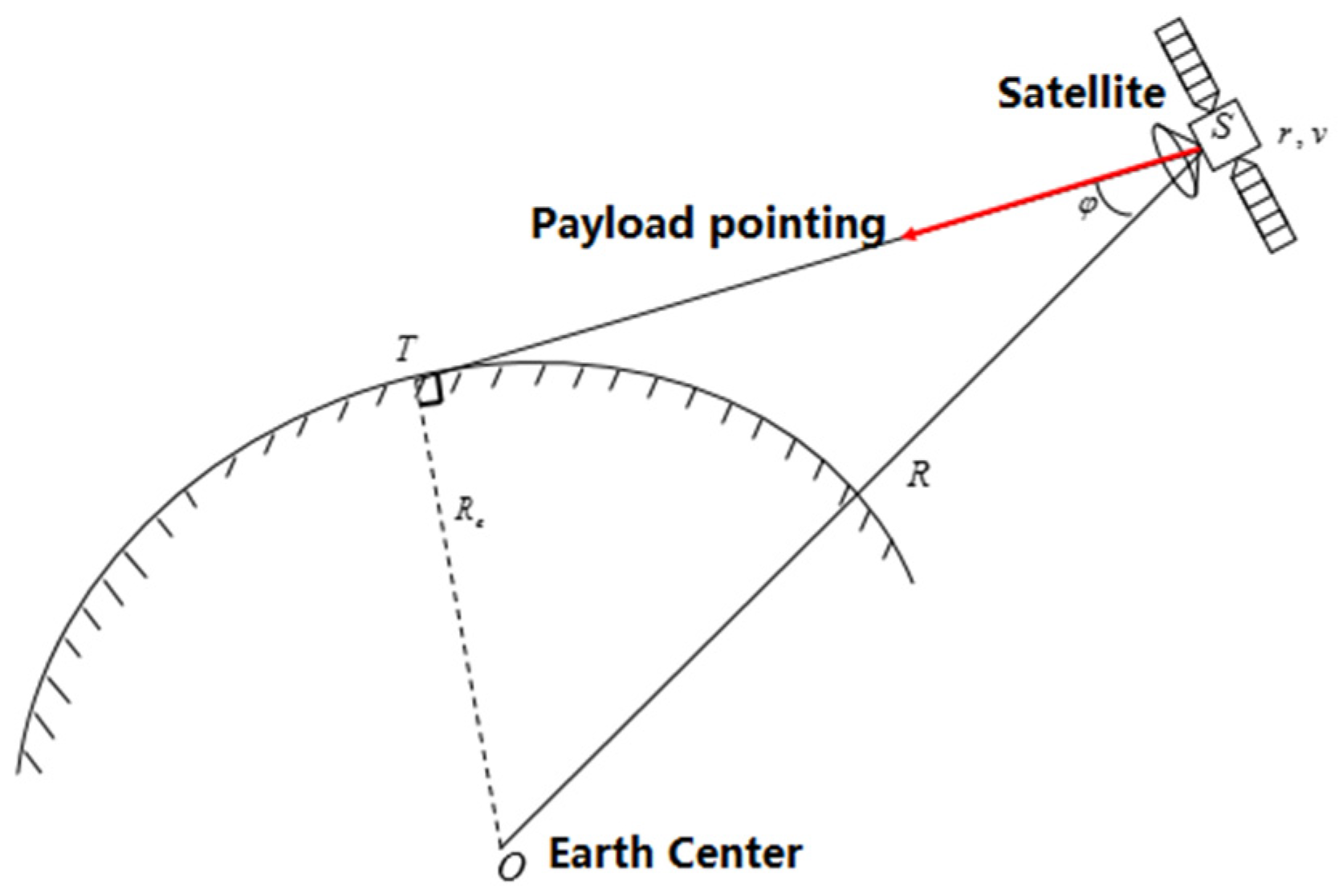
2.2.2. Mathematical Model of Payload Pointing
2.3. Optimization Modeling and Solving
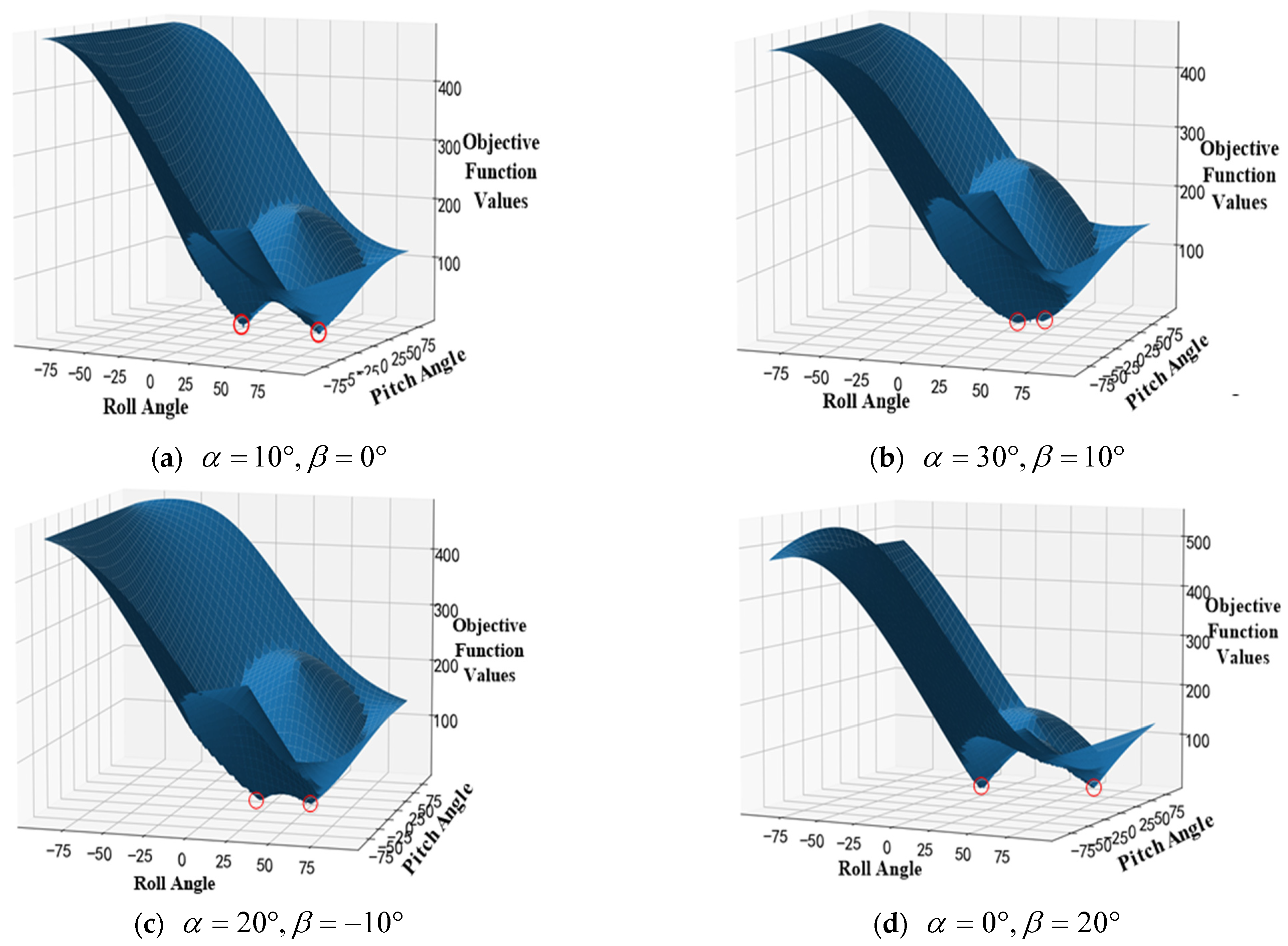
2.3.1. Objective Function
2.3.2. Constraints
Ground Reconnaissance Constraint
RCS Feature Constraint
Side-Swing Ability Constraint
2.3.3. Optimization Model
2.3.4. Modified Particle Swarm Optimization
3. Results
3.1. Simulation Verification
3.1.1. Single-Station Measurement
3.1.2. Multi-Station Measurement
3.1.3. Comparison of Single-Station and Multi-Station Results
3.2. Precision Analysis
3.2.1. Errors Caused by the Accuracy of Time Extraction
3.2.2. Errors Caused by the Optimization Algorithm
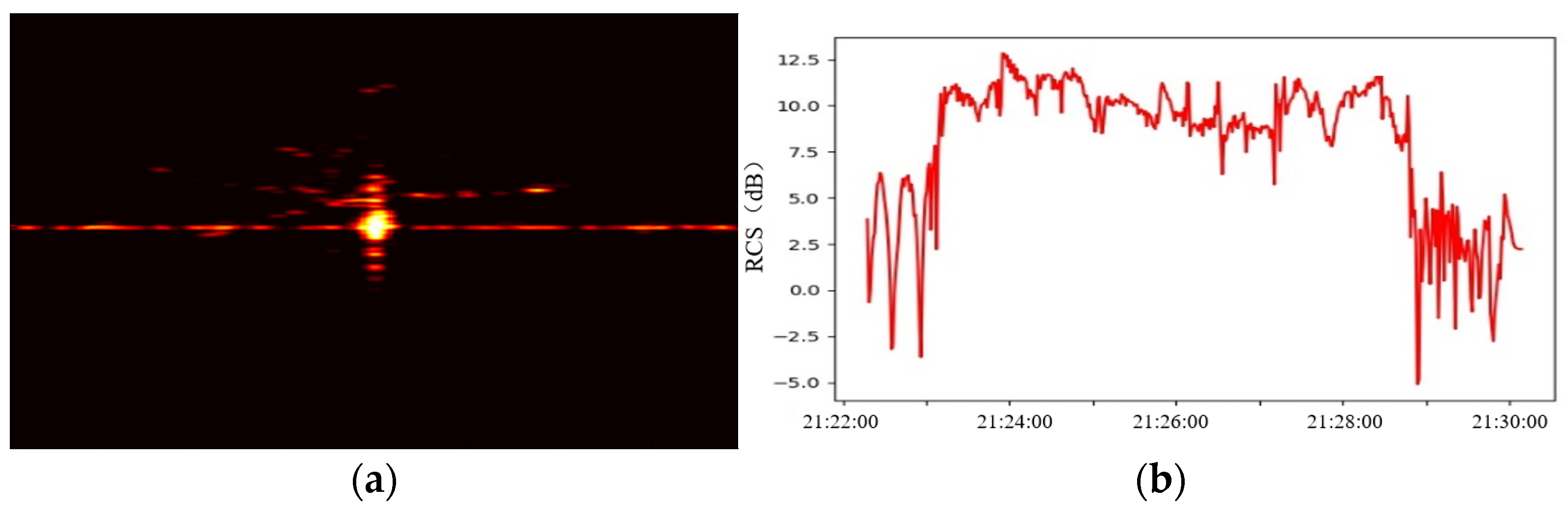
3.3. Application of Empirical Data
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lin, H.-Y.; Zhao, C.-Y. An estimation of Envisat’s rotational state accounting for the precession of its rotational axis caused by gravity gradient torque. Adv. Space Res. 2018, 61, 182–188. [Google Scholar] [CrossRef]
- Zhong, W.J.; Wang, J.S.; Ji, W.J.; Lei, X.; Zhou, X.W. The attitude estimation of three-axis stabilized satellites using hybrid particle swarm optimization combined with radar cross section precise prediction. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2015, 31, 222–224. [Google Scholar] [CrossRef]
- Lyu, J.T.; Zhong, W.J.; Liu, H.; Geng, Y.; Ben, D. Novel Approach to Determine Spinning Satellites’ Attitude by RCS Measurements. J. Aerosp. Eng. 2021, 34, 04021023. [Google Scholar] [CrossRef]
- D’Amico, S.; Benn, M.; Jørgensen, J.L. Pose estimation of an uncooperative spacecraft from actual space imagery. Int. J. Space Sci. Eng. 2014, 1, 171–189. [Google Scholar]
- Sommer, S.; Rosebrock, J.; Cerutti-Maori, D.; Leushacke, L. Temporal analysis of ENVISAT’s rotational motion. In Proceedings of the 7th European Conference on Space Debris, Darmstadt, Germany, 18–21 April 2017; pp. 18–21. [Google Scholar]
- Lemmens, S.; Krag, H.; Rosebrock, J. Radar mappings for attitude analysis of objects in orbit. In Proceedings of the 6th European Conference on Space Debris, Darmstadt, Germany, 22–25 April 2013; pp. 20–24. [Google Scholar]
- Lemmens, S.; Krag, H. Sensitivity of automated attitude determination form ISAR radar mappings. In Proceedings of the Advanced Maui Optical and Space Surveillance Technologies Conference (AMOS), Maui, HI, USA, 10–13 September 2013. [Google Scholar]
- Wang, Q.Y.; Wang, Z.Y. Position and pose measurement of spacecraft based on monocular vision. J. Appl. Opt. 2017, 38, 250–255. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhang, L.; Cao, Y.; Wu, Z. Attitude estimation and geometry reconstruction of satellite targets based on ISAR image sequence interpretation. IEEE Trans. Aerosp. Electron. Syst. 2018, 55, 1698–1711. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhang, L.; Cao, Y. Dynamic Estimation of Spin Spacecraft Based on Multiple-Station ISAR Images. IEEE Trans. Geosci. Remote Sens. 2019, 58, 2977–2989. [Google Scholar] [CrossRef]
- Avilés, M.; Margarit, G.; Canetri, M.; Lemmens, S. Automated attitude estimation from ISAR images. In Proceedings of the 7th European Conference on Space Debris, Darmstadt, Germany, 18–21 April 2017; pp. 1–13. [Google Scholar]
- Xie, P.; Zhang, L.; Du, C.; Wang, X.; Zhong, W. Space Target Attitude Estimation from ISAR Image Sequences with Key Point Extraction Network. IEEE Signal Process. Lett. 2021, 28, 1041–1045. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhang, L.; Cao, Y.; Huang, Y. Optical-and-radar image fusion for dynamic estimation of spin satellites. IIEEE Trans. Image Process. 2019, 29, 2963–2976. [Google Scholar] [CrossRef] [PubMed]
- Suwa, K.; Wakayama, T.; Iwamoto, M. Three-dimensional target geometry and target motion estimation method using multi-static ISAR movies and its performance. IEEE Trans. Geosci. Remote Sens. 2011, 49, 2361–2373. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhang, L.; Cao, Y. Attitude estimation for space targets by exploiting the quadratic phase coefficients of inverse synthetic aperture radar imagery. IEEE Trans. Geosci. Remote Sens. 2019, 57, 3858–3872. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhang, L.; Wei, S.; Cao, Y. Dynamic Analysis of Spin Satellites Through the Quadratic Phase Estimation in Multiple-Station Radar Images. IEEE Trans. Comput. Imaging 2020, 6, 894–907. [Google Scholar] [CrossRef]
- Lefferts, E.; Markley, F.; Shuster, M.D. Kalman filtering for spacecraft attitude estimation. J. Guid. Control. Dyn. 1982, 5, 417–429. [Google Scholar] [CrossRef]
- Virgili, B.B.; Lemmens, S.; Krag, H. Investigation on Envisat attitude motion. In Proceedings of the e. Deorbit Workshop, Noordwijk, The Netherlands, 6 May 2014. [Google Scholar]
- Huo, C.Y.; Yin, H.C.; Xing, X.Y.; Liang, M. Attitude direction estimation for space target antenna load based on radar image features. Chin. J. Radio Sci. 2019, 34, 45–51. [Google Scholar]
- Zhang, Y.; Yang, X.; Jiang, X.R. Attitude direction estimation of space target parabolic antenna loads using sequential terahertz ISAR images. J. Infrared Millim. Waves 2021, 40, 496–507. [Google Scholar]




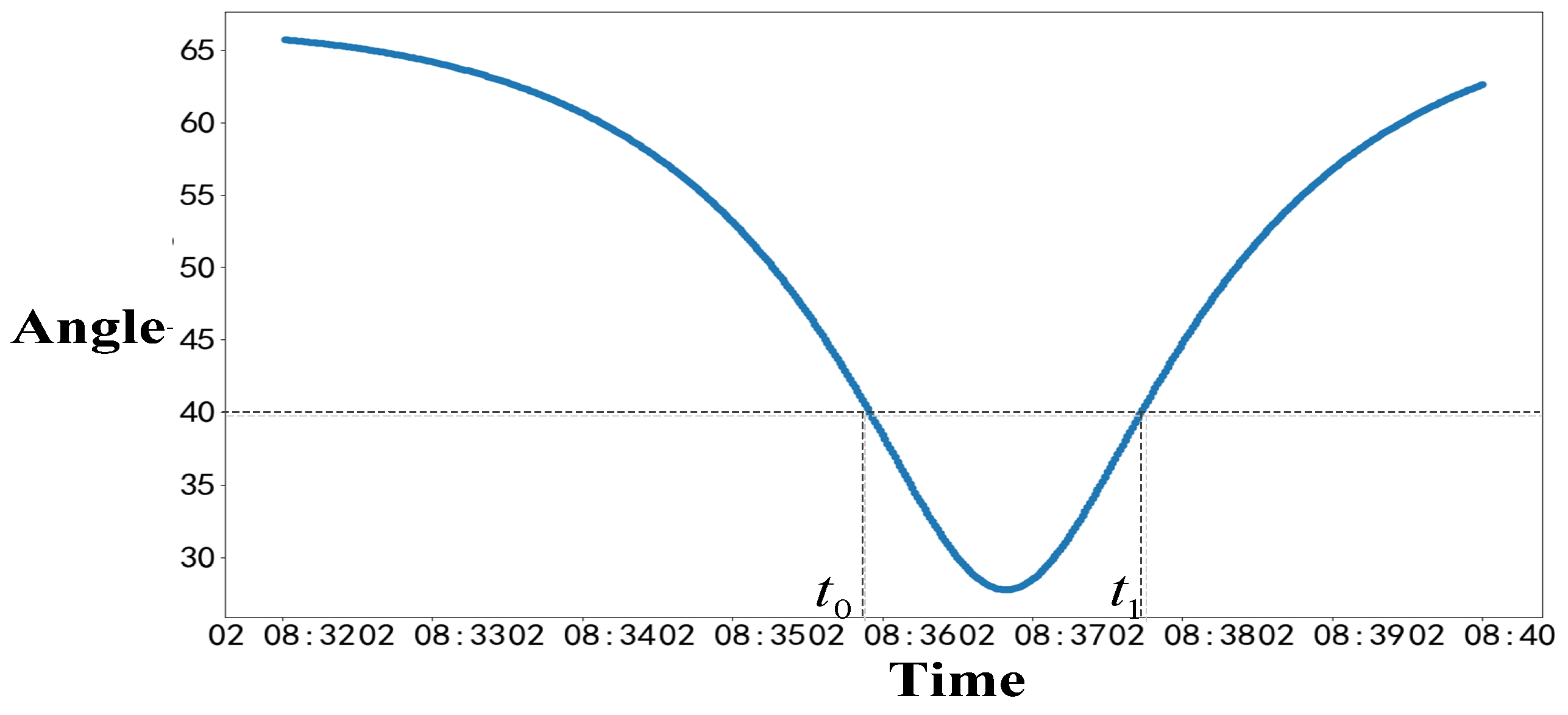



| Time Setting (UTC) | Start Time: | 2022-4-2 8:32:00 |
| End Time: | 2022-4-2 8:40:00 | |
| Satellite Orbital Parameters | Inclination: | 140.9213 |
| RA of the Ascending Node: | 208.9937 | |
| Eccentricity: | 0.0001218 | |
| Perigee Argument: | 162.7295 | |
| Mean Anomaly: | 1.2422 | |
| Mean angular velocity: | 15.16888613 | |
| Radar Station Position | Longitude: | 110 |
| Latitude: | 32 | |
| Height: | 0.1 |
| Setting Value | Inversion Value |
|---|---|
| Value 1: | |
| Value 2: |
| Setting Value | Inversion Value Single-Station | Inversion Value Multi-Station |
|---|---|---|
| 1: | ||
| 2: | ||
| 1: | ||
| 2: | ||
| 1: | ||
| 2: | ||
| 1: | ||
| 2: |
| Number | Orbital Altitude/km | Roll Angle | Pitch Angle | Station Layout Position | Inversion Result | Error |
|---|---|---|---|---|---|---|
| 1 | 500 | 10 | −25 | Lon1 = Lon2 Lat1 Lat2 | ||
| 2 | 500 | 25 | 0 | Lon1 Lon2 Lat1 = Lat2 | ||
| 3 | 500 | 40 | 25 | Lon1 Lon2 Lat1 Lat2 | ||
| 4 | 750 | 10 | 0 | Lon1 Lon2 Lat1 Lat2 | ||
| 5 | 750 | 25 | 25 | Lon1 = Lon2 Lat1 Lat2 | ||
| 6 | 750 | 40 | −25 | Lon1 Lon2 Lat1 = Lat2 | ||
| 7 | 1000 | 10 | 25 | Lon1 Lon2 Lat1 = Lat2 | ||
| 8 | 1000 | 25 | −25 | Lon1 Lon2 Lat1 Lat2 | ||
| 9 | 1000 | 40 | 0 | Lon1 = Lon2 Lat1 Lat2 |
| System Input | System Output |
|---|---|
| Satellite orbital elements | |
| Location of the tracking station | |
| : 20:47:33 | |
| : 20:50:44 | |
| Semi-conical angle of the parabolic antenna: | |
| Max side-swing angle of the antenna: |
| Satellite | Arc Segment | Measurement Time (UTC) | Moments | Inversion Result |
|---|---|---|---|---|
| Haiwangxing 01 | 1 | Start Time: 2024-03-24 04:35:00 | 04:36:05 | |
| End Time: 2024-03-24 04:40:10 | 04:39:10 | |||
| 2 | Start Time: 2024-10-11 03:22:00 | 03:23:04 | ||
| End Time: 2024-10-11 03:25:10 | 03:24:32 | |||
| TECSAR-2 | 1 | Start Time: 2022-04-28 05:10:00 | 05:11:08 | |
| End Time: 2022-04-28 05:16:10 | 05:15:06 | |||
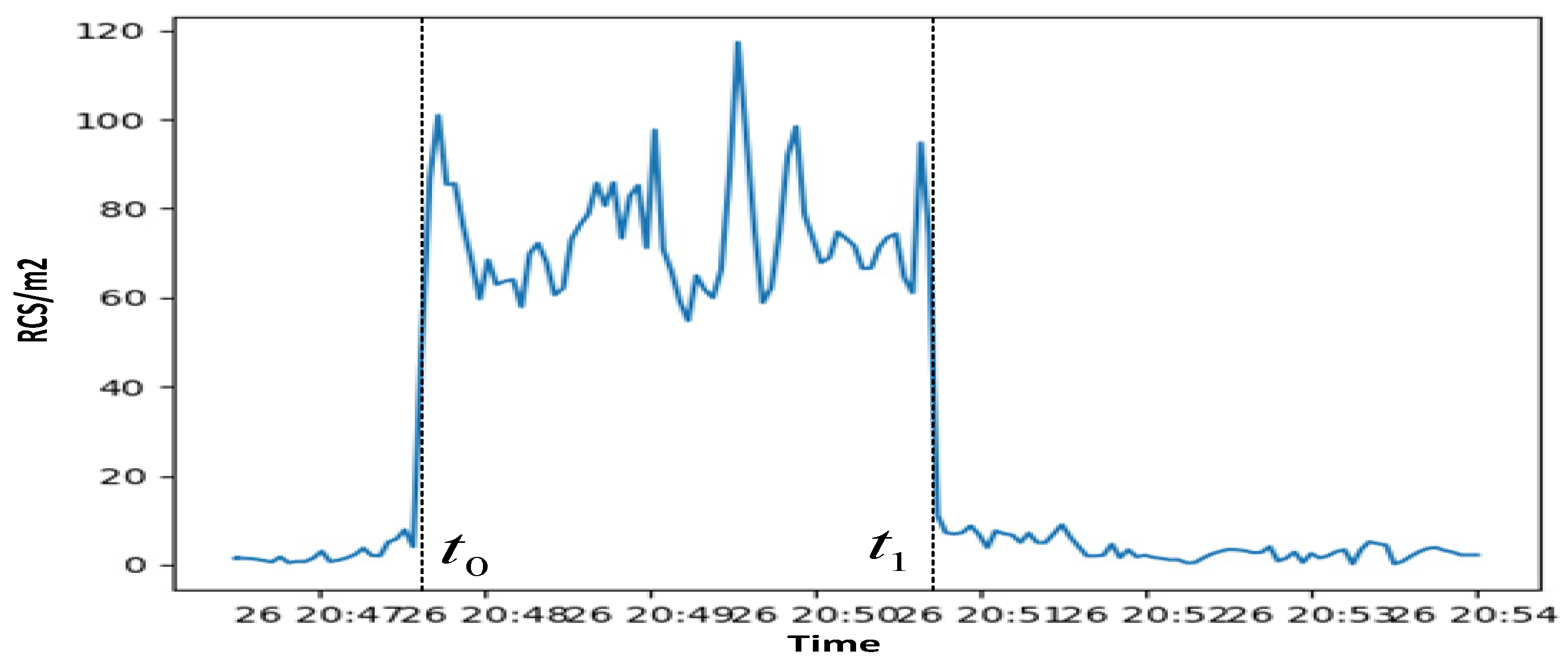
| 2 | Start Time: 2022-05-26 20:47:00 | 20:47:33 | ||
| End Time: 2022-05-26 20:54:00 | 20:50:44 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Ning, X. The Spacecraft Parabolic Antenna Payload Orientation Estimation Method Based on the Step Effect of Measured Radar Cross Section Sequences. Remote Sens. 2024, 16, 4259. https://doi.org/10.3390/rs16224259
Li J, Ning X. The Spacecraft Parabolic Antenna Payload Orientation Estimation Method Based on the Step Effect of Measured Radar Cross Section Sequences. Remote Sensing. 2024; 16(22):4259. https://doi.org/10.3390/rs16224259
Chicago/Turabian StyleLi, Junzhi, and Xin Ning. 2024. "The Spacecraft Parabolic Antenna Payload Orientation Estimation Method Based on the Step Effect of Measured Radar Cross Section Sequences" Remote Sensing 16, no. 22: 4259. https://doi.org/10.3390/rs16224259
APA StyleLi, J., & Ning, X. (2024). The Spacecraft Parabolic Antenna Payload Orientation Estimation Method Based on the Step Effect of Measured Radar Cross Section Sequences. Remote Sensing, 16(22), 4259. https://doi.org/10.3390/rs16224259





