Dual-Mode Sea Ice Extent Retrieval for the Rotating Fan Beam Scatterometer
Abstract
1. Introduction
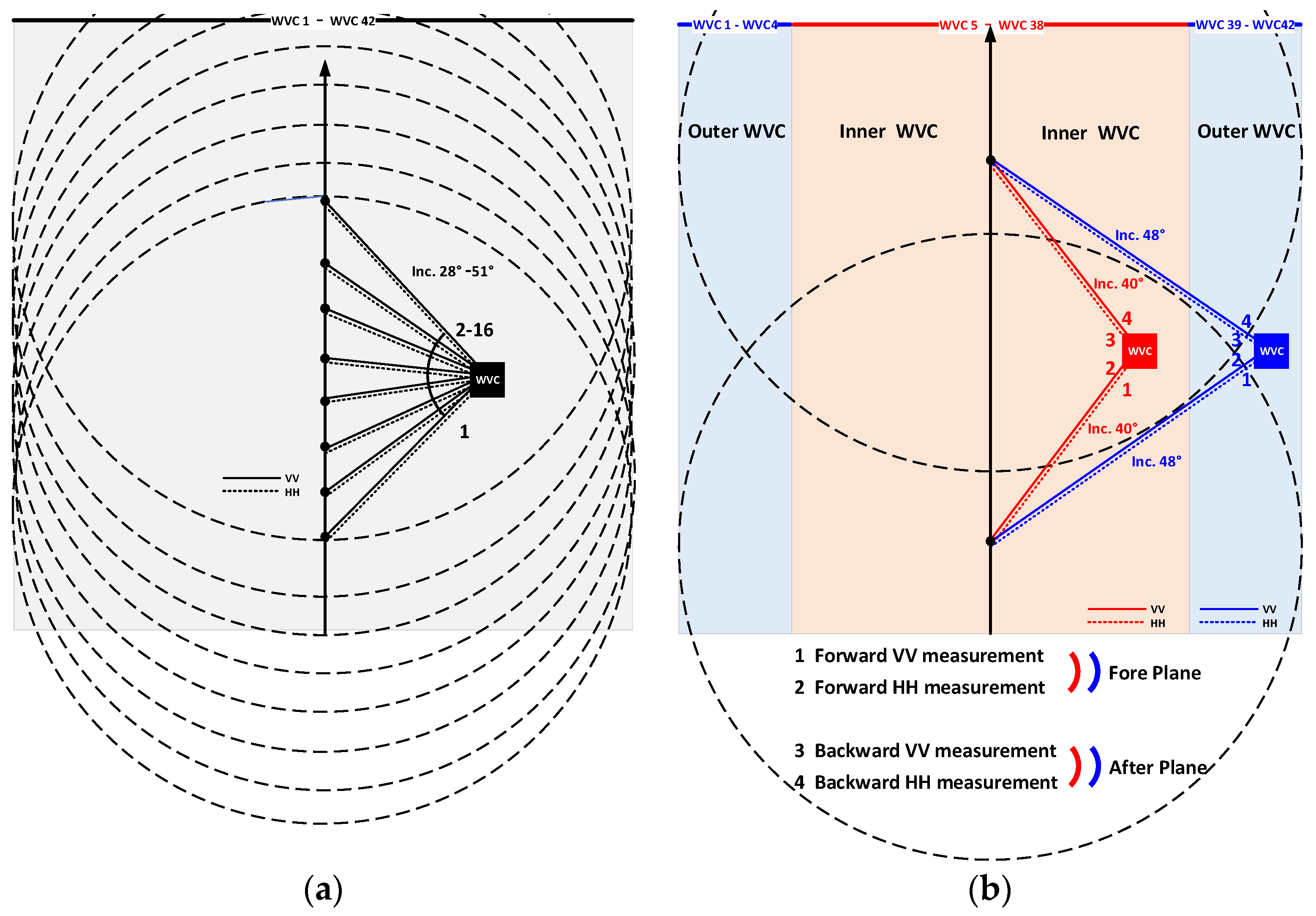
2. The Equivalent Rotating Pencil Beam Mode
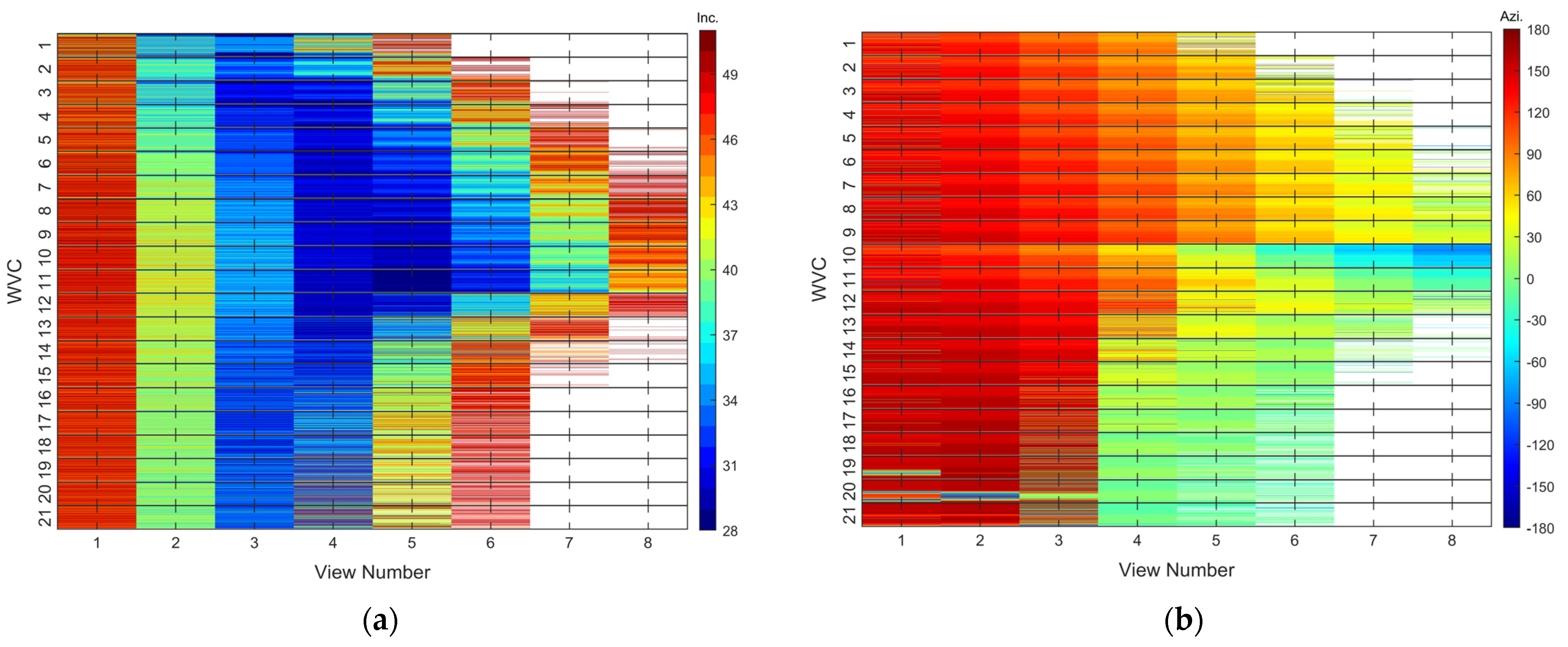
2.1. Data Preprocessing
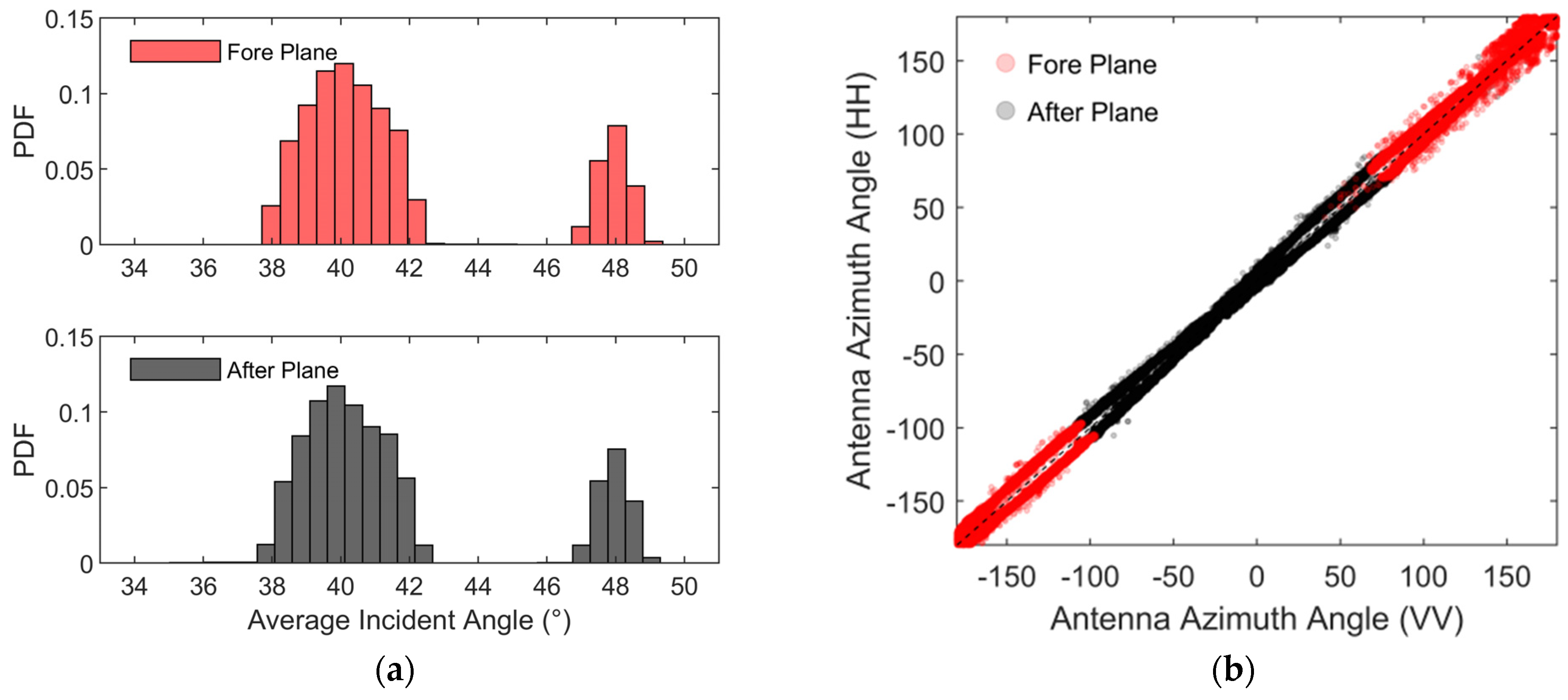
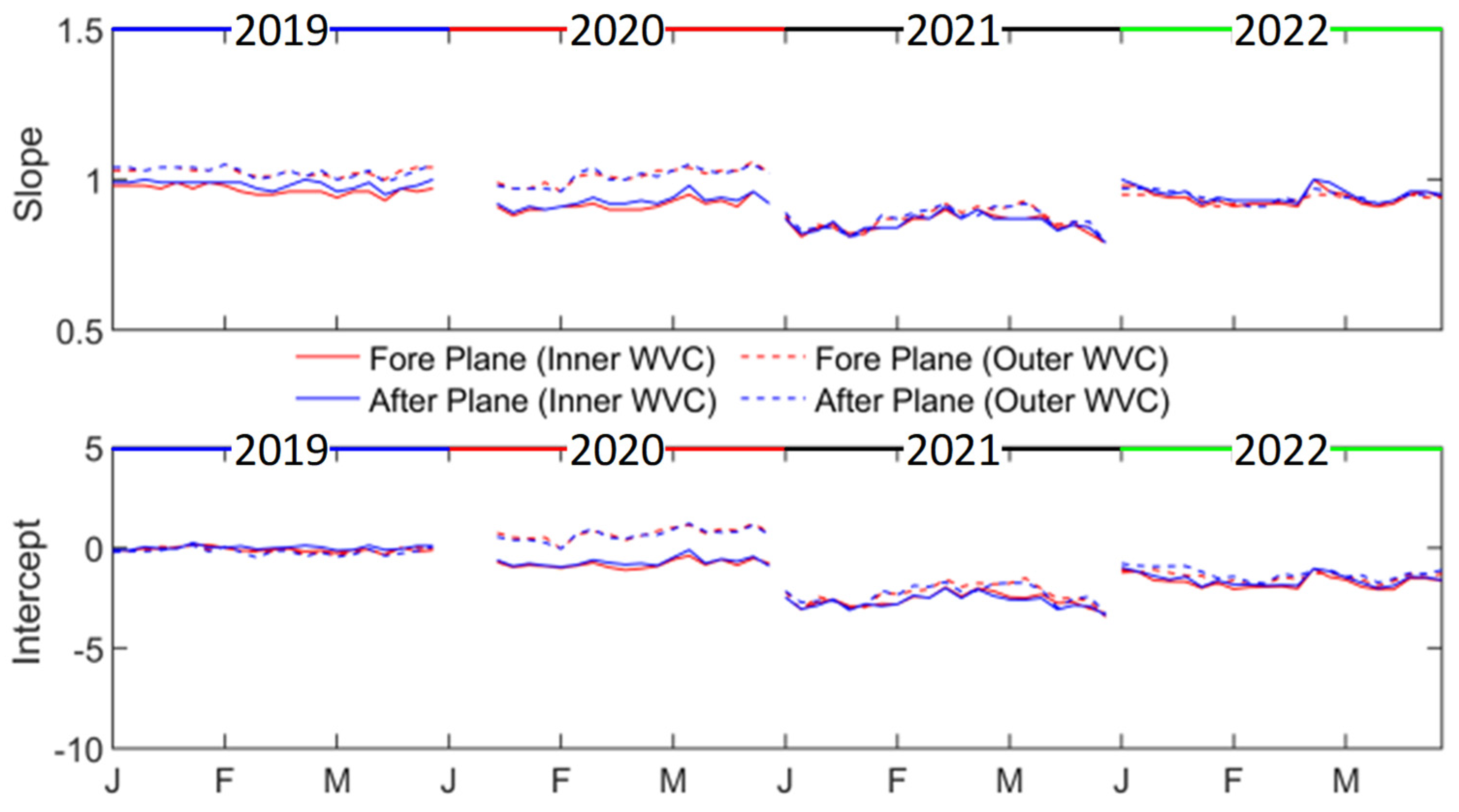
2.2. Algorithm Parameters
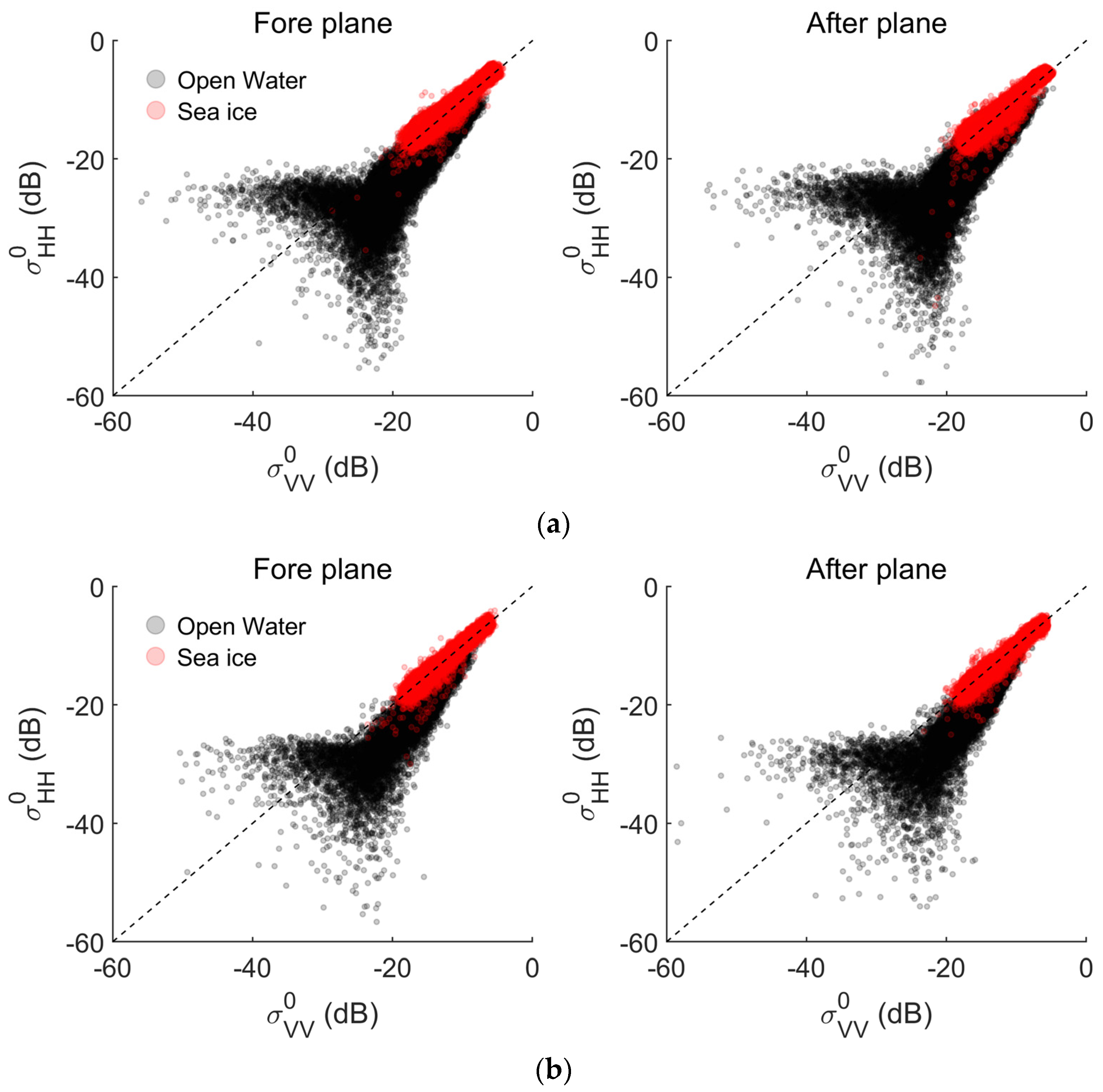
2.2.1. GMFs
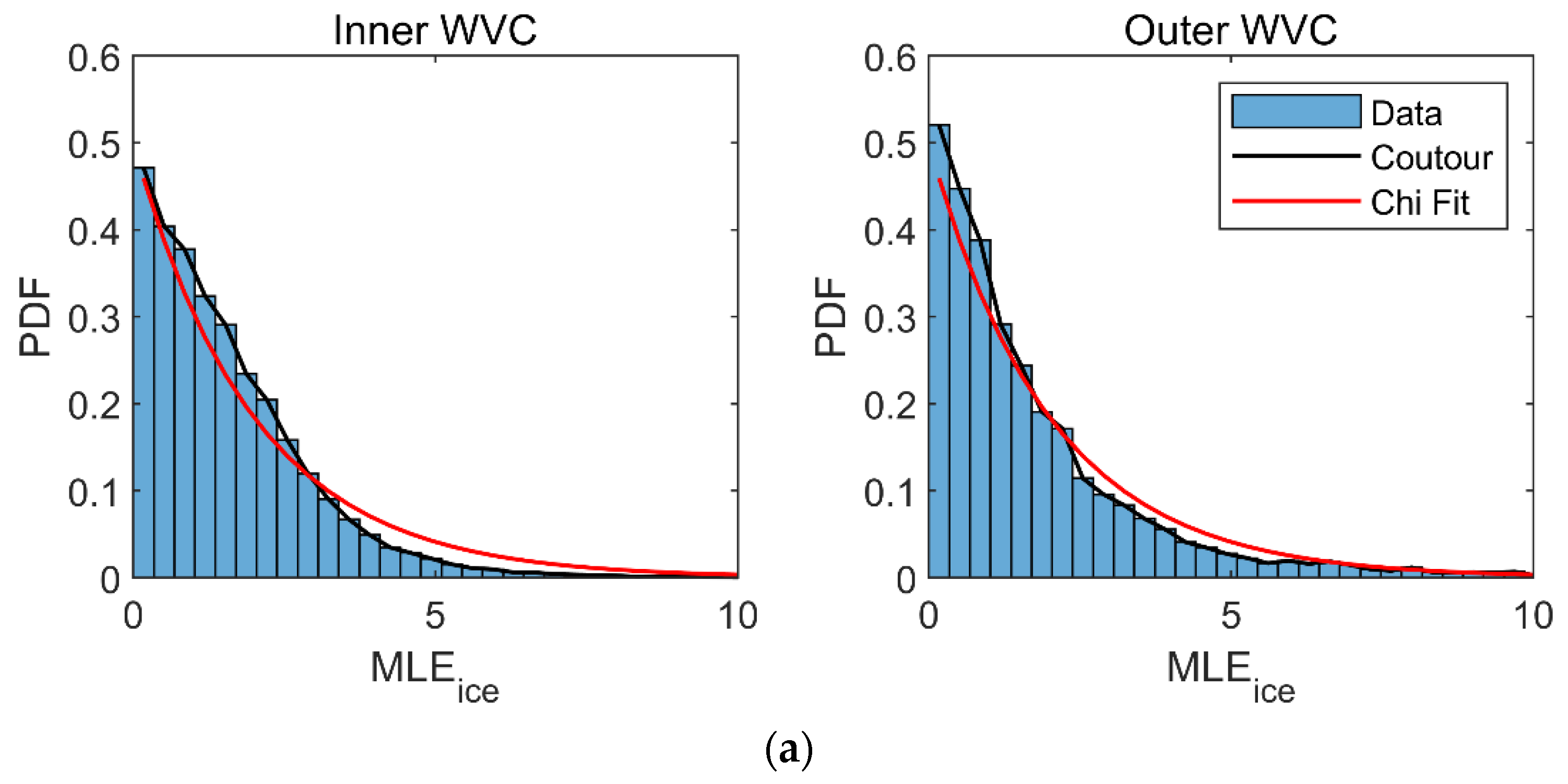
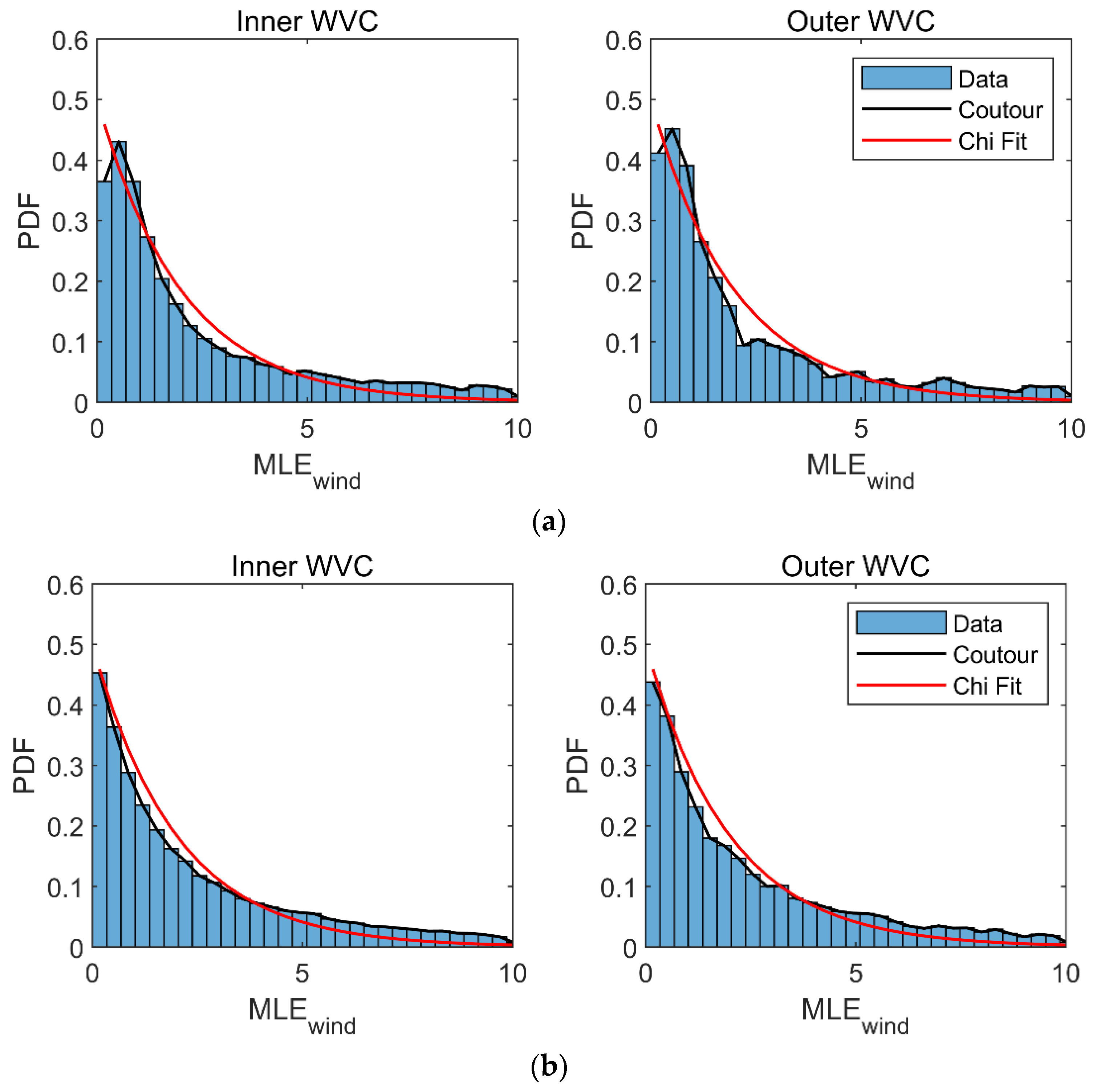
2.2.2. MLEs
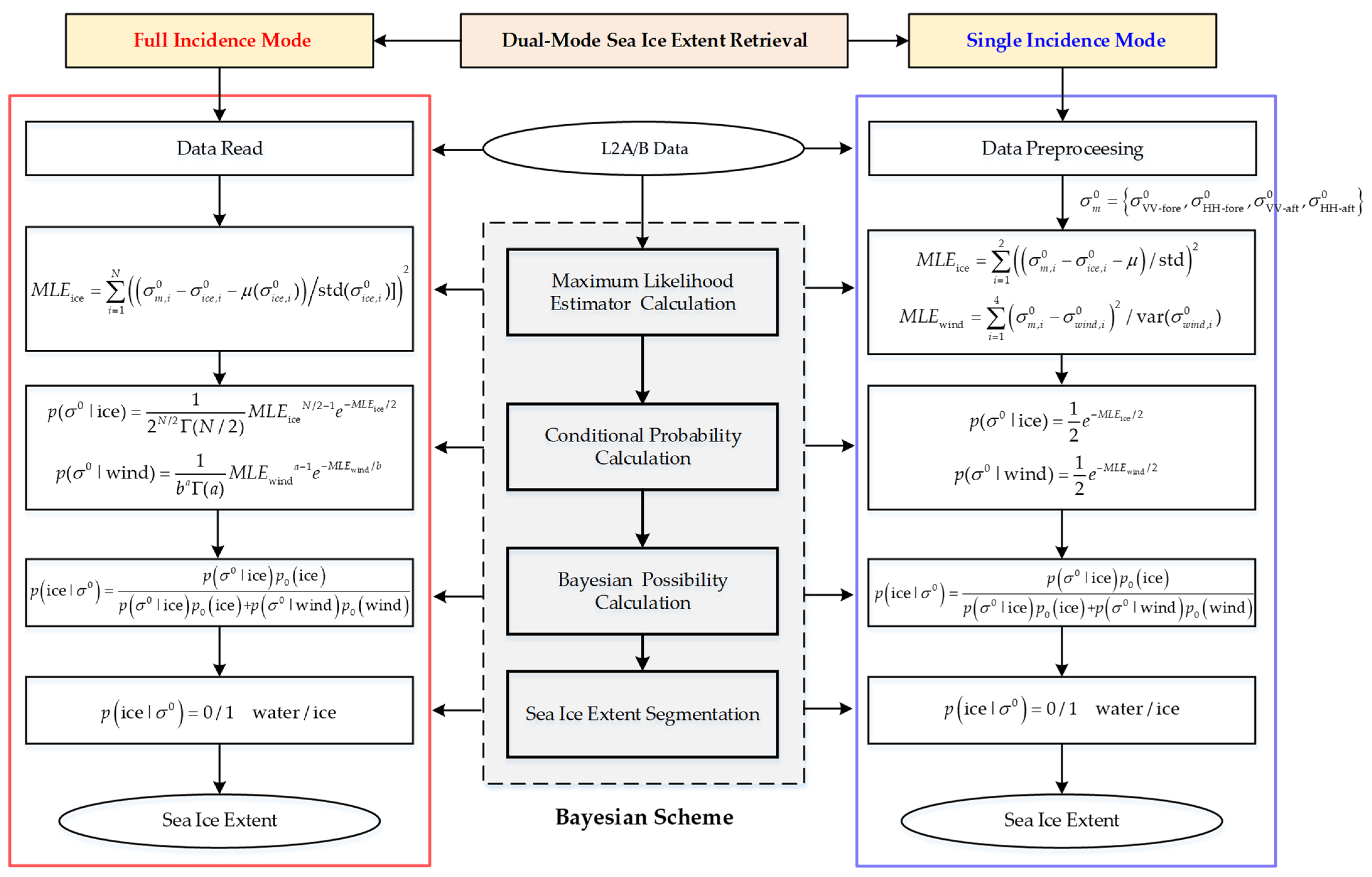
3. Dual-Mode Sea Ice Extent Retrieval Process
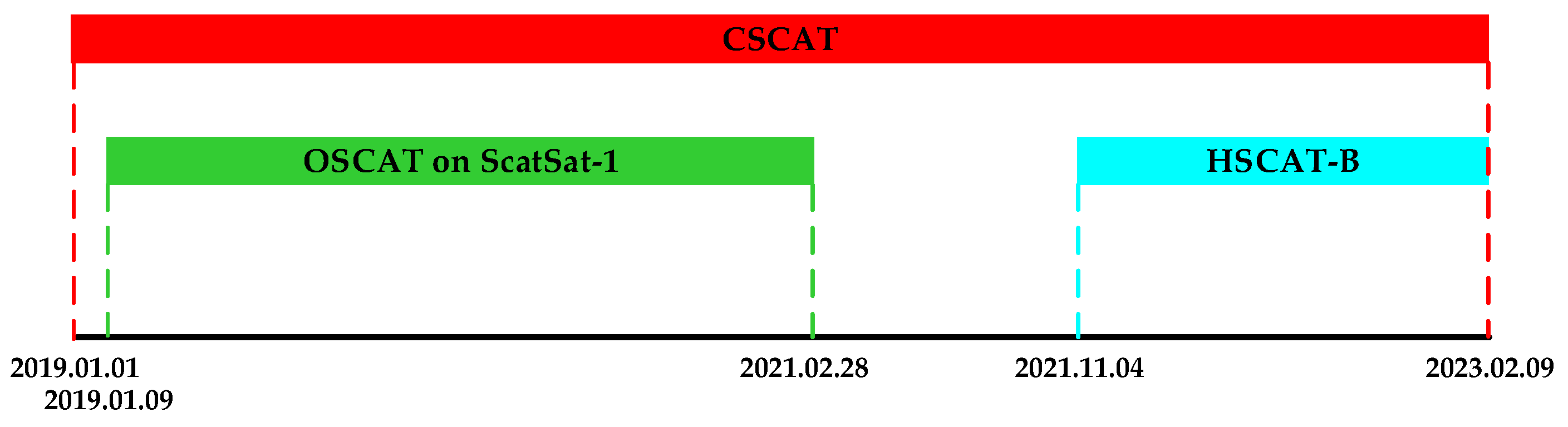
4. Results and Discussion
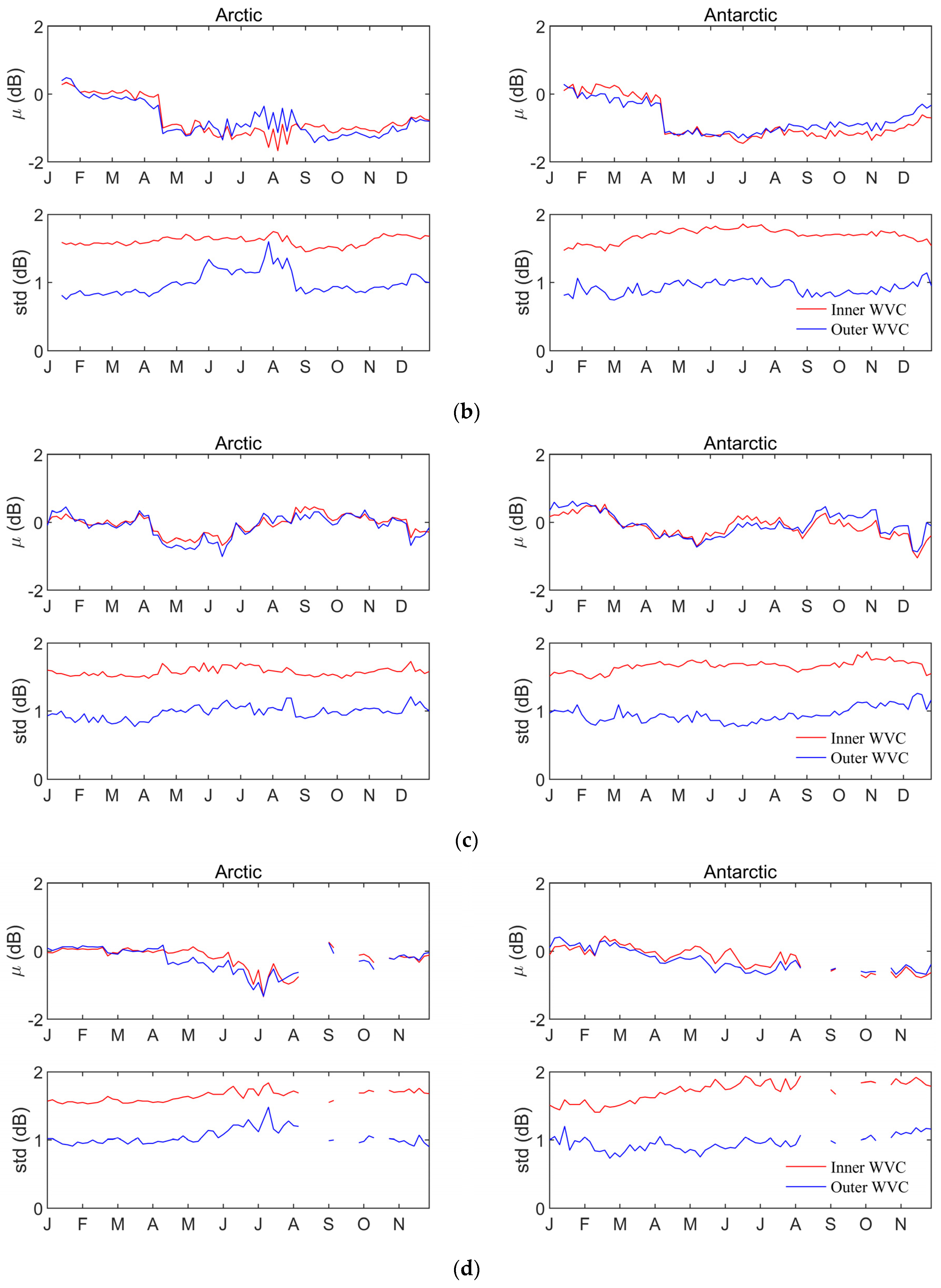
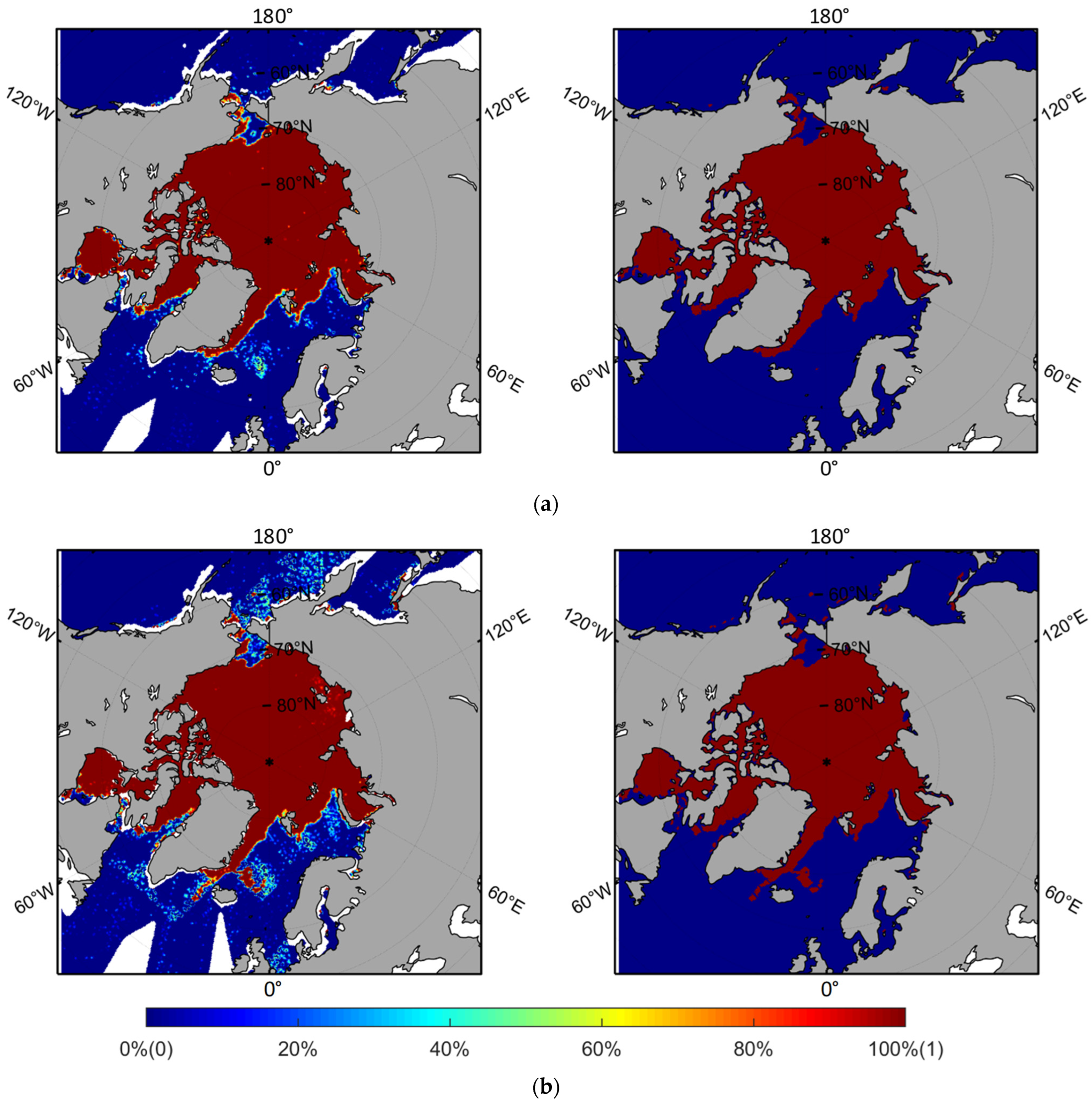
4.1. Dual-Mode Sea Ice Extent
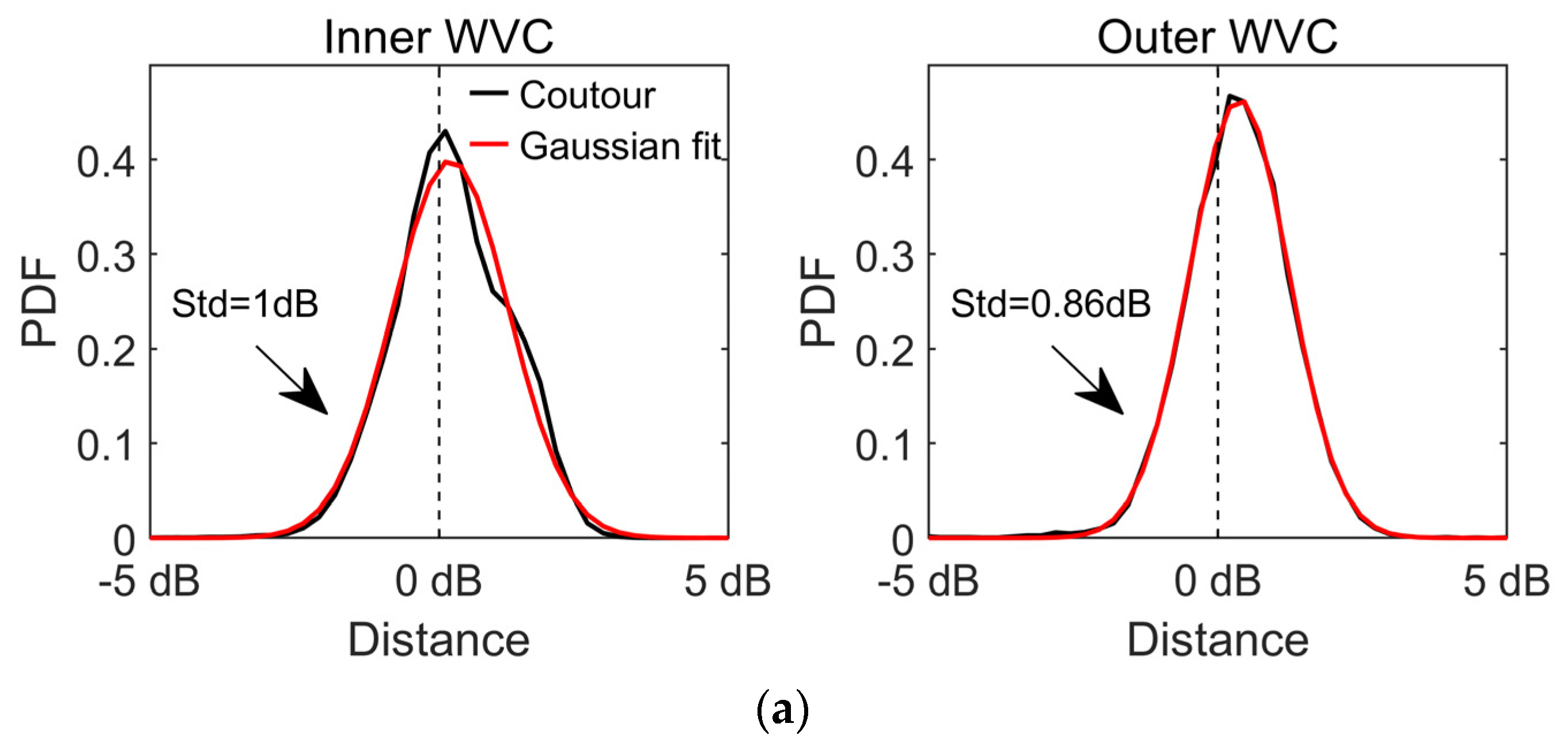
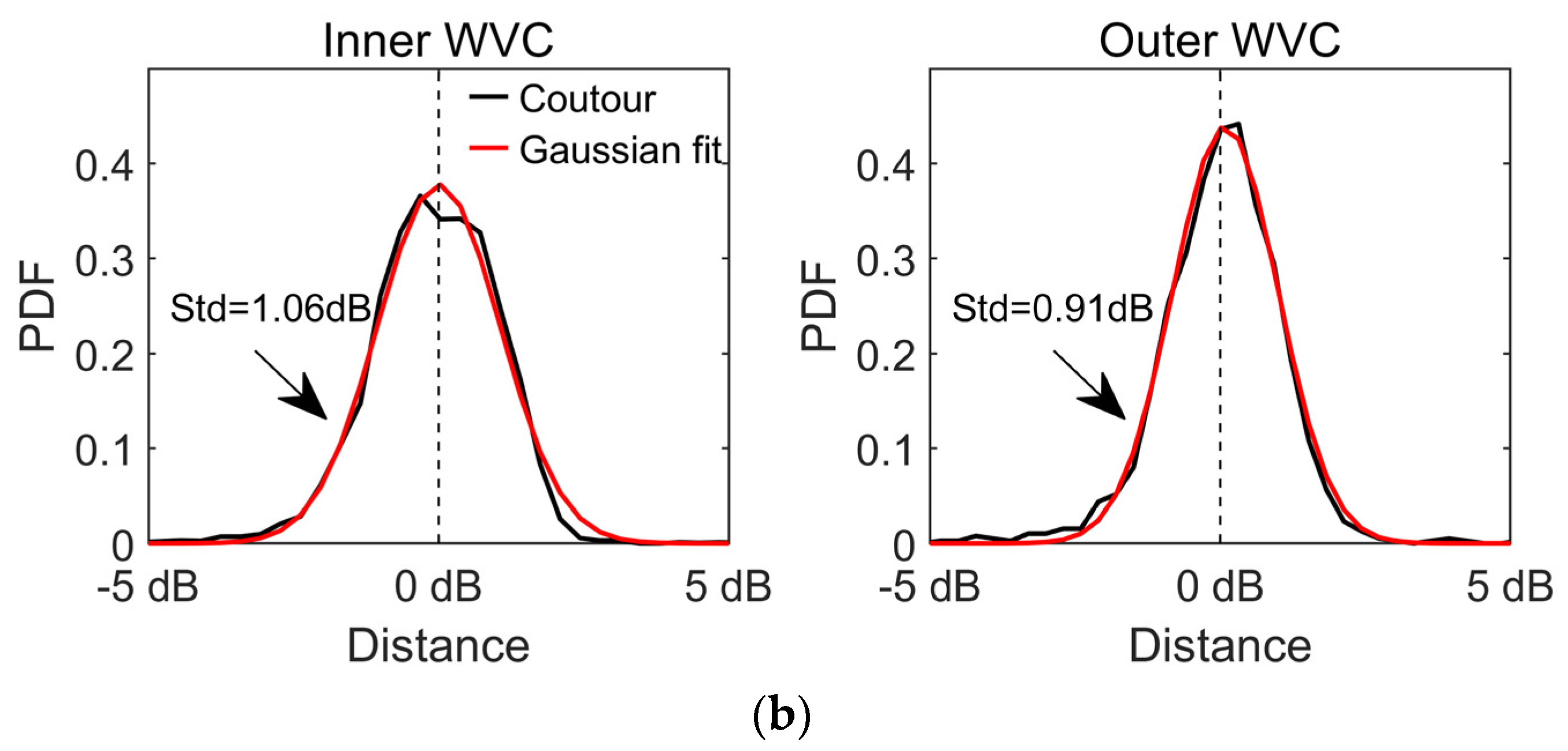
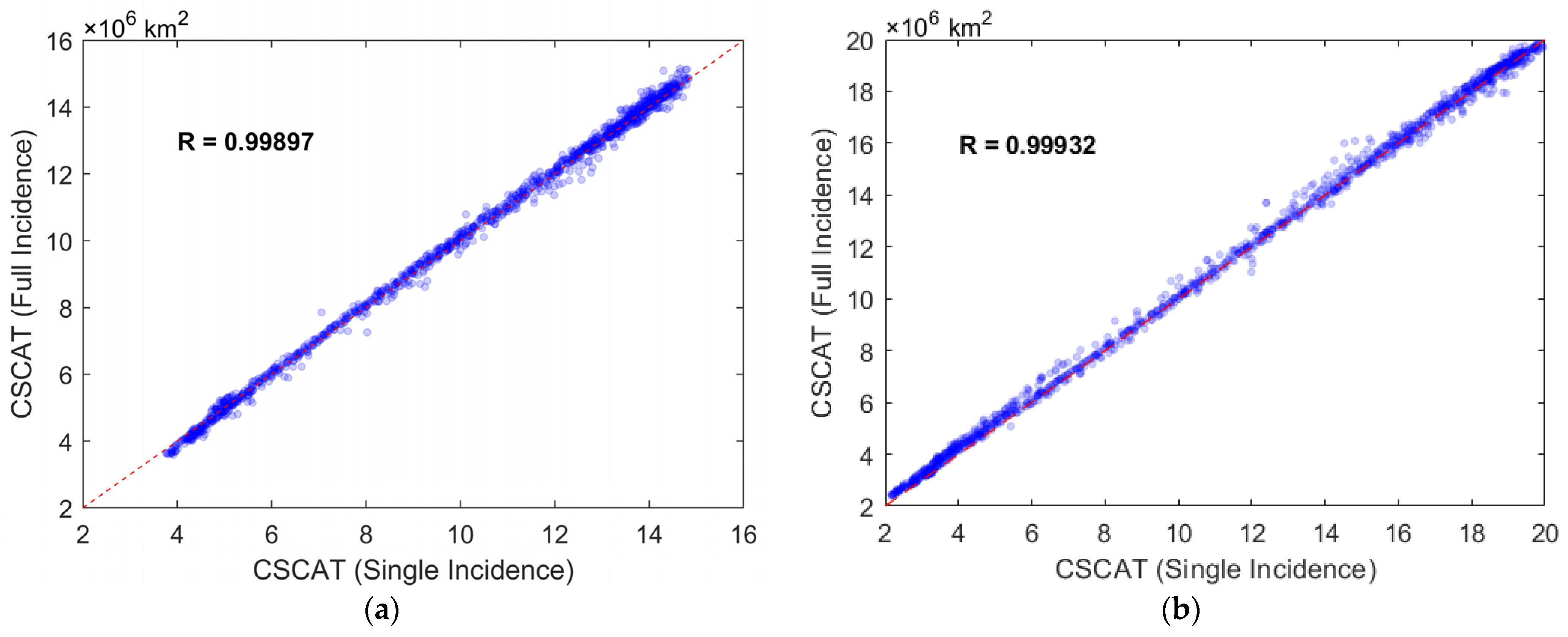
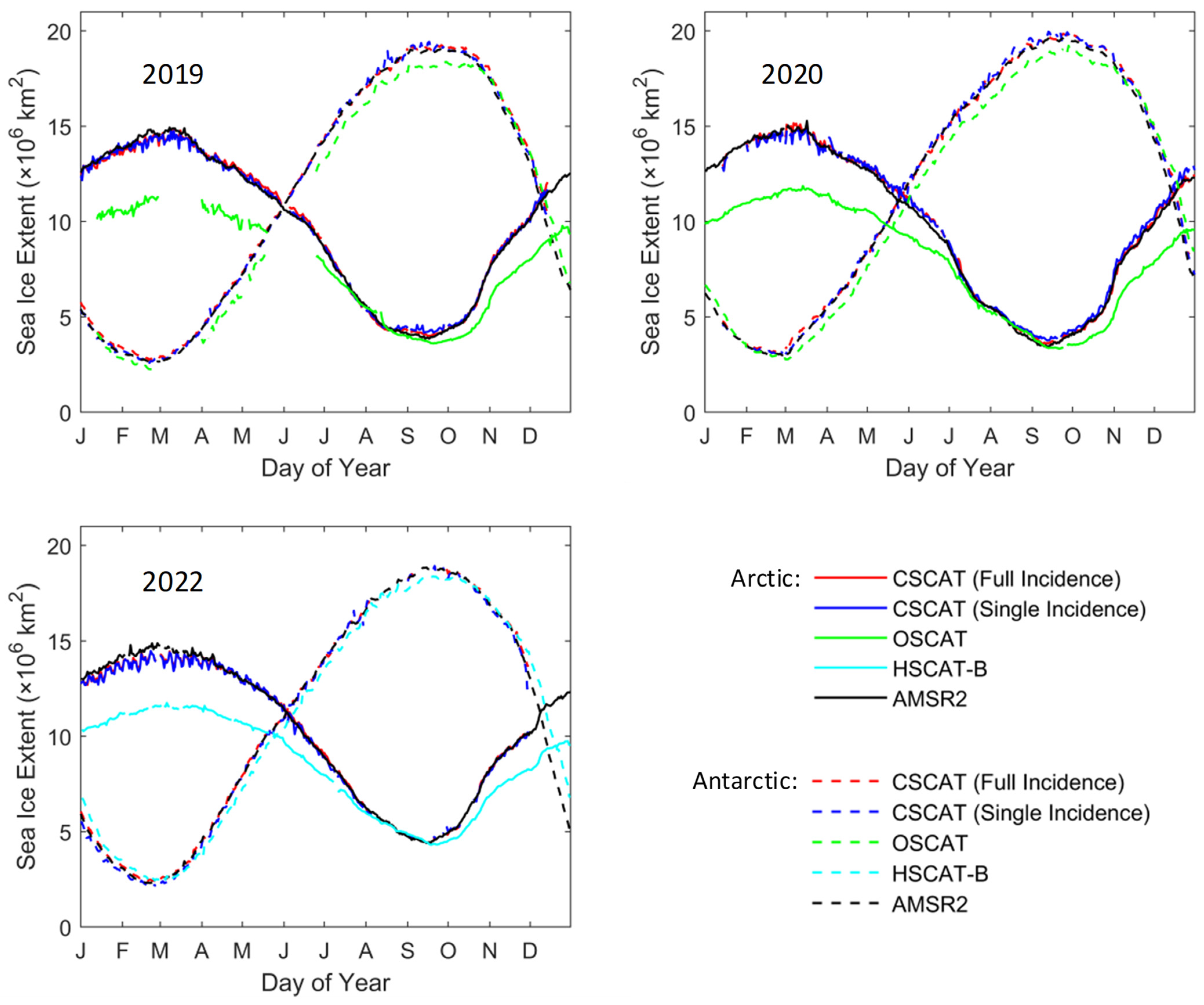
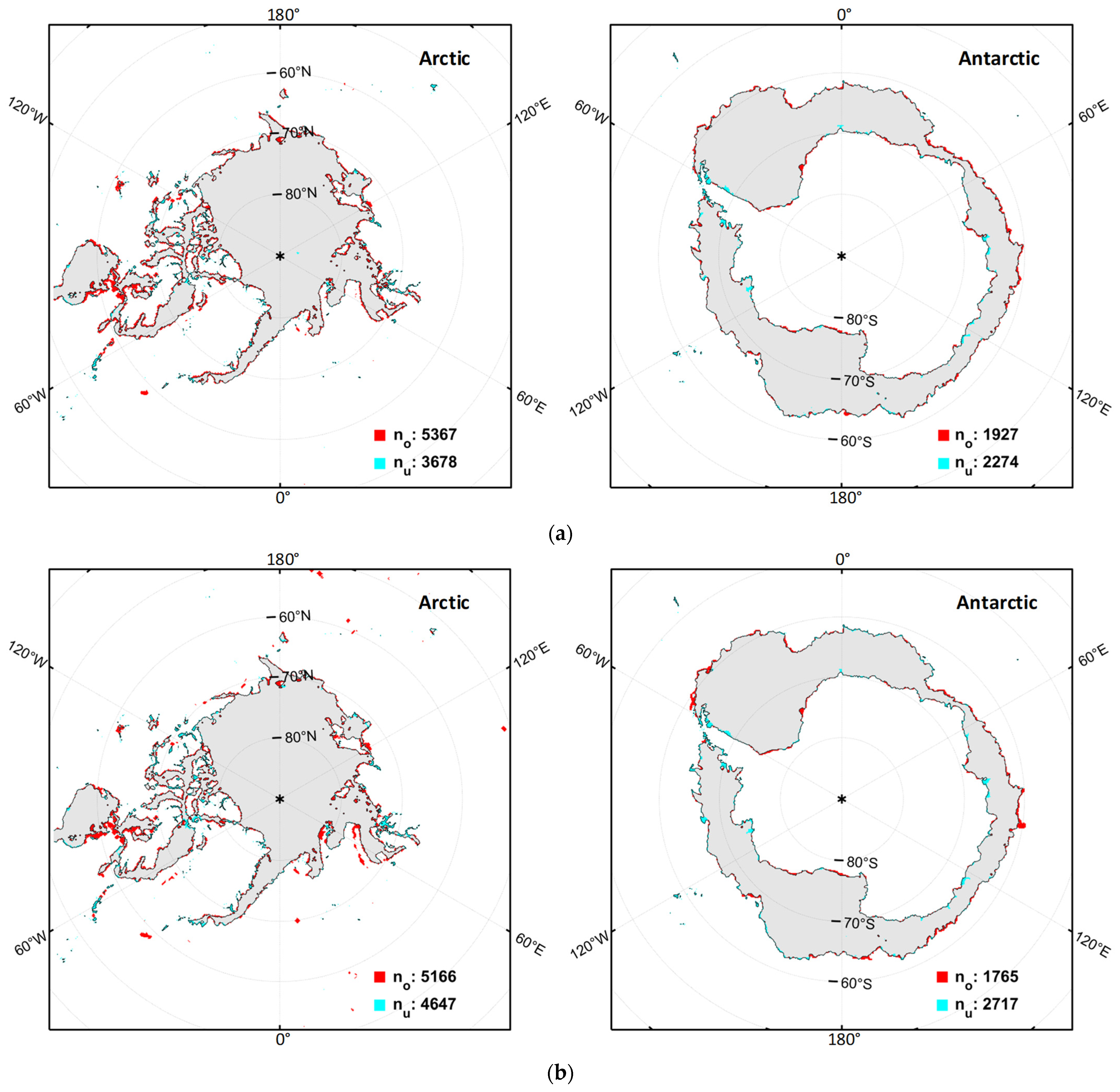
4.2. Validation
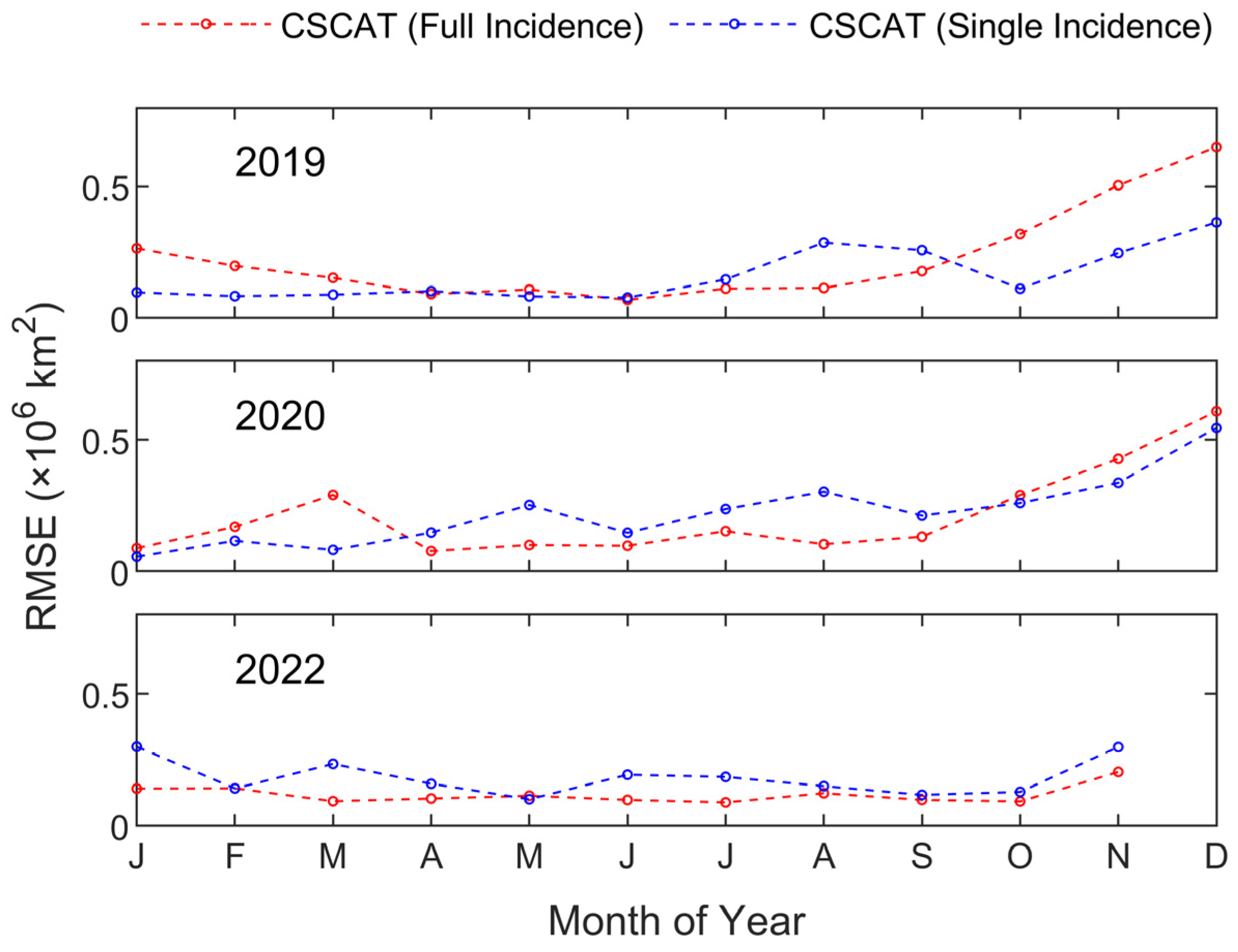
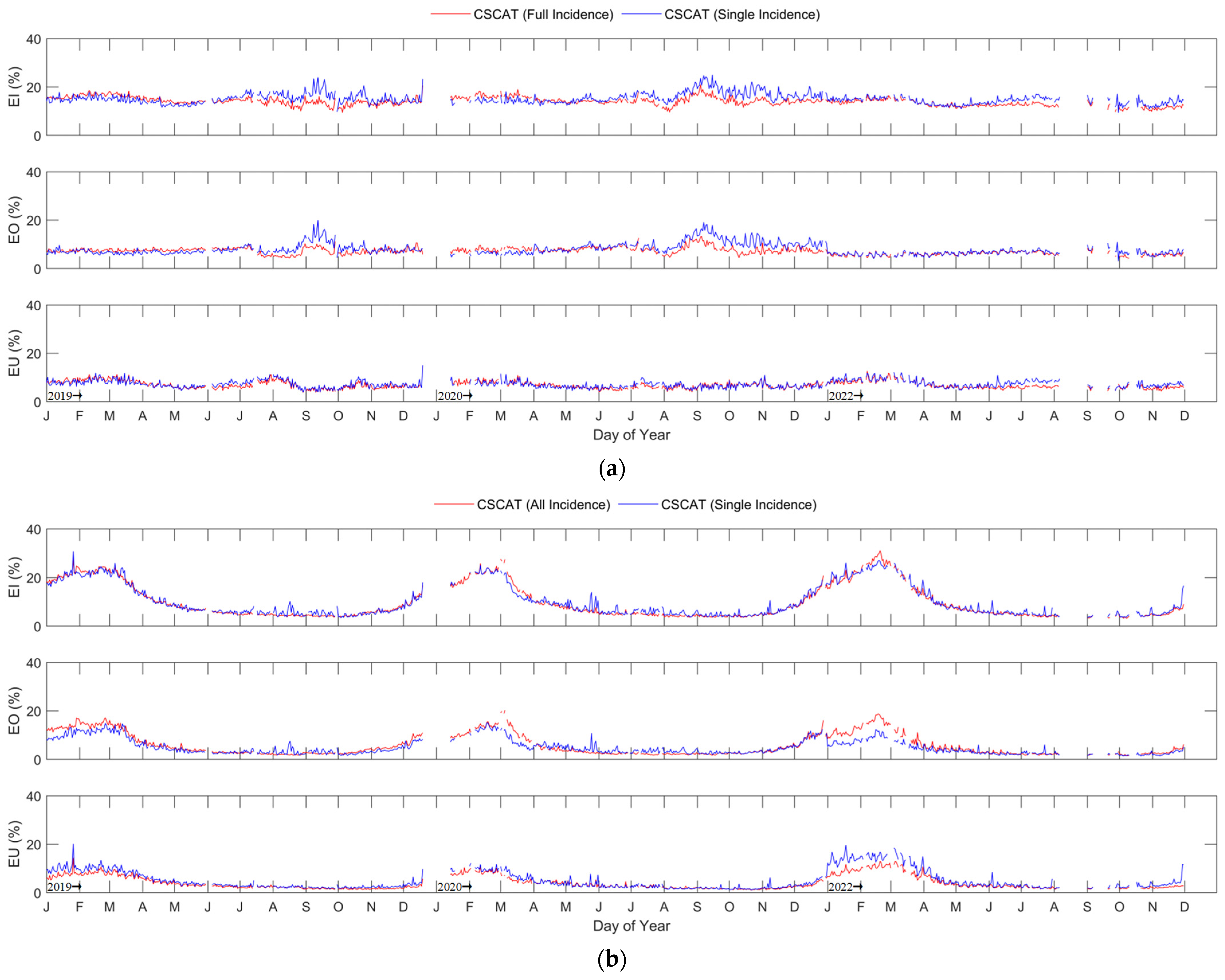
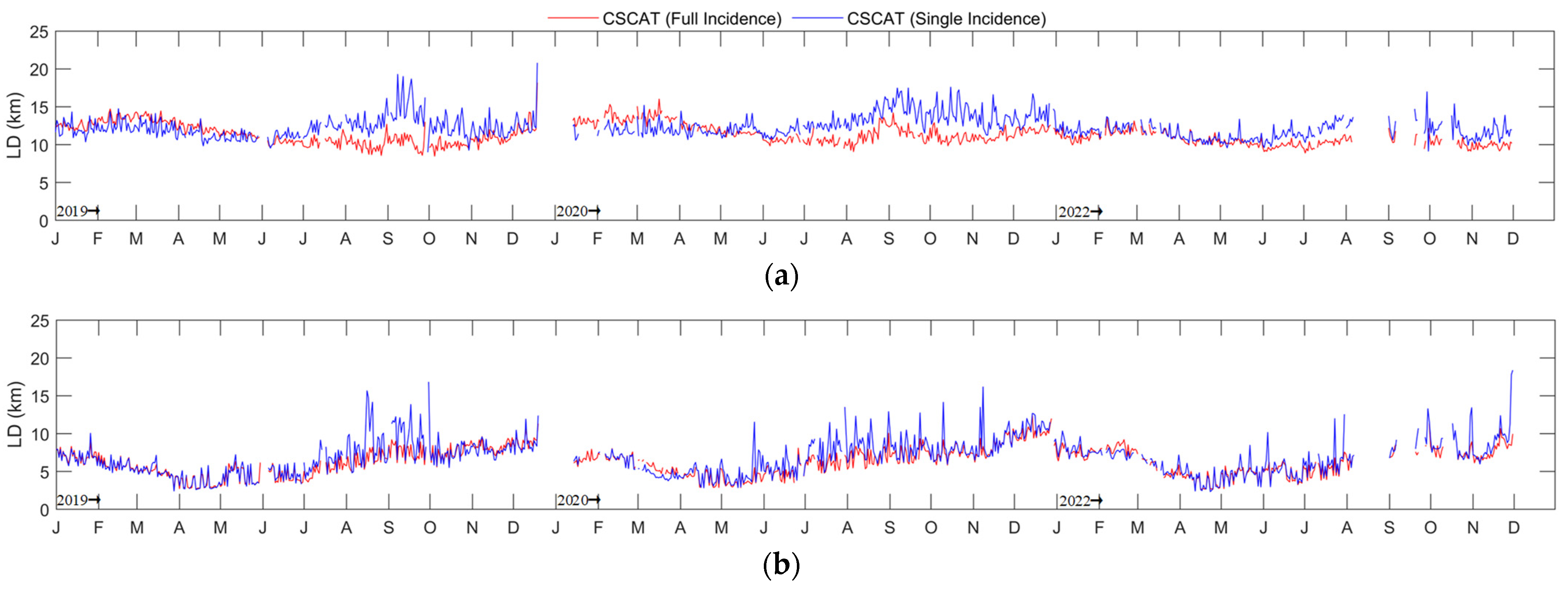
4.3. Evaluation of the Dual-Mode Sea Ice Extent
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Singh, K. Studies of Polar Ice Using SCATSAT-1 Data. Ph.D. Thesis, National Institute of Technology Manipur, Imphal, India, 2024. [Google Scholar]
- Willis, M.D.; Lannuzel, D.; Else, B.; Angot, H.; Campbell, K.; Crabeck, O.; Delille, B.; Hayashida, H.; Lizotte, M.; Loose, B.; et al. Polar oceans and sea ice in a changing climate. Elem. Sci. Anthr. 2023, 11, 00056. [Google Scholar] [CrossRef]
- Singh, S.; Tiwari, R.K.; Sood, V.; Kaur, R.; Prashar, S. The Legacy of Scatterometers: Review of applications and perspective. IEEE Geosci. Remote Sens. Mag. 2022, 10, 39–65. [Google Scholar] [CrossRef]
- Sandven, S.; Spreen, G.; Heygster, G.; Girard-Ardhuin, F.; Farrell, S.L.; Dierking, W.; Allard, R.A. Sea Ice Remote Sensing—Recent Developments in Methods and Climate Data Sets. SGeo 2023, 44, 1653–1689. [Google Scholar] [CrossRef]
- Long, D.G. Polar Applications of Spaceborne Scatterometers. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2017, 10, 2307–2320. [Google Scholar] [CrossRef]
- Amani, M.; Mohseni, F.; Layegh, N.F.; Nazari, M.E.; Fatolazadeh, F.; Salehi, A.; Ahmadi, S.A.; Ebrahimy, H.; Ghorbanian, A.; Jin, S.; et al. Remote Sensing Systems for Ocean: A Review (Part 2: Active Systems). IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2022, 15, 1421–1453. [Google Scholar] [CrossRef]
- Yun, R.; Dong, X.; Liu, J.; Lin, W.; Zhu, D.; Ma, J.; Lang, S.; Wang, Z. CFOSAT Rotating Fan-Beam Scatterometer Backscatter Measurement Processing. Earth Space Sci. 2021, 8, 1–18. [Google Scholar] [CrossRef]
- Shang, J.; Wang, Z.; Dou, F.; Yuan, M.; Yin, H.; Liu, L.; Wang, Y.; Hu, X.; Zhang, P. Preliminary Performance of the WindRAD Scatterometer Onboard the FY-3E Meteorological Satellite. IEEE Trans. Geosci. Remote Sens. 2024, 62, 1–13. [Google Scholar] [CrossRef]
- Zhai, X.; Wang, Z.; Zheng, Z.; Xu, R.; Dou, F.; Xu, N.; Zhang, X. Sea Ice Monitoring with CFOSAT Scatterometer Measurements Using Random Forest Classifier. Remote Sens. 2021, 13, 4686. [Google Scholar] [CrossRef]
- Li, Z.; Verhoef, A.; Stoffelen, A. Bayesian Sea Ice Detection Algorithm for CFOSAT. Remote Sens. 2022, 14, 3569. [Google Scholar] [CrossRef]
- Xu, C.; Wang, Z.; Zhai, X.; Lin, W.; He, Y. SVM-Based Sea Ice Extent Retrieval Using Multisource Scatterometer Measurements. Remote Sens. 2023, 15, 1630. [Google Scholar] [CrossRef]
- Liu, L.; Dong, X.; Lin, W.; Lang, S. Polar Sea Ice Detection Using a Rotating Fan Beam Scatterometer. Remote Sens. 2023, 15, 5063. [Google Scholar] [CrossRef]
- Liu, L.; Dong, X.; Yang, L.; Lin, W.; Lang, S. Sea Ice Extent Retrieval Using CSCAT 12. 5 km Sampling Data. Remote Sens. 2024, 16, 700. [Google Scholar] [CrossRef]
- Xu, R.; Zhao, C.; Zhai, X.; Chen, G. Arctic Sea Ice Type Classification by Combining CFOSCAT and AMSR-2 Data. Earth Space Sci. 2022, 9, 1–24. [Google Scholar] [CrossRef]
- Zhai, X.; Xu, R.; Wang, Z.; Zheng, Z.; Shou, Y.; Tian, S.; Tian, L.; Hu, X.; Xu, N. Classification of Arctic Sea Ice Type in CFOSAT Scatterometer Measurements Using a Random Forest Classifier. Remote Sens. 2023, 15, 1310. [Google Scholar] [CrossRef]
- Zhai, X.; Tian, S.; Ye, Y.; Cao, G.; Chen, L.; Xu, N.; Zheng, Z. First Results of Antarctic Sea Ice Classification Using Spaceborne Dual-Frequency Scatterometer FY-3E WindRAD. IEEE Geosci. Remote Sens. Lett. 2024, 21, 1–5. [Google Scholar] [CrossRef]
- Zhang, Z.; Yu, Y.; Shokr, M.; Li, X.; Ye, Y.; Cheng, X.; Chen, Z.; Hui, F. Intercomparison of Arctic Sea Ice Backscatter and Ice Type Classification Using Ku-Band and C-Band Scatterometers. IEEE Trans. Geosci. Remote Sens. 2022, 60, 1–18. [Google Scholar] [CrossRef]
- Rivas, M.B.; Stoffelen, A. New Bayesian Algorithm for Sea Ice Detection with QuikSCAT. IEEE Trans. Geosci. Remote Sens. 2011, 49, 1894–1901. [Google Scholar] [CrossRef]
- Rivas, M.B.; Otosaka, I.; Stoffelen, A.; Verhoef, A. A scatterometer record of sea ice extents and backscatter: 1992–2016. Cryosphere 2018, 12, 2941–2953. [Google Scholar] [CrossRef]
- OSCAT Winds at 25 km Swath Grid—ScatSat, EUMETSAT SAF on Ocean and Sea Ice. Available online: https://navigator.eumetsat.int/product/EO:EUM:DAT:0031 (accessed on 1 April 2024).
- HSCAT Winds at 25 km Swath Grid—Hai Yang 2B, EUMETSAT SAF on Ocean and Sea Ice. Available online: https://navigator.eumetsat.int/product/EO:EUM:DAT:0537 (accessed on 5 April 2024).
- Rivas, M.B.; Verspeek, J.; Verhoef, A.; Stoffelen, A. Bayesian Sea Ice Detection with the Advanced Scatterometer ASCAT. IEEE Trans. Geosci. Remote Sens. 2012, 50, 2649–2657. [Google Scholar] [CrossRef]
- Otosaka, I.; Rivas, M.B.; Stoffelen, A. Bayesian Sea Ice Detection with the ERS Scatterometer and Sea Ice Backscatter Model at C-Band. IEEE Trans. Geosci. Remote Sens. 2018, 56, 2248–2254. [Google Scholar] [CrossRef]
- Yang, S.; Zhang, L.; Lin, M.; Zou, J.; Mu, B.; Peng, H. Evaluation of Sea Surface Wind Products from Scatterometer Onboard the Chinese HY-2D Satellite. Remote Sens. 2023, 15, 852. [Google Scholar] [CrossRef]
- A Guide to NSIDC’s Polar Stereographic Projection, National Snow and Ice Data Center. Available online: https://nsidc.org/data/user-resources/help-center/guide-nsidcs-polar-stereographic-projection (accessed on 7 October 2023).
- AMSR-E/AMSR2 Unified L3 Daily 12.5 km Brightness Temperatures, Sea Ice Concentration, Motion & Snow Depth Polar Grids V001. Version 1. Available online: https://catalog.data.gov/dataset/amsr-e-amsr2-unified-l3-daily-12-5-km-brightness-temperatures-sea-ice-concentration-motion (accessed on 7 December 2023).
- Sun, Y.; Ye, Y.; Wang, S.; Liu, C.; Chen, Z.; Cheng, X. Evaluation of the AMSR2 Ice Extent at the Arctic Sea Ice Edge Using an SAR-Based Ice Extent Product. IEEE Trans. Geosci. Remote Sens. 2023, 61, 1–15. [Google Scholar] [CrossRef]






| Parameters | Rotating Pencil Beam | Rotating Fan Beam |
|---|---|---|
| Swath | continuous | continuous |
| Antenna | large | small |
| Scan speed | fast | low |
| Measurement resolution | >30 km | ~10 km |
| WVC size | 50/25 km | 25/12.5 km |
| Views of WVC | 2–4 | 2–16 |
| Data processing complexity | easy | complicated |
| Arctic Region | Antarctic Region | |||||
|---|---|---|---|---|---|---|
| 2019 | 2020 | 2022 | 2019 | 2020 | 2022 | |
| ×106 km2 | ||||||
| CSCAT (Full Incidence) vs. AMSR2 | 0.22 | 0.22 | 0.28 | 0.26 | 0.27 | 0.12 |
| CSCAT (Single Incidence) vs. AMSR2 | 0.23 | 0.31 | 0.32 | 0.18 | 0.27 | 0.20 |
| Arctic Region | Antarctic Region | |||||
|---|---|---|---|---|---|---|
| 2019 | 2020 | 2022 | 2019 | 2020 | 2022 | |
| RMSE (×106 km2) | ||||||
| CSCAT (Full Incidence) vs. OSCAT | 0.39 | 0.34 | × | 0.54 | 0.57 | × |
| CSCAT (Single Incidence) vs. HSCAT-B | × | × | 0.39 | × | × | 0.82 |
| AMSR2 vs. OSCAT | 0.34 | 0.36 | × | 0.75 | 0.81 | × |
| AMSR2 vs. HSCAT-B | × | × | 0.34 | × | × | 0.99 |
| AMSR2 vs. CSCAT (Single Incidence) | 0.10 | 0.12 | 0.10 | 0.19 | 0.22 | 0.12 |
| Relative coefficient (R) | ||||||
| CSCAT (Full Incidence) vs. OSCAT | 0.9948 | 0.9975 | × | 0.9984 | 0.9974 | × |
| CSCAT (Single Incidence) vs. HSCAT-B | × | × | 0.9972 | × | × | 0.9970 |
| AMSR2 vs. OSCAT | 0.9964 | 0.9974 | × | 0.9967 | 0.9956 | × |
| AMSR2 vs. HSCAT-B | × | × | 0.9982 | × | × | 0.9951 |
| AMSR2 vs. CSCAT (Single Incidence) | 0.9994 | 0.9994 | 0.9994 | 0.9998 | 0.9996 | 0.9998 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, L.; Dong, X.; Lin, W.; Lang, S. Dual-Mode Sea Ice Extent Retrieval for the Rotating Fan Beam Scatterometer. Remote Sens. 2024, 16, 2378. https://doi.org/10.3390/rs16132378
Liu L, Dong X, Lin W, Lang S. Dual-Mode Sea Ice Extent Retrieval for the Rotating Fan Beam Scatterometer. Remote Sensing. 2024; 16(13):2378. https://doi.org/10.3390/rs16132378
Chicago/Turabian StyleLiu, Liling, Xiaolong Dong, Wenming Lin, and Shuyan Lang. 2024. "Dual-Mode Sea Ice Extent Retrieval for the Rotating Fan Beam Scatterometer" Remote Sensing 16, no. 13: 2378. https://doi.org/10.3390/rs16132378
APA StyleLiu, L., Dong, X., Lin, W., & Lang, S. (2024). Dual-Mode Sea Ice Extent Retrieval for the Rotating Fan Beam Scatterometer. Remote Sensing, 16(13), 2378. https://doi.org/10.3390/rs16132378





