Pre-Launch Assessment of PACE OCI’s Polarization Sensitivity

Abstract

To provide ongoing continuity for the ocean, cloud, and aerosol science data records, NASA will launch the Plankton, Aerosol, Cloud, ocean Ecosystem (PACE) mission on 8 February 2024. The Ocean Color Instrument (OCI) is the primary sensor onboard PACE and will provide ocean color science data to continue the data sets collected by heritage sensors MODIS, SeaWiFS, and VIIRS, but with increased spectral coverage and improved accuracy. The OCI is a grating spectrometer with hyperspectral coverage from the ultraviolet (about 315 nm) to near-infrared (about 900 nm), with additional filtered channels in the short-wave infrared (940 nm–2260 nm). A rigorous ground test program was conducted to calibrate the instrument and ensure that the calibration can be transferred to on-orbit operations in order to achieve the high levels of accuracy demanded by the science community. Some calibration parameters, such as polarization sensitivity, can only be measured during pre-launch testing. Tests were performed to measure the Mueller matrix components necessary to correct polarized scenes encountered on orbit. Measurements covered all spectral bands and a series of telescope scan angles encompassing the expected on-orbit scan range. The sensitivity (linear diattenuation) was measured above 340 nm to be below 0.6%, except at wavelengths, and was characterized as better than 0.1%. Below 340 nm, the sensitivity can be much higher, but this is not expected to affect the science data significantly. These results indicate that any polarized scenes encountered on orbit can be corrected with a high degree of confidence.

1. Introduction

The Plankton Aerosol Cloud ocean Ecosystem (PACE) satellite represents the next generation in remote sensing for ocean, cloud, and aerosol science, with a payload to include the Ocean Color Instrument (OCI) and a pair of polarimeters (SPEXone and HARP2), and is scheduled for launch in early 2024 [1,2,3]. The design of the OCI owes its heritage to earlier sensors, such as MODIS, VIIRS, and SeaWiFS [4,5], which were widely used for ocean research, but also incorporates a number of design improvements. While these earlier sensors were filtered radiometers, the OCI is a grating spectrometer with hyperspectral coverage from about 315 nm to 900 nm, with nine additional filtered channels in the short-wave infrared (SWIR) spectral region. A comprehensive ground test campaign was performed to assess the instrument’s performance against a series of design requirements (intended to ensure high-quality performance once on orbit) and to characterize the performance metrics necessary to transfer the calibration to on-orbit operations [6]. One of the key performance metrics measured was polarization sensitivity, which cannot be measured post-launch. Rayleigh scattering or Sun glint can cause scenes on orbit to be highly polarized, so it is essential to understand the sensitivity of the OCI to linearly polarized light in order to convert the top-of-atmosphere radiance to water-leaving radiance and maintain accuracy for the scientific community [7]. The measurements and results of the pre-launch characterization of this sensitivity (or linear diattenuation) for the OCI will be described in this work (preliminary results were presented in [6,8,9]). The results of the OCI engineering unit were described in [10]. Section 2 will briefly describe the OCI and the polarization tests; Section 3 will provide an overview of the analysis methodology; and Section 4 will review the analysis results, including an uncertainty analysis and comparison to design requirements, followed by conclusions in Section 5.

2. Overview

2.1. OCI Overview

The optical design of the OCI is based on heritage sensors, such as VIIRS [4] and SeaWiFS [5], with some design improvements. Light enters the aperture of a rotating telescope that spins at roughly 6 Hz in a plane tilted ±20 degrees from perpendicular to the direction of the spacecraft motion [1,2,3]. The OCI avoids highly polarized scenes by using a mechanism to tilt the telescope −20 degrees in the direction of motion in the southern hemisphere and +20 degrees in the direction of motion in the northern hemisphere. Inside the telescope, there is a primary mirror, a depolarizer, and a secondary mirror. As the telescope sweeps across the Earth on orbit, the OCI images a swath of the Earth covering ±56 degrees off nadir. There are also two calibration views: a dark view at roughly 180 degrees off nadir and a solar calibration view at about −90 degrees off nadir (which will use solar views via a diffuser to calibrate the instrument once on orbit). The light passes out of the telescope and onto a half-angle mirror (HAM), rotating at half the speed of the telescope, which de-rotates the light beam. The light then illuminates a slit, whose image on the ground is oriented such that it is also perpendicular to the direction of spacecraft motion. After a weak-field lens, the light is collimated via an off-axis parabolic mirror. The light is separated into three wavelength ranges by two dichroic beamsplitters. The first reflects wavelengths below about 600 nm through a clear aperture and onto a grating; this separates the light, which is then focused through a lenslet array onto a CCD detector (referred to as the blue CCD). Light above roughly 600 nm is transmitted by the first dichroic, and then the second beamsplitter reflects wavelengths below 900 nm through another clear aperture and onto a second grating; the light is separated and focused through a lenslet array onto a second CCD detector (referred to as the red CCD). Wavelengths above 900 nm are transmitted by the second dichroic, reflected off a collimating mirror into a microlens array and a series of 16 fibers that terminate in the SWIR detector assembly. The beam exits each fiber and is separated by a dichroic, passes through a bandpass filter, and is then incident on either an InGaAs or HgCdTe detector. To improve SNR, the 32 SWIR detectors are aggregated into nine separate SWIR bands.

Each CCD comprises a 128 × 512 array of physical detectors. The light incident on each grating is separated in the direction of the larger dimension on the CCD (referred to as the spectral direction); the smaller dimension on the CCD is aligned with the long axis of the slit (referred to as the spatial direction). The short axis of the slit image corresponds to eight physical detectors in the spectral direction. As the telescope rotates, an image on the ground enters the FOV of the slit on the leading edge of the long axis and is imaged by the CCD. The image moves along the long axis of the slit and is imaged multiple times across the spatial dimension of the CCD. These images are summed via time delay integration (TDI) to improve the SNR. Both CCDs are also capable of variable aggregation of one, two, four, or eight physical detectors in either the spatial or spectral dimensions. While the main science mode expected to be used on orbit is an 8 × 2 or 8 × 4 aggregation of physical detectors (depending on the CCD tap), the polarization data were collected in an 8 × 1 aggregation, or the maximum aggregation in the spatial direction while retaining the full spectral resolution.

In order to ensure high-quality data products for the scientific community from the OCI once on orbit, a number of design requirements were assessed during the ground test campaign. There are two requirements relevant to polarization of the OCI. One limits the maximum allowed linear diattenuation: less than 1% for the hyperspectral bands above 345 nm and less than 2% for the SWIR bands. The maximum uncertainty is defined by the second requirement: less than 0.17% from 345 nm to 400 nm and less than 0.11% from 400 nm to 900 nm for the hyperspectral bands (there is no specified uncertainty limit for the SWIR bands). The compliance of the OCI with respect to these two requirements will be assessed in Section 4.

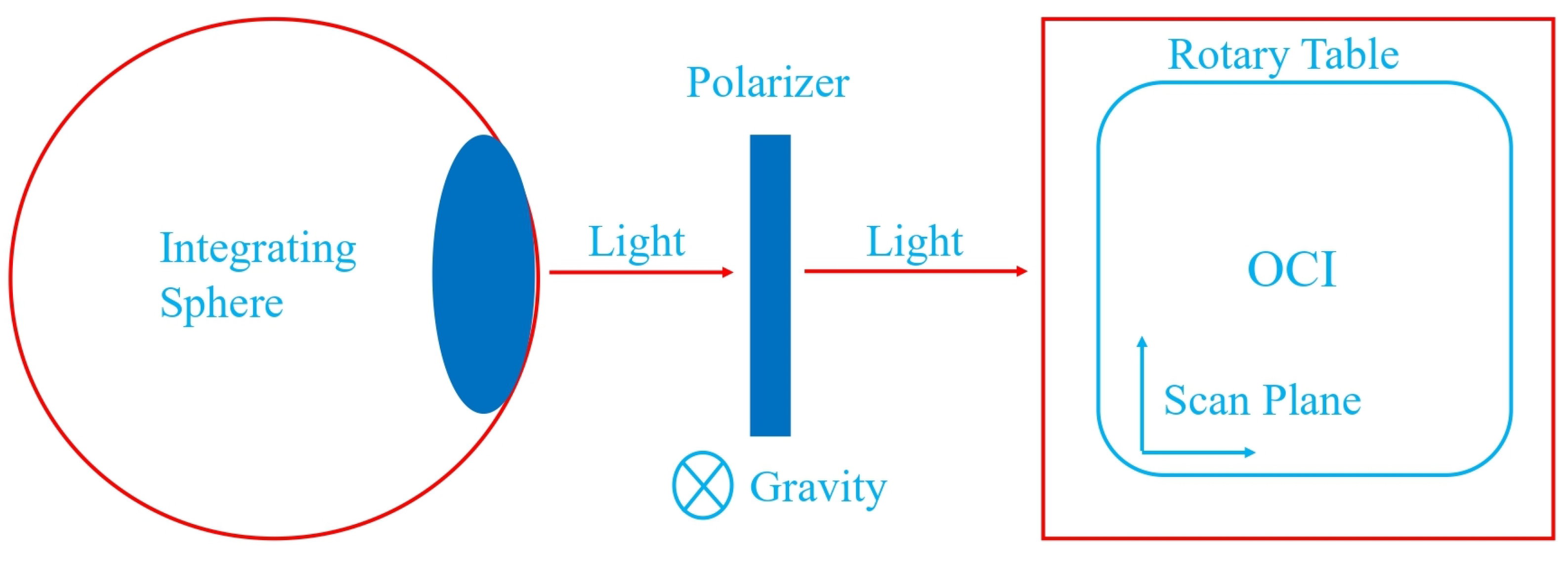
2.2. Testing Overview

Polarization testing was performed for the OCI at the instrument level under both ambient and environmental conditions. A schematic of the test setup is shown in Figure 1. A 20-inch integrating sphere with an 8-inch circular aperture was used, illuminated by a 150 W halogen lamp (covering the visible, near-infrared, and SWIR wavelength regions) and a plasma source (covering the ultraviolet and visible wavelength regions). The light beam exiting the sphere then passed through a MOXTEK™wire grid polarizer mounted in a rotary stage. The polarizer was oriented with the wire grid on the exit surface, and its transmission axis was horizontal when the rotary stage was set to 0 degrees. The polarizer was also tilted 5 degrees around the vertical to help direct any retro-reflections out of the path. Stray light curtains and baffling were installed to control unwanted stray light. During the test, the polarizer was rotated from 0 to 720 degrees in 15-degree increments.

During the ambient test, the OCI was mounted on a rotating table. The OCI was oriented such that the scan plane was perpendicular to gravity; the direction of spacecraft motion was twoards the floor. The table was rotated such that the OCI viewed the source through the polarizer at a series of scan angles (±50, ±25, and 0). The 0 degree measurement was performed first and then repeated at the end of the sequence. One additional measurement was made at −90 degrees (the solar calibration view prior to the installation of the solar calibration assembly); for this portion of the test, the OCI was re-positioned on the rotating table as the solar calibration view was obstructed in the nominal configuration. For the environmental test, the OCI was inside the vacuum chamber and could only view the source through a window at a 0 degree scan angle. The orientation of the OCI was the same as for the ambient test, but without the ability to rotate the instrument.

An additional test of the polarizer efficiency was performed during the ambient test, where a second identical polarizer was inserted in the path between the rotating polarizer and the OCI. The transmission axis of this polarizer was fixed, so it acted as an analyzer. This measured efficiency was then used as a correction for the main polarization analysis.

Before the testing began, the residual sphere polarization was also measured. The sphere was configured with the same sources used during the main polarization test. The rotating polarizer was also in the same configuration. A filtered radiometer was placed 1.35 m from the polarizer aperture. Measurements were made for a number of wavelengths from the visible to the SWIR. Because the estimated sphere polarization was small, it was simply carried as a component in the uncertainty analysis.

3. Methodology

In order to transfer the top-of-atmosphere radiance to the water-leaving radiance useful in ocean science, many scenes require corrections to remove polarization. Rayleigh scattering in the atmosphere causes many scenes to be linearly polarized, up to 50% depending on the geometry. The corrections are derived from ground measurements using the Mueller matrix formulism. Then, the corrections can be compared to the design requirements (both in magnitude and uncertainty) to determine the expected quality of the on-orbit corrections.

3.1. Mueller Matrix Components

On orbit, the Stokes vector $S^{\prime }$ of the light beam incident on the detector is related to the Stokes vector of the light beam at the telescope aperture via a Mueller matrix, or [11]

$$\left[\begin{array}{c}{S}_0^{\prime }\\ S_1^{\prime }\\ S_2^{\prime }\\ S_3^{\prime }\end{array}\right]=\left[\begin{array}{cccc}{M}_{00}& M_{01}& M_{02}& M_{03}\\ M_{10}& M_{11}& M_{12}& M_{13}\\ M_{20}& M_{21}& M_{22}& M_{23}\\ M_{30}& M_{31}& M_{32}& M_{33}\end{array}\right]\left[\begin{array}{c}{S}_0\\ S_1\\ S_2\\ S_3\end{array}\right]$$

(1)

Since the OCI cannot distinguish a polarization incident on the detector, only $S_0^{\prime }$ can be measured. In addition, the expected scene polarization encountered on orbit is linearly polarized, so $S_3$ is set to zero. The Mueller matrix equation is then reduced to

$$S_0^{\prime }=M_{00}{S}_0+M_{01}{S}_1+M_{02}{S}_2$$

(2)

The components of S are defined as the product of the Mueller matrix for an ideal rotating linear polarizer and an unpolarized light beam. $S_0^{\prime }$ is the OCI measurement (here, the offset corrected digital counts [dn] as a function of polarization angle [$\theta$]). Thus, the equation can be rewritten as

$$\begin{array}{ccc}\hfill dn\left(\theta \right)& =& \frac{1}{2}{M}_{00}+\frac{1}{2}{M}_{01}cos\left(2\theta \right)+\frac{1}{2}{M}_{02}sin\left(2\theta \right)\hfill \end{array}$$

(3)

$$\begin{array}{ccc}& =& \frac{1}{2}{M}_{00}\left[1+m_{01}cos\left(2\theta \right)+m_{02}sin\left(2\theta \right)\right]\hfill \end{array}$$

(4)

where $\frac{1}{2}{M}_{00}$ is the average signal. This formulation is just the zeroth and second-order terms of a Fourier series; these are the terms required for the on-orbit correction.

During the ground measurements, additional sources of polarization may be present (such as a transmission gradient in the polarizer and straylight) that do not appear on orbit; as a result, a fourth-order Fourier series was used to analyze the polarization data sets to account for other effects (which will be described in Section 4), as was the case for heritage sensors [12]

$$dn\left(\theta \right)=\frac{1}{2}{c}_0+\sum _{n=1}^4{c}_{n}sin\left(n\theta \right)+d_{n}cos\left(n\theta \right)$$

(5)

where the coefficients are given by

$$\frac{1}{2}{c}_0=\frac{1}{\pi }{\int }_0^{2\pi }dn\left(\theta \right)d\theta$$

(6)

$$C_n=\frac{2c_n}{c_0}=\frac{2}{\pi c_0}{\int }_0^{2\pi }dn\left(\theta \right)cos\left(n\theta \right)d\theta$$

(7)

$$D_n=\frac{2d_n}{c_0}=\frac{2}{\pi c_0}{\int }_0^{2\pi }dn\left(\theta \right)sin\left(n\theta \right)d\theta$$

(8)

Here, the coefficients $C_2$ and $D_2$ are the Mueller matrix components $m_{01}$ and $m_{02}$ [7]. Equation (5) can be rewritten in terms of the amplitude and phase angle of the electric field vector, or

$$dn\left(\theta \right)=\frac{1}{2}{c}_0\left[1+\sum _{n=1}^4{a}_{n}cos\left(n\theta +2{\delta }_n\right)\right]$$

(9)

where the amplitude of the polarization is given by

$$a_n=\frac{\sqrt{C_n^2+D_n^2}}{\sqrt{a_2^{eff}}}$$

(10)

Here, $a_2^{eff}$ is the polarizer efficiency determined from Equation (10) without the denominator using the data from the polarizer efficiency testing. Note that $a_2$ is the linear diattenuation (LD). The phase angle is determined from

$${\delta }_n=\frac{1}{2}arctan\left[\frac{D_n}{C_n}\right]$$

(11)

This methodology was used to analyze the measured data from the sphere polarization, polarizer efficiency, and polarization sensitivity tests.

3.2. Uncertainty

The polarization uncertainty was estimated in this work following the methodology established in [13,14]. Individual uncertainty contributors are propagated through the equations in the previous section to generate the total uncertainty for the polarization correction on orbit. Uncertainty contributions from noise, instrument drift, and stray light were propagated into a combined OCI measurement uncertainty for each polarizer/scan angle, or

$$u^2\left(dn\right)=u^2\left(noise\right)+u^2\left(drift\right)+u^2\left(straylight\right)$$

(12)

This measurement uncertainty is then propagated through the Fourier analysis along with an uncertainty on the polarizer angle to generate the uncertainty on the Fourier coefficients. The Fourier coefficient uncertainty is combined with uncertainties associated with measurement repeatability and scan angle interpolation, or

$$u^2\left(c_2^{final}\right)=u^2\left(c_2\right)+u^2\left(repeatability\right)+u^2\left(inter polation\right)$$

(13)

$$u^2\left(d_2^{final}\right)=u^2\left(d_2\right)+u^2\left(repeatability\right)+u^2\left(inter polation\right)$$

(14)

These uncertainties are then propagated to the linear diattenuation and phase, while including an uncertainty contribution from the polarizer efficiency and sphere polarization:

$$\begin{array}{ccc}\hfill u^2\left(a_2\right)& =& \frac{1}{c_0^2{a}_2^{eff}}\frac{1}{\left(c_2^2+d_2^2\right)}\left[c_2^2{u}^2\left(c_2\right)+d_2^2{u}^2\left(d_2\right)\right]\hfill \\ \hfill & & +a_2^2\left[\frac{u^2\left(c_0\right)}{c_0^2}+\frac{u^2\left(a_2^{eff}\right)}{4{\left(a_2^{eff}\right)}^2}\right]+u^2\left(a_2^{sphere}\right)\hfill \end{array}$$

(15)

$$u^2\left({\delta }_2\right)=\frac{1}{4c_2^2}{\left[\frac{c_2^2}{c_2^2+d_2^2}\right]}^2\left[\frac{d_2^2}{c_2^2}{u}^2\left(c_2\right)+u^2\left(d_2\right)\right]$$

(16)

The efficiency uncertainty was determined in the same manner as above, except that it used the cross-polarizer data sets. Finally, the uncertainty on the linear diattenuation and phase were combined into a total uncertainty, or

$$u^2={cos}^2\left(2\theta +2{\delta }_2\right)u^2\left(a_2\right)+4a_2^2{sin}^2\left(2\theta +2{\delta }_2\right)u^2\left({\delta }_2\right)$$

(17)

The measurement noise for a particular polarizer angle/scan angle combination is estimated by the standard deviation of the response over the 35 s data collection. The stray light uncertainty was determined using the maximum over a 0–720 degree cycle from a section of the scan line away from the source (which is expected to be dark). The drift uncertainty was estimated by comparing the repeated measurements at polarizer angles in a given 0–720 degree cycle (i.e., 0, 360, and 720 degrees) and taking the maximum change. The polarizer angle uncertainty estimated how well the alignment of the transmission axis was known (0.2 degrees). Two measurements were made at a 0 degree scan angle; these were used to estimate the repeatability uncertainty. The scan angle interpolation uncertainty was estimated as the maximum residual from a quadratic fit to all scan angle measurements. The sphere polarization uncertainty was measured using an external radiometer.

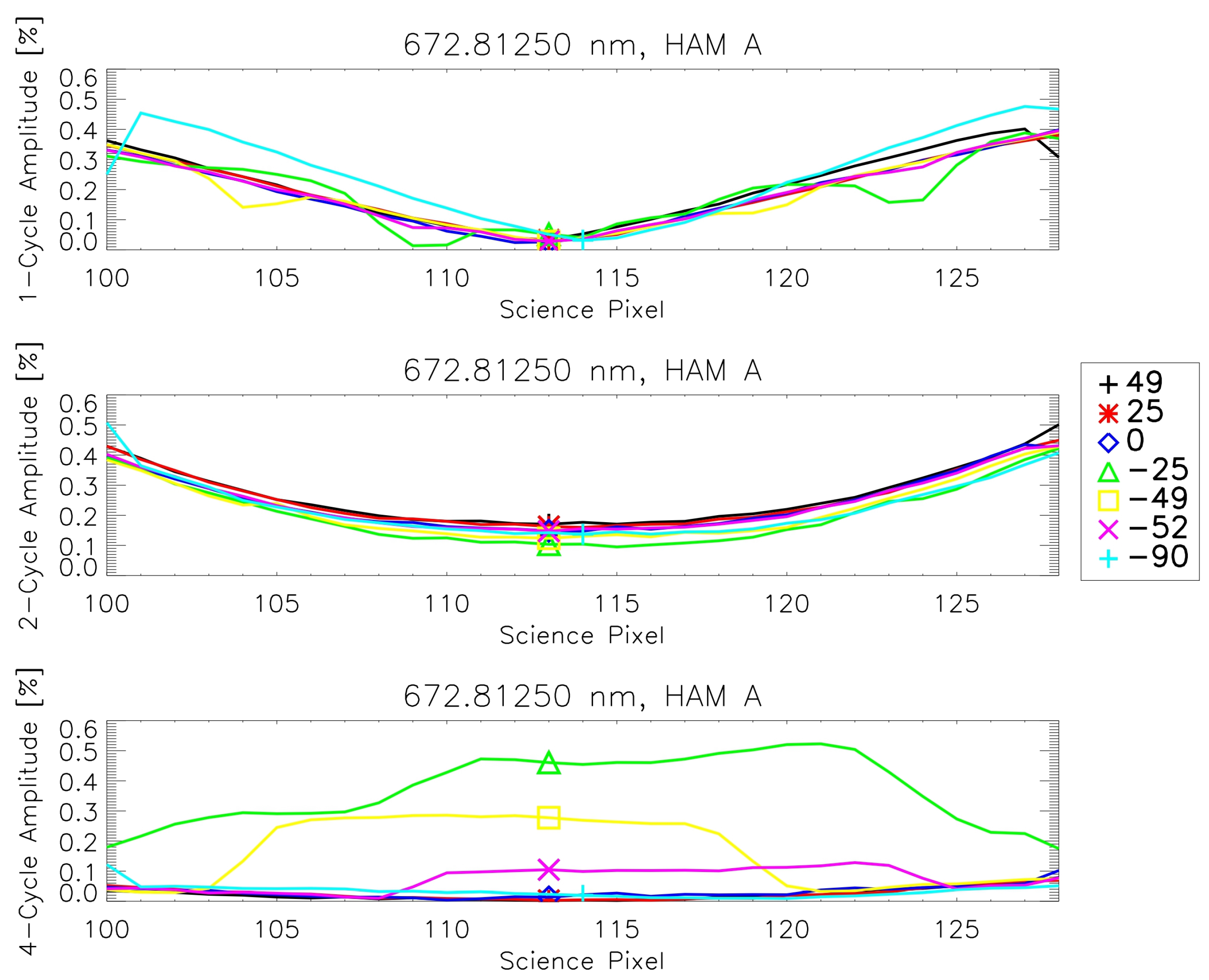
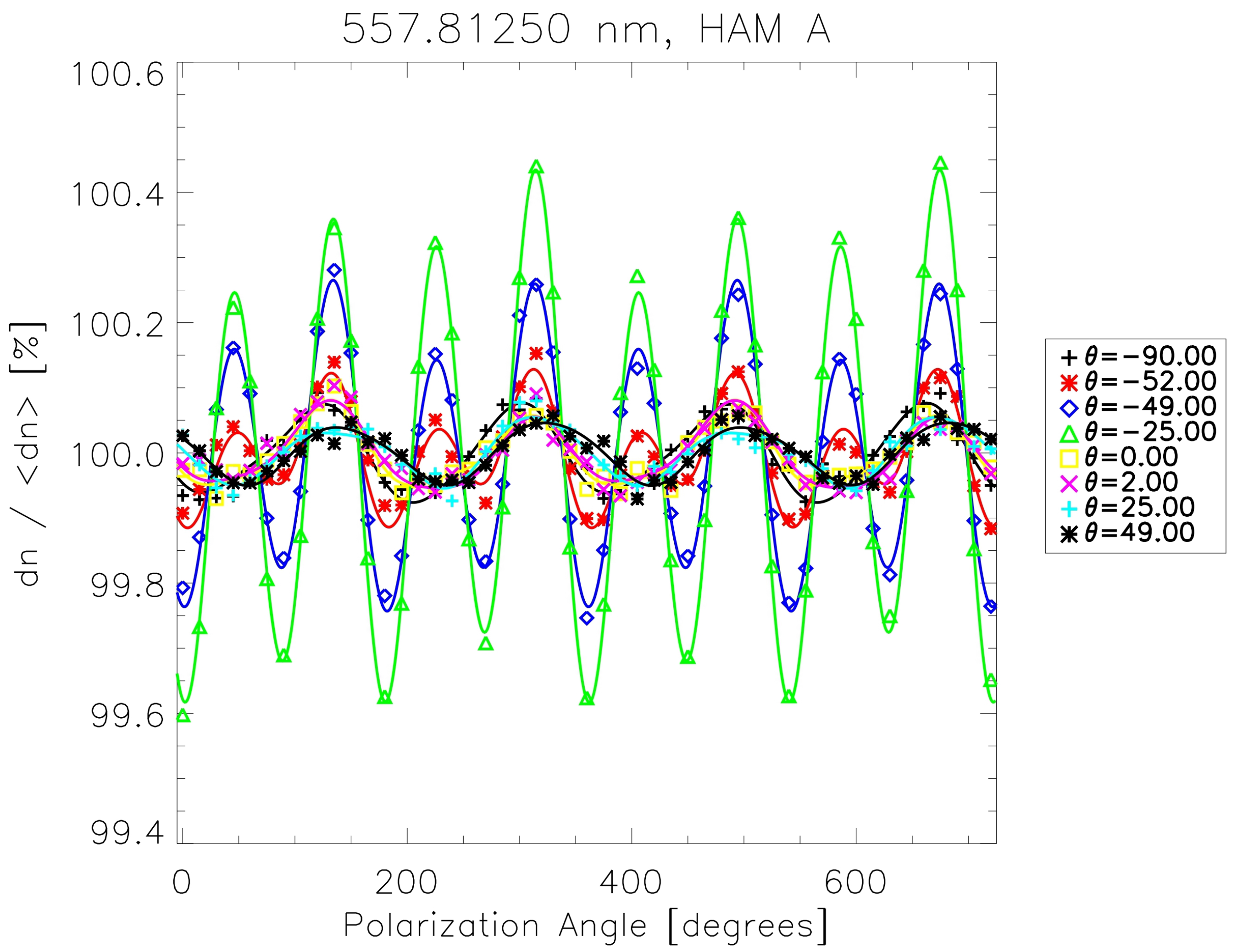
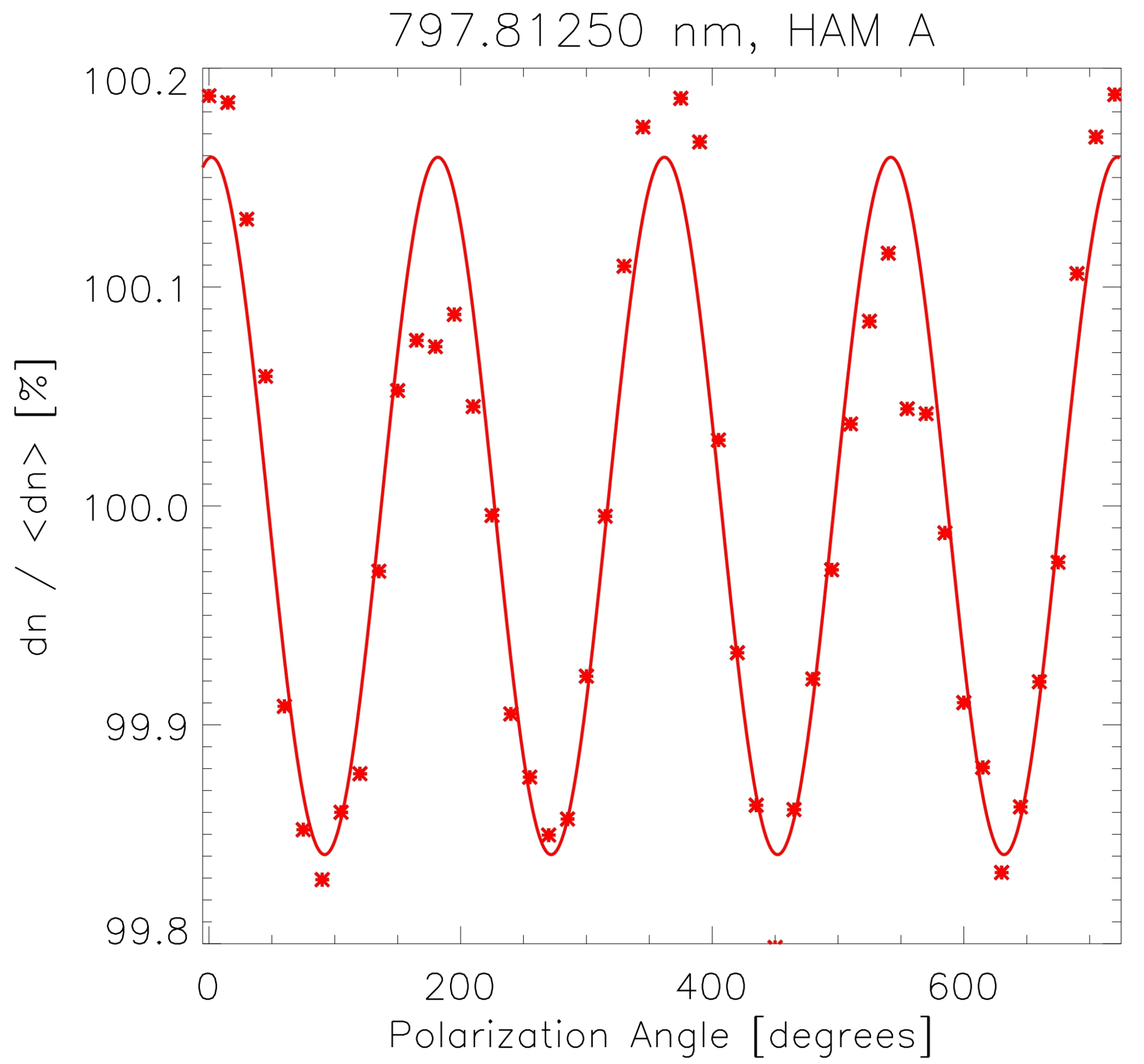
4. Results

4.1. Data Reduction and Quality

The OCI recorded data as the telescope scanned past the source as seen through the polarizer. This is visualized for a specific band (557.8125 nm) in the upper plot of Figure 2. The OCI footprint is outside the source aperture on the wings, partially views the aperture on the slopes, and fully views within the source aperture on the plateau of the response curve (the offset corrected digital counts). The offset derived from a dark view for each scan is subtracted from every pixel in the OCI Earth view for the same scan. Averaging is then performed over all scans for data collection (about 35 s of data), and outlier rejection is performed before averaging. The lower plot of Figure 2 shows the response variation within the plateau for the different polarizer angles listed in the legend. Note that the variation is greater towards the edges of the plateau and smallest in the middle. As a result, a Fourier analysis was performed on a per-pixel basis. The cause of this variation is a linear transmission gradient on the polarizer, which will become more evident in the analysis of Section 4.4.

While the source was actively monitored, the radiance correction (relative to the start of every 0–720 degree polarizer cycle) in some cases added a small amount of noise to the data sets. As a result, a linear drift correction was used to detrend the data, using the polarizer measurements at 0, 360, and 720 degrees during each 0–720 degree polarizer cycle. This improved repeatability from about 0.2–0.3% to 0.1% or less.

4.2. Sphere Polarization

The sphere polarization was measured by an external radiometer, as described in Section 2.2. While this radiometer measured the polarization at 11 wavelengths, the data quality was low for some of these. For wavelengths below 460 nm, the measured amplitude using the radiometer response was below the noise level. For higher wavelengths (above 1000 nm), the radiometer response appeared to be polarization-sensitive, with amplitudes higher than any expected sphere polarization. The remaining wavelengths (640 nm and 840 nm) were then used to estimate one sphere polarization value, which was used for all OCI bands. The average across these wavelengths, as well as configuration changes, was 0.09%. Because the expected maximum on-orbit scene polarization is 50%, this value was reduced by half and carried as part of the uncertainty analysis (highly polarized scenes are avoided due to the telescope tilt mechanism).

4.3. Polarizer Efficiency

The efficiency of the polarizer was measured using a second polarizer with a fixed transmission axis, as described in Section 2.2. The test data were then analyzed using the Fourier analysis outlined in Section 3. The resulting polarizer efficiency is plotted for all bands in Figure 3. The upper, middle, and lower plots correspond to the blue CCD, red CCD, and SWIR bands, respectively. Note that, as this test was performed under ambient conditions, the SWIR bands using HgCdTe detectors (1615 nm, 2130 nm, and 2260 nm) were not cooled, and so no meaningful data were collected. Results using the different HAM sides are plotted in blue and green lines, but are nearly identical. The efficiency is greater than 99.5% for all bands above 430 nm. Below 430 nm, the efficiency starts to decline to around 95% at 330 nm; measurements below this wavelength had very low SNR and so were not used in the analysis. The values shown in Figure 3 were used in subsequent analyses via Equation (10).

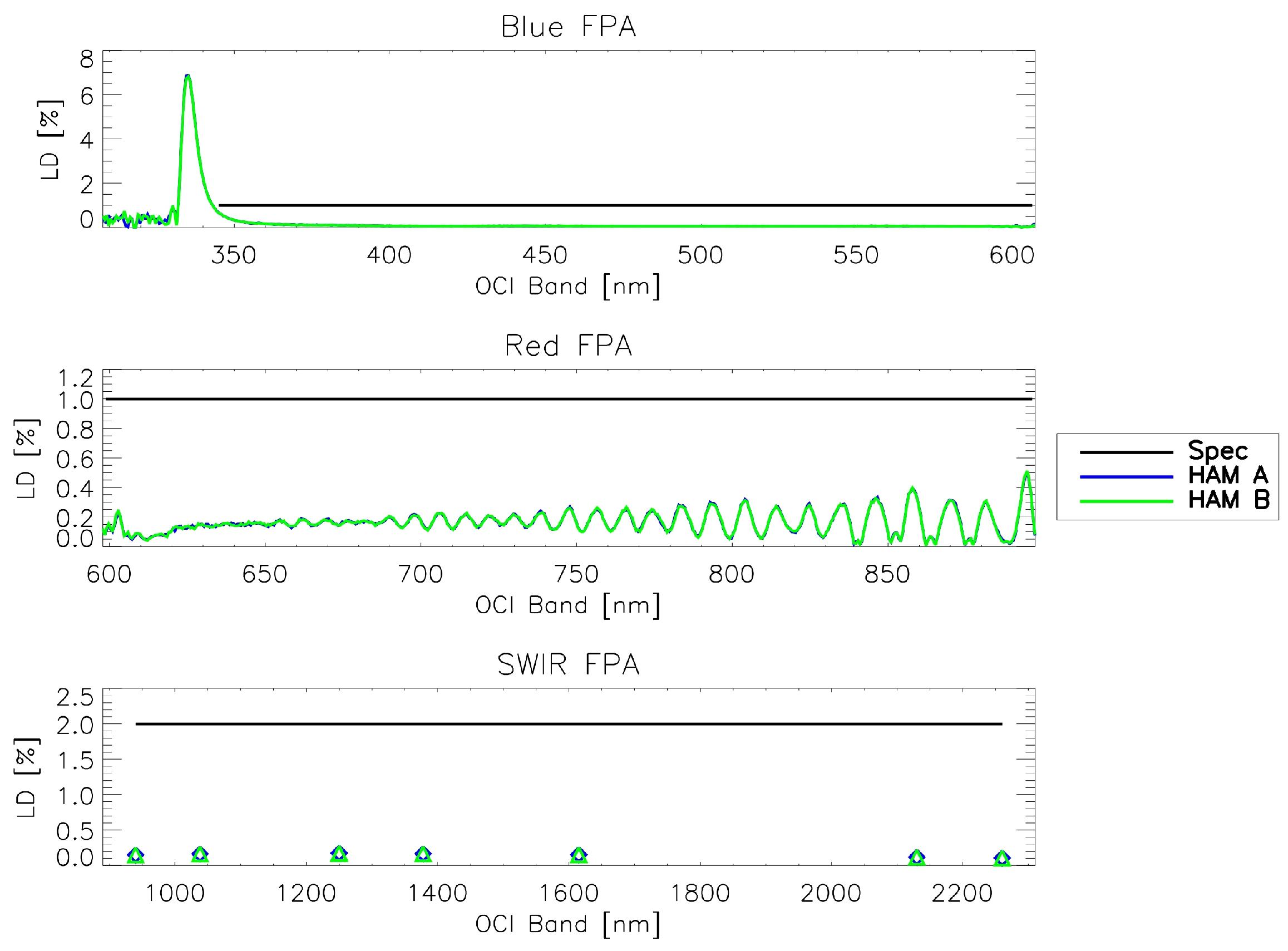
4.4. Linear Diattenuation

The Fourier analysis described in Section 3 was applied to the response as a function of the polarizer angle (the data sets from each scan angle are treated separately). This analysis was performed for every pixel location along the flat top of the response curve shown in Figure 2. The extracted amplitudes for the one-cycle, two-cycle, and four-cycle Fourier components are plotted as a function of pixels in Figure 4 (upper, middle, and lower plots, respectively). The different colors indicate the different scan angle measurements. The one-cycle amplitude shows a V pattern at the center of the response curve plateau (or the middle of the polarizer aperture). This behavior is caused by a linear transmission gradient in the polarizer [10]. To avoid this behavior, the pixel with the lowest one-cycle amplitude was selected to generate all subsequent results; in Figure 4, this is represented by the symbol. The four-cycle amplitude is small for most cases; however, three scan angle measurements did exhibit large four-cycle oscillations (−25, −49, and −52 degrees). This is likely caused by an uncontrolled path in which light intersects with the polarizer twice; as such, it is considered a feature of the test setup and not characteristic of the OCI. The two-cycle amplitudes shown in the middle plot show a gradual variation with the pixel at the center of the plateau.

The normalized instrument response variation as a function of polarization angle is shown in Figure 5 for the pixel location selected in Figure 4. Here, the symbols represent the measured data, and the solid lines signify the fourth-order Fourier series. The legend defines which color/symbol corresponds to which scan angle measurement. The measurements at −25, −49, and −52 degree scan angles have larger amplitudes, which are the result of fourth-order Fourier components. The other scan angle measurements are generally dominated by two-cycle oscillations, as expected from Malus’s law, as shown by the example plotted in Figure 6 (at a 49-degree scan angle), where the solid line now signifies the second-order Fourier series. Note that the two-cycle and four-cycle in a Fourier analysis are orthogonal, so the four-cycle oscillations, while unsightly, do not generally compromise the test results.

The linear diattenuation (or the two-cycle Fourier amplitudes) is plotted for all bands in Figure 7, as measured during environmental testing. The upper, middle, and lower plots show the results from the blue CCD, the red CCD, and the SWIR bands, respectively. The results were calculated separately for each side of the HAM as the different mirror sides can transmit polarized light differently (see heritage sensors such as JPSS-1 VIIRS [15]); in the OCI case, the two HAM side results are very similar. The solid black lines denote the sensor design requirement. Note that all bands are well below this requirement (1% above 345 nm for the hyperspectral bands and 2% for the SWIR bands). There are two features of note: the spike around 335 nm and the oscillations in the near-infrared. Between 330 nm and 340 nm, the linear diattenuation rises to almost 7% (this feature is not currently understood and is under investigation). The oscillations in wavelength observed in the near-infrared spectral region are caused by the double-wedge depolarizer, where the optical path is a function of thickness and refractive indices [16]. Some wavelengths observe a predominately full waveplate behavior (no change in polarization), others observe a predominately half-waveplate behavior (90 degree rotation), and most observe something in between. As a result, the net polarization at some wavelengths weights towards transmission while other wavelengths weight towards rejection. This manifests as a sinusoidal oscillation versus wavelength. The dependence of the diattenuation on the scan angle is shown in Figure 8, where the different colors represent different scan angle measurements. The behavior is very similar accross the different measurements. The −25 degree measurements were systematically low (this was the measurement with the largest four-cycle contamination). Attempts to fit a quadratic function versus scan angle (used to interpolate any scan angle for the on-orbit correction) determined that this measurement was out of family. As a result, the data were excluded from the final analysis. The oscillations in the near-infrared are present for all measurements, although there are slight shifts with wavelength. In addition, the 1378 nm measurements exhibited some oscillatory behavior due to water vapor contamination in the path. This reduced the quality of these results even after a correction was made. The decision was made to interpolate between the 1250 nm and 1615 nm environmental testing results to estimate the diattenuation at 1378 nm. This was more in line with expectations, although the scan angle dependence was lost.

Although the linear diattenuation was highlighted in the discussion, the related Mueller matrix components were also determined. The measurements were only made at discrete scan angles, whereas the on-orbit correction will need to be at any angle within the scan view. A quadratic fit to each of the Mueller matrix components was made, and model values every 5 degrees within the expected scan range on orbit (±56 degrees) were provided along with additional data at the solar calibration angle.

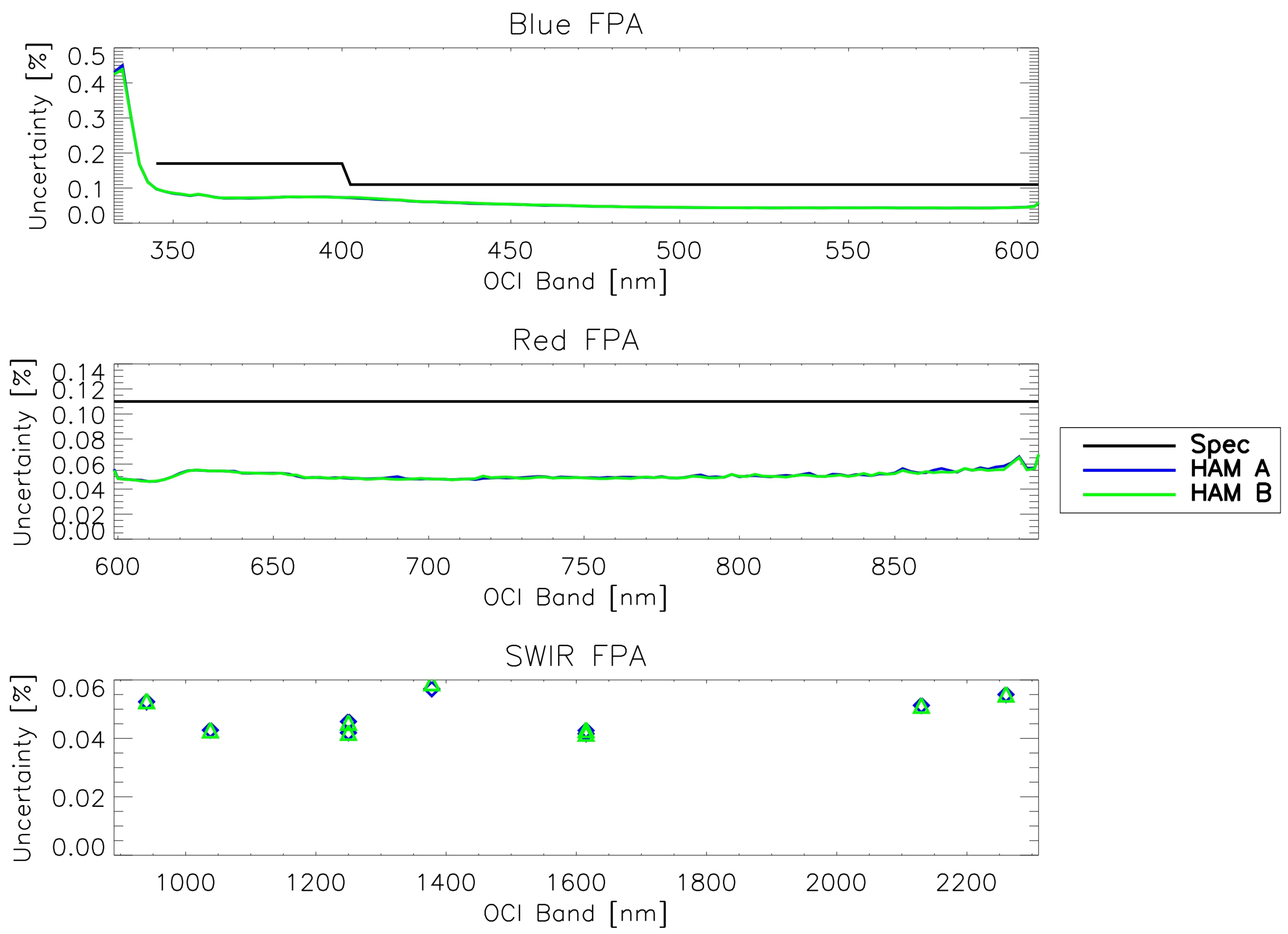
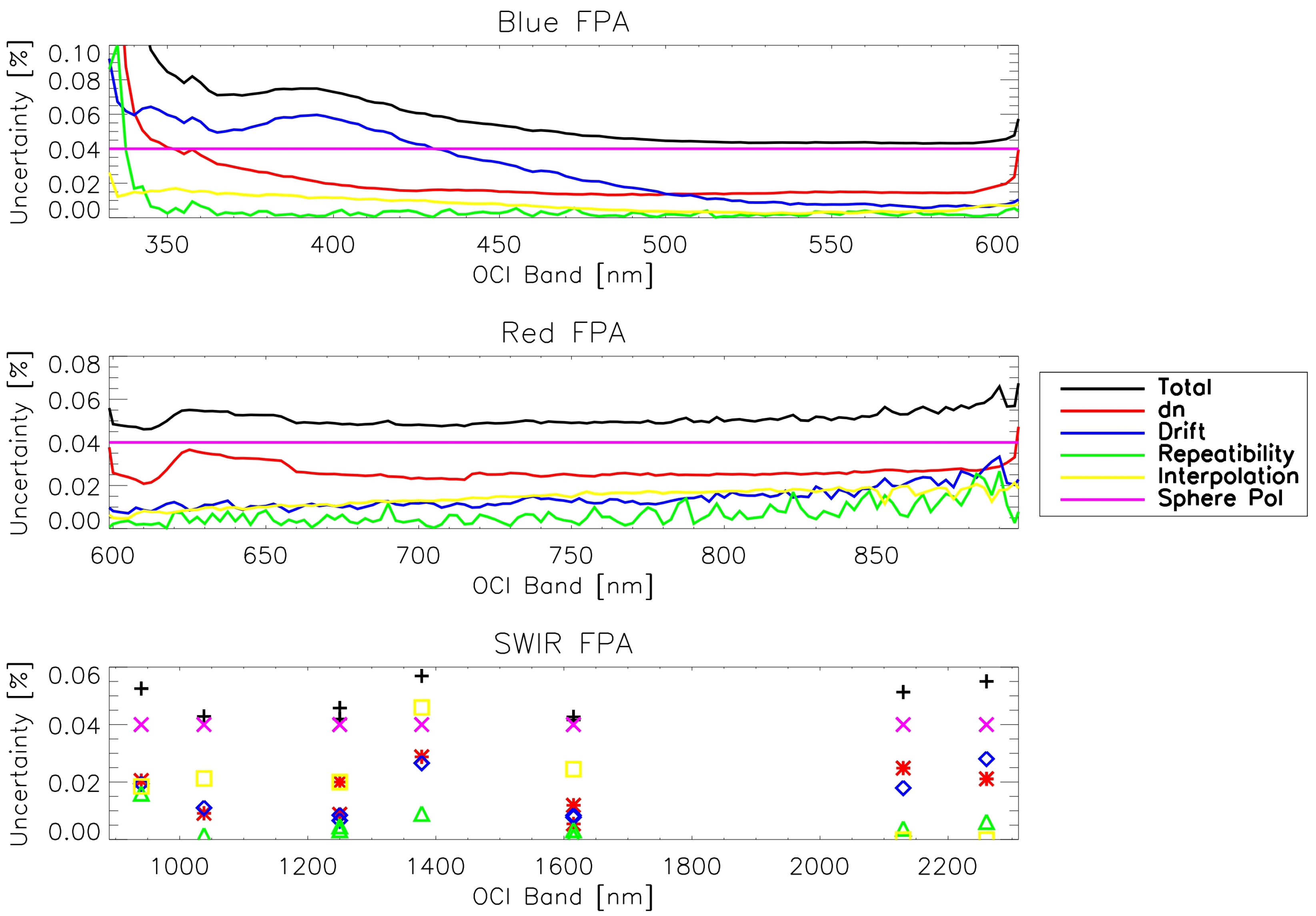
4.5. Uncertainty

The final uncertainty estimate is shown in Figure 9 for all bands. Again, the upper, middle, and lower plots show the results from the blue CCD, the red CCD, and the SWIR bands, respectively. The results for both HAM sides are shown, although they are very similar. The solid black line represents the design requirements; all bands were well within the uncertainty requirement. The total uncertainties were around 0.05% above 450 nm. Below this, the uncertainty gradually increased to about 0.08% and then sharply increased below 350 nm. The uncertainty estimates are also consistent over scan angle measurements. Figure 10 shows the largest contributors to the overall uncertainty as a function of wavelength (separated by the focal plane). The largest contributor was sphere polarization, which was included as 0.04% for all bands. Other contributors that were significant were the noise on the response, drift in the response, and scan angle interpolation, which were generally between 0.01% and 0.03%. These uncertainty estimates give confidence that the corrections supplied for use on orbit were well characterized.

5. Conclusions

The sensitivity of the PACE OCI to linearly polarized light has been characterized prior to its launch at a NASA Goddard Space Flight Center facility in 2022. The results indicate that the linear diattenuation is below 0.6% for all spectral bands above 345 nm and usually between 0.1 and 0.2%. The uncertainties on the linear diattenuation were below 0.08% above about 345 nm, and generally closer to 0.05%. Design requirements for both linear diattenuation and uncertainty were met in all cases. The Mueller matrix components necessary to perform the on-orbit correction were produced and delivered. The quality of the measurements and the results indicate that the instrument was well characterized and that these corrections will help to ensure that high-quality science data products will be produced from the PACE OCI.