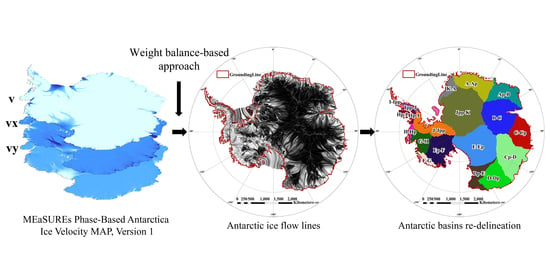
Antarctic-Scale Ice Flow Lines Map Generation and Basin Delineation
Abstract
1. Introduction
- (1)
- This paper proposes a weight balance-based method that utilizes an ice flow velocity map for the extraction of the most complete, high-density ice flow lines currently available that track the trajectory of ice flow from the origins on the ice sheet into the ice shelves. We innovatively define angular, distance, and velocity differences as impact factors to determine the pointing pixel (point or subpixel of the ice flow line). position, which considers the geometric and physical properties of ice flow lines.
- (2)
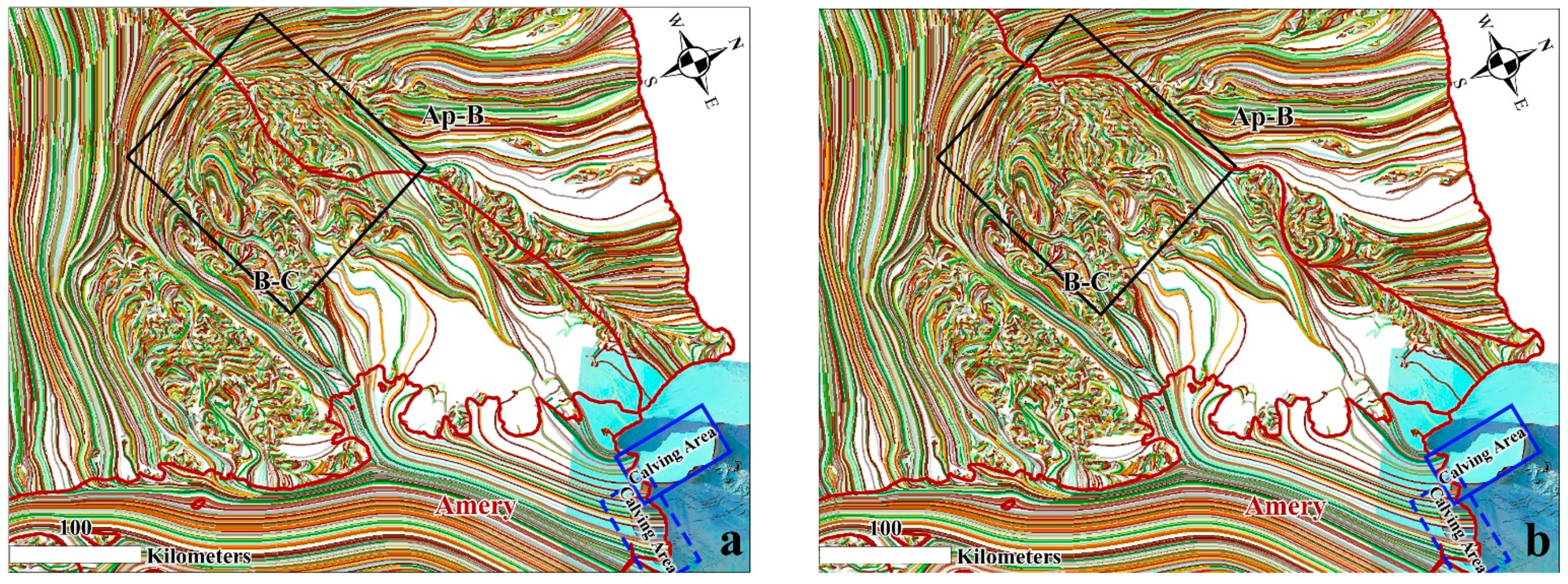
- Considering the importance of Antarctic basin systems, the differential flow directions occurring at adjacent basin boundaries from ice flow lines are used as the crucial criterion to achieve the fine delineation of Antarctic basin systems and the collapse event of Amery ice shelf occurred in 2019 as the case study for verification.
2. Materials and Methods
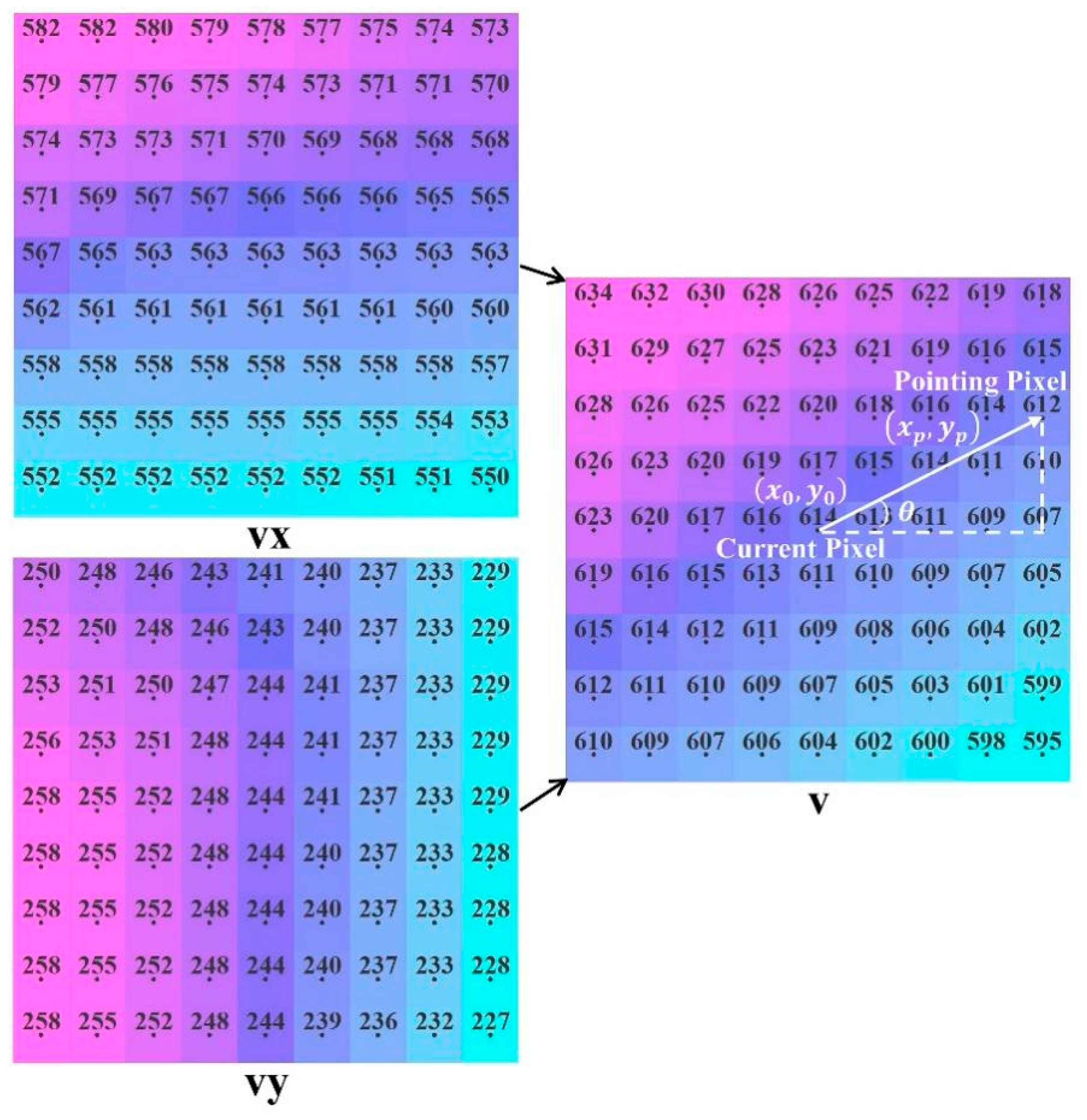
2.1. Three Features That Determine the Position of a Pointing Pixel
2.2. Direction Accuracy of Pointing Pixel
2.2.1. Smoothness of the Ice Flow Lines
2.2.2. Velocity Difference of Current Pixel and Pointing Pixel
2.3. Weight-Balance Method
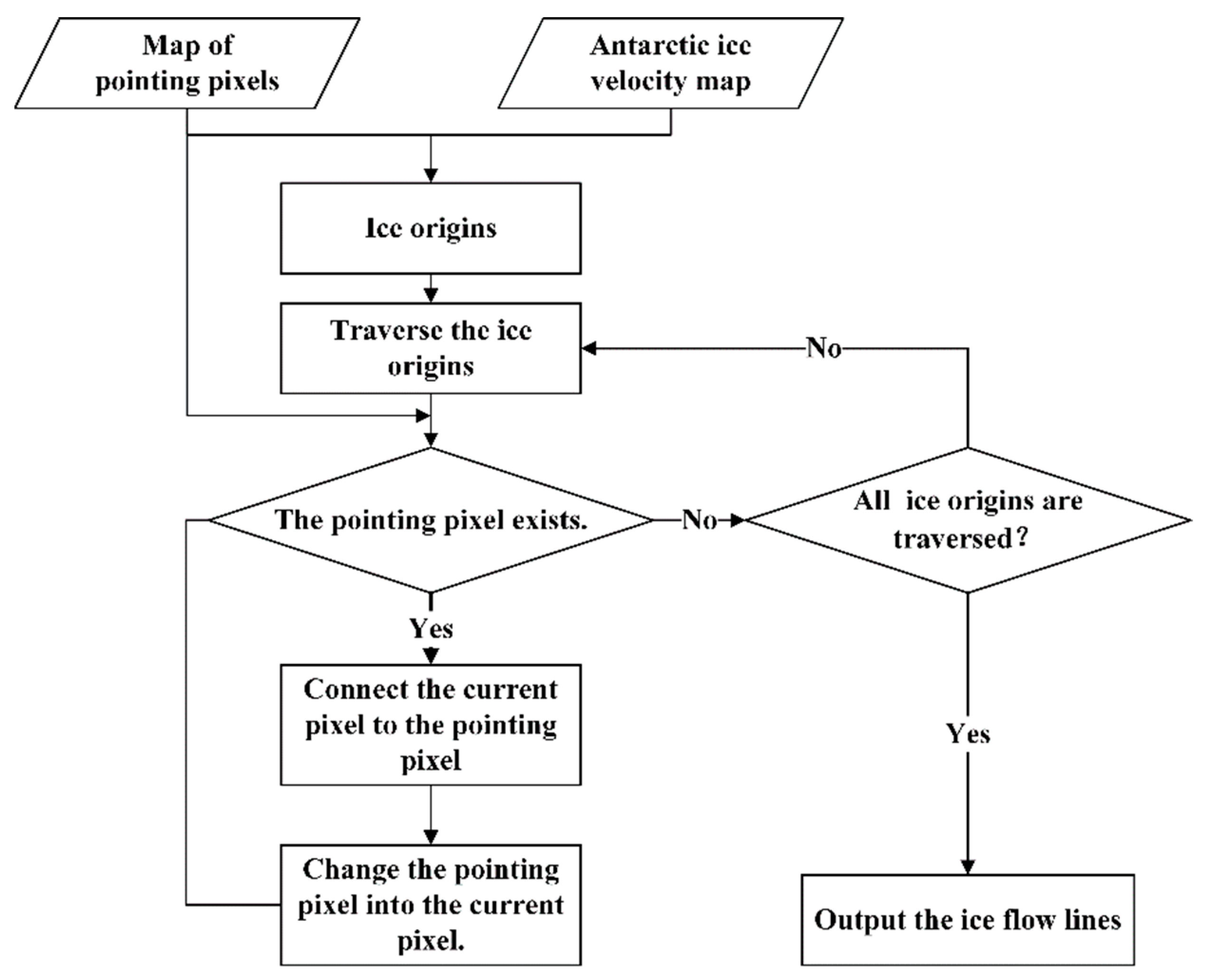
2.4. Determine the Ice Origins
- (1)
- The ice flow velocity at the ice origin velocity is close to 0 m/yr.
- (2)
- The ice origins are not pointed to by any of the pixels.
2.5. Antarctic Ice Flow Lines Map Generation
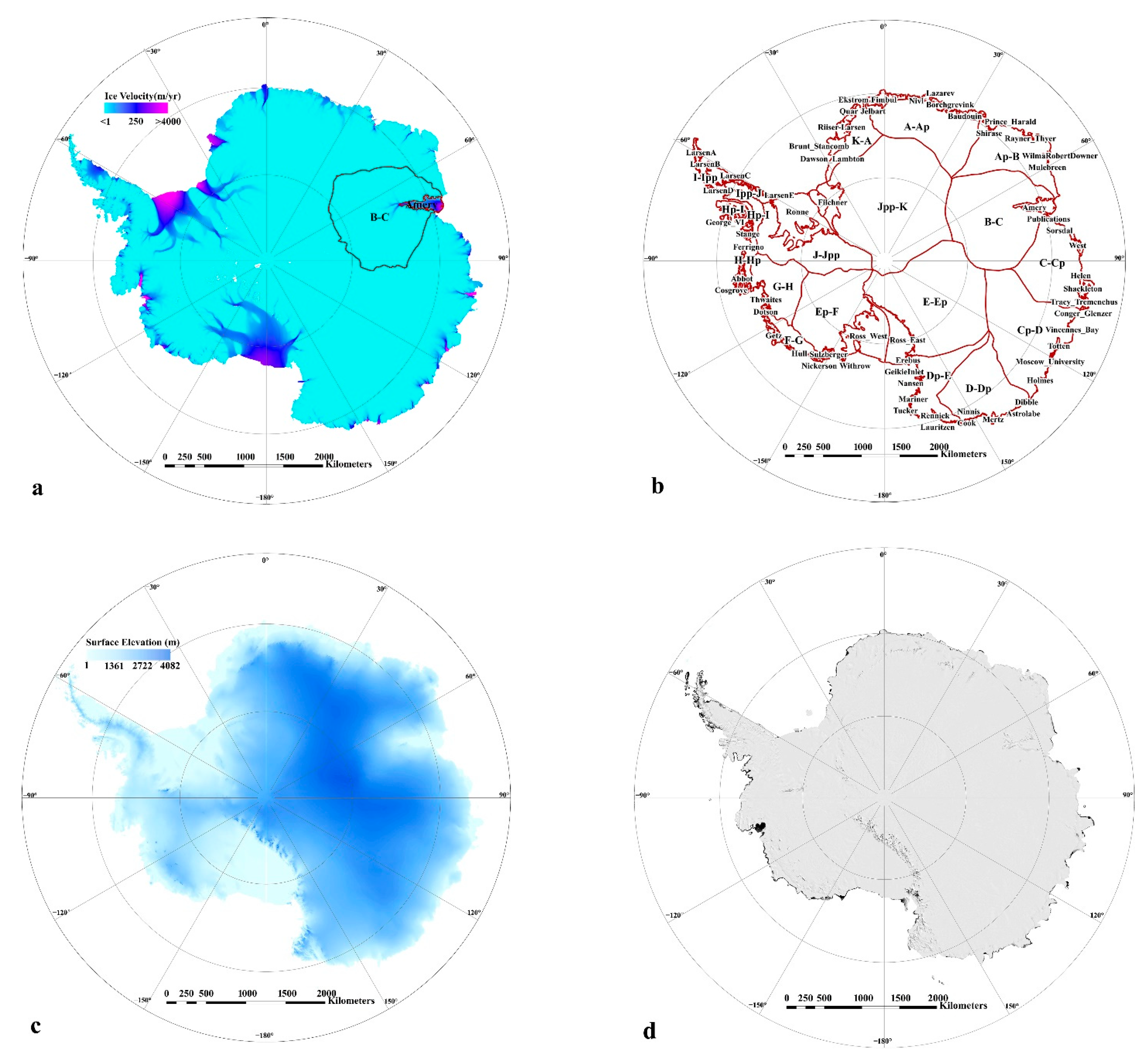
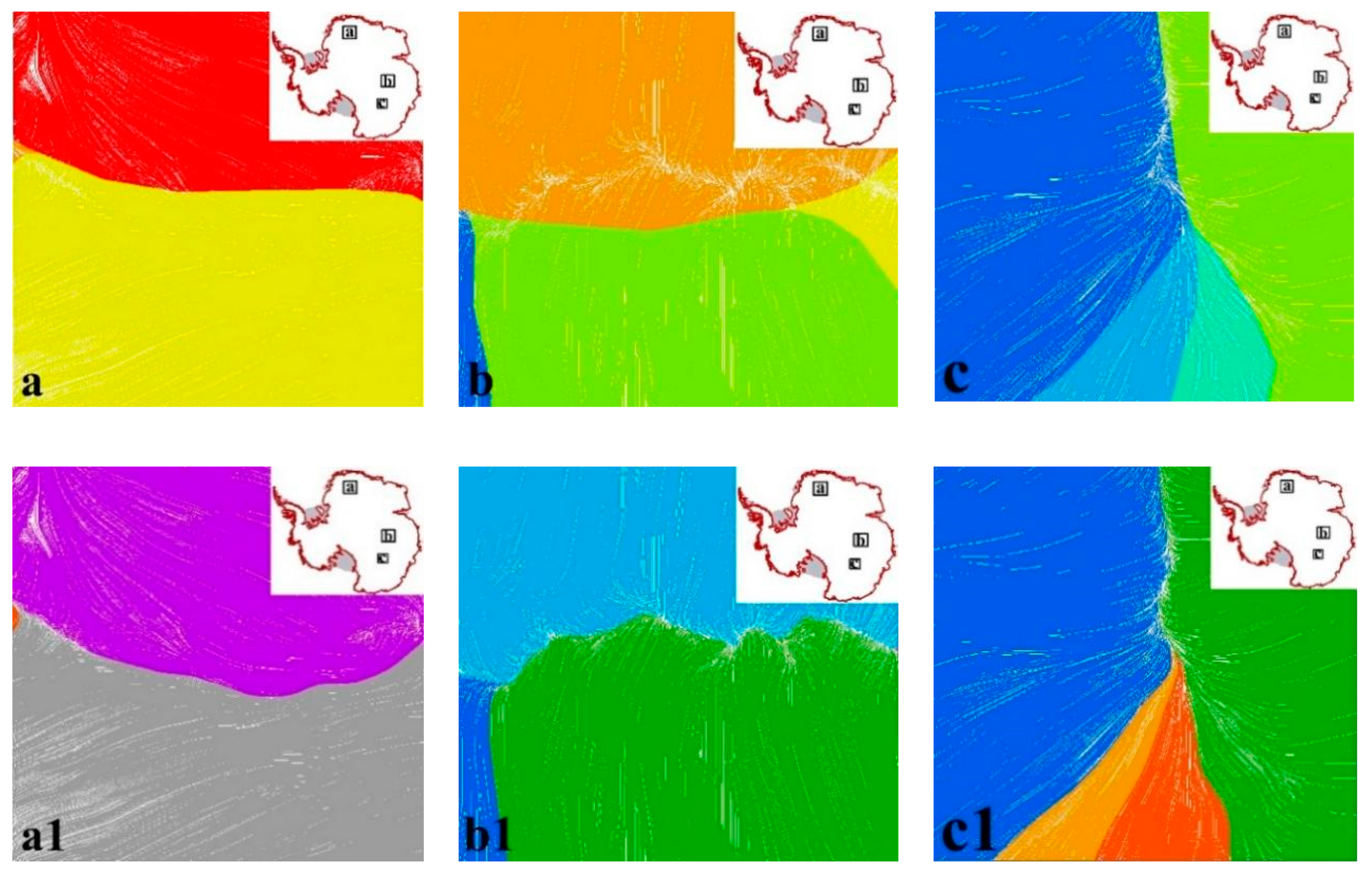
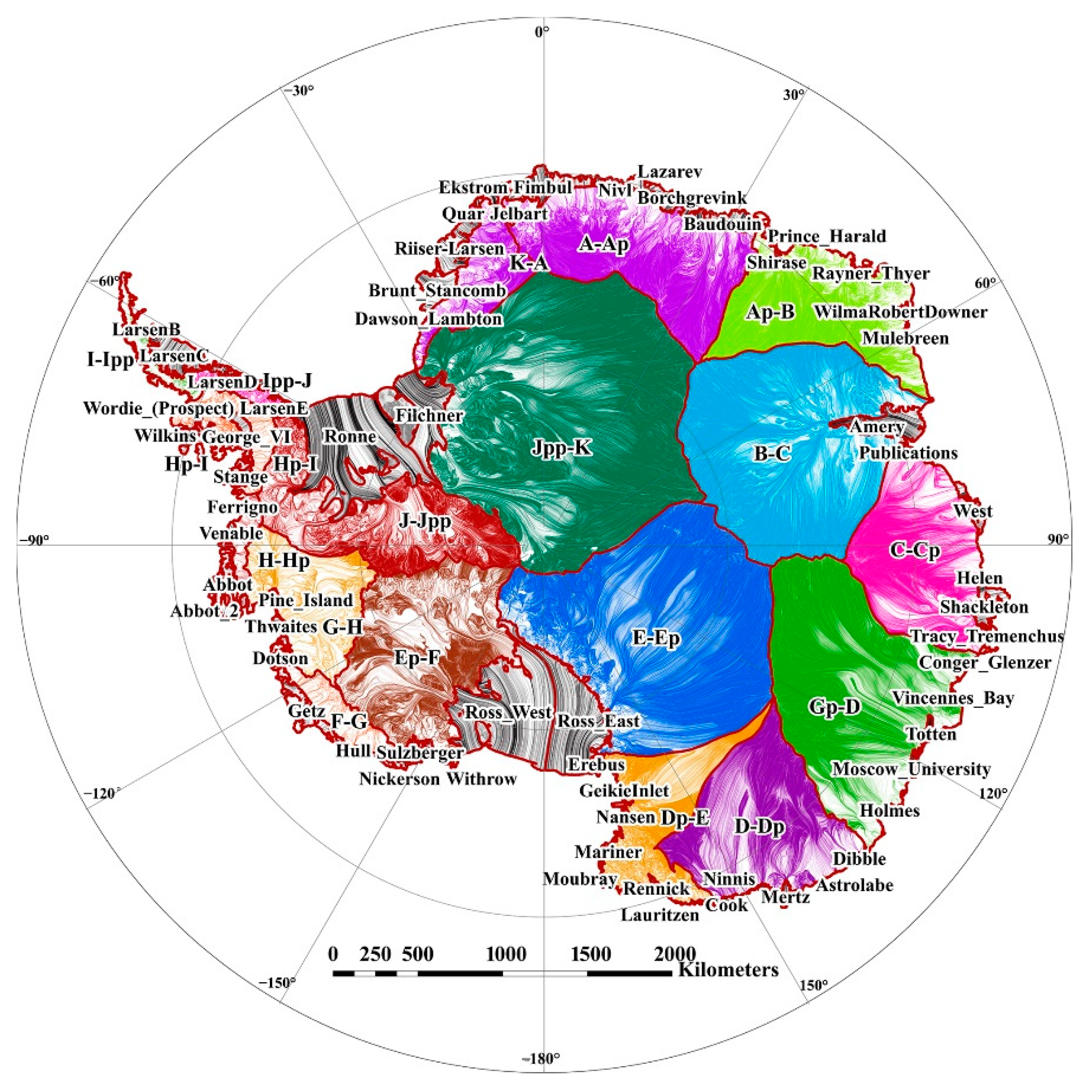
2.6. Antarctic Basins Delineation Based on Ice Flow Lines Map
3. Experimentation and Analysis
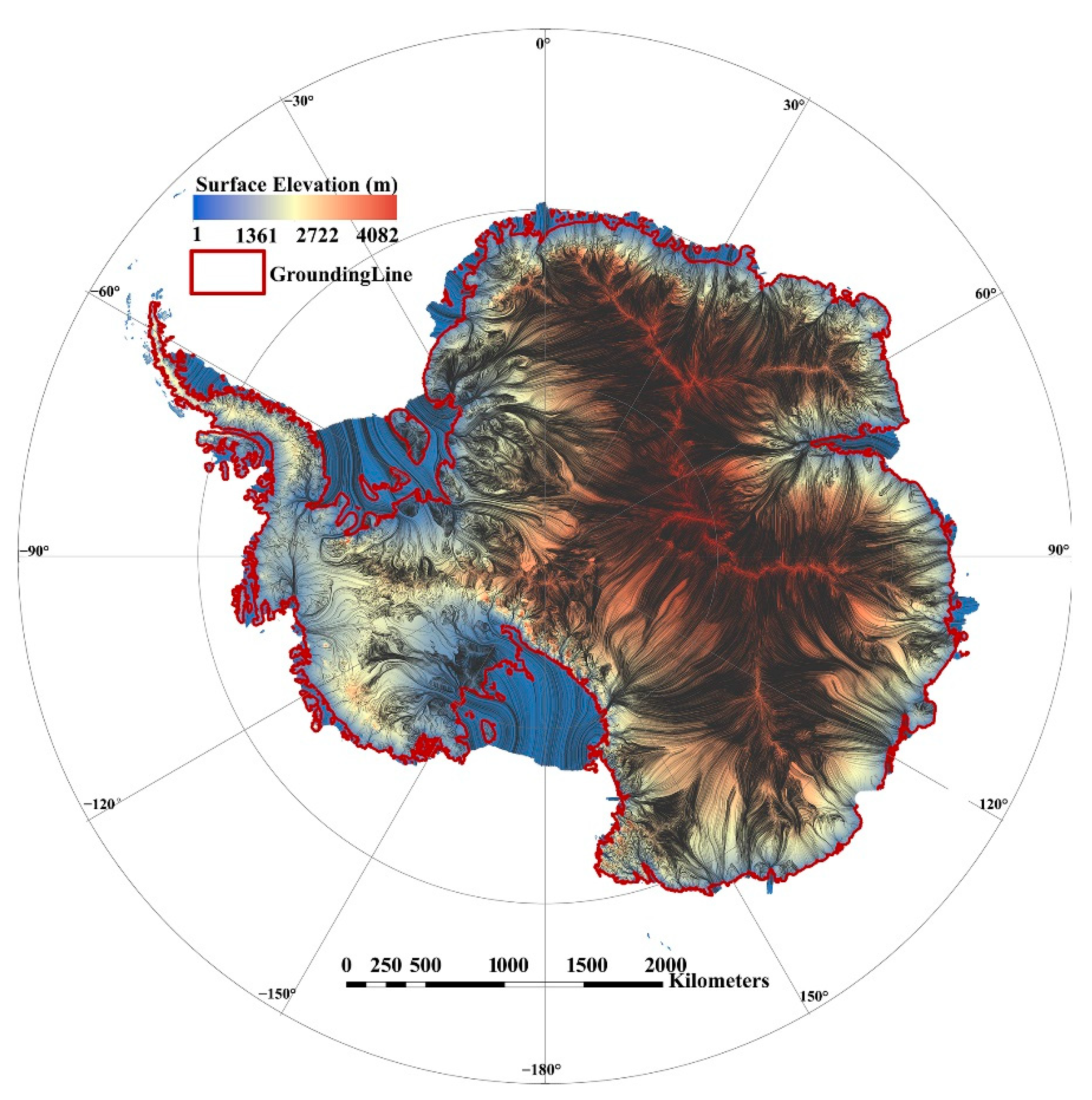
3.1. Experimental Data
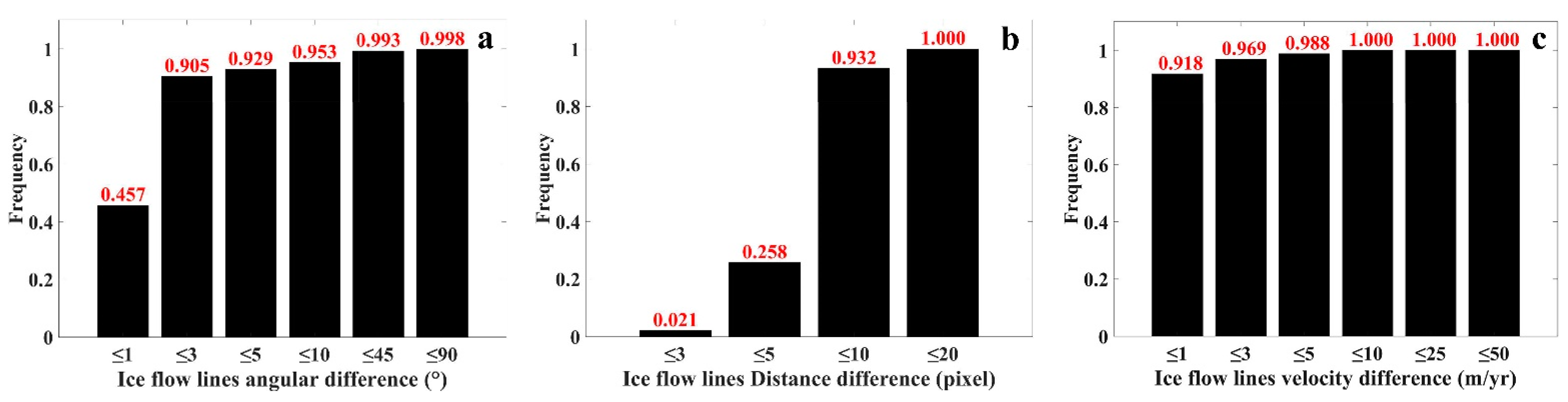
3.2. Quantitative Assessment of Ice Flow Lines Extraction Results
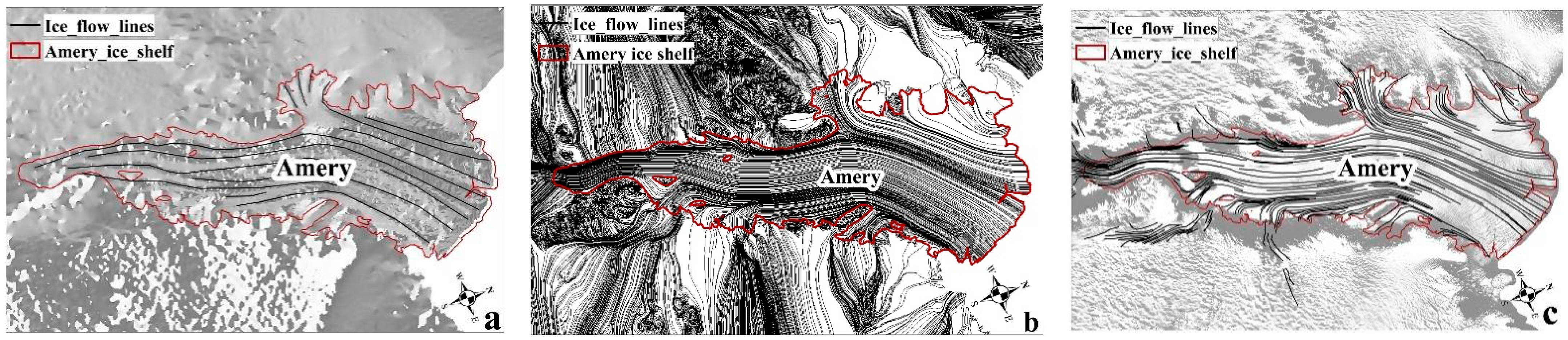
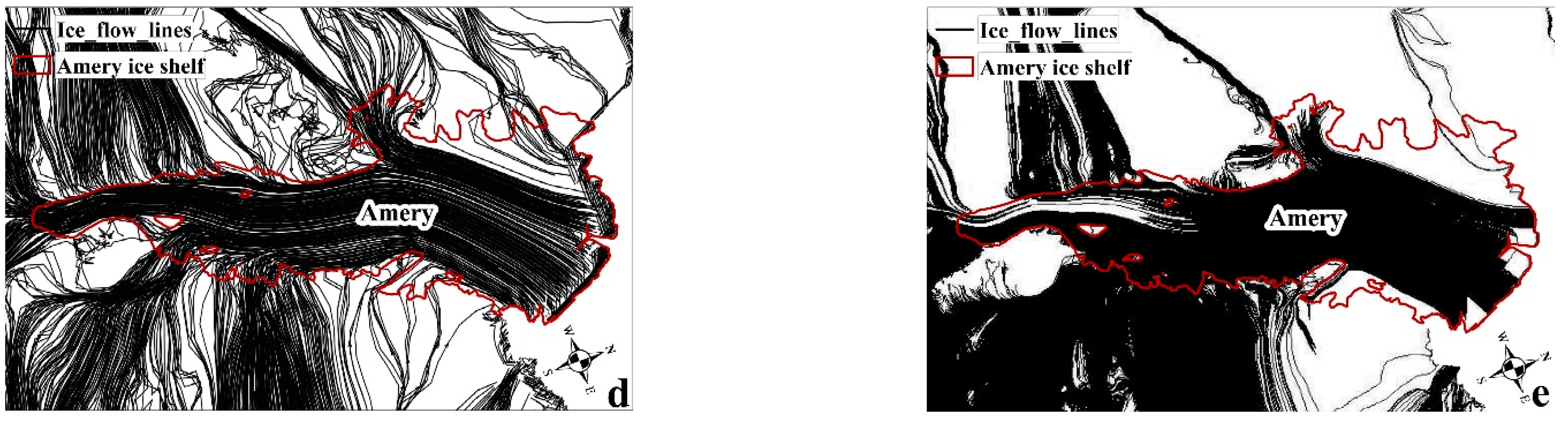
3.3. Qualitative Assessment of Ice Flow Lines Extraction Results
3.4. Comparison of the Proposed Method with Other Flow Lines Extraction Methods
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dowdeswell, J.A.; Mcintyre, N. The surface topography of large ice masses from Landsat imagery. J. Glaciol. 1987, 33, 16–23. [Google Scholar] [CrossRef]
- Ely, J.C.; Clark, C.D. Flow-stripes and foliations of the Antarctic ice sheet. J. Maps 2016, 12, 249–259. [Google Scholar] [CrossRef]
- Crabtree, R.D.; Doake, C.S.M. Flow lines on Antarctic ice shelves. Polar Rec. 1980, 20, 31–37. [Google Scholar] [CrossRef]
- Fahnestock, M.; Scambos, T.; Bindschadler, R.; Kvaran, G. A millennium of variable ice flow recorded by the Ross Ice Shelf, Antarctica. J. Glaciol. 2000, 46, 652–664. [Google Scholar] [CrossRef]
- Glasser, N.; Jennings, S.J.A.; Hambrey, M.; Hubbard, B. Origin and dynamic significance of longitudinal structures (“flow stripes”) in the Antarctic Ice Sheet. Earth Surf. Dyn. 2015, 3, 239–249. [Google Scholar] [CrossRef]
- Liu, Y.; Moore, J.C.; Cheng, X.; Gladstone, R.M.; Bassis, J.N.; Liu, H.; Wen, J.; Hui, F. Ocean-driven thinning enhances iceberg calving and retreat of Antarctic ice shelves. Proc. Natl. Acad. Sci. USA 2015, 112, 3263–3268. [Google Scholar] [CrossRef]
- Glasser, N.; Scambos, T.A. A structural glaciological analysis of the 2002 Larsen B ice-shelf collapse. J. Glaciol. 2008, 54, 3–16. [Google Scholar] [CrossRef]
- Glasser, N.F.; Gudmundsson, H. Longitudinal surface structures (flowstripes) on Antarctic glaciers. Cryosphere 2012, 6, 383–391. [Google Scholar] [CrossRef]
- Das, I.; Padman, L.; Bell, R.E.; Fricker, H.A.; Tinto, K.J.; Hulbe, C.L.; Siddoway, C.S.; Dhakal, T.; Frearson, N.P.; Mosbeux, C. Multidecadal Basal Melt Rates and Structure of the Ross Ice Shelf, Antarctica, Using Airborne Ice Penetrating Radar. J. Geophys. Res. Earth Surf. 2020, 125, e2019JF005241. [Google Scholar] [CrossRef]
- Parrenin, F.; Remy, F.; Ritz, C.; Siegert, M.J.; Jouzel, J. New modeling of the Vostok ice flow line and implication for the glaciological chronology of the Vostok ice core. J. Geophys. Res. Atmos. 2004, 109, D20102. [Google Scholar] [CrossRef]
- Casassa, G.; Brecher, H. Relief and decay of flow stripes on Byrd Glacier, Antarctica. Ann. Glaciol. 1993, 17, 255–261. [Google Scholar] [CrossRef][Green Version]
- Raup, B.H.; Scambos, T.A.; Haran, T. Topography of streaklines on an Antarctic ice shelf from photoclinometry applied to a single Advanced Land Imager (ALI) image. IEEE Trans. Geosci. Remote Sens. 2005, 43, 736–742. [Google Scholar] [CrossRef]
- Young, N.; Hyland, G. Velocity and strain rates derived from InSAR analysis over the Amery Ice Shelf, East Antarctica. Ann. Glaciol. 2002, 34, 228–234. [Google Scholar] [CrossRef][Green Version]
- Shepherd, A.; Ivins, E.R.; Geruo, A.; Barletta, V.R.; Bentley, M.J.; Bettadpur, S.; Briggs, K.; Bromwich, D.H.; Forsberg, R.; Galin, N. A Reconciled Estimate of Ice-Sheet Mass Balance. Science 2012, 338, 1183–1189. [Google Scholar] [CrossRef]
- Pritchard, H.; Ligtenberg, S.R.; Fricker, H.A.; Vaughan, D.G.; van den Broeke, M.R.; Padman, L. Antarctic ice-sheet loss driven by basal melting of ice shelves. Nature 2012, 484, 502–505. [Google Scholar] [CrossRef] [PubMed]
- Rignot, E.; Velicogna, I.; van den Broeke, M.R.; Monaghan, A.; Lenaerts, J.T. Acceleration of the contribution of the Greenland and Antarctic ice sheets to sea level rise. Geophys. Res. Lett. 2011, 38, L05503. [Google Scholar] [CrossRef]
- Zhan, J.; Shi, H.; Wang, Y.; Yao, Y. Complex Principal Component Analysis of Antarctic Ice Sheet Mass Balance. Remote Sens. 2021, 13, 480. [Google Scholar] [CrossRef]
- Ferreira, V.G.; Yong, B.; Seitz, K.; Heck, B.; Grombein, T. Introducing an Improved GRACE Global Point-Mass Solution—A Case Study in Antarctica. Remote Sens. 2020, 12, 3197. [Google Scholar] [CrossRef]
- Shepherd, A.; Ivins, E.; Rignot, E.; Smith, B.; Van Den Broeke, M.; Velicogna, I.; Whitehouse, P.; Briggs, K.; Joughin, I.; Krinner, G. Mass balance of the Antarctic Ice Sheet from 1992 to 2017. Nature 2018, 558, 219–222. [Google Scholar]
- Monaghan, A.J.; Bromwich, D.H.; Fogt, R.L.; Wang, S.-H.; Mayewski, P.A.; Dixon, D.A.; Ekaykin, A.; Frezzotti, M.; Goodwin, I.; Isaksson, E. Insignificant change in Antarctic snowfall since the International Geophysical Year. Science 2006, 313, 827–831. [Google Scholar] [CrossRef]
- Rignot, E.; Bamber, J.L.; Van Den Broeke, M.R.; Davis, C.; Li, Y.; Van De Berg, W.J.; Van Meijgaard, E. Recent Antarctic ice mass loss from radar interferometry and regional climate modelling. Nat. Geosci. 2008, 1, 106–110. [Google Scholar] [CrossRef]
- Boening, C.; Lebsock, M.; Landerer, F.; Stephens, G. Snowfall-driven mass change on the East Antarctic ice sheet. Geophys. Res. Lett. 2012, 39, L21501. [Google Scholar] [CrossRef]
- Mouginot, J.; Rignot, E.; Bjørk, A.A.; Van den Broeke, M.; Millan, R.; Morlighem, M.; Noël, B.; Scheuchl, B.; Wood, M. Forty-six years of Greenland Ice Sheet mass balance from 1972 to 2018. Proc. Natl. Acad. Sci. USA 2019, 116, 9239–9244. [Google Scholar] [CrossRef] [PubMed]
- Rignot, E.; Mouginot, J.; Scheuchl, B.; Van Den Broeke, M.; Van Wessem, M.J.; Morlighem, M. Four decades of Antarctic Ice Sheet mass balance from 1979–2017. Proc. Natl. Acad. Sci. USA 2019, 116, 1095–1103. [Google Scholar] [CrossRef]
- Wingham, D.; Shepherd, A.; Muir, A.; Marshall, G. Mass balance of the Antarctic ice sheet. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2006, 364, 1627–1635. [Google Scholar] [CrossRef] [PubMed]
- Floricioiu, D. Drainage basin delineation for outlet glaciers of Northeast Greenland based on Sentinel-1 ice velocities and TanDEM-X elevations. Remote Sens. Environ. 2020, 237, 111483. [Google Scholar]
- Rignot, E.; Mouginot, J. Ice flow in Greenland for the international polar year 2008–2009. Geophys. Res. Lett. 2012, 39, L11501. [Google Scholar] [CrossRef]
- Weidick, A.; Williams, R.S.; Ferrigno, J.G. Satellite Image Atlas of Glaciers of the World, Greenland. In U.S. Geological Survey Professional Paper 1386-C; Williams, R.S., Ferrigno, J., Eds.; U.S. Geological Survey: Denver, CO, USA, 1995; p. 153. [Google Scholar]
- Mouginot, J.; Rignot, E.; Scheuchl, B.; Fenty, I.; Khazendar, A.; Morlighem, M.; Buzzi, A.; Paden, J. Fast retreat of Zachariæ Isstrøm, northeast Greenland. Science 2015, 350, 1357–1361. [Google Scholar] [CrossRef]
- Seleem, T.A. Analysis and Tectonic Implication of DEM-Derived Structural Lineaments, Sinai Peninsula, Egypt. Int. J. Geosci. 2013, 4, 183–201. [Google Scholar] [CrossRef]
- Glasser, N.; Jennings, S.; Hambrey, M.; Hubbard, B. Are longitudinal ice-surface structures on the Antarctic Ice Sheet indicators of long-term ice-flow configuration? Earth Surf. Dyn. Discuss. 2014, 2, 911–933. [Google Scholar]
- Budd, W.; Warner, R. A computer scheme for rapid calculations of balance-flux distributions. Ann. Glaciol. 1996, 23, 21–27. [Google Scholar] [CrossRef]
- Popov, S. Flow-lines computation and their use in subglacial geomorphology and glacial erosion modeling: The Princess Elizabeth land (East Antarctica) case study. Geomorfologiya 2017, 1, 46–54. [Google Scholar] [CrossRef]
- Glasser, N.; Scambos, T.; Bohlander, J.; Truffer, M.; Pettit, E.; Davies, B. From ice-shelf tributary to tidewater glacier: Continued rapid recession, acceleration and thinning of Röhss Glacier following the 1995 collapse of the Prince Gustav Ice Shelf, Antarctic Peninsula. J. Glaciol. 2011, 57, 397–406. [Google Scholar] [CrossRef]
- Ely, J.; Clark, C.; Ng, F.; Spagnolo, M. Insights on the formation of longitudinal surface structures on ice sheets from analysis of their spacing, spatial distribution, and relationship to ice thickness and flow. J. Geophys. Res. Earth Surf. 2017, 122, 961–972. [Google Scholar] [CrossRef]
- Tarboton, D.G. A new method for the determination of flow directions and upslope areas in grid digital elevation models. Water Resour. Res. 1997, 33, 309–319. [Google Scholar] [CrossRef]
- Casassa, G.; Jezek, K.; Turner, J.; Whillans, I. Relict flow stripes on the Ross Ice Shelf. Ann. Glaciol. 1991, 15, 132–138. [Google Scholar] [CrossRef]
- Glasser, N.; Kulessa, B.; Luckman, A.; Jansen, D.; King, E.C.; Sammonds, P.; Scambos, T.; Jezek, K. Surface structure and stability of the Larsen C ice shelf, Antarctic Peninsula. J. Glaciol. 2009, 55, 400–410. [Google Scholar] [CrossRef]
- Gudmundsson, G.H.; Raymond, C.F.; Bindschadler, R. The origin and longevity of flow stripes on Antarctic ice streams. Ann. Glaciol. 1998, 27, 145–152. [Google Scholar] [CrossRef]
- Giovinetto, M. Surface balance in ice drainage systems of Antarctica. Antarct. J. USA 1985, 20, 6–13. [Google Scholar]
- Glovinetto, M.; Zwally, H. Spatial distribution of net surface accumulation on the Antarctic ice sheet. Ann. Glaciol. 2000, 31, 171–178. [Google Scholar] [CrossRef]
- Ze, Y.; Zhizhong, K. Data from:Antarctic Drainage Basins Delineation; Science Data Bank: Beijing, China, 2022. [Google Scholar] [CrossRef]
- Mouginot, J.; Rignot, E.; Scheuchl, B. Continent-wide, interferometric SAR phase, mapping of Antarctic ice velocity. Geophys. Res. Lett. 2019, 46, 9710–9718. [Google Scholar] [CrossRef]
- Rignot, E.; Mouginot, J.; Scheuchl, B. Ice flow of the Antarctic ice sheet. Science 2011, 333, 1427–1430. [Google Scholar] [CrossRef]
- Rignot, E.; Jacobs, S.; Mouginot, J.; Scheuchl, B. Ice-shelf melting around Antarctica. Science 2013, 341, 266–270. [Google Scholar] [CrossRef]
- Mouginot, J.; Scheuchl, B.; Rignot, E. Mapping of ice motion in Antarctica using synthetic-aperture radar data. Remote Sens. 2012, 4, 2753–2767. [Google Scholar] [CrossRef]
- Fretwell, P.; Pritchard, H.D.; Vaughan, D.G.; Bamber, J.L.; Barrand, N.E.; Bell, R.; Bianchi, C.; Bingham, R.; Blankenship, D.D.; Casassa, G. Bedmap2: Improved ice bed, surface and thickness datasets for Antarctica. Cryosphere 2013, 7, 375–393. [Google Scholar] [CrossRef]
- Scambos, T.A.; Haran, T.M.; Fahnestock, M.; Painter, T.; Bohlander, J. MODIS-based Mosaic of Antarctica (MOA) data sets: Continent-wide surface morphology and snow grain size. Remote Sens. Environ. 2007, 111, 242–257. [Google Scholar] [CrossRef]
- Qi, M.; Liu, Y.; Lin, Y.; Hui, F.; Li, T.; Cheng, X. Efficient Location and Extraction of the Iceberg Calved Areas of the Antarctic Ice Shelves. Remote Sens. 2020, 12, 2658. [Google Scholar] [CrossRef]
- Francis, D.; Mattingly, K.S.; Lhermitte, S.; Temimi, M.; Heil, P. Atmospheric extremes triggered the biggest calving event in more than 50 years at the Amery Ice shelf in September 2019. Cryosphere 2021, 15, 2147–2165. [Google Scholar] [CrossRef]

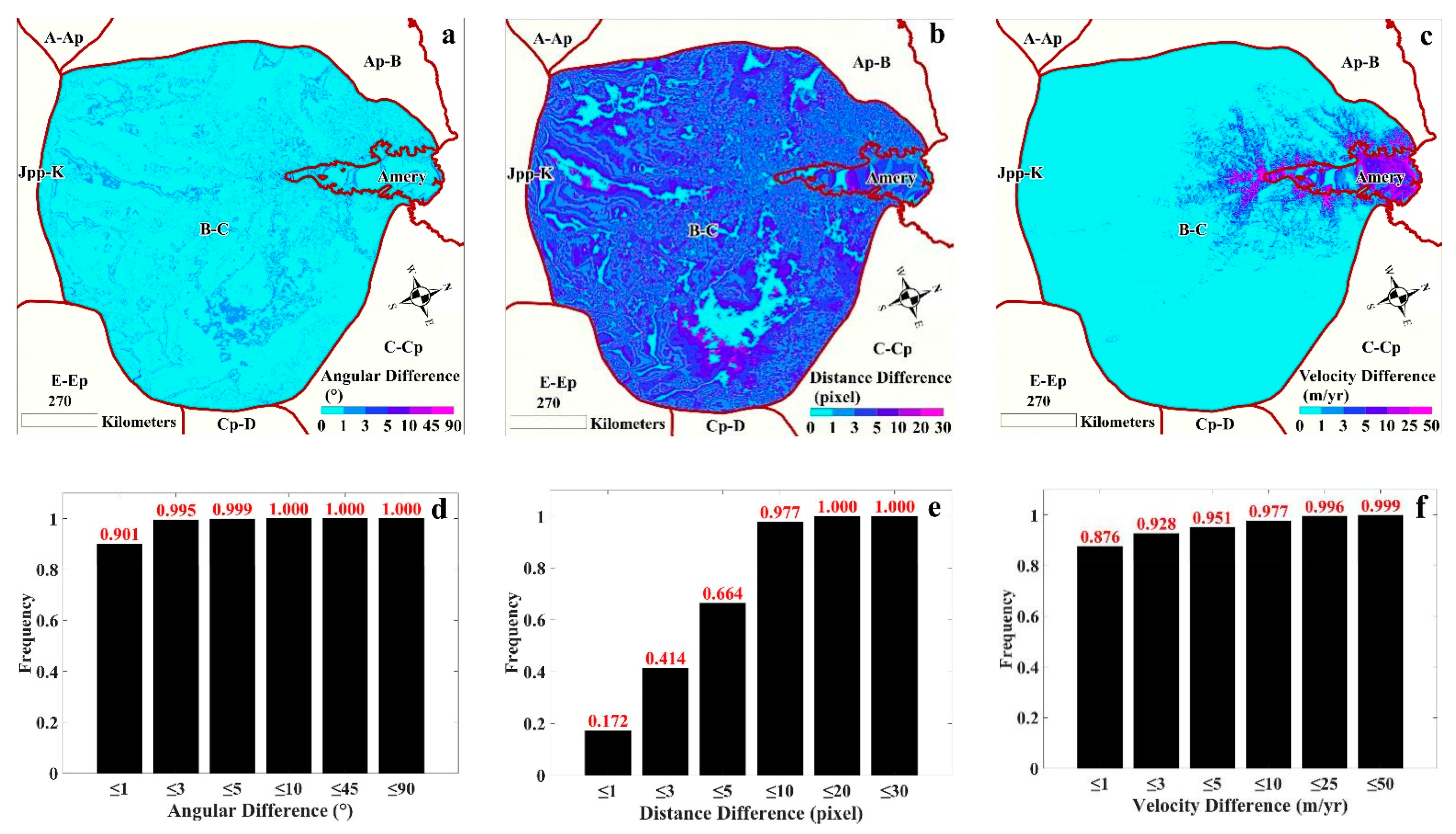




| The Maximum Value of the Angular Difference of the Ice Flow Lines (°) | Value | The Maximum Value of the Distance Difference of the Ice Flow Lines (Pixel) | Value | The Maximum Value of the Velocity Difference of the Ice Flow Lines (m/yr) | Value |
|---|---|---|---|---|---|
| ≤1 | 39.298% | ≤1 | 4.957% | ≤1 | 90.487% |
| ≤3 | 82.966% | ≤3 | 9.968% | ≤3 | 94.978% |
| ≤5 | 90.836% | ≤5 | 19.015% | ≤5 | 96.457% |
| ≤10 | 92.312% | ≤10 | 76.323% | ≤10 | 97.888% |
| Cumulative Frequency of Ice Flow Lines Angular Difference | Cumulative Frequency of Ice Flow Lines Distance Difference | Cumulative Frequency of Ice Flow Lines Velocity Difference | ||||||
|---|---|---|---|---|---|---|---|---|
| Value (°) | Method Only Considering Angular Difference | Proposed Method | Value (Pixel) | Method Only Considering Angular Difference | Proposed Method | Value (m/yr) | Method Only Considering Angular Difference | Proposed Method |
| ≤1 | 81.793% | 45.743% | ≤1 | 0.001% | 0.001% | ≤1 | 78.943% | 91.752% |
| ≤3 | 98.906% | 90.451% | ≤3 | 0.077% | 2.073% | ≤3 | 84.760% | 96.920% |
| ≤5 | 99.770% | 92.897% | ≤5 | 0.923% | 25.756% | ≤5 | 86.333% | 98.203% |
| ≤10 | 99.986% | 95.281% | ≤10 | 35.071% | 93.180% | ≤10 | 87.781% | 99.296% |
| ≤45 | 100.000% | 99.284% | ≤20 | 94.697% | 99.996% | ≤25 | 89.067% | 99.902% |
| ≤90 | 100.000% | 99.795% | ≤50 | 89.712% | 99.979% | |||
| Regions | Sub-Regions | Length (km) | Area (km2) | Redefined Length (km) | Redefined Area (km2) | Length Change | Area Change |
|---|---|---|---|---|---|---|---|
| Islands | 17,589.189 | 139,160.180 | 17,589.189 | 139,160.180 | 0.000% | 0.000% | |
| East | A-Ap | 5703.677 | 770,384.207 | 6128.055 | 771,684.087 | 7.440% | 0.169% |
| East | Ap-B | 6620.776 | 651,006.313 | 6714.151 | 655,561.090 | 1.410% | 0.700% |
| East | B-C | 5977.122 | 1,310,514.876 | 6404.936 | 1,297,067.889 | 7.158% | −1.026% |
| East | C-Cp | 4394.592 | 707,717.272 | 4264.744 | 668,426.326 | −2.955% | −5.552% |
| East | Cp-D | 6458.859 | 1,161,381.347 | 6630.339 | 1,192,855.358 | 2.655% | 2.710% |
| East | D-Dp | 4578.005 | 696,333.432 | 4895.891 | 714,171.936 | 6.944% | 2.562% |
| East | Dp-E | 6999.412 | 427,433.725 | 7160.848 | 426,510.377 | 2.306% | −0.216% |
| East | E-Ep | 6558.617 | 1,580,595.000 | 6628.415 | 1,566,231.895 | 1.064% | −0.909% |
| West | Ep-F | 6804.555 | 803,191.560 | 7357.352 | 799,815.600 | 8.124% | −0.420% |
| West | F-G | 2928.422 | 131,180.136 | 3009.061 | 128,094.479 | 2.754% | −2.352% |
| West | G-H | 4601.994 | 419,498.184 | 5080.809 | 415,012.018 | 10.405% | −1.069% |
| West | H-Hp | 2459.309 | 64,903.260 | 2532.910 | 62,281.978 | 2.993% | −4.039% |
| Peninsula | Hp-I | 5176.313 | 147,597.602 | 5255.109 | 147,858.341 | 1.522% | 0.177% |
| Peninsula | I-Ipp | 5721.814 | 71,719.604 | 5730.458 | 70,845.386 | 0.151% | −1.219% |
| Peninsula | Ipp-J | 3020.307 | 61,336.468 | 3097.389 | 60,618.217 | 2.552% | −1.171% |
| West | J-Jpp | 6817.228 | 619,486.252 | 7396.829 | 638,383.950 | 8.502% | 3.051% |
| East | Jpp-K | 6493.182 | 2,056,335.542 | 6970.393 | 2,068,936.419 | 7.349% | 0.613% |
| East | K-A | 3927.796 | 259,047.918 | 4214.729 | 255,306.078 | 7.305% | −1.444% |
| Angular Difference (°) | Cumulative Frequency |
|---|---|
| ≤1 | 90.529% |
| ≤3 | 98.948% |
| ≤5 | 99.716% |
| ≤10 | 99.947% |
| ≤90 | 100.000% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Z.; Kang, Z. Antarctic-Scale Ice Flow Lines Map Generation and Basin Delineation. Remote Sens. 2022, 14, 1958. https://doi.org/10.3390/rs14091958
Yang Z, Kang Z. Antarctic-Scale Ice Flow Lines Map Generation and Basin Delineation. Remote Sensing. 2022; 14(9):1958. https://doi.org/10.3390/rs14091958
Chicago/Turabian StyleYang, Ze, and Zhizhong Kang. 2022. "Antarctic-Scale Ice Flow Lines Map Generation and Basin Delineation" Remote Sensing 14, no. 9: 1958. https://doi.org/10.3390/rs14091958
APA StyleYang, Z., & Kang, Z. (2022). Antarctic-Scale Ice Flow Lines Map Generation and Basin Delineation. Remote Sensing, 14(9), 1958. https://doi.org/10.3390/rs14091958