Seasonal Variations in the Vertical Wavenumber Spectra of Stratospheric Gravity Waves in the Asian Monsoon Region Derived from COSMIC-2 Data
Abstract
1. Introduction
2. Materials and Methods
3. Results
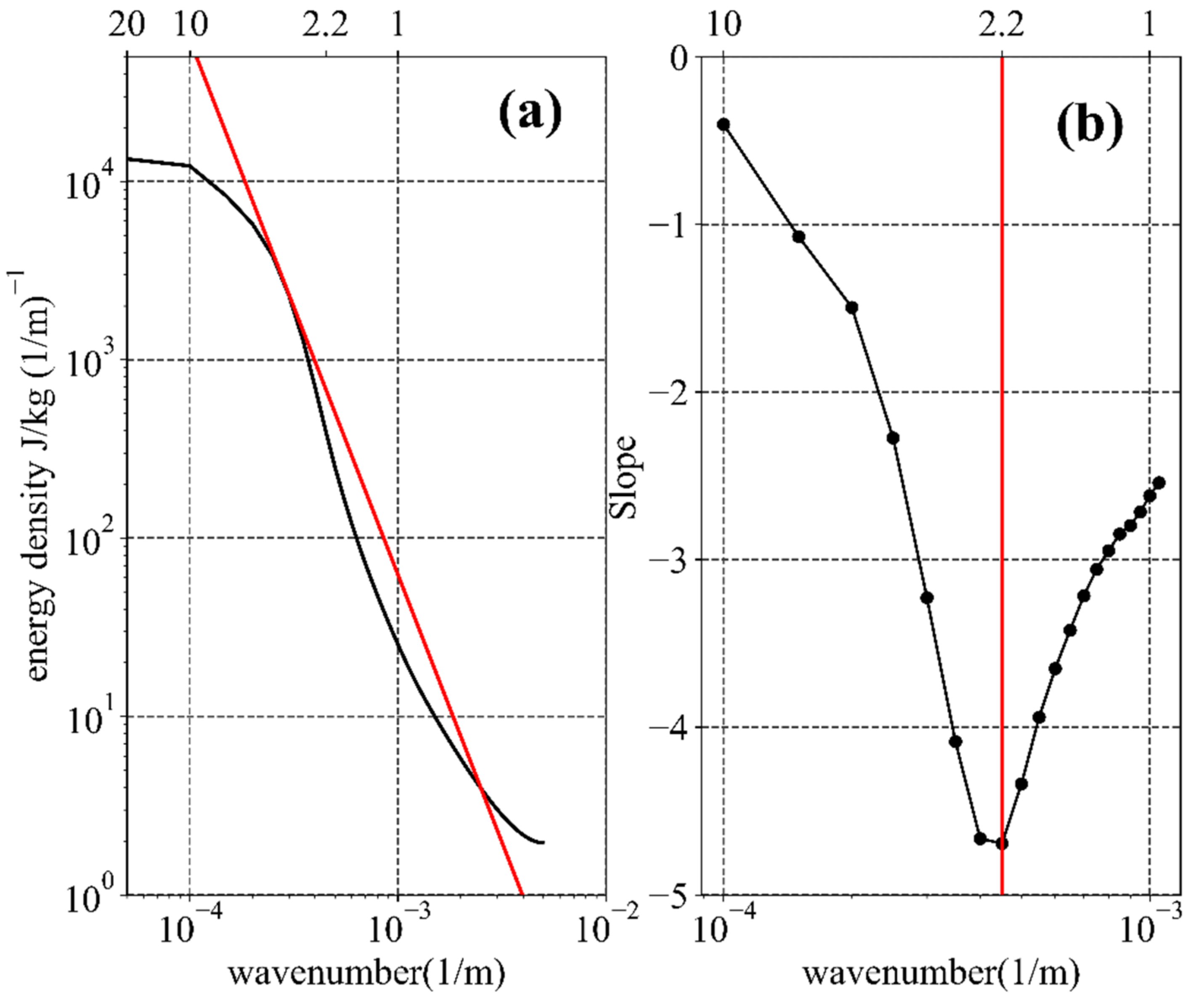
3.1. Distribution and Seasonal Variations of the Vertical Wavenumber Spectra
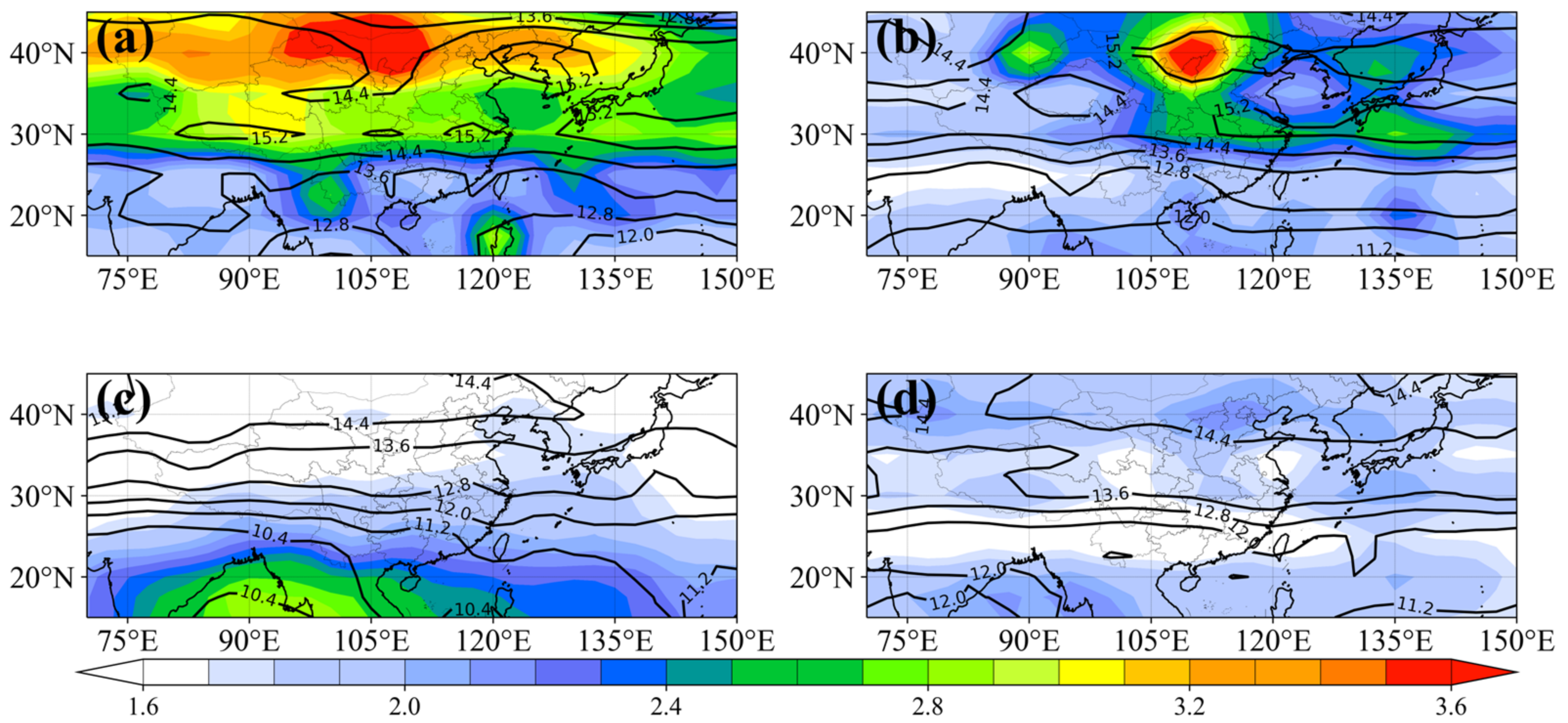
3.2. Latitude–Longitude Distribution and Seasonal Variations of the Vertical Wavenumber Spectra
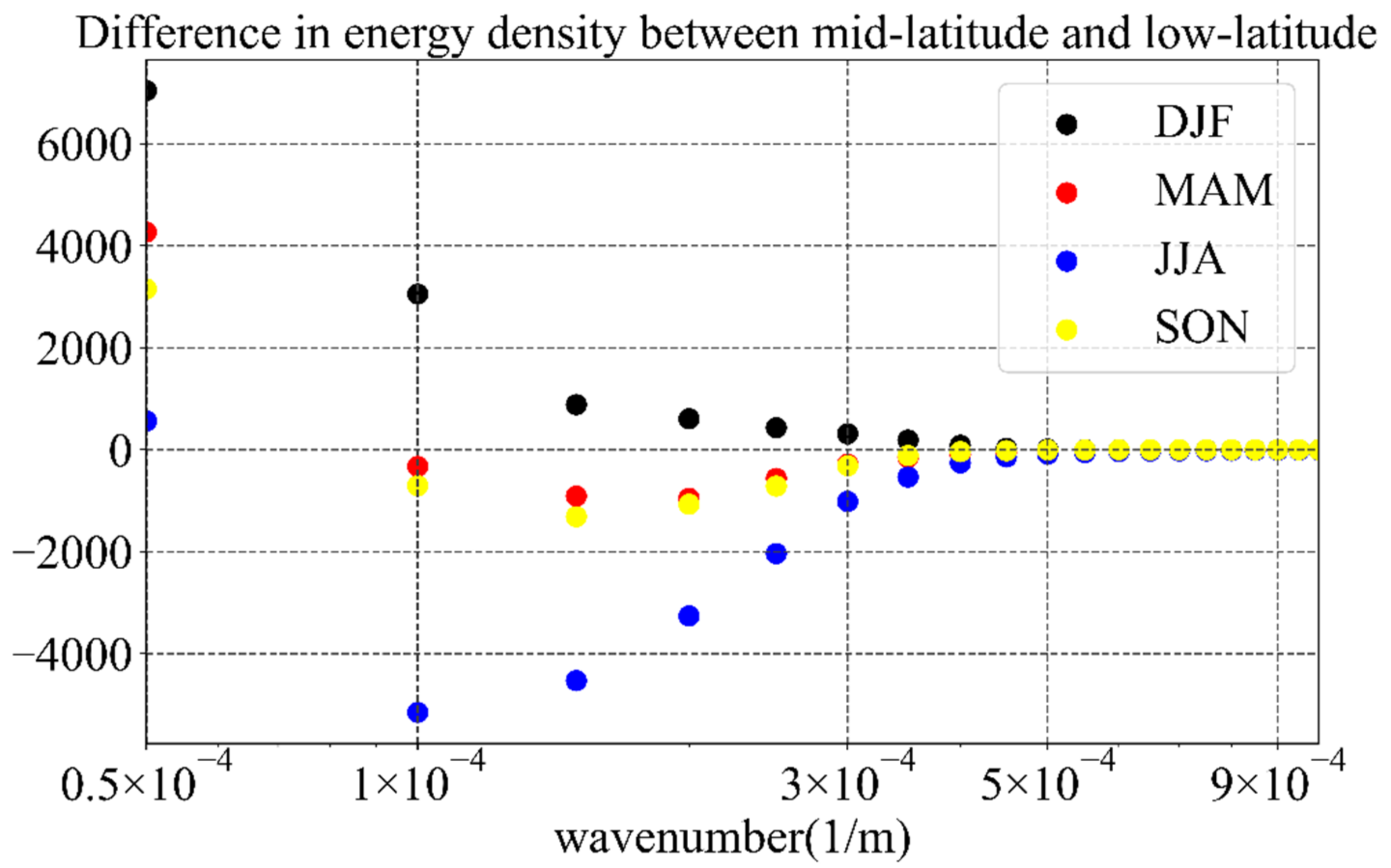
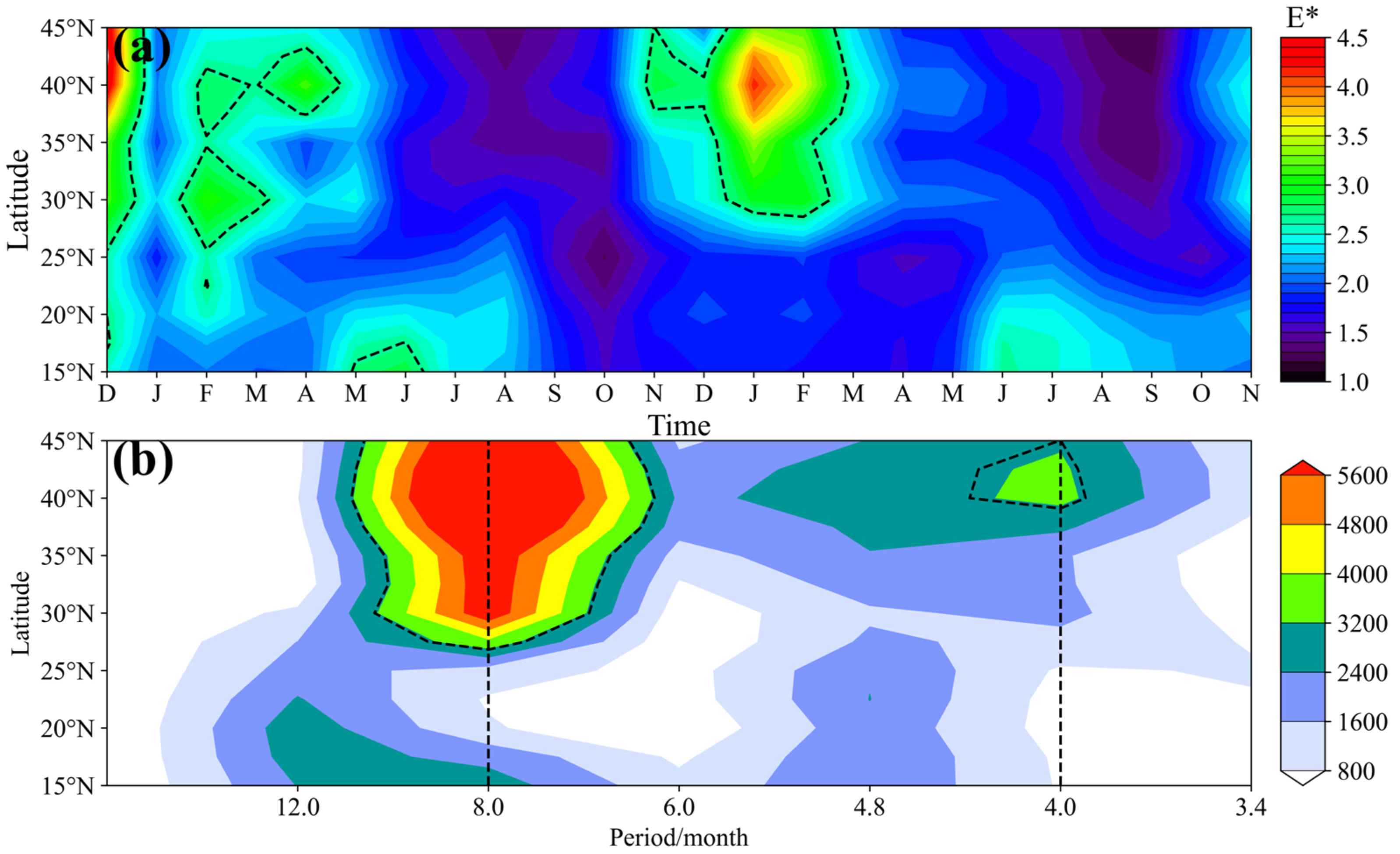
3.3. Latitude–Temporal Distribution of the Spectral Amplitude
4. Discussion
5. Conclusions
- (1)
- In the Asian monsoon region, the Ep spectral density of the stratospheric GWs at heights of 20–40 km decreases with increasing vertical wavenumber, and the spectral slope S varies with wavenumber. From 0.1 to 0.45 km−1, S becomes smaller as the wavenumber increases, and from 0.45 to 1 km−1, S becomes larger with increasing wavenumber.
- (2)
- In different seasons, there are also differences in the spectral distributions at MLs and LLs. In winter, is larger at MLs than at LLs for wavenumbers of 0.05–0.5 km−1. In summer, is larger at LLs than at MLs for wavenumbers of 0.1 to 1 km−1.
- (3)
- Both and exhibit a band-like distribution, and the high-value bands and their centers show obvious seasonal variations. In winter, at MLs is larger than that at LLs, and the high value band is at ~40°N. In contrast, in summer, at LLs is greater than that at MLs, and there are high value regions over the Bay of Bengal and Indo-China Peninsula. The intensification of the subtropical jet at MLs in winter and an increase in convection at LLs in summer may explain these observations. The high values at MLs correspond to a longer characteristic wavelength with values of >15.2 km. The high values of at LLs correspond to a shorter vertical wavelength with value of 10.4 km.
- (4)
- The distribution of S also exhibits significant seasonal variations. In winter, S at MLs is smaller and at LLs is larger, whereas in summer this pattern is reversed.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Fritts, D.C.; Alexander, M.J. Gravity wave dynamics and effects in the middle atmosphere. Rev. Geophys. 2003, 41, 1003. [Google Scholar] [CrossRef]
- Schmidt, T.; Alexander, P.; Torre, A. Stratospheric gravity wave momentum flux from radio occultations. J. Geophys. Res. 2016, 121, 4443–4467. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, L.; Zhang, Y.; Guan, J. Effects of Tropospheric Vertical Wind Shear on Gravity Waves Generated by Tropical Cyclones. Geophys. Res. Lett. 2019, 46, 4523–4530. [Google Scholar] [CrossRef]
- Zhang, S.D.; Yi, F.; Huang, C.M.; Zhou, Q.J. Latitudinal and seasonal variations of lower atmospheric inertial gravity wave energy revealed by US radiosonde data. Ann. Geophys. 2010, 28, 1065–1074. [Google Scholar] [CrossRef]
- VanZandt, T.E. A universal spectrum of buoyancy waves in the atmosphere. Geophys. Res. Lett. 1982, 9, 575–578. [Google Scholar] [CrossRef]
- Dewan, E.M.; Good, R.E. Saturation and the Universal Spectrum for Vertical Profiles of Horizontal Scalar Winds in the Atmosphere. J. Geophys. Res. 1986, 91, 2742–2748. [Google Scholar] [CrossRef]
- Smith, S.A.; Fritts, D.C.; Vanzandt, T.E. Evidence for a Saturated Spectrum of Atmospheric Gravity Waves. J. Atmos. Sci. 1987, 44, 1404–1410. [Google Scholar] [CrossRef]
- Dewan, E.M.; Grossbard, N.J. Power spectral artifacts in published balloon data and implications regarding saturated gravity wave theories. J. Geophys. Res. 2000, 105, 4667–4683. [Google Scholar] [CrossRef]
- Zhang, S.; Huang, C.; Yi, F. Radiosonde observations of vertical wave number spectra for gravity waves in the lower atmosphere over Central China. Ann. Geophys. 2006, 24, 3257–3265. [Google Scholar] [CrossRef]
- Zhang, S.; Yi, F.; Huang, C.; Chen, Z. Intensive radiosonde observations of gravity waves in the lower atmosphere over Yichang (111°18″ E, 30°42″ N), China. Ann. Geophys. 2008, 26, 2005–2018. [Google Scholar] [CrossRef]
- Zhang, S.; Huang, C.; Huang, K.; Zhang, Y.; Gong, Y.; Gan, Q. Vertical wavenumber spectra of three-dimensional winds revealed by radiosonde observations at midlatitude. Ann. Geophys. 2017, 35, 107–116. [Google Scholar] [CrossRef]
- Wu, Y.; Yuan, W.; Xu, J. Gravity wave activity in the troposphere and lower stratosphere: An observational study of seasonal and interannual variations(Article). J. Geophys. Res. 2013, 118, 11352–11359. [Google Scholar] [CrossRef]
- Allen, S.J.; Vincent, R.A. Gravity wave activity in the lower atmosphere-seasonal and latitudinal variations. J. Geophys. Res. 1995, 100, 1327–1350. [Google Scholar] [CrossRef]
- Steiner, A.K.; Kirchengast, G. Gravity wave spectra from GPS/MET occultation observations. J. Atmos. Ocean. Technol. 2000, 17, 495–503. [Google Scholar] [CrossRef]
- Noersomadi; Tsuda, T. Global distribution of vertical wavenumber spectra in the lower stratosphere observed using high-vertical-resolution temperature profiles from COSMIC GPS radio occultation. Ann. Geophys. 2016, 34, 203–213. [Google Scholar] [CrossRef]
- Tsuda, T.; Nishida, M.; Rocken, C.; Ware, R.H. A global morphology of gravity wave activity in the stratosphere revealed by the GPS occultation data (GPS/MET). J. Geophys. Res. 2000, 105, 7257–7273. [Google Scholar] [CrossRef]
- Wright1, C.J.; Rivas1, M.B.; Gille, J.C. Intercomparisons of HIRDLS, COSMIC and SABER for the detection of stratospheric gravity waves. Atmos. Meas. Tech. 2011, 4, 1581–1591. [Google Scholar] [CrossRef]
- Qu, T.; Zhang, L.; Wang, Y.; Wang, X.; Guan, J. Seasonal Variation of Stratospheric Gravity Waves in the Asian Monsoon Region Derived from COSMIC-2 Data. Remote Sens. 2022, 14, 4096. [Google Scholar] [CrossRef]
- Hindley, N.P.; Wright, C.J.; Smith, N.D.; Mitchell, N.J. The southern stratospheric gravity-wave hot spot: Individual waves and their momentum fluxes measured by COSMIC GPS-RO. Atmos. Chem. Phys. Discuss. 2015, 15, 3173–3217. [Google Scholar] [CrossRef]
- Ho, S.P.; Zhou, X.; Shao, X.; Zhang, B.; Adhikari, L.; Kireev, S.; He, Y.; Yoe, J.G.; Xia-Serafino, W.; Lynch, E. Initial Assessment of the COSMIC-2/FORMOSAT-7 Neutral Atmosphere Data Quality in NESDIS/STAR Using In Situ and Satellite Data. Remote Sens. 2020, 12, 4099. [Google Scholar] [CrossRef]
- Schreiner, W.S.; Weiss, J.P.; Anthes, R.A.; Braun, J.; Chu, V.; Fong, J.; Hunt, D.; Kuo, Y.H.; Meehan, T.; Serafino, W.; et al. COSMIC-2 Radio Occultation Constellation: First Results. Geophys. Res. Lett. 2020, 47, e2019GL086841. [Google Scholar] [CrossRef]
- Wang, L.; Alexander, M.J. Global estimates of gravity wave parameters from GPS radio occultation temperature data. J. Geophys. Res. Atmos. 2010, 115. [Google Scholar] [CrossRef]
- Faber, A.; Llamedo, P.; Schmidt, T.; Torre, A.D.; Wickert, J. On the determination of gravity wave momentum flux from GPS radio occultation data. Atmos. Meas. Tech. 2013, 11, 3169–3180. [Google Scholar] [CrossRef]
- Wang, L.; Geller, M.A. Morphology of gravity-wave energy as observed from 4 years (1998–2001) of high vertical resolution U.S. radiosonde data. J. Geophys. Res. 2003, 108, 4489. [Google Scholar] [CrossRef]
- Strelnikova, I.; Almowafy, M.; Baumgarten, G.; Baumgarten, K.; Ern, M.; Gerding, M.; Lubken, F.J. Seasonal Cycle of Gravity Wave Potential Energy Densities from Lidar and Satellite Observations at 54° and 69° N. J. Atmos. Sci. 2021, 78, 1359–1386. [Google Scholar] [CrossRef]
- Yan, Y.Y.; Zhang, S.D.; Huang, C.M.; Huang, K.M.; Gong, Y.; Gan, Q. The vertical wave number spectra of potential energy density in the stratosphere deduced from the COSMIC satellite observation. Q. J. R. Meteorol. Soc. 2019, 145, 318–336. [Google Scholar] [CrossRef]
- Lu, X.; Chu, X.; Fong, W.; Chen, C.; Yu, Z.; Roberts, B.R.; McDonald, A.J. Vertical evolution of potential energy density and vertical wave number spectrum of Antarctic gravity waves from 35 to 105km at McMurdo (77.8° S, 166.7° E). J. Geophys. Res. 2015, 120, 2719–2737. [Google Scholar] [CrossRef]
- Marquardt, C.; Healy, S.B. Measurement Noise and Stratospheric Gravity Wave Characteristics Obtained from GPS Occultation Data. J. Meteorol. Soc. Jpn. Ser. II 2005, 83, 417–428. [Google Scholar] [CrossRef]
- Liu, X.; Xu, J.; Jia, Y.; Kogure, M. Persistent Layers of Enhanced Gravity Wave Dissipation in the Upper Mesosphere Revealed From SABER Observations. Geophys. Res. Lett. 2022, 49, e2021GL097038. [Google Scholar] [CrossRef]
- Dunkerton, T.J. Inertia–Gravity Waves in the Stratosphere. J. Atmos. Sci. 1984, 41, 3396–3404. [Google Scholar] [CrossRef]
- Khan, A.; Jin, S. Gravity wave activities in Tibet observed by COSMIC GPS radio occultation. Geod. Geodyn. 2018, 9, 504–511. [Google Scholar] [CrossRef]
- Ratnam, M.; Tetzlaff, G.; Jacobi, C. Global and seasonal variations of stratospheric gravity wave activity deduced from the CHAMP/GPS satellite. J. Atmos. Sci. 2004, 61, 1610–1620. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qu, T.; Zhang, L.; Wang, Y.; Wang, X.; Guan, J. Seasonal Variations in the Vertical Wavenumber Spectra of Stratospheric Gravity Waves in the Asian Monsoon Region Derived from COSMIC-2 Data. Remote Sens. 2022, 14, 6336. https://doi.org/10.3390/rs14246336
Qu T, Zhang L, Wang Y, Wang X, Guan J. Seasonal Variations in the Vertical Wavenumber Spectra of Stratospheric Gravity Waves in the Asian Monsoon Region Derived from COSMIC-2 Data. Remote Sensing. 2022; 14(24):6336. https://doi.org/10.3390/rs14246336
Chicago/Turabian StyleQu, Tao, Lifeng Zhang, Yuan Wang, Xu Wang, and Jiping Guan. 2022. "Seasonal Variations in the Vertical Wavenumber Spectra of Stratospheric Gravity Waves in the Asian Monsoon Region Derived from COSMIC-2 Data" Remote Sensing 14, no. 24: 6336. https://doi.org/10.3390/rs14246336
APA StyleQu, T., Zhang, L., Wang, Y., Wang, X., & Guan, J. (2022). Seasonal Variations in the Vertical Wavenumber Spectra of Stratospheric Gravity Waves in the Asian Monsoon Region Derived from COSMIC-2 Data. Remote Sensing, 14(24), 6336. https://doi.org/10.3390/rs14246336




