Real-Time Multi-GNSS Precise Orbit Determination Based on the Hourly Updated Ultra-Rapid Orbit Prediction Method
Abstract
:1. Introduction
2. Methods
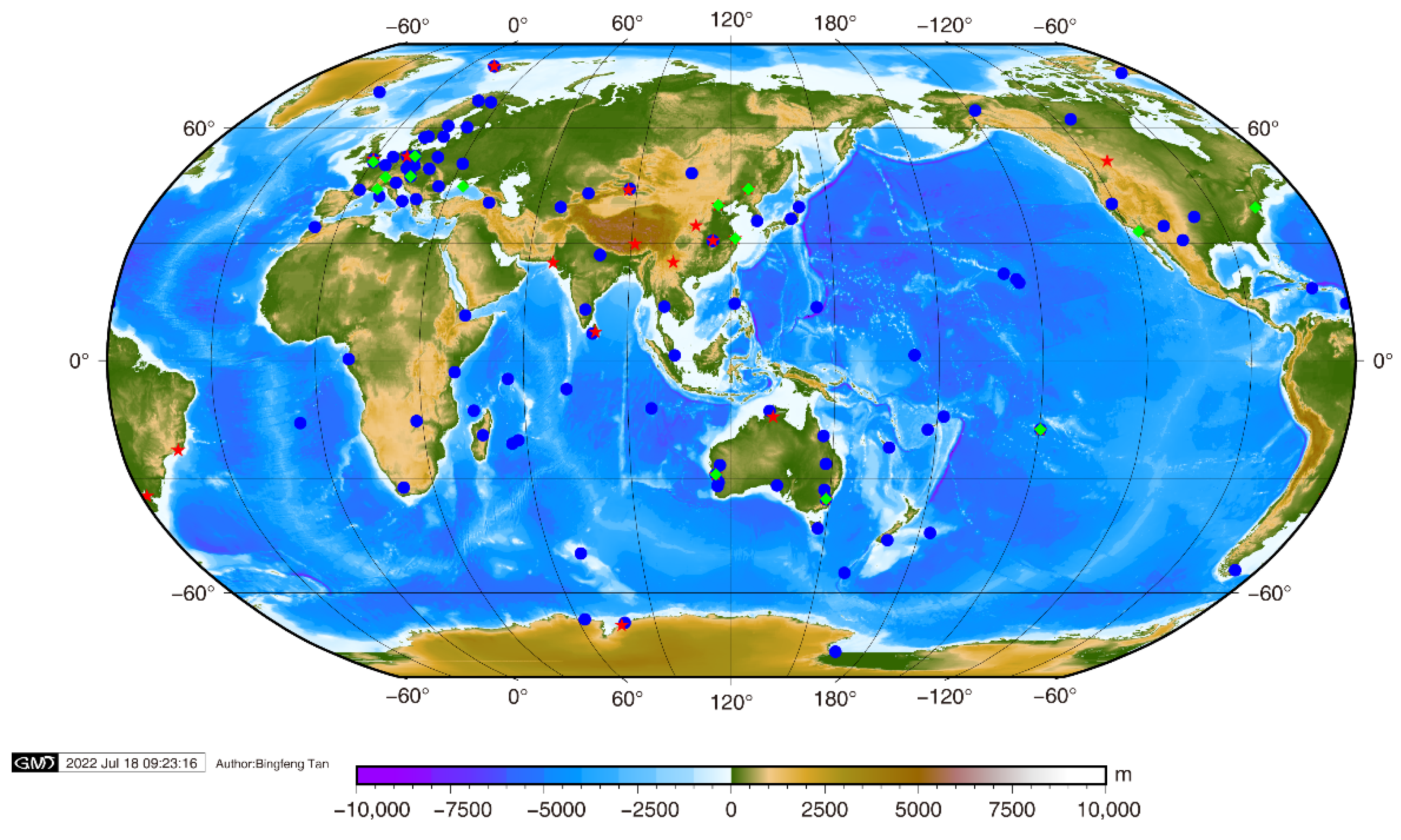
2.1. GNSS Tracking Networks
2.1.1. iGMAS Tracking Network
2.1.2. IGS Tracking Network
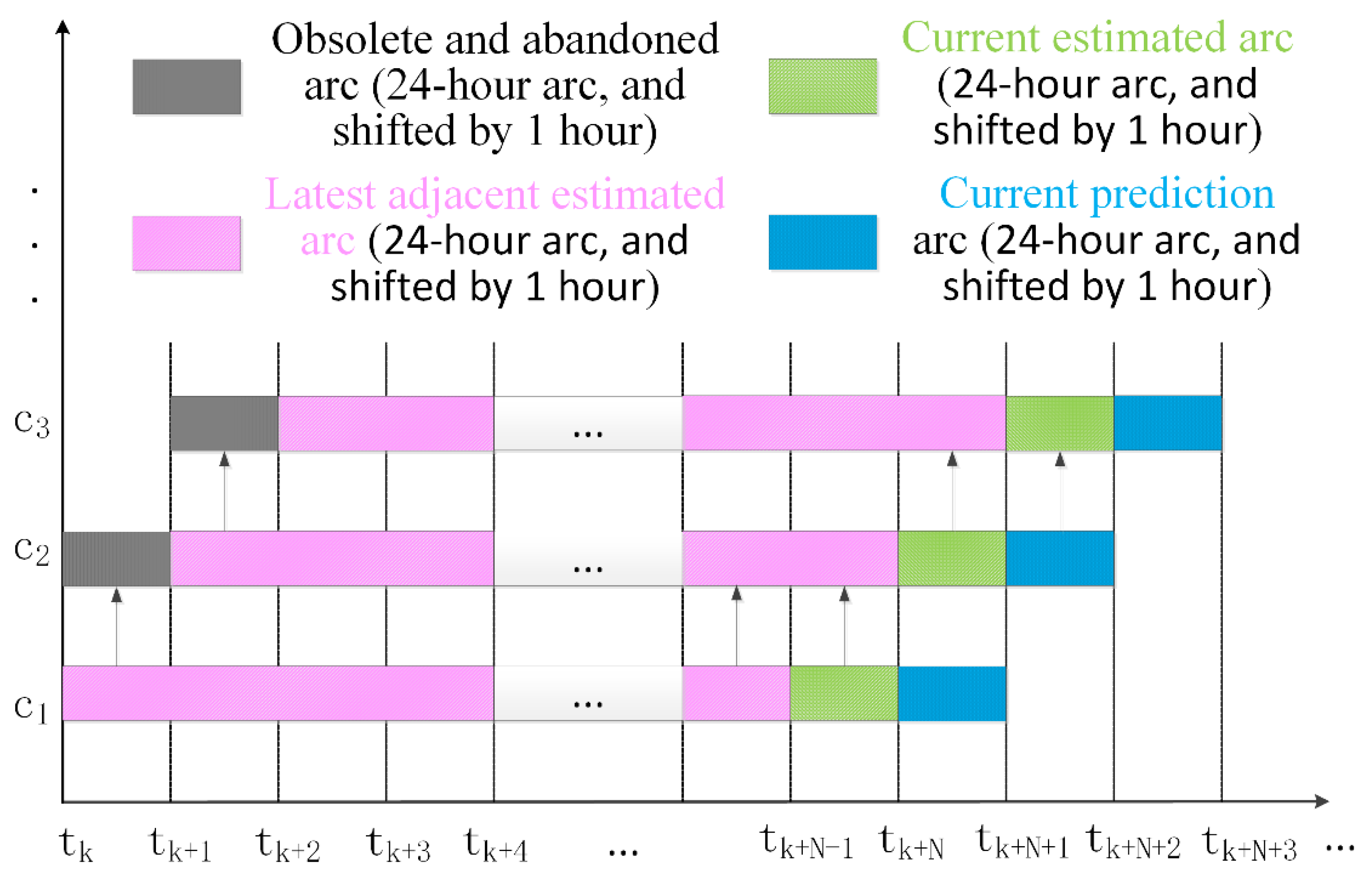
2.2. Hourly Updated Ultrarapid POD Strategy
3. Results
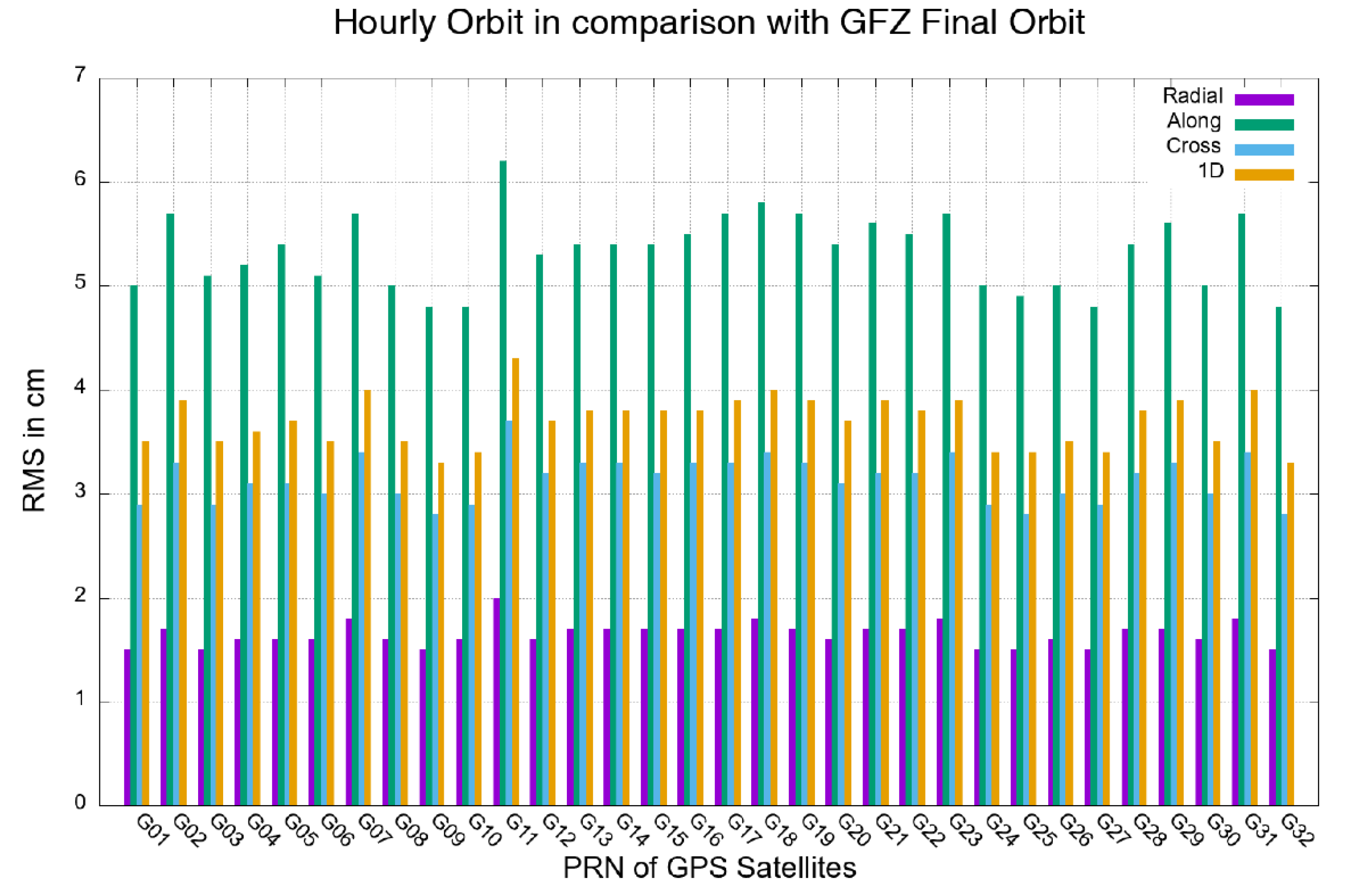
3.1. Orbit Comparisons with GFZ Final Multi-GNSS Orbits
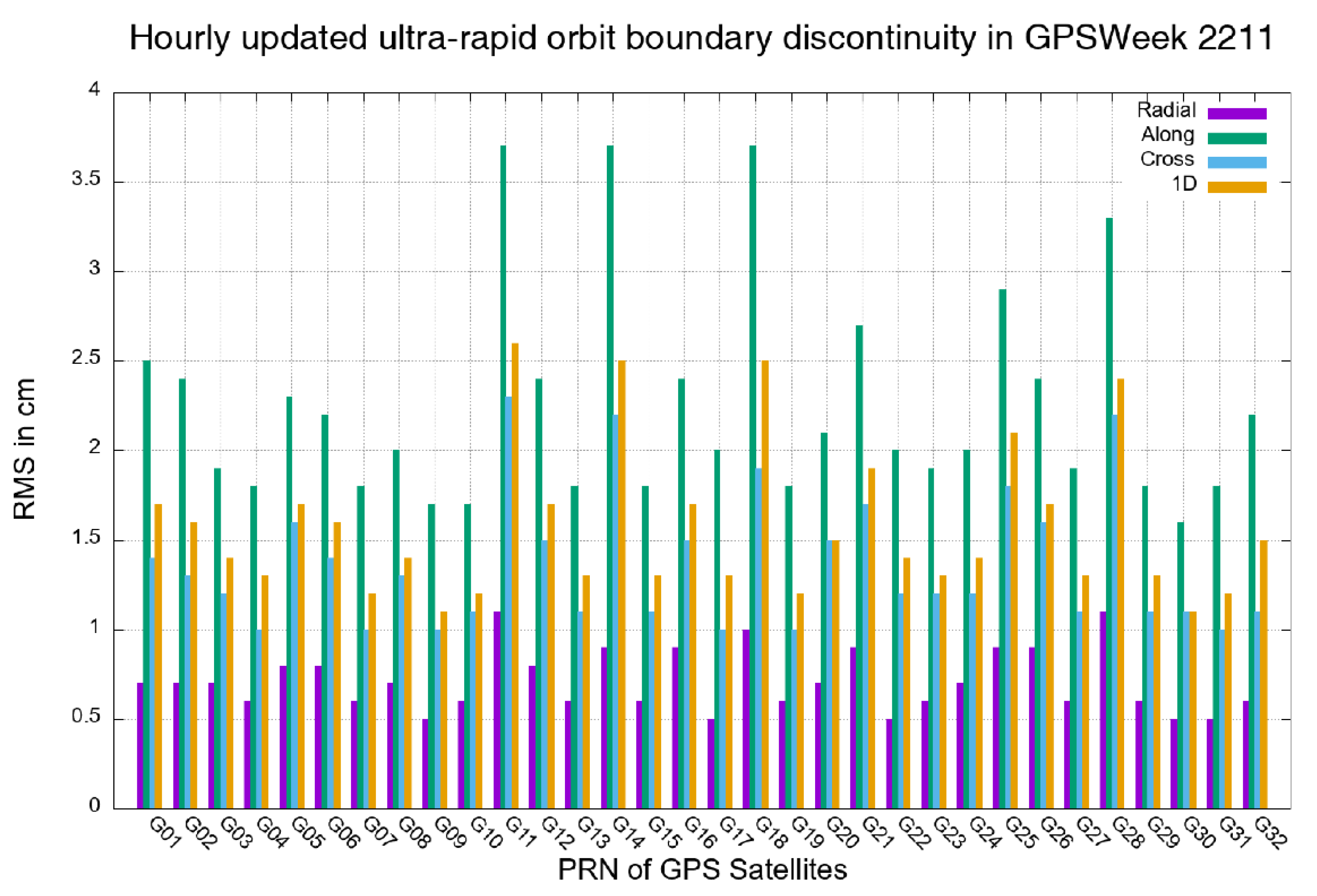
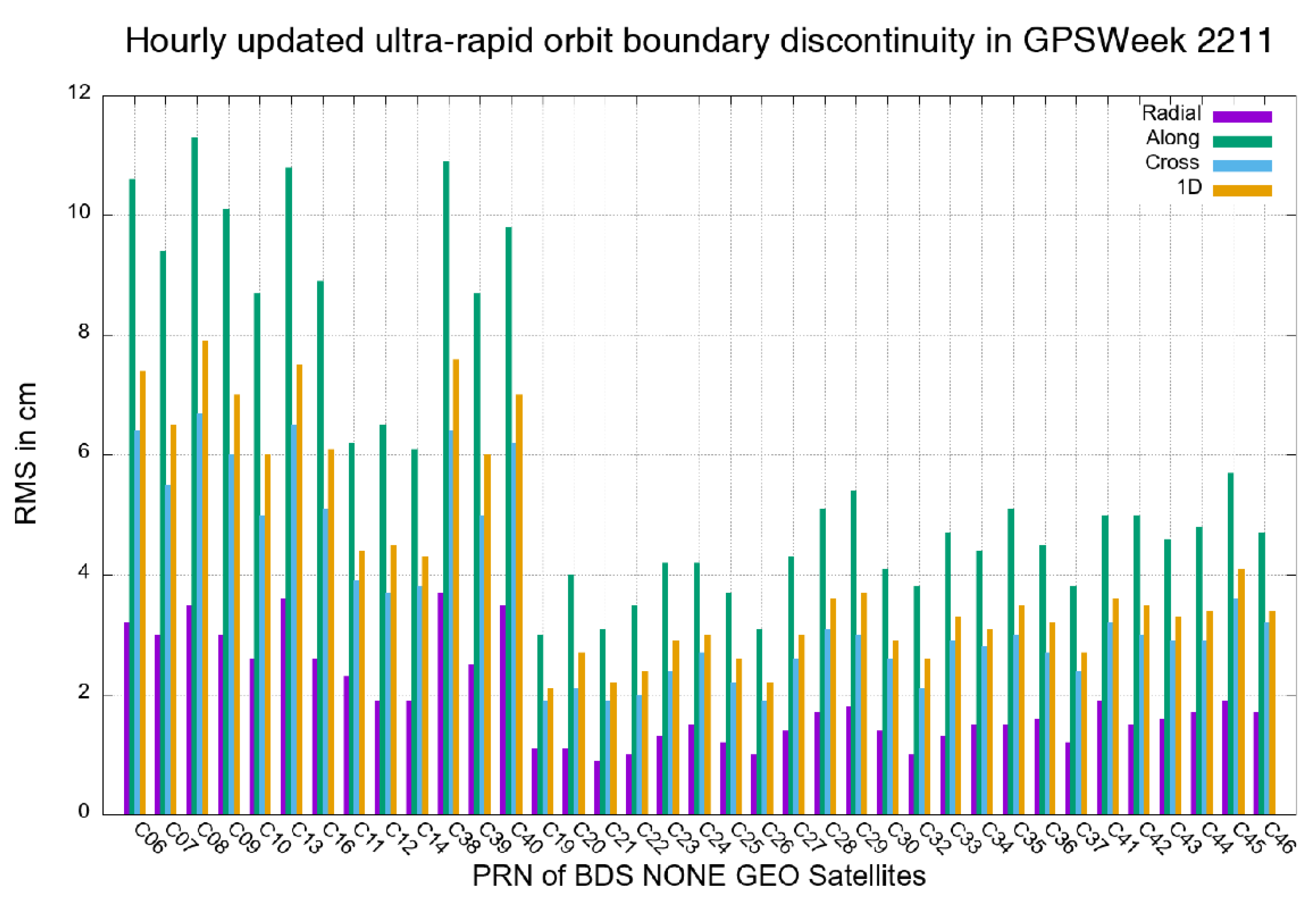
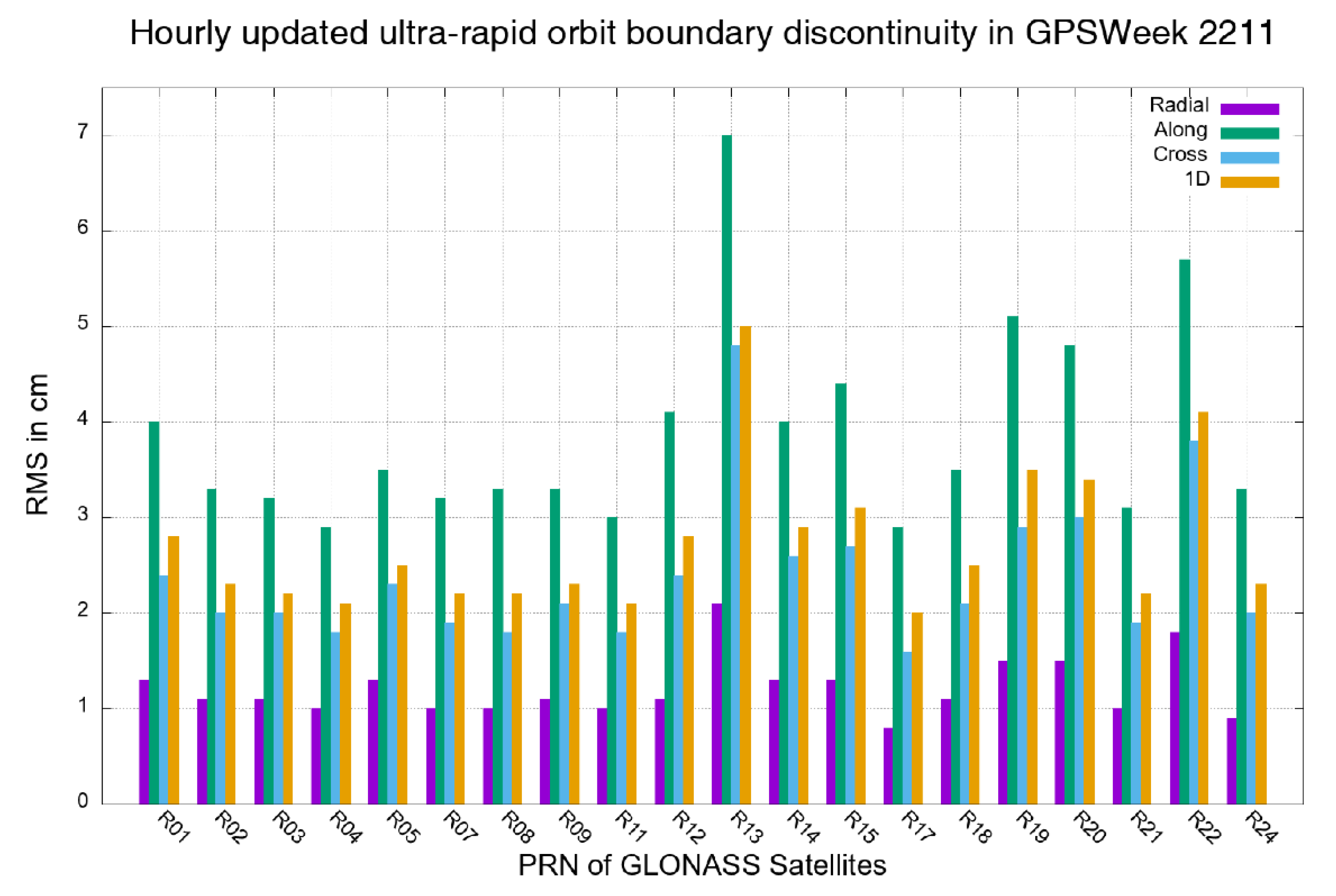
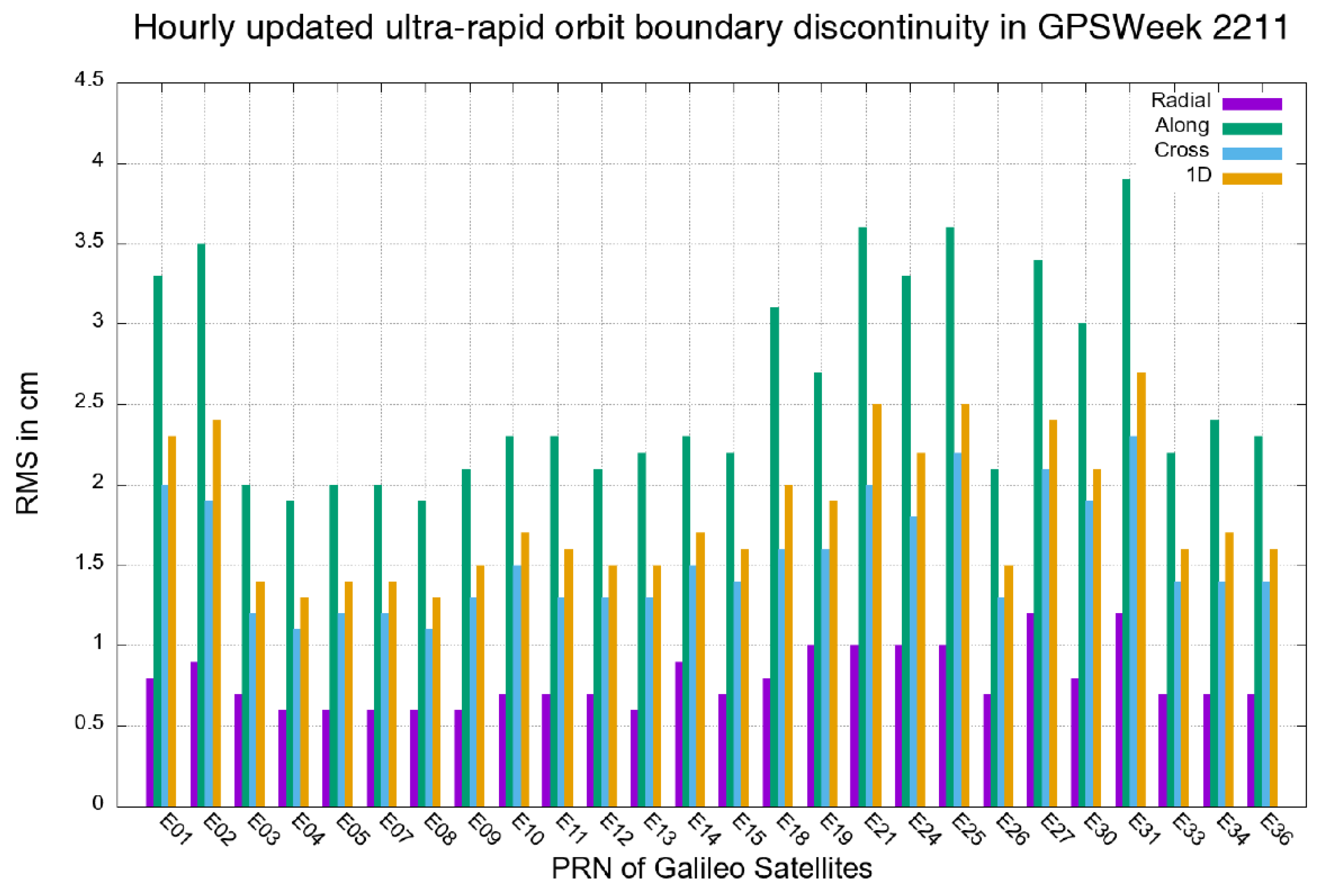
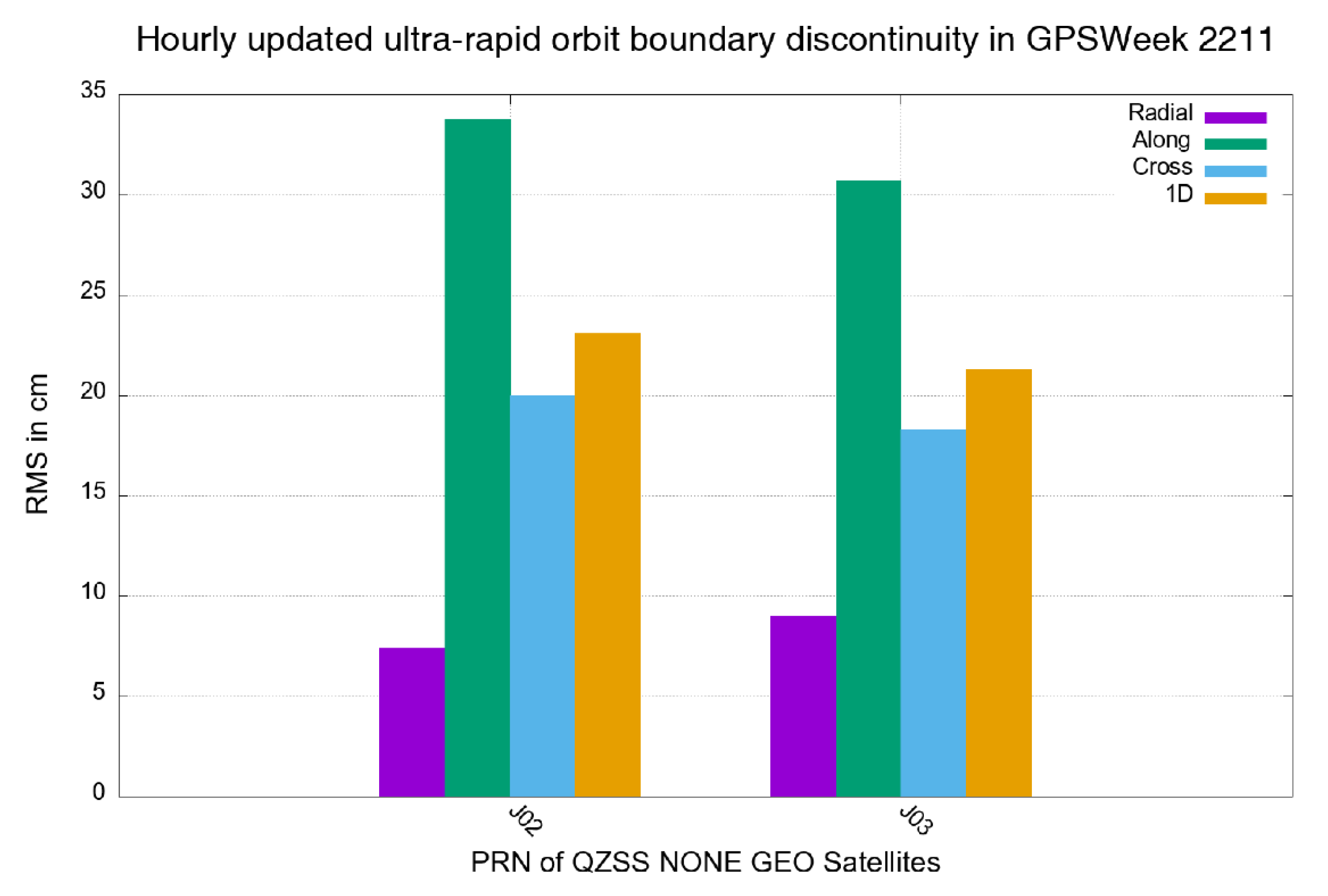
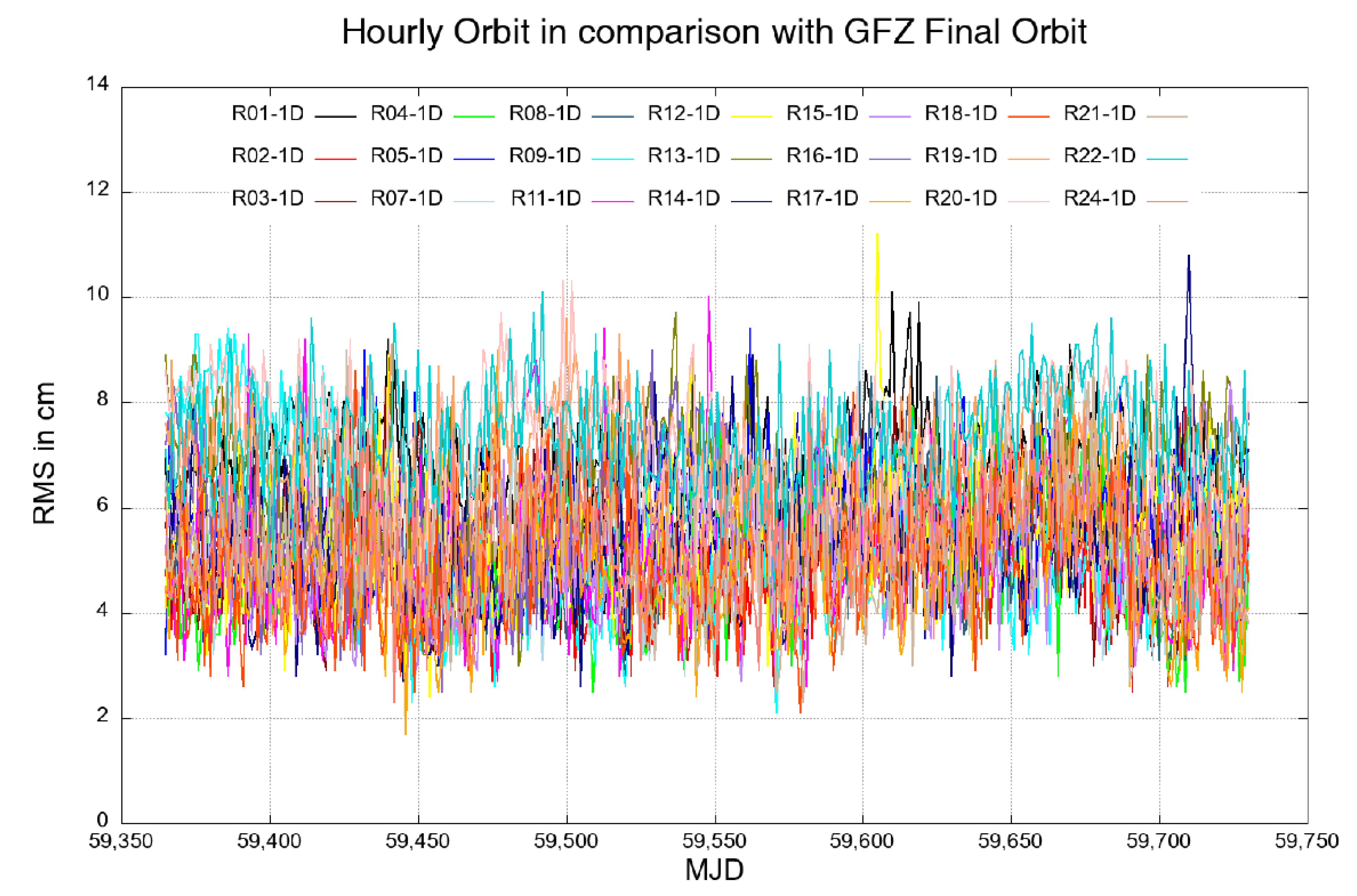
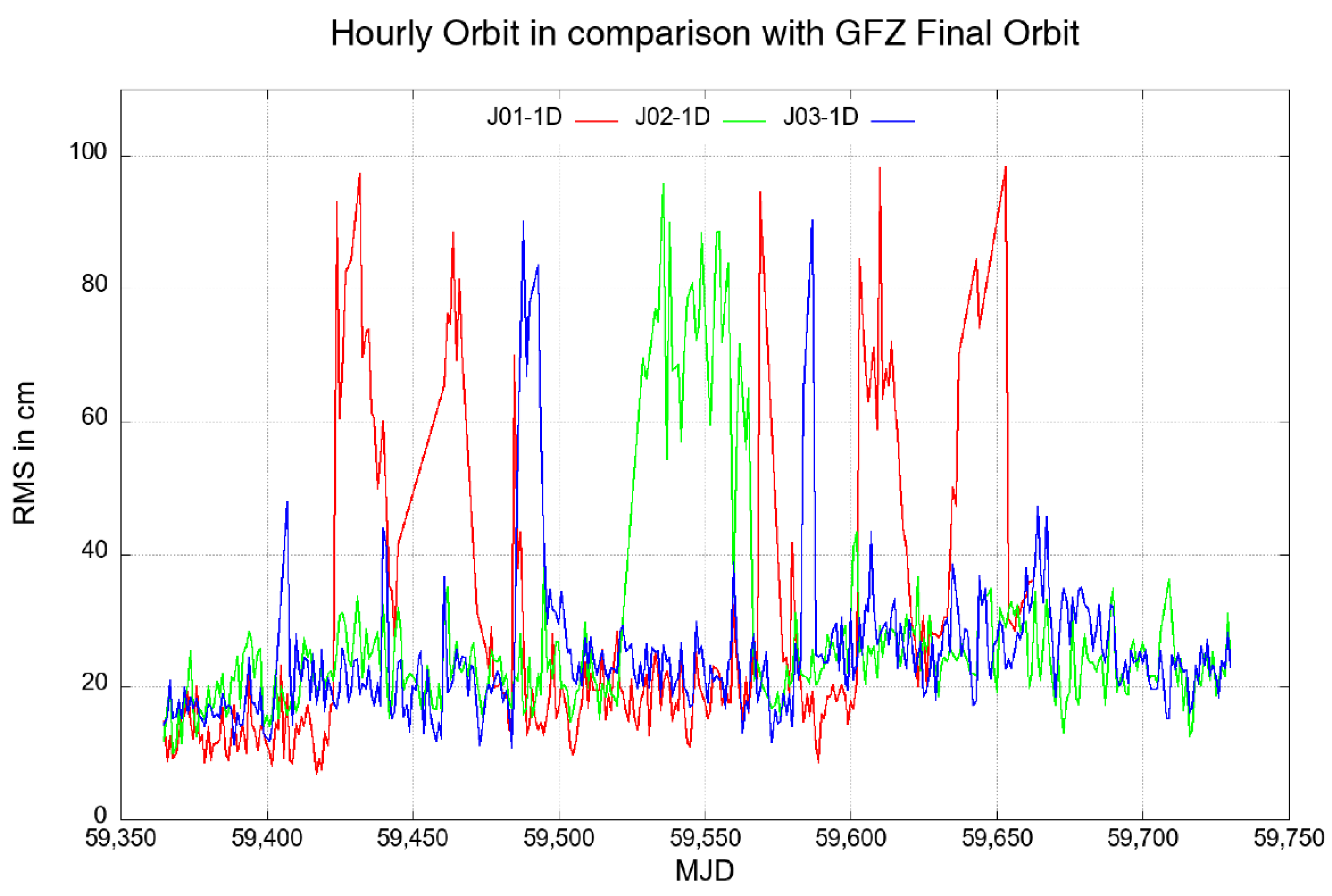
3.2. Hourly Orbit Boundary Discontinuities
3.3. SLR Residuals
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yang, Y.; Mao, Y.; Sun, B. Basic performance and future developments of BeiDou global navigation satellite system. Satell. Navig. 2020, 1, 1. [Google Scholar] [CrossRef]
- Yang, Y.; Xu, Y.; Li, J.; Yang, C. Progress and performance evaluation of BeiDou global navigation satellite system: Data analysis based on BDS-3 demonstration system. Sci. China Earth Sci. 2018, 61, 614–624. [Google Scholar] [CrossRef]
- Guo, J. The Impacts of Attitude, Solar Radiation and Function Model on Precise Orbit Determination for GNSS Satellites. Ph.D. Thesis, Wuhan University, Wuhan, China, 2014. [Google Scholar]
- Prange, L.; Orliac, E.; Dach, R.; Arnold, D.; Beutler, G.; Schaer, S.; Jäggi, A. CODE’s five-system orbit and clock solution—the challenges of multi-GNSS data analysis. J. Geod. 2017, 91, 345–360. [Google Scholar] [CrossRef]
- Prange, L.; Villiger, A.; Sidorov, D.; Schaer, S.; Beutler, G.; Dach, R.; Jäggi, A. Overview of CODE’s MGEX solution with the focus on Galileo. Adv. Space Res. 2020, 66, 2786–2798. [Google Scholar] [CrossRef]
- Zhang, B.; Chen, Y.; Yuan, Y. PPP-RTK based on undifferenced and uncombined observations: Theoretical and practical aspects. J. Geod. 2019, 93, 1011–1024. [Google Scholar] [CrossRef]
- Liu, T.; Zhang, B.; Yuan, Y.; Li, M. Real-Time Precise Point Positioning (RTPPP) with raw observations and its application in real-time regional ionospheric VTEC modeling. J. Geod. 2018, 92, 1267–1283. [Google Scholar] [CrossRef]
- Zha, J.; Zhang, B.; Liu, T.; Hou, P. Ionosphere-weighted undifferenced and uncombined PPP-RTK: Theoretical models and experimental results. GPS Solut. 2021, 25, 135. [Google Scholar] [CrossRef]
- Ai, Q.; Zhang, B.; Yuan, Y.; Xu, T.; Chen, Y.; Tan, B. Evaluation and mitigation of the influence of pseudorange biases on GNSS satellite clock offset estimation. Measurement 2022, 193, 111015. [Google Scholar] [CrossRef]
- Ai, Q.; Yuan, Y.; Zhang, B.; Xu, T.; Chen, Y. Refining GPS/GLONASS Satellite Clock Offset Estimation in the Presence of Pseudo-Range Inter-Channel Biases. Remote Sens. 2020, 12, 1821. [Google Scholar] [CrossRef]
- Zhang, B.; Teunissen, P.J. Characterization of multi-GNSS between-receiver differential code biases using zero and short baselines. Sci. Bull. 2015, 60, 1840–1849. [Google Scholar] [CrossRef] [Green Version]
- Zhang, B.; Teunissen, P.J.; Odijk, D. A novel un-differenced PPP-RTK concept. J. Navig. 2011, 64, S180–S191. [Google Scholar] [CrossRef]
- Bertiger, W.; Bar-Sever, Y.; Dorsey, A.; Haines, B.; Harvey, N.; Hemberger, D.; Heflin, M.; Lu, W.; Miller, M.; Moore, A.W. GipsyX/RTGx, a new tool set for space geodetic operations and research. Adv. Space Res. 2020, 66, 469–489. [Google Scholar] [CrossRef]
- Zhang, Q.; Moore, P.; Hanley, J.; Martin, S. Auto-BAHN: Software for near real-time GPS orbit and clock computations. Adv. Space Res. 2007, 39, 1531–1538. [Google Scholar] [CrossRef]
- Kazmierski, K.; Sośnica, K.; Hadas, T. Quality assessment of multi-GNSS orbits and clocks for real-time precise point positioning. Gps Solut. 2018, 22, 11. [Google Scholar] [CrossRef]
- Zhang, S.; Du, S.; Li, W.; Wang, G. Evaluation of the GPS precise orbit and clock corrections from MADOCA real-time products. Sensors 2019, 19, 2580. [Google Scholar] [CrossRef]
- Dai, X.; Lou, Y.; Dai, Z.; Qing, Y.; Li, M.; Shi, C. Real-time precise orbit determination for BDS satellites using the square root information filter. GPS Solut. 2019, 23, 45. [Google Scholar] [CrossRef]
- Duan, B.; Hugentobler, U.; Chen, J.; Selmke, I.; Wang, J. Prediction versus real-time orbit determination for GNSS satellites. GPS Solut. 2019, 23, 39. [Google Scholar] [CrossRef]
- Montenbruck, O.; Steigenberger, P.; Prange, L.; Deng, Z.; Zhao, Q.; Perosanz, F.; Romero, I.; Noll, C.; Stürze, A.; Weber, G. The Multi-GNSS Experiment (MGEX) of the International GNSS Service (IGS)–achievements, prospects and challenges. Adv. Space Res. 2017, 59, 1671–1697. [Google Scholar] [CrossRef]
- Jiao, W. International GNSS Monitoring and Assessment System (iGMAS) and latest progress. In Proceedings of the China Satellite Navigation Conference (CSNC), Nanjing, China, 21–23 May 2014. [Google Scholar]
- Deng, Z.; Fritsche, M.; Nischan, T.; Bradke, M. Multi-GNSS Ultra Rapid Orbit-, Clock-& EOP Product Series, GFZ Data Services; Technical Report; 2016. [Google Scholar]
- Guo, J.; Xu, X.; Zhao, Q.; Liu, J. Precise orbit determination for quad-constellation satellites at Wuhan University: Strategy, result validation, and comparison. J. Geod. 2016, 90, 143–159. [Google Scholar] [CrossRef]
- Kuang, K.; Zhang, S.; Li, J. Real-time GPS satellite orbit and clock estimation based on OpenMP. Adv. Space Res. 2019, 63, 2378–2386. [Google Scholar] [CrossRef]
- Li, X.; Ge, M.; Dai, X.; Ren, X.; Fritsche, M.; Wickert, J.; Schuh, H. Accuracy and reliability of multi-GNSS real-time precise positioning: GPS, GLONASS, BeiDou, and Galileo. J. Geod. 2015, 89, 607–635. [Google Scholar] [CrossRef]
- Shi, C.; Zhao, Q.; Li, M.; Tang, W.; Hu, Z.; Lou, Y.; Zhang, H.; Niu, X.; Liu, J. Precise orbit determination of Beidou Satellites with precise positioning. Sci. China Earth Sci. 2012, 55, 1079–1086. [Google Scholar] [CrossRef]
- Li, X.; Chen, X.; Ge, M.; Schuh, H. Improving multi-GNSS ultra-rapid orbit determination for real-time precise point positioning. J. Geod. 2019, 93, 45–64. [Google Scholar] [CrossRef]
- Choi, K.K.; Ray, J.; Griffiths, J.; Bae, T.-S. Evaluation of GPS orbit prediction strategies for the IGS Ultra-rapid products. GPS Solut. 2013, 17, 403–412. [Google Scholar] [CrossRef]
- Hadas, T.; Bosy, J. IGS RTS precise orbits and clocks verification and quality degradation over time. GPS Solut. 2015, 19, 93–105. [Google Scholar] [CrossRef]
- Rülke, A.; Agrotis, L.; Enderle, W.; MacLeod, K. IGS real time service–status, future tasks and limitations. In Proceedings of the IGS Workshop, Sydney, Australia, 8–12 February 2016; Federal Agency for Cartography and Geodesy: Frankfurt, Germany, 2016. [Google Scholar]
- Montenbruck, O.; Schmid, R.; Mercier, F.; Steigenberger, P.; Noll, C.; Fatkulin, R.; Kogure, S.; Ganeshan, A.S. GNSS satellite geometry and attitude models. Adv. Space Res. 2015, 56, 1015–1029. [Google Scholar] [CrossRef]
- Petit, G.; Luzum, B. IERS Conventions; Verlag des Bundesamts für Kartographie und Geodäsie: Frankfurt, Germany, 2010; ISBN 3-89888-989-6. [Google Scholar]
- Song, W.; Li, C.; Dai, X.; Lou, Y.; Xu, Y. BDS near real-time maneuver detection based on triple-differenced phase observations. Adv. Space Res. 2022, 69, 3032–3043. [Google Scholar] [CrossRef]
- Ye, F.; Yuan, Y.; Tan, B.; Ou, J. A robust method to detect BeiDou navigation satellite system orbit maneuvering/anomalies and its applications to precise orbit determination. Sensors 2017, 17, 1129. [Google Scholar] [CrossRef]
- Beutler, G.; Brockmann, E.; Gurtner, W.; Hugentobler, U.; Mervart, L.; Rothacher, M.; Verdun, A. Extended orbit modeling techniques at the CODE processing center of the international GPS service for geodynamics (IGS): Theory and initial results. Manuscr. Geod. 1994, 19, 367–386. [Google Scholar]
- Arnold, D.; Meindl, M.; Beutler, G.; Dach, R.; Schaer, S.; Lutz, S.; Prange, L.; Sośnica, K.; Mervart, L.; Jäggi, A. CODE’s new solar radiation pressure model for GNSS orbit determination. J. Geod. 2015, 89, 775–791. [Google Scholar] [CrossRef]
- Montenbruck, O.; Steigenberger, P.; Hugentobler, U. Enhanced solar radiation pressure modeling for Galileo satellites. J. Geod. 2015, 89, 283–297. [Google Scholar] [CrossRef]
- Sidorov, D.; Dach, R.; Polle, B.; Prange, L.; Jäggi, A. Adopting the empirical CODE orbit model to Galileo satellites. Adv. Space Res. 2020, 66, 2799–2811. [Google Scholar] [CrossRef]
- Wang, C. Solar Radiation Pressure Modelling for BeiDou Navigation Satellites. Ph.D. Thesis, Wuhan University, Wuhan, China, 2019. [Google Scholar]
- Liu, Y.; Liu, Y.; Tian, Z.; Dai, X.; Qing, Y.; Li, M. Impact of ECOM Solar Radiation Pressure Models on Multi-GNSS Ultra-Rapid Orbit Determination. Remote Sens. 2019, 11, 3024. [Google Scholar] [CrossRef]
- Xia, F.; Ye, S.; Chen, D.; Tang, L.; Wang, C.; Ge, M.; Neitzel, F. Advancing the Solar Radiation Pressure Model for BeiDou-3 IGSO Satellites. Remote Sens. 2022, 14, 1460. [Google Scholar] [CrossRef]
- Rodriguez-Solano, C.; Hugentobler, U.; Steigenberger, P. Adjustable box-wing model for solar radiation pressure impacting GPS satellites. Adv. Space Res. 2012, 49, 1113–1128. [Google Scholar] [CrossRef]
- China Satellite Navigation Office. BeiDou Satellite Metadata. Available online: http://en.beidou.gov.cn/SYSTEMS/Officialdocument/201912/P020200323536298695483.zip (accessed on 8 March 2021).
- Pearlman, M.R.; Degnan, J.J.; Bosworth, J.M. The international laser ranging service. Adv. Space Res. 2002, 30, 135. [Google Scholar] [CrossRef]









| System | Type | Signals | Sats |
|---|---|---|---|
| GPS | Block IIR | L1 C/A, L1/L2 P(Y) | 8 |
| Block IIR-M | L1 C/A, L1/L2 P(Y), L2C, L1/L2 M | 7 | |
| Block IIF | L1 C/A, L1/L2 P(Y), L1/L2 M, L2C, L5 | 12 | |
| Block IIIA | L1 C/A, L1/L2 P(Y), L1/L2 M, L2C, L5 | 5 | |
| GLONASS | GLONASS-M | L1/L2 C/A and P, L3, FDMA | 18 |
| GLONASS-K1 | L1/L2 C/A and P, L3, FDMA+CDMA | 3 | |
| BDS-2 | GEO | B1I, B2I, B3I | 5 |
| IGSO | 7 | ||
| MEO | 3 | ||
| BDS-3 | GEO | B1C, B2a, B2b, B3C, B1I, B3I | 3 |
| IGSO | 3 | ||
| MEO | 24 | ||
| Galileo | IOV | E1, (E6), E5a/b/ab | 4 |
| FOC | 24 | ||
| QZSS | GEO | L1 C/A (L1 C/B), L1-SAIF, L1C, L2C, L5, L6-LEX | 1 |
| IGSO | 4 |
| Center | Description | Mountpoint |
|---|---|---|
| IGS01 | GPS-only orbit and clock corrections based on IGS ultrarapid orbits; Single-Epoch GPS clock combination, RETINA solution | SSRA01IGS0 |
| IGS02 | GPS-only orbit and clock corrections based on IGS ultrarapid orbits; Kalman Filter GPS clock combination, BNC solution | SSRA02IGS0 |
| IGS03 | GPS+GLONASS orbit and clock corrections based on IGS ultrarapid orbits; Kalman Filter GPS+GLONASS clock combination, BNC solution | SSRA03IGS0 |
| BKG | GPS+GLONASS orbit and clock corrections based on IGS ultrarapid orbits | SSRA00BKG0 |
| CNES | GPS+GLONASS orbit and clock corrections based on IGS ultrarapid orbits | SSRA00CNE0 |
| CAS | GPS+GLONASS orbit and clock corrections based on internal ultrarapid orbits | SSRA00CAS0 |
| DLR/GSOC | GPS+GLONASS orbit and clock corrections based on IGS ultrarapid orbits | SSRA00DLR0 |
| ESA/ESOC | GPS-only orbit and clock corrections based on IGS ultrarapid orbits | SSRA00ESA0 |
| GPS-only orbit and clock corrections based on internal ultrarapid orbits | SSRA01ESA0 | |
| GFZ | GPS+GLONASS orbit and clock corrections based on IGS ultrarapid orbits | SSRA00GFZ0 |
| GMV | GPS+GLONASS orbit and clock corrections based on internal ultrarapid orbits | SSRA00GMV0 |
| NRCan | GPS-only orbit and clock corrections using NRT batch orbits every hour (APC) | SSRA00NRC0 |
| SHAO | GPS+GLONASS orbit and clock corrections based on internal ultrarapid orbits | SSRA00SHA0 |
| WUHAN | GPS+GLONASS orbit and clock corrections based on IGS ultrarapid orbits | SSRA00WHU0 |
| Aspect | Summary |
|---|---|
| Observations | Double-differenced ionosphere-free phase. GPS/GLONASS/QZSS: L1, L2; BDS: B1I, B3I; Galileo: E1, E5a |
| Elevation angle cut-off | 7° |
| Stations | 21 iGMAS stations and 100 IGS/MGEX stations |
| Sampling rate | 300 s |
| Data coverage | A length of 72 h arc combined through three adjacent 24 h arc normal equations |
| Orbits | Initial positions and velocities from broadcast ephemeris |
| Weighting | 6 mm for phase observationswith elevation-dependent weighting |
| Satellite antenna PCO and PCV | Satellite antenna PCOs and PCVs of GPS/GLONASS/QZSS L1/L2, Galileo E1/E5a and BDS B1I/B3I from igs14_2196.atx. For BDS and Galileo receiver antenna, the values of GPS L1/L2 have been used for the BDS B1I/B3I and Galileo E1/E5a, as their calibrations are unavailable in the igs14.atx [30] |
| Attitude model | GPS/GLONASS/Galileo/BDS-3: Yaw steering BDS-2/QZSS: Yaw steering + orbit normal |
| Troposphere | GMF mapping functions; ZTDs estimated at intervals of 2 h |
| Precession and nutation | IAU 2010 model |
| Geopotential | EGM2008 12 × 12 |
| Solid Earth tides, ocean tides and solid Earth pole tides | IERS Conventions 2010 [31] |
| N body gravitation | DE405 ephemeris from JPL |
| Solar Radiation Pressure (SRP) model | GPS/GLONASS/QZSS: seven-parameter ECOM2 (D1B1) BDS-2: five-parameter ECOM1 BDS-3/Galileo: cuboid a priori plus five-parameter ECOM1 |
| Pseudo-stochastic orbit parameters | Every half day; constrained to 1 × 10−6 m/s in the radial, 1 × 10−5 m/s in the along-track and 1 × 10−8 m/s in the cross-track direction |
| Ambiguity | Fixed for GPS, GLONASS, Galileo and BDS NONE GEO satellites |
| Satellite Maneuver | GPS/GLONASS/Galileo maneuver information from GFZ (https://semisys.gfz-potsdam.de/semisys/scripts/satellites/maneuver.php) (accessed on 25 July 2022) BDS/QZSS detected in real-time mode [32,33] |
| Ultrarapid orbit update interval | Hourly updated based on five-system POD processing within one hour |
| PRN of GPS | Radial (cm) | Along (cm) | Cross (cm) | 1-D (cm) |
|---|---|---|---|---|
| G01 | 1.5 | 5.0 | 2.9 | 3.5 |
| G02 | 1.7 | 5.7 | 3.3 | 3.9 |
| G03 | 1.5 | 5.1 | 2.9 | 3.5 |
| G04 | 1.6 | 5.2 | 3.1 | 3.6 |
| G05 | 1.6 | 5.4 | 3.1 | 3.7 |
| G06 | 1.6 | 5.1 | 3.0 | 3.5 |
| G07 | 1.8 | 5.7 | 3.4 | 4.0 |
| G08 | 1.6 | 5.0 | 3.0 | 3.5 |
| G09 | 1.5 | 4.8 | 2.8 | 3.3 |
| G10 | 1.6 | 4.8 | 2.9 | 3.4 |
| G11 | 2.0 | 6.2 | 3.7 | 4.3 |
| G12 | 1.6 | 5.3 | 3.2 | 3.7 |
| G13 | 1.7 | 5.4 | 3.3 | 3.8 |
| G14 | 1.7 | 5.4 | 3.3 | 3.8 |
| G15 | 1.7 | 5.4 | 3.2 | 3.8 |
| G16 | 1.7 | 5.5 | 3.3 | 3.8 |
| G17 | 1.7 | 5.7 | 3.3 | 3.9 |
| G18 | 1.8 | 5.8 | 3.4 | 4.0 |
| G19 | 1.7 | 5.7 | 3.3 | 3.9 |
| G20 | 1.6 | 5.4 | 3.1 | 3.7 |
| G21 | 1.7 | 5.6 | 3.2 | 3.9 |
| G22 | 1.7 | 5.5 | 3.2 | 3.8 |
| G23 | 1.8 | 5.7 | 3.4 | 3.9 |
| G24 | 1.5 | 5.0 | 2.9 | 3.4 |
| G25 | 1.5 | 4.9 | 2.8 | 3.4 |
| G26 | 1.6 | 5.0 | 3.0 | 3.5 |
| G27 | 1.5 | 4.8 | 2.9 | 3.4 |
| G28 | 1.7 | 5.4 | 3.2 | 3.8 |
| G29 | 1.7 | 5.6 | 3.3 | 3.9 |
| G30 | 1.6 | 5.0 | 3.0 | 3.5 |
| G31 | 1.8 | 5.7 | 3.4 | 4.0 |
| G32 | 1.5 | 4.8 | 2.8 | 3.3 |
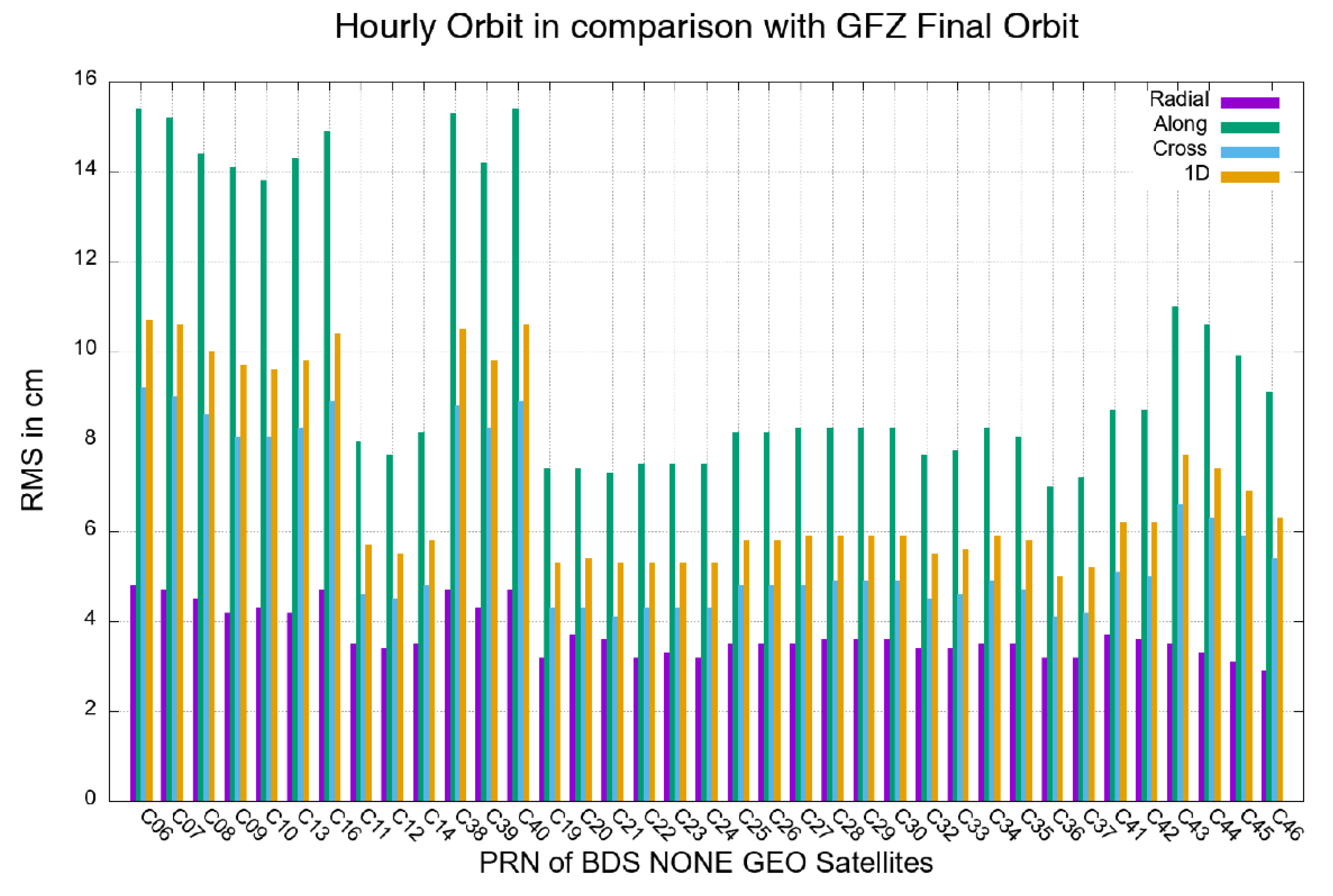
| PRN of BDS NONE GEOs | Radial (cm) | Along (cm) | Cross (cm) | 1-D (cm) |
|---|---|---|---|---|
| BDS-2 IGSOs | ||||
| C06 | 4.8 | 15.4 | 9.2 | 10.7 |
| C07 | 4.7 | 15.2 | 9.0 | 10.6 |
| C08 | 4.5 | 14.4 | 8.6 | 10.0 |
| C09 | 4.2 | 14.1 | 8.1 | 9.7 |
| C10 | 4.3 | 13.8 | 8.1 | 9.6 |
| C13 | 4.2 | 14.3 | 8.3 | 9.8 |
| C16 | 4.7 | 14.9 | 8.9 | 10.4 |
| BDS-2 MEOs | ||||
| C11 | 3.5 | 8.0 | 4.6 | 5.7 |
| C12 | 3.4 | 7.7 | 4.5 | 5.5 |
| C14 | 3.5 | 8.2 | 4.8 | 5.8 |
| BDS-3 IGSOs | ||||
| C38 | 4.7 | 15.3 | 8.8 | 10.5 |
| C39 | 4.3 | 14.2 | 8.3 | 9.8 |
| C40 | 4.7 | 15.4 | 8.9 | 10.6 |
| BDS-3 MEOs | ||||
| C19 | 3.2 | 7.4 | 4.3 | 5.3 |
| C20 | 3.7 | 7.4 | 4.3 | 5.4 |
| C21 | 3.6 | 7.3 | 4.1 | 5.3 |
| C22 | 3.2 | 7.5 | 4.3 | 5.3 |
| C23 | 3.3 | 7.5 | 4.3 | 5.3 |
| C24 | 3.2 | 7.5 | 4.3 | 5.3 |
| C25 | 3.5 | 8.2 | 4.8 | 5.8 |
| C26 | 3.5 | 8.2 | 4.8 | 5.8 |
| C27 | 3.5 | 8.3 | 4.8 | 5.9 |
| C28 | 3.6 | 8.3 | 4.9 | 5.9 |
| C29 | 3.6 | 8.3 | 4.9 | 5.9 |
| C30 | 3.6 | 8.3 | 4.9 | 5.9 |
| C32 | 3.4 | 7.7 | 4.5 | 5.5 |
| C33 | 3.4 | 7.8 | 4.6 | 5.6 |
| C34 | 3.5 | 8.3 | 4.9 | 5.9 |
| C35 | 3.5 | 8.1 | 4.7 | 5.8 |
| C36 | 3.2 | 7.0 | 4.1 | 5.0 |
| C37 | 3.2 | 7.2 | 4.2 | 5.2 |
| C41 | 3.7 | 8.7 | 5.1 | 6.2 |
| C42 | 3.6 | 8.7 | 5.0 | 6.2 |
| C43 | 3.5 | 11.0 | 6.6 | 7.7 |
| C44 | 3.3 | 10.6 | 6.3 | 7.4 |
| C45 | 3.1 | 9.9 | 5.9 | 6.9 |
| C46 | 2.9 | 9.1 | 5.4 | 6.3 |
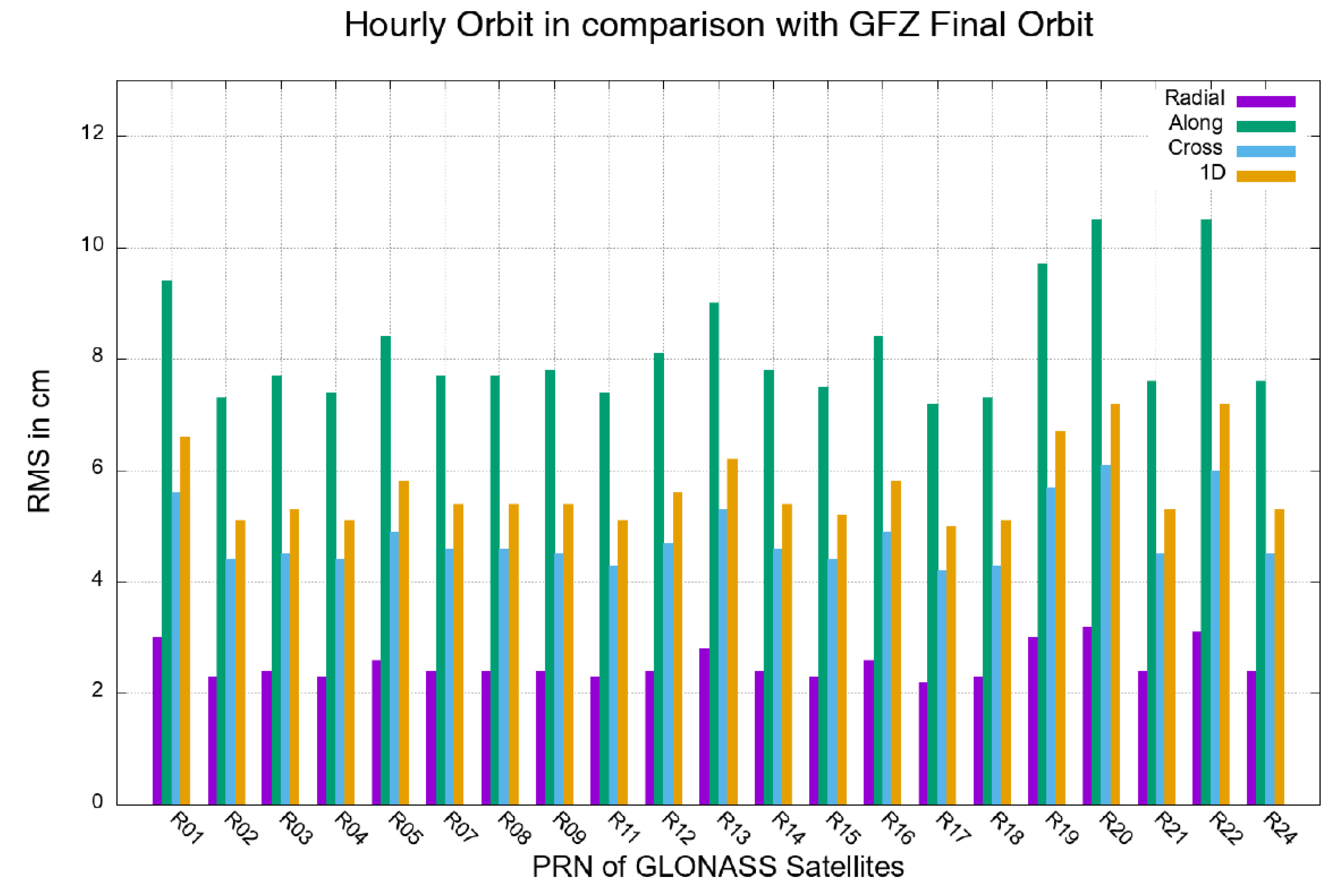
| PRN of GLONASS | Radial (cm) | Along (cm) | Cross (cm) | 1-D (cm) |
|---|---|---|---|---|
| R01 | 3.0 | 9.4 | 5.6 | 6.6 |
| R02 | 2.3 | 7.3 | 4.4 | 5.1 |
| R03 | 2.4 | 7.7 | 4.5 | 5.3 |
| R04 | 2.3 | 7.4 | 4.4 | 5.1 |
| R05 | 2.6 | 8.4 | 4.9 | 5.8 |
| R07 | 2.4 | 7.7 | 4.6 | 5.4 |
| R08 | 2.4 | 7.7 | 4.6 | 5.4 |
| R09 | 2.4 | 7.8 | 4.5 | 5.4 |
| R11 | 2.3 | 7.4 | 4.3 | 5.1 |
| R12 | 2.4 | 8.1 | 4.7 | 5.6 |
| R13 | 2.8 | 9.0 | 5.3 | 6.2 |
| R14 | 2.4 | 7.8 | 4.6 | 5.4 |
| R15 | 2.3 | 7.5 | 4.4 | 5.2 |
| R16 | 2.6 | 8.4 | 4.9 | 5.8 |
| R17 | 2.2 | 7.2 | 4.2 | 5.0 |
| R18 | 2.3 | 7.3 | 4.3 | 5.1 |
| R19 | 3.0 | 9.7 | 5.7 | 6.7 |
| R20 | 3.2 | 10.5 | 6.1 | 7.2 |
| R21 | 2.4 | 7.6 | 4.5 | 5.3 |
| R22 | 3.1 | 10.5 | 6.0 | 7.2 |
| R24 | 2.4 | 7.6 | 4.5 | 5.3 |
| PRN of Galileo | Radial (cm) | Along (cm) | Cross (cm) | 1-D (cm) |
|---|---|---|---|---|
| E01 | 1.8 | 5.9 | 3.4 | 4.0 |
| E02 | 1.7 | 5.8 | 3.3 | 4.0 |
| E03 | 1.8 | 5.6 | 3.3 | 3.9 |
| E04 | 1.7 | 5.5 | 3.2 | 3.8 |
| E05 | 1.7 | 5.5 | 3.2 | 3.8 |
| E07 | 1.8 | 5.6 | 3.3 | 3.9 |
| E08 | 1.7 | 5.5 | 3.3 | 3.8 |
| E09 | 1.7 | 5.6 | 3.3 | 3.9 |
| E10 | 2.3 | 7.2 | 4.3 | 5.0 |
| E11 | 1.8 | 5.9 | 3.5 | 4.1 |
| E12 | 1.9 | 6.0 | 3.5 | 4.1 |
| E13 | 1.8 | 5.9 | 3.4 | 4.1 |
| E14 | 2.0 | 6.4 | 3.8 | 4.5 |
| E15 | 1.8 | 6.0 | 3.5 | 4.1 |
| E18 | 2.0 | 6.3 | 3.7 | 4.4 |
| E19 | 1.8 | 5.7 | 3.3 | 3.9 |
| E21 | 1.7 | 5.8 | 3.3 | 4.0 |
| E24 | 1.7 | 5.8 | 3.3 | 4.0 |
| E25 | 1.8 | 5.8 | 3.4 | 4.0 |
| E26 | 1.8 | 6.0 | 3.5 | 4.1 |
| E27 | 1.8 | 5.8 | 3.4 | 4.0 |
| E30 | 1.8 | 5.9 | 3.4 | 4.1 |
| E31 | 1.7 | 5.7 | 3.3 | 4.0 |
| E33 | 1.8 | 6.0 | 3.5 | 4.1 |
| E34 | 2.1 | 6.9 | 4.0 | 4.7 |
| E36 | 1.8 | 6.0 | 3.5 | 4.1 |
| PRN of QZSS NONE GEOs | Radial (cm) | Along (cm) | Cross (cm) | 1-D (cm) |
|---|---|---|---|---|
| J01 | 12.1 | 37.9 | 22.9 | 26.5 |
| J02 | 10.9 | 35.5 | 20.6 | 24.5 |
| J03 | 9.6 | 35.7 | 20.6 | 24.4 |
| PRN of BDS-3 | AVE (cm) | RMS (cm) |
|---|---|---|
| M2, C20 (CAST) | 3.29 | 4.39 |
| M3, C21 (CAST) | 3.08 | 4.61 |
| M9, C29 (SECM) | −4.09 | 4.64 |
| M10, C30 (SECM) | −3.93 | 4.48 |
| PRN of BDS-2 | AVE (cm) | RMS (cm) |
| I3, C08 (IGSO) | 0.99 | 4.76 |
| I5, C10 (IGSO) | 1.95 | 4.73 |
| I6, C13 (IGSO) | 1.13 | 4.74 |
| M3, C11 (MEO) | 1.29 | 4.67 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tan, B.; Yuan, Y.; Ai, Q.; Zha, J. Real-Time Multi-GNSS Precise Orbit Determination Based on the Hourly Updated Ultra-Rapid Orbit Prediction Method. Remote Sens. 2022, 14, 4412. https://doi.org/10.3390/rs14174412
Tan B, Yuan Y, Ai Q, Zha J. Real-Time Multi-GNSS Precise Orbit Determination Based on the Hourly Updated Ultra-Rapid Orbit Prediction Method. Remote Sensing. 2022; 14(17):4412. https://doi.org/10.3390/rs14174412
Chicago/Turabian StyleTan, Bingfeng, Yunbin Yuan, Qingsong Ai, and Jiuping Zha. 2022. "Real-Time Multi-GNSS Precise Orbit Determination Based on the Hourly Updated Ultra-Rapid Orbit Prediction Method" Remote Sensing 14, no. 17: 4412. https://doi.org/10.3390/rs14174412
APA StyleTan, B., Yuan, Y., Ai, Q., & Zha, J. (2022). Real-Time Multi-GNSS Precise Orbit Determination Based on the Hourly Updated Ultra-Rapid Orbit Prediction Method. Remote Sensing, 14(17), 4412. https://doi.org/10.3390/rs14174412






