Relationship between Urban Three-Dimensional Spatial Structure and Population Distribution: A Case Study of Kunming’s Main Urban District, China
Abstract
:1. Introduction
2. Materials and Methods
2.1. Concept and Calculation Method of 3D Space-Filling Degree
2.2. Concept and Evaluation Methods of Urban Population Distribution
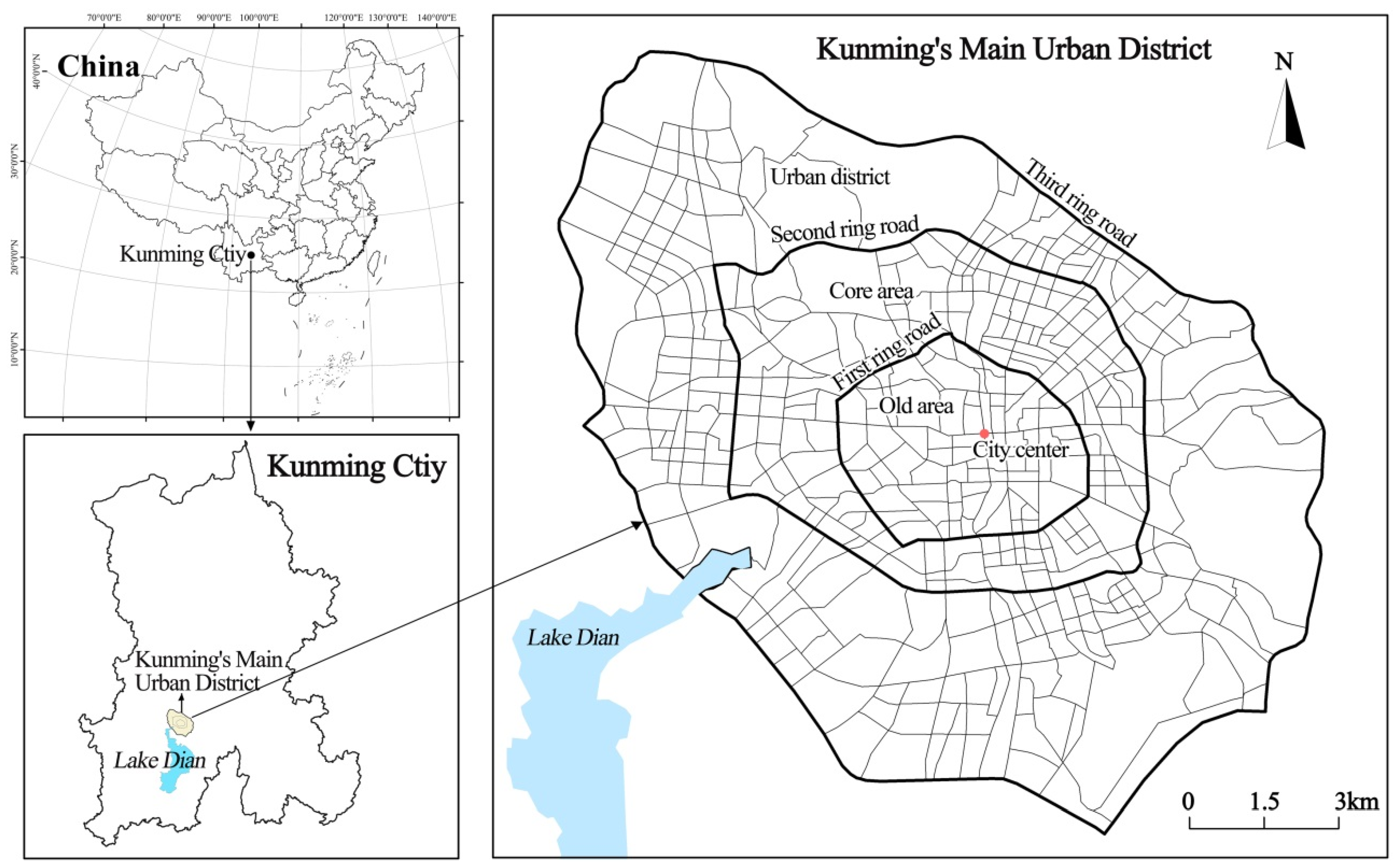
2.3. Research Area
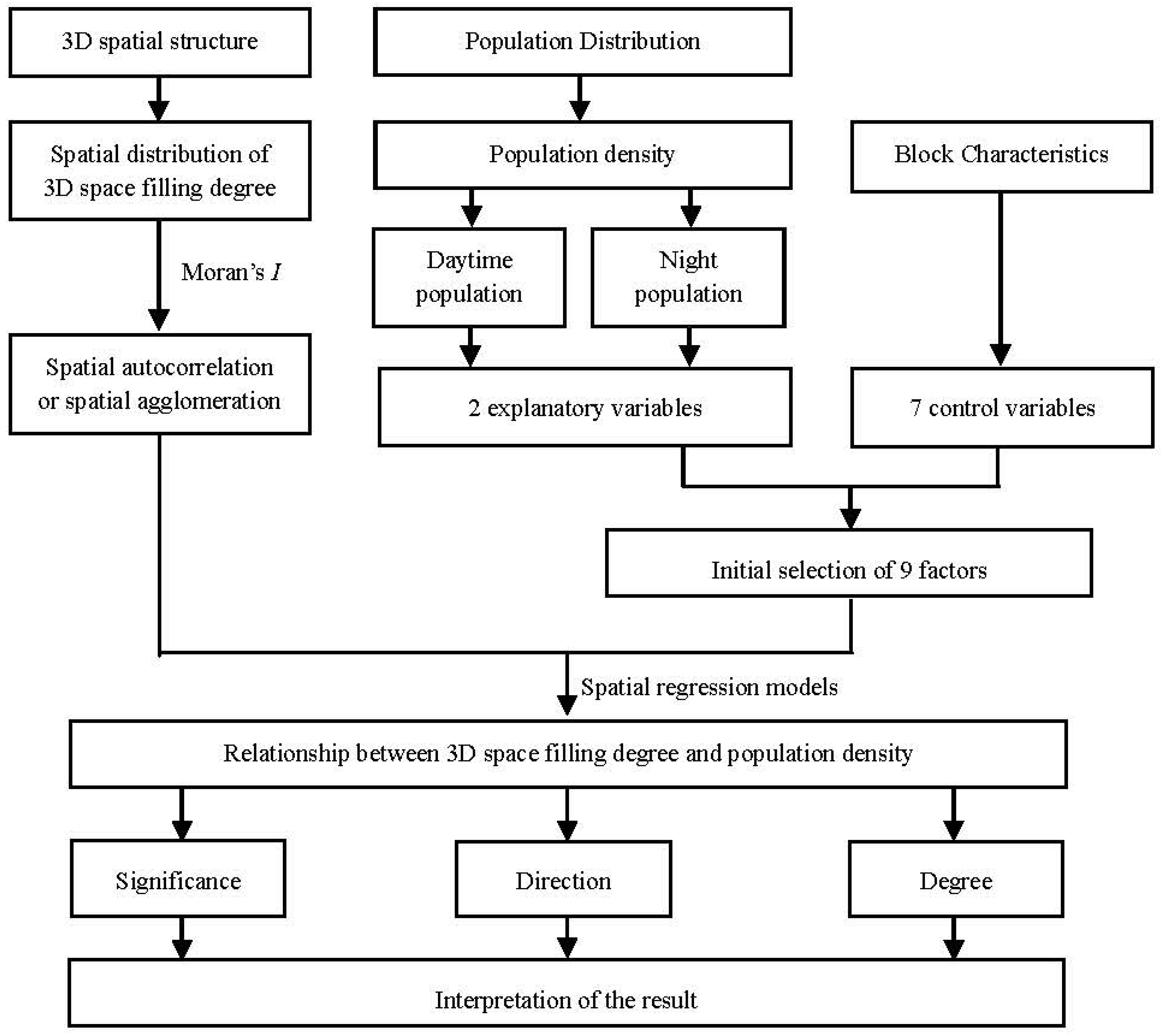
2.4. Research Design, Indicator System, and Model
2.5. Data and Data Sources
2.6. Spatial Regression Model
3. Results
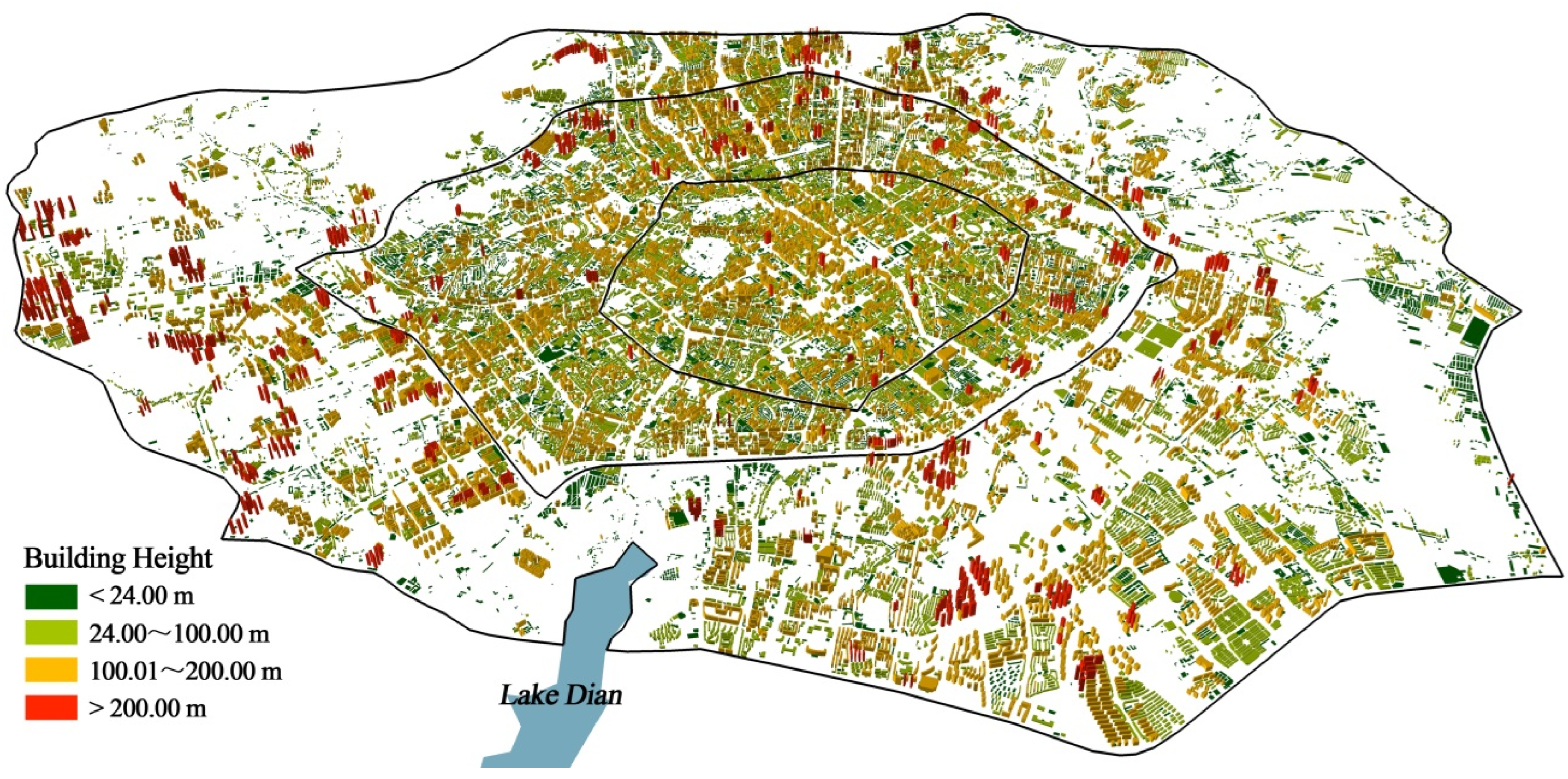
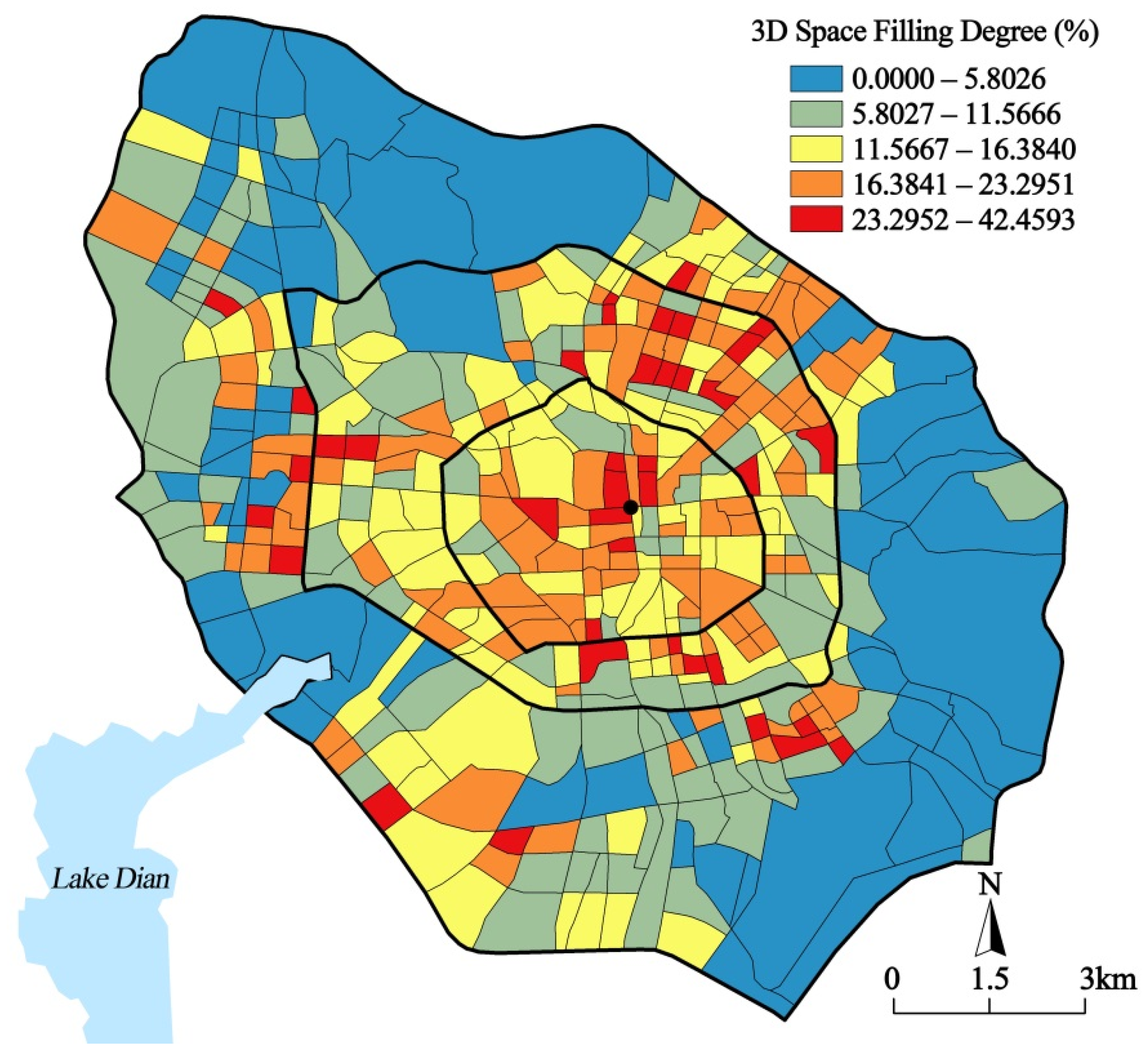
3.1. Urban 3D Spatial Structure
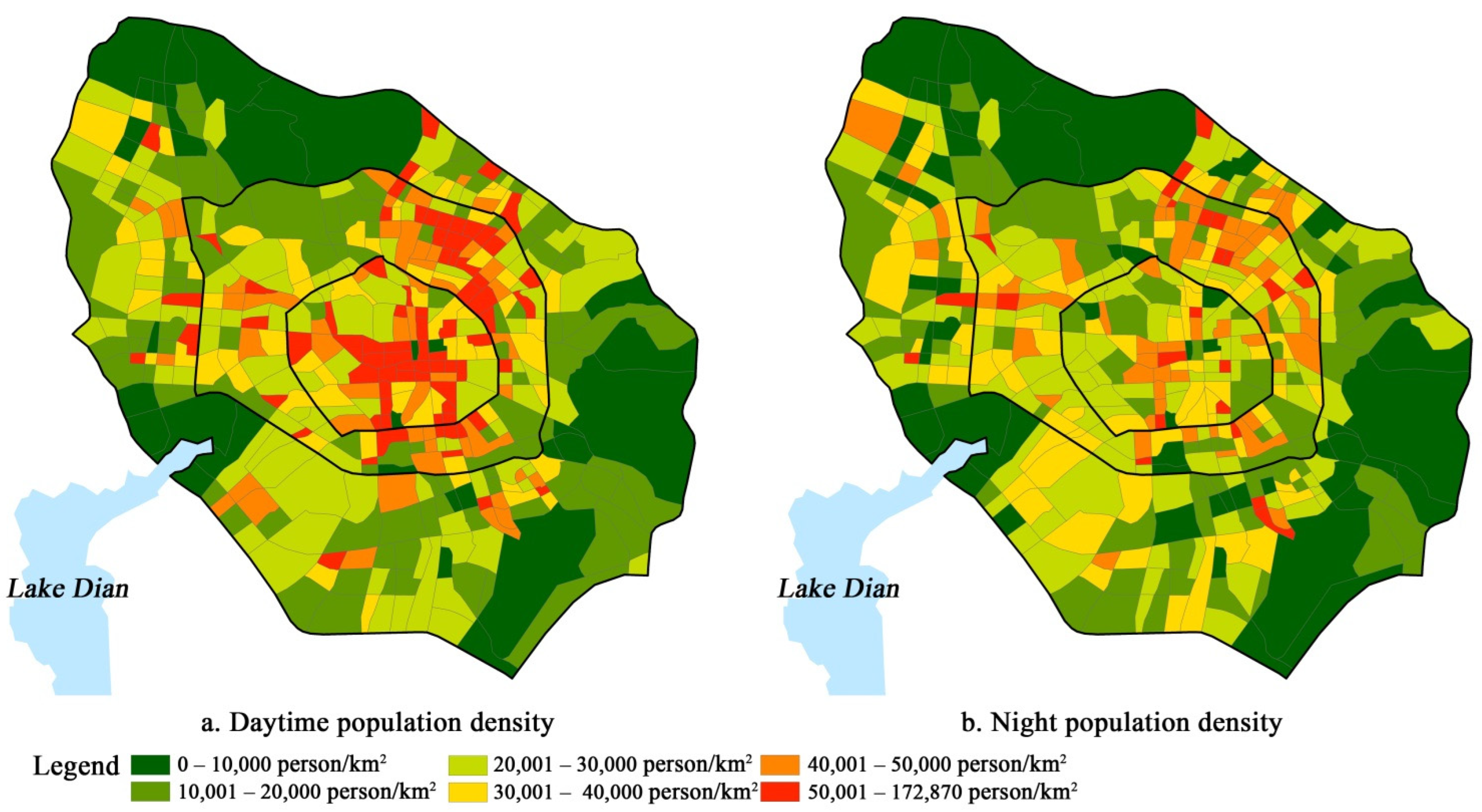
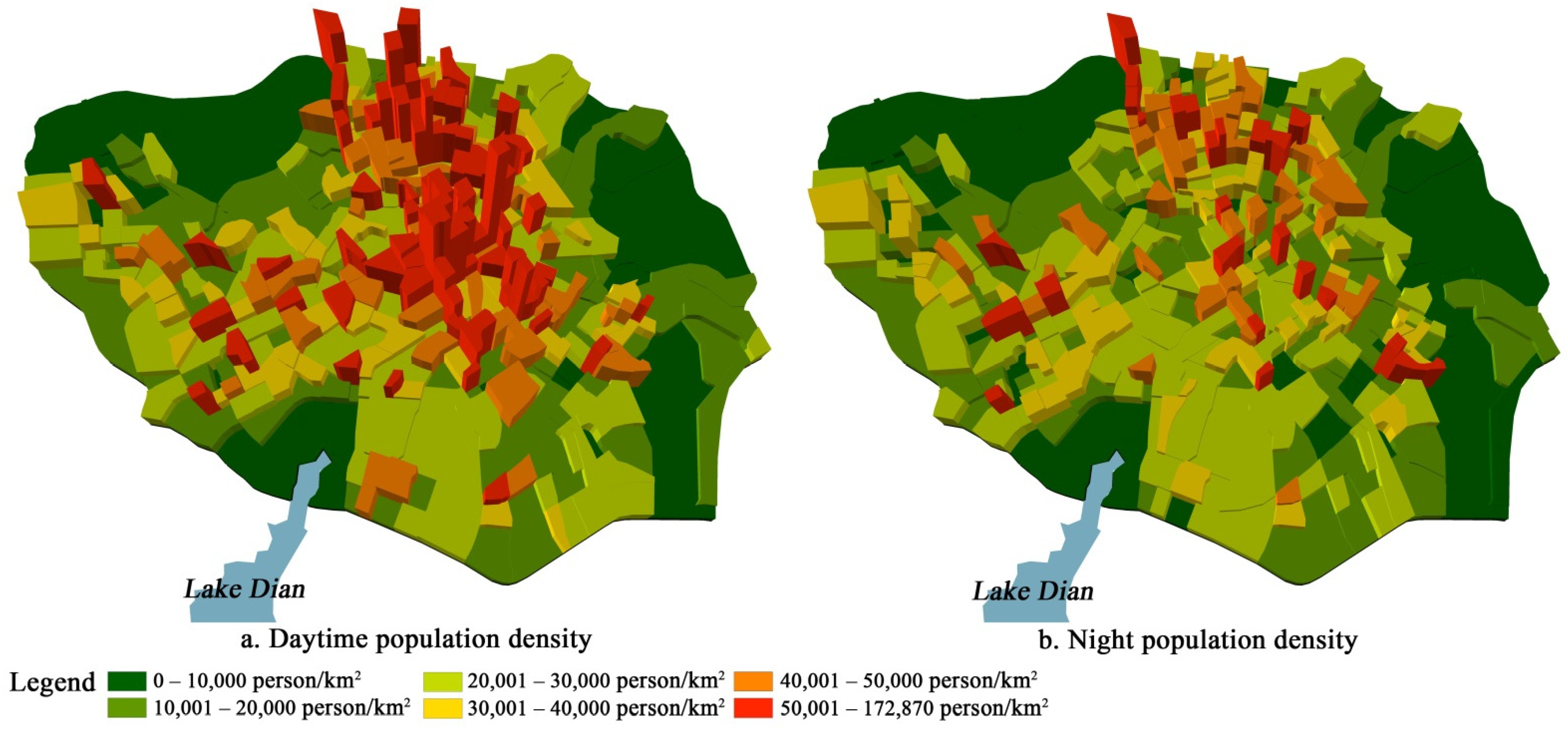
3.2. Spatial Pattern of Population Distribution
3.3. Relationship between Urban 3D Spatial Structure and Population Distribution in Kunming’s Main Urban District
4. Discussion and Conclusions
4.1. Discussion
4.2. Conclusions
4.3. Policy Implications
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xia, F.Z.; Shen, Y.; Yan, J.M.; Bao, H.X.H. On the potential of urban three-dimensional space development: The case of Liuzhou, China. Habitat Int. 2016, 51, 48–58. [Google Scholar] [CrossRef]
- Peng, J.; Liu, Q.H.; Blaschke, T.; Zhang, Z.; Liu, Y.X.; Hu, Y.N.; Wang, M.; Xu, Z.H.; Wu, J.S. Integrating land development size, pattern, and density to identify urban-rural fringe in a metropolitan region. Landsc. Ecol. 2020, 35, 2045–2059. [Google Scholar] [CrossRef]
- Zhang, W.P.; Shi, P.J.; Tong, H.L. Research on construction land use benefit and the coupling coordination relationship based on a three-dimensional frame model—A case study in the Lanzhou-Xining urban agglomeration. Land 2022, 11, 460. [Google Scholar] [CrossRef]
- Koziatek, O.; Dragicevic, S. A local and regional spatial index for measuring three-dimensional urban compactness growth. Environ. Plan. B-Urban Anal. City Sci. 2019, 46, 143–164. [Google Scholar] [CrossRef]
- Wei, Y.G.; Huang, C.; Lam, P.T.I.; Yuan, Z.Y. Sustainable urban development: A review on urban carrying capacity assessment. Habitat Int. 2015, 46, 64–71. [Google Scholar] [CrossRef]
- Gao, S.H.; Zhan, Q.M.; Yang, C.; Liu, H.M. The diversified impacts of urban morphology on land surface temperature among urban functional zones. Int. J. Environ. Res. Public Health 2020, 17, 9578. [Google Scholar] [CrossRef]
- Xu, X.; Ou, J.; Liu, P.; Liu, X.P.; Zhang, H.H. Investigating the impacts of three-dimensional spatial structures on CO2 emissions at the urban scale. Sci. Total Environ. 2021, 762, 143096. [Google Scholar] [CrossRef]
- Lin, J.Y.; Lu, S.Y.; He, X.Y.; Wang, F. Analyzing the impact of three-dimensional building structure on CO2 emissions based on random forest regression. Energy 2021, 236, 121502. [Google Scholar] [CrossRef]
- Yin, C.; Yuan, M.; Lu, Y.; Huang, Y.; Liu, Y. Effects of urban form on the urban heat island effect based on spatial regression model. Sci. Total Environ. 2018, 634, 696–704. [Google Scholar] [CrossRef]
- Qiao, W.F.; Gao, J.B.; Guo, Y.Z.; Ji, Q.Q.; Wu, J.; Cao, M. Multi-dimensional expansion of urban space through the lens of land use: The case study of Nanjing City, China. J. Geogr. Sci. 2019, 29, 749–761. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.B.; Cai, M.; Yao, Y.F. A modified 3D algorithm for road traffic noise attenuation calculations in large urban areas. J. Environ. Manag. 2017, 196, 614–626. [Google Scholar] [CrossRef] [PubMed]
- Zheng, Z.; Zhou, W.Q.; Wang, J.; Hu, X.F.; Qian, Y.G. Sixty-year changes in residential landscapes in Beijing: A perspective from both the horizontal (2D) and vertical (3D) dimensions. Remote Sens. 2017, 9, 992. [Google Scholar] [CrossRef] [Green Version]
- Zhou, W.Q.; Wang, J.; Cadenasso, M.L. Effects of the spatial configuration of trees on urban heat mitigation: A comparative study. Remote Sens. Environ. 2017, 195, 1–12. [Google Scholar] [CrossRef]
- Li, X.M.; Zhang, D.H.; Tian, S.Z.; Wang, M. Spatial and temporal differences of urban residential quarter floor area ratio: A case study of four districts in Dalian. Sci. Geogr. Sin. 2018, 38, 531–538. [Google Scholar]
- Lee, J.M.; Braham, W. Right-sizing cities for maximum power: Urban form parameters for New York City and the Greater Philadelphia Region. Sustainability 2019, 11, 2352. [Google Scholar] [CrossRef] [Green Version]
- Usui, H. Building height distribution under zoning regulations: Theoretical derivation based on allometric scaling analysis and application to harmonise building heights. Environ. Plan. B-Urban Anal. City Sci. 2021, 48, 2520–2535. [Google Scholar] [CrossRef]
- Rao, Y.; Zhou, J.; Zhou, M.; He, Q.S.; Wu, J.Y. Comparisons of three-dimensional urban forms in different urban expansion types: 58 sample cities in China. Growth Chang. 2020, 51, 1766–1783. [Google Scholar] [CrossRef]
- He, S.J.; Wang, X.Y.; Dong, J.R.; Wei, B.C.; Duan, H.M.; Jiao, J.Z.; Xie, Y.W. Three-dimensional urban expansion analysis of valley-type cities: A case study of Chengguan district, Lanzhou, China. Sustainability 2019, 11, 5663. [Google Scholar] [CrossRef] [Green Version]
- Jayasinghe, A.; Madusanka, N.B.S.; Abenayake, C.; Mahanama, P.K.S. A modeling framework: To analyze the relationship between accessibility, land use and densities in urban areas. Sustainability 2021, 13, 467. [Google Scholar] [CrossRef]
- Zhao, S.C.; Liu, Y.; Liang, S.; Wang, C.; Arora, M. Effects of urban forms on energy consumption of water supply in China. J. Clean. Prod. 2020, 253, 119960. [Google Scholar] [CrossRef]
- Dong, T.; Jiao, L.M.; Xu, G.; Liu, J.F. Towards sustainability? Analyzing changing urban form patterns in the United States, Europe, and China. Sci. Total Environ. 2019, 671, 632–643. [Google Scholar] [CrossRef] [PubMed]
- Koziatek, O.; Dragićević, S. iCity 3D: A geosimualtion method and tool for three-dimensional modeling of vertical urban development. Landsc. Urban Plan. 2017, 167, 356–367. [Google Scholar] [CrossRef]
- Zuo, T.; Wei, H.; Liu, H.; Yang, Y.J. Bi-level optimization approach for configuring population and employment distributions with minimized vehicle travel demand. J. Transp. Geogr. 2019, 74, 161–172. [Google Scholar] [CrossRef]
- Peng, Y.; Liu, J.; Zhang, T.; Li, X. The Relationship between urban population density distribution and land use in Guangzhou, China: A spatial spillover perspective. Int. J. Environ. Res. Public Health 2021, 18, 12160. [Google Scholar] [CrossRef]
- Li, J.G.; Li, J.W.; Yuan, Y.; Li, G.F. Spatiotemporal distribution characteristics and mechanism analysis of urban population density: A case of Xi’an, Shaanxi, China. Cities 2019, 86, 62–70. [Google Scholar] [CrossRef]
- Li, Z.H.; Jiao, L.M.; Zhang, B.E.; Liu, J.F. Understanding the pattern and mechanism of spatial concentration of urban land use, population and economic activities: A case study in Wuhan, China. Geo-Spatial Inf. Sci. 2021, 24, 678–694. [Google Scholar] [CrossRef]
- Joshi, K.K.; Kono, T. Optimization of floor area ratio regulation in a growing city. Reg. Sci. Urban Econ. 2009, 39, 502–511. [Google Scholar] [CrossRef]
- Koohsari, M.J.; Badland, H.; Giles-Corti, B. (Re)Designing the built environment to support physical activity: Bringing public health back into urban design and planning. Cities 2013, 35, 294–298. [Google Scholar] [CrossRef]
- Salgado, M.; Madureira, J.; Mendes, A.S.; Torres, A.; Teixeira, J.P.; Oliveira, M.D. Environmental determinants of population health in urban settings. A systematic review. BMC Public Health 2020, 20, 853. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, F.; Xiao, Y.; Gao, S. Urban land uses and traffic source-sink areas: Evidence from GPS-enabled taxi data in Shanghai. Landscape Urban Plan. 2012, 106, 73–87. [Google Scholar] [CrossRef]
- Pei, T.; Sobolevsky, S.; Ratti, C.; Shaw, S.L.; Li, T.; Zhou, C. A new insight into land use classification based on aggregated mobile phone data. Int. J. Geogr. Inf. Sci. 2014, 28, 1988–2007. [Google Scholar] [CrossRef] [Green Version]
- Louail, T.; Lenormand, M.; Cantu Ros, O.G.; Picornell, M.; Herranz, R.; Frias-Martinez, E.; Ramasco, J.J.; Barthelemy, M. From mobile phone data to the spatial structure of cities. Sci. Rep. 2014, 4, 5276. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Song, Y.; Tan, Y.; Song, Y.; Wu, P.; Cheng, J.C.P.; Kim, M.J.; Wang, X. Spatial and temporal variations of spatial population accessibility to public hospitals: A case study of rural-urban comparison. Gisci. Remote Sens. 2018, 55, 718–744. [Google Scholar] [CrossRef]
- Lan, F.; Gong, X.; Da, H.; Wen, H. How do population inflow and social infrastructure affect urban vitality? Evidence from 35 large- and medium-sized cities in China. Cities 2020, 100, 102454. [Google Scholar] [CrossRef]
- Zeng, P.; Sun, Z.Y.; Chen, Y.Q.; Qiao, Z.; Cai, L.W. COVID-19: A comparative study of population aggregation patterns in the Central Urban Area of Tianjin, China. Int. J. Environ. Res. Public Health 2021, 18, 2135. [Google Scholar] [CrossRef] [PubMed]
- Dovey, K.; Pafka, E. The urban density assemblage: Modelling multiple measures. Urban Design Int. 2014, 19, 66–76. [Google Scholar] [CrossRef]
- Shi, G.; Shan, J.; Ding, L.; Ye, P.; Li, Y.; Jiang, N. Urban road network expansion and its driving variables: A case study of Nanjing City. Int. J. Environ. Res. Public Health 2019, 16, 2318. [Google Scholar] [CrossRef] [Green Version]
- Liu, G.; Yao, X.B.; Luo, Z.M.; Kang, S.W.; Long, W.; Fan, Q.J.; Gao, P.C. Agglomeration centrality to examine spatial scaling law in cities. Comput. Environ. Urban Syst. 2019, 77, 10. [Google Scholar] [CrossRef]
- Sakamoto, K.; Iida, A.; Yokohari, M. Spatial patterns of population turnover in a Japanese Regional City for urban regeneration against population decline: Is Compact City policy effective? Cities 2018, 81, 230–241. [Google Scholar] [CrossRef]
- Tsai, Y.H. Quantifying urban form: Compactness versus ‘Sprawl’. Urban Stud. 2016, 42, 141–161. [Google Scholar] [CrossRef]
- Alahmadi, M.; Atkinson, P.; Martin, D. Estimating the spatial distribution of the population of Riyadh, Saudi Arabia using remotely sensed built land cover and height data. Comput. Environ. Urban Syst. 2013, 41, 167–176. [Google Scholar] [CrossRef]
- Yu, G.J.; Xie, Z.X.; Li, X.C.; Wang, Y.X.; Huang, J.X.; Yao, X.C. The potential of 3-D building height data to characterize socioeconomic activities: A case study from 38 cities in China. Remote Sens. 2022, 14, 2087. [Google Scholar] [CrossRef]
- Yao, Y.; Chen, W.Z.; Chen, X.D.; Yu, J.S. Mapping fine-scale population distributions at the building level by integrating multisource geospatial big data. Int. J. Geogr. Inf. Sci. 2017, 31, 1220–1244. [Google Scholar] [CrossRef]
- Zhu, S.N.; Dai, Q.; Zhao, B.R.; Shao, J.Q. Assessment of population exposure to urban flood at the building scale. Water 2020, 12, 3253. [Google Scholar] [CrossRef]
- Zhao, Y.; Ovando-Montejo, G.A.; Frazier, A.E.; Mathews, A.J.; Flynn, K.C.; Ellis, E.A. Estimating work and home population using LIDAR-derived building volumes. Int. J. Remote Sens. 2017, 38, 1180–1196. [Google Scholar] [CrossRef]
- Qiu, F.; Sridharan, H.; Chun, Y. Spatial autoregressive model for population estimation at the census block level using LiDAR−derived building volume information. Cartogr. Geogr. Inf. Sci. 2010, 37, 239–257. [Google Scholar] [CrossRef]
- Kono, T.; Kaneko, T.; Morisugi, H. Necessity of minimum floor area ratio regulation: A second-best policy. Ann. Reg. Sci. 2010, 44, 523–539. [Google Scholar] [CrossRef]
- Tan, S.K.; Liu, Q.; Han, S.Y. Spatial-temporal evolution of coupling relationship between land development intensity and resources environment carrying capacity in China. J. Environ. Manag. 2022, 301, 113778. [Google Scholar] [CrossRef]
- Zhong, W.; Wang, D.; Xie, D.; Yan, L. Dynamic characteristics of Shanghai’s population distribution using cell phone signaling data. Geogr. Res. 2017, 36, 972–984. [Google Scholar]
- Biljecki, F.; Arroyo Ohori, K.; Ledoux, H.; Peters, R.; Stoter, J. Population estimation using a 3D city model: A multi-scale country-wide study in the Netherlands. PLoS ONE 2016, 11, e0156808. [Google Scholar] [CrossRef]
- Ding, C. Building height restrictions, land development and economic costs. Land Use Pol. 2013, 30, 485–495. [Google Scholar] [CrossRef]
- Lin, J.; Wan, H.; Cui, Y. Analyzing the spatial factors related to the distributions of building heights in urban areas: A comparative case study in Guangzhou and Shenzhen. Sust. Cities Soc. 2020, 52, 101854. [Google Scholar] [CrossRef]
- Sun, W.; Li, T. Building height trends and their influencing factors under China’s rapid urbanization: A case study of Guangzhou, 1960–2017. Chin. Geogr. Sci. 2020, 30, 993–1004. [Google Scholar] [CrossRef]
- Gong, P.; Chen, B.; Li, X.; Liu, H.; Wang, J.; Bai, Y.; Chen, J.; Chen, X.; Fang, L.; Feng, S.; et al. Mapping essential urban land use categories in China (EULUC-China): Preliminary results for 2018. Sci. Bull. 2019, 65, 182–187. [Google Scholar] [CrossRef] [Green Version]
- Anselin, L. Spatial Econometrics: Methods and Models; Springer: Dordrecht, The Netherlands, 1988; pp. 1–254. [Google Scholar]
- Anselin, L.; Syabri, I.; Kho, Y. GeoDa: An introduction to spatial data analysis. Geogr. Anal. 2006, 38, 5–22. [Google Scholar] [CrossRef]
- Arbia, G. Spatial Econometrics: Statistical Foundations and Applications to Regional Economic Growth; Springer: Berlin/Heidelberg, Germany, 2006; pp. 1–146. [Google Scholar]

| Variable (Symbol) | Unit | Expected Impact Direction |
|---|---|---|
| Dependent Variable | ||
| 3D space filling degree (3DSFD) | % | |
| Explanatory Variables—Population Density | ||
| Daytime population density (DPD) | Person/km2 | + |
| Night population density (NPD) | Person/km2 | + |
| Control Variables—Block Characteristics | ||
| Distance from the city center (DFCC) | m | + |
| Building density (BD) | % | + |
| Road density (RD) | km/km2 | + |
| Functional place density (FPD) | Point/km2 | + |
| Proportion of undevelopable land area (ULA) | % | - |
| Housing prices(HP) | Yuan/m2 | + |
| Land use type(LUP) | Score | + |
| Tolerance | VIF Value | |
|---|---|---|
| DPD | 0.171 | 5.833 |
| NPD | 0.200 | 5.002 |
| DFCC | 0.548 | 1.826 |
| BD | 0.375 | 2.668 |
| RD | 0.823 | 1.215 |
| FPD | 0.326 | 3.066 |
| PULA | 0.934 | 1.071 |
| HP | 0.836 | 1.196 |
| LUP | 0.892 | 1.121 |
| Model | R2 | AIC | Log Likelihood |
|---|---|---|---|
| OLS | 0.518041 | 2683.99 | −1332.00 |
| SLM | 0.524701 | 2681.01 | −1329.50 |
| SEM | 0.546415 | 2664.95 | −1322.48 |
| Coefficient | Std. Error | t/z-Value | p | |
|---|---|---|---|---|
| DPD | −1.2279 | 0.8084 | −1.5190 | 0.1288 |
| NPD | 2.8307 ** | 0.7713 | 3.6702 | 0.0002 |
| DFCC | −1.0071 | 0.7084 | −1.4216 | 0.1551 |
| BD | 4.5480 ** | 0.5705 | 7.9720 | 0.0000 |
| RD | 0.9098 | 0.5804 | 1.5675 | 0.1170 |
| FPD | 0.9073 * | 0.3889 | 2.3328 | 0.0197 |
| PULA | −0.5323 * | 0.2170 | −2.4526 | 0.0142 |
| HP | 1.3390 | 1.3304 | 1.0065 | 0.3142 |
| LUP | 2.9564 * | 1.3833 | 2.1373 | 0.0326 |
| CONSTANT | −31.1550 * | 15.7597 | −1.9769 | 0.0481 |
| LAMBDA | 0.3999 ** | 0.0825 | 4.8461 | 0.0000 |
| R2: 0.546415; AIC: 2664.95; Log likelihood: −1322.48 | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Yue, X.; Li, C.; Wang, M.; Zhang, H.; Su, Y. Relationship between Urban Three-Dimensional Spatial Structure and Population Distribution: A Case Study of Kunming’s Main Urban District, China. Remote Sens. 2022, 14, 3757. https://doi.org/10.3390/rs14153757
Wang Y, Yue X, Li C, Wang M, Zhang H, Su Y. Relationship between Urban Three-Dimensional Spatial Structure and Population Distribution: A Case Study of Kunming’s Main Urban District, China. Remote Sensing. 2022; 14(15):3757. https://doi.org/10.3390/rs14153757
Chicago/Turabian StyleWang, Yang, Xiaoli Yue, Cansong Li, Min Wang, Hong’ou Zhang, and Yongxian Su. 2022. "Relationship between Urban Three-Dimensional Spatial Structure and Population Distribution: A Case Study of Kunming’s Main Urban District, China" Remote Sensing 14, no. 15: 3757. https://doi.org/10.3390/rs14153757
APA StyleWang, Y., Yue, X., Li, C., Wang, M., Zhang, H., & Su, Y. (2022). Relationship between Urban Three-Dimensional Spatial Structure and Population Distribution: A Case Study of Kunming’s Main Urban District, China. Remote Sensing, 14(15), 3757. https://doi.org/10.3390/rs14153757







