Comparison between the Mesospheric Winds Observed by Two Collocated Meteor Radars at Low Latitudes
Abstract
:1. Introduction
2. Instruments and Data Sets
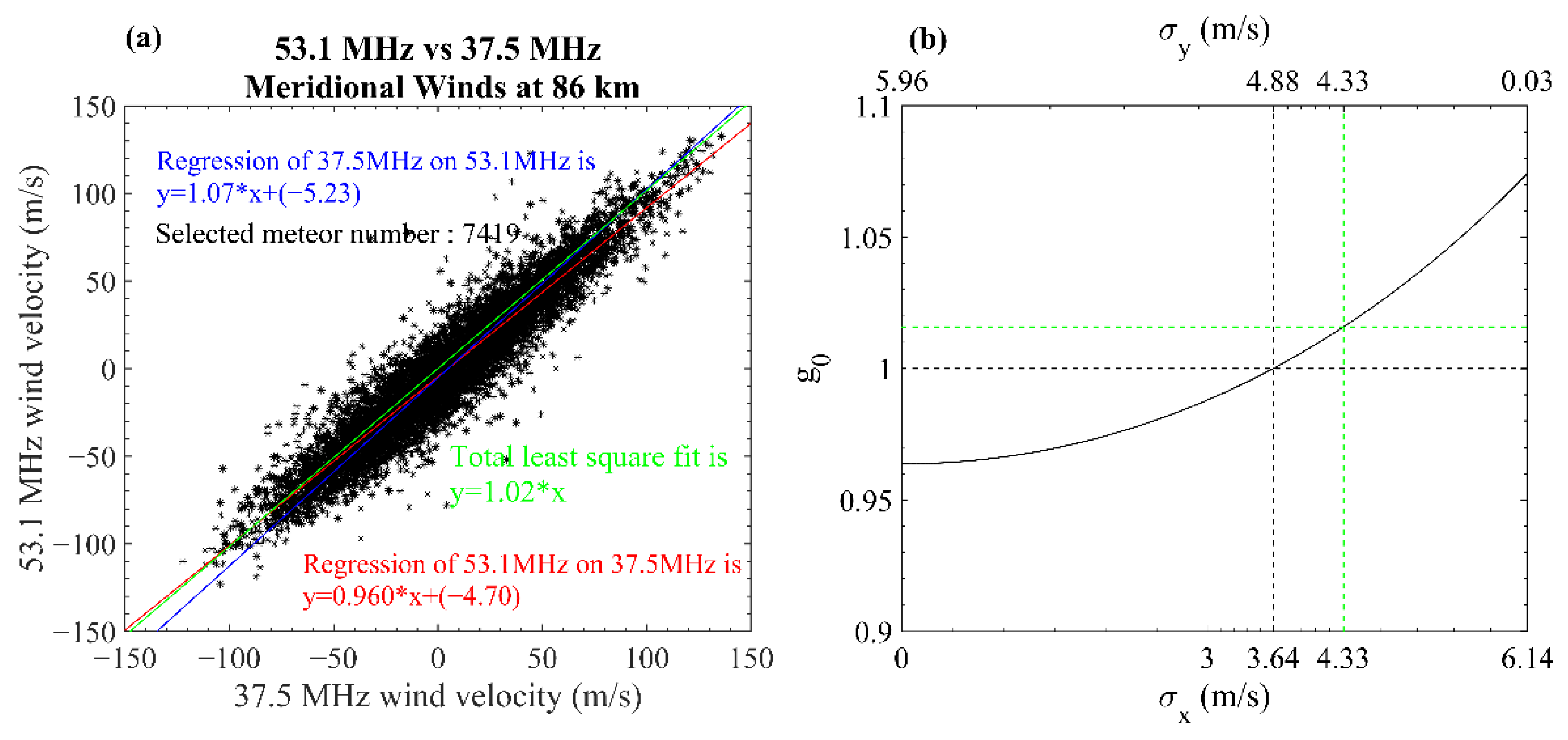
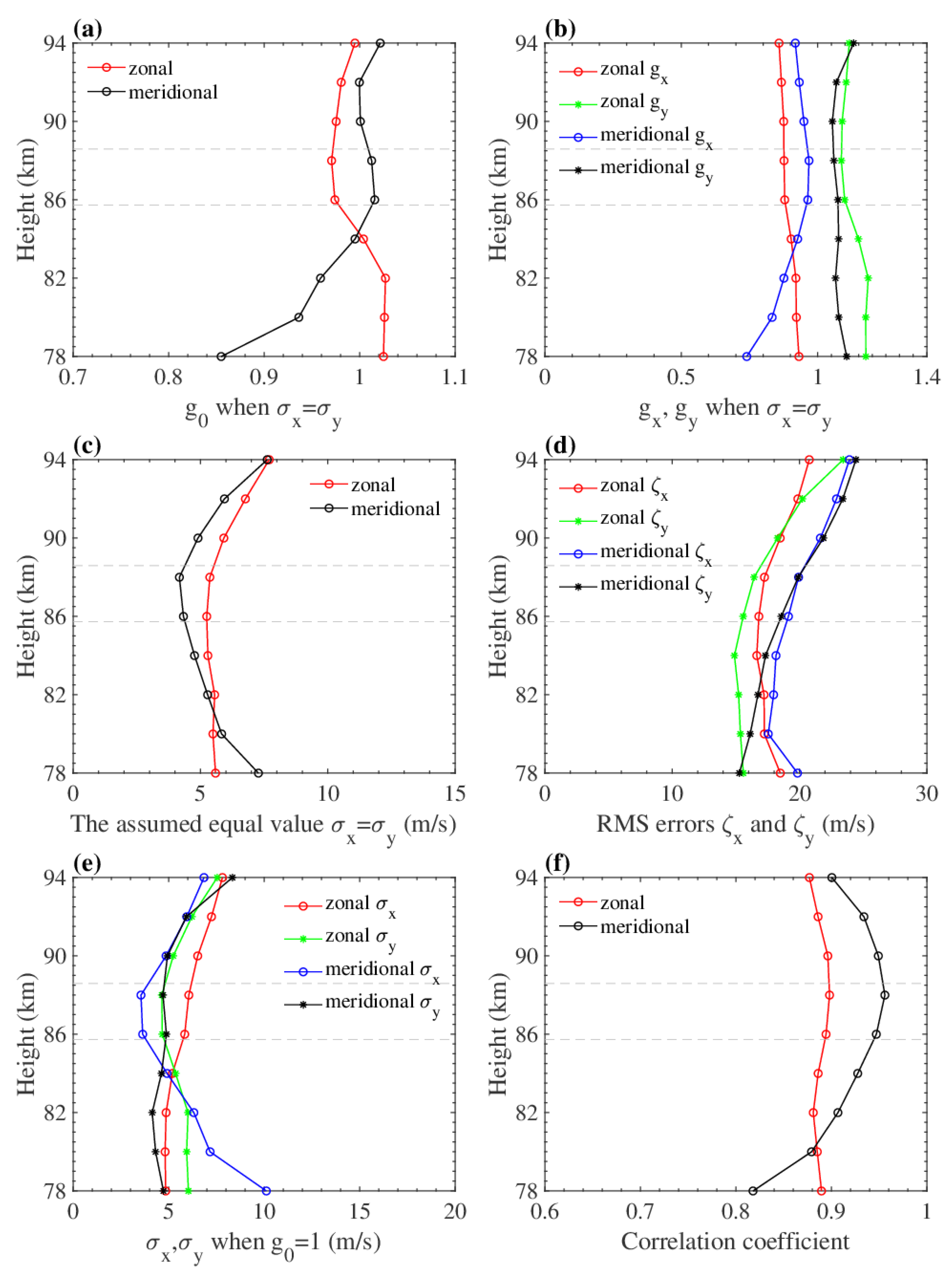
3. Method
4. Results
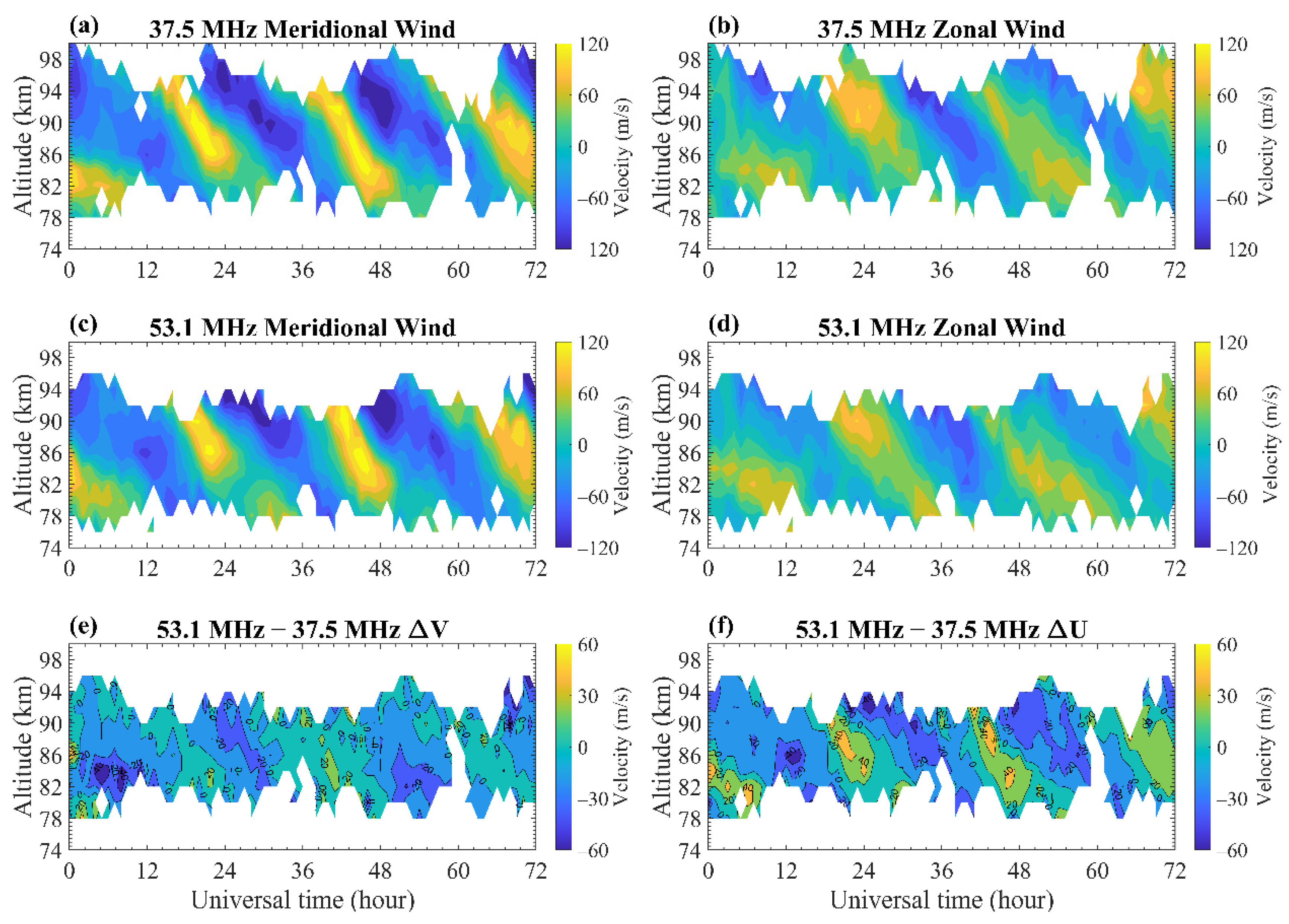
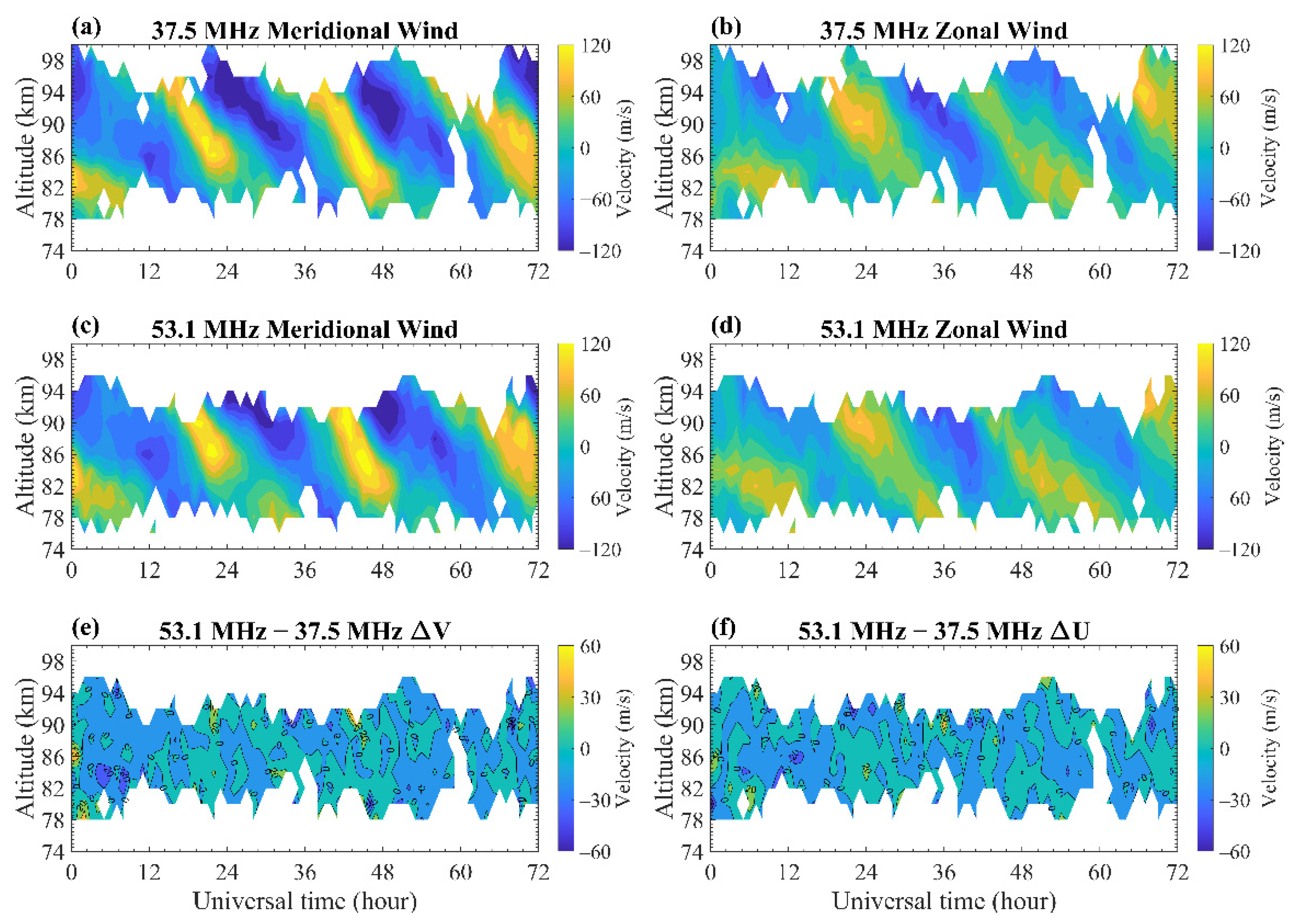
4.1. Comparison between the Kunming 37.5 MHz and 53.1 MHz Meteor Radar Winds
4.2. Comparison with Corrected Winds
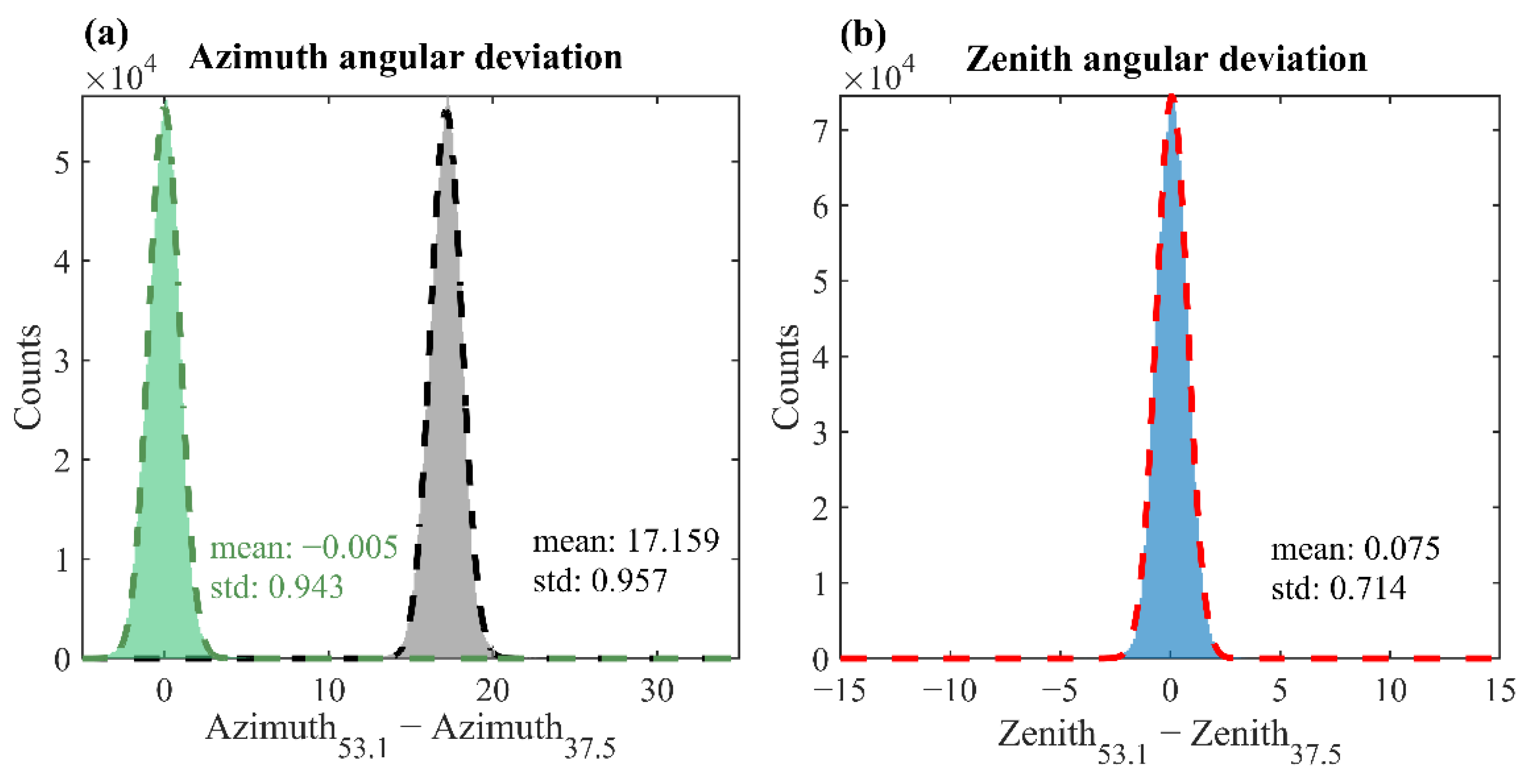
4.2.1. Proposed Method for Correcting the Antenna Angular Deviation
4.2.2. Comparison of the Wind Results after an Angular Correction
5. Discussion
6. Summary
- Statistical analyses of the winds measured by the two MRs reveal an estimate of the uncertainties in the winds from the two systems, meaning the wind fields obtained by the different radars can be calibrated. The least squares regression fitting line is close to the y = x line, which means that the random errors of the two techniques are similar.
- Our correction method identifies the relative deviation between the reference directions of two collocated MR systems by selecting simultaneously observed meteors. This enables us to estimate the deviation in the azimuth reference direction. Fortunately, this method requires no additional hardware or data. After the correction has been implemented, the mean deviation between the reference directions of the two MR receiving systems approaches 0°; thus, the two systems can be regarded as being aligned. For these two MRs, correcting the angular deviation facilitates a qualitative and quantitative comparison, as well as the joint observation and verification of atmospheric dynamics in the MLT region, such as atmospheric tides and gravity waves.
- The winds measured by the two collocated MRs show strong agreement. The results are highly correlated with the meteor distributions and are best at the peak height. Within the altitude range of 78–94 km, the correlation coefficients are higher than 0.78, and the wind velocities observed by the 53.1 MHz MR are generally lower than those observed by the 37.5 MHz MR by approximately 5–20%. After the angular deviation is corrected, the correlation coefficients increase by ~0.05 and are essentially greater than 0.9 over the entire height range. Furthermore, the uncertainties and are reduced by approximately 20%, and the differences in the wind components can basically be ignored. The comparison of the results from before and after implementing the correction confirms the consistent performance of both KMMRs for the entire detection height range. When compared to other techniques, MRs seem to provide a benchmark for MLT wind measurements, at least in this study within the altitude range of 78–94 km, where enough meteor counts are obtained.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Vincent, R.A. The dynamics of the mesosphere and lower thermosphere: A brief review. Prog. Earth Planet. Sci. 2015, 2, 4. [Google Scholar] [CrossRef] [Green Version]
- Reid, I.M. MF and HF radar techniques for investigating the dynamics and structure of the 50 to 110 km height region: A review. Prog. Earth Planet. Sci. 2015, 2, 33. [Google Scholar] [CrossRef] [Green Version]
- Sato, K.; Watanabe, S.; Kawatani, Y.; Tomikawa, Y.; Miyazaki, K.; Takahashi, M. On the origins of mesospheric gravity waves. Geophys. Res. Lett. 2009, 36, L19801. [Google Scholar] [CrossRef] [Green Version]
- Hocking, W.K.; Thayaparan, T.; Franke, S.J. Method for statistical comparison of geophysical data by multiple instruments which have differing accuracies. Adv. Space Res. 2001, 27, 1089–1098. [Google Scholar] [CrossRef]
- Holdsworth, D.A.; Reid, I.M.; Cervera, M.A. Buckland Park all-sky interferometric meteor radar. Radio Sci. 2004, 39, RS5009. [Google Scholar] [CrossRef]
- Yu, Y.; Wan, W.; Ning, B.; Liu, L.; Wang, Z.; Hu, L.; Ren, Z. Tidal wind mapping from observations of a meteor radar chain in December 2011. J. Geophys. Res. Space Phys. 2013, 118, 2321–2332. [Google Scholar] [CrossRef] [Green Version]
- Chau, J.L.; Stober, G.; Hall, C.M.; Tsutsumi, M.; Laskar, F.I.; Hoffmann, P. Polar mesospheric horizontal divergence and relative vorticity measurements using multiple specular meteor radars. Radio Sci. 2017, 52, 811–828. [Google Scholar] [CrossRef]
- Jia, M.; Xue, X.; Gu, S.; Chen, T.; Ning, B.; Wu, J.; Zeng, X.; Dou, X. Multiyear Observations of Gravity Wave Momentum Fluxes in the Midlatitude Mesosphere and Lower Thermosphere Region by Meteor Radar. J. Geophys. Res. Space Phys. 2018, 123, 5684–5703. [Google Scholar] [CrossRef] [Green Version]
- Kishore Kumar, G.; Hocking, W.K. Climatology of northern polar latitude MLT dynamics: Mean winds and tides. Ann. Geophys. 2010, 28, 1859–1876. [Google Scholar] [CrossRef]
- Wilhelm, S.; Stober, G.; Chau, J.L. A comparison of 11-year mesospheric and lower thermospheric winds determined by meteor and MF radar at 69°N. Ann. Geophys. 2017, 35, 893–906. [Google Scholar] [CrossRef] [Green Version]
- Yi, W.; Reid, I.M.; Xue, X.; Murphy, D.J.; Hall, C.M.; Tsutsumi, M.; Ning, B.; Li, G.; Younger, J.P.; Chen, T.; et al. High- and Middle-Latitude Neutral Mesospheric Density Response to Geomagnetic Storms. Geophys. Res. Lett. 2018, 45, 436–444. [Google Scholar] [CrossRef] [Green Version]
- Yi, W.; Chen, J.-S.; Ma, C.-B.; Li, N.; Zhao, Z.-W. Observation of Upper Atmospheric Temperature by Kunming All-Sky Meteor Radar. Chin. J. Geophys. 2014, 57, 750–760. [Google Scholar] [CrossRef]
- Reid, I.M.; Holdsworth, D.A.; Morris, R.J.; Murphy, D.J.; Vincent, R.A. Meteor observations using the Davis mesosphere-stratosphere-troposphere radar. J. Geophys. Res.-Space Phys. 2006, 111, A05305. [Google Scholar] [CrossRef] [Green Version]
- McKinley, D.W.R. Meteor Science and Engineering, 1st ed.; McGraw-Hill: New York, NY, USA, 1961; pp. 5–104. [Google Scholar]
- Ceplecha, Z.; Borovička, J.; Elford, W.G.; ReVelle, D.O.; Hawkes, R.L.; Porubčan, V.; Šimek, M. Meteor Phenomena and Bodies. Space Sci. Rev. 1998, 84, 327–471. [Google Scholar] [CrossRef]
- Yi, W.; Xue, X.H.; Reid, I.M.; Younger, J.P.; Chen, J.S.; Chen, T.D.; Li, N. Estimation of Mesospheric Densities at Low Latitudes Using the Kunming Meteor Radar Together With SABER Temperatures. J. Geophys. Res.-Space Phys. 2018, 123, 3183–3195. [Google Scholar] [CrossRef]
- Younger, J.P.; Lee, C.S.; Reid, I.M.; Vincent, R.A.; Kim, Y.H.; Murphy, D.J. The effects of deionization processes on meteor radar diffusion coefficients below 90 km. J. Geophys. Res.-Atmos. 2014, 119, 10027–10043. [Google Scholar] [CrossRef] [Green Version]
- Hocking, W.K.; Fuller, B.; Vandepeer, B. Real-time determination of meteor-related parameters utilizing modem digital technology. J. Atmos. Sol.-Terr. Phys. 2001, 63, 155–169. [Google Scholar] [CrossRef]
- Holdsworth, D.A.; Tsutsumi, M.; Reid, I.M.; Nakamura, T.; Tsuda, T. Interferometric meteor radar phase calibration using meteor echoes. Radio Sci. 2004, 39, RS5012. [Google Scholar] [CrossRef]
- Reid, I.M.; McIntosh, D.L.; Murphy, D.J.; Vincent, R.A. Mesospheric radar wind comparisons at high and middle southern latitudes. Earth Planets Space 2018, 70, 84. [Google Scholar] [CrossRef]
- McIntosh, D.L. Comparisons of VHF Meteor Radar Observations in the Middle Atmosphere with Multiple Independent Remote Sensing Techniques. Ph.D. Thesis, University of Adelaide, Adelaide, Australia, 2010. [Google Scholar]
- Yi, W.; Xue, X.H.; Reid, I.M.; Murphy, D.J.; Hall, C.M.; Tsutsumi, M.; Ning, B.Q.; Li, G.Z.; Vincent, R.A.; Chen, J.S.; et al. Climatology of the mesopause relative density using a global distribution of meteor radars. Atmos. Chem. Phys. 2019, 19, 7567–7581. [Google Scholar] [CrossRef] [Green Version]
- Vincent, R.A.; Kovalam, S.; Murphy, D.J.; Reid, I.M.; Younger, J.P. Trends and Variability in Vertical Winds in the Southern Hemisphere Summer Polar Mesosphere and Lower Thermosphere. J. Geophys. Res.-Atmos. 2019, 124, 11070–11085. [Google Scholar] [CrossRef]
- Cervera, M.A.; Reid, I.M. Comparison of simultaneous wind measurements using colocated VHF meteor radar and MF spaced antenna radar systems. Radio Sci. 1995, 30, 1245–1261. [Google Scholar] [CrossRef]
- Hall, C.M.; Aso, T.; Tsutsumi, M.; Nozawa, S.; Manson, A.H.; Meek, C.E. A comparison of mesosphere and lower thermosphere neutral winds as determined by meteor and medium-frequency radar at 70°N. Radio Sci. 2005, 40, RS4001. [Google Scholar] [CrossRef]
- Jones, G.O.L.; Berkey, F.T.; Fish, C.S.; Hocking, W.K.; Taylor, M.J. Validation of imaging Doppler interferometer winds using meteor radar. Geophys. Res. Lett. 2003, 30, 1743. [Google Scholar] [CrossRef] [Green Version]
- Holdsworth, D.A.; Reid, I.M. The Buckland Park MF radar: Routine observation scheme and velocity comparisons. Ann. Geophys. 2004, 22, 3815–3828. [Google Scholar] [CrossRef]
- Liu, A.Z.; Hocking, W.K.; Franke, S.J.; Thayaparan, T. Comparison of Na lidar and meteor radar wind measurements at Starfire Optical Range, NM, USA. J. Atmos. Sol.-Terr. Phys. 2002, 64, 31–40. [Google Scholar] [CrossRef] [Green Version]
- Franke, S.J.; Chu, X.; Liu, A.Z.; Hocking, W.K. Comparison of meteor radar and Na Doppler lidar measurements of winds in the mesopause region above Maui, Hawaii. J. Geophys. Res. Atmos. 2005, 110, D09S02. [Google Scholar] [CrossRef] [Green Version]
- Lee, C.; Jee, G.; Kam, H.; Wu, Q.; Ham, Y.B.; Kim, Y.H.; Kim, J.H. A Comparison of Fabry–Perot Interferometer and Meteor Radar Wind Measurements Near the Polar Mesopause Region. J. Geophys. Res. Space Phys. 2021, 126, e2020JA028802. [Google Scholar] [CrossRef]
- Gu, S.; Hou, X.; Li, N.; Yi, W.; Ding, Z.; Chen, J.; Hu, G.; Dou, X. First Comparative Analysis of the Simultaneous Horizontal Wind Observations by Collocated Meteor Radar and FPI at Low Latitude through 892.0-nm Airglow Emission. Remote Sens. 2021, 13, 4337. [Google Scholar] [CrossRef]

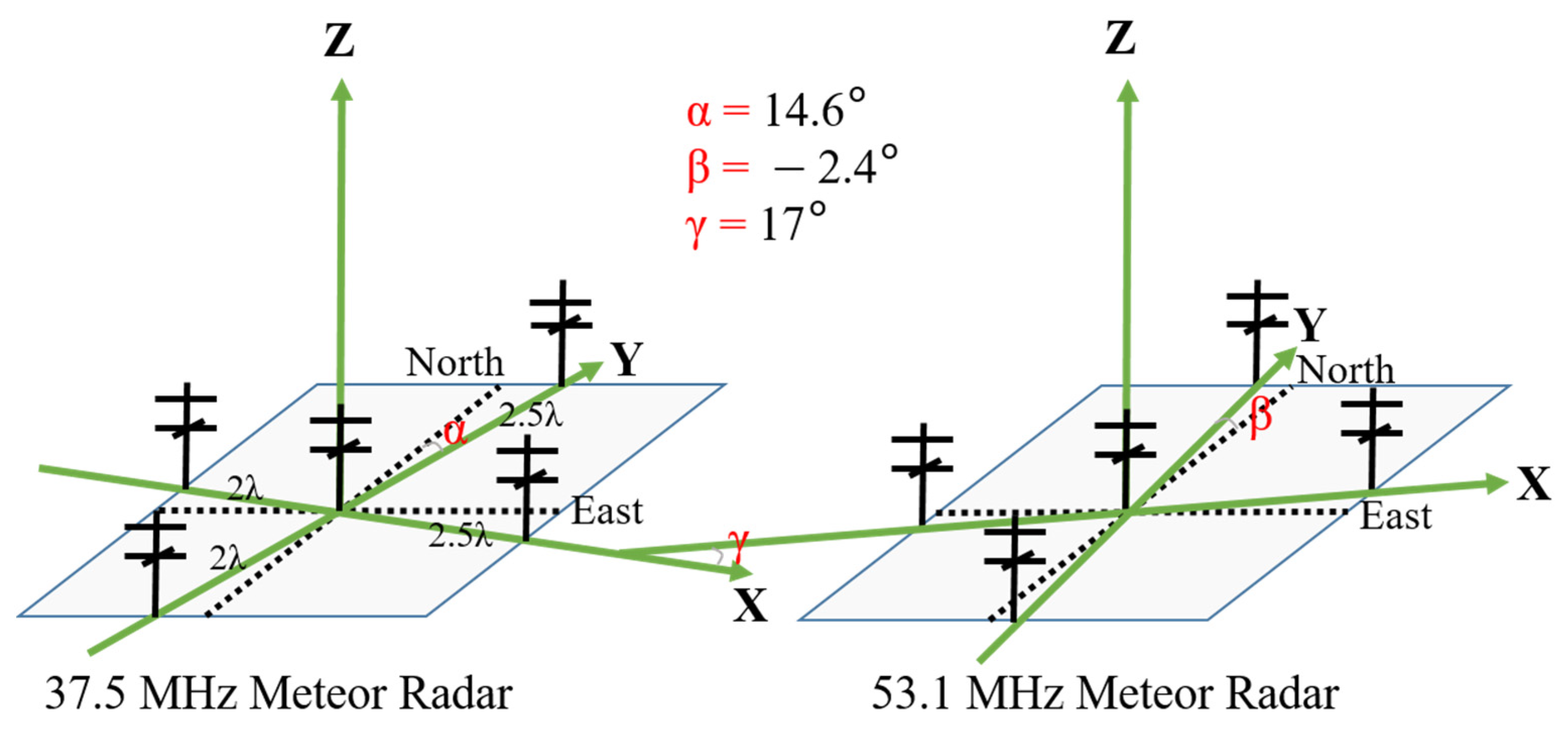



| Parameter | 37.5 MHz | 53.1 MHz |
| Pulse repetition frequency (PRF) | 430 Hz | 430 Hz |
| Peak power | 20 kW | 40 kW |
| Range resolution | 1.8 km | 1.8 km |
| Pulse type | Gaussian | Gaussian |
| Detection range | 70–110 km | 70–110 km |
| Pulse width | 24 μs | 24 μs |
| Case | Height Range (km) | Wind Component | Correlation | Mean Difference (m/s) | Standard Deviation (m/s) | Instruments (x and y) and Sites (Lat, Lon) | |
|---|---|---|---|---|---|---|---|
| This study | 76–94 | Zonal | 0.92–0.96 | 0.98–1.02 | −0.43–0.55 | 6.03–11.23 | 37.5 MHz and 53.1 MHz MRs, Kunming (25.6°N, 103.8°E) |
| Meridional | 0.87–0.97 | 0.94–1.00 | −0.03–0.60 | 5.89–11.24 | |||
| Reid et al. (2018) and McIntosh (2010) | 80–98 | Zonal | 0.60–0.78 | 1.05–1.24 | −1.82–1.94 | 13.64–17.16 | 33.2 MHz and 55 MHz MRs, Davis (69°S, 78°E) |
| Meridional | 0.45–0.85 | 0.98–1.23 | 1.08–3.85 | 12.83–23.91 | |||
| Reid et al. (2018) and McIntosh (2010) | 80–98 | Zonal | 0.5–0.8 | 0.38–0.95 | −1–4.5 | 14.7–27.5 | 33.2 MHz MR and 1.98 MHz MF radar (O-mode), Davis (69°S, 78°E), |
| Meridional | 0.5–0.8 | 0.37–0.85 | 0.8–4.2 | 13.2–28.5 | |||
| Zonal | 0.5–0.8 | 0.48–1.12 | −1–6.8 | 16–25 | 55 MHz MR and 1.98 MHz MF radar (FCA), Buckland Park (34.6°S, 138.5°E) | ||
| Meridional | 0.5–0.8 | 0.8–1.22 | −4–1.5 | 15–23 | |||
| Cervera and Reid (1995) | 80–98 | Zonal | N/A | 0.38 (>90 km), 0.80 (<90 km) | −12.6–5.9 | 11.7–26.5 | Narrow beam MR and 1.98 MHz MF radar, Buckland Park (34.6°S, 138.5°E) |
| Jones et al. (2003) | 80–95 | Zonal | N/A | N/A | 2.0–7.8 | 16.7–26.4 | MR and IDI, Bear Lake Observatory (41.9°N, 111.4°W) |
| Meridional | |||||||
| Liu et al. (2002) | 86, 93 | Zonal | 0.84, 0.95 | 0.816, 1.149 | −1.6, 5.6 | >20, >30 | Lidar and MR, Kirtland Air Force Base (35°N, 106.5°W) |
| Meridional | 0.93, 0.88 | 0.675, 0.801 | −3.1, 4.8 | >20, >20 | |||
| Franke et al. (2005) | 82–98 | Zonal | 0.89 | N/A | −0.2 | N/A | Lidar and MR, Maui (20.75°N, 156.43°W) |
| Meridional | 0.91 | N/A | 0.8 | N/A | |||
| Lee et al. (2021) | 87, 97 | Zonal | 0.92, 0.83 | 0.78, 0.79 | −0.7, −1.1 | >20, >30 | MR and FPI, King Sejong Station (KSS) in Antarctica (62.22°S, 58.79°W) |
| Meridional | 0.88, 0.86 | 0.88, 0.62 | −1.2, −2.9 | >20, >30 | |||
| Gu et al. (2021) | 85–89 | Zonal | 0.76–0.88 | 1.31 | N/A | N/A | FPI and MR, Kunming (25.6°N, 103.8°E) |
| Meridional | 0.75–0.91 | 1.31 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zeng, J.; Yi, W.; Xue, X.; Reid, I.; Hao, X.; Li, N.; Chen, J.; Chen, T.; Dou, X. Comparison between the Mesospheric Winds Observed by Two Collocated Meteor Radars at Low Latitudes. Remote Sens. 2022, 14, 2354. https://doi.org/10.3390/rs14102354
Zeng J, Yi W, Xue X, Reid I, Hao X, Li N, Chen J, Chen T, Dou X. Comparison between the Mesospheric Winds Observed by Two Collocated Meteor Radars at Low Latitudes. Remote Sensing. 2022; 14(10):2354. https://doi.org/10.3390/rs14102354
Chicago/Turabian StyleZeng, Jie, Wen Yi, Xianghui Xue, Iain Reid, Xiaojing Hao, Na Li, Jinsong Chen, Tingdi Chen, and Xiankang Dou. 2022. "Comparison between the Mesospheric Winds Observed by Two Collocated Meteor Radars at Low Latitudes" Remote Sensing 14, no. 10: 2354. https://doi.org/10.3390/rs14102354
APA StyleZeng, J., Yi, W., Xue, X., Reid, I., Hao, X., Li, N., Chen, J., Chen, T., & Dou, X. (2022). Comparison between the Mesospheric Winds Observed by Two Collocated Meteor Radars at Low Latitudes. Remote Sensing, 14(10), 2354. https://doi.org/10.3390/rs14102354






