GNSS Imaging of Strain Rate Changes and Vertical Crustal Motions over the Tibetan Plateau
Abstract
:1. Introduction
2. Datasets and Methods
2.1. GNSS Datasets
2.2. GRACE Datasets
2.3. Strain Rates Estimation
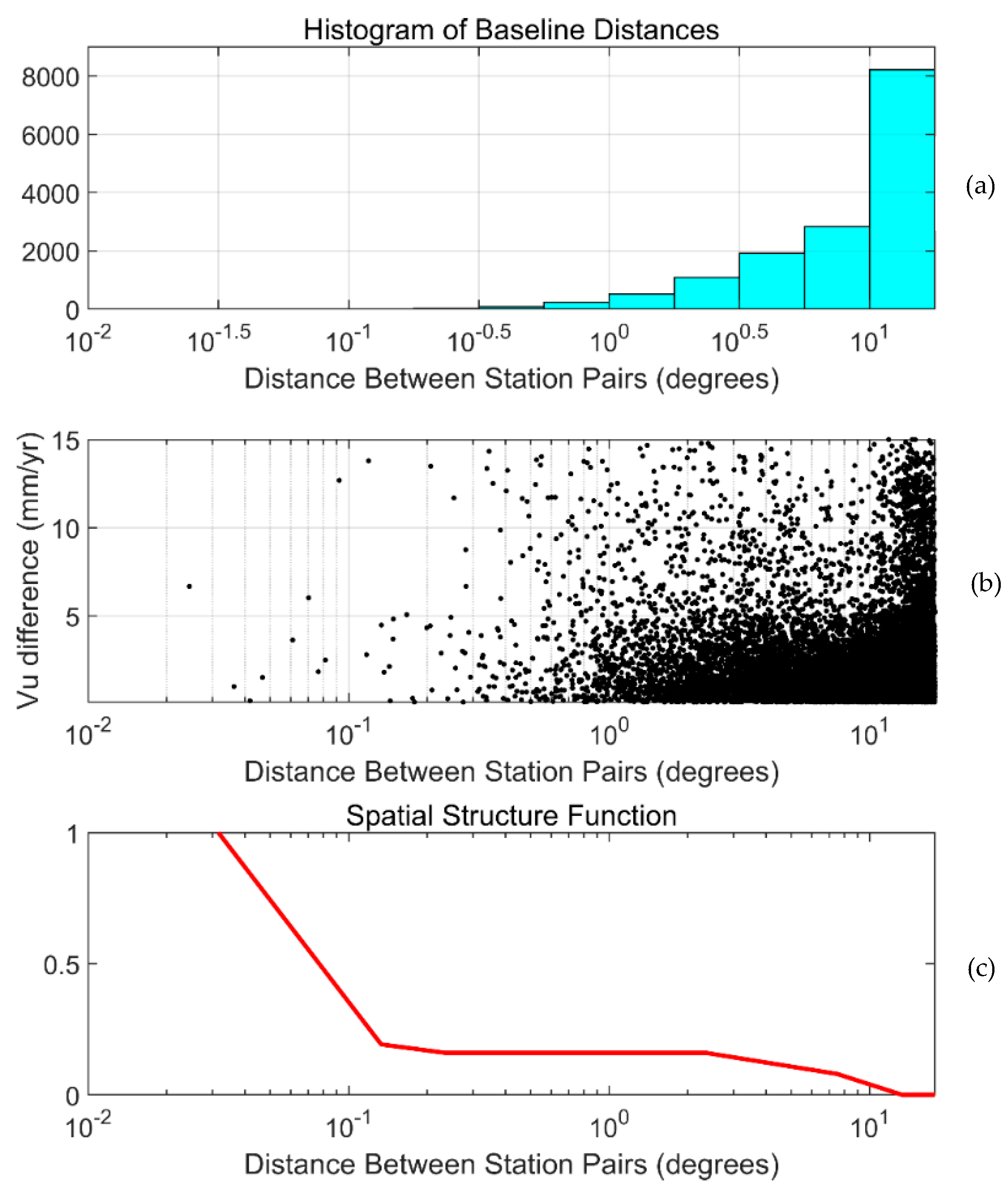
2.4. GNSS Imaging Scheme
- (1)
- Spatial structure function
- (2)
- Median spatial filtering
3. Results
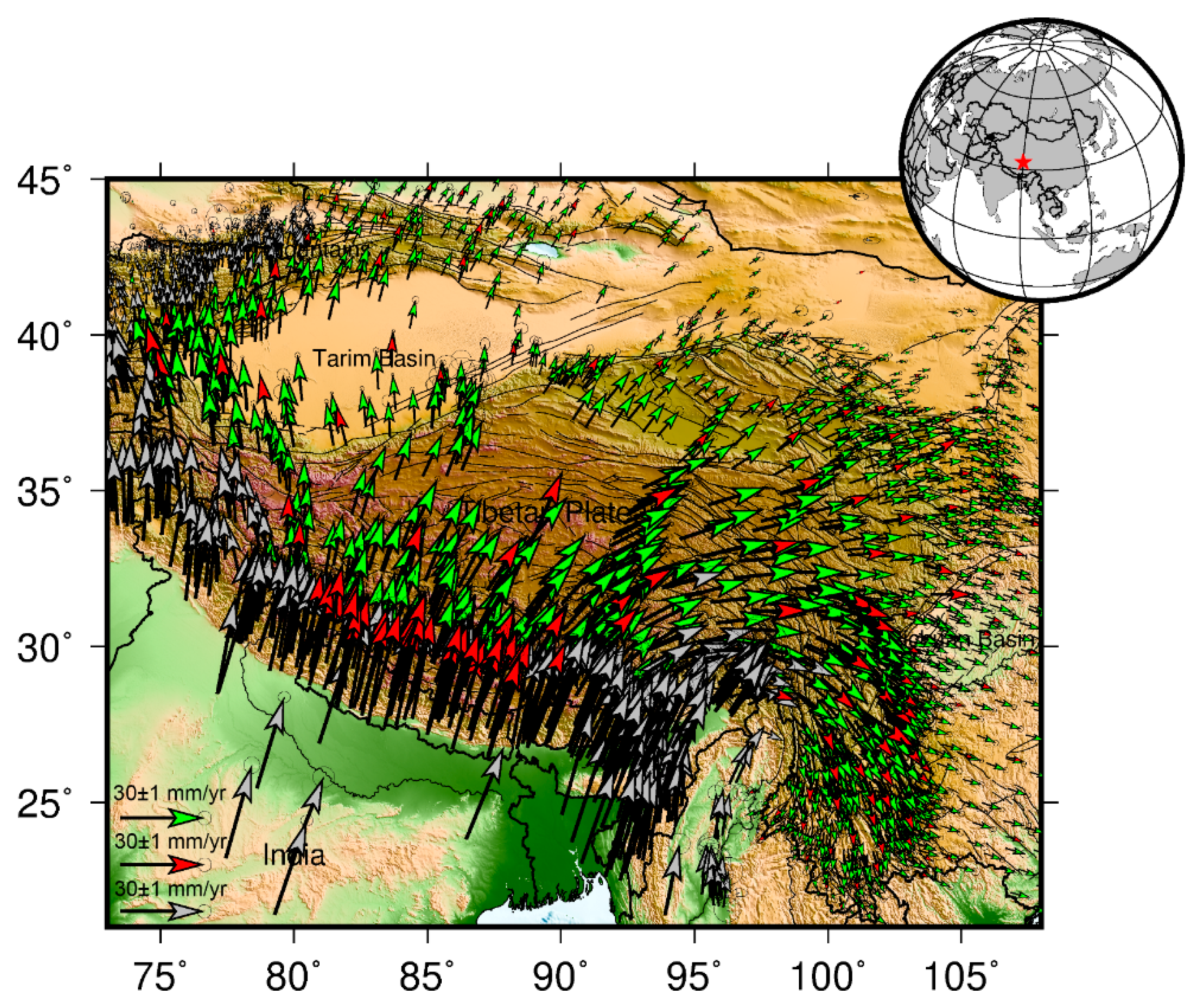
3.1. GNSS Horizontal Velocity Field
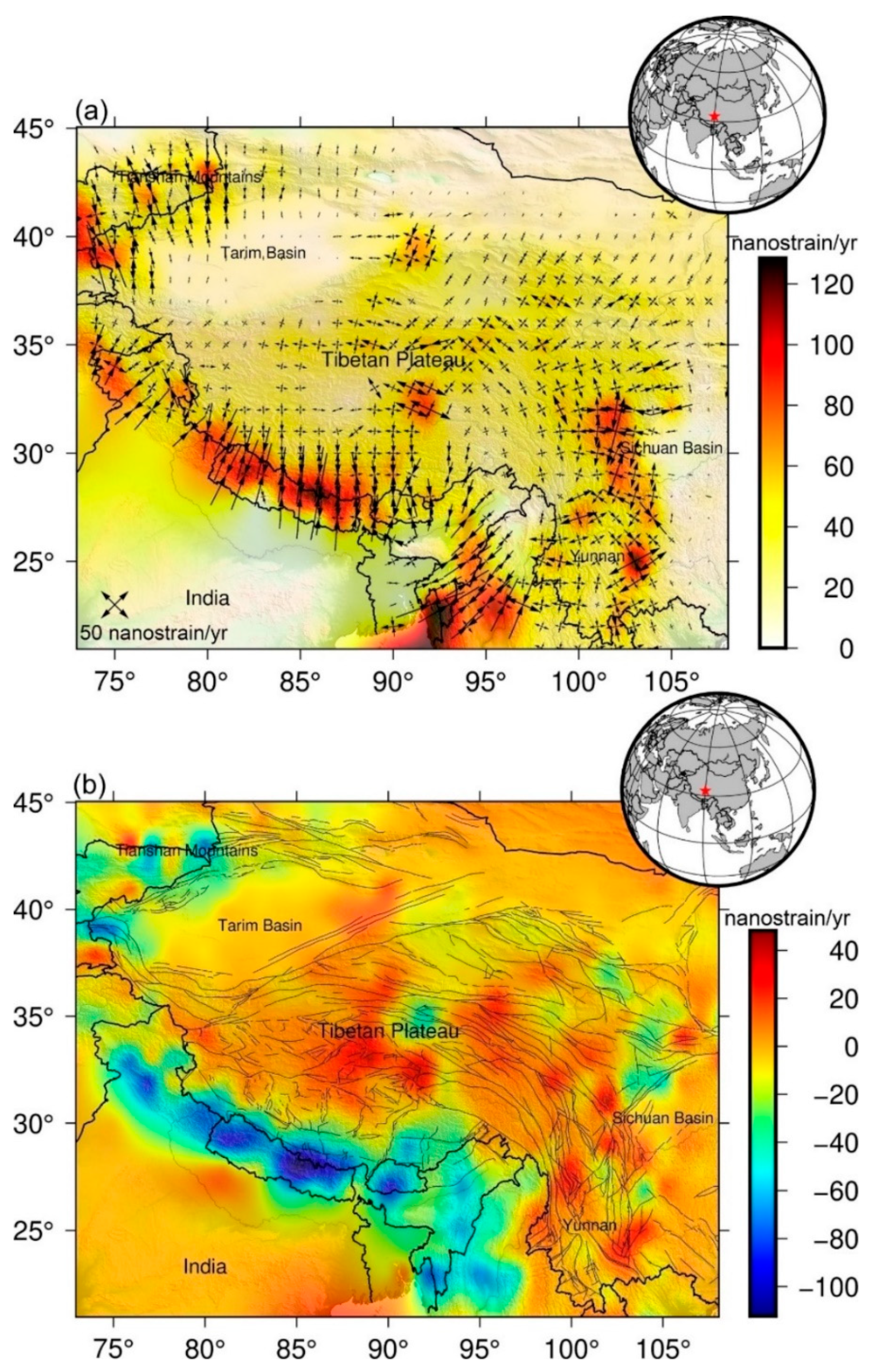
3.2. Strain Rate Changes
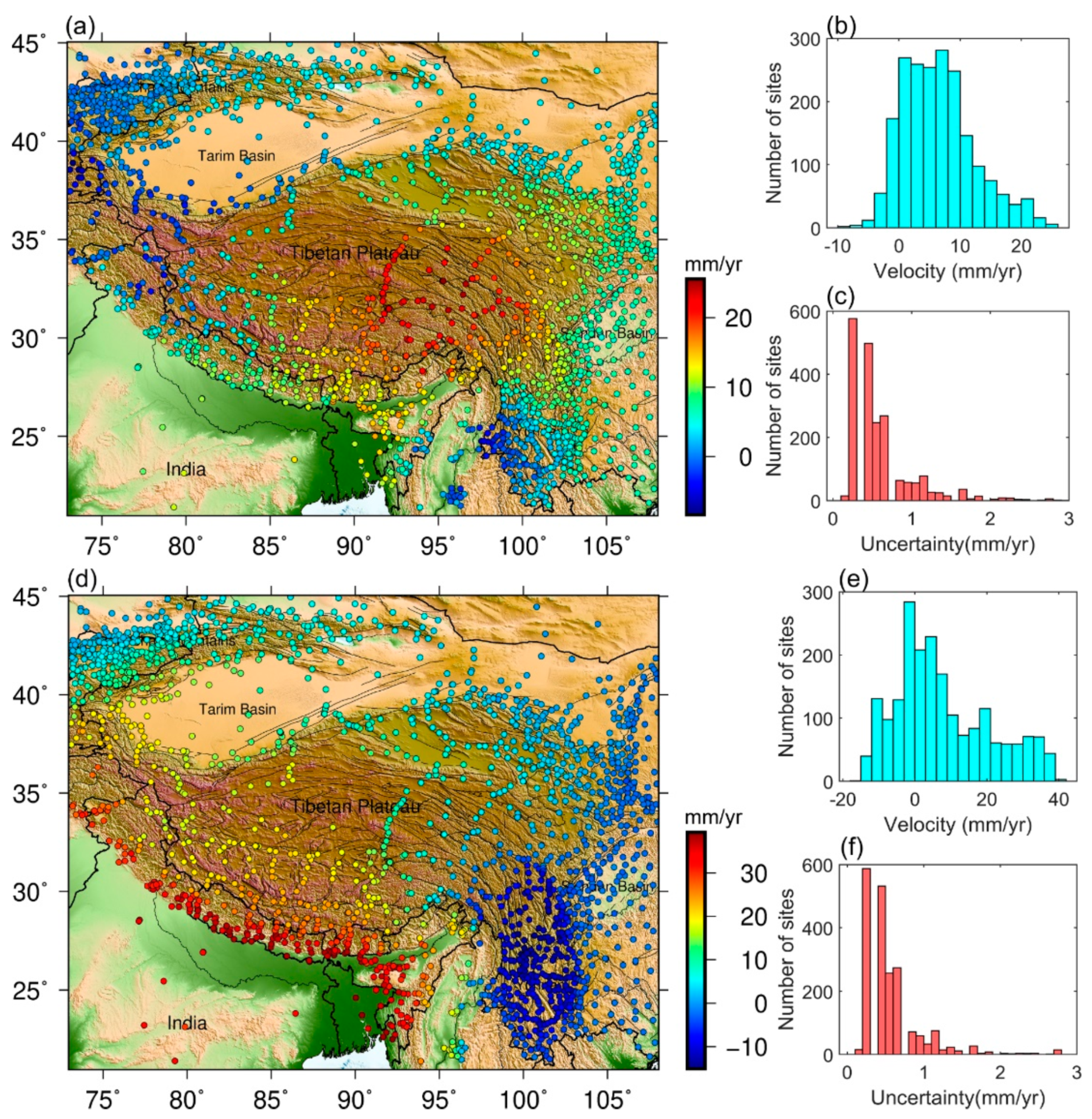
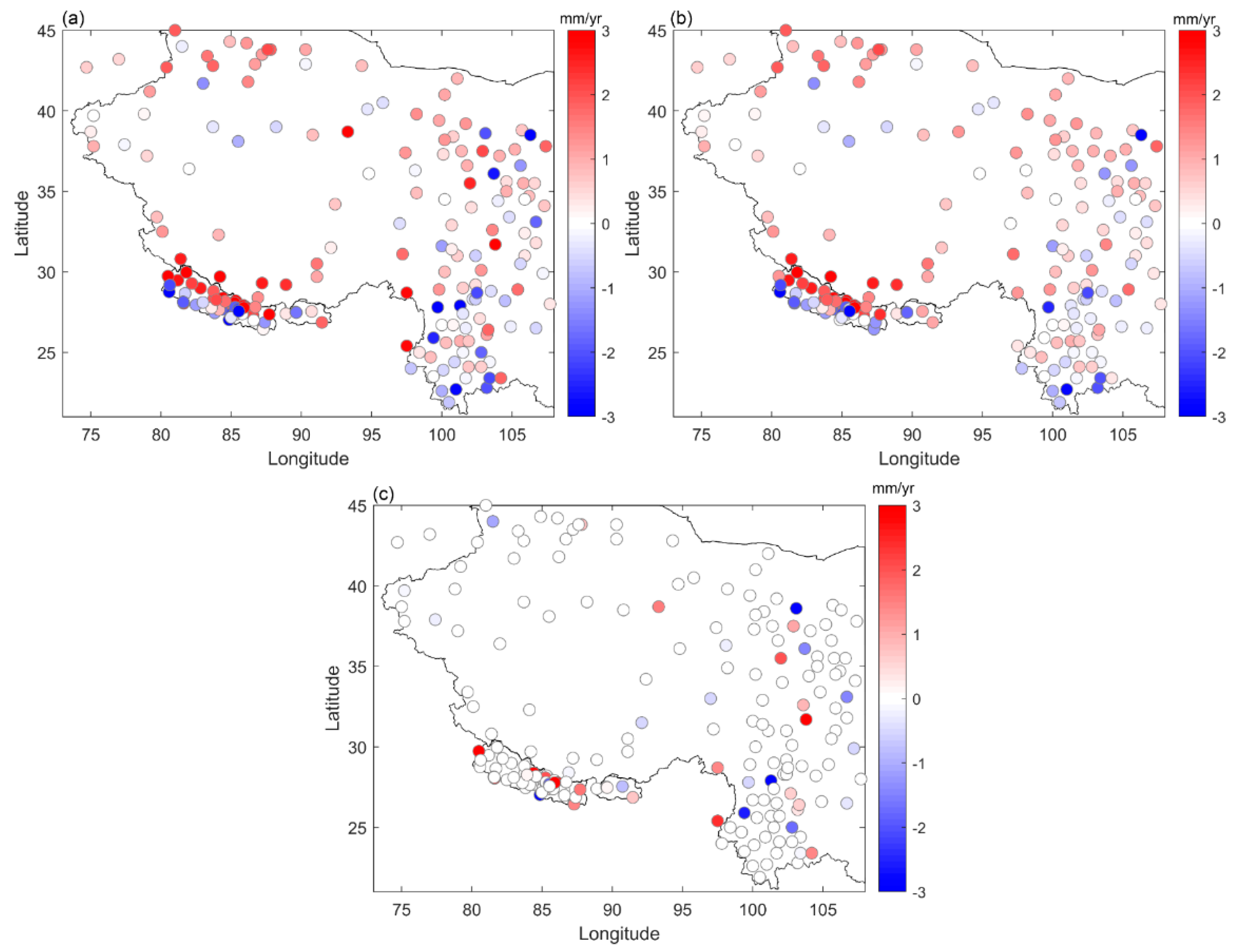
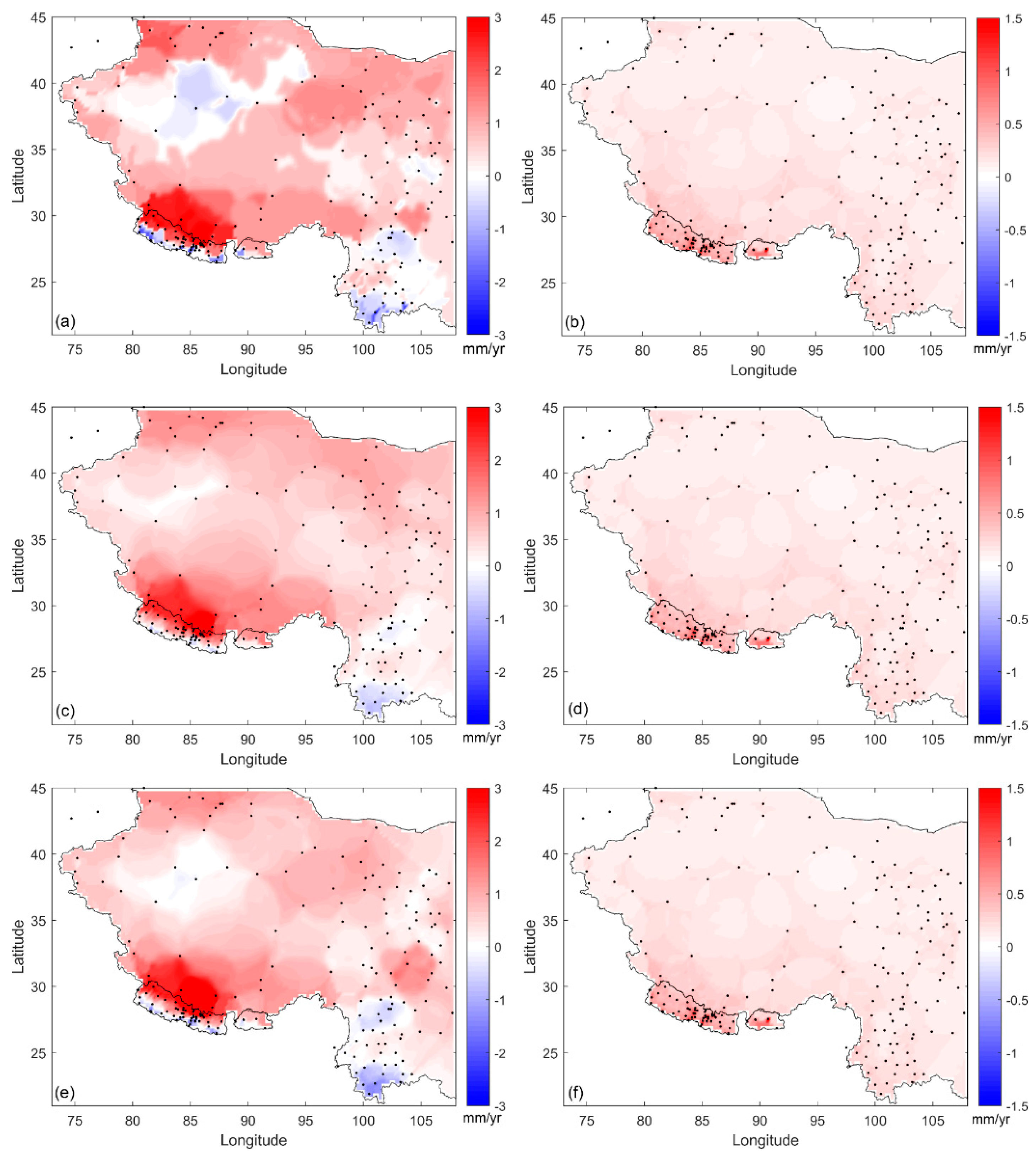
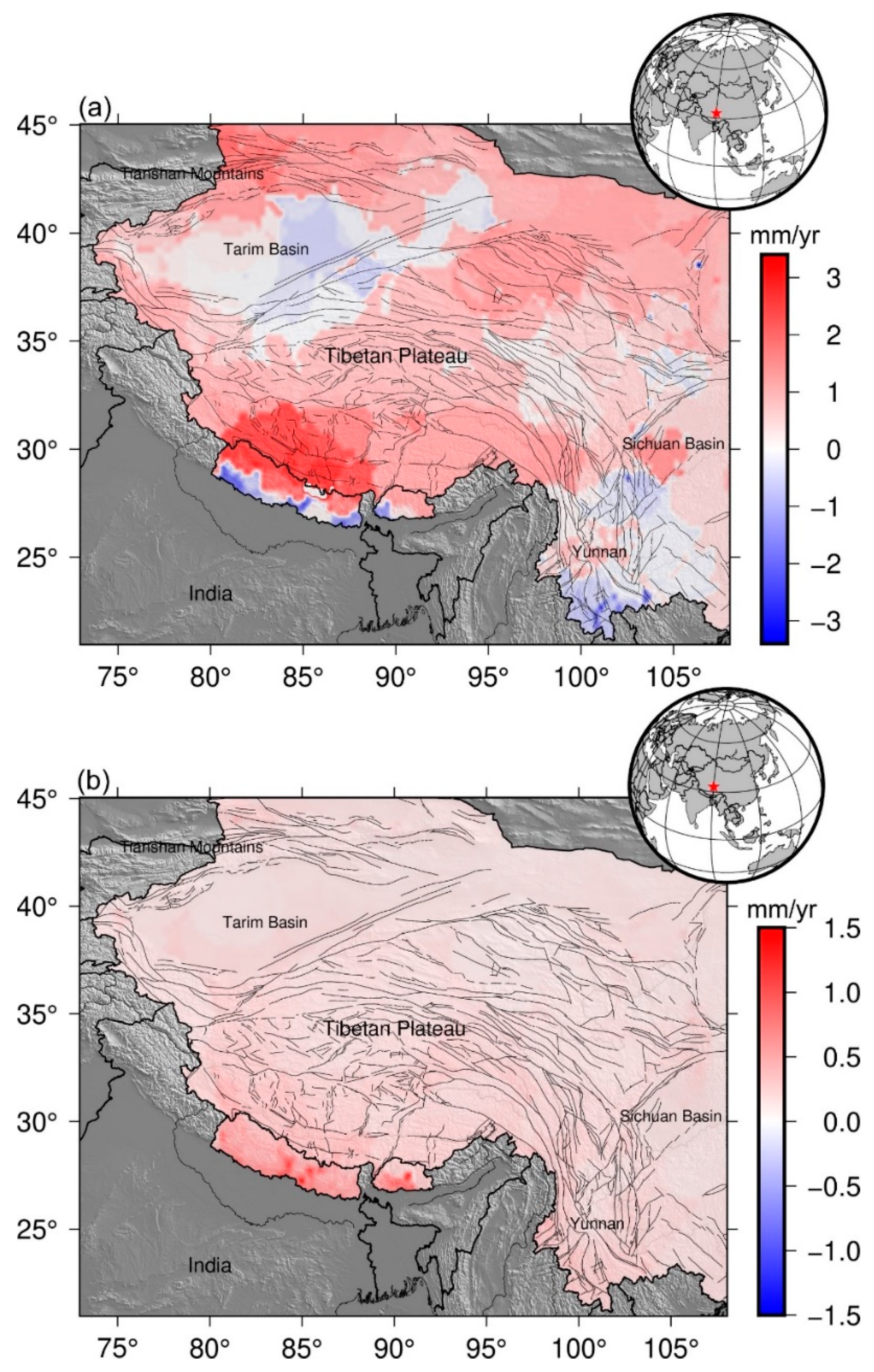
3.3. GNSS Imaging of Vertical Crustal Motions
3.4. Surface Mass Changes over the Tibetan Plateau
4. Discussion
4.1. Tectonic Process of Tibetan Plateau
4.2. Spatial Patterns of Vertical Crustal Motions in the Tibetan Plateau
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rowley, D.B. Age of initiation of collision between India and Asia: A review of stratigraphic data. Earth Planet. Sci. Lett. 1996, 145, 1–13. [Google Scholar] [CrossRef]
- Yin, A.; Harrison, T.M. Geologic evolution of the Himalayan-Tibetan orogen. Annu. Rev. Earth Planet. Sci. 2000, 28, 211–280. [Google Scholar] [CrossRef] [Green Version]
- Royden, L.H.; Burchfiel, B.C.; van der Hilst, R.D. The geological evolution of the Tibetan Plateau. Science 2008, 321, 1054–1058. [Google Scholar] [CrossRef] [Green Version]
- Walters, R.J.; England, P.C.; Houseman, G.A. Constraints from GPS measurements on the dynamics of the zone of convergence between Arabia and Eurasia. J. Geophys. Res. Solid Earth 2017, 122, 1470–1495. [Google Scholar] [CrossRef] [Green Version]
- Wang, Q.; Zhang, P.Z.; Freymueller, J.T.; Bilham, R.; Larson, K.M.; Lai, X.; You, X.; Niu, Z.; Wu, J.; Li, Y.; et al. Present-day crustal deformation in China constrained by Global Positioning System measurements. Science 2001, 294, 574–577. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hu, X.; Garzanti, E.; Wang, J.; Huang, W.; An, W.; Webb, A. The timing of India-Asia collision onset—Facts, theories, controversies. Earth-Sci. Rev. 2016, 160, 264–299. [Google Scholar] [CrossRef]
- Qu, W.; Gao, Y.; Chen, H.; Liang, S.; Han, Y.; Zhang, Q.; Wang, Q.; Hao, M. Review on characteristics of present crustal tectonic movement and deformation in Qinghai-Tibetan Plateau, China using GPS high precision monitoring data. J. Earth Sci. Environ. 2021, 43, 182–204. [Google Scholar]
- Zhang, P.Z.; Shen, Z.K.; Wang, M.; Gan, W.; Burgmann, R.; Molnar, P.; Wang, Q.; Niu, Z.; Sun, J.; Wu, J.; et al. Continuous deformation of the Tibetan Plateau from global positioning system data. Geology 2004, 32, 809–812. [Google Scholar] [CrossRef]
- Zhang, Z.; McCaffrey, R.; Zhang, P. Relative motion across the eastern Tibetan plateau: Contributions from faulting, internal strain and rotation rates. Tectonophysics 2013, 584, 240–256. [Google Scholar] [CrossRef]
- Zhao, B.; Du, R.L.; Zhang, R.; Qiao, X.; Zhang, C.; Tan, K.; Huang, Y. Co-seismic displacements associated with the 2015 Nepal Mw 7.9 Earthquake and Mw 7.3 Aftershock constrained by Global Positioning System measurements. Chin. Sci. Bull. 2015, 60, 2758–2764. [Google Scholar]
- Fan, W.; Shearer, P.M. Detailed rupture imaging of the 25 April 2015 Nepal earthquake using teleseismic P Waves. Geophys. Res. Lett. 2015, 42, 5744–5752. [Google Scholar] [CrossRef]
- Jiang, Z.S.; Yuan, L.G.; Huang, D.F.; Yang, Z.R.; Hassan, A. Postseismic deformation associated with the 2015 Mw 7.8 Gorkha earthquake, Nepal: Investigating ongoing afterslip and constraining crustal rheology. J. Asian Earth Sci. 2018, 156, 1–10. [Google Scholar] [CrossRef]
- Li, C.; Li, Q.; Tan, K.; Lu, X. Coseismic deformation characteristics of the 2020 Nima, Xizang Mw 6.3 earthquake from Sentinel-1A/B InSAR data and rupture slip distribution. Chin. J. Geophys. 2021, 64, 2297–2310. [Google Scholar]
- Thatcher, W. Microplate model for the present-day deformation of Tibet. J. Geophys. Res. Solid Earth 2007, 112, B01401. [Google Scholar] [CrossRef]
- Priestley, K.; Jackson, J.; McKenzie, D. Lithospheric structure and deep earthquakes beneath India, the Himalaya and southern Tibet. Geophys. J. Int. 2008, 172, 345–362. [Google Scholar] [CrossRef] [Green Version]
- Kirby, E.; Harkins, N. Distributed deformation around the eastern tip of the Kunlun Fault. Int. J. Earth Sci. 2013, 102, 1759–1772. [Google Scholar] [CrossRef]
- Gan, W.; Zhang, P.; Shen, Z.K.; Niu, Z.; Wang, M.; Wan, Y.; Zhou, D.; Cheng, J. Present-day crustal motion within the Tibetan Plateau inferred from GPS measurements. J. Geophys. Res. Solid Earth 2007, 112, B08416. [Google Scholar] [CrossRef] [Green Version]
- Liang, S.; Gan, W.; Shen, C.; Xiao, G.; Liu, J.; Chen, W.; Ding, X.; Zhou, D. Three-dimensional velocity field of present-day crustal motion of the Tibetan plateau derived from GPS measurements. J. Geophys. Res. Solid Earth 2013, 118, 5722–5732. [Google Scholar] [CrossRef]
- Kreemer, C.; Blewitt, G.; Klein, E. A geodetic plate motion and Global Strain Rate Model. Geochem. Geophys. Geosyst. 2014, 15, 3849–3889. [Google Scholar] [CrossRef]
- Hao, M.; Freymueller, J.T.; Wang, Q.; Cui, D.; Qin, S. Vertical crustal movement around the southeastern Tibetan Plateau constrained by GPS and GRACE data. Earth Planet. Sci. Lett. 2016, 437, 1–8. [Google Scholar] [CrossRef]
- Zheng, G.; Wang, H.; Wright, T.J.; Lou, Y.; Zhang, R.; Zhang, W.; Shi, C.; Huang, J.; Wei, N. Crustal deformation in the India-Eurasia collision zone from 25 years of GPS measurements. J. Geophys. Res. Solid Earth 2017, 122, 9290–9312. [Google Scholar] [CrossRef]
- Pan, Y.; Shen, W.B.; Shum, C.K.; Chen, R. Spatially varying surface seasonal oscillations and 3-D crustal deformation of the Tibetan Plateau derived from GPS and GRACE data. Earth Planet. Sci. Lett. 2018, 502, 12–22. [Google Scholar] [CrossRef]
- Wang, M.; Shen, Z.K. Present-day crustal deformation of continental China derived from GPS and its tectonic implications. J. Geophys. Res. Solid Earth 2020, 125, e2019JB018774. [Google Scholar] [CrossRef] [Green Version]
- Herring, T.A.; King, R.W.; McClusky, S.C. GAMIT reference manual: GPS analysis at MIT, Release 10.4. Mass. Inst. Technol. Camb. 2010. [Google Scholar]
- Xiang, Y.; Yue, J.; Jiang, Z.; Xing, Y. Spatial-temporal properties of afterslip associated with the 2015 Mw 8.3 Illapel earthquake, Chile. Earth Planets Space 2021, 73, 1–15. [Google Scholar] [CrossRef]
- He, X.; Bos, M.S.; Montillet, J.P.; Fernandes, R.M.S. Investigation of the noise properties at low frequencies in long GNSS time series. J. Geod. 2019, 93, 1271–1282. [Google Scholar] [CrossRef]
- He, X.; Bos, M.S.; Montillet, J.P.; Fernandes, R.M.S.; Melbourne, T.; Jiang, W.; Li, W. Spatial Variations of Stochastic Noise Properties in GPS Time Series. Remote Sens. 2021, 13, 4534. [Google Scholar] [CrossRef]
- He, X.; Yu, K.; Montillet, J.P.; Xiong, C.; Lu, T.; Zhou, S.; Ma, X.; Cui, H.; Ming, F. GNSS-TS-NRS: An Open-source MATLAB-Based GNSS time series noise reduction software. Remote Sens. 2020, 12, 3532. [Google Scholar] [CrossRef]
- Ren, Y.; Lian, L.; Wang, J. Analysis of Seismic Deformation from Global Three-Decade GNSS Displacements: Implications for a Three-Dimensional Earth GNSS Velocity Field. Remote Sens. 2021, 13, 3369. [Google Scholar] [CrossRef]
- Yang, X.; Tian, S.; Feng, W.; Ran, J.; You, W.; Jiang, Z.; Gong, X. Spatio-temporal evaluation of water storage trends from hydrological models over Australia using GRACE mascon solutions. Remote Sens. 2020, 12, 3578. [Google Scholar] [CrossRef]
- Loomis, B.D.; Luthcke, S.B.; Sabaka, T.J. Regularization and error characterization of GRACE mascons. J. Geod. 2019, 93, 1381–1398. [Google Scholar] [CrossRef] [PubMed]
- Shen, Z.K.; Jackson, D.D.; Ge, B.X. Crustal deformation across and beyond the Los Aangeles basin from geodetic measurements. J. Geophys. Res. 1996, 101, 27957–27980. [Google Scholar] [CrossRef]
- Shen, Z.K.; Wang, M.; Zeng, Y.; Wang, F. Optimal interpolation of spatially discretized geodetic data. Bull. Seismol. Soc. Am. 2015, 105, 2117–2127. [Google Scholar] [CrossRef] [Green Version]
- Hammond, W.C.; Blewitt, G.; Kreemer, C. GPS imaging of vertical land motion in California and Nevada: Implications for Sierra Nevada uplift. J. Geophys. Res. Solid Earth 2016, 121, 7681–7703. [Google Scholar] [CrossRef] [PubMed]
- Argus, D.F.; Landerer, F.W.; Wiese, D.N.; Martens, H.R.; Fu, Y.; Famiglietti, J.S.; Thomas, B.F.; Farr, T.G.; Moore, A.W.; Watkins, M.M. Sustained water loss in California’s Mountain ranges during severe drought from 2012 to 2015 inferred from GPS. J. Geophys. Res. Solid Earth 2017, 122, 10559–10585. [Google Scholar] [CrossRef] [Green Version]
- Kreemer, C.; Hammond, W.C.; Blewitt, G. A robust estimation of the 3-D intraplate deformation of the North American plate from GPS. J. Geophys. Res. Solid Earth 2018, 123, 4388–4412. [Google Scholar] [CrossRef]
- Zhou, X.; Yang, Y.; Jiang, W.; Zhou, X. Preliminary spatial-temporal pattern of vertical deformation revealed by GNSS imaging. Chin. J. Geophys. 2020, 63, 155–171. [Google Scholar]
- Pan, Y.; Hammond, W.C.; Ding, H.; Mallick, R.; Jiang, W.; Xu, X.; Shum, C.K.; Shen, W. GPS Imaging of Vertical Bedrock Displacements: Quantification of Two-Dimensional Vertical Crustal Deformation in China. J. Geophys. Res. Solid Earth 2021, 126, 2020JB020951. [Google Scholar] [CrossRef]
- Chew, C.C.; Small, E.E. Terrestrial water storage response to the 2012 drought estimated from GPS vertical position anomalies. Geophys. Res. Lett. 2014, 41, 6145–6151. [Google Scholar] [CrossRef]
- Fu, Y.; Argus, D.F.; Landerer, F.W. Seasonal and interannual variations of water storage in Washington and Oregon estimated from GPS measured surface loading deformation. J. Geophys. Res. Solid Earth 2015, 120, 552–566. [Google Scholar] [CrossRef]
- Zhang, B.; Liu, L.; Khan, S.A.; Dam, T.; Zhang, E.; Yao, Y. Transient variations in glacial mass near Upernavik Isstrøm (west Greenland) detected by the combined use of GPS and GRACE data. J. Geophys. Res. Solid Earth 2017, 122, 10626–10642. [Google Scholar] [CrossRef] [Green Version]
- Zhang, B.; Liu, L.; Yao, Y.; van Dam, T.; Khan, S.A. Improving the estimate of the secular variation of Greenland ice mass in the recent decades by incorporating a stochastic process. Earth Planet. Sci. Lett. 2020, 549, 116518. [Google Scholar] [CrossRef]
- Chen, Z.H.; Burchfiel, B.C.; Liu, Y.; King, R.W.; Royden, L.H.; Tang, W.; Wang, E.; Zhao, J.; Zhang, X. Global Positioning System measurements from eastern Tibet and their implications for India/Eurasia intercontinental deformation. J. Geophys. Res. Solid Earth 2000, 105, 16215–16227. [Google Scholar] [CrossRef]
- Qu, W.; Lu, Z.; Zhang, Q.; Hao, M.; Wang, Q.; Qu, F.; Zhu, W. Present-day crustal deformation characteristics of the southeastern Tibetan Plateau and surrounding areas by using GPS analysis. J. Asian Earth Sci. 2018, 163, 22–31. [Google Scholar] [CrossRef]
- Zhan, W.; Tian, Y.; Zhang, Z.; Zhu, C.; Wang, Y. Seasonal patterns of 3D crustal motions across the seismically active southeastern Tibetan Plateau. J. Asian Earth Sci. 2020, 192, 104274. [Google Scholar] [CrossRef]
- Li, Y.; Liu, M.; Li, Y.; Chen, L. Active crustal deformation in southeastern Tibetan Plateau: The kinematics and dynamics. Earth Planet. Sci. Lett. 2019, 523, 115708. [Google Scholar] [CrossRef]
- Hao, M.; Wang, Q.L.; Shen, Z.K.; Cui, D.X.; Ji, L.Y.; Li, Y.H.; Qin, S.L. Present day crustal vertical movement inferred from precise leveling data in eastern margin of Tibetan Plateau. Tectonophysics 2014, 632, 281–292. [Google Scholar] [CrossRef]
- Zhao, J.Y.; Du, K.F.; Yang, G.H. Vertical motion characteristics of Tianshan and its adjacent areas measured by continuous GPS. Prog. Geophys. 2019, 34, 1835–1841. (In Chinese) [Google Scholar]
- Wang, W.; Qiao, X.; Yang, S.; Wang, D. Present-day velocity field and block kinematics of Tibetan Plateau from GPS measurements. Geophys. J. Int. 2017, 208, 1088–1102. [Google Scholar] [CrossRef]
- Avouac, J.P.; Tapponnier, P.; Bai, M.; You, H.; Wang, G. Active thrusting and folding along the northern Tian Shan and late Cenozoic rotation of the Tarim relative to Dzungaria and Kazakhstan. J. Geophys. Res. Solid Earth 1993, 98, 6755–6804. [Google Scholar] [CrossRef] [Green Version]
- Zubovich, A.V.; Wang, X.Q.; Scherba, Y.G.; Schelochkov, G.G.; Reilinger, R.; Reigber, C.; Mosienko, O.I.; Molnar, P.H.; Michajljow, W.; Makarov, V.I.; et al. GPS velocity field for the Tien Shan and surrounding regions. Tectonics 2010, 29, TC6014. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiang, Y.; Wang, H.; Chen, Y.; Xing, Y. GNSS Imaging of Strain Rate Changes and Vertical Crustal Motions over the Tibetan Plateau. Remote Sens. 2021, 13, 4937. https://doi.org/10.3390/rs13234937
Xiang Y, Wang H, Chen Y, Xing Y. GNSS Imaging of Strain Rate Changes and Vertical Crustal Motions over the Tibetan Plateau. Remote Sensing. 2021; 13(23):4937. https://doi.org/10.3390/rs13234937
Chicago/Turabian StyleXiang, Yunfei, Hao Wang, Yuanyuan Chen, and Yin Xing. 2021. "GNSS Imaging of Strain Rate Changes and Vertical Crustal Motions over the Tibetan Plateau" Remote Sensing 13, no. 23: 4937. https://doi.org/10.3390/rs13234937
APA StyleXiang, Y., Wang, H., Chen, Y., & Xing, Y. (2021). GNSS Imaging of Strain Rate Changes and Vertical Crustal Motions over the Tibetan Plateau. Remote Sensing, 13(23), 4937. https://doi.org/10.3390/rs13234937





