An Integrated Approach for Mapping Three-Dimensional CoSeismic Displacement Fields from Sentinel-1 TOPS Data Based on DInSAR, POT, MAI and BOI Techniques: Application to the 2021 Mw 7.4 Maduo Earthquake
Abstract
:1. Introduction
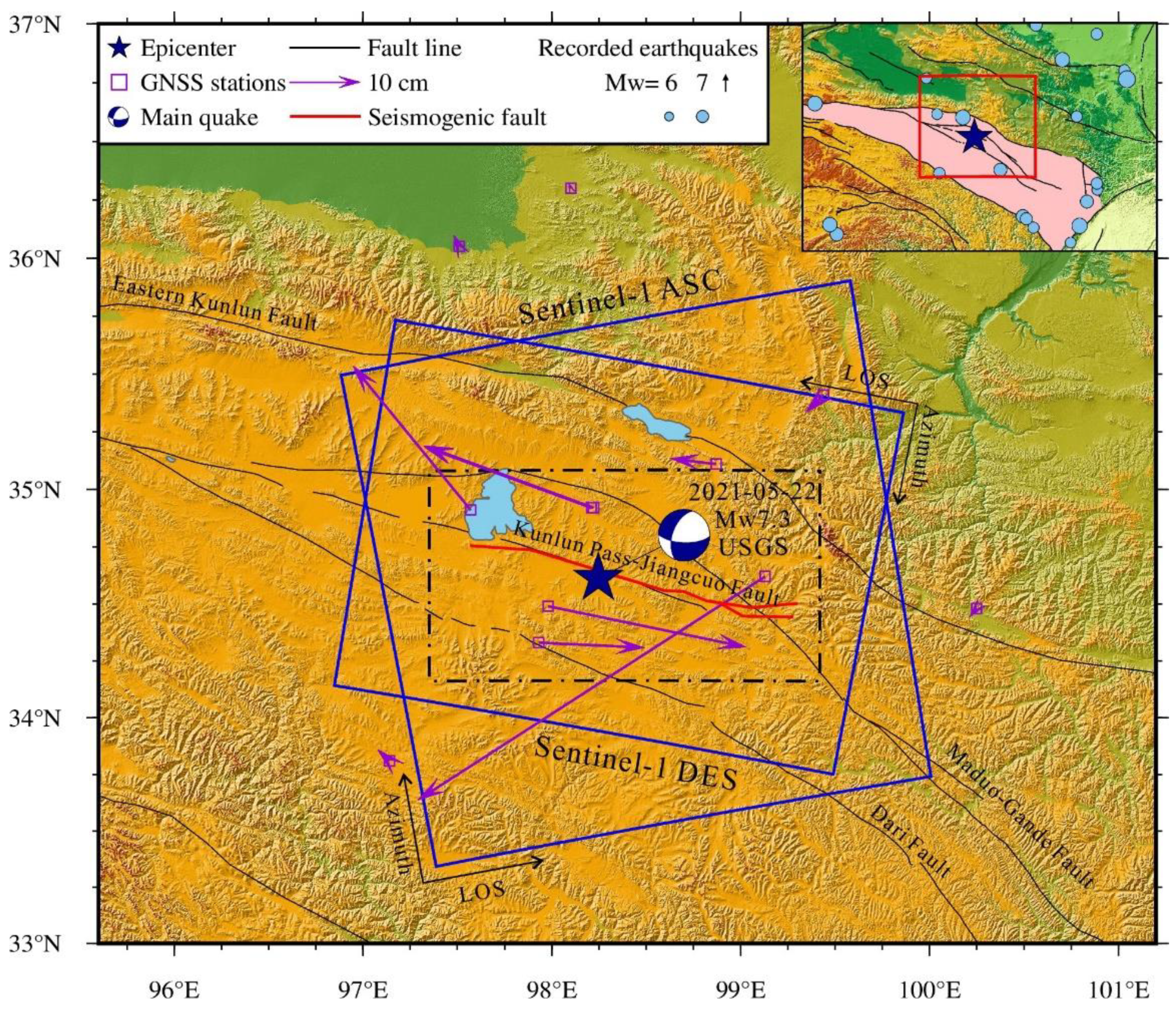
2. Datasets and Processing
2.1. InSAR Data
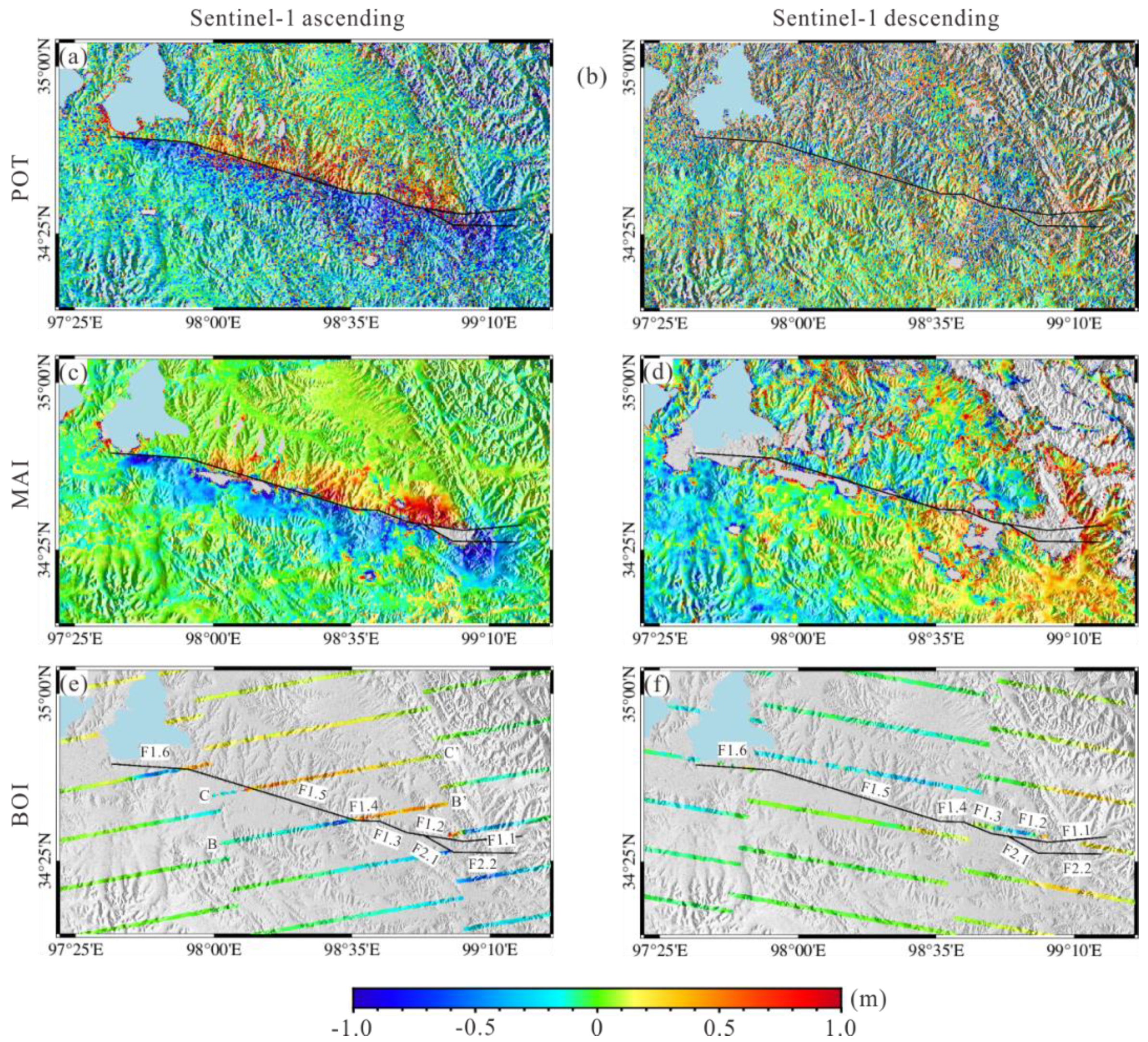
2.2. Across-Track Displacements Derived from DInSAR and POT Techniques
2.3. Along-Track Displacements Derived from POT, MAI and BOI Techniques
2.4. Quality Assessment of the Across- and Along-Track Displacements
3. Methods
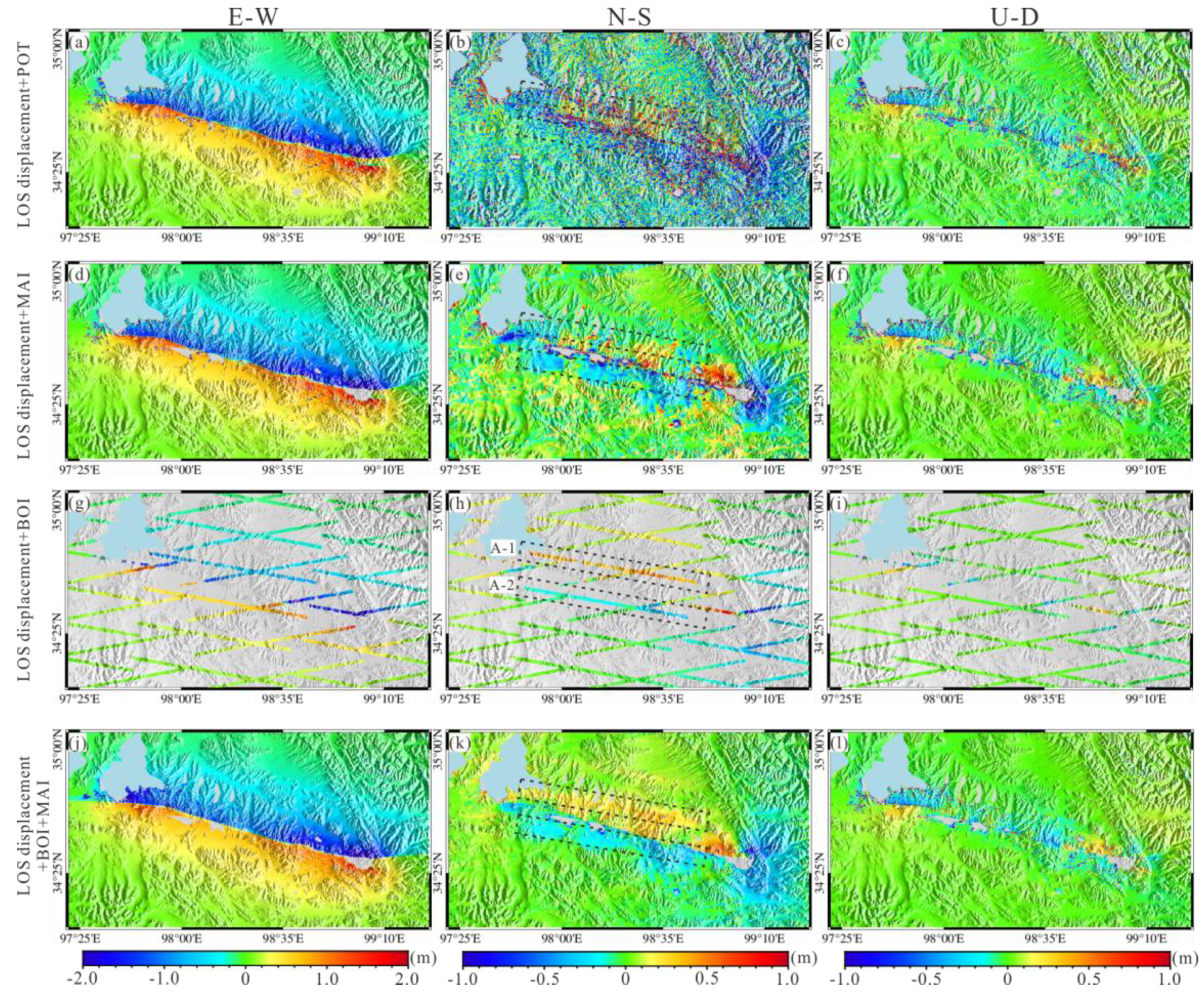
3.1. 3D Displacement Modeling
3.2. Geodetic Modeling
4. Results
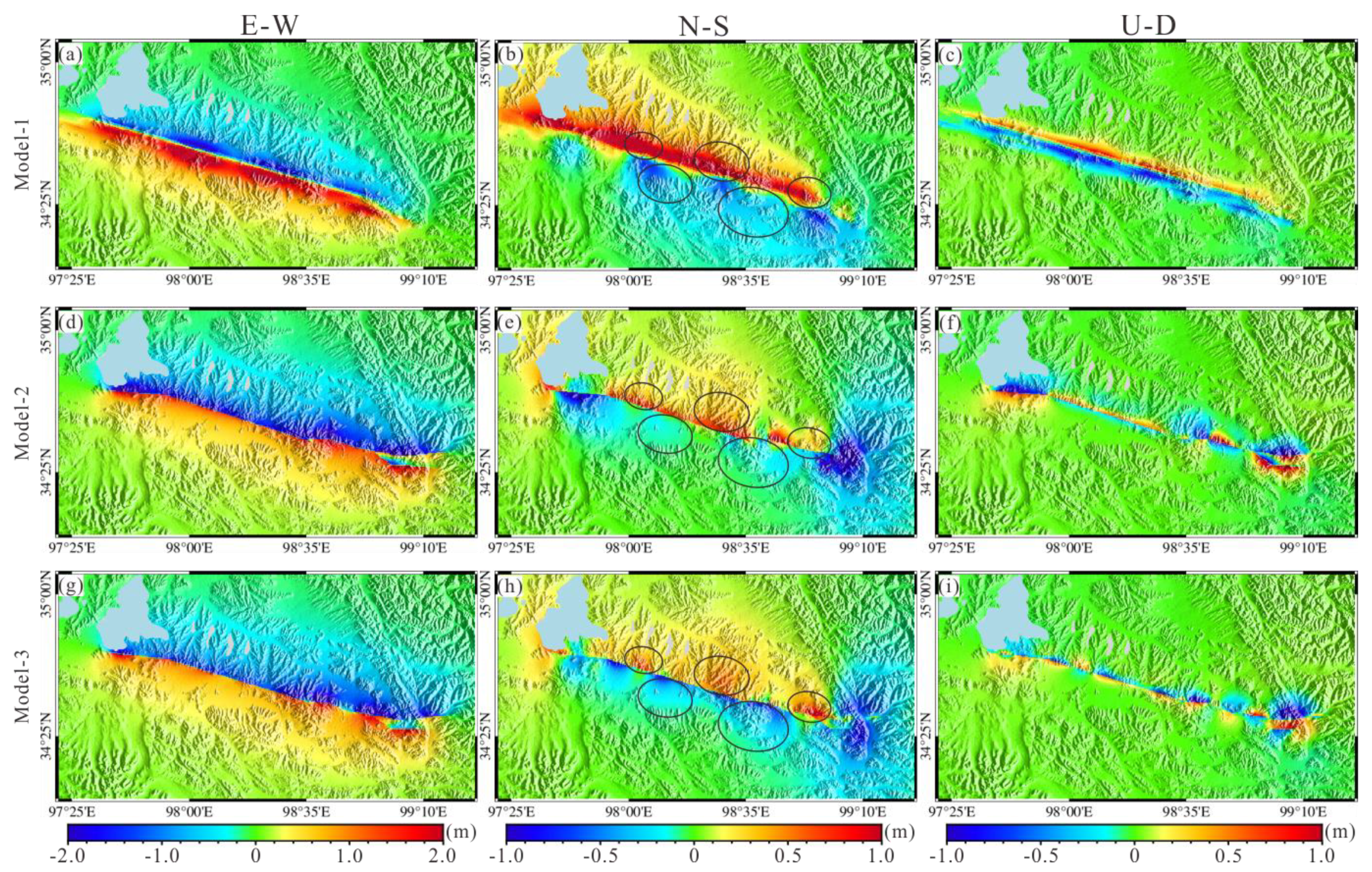
4.1. The 3D Coseismic Displacement Maps of the 2021 Maduo Earthquake
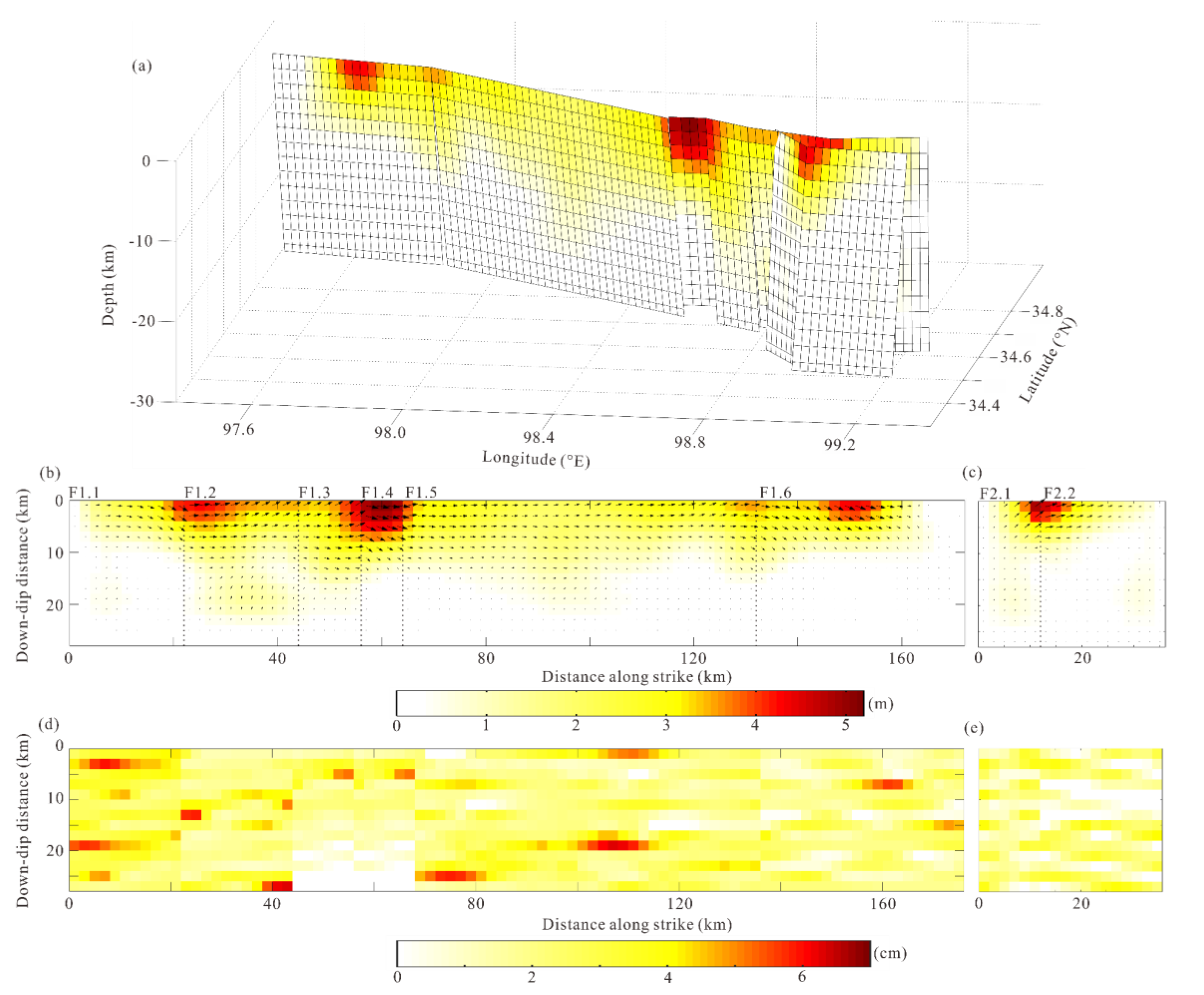
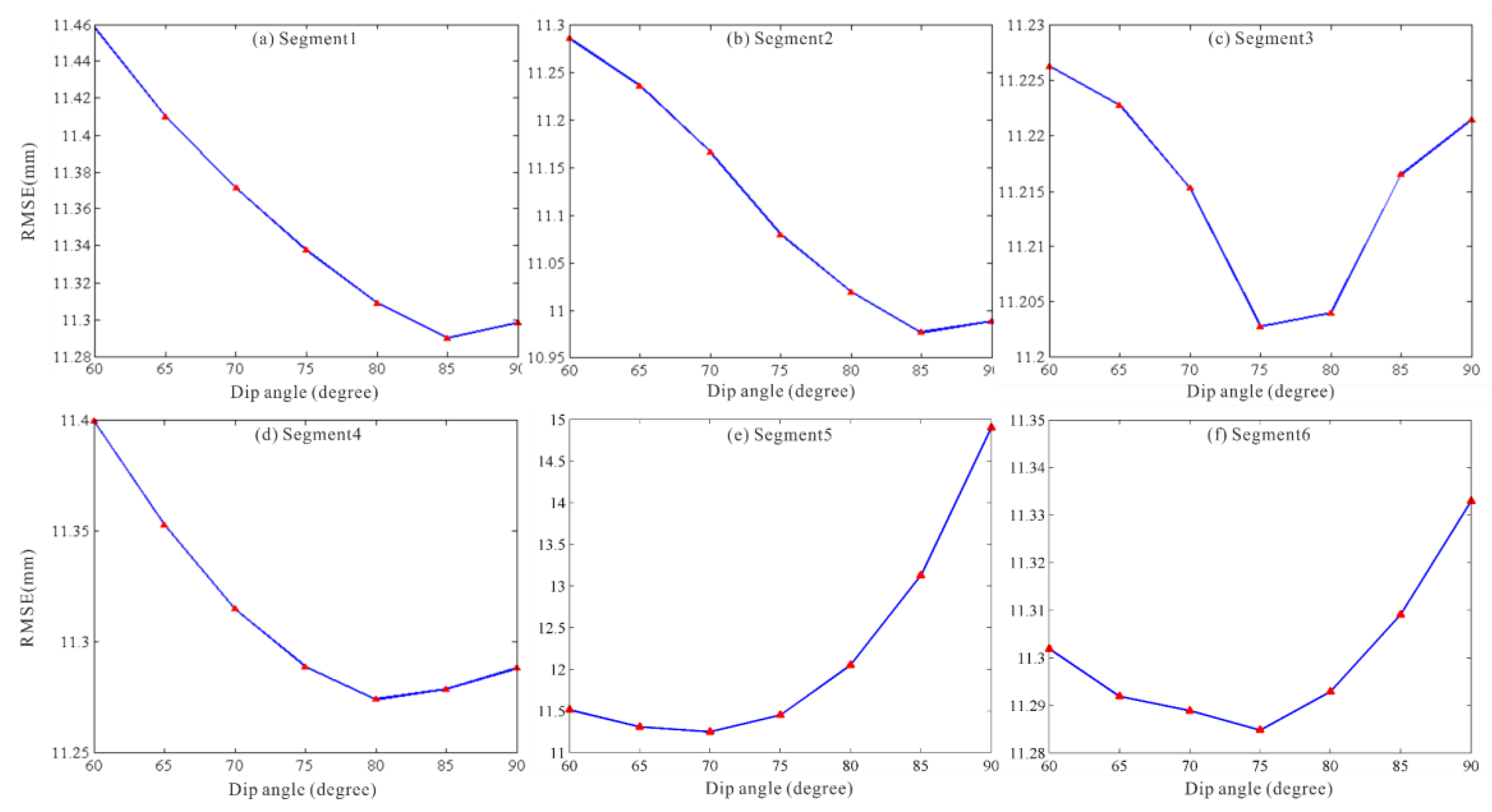
4.2. Fault Geometry and Slip Model
4.3. Coulomb Failure Stress Change
5. Discussion
5.1. Fault Slip Model Estimated by the Joint Utilization of DInSAR and BOI
5.2. Synthetic 3D Displacement Maps
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Biggs, J.; Wright, T.J. How satellite InSAR has grown from opportunistic science to routine monitoring over the last decade. Nat. Commun. 2020, 11, 3863. [Google Scholar] [CrossRef] [PubMed]
- Bischoff, C.A.; Ferretti, A.; Novali, F.; Uttini, A.; Giannico, C.; Meloni, F. Nationwide deformation monitoring with SqueeSAR using Sentinel-1 data. Proc. Int. Assoc. Hydrol. Sci. 2020, 382, 31–37. [Google Scholar] [CrossRef] [Green Version]
- Morishita, Y.; Lazecky, M.; Wright, T.J.; Weiss, J.R.; Elliott, J.R.; Hooper, A. LiCSBAS: An open-source InSAR time series analysis package integrated with the LiCSAR automated Sentinel-1 InSAR processor. Remote. Sens. 2020, 12, 424. [Google Scholar] [CrossRef] [Green Version]
- Wright, T.J.; Parsons, B.E.; Lu, Z. Toward mapping surface deformation in three dimensions using InSAR. Geophys. Res. Lett. 2004, 31, L01607. [Google Scholar] [CrossRef] [Green Version]
- Hu, J.; Li, Z.W.; Ding, X.L.; Zhu, J.J.; Zhang, L.; Sun, Q. Resolving three-dimensional surface displacements from InSAR measurements: A review. Earth-Sci. Rev. 2014, 133, 1–17. [Google Scholar] [CrossRef]
- He, P.; Wen, Y.; Xu, C.; Chen, Y. High-quality three-dimensional displacement fields from new-generation SAR imagery: Application to the 2017 Ezgeleh, Iran, earthquake. J. Geod. 2019, 93, 573–591. [Google Scholar] [CrossRef]
- Fialko, Y.; Simons, M.; Agnew, D. The complete (3-D) surface displacement field in the epicentral area of the 1999 Mw7. 1 Hector Mine earthquake, California, from space geodetic observations. Geophys. Res. Lett. 2001, 28, 3063–3066. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.; Liu, G.; Yu, B.; Dai, K.; Zhang, R.; Ma, D.; Li, Z. An integrated method based on DInSAR, MAI and displacement gradient tensor for mapping the 3D coseismic deformation field related to the 2011 Tarlay earthquake (Myanmar). Remote. Sens. Environ. 2015, 170, 388–404. [Google Scholar] [CrossRef]
- Grandin, R.; Klein, E.; Métois, M.; Vigny, C. Three-dimensional displacement field of the 2015 Mw8. 3 Illapel earthquake (Chile) from across-and along-track Sentinel-1 TOPS interferometry. Geophys. Res. Lett. 2016, 43, 2552–2561. [Google Scholar] [CrossRef] [Green Version]
- Michel, R.; Avouac, J.P.; Taboury, J. Measuring near field coseismic displacements from SAR images: Application to the Landers earthquake. Geophys. Res. Lett. 1999, 26, 3017–3020. [Google Scholar] [CrossRef] [Green Version]
- Jiang, H.; Feng, G.; Wang, T.; Bürgmann, R. Toward full exploitation of coherent and incoherent information in Sentinel-1 TOPS data for retrieving surface displacement: Application to the 2016 Kumamoto (Japan) earthquake. Geophys. Res. Lett. 2017, 44, 1758–1767. [Google Scholar] [CrossRef] [Green Version]
- Casu, F.; Manconi, A.; Pepe, A.; Lanari, R. Deformation time-series generation in areas characterized by large displacement dynamics: The SAR amplitude pixel-offset SBAS technique. IEEE Trans. Geosci. Remote. Sens. 2011, 49, 2752–2763. [Google Scholar] [CrossRef]
- Chen, Q.; Luo, R.; Yang, Y.H.; Yong, Q. Method and Accuracy of Ex tracting Surface Deformation Field from SAR Image Coregistration. Acta Geod. Cartogr. Sin. 2015, 44, 301–308. (In Chinese) [Google Scholar] [CrossRef]
- Bechor, N.B.; Zebker, H.A. Measuring two-dimensional movements using a single InSAR pair. Geophys. Res. Lett. 2006, 33, L16311. [Google Scholar] [CrossRef] [Green Version]
- Jung, H.S.; Won, J.S.; Kim, S.W. An improvement of the performance of multiple-aperture SAR interferometry (MAI). IEEE Trans. Geosci. Remote. Sens. 2009, 47, 2859–2869. [Google Scholar] [CrossRef]
- Jung, H.S.; Lu, Z.; Zhang, L. Feasibility of along-track displacement measurement from Sentinel-1 interferometric wide-swath mode. IEEE Trans. Geosci. Remote. Sens. 2012, 51, 573–578. [Google Scholar] [CrossRef]
- De Zan, F.; Guarnieri, A.M. TOPSAR: Terrain observation by progressive scans. IEEE Trans. Geosci. Remote. Sens. 2006, 44, 2352–2360. [Google Scholar] [CrossRef]
- Liu, J.; Hu, J.; Li, Z.; Ma, Z.; Wu, L.; Jiang, W.; Zhu, J. Complete three-dimensional coseismic displacements related to the 2021 Maduo earthquake in Qinghai Province, China from Sentinel-1 and ALOS-2 SAR images. Sci. Bull. 2021. [Google Scholar] [CrossRef]
- Hooper, A. Large-scale, high-resolution maps of interseismic strain accumulation from Sentinel-1, and incorporation of along-track measurements. In Proceedings of the EGU General Assembly 2021, online, 19–30 April 2021. [Google Scholar] [CrossRef]
- Li, X.; Jónsson, S.; Cao, Y. Interseismic Deformation from Sentinel-1 Burst-Overlap Interferometry: Application to the Southern Dead Sea Fault. Geophys. Res. Lett. 2021, 48, e2021GL093481. [Google Scholar] [CrossRef]
- Li, P.; Liao, L.; Feng, J.; Liu, P. Numerical simulation of relationship between stress evolution and strong earthquakes around the Bayan Har block since 1900. Chin. J. Geophys. 2019, 62, 4170–4188. (In Chinese) [Google Scholar] [CrossRef]
- Pan, J.; Bai, M.; Li, C.; Liu, F.; Li, H.; Liu, D.; Li, C. Coseismic surface rupture and seismogenic structure of the 2021-05-22 Maduo (Qinghai) MS 7.4 earthquake. Acta Geol. Sin. 2021, 95, 1655–1670. [Google Scholar]
- Ren, J.; Zhang, Z.; Gai, H.; Kang, W. Typical Riedel shear structures of the coseismic surface rupture zone produced by the 2021 Mw 7.3 Maduo earthquake, Qinghai, China, and the implications for seismic hazards in the block interior. Nat. Hazards Res. 2021. [Google Scholar] [CrossRef]
- Zhan, Y.; Liang, M.; Sun, X.; Huang, F.; Zhao, L.; Gong, Y. Deep structure and seismogenic pattern of the 2021.5.22 Madoi (Qinghai) Ms7.4 earthquake. Chin. J. Geophys. 2021, 64, 2232–2252. (In Chinese) [Google Scholar] [CrossRef]
- Wang, W.; Fang, L.; Wu, J.; Tu, H.; Chen, L.; Lai, G. Aftershock sequence relocation of the 2021 MS7.4 Maduo Earthquake, Qinghai, China. Sci. China Earth Sci. 2021, 64, 1371–1380. [Google Scholar] [CrossRef]
- U.S. Geological Survey (USGS). M7.3-Southern Qinghai, China. 2021. Available online: https://earthquake.usgs.gov/earthquakes/eventpage/us7000e54r/executive (accessed on 7 June 2021).
- Li, Z.C.; Ding, K.H.; Zhang, P.; Wen, Y.; Zhao, L.; Chen, J. Co-seismic deformation and slip distribution of 2021 M W 7.4 Madoiearthquake from GNSS observation. Geomat. Inf. Sci. Wuhan Univ. 2021, 46, 1489–1497. [Google Scholar] [CrossRef]
- Wegmüller, U.; Werner, C. Gamma SAR Processor and Interferometry Software; ESA SP (Print); ESA Publications Division: Noordwijk, The Netherlands, 1997; pp. 1687–1692. [Google Scholar]
- Scheiber, R.; Moreira, A. Coregistration of interferometric SAR images using spectral diversity. IEEE Trans. Geosci. Remote. Sens. 2000, 38, 2179–2191. [Google Scholar] [CrossRef]
- Liu, G.X.; Ding, X.L.; Li, Z.L.; Chen, Y.Q.; Zhang, G.B. Co-registration of satellite SAR complex images. Acta Geod. Cartogr. Sin. 2001, 30, 60–66. [Google Scholar]
- Prats-Iraola, P.; Scheiber, R.; Marotti, L.; Wollstadt, S.; Reigber, A. TOPS interferometry with TerraSAR-X. IEEE Trans. Geosci. Remote. Sens. 2012, 50, 3179–3188. [Google Scholar] [CrossRef] [Green Version]
- Ma, Z.F.; Jiang, M.; Huang, T. A sequential approach for Sentinel-1 TOPS time-series co-registration over low coherence scenarios. IEEE Trans. Geosci. Remote. Sens. 2020, 59, 4818–4826. [Google Scholar] [CrossRef]
- Goldstein, R.M.; Werner, C.L. Radar interferogram filtering for geophysical applications. Geophys. Res. Lett. 1998, 25, 4035–4038. [Google Scholar] [CrossRef] [Green Version]
- Chen, C.W.; Zebker, H.A. Network approaches to two-dimensional phase unwrapping: Intractability and two new algorithms. JOSA A 2000, 17, 401–414. [Google Scholar] [CrossRef]
- Simons, M.; Fialko, Y.; Rivera, L. Coseismic deformation from the 1999 M w 7.1 Hector Mine, California, earthquake as inferred from InSAR and GPS observations. Bulletin of the Seismological. Soc. Am. 2002, 92, 1390–1402. [Google Scholar] [CrossRef]
- Hu, J.; Li, Z.W.; Ding, X.L.; Zhu, J.J.; Zhang, L.; Sun, Q. 3D coseismic displacement of 2010 Darfield, New Zealand earthquake estimated from multi-aperture InSAR and D-InSAR measurements. J. Geod. 2012, 86, 1029–1041. [Google Scholar] [CrossRef]
- Welstead, S.T. Fractal and Wavelet Image Compression Techniques; SPIE Optical Engineering Press: Bellingham, WA, USA, 1999. [Google Scholar]
- Okada, Y. Surface deformation to shear and tensile fault in a half-space. Bull. Seismol. Soc. Am. 1985, 75, 1135–1154. [Google Scholar] [CrossRef]
- Yang, Y.; Chen, Q.; Xu, Q.; Liu, G.; Hu, J.C. Source model and Coulomb stress change of the 2015 Mw 7.8 Gorkha earthquake determined from improved inversion of geodetic surface deformation observations. J. Geod. 2019, 93, 333–351. [Google Scholar] [CrossRef]
- Yang, Y.H.; Chen, Q.; Xu, Q.; Zhao, J.J.; Hu, J.C.; Li, H.L.; Xu, L. Comprehensive Investigation of Capabilities of the Left-Looking InSAR Observations in Coseismic Surface Deformation Mapping and Faulting Model Estimation Using Multi-Pass Measurements: An Example of the 2016 Kumamoto, Japan Earthquake. Remote. Sens. 2021, 13, 2034. [Google Scholar] [CrossRef]
- Hanks, T.H. Earthquake stress-drops, ambient tectonic stresses, and the stresses that drive plates. Pure Appl. Geophys. 1997, 115, 441–458. [Google Scholar] [CrossRef]
- Harris, R.A. Introduction to special session: Stress triggers, stress shadows, and implications for seismic hazard. J. Geophys. Res. 1998, 103, 24347–24358. [Google Scholar] [CrossRef]
- Styron, R.; Marco, P. The GEM Global Active Faults Database. Earthq. Spectra 2020, 36, 160–180. [Google Scholar] [CrossRef]





| Sensor | OrbitDirection | Acquisition Time | Perp. Baseline (m) | Heading Angle (°) | Incidence Angle (°) | Imaging Mode |
|---|---|---|---|---|---|---|
| Sentinel-1A/B | Ascending | 20 May 2021–26 May 2021 | 55 | −12.9 | 39.2 | TOPS |
| Sentinel-1A | Descending | 20 May 2021–26 May 2021 | 29 | −167.0 | 39.1 | TOPS |
| Methods | Ascending Orbit | Descending Orbit | |||
|---|---|---|---|---|---|
| mean | std | mean | std | ||
| Across-track displacements | DInSAR | 0.8 | 2.8 | −0.3 | 2.9 |
| POT | 1.3 | 8.5 | 3.2 | 14.6 | |
| Along-track displacements | POT | 18.3 | 42.4 | 6.3 | 98.4 |
| MAI | 1.5 | 21.1 | 3.7 | 66.1 | |
| BOI | 0.5 | 4.3 | 1 | 3.6 | |
| Datasets | E–W | N–S | U–D | |
|---|---|---|---|---|
| RMSEs | ||||
| LOS displacement + POT | 3.9 | 21.3 | 2.7 | |
| LOS displacement + MAI | 4.0 | 13.6 | 1.5 | |
| LOS displacement + BOI | 6.6 | 5.5 | 2.4 | |
| LOS displacement + BOI + MAI | 5.8 | 6.3 | 1.7 | |
| Constraint Data | Seismic Fault | Strike Angle (°) | Dip Angle (°) | Rake Angle (°) |
|---|---|---|---|---|
| DInSAR observations | F1 | 263.9 ± 3.1 | 85.7 ± 3.8 | 3.6 |
| 280.5 ± 2.7 | 87.2 ± 3.2 | |||
| 287.2 ± 2.9 | 77.2 ± 3.3 | |||
| 271.3 ± 3.5 | 80.3 ± 3.7 | |||
| 288.0 ± 1.5 | 71.5 ± 2.1 | |||
| 275.0 ± 1.3 | 75.4 ± 2.4 | |||
| F2 | 126.4 ± 2.5 | 84.7 ± 2.3 | 5.4 | |
| 93.2 ± 2.1 | 71.0 ± 1.9 |
| Seismic Fault | Strike Angle (°) | Dip Angle (°) | Rake Angle (°) | |
|---|---|---|---|---|
| DInSAR +BOI observations | F1 | 262.0 + 3.6 | 82.8 + 4.0 | 5.8 |
| 278.3 + 3.2 | 84.4 + 3.8 | |||
| 289.8 + 3.7 | 79.4 + 3.5 | |||
| 272.8 + 3.4 | 78.2 + 2.9 | |||
| 286.3 + 2.6 | 73.2 + 3.4 | |||
| 277.1 + 2.1 | 77.7 + 3.2 | |||
| F2 | 124.8 + 2.8 | 83.3 + 3.1 | −19.5 | |
| 92.3 + 2.6 | 73.2 + 3.3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, L.; Chen, Q.; Zhao, J.-J.; Liu, X.-W.; Xu, Q.; Yang, Y.-H. An Integrated Approach for Mapping Three-Dimensional CoSeismic Displacement Fields from Sentinel-1 TOPS Data Based on DInSAR, POT, MAI and BOI Techniques: Application to the 2021 Mw 7.4 Maduo Earthquake. Remote Sens. 2021, 13, 4847. https://doi.org/10.3390/rs13234847
Xu L, Chen Q, Zhao J-J, Liu X-W, Xu Q, Yang Y-H. An Integrated Approach for Mapping Three-Dimensional CoSeismic Displacement Fields from Sentinel-1 TOPS Data Based on DInSAR, POT, MAI and BOI Techniques: Application to the 2021 Mw 7.4 Maduo Earthquake. Remote Sensing. 2021; 13(23):4847. https://doi.org/10.3390/rs13234847
Chicago/Turabian StyleXu, Lang, Qiang Chen, Jing-Jing Zhao, Xian-Wen Liu, Qian Xu, and Ying-Hui Yang. 2021. "An Integrated Approach for Mapping Three-Dimensional CoSeismic Displacement Fields from Sentinel-1 TOPS Data Based on DInSAR, POT, MAI and BOI Techniques: Application to the 2021 Mw 7.4 Maduo Earthquake" Remote Sensing 13, no. 23: 4847. https://doi.org/10.3390/rs13234847
APA StyleXu, L., Chen, Q., Zhao, J.-J., Liu, X.-W., Xu, Q., & Yang, Y.-H. (2021). An Integrated Approach for Mapping Three-Dimensional CoSeismic Displacement Fields from Sentinel-1 TOPS Data Based on DInSAR, POT, MAI and BOI Techniques: Application to the 2021 Mw 7.4 Maduo Earthquake. Remote Sensing, 13(23), 4847. https://doi.org/10.3390/rs13234847





