Spatiotemporal Trends and Variations of the Rainfall Amount, Intensity, and Frequency in TRMM Multi-satellite Precipitation Analysis (TMPA) Data
Abstract
1. Introduction
2. Data and Method
2.1. TRMM 3B42V7 Data
2.2. Precipitation Parameters
- (1)
- The mean rain rate (MR) is defined by the total rainfall amount A over the study region and time period divided by the total number of data pixels (or time of observations) accumulated within the study region and period , based on Equation (1):
- (2)
- The rain frequency (RF) is the frequency of the precipitation occurrence within the study region and period, which is defined as Equation (2):where is the number of pixels that have a rain rate larger than 0.
- (3)
- The conditional rain rate (CR) is the average rainfall density within the rainy pixels (rain rate > 0), which can be expressed as Equation (3):
2.3. Analytical Method
- (1)
- The seasonal timeseries of anomalies (obtained by subtracting the seasonal mean over the study period from the parameter value in the corresponding season) and spatiotemporal means of each precipitation parameter are calculated for the land, ocean, and globe, respectively. The linear trends of these timeseries are computed and their significance levels are examined using the Null Hypothesis Test (NHT).
- (2)
- The spatial patterns of the trend for each precipitation parameter are analyzed through the investigation of its trend in every 0.25° grid point of TRMM data. In this step, only trends that pass the 0.05 significance level are visualized and analyzed.
- (3)
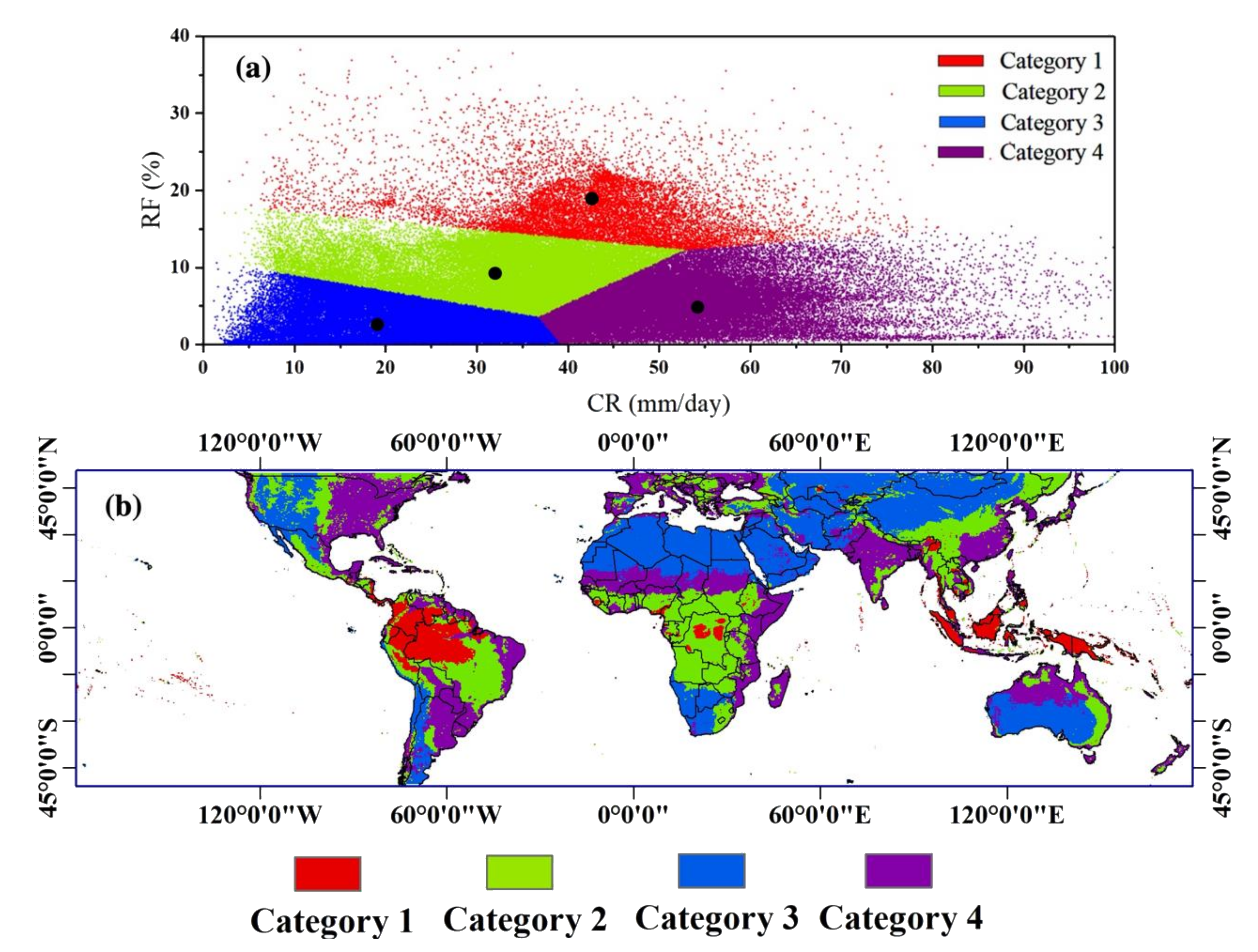
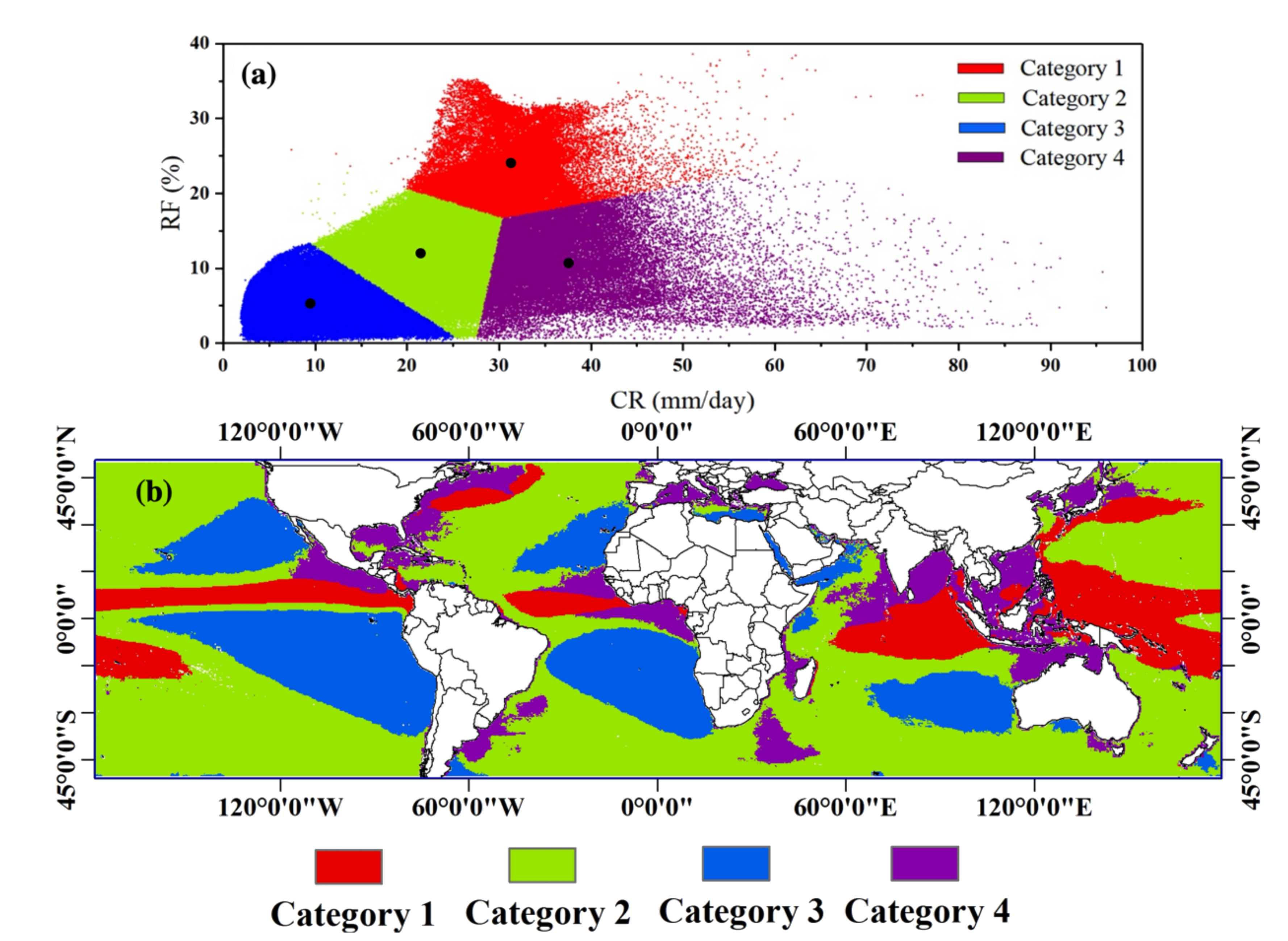
- Clustering analysis is performed to categorize precipitation over land and ocean separately based on the covariability of CR and RF using the K-means method. The K-means algorithm divides a set of N samples X = (…,) into k (k N) disjoint clusters C, in which each observation belongs to the cluster with the nearest mean [32], and means are usually called the cluster centroids. The K-means clustering aims to choose centroids that minimize the inertia or sum-of-squares criterion within the cluster, using Equation (1):land and ocean are clustered into four optimized categories separately.
- (4)
- Empirical orthogonal function (EOF) analysis is conducted on the seasonal anomalies of the parameters to confirm their dominant pattern and factors that introduce these patterns. The EOF analysis decomposes a spatiotemporal dataset into a set of orthogonal functions to represent the times series and spatial distribution [33] according to Equation (2):where Z (x, y, t) is the original space/time dataset of precipitation data as a function of time (t) and space (x, y). EOFk (x, y) is the kth spatial structures that correspond to the kth PCk(t) principal component that shows the evolution of the pattern over time. The covariance matrix is decomposed using singular-value decomposition (SVD) to calculate the EOFs and associated PCs according to Equation (3):where Z (n, t) is the data matrix, the columns of U (n by n matrix) are eigenvectors (EOFs); the columns of V (t by t matrix) are the PCs; the diagonal values of Σ are the eigenvalues represent the amplitudes of the EOFs. In this paper, n is the number of pixels in each TMPA image, and x and y are the pixel numbers in horizontal (longitude) and vertical (latitude) dimensions, respectively (x = 1440, y = 400).
3. Results
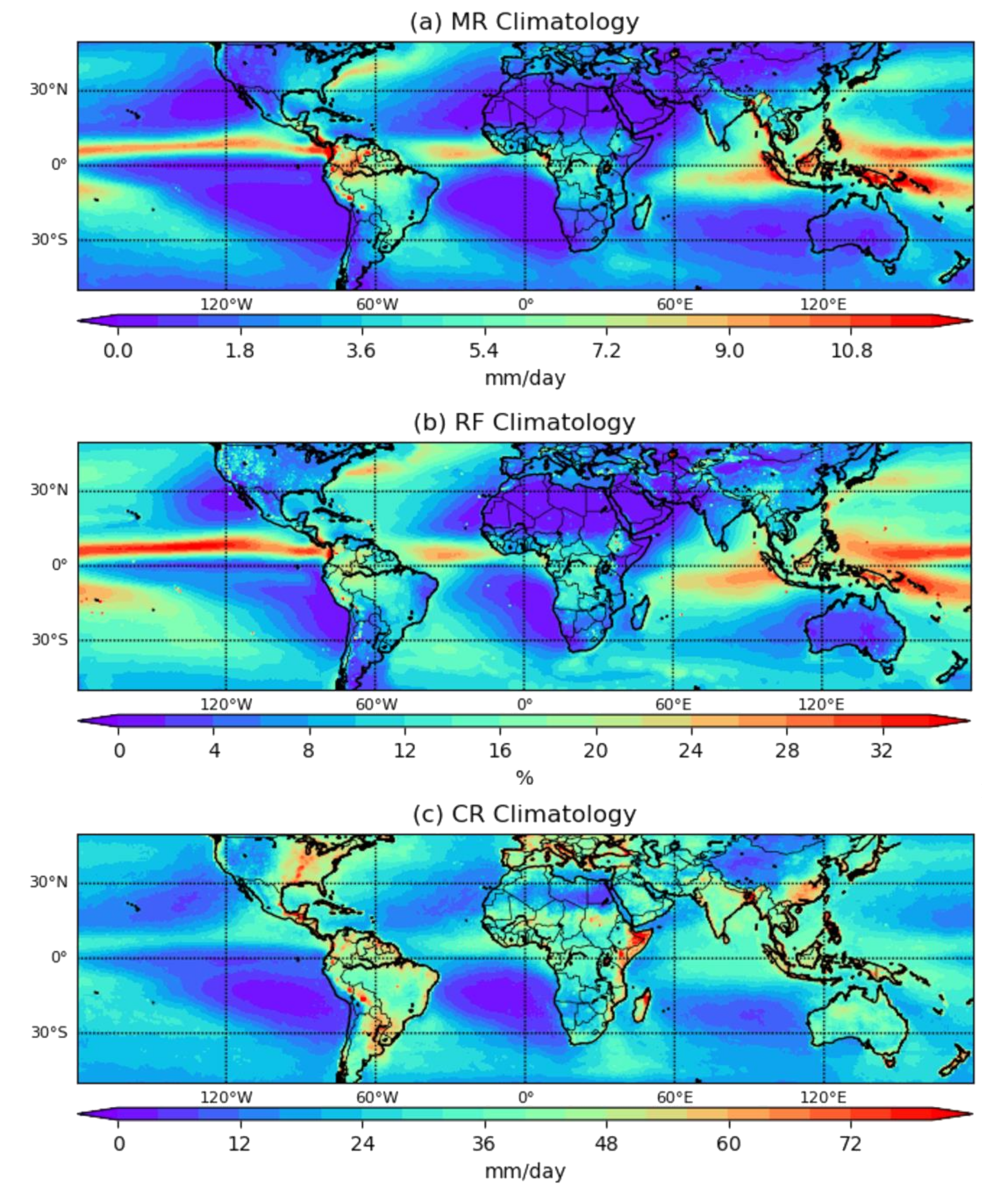
3.1. Global Means
3.2. Spatial Patterns of Trends
3.3. Precipitation Categories Analysis
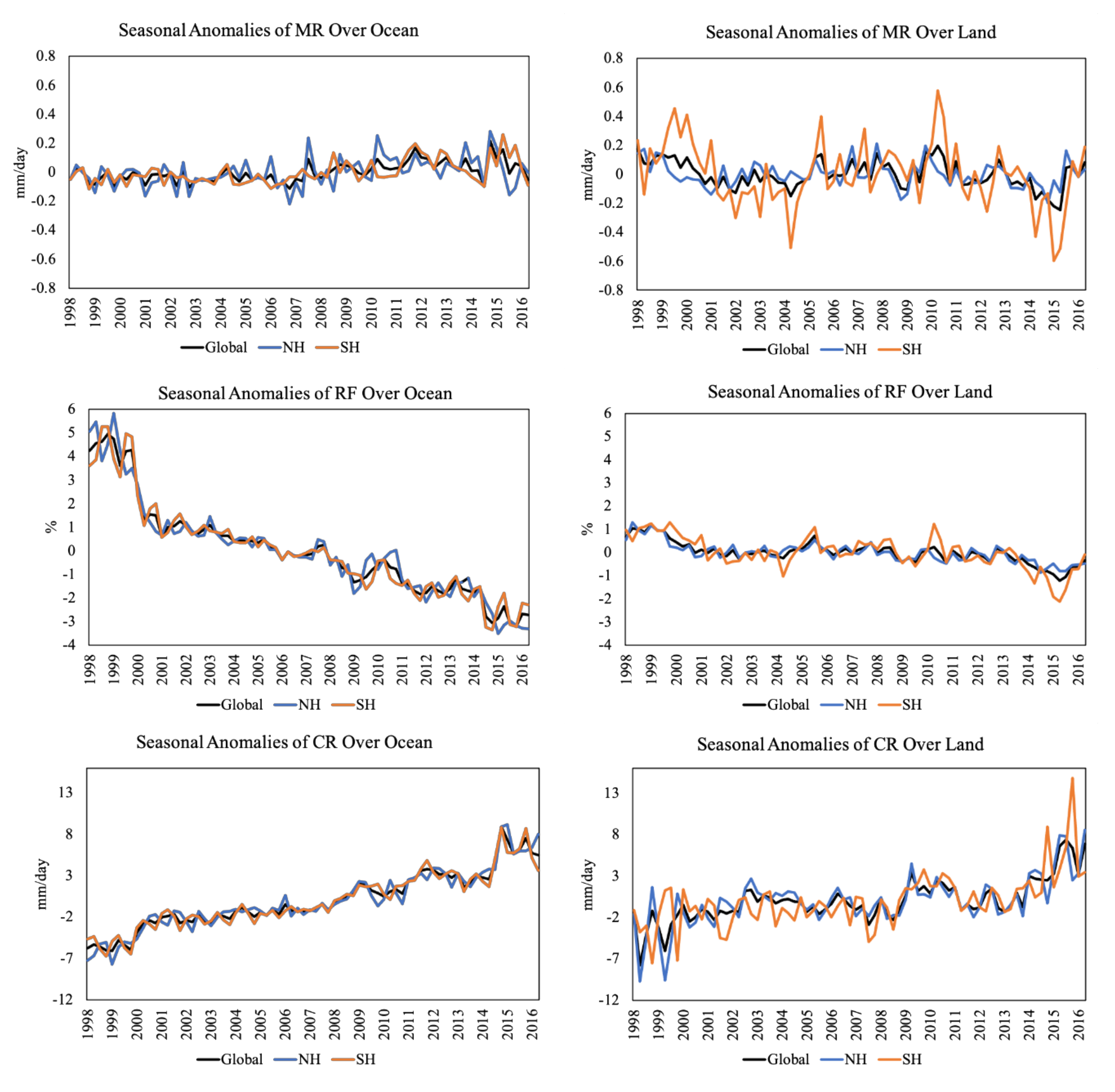
3.4. Inter-Annual Analysis
4. Discussions
5. Conclusions
- (1)
- Globally, the total rainfall amount is higher over ocean than over land, which is accounted by a much higher frequency but a slightly lower intensity over the ocean.
- (2)
- The frequency of rainfall events significantly decreased, while the intensity largely increased over the study period. The precipitation amounts slightly increased due to a combined effect of frequency and intensity. The ocean takes a relatively larger proportion in the global trends of frequency and intensity than land.
- (3)
- The precipitation categories are consistent with the climate classes over land and are mainly determined by oceanic precipitation climatology over the ocean. The clustering results also stand out as some coastal and bay areas do not have the largest rainfall amount but are high in intensity. This information can be useful for precipitation disaster management in these regions.
- (4)
- The total rainfall amount is dominated by the ENSO signal globally, but the frequency and intensity are mostly influenced by their trends in TMPA data. The amount and frequency over the land of the southern hemisphere are also largely influenced by the SOI.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xu, H.; Yu, T.; Cheng, T.; Li, J.; Lai, J.; Liu, Q. The research of remote sensing duststorm with FY-3B three infrared channels. In Infrared, Millimeter-Wave, and Terahertz Technologies II; International Society for Optics and Photonics: Bellingham, WA, USA, 2012; Volume 8562, p. 856212. [Google Scholar]
- Xu, H.; Cheng, T.; Gu, X.; Yu, T.; Xie, D.; Zheng, F. Spatiotemporal variability in dust observed over the Sinkiang and Inner Mongolia regions of Northern China. Atmos. Pollut. Res. 2015, 6, 562–571. [Google Scholar] [CrossRef]
- Oldenborgh, G.J.; van der Wiel, K.; Sebastian, A.; Singh, R.; Arrighi, J.; Otto, F.; Cullen, H. Attribution of extreme rainfall from Hurricane Harvey, August 2017. Environ. Res. Lett. 2017, 12, 124009. [Google Scholar] [CrossRef]
- Kawecki, S.; Steiner, A.L. The influence of aerosol hygroscopicity on precipitation intensity during a mesoscale convective event. J. Geophys. Res. Atmos. 2018, 123, 424–442. [Google Scholar] [CrossRef]
- Black, A.W.; Mote, T.L. Characteristics of winter-precipitation-related transportation fatalities in the United States. Weather Clim. Soc. 2015, 7, 133–145. [Google Scholar] [CrossRef]
- Sloat, L.L.; Gerber, J.S.; Samberg, L.H.; Smith, W.K.; Herrero, M.; Ferreira, L.G.; West, P.C. Increasing importance of precipitation variability on global livestock grazing lands. Nat. Clim. Chang. 2018, 8, 214. [Google Scholar] [CrossRef]
- Horn, L.; Hajat, A.; Sheppard, L.; Quinn, C.; Colborn, J.; Zermoglio, M.; Ebi, K. Association between precipitation and diarrheal disease in Mozambique. Int. J. Environ. Res. Public Health 2018, 15, 709. [Google Scholar] [CrossRef] [PubMed]
- Liu, Q.; Harris, J.T.; Chiu, L.S.; Sun, D.; Houser, P.R.; Yu, M.; Duffy, D.Q.; Little, M.M.; Yang, C. Spatiotemporal impacts of COVID-19 on air pollution in California, USA. Sci. Total Environ. 2021, 750, 141592. [Google Scholar] [CrossRef]
- Chiu, L.S.; Gao, S.; Shin, D.-B. Climate-Scale Oceanic Rainfall Based on Passive Microwave Radiometry. In Satellite-Based Applications on Climate Change; Qu, J., Ed.; Springer: New York, NY, USA, 2013; pp. 225–245. [Google Scholar]
- Carson, M.; Lyu, K.; Richter, K.; Becker, M.; Domingues, C.M.; Han, W.; Zanna, L. Climate model uncertainty and trend detection in regional sea level projections: A review. Surv. Geophys. 2019, 40, 1631–1653. [Google Scholar] [CrossRef]
- Donat, M.G.; Lowry, A.L.; Alexander, L.V.; O’Gorman, P.A.; Maher, N. More extreme precipitation in the world’s dry and wet regions. Nat. Clim. Chang. 2016, 6, 508. [Google Scholar] [CrossRef]
- Valdes-Abellan, J.; Pardo, M.A.; Tenza-Abril, A.J. Observed precipitation trend changes in the western Mediterranean region. Int. J. Climatol. 2017, 37, 1285–1296. [Google Scholar] [CrossRef]
- Trenberth, K.E. Conceptual Framework for Changes of Extremes of the Hydrological Cycle with Climate Change. Limatic Chang. 1999, 42, 327–339. [Google Scholar] [CrossRef]
- Jakob, C.; Schumacher, C. Precipitation and Latent Heating Characteristics of the Major Tropical Western Pacific Cloud Regimes. J. Clim. 2007, 21, 4348–4364. [Google Scholar] [CrossRef][Green Version]
- Pendergrass, A.G.; Hartmann, D.L. Changes in the Distribution of Rain Frequency and Intensity in Response to Global Warming. J. Clim. 2014, 27, 8372–8383. [Google Scholar] [CrossRef]
- Adler, R.F.; Gu, G.; Sapiano, M.; Wang, J.-J.; Huffman, G.J. Global Precipitation: Means, Variations and Trends During the Satellite Era (1979–2014). Surv. Geophys. 2017, 38, 679–699. [Google Scholar] [CrossRef]
- Gu, G.; Adler, R.F. Precipitation intensity changes in the tropics from observations and models. J. Clim. 2018, 31, 4775–4790. [Google Scholar] [CrossRef]
- Shang, H.; Xu, M.; Zhao, F.; Tijjani, S.B. Spatial and Temporal Variations in Precipitation Amount, Frequency, Intensity, and Persistence in China, 1973–2016. J. Hydrometeorol. 2019, 20, 2215–2227. [Google Scholar] [CrossRef]
- Trenberth, K.E.; Zhang, Y. Near-Global Covariability of Hourly Precipitation in Space and Time. J. Hydrometeorol. 2018, 19, 695–713. [Google Scholar] [CrossRef]
- Miao, C.; Duan, Q.; Sun, Q.; Lei, X.; Li, H. Non-uniform changes in different categories of precipitation intensity across China and the associated large-scale circulations. Environ. Res. Lett. 2018, 14, 025004. [Google Scholar] [CrossRef]
- GES DISC. TRMM (TMPA) Rainfall Estimate L3 3 Hour 0.25 Degree x 0.25 Degree V7, Greenbelt, MD, Goddard Earth Sciences Data and Information Services Center (GES DISC). 2011. Available online: https://disc.gsfc.nasa.gov/ (accessed on 8 April 2017).
- Kummerow, C.; Barnes, W.; Kozu, T.; Shiue, J.; Simpson, J. The tropical rainfall measuring mission (TRMM) sensor package. J. Atmos. Ocean. Technol. 1998, 15, 809–817. [Google Scholar] [CrossRef]
- Yu, Z.; Yu, H.; Chen, P.; Qian, C.; Yue, C. Verification of tropical cyclone—Related satellite precipitation estimates in mainland China. J. Appl. Meteorol. Climatol. 2009, 48, 2227–2241. [Google Scholar] [CrossRef]
- Sahoo, A.K.; Sheffield, J.; Pan, M.; Wood, E.F. Evaluation of the tropical rainfall measuring mission multi-satellite precipitation analysis (TMPA) for assessment of large-scale meteorological drought. Remote Sens. Environ. 2015, 159, 181–193. [Google Scholar] [CrossRef]
- Nguyen, P.; Ombadi, M.; Sorooshian, S.; Hsu, K.; AghaKouchak, A.; Braithwaite, D.; Ashouri, H.; Thorstensen, A.R. The PERSIANN family of global satellite precipitation data: A review and evaluation of products. Hydrol. Earth Syst. Sci. 2018, 22, 5801–5816. [Google Scholar] [CrossRef]
- Huffman, G.J.; Adler, R.F.; Bolvin, D.T.; Nelkin, E.J. The TRMM Multi-Satellite Precipitation Analysis (TMPA). In Satellite Rainfall Applications for Surface Hydrology; Springer: Dordrecht, The Netherlands, 2010; pp. 3–22. [Google Scholar]
- Karbalaee, N.; Hsu, K.; Sorooshian, S.; Braithwaite, D. Bias adjustment of infrared-based rainfall estimation using passive microwave satellite rainfall data. J. Geophys. Res. Atmos. 2017, 122, 3859–3876. [Google Scholar] [CrossRef]
- Trenberth, K.E.; Zhang, Y. How often does it really rain? BAMS 2017, 99, 289–298. [Google Scholar] [CrossRef]
- Liu, Q.; Chiu, L.S.; Hao, X. The Impact of Spatial and Temporal Resolutions in Tropical Summer Rainfall Distribution: Preliminary Results. ISPRS Ann. Photogramm. Remote Sens. Spat. Inf. Sci. 2017, 4, 183. [Google Scholar] [CrossRef]
- Chen, D.; Dai, A. Dependence of estimated precipitation frequency and intensity on data resolution. Clim. Dyn. 2018, 50, 3625–3647. [Google Scholar] [CrossRef]
- Blamey, R.C.; Reason, C.J.C. The role of mesoscale convective complexes in southern Africa summer rainfall. J. Clim. 2013, 26, 1654–1668. [Google Scholar] [CrossRef]
- Zahra, S.; Ghazanfar, M.A.; Khalid, A.; Azam, M.A.; Naeem, U.; Prugel-Bennett, A. Novel centroid selection approaches for KMeans-clustering based recommender systems. Inf. Sci. 2015, 320, 156–189. [Google Scholar] [CrossRef]
- Von Storch, H.; Zwiers, F.W. Statistical Analysis in Climate Research; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Liu, Z. Comparison of Integrated Multisatellite Retrievals for GPM (IMERG) and TRMM Multisatellite Precipitation Analysis (TMPA) Monthly Precipitation Products: Initial Results. J. Hydrometeorol. 2016, 17, 777–790. [Google Scholar] [CrossRef]
- Venugopal, V.; Wallace, J.M. Climatology of contribution-weighted tropical rain rates based on TRMM 3B42. Geophys. Res. Lett. 2016, 43, 10439–10447. [Google Scholar] [CrossRef]
- Marciano, C.G.; Lackmann, G.M.; Robinson, W.A. Changes in US East Coast cyclone dynamics with climate change. J. Clim. 2015, 28, 468–484. [Google Scholar] [CrossRef]
- Adler, R.F.; Sapiano, M.R.; Huffman, G.J.; Wang, J.-J.; Gu, G.; Bolvin, D.; Shin, D.-B. The Global Precipitation Climatology Project (GPCP) Monthly Analysis (New Version 2.3) and a Review of 2017 Global Precipitation. Atmosphere 2018, 9, 138. [Google Scholar] [CrossRef]
- Lashkari, H.; Mohammadi, Z.; Keikhosravi, G. Annual fluctuations and displacements of inter tropical convergence zone (ITCZ) within the range of Atlantic Ocean-India. Open J. Ecol. 2017, 7, 12–33. [Google Scholar] [CrossRef]
- Jaramillo, L.; Poveda, G.; Mejía, J.F. Mesoscale convective systems and other precipitation features over the tropical Americas and surrounding seas as seen by TRMM. Int. J. Climatol. 2017, 37, 380–397. [Google Scholar] [CrossRef]
- Tan, H.; Ray, P.; Barrett, B.; Dudhia, J.; Moncrieff, M.W. Systematic patterns in land precipitation due to convection in neighboring islands in the Maritime Continent during MJO propagation. J. Geophys. Res. Atmos. 2021, 126, e2020JD033465. [Google Scholar] [CrossRef]
- NOAA. Southern Oscillation Index (SOI). Retrieved 19 April 2021, from National Centers for Environmental Information; 2016. Available online: https://www.ncdc.noaa.gov/teleconnections/enso/indicators/soi/ (accessed on 22 August 2019).
- Rohlf, F.J.; Sokal, R.R. Statistical Tables; Macmillan: New York, NY, USA, 1995. [Google Scholar]
- McBride, J.L.; Haylock, M.R.; Nicholls, N. Relationships between the Maritime Continent heat source and the El Niño–Southern Oscillation phenomenon. J. Clim. 2003, 16, 2905–2914. [Google Scholar] [CrossRef]
- Beck, H.E.; Zimmermann, N.E.; McVicar, T.R.; Vergopolan, N.; Berg, A.; Wood, E.F. Present and future Köppen-Geiger climate classification maps at 1-km resolution. Sci. Data 2018, 214, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Henderson, D.S.; Kummerow, C.D.; Berg, W. ENSO influence on TRMM tropical oceanic precipitation characteristics and rain rates. J. Clim. 2018, 31, 3979–3998. [Google Scholar] [CrossRef]
- North, G.R.; Bell, T.L.; Cahalan, R.F.; Moeng, F.J. Sampling Errors in the Estimation of Empirical Orthogonal Functions. Mon. Weather Rev. 1982, 110, 699–705. [Google Scholar] [CrossRef]
- Sun, Q.; Miao, C.; Duan, Q.; Ashouri, H.; Sorooshian, S.; Hsu, K.-L. A Review of Global Precipitation Data Sets: Data Sources, Estimation, and Intercomparisons. Rev. Geophys. 2018, 56, 79–107. [Google Scholar] [CrossRef]
- Yang, S.; Nesbitt, S.W. Statistical properties of precipitation as observed by the TRMM precipitation radar. Geophys. Res. Lett. 2014, 41, 5636–5643. [Google Scholar] [CrossRef]
- Mukherjee, S.; Aadhar, S.; Stone, D.; Mishra, V. Increase in extreme precipitation events under anthropogenic warming in India. Weather Clim. Extrem. 2018, 20, 45–53. [Google Scholar] [CrossRef]
- Güner Bacanli, Ü. Trend analysis of precipitation and drought in the A egean region, Turkey. Meteorol. Appl. 2017, 24, 239–249. [Google Scholar] [CrossRef]
- Bartiko, D.; Oliveira, D.Y.; Bonumá, N.B.; Chaffe, P.L.B. Spatial and seasonal patterns of flood change across Brazil. Hydrol. Sci. J. 2019, 64, 1071–1079. [Google Scholar] [CrossRef]
- Li, Y.; Guo, B.; Wang, K.; Wu, G.; Shi, C. Performance of TRMM Product in Quantifying Frequency and Intensity of Precipitation during Daytime and Nighttime across China. Remote Sens. 2020, 12, 740. [Google Scholar] [CrossRef]
- Fischer, E.M.; Knutti, R. Anthropogenic contribution to global occurrence of heavy-precipitation and high-temperature extremes. Nat. Clim. Chang. 2015, 5, 560. [Google Scholar] [CrossRef]
- Liu, Q.; Li, Y.; Yu, M.; Chiu, L.S.; Hao, X.; Duffy, D.Q.; Yang, C. Daytime Rainy Cloud Detection and Convective Precipitation Delineation Based on a Deep Neural Network Method Using GOES-16 ABI Images. Remote Sens. 2019, 11, 2555. [Google Scholar] [CrossRef]
- Field, C.B.; Barros, V.R. (Eds.) Climate Change 2014–Impacts, Adaptation and Vulnerability: Regional Aspects; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Bannister, D.; Orr, A.; Jain, S.K.; Holman, I.P.; Momblanch, A.; Phillips, T.; Adeloye, A.J.; Snapir, B.; Waine, T.W.; Hosking, J.S.; et al. Bias correction of high-resolution regional climate model precipitation output gives the best estimates of precipitation in Himalayan catchments. J. Geophys. Res. Atmos. 2019, 124, 14220–14239. [Google Scholar] [CrossRef]
- Rauniyar, S.P.; Protat, A.; Kanamori, H. Uncertainties in TRMM-Era multisatellite-based tropical rainfall estimates over the Maritime Continent. Earth Space Sci. 2017, 4, 275–302. [Google Scholar] [CrossRef]
- Bharti, V.; Singh, C. Evaluation of error in TRMM 3B42V7 precipitation estimates over the Himalayan region. J. Geophys. Res. Atmos. 2015, 120, 12458–12473. [Google Scholar] [CrossRef]
- Kim, K.; Park, J.; Baik, J.; Choi, M. Evaluation of topographical and seasonal feature using GPM IMERG and TRMM 3B42 over Far-East Asia. Atmos. Res. 2017, 187, 95–105. [Google Scholar] [CrossRef]
- Chen, F.; Li, X. Evaluation of IMERG and TRMM 3B43 monthly precipitation products over mainland China. Remote Sens. 2016, 8, 472. [Google Scholar] [CrossRef]
- Fang, J.; Yang, W.; Luan, Y.; Du, J.; Lin, A.; Zhao, L. Evaluation of the TRMM 3B42 and GPM IMERG products for extreme precipitation analysis over China. Atmos. Res. 2019, 223, 24–38. [Google Scholar] [CrossRef]



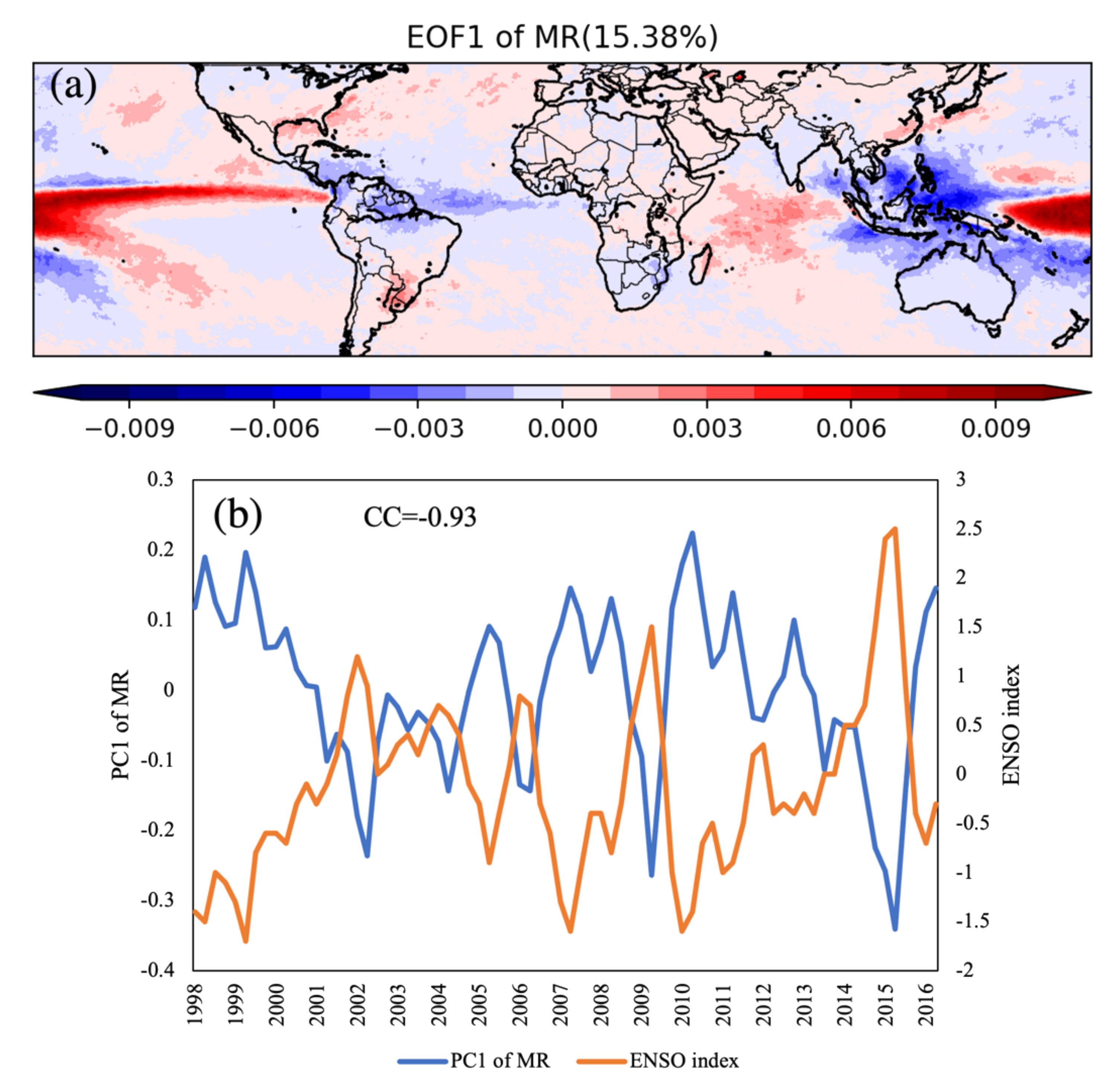
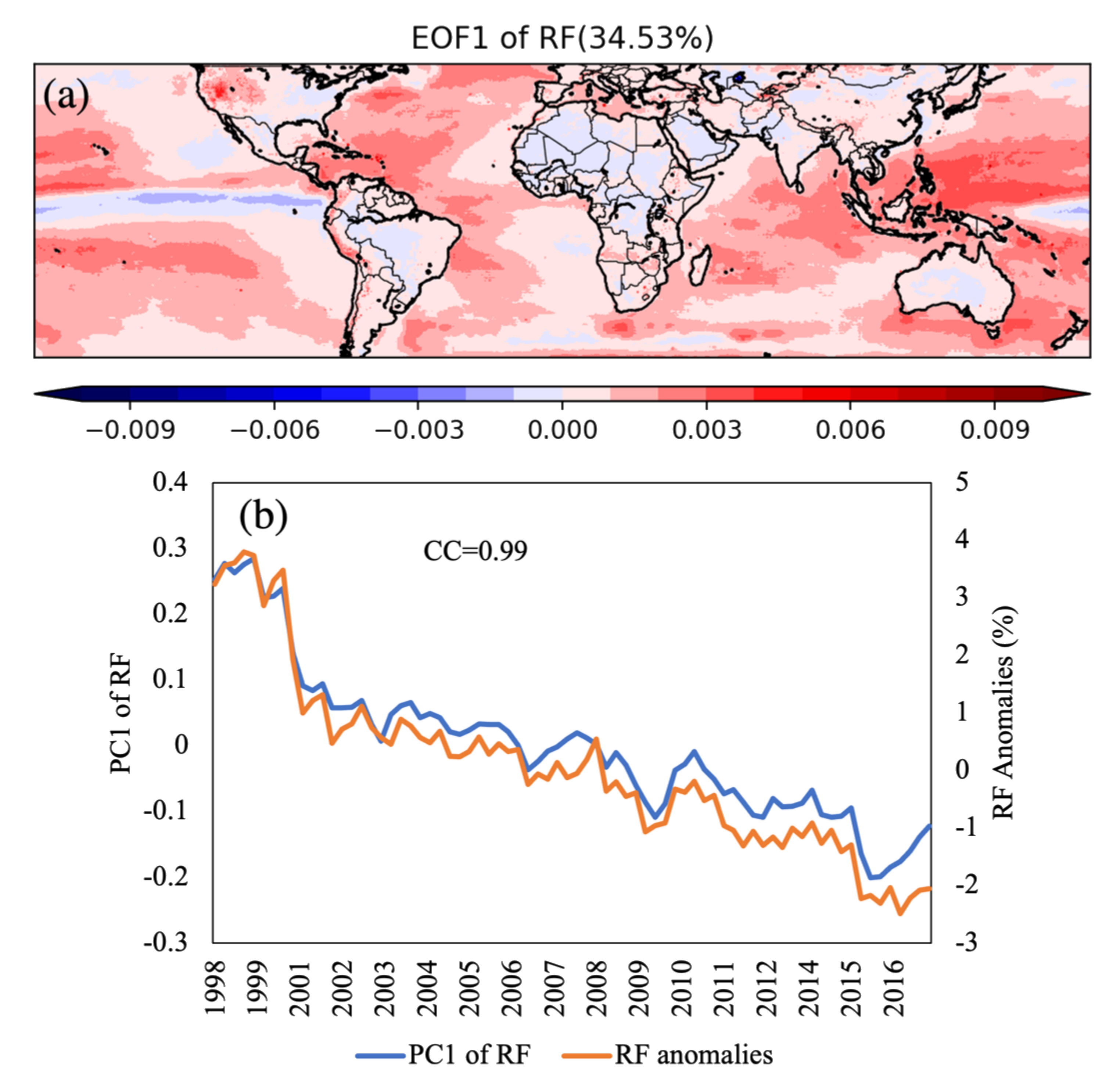
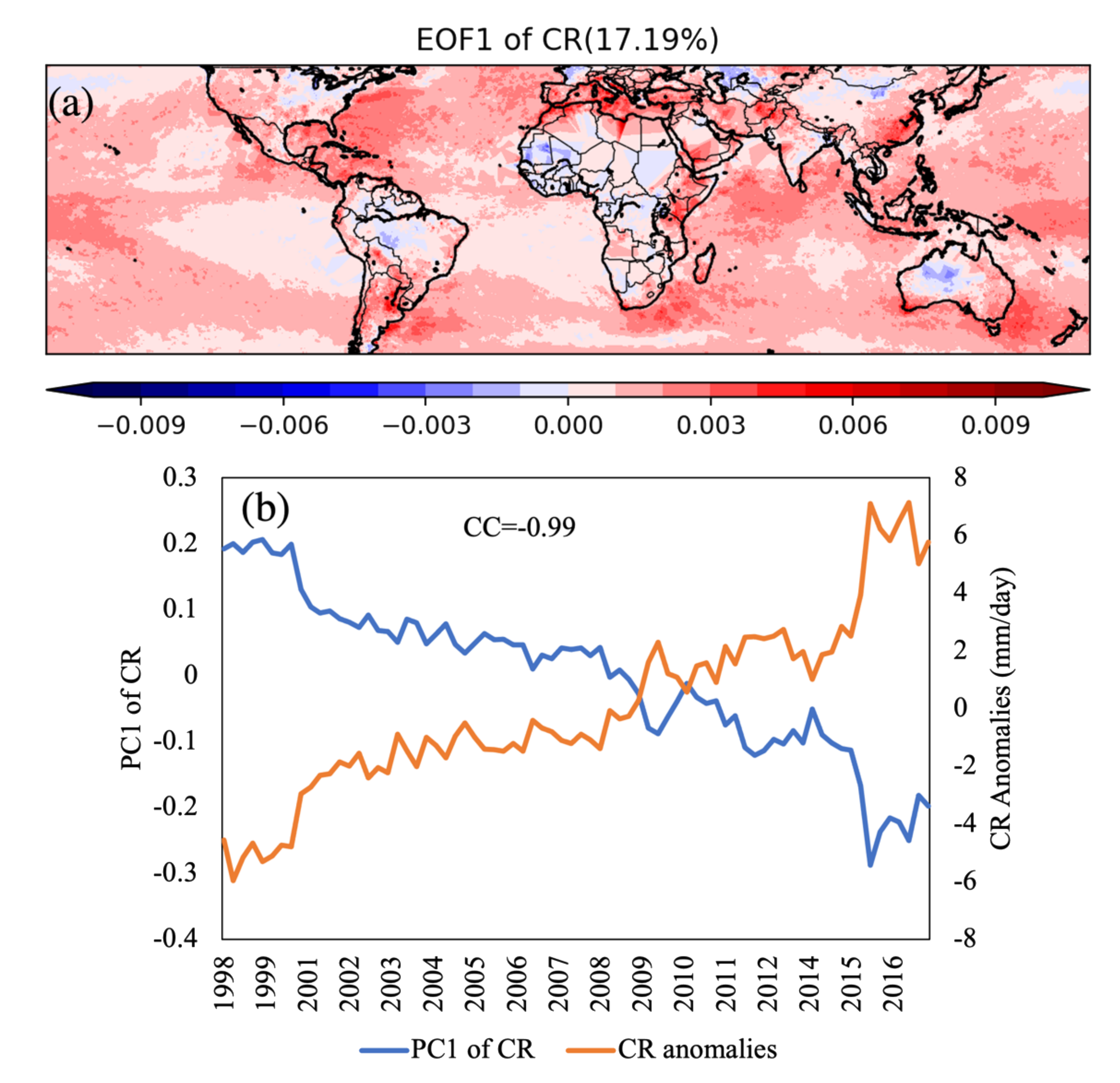
| MR | RF | CR | |||||
|---|---|---|---|---|---|---|---|
| Mean (mm/Day) | Trend (mm/d/Decade) | Mean (%) | Trend (%/Decade) | Mean (mm/Day) | Trend (mm/d/Decade) | ||
| 50°N−50°S (Global) | 2.83 | 0.04 * | 10.55 | −2.65 ** | 25.05 | 5.26 ** | |
| Ocean | Total | 2.96 | 0.07 ** | 12.15 | −3.44 ** | 21.19 | 6.03 ** |
| NH | 3.58 | 0.07 * | 13.45 | −3.47 ** | 23.87 | 6.22 ** | |
| SH | 2.50 | 0.08 ** | 11.17 | −3.43 ** | 19.18 | 5.87 ** | |
| Land | Total | 2.48 | −0.05 | 6.47 | −0.66 ** | 35.17 | 3.32 ** |
| NH | 2.10 | −0.03 | 5.61 | −0.59 ** | 34.24 | 3.30 ** | |
| SH | 3.29 | −0.10 * | 8.31 | −0.80 ** | 37.11 | 3.47 ** | |
| Mean Rain Rate | Rain Frequency | Conditional Rain Rate | ||||
|---|---|---|---|---|---|---|
| Mean (mm/Day) | Trend (mm/d/Decade) | Mean (%) | Trend (%/Decade) | Mean (mm/Day) | Trend (mm/d/Decade) | |
| NH Spring (MAM) | 2.75 | −0.007 | 10.65 | −2.82 ** | 24.91 | 5.10 ** |
| NH Summer (JJA) | 2.92 | 0.04 ** | 10.70 | −2.86 ** | 24.62 | 5.68 ** |
| NH Autumn (SON) | 2.81 | 0.101 ** | 10.45 | −2.65 ** | 25.20 | 5.12 ** |
| NH Winter (DJF) | 2.82 | 0.024 | 10.38 | −2.37 ** | 25.42 | 5.18 ** |
| MR | RF | CR | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Variance Explained (%) | Correlation with Seasonal Anomalies | Correlation with ENSO | Variance Explained (%) | Correlation with Seasonal Anomalies | Correlation with ENSO | Variance Explained (%) | Correlation with Seasonal Anomalies | Correlation with ENSO | |
| EOF1 | 15.38 | 0.24 | −0.93 | 34.53 | 0.99 | −0.51 | 17.19 | −0.99 | −0.46 |
| EOF2 | 4.40 | 0.23 | - | 9.09 | - | 0.78 | 3.95 | - | 0.49 |
| EOF3 | 3.55 | 0.39 | - | 4.39 | - | - | 3.65 | - | −0.55 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Q.; Chiu, L.S.; Hao, X.; Yang, C. Spatiotemporal Trends and Variations of the Rainfall Amount, Intensity, and Frequency in TRMM Multi-satellite Precipitation Analysis (TMPA) Data. Remote Sens. 2021, 13, 4629. https://doi.org/10.3390/rs13224629
Liu Q, Chiu LS, Hao X, Yang C. Spatiotemporal Trends and Variations of the Rainfall Amount, Intensity, and Frequency in TRMM Multi-satellite Precipitation Analysis (TMPA) Data. Remote Sensing. 2021; 13(22):4629. https://doi.org/10.3390/rs13224629
Chicago/Turabian StyleLiu, Qian, Long S. Chiu, Xianjun Hao, and Chaowei Yang. 2021. "Spatiotemporal Trends and Variations of the Rainfall Amount, Intensity, and Frequency in TRMM Multi-satellite Precipitation Analysis (TMPA) Data" Remote Sensing 13, no. 22: 4629. https://doi.org/10.3390/rs13224629
APA StyleLiu, Q., Chiu, L. S., Hao, X., & Yang, C. (2021). Spatiotemporal Trends and Variations of the Rainfall Amount, Intensity, and Frequency in TRMM Multi-satellite Precipitation Analysis (TMPA) Data. Remote Sensing, 13(22), 4629. https://doi.org/10.3390/rs13224629








