Analysis of the BDGIM Performance in BDS Single Point Positioning
Abstract
:1. Introduction
2. The Mathematical Model of SPP with the BDGIM
2.1. Observation Equation of SPP
2.2. Correction of Hardware Delay
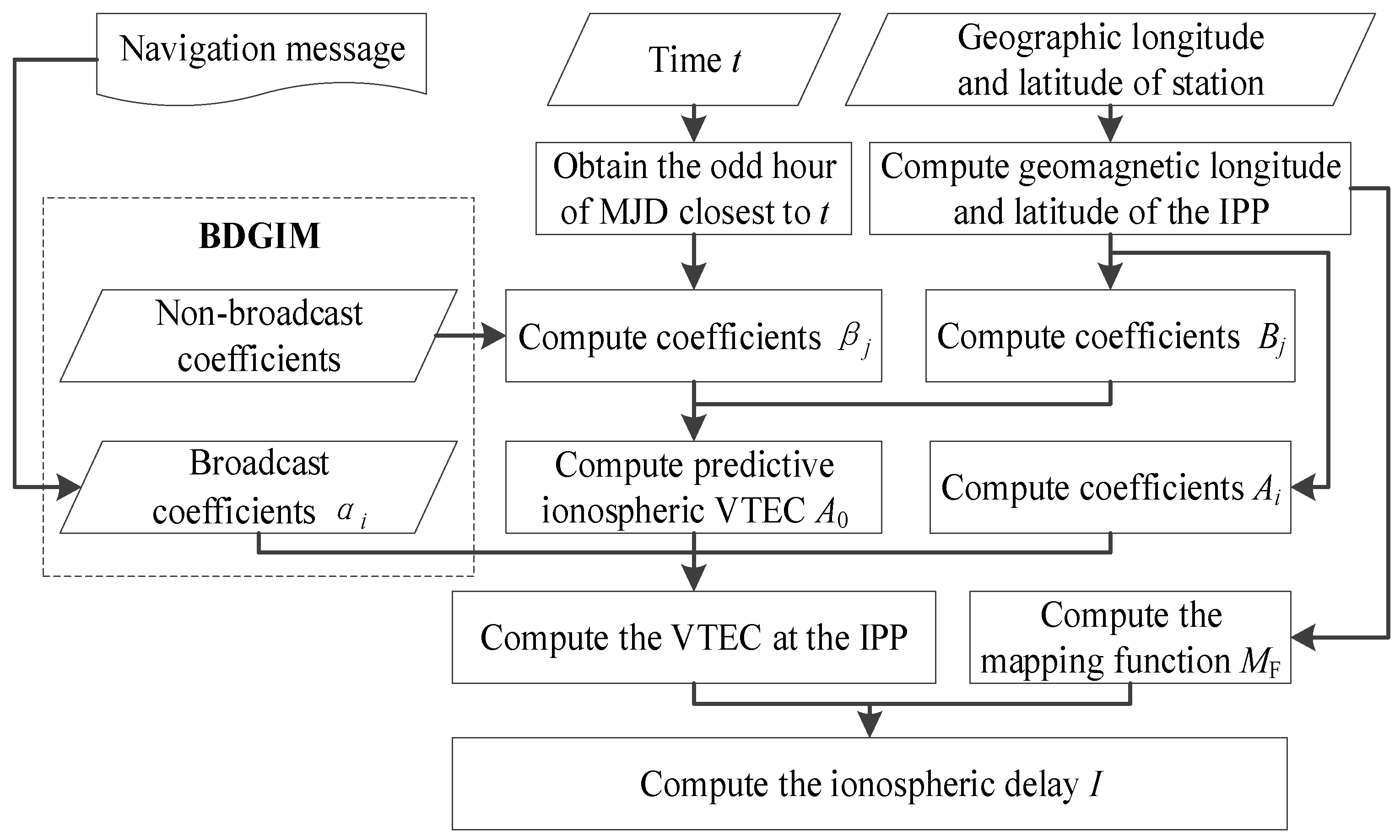
2.3. Algorithm of the BDGIM
3. Processing Strategies
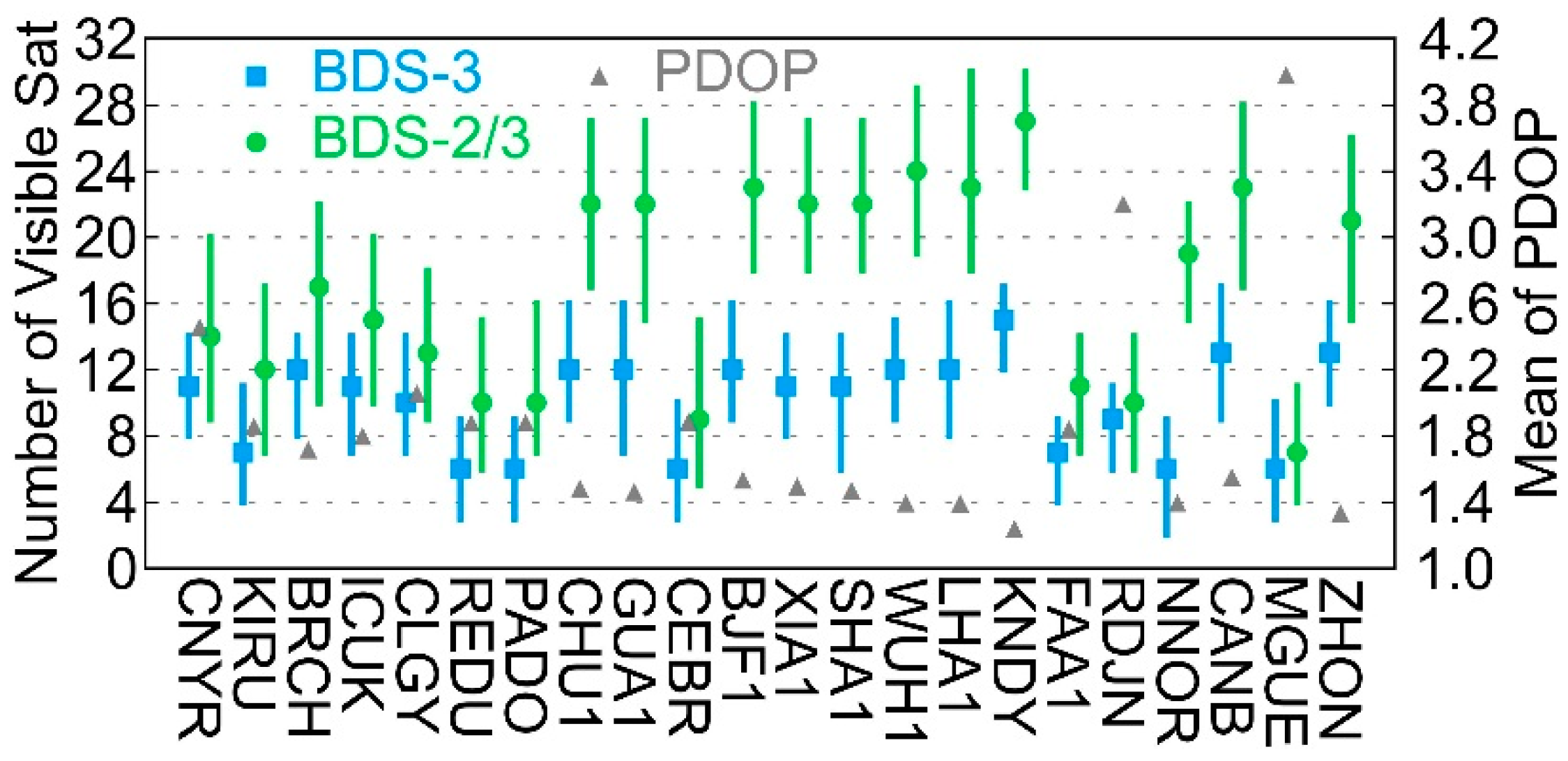
3.1. Data Selection
3.2. SPP Strategies
4. Results
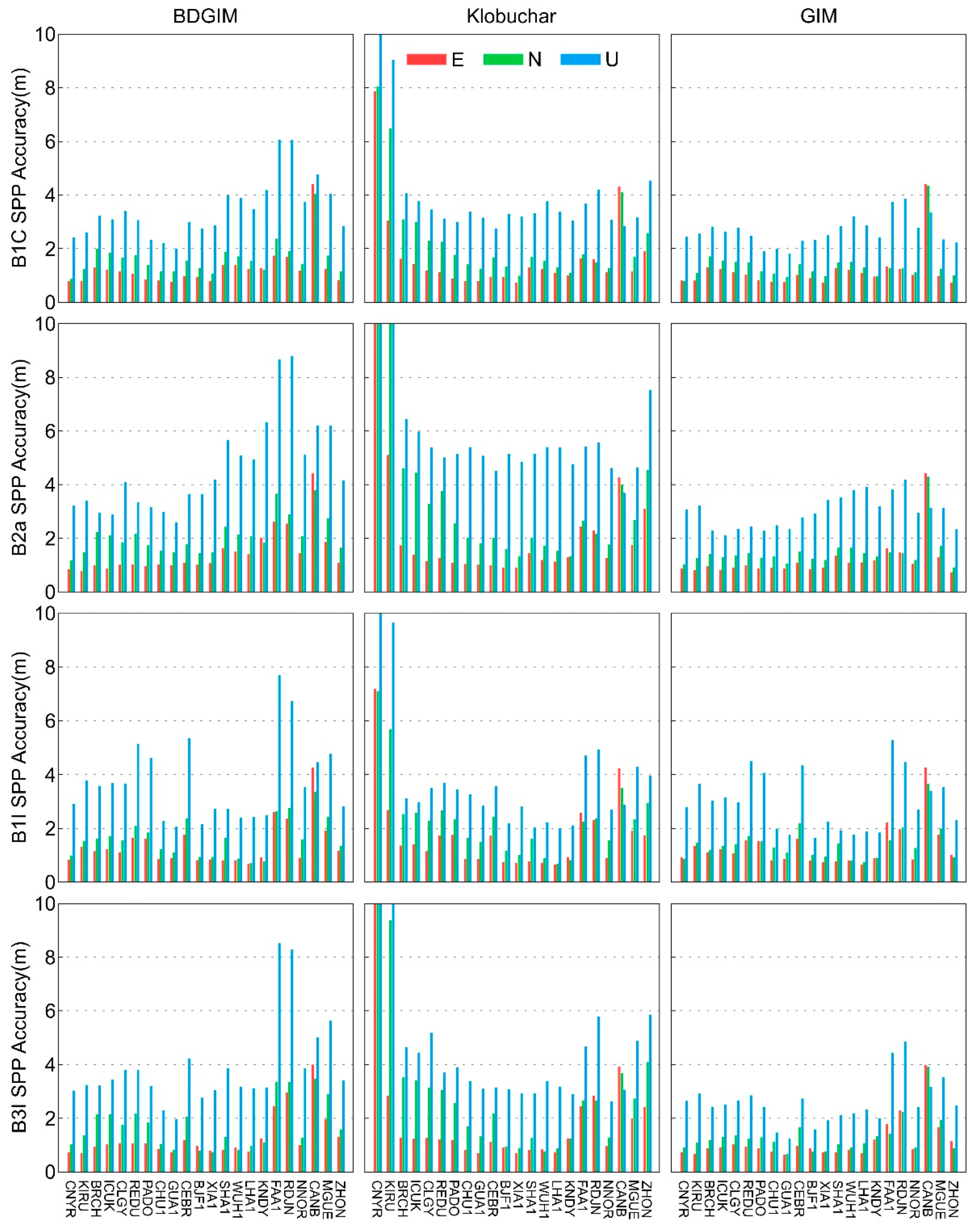
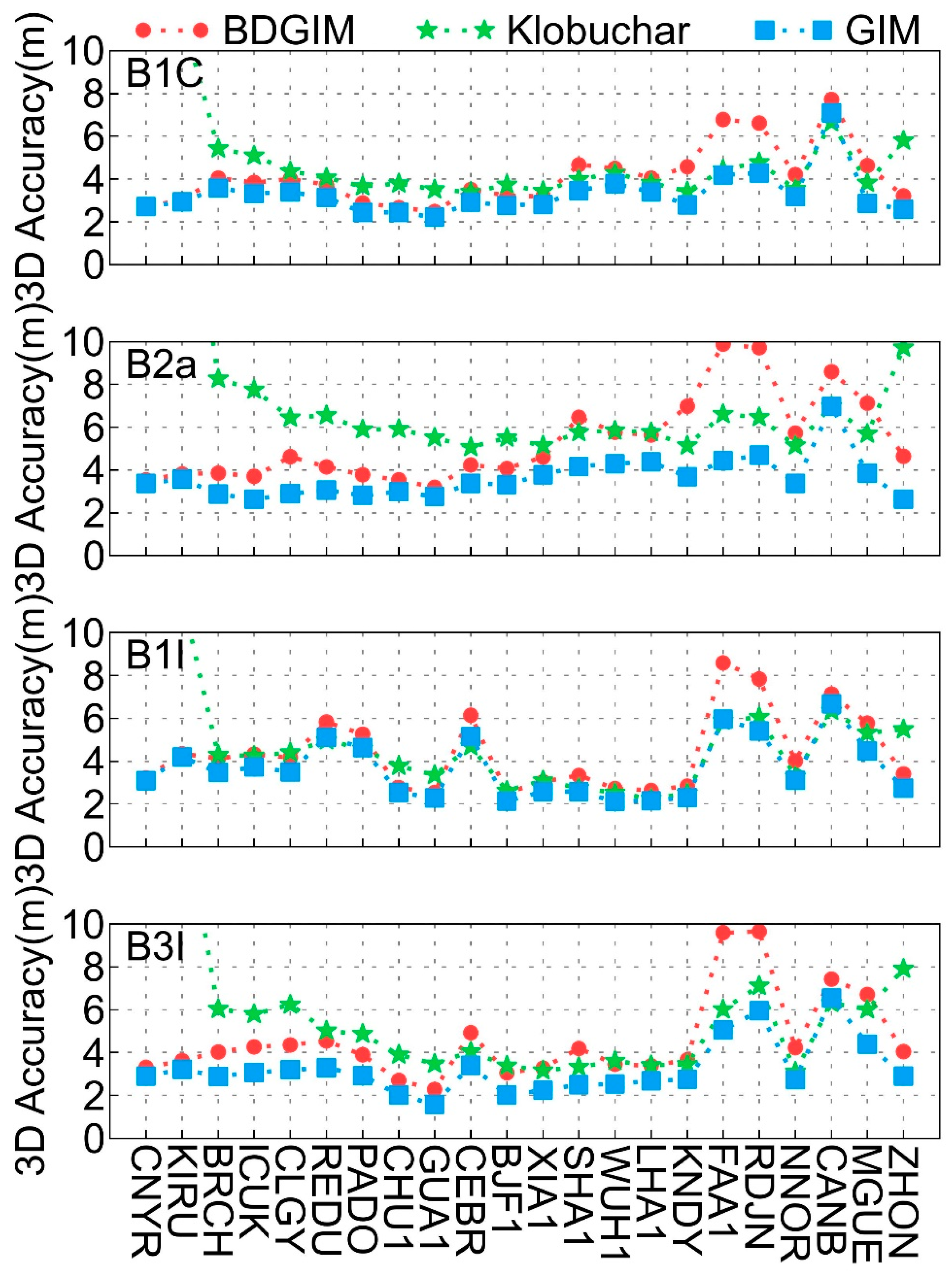
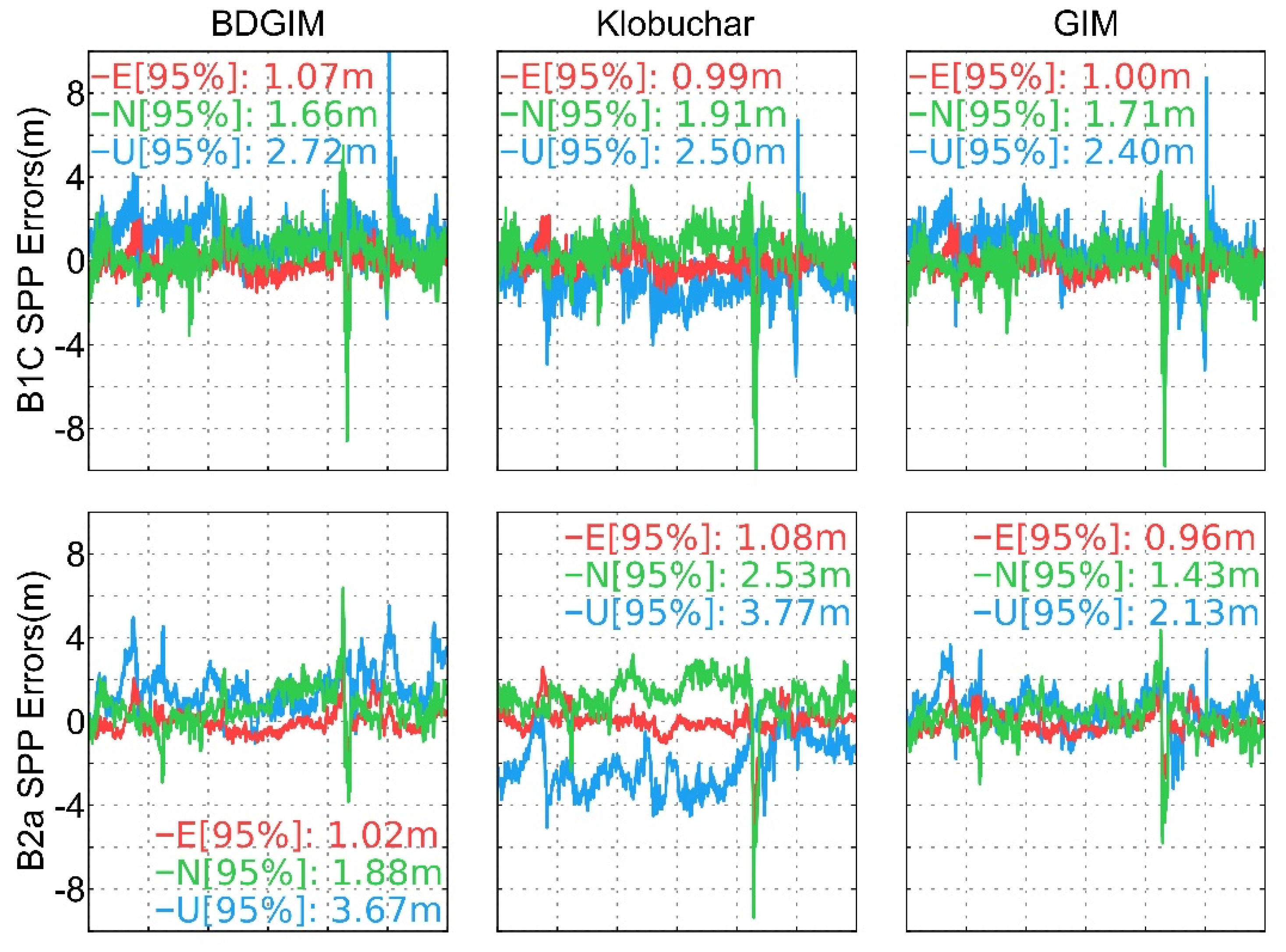
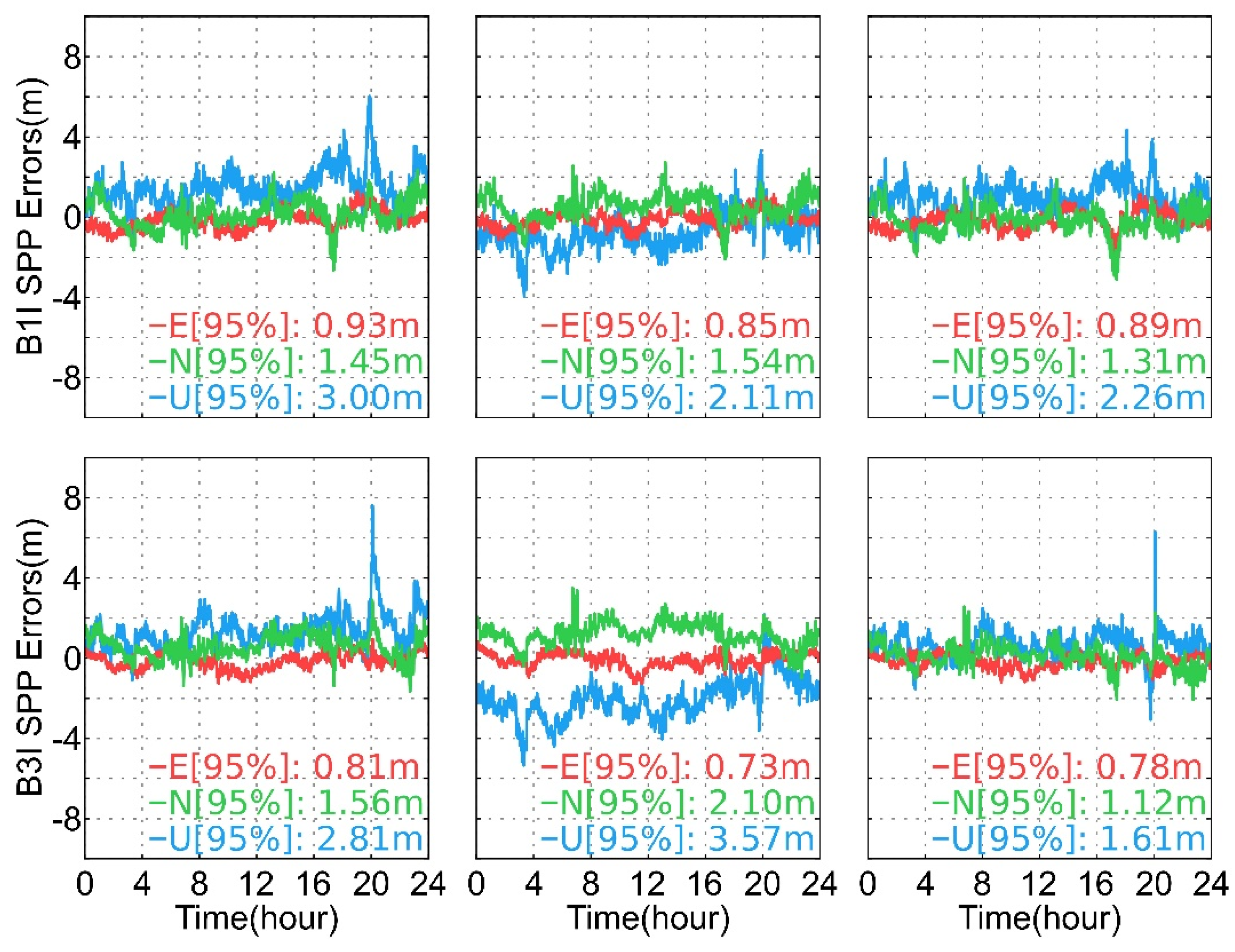
4.1. Global Positioning Accuracies
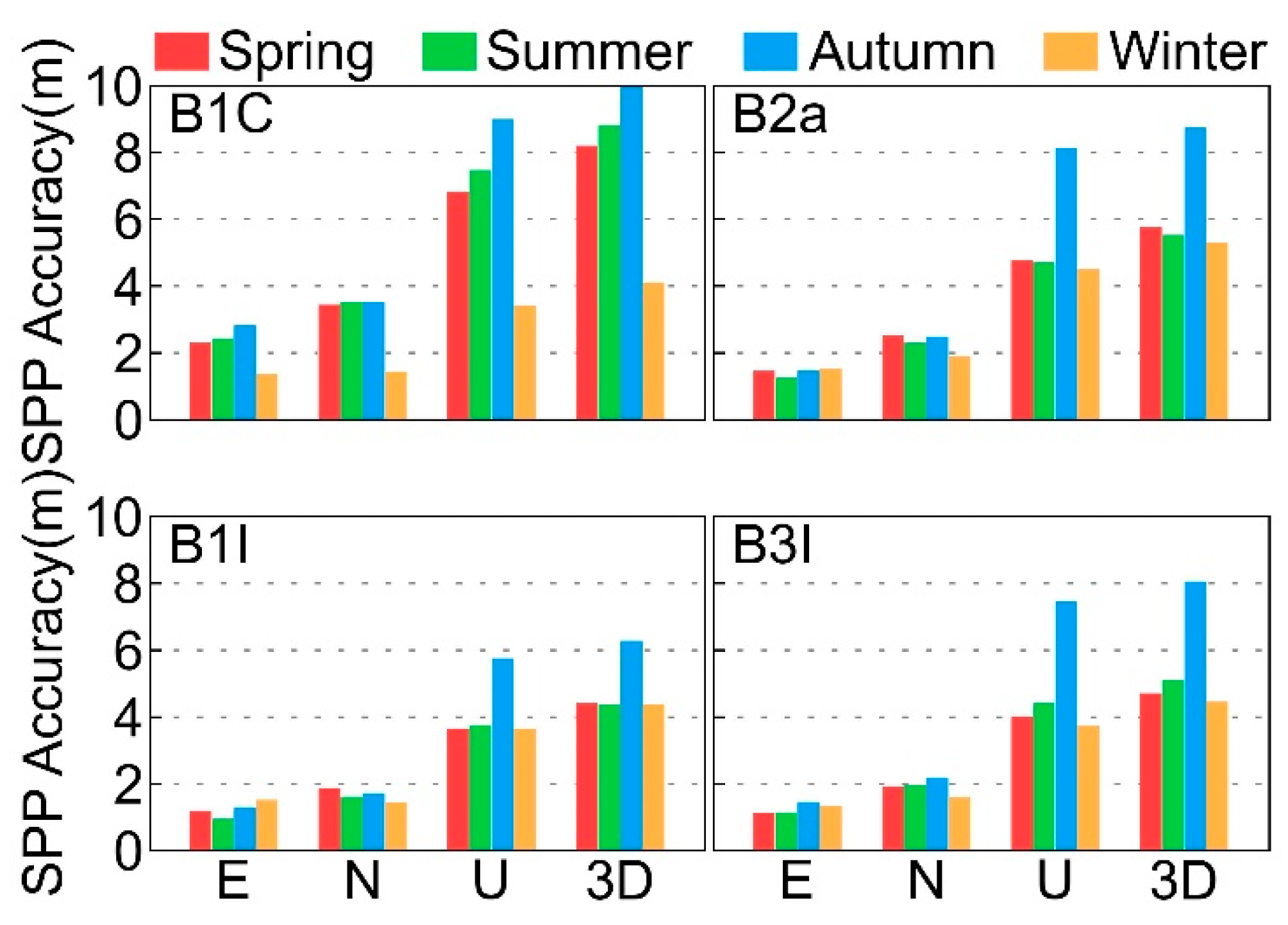
4.2. Positioning Accuracies in Different Seasons
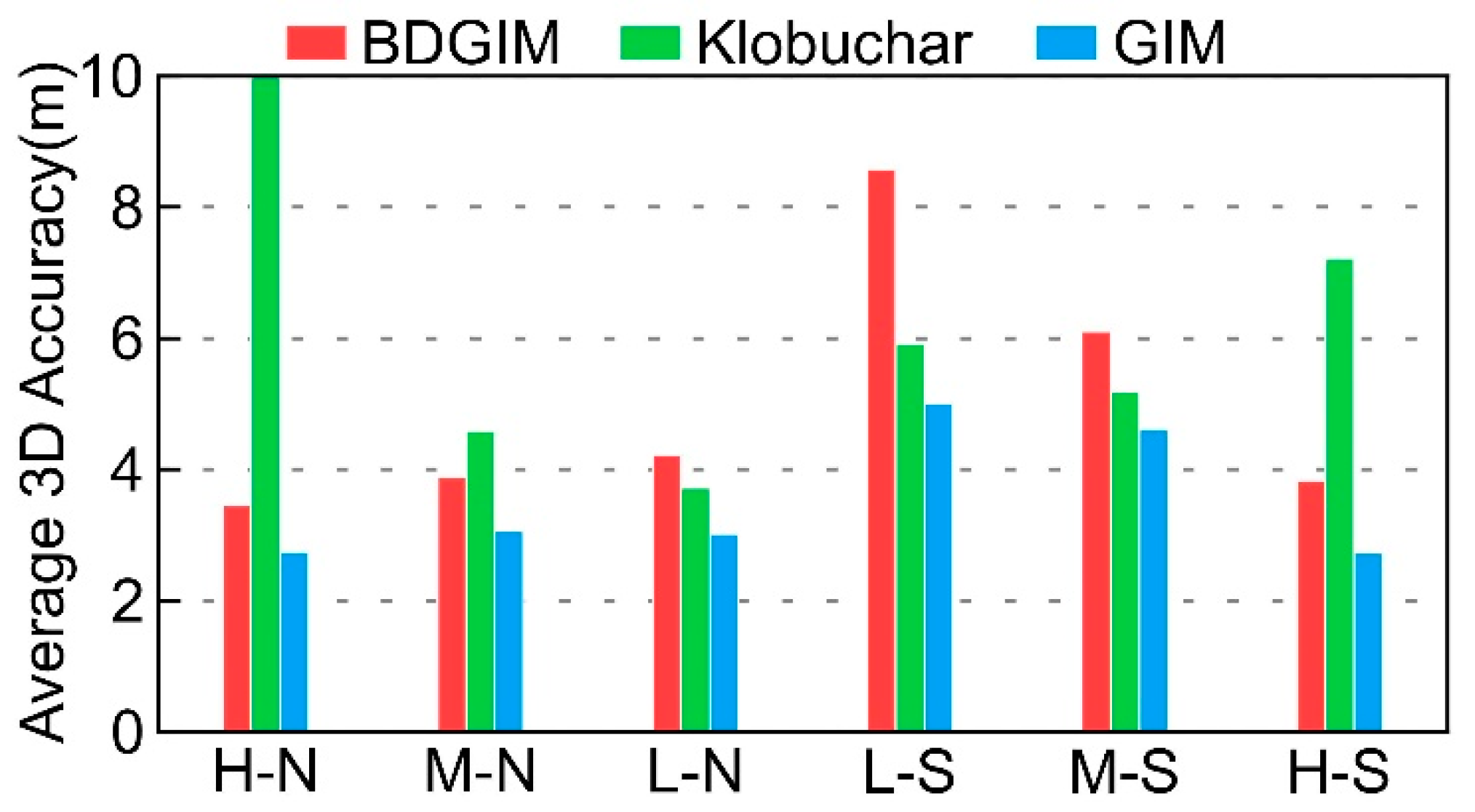
4.3. Positioning Accuracies during the Geomagnetic Storm
5. Conclusions and Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Klobuchar, J.A. Ionospheric Time-Delay Algorithm for Single-Frequency GPS Users. IEEE Trans. Aerosp. Electron. Syst. 1987, AES-23, 325–331. [Google Scholar] [CrossRef]
- Yu, S.; Ma, F.J.; Wang, W.; Wu, R.P.; Zeng, Q. Preliminary Evaluation on the Precision of the BDS-3 Global Ionospheric Model. In China Satellite Navigation Conference; Sun, J., Yang, C., Yang, Y., Eds.; Lecture Notes in Electrical Engineering; Springer Singapore Pte Ltd.: Singapore, 2019; Volume 563, pp. 131–139. [Google Scholar]
- Gioia, C.; Borio, D.; Realini, E.; Gatti, A.; Tagliaferro, G. From Single to Precise Point Positioning: The Impact on Time Retrieval. In Proceedings of the 2020 IEEE/ION Position, Location and Navigation Symposium (PLANS), Portland, OR, USA, 20–23 April 2020; pp. 1104–1110. [Google Scholar]
- Paziewski, J.; Sieradzki, R.; Baryla, R. Multi-GNSS high-rate RTK, PPP and novel direct phase observation processing method: Application to precise dynamic displacement detection. Meas. Sci. Technol. 2018, 29, 035002. [Google Scholar] [CrossRef]
- Wang, N.; Yuan, Y.; Li, Z.; Huo, X. Improvement of Klobuchar model for GNSS single-frequency ionospheric delay corrections. Adv. Space Res. 2016, 57, 1555–1569. [Google Scholar] [CrossRef]
- Zhu, Y.; Tan, S.; Zhang, Q.; Ren, X.; Jia, X. Accuracy evaluation of the latest BDGIM for BDS-3 satellites. Adv. Space Res. 2019, 64, 1217–1224. [Google Scholar] [CrossRef]
- Gioia, C.; Borio, D. NeQuick-G and Android Devices: A Compromise between Computational Burden and Accuracy. Sensors 2020, 20, 5908. [Google Scholar] [CrossRef]
- Yuan, Y.; Wang, N.; Li, Z.; Huo, X. The BeiDou global broadcast ionospheric delay correction model (BDGIM) and its preliminary performance evaluation results. J. Inst. Navig. 2019, 66, 55–69. [Google Scholar] [CrossRef] [Green Version]
- Wu, X.; Hu, X.; Wang, G.; Zhong, H.; Tang, C. Evaluation of COMPASS ionospheric model in GNSS positioning. Adv. Space Res. 2013, 51, 959–968. [Google Scholar] [CrossRef]
- Zhou, R.Y.; Hu, Z.G.; Su, M.D.; Li, J.Z.; Li, P.B.; Zhao, Q.L. Preliminary Performance Evaluation of BeiDou Global Ionospheric Delay Correction Model; Geomatics and Information Science of Wuhan University: Wuhan, China, 2019; Volume 44, pp. 1457–1464. (In Chinese) [Google Scholar]
- Bi, T.; An, J.; Yang, J.; Liu, S. A modified Klobuchar model for single-frequency GNSS users over the polar region. Adv. Space Res. 2017, 59, 833–842. [Google Scholar] [CrossRef]
- Pongracic, B.; Wu, F.; Fathollahi, L.; Brčić, D. Midlatitude Klobuchar correction model based on the k-means clustering of ionospheric daily variations. GPS Solut. 2019, 23, 80. [Google Scholar] [CrossRef]
- Liu, C.; Liu, C.J.; Feng, X.; Xu, L.F.; Du, Y. Klobuchar-like Ionospheric Model for Different Scales Areas. Acta Geod. Cartogr. Sin. 2016, 45, 54–63. [Google Scholar] [CrossRef]
- China Satellite Navigation Office. BeiDou Navigation Satellite System Signal in Space Interface Control Document Open Service Signal B1I (Version 3.0). Available online: http://www.beidou.gov.cn/xt/gfxz/201902/P020190227593621142475.pdf (accessed on 8 June 2021).
- China Satellite Navigation Office. BeiDou Navigation Satellite System Signal in Space Interface Control Document Open Service Signal B1C (Version 1.0). Available online: http://www.beidou.gov.cn/xt/gfxz/201712/P020171226741342013031.pdf (accessed on 8 June 2021).
- China Satellite Navigation Office. BeiDou Navigation Satellite System Signal in Space Interface Control Document Open Service Signal B2a (Version 1.0). Available online: http://www.beidou.gov.cn/xt/gfxz/201712/P020171226742357364174.pdf (accessed on 8 June 2021).
- Zhang, Q.; Zhao, Q.L.; Zhang, H.P.; Hu, Z.G.; Wu, Y. Evaluation on the Precision of Klobuchar Model for BeiDou Navigation Satellite System; Geomatics and Information Science of Wuhan University: Wuhan, China, 2014; Volume 39, pp. 142–146. (In Chinese) [Google Scholar]
- Wang, Z.M.; Bi, T.; Sun, W.; An, J.C.; Liu, S.L. Analysis of GNSS broadcast ionospheric model correction in polar regions. Chin. J. Polar Res. 2016, 28, 235–242. (In Chinese) [Google Scholar]
- Wang, N.; Li, Z.; Yuan, Y.; Huo, X. BeiDou Global Ionospheric delay correction Model (BDGIM): Performance analysis during different levels of solar conditions. GPS Solut. 2021, 25, 1–13. [Google Scholar] [CrossRef]
- Hoque, M.M.; Jakowski, N.; Berdermann, J.; Inst, N. An Ionosphere Broadcast Model for Next Generation GNSS. In Proceedings of the 28th International Technical Meeting of the Satellite Division of the Institute of Navigation (ION GNSS 2015), Tampa, FL, USA, 14–18 September 2015; pp. 3755–3765. [Google Scholar]
- Ye, Y.L.; Liu, C.; Liu, C.J. Evaluation of ionospheric model based on standard point positioning technique. Sci. Surv. Mapp. 2017, 42, 126–131. (In Chinese) [Google Scholar]
- Rovira-Garcia, A.; Ibáñez-Segura, D.; Orús-Perez, R.; Juan, J.M.; Sanz, J.; González-Casado, G. Assessing the quality of ionospheric models through GNSS positioning error: Methodology and results. GPS Solut. 2020, 24, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Wang, A.H.; Chen, J.P.; Zhang, Y.Z.; Meng, L.D.; Wang, J.X. Performance of Selected Ionospheric Models in Multi-Global Navigation Satellite System Single-Frequency Positioning over China. Remote Sens. 2019, 11, 2070. [Google Scholar] [CrossRef] [Green Version]
- Li, W.W.; Li, M.; Hu, Z.G.; Zhao, Q.L. Comparative Analysis of COMPASS and GPS Ionospheric Model on Positioning and Navigation Precision. In Proceedings of the 4th CSNC, Wuhan, China, 15–17 May 2013; pp. 95–99. (In Chinese). [Google Scholar]
- Wang, L.J.; Cai, C.L.; Li, Z.B.; Wei, Z.C.; Li, T.S. COMPASS User Positioning Accuracy Evaluation Based on Single Point Pseudo-Range Positioning Principle. In Proceedings of the 4th CSNC, Wuhan, China, 15–17 May 2013; pp. 168–173. (In Chinese). [Google Scholar]
- Zhang, Q.; Zhao, Q.L.; Zhang, H.P.; Hu, Z.G.; Liu, Z.Y. Research on BeiDou Navigation Satellite System Ionospheric Model Accuracy. In Proceedings of the 4th CSNC, Wuhan, China, 15–17 May 2013; pp. 271–277. (In Chinese). [Google Scholar]
- Zheng, F.; Gu, S.; Gong, X.; Lou, Y.; Fan, L.; Shi, C. Real-time single-frequency pseudorange positioning in China based on regional satellite clock and ionospheric models. GPS Solut. 2020, 24, 6. [Google Scholar] [CrossRef]
- Guo, S.R.; Cai, H.L.; Meng, Y.N.; Geng, C.J.; Jia, X.L.; Mao, Y.; Geng, T.; Rao, Y.N.; Zhang, H.J.; Xie, X. BDS-3 RNSS technical characteristics and service performance. Acta Geod. Cartogr. Sin. 2019, 48, 810–821. (In Chinese) [Google Scholar]
- Wang, W.; Liu, C.; Wang, Y.P.; Jiang, Y.; Xu, F. Analysis on the influence of geomagnetic storm of BDS pseudorange positioning. GNSS World China 2019, 44, 82–88. (In Chinese) [Google Scholar]
- Guo, F.; Zhang, X.; Wang, J. Timing group delay and differential code bias corrections for BeiDou positioning. J. Geod. 2015, 89, 427–445. [Google Scholar] [CrossRef]
- Ge, Y.; Zhou, F.; Sun, B.; Wang, S.; Shi, B. The Impact of Satellite Time Group Delay and Inter-Frequency Differential Code Bias Corrections on Multi-GNSS Combined Positioning. Sensors 2017, 17, 602. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, J.; Gong, X.; Chen, Q. The update of BDS-2 TGD and its impact on positioning. Adv. Space Res. 2020, 65, 2645–2661. [Google Scholar] [CrossRef]
- Li, Z.; Yuan, Y.; Wang, N.; Hernandez-Pajares, M.; Huo, X. SHPTS: Towards a new method for generating precise global ionospheric TEC map based on spherical harmonic and generalized trigonometric series functions. J. Geod. 2015, 89, 331–345. [Google Scholar] [CrossRef]
- Yang, Y.; Tang, J.; Montenbruck, O. Chinese Navigation Satellite Systems. In Springer Handbook of Global Navigation Satellite Systems; Teunissen, P.J.G., Montenbruck, O., Eds.; Springer: Cham, Switzerland, 2017; pp. 273–304. [Google Scholar]
- Hernández-Pajares, M.; Juan, J.M.; Sanz, J.; Orus, R.; Garcia-Rigo, A.; Feltens, J.; Komjathy, A.; Schaer, S.C.; Krankowski, A. The IGS VTEC maps: A reliable source of ionospheric information since 1998. J. Geod. 2009, 83, 263–275. [Google Scholar] [CrossRef]
- Li, Z.S.; Wang, N.B.; Li, M.; Zhou, K.; Yuan, Y.B.; Yuan, H. Evaluation and analysis of the global ionospheric TEC map in the frame of international GNSS services. Chin. J. Geophys. 2017, 60, 3718–3729. [Google Scholar] [CrossRef]

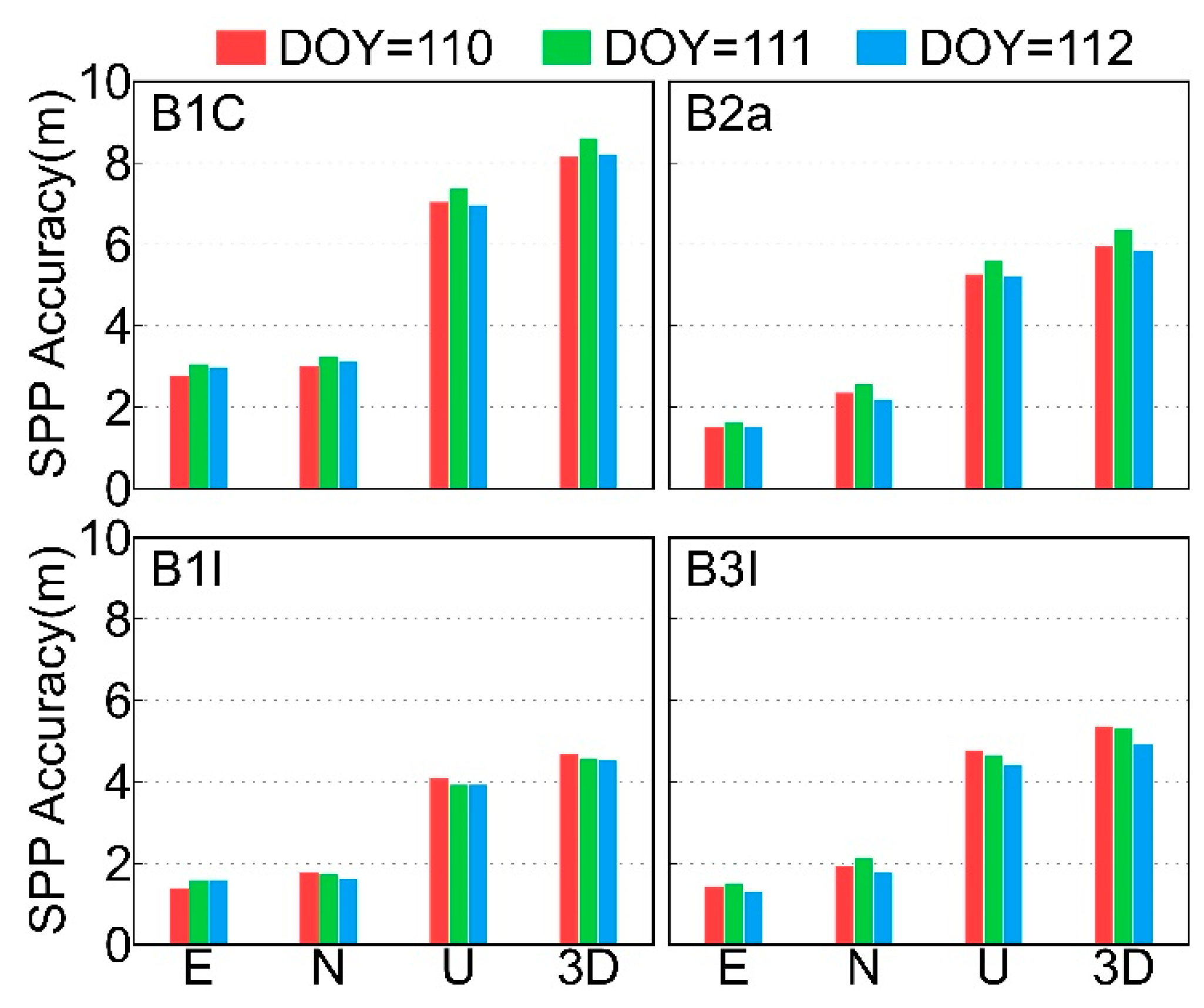
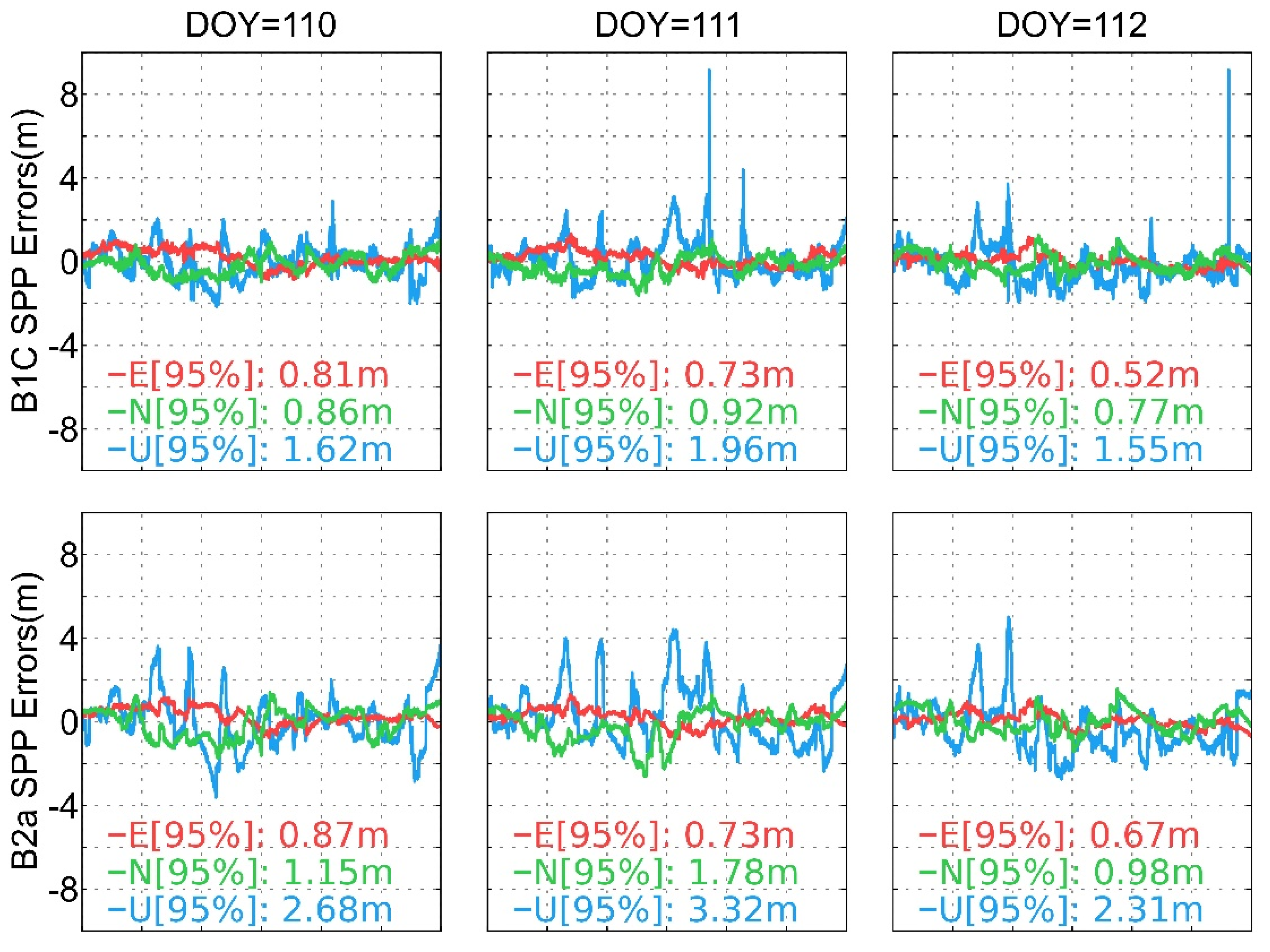
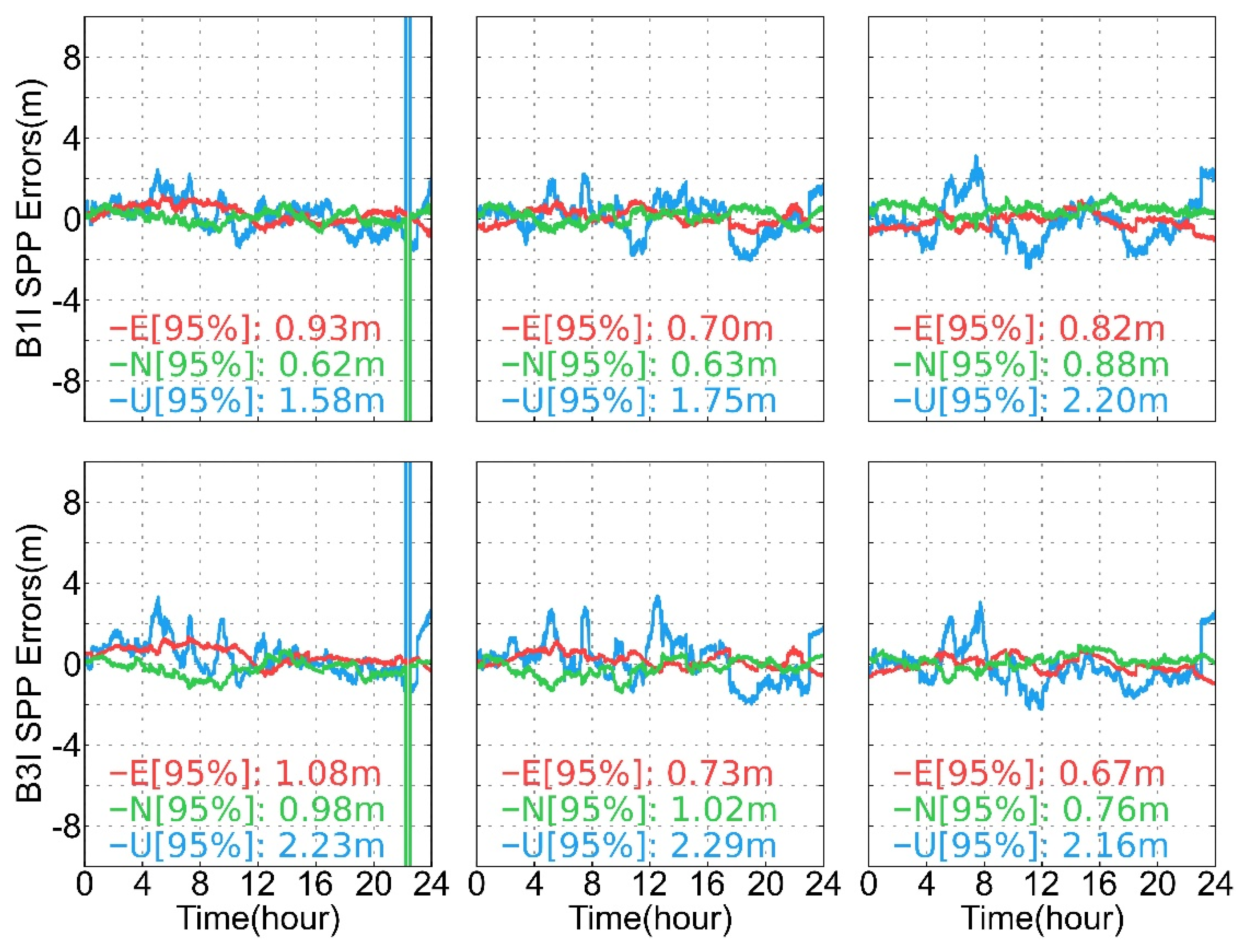
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | |
|---|---|---|---|---|---|---|---|---|---|
| / | 0/0 | 1/0 | 1/1 | 1/−1 | 2/0 | 2/1 | 2/−1 | 2/2 | 2/−2 |
| Station | Latitude (°) | Longitude (°) | Location | Receiver Type | Source |
|---|---|---|---|---|---|
| CNYR | 78.92 | 11.93 | Arctic | UNICORE UB4B0I | iGMAS |
| KIRU | 67.86 | 20.97 | Kiruna, Sweden | SEPT POLARX5 | IGS |
| BRCH | 52.26 | 10.53 | Braunschweig, Germany | CETC-54-GMR-4016 | iGMAS |
| ICUK | 51.51 | −0.13 | London, England | CETC-54-GMR-4016 | iGMAS |
| CLGY | 51.05 | −114.07 | Calgary, Canada | CETC-54-GMR-4016 | iGMAS |
| REDU | 50.00 | 5.14 | Redu, Belgium | SEPT POLARX5 | IGS |
| PADO | 45.41 | 11.90 | Padova, Italy | SEPT POLARX5 | IGS |
| CHU1 | 43.79 | 125.44 | Changchun, China | BD070 | iGMAS |
| GUA1 | 43.47 | 87.18 | Urumqi, China | BD070 | iGMAS |
| CEBR | 40.45 | −4.37 | Cebreros, Spain | SEPT POLARX5TR | IGS |
| BJF1 | 39.61 | 115.89 | Beijing, China | CETC-54-GMR-4016 | iGMAS |
| XIA1 | 34.37 | 109.22 | Xian, China | GNSS_GGR | iGMAS |
| SHA1 | 31.10 | 121.20 | Shanghai, China | UNICORE UB4B0I | iGMAS |
| WUH1 | 30.52 | 114.49 | Wuhan, China | CETC-54-GMR-4016 | iGMAS |
| LHA1 | 29.66 | 91.10 | Lhasa, China | CETC-54-GMR-4016 | iGMAS |
| KNDY | 7.29 | 80.63 | Kandy, Sri Lanka | CETC-54-GMR-4016 | iGMAS |
| FAA1 | −17.56 | −149.61 | Papeete, SOCI | SEPT POLARX5 | IGS |
| RDJN | −22.91 | −43.20 | Rio, Brazil | UNICORE UB4B0I | iGMAS |
| NNOR | −31.05 | 116.19 | New Norcia, Australia | SEPT POLARX5TR | IGS |
| CANB | −35.30 | 149.20 | Canberra, Australia | CETC-54-GMR-4011 | iGMAS |
| MGUE | −35.78 | −69.40 | Malargue, Argentina | SEPT POLARX5TR | IGS |
| ZHON | −69.37 | 76.37 | Antarctic | CETC-54-GMR-4011 | iGMAS |
| Items | Settings |
|---|---|
| Sampling interval | 30s |
| Elevation cutoff | 5° |
| Orbits and clocks | Broadcast ephemeris |
| Tropospheric delay | Saastamoinen model |
| Ionospheric delay | Klobuchar/BDGIM/GIM |
| Hardware delay | B1I: TGD; B1C/B2a: DCB |
| Weight | Elevation-based |
| Estimator | Weighted least squares |
| Regions | B1C | B2a | ||||
|---|---|---|---|---|---|---|
| BDGIM (m) | Klobuchar (m) | Improvement Percentage (%) | BDGIM (m) | Klobuchar (m) | Improvement Percentage (%) | |
| High-latitude | 2.98 | 13.68 | 78.2 | 4.00 | 23.77 | 83.2 |
| Mid-latitude | 3.95 | 4.18 | 5.5 | 4.89 | 6.10 | 19.8 |
| Low-latitude | 5.50 | 4.12 | −33.5 | 8.05 | 5.99 | −34.4 |
| Global | 4.10 | 5.46 | 24.9 | 5.35 | 8.49 | 37.0 |
| Region | B1I | B3I | ||||
|---|---|---|---|---|---|---|
| BDGIM (m) | Klobuchar (m) | Improvement Percentage (%) | BDGIM (m) | Klobuchar (m) | Improvement Percentage (%) | |
| High-latitude | 3.66 | 12.40 | 70.5 | 3.66 | 17.77 | 79.4 |
| Mid-latitude | 4.25 | 4.02 | −5.7 | 4.22 | 4.55 | 7.3 |
| Low-latitude | 5.48 | 4.17 | −31.4 | 6.57 | 5.00 | −31.4 |
| Global | 4.39 | 5.19 | 15.4 | 4.57 | 6.44 | 29.0 |
| Season | B1C | B2a | ||||
|---|---|---|---|---|---|---|
| BDGIM | Klobuchar | GIM | BDGIM | Klobuchar | GIM | |
| Spring | 8.23 | 14.71 | 7.66 | 5.78 | 20.51 | 3.88 |
| Summer | 8.84 | 8.23 | 8.16 | 5.56 | 4.99 | 3.47 |
| Autumn | 10.28 | 8.45 | 8.36 | 8.77 | 5.70 | 3.71 |
| Winter | 4.13 | 4.92 | 3.29 | 5.32 | 7.38 | 3.64 |
| Season | B1I | B3I | ||||
|---|---|---|---|---|---|---|
| BDGIM | Klobuchar | GIM | BDGIM | Klobuchar | GIM | |
| Spring | 4.43 | 11.00 | 3.79 | 4.74 | 15.83 | 3.56 |
| Summer | 4.38 | 3.56 | 3.33 | 5.13 | 4.23 | 3.50 |
| Autumn | 6.31 | 3.62 | 3.60 | 8.09 | 4.80 | 3.85 |
| Winter | 4.40 | 4.69 | 3.50 | 4.49 | 5.65 | 2.99 |
| Station | BDGIM | Klobuchar | ||||||
|---|---|---|---|---|---|---|---|---|
| E | N | U | 3D | E | N | U | 3D | |
| CNYR | 1.71 | 1.60 | 5.26 | 5.76 | 19.07 | 24.41 | 59.20 | 66.81 |
| KIRU | 1.18 | 1.98 | 4.19 | 4.78 | 11.82 | 25.34 | 36.68 | 46.12 |
| BRCH | 1.34 | 2.74 | 5.61 | 6.39 | 2.08 | 8.03 | 8.77 | 12.07 |
| ICUK | 1.29 | 3.03 | 5.91 | 6.77 | 1.86 | 8.28 | 8.48 | 12.00 |
| CLGY | 1.33 | 1.81 | 5.07 | 5.55 | 1.85 | 6.84 | 10.85 | 12.96 |
| REDU | 1.41 | 2.71 | 5.06 | 5.91 | 2.87 | 8.64 | 6.16 | 10.99 |
| PADO | 1.14 | 2.31 | 4.29 | 5.00 | 1.78 | 5.93 | 4.59 | 7.71 |
| CHU1 | 0.96 | 1.43 | 4.54 | 4.86 | 1.22 | 2.52 | 4.76 | 5.52 |
| GUA1 | 0.72 | 1.09 | 2.33 | 2.67 | 0.75 | 1.86 | 3.30 | 3.86 |
| CEBR | 1.12 | 2.35 | 5.75 | 6.31 | 1.24 | 3.00 | 4.14 | 5.26 |
| BJF1 | 1.68 | 2.07 | 4.44 | 5.18 | 1.55 | 2.39 | 4.93 | 5.69 |
| XIA1 | 1.02 | 1.18 | 3.10 | 3.47 | 0.95 | 1.31 | 3.46 | 3.82 |
| SHA1 | 2.15 | 3.02 | 6.30 | 7.31 | 2.05 | 2.99 | 6.12 | 7.11 |
| WUH1 | 1.97 | 3.53 | 6.76 | 7.88 | 1.88 | 3.15 | 6.78 | 7.71 |
| LHA1 | 2.83 | 4.57 | 9.45 | 10.87 | 2.79 | 4.18 | 9.18 | 10.47 |
| KNDY | 1.72 | 2.71 | 6.19 | 6.97 | 1.57 | 2.12 | 5.04 | 5.69 |
| FAA1 | 3.67 | 3.57 | 11.02 | 12.15 | 3.52 | 3.70 | 6.75 | 8.46 |
| RDJN | 2.57 | 2.84 | 6.67 | 7.69 | 2.56 | 2.89 | 8.10 | 8.97 |
| NNOR | 1.05 | 1.71 | 4.35 | 4.79 | 1.11 | 2.06 | 5.97 | 6.41 |
| CANB | 9.14 | 3.48 | 5.38 | 11.16 | 9.10 | 4.47 | 4.97 | 11.29 |
| MGUE | 1.19 | 1.50 | 3.78 | 4.24 | 1.24 | 1.51 | 4.46 | 4.87 |
| ZHON | 1.74 | 2.00 | 3.14 | 4.11 | 7.27 | 13.41 | 17.91 | 23.53 |
| Mean | 1.95 | 2.42 | 5.39 | 6.22 | 3.64 | 6.32 | 10.48 | 12.77 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, G.; Yin, Z.; Hu, Z.; Chen, G.; Li, W.; Bo, Y. Analysis of the BDGIM Performance in BDS Single Point Positioning. Remote Sens. 2021, 13, 3888. https://doi.org/10.3390/rs13193888
Wang G, Yin Z, Hu Z, Chen G, Li W, Bo Y. Analysis of the BDGIM Performance in BDS Single Point Positioning. Remote Sensing. 2021; 13(19):3888. https://doi.org/10.3390/rs13193888
Chicago/Turabian StyleWang, Guangxing, Zhihao Yin, Zhigang Hu, Gang Chen, Wei Li, and Yadong Bo. 2021. "Analysis of the BDGIM Performance in BDS Single Point Positioning" Remote Sensing 13, no. 19: 3888. https://doi.org/10.3390/rs13193888
APA StyleWang, G., Yin, Z., Hu, Z., Chen, G., Li, W., & Bo, Y. (2021). Analysis of the BDGIM Performance in BDS Single Point Positioning. Remote Sensing, 13(19), 3888. https://doi.org/10.3390/rs13193888









