A Comparative Study on the Solar Radiation Pressure Modeling in GPS Precise Orbit Determination
Abstract
:1. Introduction
2. Methodology
- Full phase: the Sun is fully visible to the satellite, therefore ;
- Penumbra: the satellite is in the penumbra area of the Earth and the Moon and it can receive only part of the solar irradiance from the Sun, therefore [35] (pp. 81–83):where denotes the total area of the Sun occulted by the Earth and the Moon, and the apparent radius of the Sun;
- Umbra: the satellite is in the umbra of satellite, therefore
3. Data Processing Strategy
- Analyses of the estimated SRP parameters: the estimated ECOM parameters should not show systematic biases if the a priori model can describe all important characteristics of the satellite. The correlation between the satellite orbit dynamic parameters can also measure the association of these parameters;
- Day boundary discontinuities (DBD) of the satellite orbits: 3D distance between the orbital positions of two consecutive arcs are commonly used to assess the internal consistency of the orbit solution;
- Agreement of the Earth rotation parameters (ERPs) to the IERS EOP 14C04 product [45]: the GPS technique provides precise polar motion and LoD estimates, and thus the ERP estimates from different solutions can be compared to the IERS product. We calculated the weighted STD (WSTD) of the differences between the GPS POD solution and the IERS 14C04 product [48] as:
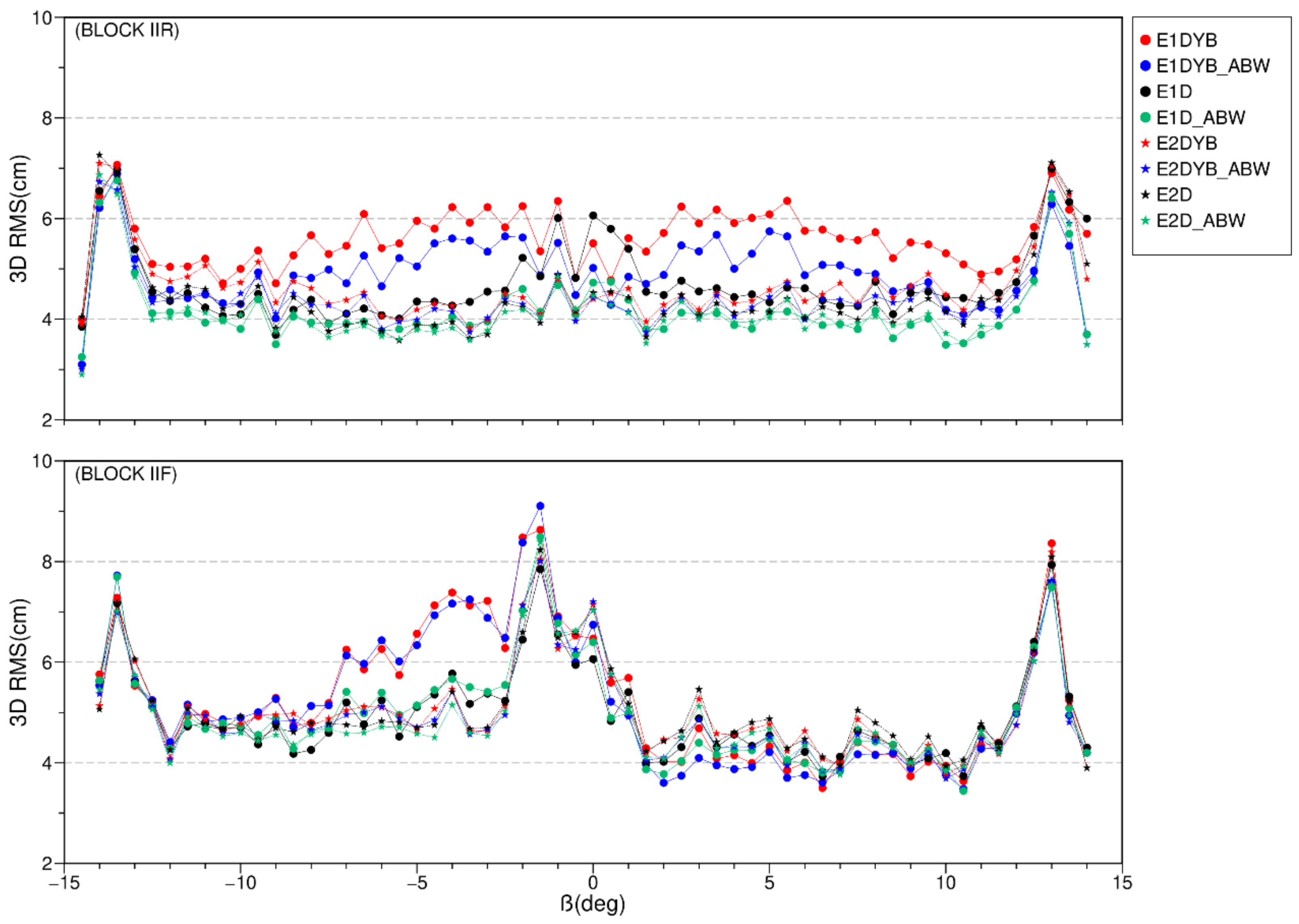
4. Results and Discussions
4.1. Estimated ECOM Parameters
4.2. Day Boundary Discontinuity of Satellite Orbits
4.3. Impact on the Earth Rotation Parameters
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Altamimi, Z.; Rebischung, P.; Métivier, L.; Collilieux, X. ITRF2014: A new release of the International Terrestrial Reference Frame modeling nonlinear station motions. J. Geophys. Res. Solid Earth 2016, 121, 6109–6131. [Google Scholar] [CrossRef] [Green Version]
- Sośnica, K.; Bury, G.; Zajdel, R.; Strugarek, D.; Drożdżewski, M.; Kazmierski, K. Estimating global geodetic parameters using SLR observations to Galileo, GLONASS, BeiDou, GPS, and QZSS. Earth Planets Space 2019, 71, 20. [Google Scholar] [CrossRef] [Green Version]
- Argus, D.F.; Heflin, M.B. Plate motion and crustal deformation estimated with geodetic data from the Global Positioning System. Geophys. Res. Lett. 1995, 22, 1973–1976. [Google Scholar] [CrossRef]
- Calais, E.; Han, J.-Y.; DeMets, C.; Nocquet, J.-M. Deformation of the North American plate interior from a decade of continuous GPS measurements. J. Geophys. Res. Space Phys. 2006, 111, B06402. [Google Scholar] [CrossRef] [Green Version]
- Wang, J.; Wu, Z.; Semmling, M.; Zus, F.; Gerland, S.; Ramatschi, M.; Ge, M.; Wickert, J.; Schuh, H. Retrieving Precipitable Water Vapor From Shipborne Multi-GNSS Observations. Geophys. Res. Lett. 2019, 46, 5000–5008. [Google Scholar] [CrossRef] [Green Version]
- Wu, Z.; Liu, Y.; Liu, Y.; Wang, J.; He, X.; Xu, W.; Ge, M.; Schuh, H. Validating HY-2A CMR precipitable water vapor using ground-based and shipborne GNSS observations. Atmos. Meas. Tech. 2020, 13, 4963–4972. [Google Scholar] [CrossRef]
- Griffiths, J. Combined orbits and clocks from IGS second reprocessing. J. Geod. 2019, 93, 177–195. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Duan, B.; Hugentobler, U. Enhanced solar radiation pressure model for GPS satellites considering various physical effects. GPS Solut. 2021, 25, 1–14. [Google Scholar] [CrossRef]
- Kouba, J. A simplified yaw-attitude model for eclipsing GPS satellites. GPS Solut. 2009, 13, 1–12. [Google Scholar] [CrossRef]
- Fliegel, H.F.; Gallini, T.E.; Swift, E.R. Global Positioning System Radiation Force Model for geodetic applications. J. Geophys. Res. Space Phys. 1992, 97, 559. [Google Scholar] [CrossRef]
- Fliegel, H.F.; Gallini, T.E. Solar force modeling of block IIR Global Positioning System satellites. J. Spacecr. Rocket. 1996, 33, 863–866. [Google Scholar] [CrossRef]
- Rodriguez-Solano, C.J. Impact of Non-Conservative Force Modelling on GNSS Satellite Orbits and Global Solutions. Ph.D. Dissertation, Technische Universität München, Munich, Germany, 2014. [Google Scholar]
- Rodriguez-Solano, C.; Hugentobler, U.; Steigenberger, P. Adjustable box-wing model for solar radiation pressure impacting GPS satellites. Adv. Space Res. 2012, 49, 1113–1128. [Google Scholar] [CrossRef]
- Fliegel, H.; Feess, W.; Layton, W.; Rhodus, N. The GPS radiation Force Model. In Proceedings of the 1st International Symposium on Precise Positioning with the Global Positioning System, Rockville, MA, USA, 15–19 April 1985. [Google Scholar]
- Fliegel, H. Radiation pressure models for Block II GPS satellites. In Proceedings of the Fifth International Geodetic Symposium on Satellite Positioning, Las Cruces, NM, USA, 13–17 March 1989; pp. 789–798. [Google Scholar]
- Bar-Sever, Y.; Kuang, D. New empirically derived solar radiation pressure model for global positioning system satellites during eclipse seasons. IPN Prog. Rep. 2005, 1, 42–160. [Google Scholar]
- Beutler, G.; Brockmann, E.; Gurtner, W.; Hugentobler, U.; Mervart, L.; Rothacher, M.; Verdun, A. Extended orbit modeling techniques at the CODE processing center of the international GPS service for geodynamics (IGS): Theory and initial results. Manuscr. Geod. 1994, 19, 367–386. [Google Scholar]
- Springer, T.A.; Beutler, G.; Rothacher, M. A New Solar Radiation Pressure Model for GPS Satellites. GPS Solut. 1999, 2, 50–62. [Google Scholar] [CrossRef]
- Arnold, D.; Meindl, M.; Beutler, G.; Dach, R.; Schaer, S.; Lutz, S.; Prange, L.; Sośnica, K.; Mervart, L.; Jäggi, A. CODE’s new solar radiation pressure model for GNSS orbit determination. J. Geod. 2015, 89, 775–791. [Google Scholar] [CrossRef] [Green Version]
- Prange, L.; Villiger, A.; Sidorov, D.; Schaer, S.; Beutler, G.; Dach, R.; Jäggi, A. Overview of CODE’s MGEX solution with the focus on Galileo. Adv. Space Res. 2020, 66, 2786–2798. [Google Scholar] [CrossRef]
- Deng, Z.; Nischan, T.; Bradke, M. Multi-GNSS Rapid Orbit-, Clock- & EOP-Product Series; GFZ Data Services: Potsdam, Germany, 2017. [Google Scholar]
- Guo, J.; Xu, X.; Zhao, Q.; Liu, J. Precise orbit determination for quad-constellation satellites at Wuhan University: Strategy, result validation, and comparison. J. Geod. 2016, 90, 143–159. [Google Scholar] [CrossRef]
- Rodriguez-Solano, C.J.; Hugentobler, U.; Steigenberger, P.; Blosfeld, M.; Fritsche, M. Reducing the draconitic errors in GNSS geodetic products. J. Geod. 2014, 88, 559–574. [Google Scholar] [CrossRef]
- Meindl, M.; Beutler, G.; Thaller, D.; Dach, R.; Jäggi, A. Geocenter coordinates estimated from GNSS data as viewed by perturbation theory. Adv. Space Res. 2013, 51, 1047–1064. [Google Scholar] [CrossRef]
- Montenbruck, O.; Steigenberger, P.; Hugentobler, U. Enhanced solar radiation pressure modeling for Galileo satellites. Bull. Géod. 2014, 89, 283–297. [Google Scholar] [CrossRef]
- Steigenberger, P.; Montenbruck, O.; Hugentobler, U. GIOVE-B solar radiation pressure modeling for precise orbit determination. Adv. Space Res. 2014, 55, 1422–1431. [Google Scholar] [CrossRef]
- Bury, G.; Zajdel, R.; Sośnica, K. Accounting for perturbing forces acting on Galileo using a box-wing model. GPS Solut. 2019, 23, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Li, X.; Yuan, Y.; Huang, J.; Zhu, Y.; Wu, J.; Xiong, Y.; Li, X.; Zhang, K. Galileo and QZSS precise orbit and clock determination using new satellite metadata. J. Geod. 2019, 93, 1123–1136. [Google Scholar] [CrossRef]
- Sibthorpe, A.; Bertiger, W.; Desai, S.D.; Haines, B.; Harvey, N.; Weiss, J.P. An evaluation of solar radiation pressure strategies for the GPS constellation. J. Geod. 2011, 85, 505–517. [Google Scholar] [CrossRef]
- Chang, X.; Männel, B.; Schuh, H. An analysis of a priori and empirical solar radiation pressure models for GPS satellites. Adv. Geosci. 2021, 55, 33–45. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, Y.; Tian, Z.; Dai, X.; Qing, Y.; Li, M. Impact of ECOM Solar Radiation Pressure Models on Multi-GNSS Ultra-Rapid Orbit Determination. Remote Sens. 2019, 11, 3024. [Google Scholar] [CrossRef] [Green Version]
- Guo, J.; Chen, G.; Zhao, Q.; Liu, J.; Liu, X. Comparison of solar radiation pressure models for BDS IGSO and MEO satellites with emphasis on improving orbit quality. GPS Solut. 2017, 21, 511–522. [Google Scholar] [CrossRef] [Green Version]
- Sidorov, D.; Dach, R.; Polle, B.; Prange, L.; Jäggi, A. Adopting the empirical CODE orbit model to Galileo satellites. Adv. Space Res. 2020, 66, 2799–2811. [Google Scholar] [CrossRef]
- Montenbruck, O.; Schmid, R.; Mercier, F.; Steigenberger, P.; Noll, C.; Fatkulin, R.; Kogure, S.; Ganeshan, A. GNSS satellite geometry and attitude models. Adv. Space Res. 2015, 56, 1015–1029. [Google Scholar] [CrossRef] [Green Version]
- Montenbruck, O.; Gill, E.; Lutze, F. Satellite Orbits: Models, Methods, and Applications. Appl. Mech. Rev. 2002, 55, B27–B28. [Google Scholar] [CrossRef]
- Marquis, W.; Krier, C. Examination of the GPS block IIR solar pressure model. In Proceedings of the 13th International Technical Meeting of the Satellite Division of The Institute of Navigation (ION GPS 2000), Salt Lake City, UT, USA, September 19–22 2000; pp. 407–415. [Google Scholar]
- Liu, J.; Ge, M. PANDA software and its preliminary result of positioning and orbit determination. Wuhan Univ. J. Nat. Sci. 2003, 8, 603. [Google Scholar]
- Bar-Sever, Y.E. A new model for GPS yaw attitude. J. Geod. 1996, 70, 714–723. [Google Scholar] [CrossRef]
- Dilssner, F.; Springer, T.; Enderle, W. GPS IIF yaw attitude control during eclipse season. In Proceedings of the AGU Fall Meeting Abstracts, San Francisco, CA, USA, 5–9 December 2011. [Google Scholar]
- Kuang, D.; Desai, S.; Sibois, A. Observed features of GPS Block IIF satellite yaw maneuvers and corresponding modeling. GPS Solut. 2017, 21, 739–745. [Google Scholar] [CrossRef]
- Petit, G.; Luzum, B. IERS Conventions (2010); Bureau International des Poids et Mesures: Sevres, France, 2010. [Google Scholar]
- Böhm, J.; Moeller, G.; Schindelegger, M.; Pain, G.; Weber, R. Development of an improved empirical model for slant delays in the troposphere (GPT2w). GPS Solut. 2015, 19, 433–441. [Google Scholar] [CrossRef] [Green Version]
- Boehm, J.; Niell, A.; Tregoning, P.; Schuh, H. Global Mapping Function (GMF): A new empirical mapping function based on numerical weather model data. Geophys. Res. Lett. 2006, 33, 33. [Google Scholar] [CrossRef] [Green Version]
- Chen, G.; Herring, T. Effects of atmospheric azimuthal asymmetry on the analysis of space geodetic data. J. Geophys. Res. Space Phys. 1997, 102, 20489–20502. [Google Scholar] [CrossRef]
- Ge, M.; Gendt, G.; Dick, G.; Zhang, F.P. Improving carrier-phase ambiguity resolution in global GPS network solutions. J. Geod. 2005, 79, 103–110. [Google Scholar] [CrossRef]
- Ge, M.; Gendt, G.; Dick, G.; Zhang, F.P.; Rothacher, M. A New Data Processing Strategy for Huge GNSS Global Networks. J. Geod. 2006, 80, 199–203. [Google Scholar] [CrossRef]
- Sośnica, K.; Thaller, D.; Dach, R.; Steigenberger, P.; Beutler, G.; Arnold, D.; Jaggi, A. Satellite laser ranging to GPS and GLONASS. J. Geod. 2015, 89, 725–743. [Google Scholar] [CrossRef] [Green Version]
- Bizouard, C.; Lambert, S.; Gattano, C.; Becker, O.; Richard, J.-Y. The IERS EOP 14C04 solution for Earth orientation parameters consistent with ITRF 2014. J. Geod. 2019, 93, 621–633. [Google Scholar] [CrossRef]
- Rodriguez-Solano, C.; Hugentobler, U.; Steigenberger, P.; Allende-Alba, G. Improving the orbits of GPS block IIA satellites during eclipse seasons. Adv. Space Res. 2013, 52, 1511–1529. [Google Scholar] [CrossRef]
- Bury, G.; Sośnica, K.; Zajdel, R.; Strugarek, D. Toward the 1-cm Galileo orbits: Challenges in modeling of perturbing forces. J. Geod. 2020, 94, 16. [Google Scholar] [CrossRef] [Green Version]
- Rothacher, M.; Beutler, G.; Herring, T.; Weber, R. Estimation of nutation using the Global Positioning System. J. Geophys. Res. Space Phys. 1999, 104, 4835–4859. [Google Scholar] [CrossRef]
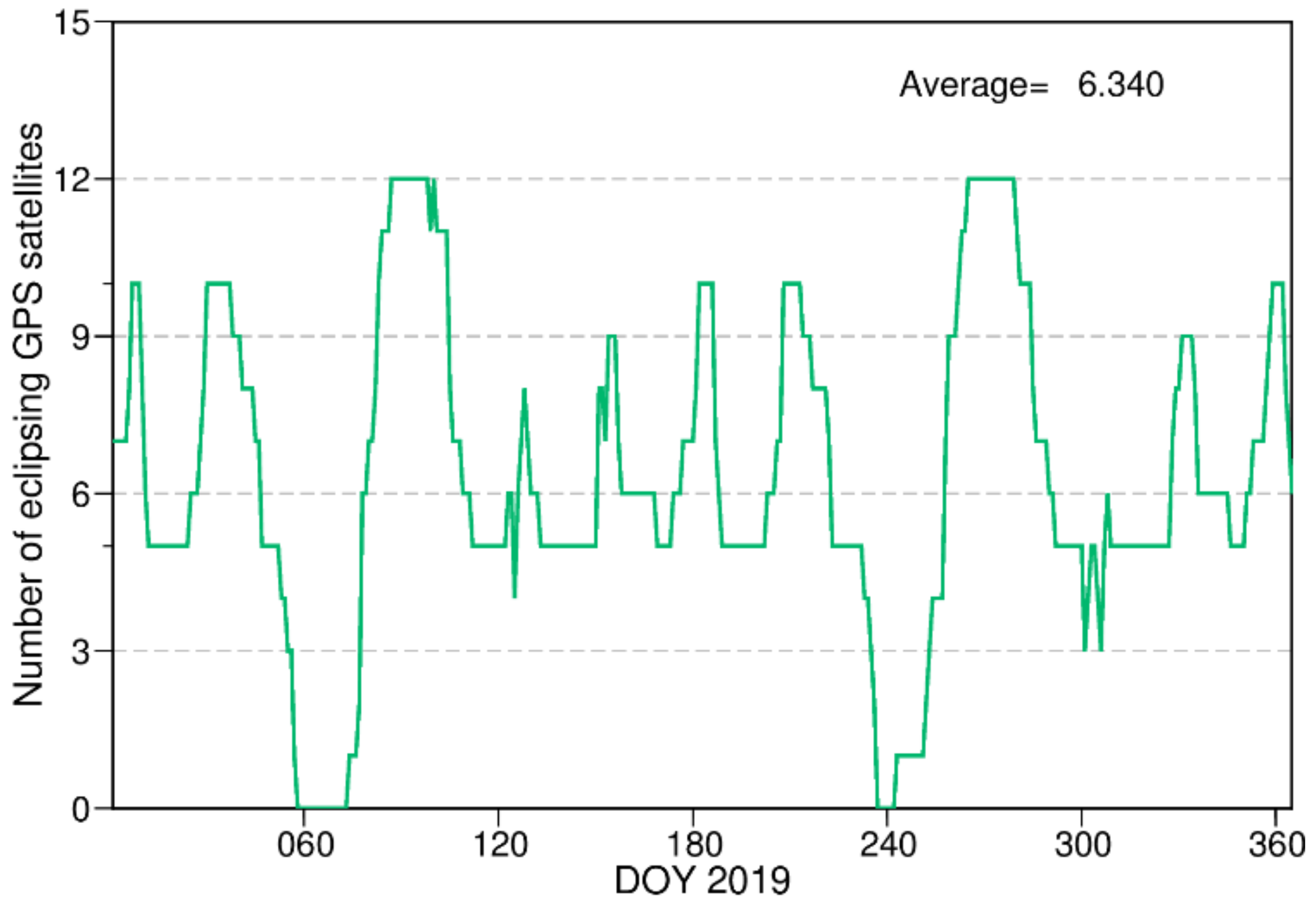

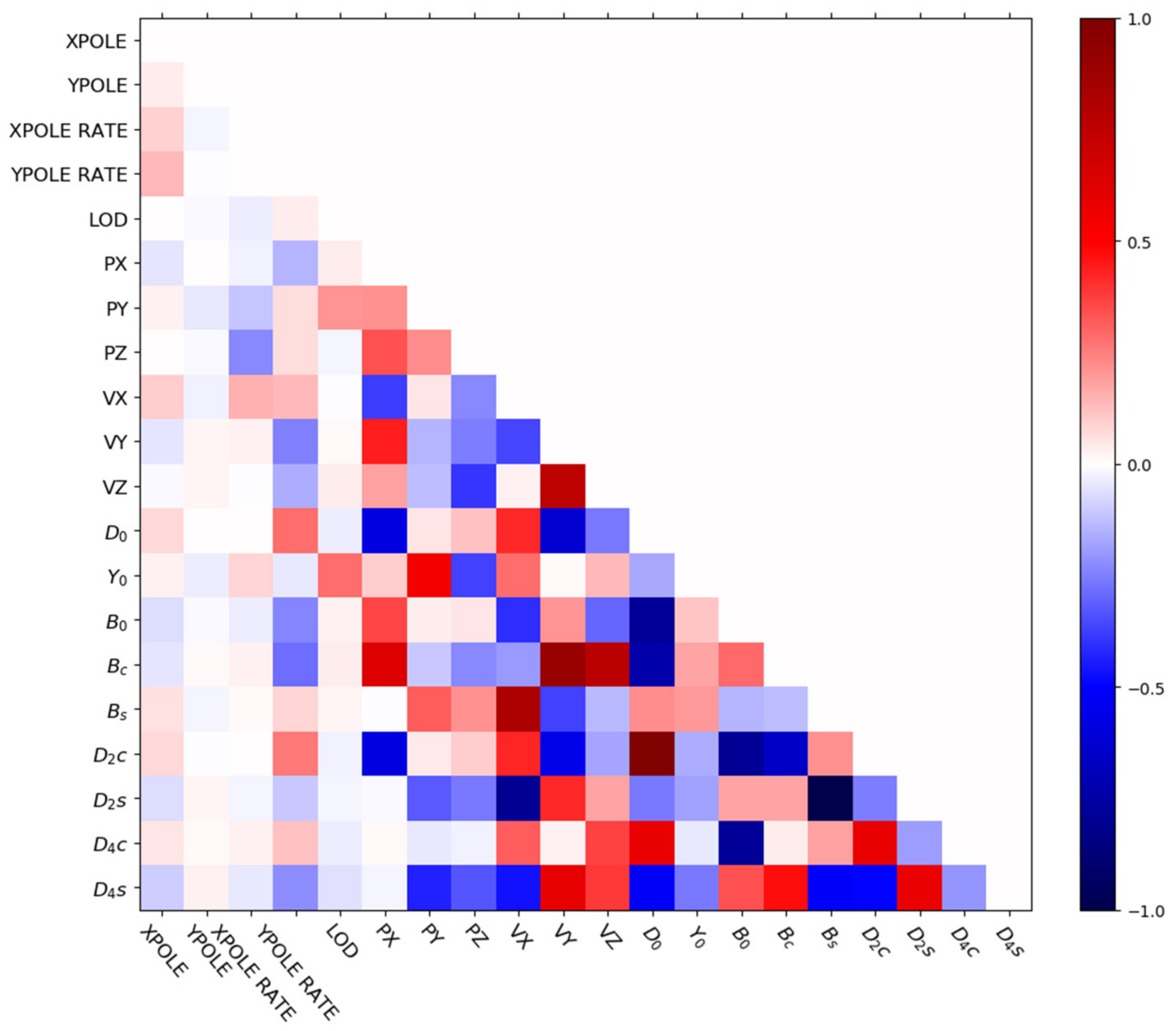
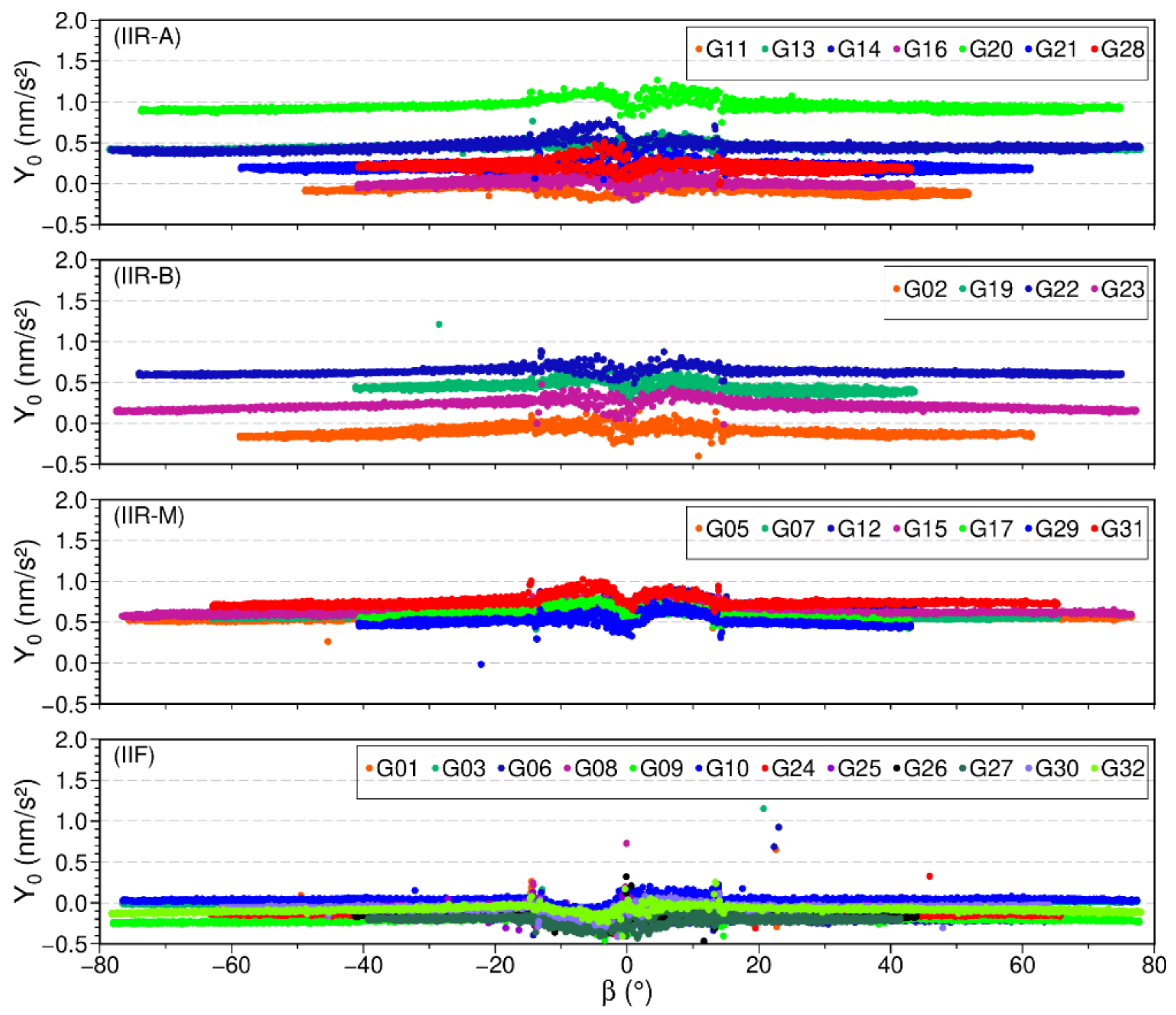

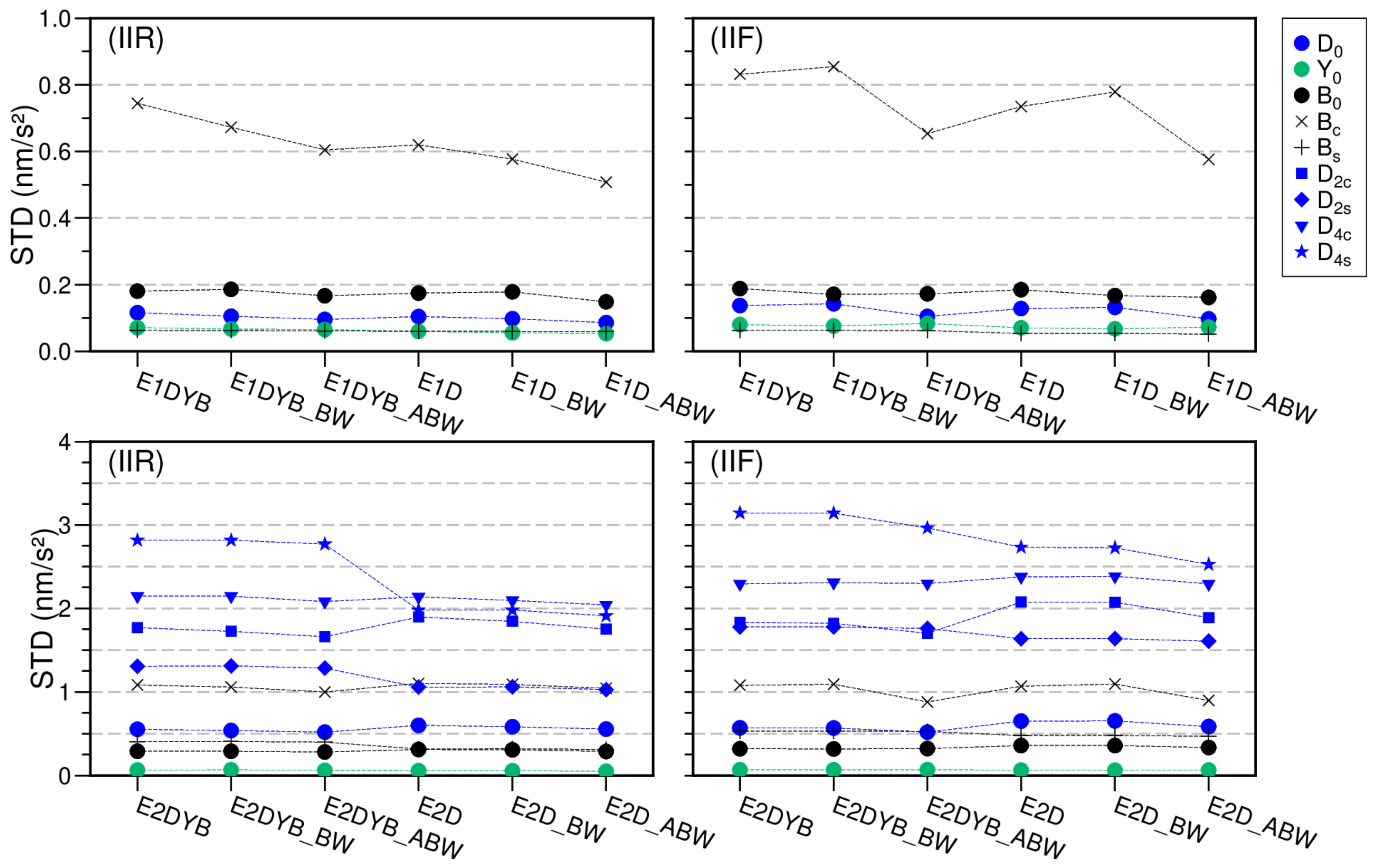
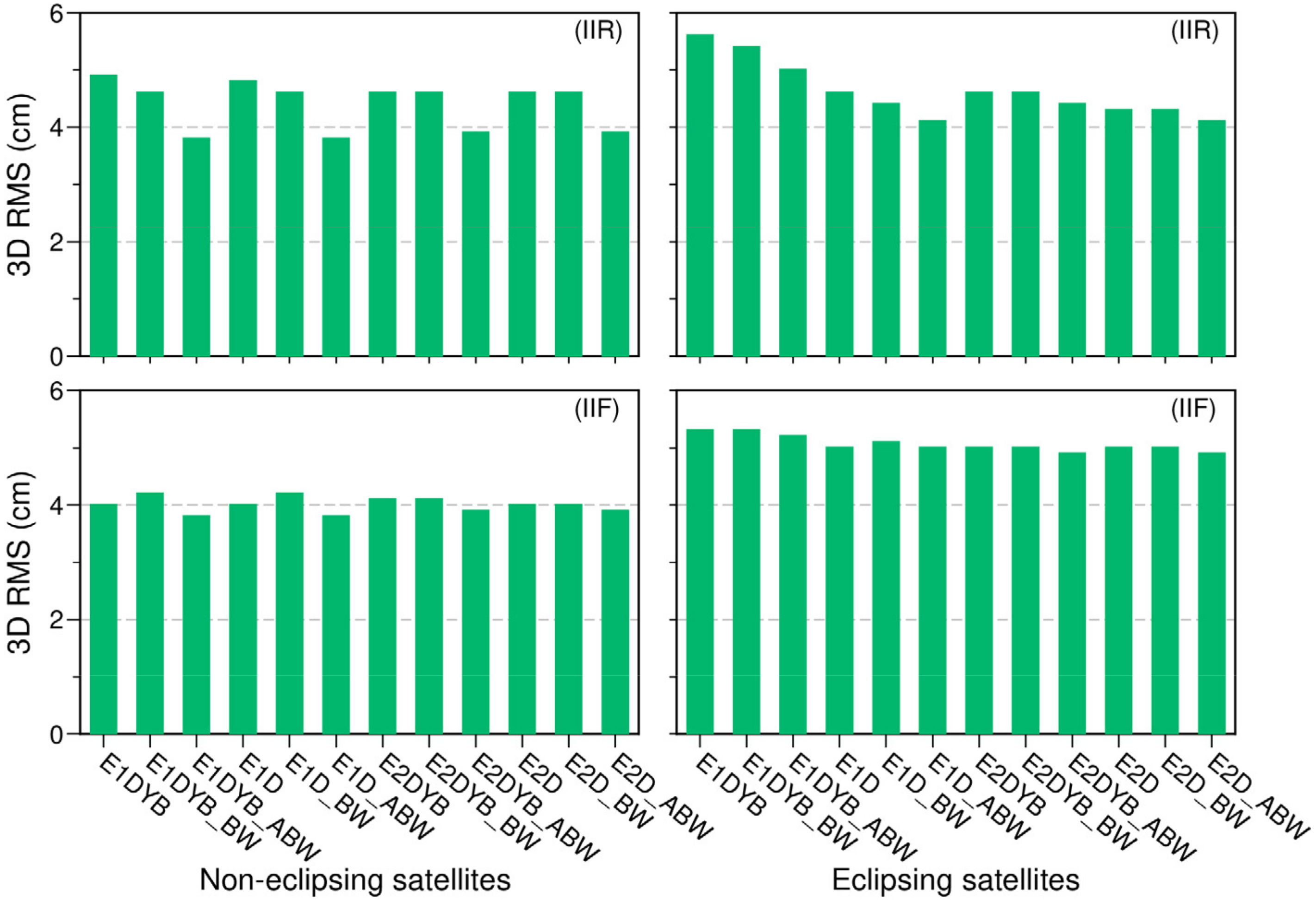
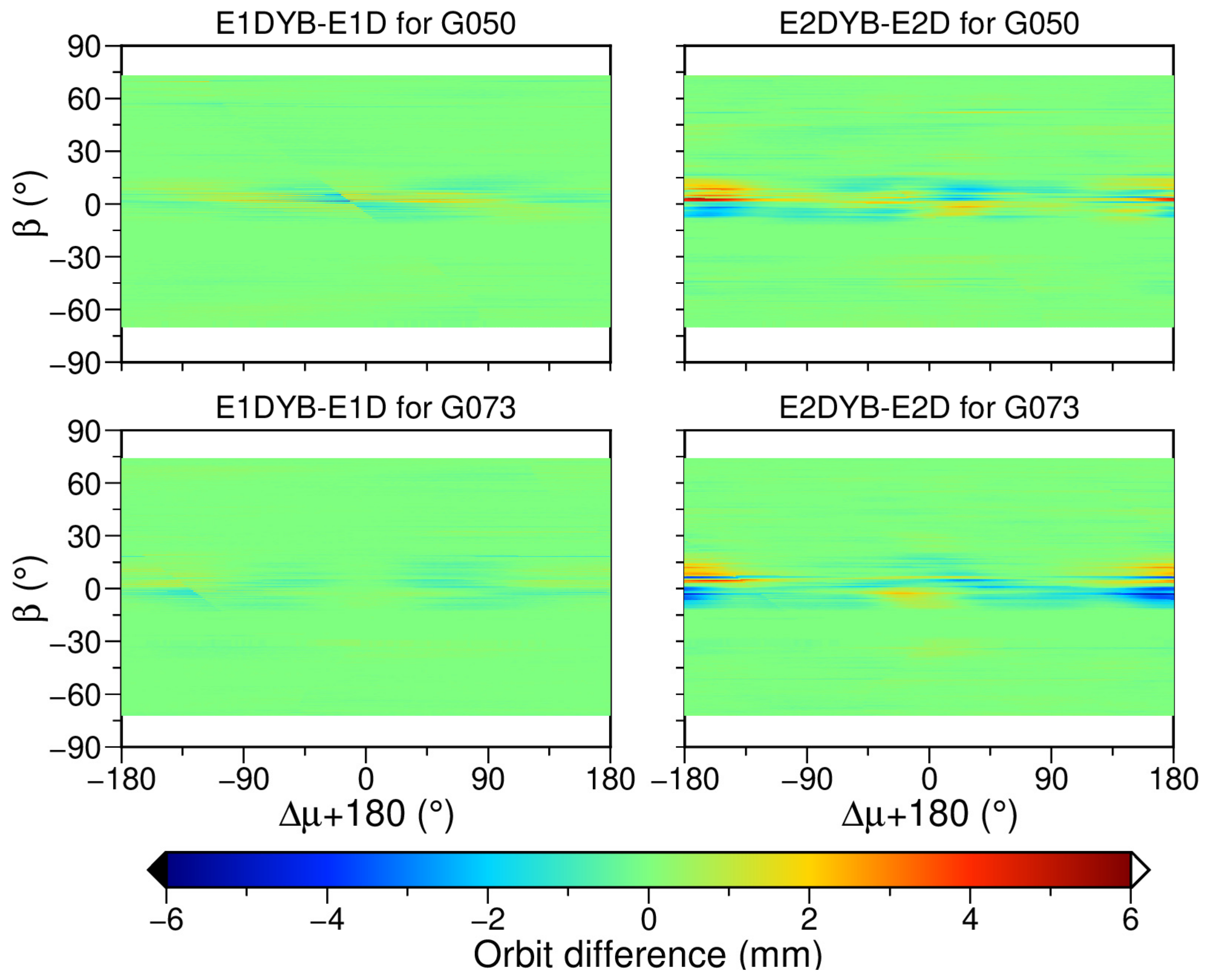
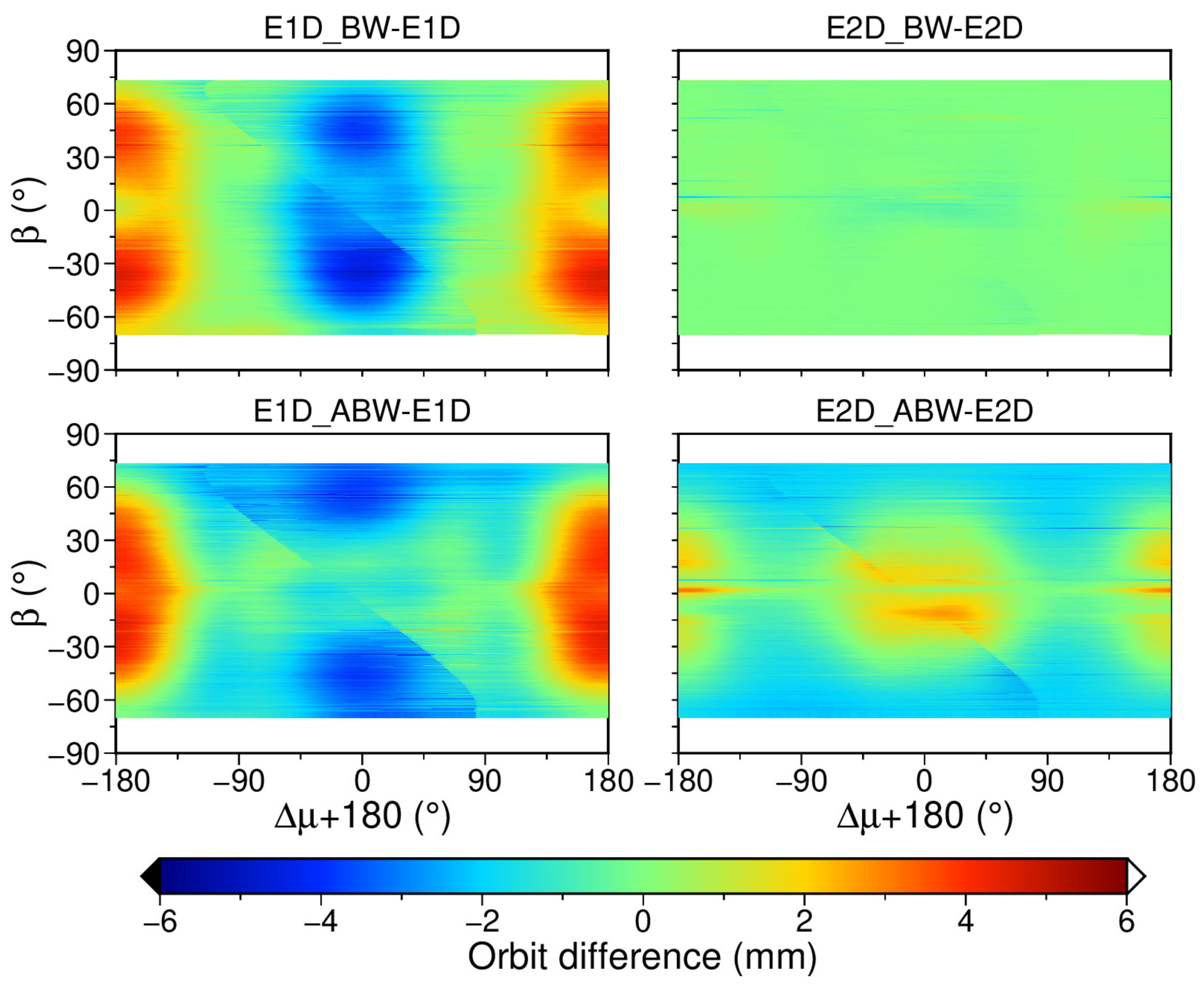
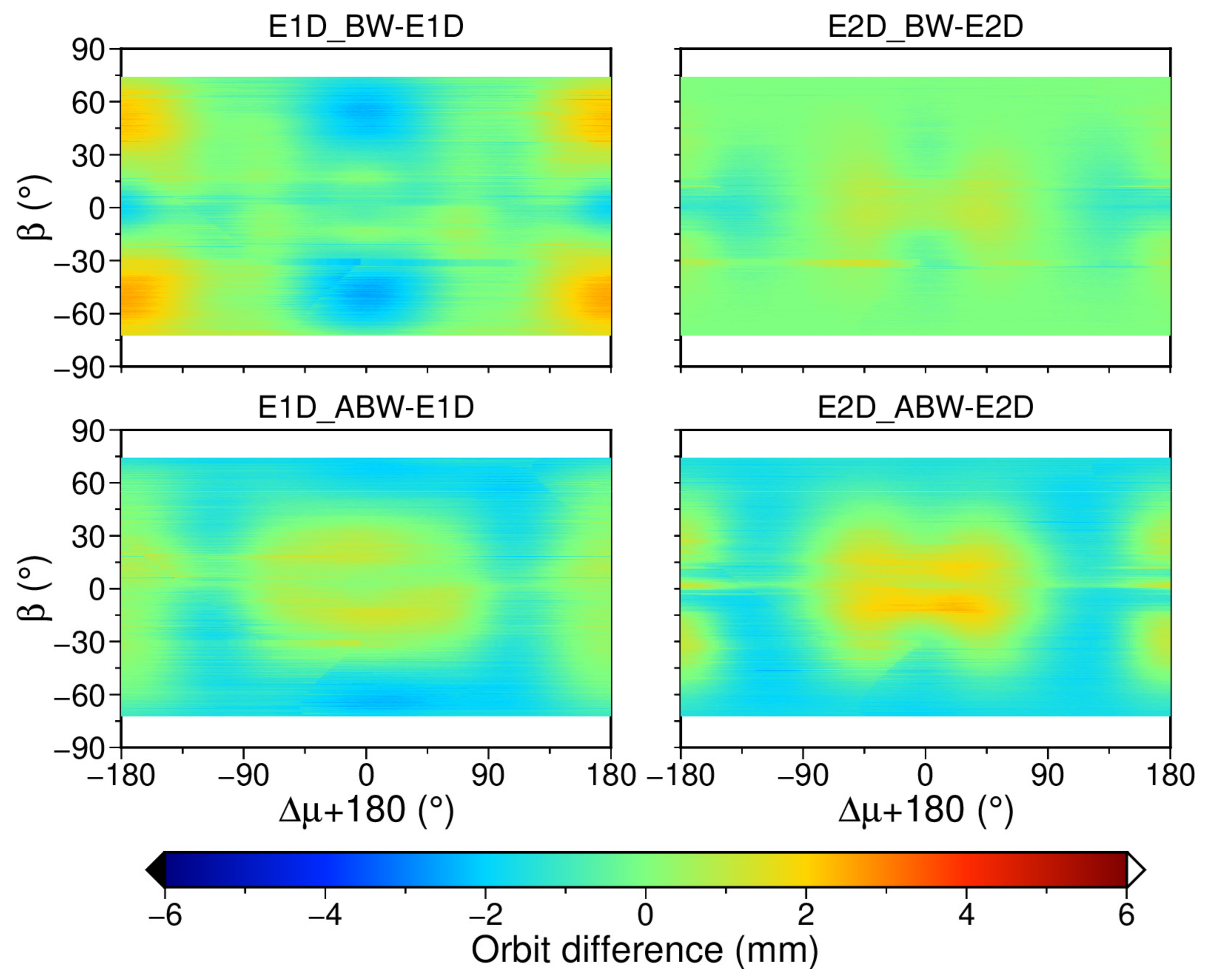

| Solution | Empirical SRP Model | Shadow Factor | A Priori SRP Model |
|---|---|---|---|
| E1DYB | ECOM1 | D,Y,B | None |
| E1DYB_BW | ECOM1 | D,Y,B | Box-wing |
| E1DYB_ABW | ECOM1 | D,Y,B | Adjustable box-wing |
| E1D | ECOM1 | D | None |
| E1D_BW | ECOM1 | D | Box-wing |
| E1D_ABW | ECOM1 | D | Adjustable box-wing |
| E2DYB | ECOM2 | D,Y,B | None |
| E2DYB_BW | ECOM2 | D,Y,B | Box-wing |
| E2DYB_ABW | ECOM2 | D,Y,B | Adjustable box-wing |
| E2D | ECOM2 | D | None |
| E2D_BW | ECOM2 | D | Box-wing |
| E2D_ABW | ECOM2 | D | Adjustable box-wing |
| Item | Description |
|---|---|
| Time span | 2017–2019 |
| Arc length | 24-h |
| Number of stations | ~125 stations |
| Observation | Ionospheric-free combined GPS pseudorange and phase observations, 5-min sampling |
| Observation weighting | Pseudorange: 0.5 m, phase: 0.01 cycle; elevation-dependent downweighing |
| Cut-off elevation angle | 7 degrees |
| Satellite orbits and clocks | Orbit estimated using different SRP models (see Table 1 for details). Satellite clocks estimated as epochwise white noise |
| Orbit integration | RKF+ADMAS (change to RKF when entering and out of shadow) |
| Satellite attitude | GPS yaw model [9,38,39,40] |
| Earth radiation | Applied [12] |
| Transmitter thrust | Applied [12] |
| Station coordinates | Estimated as daily constant, no-net-rotation constraints on the datum stations to IGS14 [37] |
| Station clock | Estimated as epochwise white noise |
| Surface displacement | Solid Earth tides, ocean tides (FES2004), pole tide, and ocean pole tide according to IERS 2010 Conventions [41] |
| Tropospheric delay | GPT2w [42] for the a priori zenith hydrostatic and wet delays, residual zenith wet delay estimated as 2-h piece wise constant, north and east gradients estimated as daily constant. Mapping functions: GMF [43] for zenith delays and Chen-Herring [44] for horizontal gradients. |
| Ambiguity fixing | Double-differenced ambiguity resolution [45,46] |
| Earth rotation parameters | A priori value: IERS finals 2000A productPolar motion components estimated as daily offset and rate, and only daily rate (LoD) for UT1-UTC. The sub-daily variations of ERP are modeled according to IERS 2010 Conventions. |
| Solutions | BLOCK IIR | BLOCK IIF | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Eclipse | Noneclipse | Eclipse | Noneclipse | |||||||||
| A | C | R | A | C | R | A | C | R | A | C | R | |
| E1DYB | 2.6 | 2.8 | 3.1 | 2.8 | 2.2 | 2.3 | 3.1 | 2.2 | 2.7 | 2.5 | 1.7 | 1.8 |
| E1DYB_ABW | 2.5 | 2.4 | 2.7 | 2.3 | 1.7 | 1.7 | 3.1 | 2.1 | 2.7 | 2.4 | 1.7 | 1.7 |
| E1D | 2.5 | 2.1 | 2.4 | 2.8 | 2.2 | 2.3 | 3.1 | 2.1 | 2.3 | 2.5 | 1.7 | 1.8 |
| E1D_ABW | 2.4 | 1.9 | 2.0 | 2.3 | 1.7 | 1.7 | 3.1 | 2.1 | 2.3 | 2.4 | 1.6 | 1.7 |
| E2DYB | 2.4 | 2.1 | 2.4 | 2.7 | 2.1 | 2.2 | 3.0 | 2.0 | 2.6 | 2.5 | 1.8 | 1.8 |
| E2DYB_ABW | 2.3 | 2.0 | 2.3 | 2.4 | 1.8 | 1.7 | 3.0 | 1.9 | 2.6 | 2.4 | 1.7 | 1.7 |
| E2D | 2.4 | 1.8 | 2.3 | 2.7 | 2.0 | 2.2 | 3.1 | 1.9 | 2.5 | 2.5 | 1.8 | 1.8 |
| E2D_ABW | 2.3 | 1.7 | 2.2 | 2.3 | 1.7 | 1.7 | 3.0 | 1.8 | 2.5 | 2.4 | 1.7 | 1.7 |
| Solutions | x-Pole [μas] | y-Pole [μas] | x-Pole Rate [μas/day] | y-Pole Rate [μas/day] | LoD [μs/day] |
|---|---|---|---|---|---|
| E1DYB | 44.1 | 24.8 | 214.4 | 205.3 | 9.2 |
| E1DYB_ABW | 43.7 | 23.4 | 214.4 | 184.9 | 9.3 |
| E1D | 43.6 | 24.4 | 214.6 | 199.5 | 9.1 |
| E1D_ABW | 43.9 | 23.3 | 214.7 | 193.2 | 8.7 |
| E2DYB | 44.3 | 23.6 | 215.8 | 190.6 | 9.8 |
| E2DYB_ABW | 43.8 | 23.3 | 213.3 | 185.6 | 9.7 |
| E2D | 44.7 | 23.5 | 216.0 | 200.3 | 9.2 |
| E2D_ABW | 44.0 | 23.1 | 213.2 | 191.4 | 9.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, L.; Wang, J.; Zhu, H.; Ge, M.; Xu, A.; Schuh, H. A Comparative Study on the Solar Radiation Pressure Modeling in GPS Precise Orbit Determination. Remote Sens. 2021, 13, 3388. https://doi.org/10.3390/rs13173388
Tang L, Wang J, Zhu H, Ge M, Xu A, Schuh H. A Comparative Study on the Solar Radiation Pressure Modeling in GPS Precise Orbit Determination. Remote Sensing. 2021; 13(17):3388. https://doi.org/10.3390/rs13173388
Chicago/Turabian StyleTang, Longjiang, Jungang Wang, Huizhong Zhu, Maorong Ge, Aigong Xu, and Harald Schuh. 2021. "A Comparative Study on the Solar Radiation Pressure Modeling in GPS Precise Orbit Determination" Remote Sensing 13, no. 17: 3388. https://doi.org/10.3390/rs13173388
APA StyleTang, L., Wang, J., Zhu, H., Ge, M., Xu, A., & Schuh, H. (2021). A Comparative Study on the Solar Radiation Pressure Modeling in GPS Precise Orbit Determination. Remote Sensing, 13(17), 3388. https://doi.org/10.3390/rs13173388









