LEO-Constellation-Augmented BDS Precise Orbit Determination Considering Spaceborne Observational Errors
Abstract
:1. Introduction
2. Constellation Design and Data Simulation
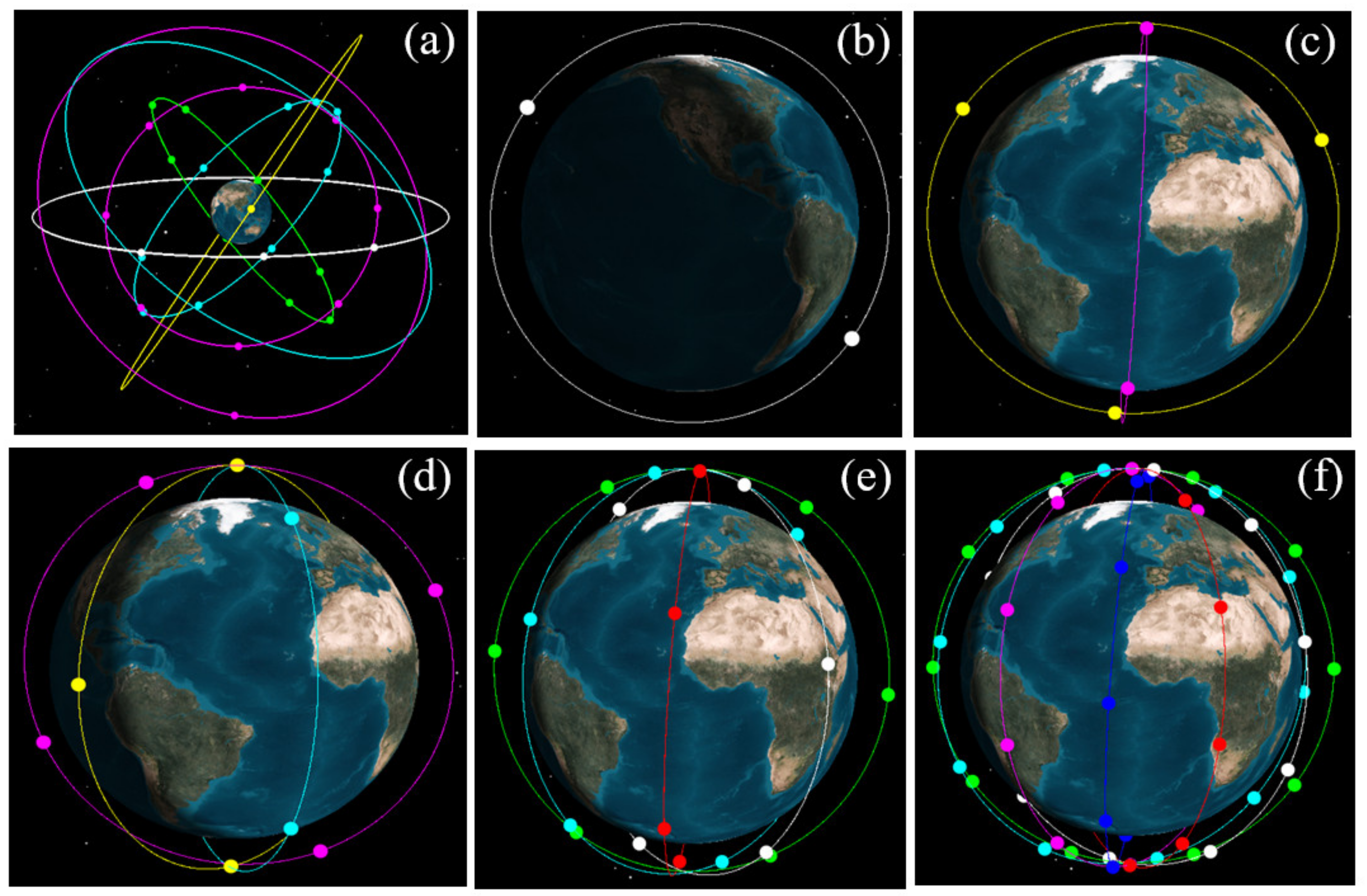
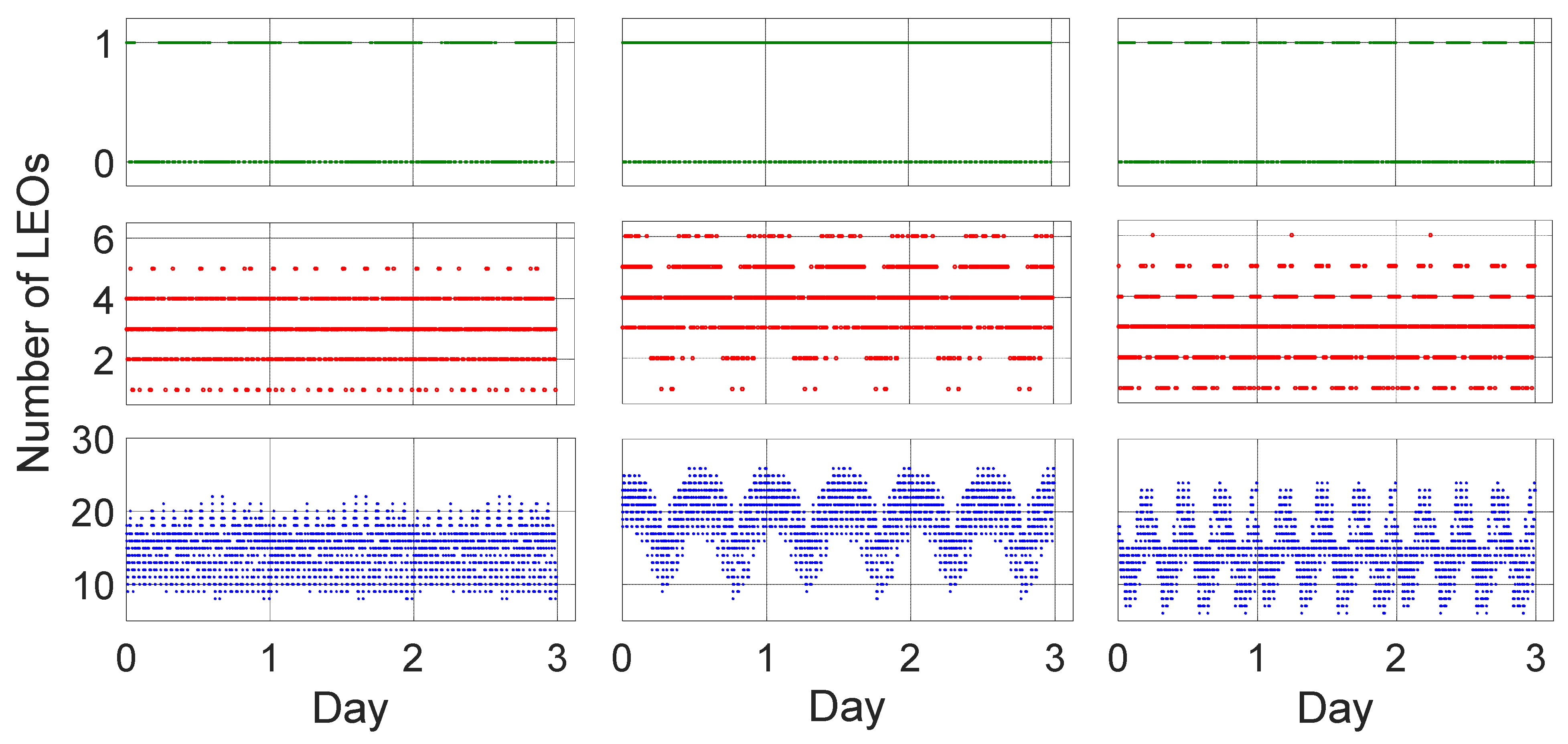
2.1. BDS/LEO Combined Constellation Configurations
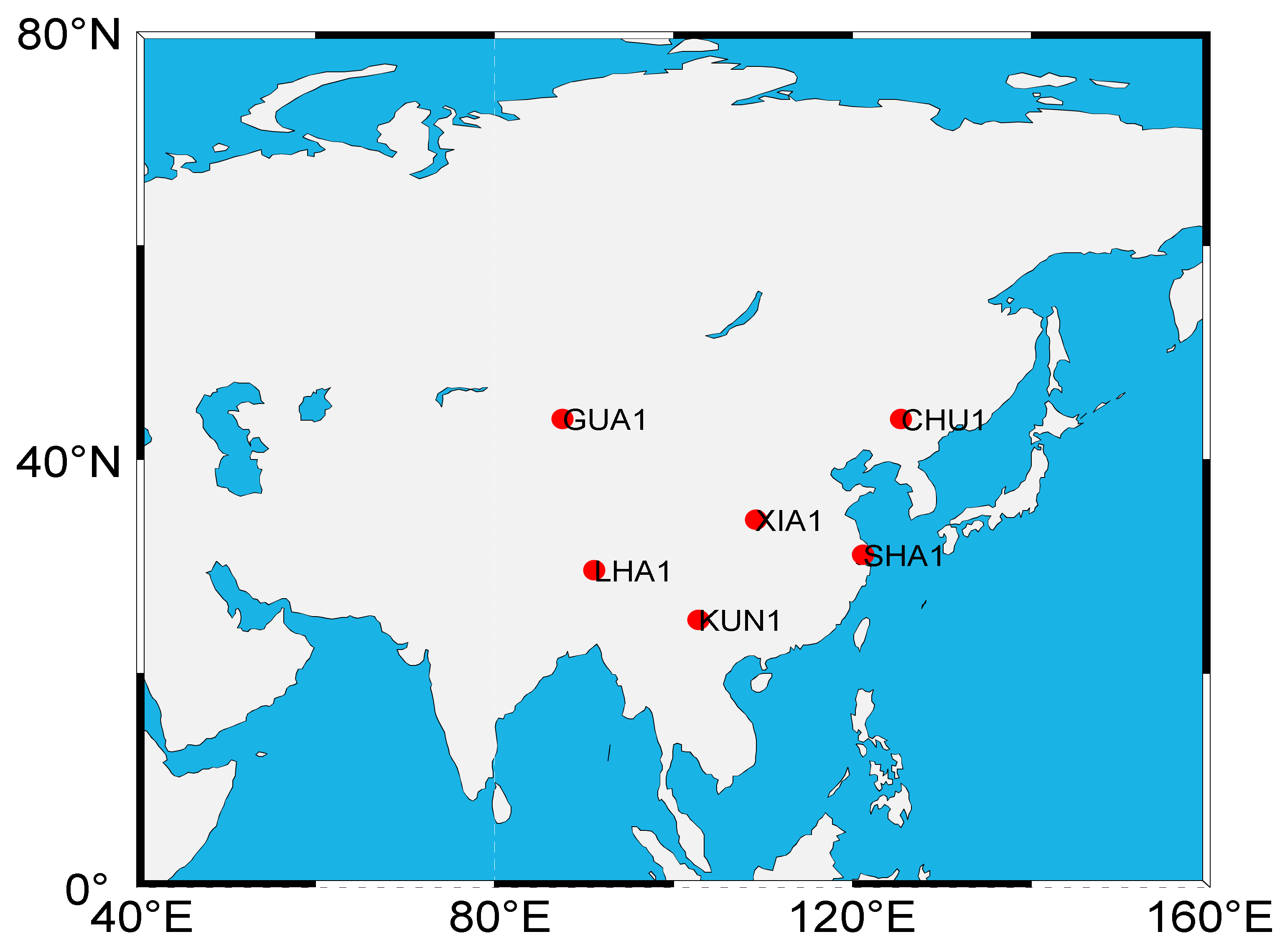
2.2. Simulation of Observations
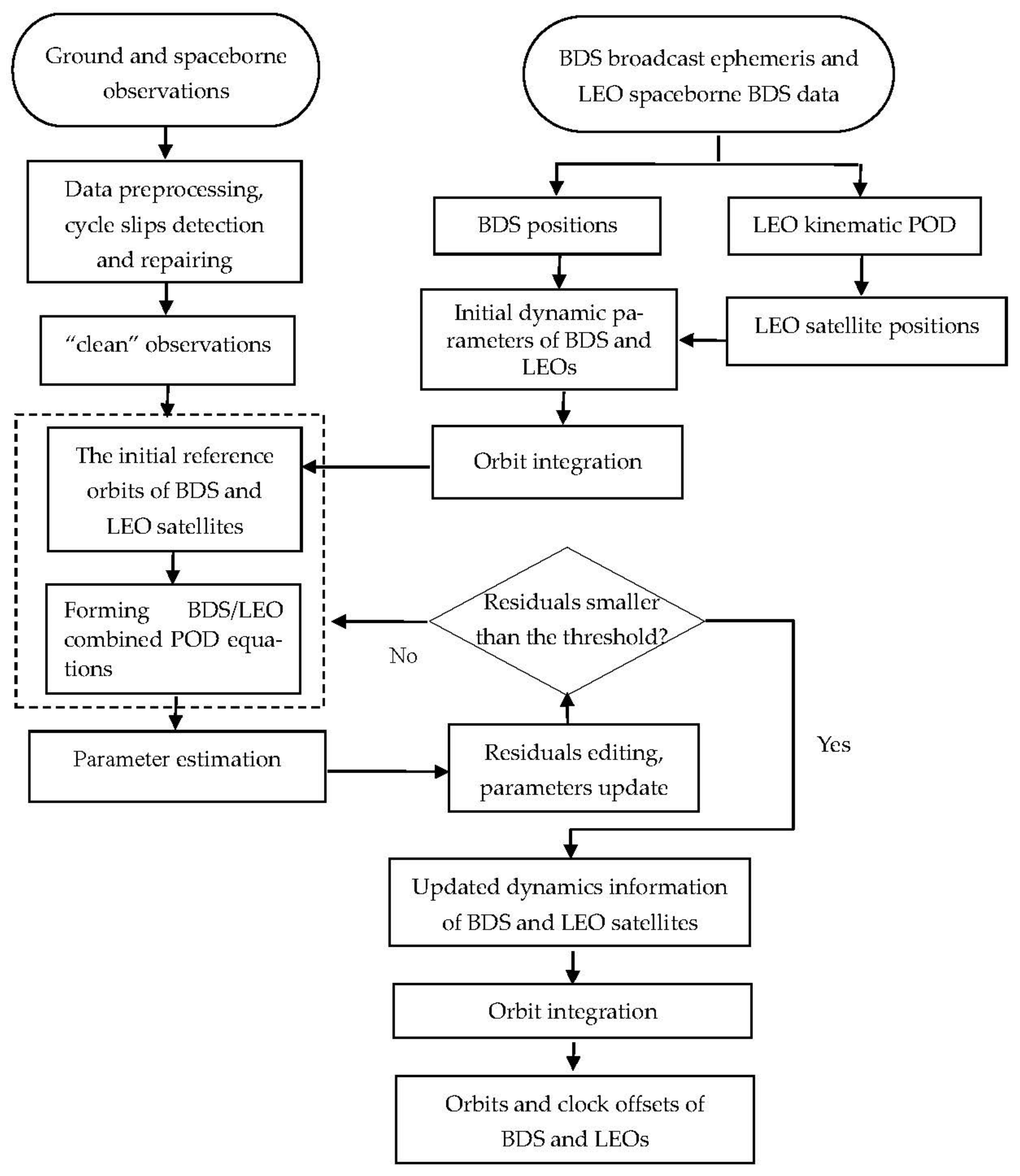
3. LEO-Constellation-Augmented BDS POD Strategies
- (1)
- First, the designed BDS and LEO ephemerides are converted to a broadcast ephemeris and used as the initial values for the POD. An 18-parameter model [31] is used for the BDS broadcast ephemeris design.
- (2)
- The initial BDS positions are obtained from the generated broadcast ephemeris and then the BDS initial dynamics information is derived via orbit dynamics fitting. The positions of the LEO satellites are derived from the kinematic POD using the BDS broadcast ephemeris and LEO spaceborne data, and then the LEO orbit initial dynamics information is obtained via orbit dynamics fitting.
- (3)
- Orbit integration is applied for the BDS and LEO satellite dynamics to obtain the initial reference orbit and state transition matrix.
- (4)
- The unknown parameters are ranked, combined, and added with the constraints listed in Table 3, and the POD equations are linearized at the reference initial orbit. The observations at all epochs are stacked on a combined adjustment for parameter estimation.
- (5)
- The adjusted BDS and LEO reference orbits are obtained via orbit integration after having resolved the normal equations.
- (6)
- Considering several random cycles of cycle slips are added to the observations, posterior residual editing is conducted until new cycle slips are detected and calibrated.
- (7)
- Steps 3–5 are repeated until the residuals are smaller than the given threshold values. In terms of the carrier-phase measurements, the threshold is usually defined as three times the post-fit STD value. The carrier-phase measurement can be regarded as “bad” and abandoned if the corresponding residual is larger than the threshold value. An ambiguity parameter is introduced and involved in calculation when the residual is larger than one and a half times the STD value. Otherwise, the residuals are regarded as white noises with normal distribution. This step is necessary because the actual-measured code and carrier-phase residuals are included in the observation simulation.
- (8)
- Finally, the BDS and LEO satellite orbit dynamics and clock products are calculated and the precise orbits can be derived via orbit integration with the orbit dynamics information. The estimated final orbits are compared with the “true” orbits, which are described in Section 2.1. A flowchart of the above procedures is given in Figure 3.
4. Experimental Analysis
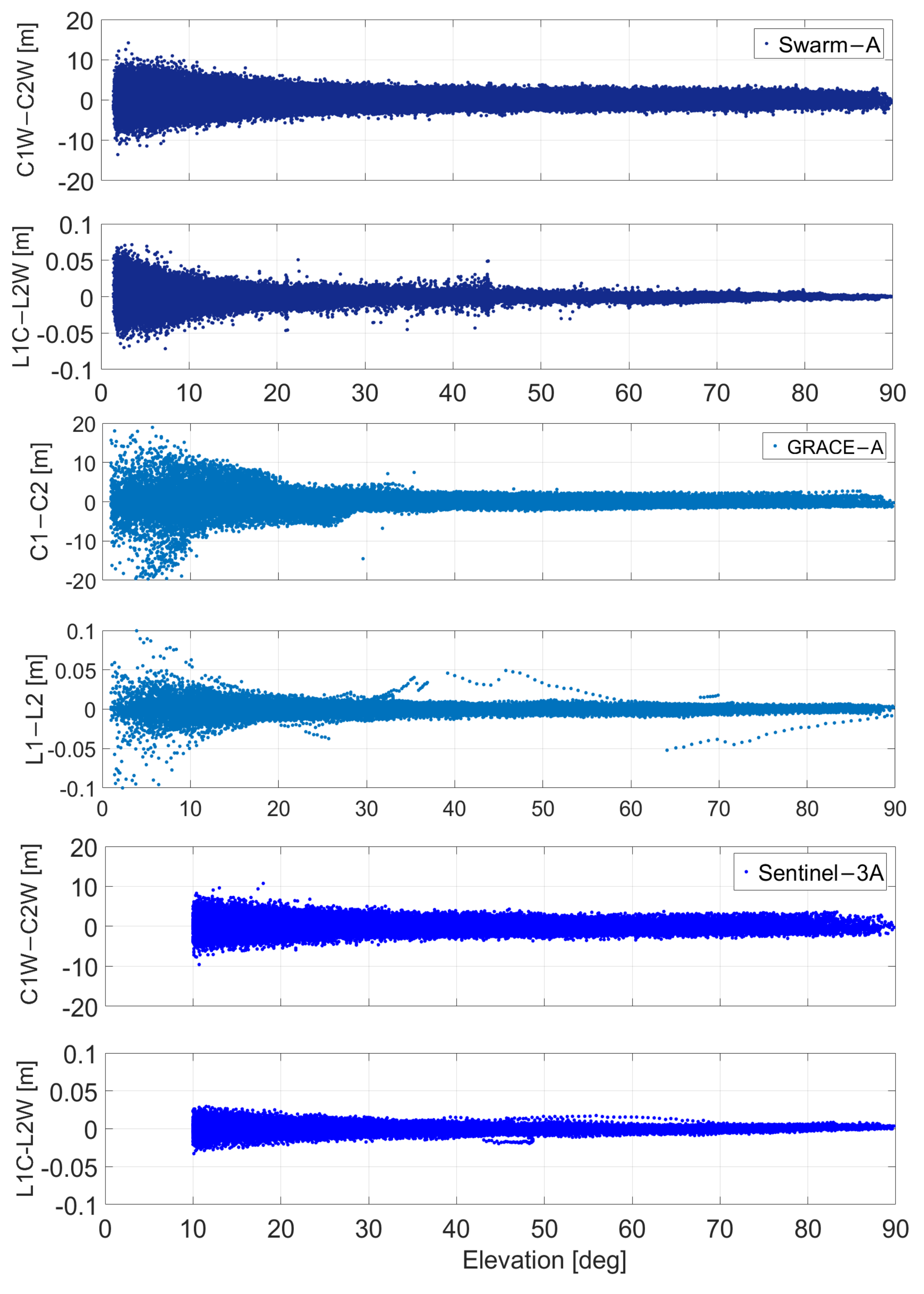
4.1. Analysis of the Spaceborne Measurement Noise
4.2. BDS/LEO Combined POD during a Quiet Period of Solar Activity
4.3. GNSS/LEO Combined POD during a Solar Storm
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Dai, X.; Ge, M.; Lou, Y.; Shi, C.; Wickert, J.; Schuh, H. Estimating the yaw-attitude of BDS IGSO and MEO satellites. J. Geod. 2015, 89, 1005–1018. [Google Scholar] [CrossRef]
- Guo, J.; Chen, G.; Zhao, Q.; Liu, J.; Liu, X. Comparison of solar radiation pressure models for BDS IGSO and MEO satellites with emphasis on improving orbit quality. GPS Solut. 2017, 21, 511–522. [Google Scholar] [CrossRef] [Green Version]
- Montenbruck, O.; Steigenberger, P.; Hugentobler, U. Enhanced solar radiation pressure modeling for Galileo satellites. J. Geod. 2014, 89, 283–297. [Google Scholar] [CrossRef]
- Zhang, Q.; Zhu, Y.; Chen, Z. An In-Depth Assessment of the New BDS-3 B1C and B2a Signals. Remote Sens. 2021, 13, 788. [Google Scholar] [CrossRef]
- Guo, F.; Li, X.; Zhang, X.; Wang, J. Assessment of precise orbit and clock products for Galileo, BeiDou, and QZSS from IGS Multi-GNSS Experiment (MGEX). GPS Solut. 2017, 21, 279–290. [Google Scholar] [CrossRef]
- Joerger, M.; Gratton, L.; Pervan, B.; Cohen, C.E. Analysis of Iridium-Augmented GPS for Floating Carrier Phase Positioning. Navigation 2010, 57, 137–160. [Google Scholar] [CrossRef]
- Li, B.; Ge, H.; Ge, M.; Nie, L.; Shen, Y.; Schuh, H. LEO enhanced Global Navigation Satellite System (LeGNSS) for real-time precise positioning services. Adv. Space Res. 2019, 63, 73–93. [Google Scholar] [CrossRef]
- Ge, H.; Li, B.; Ge, M.; Zang, N.; Nie, L.; Shen, Y.; Schuh, H. Initial Assessment of Precise Point Positioning with LEO Enhanced Global Navigation Satellite Systems (LeGNSS). Remote Sens. 2018, 10, 984. [Google Scholar] [CrossRef] [Green Version]
- Li, X.; Zhang, K.; Ma, F.; Zhang, W.; Bian, L. Integrated Precise Orbit Determination of Multi-GNSS and Large LEO Constel-lations. Remote Sens. 2019, 11, 2514. [Google Scholar] [CrossRef] [Green Version]
- Li, X.; Ma, F.; Li, X.; Lv, H.; Bian, L.; Jiang, Z.; Zhang, X. LEO constellation-augmented multi-GNSS for rapid PPP convergence. J. Geod. 2019, 93, 749–764. [Google Scholar] [CrossRef]
- Rabinowitz, M.; Parkinson, B.; Cohen, C.; O’Connor, M.; Lawrence, D. A system using LEO telecommunication satellites for rapid acquisition of integer cycle ambiguities. In Proceedings of the IEEE 1998 Position Location and Navigation Symposium (Cat. No. 98CH36153), Palm Springs, CA, USA, 20–23 April 1996; pp. 137–145. [Google Scholar] [CrossRef]
- Skone, S.H. The impact of magnetic storms on GPS receiver performance. J. Geod. 2001, 75, 457–468. [Google Scholar] [CrossRef]
- Hu, Y.; Cai, Z. Influences of Space Weather Events on the Spacecraft. Adv. Meteorol. Sci. Technol. 2011, 1, 13–17. [Google Scholar]
- Janssen, V. Likely impact of the approaching solar maximumon GNSS surveys: Be alert but not alarmed. In Proceedings of the 17th Association of the Public Authority Surveyors Conference (APAS2012), Wollongong, Australia, 19–21 March 2012. [Google Scholar]
- Cerruti, A.P.; Kintner, P.M.; Gary, D.; Lanzerotti, L.J.; De Paula, E.R.; Vo, H. Observed solar radio burst effects on GPS/Wide Area Augmentation System carrier-to-noise ratio. Space Weather 2006, 4, 10006. [Google Scholar] [CrossRef]
- Chen, Z.; Gao, Y.; Liu, Z. Evaluation of solar radio bursts’ effect on GPS receiver signal tracking within International GPS Service network. Radio Sci. 2005, 40, 3012. [Google Scholar] [CrossRef]
- Kintner, P.M.; Ledvina, B.M.; De Paula, E.R. GPS and ionospheric scintillations. Space Weather 2007, 5, 09003. [Google Scholar] [CrossRef]
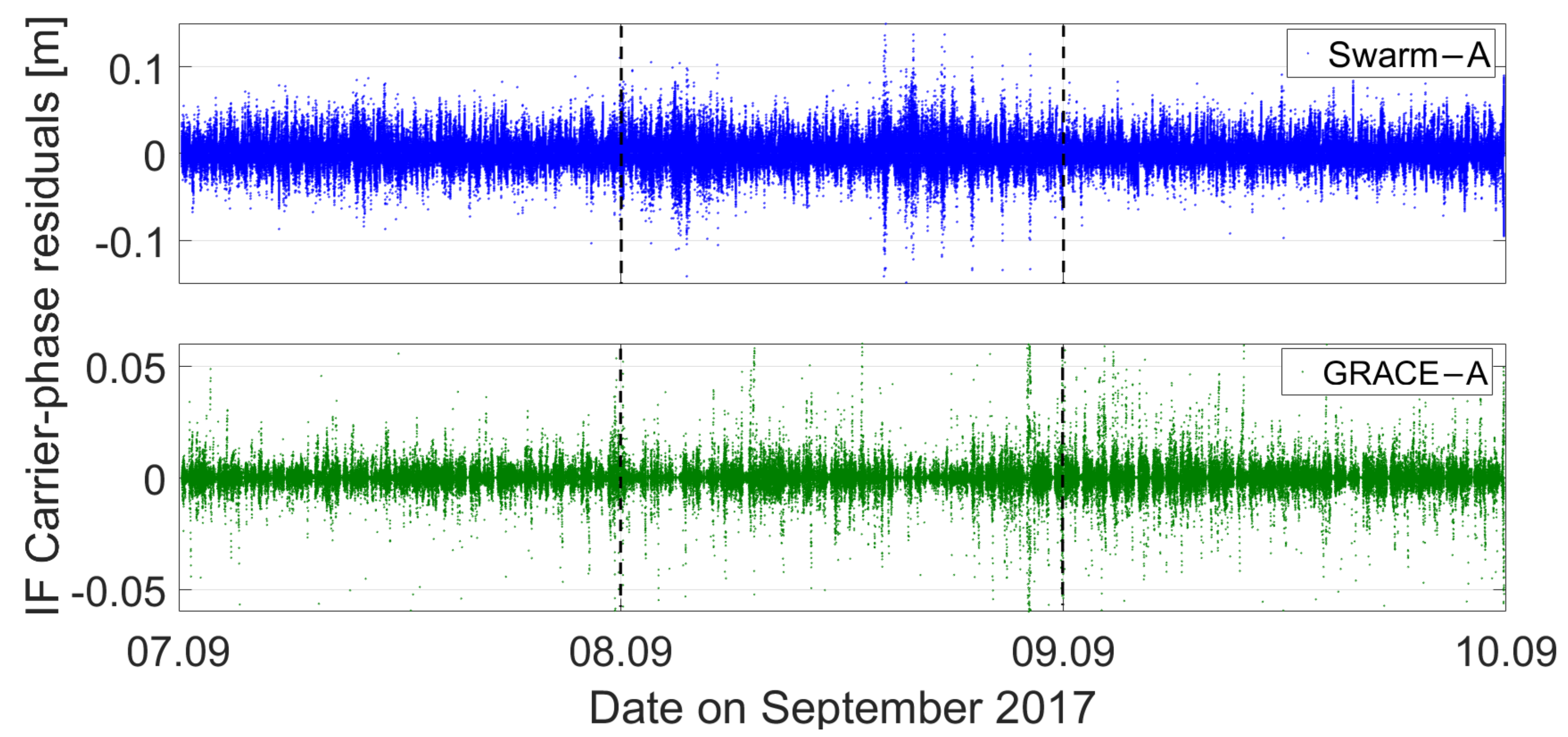
- Zhang, K.; Li, X.; Xiong, C. The Influence of Geomagnetic Storm of 7September 2017 on the Swarm Precise Orbit Determination. J. Geophys. Res. Space Phys. 2019, 124, 6971–6984. [Google Scholar] [CrossRef]
- Walker, J.G. Satellite constellations. J. Br. Interplanet. Soc. 1984, 37, 559–572. [Google Scholar]
- Förste, C.; Bruinsma, S.; Shako, R.; Marty, J.-C.; Flechtner, F.; Abrykosov, O.; Dahle, C.; Lemoine, J.-M.; Neumayer, K.-H.; Biancale, R. EIGEN-6—A New Combined Global Gravity Field Model Including GOCE Data from the Collaboration of GFZ Potsdam and GRGS Toulouse (Geophysical Research Abstracts Vol.13, EGU2011-3242-2, 2011); General Assembly European Geosciences Union: Vienna, Austria, 2011. [Google Scholar]
- Petit, G.; Luzum, B. IERS Conventions 2010. No.36 in IERS Technical Note; Verlag des Bundesamts für Kartographie und Geodäsie: Frankfurt am Main, Germany, 2010. [Google Scholar]
- Lyard, F.; Lefevre, F.; Letellier, T.; Francis, O. Modelling the global ocean tides: Modern insights from FES2004. Ocean Dyn. 2006, 56, 394–415. [Google Scholar] [CrossRef]
- Arnold, D.; Meindl, M.; Beutler, G.; Dach, R.; Jggi, A. CODE’s new solar radiation pressure model for GNSS orbit determination. J. Geod. 2015, 89, 775–791. [Google Scholar] [CrossRef] [Green Version]
- Berger, C.; Biancale, R.; Ill, M.; Barlier, F. Improvement of the empirical thermospheric model DTM: DTM94—A comparative review of various temporal variations and prospects in space geodesy applications. J. Geod. 1998, 72, 161–178. [Google Scholar] [CrossRef]
- Kouba, J.; Héroux, P. Precise Point Positioning Using IGS Orbit and Clock Products. GPS Solut. 2001, 5, 12–28. [Google Scholar] [CrossRef]
- Li, M.; He, K.; Xu, T.; Lu, B. Robust adaptive filter for shipborne kinematic positioning and velocity determination during the Baltic Sea experiment. GPS Solut. 2018, 22, 81. [Google Scholar] [CrossRef]
- Zhang, B.; Chen, Y.; Yuan, Y. PPP-RTK based on undifferenced and uncombined observations: Theoretical and practical aspects. J. Geod. 2018, 93, 1011–1024. [Google Scholar] [CrossRef]
- Saastamoinen, J. Atmospheric Correction for the Troposphere and Stratosphere in Radio Ranging Satellites. In Use of Aritificial Satellites for Geodesy; Wiley: Hoboken, NJ, USA, 1972; Volume 15, pp. 247–251. [Google Scholar]
- Boehm, J.; Niell, A.; Tregoning, P.; Schuh, H. Global Mapping Function (GMF): A new empirical mapping function based on numerical weather model data. Geophys. Res. Lett. 2006, 33, 07304. [Google Scholar] [CrossRef] [Green Version]
- Zhang, B.; Ou, J.; Yuan, Y.; Li, Z. Extraction of line-of-sight ionospheric observables from GPS data using precise point positioning. Sci. China 2012, 55, 1919–1928. [Google Scholar] [CrossRef]
- Montenbruck, O.; Steigenberger, P.; Hauschild, A. Broadcast versus precise ephemerides: A multi-GNSS perspective. GPS Solut. 2015, 19, 321–333. [Google Scholar] [CrossRef]
- Friis-Christensen, E.; Lühr, H.; Knudsen, D.; Haagmans, R. Swarm—An Earth Observation Mission investigating Geospace. Adv. Space Res. 2008, 41, 210–216. [Google Scholar] [CrossRef]
- Montenbruck, O.; Hackel, S.; Jäggi, A. Precise orbit determination of the Sentinel-3A altimetry satellite using ambiguity-fixed GPS carrier phase observations. J. Geod. 2018, 92, 711–726. [Google Scholar] [CrossRef] [Green Version]
- Wagner, C.; McAdoo, D.; Klokočník, J.; Kostelecký, J. Degradation of Geopotential Recovery from Short Repeat-Cycle Orbits: Application to GRACE Monthly Fields. J. Geod. 2006, 80, 94–103. [Google Scholar] [CrossRef]
- Tassev, Y.; Velinov, P.I.; Tomova, D.; Mateev, L. Analysis of extreme solar activity in early September 2017: G4—Severe geomagnetic storm (07-08.09) and GLE72 (10.09) in solar minimum. Comptes Rendus l’Acade’mie Bulg. Sci. 2017, 70, 1437–1444. [Google Scholar]


| System | BDS-3 | LEO | ||
|---|---|---|---|---|
| Orbit Type | GEO | IGSO | MEO | Polar |
| Satellite number | 3 | 3 | 24 | 2, 6, 12, 24, and 60, respectively |
| Constellation | Placed at east longitude 80°, 110.5°, and 140° | Placed at geographical longitude 0°, 120°, and 240° | Walker 24/3/1 | 1, 2, 3, 4, and 6 planes, respectively |
| Inclination | 0 | 55° | 55° | 86.4° |
| altitude [km] | 35,786 | 35,786 | 21,528 | 1200 |
| Dynamic Model | BDS | LEO |
|---|---|---|
| Earth gravity | EIGEN-6C (12 × 12) [20] | EIGEN-6C (120 × 120) |
| N-body | JPL DE405 | JPL DE405 |
| Solid tide and pole tide | IERS 2010 [21] | IERS 2010 |
| Ocean tide | FES 2004 [22] | FES 2004 |
| Relatively | IERS 2010 | IERS 2010 |
| Solar radiation pressure | ECOM2 7-parameter model [3] | Box-Wing model [23] |
| Atmospheric drag | None | DTM94 [24] |
| Item | Description | A Priori Constraints |
|---|---|---|
| Observations | Undifferenced dual-frequency IF LC and PC | Ground pseudo-range observations: 1 m; carrier-phase: 0.02 cycles; spaceborne observations: calibrated with real-calculated observations |
| Cut-off elevation | Spaceborne observations: 1°; ground observations: 7° | |
| Arc length | 72 h | |
| Sampling interval | Ground and spaceborne BDS observations: 10 s | |
| Satellite position and velocity | Initial value from the broadcast ephemeris | Position: 10 m; velocity: 0.1 m/s |
| Station coordinates | PPP static solutions | 2 mm for horizontal and 5 mm for vertical component |
| Tropospheric delay | LEO spaceborne: none; ground stations: Saastamoinen model/GMF, the zenith wet delay estimated every 2 h | 0.2 + 0.2/sqrt (hours) |
| BDS and LEO satellite clock offsets | Broadcast ephemeris and white noise (estimated with a BDS clock as reference) | 5000 m |
| Spaceborne receiver clock offset | Estimated as white noise | 1000 m |
| Ground receiver clock offset | Estimated as white noise | 9000 m |
| EOP | Fixed | IGS final products |
| Ambiguity | Float solution |
| LEO Constellation | Swarm | GRACE | Sentinel-3 |
|---|---|---|---|
| Satellite | Swarm-A/B/C | GRACE-A/B | Sentinel-3A/B |
| Spaceborne observations | GPS | GPS | GPS |
| Altitude | A/C: ~460 km, B: ~510 km | ~500 km | ~814.5 km |
| Inclination | A/C: 87.35°, B: 87.75° | 89.5° | 98.65° |
| Orbit type | Circular near-polar orbits | Circular near-polar orbits | Repeating frozen sun-synchronous orbit [33] |
| Repeat cycle | 7–10 months | A sparse repeat track of 61 revolutions every four days [34] | 27-day repeat cycle, 14 + 7/27 orbits per day [34] |
| Goal | Geomagnetic observation | Detection of the Earth’s gravity variations | Earth monitoring and operational oceanography |
| Satellite | S2 (W2/1/0) | S4 (W/12/3/1) | S6 (60/6/4) | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Item | C02 | C06 | C11 | C02 | C06 | C11 | C02 | C06 | C11 | |
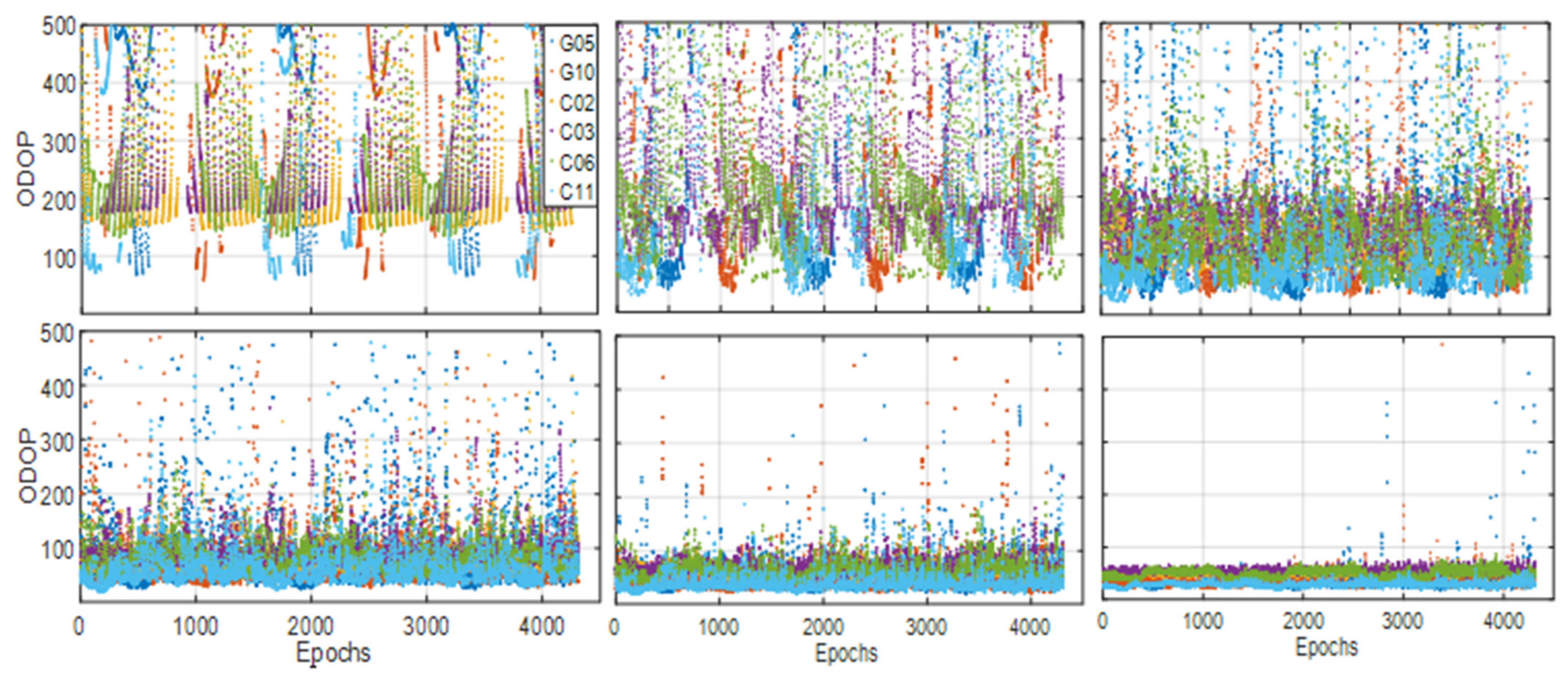
| Mean ODOP | 297.5 | 237.5 | 203.1 | 84.0 | 96.4 | 72.6 | 40.2 | 38.8 | 18.7 | |
| [m2] | ||||||||||
| 371.9 | 296.9 | 253.9 | 281.4 | 322.9 | 243.2 | 31.0 | 29.9 | 14.4 | ||
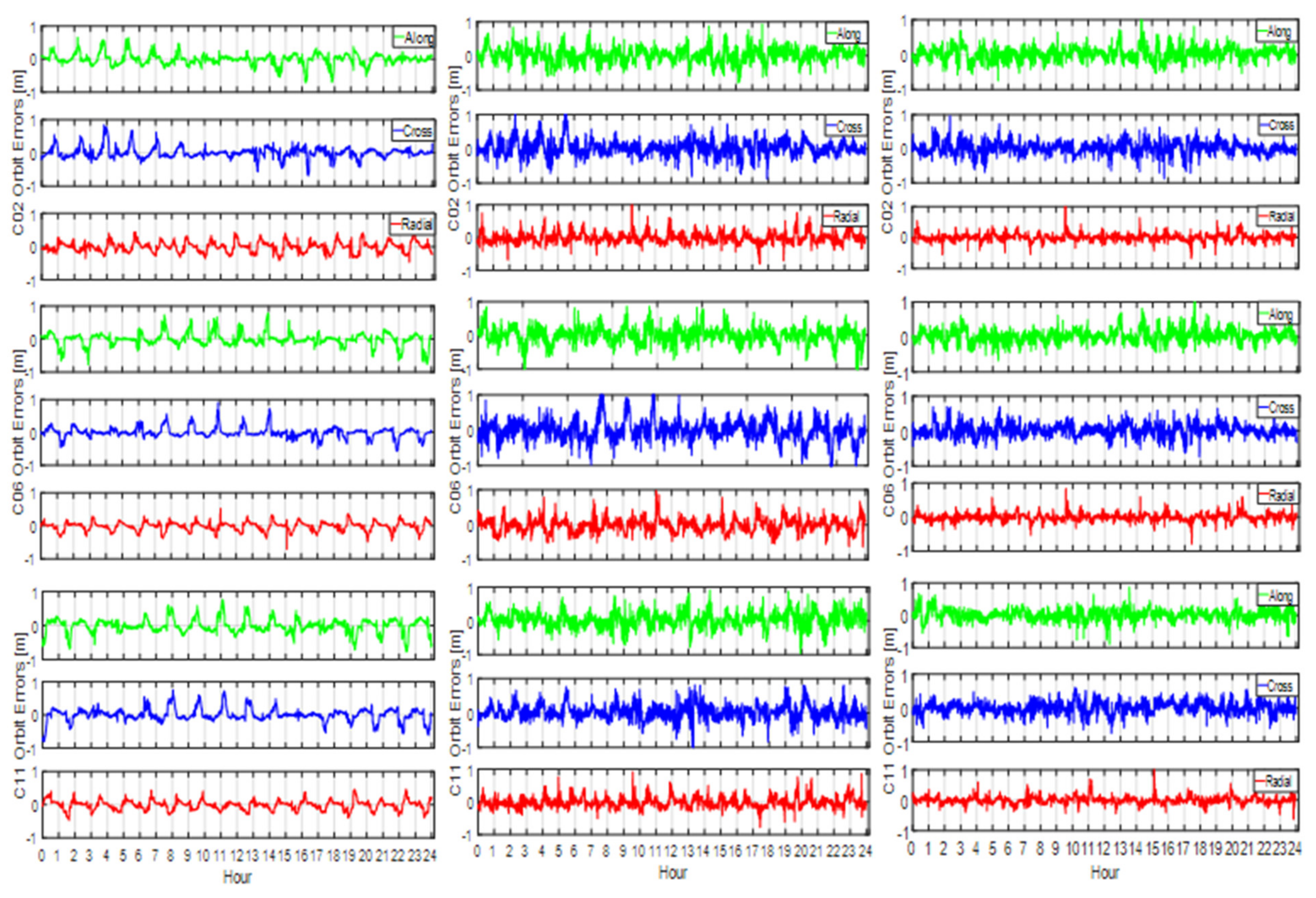
| Orbital 3D RMS [m] | 1.18 | 1.07 | 0.75 | 1.10 | 1.15 | 0.69 | 0.60 | 0.43 | 0.44 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, M.; Xu, T.; Ge, H.; Guan, M.; Yang, H.; Fang, Z.; Gao, F. LEO-Constellation-Augmented BDS Precise Orbit Determination Considering Spaceborne Observational Errors. Remote Sens. 2021, 13, 3189. https://doi.org/10.3390/rs13163189
Li M, Xu T, Ge H, Guan M, Yang H, Fang Z, Gao F. LEO-Constellation-Augmented BDS Precise Orbit Determination Considering Spaceborne Observational Errors. Remote Sensing. 2021; 13(16):3189. https://doi.org/10.3390/rs13163189
Chicago/Turabian StyleLi, Min, Tianhe Xu, Haibo Ge, Meiqian Guan, Honglei Yang, Zhenlong Fang, and Fan Gao. 2021. "LEO-Constellation-Augmented BDS Precise Orbit Determination Considering Spaceborne Observational Errors" Remote Sensing 13, no. 16: 3189. https://doi.org/10.3390/rs13163189
APA StyleLi, M., Xu, T., Ge, H., Guan, M., Yang, H., Fang, Z., & Gao, F. (2021). LEO-Constellation-Augmented BDS Precise Orbit Determination Considering Spaceborne Observational Errors. Remote Sensing, 13(16), 3189. https://doi.org/10.3390/rs13163189








